加拿大国际袋鼠数学竞赛试题 及答案-2018年
袋鼠数学数学竞赛试题

袋鼠数学数学竞赛试题
题目,在一个房间里,有一只袋鼠和一只狗。
袋鼠的身高是狗的1/4,袋鼠的体重是狗的1/2。
如果袋鼠的体重增加了20%,那么袋鼠的身高将增加多少?
解答:
1. 利用代数方法解答:
设狗的身高为h,袋鼠的身高为1/4h。
袋鼠的体重为w,狗的体重为2w。
根据题意可得,袋鼠的体重增加20%,即原体重的1.2倍,即1.2w。
设袋鼠身高增加后的身高为x,则有,x = 1/4h + Δh(Δh为身高增加的值)。
根据题意可得,1.2w = 2w (x/h)^3(袋鼠体重的增加与身高的关系)。
整理方程得,(x/h)^3 = 0.6。
解方程可得,x/h ≈ 0.843。
因此,袋鼠的身高增加约为84.3%。
2. 利用比例方法解答:
根据题意可得,袋鼠的身高与狗的身高的比例为1:4,袋鼠的体重与狗的体重的比例为1:2。
设袋鼠的身高增加后的身高为x,根据比例可得,x/h = 1.2。
解方程可得,x = 1.2h。
因此,袋鼠的身高增加了20%。
3. 利用图形方法解答:
设狗的身高为h,袋鼠的身高为1/4h。
袋鼠的体重为w,狗的体重为2w。
根据题意可得,袋鼠的体重增加20%,即原体重的1.2倍,即1.2w。
画出狗和袋鼠的身高和体重的比较图,可以观察到袋鼠的身高增加了20%后,狗和袋鼠的身高之间的比例关系仍然保持不变。
综上所述,袋鼠的身高增加了约84.3%。
袋鼠数学数学题

袋鼠数学题,也被称为“袋鼠数学竞赛”,是一个面向中
学生的数学竞赛,其题目以问题解决和数学应用为主。
以下
是一份袋鼠数学竞赛的样题:
选择题
1. 如果一只袋鼠一年跳300次,那么五只袋鼠跳多少次?
A. 1500次
B. 1400次
C. 1300次
D. 1200次
E. 1100次
2. 在一个等边三角形中,一个顶点到一个对边的中点的
距离是3米,那么这个三角形的边长是多少?
A. 3米
B. 4米
C. 5米
D. 6米
E. 8米
3. 一个圆和一个扇形的半径相等。
已知圆的面积是50平
方厘米,而扇形所在圆的面积是200平方厘米,这个扇形的
圆心角是多少度?
A. 72度
B. 108度
C. 180度
D. 360度
E. 720度
答案:A,C,B。
解释:第一题根据比例关系计算;第二题利用勾股定理计算;第三题根据面积比计算圆心角。
通过这些题目可以看出,袋鼠数学竞赛的题目强调数学的应用和问题解决能力,而不仅仅是理论知识和计算能力。
因此,想要在袋鼠数学竞赛中取得好成绩,需要具备广泛的数学知识、敏锐的观察力和良好的思维习惯。
加拿大国际袋鼠数学竞赛试题 及答案-2016年 Parents Questions
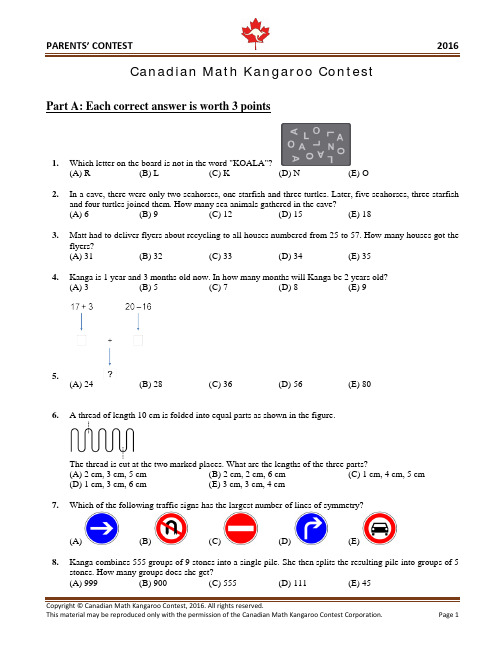
Canadian Math Kangaroo ContestPart A: Each correct answer is worth 3 points1.Which letter on the board is not in the word "KOALA"?(A) R (B) L (C) K (D) N (E) O2.In a cave, there were only two seahorses, one starfish and three turtles. Later, five seahorses, three starfishand four turtles joined them. How many sea animals gathered in the cave?(A) 6 (B) 9 (C) 12 (D) 15 (E) 183.Matt had to deliver flyers about recycling to all houses numbered from 25 to 57. How many houses got theflyers?(A) 31 (B) 32 (C) 33 (D) 34 (E) 354.Kanga is 1 year and 3 months old now. In how many months will Kanga be 2 years old?(A) 3 (B) 5 (C) 7 (D) 8 (E) 95.(A) 24 (B) 28 (C) 36 (D) 56 (E) 806. A thread of length 10 cm is folded into equal parts as shown in the figure.The thread is cut at the two marked places. What are the lengths of the three parts?(A) 2 cm, 3 cm, 5 cm (B) 2 cm, 2 cm, 6 cm (C) 1 cm, 4 cm, 5 cm(D) 1 cm, 3 cm, 6 cm (E) 3 cm, 3 cm, 4 cm7.Which of the following traffic signs has the largest number of lines of symmetry?(A) (B) (C) (D) (E)8.Kanga combines 555 groups of 9 stones into a single pile. She then splits the resulting pile into groups of 5stones. How many groups does she get?(A) 999 (B) 900 (C) 555 (D) 111 (E) 459.What is the shaded area?(A) 50 (B) 80 (C) 100 (D) 120 (E) 15010.In a coordinate system four of the following points are the vertices of a square. Which point is not a vertexof this square?(A) (−1;3)(B) (0;−4)(C) (−2;−1)(D) (1;1)(E) (3;−2)Part B: Each correct answer is worth 4 points11.There are twelve rooms in a building and each room has two windows and one light. Last evening, eighteenwindows were lighted. In how many rooms was the light off?(A) 2 (B) 3 (C) 4 (D) 5 (E) 612.Which three of the five jigsaw pieces shown can be joined together to form a square?(A) 1, 3 and 5 (B) 1, 2 and 5 (C) 1, 4 and 5 (D) 3, 4 and 5 (E) 2, 3 and 513.John has a board with 11 squares. He puts a coin in each of eight neighbouring squareswithout leaving any empty squares between the coins. What is the maximum numberof squares in which one can be sure that there is a coin?(A) 1 (B) 3 (C) 4 (D) 5 (E) 614.Which of the following figures cannot be formed by gluing these two identical squares of paper together?(A) (B) (C) (D) (E)15.Each letter in BENJAMIN represents one of the digits 1, 2, 3, 4, 5, 6 or 7. Different letters represent differentdigits. The number BENJAMIN is odd and divisible by 3. Which digit corresponds to N?(A) 1 (B) 2 (C) 3 (D) 5 (E) 716.Seven standard dice are glued together to make the solid shown. The faces of the dice thatare glued together have the same number of dots on them. How many dots are on the surfaceof the solid?(A) 24 (B) 90 (C) 95 (D) 105 (E) 12617.Jill is making a magic multiplication square using the numbers 1, 2, 4, 5, 10, 20, 25, 50 and 100. The productsof the numbers in each row, in each column and in the two diagonals should all be the same. In the figure you can see how she has started. Which number should Jill place in the cell with the question mark?(A) 2 (B) 4 (C) 5 (D) 10 (E) 2518.What is the smallest number of planes that are needed to enclose a bounded part in three-dimensional space?(A) 3 (B) 4 (C) 5 (D) 6 (E) 719.Each of ten points in the figure is marked with either 0 or 1 or 2. It is known thatthe sum of numbers in the vertices of any white triangle is divisible by 3, while thesum of numbers in the vertices of any black triangle is not divisible by 3. Three ofthe points are marked as shown in the figure. What numbers can be used to markthe central point?(A) Only 0. (B) Only 1. (C) Only 2. (D) Only 0 and 1. (E) Either 0 or 1 or 2.20.Betina draws five points AA,BB,CC,DD and EE on a circle as well as the tangent tothe circle at AA, such that all five angles marked with xx are equal. (Note thatthe drawing is not to scale.) How large is the angle ∠AABBDD ?(A) 66°(B) 70.5°(C) 72°(D) 75°(E) 77.5°Part C: Each correct answer is worth 5 points21.Which pattern can we make using all five cards given below?(A) (B) (C) (D) (E)22.The numbers 1, 5, 8, 9, 10, 12 and 15 are distributed into groups with one or more numbers. The sum of thenumbers in each group is the same. What is the largest number of groups?(A) 2 (B) 3 (C) 4 (D) 5 (E) 623.My dogs have 18 more legs than noses. How many dogs do I have?(A) 4 (B) 5 (C) 6 (D) 8 (E) 924.In the picture you see 5 ladybirds.Each one sits on its flower. Their places are defined as follows: the difference of the dots on their wings is the number of the leaves and the sum of the dots on their wings is the number of the petals. Which of the following flowers has no ladybird?(A) (B) (C) (D) (E)25.On each of six faces of a cube there is one of the following six symbols: ♣, ♦, ♥, ♠, ∎ and Ο. On each facethere is a different symbol. In the picture we can see this cube shown in two different positions.Which symbol is opposite the ∎?(A) Ο(B)♦(C) ♥(D) ♠(E) ♣26.What is the greatest number of shapes of the form that can be cut out from a5 × 5 square?(A) 2 (B) 4 (C) 5 (D) 6 (E) 727.Kirsten wrote numbers in 5 of the 10 circles as shown in the figure. She wants to writea number in each of the remaining 5 circles such that the sums of the 3 numbers alongeach side of the pentagon are equal. Which number will she have to write in the circlemarked by XX?(A) 7 (B) 8 (C) 11 (D) 13 (E) 1528. A 3×3×3 cube is built from 15 black cubes and 12 white cubes. Five faces of the larger cube are shown.Which of the following is the sixth face of the large cube?(A) (B) (C) (D) (E)29.Jakob wrote down four consecutive positive integers. He then calculated the four possible totals made bytaking three of the integers at a time. None of these totals was a prime. What is the smallest integer Jakob could have written?(A) 12 (B) 10 (C) 7 (D) 6 (E) 330.Four sportsmen and sportswomen - a skier, a speed skater, a hockey player and a snowboarder - had dinnerat a round table. The skier sat at Andrea's left hand. The speed skater sat opposite Ben. Eva and Filip sat next to each other. A woman sat at the hockey player`s left hand. Which sport did Eva do?(A) speed skating (B) skiing (C) ice hockey (D) snowboarding(E) It`s not possible to find out with the given information.International Contest-Game Math Kangaroo Canada, 2016Answer KeyParents Contest。
袋鼠数学数学竞赛中文试题

袋鼠数学数学竞赛中文试题袋鼠数学数学竞赛中文试题Ⅰ.选择题(每题2分,共10分)1. 下列哪个数是一个素数?A. 25B. 31C. 42D. 502. A、B、C三个人分别携带了2本、3本、5本书,他们总共带了多少本书?A. 6B. 10C. 9D. 73. 一些苹果在3个篮子中平均分配,每个篮子得到10个苹果,若再将这些苹果平均分配到6个篮子中,则每个篮子得到多少个苹果?A. 5B. 10C. 15D. 204. 甲、乙、丙三个人分别花费400元、600元、800元购买了一些物品,他们所花费的总金额是多少元?A. 800B. 1200C. 1800D. 16005. 若9+4x=25,则x的值是多少?A. 4B. 3C. 5D. 2Ⅱ.填空题(每题3分,共15分)1. 一个整数减去两个负整数之和能是正整数吗?为什么?________________________________________________2. 一个多边形的内角和是2160°,这个多边形有多少个角?________________________________________________3. 甲、乙两个容器分别装有2升和3升的水,如何只用这两个容器倒水,可以得到1升的水?________________________________________________4. 如果一个数的平方加上这个数的2倍等于18,求出这个数。
________________________________________________5. 某树在一年内的生长长度是150厘米,第一季度它的生长长度是前两个季度长度之和的1.5倍,第二季度它的生长长度是前两个季度长度之和的0.5倍,求出第三季度它的生长长度。
________________________________________________Ⅲ.解答题(每题10分,共30分)1. 中国的国旗是由什么颜色组成的?每种颜色的面积占比是多少?________________________________________________2. 一辆火车从A站出发,以每小时100千米的速度前进,过了1小时到达B站。
袋鼠数学竞赛题

袋鼠数学竞赛题在我国,数学一直被视为学科中的重头戏。
为了提高孩子们的数学素养和思维能力,许多学校和机构举办了各种各样的数学比赛。
袋鼠数学竞赛就是其中之一,它的名称来源于袋鼠在澳大利亚是一种非常普遍的动物,象征着这个竞赛适合广大学生参加。
下面我将带您了解一下袋鼠数学竞赛的特点及其挑战性的数学问题。
袋鼠数学竞赛是一个国际性的数学竞赛,始于1980年,至今已有40年历史。
为了鼓励学生对数学的兴趣和探究,竞赛制定了一套独特而有趣的考题,不仅能考察学生的数学知识,更能考察他们的逻辑思维和问题解决能力。
与其他数学竞赛不同的是,袋鼠数学竞赛有三个不同的组别:普及组、提高组和国际组,每个组别的试题难度和题型也不同。
普及组的题目结构比较简单,主要考察学生在基础数学知识的掌握程度上。
而提高组的题目就相对难一些,主要考察学生在更加高级的数学知识和技能上的掌握情况。
而在国际组的竞赛中,一些挑战性的数学问题会让参赛选手挑战自我、超越自我。
在袋鼠数学竞赛中,题目形式多样,短问题、选择题、填空题、计算题、解决问题和证明题都会出现。
每个题目都有一定深度和难度,一些题目还要求学生在短时间内分析和解决,是一种很好的对数学运用和思考能力的考验。
接下来,我们来看看几道典型的袋鼠数学竞赛题目。
第一道题目是普及组中的一道计算题:小李要从1元、2元、5元三种面额的货币中选择70元钱,共有多少种不同的选择方法?这道题主要考察了学生的组合数学基础。
第二道选自提高组的题目:一个正整数被九除余八,被水平线翻转后得到一个六位正整数,求这个六位正整数的值。
这道题主要考察了学生在数论和数字推理中的能力。
此外,还有一道难度较大的题目:有100个棋子,其中有99个正常重量,还有1个轻重袋子。
通过使用一个只能测3次的天平,你能找到轻重袋子吗?这道题考察了学生的逻辑思维和问题解决能力,是一道非常挑战性的数学题目。
总的来说,袋鼠数学竞赛是一种很好的对学生数学能力的考察和锻炼。
加拿大国家中小学数学竞赛( kangaroo math 袋鼠竞赛)2018年一二年级(含答案)

I N T E R N A T I O N A L C O N T E S T-G A M EM A T H K A N G A R O OC A N AD A,2018I N S T R U C T I O N SG R A D E1-21.You have 45 minutes to solve 18 multiple choice problems. For eachproblem, circle only one of the proposed five choices. If you circle more than one choice, your response will be marked as wrong.2.Record your answers in the response form. Remember that this is the onlysheet that is marked, so make sure you have all your answers transferred to the response form before giving it back to the contest supervisor.3.The problems are arranged in three groups. A correct answer of the first 6problems is worth 3 points. A correct answer of the problems 7-12 is worth4 points. A correct answer of the problems 13-18 is worth5 points. Foreach incorrect answer, one point is deducted from your score. Each unanswered question is worth 0 points. To avoid negative scores, you start from 18 points. The maximum score possible is 90.4.The use of external material or aid of any kind is not permitted.5.The figures are not drawn to scale. They should be used only for illustrationpurposes.6.Remember, you have about 2 to 3 minutes for each problem; hence, if aproblem appears to be too difficult, save it for later and move on to another problem.7.At the end of the allotted time, please give the response form to thecontest supervisor.8.Do not forget to pick up your Certificate of Participation on your way out!Good luck!Canadian Math Kangaroo Contest teamCanadian Math Kangaroo ContestPart A: Each correct answer is worth 3 points1.Which shape cannot be formed using and ?(A) (B) (C) (D) (E)2.At least how many 4-ray stars like this are glued together tomake this shape ?(A) 5 (B) 6 (C) 7 (D) 8 (E) 93.This pizza was divided into equal slices.How many slices are missing?(A) 1 (B) 2 (C) 3 (D) 4 (E) 54.How many kangaroos must be moved from one park to the other in order toget the same number of kangaroos in each park?(A) 4 (B) 5 (C) 6 (D) 8 (E) 95.Which of these ladybugs has to fly away so that the rest of them have 20dots in total?(A) (B) (C) (D) (E)6.Emilie builds towers in the following patternWhich one will be the tower number 6?(A) (B) (C) (D) (E)Part B: Each correct answer is worth 4 points7.If ◊+ ◊ = 4 and ∆ + ∆ + ∆ = 9, what is the value of ◊ + ∆ = ?(A) 2 (B) 3 (C) 4 (D) 5 (E) 68.Lisa has 4 pieces , but she only needs 3 forcompleting her puzzle frame . Which piece will be left over?(A)(B)(C)(D) (E)or9.How many right hands are in this picture?(A) 3 (B) 4 (C) 5 (D) 6 (E) 710.The dog went to its food following a path. In total it made 3 right turns and2 left turns. Which path did the dog follow?(A) (B) (C)(D) (E)11.What number is in the box marked "?" ?(A) 6 (B) 13 (C) 24 (D) 29 (E) Some other number12.Charles cut a rope in three equal pieces and then made some equal knotswith them. Which figure correctly shows the three pieces with the knots?(A) (B)(C) (D)(E)Part C: Each correct answer is worth 5 points13.How many circles and how many squares are covered by the blot in thepicture?(A) 1 circle and 3 squares(B) 2 circles and 1 square(C) 3 circles and 1 square(D) 1 circles and 2 squares(E) 2 circles and 2 squares14.Diana shoots three arrows at a target.On her first try, she gets 6 points and the arrows land like this: 6 pointsOn her second try, she gets 8 points and the arrows land like this: 8 pointsOn her third try, the arrows land like this:? points How many points will she get the third time?(A) 8 (B) 10 (C) 12 (D) 14 (E) 1615.How many different numbers greater than 10 and smaller than 25 with distinct digits can we make by using any two of the digits 2, 0, 1, and 8?(A) 4 (B) 5 (C) 6 (D) 7 (E) 816.Mark had some sticks of length 5 cm and width 1 cm.With the sticks he constructed the fence below.What is the length of the fence?(A) 20 cm(B) 21 cm(C) 22 cm (D) 23 cm (E) 25 cmlength17.The road from Anna's house to Mary's house is 16 km long.The road from Mary's house to John's house is 20 km long.The road from the crossroad to Mary's house is 9 km long.How long is the road from Anna’s house to John's house?(A) 7 km (B) 9 km (C) 11 km (D) 16 km (E) 18 km18.There are four ladybugs on a 4×4 board. Two are asleep and do not move.The other two ladybugs move one square every minute (up, down, left, or right). Here are pictures of the board for the first four minutes:Minute 1 Minute 2 Minute 3 Minute 4Which of these is a picture of the fifth minute (Minute 5)?(A) (B) (C) (D) (E)International Contest-GameMath Kangaroo Canada, 2018Answer KeyGrade 1-21 A B C D E 7 A B C D E 13 A B C D E2 A B C D E 8 A B C D E 14 A B C D E3 A B C D E 9 A B C D E 15 A B C D E4 A B C D E 10 A B C D E 16 A B C D E5 A B C D E 11 A B C D E 17 A B C D E6 A B C D E 12 A B C D E 18 A B C D E。
加拿大国家中小学数学竞赛( kangaroo math 袋鼠竞赛)2018年三四年级(含答案)

I N T E R N A T I O N A L C O N T E S T-G A M EM A T H K A N G A R O OC A N AD A,2018I N S T R U C T I O N SG R A D E3-41.You have 60 minutes to solve 24 multiple choice problems. For each problem,circle only one of the proposed five choices. If you circle more than one choice, your response will be marked as wrong.2.Record your answers in the response form. Remember that this is the only sheetthat is marked, so make sure you have all your answers transferred to the response form before giving it back to the contest supervisor.3.The problems are arranged in three groups. A correct answer of the first 8problems is worth 3 points. A correct answer of the problems 9-16 is worth 4 points. A correct answer of the problems 17-24 is worth 5 points. For each incorrect answer, one point is deducted from your score. Each unanswered question is worth 0 points. To avoid negative scores, you start from 24 points. The maximum score possible is 120.4.The use of external material or aid of any kind is not permitted.5.The figures are not drawn to scale. They should be used only for illustrationpurposes.6.Remember, you have about 2 to 3 minutes for each problem; hence, if a problemappears to be too difficult, save it for later and move on to another problem.7.At the end of the allotted time, please give the response form to the contestsupervisor.8.Do not forget to pick up your Certificate of Participation on your way out!Good luck!Canadian Math Kangaroo Contest teamCanadian Math Kangaroo Contest Part A: Each correct answer is worth 3 points1.Lea has 10 rubber stamps. Each stamp has one of the digits:0, 1, 2, 3, 4, 5, 6, 7, 8, 9.She prints the date of St. Patrick’s Day 2018:How many different stamps does she use?(A) 5(B) 6 (C) 7 (D) 9 (E) 102.The picture shows three flying arrows and nine fixedballoons. When an arrow hits a balloon, it bursts,and the arrow flies further in the same direction.How many balloons will be hit by the arrows?(A) 2 (B) 3 (C) 4(D) 5 (E) 63.Susan is six years old. Her sister is one year younger, and her brother is one yearolder. What is the sum of the ages of the three siblings?(A) 10 (B) 15 (C) 18 (D) 21 (E) 304.Here is a picture of Sophie the ladybug. She turns around. Which picture ofthe ladybugs below is not Sophie?(A)(B)(C)(D)(E)5.Lucy folds a sheet of paper in half. Then she cuts a piece out of it. What willshe see when she unfolds the paper?(A) (B) (C) (D)(E)1 70320186. A table is set for 8 people.How many settings have the fork to the left of the plate and the knife to the right of the plate?(A) 5(B) 4 (C) 6 (D) 2 (E) 3 7.Emily added two 2-digit numbers correctly on paper. Then she painted out two cells,as shown below.What is the sum of two digits in the painted cells?(A) 5(B) 7 (C) 8 (D) 9 (E) 13 8.First, Diana scores 12 points in total with three arrows. On her second turn shescores 15 points.How many points does she score on her third turn?(A) 18 (B) 19 (C) 20 (D) 21 (E) 22 Part B: Each correct answer is worth 4 points9.How many different numbers greater than 12 and smaller than 58 with distinct digitscan we make by using any two of the digits 0, 1, 2, 5, and 8?(A) 3(B) 5(C) 7 (D) 8 (E) 912 points15 points ? points10.Roberto makes designs using tiles like this .How many of the following five designs can he make?(A) 1 (B) 2 (C) 3 (D) 4 (E) 511.Each of these five figures ,, , , , appears exactly once in everycolumn and every row of the given table.Which figure must we put in the cell with the question mark?(A) (B) (C) (D) (E)12.Toby glues 10 cubes together to make the structure shown.He paints the whole structure, even the bottom.How many cubes are painted on exactly four of their faces?(A) 6 (B) 7 (C) 8 (D) 9 (E) 1013.The opposite faces of a cube are identical, being dark, bright or patterned.Which picture below is the unfolded net of this cube?(A)14.Tom cuts two types of pieces out of grid paper.What is the smallest number of pieces identical to the ones shown that Tom needs to build the boat in the picture?(A) 5 (B) 6 (C) 7 (D) 8 (E) 915.The rooms in Kanga's house are numbered. Baby Roo entersthe main door, passes through some rooms and leaves thehouse. The numbers of the rooms that he visits are alwaysincreasing. Through which door does he leave the house?(A) A (B) B (C) C (D) D (E) E16.Peta rabbit had 20 carrots. She ate two carrots every day. She ate the twelfth carroton Wednesday. On which day did she start eating the carrots?(A) Monday (B) Tuesday (C) Wednesday (D) Thursday (E) FridayPart C: Each correct answer is worth 5 points17.The belt shown in the drawing can be fastened in five ways.How much longer is the belt fastened in one hole than the belt fastened in all five holes?(A) 4 cm (B) 8 cm (C) 10 cm (D) 16 cm (E) 20 cm18.In an ancient writing the symbols represent thenumbers 1, 2, 3, 4, and 5. Nobody knows which symbol represents which number.We know thatWhich symbol represents the number 3?(A)(B) (C) (D) (E)19. A stained-glass tile is flipped along the black line. The figure shows the tile after thefirst flip.What will the stained-glass tile look like after the third flip (at the far right)?(A)(B)(C)(D)(E)20.The large rectangle is made up of squares of varied sizes. The three smallest squareseach have an area of 1, as shown.What is the area of the largest square?(A) 81 (B) 100 (C) 110 (D) 121 (E) 14421.Five ducklings walk behind the mother duck in a row from the oldest to the youngestlike this: Dina and Becca walk right one after the other, Mingo walks behind Lisa butin front of Becca, Becca walks directly in front of Pip. What is the name of theyoungest duckling?(A) Dina (B) Pip (C) Becca (D) Lisa (E) Mingo22.Four balls each weigh 10, 20, 30 and 40 grams. Which ball weighs 30 grams?(A) A (B) B (C) C (D) D (E) it could be A or B23.Lois wants to write the numbers from 1 to 7 in the grid shown.Two consecutive numbers cannot be written in two neighbouringcells. Neighbouring cells meet at the edge or at a corner. Whatnumbers can she write in the cell marked with a question mark?(A) all seven numbers (B) only odd numbers(C) only even numbers (D) only number 4(E) only the numbers 1 or 7 24.The distance from Anna's to Mary's house is 16 kilometers along the shown road.The distance from Mary's to Nick's house is 20 kilometers.The distance from Nick's to John's house is 19 kilometers.How far is Anna's house from John's?(A) 15 (B) 16(C) 18(D) 19 (E) 20 ?International Contest-GameMath Kangaroo Canada, 2018Answer KeyGrade 3-41 A B C D E 9 A B C D E17 A B C D E2 A B C D E10 A B C D E 18 A B C D E3 A B C D E 11 A B C D E 19 A B C D E4 A B C D E 12 A B C D E 20 A B C D E5 A B C D E 13 A B C D E21 A B C D E6 A B C D E 14 A B C D E 22 A B C D E7 A B C D E 15 A B C D E 23 A B C D E8 A B C D E 16 A B C D E24 A B C D E。
袋鼠数学-3年级-Kangroo sample Primary-3 2018

Singapore Math Kangaroo Contest 2018Rough Working–0points|Wrong–deduct1point)has one of the digits:0,1,2,3,4,5,6,7,8and9.Sheas shown below.How many stamps does she use?810320(A)5(B)6(C)7(D)9(E)10Question2The picture shows3flying arrows and9fixed balloons.When an arrow hits a balloon,it bursts, and the arrowflies further in the same direction.How many balloons will be hit by the arrows?(A)2(B)3(C)4(D)5(E)6Question3Susan is6years old.Her sister is one year younger and her brother is one year older.What is the sum of the ages of the three siblings?(A)10(B)15(C)18(D)21(E)30Question4The picture showsfive screws in a block.Among the5screws,only1of them is shorter than the other4screws.Which screw is the shortest?(A)1(B)2(C)3(D)4(E)5Question5The picture of the ladybird is shown below.Which option is not the same ladybird?(A)(B)(C)(D)(E)Question6Lucy folds a sheet of paper in half.Then she cuts a piece out of it as shown in the picture below. What will she see when she unfolds the paper?(A)(B)(C)(D)(E)Question7In herfirst try,Diana scores12points in total with three arrows.On her second try she scores15 points.How much points does(A)18(B)19(C)20(D)21(E)22Question8Mike sets the table for8guests as shown in the picture below.He wants to serve each guest with the correct arrangement,which means a fork on the left of each plate and a knife on the right.How many guest will have the correct(A)5(B)4(C)6(D)2(E)3|Wrong–deduct1point)Question9Roberto makes designs using tiles like this.How many of the5designs can he make?(A)1(B)2(C)3(D)4(E)5Question10Albertfills the grid below withfivefigures.Eachfigure appears exactly once in every column and every row.Whichfigure must mark?(A(B)(C)(D)(E)Question11Tom wants to cover the boat completely using the2types of shapes,a square and a trapezium as shown in the picture below.If no shapes can overlap each other,what is the least number of square and trapezium pieces does Tom needs to cover the boat completely?(A)5(B)6(C)7(D)8(E)9The colours in the picture below are inverted.Then the picture was rotated.What does the new picture looklike?(A )11111Question 13A rabbit has 20carrots.It eats 2carrots every day.If it ate the 12th carrot on Wednesday,which day did the rabbit start eating the carrots?(A )Monday (B )Tuesday (C )Wednesday (D )Thursday (E )Friday Question 14Toby glues 10cubes together to make the structure shown below.He paints the surface of the structure,including the bottom.How many cubes 4faces painted?(A )6(B )7(C )8(D )9(E )10Question 15There are 8flowers on a rose bush.There are no more than one insect per flower.More than half of the flowers are occupied.The number of butterflies on the flowers is twice the number of dragonflies on the flowers.How many butterflies are on the flowers?(A )2(B )3(C )4(D )5(E )6Kook wants to sail from the island called Easter through every island on the map and back to The total journey is 100km long.Some of the distances between each island have been written in the picture as shown below.For example,the distance between Easter Island andVolcano Island is 17km.The distance between Desert Island and Lake Island is the sameas the distance between Easter Island and Flower Island through Volcano Island.What is the distance between Easter Island and Lake Island?(A )17km (B )23km (C )26km (D )33km (E )35kmSection C (Correct –5points |Unanswered –0points |Wrong –deduct 1point)Question 17The rooms in Kanga’s house are numbered from 1to 14.Baby Roo enters the main door as indicated by the arrow show in the picture below.He passes through some rooms before leaving the house in one of the doors labeled A,B,C,D and E.The numbers of the rooms that he visits are always increasing.Through which door does he leave the house?(A )A (B )B (C )C (D )D (E )EQuestion 18Four balls each weigh 10,weighs 30?(A )A(B )B (C )C (D )D(E )It could be A or B2018–Primary 3/Grade 3in five ways.How much longer is the band fastened (A )4cm (B )8cm (C )10cm (D )16cm (E )20cmQuestion 20In an ancient language the symbolsIt is knownthat:represent the followingnumbers 1,2,3,4,and 5.Nobody knows which symbol represents which number.We know that:It is known that:Which (A )t is known that:It is known that:It is known that:It is known that:)It is known that:(A )(B )(C )(D )(E )Singapore Math Kangaroo Contest 2018–Primary 3/Grade 3Question 22The large rectangle is made up of different sized squares as shown in the picture below.The 3small squares each have an area of 1.1(A )165(B )176(C )187(D )198(E )200Question 23Loes wants to write the numbers from 1to 7in the boxes shown below.Two consecutive numbers cannot be written in twoneighbouring boxes.Neighbouring boxes share a common side.What numbers can she write in the box marked with a question mark?(A )all seven numbers (B )only odd numbers (C )only even numbers (D )only number 4(E )only the numbers 1or 7Question 24To defeat a dragon Mathias has to cut offall the dragon’s heads.If he can cut off3dragon’s heads,one new head immediately grows.Mathias defeats the dragon by cutting off13heads in total.How many heads did the dragon have in the beginning?(A )8(B )9(C )10(D )11(E )12Rough Working。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
I N T E R N A T I O N A L C O N T E S T-G A M EM A T H K A N G A R O OC A N AD A,2018I N S T R U C T I O N SG R A D E1-21.You have 45 minutes to solve 18 multiple choice problems. For eachproblem, circle only one of the proposed five choices. If you circle more than one choice, your response will be marked as wrong.2.Record your answers in the response form. Remember that this is the onlysheet that is marked, so make sure you have all your answers transferred to the response form before giving it back to the contest supervisor.3.The problems are arranged in three groups. A correct answer of the first 6problems is worth 3 points. A correct answer of the problems 7-12 is worth4 points. A correct answer of the problems 13-18 is worth5 points. Foreach incorrect answer, one point is deducted from your score. Each unanswered question is worth 0 points. To avoid negative scores, you start from 18 points. The maximum score possible is 90.4.The use of external material or aid of any kind is not permitted.5.The figures are not drawn to scale. They should be used only for illustrationpurposes.6.Remember, you have about 2 to 3 minutes for each problem; hence, if aproblem appears to be too difficult, save it for later and move on to another problem.7.At the end of the allotted time, please give the response form to thecontest supervisor.8.Do not forget to pick up your Certificate of Participation on your way out!Good luck!Canadian Math Kangaroo Contest teamCanadian Math Kangaroo ContestPart A: Each correct answer is worth 3 points1.Which shape cannot be formed using and ?(A) (B) (C) (D) (E)2.At least how many 4-ray stars like this are glued together tomake this shape ?(A) 5 (B) 6 (C) 7 (D) 8 (E) 93.This pizza was divided into equal slices.How many slices are missing?(A) 1 (B) 2 (C) 3 (D) 4 (E) 54.How many kangaroos must be moved from one park to the other in order toget the same number of kangaroos in each park?(A) 4 (B) 5 (C) 6 (D) 8 (E) 95.Which of these ladybugs has to fly away so that the rest of them have 20dots in total?(A) (B) (C) (D) (E)6.Emilie builds towers in the following patternWhich one will be the tower number 6?(A) (B) (C) (D) (E)Part B: Each correct answer is worth 4 points7.If ◊+ ◊ = 4 and ∆ + ∆ + ∆ = 9, what is the value of ◊ + ∆ = ?(A) 2 (B) 3 (C) 4 (D) 5 (E) 68.Lisa has 4 pieces , but she only needs 3 forcompleting her puzzle frame . Which piece will be left over?(A)(B)(C)(D) (E)or9.How many right hands are in this picture?(A) 3 (B) 4 (C) 5 (D) 6 (E) 710.The dog went to its food following a path. In total it made 3 right turns and2 left turns. Which path did the dog follow?(A) (B) (C)(D) (E)11.What number is in the box marked "?" ?(A) 6 (B) 13 (C) 24 (D) 29 (E) Some other number12.Charles cut a rope in three equal pieces and then made some equal knotswith them. Which figure correctly shows the three pieces with the knots?(A) (B)(C) (D)(E)Part C: Each correct answer is worth 5 points13.How many circles and how many squares are covered by the blot in thepicture?(A) 1 circle and 3 squares(B) 2 circles and 1 square(C) 3 circles and 1 square(D) 1 circles and 2 squares(E) 2 circles and 2 squares14.Diana shoots three arrows at a target.On her first try, she gets 6 points and the arrows land like this: 6 pointsOn her second try, she gets 8 points and the arrows land like this: 8 pointsOn her third try, the arrows land like this:? points How many points will she get the third time?(A) 8 (B) 10 (C) 12 (D) 14 (E) 1615.How many different numbers greater than 10 and smaller than 25 with distinct digits can we make by using any two of the digits 2, 0, 1, and 8?(A) 4 (B) 5 (C) 6 (D) 7 (E) 816.Mark had some sticks of length 5 cm and width 1 cm.With the sticks he constructed the fence below.What is the length of the fence?(A) 20 cm(B) 21 cm(C) 22 cm (D) 23 cm (E) 25 cmlength17.The road from Anna's house to Mary's house is 16 km long.The road from Mary's house to John's house is 20 km long.The road from the crossroad to Mary's house is 9 km long.How long is the road from Anna’s house to John's house?(A) 7 km (B) 9 km (C) 11 km (D) 16 km (E) 18 km18.There are four ladybugs on a 4×4 board. Two are asleep and do not move.The other two ladybugs move one square every minute (up, down, left, or right). Here are pictures of the board for the first four minutes:Minute 1 Minute 2 Minute 3 Minute 4Which of these is a picture of the fifth minute (Minute 5)?(A) (B) (C) (D) (E)International Contest-GameMath Kangaroo Canada, 2018Answer KeyGrade 1-21 A B C D E 7 A B C D E 13 A B C D E2 A B C D E 8 A B C D E 14 A B C D E3 A B C D E 9 A B C D E 15 A B C D E4 A B C D E 10 A B C D E 16 A B C D E5 A B C D E 11 A B C D E 17 A B C D E6 A B C D E 12 A B C D E 18 A B C D EI N T E R N A T I O N A L C O N T E S T-G A M EM A T H K A N G A R O OC A N AD A,2018I N S T R U C T I O N SG R A D E3-41.You have 60 minutes to solve 24 multiple choice problems. For each problem,circle only one of the proposed five choices. If you circle more than one choice, your response will be marked as wrong.2.Record your answers in the response form. Remember that this is the only sheetthat is marked, so make sure you have all your answers transferred to the response form before giving it back to the contest supervisor.3.The problems are arranged in three groups. A correct answer of the first 8problems is worth 3 points. A correct answer of the problems 9-16 is worth 4 points. A correct answer of the problems 17-24 is worth 5 points. For each incorrect answer, one point is deducted from your score. Each unanswered question is worth 0 points. To avoid negative scores, you start from 24 points. The maximum score possible is 120.4.The use of external material or aid of any kind is not permitted.5.The figures are not drawn to scale. They should be used only for illustrationpurposes.6.Remember, you have about 2 to 3 minutes for each problem; hence, if a problemappears to be too difficult, save it for later and move on to another problem.7.At the end of the allotted time, please give the response form to the contestsupervisor.8.Do not forget to pick up your Certificate of Participation on your way out!Good luck!Canadian Math Kangaroo Contest teamGrade 3-42018 Canadian Math Kangaroo ContestPart A: Each correct answer is worth 3 points1.Lea has 10 rubber stamps. Each stamp has one of the digits:0, 1, 2, 3, 4, 5, 6, 7, 8, 9.She prints the date of St. Patrick’s Day 2018:How many different stamps does she use?(A) 5(B) 6 (C) 7 (D) 9 (E) 102.The picture shows three flying arrows and nine fixedballoons. When an arrow hits a balloon, it bursts,and the arrow flies further in the same direction.How many balloons will be hit by the arrows?(A) 2 (B) 3 (C) 4(D) 5 (E) 63.Susan is six years old. Her sister is one year younger, and her brother is one yearolder. What is the sum of the ages of the three siblings?(A) 10 (B) 15 (C) 18 (D) 21 (E) 304.Here is a picture of Sophie the ladybug. She turns around. Which picture ofthe ladybugs below is not Sophie?(A)(B)(C)(D)(E)5.Lucy folds a sheet of paper in half. Then she cuts a piece out of it. What willshe see when she unfolds the paper?(A)(B)(C) (D)(E)1 70320186. A table is set for 8 people.How many settings have the fork to the left of the plate and the knife to the right of the plate?(A) 5(B) 4 (C) 6 (D) 2 (E) 3 7.Emily added two 2-digit numbers correctly on paper. Then she painted out two cells,as shown below.What is the sum of two digits in the painted cells?(A) 5(B) 7 (C) 8 (D) 9 (E) 13 8.First, Diana scores 12 points in total with three arrows. On her second turn shescores 15 points.How many points does she score on her third turn?(A) 18 (B) 19 (C) 20 (D) 21 (E) 22 Part B: Each correct answer is worth 4 points9.How many different numbers greater than 12 and smaller than 58 with distinct digitscan we make by using any two of the digits 0, 1, 2, 5, and 8?(A) 3(B) 5 (C) 7(D) 8 (E) 912 points15 points ? points10.Roberto makes designs using tiles like this .How many of the following five designs can he make?(A) 1 (B) 2 (C) 3 (D) 4 (E) 511.Each of these five figures ,, , , , appears exactly once in everycolumn and every row of the given table.Which figure must we put in the cell with the question mark?(A) (B) (C) (D) (E)12.Toby glues 10 cubes together to make the structure shown.He paints the whole structure, even the bottom.How many cubes are painted on exactly four of their faces?(A) 6 (B) 7 (C) 8 (D) 9 (E) 1013.The opposite faces of a cube are identical, being dark, bright or patterned.Which picture below is the unfolded net of this cube?(A)14.Tom cuts two types of pieces out of grid paper.What is the smallest number of pieces identical to the ones shown that Tom needs to build the boat in the picture?(A) 5 (B) 6 (C) 7 (D) 8 (E) 915.The rooms in Kanga's house are numbered. Baby Roo entersthe main door, passes through some rooms and leaves thehouse. The numbers of the rooms that he visits are alwaysincreasing. Through which door does he leave the house?(A) A (B) B (C) C (D) D (E) E16.Peta rabbit had 20 carrots. She ate two carrots every day. She ate the twelfth carroton Wednesday. On which day did she start eating the carrots?(A) Monday (B) Tuesday (C) Wednesday (D) Thursday (E) FridayPart C: Each correct answer is worth 5 points17.The belt shown in the drawing can be fastened in five ways.How much longer is the belt fastened in one hole than the belt fastened in all five holes?(A) 4 cm (B) 8 cm (C) 10 cm (D) 16 cm (E) 20 cm18.In an ancient writing the symbols represent thenumbers 1, 2, 3, 4, and 5. Nobody knows which symbol represents which number.We know thatWhich symbol represents the number 3?(A)(B) (C) (D) (E)19. A stained-glass tile is flipped along the black line. The figure shows the tile after thefirst flip.What will the stained-glass tile look like after the third flip (at the far right)?(A)(B)(C)(D)(E)20.The large rectangle is made up of squares of varied sizes. The three smallest squareseach have an area of 1, as shown.What is the area of the largest square?(A) 81 (B) 100 (C) 110 (D) 121 (E) 14421.Five ducklings walk behind the mother duck in a row from the oldest to the youngestlike this: Dina and Becca walk right one after the other, Mingo walks behind Lisa but in front of Becca, Becca walks directly in front of Pip. What is the name of theyoungest duckling?(A) Dina (B) Pip (C) Becca (D) Lisa (E) Mingo22.Four balls each weigh 10, 20, 30 and 40 grams. Which ball weighs 30 grams?(A) A (B) B (C) C (D) D (E) it could be A or B23.Lois wants to write the numbers from 1 to 7 in the grid shown.Two consecutive numbers cannot be written in two neighbouringcells. Neighbouring cells meet at the edge or at a corner. Whatnumbers can she write in the cell marked with a question mark?(A) all seven numbers (B) only odd numbers(C) only even numbers (D) only number 4(E) only the numbers 1 or 7 24.The distance from Anna's to Mary's house is 16 kilometers along the shown road.The distance from Mary's to Nick's house is 20 kilometers.The distance from Nick's to John's house is 19 kilometers.How far is Anna's house from John's?(A) 15 (B) 16(C) 18(D) 19 (E) 20 ?International Contest-GameMath Kangaroo Canada, 2018Answer KeyGrade 3-41 A B C D E 9 A B C D E17 A B C D E2 A B C D E10 A B C D E 18 A B C D E3 A B C D E 11 A B C D E 19 A B C D E4 A B C D E 12 A B C D E 20 A B C D E5 A B C D E 13 A B C D E21 A B C D E6 A B C D E 14 A B C D E 22 A B C D E7 A B C D E 15 A B C D E 23 A B C D E8 A B C D E 16 A B C D E24 A B C D EI N T E R N A T I O N A L C O N T E S T-G A M EM A T H K A N G A R O OC A N AD A,2018I N S T R U C T I O N SG R A D E5-121.You have 75 minutes to solve 30 multiple choice problems. For eachproblem, circle only one of the proposed five choices. If you circle more than one choice, your response will be marked as wrong.2.Record your answers in the response form. Remember that this is theonly sheet that is marked, so make sure you have all your answers transferred to that form before giving it back to the contest supervisor. 3.The problems are arranged in three groups. A correct answer of the first10 problems is worth 3 points. A correct answer of problems 11 -20 isworth 4 points. A correct answer of problems 21-30 is worth 5 points. For each incorrect answer, one point is deducted from your score. Each unanswered question is worth 0 points. To avoid negative scores, you start from 30 points. The maximum score possible is 150.4.The use of external material or aid of any kind is not permitted.5.The figures are not drawn to scale. They should be used only forillustration purposes.6.Remember, you have about 2 to 3 minutes for each problem; hence, if aproblem appears to be too difficult, save it for later and move on to another problem.7.At the end of the allotted time, please give the response form to thecontest supervisor.8.Do not forget to pick up your Certificate of Participation on your way out!Good luck!Canadian Math Kangaroo Contest teamCanadian Math Kangaroo ContestPart A: Each correct answer is worth 3 points1.The drawing shows 3flying arrows and 9fixed balloons. Whenan arrow hits a balloon, it bursts, and the arrow flies further inthe same direction. How many balloons will not be hit byarrows?(A) 3 (B) 2(C) 6(D) 5(E) 42.The image shows a structure made of three objects.What does Peter see if he looks at the structure from above?(A)(B)(C) (D) (E)3.Diana played darts throwing arrows toward a target with three sections. First she got 14 points with twoarrows on the target. The second time she got 16 points. How many points did she get the third time?(A) 17(B) 18(C) 19 (D) 20 (E) 22 4. A garden is divided into identical squares. A fast snail and a slow snail move along the perimeter of thegarden starting simultaneously from the corner S but in different directions. The slow snail moves at the speed of 1 metre per hour (1 m/h) and the fast one at 2 metres per hour (2 m/h).At what point will the two snails meet?(A) A (B) B (C) C (D) D(E) E 14 points16 points ? A B CDE S 1 m/h2 m/h5.In which of the four squares is the fraction of the black area the largest?(A) A (B) B (C) C (D) D (E) they are all the same6. A star is made out of four equilateral triangles and a square. The perimeter of thesquare is 36 cm. What is the perimeter of the star?(A) 144 cm (B) 120 cm (C) 104 cm (D) 90 cm (E) 72 cm7.From the list 3, 5, 2, 6, 1, 4, 7 Masha chose 3 different numbers whose sum is 8. From the same list Dashachose 3 different numbers whose sum is 7. How many common numbers have been chosen by both girls?(A) none (B) 1 (C) 2 (D) 3 (E) impossible to determine8.We move a bead along a piece of wire. What shall we see when the beadcomes to the end of the wire?(A) (B) (C)(D) (E)9.There are 3squares in the figure. The side length of the smallest square is 6 cm.What is the side length of the biggest square?(A) 8(B) 10(C) 12(D) 14(E) 1610.In the following figure, the circles are light bulbs connected to some other lightbulbs. Initially, all light bulbs are off. When you touch a light bulb, this light bulband all its neighbours (e.g., the light bulbs connected to it) are lit.At least how many light bulbs do you have to touch to turn on all the light bulbs?(A) 2 (B) 3 (C) 4 (D) 5 (E) 6Part B: Each correct answer is worth 4 points11.Each square contains one of the numbers 1, 2, 3, 4, or 5, so that both of thecalculations following the arrows are correct. A number may be used morethan once. What number goes into the box with the question mark?(A) 1 (B) 2 (C) 3 (D) 4 (E) 5 12. Nine cars arrive at a crossroads and drive off as indicated by the arrows. Which figure shows these cars after leaving the crossroads?(A)(B) (C) (D) (E) 13. The faces of a cube are painted black, white or grey so that opposite faces are of different colour. Which of the following is not a possible net of this cube?(A)(B) (C) (D) (E)14.In a box there are many one-euro, two-euro and five-euro coins. A dispenser draws coins out of the box – one at a time, and stops when three identical coins are taken out. What is the largest possible amount that can be withdrawn? (A) 24 (B) 23 (C) 22 (D) 21 (E) 1515.Two girls, Eva and Olga and three boys, Adam, Isaac and Urban play with a ball. When a girl has the ball, she throws it to the other girl or to a boy. When a boy has the ball, he throws it to another boy but never to the boy from whom he just received it. Eva starts by throwing the ball to Adam. Who will do the fifth throw?(A) Adam (B) Eva (C) Isaac (D) Olga (E) Urban16.Emily wants to enter a number into each cell of the triangular table. The sum of thenumbers in any two cells with a common edge must be the same. She has alreadyentered two numbers. What is the sum of all the numbers in the table?(A) 18 (B) 20 (C) 21 (D) 22 (E) impossible to determine17.John coded a correct addition calculation naming the digits AA , BB , CC and DD .Which digit is represented by BB ?(A) 0 (B) 2 (C) 4 (D) 5(E) 6 + A B C C B A D D DD18.On Monday Alexandra shares a picture with 5 friends. For several days, everybody who receives thepicture, sends it once on the next day to two friends. On which day does the number of people who have seen the picture (including Alexandra) become greater than 75, if it is known that no one receives the picture more than once?(A) Wednesday (B) Thursday (C) Friday (D) Saturday (E) Sunday 19.The sum of the ages of Kate and her mother is 36, and the sum of the ages of her mother and her grandmother is 81. How old was the grandmother when Kate was born? (A) 28 (B) 38 (C) 45 (D) 53 (E) 56 20.Annie replaced the letters with numbers in the word KANGAROO (identical letters with the same digits, different letters with different digits) so that she got the largest possible 8-digit number, which is not a multiple of 4. What is the sum of the last three digits replacing the word ROO? (A) 13 (B) 14 (C) 12 (D) 15 (E) 11Part C: Each correct answer is worth 5 points21.Captain Hook has plundered a safe that contains 2520 gold coins. During the night, each of his pirates secretly took out some coins just for themselves. The first one took out �12�of the coins, the second one�13�of the remaining coins, the third one �14�of the remaining coins and so on. When Captain Hook opened the safe in the morning, he found only 252 coins inside. How many pirates are commanded by Captain Hook?(A) 8 (B) 9 (C) 10 (D) 11 (E) 12 22.In the figure on the right, the five balls A, B, C, D and E weigh 30, 50, 50, 50 and 80 grams, but not necessarily in this order. Which ball weighs 30 grams? (A) A (B) B (C) C (D) D (E) E23.If A, B, C are distinct digits, which of the following numbers cannot be the largest possible 6-digit number written using three digits A, two digits B, and one digit C? (A) AAABBC (B) CAAABB (C) BBAAAC (D) AAABCB (E) AAACBB 24.In the World of Numbers, there are many number-machines, which work in the following way: the machine adds the two beginning digits of the number and replaces them by their sum. For example, beginning with the number 87312 and using six such machines we obtain:How many such machines should be used in order to get the number times509...9 from the numbertimes1009...9? (A) 50(B) 60(C) 100(D) 80(E) Not possible to obtain this number8731215312 6312 91210212 3Page 525.Nick wants to arrange the numbers 2, 3, 4, ..., 10 into several groups such that the sum of the numbers in each group is the same. What is the largest number of groups he can get?(A) 2 (B) 3 (C) 4 (D) 6 (E) other answer 26.Peter cut an 8-cm wide wooden plank with a saw into 9 parts across the width of the plank.One piece was a square, the other were rectangles. Then he arranged all the pieces together as shown in the picture. What was the length of the plank?(A) 150 cm (B) 168 cm (C) 196 cm (D) 200 cm (E) 232 cm 27.Write 0 or 1 in each cell of the 5×5 table so that each 2×2 square of the 5×5 table contains exactly 3 equal numbers. What is the largest possible sum of all the numbers in the table?(A) 22 (B) 21 (C) 19 (D) 17 (E) 1528.14 people are seated at a round table.Each person is either a liar or tells the truth. Everybody says: "Both my neighbours are liars". What is themaximum number of liars at the table?(A) 7 (B) 8 (C) 9(D) 10(E) 1429.There are eight domino tiles on the table (pic 1). One half of one tile is covered. The 8 tiles can be arranged into a 4×4 square (pic 2), so that the number of dots in each row and column is the same.How many dots are on the covered part? (A) 1 (B) 2 (C) 3 (D) 4(E) 530.Four ladybugs sit on different cells of a 4×4 grid.One of them is sleeping and does not move. Each time you whistle, the other three ladybugs move toa free neighbouring cell. They can move up, down,right or left but they are not allowed to go back tothe cell they just came from. Which of the following images might show the result after the fourth whistle?(A)(B)(C)(D)(E)pic 1pic 2initial position after firstwhistleafter second whistle after third whistle Both my neighboursare liars.International Contest-GameMath Kangaroo Canada, 2018Answer KeyGrade 5-61 A B C D E 11 A B C D E21 A B C D E2 A B C D E 12 A B C D E 22 A B C D E3 A B C D E 13 A B C D E23 A B C D E4 A B C D E 14 A B C D E 24 A B C D E5 A B C D E15 A B C D E 25 A B C D E6 A B C D E16 A B C D E 26 A B C D E7 A B C D E 17 A B C D E 27 A B C D E8 A B C D E 18 A B C D E 28 A B C D E9 A B C D E 19 A B C D E 29 A B C D E10 A B C D E 20 A B C D E30 A B C D EI N T E R N A T I O N A L C O N T E S T-G A M EM A T H K A N G A R O OC A N AD A,2018I N S T R U C T I O N SG R A D E5-121.You have 75 minutes to solve 30 multiple choice problems. For eachproblem, circle only one of the proposed five choices. If you circle more than one choice, your response will be marked as wrong.2.Record your answers in the response form. Remember that this is theonly sheet that is marked, so make sure you have all your answers transferred to that form before giving it back to the contest supervisor. 3.The problems are arranged in three groups. A correct answer of the first10 problems is worth 3 points. A correct answer of problems 11 -20 isworth 4 points. A correct answer of problems 21-30 is worth 5 points. For each incorrect answer, one point is deducted from your score. Each unanswered question is worth 0 points. To avoid negative scores, you start from 30 points. The maximum score possible is 150.4.The use of external material or aid of any kind is not permitted.5.The figures are not drawn to scale. They should be used only forillustration purposes.6.Remember, you have about 2 to 3 minutes for each problem; hence, if aproblem appears to be too difficult, save it for later and move on to another problem.7.At the end of the allotted time, please give the response form to thecontest supervisor.8.Do not forget to pick up your Certificate of Participation on your way out!Good luck!Canadian Math Kangaroo Contest teamPage 1Canadian Math Kangaroo ContestPart A: Each correct answer is worth 3 points1.When the letters of the word MAMA are written vertically above one another, the word has a vertical line of symmetry. Which of these words also has a vertical line of symmetry when written in the same way?(A) ROOT (B) BOOM (C) BOOT (D) LOOT (E) TOOT2.A triangle has sides of length 6, 10 and 11. An equilateral triangle has the same perimeter. What is the length of each side of the equilateral triangle?(A) 6 (B) 9 (C) 10 (D) 11 (E) 273.Which number should replace ∗in the equation 2 ∙ 18 ∙ 14 = 6 ∙ ∗ ∙ 7to make it correct?(A) 8 (B) 9 (C) 10 (D) 12 (E) 154.The panels of Fergus' fence are full of holes. One morning, one of the panels fell flat on the floor.Which of the following could Fergus see as he approaches his fence?(A) (B) (C) (D) (E)5.How many possible routes are there to go from A to B in the direction indicated by the arrows?(A) 2 (B) 3 (C) 4 (D) 5 (E) 66.Martha multiplied two 2-digit numbers correctly on a piece of paper.Then she scribbled out three digits as shown.What is the sum of the three digits she scribbled out? (A) 5 (B) 6 (C) 9 (D) 12 (E) 14 7.A large rectangle is made up of nine identical rectangles whose longest sides are 10 cm long. What is the perimeter of the large rectangle?(A) 40 cm(B) 48 cm(C) 76 cm(D) 81 cm(E) 90 cm8. A hotel on an island in the Caribbean advertises using the slogan "350 days of sun every year!''. According tothe advert, what is the smallest number of days Willi Burn has to stay at the hotel in 2018 to be certain of having two consecutive days of sun?(A) 17 (B) 21 (C) 31 (D) 32 (E) 359.The diagram shows a rectangle of dimensions 7 × 11 containing two circles eachtouching three of the sides of the rectangle. What is the distance between the centres of the two circles?(A) 1 (B) 2(C) 3(D) 4 (E) 510.Only one of the digits in the year 2018 is a prime number. How many years will pass till the next year whenall of the digits in the year number are prime numbers?(A) 201 (B) 202 (C) 203 (D) 204 (E) 205Part B: Each correct answer is worth 4 points11.Square AAAAAAAA has sides of length 3 cm. The points MM and NN lie on AAAA and AAAA so that AAMMand AANN split the square into three pieces of the same area. What is the length of AAMM?(A) 0.5 cm (B) 1 cm (C) 1.5 cm (D) 2 cm (E) 2.5 cm12.A rectangle is divided into 40 identical squares. The rectangle contains more than one row of squares. Avacoloured the middle row. What is the largest possible number of squares that remain uncoloured?(A) 20 (B) 30 (C) 32 (D) 35 (E) 3913.A lion is hidden in one of three rooms. A note on the door of room 1 reads "The lion is here". A note on thedoor of room 2 reads "The lion is not here". A note on the door of room 3 reads "2+3=2×3". Only one of these statements is true. In which room is the lion hidden?(A) In room 1 (B) In room 2 (C) In room 3 (D) It may be in any room(E) It may be in either room 1 or room 214.Valeriu draws a zig-zag line inside a rectangle, creating angles of 10°,14°,33°, and 26°as shown.What is the size of angle θθ?(A) 11°(B) 12°(C) 16°(D) 17°(E)33°。
