2017-2018年美国“数学大联盟杯赛”(中国赛区)初赛高中年级试卷及答案

2017-2018年度美国“数学大联盟杯赛”(中国赛区)初赛
(十、十一、十二年级)
(初赛时间:2017年11月26日,考试时间90分钟,总分300分)
学生诚信协议:考试期间,我确定没有就所涉及的问题或结论,与任何人、用任何方式交流或讨论,
我确定我所填写的答案均为我个人独立完成的成果,否则愿接受本次成绩无效的处罚。
请在装订线内签名表示你同意遵守以上规定。
考前注意事项:
1. 本试卷是十、十一、十二年级试卷,请确保和你的参赛年级一致;
2. 本试卷共4页(正反面都有试题),请检查是否有空白页,页数是否齐全;
3. 请确保你已经拿到以下材料:
本试卷(共4页,正反面都有试题)、答题纸、英文词汇手册、草稿纸。考试完
毕,请务必将英文词汇手册带回家,上面有如何查询初赛成绩、及如何参加复
赛的说明。其他材料均不能带走,请留在原地。
填空题(每小题10分,答对加10分,答错不扣分,共300分。)
1.Each pirate wants his own treasure chest, but there is 1 more pirate than there
are treasure chests. If the pirates would agree to pair up so each pirate
shares a treasure chest with another pirate, then 1 treasure chest would
not be assigned to any pirate. How many treasure chests are there?
Answer: ________________.
2.If m and n
are positive integers that satisfy 10
=, what is the greatest possible
value of m + n?
Answer: ________________.
3.There are an infinite number of points with positive coordinates
(x,y) the sum of whose coordinates is the square of an integer.
Among all such points (x,y), which one satisfies y = 2x and has
x as small as possible?
Answer: ________________.
4.As shown, a small square is inscribed in one of the triangles formed when
both diagonals of a larger square are drawn. If the area of the larger square
is 144, what is the area of the smaller square?
Answer: ________________.
5.Trisection points on opposite sides of a rectangle are joined, as shown. If
the area of the shaded region is 2018, what is the area of the rectangle?
Answer: ________________.
6. A unit fraction is a fraction whose numerator is 1 and whose
denominator is a positive integer. What is the largest rational
number that can be written as the sum of 3 different unit
fractions?
Answer: ________________.
7.What is the greatest possible perimeter of a rectangle whose length and width are different
prime numbers, each less than 120?
Answer: ________________.
8.Mom, Dad, and I each write a positive integer. My number is least
and Dad's is greatest. The average of all 3 numbers is 20. The
average of the 2 smallest numbers is 8. If Dad's number is d and
if my number is m, what is the greatest possible value of d–m?
Answer: ________________.
9.If 8 different integers are chosen at random from the first 15 positive integers, what is the
probability that an additional number chosen at random from the remaining 7 positive
integers is smaller than every one of the 8 originally chosen positive integers?
Answer: ________________.
10.What sequence of 5 positive integers has these three properties:
1) All but one of the numbers is a multiple of 5.
2) Every number after the first is 1 more than the sum of all the preceding numbers.
3) The first number is as small as possible.
Answer: ________________.
11.Three beavers (one not shown) take turns biting a tree until it falls. The
second beaver is twice as likely as the first to make the tree fall. The
third is twice as likely as the second to make the tree fall. What is
the probability that a bite taken by the third beaver causes the
tree to fall?
Answer: ________________.
12.What is the ratio, larger to smaller, of a rectangle's dimensions if half
of the rectangle is similar to the original rectangle?
Answer: ________________.
第1页,共4页第2页,共4页
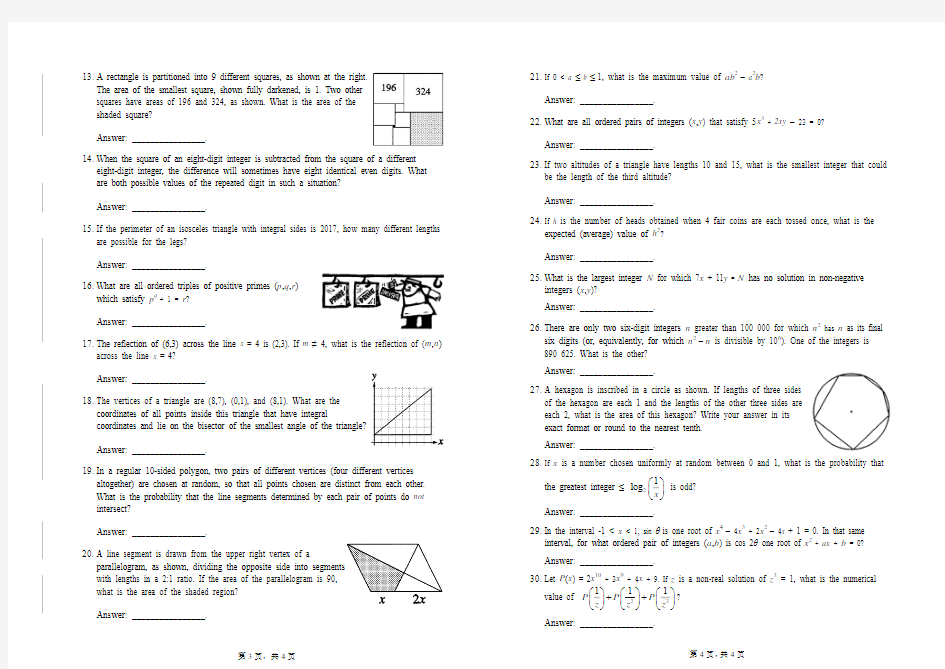
A rectangle is partitioned into 9 different squares, as shown at the right. The area of the smallest square, shown fully darkened, is 1. Two other squares have areas of 196 and 324, as shown. What is the area of the shaded square? Answer: ________________.
When the square of an eight-digit integer is subtracted from the square of a different
eight-digit integer, the difference will sometimes have eight identical even digits. What are both possible values of the repeated digit in such a situation? Answer: ________________.
If the perimeter of an isosceles triangle with integral sides is 2017, how many different lengths
are possible for the legs? Answer: ________________.
What are all ordered triples of positive primes (p ,q ,r ) which satisfy p q + 1 = r ? Answer: ________________.
The reflection of (6,3) across the line x = 4 is (2,3). If m ≠ 4, what is the reflection of (m ,n )
across the line x = 4? Answer: ________________.
The vertices of a triangle are (8,7), (0,1), and (8,1). What are the
coordinates of all points inside this triangle that have integral
coordinates and lie on the bisector of the smallest angle of the triangle? Answer: ________________.
In a regular 10-sided polygon, two pairs of different vertices (four different vertices
altogether) are chosen at random, so that all points chosen are distinct from each other. What is the probability that the line segments determined by each pair of points do not intersect? Answer: ________________.
A line segment is drawn from the upper right vertex of a
parallelogram, as shown, dividing the opposite side into segments with lengths in a 2:1 ratio. If the area of the parallelogram is 90, what is the area of the shaded region?
Answer: ________________.
21. If 0 < a ≤ b ≤ 1, what is the maximum value of ab 2 – a 2b ? Answer: ________________.
22. What are all ordered pairs of integers (x ,y ) that satisfy 5x 3 + 2xy – 23 = 0? Answer: ________________.
23. If two altitudes of a triangle have lengths 10 and 15, what is the smallest integer that could
be the length of the third altitude?
Answer: ________________.
24. If h is the number of heads obtained when 4 fair coins are each tossed once, what is the
expected (average) value of h 2? Answer: ________________.
25. What is the largest integer N for which 7x + 11y = N has no solution in non-negative
integers (x ,y )? Answer: ________________.
26. There are only two six-digit integers n greater than 100 000 for which n 2 has n as its final
six digits (or, equivalently, for which n 2 – n is divisible by 106). One of the integers is 890 625. What is the other?
Answer: ________________.
27. A hexagon is inscribed in a circle as shown. If lengths of three sides
of the hexagon are each 1 and the lengths of the other three sides are each 2, what is the area of this hexagon? Write your answer in its exact format or round to the nearest tenth. Answer: ________________.
28. If x is a number chosen uniformly at random between 0 and 1, what is the probability that
the greatest integer ≤ 21log x ??
??? is odd?
Answer: ________________.
29. In the interval -1 < x < 1, sin θ is one root of x 4 – 4x 3 + 2x 2 – 4x + 1 = 0. In that same
interval, for what ordered pair of integers (a ,b ) is cos 2θ one root of x 2 + ax + b = 0? Answer: ________________.
30. Let P (x ) = 2x 10 + 3x 9 + 4x + 9. If z is a non-real solution of z 3 = 1, what is the numerical
value of 23111P P P z z z ??????
++ ? ? ???????
?
Answer: ________________.
第3页,共4页
第4页,共4页
HIMCM 2014美国中学生数学建模竞赛试题
HIMCM 2014美国中学生数学建模竞赛试题 Problem A: Unloading Commuter Trains Trains arrive often at a central Station, the nexus for many commuter trains from suburbs of larger cities on a “commuter” line. Most trains are long (perhaps 10 or more cars long). The distance a passenger has to walk to exit the train area is quite long. Each train car has only two exits, one near each end so that the cars can carry as many people as possible. Each train car has a center aisle and there are two seats on one side and three seats on the other for each row of seats.To exit a typical station of interest, passengers must exit the car, and then make their way to a stairway to get to the next level to exit the station. Usually these trains are crowded so there is a “fan” of passengers from the train trying to get up the stairway. The stairway could accommodate two columns of people exiting to the top of the stairs.Most commuter train platforms have two tracks adjacent to the platform. In the worst case, if two fully occupied trains arrived at the same time, it might take a long time for all the passengers to get up to the main level of the station.Build a mathematical model to estimate the amount of time for a passenger to reach the street level of the station to exit the complex. Assume there are n cars to a train, each car has length d. The length of the platform is p, and the number of stairs in each staircase is q. Use your model to specifically optimize (minimize) the time traveled to reach street level to exit a station for the following: 问题一:通勤列车的负载问题 在中央车站,经常有许多的联系从大城市到郊区的通勤列车“通勤”线到达。大多数火车很长(也许10个或更多的汽车长)。乘客走到出口的距离也很长,有整个火车区域。每个火车车厢只有两个出口,一个靠近终端, 因此可以携带尽可能多的人。每个火车车厢有一个中心过道和过道两边的座椅,一边每排有两个座椅,另一边每排有三个座椅。走出这样一个典型车站,乘客必须先出火车车厢,然后走入楼梯再到下一个级别的出站口。通常情况下这些列车都非常拥挤,有大量的火车上的乘客试图挤向楼梯,而楼梯可以容纳两列人退出。大多数通勤列车站台有两个相邻的轨道平台。在最坏的情况下,如果两个满载的列车同时到达,所有的乘客可能需要很长时间才能到达主站台。建立一个数学模型来估计旅客退出这种复杂的状况到达出站口路上的时间。假设一列火车有n个汽车那么长,每个汽车的长度为d。站台的长度是p,每个楼梯间的楼梯数量是q。使用您的模型具体来优化(减少)前往主站台的时间,有如下要求: Requirement 1. One fully occupied train's passengers to exit the train, and ascend the stairs to reach the street access level of the station. 要求1.一个满载乘客的火车,所有乘客都要出火车。所有乘客都要出楼梯抵达出主站台的路上。 Requirement 2. Two fully occupied trains' passengers (all passengers exit onto a common platform) to exit the trains, and ascend the stairs to reach the street access level
AMC美国高中数学竞赛难题精选
F组题 1.Jar A contains four liters of a solution that is 45% acid. Jar B contains five liters of a solution that is 48% acid. Jar C contains one liter of a solution that is acid. From jar C, liters of the solution is added to jar A, and the remainder of the solution in jar C is added to jar B. At the end both jar A and jar B contain solutions that are 50% acid. Given that and are relatively prime positive integers, find . Answer: Solution:Omited. Resource: 2011 AIME I Problems1 2.Let be the line with slope that contains the point , and let be the line perpendicular to line that contains the point . The original coordinate axes are erased, and line is made the -axis and line the -axis. In the new coordinate system, point is on the positive -axis, and point is on the positive -axis. The point with coordinates in the original system has coordinates in the new coordinate system. Find . Answer: Solution:Omited. Resource: 2011 AIME I Problems3 3. Suppose that a parabola has vertex and equation , where and is an integer. The minimum possible value of can be written in the form , where and are relatively prime positive integers. Find . Answer: Solution:Omited. Resource: 2011 AIME I Problems6 4. Suppose is in the interval and . Find .
2018年美国数学竞赛 AMC 试题
2018 AIME I Problems Problem 1 Let be the number of ordered pairs of integers with and such that the polynomial can be factored into the product of two (not necessarily distinct) linear factors with integer coefficients. Find the remainder when is divided by . Problem 2 The number can be written in base as , can be written in base as , and can be written in base as , where . Find the base- representation of . Problem 3 Kathy has red cards and green cards. She shuffles the cards and lays out of the cards in a row in a random order. She will be happy if and only if all the red cards laid out are adjacent and all the green cards laid out are adjacent. For example, card orders RRGGG, GGGGR, or RRRRR will make Kathy happy, but RRRGR will not. The probability that Kathy will be happy is , where and are relatively prime positive integers. Find . Problem 4 In and . Point lies strictly between and on and point lies strictly between and on so that . Then can be expressed in the form , where and are relatively prime positive integers. Find . Problem 5 For each ordered pair of real numbers satisfying there is a real number such that
2018年美国“数学大联盟杯赛”(中国赛区)初赛三年级试卷及答案
2017-2018年度美国“数学大联盟杯赛”(中国赛区)初赛 (三年级) (初赛时间:2017年11月26日,考试时间90分钟,总分200分) 学生诚信协议:考试期间,我确定没有就所涉及的问题或结论,与任何人、用任何方式交流或讨论, 我确定我所填写的答案均为我个人独立完成的成果,否则愿接受本次成绩无效的处罚。 请在装订线内签名表示你同意遵守以上规定。 考前注意事项: 1. 本试卷是三年级试卷,请确保和你的参赛年级一致; 2. 本试卷共4页(正反面都有试题),请检查是否有空白页,页数是否齐全; 3. 请确保你已经拿到以下材料: 本试卷(共4页,正反面都有试题)、答题卡、答题卡使用说明、英文词汇手册、草稿纸。考试完毕,请务必将英文词汇手册带回家,上面有如何查询初赛成绩、及如何参加复赛的说明。其他材料均不能带走,请留在原地。 选择题:每小题5分,答对加5分,答错不扣分,共200分,答案请填涂在答题卡上。 1. 5 + 6 + 7 + 1825 + 175 = A) 2015 B) 2016 C) 2017 D) 2018 2.The sum of 2018 and ? is an even number. A) 222 B) 223 C) 225 D) 227 3.John and Jill have $92 in total. John has three times as much money as Jill. How much money does John have? A) $60 B) $63 C) $66 D) $69 4.Tom is a basketball lover! On his book, he wrote the phrase “ILOVENBA” 100 times. What is the 500th letter he wrote? A) L B) B C) V D) N 5.An 8 by 25 rectangle has the same area as a rectangle with dimensions A) 4 by 50 B) 6 by 25 C) 10 by 22 D) 12 by 15 6.What is the positive difference between the sum of the first 100 positive integers and the sum of the next 50 positive integers? A) 1000 B) 1225 C) 2025 D) 5050 7.You have a ten-foot pole that needs to be cut into ten equal pieces. If it takes ten seconds to make each cut, how many seconds will the job take? A) 110 B) 100 C) 95 D) 90 8.Amy rounded 2018 to the nearest tens. Ben rounded 2018 to the nearest hundreds. The sum of their two numbers is A) 4000 B) 4016 C) 4020 D) 4040 9.Which of the following pairs of numbers has the greatest least common multiple? A) 5,6 B) 6,8 C) 8,12 D) 10,20 10.For every 2 pencils Dan bought, he also bought 5 pens. If he bought 10 pencils, how many pens did he buy? A) 25 B) 50 C) 10 D) 13 11.Twenty days after Thursday is A) Monday B) Tuesday C) Wednesday D) Thursday 12.Of the following, ? angle has the least degree-measure. A) an obtuse B) an acute C) a right D) a straight 13.Every student in my class shouted out a whole number in turn. The number the first student shouted out was 1. Then each student after the first shouted out a number that is 3 more than the number the previous student did. Which number below is a possible number shouted out by one of the students? A) 101 B) 102 C) 103 D) 104 14.A boy bought a baseball and a bat, paying $1.25 for both items. If the ball cost 25 cents more than the bat, how much did the ball cost? A) $1.00 B) $0.75 C) $0.55 D) $0.50 15.2 hours + ? minutes + 40 seconds = 7600 seconds A) 5 B) 6 C) 10 D) 30 16.In the figure on the right, please put digits 1-7 in the seven circles so that the three digits in every straight line add up to 12. What is the digit in the middle circle? A) 3 B) 4 C) 5 D) 6 17.If 5 adults ate 20 apples each and 3 children ate 12 apples in total, what is the average number of apples that each person ate? A) 12 B) 14 C) 15 D) 16 18.What is the perimeter of the figure on the right? Note: All interior angles in the figure are right angles or 270°. A) 100 B) 110 C) 120 D) 160 19.Thirty people are waiting in line to buy pizza. There are 10 people in front of Andy. Susan is the last person in the line. How many people are between Andy and Susan? A) 18 B) 19 C) 20 D) 21
2018年美国“数学大联盟杯赛”(中国赛区)初赛五年级试卷(1)
2017-2018年度美国“数学大联盟杯赛”(中国赛区)初赛 (五年级) (初赛时间:2017年11月26日,考试时间90分钟,总分200分) 学生诚信协议:考试期间,我确定没有就所涉及的问题或结论,与任何人、用任何方式交流或讨论, 我确定我所填写的答案均为我个人独立完成的成果,否则愿接受本次成绩无效的处罚。 请在装订线内签名表示你同意遵守以上规定。 考前注意事项: 1. 本试卷是五年级试卷,请确保和你的参赛年级一致; 2. 本试卷共4页(正反面都有试题),请检查是否有空白页,页数是否齐全; 3. 请确保你已经拿到以下材料: 本试卷(共4页,正反面都有试题)、答题卡、答题卡使用说明、英文词汇手册、 草稿纸。考试完毕,请务必将英文词汇手册带回家,上面有如何查询初赛成绩、 及如何参加复赛的说明。其他材料均不能带走,请留在原地。 选择题:每小题5分,答对加5分,答错不扣分,共200分,答案请填涂在答题卡上。 1. The smallest possible sum of two different prime numbers is A) 3 B) 4 C) 5 D) 6 2. The greatest common factor of two numbers is 3. The product of these two numbers must be divisible by A) 6 B) 9 C) 12 D) 18 3. The sum of 5 consecutive one-digit integers is at most A) 15 B) 25 C) 35 D) 45 4. How many two-digit multiples of 10 are also multiples of 12? A) 4 B) 3 C) 2 D) 1 5. I have read exactly 1 3 of the total number of chapters in my 120-page book. If each chapter has the same whole number of pages, then the total number of chapters I have left could be A) 16 B) 24 C) 32 D) 50 6. What is the greatest odd factor of 44 × 55 × 66? A) 36 B) 55 C) 35 × 55 D) 36 × 55 7. What is the sum of the factors of the prime number 2017? A) 2016 B) 2017 C) 2018 D) 2019 8. Lynn ran in 6 times as many races as the number of races she won. How many of her 126 races did Lynn not win? A) 21 B) 90 C) 96 D) 105 9. The least common multiple of 8 and 12 is the greatest common factor of 120 and A) 80 B) 124 C) 144 D) 180 10. January has the greatest possible number of Saturdays when January 1 occurs on any of the following days of the week except A) Thursday B) Friday C) Saturday D) Sunday 11. The number that is 10% of 1000 is 10 more than 10% of A) 90 B) 100 C) 900 D) 990 12. The sum of 16 fours has the same value as the product of ? fours. A) 2 B) 3 C) 4 D) 16 13. Of the following, which is the sum of two consecutive integers? A) 111 111 B) 222 222 C) 444 444 D) 888 888 14. Abe drove for 2 hours at 30 km/hr. and for 3 hours at 50 km/hr. What was Abe’s average speed over the 5 hours? A) 35 km/hr. B) 40 km/hr. C) 42 km/hr. D) 45 km/hr. 15. My broken watch runs twice as fast as it should. If my watch first broke at 6:15 P.M., what time was displayed on my watch 65 minutes later? A) 7:20 P.M. B) 7:25 P.M. C) 8:20 P.M. D) 8:25 P.M. 16. (2018 × 2017) + (2018 × 1) = A) 20172 B) 20182 C) 20183 D) (2018 + 2017)2 17. A prized bird lays 2, 3, or 4 eggs each day. If the bird laid 17 eggs in 1 week, on at most how many days that week did the bird lay exactly 2 eggs? A) 2 B) 3 C) 4 D) 5 18. Of the following, which could be the perimeter of a rectangle whose side-lengths, in cm, are prime numbers? A) 10 cm B) 22 cm C) 34 cm D) 58 cm 19. The average of all possible total values of a 4-coin stack of nickels and dimes (containing at least one of each coin) is A) 20¢ B) 30¢ C) 40¢ D) 60¢ 20. The diameter of Ann’s drum i s 40 cm more than the radius. What is half the circumference of the drum? A) 120π cm B) 80π cm C) 60π cm D) 40π cm 21. Of the following, which expression has the greatest number of factors that are multiples of 2018? A) 2018 × 12 B) 20182 C) 20192 D) 20192019 第1页,共4页 第2页,共4页
AMC美国数学竞赛AMCB试题及答案解析
2003 AMC 10B 1、Which of the following is the same as 2、Al gets the disease algebritis and must take one green pill and one pink pill each day for two weeks. A green pill costs more than a pink pill, and Al’s pills cost a total of for the two weeks. How much does one green pill cost? 3、The sum of 5 consecutive even integers is less than the sum of the ?rst consecutive odd counting numbers. What is the smallest of the even integers? 4、Rose fills each of the rectangular regions of her rectangular flower bed with a different type of flower. The lengths, in feet, of the rectangular regions in her flower bed are as shown in the ?gure. She plants one flower per square foot in each region. Asters cost 1 each, begonias each, cannas 2 each, dahlias each, and Easter lilies 3 each. What is the least possible cost, in dollars, for her garden? 5、Moe uses a mower to cut his rectangular -foot by -foot lawn. The swath he cuts is inches wide, but he overlaps each cut by inches to make sure that no grass is missed. He walks at the rate of
美国高中数学测试题 MathPlacement
Emma Willard School Troy, New York 12180 MATHEMATICS PLACEMENT TEST Purpose: The tests in this booklet are to help determine proper mathematics placement and minimize the need for course changes after the start of the academic year. It is important that the student work independently so that the test will give us a fair representation of her current knowledge and skills. The tests are for placement purposes only. They do not affect admissions decisions in any way. However, it is important to answer questions to the best of your ability in order for the mathematics department to place you properly. Date student received test:_________________ Name: (please print)_______________________________ Date test taken:___________ Circle grade you are entering at Emma Willard School: 9 10 11 12 PG Phone number (with area code): _________________________________________________ E-mail address: _____________________________________________ (print legibly please) Name of most recent school attended and the city and state/country where it is located. ______________________________________________________________________________ What math course did you take this year, and what is your average grade at the time you are taking this test? ______________________________________________________________________________ Have you taken a full-year course called Geometry? Circle your answer. YES NO If you were remaining in your current school district or at your current school, what would be the name of the course you would take next year? ______________________________________________________________________________ How do you view yourself as a mathematics student?
美国数学大联盟杯赛五年级试卷
2015-2016年度美国“数学大联盟杯赛”(中国赛区)初赛 (五年级) (初赛时间:2015年11月14日,考试时间90分钟,总分200分) 学生诚信协议:考试期间,我确定没有就所涉及的问题或结论,与任何人、用任何方式交流或讨论, 我确定以下的答案均为我个人独立完成的成果,否则愿接受本次成绩无效的处罚。 如果您同意遵守以上协议请在装订线内签名 选择题:每小题5分,答对加5分,答错不扣分,共200分,答案请填涂在答题卡上。 1. A 6 by 6 square has the same area as a 4 by ? rectangle. A) 3 B) 6 C) 8 D) 9 2.Every prime has exactly ? positive divisors. A) 1 B) 2 C) 3 D) 4 or more 3.If I answered 34 out of 40 questions on my math test correctly, I answered ? % of the questions correctly. A) 75 B) 80 C) 85 D) 90 4.120 ÷ 3 ÷ 4 × 12 = A) 1 B) 10 C) 12 D) 120 5.10 × 20 × 30 × 40 = 24 ×? A) 1000 B) 10 000 C) 100 000 D) 1000 000 6.One of my boxes contains 1 pencil and the others each contain 5 pencils. If there are 101 pencils in my boxes, how many boxes do I have? A) 19 B) 20 C) 21 D) 22 7.An electrical company imports 2016 light bulbs. Unfortunately, 25% of those are damaged. How many light bulbs are not damaged? A) 25 B) 504 C) 1512 D) 2016 8.50 × (16 + 24) is the square of A) -40 B) -4 C) 4 D) 80 9.Which of the following numbers has exactly 3 positive divisors? A) 49 B) 56 C) 69 D) 100 10.Ten people stand in a line. Counting from the left, Jerry stands at the 5th position. Counting from the right, which position is he at? A) 4 B) 5 C) 6 D) 7 11.On a teamwork project, Jack contributed 2/7 of the total amount of work, Jill contributed 1/4 of the work, Pat contributed 1/5 of the work, and Matt contributed the rest. Who contributed the most toward this project? A) Jack B) Jill C) Pat D) Matt 12.Which of the following numbers is a factor of 2016? A) 5 B) 11 C) 48 D) 99 13.2 × 4 × 8 × 16 × 32 × 64 = A) 210B) 215C) 221D) 2120 14.On a game show, Al won four times as much as Bob, and Bob won four times as much as Cy. If Al won $1536, how much did Al, Bob, and Cy win together? A) $96 B) $384 C) $1920 D) $2016 15.The sum of two composites cannot be A) odd B) even C) 11 D) 17 16.If a and b are positive integers such that a/b = 5/7, then a + b is A) 12 B) 24 C) 36 D) not able to be determined 17.What is the greatest odd factor of the number of hours in all the days of the year 2015? A) 3 B) 365 C) 1095 D) 3285 18.If the current month is February, what month will it be 1 199 999 months from now? A) January B) February C) March D) April 19.Two angles are complementary. One of these angles is 36° less than the other. What is the measure of the larger angle? A) 36°B) 54°C) 63°D) 72° 20.(The square root of 16) + (the cube root of 64) + (the 4th root of 256) = A) 12 B) 24 C) 32 D) 64 21.In ?ABC, m∠A–m∠B = m∠B–m∠C. What is the degree measure of ∠B? A) 30 B) 60 C) 90 D) 120 22.For every 3 math books I bought, I bought 2 biology books. I bought 55 books in all. How many of those are math books? A) 11 B) 22 C) 33 D) 44 23.John wrote a number whose digits consists entirely of 1s. This number was a composite number. His number could contain exactly ? 1s. A) 17 B) 19 C) 29 D) 32 24.Weird Town uses three types of currencies: Cons, Flegs, and Sels. If 3 Sels = 9 Cons and 2 Cons = 4 Flegs, then 5 Sels = ? Flegs. A) 12 B) 24 C) 30 D) 36 第1页,共4页
美国高中学生数学竞赛题
美国高中学生数学竞赛题 1.(1995年文理)设(3x-1)6=a6x6+a5x5+a4x4+a3x3+a2x2+a1x+a0,求a6+a5+a4+a3+a2+a1+a0的值。 答案:64。 2.(1989年文)如果(1-2x)7=a0+a1x+a2x2+…+a7x7,那么a1+a2+…+a7的值等于() A.-2 B.-1 C.0 D.2 答案:(A) 3.(1989年理)已知(1-2x)7=a0+a1x+a2x2+…+a7x7,那么a1+a2+…+a7=____。 答案:-2。 题源:(美28届10题)若(3x-1)7=a7x7+a6x6+…+a0,那么a7+a6+…+a0等于() A.0 B.1 C.64 D.-64 E.128 答案:(E) 改编点评:1题将指数7改为6,改为简答题;2题将底数(3x-1)改为(1-2x),展开式改为x的升幂排列,所求结论中去掉了常数项a0,3题改编方法同2题,改为填空题。 4.(1990年文)已知f(x)=x5+ax3+bx-8,且f(-2)=10,那么f(2)等于() A.-26 B.-18 C.-10 D.10 答案:(A) 题源:(美33届12题)设f(x)=ax7+bx3+cx-5,其中a.b和c是常数,如图f(-7)=7,那么f(7)等于() A.-17 B.-7 C.14 D.21 E.不能唯一确定 答案:(A) 改编点评:降低了次数,减少了一个字母系数,降低了难度。 5.(1990年文理)如果实数x、y满足等式(x-2)2+y2=3,那么的最大值是() A. B. C. D. 答案:(D)
题源:(美35届29题)在满足方程(x-3)2+(y-3)2=6的实数对(x,y)中,的最大值是() A.3+2 B.2+ C.3 D.6 E.6+2 答案:(A) 改编点评:圆方程中的圆心坐标、半径作了改变,题设的叙述方式也作了变化。 6.(1990年文理)函数y=+++的值域是() A.{-2,4} B.{-2,0,4} C.{-2,0,2,4} D.{-4,-2,0,4} 答案:(B) 题源:(美28届8题)非零实数的每一个三重组(a,b,c)构成一个数。如此构成的所有数的集是() A.{0} B.{-4,0,4} C.{-4,-2,0,2,4} D.{-4,-2,2,4} E.这些都不对 答案:(B) 改编点评:将a、b、c改为三角函数,考查的知识面更广。 7.(1992年文理)长方体的全面积为11,十二条棱长度之和为24,则这个长方体的一条对角线长为() A.2 B. C.5 D.6 答案:(C) 题源:(美35届25题)一个长方体的表面积为22cm2,并且它的所有棱的总长度为24cm,那么它的对角线的长度(按cm计)是() A. B. C. D. E.不能被唯一确定 答案:(D) 改编点评:作了两个方面的变化:将全面积22cm2改为11,去掉单位。 8.(1992年文理)如果函数f(x)=x2+bx+c对任意实数t都有f(2+t)=f(2-t),那么() A.f(2)<f(1)<f(4) B.f(1)<f(2)<f(4)
高中数学竞赛历届IMO竞赛试题届完整中文版
第1届I M O 1.? 求证(21n+4)/(14n+3) 对每个自然数 n都是最简分数。 2.??设√(x+√(2x-1))+√(x-√(2x-1))=A,试在以下3种情况下分别求出x的实数解:? (a) A=√2;(b)A=1;(c)A=2。 3.?a、b、c都是实数,已知 cos x的二次方程 a cos2x + b cos x + c = 0, 试用a,b,c作出一个关于 cos 2x的二次方程,使它的根与原来的方程一样。当 a=4,b=2,c=-1时比较 cos x和cos 2x的方程式。 4.? 试作一直角三角形使其斜边为已知的 c,斜边上的中线是两直角边的几何平均值。 5.? 在线段AB上任意选取一点M,在AB的同一侧分别以AM、MB为底作正方形AMCD、MBEF,这两个正方形的外接圆的圆心分别是P、Q,设这两个外接圆又交于M、N, ??? (a.) 求证 AF、BC相交于N点; ?? (b.) 求证不论点M如何选取直线MN 都通过一定点 S; ??? (c.) 当M在A与B之间变动时,求线断 PQ的中点的轨迹。 6.? 两个平面P、Q交于一线p,A为p上给定一点,C为Q上给定一点,并且这两点都不在直线p上。试作一等腰梯形ABCD(AB平行于CD),使得它有一个内切圆,并且顶点B、D分别落在平面P和Q上。 第2届IMO 1.? 找出所有具有下列性质的三位数 N:N能被11整除且 N/11等于N的各位数字的平方和。 2.? 寻找使下式成立的实数x: 4x2/(1 - √(1 + 2x))2 ?< ?2x + 9
3.? 直角三角形ABC的斜边BC的长为a,将它分成 n 等份(n为奇数),令?为从A点向中间的那一小段线段所张的锐角,从A到BC边的高长为h,求证: tan ? = 4nh/(an2 - a). 4.? 已知从A、B引出的高线长度以及从A引出的中线长,求作三角形ABC。 5.? 正方体ABCDA'B'C'D'(上底面ABCD,下底面A'B'C'D')。X是对角线AC上任意一点,Y是B'D'上任意一点。 a.求XY中点的轨迹; b.求(a)中轨迹上的、并且还满足 ZY=2XZ的点Z的轨迹。 6.? 一个圆锥内有一内接球,又有一圆柱体外切于此圆球,其底面落在圆锥的底面上。令V1为圆锥的体积,V2为圆柱的体积。 ??? (a).? 求证:V1不等于 V2; ??? (b).? 求V1/V2的最小值;并在此情况下作出圆锥顶角的一般。 7.? 等腰梯形ABCD,AB平行于DC,BC=AD。令AB=a,CD=c,梯形的高为 h。X点在对称轴上并使得角BXC、AXD都是直角。试作出所有这样的X点并计算X到两底的距离;再讨论在什么样的条件下这样的X点确实存在。 第3届IMO 1.? 设a、b是常数,解方程组 x + y + z = a; ? ? x2 + y2 + z2 = b2; ? ? xy=z2 并求出若使x、y、z是互不相同的正数,a、b应满足什么条件? 2.? 设a、b、c是某三角形的边,A 是其面积,求证: a2 + b2 + c2>= 4√3 A. 并求出等号何时成立。 3.? 解方程 cos n x - sin n x = 1, 其中n是一个自然数。 4.? P是三角形ABC内部一点,PA交BC于D,PB交AC于E,PC交AB于F,求证AP/PD,
