计算科学大学物理双语A卷(2014)
2024年AP Calculus AB真题集锦

2024年AP Calculus AB真题集锦在2024年的AP Calculus AB考试中,考生们将面临一系列挑战,需要全面掌握各个知识点和技巧来应对。
本文将为大家提供一个真题集锦,帮助大家更好地备考。
第一部分:选择题选择题是AP Calculus AB考试中的重要组成部分,考察学生对基础知识的理解和应用能力。
在这个部分,我们将精选几道典型的选择题,供大家练习。
题目1:设函数f(x)为连续函数,且在区间[0,1]上满足f(0)=0,f(1)=2。
已知f'(x)>0,且在[0,1]上有f''(x)<0,则下列哪一个选项是正确的?A. f(x)在(0,1)内取值为负数B. f(x)在(0,1)内取值为正数C. f(x)在(0,1)内取值为0D. 无法确定f(x)在(0,1)内的取值解析:根据题意可知,函数f(x)在[0,1]上是单调递增的,同时具有凹向下的图像形状。
因此,f(x)在(0,1)内取值为正数,选项B为正确答案。
题目2:已知函数f(x)=2x^3-3x^2-12x+5,求f'(2)的值。
A. 12B. 14C. 16D. 18解析:首先,我们对f(x)进行求导,得到f'(x)=6x^2-6x-12。
将x=2代入f'(x)中,得到f'(2)=6(2)^2-6(2)-12=12。
因此,选项A为正确答案。
第二部分:解答题解答题是AP Calculus AB考试中的较为复杂和综合性要求较高的部分,需要考生们综合运用所学知识来解决问题。
下面,我们选择两道典型的解答题供大家参考。
题目3:已知函数f(x)在区间[-1,3]上连续,且满足f'(x)=2(x-1)和f(1)=4。
若曲线y=f(x)与x轴所围成的面积为8,求函数f(x)在区间[-1,3]上的最小值和最大值。
解析:首先,根据题意可以确定f(x)的一阶导数为f'(x)=2(x-1),再对f'(x)进行积分,得到f(x)=x^2-2x+C,其中C为常数。
大学海洋科学专业《大学物理(二)》期末考试试题 附解析

大学海洋科学专业《大学物理(二)》期末考试试题附解析姓名:______ 班级:______ 学号:______考试须知:1、考试时间:120分钟,本卷满分为100分。
2、请首先按要求在试卷的指定位置填写您的姓名、班级、学号。
一、填空题(共10小题,每题2分,共20分)1、一个质点的运动方程为(SI),则在由0至4s的时间间隔内,质点的位移大小为___________,在由0到4s的时间间用内质点走过的路程为___________。
2、一个绕有500匝导线的平均周长50cm的细螺绕环,铁芯的相对磁导率为600,载有0.3A 电流时, 铁芯中的磁感应强度B的大小为___________;铁芯中的磁场强度H的大小为___________ 。
3、长为的匀质细杆,可绕过其端点的水平轴在竖直平面内自由转动。
如果将细杆置与水平位置,然后让其由静止开始自由下摆,则开始转动的瞬间,细杆的角加速度为_____,细杆转动到竖直位置时角加速度为_____。
4、一长为的均匀直棒可绕过其一端且与棒垂直的水平光滑固定轴转动。
抬起另一端使棒向上与水平面呈60°,然后无初转速地将棒释放,已知棒对轴的转动惯量为,则(1) 放手时棒的角加速度为____;(2) 棒转到水平位置时的角加速度为____。
()5、将热量Q传给一定量的理想气体:(1)若气体的体积不变,则热量转化为_____________________________。
(2)若气体的温度不变,则热量转化为_____________________________。
(3)若气体的压强不变,则热量转化为_____________________________。
6、一质点作半径为0.1m的圆周运动,其运动方程为:(SI),则其切向加速度为=_____________。
7、从统计的意义来解释, 不可逆过程实质上是一个________________的转变过程, 一切实际过程都向着________________ 的方向进行。
大学物理活页作业答案(全套)马文蔚(二)2024

大学物理活页作业答案(全套)马文蔚(二)引言概述:在本文中,我们将提供马文蔚的《大学物理活页作业答案(全套)》第二部分的答案。
该答案集包含了大学物理课程中的各种难题和练习题的解答,将帮助学生更好地理解和掌握物理知识。
下面将分为五个大点,详细阐述每个大点下的小点内容。
1. 力学:- 物体的运动:包括匀速直线运动、加速直线运动、自由落体等运动形式的求解方法;- 牛顿运动定律:分析力的作用、摩擦力、弹力等的计算方法;- 循环运动:旋转、圆周运动等相关知识;- 力的合成与分解:应用向量运算解决力的合成与分解问题;- 动量与能量:动量守恒定律、机械能守恒定律等的应用。
2. 热学:- 温度与热量:温标、热量的单位、热量传递等的概念和计算;- 热力学第一定律:内能和热功的关系,热机效率的计算;- 热传导:导热系数、传热方程等内容;- 热膨胀:线膨胀、面膨胀和体膨胀等相关知识;- 气体定律:理想气体状态方程、等温过程和绝热过程的分析。
3. 光学:- 光的传播:光速、光线传播的规律等;- 光的折射与反射:折射定律、反射定律的应用;- 光的干涉与衍射:双缝干涉、单缝衍射等基本原理;- 光的色散与光谱:光的色散现象、光谱的特性和应用;- 光学仪器:透镜、显微镜、望远镜等光学仪器的工作原理和使用方法。
4. 电磁学:- 静电场:库仑定律、电场强度的计算等;- 电场的能量:电场能的计算、电场的静电势和电势差的概念;- 电流和电阻:电流的计算、欧姆定律的应用;- 磁场:磁感应强度、电流在磁场中受力等基本概念;- 电磁感应:法拉第电磁感应定律、应用于电感和互感等。
5. 物理实验:- 实验仪器与测量:常用物理实验仪器的常规使用方法;- 实验技巧和数据处理:实验数据的处理与分析方法;- 实验设计和报告:实验设计的基本原则、报告撰写的要点;- 实验安全与管理:实验过程中的安全措施和实验室规章制度;- 物理实验的应用与发展:物理实验在科学研究和工程技术中的应用和发展。
Entanglement of the orbital angular momentum states of photons

15.Zhu,X.K.,O'Nions,R.K.,Guo,Y.&Reynolds,B.C.Secular variation of iron isotopes in NorthAtlantic deep water.Science287,2000±2002(2000).16.Belshaw,N.S.,Zhu,X.K.,Guo,Y.&O'Nions,R.K.High precision measurement of iron isotopes byplasma source mass spectrometry.Int.J.Mass.Spectrom.197,191±195(2000).17.Marechal,C.N.,T elouk,P.&AlbareÁde,F.Precise analysis of copper and zinc isotopic compositions byplasma-source mass spectrometry.Chem.Geol.156,251±273(1999).18.Clayton,R.N.&Mayeda,T.K.Formation of ureilites by nebular processes.Geochim.Cosmochim.Acta52,1313±1318(1988).19.Clayton,R.N.&Mayeda,T.K.Oxygen isotope studies of achondrites.Geochim.Cosmochim.Acta60,1999±2017(1996).20.Clayton,R.N.Mayeda,T.K.,Goswami,J.N.&Olsen,E.J.Oxygen isotope studies of ordinarychondrites.Geochim.Cosmochim.Acta55,2317±2337(1991).21.Volkening,J.&Papanastassiou,D.A.Iron isotope anomaly.Astrophys.J.347,L43±L46(1989).22.Volkening,J.&Papanastassiou,D.A.Zinc isotope anomaly.Astrophys.J.358,L29±L32(1990).23.Birck,J.L.&Lugmair,G.W.Nickel and chromium isotopes in Allende inclusions.Earth Planet.Sci.Lett.90,131±143(1988).24.Rotaru,M.,Birck,J.L.&AlleÁgre,C.J.Clue to early solar system history from chromium isotopes incarbonaceous chondrites.Nature358,465±470(1992).25.Podosek,F.A.et al.Thoroughly anomalous chromium in Orgueil.Meteorit.Planet.Sci.32,617±627(1997).26.Niemeyer,S.&Lugmair,G.W.Titanium isotopic anomalies in meteorites.Geochim.Cosmochim.Acta48,1401±1416(1984).27.Niemeyer,S.&Lugmair,G.W.Ubiquitous isotopic anomalies in Ti from normal Allende inclusions.Earth Planet.Sci.Lett.53,211±225(1981).28.Papanastassiou,D.A.Chromium isotopic anomalies in the Allende meteorite.Astrophys.J.308,L27±L30(1986).AcknowledgementsWe thank N.S.Belshaw for assistance with mass spectrometry;A.Galy for discussions and for providing solution aliquots of some samples analysed;G.Turner and F.AlbareÁde for comments on the manuscript;and M.Price for providing samples of the Mt and OUM series.This work was supported by the Natural Environment Research Council. Correspondence and requests for materials should be addressed to X.K.Z.(e-mail:xiangz@). ................................................................. Entanglement of the orbital angular momentum states of photonsAlois Mair*,Alipasha Vaziri,Gregor Weihs&Anton ZeilingerInstitut fuÈr Experimentalphysik,UniversitaÈt Wien,Boltzmanngasse5,1090Wien, Austria .............................................................................................................................................. Entangled quantum states are not separable,regardless of the spatial separation of their components.This is a manifestation of an aspect of quantum mechanics known as quantum non-locality1,2.An important consequence of this is that the measure-ment of the state of one particle in a two-particle entangled state de®nes the state of the second particle instantaneously,whereas neither particle possesses its own well-de®ned state before the measurement.Experimental realizations of entanglement have hitherto been restricted to two-state quantum systems3±6,involv-ing,for example,the two orthogonal polarization states of photons.Here we demonstrate entanglement involving the spatial modes of the electromagnetic®eld carrying orbital angular mo-mentum.As these modes can be used to de®ne an in®nitely dimensional discrete Hilbert space,this approach provides a practical route to entanglement that involves many orthogonal quantum states,rather than just two Multi-dimensional entangled states could be of considerable importance in the®eld of quantum information7,8,enabling,for example,more ef®cient use of communication channels in quantum cryptography9±11. Multi-dimensional entanglement is a wayÐin addition to multi-particle entanglementÐto extend the usual two-dimensional two-particle state.There have been suggestions12,13(and only a proof-of-*Present address:Harvard-Smithsonian Center for Astrophysics,60Garden Street,Cambridge,Massa-chusetts02138,USA.principle experiment14)as to how to realize higher-order entangle-ment via multiport beam splitters.Here we present an experiment in which we used the spatial modes of the electromagnetic®eld carrying orbital angular momentum to create multi-dimensional entanglement.The advantage of using these modes to create entanglement is that they can be used to de®ne an in®nitely dimensional discrete(because of the quantization of angular momentum)Hilbert space.The experimental realization proceeded in the following two steps.First,we con®rmed that spontaneous parametric down-conversion conserves the orbital angular momentum of photons. This was done for pump beams carrying orbital angular momenta of2~,0and ~per photon,respectively.Second,we showed that the state of the down-converted photons can not be explained by assuming classical correlationÐin the sense that the photon pairs produced are just a mixture of the combinations allowed by conservation of angular momentum.We proved that,in contrast, they are a coherent superposition of these combinations,and hence they have to be considered as entangled in their orbital angular momentum.After completion of the experimental work presented here,related theoretical work was brought to our attention15,16. We will now discuss in order the two steps mentioned above.For paraxial light beams,Laguerre±gaussian(LG)modes(Fig.1)de®ne a possible set of basis vectors.As predicted17and observed18,LG modes carry an orbital angular momentum for linearly polarized light that is distinct from the intrinsic angular momentum of photons associated with their polarizations.This external angular momentum of the photon states is the reason why they have been suggested for gearing micromachines,and it has been shown that they can be used as optical tweezers19±21.Figure1Laguerre±gaussian(LG l p)or`doughnut'mode.The index l is referred to as the winding number,and p 1 is the number of radial nodes.Here we only consider cases of p 0.The customary gaussian mode can be viewed as an LG mode with l 0.The handedness of the helical wave fronts of the LG modes is linked to the sign of the index l,and can be chosen by convention.The azimuthal phase term exp il f of the LG modes results in helical wave fronts.The phase variation along a closed path C around the beam centre is2p l. Therefore,in order to ful®l the wave equation,the intensity has to vanish in the centre of the beam.To demonstrate the conservation of the orbital angular momen-tum carried by the LG modes in spontaneous parametric down-conversion,we investigated three different casesÐfor pump photons possessing orbital angular momenta of2~,0and ~ per photon,respectively.As a pump beam,we used an argon-ion laser(wavelength351nm)which we could operate either with a simple gaussian mode pro®le(l 0)or in the®rst-order LG modes (l 61)after astigmatic mode conversion(for a description of this technique,see ref.22).Spontaneous parametric down-conver-sion was done in a1.5-mm-thick BBO(b-barium borate)crystal cut for type-I phase matching(that is,both photons carry the same linear polarization).The crystal cut was chosen so as to produce down-converted photons at a wavelength of702nm at an angle of48 off the pump direction.The mode detection of the down-converted photons was per-formed for gaussian and LG modes.The gaussian mode(l 0)was identi®ed using mono-mode®bres(Fig.2)in connection with avalanche detectors.All other modes have a larger spatial extension, and therefore cannot be coupled into the mono-mode®bre.The LG modes(lÞ0)were identi®ed using mode detectors consisting of computer-generated holograms and mono-mode optical®bres (Fig.2).Computer-generated holograms have often been exploited for creating LG modes of various orders23.Our holograms were phase gratings5mm35mm in size,with20lines mm-1,which we®rst recorded on holographic®lms and bleached afterwards to increase the transmission ef®ciency(Fig.2).We made holograms that had one or two dislocations in the centre,and designed them to have their maximum intensity in the®rst diffraction order.This enabled us to distinguish between LG modes l 22;21;0;1;2using all holograms in the®rst diffraction orderÐfor which they have been blazed.For analysing an LG mode with a negative index,the holograms were rotated by1808around the axis perpendicular to the grating lines.The total transmission ef®ciency of all our holograms was about80%,and they diffracted18%of the incoming beam into the desired®rst order.These characteristics were meas-ured at632nm wavelength because a laser source at702nm was not available.The diffraction ef®ciency is not the only loss that occurs.We also have to account for Fresnel losses at all optical surfaces(95% transmission),imperfect coupling into the optical®bres(70%for a gaussian beam),non-ideal interference®lters(75%centre trans-mission),and the ef®ciency of the detectors(30%).A conservative estimate of all the losses yields an overall collection ef®ciency of2±3%.Comparing the unnormalized(l pump l1 l2 0)coin-cidence rates of about2,000s-1to the single count rates of about 100,000s-1we deduce an ef®ciency of2%,in agreement with the above estimation.The mode analysis was performed in coincidence for all cases where mode®lter1was prepared for analysing LG modes l1 0;1;2 and mode®lter2for those with l2 22;21;0;1;2.For analys-ing an LG mode with mode index l 0Ðthat is,a gaussian modeÐthe dislocation of the hologram was shifted out of the beam path.Thus the beam was sent through the border of the hologram where it acts as a conventional grating without changing the photons'angular momentum.The results are shown in Fig.3for different values of orbital angular momenta of the pump beam.Within experimental accuracy,coincidences were only observed in those cases where the sum of the orbital angular momenta of the down-converted photons was equal to the pump beam's orbital angular momentum.However,the absolute count rates of these cases are not equal.This is probably due to unequal emission probabilities of the photons into the different modes in the down-conversion process.These results con®rm conservation of the orbital angular momentum in parametric down-conversion.The signal-to-noise ratios achieved were as high as V 0:97660:038and V 0:91660:009for pump beams with and without orbital angular momentum,respectively.V is de®ned as I out2I in = I out I in ,where I in and I out denote the maximum and the mini-mum of the coincidences with the dislocation of the hologram respectively in and out of the beam.It is only by using a coincidence measurement that we could show that the conservation of the orbital angular momentum holds for each single photon pair.In contrast,cumulative detection methods using many photons result in an incoherent pattern24,becauseFigure2Experimental set-up for single-photon mode detection.After parametric down-conversion,each of the photons enters a mode detector consisting of a computer-generated hologram and a mono-mode optical®bre.By diffraction at the hologram,the incoming mode undergoes a mode transformation in such a way that an LG mode can be transformed into a gaussian mode.As it has a smaller spatial extension than all LG modes, only the gaussian mode can be coupled into the mono-mode®bre.Thus observation of a click projects the mode incident on the®bre coupler into the gaussian mode.The hologram is a phase grating with D m dislocations in the centre blazed for®rst-order diffraction.An incoming gaussian laser beam passing through the dislocation of the hologram is diffracted by the grating,and the n th diffraction order becomes an LG mode with an index l n D m and vice versa.Intuitively speaking,the phase dislocation exerts a `torque'onto the diffracted beam because of the difference of the local grating vectors in the upper and lower parts of the grating.This`torque'depends on the diffraction order n and on D m.Consequently the right and left diffraction orders gain different handedness. Reversing this process,a photon with angular momentum D m~before the grating can be detected by the mono-mode®bre detector placed in the®rst diffraction order.A photon with zero angular momentum(gaussian mode)is detected by diffracting the beam at the border of the hologram far away from the dislocation.All our measurements were performed in coincidence detection between the two down-converted photons.each beam from parametric down-conversion by itself is an inco-herent mixture.Therefore some previous workers24using these classical detection methodsÐwhich are in principle unsuitable at the single photon levelÐwere led to believe that the orbital angular momentum is not conserved in spontaneous parametric down-conversion.Given this experimental veri®cation of the conservation of orbital angular momentum,entanglement between the two photons pro-duced in the conversion process might be expected.But to explain the conservation of the orbital angular momentum,the photons do not necessarily have to be entangled:it would be suf®cient to assume classical correlation.But further experimental results(see below) showed that the two-photon state goes beyond classical correlation, and indeed,we were able to prove the entanglement for photon states with phase singularities.To con®rm entanglement,we have to demonstrate that the two-photon state is not just a mixture but a coherent superposition of product states of the various gaussian and LG modes which obey angular momentum conservation.For simplicity,we restricted ourselves to superpositions of two basis states only.An important distinction between coherent superposition and incoherent mixture of gaussian and LG modes is that the latter possess no phase singularity.This is because adding the spatial intensity distributions of these two modes will yield a®nite intensity everywhere in the resulting pattern.In contrast,in a coherent superposition the amplitudes are added,and therefore the phase singularity must remain and is displaced to an eccentric location(Fig.4).It will appear at that location where the amplitudes of the two modes are equal,with opposite phase.Therefore the radial distance of the singularity from the beam centre is a measure of the amplitude ratio of the gaussian to the LG components,whereas the angular position of the singularity is determined by their relative phase.Intuitively speaking,the position of the dislocation with respect to the beam is equivalent to the orientation of a polarizer. Superpositions of LG and gaussian modes can be realized experi-mentally by shifting the dislocation of the hologram out of the centre of the beam by a certain(small)amount.Hence in order to detect a photon having an orbital angular momentum that is a superposition of the gaussian and the LG mode,the hologram was placed in a position such that the dislocation was slightly displaced from the beam centre.In the intensity pattern these modes possess an eccentric singularity(Fig.4).To demonstrate the entanglement, we therefore shifted one of the holograms and scanned the gaussian mode®lter on the other side while recording the coincidences. The results shown in Fig.4verify the correlation in superposition bases of the LG(l 62)and gaussian(l 0)modes.A closer analysis shows that there are two conditions necessary to obtain the measured curves.First,the shifted hologram has to work as described above,and second,the source must emit an angular-momentum-entangled state.Assume that the source only emits classically correlated but not entangled singularities.Then on the side with the shifted hologram,the various terms of the classical mixture would be projected onto a state with displaced singularity leaving the total state again in a mixture.Respecting the conserva-tion of angular momentum we would then have to sum the probabilities of the various components on the other side,resulting Figure3photon1and photon2in15possible combinations of orthogonal states were performed.This was done for a pump beam having an orbital angular momentum of2~,0and ~per photon,respectively.Coincidences were observed in all cases where the sum of thethe pump beam'sorbital angular momentum.The coincidence counts for each®xed value of the orbitalangular momentum of photon1were normalized by the total number of coincidencesvarying the orbital angular momentum of photon2.–750+75Detector position (µm)Experimental evidence(left;right,simulation)of entanglementwith phase singularities.The dislocation of the hologram in the beam of photon1is shiftedout of the beam centre step by step(top,middle,bottom).In these positions,thishologramÐtogether with the mono-mode®bre detectorÐprojects the state of photon1into a coherent superposition of LG and gaussian modes.The mode®lter for photon2withthe hologram taken out makes a scan of the second photon's intensity distribution(detector position)in order to identify the location of its singularity with respect to the beamcentre.The coincidences show that the second photon is also detected in a superpositionof LG and gaussian modes.Classical correlation would yield a coincidence picture whichis just a mixture of gaussian and LG modes.In that case,the intensity minimum wouldremain in the beam centre but would become washed out.In the experiment a hologramwith two dislocations in the®rst diffraction order was used.in a coincidence pattern not containing any intensity zeroes.Such a coincidence pattern would also be observed if a shifted hologram together with a mono-mode detector were not able to analyse for superposition states.An entangled state represents correctly both the correlation of the eigenmodes and the correlations of their superpositions.Having experimentally con®rmed the quantum superposition for l 0and l 62,it is reasonable to expect the quantum superposition will also occur for the other states.Nevertheless,ultimate con®rmation of entanglement will be a Bell inequality experiment generalized to more states25.Such an experiment will be a major experimental challenge,and we are preparing to perform it.For a pump beam with zero angular momentum,the emitted state must then be represented byw C0;0j0i j0i C1;21j1i j21i C21;1j21i j1iC2;22j2i j22i C22;2j22i j2i ¼¼1as the LG modes form an in®nite dimensional basis.Here the numbers in the brackets represent the indices l of the LG modes, and the C i,j denote the corresponding probability amplitude for measuring j i i j j i.The state(1)is a multi-dimensional entangled state for two photons,which in general will also contain terms with radial mode index pÞ0.It means neither photon in state (1)possesses a well-de®ned orbital angular momentum after parametric down-conversion.The measurement of one photon de®nes its orbital angular momentum state,and projects the second one into the corresponding orbital angular momentum state.It is conceivable that these states could in the future be extended to multi-dimensional multi-particle entanglement.A growing body of theoretical work calls for entanglement of quantum systems of higher dimensions7,8.These states have applications in quantum cryptography with higher alphabets and in quantum teleportation. As such states increase the¯ux of information,it is conceivable that they could be important for many other applications in quantum communication and in quantum information.The possibility of using these photon states to drive micromachines,and the applica-tion of these states as optical tweezers,make them versatile and potentially suitable for future technologies19±21.M Received12March;accepted5June2001.1.SchroÈdinger,E.Die gegenwaÈrtige Situation in der Quantenmechanik.Naturwissenschaften23,807±812;823±828;844±849(1935).2.SchroÈdinger,E.Discussion of probability relations between separated systems.Proc.Camb.Phil.Soc.31,555±563(1935).3.Bouwmeester,D.,Pan,J.-W.,Daniell,M.,Weinfurter,H.&Zeilinger,A.Observation of a three-photon Greenberger-Horne-Zeilinger state.Phys.Rev.Lett.82,1345±1349(1999).4.Pan,J.-W.,Bouwmeester,D.,Daniell,M.,Weinfurter,H.&Zeilinger,A.Experimental test of quantumnonlocality in three-photon Greenberger-Horne-Zeilinger entanglement.Nature403,515±519 (2000).5.Sackett,C.A.et al.Experimental entanglement of four particles.Nature404,256±259(2000).6.Pan,J.-W.,Daniell,M.,Gasparoni,S.,Weihs,G.&Zeilinger,A.Experimental demonstration of four-photon entanglement and high-®delity teleportation.Phys.Rev.Lett.86,4435±4438(2001).7.DiVincenzo,D.P.,More,T.,Shor,P.W.,Smolin,J.A.&Terhal,B.M.Unextendible product bases,uncompletable product bases and bound entanglement.Preprint quant-ph/9908070ath i(1999).8.Bartlett,S.D.,de Guise,H.&Sanders,B.C.Quantum computation with harmonic oscillators.Preprint quant-ph/0011080at h i(2000).9.Bechmann-Pasquinucci,H.&Peres,A.Quantum cryptography with3-state systems.Phys.Rev.Lett.85,3313±3316(2000).10.Bechmann-Pasquinucci,H.&Tittel,W.Quantum cryptography using larger alphabets.Phys.Rev.A61,62308±62313(2000).11.Bourennane,M.,Karlsson,A.&BjoÈrk,G.Quantum key distribution using multilevel encoding.Phys.Rev.A(in the press).12.Reck,M.,Zeilinger,A.,Bernstein,H.J.&Bertani,P.Experimental realization of any discrete unitaryoperator.Phys.Rev.Lett.73,58±61(1994).13.Zukowski,M.,Zeilinger,A.&Horne,M.Realizable higher-dimensional two-particle entanglementsvia multiport beam splitters.Phys.Rev.A55,2564±2579(1997).14.Reck,M.Quantum Interferometry with Multiports:Entangled Photons in Optical Fibers.Thesis,Univ.Innsbruck(1996).15.Arnaut,H.H.&Barbosa,G.A.Orbital and angular momentum of single photons andentangled pairs of photons generated by parametric down-conversion.Phys.Rev.Lett.85,286±289 (2000).16.Franke-Arnold,S.,Barnett,S.M.,Padgett,M.J.&Allen,L.Two-photon entanglement of orbitalangular momentum states.Phys.Rev.A(in the press).17.Allen,L.,Beijersbergen,M.W.,Spreeuw,R.J.C.&Woerdman,J.P.Orbital angular momentumof light and the transformation of laguerre-gaussian laser modes.Phys.Rev.A45,8185±8189 (1992).18.He,H.,Fries,M.,Heckenberg,N.&Rubinsztein-Dunlop,H.Direct observation of transfer of angularmomentum to absorbtive particles from a laser beam with a phase singularity.Phys.Rev.Lett.75,826±829(1995).19.Simpson,N.B.,Dholakia,K.,Allen,L.&Padgett,M.J.Mechanical equivalence of spin and orbitalangular momentum of light:An optical spanner.Opt.Lett.22,52±54(1997).20.Galajda,P.&Ormos,plex micromachines produced and driven by light.Appl.Phys.Lett.78,249±251(2001).21.Friese,M.E.J.,Enger,J.,Rubinsztein-Dunlop,H.&Heckenberg,N.Optical angular-momentumtransfer to trapped absorbing particles.Phys.Rev.A54,1593±1596(1996).22.Bejersbergen,M.W.,Allen,L.,van der Veen,H.E.L.O.&Woerdman,J.P.Astigmatic laser modeconverters and transfer of orbital angular mun.96,123±132(1993).23.Arlt,J.,Dholakia,K.,Allen,L.&Padgett,M.J.The production of multiringed laguerre-gaussianmodes by computer-generated holograms.J.Mod.Opt.45,1231±1237(1998).24.Arlt,J.,Dholakia,K.,Allen,L.&Padgett,M.Parametric down-conversion for light beams possessingorbital angular momentum.Phys.Rev.A59,3950±3952(1999).25.Kaszlikowski,D.,Gnacinski,P.,Zukowski,M.,Miklaszewski,W.&Zeilinger,A.Violation of localrealism by two entangled n-dimensional systems are stronger than for two qubits.Phys.Rev.Lett.85, 4418±4421(2000).AcknowledgementsThis work was supported by the Austrian Fonds zur FoÈrderung der wissenschaftlichen Forschung(FWF).Correspondence and requests for materials should be addressed to A.Z.(e-mail:anton.zeilinger@univie.ac.at).................................................................. Superconductivity in the non-magnetic state of iron under pressureKatsuya Shimizu*²,Tomohiro Kimura*,Shigeyuki Furomoto*,Keiki Takeda*,Kazuyoshi Kontani*,Yoshichika Onuki³&Kiichi Amaya*²*Department of Physical Science,Graduate School of Engineering Science, Osaka University,Toyonaka,Osaka560-8531,USA²Research Center for Materials Science at Extreme Conditions,Osaka University, Toyonaka,Osaka560-8531,Japan³Department of Physics,Graduate School of Science,Osaka University, Osaka560-0043,Japan .............................................................................................................................................. Ferromagnetism and superconductivity are thought to compete in conventional superconductors,although in principle it is possible for any metal to become a superconductor in its non-magnetic state at a suf®ciently low temperature.At pressures above10GPa, iron is known to transform to a non-magnetic structure1,2and the possibility of superconductivity in this state has been predicted3,4. Here we report that iron does indeed become superconducting at temperatures below2K at pressures between15and30GPa.The transition to the superconducting state is con®rmed by both a drop in resistivity and observation of the Meissner effect.An iron sample with purity of99.995%(Johnson Matthey)was puri®ed further and degassed by heating close to the melting point in an ultra-high-vacuum chamber.The sample was cut into a rectangular shape of0:0430:16030:07mm3and placed in the sample chamber of a non-magnetic diamond-anvil cell(DAC)made of BeCu alloy.For electrical resistivity measurements the BeCu metal gasket was covered with a thin Al2O3layer for electrical insulation.Electrical resistivity measurements are performed using the a.c.four-terminal method with a typical measuring current of0:131026A at low temperatures below10K.The sample chamber was®lled with NaCl as the pressure-transmitting medium(Fig.1a).Several ruby chips of less than0.002mm in diameter were located around the sample and the applied pressure。
06级《大学物理A2》期末考试试卷 (甲卷)

注意事项:1.请在本试卷上直接答题. 2.密封线下面不得写班级,姓名,学号等.教师姓名__________________ 作业序号_________专业__________________学号__________________姓名________________…………………………………………………07~08学年第一学期………………………密封装订线………………………08年1月15日……………………………………安徽工业大学06级《大学物理A 2》期末考试试卷 (甲卷)一、选择题: 请将你所选的各题答案的序号填入下表(每题3分,共30分).1、若匀强电场的场强为E v,其方向平行于半径为R的半球面的轴,如图所示.则通过此半球面的电场强度通量Φe 为 (A)(B)E R 2πE R 22π (C) E R 221π (D) E R 22π2、如图所示,半径为R 的均匀带电球面,总电荷为Q ,设无穷远处的电势为零,则球内距离球心为r 的P 点处的电场强度的大小和电势为:(A) E =0,r Q U 04επ=. (B) E =0,RQ U 04επ=.(C) 204r QE επ=,r Q U 04επ=. (D) 204r Q E επ=,RQU 04επ=.3、如图所示,边长为a 的等边三角形的三个顶点上,分别放置着三个点电荷-q 、q 、2q .若将另一正点电荷Q 从无穷远处移到三角形的中心O 处,外力所作的功为: (A)a qQ 032επ. (B) aqQ03επ. (C)a qQ 0233επ. (D) aqQ023επ.4、在一不带电荷的导体球壳的球心处放一点电荷,并测量球壳内外的场强分布.如果将此点电荷从球心移到球壳内其它位置,重新测量球壳内外的场强分布,则将发现: (A) 球壳内、外场强分布均无变化. (B) 球壳外场强分布改变,球壳内不变. (C) 球壳内场强分布改变,球壳外不变. (D) 球壳内、外场强分布均改变.5、如图所示,一厚度为d 的“无限大”均匀带电导体板,电荷面密度为σ ,则板的两侧离板面距离均为h 的两点a 、b 之间的电势差为:(A) 0. (B) 02εσ. (C) 0εσh . (D) 02εσh.6、一平行板电容器充电后仍与电源连接,若用绝缘手柄将电容器两极板间距离拉大,则极板上的电荷Q 、电场强度的大小E 和电场能量W 将发生如下变化(A) Q 增大,E 增大,W 增大. (B) Q 增大,E 增大,W 减小. (C) Q 增大,E 减小,W 增大. (D) Q 减小,E 减小,W 减小.7、边长为l 的正方形线圈,分别用图示两种方式通以电流I (其中ab 、cd 与正方形共面),在这两种情况下,线圈在其中心产生的磁感强度的大小分别为 (A) 01=B ,02=B .(B) 01=B ,lI B π=0222μ. (C) lIB π=0122μ,02=B . (D) l I B π=0122μ,lI B π=0222μ.8、圆铜盘水平放置在均匀磁场中,B v的方向垂直盘面向上.当铜盘绕通过中心垂直于盘面的轴沿图示方向转动时,(A) 铜盘上有感应电流产生,沿铜盘转动的相反方向流动.(B) 铜盘上有感应电流产生,沿铜盘转动的方向流动. (C) 铜盘上产生涡流. (D) 铜盘上有感应电动势产生,铜盘边缘处电势最高.9、根据玻尔的理论,氢原子在n =7轨道上的动量矩与在第一激发态的轨道动量矩之比为(A) 7/6. (B) 7/2. (C) 7/4. (D) 7.10、若α粒子(电荷为2e )在磁感应强度为B r均匀磁场中沿半径为R 的圆形轨道运动,则α粒子的德布罗意波长是(A) . (B) . )2/(eRB h )/(eRB h (C) . (D) .)2/(1eRBh )/(1eRBh二、填空题:(共 36 分) . 1、真空中一半径为R 的均匀带电球面带有电荷Q (Q >0).今在球面上挖去非常小块的面积△S (连同电荷),如图所示,假设不影响其他处原来的电荷分布,则挖去△S 后球心处电场强度的大小E =____________,其方向为_________.2、一空气平行板电容器,两极板间距为d ,充电后板间电压为U .然后将电源断开,在两板间平行地插入一厚度为d /4的金属板,则板间电压变成 U ' =________________3、如图所示的空间区域内,分布着方向垂直于纸面的匀强磁场,在纸面内有一正方形边框abcd (磁场以边框为界).而a 、b 、c 三个角顶处开有很小的缺口.今有一束具有不同速度的电子由a 缺口沿ad 方向射入磁场区域,若b 、c 两缺口处分别有电子射出,则此两处出射电子的速率之比v b /v c =_________________.4、已知面积相等的载流圆线圈与载流正方形线圈的磁矩之比为2∶1,圆线圈在其中心处产生的磁感强度为0B v,那么正方形线圈(边长为a )在磁感强度为B v的均匀外磁场中所受最大磁力矩大小为______________________.( 反面还有试题 )题类,题号 选择题 填 空 题 计算题 1 计算题 2 计算题 3 计算题 4 计算题 5 总 分 累分人复累人得分评阅教师题号 1 2 3 4 5 678910选择 得分 q2 IvS Q得 分(1∼5)c d5、平行的无限长直载流导线A 和B ,电流均为I ,垂直纸面, 方向如图示,两根载流导线之间相距为a ,则(1) AB 中点(P 点)的磁感强度_________. =p B (2) 磁感强度B v 沿图中环路L 的线积分 =∫⋅L l B v v d __________________.6、如图所示,aOc 为一折成∠形的金属导线(aO =Oc =L ),位于xy 平面中;磁感强度为B v 的匀强磁场垂直于xy 平面.当aOc 以速度v沿x 轴正向运动时,导线上a 、c 两点间电势差U v ac =____________;当aOc 以速度v v 沿y 轴正向运动时,a 、c 两点的电势相比较, 是____________点电势高.7、两根很长的平行直导线与电源组成回路,如图.已知导线上的电流为I ,两导线单位长度的自感系数为L ,则沿导线单位长度的空间内的总磁能W m =______________.8、用某频率的单色光照射基态氢原子气体,使气体发射出三种频率的谱线,原照射单色光的频率是______________________.(普朗克常量h =6.63×10-34 J ·s ,1 eV =1.60×10-19 J)9、如图所示,一频率为ν 的入射光子与起始静止的自由电子发生碰撞和散射.如果散射光子的频率为ν′,反冲电子的动量为p ,则在与入射光子平行的方向上的动量守恒定律的分量形式为_______________.10、波长为λ0 = 0.500 Å的X 射线被静止的自由电子所散射,若散射线的波长变为λ = 0.522 Å,反冲电子的动能为______________________.(普朗克常量h =6.63×10-34 J ·s)三、计算题:要求写出解题主要步骤 (34分).(6分) 1、一半径为R 的带电球体,其电荷体密度分布为ρ =A/r (r ≤R ) ,ρ =0 (r >R )A 为一常量.试求球体内外的场强分布.(8分)2、一电容器由两个很长的同轴薄圆筒组成,内、外圆筒半径分别 为R 1 = 2 cm ,R 2 = 6 cm .电容器接在电压U = 30 V 的电源上,(如图所示),试求距离轴线R = 3 cm 处的A 点的电场强度和A 点与外筒间的电势差.(6分)3、一维运动的粒子,设其动量的不确定量等于它的动量,试求此粒子的位置不确定量与它的德布罗意波长的关系.(不确定关系式).h x p x ≥ΔΔ(6分)4、通有电流I的长直导线在一平面内被弯成如图形状,放于垂直进入纸面的均匀磁场B v中,求整个导线所受的安培力(R 为已知).(8分 )5、载有电流为I 的长直导线附近,放一导体半圆环MeN 与长直导线共面,且端点MN 的连线与长直导线垂直.半圆环的半径为b ,环心O 与导线相距a .设半圆环以速度 v v 平行导线平移,求半圆环内感应电动势的大小和方向以及MN 两端的电压U M − U N .××××× 得分(6∼10) ……………………………………………………………此线以下答题无效…………………………………………………………得分得分 得分 得分Bv得分A2甲卷 3。
大学物理期末考试题(光学)

大学物理(下)(强物理类)期末考试试卷 (B)
一、选择题(每小题4分,共5小题,20分)
1、 在单缝夫琅和费衍射实验中,波长为λ的单色光垂直入射在宽度为
3λ的单缝上,对应于衍射角为30°的方向,单缝处波阵面可分成的半
波带数目为( )
(a) 2个
大学物理(下)(强物理类)期末考试试卷 (A)参考答案及评分标 准
一、选择题(每小题4分,共5小题,20分) 1~5题答案:B E C D A 二、填空题(6~13每小题3分,14题6分,合计30分) 6题答案:100nm 7题答案: 8题答案:米 9题答案:
10题答案: 11题答案: 12题答案:10条 13题答案:9.3或9.4 14题答案:电子动能, 动量
9、在两个正交的偏振片之间再插入一个偏振片,该偏振片的透振方
向与第一个偏振片的夹角为,入射自然光的光强为,则透射光的光强为
。
10、一列固有长度长为的火车,以高速通过一个固有长度为的大
桥,火车上的观察者测得火车通过大桥所需的时间为
。
11、实验室中观察到静止质量均为的两个粒子,同时相对于实验室 以0.8C的速度相向飞行,并作完全非弹性碰撞,碰撞后结合为一个新粒 子。则该新粒子的静止质量为:
2007—2008学年第一学期
大学物理(下)(强物理类)期末考试试卷 (A)
院系
专业
姓名
学号
.
一、选择题(每小题4分,共5小题,20分)
1、右图所示是迈克耳逊干涉仪的光路图及其干涉图照片,S是单色 点光源,M1、M2是两个平面反射镜。下列说法正确的是:( )
M1 M2 S 第1题图
A、这是等倾干涉图,M1、M2不严格垂直,中间条纹的干涉级次 较高;
信息与计算科学)(大一新生必看)大学专业必修课程
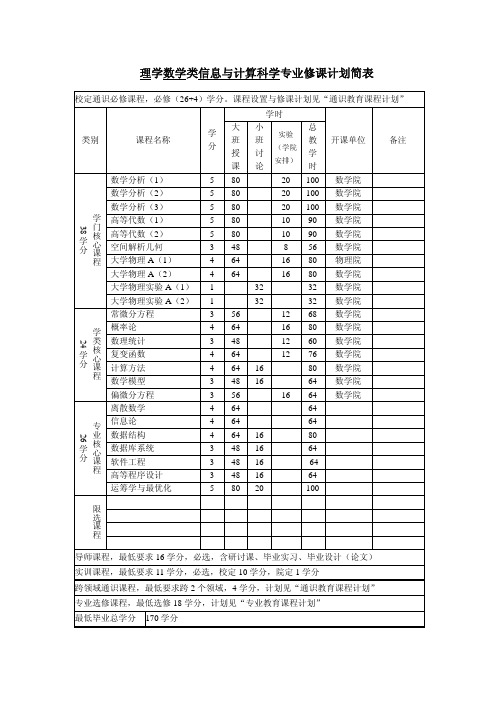
校定通识必修课程,必修(26+4)学分。课程设置与修课计划见“通识教育课程计划”
学时
类别
课程名称
学 分
大 班
小
总
实验
班
教
(学院
开课单位
授讨
学
安排)
课论
时
备注
数学分析(1)
5 80
20 100 数学院
数学分析(2)
5 80
20 100 数学院
38
学 数学分析(3)
3 56
16 64 数学院
26
离散数学
专 业
信息论
学 分
核 心 课
数据结构 数据库系统
程 软件工程
4 64
64
4 64
64
4 64 16
80
3 48 16
64
3 48 16
64
高等程序设计
3 48 16
64
运筹学与最优化
5 80 20
100
限 选 课 程
导师课程,最低要求 16 学分,必选,含研讨课、毕业实习、毕业设计(论文) 实训课程,最低要求 11 学分,必选,校定 10 学分,院定 1 学分 跨领域通识课程,最低要求跨 2 个领域,4 学分,计划见“通识教育课程计划” 专业选修课程,最低选修 18 学分,计划见“专业教育课程计划” 最低毕业总学分 170 学分
门 高等代数(1)
学 分
核 心 课
高等代数(2) 空间解析几何
程 大学物理 A(1)
5 80 5 80 5 80 3 48 4 64
20 100 数学院 10 90 数学院 10 90 数学院 8 56 数学院 16 80 物理院
本科物理“双语教学”的范本——《费曼物理学讲义》

第28卷第5期 唐山师范学院学报 2006年9月 Vol. 28 No.5 Journal of Tangshan Teachers College Sep. 2006 ────────── 收稿日期:2005-02-29作者简介:王贺清(1965-),女,河北唐山人,唐山师范学院物理系讲师。
- 137 -本科物理“双语教学”的范本——《费曼物理学讲义》王贺清(唐山师范学院 物理系,河北 唐山 063000)摘 要:费曼独特的教学理念对物理教学的启迪是非同寻常的,其《费曼物理学讲义》中深刻的物理学思想和精练的阐述,是物理系本科学生双语学习难得的经典教材,而且还能为学生毕业后从事物理教学和科研打下良好的基础。
关键词:费曼物理学讲义;双语教学;启迪中图分类号:G658.3 文献标识码:B 文章编号:1009-9115(2006)05-0137-021 《费曼物理学讲义》为创设“双语教学”环境提供了良好的开端物理系“双语教学”课程是为大三学生开设的。
经过大一和大二两年的大学英语学习,学生已经具有了基本的听、说、读、写的语言能力,有些学生已经具备了较高的英语水平;同时学生也具备了物理专业背景知识。
《费曼物理学讲义》能把学生从“做题,过级”的怪圈中解放出来,以一种既符合学生学习心理特点,也符合英语教学规律的方式来创设“双语教学”环境。
《费曼物理学讲义》的前言用了很长篇幅介绍了费曼的生平、费曼对科学和教育的贡献及《费曼物理学讲义》产生的背景,使学生在“双语”环境中不仅能轻松地感受到费曼多方面的个性,而且更能体会到费曼简单而巧妙的教学技巧。
例如,在一次讲座中,他试图解释为什么千万不能用第一次提出的观点所用的数据来证明这个观点。
费曼开始谈论汽车车牌,看上去像是漫不经心偏离了主题,实际上已经严密地论证了他所论述的物理内容:Once, during a public lecture, he was trying to explain why one must not verify an idea using the same data that suggested the idea in the first place. Seeming to wander off the subject. Feynman began talking about the license plates. “You know, the most amazing thing happened to me tonight. I was coming here, on the way to the lecture, and I came in through the parking lot. And you won’t believe what happened. I saw a car with the license plate ARW 357. Can you imagine? Of all the millions of license plates in the state, what was the chance that I would see that particular one tonight? Amazing!”(“你知道吗!今天晚上发生了最令我惊奇的事情。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
2013 - 2014 学年第 二 学期期末考试卷(A 卷)课程名称: College Physics 课程类别: 必修 考试方式: 闭卷注意: 1、本试卷满分100分。
2、考试时间 120分钟。
I. There are 6 statements in this part. Please mark a “√” in the bracket if it is right, or a “×” if it is wrong.(2×6=12 points )1 If an object is moving without any kind of force, it will continue to move along in a perfectly straight line at a constant speed. ( )2. The work of the gravity is not related with the motion path of the particle, and only depends on the initial and final positions of the particle. ( )3 Electric flux is a vector.( )4 The line of magnetic field is closed. ( )5 The optical wave is a longitudinal wave (纵波). ( )6 At a given temperature, lighter molecules move faster, on average, than heavier ones ( ) II. Choose one correct answer among following choices (4×6=24 points )1 When a mass point is in a circular motion, then ()学院: 专业班级: 姓名: 学号:装 订 线 内 不 要 答 题(A) The tangential acceleration definitely change, the normal acceleration also change. (B) The tangential acceleration may not change, the normal acceleration definitely change. (C) The tangential acceleration may not change, the normal acceleration does not change. (D) The tangential acceleration definitely change, the normal acceleration does not change.2 An object move along a smooth inclined plane from rest, as shown in figure, which statement is correct ( )(A) Both of the time and the speed of the object at the end is independent on the inclined angular.(B) Only the speed of the object at the end is independent on the inclined angular.(C) Only the time of the object passing through the inclined plane is independent on the inclined angular.(D) Both of the time and the speed of the object at the end is depended on the inclined angular.3 In following statements which one is the correct ( )(A) The electric potential is definitely zero at the point where the electric field is zero. (B) The electric potential is non-zero at the point where the electric field is not zero. (C) The electric field intensity is definitely zero at the point where the electric potential is zero.(D) The electric field intensity is definitely zero in the space where the electric potential is constant.4 The segment of wire in figure carries a current of I, where the radius of the circular arc is R, the magnitude of the magnetic field at O is ( )(A) R I80μ (B) R I 40μ(C) RI πμ80 (D) R I πμ205 The light with the wavelength 550nm irradiates on the single slit with the width 1800nm, the highest order spectrum that can be observed is ( ) (A) 1 (B) 2 (C) 3 (D) 46 The same kind of ideal gas are in three containers A, B and C, their molecular numberdensity are also the same whereas the ratio of the mean square speed is 1:2:4,what is the ratio of the pressure ( )(A) 1:2:4 (B) 1:4:8 (C) 1:4:16 (D) 4:2:1III. Filling the following blanks (4×6=24 points )1. The motion equations of a particle is 23010t t x +-= and 22015t t y -= in SI, then the magnitude of the initial velocity is 。
2. A semi-sphere surface of radius R is charged uniformly, the surface density of electric charge is 0σ, so the electric potential at the sphere center is 。
3. If 500 J of heat added to ideal gas that is expanding from 0.2 m 3to 0.3 m 3 at a constant pressure of 4000 Pa, the change in its internal energy is 。
4. Two objects m 1=4.00 kg and m 2=7.00 kg are connected by a light string that passes over a frictionless pulley. The sliding friction between m 1 and the surface is neglected. The acceleration of the two objects is .5. When applying the wedge interference to examine theroughness of the optical device, we find that the interference fringes are shown as the right figure, then the upper surface of the device is (concave 凹的,convex ,凸的)。
6 In general, point of zero potential is taken at an infinite distance from the charge. Withm1m2this choice, a potential due to point changes Q can be expressed as 。
IV. Calculation(4×10=40 points)1. There is a small boat on the lake. Some people on thebank draw the boat to the bank with the string carryingthe fixed pulley. Suppose people draw the string withinvariable speed v0, the height of the pulley from thewater surface is h, and the length of the string from theboat to the pulley is L, determine the speed of the boat.2.An insulating sphere of radius R has a uniform charge distribution and a total positive charge Q. Calculate the electric field intensity at a point outside or inside the sphere.3. In the experiment of double-slit interference, the distance between two slits is 0.30mm, and the monochromatic light (单色光) irradiates two slits vertically. On the screen that is 1.20m away from slits, the distance between two fifth dark fringes locating by opposite sides of the central bright fringe is 22.78mm. How much is the wavelength of the light?4. Finish the table of the each process of thermodynamics where Q is heat, E is the internal energy and W is work.。
