高分子材料加工计算机模拟第四章_有限元软件介绍
计算机在高分子材料中的应用上机

计算机在高分子材料中的应用上机引言随着计算机科学的发展和计算能力的提高,计算机在各个领域的应用也越来越广泛。
高分子材料作为一类重要的材料,在材料科学领域中起着举足轻重的作用。
本文将介绍计算机在高分子材料研究中的应用,着重阐述计算机在高分子材料的模拟与设计、性能预测以及高分子材料在生物医学中的应用等方面的上机实验。
计算机模拟与设计计算机模拟与设计在高分子材料研究中扮演着重要的角色。
通过计算机模拟,可以对高分子材料的结构进行建模和优化设计,从而提高材料的性能。
在计算机模拟过程中,分子动力学模拟和量子化学计算是两个常用的方法。
分子动力学模拟分子动力学模拟可以通过模拟高分子材料的原子和分子之间的相互作用,来研究材料的力学性质和热力学行为。
通过分子动力学模拟,可以预测材料的力学性能、热膨胀性质和热导率等物理特性。
计算机可以通过数值计算求解分子动力学方程,来模拟高分子材料的行为。
量子化学计算量子化学计算是基于量子力学的方法,用于计算分子和原子的结构、能量和其他性质。
在高分子材料研究中,量子化学计算可以用于模拟高分子材料的电子结构和光谱特性。
通过计算机进行量子化学计算,可以预测高分子材料的光学性质、电导率以及催化反应等特性。
性能预测计算机在高分子材料中的应用还包括性能预测。
通过计算机模拟和数值计算,可以预测高分子材料在不同条件下的性能表现,从而提供重要的参考和指导。
力学性能预测通过计算机模拟和有限元分析等方法,可以预测高分子材料在受力情况下的力学性能,如强度、刚度、韧性等。
这对于材料的设计和应用具有重要意义,可以帮助研究人员更好地选择和设计高分子材料。
热学性能预测计算机模拟和数值计算还可以用于预测高分子材料的热学性能,包括热膨胀性、热传导性以及热稳定性等。
这些性能的预测对于高分子材料在高温、低温等极端条件下的应用具有重要意义。
高分子材料在生物医学中的应用高分子材料在生物医学领域中的应用也得到了广泛关注。
计算机在高分子材料在生物医学中的应用上机实验中发挥着重要作用。
Deform有限元模拟技术介绍及应用
。把整个系统分解成性态容易了解的单个的元件 或“ 单元”,然后由这些元件重建原来的系统, 以研究其性态变化等。
静态 隐式
有限元模拟算法
动态 显式
数值模拟:求偏微分方程的数值解
数值模 拟方法
•1.有限元法、 • 2.边界元法、 • 3.离散单元法 •4.有限差分法
综上本模拟试验中所选取的基本参数:
• TiAl合金坯料的直径为14mm • TiAl合金坯料髙径比为2,2.5,3 • 包套材料为321不锈钢,厚度为3,5,8mm • 上下模尺寸为50×50×15mm,温度为20 ℃ • TiAl合金的温度为1150℃ • 上模向下运动的速度为1mm/sec • 各物体之间的接触摩擦系数为0.3 • 公差设为最佳值 • 模拟控制中步长设为0.7mm
5 DEFORM模拟软件在材料加工中的应用
以DEFORM软件在塑性成形中的应用为主,以 TiAl合金的包套镦粗以及方形件的锻压为例,详 细介绍deform的功能,应用的步骤,以及可以 解决的一些主要问题
A
前期造型
B
模拟的前处理
C
启动模拟计算器
D
后处理操作
前期造型
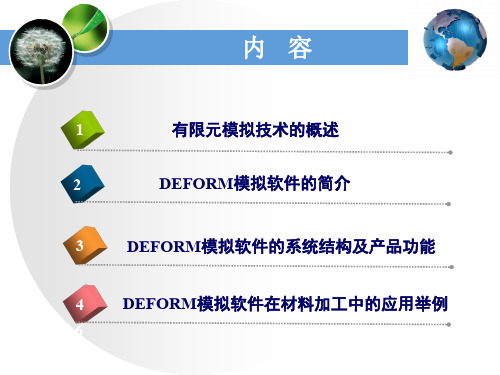
内容
1
Hale Waihona Puke 有限元模拟技术的概述2DEFORM模拟软件的简介
3
DEFORM模拟软件的系统结构及产品功能
4 DEFORM模拟软件在材料加工中的应用举例 6
有限元模拟软件的概述
有限元的基本思想在20世纪40年代初就已经提出, 到70年代才开始逐渐被应用,应用的范围,分析 的对象也越来越广,分析的作用也更加强大
• 专门适用于塑性加工领域的CAE分析软件
有限元模拟软件使用说明

Magnetic Prospecting of Iron Ore DepositsIntroductionMagnetic prospecting is one of the methods of geological exploration applicable to certain types of iron ore deposits, in particular those made up of magnetite andhematite. Estimates of center-of-mass coordinates and the spatial extent of iron-rich layers help decrease the cost of exploration. Passive magnetic prospecting relies on accurate mapping of local geomagnetic anomalies—deviations of the naturalmagnetostatic fields from their predicted values based on the magnetic dipole model of the Earth’s field. This model investigates magnetic anomaly estimation for both surface and aerial prospecting.Crustal magnetic anomalies may result from either induced or remnant magnetization of iron-rich rocks. Amongst all terrestrial iron minerals, magnetite has the largest magnetic permeability (up to μr = 650 in small-grain magnetite) and the strongest naturally occurring thermo-remnant magnetization (up to M r = 5000 A/m) (Ref. 1).Another mineral with relatively strong magnetization is hematite (Ref. 1). Magnetite and hematite are the major minerals of high-grade iron ore. This model uses modest values of magnetic permeability (μr = 3.5) and remnant magnetization (M r = 60 A/m) that are typical for terrestrial magnetite with a grain size distribution of 20–200μm (Ref. 1). The concentration of magnetite in the iron-rich ore is assumed to be 25%.Model DefinitionMagnetostatic problems without significant electrical currents can be solved in terms of a scalar magnetic potential. Variations of the magnetic permeability or remnant magnetization cause small deviations of magnetic fields from the norm (localgeomagnetic anomalies), which you can model accurately using the Reduced Potential formulation of the Magnetic Fields, No Currents interface. The background magnetic field is assumed to be uniform within the simulation domain. Intensity and orientation of the natural magnetic field is estimated using the data from National Geophysical Data Center of the U.S. Government (Ref. 2).This model neglects the induced and remnant magnetization of the rocks outside the iron ore body. The iron ore bed is approximated by a uniform flat ellipsoid with amaximum vertical thickness of 100 m, a maximum North-South width of 400 m, and an East-West extent of 2000 m. Center-of-mass coordinates of the ore bed (2500, 1500) meters from the lower-left corner (200 meters above sea level) are positioned underneath the Eastern Pit of the Eagle Mountain Mine, a former Kaiser Steel Co. mining operation in Riverside County, California (Ref. 3). The specific location and shape of magnetic ore in this model are not based on any actual geological prospecting but are chosen to simulate a basic size and shape of such an ore. Figure 1 shows the model geometry.Figure 1: The model geometry.T O P O G R A P H YThe topographical map used for this model consists of a rectangular grid containing 157-by-111 points spaced 1 arc second (1/3600°) apart in both horizontal directions. The curvature of geoid is neglected in drawing this geometry from elevation data. The lower-left (south-west) corner of the map is located at a latitude 33.85° (North) and longitude 115.5° (West). The size of the simulation domain is 4836 m in an East-West (x-axis), 3410 m in a North-South direction (y-axis), and 1934.4 m in the up-down direction (z-axis). A satellite image of this area can be seen in (Ref. 4).A digital elevation model (DEM) for this example can be derived from the National Elevation Dataset (NED) of the U.S. Geological Survey data center (Ref. 5). The free program MicroDEM (Ref. 6) converts the USGS elevation data into an ASCII filecontaining the elevation matrix, which you can import into COMSOL Multiphysics as an Interpolation function feature. From the interpolation function you can then create a geometry by using a Parametric Surface feature. Optionally, the resulting geometry can be saved in COMSOL’s internal binary CAD file format (.mphbin). Importing the saved CAD file into the COMSOL Desktop is the starting point for the step-by-step instructions for this model. Figure 2 contains a plot of the resulting elevation map as it appears in COMSOL Multiphysics.Figure 2: Elevation map of the simulation domain (boundary plot of z-coordinate on Boundary 6).D O M A I NE Q U A T I O N SIn a current-free region, whereit is possible to define a scalar magnetic potential, V m , such thatThis is analogous to the definition of the electric potential for static electric fields. Using the constitutive relation between the magnetic flux density and magnetic field,together with the equation∇H ×0=H V m∇–=B μH B r+=one can derive an equation for V m :The Reduced potential formulation used in this model splits the total magnetic potential into external and reduced parts, V tot = V ext + V red , where the reduced potential V red is the dependent variable:The external magnetic potential is more easily defined as an external magnetic field, so the equation that is used in practice is ratherTo simulate the background geomagnetic field, components of the external magnetic field are expressed through total intensity, magnetic declination, and inclination angles as follows:Based on the data provided by NOAA’s data center (Ref. 3), inclination and declination angles for this location (N 33.85°, W 115.5°) are approximatelyIncl = 59.357° and Decl = 12.275°, respectively. The magnitude of natural magnetic flux density (B 0 = μ0 H 0) is estimated as 48.163 μT.Remnant magnetic flux density is assumed to be aligned with the local contemporary direction of geomagnetic field:In general, thermo-remnant magnetization does not have to be aligned with the Earth’s magnetic field.The values of magnetic permeability and remnant flux used for the iron ore are based on the typical parameters of magnetite ore and a simple homogenization model taking into account dilution of magnetization in the ore bed:∇B ⋅0=∇μ–∇V m B r +()⋅0=∇μ–∇V ext V red +()B r +()⋅0=∇μ–∇V red ()B r μH ext ++()⋅0=extHOx HO Incl ()cos Decl ()sin ⋅⋅=extHOy HO Incl ()cos Decl ()cos ⋅⋅=extHOz HO –Incl ()sin ⋅=B r x ,B r ore ,Incl ()cos Decl ()sin ⋅⋅=B r y ,B r ore ,Incl ()cos Decl ()cos ⋅⋅=B r z ,B –r ore ,Incl ()sin ⋅=Concentration of magnetite c magn in the ore is assumed to be 25%.B O U N D A R YC O ND I T I O N SAlong the exterior boundaries of the surrounding box, the perturbation of the magnetic field (H red ) is assumed to be tangential to the boundaries. The natural boundary condition from the equation isThus, the reduced magnetic field is made tangential to the boundary by a Neumann condition on the reduced potential. Interior boundaries are modeled as continuity boundary conditions and require no user input.The Magnetic Fields, No Currents interface automatically adds a point constraint, V m = 0, using a weak term on the equation-system level if there are no boundary conditions that constrain the value of V m (such as Magnetic potential or Zero potential). This ensures that the scalar potential V m is uniquely defined in problems with pure Neumann boundary conditions.Results and DiscussionFigure 3 shows deviations of the magnetic field intensity on the ground (boundary plot), and at an altitude of 1300 meters. The maximum magnetic field perturbation on the ground is 2 A/m and at 1300 meters altitude it has fallen to 0.1 A/m. In both locations the maximum is located approximately above the center of mass of themagnetite ore body. These results show that aerial magnetic prospecting may reveal the location and provide estimates of the horizontal extent of magnetite-rich deposits but also that ground-based magnetic prospecting apparently is a much more sensitive exploration tool than aerial prospecting.μr ore ,1–μr magn ,1–()c magn⋅=B r ore ,B r magn ,c magn⋅=n B red ⋅n μV m ()∇⋅⋅0==Figure 3: The slice color plot shows perturbations of magnetic intensity (norm of reduced magnetic field) at an altitude 1300 m. The boundary plot shows the same quantity on theground.Figure 4: Arrows show the direction and relative magnitude of the reduced magnetic field on the Earth’s surface.Figure 5 shows a slice plot of the dimensionless ratio of remanent and induced magnetizations, B r/(μ0M), inside the iron ore body, at an elevation of 200 m above sea level. This plot indicates that contributions of remanent and induced magnetizations to the magnetic anomaly can be comparable for realistic magnetic parameters ofmagnetite (Ref. 1).Figure 5: Slice plot of the dimensionless ratio of remanent to induced magnetizations inside the iron ore body at an elevation of 200 m above sea level. The two contributions to the magnetic anomaly are of comparable magnitude.References1. G. Kletetschka, P.J. Wasilewski, and P.T. Taylor, “Hematite vs. magnetite as the signature for planetary magnetic anomalies,” Physics of the Earth and Planetary Interiors, vol. 119, pp. 259–267, 2000.2. NOAA’s Geophysical Data Center, /geomagmodels/ IGRFGrid.jsp3. E.R. Force, “Eagle Mountain Mine – Geology of the former Kaiser Steel operation in Riverside county, California,” U.S. Geological Survey Open-File Report 01-237.4. Google Maps, /maps?f=q&hl=en&geocode=&q=33.865,-115.475&ie=UTF8&t=h&z=16&iwloc=addr5. Data available from U.S. Geological Survey, EROS Data Center, Sioux Falls, SD. The National Map Seamless Server of U.S. Geological Survey, http:// /6. MICRODEM Home Page, /Users/oceano/pguth/ website/microdem/microdem.htmModel Library path: ACDC_Module/Magnetostatics/magnetic_prospecting Modeling InstructionsM O D E L W I Z A R D1Go to the Model Wizard window.2Click Next.3In the Add physics tree, select AC/DC>Magnetic Fields, No Currents (mfnc).4Click Next.5Find the Studies subsection. In the tree, select Preset Studies>Stationary.6Click Finish.G L O B A L D E F I N I T I O N SParameters1In the Model Builder window, right-click Global Definitions and choose Parameters. 2In the Parameters settings window, locate the Parameters section.3In the table, enter the following settings:Name Expression Descriptionmur_magn 3.5Relative permittivity ofmagnetitec_magn0.25Magnetite concentration in ore Mr_magn60[A/m]Remanent magnetization ofmagnetiteH048163[nT]/mu0_const Geoelectric fieldIncl59.357[deg]Local inclinationDecl12.275[deg]Local declinationVariables 11Right-click Global Definitions and choose Variables.2In the Variables settings window, locate the Variables section.3In the table, enter the following settings:Name Expression DescriptionBr_magn Mr_magn*mu0_const Remanent flux density ofmagnetiteBr Br_magn*c_magn Remanent flux density of ore Gx cos(Incl)*sin(Decl)Geomagnetic field direction,x-componentGy cos(Incl)*cos(Decl)Geomagnetic field direction,y-componentGz-sin(Incl)Geomagnetic field direction,z-componentmur_ore1+(mur_magn-1)*c_magn Model for the relativepermittivity of oreG E O M E T R Y1The geometry obtained from the height map is available in an MPHBIN-file (COMSOL Multiphysics' native CAD file format) that can be imported in the geometry node.Import 11In the Model Builder window, under Model 1 right-click Geometry 1 and choose Import.2In the Import settings window, locate the Import section.3Click the Browse button.4Browse to the model’s Model Library folder and double-click the file magnetic_prospecting.mphbin.5Click the Import button.Ellipsoid 1Create an ellipsoid that models the iron ore deposit.1In the Model Builder window, right-click Geometry 1 and choose More Primitives>Ellipsoid.2In the Ellipsoid settings window, locate the Size and Shape section.3In the a-semiaxis edit field, type 1000.4In the b-semiaxis edit field, type 200.5In the c-semiaxis edit field, type 50.6Locate the Position section. In the x edit field, type 2500.7In the y edit field, type 1500.8In the z edit field, type 200.9Click the Build All button.Activate the transparency to verify that the deposit is in the correct position.10Click the Transparency button on the Graphics toolbar.M A T E R I A L SUse one material for the background (air and nonmagnetic rocks) and another for the iron ore. For the ore, you specify the relative permeability as a material property but add the remanent magnetization in the physics settings.Material 11In the Model Builder window, under Model 1 right-click Materials and choose Material. 2In the Material settings window, locate the Material Contents section.3In the table, enter the following settings:Property Name ValueRelative permeability mur14Right-click Material 1 and choose Rename.5Go to the Rename Material dialog box and type Background Material in the New name edit field.6Click OK.Material 21Right-click Materials and choose Material.2Select Domain 3 only.3In the Material settings window, locate the Material Contents section.4In the table, enter the following settings:Property Name ValueRelative permeability mur mur_ore5Right-click Material 2 and choose Rename.6Go to the Rename Material dialog box and type Ore in the New name edit field.7Click OK.M A G N E T I C F I E L D S,N O C U R R E N T SIn the Model Builder window, expand the Magnetic Fields, No Currents node.Magnetic Flux Conservation 21Right-click Model 1>Magnetic Fields, No Currents and choose Magnetic Flux Conservation.2Select Domain 3 only.3In the Magnetic Flux Conservation settings window, locate the Magnetic Field section. 4From the Constitutive relation list, choose Remanent flux density.5In the B r table, enter the following settings:Br*Gx xBr*Gy yBr*Gz zSpecify the local geomagnetic field as the background field for the problem.6In the Model Builder window, click Magnetic Fields, No Currents.7In the Magnetic Fields, No Currents settings window, locate the Background Magnetic Field section.8From the Solve for list, choose Reduced field.9In the H b table, enter the following settings:H0*Gx xH0*Gy yH0*Gz z10Right-click Magnetic Fields, No Currents and choose External Magnetic Flux Density.External Magnetic Flux Density 1Apply External Magnetic Flux Density as the boundary condition instead of Magnetic Insulation. The former operates only on the relative field, while the latter sets a condition for the total field.1In the External Magnetic Flux Density settings window, locate the Boundary Selection section.2From the Selection list, choose Manual.3Click Clear Selection.4Select Boundaries 1–5, 7–9, 18, and 19 only.S T U D Y1Disable the automatic generation of the default plots. You will manually create the plots after the solution.1In the Model Builder window, click Study 1.2In the Study settings window, locate the Study Settings section.3Clear the Generate default plots check box.4Click the Compute button.R E S U L T SIn the Model Builder window, expand the Results node.Data SetsCreate a second solution with a selection active only on the boundary corresponding to the Earth's surface. You will use this solution when plotting surface data.1In the Model Builder window, expand the Results>Data Sets node.2Right-click Data Sets and choose Solution.3Right-click Results>Data Sets>Solution 2 and choose Add Selection.4In the Selection settings window, locate the Geometric Entity Selection section.5From the Geometric entity level list, choose Boundary.6Select Boundary 6 only.3D Plot Group 11In the Model Builder window, right-click Results and choose 3D Plot Group.2Right-click 3D Plot Group 1 and choose Surface.3In the Surface settings window, locate the Data section.4From the Data set list, choose Solution 2.5Click Replace Expression in the upper-right corner of the Expression section. From the menu, choose Magnetic Fields, No Currents>Magnetic>Reduced magnetic field norm (mfnc.normredH).The plot of the relative magnetic field at the altitude of 1300 m can be obtained with a simple slice plot.6In the Model Builder window, right-click 3D Plot Group 1 and choose Slice.7In the Slice settings window, locate the Plane Data section.8From the Plane list, choose xy-planes.9From the Entry method list, choose Coordinates.10In the z-coordinates edit field, type 1300.11Click Replace Expression in the upper-right corner of the Expression section. From the menu, choose Magnetic Fields, No Currents>Magnetic>Reduced magnetic field norm (mfnc.normredH).12Locate the Coloring and Style section. From the Color table list, choose Thermal.13Click the Plot button.The plot shows the alteration of the geomagnetic field due to the presence of a magnetized material. Note how the relative magnetic field intensity at ground level is about 20 times larger than at 1300 m.14Click the Transparency button on the Graphics toolbar.Next, add another plot group to visualize the vector field.3D Plot Group 21In the Model Builder window, right-click Results and choose 3D Plot Group.2In the 3D Plot Group settings window, click to expand the Window Settings section. Add a uniform gray surface representing the ground.3Right-click Results>3D Plot Group 2 and choose Surface.4In the 3D Plot Group settings window, locate the Data section.5From the Data set list, choose Solution 2.6In the Model Builder window, under Results>3D Plot Group 2 click Surface 1.7In the Surface settings window, locate the Expression section.8In the Expression edit field, type 0.9Locate the Coloring and Style section. From the Coloring list, choose Uniform.10From the Color list, choose Gray.Now plot the relative magnetic field at ground level.11In the Model Builder window, right-click 3D Plot Group 2 and choose Arrow Surface.12In the Arrow Surface settings window, click Replace Expression in the upper-right corner of the Expression section. From the menu, choose Magnetic Fields, NoCurrents>Magnetic>Reduced magnetic field (mfnc.redHx,...,mfnc.redHz).13Locate the Coloring and Style section. In the Number of arrows edit field, type 2000. 14Click the Plot button.15Click the Zoom In button on the Graphics toolbar.The plot shows the vector field for the magnetic field perturbation caused by the iron ore deposit.Finally, plot the ratio between the remanent flux density and the induced magnetization.3D Plot Group 31In the Model Builder window, right-click Results and choose 3D Plot Group.2Right-click 3D Plot Group 3 and choose Slice.3In the Slice settings window, locate the Plane Data section.4From the Plane list, choose xy-planes.5From the Entry method list, choose Coordinates.6In the z-coordinates edit field, type 200.7Locate the Expression section. In the Expression edit field, type mfnc.normBr/ (mu0_const*mfnc.normM).8Click the Plot button.The plot shows that the two contributions are comparable in magnitude.。
分子模拟方法及模拟软件MaterialsStudio在高分子材料中的应用

分子模拟方法及模拟软件MaterialsStudio在高分子材料中的应用高分子材料是当今工业界和科学界中的一种重要材料,广泛应用于各个领域。
为了进一步了解高分子材料的性质和行为,探究人员接受了许多不同的方法进行探究。
其中,分子模拟方法是一种有效的工具,可用于猜测高分子材料的结构、动力学和性质。
二、分子模拟方法1. 分子动力学模拟分子动力学模拟是分子模拟方法中最常用的方法之一。
它通过模拟分子系统中原子之间的互相作用,通过求解牛顿方程来探究粒子在给定势场中的运动行为。
这种方法可以模拟高分子材料的力学性质、热力学性质和动态行为。
2. 蒙特卡洛模拟蒙特卡洛模拟是一种基于概率统计方法的模拟方法。
它通过随机生成分子的构象,计算系统的能量,然后依据一定的概率准则来决定是否接受这个构象。
通过大量的随机试验,蒙特卡洛模拟可以得到高分子材料的平衡态性质和相变行为。
三、MaterialsStudio软件介绍MaterialsStudio是由Accelrys公司(此刻是Biovia公司的一部分)开发的一款功能强大的分子模拟软件。
它提供了许多用于高分子材料模拟的工具和模块,包括分子动力学模拟、蒙特卡洛模拟、量子力学计算等。
通过MaterialsStudio软件,探究人员可以模拟高分子材料的结构、性质和行为。
四、MaterialsStudio在高分子材料中的应用1. 高分子材料的结构模拟MaterialsStudio软件可以进行高分子材料的结构模拟。
通过分子动力学模拟,探究人员可以了解高分子材料的构象分布、空间排布和互相作用。
通过蒙特卡洛模拟,探究人员可以得到高分子材料的稳定结构和相变行为。
这些模拟结果可以援助探究人员理解高分子材料的结构特征,指导高分子材料的设计和合成。
2. 高分子材料的热力学性质模拟MaterialsStudio软件可以进行高分子材料的热力学性质模拟。
通过分子动力学模拟,探究人员可以计算高分子材料的力学性质、热胀缩性和热导率等热力学性质。
有限元分析软件

有限元分析软件简介有限元分析软件(Finite Element Analysis Software)是一种通过有限元方法(Finite Element Method,简称FEM)对工程结构进行数值计算和模拟的工具。
有限元分析软件可以用于分析和解决各种工程问题,包括结构强度、热传导、流体流动、电磁场等。
本文将介绍有限元分析软件的基本原理、主要功能和一些常见的应用领域。
原理有限元分析软件的基本原理是将连续体划分为有限数量的小元素,每个小元素在一个局部坐标系内进行计算。
通过将整个结构划分为多个小元素,并在每个小元素内进行计算,最终可以得到整个结构的物理行为。
有限元软件使用数学模型描述物体的力学特性和材料特性,例如弹性模量、材料的热传导系数等。
在分析过程中,可以通过施加不同的边界条件和加载情况来模拟结构的行为。
主要功能有限元分析软件通常具有以下主要功能:1.几何建模:可以通过创建、编辑和修改几何模型来描述结构的形状和尺寸。
2.网格生成:将几何模型划分为有限数量的小元素,生成网格以进行计算。
3.边界条件和加载设置:可以设置结构的边界条件,如固定支撑、力和热流等加载条件。
4.材料属性设置:可以定义结构的材料属性,如弹性模量、热传导系数等。
5.计算求解:通过求解数学模型的方程组,得到结构的应力、位移、温度等结果。
6.结果后处理:对计算结果进行可视化和分析,如绘制应力云图、位移矢量图等。
应用领域有限元分析软件在各个工程领域都有广泛的应用,包括但不限于以下几个方面:结构分析有限元分析软件可以对各种结构进行静力学和动力学分析。
例如,在建筑工程中可以分析房屋的承重能力、抗风性能等;在机械工程中可以分析零件的强度、刚度等。
热传导分析有限元分析软件可以模拟材料或结构中的热传导过程。
例如,在电子设备中可以分析散热器的性能,以确保设备的正常运行;在汽车行业中可以优化发动机的冷却系统。
流体力学分析有限元分析软件可以模拟流体在管道、泵和阀门等设备中的流动。
《有限元软件应用》课件

化学反应器
燃料电池
水处理厂
通过有限元分析,确定反应器的 结构和尺寸,以及承受高温和高 压的能力。
通过有限元分析,优化燃料电池 的设计,提高其输出能力和稳定 性。
通过有限元分析,考虑反应槽的 加热和冷却、搅拌和流动等因素, 优化水处理设施的设计和运行。
有限元分析在化学工程领域,有助于提高化学反应器、燃料电池和水处理设施等设施的安全性和效率性。
Abaqus
用于研究结构和复杂系统的完整响应。
LS-Dyna
用于分析动态和非线性问题。
有限元软件的选择要基于具体问题的需求和程序特点进行综合考虑。
有限元模型的建立方法
绘图模型
三维建模
三维扫描
以手绘图或CAD软件绘制模型, 再转为有限元模型。
直接利用三维建模软件建立模型, 再导入有限元软件进行分析。
模拟火箭的发射过程,评估架子 的承载能力和稳定性。
模拟卫星在发射、运输、维护过 程中的各种受力情况,评估其结 构的安全性和可靠性。
有限元分析在航空航天领域的结构设计、可靠性评估等方面有着重要应用。
有限元分析在电子电气中的应用举例
手机
变电器
电路板
模拟手机的载荷、震动和温度等 情况,评估手机的结构和性能。
后处理结果的分析方法
位移和应力云图
通过生成图示来显示结构的位移和应力分布。
变形和应力云图
通过对比不同加载情况下的结构变形和应力输出云图。
位移、应变和应力的量化结果
包括最大值、最小值、平均值和范围等。 有限元分析结果的后处理需要根据具体需要进行分析和展示,包括图表输出等多种形式。
有限元分析在机械制造中的应用举例
通过有限元分析,评估管道在不
第2讲 有限元软件功能概览

11
加载
可在实体模型或 FEA 模型 (节点和单元) 上加载.
沿线均布的压力
沿单元边界均布的压力
在关键点 处约束
实体模型 FEA 模型
在节点处 约束
在关键点加集中力
在节点加集中力
12
求解器——有限元的核心
求解结果保存在数据库中并输出到结果文件
输入数据
数据库
求解器
结果
结果数据
结果文件
13
后处理——结果的绘图和列表
2012-8-2
9
2、前处理、求解器和后处理
前处理——有限元的建模工具
• • • • • • • 功能强大的有限元网格生成功能 自动与半自动网格剖分 网格优化功能 网格加密功能 属性定义 加载 约束处理
载荷分类
• 自由度DOF - 定义节点的自由度( DOF ) 值 (结构分析_位移 、热分析_ 温度、电磁分析_磁势等) • 集中载荷 - 点载荷 (结构分析_力、热分析_ 热导率、电磁分析_ magnetic current segments) • 面载荷 - 作用在表面的分布载荷 (结构分析_压力、热分析_热 对流、电磁分析_magnetic Maxwell surfaces等) • 体积载荷 - 作用在体积或场域内 (热分析_ 体积膨胀、内生成热 、电磁分析_ magnetic current density等) • 惯性载荷 - 结构质量或惯性引起的载荷 (重力、角速度等)
.
. .
June 3, 1996
25
有限元建模 -分析目的(续)
模态分析: 简单模态振型和频率可以忽略细节因素而使用相对较粗糙的单元 网格进行分析计算。
.
热分析:
.温度分布梯度变化不大时可以忽略细节,划分均匀且相对稀疏的
材料成型计算机模拟分析(各种仿真软件介绍)课件

PPT学习交流
8
• 现代成形加工与模具正朝着高效率、高速度、 高精度、高性能、低成本、节省资源等方向发 展,因此传统的设计方式已远远无法满足要求。 20 多年来,随着计算机技术和数值仿真技
• 术的发展,出现了计算机辅助工程分析 (Computer Aided Engineering)这一新兴的技术, 该技术在成形加工和模具行业中的应用,即模 具CAE。模具CAE 是广义模具CAD/CAM 中的一 个主要内容,现已在实际中体现出了越来越重 要的作用,也得到越来越广泛的应用。
PPT学习交流
19
有限元法分析的基本
过程
• 根据有限元法的基本概念,其分析过程概括起来有如下 内容,现以连续结构的应力应变分析为例,逐
• 步加以说明。
•
有限元分析的第一步是结构的离散化,这也是有
限元法的基础。简单来说,离散化就是将结构划分成
• 为有限个单元体,并在单元体的指定点设置节点,将相 邻单元体通过节点连接起来组成单元的集合体,并
优化模块、超单元分析模块、气动弹性分析模 块、DMAP 用户开发工具模块及高级对称分析 模块。
PPT学习交流
23
• 结构动力学分析是MSC.NASTRAN 的主要强项 之一,其主要功能包括:正则模态及复特征值 分析、频率及瞬态响应分析、(噪)声学分析、随 机响应分析、响应及冲击谱分析、动力灵敏度 分析等。
PPT学习交流
15
PPT学习交流
16
• 从数学角度理解,是将图7-1 所示的求解区域 剖分成许多三角形子区域,子域内的位移可以 由相应各节点的待定位移合理插值来表示。根 据原问题的控制方程(如最小势能原理)和约 束条件,可以求解出各节点的待定位移,进而 求得其它场量。推广到其它连续域问题,节点 未知量也可以是压力、温度、速度等
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
ANSYS 入门
…启动 ANSYS
启动 (续) 选择您喜欢的启动方式之后,请按键运行
ANSYS.
命令方式 在当前系统下键入一个命令,ANSYS将被启动 。
例如:
• ansys57 • ansys57 -g • ansys57 -g -j plate • ansys57 -g -p ANE3FL -d 3d -j proj1 -m 128
如果不采用交互方式,输入文件的错误将 导致批处理终止。
例如求解,最好的操作方式是不需要用 户干预的。
• 在本学习过程中,我们将主要讲解 交互式操作。
ANSYS 入门
…启动 ANSYS
启动 (续)
在弹出的 启动对话框中按 Interactive按钮:
• ANSYS产品
• 工作目录 –所有文件将要存贮的目 录。
ANSYS入门
…启动 ANSYS
启动 (cont’d)
当ANSYS 以交互方式启动后,用户图形界面(GUI) 将自动显示。
交互方式与批处理模式的比较: • 交互方式允许您与ANSYS面对面的 “交流”,检查您的每一步操作 • 批处理方式 以输入命令文件的方式 工作,允许您在后台运行 ANSYS 。
ANSYS 入门
... GUI方式
应用菜单 包含ANSYS运行过程中通常使用的功能,如:图形,
在线帮助,选择, 文件管理等. 与主菜单的约定类似:
-- “…”表示产生一个对话框 -- “ +”表示图形拾取 -- “ >”表示将产生下一个子菜单 -- “ ” (空缺)表示进行一个操作
ANSYS 入门
ANSYS/ Mechanical
ANSYS/ Multiphysicral
ANSYS/ LS-DYNA
ANSYS/ PrepPost
ANSYS/ FLOTRAN
ANSYS/ ED
ANSYS/ ProFEA
ANSYS/ University
DesignSpace
图形
显示由 ANSYS 创 建或传入ANSYS的 图形。
ANSYS入门
... GUI方式
主菜单
包括分析所需的主要功能。
在进行下一个功能之前,重叠的独立窗 口允许您完成所有必须的操作。
约定:
“…”表示产生一个对话框 “ +”表示图形拾取 “ >”表示将产生下一个子菜 单 “ ” (空缺)表示运行一个 ANSYS命令
• 图形设置 – 如果您配置了 3-D显 示卡请您选择 3-D。否则, 在Unix 系统上请您选择 X11 , 在 Windows系统上请您选择win32.
• 作业名 –作业名, 不超过 32 个字 符, 该作业名将作为所有生成文件 的前缀。缺省为 file或为上一次定 义的作业文件名。
• 内存空间 –缺省值将满足大多数情 况
求解方法 在 ANSYS 产品中,求解结构问题有两 种方法:h-方法和p-方法。
h-方法可用于任何类型的结构分析,而 p-方法只能用于线性结构静力分析。根据所 求的问题,h-方法通常需要比p-方法更密的 网格。p-方法在应用较粗糙的网格时,提供 了求得适当精度的一种很好的途径。
ANSYS 入门
后显示如下的
GUI窗口:
显示提示信息,输 入ANSYS命令。能 够方便的获取以前 输入的所有命令。
功能菜单
包含例如文件管理、 选择、显示控制、参 数设置等功能。
主菜单
包含主要的 ANSYS 功能,分为前处理、 求解、后处理等。
工具条
将常用的命令制成工 具条,方便使用。
输出
显示软件的文本输 出,通常在其它窗 口后面,需要查看 时可提到前面。
• 微机电系统
• 运动器械
有限元分析与ANSYS
…关于ANSYS
ANSYS/Multiphysics 是ANSYS产品的“旗舰”,它包 括所有工程学科的所有性能
ANSYS/Multiphysics有三个主要的组成产品
• ANSYS/Mechanical - ANSYS/机械-结构及热 • ANSYS/Emag -ANSYS电磁学 • ANSYS/FLOTRAN - ANSYS计算流体动力学
ANSYS软件介绍
ANSYS是一个完整的FEA软件包,它适合世界范围各个 工程领域的工程师们使用:
• 结构分析
• 热分析
• 流体分析,包括CFD(计算流体动力学)
• 电 / 静电场分析
• 电磁场分析
ANSYS在部分工业领域中的应用如下:
• 航空航天 • 汽车工业 • 生物医学 • 桥梁、建筑
• 电子产品 • 重型机械
ANSYS 入门
…启动 ANSYS
命令行 (续)
典型的开始选项中,通常命令选择项有:
-g
(开始后将自动显示 GUI)
-p
产品代码
-d
图形设备
-j 工作名称
-m
内存
工作目录是命令运行的目录。
参考您的 ANSYS安装和配置手册获取命令行的 详细信息。
ANSYS 入门
图形用户界面
进入 ANSYS 输入
其它产品:
• ANSYS/LS-DYNA -高度非线性结构问题 • DesignSpace –CAD环境下,适合快速分析容易使用的设计和分
析工具 • ANSYS/ProFEA –Pro/ENGINEER的ANSYS 分析接口。
有限元分析与ANSYS
…关于ANSYS
ANSYS/ Professional
启动 ANSYS
有两种方式启动 ANSYS:
• 通过交互方式 • 通过命令
交互方式 通过菜单上的相应按钮启动
ANSYS 在Unix 系统中, 进入
xansys57 & 导出操作平台。 在Windows 系统中, 按 Start
> Programs > ANSYS 5.7。
Unix 平台
Windows 平台
... GUI方式
输入窗口
允许您输入命令。 (大多数 GUI功能都能通过输 入命令来实现. 如果您知道这些命令,可以通过输 入窗口键入。)
在拾取图形时您也可以通过键入命令的方式实现。
计算机在材料科学中的应用
ANSYS软件介绍
制作人
周亚运 张自强 高俊豪 陈威 葛云
简介
ANSYS(Anzlysis System)—世界著名 力学分析专家、匹兹堡大学教 J.Swanson 创立的SASI(Swanson Analysis System Inc)的大型通用有限元分析软件 ,世界最权威的有限元软件。
