Free differential Rota-Baxter Lie algebras and Grobner-Shirshov bases
freesurfer对结构核磁共振成像分割输出结果介绍 -回复

freesurfer对结构核磁共振成像分割输出结果介绍-回复Freensurfer是一个被广泛使用的开源软件包,用于自动进行结构核磁共振成像的脑结构分割和配准。
它提供了一种非常有效的方法,可以对脑图像进行分割和分类,将脑组织分为不同的区域,从而对疾病诊断、脑结构研究和大脑功能定位等领域提供有力的支持。
本文将详细介绍freesurfer 的分割输出结果。
首先,freesurfer能够自动将脑图像分割为一系列的区域。
这些区域包括大脑皮层、皮层下灰质结构、白质结构和深灰质结构等。
每个区域都会被分配一个特定的标签,用于标识其在脑中的位置和类型。
这些标签是根据大量的脑图像数据库来进行训练和优化的,因此其准确度非常高。
其次,freesurfer还能够提供脑皮层的各个解剖学表面的详细信息。
它可以生成脑皮层的灰质厚度图,用于评估不同脑区的形态特征。
此外,freesurfer还可以提供脑皮层的曲率信息、皮层纹理映射和表面网络连接信息等,这些信息对于理解脑皮层的功能组织和连接具有重要意义。
在分割输出结果中,freesurfer还提供了其他一些有用的指标和测量结果。
例如,它可以计算大脑和各个脑区的体积、厚度和表面积等。
这些测量值对于研究不同脑区之间的差异、脑发育和衰老过程中的变化以及神经系统疾病的诊断和评估具有重要意义。
此外,freesurfer还提供了一些可视化工具,用于展示分割结果和测量数据。
它可以生成三维脑模型,显示不同脑区的位置和形状。
同时,freesurfer 还支持将测量结果绘制在脑模型上,比如将体积和厚度信息用伪彩色或颜色映射的方式显示出来,从而使得研究人员能够更直观地观察和分析数据。
值得一提的是,freesurfer的分割输出结果可以根据研究人员的需求进行进一步的定制和分析。
例如,用户可以选择性地提取特定的脑区进行详细分析,或者将不同的脑区合并或分割成更小的子区域。
这为研究人员提供了丰富的数据和选项,从而可以开展更加深入和精确的研究。
垂直U型地埋管换热器性能的动态仿真

总756期第二十二期2021年8月河南科技Journal of Henan Science and Technology垂直U型地埋管换热器性能的动态仿真代兰花肖瑶瑶(常州工学院,江苏常州213032)摘要:本文基于圆柱内热源理论建立了垂直U型地埋管换热器的数学模型。
在MATLAB软件中建立了垂直U型地埋管换热器的动态仿真平台。
利用该仿真平台研究进口温度、进口流速、钻井深度、回填材料、土壤以及间歇运行时间比等因素对垂直U型地埋管换热器换热性能的影响规律。
结果表明,在一定范围内提高进口温度可以提升垂直U型地埋管换热器的换热性能;当进口流速为0.4~0.6m/s时,增大进口流速可以有效提升垂直U型地埋管换热器的换热量;钻井深度越深,钻孔总换热量增加明显,单位钻孔深度换热量略微减小;但随着运行时间的增加,回填材料导热系数对换热的影响逐渐降低;土壤的导热系数越大,垂直U型地埋管换热器的换热量越大;采用间歇运行的方式可以提升垂直U型地埋管换热器的换热性能。
关键词:垂直U型地埋管换热器;动态仿真;影响因素;换热性能中图分类号:TU831.4文献标识码:A文章编号:1003-5168(2021)22-0104-06 Dynamic Simulation on the Performance of Vertical U-Shaped Buried PipeHeat ExchangerDAI Lanhua XIAO Yaoyao(Changzhou Institute of Technology,Changzhou Jiangsu213032)Abstract:In this paper,a mathematical model of the vertical U-shaped borehole heat exchanger is established based on the theory of the heat source inside the cylinder.The dynamic simulation platform of the vertical U-shaped buried pipe heat exchanger is established in MATLAB software.The simulation platform is used to study the influence of fac⁃tors such as inlet temperature,inlet flow rate,drilling depth,backfill material,soil,and intermittent operation time ra⁃tio on the heat transfer performance of the vertical U-shaped buried heat exchanger.The results show that increasing the inlet temperature within a certain range can improve the heat transfer performance of the vertical U-shaped bore⁃hole heat exchanger;when the inlet flow velocity is0.4~0.6m/s,increasing the inlet flow velocity can effectively in⁃crease the heat transfer of the vertical U-shaped borehole heat exchanger;the deeper the drilling depth,the increase in the total heat transfer of the borehole is obvious,and the heat transfer per unit depth of the borehole slightly de⁃creases;however,as the operating time increases,the thermal conductivity of the backfill material gradually reduces its impact on heat transfer;the greater the thermal conductivity of the soil,the greater the heat transfer of the vertical U-shaped buried heat exchanger;the use of intermittent operation can improve the heat exchange performance of the vertical U-shaped borehole heat exchanger.Keywords:vertical U-shaped boried pipe heat exchange;dynamic simulation;influencing factors;heat transfer per⁃formance地源热泵技术的重点是设计出高效的地埋管换热器,地埋管换热器直接影响了整个系统的效率。
trimAl Phylogenetics Alignment Trimming Tool说明书

trimAl: a tool for automated alignment trimming in large-scale phylogenetics analyses Salvador Capella-Gutiérrez, Jose M. Silla-Martínez and Toni GabaldónTutorialVersion 1.2trimAl tutorialtrimAl is a tool for the automated trimming of Multiple Sequence Alignments. A format inter-conversion tool, called readAl, is included in the package. You can use the program either in the command line or webserver versions. The command line version is faster and has more possibilities,so it is recommended if you are going to use trimAl extensively.The trimAl webserver included in Phylemon 2.0 provides a friendly user interface and the opportunity to perform many different downstream phylogenetic analyses on your trimmed alignment. This document is a short tutorial that will guide you through the different possibilities of the program.Additional information can be obtained from where a more comprehensive documentation is available.If you use trimAl or readAl please cite our paper:trimAl: a tool for automated alignment trimming in large-scale phylogenetic analyses.Salvador Capella-Gutierrez;Jose M.Silla-Martinez;Toni Gabaldon.Bioinformatics 2009 25: 1972-1973.If you use the online webserver phylemon or phylemon2, please cite also this reference:Phylemon:a suite of web tools for molecular evolution,phylogenetics and phylogenomics.Tárraga J, Medina I, Arbiza L, Huerta-Cepas J, Gabaldón T, Dopazo J, Dopazo H. Nucleic Acids Res. 2007 Jul;35 (Web Server issue):W38-42.1. Program Installation.If you have chosen the trimAl command line version you can download the source code from the Download Section in trimAl's wikipage.For Windows OS users, we have prepared a pre-compiled trimAl version to use in this OS. Once the user has uncompressed the package, the user can find a directory,called trimAl/bin, where trimAl and readAl pre-compiled version can be found.Meanwhile for the OS based on Unix platform, e.g. GNU/Linux or MAC OS X, the user should compile the source code before to use these programs. To compile the source code, you have to change your current directory to trimAl/source and just execute "make".Once you have the trimAl and readAl binaries program, you should check if trimAl is running in appropriate way executing trimal program before starting this tutorial.2. trimAl. Multiple Sequence Alignment dataset.In order to follow this tutorial, we have prepared some examples. These examples have been taken from and you can use the codes from these files to get more information about it in this database.You can find three different directories called Api0000038, Api0000040 and Api0000080 with different files. The directory contains these files:A file .seqs with all the unaligned sequences.A file .tce with the Multiple Sequence Alignment produced by T-Coffee1.A file .msl with the Multiple Sequence Alignment produced by Muscle2.A file .mft with the Multiple Sequence Alignment produced by Mafft3.A file .clw with the Multiple Sequence Alignment produced by Clustalw4.A file .cmp with the different names of the MSAs in the directory. This file would be used by trimAl to get the most consistent MSA among the different alignments.You can use any directory to follow the present tutorial.3. Useful trimAl's features.Among the different trimAl parameters, there are some features that can be useful to interpret your alignment results:-htmlout filename. Use this parameter to have the trimAl output in an html file. In this way you can see the columns/sequences that trimAl maintains in the new alignment in grey color while the columns/sequences that have been deleted from the original alignment are in white color.-colnumbering. This parameter will provide you the relationship between the column numbers in the trimmed and the original alignment.-complementary. This parameter lets the user get the complementary alignment, in other words,when the user uses this parameter trimAl will render the columns/sequences that would be deleted from the original alignment.-w number. The user can change the windows size, by default 1, to take into account the surrounding columns in the trimAl's manual methods. When this parameter is fixed, trimAl take into account number columns to the right and to the left from the current position to compute any value, e.g. gap score, similarity score, etc. If the user wants to change a specific windows size value should use the correspond parameter-gw to change window size applied only a gap score assessments, -sc to change window size applied only to similiraty score calculations or -cw to change window size applied only to consistency part.4. Useful trimAl's/readAl's features.Both programs, trimAl and readAl, share common features related to the MSA conversion. It is possible to change the output format for a given alignment, by default the output format is the same than the input one, you can produce an output in different format with these options: -clustal. Output in CLUSTAL format.-fasta. Output in FASTA format.-nbrf. Output in PIR/NBRF format.-nexus. Output in NEXUS format.-mega. Output in MEGA format.-phylip3.2. Output in Phylip NonInterleaved format.-phylip. Output in Phylip Interleaved format.5. Getting Information from Multiple Sequence Alignment.trimAl computes different scores, such as gap score or similarity score distribution, from a given MSA. In order to obtain this information, we can use different parameters through the command line version.To do this part,we are going to use the MSA called Api0000038.msl.This file is in the Api0000038 directory.$ cd Api0000038$ trimal -in Api0000038.msl -sgt$ trimal -in Api0000038.msl -sgc$ trimal -in Api0000038.msl -sct$ trimal -in Api0000038.msl -scc$ trimal -in Api0000038.msl -sidentYou can redirect the trimAl output to a file. This file can be used in subsequent steps as input of other programs, e.g.gnuplot,,microsoft excel,etc,to do plots of this information.$ trimal -in Api0000038.msl -scc > SimilarityColumnsFor instance, in the lines below you can see how to plot the information generated by trimAl using the GNUPLOT program.$ gnuplotplot 'SimilarityColumns' u 1:2 w lp notitleset yrange [-0.05:1.05]set xrange [-1:1210]set xlabel 'Columns'set ylabel 'Residue Similarity Score'plot 'SimilarityColumns' u 1:2 w lp notitleexitIn this other example you can see the gaps distribution from the alignment. This plot also was generated using GNUPLOT$ trimal -in Api0000038.msl -sgt > gapsDistribution$ gnuplotset xlabel '% Alignment'set ylabel 'Gaps Score'plot 'gapsDistribution' u 7:4 w lp notitleexit6. Using user-defined thresholds.If you do not want to use any of the automated procedures included in trimAl (see sections 7 and 8) you can set your own thresholds to trim your alignment. We will use the parameter -htmlout filename for each example so differences can be visualized. In this example, we will use the Api0000038.msl file from the Api0000038 directory.Firstly, we are going to trim the alignment only using the -gt value which is defined in the [0 - 1] range. In this specific example, those columns that do not achieve a gap score, at least, equal to 0.190, meaning that the fraction of gaps on these columns are smaller than this value, will be deleted from the input alignment.$ trimal -in Api0000038.msl -gt 0.190 -htmlout ex01.htmlYou can see different parts of the alignment in the image below.This figure has been generated from the trimAl's HTML file for the previous example.In this other example, we can see the effect to be more strict with our threshold. An usual consequence of higher stringency is that the trimmed MSA has fewer columns. Be careful so you do not remove too much signal$ trimal -in Api0000038.msl -gt 0.8 -htmlout ex02.htmlTo be on the safe side, you can set a minimal fraction of your alignment to be conserved. In this example,we have reproduced the previous example with the difference that here we required to the program that, at least, conserve the 80% of the columns from the original alignment. This will remove the most gappy 20% of the columns or stop at the gap threshold set.$ trimal -in Api0000038.msl -gt 0.8 -cons 80 -htmlout ex03.htmlSecondly,we are going to introduce other manual threshold-st value.In this case,this threshold,also defined in the[0-1]range,is related to the similarity score.This score measures the similarity value for each column from the alignment using the Mean Distance method, by default we use Blosum62 similarity matrix but you can introduce any other matrix (see the manual). In the example below, we have used a smaller threshold to know its effect over the example.$ trimal -in Api0000038.msl -st 0.003 -htmlout ex04.htmlIn this example, similar to the previous example, we have required to conserve a minimum percentage of the original alignment in a independent way to fixed by the similarity threshold.A given threshold maintains a larger number of columns than the cons threshold, trimAl selects this first one.$ trimal -in Api0000038.msl -st 0.003 -cons 30 -htmlout ex05.htmlThirdly, we are going to see the effect of combining two different thresholds. In this case, trimAl only maintains those columns that achieve or pass both thresholds.$ trimal -in Api0000038.msl -st 0.003 -gt 0.19 -htmlout ex06.htmlFinally, we are going to see the effect of combining two different thresholds with the cons parameter. In this case, if the number of columns that achieve or pass both thresholds is equal or greater than the percentage fixed by cons parameter, trimAl chose these columns. However, if the number of columns that achieve or pass both thresholds is less than the number of columns fixed by cons parameter, trimAl relaxes both to thresholds in order to retrieve those columns that lets to achieve this minimum percentage.$ trimal -in Api0000038.msl -st 0.003 -gt 0.19 -cons 60 -htmlout ex07.html7. Selection of the most consistent alignment.trimAl can select the most consistent alignment when more than one alignment is provided for the same sequences (and in the same order) using the -compareset filename parameter. To do this part, we are going to move to Api0000040 directory, we can find there a file calledApi0000040.cmp listing the alignment paths. Using this file, we execute the instruction below to select the most consistent alignment among the alignment provided$ trimal -compareset Api0000040.cmpAs in previous section, once trimAl has selected the most consistent alignment, we can get information about the alignment selected using the appropriate parameters. For example, we can use the follow instructions to know the consistency value for each column in the alignment or its consistency values distribution$ trimal -compareset Api0000040.cmp -sct$ trimal -compareset Api0000040.cmp -sccAlso, we can trim the selected alignment using a specific threshold related to the consistency value. To do that, we should use the -ct value where the value is a number defined in the [0 - 1] range. This number refers to the average conservation of residue pars in that column with respect to the other alignments.$ trimal -compareset Api0000040.cmp -ct 0.6 -htmlout ex08.htmlOn the same way than the previous section, we can define a minimum percentage of columns that should be conserve in the new alignment. For this purpose, we have to use the cons parameter as we explained before.$ trimal -compareset Api0000040.cmp -ct 0.6 -cons 50 -htmlout ex09.htmlFinally, we can combine different thresholds, in fact, we can use all of them as well as we can define a minimum percentage of columns that should be conserve in the output alignment. In the line below, you can see an example of this situation.$ trimal -compareset Api0000040.cmp -ct 0.6 -cons 50 -gt 0.8 -st 0.01-htmlout ex10.html8. Applying automated methods.One of the most powerful aspects of trimAl is that it provides you with several automated options.This option will automatically select the most appropriate thresholds for your alignment after examining the distribution of various parameters along your alignment. Among the alignment features that trimAl takes into account to compute these optimal cut-off are the gap distribution, the similarity distribution, the identity score, etc.You can find a complete explanation about all of these methods in the trimAl's Publications Section.Here,we provide some examples on how to use these methods.The automated methods, gappyout, strict and strictpus, can be used independently if you are working with one or more than one alignment, in the last case, for the same sequences.In the lines below, you can see how to use the gappyout method in both ways. This method will eliminate the most gappy fraction of the columns from your alignment. For this, we are going to continue using the same directory than the previous section.$ trimal -compareset Api0000040.cmp -gappyout -htmlout ex11.html$ trimal -in Api0000040.mft -gappyout -htmlout ex12.htmlIn this case, we are going to use the same files than in the example before but we have changed the method to trim the alignmnet. Now, we are using strict and strictplus methods. These two methods combine the information on the fraction of gaps in a column and their similarity scores, being strictplus for more stringent than strict method.$ trimal -compareset Api0000040.cmp -strict -htmlout ex13.html$ trimal -in Api0000040.clw -strictplus -htmlout ex14.htmling an heuristic method to decide which is the best automated method for a given MSA.Finally, we implemented an heuristic method to decide which is the best automated method to trim a given alignment. The heuristic method takes into account alignment features such as the number of sequences in the alignment as well as some measures about the identity score among the sequences in the alignment or among the best pairwise sequences in that MSA. According to these characteristics trimAl will decide upon one of the two automated methods (gappyout or strictplus).To illustrate how to use this method, we provide a couple of example using the same directory than the section before. First, we used trimAl to selecte the most consistent alignment and then we trimmed that alignmnet using our heuristic method.$ trimal -compareset Api0000040.cmp -automated1 -htmlout ex15.htmlThen, we trim a single MSA using the previously mentioned method.$ trimal -in Api0000040.msl -automated1 -htmlout ex16.html10. Getting more information.We hope that this short introduction to trimAl's features has been useful to you.We advise you to visit periodically the trimAl's wikipage()where you could get the latest news about the program as well as more information, examples, etc, about trimAl's package. You can also subscribe to the mailing list if you want to be updated in new trimAl developing.11. References.1.T-Coffee: A novel method for fast and accurate multiple sequence alignment.Notredame C, Higgins DG, Heringa J. J Mol Biol. 2000 Sep 8;302(1):205-17.2.MUSCLE:multiple sequence alignment with high accuracy and highthroughput. Edgar RC.Nucleic Acids Res. 2004 Mar 19;32(5):1792-7.3.MAFFT: a novel method for rapid multiple sequence alignment based on fastFourier transform. Katoh K, Misawa K, Kuma K, Miyata T. Nucleic Acids Res. 2002 Jul 15;30(14):3059-66.4.CLUSTAL W:improving the sensitivity of progressive multiple sequencealignment through sequence weighting,position-specific gap penalties and weight matrix choice. Thompson JD, Higgins DG, Gibson TJ. Nucleic Acids Res. 1994 Nov 11;22(22):4673-80.。
人体结构学 Human Structure学习通章节答案期末考试题库2023年

人体结构学 Human Structure学习通超星课后章节答案期末考试题库2023年1.Which bones belong to the shoulder girdle?答案:Scapula###Clavicle2.The paired cerebral bones are答案:parietal bone###temporal bone3.Shoulder joint is formed by答案:head of humerus###glenoid cavity of scapula4.Please deseribe the location and openings of the paranasal sinuses答案:答5.Please describe the formation, main structures and communications of the middle Cranial fossa.答案:答6.Please describe the joints of the vertebral bodies.答案:答7.Please describe the joints of the vertebral arches.答案:答8.Please describe the composition, characteristics and movements of theshoulder joint.答案:答9.Which bone belongs to the long bone?答案:Femur10.Which bones belong to the irregular bone?答案:Vertebra###Sphenoid bone11.The blood- testis barrier does NOT include the答案:gap junction between adjacent spermatogomia12.Which of the following description is true about the primordial follicles答案:The primordial follicle consists of a primary oocyte and a layer of flat follicle cells.13.(英文答题,第一空填1个单词,第二空3个单词)The axial bone contains fromup downwards _____ and_____.答案:skull###bonesoftrunk14.About the component of nephron, the correct option is答案:renal corpuscle, proximal tubules, distal tubules and thin segment15.About the features of proximal tubule, the WRONG option is答案:The cytoplasm of epithelial cell is weakly basophilic.16. A patient presents in your office after having a positive result on a homepregnancy test. Her menstrual cycle has always been the classic 28-day cycle discussed in textbooks, with ovulation occurring on the 14th day following the start of menstruation. Her menstrual period began on August 19.2019.You estimate her EDD to be答案:on May 26, 202017.Which of the following is NOT considered one of the fetal membranes答案:buccopharyngeal membrane18.Which bone does not form the anterior cranial fossa?答案:Temporal bone19.Which bone forms both the middle and posterior cranial fossa?答案:Temporal bone20.(英文答题,第一空填1个单词,第二空1个单词,第三空2个单词)Thesternum consists from up downwards of_____ , _______ and ______ .答案:manubrium###body###xiphoidprocess21.Of the following statements about epididymis, the WRONG option is答案:The ductus epididymis is lined with a simple columnar epithelium22.Of the following statements about trachea, the WRONG option is答案:The adventitia is constructed of the elastic cartilage rings.23.The interalveolar septum does NOT contain答案:ciliated cell24.All the following cells are included in the spermatogenic epithelium, EXCEPTthe答案:Leydig cells25.Please describe the general features of the vertebrae.答案:答26.Which bone forms the posteroinferior part of the bony nasal septum?答案:Vomer27.Drawing pictures of Thoracic vertebra from anterior and lateral view.答案:答28.Which bone does not form the thoracic Cage?答案:Sacrum29.About the scapula, which of the statements is not true?答案:It has three borders, three angles and three surfaces.30.About the component of the renal corpuscle, the WRONG option is答案:At the vascular pole, the efferent arteriole enters the glomerulus.31.Of the following statements about podocyte, the correct option is答案:They form the visceral layer of the Bowman's capsule.32.An infant is born with a sacrococcygeal teratoma. Biopsy(组织活检) andhistologic analysis reveal that it contains intestinal epithelia, cardiac muscle, cartilage, and integument tissue. You counsel the mother that the tumor is benign(良性的)and recommend surgical removal. This tumor was caused by which developmental anomaly?答案:Failure of primitive streak regression33.Which of the following structure is NOT included in the secondary follicle?答案:secondary oocyte34.All the following are from mesoderm EXCEPT the答案:spinal cord35.Which of the following descriptions is NOT true about the corpus luteum?答案:The corpus luteum continues to produce estrogen and progesterone during the whole process of pregnancy.36.Of the following statements about the alveolus of lung, the WRONG option is答案:It opens on the wall of terminal bronchioles.37.Which of the following descriptions is NOT true about the secretory phase ofa menstrual cycle?答案:The basal layer of endometrium becomes thicker .38.Of the following statements about Leydig cells, the correct option is答案:It secretes testosterone.39.Of the following statements about macula densa, the WRONG option is答案:It is derived from smooth muscle fibers of afferent arteriole.40.Of the following statements concerning terminal bronchioles, the WRONGoption is答案:They have some mixed gland.41.Of the following options, the blood-air barrier does NOT contain答案:typeⅡalveolar cells42.All the following cells are included in the spermatogenic cells, EXCEPT答案:Sertoli cells43.Which of the following does not belong to the joints of the vertebral arches?答案:Anterior longitudinal ligament44.Human chorionic gonadotropin is produced by the答案:syncytiotrophoblast45.Which of the followings is not enclosed in the articular capsule of shoulderjoint ?答案:Tendon of the short head of the biceps46.The pathway connecting the infratemporal fossa with the orbit is答案:inferior orbital fissure47.When does ovulation occur in a menstrual cycle?答案:the 14th day。
考虑混凝土徐变收缩的超高层偏心核心筒-框架结构水平变形和结构内力分析

第 39 卷第 3 期2023 年6 月结构工程师Structural Engineers Vol. 39 , No. 3Jun. 2023考虑混凝土徐变收缩的超高层偏心核心筒-框架结构水平变形和结构内力分析鲁正1,*周映旻1王远航2王超3张富文3(1.同济大学结构防灾减灾工程系,上海 200092; 2.中建三局集团第一建设有限责任公司,武汉 266100;3.上海市建筑科学研究院有限公司,上海 200032)摘要核心筒偏置的框架-核心筒结构在平面上不对称,可能在水平方向产生较大的不利变形。
采用混凝土徐变收缩B3模型,在ETABS中对某偏心核心筒-框架结构进行施工分析,计算了结构在不同时期的水平变形,并分析了水平变形对结构内力的影响。
计算中考虑了钢管混凝土柱中钢管的环箍效应,以及钢管与混凝土的应力重分布、施工工序等因素。
计算结果表明,偏心核心筒-框架结构的水平变形沿结构高度呈“中间大、两头小”分布。
研究结果显示徐变和收缩引起的水平变形随时间明显增加,部分幅值在后期大于弹性变形;合理规划施工顺序可有效降低结构构件内力。
通过与实测数据的对比,验证了所用模型的准确性。
关键词高层结构,水平变形,徐变收缩,施工分析Analysis of Horizontal Deformation and Internal Force of Super High-Rise Frame-Eccentrical Core Tube StructureConsidering Creep and Shrinkage of ConcreteLU Zheng1,*ZHOU Yingmin1WANG Yuanhang2WANG Chao3ZHANG Fuwen3(1.Department of Disaster Mitigation for Structures,Tongji University, Shanghai 200092, China;2.The First Construction Co.,Ltd., of China Construction Third Engineering Bureau Group, Wuhan 266100, China;3.Shanghai Research Institute of Building Sciences Co.,Ltd., Shanghai 200032, China)Abstract The frame-eccentrical core tube structure is asymmetric in plane and may produce large adverse deformation in horizontal direction. The B3 model of concrete is used to analyze the construction of a frame-eccentrical core tube structure in ETABS. The structural horizontal deformation in different periods is calculated and the influence of horizontal deformation on the internal force of the structure is analyzed. The hoop effect of steel tube in CFST column,the stress redistribution between steel tube and concrete and the construction sequences are considered. The calculation results show that the horizontal deformation of the eccentric core-frame structure is large in the middle and small at both ends along the structural height. The results show that the horizontal deformation caused by creep and shrinkage increases obviously with time, and their amplitude will be larger than the elastic deformation in partial components in the later stage; besides, the results also show that reasonable planning for construction sequence can effectively alleviate the internal force of structural members. The accuracy of the model is verified by comparing with the measured data.收稿日期:2022-05-20基金项目:国家重点研发项目(2020YFB1901402),上海市优秀学术带头人计划(20XD1423900),中央高校基本科研业务费专项资金资助(11080)* 联系作者:鲁正(1982-),男,汉族,浙江上虞人,工学博士,教授,主要从事高层建筑抗震性能研究。
Partial Differential Equations

Partial Differential Equations Partial Differential Equations (PDEs) are a fundamental tool in the field of mathematics, with applications across various scientific disciplines such as physics, engineering, and economics. These equations involve multiple independent variables and their partial derivatives, making them more complex than ordinary differential equations. Solving PDEs is crucial in understanding physical phenomena and predicting future behaviors, making them a valuable tool for researchers and scientists. One of the key challenges in working with PDEs istheir inherent complexity and nonlinearity. Unlike ordinary differential equations, which involve only one independent variable, PDEs deal with multiple variables and their partial derivatives. This complexity often makes it difficult to find analytical solutions, leading researchers to rely on numerical methods for approximation. These numerical methods, such as finite difference or finite element methods, involve discretizing the domain and solving the resulting system of equations iteratively. While these methods are powerful tools, they can be computationally expensive and time-consuming. Another challenge in working with PDEs is the need for boundary and initial conditions to uniquely determine a solution. Without proper boundary and initial conditions, the solution to a PDE may not be well-defined or may have multiple solutions. Determining these conditions can be a non-trivial task, requiring a deep understanding of the physical system being modeled. Additionally, small changes in the boundary orinitial conditions can lead to significant changes in the solution, highlighting the sensitivity of PDEs to their inputs. Despite these challenges, PDEs are incredibly versatile and powerful tools for modeling a wide range of physical phenomena. From heat conduction to fluid dynamics to quantum mechanics, PDEs provide a framework for understanding and predicting the behavior of complex systems. By solving PDEs, researchers can gain insights into the underlying mechanisms governing these systems and make informed decisions based on their predictions. This ability to model and simulate real-world phenomena is invaluable in fields such as engineering, where accurate predictions can lead to improved designs and optimized performance. In addition to their scientific applications, PDEs also have practical implications in everyday life. For example, PDEs are usedin weather forecasting models to predict the movement of storms and thedistribution of rainfall. By solving PDEs governing fluid dynamics and thermodynamics, meteorologists can generate accurate forecasts that help communities prepare for severe weather events. Similarly, PDEs are used infinancial modeling to predict stock prices and analyze risk factors. By solving PDEs describing the dynamics of financial markets, economists and analysts can make informed decisions about investments and portfolio management. In conclusion, Partial Differential Equations are a powerful tool with diverse applications in science, engineering, and economics. While they pose challenges in terms of complexity and sensitivity to initial conditions, their ability to model and simulate real-world phenomena makes them invaluable in research and practical applications. By solving PDEs, researchers can gain insights into the underlying mechanisms governing complex systems and make informed decisions based on their predictions. As technology advances and computational power increases, the use of PDEs is likely to grow, leading to new discoveries and innovations in various fields.。
2009年分子生物学quiz1参考答案(精)

2009年分子生物学quizl 参考答案2. What is central dogma? What contents have you learned in each part of the central dogma? (5’)The central dogma is the pathway for the flow of genetic information.We have learnt the maintenance of the genome (the structure of the genetic material and its faithful duplication) and the expression of the genome (the conversion of genetic instructions contained in DNA into proteins).(缺 reverse transcription 扣一分)3. Write out the structure of four bases, label the positions that participate Watson-Crick base pairing and that connect to the ribose? (10’)如果解答中可以 置等,会酌情wg/Q sugar4. What are the features of DNA structure? And how RNA structure differs from DNA? (2 Copyright © 2004 Pearson Education f I nc., publishing as Benjamin Cummings Two antiparallel polynucleotide chains are twisting around each other in the form of a double helix. 6’(polynucleotide(building block) 2’,antiparallel 2’,double helix 2’)Hydrogen Bonding determines the Specificity of base pairing (complimentarity).2’Stacking interaction between bases determines the stability of DNA double helix, hydrogen bond also contributes to stability.2’The double helix has Minor and Major grooves. (A B Z forms)2’Differences :Primary structure( building block): 2’dNTP vs. rNTP ; T vs. U ; double-stranded vs. single-stranded.Secondary structure: 4’键;AG 是二环,TC 是单环;氢键的位 The features of DNA structure :间是双键,CGDNA has stable double helical structure. (full complimentarity)RNA chains fold back on themselves to form local regions of double helix similar to A-form DNA. RNA helix are the base-paired segments between short stretches of complementary sequences, which adopt one of the various stem-loop structures, pseudoknots.( inter- and intra-molecular base pairing) Tertiary structures: 2’DNA: no tertiary structure.RNA can fold up into complex tertiary structures, because RNA has enormous rotational freedom in the backbone of its non-base-paired regions.pare the chemistry of DNA synthesis and RNA synthesis. (5’)(clues: compare=differences+the same; chemistry=substrate+direction+ energy)Differences:(1)DNA synthesis requires deoxynucleotide triphosphates while RNA synthesis requires oxynucleotide triphosphates;(1')(2)The base for DNA synthesis are A/T/C/G while that for RNA synthesis are A/U/C/G;(1')(3)DNA synthesis needs a primer:template junction while RNA synthesis do not;(1')The same:(1)The direction of both DNA synthesis and RNA synthesis is 5' to 3';(1')(2)The energy needed for DNA synthesis as well as RNA synthesis is hydrolysis of pyrophosphate (PPi).(1')6.Describe the functions of each domain of the DNA polymerase. (14’+1')DNA polymerase palm domain(1'):(1)Contains two catalytic sites, one for addition of dNTPs (1')and one for removal of the mispaired dNTP.(1')(2)The polymerization site: (1) binds to two metal ions that alter the chemical environment around the catalytic site and lead to the catalysis. (1')(2) Monitors the accuracy of base-pairing for the most recently added nucleotides by forming extensive hydrogen bond contacts with minor groove of the newly synthesized DNA. (1')(3)Exonuclease site/proof reading site . The mechanism of proof reading is kinetic selectivity(1') and the mismatched dNMP is removed by proofreading exonuclease in the direction of 3'-5'(1'). DNA polymerase finger domain:(1')(1)Binds to the incoming dNTP, encloses the correct paired dNTP to the position for catalysis;(1') (2)Bends the template to expose the only nucleotide at the template that ready for forming base pair with the incoming nucleotide;(1')(3)Stabilization of the pyrophosphate;(1')DNA polymerase thumb domain:(1')(1)Not directly involved in catalysis;(2)Interacts with the synthesized DNA to maintain correct position of the primer and the active site, (1')and to maintain a strong association between DNA Pol and its substrate.(1')7.How replication of a DNA molecule is accomplished in bacteria ?Initiation:(1)Recognition and binding of OriC by DnaA(initiator)-ATP.(2)Helicase (DnaB) loading and DnaC(helicase loader) and DNA unwinding.(3)Primase synthesizes RNA primer, and D NA Polymerase III synthesizes the new DNA strand.(The "trombone" model was developed to explain lagging strand and Elongation:leading strand synthesized simultaneously):(1)Leading strand: newly synthesized DNA strand that is continuously copied from the template strand by a DNA polymerase after the first RNA primer was made by a primase. A sliding clamp is usually loaded to the DNA polymerase to increase the polymerase processivity. The3 direction of theing strand is the same as the moving direction of the replication fork.(2)Lagging strand is discontinuously copied from the template strand. The 3 direction of the lagging strand is opposite to the moving direction of the replication fork. Primase makes RNA primers periodically after the template strand is unwound and becomes single-stranded. DNA polymerase extends each primer to synthesis short DNA fragments, called Okazaki fragments. The polymerase dissociates from the template strand when it meets the previous Okazaki fragment. Finally, RNA primers are digested by an RNase H activity, and the gaps are filled by DNA polymerase. At last, the adjacent Okazaki fragments are covalently joined together by a DNA ligase to generate a continuous, intact strand of new DNA.Termination:Type II topoisomerases separate daughter DNA molecules.8.How transcription of a RNA molecule by RNA polymerase II is initiated, elongated and terminated in eukaryotes (25’)Initiation: (11’+附加分)A.1.Promoter recognition: TBP in TFIID binds to the TATA box;(1’) TFIIA and TFIIB are recruited withTFIIB binding to the BRE;(1’)2. RNA Pol II recruitment: RNA Pol II-TFIIF complex is the recruited;(1’)3.TFIIE and TFIIH then bind upstream of Pol II (to form the pre-initiation complex).(1 ()如果提到了pre-initiation complex,有1 分的附加分)B.Promoter melting using energy from ATP hydrolysis by TFIIH .(2’)C.Promoter escapes after the phosphorylation of the CTD tail. (2’)Additional proteins are needed for transcription initiation in vivo:■The mediator complex (1 )■Transcriptional regulatory proteins (1 )■Nucleosome-modifying enzymes (1’)Tips:1、题目已经问了是真核中的情况,所以必须把相关的真核里的factor都回答出来,不然没分;2、B和C两点非常重要,分值也很大,由此可见把握keypoints的重要性;3、很少同学可以回答出in vivo状态下所需要的3种蛋白;4、如果可以答出另外一些细节,比如“TBP binds to and distorts DNA using a p sheet inserted into the minor groove”,会有少量加分。
differential algebras
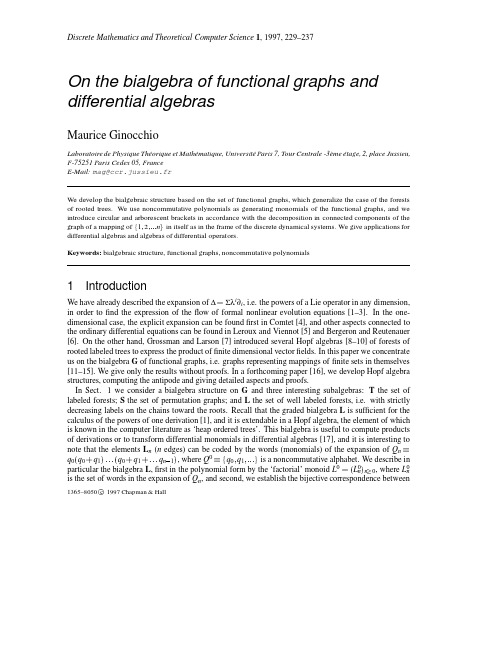
Discrete Mathematics and Theoretical Computer Science1,1997,229–237On the bialgebra of functional graphs and differential algebrasMaurice GinocchioLaboratoire de Physique Th´e orique et Math´e matique,Universit´e Paris7,Tour Centrale-3`e me´e tage,2,place Jussieu, F-75251Paris Cedex05,FranceE-Mail:mag@ccr.jussieu.fr1IntroductionWe have already described the expansion of∆Σλi∂i,i.e.the powers of a Lie operator in any dimension, in order tofind the expression of theflow of formal nonlinear evolution equations[1–3].In the one-dimensional case,the explicit expansion can be foundfirst in Comtet[4],and other aspects connected to the ordinary differential equations can be found in Leroux and Viennot[5]and Bergeron and Reutenauer [6].On the other hand,Grossman and Larson[7]introduced several Hopf algebras[8–10]of forests of rooted labeled trees to express the product offinite dimensional vectorfields.In this paper we concentrate us on the bialgebra G of functional graphs,i.e.graphs representing mappings offinite sets in themselves [11–15].We give only the results without proofs.In a forthcoming paper[16],we develop Hopf algebra structures,computing the antipode and giving detailed aspects and proofs.In Sect.1we consider a bialgebra structure on G and three interesting subalgebras:T the set of labeled forests;S the set of permutation graphs;and L the set of well labeled forests,i.e.with strictly decreasing labels on the chains toward the roots.Recall that the graded bialgebra L is sufficient for the calculus of the powers of one derivation[1],and it is extendable in a Hopf algebra,the element of which is known in the computer literature as‘heap ordered trees’.This bialgebra is useful to compute products of derivations or to transform differential monomials in differential algebras[17],and it is interesting to note that the elements L n(n edges)can be coded by the words(monomials)of the expansion of Q nq0q0q1q0q1q n1,where Q0q0q1is a noncommutative alphabet.We describe in particular the bialgebra L,first in the polynomial form by the‘factorial’monoid L0L0n n0,where L0n is the set of words in the expansion of Q n,and second,we establish the bijective correspondence between 1365–8050c1997Chapman&Hall230M.Ginocchio L and L.We show that the calculus are easier with L,and that the product on L can be expressed in a very natural way.For example,q0n Q n,hence the(exponential)generating function of all the elements of L.We describe principally the formalism in the general case G,and the calculus uses thefields F201 as well as characteristic zerofields K.In Sect.2,we describe the link with the graded differential algebra K U r0K U r and the graded algebra of differential operators K U D r0K U r D r,where U u1u2uββ1uβαα0β1is a set of indeterminates,D∂0∂1and the differential indeterminates uβασ1σp∂σ1∂σpuβαgenerate K U r[17].This shows that the above Q-calculus,which is a kind of‘dissection’on functional graphs can be used as pre-calculus in differential algebras as well as in discrete dynamical systems[18].2Bialgebra Based on the Semi-group of Functional Graphs2.1T ypes of Functional GraphsIn this paper,a connected functional graph will be called excycle[13,15].In the area of discrete dynamical systems,an excycle is known as a basin of attraction.Consider several graded andfiltered sets of labeled functional graphs(i)E(resp.G)the set of excycles(resp.functional graphs)and designated by G n(resp.G n),the set offunctional graphs having(resp.having at most)n1nodes for n0(ii)R(resp.T)the set of labeled arborescences(resp.forests).(iii)C(resp.S)the set of cycles(resp.permutation graphs).(iv)A(resp.L)the set of well labeled arborescences(resp.forests),i.e.with strictly decreasing labels on the chains toward the root(s).As in(i),we consider for(ii)–(iv)graduations andfiltrations.2.2Free Representation by Q-polynomialsLet G n be the semigroup of mappings of12n in itself(‘Semigroup of endofunctions’), Card G n n n and the subsemigroups,T n f;f G n f n f n1(i.e.f acyclic and Card T n n1n1,S n the symmetric group and Card S n n!L n f;f G n f i i(i.e.f subdiagonal and Card L n n!.We have the well known bijections F F:G n G n T n T n S n S n L n L n.Let Q q0q1be a noncommutative alphabet,Q0q0Q with q0noncommuting with the q i’s,Q n q1q2q n Q0n q0Q n and Q(resp.Q0),the corresponding free monoids.Taking F201as thefield,consider(i)the G n module F2Q n by the F2linear incidence matrix action of f G n as l f q i q f i hence l f l gl f g.On the bialgebra of functional graphs231 (ii)the generating monomial associated with f.By morphism extension,denoted again by l f,we defineQ f q f1q f2q f n l f Qιn1where Qιn q1q2q n is associated with the identityιn of G n and Qι01One again has l f l g l f g.For the following we consider(iii)The graded subsets of Q as G G n n0T T n n0S S n n0L L n n0respectively associated with G,T,S and L,with G0T0S0L01(iv)The corresponding graded F2-modules:F2G F2T F2S F2L admit components of degree n which are,respectively,G n T n S n L n modules,withdimF2G n n n dimF2T n n1n1dimF2S n dimF2L n n!(v)We will denote by R n one of the above subsemi-groups of G n(or of another category). Similarly,let R R n n0resp F2R n0F2R n be the corresponding graded subsets of Q(resp. graded F2-modules ofF2G n0F2G n.2.3Virtual Root and External ProductLet f G n I0be the set offixed points of f and H0a subset of I0,and set p q r;p r q if p q and/0otherwise.Define f0:1n0n such that f0i f i if i H0and f0i0if i H0The‘0’is the label of a virtual root added to the graph representation of f,and we will say that H0is‘confined in0’,which is a fixed point of f0We call‘extended endofunctions’such functions f0,denote by G0n0n1n their set, and we consider G n as a subset of G0n Similarly,we will have T0n T n S0n S n L0n L n Consequently, adding q0,we get the extended graded sets G0G0n n0the extended graded F2-module F2G0 n0F2G0n and their substructures F2T0F2S0F2L0Now letφG0mχ0be the characteristic function of H0φ10,and writeQφlφQιm qφ1qφ2qφmm ∏i1qφi(cf.Figures1and2).WithψG0n,consider the F2-bilinear product in F2G0defined byQφQψQψm∏i1qφi nχ0i q0q1q n12On the right-hand side we have a sum of concatened monomials,and on the right factor the substitutions q0q0q1q n and q h q h n when h0232M.Ginocchio On the other hand,the product belongs to F2G0m n This external product is associated with unit1and F2G0is‘.’graded.To see this consider i j k being0three homogeneous polynomials,A A q0;q i F2G0mB B q0;q j F2G0nC C q0;q k F2G0pthen by(2)A B B q0;q j A q0q1q n;q i n3 and so,using deg B C n p,A q0;q iB q0;q jC B q0;q j A q0q1q n;q i n CCB q0q1q p;q j p A q0q1q p q1p q n p;q i n pA q0;q i CB q0q1q p;q j pA q0;q iB q0;q j CMoreover,because T n S n L n are subsemi-groups of G n one can see that F2R0F2T0F2S0F2L0are‘.’graded subalgebras of F2G0HenceProposition1Let the sequence G0m m1of the sets of the extended endofunctions in12m and Q0q0q1be a noncommutative alphabet.ForφG0m let Qφ∏m i1qφi be the generating monomial ofφand the graded module F2-module F2G0n0F2G0n on F201generated by all the φsThen F2G0is a graded algebra for the associative product with unit1QφQψQψm∏i1qφi nχ0i q0q1q n1whereψG0n andχ0is the characteristic function ofφ10Moreover,if R0m m1is a sequence of subsets associated with subsemi-groups of the sequence G0m m1, then F2R0n0F2R0n is a graded subalgebra of F2G02.4Splitting Operatorδn F2G0This operator substitutes the n-coproduct∆n of the Leibniz–Lie type.Associate to A Q0the left linear operatorτn A acting on B Q0,such that,if A G0m B G0n,then Bτn A BA if degB n,and0 otherwise,where BA is the concatenation of B and A.(i)Now let f G m and H0as in Sect.3,and notefirst that ifτn is viewed as acting on f,then for i1m one hasτn f i n f i n,and by f0i ¯χ0i f i one hasτn f0i n¯χ0i f0i n,where¯χ01χ0According to(2),define forφG0mδn Qφτnm∏i1qφi nχ0i q0q1q n14If d0Card H0the expansion(4)gives a sum of n1d0generating monomials of functionsψκof n1n m into0n1n m,and the corresponding functional graphs factorized in commutative excycles.On the bialgebra of functional graphs233 The operatorδn A is left linear on F2G0,and(2)can be writtenQφQψQψδn Qφ5 (ii)Moreover,δp is a graded antimorphism for‘’δp A Bδp Bδp n A6 where n degB and p N.For this to compute with(5)and A B C as in Sect.3,Cδp A B A B C A B C B Cδp n A Cδp Bδp n A.If p0we recover A B Bδn A and Bδk A0if k degB(iii)Also,δn is a powerδnδnδδ1δ017 For this to compute,δpδn A q0;q iδpτn A q0q1q n;q i nτn p A q0q1q p q1pq n p;q i n pδn p A q0;q i.(iv)Define the left linear operatorµin F2G0by the expansionµ∑n0δn8By left linear action ofµA on F2G0,we get A B BµA for A B F2G0with the antimorphism propertyµA BµBµA9 which express the associativity of‘’.Proposition2Let A F2G0m B F2G0n Then the splitting linear operatorδp defined left linearly by Bδp A A B if p=n,and0otherwise,verifiesδpδp withδδ1δ01andδp A Bδp Bδp n A Moreover,µ∑n0δn is an antimorphism in F2G0such that A B BµA2.5Exponential Generating Function of the Monomials of L0All the words of L0n(i.e.subdiagonals)are obtained from the expansion of Q n q0q0q1q0q1 q n1F2L0and Q01By equation(3),one has Q m Q n Q m n,and if A F2L0m B F2L0n we have A B F2L0m n,and then we recover that F2L0is stable for the product‘’.Because Q1q0,the associativity givesQ n q0n10 With the Q[[t]]-modules on L0,one has the exponential generating functionexp tq0∑n0t nn!Q n11exp sq0exp tq0exp s t q0234M.Ginocchio2.6ExamplesConsider equations (4)and (5)for Q ψq n 0.2.6.1Rooted T rees with n=1δq 20q 1τq 0q 12q 2τq 0q 0q 2τq 0q 1q 2τq 1q 0q 2τq 1q 1q 2(Figure 3)q 20q 1q 0q 0q 0q 12q 2q 30q 2q 20q 1q 2q 0q 1q 0q 2q 0q 1q 1q 2(Figure 4)2.6.2Excycles with n=2δ2q 23q 1q 0τ2q 25q 3q 0q 1q 2τ2q 25q 3q 0τ2q 25q 3q 1τ2q 25q 3q 2(Figure 5)q 23q 1q 0q 20q 20q 25q 3q 0q 1q 2q 20q 25q 3q 0q 20q 25q 3q 1q 20q 25q 3q 2(Figure 6)3Differential Algebra3.1Differential indeterminatesLet D ∂0∂1where ∂α∂∂ξαthe αth canonical derivation in S K ξthe algebra of formal power series in ξξ0ξ1,where K is a characteristic zero field.If S N N is the set U u 1u 2u ββ1u αβα0β1with u αβS consider U as a set of indeterminates,u αβσ1σp ∂σ1∂σp u αβasdifferential indeterminates,replace S N N by KU ,and consider the graded differential algebra K Ur 0K U r and the graded algebra of differential operators K U D r 0K U r D r.To each W F 2R 0we associate the differential operator W U U D ;for example,with W r U K U r one hasW UW 0UW 1Uα∂αW 2Uαβ∂α∂βW 0U∑r 1W r U D r12We will use now the summation convention.3.2Brackets in K UDefine for u v wU the multilinear operations valued in K U .3.2.1Arborescent Brackets (Valued in K U 1)u v uv w u vβu αv βα,henceu v Du αv βα∂β(1fixed point sent to ‘0’)uv wγu αv βw γαβ,henceu v Du αv βw γαβ∂γ13Also,for AK UrBK UsA Bβ1βsA α1αr B β1βsα1αr3.2.2Circular Brackets (Valued in K U 0)uu αα(1fixed point),u vu ααv ββ(2fixed points)u vu αβv βα2cycleu v wu αγv βαw γβ3cycle14On the bialgebra of functional graphs 2353.2.3Mixed Brackets (Valued in K U 0)Let E be a proper excycle (i.e.with no fixed point);we can write it EA i 1A i 2A i p ,where the A i k ’s are arborescences with root i k If in each arborescence A i k is reduced to its root i k ,we recover simply acycle Ei 1i 2i p Now let F k be the forest under i k ,i.e.obtained by cutting the root of A i k ,and defined with F i k U F i k u j ;j N i k ,where N i k is the set of nodes of F i k :E Uu F i 1i 1u F i 2i 2u F i pi pF i 1U u i 1α1αp F i 2U u i 2α2α1F i p U u i p αp αp13.3Action of F 2R 0Moreover,F 2R 0operates K -linearly in K U with values in K U D .For this let φG 0m H 0φ1for j 0m I 1m ,and H u β1u β2u βm U ,a word on U of length m .Then the action isQ φ∏i Iq φiQ φH∏j I∏i H j∂αiu αj βj∏k H 0∂αk15The differential monomial Q φH is such that u βj is associated with j in the domain I of φIf d j is the degree in q j (in-degree of the node labeled by ‘j ’),then u αj βj is derived d j times and the indices of derivation are related to the places of the q j ’s in the word.Similarly,the differential operator D r is characterized by the number r (degree of the root)of the q 0’s and their places.So we can summarize:In a word A R 0where q j is at the place (i),then in A H the j th letter of H is derived according to i,i.e.∂αi acts.One has,in particular,taking H u 1u 2:Arborescent brackets 1U 1q 0U u 1α1∂α1u 1Dq 0q 0U u 1α1u 2α2∂α1∂α2u 1u 2D 2q 0q 1U u 1α1α2u 2α2∂α1u 2u 1D q 3q 3q 0U u 1α1u 2α2u 3α3α1α2∂α3u 1u 2u 3Dq 0q 0q 2q 2U u 1α1u 2α2α3α4u α33u α44∂α1∂α2u 1u 3u 4u 2D 2Circular brackets q 1U u 1α1α1u 1q 1q 2U u 1α1α1u 2α2α2u 1u 2q 2q 1U u 1α1α2u 2α2α1u 1u 2q 3q 1q 2U u 1α1α3u 2α2α1u 3α3α2u 1u 2u 33.4Product of Differential OperatorsThe product (2)on words with correspondence (15)gives the product of differential operators.We state,without proof,Proposition 3Let the graded differential algebra K U r 0K U r and the graded algebra of differ-ential operators K U D r 0K U r D r Let φG 0m I 1m H j φ1j for j 0m and H u β1u β2u βm a word on U of length m.Then the mapping of F 2G 0into K U D which associates to the generating monomial Q φ∏i I q φi of φthe differential operator Q φH ∏j I ∏i H j ∂αi u αj βj ∏k H 0∂αk236M.Ginocchio is a morphism,such that ifψG0n and K is a word on U of length n,one has QφH QψK QφQψKH, where KH is the concatenation of K and H.ExampleA q0B q2q1q0H u4K u1u2u3A B q2q1q0q0q1q2q3q2q1q0q0q2q1q0q1q2q1q0q2q2q1q0q3(Figure7)A H u1DB K u1u2u3DA HB K u1u2u3u4D2u4u1u2u3D u1u2u4u3DObserve that:u4u1u2u3D u1α1α2α4u2α2α1u3α3u4α4∂α3u1α1α2u2α2α1α4u3α3u4α4∂α3which corresponds to q2q1q0q1q2,i.e.the second and third terms in the graph expansion. AppendixTo view Figures1–7,click here.To return to the main paper,click on the red box.References[1]Ginocchio,M.(1995).Universal expansion of the powers of a derivation,Letters in Math.Phys.34(4),343–364.[2]Ginocchio,M.and Irac-Astaud,M.(1985).A recursive linearization process for evolution equations.Reports on Math.Phys.21,245–265.[3]Steeb,W.H.and Euler,N.(1988).Nonlinear Evolution Equations and Painlev´e Test.World Scien-tific.[4]Comtet,L.(1973).Une formule explicite pour les puissances successives de l’op´e rateur de d´e rivationde m.Roy.Acad.Sci.276A,165–168.[5]Leroux,P.and Viennot,G.(1986).Combinatorial resolution of systems of differential equations I:ordinary differential equations.Actes du colloque de combinatoire´e num´e rative,Montr´e al.Lecture Notes in Mathematics1234,pp.210–245.Springer-V erlag.[6]Bergeron, F.and Reutenauer, C.(1987).Une interpr´e tation combinatoire des puissances d’unop´e rateur diff´e rentiel lin´e aire.Ann.Sci.Math.Quebec11,269–278.[7]Grossman,R.and Larson,R.G.(1989).Hopf-algebraic structures of families of trees.J.Algebra126,184–210.[8]Joni,A.A.and Rota,G.C.(1979).Coalgebras and bialgebras in combinatorics.Studies.in Appl.Math.61,93–139.On the bialgebra of functional graphs237 [9]Nichols,W.and Sweedler,M.E.(1980).Hopf algebras and combinatorics,in‘Umbral calculus andHopf algebras’.Contemp.Math.6.[10]Sweedler,M.E.(1969).Hopf Algebras.Benjamin.[11]Berge,C.(1983).Graphes.Gauthier-Villars.[12]Comtet,L.(1974).Advanced Combinatorics.Reidel.[13]Denes,J.(1968).On transformations,transformation-semigroups and graphs.In Erd¨o s-Katona,ed-itor,Theory of Graphs.Academic Press,pp.65–75.[14]Foata,D.and Fuchs,A.(1970).R´e arrangements de fonctions et d´e b.Theory8,361–375.[15]Harary,F.(1959).The number of functional digraphs.Math.Annalen138,203–210.[16]Ginocchio,M.On the Hopf algebra of functional graphs and differential algebras.Discr.Math.Toappear.[17]Kaplansky,I.(1976).Introduction to Differential Algebras.Springer-V erlag.[18]Robert,F.(1995).Les syst`e mes Dynamiques Discrets.Springer-V erlag.。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
Free differential Rota-Baxter Lie algebras andGr¨o bner-Shirshov bases∗Jianjun QiuSchool of Mathematics and Statistics,Lingnan Normal UniversityZhanjiang524048,P.R.Chinajianjunqiu@Yuqun Chen†School of Mathematical Sciences,South China Normal UniversityGuangzhou510631,P.R.Chinayqchen@Abstract:We establish the Gr¨o bner-Shirshov bases theory for differential Lie Ω-algebras.As an application,we give a linear basis of a free differential Rota-Baxter Lie algebra on a set.Key words:Gr¨o bner-Shirshov basis,Lyndon-Shirshov word,differential Rota-Baxter Lie algebraAMS2000Subject Classification:16S15,13P10,16W99,17A501IntroductionLet k be afield andλ∈k.A differential algebra of weightλor aλ-differential algebra([19,23,29])is a k-algebra(R,·)together with a differential operator (of weightλ)D:R→R satisfyingD(x·y)=D(x)·y+x·D(y)+λD(x)·D(y),x,y∈R.The differential algebras werefirst studied by J.F.Ritt[29]and have developed to be an important branch of mathematics in both theory and applications(see for instance[15,19,33]).A Rota-Baxter algebra of weightλorλ-Rota-Baxter algebra([4,22,30])is a k-algebra(R,·)together with a Rota-Baxter operator(of weightλ)P:R→R satisfyingP(x)·P(y)=P(x·P(y))+P(P(x)·y)+λP(x·y),x,y∈R.∗Supported by the NNSF of China(11171118,11571121)and the NSF of Guangdong (2015A030310502).†Corresponding author.1The Rota-Baxter operator on an associative algebra initially appeared in proba-bility[4]and then in combinatorics[30]and quantumfield theory[14].There are a number of studies on Rota-Baxter associative algebras on both commutative and noncommutative case.For more details we refer the reader to[22]and the references given there.The Rota-Baxter operator of weight0on a Lie algebra is also called the operator form of the classical Yang-Baxter equation[31].The Rota-Baxter Lie algebras are closely related with the pre-Lie algebras.Recently, there are many results on Rota-Baxter Lie algebras and related topics(see for instance[2,3,21,26,28]).Similarly to the relation between differential operator and integral operator as in the First Fundamental Theorem of Calculus,L.Guo and W.Keigher[23] introduced the notion of differential Rota-Baxter algebra which is a k-algebra R together with a differential operator D and a Rota-Baxter operator P such that DP=Id R.As we known,the free objects of various varieties of linear algebras play an important role.Sometimes,it is difficult to give a linear basis of a free algebra,for example,it is an open problem tofind a linear basis of a free Jordan algebra.A linear basis of the free differential Rota-Baxter(mutative and associative)associative algebra on a set was given by L.Guo and W.Keigher[23].In this paper,we apply the Gr¨o bner-Shirshov bases method to constructa free differential Rota-Baxter Lie algebras.Especially,we give a linear basis of a free differential Rota-Baxter Lie algebra on a set.Gr¨o bner bases and Gr¨o bner-Shirshov bases have been proved to be very useful in different branches of mathematics,which were invented independently by A.I.Shirshov[32],H.Hironaka[24]and B.Buchberger[13]on different types of algebras.For more details on the Gr¨o bner-Shirshov bases and their applications,see for instance the surveys[8,10],the books[1,11,16,18]and the papers[9,17,20,27,28].TheΩ-algebra was introduced by A.G.Kurosh[25].A differential LieΩ-algebra over afield k is a differential Lie algebra L with a set of multilinear operatorsΩon L.It is easy to see that a differential Rota-Baxter Lie algebra is a differential LieΩ-algebra with a single operator satisfying the Rota-Baxter relation.The paper is organized as follows.In Section2,we review the Gr¨o bner-Shirshov bases theory for differential associativeΩ-algebras.In Section3,we firstly construct a free differential LieΩ-algebra by the differential nonassocia-tive Lyndon-ShirshovΩ-words,which is a generalization of the classical nonas-sociative Lyndon-Shirshov words.Secondly,we establish the Gr¨o bner-Shirshov bases theory for differential LieΩ-algebras.As an immediate application,we prove that the word problem of any one relation differential LieΩ-algebra is solv-able.In Section4,we obtain a Gr¨o bner-Shirshov basis of a freeλ-differential Rota-Baxter Lie algebra and then a linear basis of such an algebra is obtained by the Composition-Diamond lemma for differential LieΩ-algebras.22Gr¨o bner-Shirshov bases forλ-differential as-sociativeΩ-algebrasIn this section,we briefly review the Gr¨o bner-Shirshov bases theory forλ-differential associativeΩ-algebras,which can be found in[27].2.1Freeλ-differential associativeΩ-algebrasLet D be a1-ary operator andΩ:=∞m=1Ωm,whereΩm is a set of m-ary operators for any m≥1.For any set Y,we define the following notations:S(Y):the set of all nonempty associative words on Y.Y∗:the set of all associative words on Y including the empty word1.Y∗∗:the set of all nonassociative words on Y.∆(Y):= ∞m=0{D m(y)|y∈Y},where D0(y)=y,y∈Y.Ω(Y):= ∞m=1ω(m)(y1,y2,···,y m)|y i∈Y,1≤i≤m,ω(m)∈Ωm.Let X be a set.Define the differential associative and nonassociativeΩ-words on X as follows.For n=0,define D,Ω;X 0=S(∆(X)),(D,Ω;X)0= (∆(X))∗∗.For n>0,defineD,Ω;X n=S(∆(X∪Ω( D,Ω;X n−1))),(D,Ω;X)n=(∆(X∪Ω((D,Ω;X)n−1)))∗∗.SetD,Ω;X =∞n=0D,Ω;X n,(D,Ω;X)=∞n=0(D,Ω;X)n.The elements of D,Ω;X (resp.(D,Ω;X))are called differential associative (resp.nonassociative)Ω-words on X.A differential associativeΩ-word u is called prime if u∈∆(X∪Ω( D,Ω;X )).Let k be afield andλ∈k.Aλ-differential associativeΩ-algebra over k is a λ-differential associative k-algebra R together with a set of multilinear operators Ωon R.Let DA Ω;X =k D,Ω;X be the semigroup algebra of D,Ω;X .Let u=u1u2···u t∈ D,Ω;X ,where each u i is prime.If t=1,i.e.u=D i(u )for some i≥0,u ∈X∪Ω( D,Ω;X ),then we define D(u)=D i+1(u ).If t>1, then we recursively defineD(u)=D(u1)(u2···u t)+u1D(u2···u t)+λD(u1)D(u2···u t).3Extend linearly D to DA Ω;X .For anyω(m)∈Ωm,defineω(m): D,Ω;X m→ D,Ω;X ,(u1,u2,···,u m)→ω(m)(u1,u2,···,u m) and extend it linearly to DA Ω;X m.Theorem2.1([27])(DA Ω;X ,D,Ω)is a freeλ-differential associativeΩ-algebra on the set X.2.2Composition-Diamond lemma forλ-differential asso-ciativeΩ-algebrasLet is a symbol,which is not in X.By a differential -Ω-word we mean any expression in D,Ω;X∪{ } with only one occurrence of .The set of all the differential -Ω-words on X is denoted by D,Ω;X .Letπbe a differential -Ω-word and s∈DA Ω;X .Then we callπ|s=π| →s a differential s-word.Let deg(u)be the number of all occurrences of x∈X,ω∈Ωand D in u.If u=u1u2···u m,where u i is prime,then the breath of u,denoted by bre(u),is defined to be the number m.Definewt(u)=(deg(u),bre(u),u1,u2,···,u m).Let X andΩbe well-ordered sets and assume thatω>D for anyω∈Ω.Wedefine the Deg-lex order>Dl on D,Ω;X as follows.For any u=u1u2···u nand v=v1v2···v m∈ D,Ω;X ,where u i,v j are prime,defineu>Dlv if wt(u)>wt(v)lexicographically,where if u i=ω(u i1,u i2,···,u it),v i=θ(v i1,v i2,···,v il),ω,θ∈{D}∪Ωand deg(u i)=deg(v i),then u i>Dlv i if(ω,u i1,u i2,···,u it)>(θ,v i1,v i2,···,v il)lexicographically.It is easy to check that>Dl is a well order on D,Ω;X .For any0=f∈DA Ω;X ,let¯f be the leading term of f with respect to the order>Dl .Let usdenote lc(f)the coefficient of the leading term¯f of f.For1≤t≤n,defineI t n={(i1,i2,···,i n)∈{0,1}n|i1+i2+···+i n=t}.Lemma2.2([27])If u=u1u2···u n∈ D,Ω;X ,where each u i is prime, thenD(u)=(i1,i2,···,i n)∈I1nD i1(u1)D i2(u2)···D i n(u n)+nt=2(i1,i2,···,i n)∈I tnλt−1D i1(u1)D i2(u2)···D i n(u n).4Lemma 2.3([27])Let u =u 1u 2···u n ∈ D,Ω;X ,where each u i is prime.(a)If λ=0,then D i (u )=D i (u 1)u 2···u n and lc (D i (u ))=1.(b)If λ=0,then i =D i (u 1)D i (u 2)···D i (u n )and lc (D i (u ))=λ(n −1)i .It follows that if u,v ∈ D,Ω;X and u >Dl v ,then D (u )>D (v ).Proposition 2.4([27])For any u,v ∈ D,Ω;X ,π∈ D,Ω;X ,if u >Dl v ,then u >Dl v If s =π|s ,where s ∈DA Ω;X and π∈ D,Ω;X ,then π|s is called a normal differential s -word.Note that not each differential s -word is a normal differential s -word,for example,if u =D (x )P (D 2( ))and s =xy ,where P ∈Ω,x,y ∈X ,then π|s is not a normal differential s -word.However,if we take π =D (x 1)P ( ),then π|s =π |D 2(s )and π |D 2(s )is a normal differential D 2(s )-word.Lemma 2.5([27])For any differential s -word π|s ,there exist i ≥0and π such that π|s =π |D i (s )and π |D i (s )is a normal differential D i (s )-word.Let f,g ∈DA Ω;X .There are two kinds of compositions.(i)If there exists a w =D i (f )a =bD j (g )for some a,b ∈ D,Ω;X such that bre (w )<bre (¯f )+bre (¯g ),then we call(f,g )w =lc (D i (f ))−1D i (f )a −lc (D j (g ))−1bD j (g )the intersection composition of f and g with respect to the ambiguity w .(ii)If there exists a π∈ D,Ω;X such that w =i =π|D j (g ),where π|D j (g )is a normal differential D j(g )-word,then we call(f,g )w =lc (D i (f ))−1D i (f )−lc (D j (g ))−1π|D j (g )the inclusion composition of f and g with respect to the ambiguity w .Let S be a subset of DA Ω;X .Then the composition (f,g )w is called trivial modulo (S,w )if (f,g )w = αi πi |D l i (s i),where each αi ∈k ,πi ∈ D,Ω;X ,s i ∈S ,πi |D l i (si )is a normal differentialD l i (s i )-word and πi |D l i (s i )<Dl w .If this is the case,we write(f,g )w ≡ass 0mod (S,w ).In general,for any two polynomials p and q ,p ≡ass qmod (S,w )means that p −q = αi πi |D l i (s i),where each αi ∈k ,πi ∈ D,Ω;X ,s i ∈S ,πi |D l i (s i)is anormal differential D l i (s i )-word and πi |D l i (s i )<Dl w .A set S ⊂DA Ω;X is called a Gr¨o bner-Shirshov basis in DA Ω;X if any composition (f,g )w of f,g ∈S is trivial modulo (S,w ).5Theorem2.6([27],Composition-Diamond lemma for differential associative Ω-algebras)Let S be a subset of DA Ω;X ,Id DA(S)the ideal of DA Ω;Xgenerated by S and>Dl the Deg-lex order on D,Ω;X defined as before.Thenthe following statements are equivalent:(i)S is a Gr¨o bner-Shirshov basis in DA Ω;X .(ii)f∈Id DA(S)⇒¯f=π|D i(s)for someπ∈ D,Ω;X ,s∈S and i≥0. (iii)The setIrr(S)=w∈ D,Ω;Xw=π|D i(s),s∈S,i≥0,π|D i(s)isa normal differential D i(s)-wordis a linear basis of the differential associativeΩ-algebra DA Ω;X|S := DA Ω;X /Id DA(S).3Gr¨o bner-Shirshov bases forλ-differential Lie Ω-algebras3.1Lyndon-Shirshov wordsIn this subsection,we review the concept and some properties of Lyndon-Shirshov words,which can be found in[7,32].For any u∈X∗,let us denote by deg(u)the degree(length)of u.Let>be a well order on X.Define the lex-order>lex and the deg-lex order>deg−lex on X∗with respect to>by:(i)1>lex u for any nonempty word u,and if u=x i u and v=x j v ,where x i,x j∈X,then u>lex v if x i>x j,or x i=x j and u >lex v by induction.(ii)u>deg−lex v if deg(u)>deg(v),or deg(u)=deg(v)and u>lex v.A nonempty associative word w is called an associative Lyndon-Shirshov word on X,if w=uv>lex vu for any decomposition of w=uv,where1= u,v∈X∗.A nonassociative word(u)∈X∗∗is said to be a nonassociative Lyndon-Shirshov word on X with respect to the lex-order>lex,if(a)u is an associative Lyndon-Shirshov word on X;(b)if(u)=((v)(w)),then both(v)and(w)are nonassociative Lyndon-Shirshov words on X;(c)if(v)=((v1)(v2)),then v2≤lex w.Let ALSW(X)(resp.NLSW(X))denote the set of all the associative (resp.nonassociative)Lyndon-Shirshov words on X with respect to the lex-order>lex.It is well known that for any u∈ALSW(X),there exists a unique6Shirshov standard bracketing way[u](see for instance[7])on u such that[u]∈NLSW(X).Then NLSW(X)={[u]|u∈ALSW(X)}.Let k X be the free associative algebra on X over afield k and Lie(X)be the Lie subalgebra of k X generated by X under the Lie bracket(uv)=uv−vu. It is well known that Lie(X)is a free Lie algebra on the set X and NLSW(X) is a linear basis of Lie(X).3.2Differential Lyndon-ShirshovΩ-wordsLet>Dl be the Deg-lex order on D,Ω;X and the restriction of>Dlon∆(X∪Ω( D,Ω;X )).Define the differential Lyndon-ShirshovΩ-words on the set X as follows.For n=0,let Z0:=∆(X).DefineALSW(D,Ω;X)0:=ALSW(Z0),NLSW(D,Ω;X)0:=NLSW(Z0)={[u]|u∈ALSW(D,Ω;X)0}with respect to the lex-order lex on(Z0)∗,where[u]is the Shirshov standard bracketing way on u.Assume that we have definedALSW(D,Ω;X)n−1,NLSW(D,Ω;X)n−1:={[u]|u∈ALSW(D,Ω;X)n−1}.Let Z n:=∆(X∪Ω(ALSW(D,Ω;X)n−1)).DefineALSW(D,Ω;X)n:=ALSW(Z n).with respect to the lex-order lex on Z∗n.For any u∈Z n,define the bracketing way on u by[u]:=u,if u=D i(x),x∈X,D i(ω(m)([u1],[u2],···,[u m])),if u=D i(ω(m)(u1,u2,···,u m)).Let[Z n]:={[u]|u∈Z n}.Thus,the order on Z n induces an order on[Z n]by [u] [v]if u v for any u,v∈Z n.For any u=u1u2···u t∈ALSW(D,Ω;X)n, where each u i∈Z n,we define[u]:=[[u1][u2]···[u t]]the Shirshov standard bracketing way on the word[u1][u2]···[u t],which means that[u]is a nonassociative Lyndon-Shirshov word on the set{[u1],[u2],···,[u t]}. DefineNLSW(D,Ω;X)n:={[u]|u∈ALSW(D,Ω;X)n}.It is easy to see that NLSW(D,Ω;X)n=NLSW([Z n])with respect to the lex-order lex on[Z n]∗.7SetALSW(D,Ω;X):=∞n=0ALSW(D,Ω;X)n,NLSW(D,Ω;X):=∞n=0NLSW(D,Ω;X)n.Then,we haveNLSW(D,Ω;X)={[u]|u∈ALSW(D,Ω;X)}.The elements of ALSW(D,Ω;X)(resp.NLSW(D,Ω;X))are called the dif-ferential associative(resp.nonassociative)Lyndon-ShirshovΩ-words on the set X.3.3Freeλ-differential LieΩ-algebrasIn this subsection,we prove that the set NLSW(D,Ω;X)of all differential nonassociative Lyndon-ShirshovΩ-words on X forms a linear basis of the free λ-differential LieΩ-algebra on X.Aλ-differential Lie algebra is a Lie algebra L with a linear operator D:L→L satisfying the differential relationD([xy])=[D(x)y]+[xD(y)]+λ[D(x)D(y)],x,y∈L.Aλ-differential LieΩ-algebra is aλ-differential Lie algebra L with a set of multilinear operatorsΩon L.Let(R,·,D,Ω)is aλ-differential associativeΩ-algebra.Then it is easy to check that(R,[,],D,Ω)is aλ-differential LieΩ-algebra under the Lie bracket [a,a ]=a·a −a ·a,a,a ∈R.Let DLie(Ω;X)be theλ-differential LieΩ-subalgebra of DA Ω;X gener-ated by X under the Lie bracket(uv)=uv−vu.Similar to the proofs of Lemma2.6and Theorem2.8in[28],we have the following results.Lemma3.1If u∈ALSW(D,Ω;X),then[u]=u with respect to the order >Dlon D,Ω;X .Theorem3.2DLie(Ω;X)is a freeλ-differential LieΩ-algebra on the set X and NLSW(D,Ω;X)is a linear basis of DLie(Ω;X).3.4Composition-Diamond lemma forλ-differential LieΩ-algebrasIn this subsection,we establish the Composition-Diamond lemma for differential LieΩ-algebras.8Lemma3.3Letπ∈ D,Ω;X and v,π|v∈ALSW(D,Ω;X).Then there is aπ ∈ D,Ω;X and c∈ D,Ω;X such that[π|v]=[π |[vc]],where c may be empty.Let[π|v]v=[π |[vc]]|[vc]→[···[[[v][c1]][c2]]···[c m]]where c=c1c2···c m with each c i∈ALSW(D,Ω;X)and c t lex c t+1.Then,[π|v]v=π|[v]+αiπi|[v],where eachαi∈k andπi|v<Dl π|v.It follows that[π|v]v=π|v with respect tothe order>Dl.Proof.The proof is the same as the one of Lemma3.2in[28].Let0=f∈DLie(Ω;X)⊆DA Ω;X .Ifπ|¯f∈ALSW(D,Ω;X),then we call[π|f]¯f=[π|¯f]¯f|[¯f]→fa special normal differential f-word.Corollary3.4Let f∈DLie(Ω;X)andπ|¯f∈ALSW(D,Ω;X).Then[π|f]¯f=π|f+αiπi|f,where eachαi∈k andπi|¯f<Dlπ|¯f.Let f,g∈DLie(Ω;X).There are two kinds of compositions.(i)If there exists a w=D i(f)a=bD j(g)for some a,b∈ D,Ω;X such thatbre(w)<bre(¯f)+bre(¯g),then we callf,g w=lc(D i(f))−1[D i(f)a]D i(f)−lc(D j(g))−1[bD j(g)]D j(g)the intersection composition of f and g with respect to the ambiguity w.(ii)If there exists aπ∈ D,Ω;X such that w=D i(f)=π|D j(g),whereπ|D j(g)is a normal differential D j(g)-word,then we callf,g w=lc(D i(f))−1D i(f)−lc(D j(g))−1[π|Dj(g)]Dj(g)the inclusion composition of f and g with respect to the ambiguity w.9If S is a subset of DLie (Ω;X ),then the composition f,g w is called trivial modulo (S,w )if f,g w = αi [πi |D l i (s i )]D l i (s i ),where each αi ∈k,s i ∈S ,[πi |D l i (s i)]D i (s i )is a special normal differentialD l i (s i )-word and πi |D l i (s i )<Dl w .If this is the case,then we writef,g w ≡0mod (S,w ).In general,for any two polynomials p and q ,p ≡q mod (S,w )meansthat p −q = αi [πi |D l i (s i)]D l i (s i ),where each αi ∈k ,πi ∈ D,Ω;X ,s i ∈S ,[πi |D l i (s i )]D l i (s i )is a normal differential D l i (s i )-word and πi |D l i (s i )<Dl w .Definition 3.5A set S ⊂DLie (Ω;X )is called a Gr¨o bner-Shirshov basis in DLie (Ω;X )if any composition f,g w of f,g ∈S is trivial modulo (S,w ).Lemma 3.6Let f,g ∈DLie (Ω;X ).Thenf,g w −(f,g )w ≡ass 0mod ({f,g },w ).Proof.If f,g w and (f,g )w are compositions of intersection,where w =i =j thenf,g w=lc (D i (f ))−1[D i (f )a ]D i (f )−lc (D j (g ))−1[bD j (g )]D j (g )=lc (D i (f ))−1D i (f )b + αi a i D i (f )a i −lc (D j (g ))−1bD j (g )− βj b j D j (g )b j=(f,g )w + αi a i D i (f )a i − βj b j D j (g )b j ,where a i D i (f )a i ,b j D j (g )b j <Dl w .It follows that f,g w −(f,g )w ≡ass 0mod ({f,g },w ).If f,g w and (f,g )w are compositions of inclusion,where w =¯f =π|D j (g ),thenf,g w =f −lc (D j (g ))−1[π|D j (g )]D j (g )=f −lc (D j (g ))−1π|D j (g )− αi πi |D j (g ),where πi |D j (g )<Dl w .It follows thatf,g w −(f,g )w ≡ass 0mod ({f,g },w ).The proof is complete. Lemma 3.7Let S ⊂DLie (Ω;X )⊂DA (Ω;X ).Then the following two state-ments are equivalent:10(i)S is a Gr¨o bner-Shirshov basis in DLie(Ω;X),(ii)S is a Gr¨o bner-Shirshov basis in DA Ω;X .Proof.(i)=⇒(ii).Suppose that S is a Gr¨o bner-Shirshov basis in DLie(Ω;X). Then,for any composition f,g w,we havef,g w=αi[πi|D l i(s i)]D l i(s i),where eachαi∈k,s i∈S,πi|D i(s i)<Dlw.By Corollary3.4,we havef,g w=βtπt|D l t(s t),where eachβt∈k,s t∈S,πt|D l t(s t)<Dlw.Therefore,by Lemma3.6,we canobtain that(f,g)w≡ass0mod(S,w).Thus,S is a Gr¨o bner-Shirshov basis in DA Ω;X .(ii)=⇒(i).Assume that S is a Gr¨o bner-Shirshov basis in DA Ω;X .Then, for any composition f,g w in S,we have f,g w∈DLie(Ω;X)and f,g w∈Id DA(S).By Theorem2.6, f,g w=π1|D i1(s1)∈ALSW(D,Ω;X).Leth1= f,g w−α1[π1|D i1(s1)]D i1(s1),whereα1is the coefficient of w Then,h1<Dl w,h1∈Id DA(S)andh1∈DLie(Ω;X).Now,the result follows from induction on f,g w.Lemma3.8Let S⊂DLie(Ω;X)andIrr(S)={[w]|w∈ALSW(D,Ω;X),w=π|D i(s),s∈S,π∈ D,Ω;X ,i≥0}. Then,for any h∈DLie(Ω;X),h can be expressed byh=αi[u i]+βj[πj|Dl j(s j)]Dl j(s j),where eachαi,βj∈k,u i∈ALSW(D,Ω;X),u i≤Dl¯h and s j∈S,πj|D l j(s j)≤Dl¯h.Proof.By induction on h,we can obtain the result.The following theorem is the Composition-Diamond lemma for differential LieΩ-algebras.It is a generalization of Shirshov’s Composition lemma for Lie algebras[32],which was specialized to associative algebras by L.A.Bokut[6], see also G.M.Bergman[5]and B.Buchberger[12,13].11Theorem 3.9(Composition-Diamond lemma for differential Lie Ω-algebras)Let S ⊂DLie (Ω;X )be a nonempty set and Id DLie (S )the ideal of DLie (Ω;X )generated by S .Then the following statements are equivalent:(I)S is a Gr¨o bner-Shirshov basis in DLie (Ω;X ).(II)f ∈Id DLie (S )⇒¯f =π|D i (s )∈ALSW (D,Ω;X )for some s ∈S ,π∈ D,Ω;X and i ≥0.(III)The setIrr (S )={[w ]|w ∈ALSW (D,Ω;X ),w =π|D i (s ),s ∈S,π∈ D,Ω;X ,i ≥0}is a linear basis of the λ-differential Lie Ω-algebras DLie (Ω;X |S ).Proof.(I )=⇒(II ).Since f ∈Id DLie (S )⊆Id DA (S ),by Lemma 3.7and Theorem 2.6,we have ¯f =π|D i (s )for some s ∈S ,π∈ D,Ω;X and i ≥0.(II )=⇒(III ).Suppose that αi [u i ]=0in DLie (Ω;X |S ),where each[u i ]∈Irr (S )and u i >Dl u i +1.That is, αi [u i]∈Id DLie (S ).Then each αi must be 0.Otherwise,say α1=0,sincei i =u 1and by (II),we have [u 1]∈Irr (S ),a contradiction.Therefore,Irr (S )is linear independent.By Lemma 3.8,Irr (S )is a linear basis of DLie (Ω;X |S )=DLie (Ω;X )/Id DLie (S ).(III )=⇒(I ).For any composition f,g w with f,g ∈S ,we have f,g w ∈Id DLie (S ).Then,by (III)and by Lemma 3.8, f,g w = βj [π|D l j (s j )]D j (s j)where each βj ∈k,π|D l j (s j )<Dl w .This proves that S is a Gr¨o bner-Shirshovbasis in DLie (Ω;X ).As an immediate application of Theorem 3.9,similar to the Lie algebra,if S ={f },f ∈DLie (Ω;X ),is a one relation subset of DLie (Ω;X ),then S is a Gr¨o bner-Shirshov basis in DLie (Ω;X ).It follows that the word problem of any one relation differential Lie Ω-algebra is solvable.4Free λ-differential Rota-Baxter Lie algebras In this section,by using Theorem 3.9we give a Gr¨o bner-Shirshov basis of a free λ-differential Rota-Baxter Lie algebra on a set X and then a linear basis of such an algebra is obtained.4.1Gr¨o bner-Shirshov bases for free λ-differential Rota-Baxter Lie algebrasLet k be a field and λ∈k .A differential Rota-Baxter Lie algebra of weight λ,called also λ-differential Rota-Baxter Lie algebra,is a Lie algebra L with two linear operators P,D :L →L such that for any x,y ∈L ,12(a)(Rota-Baxter relation)[P (x )P (y )]=P ([xP (y )])+P ([P (x )y ])+λP ([xy ]);(b)(differential relation)D ([xy ])=[D (x )y ]+[xD (y )]+λ[D (x )D (y )];(c)(section relation)D (P (x ))=x .It is easy to see that any λ-differential Rota-Baxter Lie algebra is a λ-differential Lie {P }-algebra satisfying the relations (a)and (c).Let DLie ({P };X )be the free λ-differential Lie {P }-algebra on the set X and writeg (u ):=D (P ([u ]))−[u ],f (u,v ):=[P ([u ])P ([v ])]−P (([u ]P ([v ])))−P ((P ([u ])[v ]))−λP ([u ][v ]),u >Dl v,where u,v ∈ALSW (D,{P };X ).SetS ={f (u,v ),g (w )|u,v,w ∈ALSW (D,{P };X ),u >Dl v }.It is clear that DRBL (X ):=DLie ({P };X |S )is a free λ-differential Rota-Baxter Lie algebra on X .For any f ∈DLie ({P };X ),let us denote r (f ):=f −lc (f )[f ].Lemma 4.1The set S 1:={D (P ([u ]))−[u ]|u ∈ALSW (D,{P };X )}is a Gr¨o bner-Shirshov basis in DLie ({P };X ).Proof.It is easy to check that S 1is a Gr¨o bner-Shirshov basis in DLie ({P };X ).Lemma 4.2Let u,v ∈ALSW (D,{P };X )and u >Dl v .(a)If λ=0and j >0,thenD j (f (u,v ))≡λj ([D j (f (u,v ))]−(D j −1([u ])D j −1([v ])))mod (S 1,D j (f (u,v ))),(b)If λ=0and j >0,thenD j (f (u,v ))≡[D j (f (u,v ))]−(D j −1([u ])P ([v ]))mod (S 1,D j (f (u,v ))).Proof.(a )The proof is by induction on j .For j =1,we haveD (f (u,v ))=D ((P ([u ])P ([v ])))−D (P (([u ]P ([v ])))−D (P ((P ([u ])[v ])))−λD (P (([u ][v ])))≡λ(D (P ([u ]))D (P ([v ])))−λD (P (([u ][v ])))≡λ([D (f (u,v ))]−([u ][v ]))mod (S 1,D (f (u,v ))).Assume that the result is true for j −1,j ≥2,i.e.D j −1(f (u,v ))=λj −1(D j −1(P ([u ]))D j −1(P ([v ])))−λj −1(D j −2([u ])D j −2([v ]))+ αi [πi |D t i (s i )]D t i (s i ),13where eachαi∈k,s i∈S1,πi|D t i(s i)<DlD j−1(P(u))D j−1(P(v)).Since S1isa Gr¨o bner-Shirshov basis in DLie({P};X),D(αi[πi|D t i(s i)]D t i(s i))=βl[σl|D n l(s l)]D n(s l),where eachβl∈k,s l∈S1,[σl|D n l(s l)]D n l(s l)is a special normal differentialD k l(s l)-word.By Lemma2.3,lD n l(s l)]D n l(s l)=σl|D n l(s l)<Dlj−1j−1=D j(P(u))D j(P(v)).Thus,we haveD j(f(u,v))=D(D j−1(f(u,v)))≡λj−1D((D j−1(P([u]))D j−1(P([v]))))−λj−1D((D j−2([u])D j−2([v])))≡λj(D j(P([u]))D j(P([v])))+λj−1(D j(P([u]))D j−1(P([v])))+λj−1(D j−1(P([u]))D j(P([v])))−λj(D j−1([u])D j−1([v]))−λj−1(D j−1([u])D j−2([v]))−λj−1(D j−2([u])D j−1([v]))≡λj(D j(P([u]))D j(P([v])))+λj−1(D j−1([u])D j−2([v]))+λj−1(D j−2([u])D j−1([v])))−λj(D j−1([u])D j−1([v]))−λj−1(D j−1([u])D j−2([v]))−λj−1(D j−2([u])D j−1([v])))≡λj(D j(P([u]))D j(P([v]))−λj(D j−1([u])D j−1([v]))≡λj([j−(D j−1([u])D j−1([v])))mod(S1,j.(b)The proof is similar to Case(a).Theorem4.3With the order>Dl on D,{P};X defined as before,the set Sis a Gr¨o bner-Shirshov basis in DLie({P};X).Proof.There are two casesλ=0andλ=0to consider.Case1.Forλ=0,all possible compositions of the polynomials in S are list as below:g(π|D j(D(P(v)))),g(v) w1,w1=D j(D(P(π|Di(D(P(v)))))),g(π|D i(P(u))Di(P(v))),f(u,v) w2,w2=D j(D(P(π|Di(P(u))Di(P(v))))),f(u,v),g(v) w3,w3=D l(P(u))D l(P(v)),l>0,f(u,v),g(u) w4,w4=D l(P(u))D l(P(v)),l>0,f(π|D i(D(P(u))),v),g(u) w5,w5=D j(P(π|Di(D(P(u)))))D j(P(v)),f(u,π|D i(D(P(v))))),g(v) w6,w6=D j(P(u))D j(P(π|Di(D(P(v))))),f(u,v),f(v,w) w7,w7=D j(P(u))D j(P(v))D j(P(w)),14f(π|D i(P(u))D i(P(v)),w),f(u,v) w8,w8=D j(P(π|D i(P(u))D i(P(w))))D j(P(w)),f(u,π|D i(P(v))D i(P(w))),f(v,w) w9,w9=D j(P(u))D j(P(π|D i(P(v))D i(P(w)))),where i,j≥0.We check that all the compositions in S are trivial.Here,we just check one composition as example.If j>0,then by Lemma4.2,we havef(π|D i(P(u))D i(P(v)),w),f(u,v) w8=λ−j D j(f(π|D i(P(u))D i(P(v)),w))−λ−i[D j(P(π|D i(f(u,v)))D j(P(w))]D(i)(f(u,v))≡−(D j−1([π|D i(P(u))D i(P(v))])D j−1([w]))−λ−i(D j(P(r([π|D i(f(u,v))]D i(f(u,v)))))D j(P([w])))≡λ−i(D j−1(r([π|D i(f(u,v))]D i(f(u,v))))D j−1([w]))−λ−i(D j−1(r([π|D i(f(u,v))]D i(f(u,v))))D j−1([w]))≡0mod(S,w8). If j=0,thenf(π|D i(P(u))D i(P(v)),w),f(u,v) w8=f(π|D i(P(u))D i(P(v)),w)−λ−i[P(π|D i(f(u,v)))P(w)]D i)(f(u,v))≡−P((P([π|D i(P([u]))D i(P([v]))])[w]))−P(([π|D i(P(u))D i(P(v))]P([w])))−λP(([π|D i(P(u))D i(P(v))][w]))−λ−i(P(r([π|D i(f(u,v))]D i(f(u,v))))P([w]))≡λ−i P((P(r([π|D i(f(u,v))]D i(f(u,v))))[w]))+λ−i P((r([π|D i(f(u,v))]D i(f(u,v))))))λ−i+1P((r([π|D i(f(u,v))]D i(f(u,v)))[w]))−λ−i P((P(r([π|D i(f(u,v))]D i(f(u,v))))[w]))−λ−i P((r([π|D i(f(u,v))]D i(f(u,v))))))−λ−i+1P((r([π|D i(f(u,v))]D i(f(u,v)))[w]))≡0mod(S,w8).Case2.Forλ=0,all possible compositions of the polynomials in S are list as below:g(π|D i(D(P(v)))),g(v) w1,w1=D j(D(P(π|Di(D(P(v)))))),g(π|D i(P(u))P(v)),f(u,v) w2,w2=D j(D(P(π|Di(P(u))P(v)))),f(u,v),g(u) w3,w3=D l(P(u))P(v),l>0,f(π|D i(D(P(u))),v),g(u) w4,w4=D j(P(π|Di(D(P(u)))))P(v),f(u,π|D i(D(P(v)))),g(v) w5,w5=D j(P(u))P(π|Di(D(P(v)))),f(u,v),f(v,w) w6,w6=D j(P(u))P(v)P(w),f(π|D i(P(u))P(v),w),f(u,v) w7,w7=D j(P(π|Di(P(u))P(v)))P(w),f(u,π|D i(P(v))P(w)),f(v,w) w8,w8=D j(P(u))P(π|Di(P(v))P(w))),where i,j≥0.We check that all the compositions in S are trivial.The proof is similar to Case1.15。
