Mott-Peierls Transition in the extended Peierls-Hubbard model
考研英语一翻译技巧讲解ppt课件

副词插入语:frankly, especially, fortunately, indeed, however…
短语插入语:generally speaking(总的来说), to tell the truth(老实说), in a sense(在某种意义上讲), in a word(总而言之), strange to say(说来奇怪), to some extent(从一定程度上讲)…
具体要根据各成分的修饰关系而定。
The virgin forest with its richness and variety of trees was a real treasure-house which extended from Maine all the way down to Georgia.(2015年真题)
人生中几乎不可能不经历某种失败。那些经历过失 败的人可能活得很谨慎,以致于一事无成。
准确、完整、通顺
完整——不漏译
The growing incidence of child abuse and child neglect. 与日俱增的虐待儿童和忽视儿童事件
准确、完整、通顺
通顺——说人话,符合汉语表达习惯
研究健康和身体如何吸收并利用食物成分之间的关 系的学科被称为临床营养学,它的历史可以被分为 四个不同的阶段。
方法总结:将同位语提前翻译,作为定语修饰被修 饰的名词,可视具体情况增译
另译:临床医药学研究……
插入语
插入语的作用:为了增加句子表达的多样性,在英语 中任何成分(词/短语/句子)都可以用两个逗号或者破 折号隔开成为插入成分。
后置定语
(3)现在分词短语 A leaf floating in the wind 飘在风中的一片叶子 (4)过去分词短语 The meeting held last month 上个月举办的会议 (5)动词不定式短语 A way to solve the problem 解决问题的一个方式
2017英语二新题型原文

2017英语二新题型原文The new format of the 2017 English II exam has caused quite a stir among students and educators alike. Many are concerned about the increased difficulty and the impact it may have on students' performance. However, it is important to consider the potential benefits of the new format and how it may better prepare students for future academic and professional endeavors.From the students' perspective, the new format may initially seem daunting and overwhelming. The increased length and complexity of the exam may lead to anxiety and stress for many students. However, it is important for students to approach the new format with a positive mindset and see it as an opportunity to challenge themselves and improve their English language skills. By embracing the new format and putting in the necessary effort and preparation, students can develop a deeper understanding of the English language and improve their overall proficiency.Educators are also facing challenges with the new format, as they must adapt their teaching methods and strategies to effectively prepare students for the exam. The increased emphasis on critical thinking and analysis requires educators to provide students with opportunities to engage in higher-level thinking and develop their analytical skills. Additionally, educators must ensure that students are equipped with the necessary resources and support to succeed in the new format, such as practice materials and guidance on how to approach the longer and more complex exam questions.Despite the initial concerns and challenges, the new format of the English II exam has the potential to better prepare students for future academic and professional endeavors. The increased length and complexity of the exam may better reflect the demands of college-level coursework and the professional world, where critical thinking and analysis are essential skills. By mastering the new format, students can develop the skills and confidence needed to excel in college and beyond.In conclusion, while the new format of the 2017 English II exam may present challenges for students and educators, it also offers valuable opportunities for growth and development. By approaching the new format with a positive mindset and embracing the challenge, students can improve their English language skills and prepare themselves for future success. Educators play a crucial role in supporting students through the transition to the new format, providing them with the necessary resources and guidance to excel. Ultimately, the new format has the potential to better prepare students for the demands of college and the professional world, equipping them with the skills and confidence needed to thrive in their academic and professional endeavors.。
翻译过程论

There are three basic translation processes: (a) the interpretation and analysis of the SL text; (b) the translation procedures, which may be direct, or on the basis of SL and TL corresponding syntactic structures, or through an underlying logical ‘interlanguage’; (c) the reformulation of the text in relation to the writer’s intention, the readers’ expectation, the appropriate norms of the TL, etc. The processes are to a small degree paralleled by translation as a science, a skill and an art. (英)纽马克(Newmark, P. )著. 《翻译问题探讨》 上海: 上海外语教育出版社,2001 P144 Peter Newmark. Approaches to Translation Pergamon Press Ltd., 1982
When we are translating, we translate with four levels more or less consciously in mind: (1) the SL text level, the level of language, where we begin and which we continually (but not continuously) go back to; (2) the referential level, the level of objects and events, real or imaginary, which we progressively have to visualize and build up, and which is an essential part, first of the comprehension, then of the reproduction process; (3) the cohesive level, which is more general, and grammatical, which traces the train of thought, the feeling tone (positive or negative) and the various presuppositions of the SL text. This level encompasses both comprehension and reproduction: it presents an overall picture, to which we may have to adjust the language level; (4) the level of naturalness, of common language appropriate to the writer or the speaker in a certain situation. Again, this is a generalized level, which constitutes a band within which the translator works, unless he is translating an authoritative text, in which case he sees the level of naturalness as a point of reference to determine the deviation-if anybetween the author’s level he is pursuing and the natural level. This level of naturalness is concerned only with reproduction. Finally, there is the revision procedure, which may be concerned or staggered according to the situation. This procedure constitutes at least half of the complete process. Newmark, P. A Textbook of Translation Shanghai: Shanghai Foreign Language Education Press, 2001 (p.19)
英语翻译-句子翻译PPT

02
Sentence translation skills
Literal translation and free translation
Literal translation
This method aims to confirm the exact meaning of the source language presence to the target language, without any changes in vocabulary or grammar It is important to maintain the original context and intention
Long presence translation
要点一
Breaking down complex senses
Long senses in the source language can often require breaking them down into smaller, more manageable units for translation This helps maintain clarity and flow in the target language version
Target language word order
Adjusting word order in the target language can help ensure a more natural and readable translation It is essential to maintain graphical correctness and logical flow while making these adjustments
研究生英语读写教程提高级unit13

研究生英语读写教程提高级unit13In the backdrop of globalization, the exchange and integration of cultures have become increasingly significant. As the world becomes more interconnected, the boundaries that once separated different cultures are gradually disappearing, giving way to a new era of cultural diversity and hybridity. This phenomenon is not merely a product of economic integration and technological advancements; it is also a reflection of the deep-seated human desire to connect, understand, and appreciate other cultures.One of the most significant impacts of globalization on cultural exchange is the ease with which information and ideas can now travel across borders. The internet, in particular, has revolutionized the way we access and share cultural content. Movies, music, books, and other forms of media are now accessible to a global audience, enabling people to experience diverse cultures without leaving their homes. This has not only broadened people's horizons but has also fostered a newfound respect and appreciation for other cultures.However, the process of cultural exchange is not always smooth. Differences in language, values, traditions, and beliefs can sometimes lead to misunderstandings and conflicts. It is, therefore, crucial that we approach cultural exchange with an open and tolerant mindset, respecting the differences while seeking commonalities. By doing so, we can not only avoid misunderstandings but also learn from other cultures, enriching our own cultural identity.Integration, on the other hand, refers to the blending of different cultures into a new, unique cultural identity. This process often occurs when cultures come into contact with each other, resulting in the emergence of new cultural forms and practices. For instance, the fusion of Western and Eastern music styles has given birth to a new genre of music that is both unique and appealing to a global audience. Similarly, the integration of different cuisines has led to the creation of new and exciting dishes that are a testament to the beauty of cultural diversity.In conclusion, the exchange and integration of cultures are inevitable in the age of globalization. It is ourresponsibility to ensure that this process is positive and constructive, fostering mutual understanding and respect among different cultures. By doing so, we can create a more inclusive and harmonious world where the richness ofcultural diversity is celebrated and cherished.**全球化背景下的文化交流与融合**在全球化的背景下,文化交流与融合变得越来越重要。
高考试卷英语选择题

第一部分:选择题Section A以下每小题各有一项选择,请从每题的四个选项中选出最佳答案。
1. My brother always keeps a ________ diary, which records his daily activities.A. accurateB. detailedC. simpleD. brief2. The teacher's explanation was so ________ that even the students who were not good at math understood it.A. difficultB. clearC. complicatedD. simple3. The government has announced a new policy to ________ the pollution in the city.A. reduceB. eliminateC. increaseD. control4. She ________ her phone and missed the important call.A. leftB. foundC. lostD. returned5. The company is planning to ________ its production line to improve efficiency.A. extendB. expandC. reduceD. decrease6. Despite the bad weather, the football match ________ as scheduled.A. was heldB. was canceledC. was postponedD. was played7. It's important to ________ your body with exercise and a healthy diet.A. strengthenB. weakenC. strainD. fatigue8. The museum ________ a new exhibition on ancient Chinese art next month.A. is holdingB. will holdC. heldD. has held9. The students were asked to ________ their papers and hand them in before the bell rings.A. finishB. writeC. collectD. correct10. The doctor recommended that the patient ________ more water to stay hydrated.A. drinksB. drinkC. drinkingD. had drunkSection B以下每小题各有一项选择,请从每题的四个选项中选出最佳答案。
Spin-Peierls transition with strong structural fluctuations in the vanadium oxide VOSb$_{2}

a r X i v :c o n d -m a t /0103441v 1 [c o n d -m a t .s t r -e l ] 21 M a r 2001Spin-Peierls transition with strong structural fluctuations in the vanadium oxideVOSb 2O 4V.A.Pashchenko 1,2,A.Sulpice 3,a 4,let 5,A.Stepanov 6,and P.Wyder 11Grenoble High Magnetic Field Laboratory,MPI-FKF and CNRS,BP 166,38042Grenoble Cedex 9,France 2B.Verkin Institute for Low Temperature Physics and Engineering,NASU,310164Kharkov,Ukraine 3Centre de Recherches sur les Tr`e s Basses Temp´e rautres,CNRS,BP 166,38042Grenoble Cedex 9,France4Institut de Physique Th´e orique,Universit´e de Lausanne,CH-1015Lausanne,Switzerland5Centre d’Elaboration des Mat´e riaux et d’Etudes Structurales,CNRS,BP 4347,31055Toulouse Cedex 4,France6Laboratoire Mat´e riaux et Micro´e lectronique de Provence,Universit´e d’Aix-Marseille III,CNRS,Facult´e des Sciences de Saint-J´e rˆo me,C-151,13397Marseille Cedex 20,France(February 1,2008)We report on the magnetic susceptibility and electron spin resonance measurements on polycrys-talline samples of the vanadium oxide VOSb 2O 4,a quasi-one dimensional S=1/2Heisenberg system.We show that the susceptibility vanishes at zero temperature,as in a gapped system,and we argue that this is due to a spin-Peierls transition with strong structural fluctuations.PACS numbers:75.45.+j,75.50.Ee,75.40.Cx,76.30.-vAlthough the study of the spin-Peierls (SP)transition in S =1/2antiferromagnetic (AF)Heisenberg chains has started a long time ago with the discovery of the first SP transition in the organic system TTFCuBDT in 1975[1,2],a major breakthrough in the field was the discovery in 1993by Hase et al.[3]of the first inorganic system exhibiting a SP transition,namely CuGeO 3.The possibility to grow large single crystals has led to a very intensive experimental activity,and the understanding of the properties of such systems,in particular in strong magnetic fields,has been dramatically improved.However CuGeO 3is representative of only one class of spin-Peierls systems,namely systems in which struc-tural fluctuations are to a certain extent negligible.In such systems,the dimerization of the lattice is very bru-tal,and the susceptibility exhibits a characteristic cusp at the transition temperature.The irrelevance of struc-tural fluctuations in a 1D system is very surprising,and the first theories actually predicted a strongly fluctuat-ing regime above the transition [4].This discrepancy was resolved by Cross and Fisher [5],who showed that an appropriate treatment of 3D phonons can lead to a significant suppression of fluctuations.The study of fluctuations in spin-Peierls systems has recently restarted however with the careful analy-sis of the spin-Peierls transition in the organic system (BCPTTF)2X by Dumoulin et al.[6]in 1996who con-vincingly showed the presence of very strong structural fluctuations above the spin-Peierls transition.Judging from the impact of CuGeO 3on the field,the search for inorganic systems with similar properties is a real chal-lenge.However the inorganic spin 1/2chains synthetized so far do not seem to fill this gap.Most of them just do not show any sign of a SP instability,like Sr 2CuO 3[7]or MgVO 3[8],while the transition observed in NaV 2O 5[9]is very abrupt and is now believed to involve charge degrees of freedom as well.In this paper we report on the magnetic properties of a vanadium oxide,VOSb 2O 4,which we believe is the first example of an inorganic system that undergoes a SP tran-sition with very strong fluctuation effects.This system is made of almost isolated chains of VO 5pyramids.Ac-cording to Darriet,Bovin and Galy [10]VOSb 2O 4crys-tallizes in the monoclinic system,space group C 2/c ,with the unit cell dimensions a =18.03˚A ,b =4.800˚A ,c =5.497˚A ,β=94.58◦(Z=4).The vanadium atoms are fivefoldcoordinated in a slightly distorted square pyramids with one characteristic short vanadyl bond V-O close to 1.59˚A towards its apex and 2×2longer bonds at 1.91and 2.04˚A with the oxygens of the square base.Along the [001]direction the apices of the VO 5pyramids alternately point up and down relative to a plane of the square base (see Fig.1(a)).The smallest in-chain V-V distance is approximately 3.01˚A .The distances between the chains are 4.80and 18.03˚A for the [010]and the [100]direc-tion,respectively.Thus from a magnetic point of view,the VOSb 2O 4structure can be viewed as infinite isolated chains of V 4+ions running along the [001]direction.The antimony atoms exhibit the typical one-sided threefold coordination of the oxygen atoms having a stereoactive lone pair E [10](see Fig.1(b)).Polycrystalline samples of VOSb 2O 4having a light-green color were synthesized by solid-state reaction [10].ESR X-band spectra were collected using a Bruker ESP300spectrometer equipped with a standard TE 102cavity and a continuous helium flow cryostat that allows temperature scans between 4and 300K.The temper-ature and the field variation of the magnetization was measured with a Quantum Design SQUID magnetome-ter from 300to 1.8K in fields up to 4T.(a)(b)FIG.1.(a)Polyhedral representation of the VOSb2O4 structure illustrating the infinite isolated chains of VO5pyra-mids.(b)the(ac)plan projection of the crystal structure of VOSb2O4.The temperature dependence of the magnetic suscep-tibilityχraw(T)of a100mg polycrystalline sample of VOSb2O4in afield of2T is shown in Fig. 2.Be-low room temperature,when the temperature is lowered,χ(T)passes through a broad maximum at T max≈160 K,which is typical of a S=1/2Heisenberg chain withJ/k≃250K.However,on further cooling the sample, there is a rapid decrease which starts around40K,and the slope exhibits a clear maximum at T sp=13K.Fi-nally,there is a minimum at10K followed by an increase of the susceptibility indicating the presence of magnetic impurities.The drop that starts around40K is remi-niscent of a SP transition,but there is a dramatic differ-ence with CuGeO3:there is no cusp in the susceptibility around the temperature where it becomes much smaller than the Bonner-Fisher prediction.On the contrary,the susceptibility drops rapidly but smoothly below35K, very much like in(BCPTTF)2X.FIG.2.The temperature dependence of the magnetic sus-ceptibility of a100mg VOSb2O4polycrystalline sample χraw(T)at H=2T(open diamonds).Open triangles,dashed line and solid line represent the corrected for the impurity con-tribution VOSb2O4susceptibilityχcor(T),the impurity con-tribution toχraw(T)and the bestfit to theχ(T)of S=1/2 HAF according to Eq.(50),Ref.18(J/k=245±5K,χ0=0.00021cm3/mol,g=1.66),respectively.The insert details low temperature behavior of dχcor(T)/dT.Before we can start discussing the physical origin of this unusual behaviour,thefirst thing we must check is whether the susceptibility indeed goes to zero at zero temperature,as in a SP transition.Let usfirst study in more details the impurity contribution.To characterize more quantitatively this impurity contributionχimp(T) toχraw(T),particularly with an idea to separate it from the intrinsic susceptibility of the VOSb2O4phase,which we will callχcor(T),we have carried out magnetization measurements vs.H at variousfixed temperatures from 80K to1.8K.The results are shown in Fig.3.An impor-tant information aboutχimp(T)is contained in the low temperature nonlinear dependence of M imp(H,T)when µH>kT.Therefore we have to examine the data in Fig. 3using the following equation M(H,T)=M imp(H,T)+χcor(T)·H,where M imp(H,T)is expressed in a standard way as M imp(H,T)=p imp N A gµB S·B S(gµB SH/kT), B S is the Brillioun function,S is an impurity spin value and p imp defines the relative impurity concentra-tion.The results of afit of the experimental data with this equation are shown in Fig.3as solid lines.The following parameters S=1/2,p imp=0.00573(5)were extracted(g=1.975wasfixed in thefit procedure)to-gether with the AF Curie-Weiss constantθ≈0.6K obtained from the low-T dependence ofχ−1raw(T).We are now in a position to correctχraw(T)for the impu-rity contribution.In Fig.2χimp(T)=p imp C/(T+θ) (C=0.366cm3·K/mol)is plotted(dashed line)along with theχcor(T)=χraw(T)−χimp(T).This behaviour is consistent with a zero contribution to the spin sus-ceptibility χspin (T )=χcor (T )−χ0at zero temperature if the sum of the diamagnetic and Van Vleck contribu-tions χ0is equal to χcor (T =0).While the diamag-netic contribution can beestimated from standard tables (χdia ≃−1.01×10−4cm 3/mol [11]),an unbiased estimate of the Van Vleck susceptibility would require susceptibil-ity data at temperatures much larger than the typical exchange integrals,a regime which is not accessible.M o m e n t (e m u /m o l )FIG.3.The magnetization of VOSb 2O 4vs.magnetic field at different temperatures.The symbols are experimental data,the solid lines are the fit results according to the equa-tion of M (H,T )discussed in the text.To go around this difficulty,we have performed ex-tensive ESR measurements.A representative series of X-band ESR spectra recorded from 320to 7.3K on a polycrystalline sample (12mg)is presented in Fig.4(a).We note the axial symmetry of the obtained spectra,especially apparent at T =20K,reflecting the axial symmetry of the crystal field acting on V 4+ions in the fivefold pyramidal environment.The computer spectra simulations over the temperature range 13-320K give two T -independent g -factors:g ⊥=1.978,g =1.930with the average value of 1.962±0.002already reported for low-dimensional vanadates [8,12].At low T it is found that the measured spectra contain an additional ESR signal.The intensity of this additional signal roughly follows Curie law and the average g −factor is found to be 1.975±0.005.We ascribe this signal to the magnetic impurities which are responsible for the steep increase of the magnetic susceptibility at low temperatures (see above).To extract information from these ESR spectra,we have proceeded in the following way.Since the ESR is insensitive to the diamagnetic and Van Vleck contribu-tions to the susceptibility,we are able,by double in-tegration of the ESR spectra,to reconstruct the sum χspin (T )+χimp (T )and then using an appropriate pro-cedure for the substraction of χimp (T )to restore the T -dependence of χspin (T ).(a)(b)FIG.4.(a)The temperature evolution of ESR spectra in the VOSb 2O 4polycrystalline sample from 320to 7.3K;(b)the temperature dependence of the peak-to-peak linewidth ∆H pp in VOSb 2O 4extracted from computer simulation of experimental spectra.The insert shows the double inte-grated ESR spectra intensity χESR spin (T )(solid diamonds).χspin (T )=χcor (T )−χ0deduced from Fig.2is also shownscaled with χESR spin (T )so,that χspin (T max )=χESRspin (T max ).In the case of VOSb 2O 4the substraction of χimp (T )is a rather tedious but unambiguous procedure because i)the impurity ESR spectra have quite different line pa-rameters (such as the linewidth,g-factor and the tem-perature dependence of spectra intensity)as compared to the main spectra;ii)from the magnetization measure-ments we know the T −evolution of χimp (T )so,we can use this information to check the correctness of the sub-straction at each T .We drop out the technical details of this procedure and postpone them to our forthcomingpaper.TheχESRspin (T)data as extracted from ESR aregiven in an inset of Fig.4(b).It is clearly seen that the spin magnetic susceptibility of VOSb2O4goes to zero in the limit T→0,a result which is qualitatively appar-ent from the examination of ESR spectra at20,13and 7.3K in Fig.4(a).For example,the7.3K ESR spec-trum is almost for95%an impurity one.The fact that χspin(T→0)≈0clearly evidences that the VOSb2O4 ground state at low temperature is a nonmagnetic singlet S=0.Note that the temperature dependence is consis-tent with that deduced from the susceptibility measure-ments after substraction of the impurity contributions and assuming that the Van Vleck contribution is such thatχcor=0(see inset of Fig.4(b)).Another very useful information is contained in the temperature dependence of the line width.As seen from Fig.4(b),the peak-to-peak linewidth∆H pp shows a characteristic V-like temperature dependence(a strong decrease of∆H replaced at T≈13K by a rapid increase of∆H).Such a behaviour has been previously observed in both SP materials NaV2O5and CuGeO3[13],the min-imum temperature being equal to the SP transition tem-perature.Let us now discuss the various possibilities to explain this behaviour.Assuming that the chains are well iso-lated magnetically,which is very reasonable given the ge-ometry,we can think of only two possibilities to explain a vanishing susceptibility,hence the presence of a spin gap, at zero temperature:Frustration or dimerization due to a SP transition.Let us analyze both possibilities. Frustration:It is well known that a coupling J2to second neighbours can lead to a spin gap if its ratio to thefirst-neighbour coupling J1is larger than0.24[14,15]. However the presence of a significant coupling between second neighbours will not just open a gap at low temper-ature,but will modify the temperature dependence of the susceptibility at high temperature as well.We have thus tried tofitχspin(T)with a significant value of J2.The resultingfit is very bad,and much worse actually than without J2between40K and300K.So this possibility seems unlikely.Besides,if we compare with MgVO3,an-other quasi-1D vanadium oxide which does not show any anomaly at low temperature[8],the chains have the same structure.But the magnetic measurements performed on MgVO3show no indication whatsoever of intra-chain frustration.So it seems more plausible that the differ-ence between the magnetic properties of these systems comes from the interaction between the chains.In fact, the chains are further apart in VOSb2O4than in MgVO3, especially in the a direction,where most of the residual coupling is believed to occur in MgVO3.So it is not sur-prising that typical1D effects show up in VOSb2O4and not in MgVO3.Dimerization due to SP transition:In principle,a S=1/2chain is always unstable towards dimerization, but the transition temperature can be strongly reduced due tofluctuations of the lattice,especially if the system is very one-dimensional.In the present case,a goodfit of the high-temperature susceptibility with the suscep-tibility of the S=1/2chain[16–18]is possible(see Fig.2),although with an effective g-factor smaller than the actual one measured in ESR.This discrepancy is actu-ally ubiquitous in V4+vanadates,whose properties are quite well understood otherwise,and it seems legitimate not to worry too much about it.It might come from dif-ferent factors ranging from a poor determination of the weight due to the absorption of water by the sample to the presence of some non-magnetic impurity phase. The next question is whether we do have a SP transi-tion.From the susceptibility measurements alone,it is not possible to conclude.But if there is a transition,it seems likely that it does not take place at the onset of the drop,like in CuGeO3,but at the temperature where the derivative of the susceptibility is maximal,i.e.13K.This scenario is actually favoured by ESR measurements since the line-width changes dramatically at the same temper-ature.However,clear signatures of the transitions,like new Bragg peaks or new phonons lines below13K,are not available yet.If on the contrary the system remainsfluctuating with a pseudo-gap down to zero temperature,as in the Lee-Rice-Anderson theory of the Peierls transition in metal-lic systems[4],the susceptibility is expected to decrease smoothly to zero.This would be consistent with our data.The behaviour of the line-width under such circum-stances is not known however,and more work is needed to check whether our data can exclude this possibility. To summarize,we have presented clear evidence that a spin gap opens in the quasi-1D vanadium oxide VOSb2O4 from susceptibility,magnetization and ESR data.The overall behaviour strongly suggests that this is due to the inherent SP instability of this spin1/2chain,but with very strongfluctuations.Given the lack of inorganic materials exhibiting this physics so far,the properties of this system are likely to attract a lot of attention in the future.[9]M.Isobe and Y.Ueda,J.Phys.Soc.Jpn.65,1178(1996).[10]B.Darriet,J.Bovin and J.Galy,J.Solid State Chem.19,205(1976).[11]P.W.Selwood,Magnetochemistry,2nd ed.(Interscience,New York,1956).[12]I.Yamada et al.,J.Phys.Soc.Jpn.67,4269(1998).[13]M.Lohmann et al.,Solid State Commun.104,649(1997).A.N.Vasil’ev et al.,Phys.Rev.B56,5065 (1997).S.Shmidt et al.,Phys.Rev.B57,2887(1998).B.Grenier et al.,Physica B259-261,961(1999).[14]C.K.Majumdar and D.K.Ghosh,J.Math.Phys.(N.Y.)10,1388(1969).[15]K.Okamoto and K.Nomura,Phys.Lett.A169,433(1992).[16]J.C.Bonner and M.E.Fisher,Phys.Rev.135,A640(1964).[17]S.Eggert,I.Affleck,M.Takahashi,Phys.Rev.Lett.73,332(1994).[18]D.C.Johnston at al.,cond-mat/0003271v223Mar2000.。
湍流燃烧模型
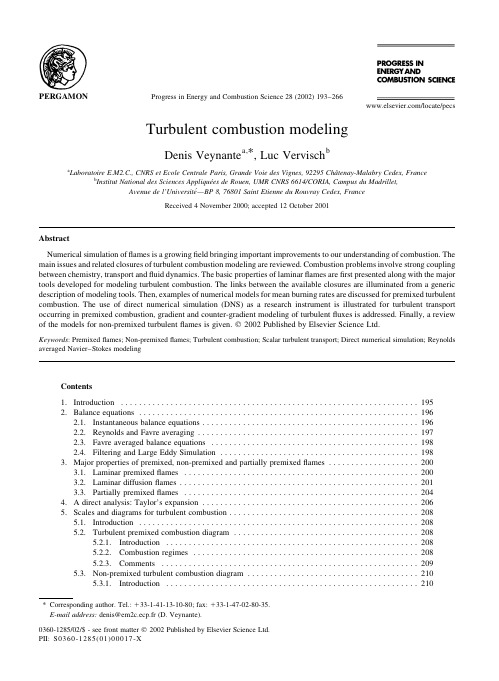
Contents
1. Introduction . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 195 2. Balance equations . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 196
194
D. Veynante, L. Vervisch / Progress in Energy and Combustion Science 28 (2002) 193±266
6. Tools for turbulent combustion modeling . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 212 6.1. Introduction . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 212 6.2. Scalar dissipation rate . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 214 6.3. Geometrical description . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 214 6.3.1. G-®eld equation . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 214 6.3.2. Flame surface density description . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 216 6.3.3. Flame wrinkling description . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 218 6.4. Statistical approaches: probability density function . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 218 6.4.1. Introduction . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 218 6.4.2. Presumed probability density functions . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 219 6.4.3. Pdf balance equation . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 219 6.4.4. Joint velocity/concentrations pdf . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 221 6.4.5. Conditional moment closure (CMC) . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 221 6.5. Similarities and links between the tools . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 221
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
a r X i v :c o n d -m a t /9711266v 1 [c o n d -m a t .s t r -e l ] 25 N o v 1997Mott-Peierls Transition in the extended Peierls-Hubbard modelEric JeckelmannDepartment of Physics and Astronomy,University of California,Irvine,CA 92697.(February 1,2008)The one-dimensional extended Peierls-Hubbard model is studied at several band fillings using the density matrix renormalization group method.Results show that the ground state evolves from a Mott-Peierls insulator with a correlation gap at half-filling to a soliton lattice with a small band gap away from half-filling.It is also confirmed that the ground state of the Peierls-Hubbard model undergoes a transition to a metallic state at finite doping.These results show that electronic correlations effects should be taken into account in theoretical studies of doped polyacetylene.They also show that a Mott-Peierls theory could explain the insulator-metal transition observed in this material.71.10.Fd,71.30.+h,71.10.Pm,71.20.RvSince the discovery of the metallic phase of doped polyacetylene,this material has been extensively stud-ied [1,2],but the mechanism of the insulator-metal transi-tion observed upon doping is still poorly understood.It is known that both the Peierls instability and electronic cor-relations play a fundamental role in the formation and the properties of the insulating phase [3]and thus undoped polyacetylene is a Mott-Peierls insulator [4].Therefore,ten years ago,Baeriswyl,Carmelo and Maki [5]proposed that the insulator-metal transition was also driven by the interplay of electron-electron and electron-phonon in-teractions.Within the restricted Hartree-Fock approxi-mation they have shown the possibility of such a Mott-Peierls insulator-metal transition in the Peierls-Hubbard model,which is the simplest model of polyacetylene in-cluding both interactions.Recently,several works us-ing sophisticated numerical many-body methods,such as the Gutzwiller variational wavefunction [6]and quantum Monte Carlo (QMC)simulations [7],have confirmed the occurrence of an insulator-metal transition in this model.On the other hand,Wen and Su,which have applied the the density matrix renormalization group (DMRG)tech-nique to this problem,have disputed the existence of this transition [8].Hartree-Fock [9]and QMC [7]simulations have also shown that the nearest-neighbor Coulomb re-pulsion opposes and can prevent the formation of a metal-lic state in the extended Peierls-Hubbard model.As an attempt to clarify this issue I have studied the properties of the extended Peierls-Hubbard model with parameters leading to a Mott-Peierls insulating ground state at half-filling.Accurate ground states and gaps are obtained for open chains up to 200sites and different band fillings using the DMRG method [10]and finite size effects have been carefully analyzed.Results show that the ground state of a Mott-Peierls insulator evolves to a soliton lattice upon doping.This soliton lattice is quali-tatively similar to the ground state predicted by simple electron-phonon models [11,12],but both the gap and the amplitude of the lattice distortion decrease faster in the extended Peierls-Hubbard model than in these mod-els when the doping increases.An insulator-metal tran-sition occurs at a finite doping concentration in the ab-sence of nearest-neighbor electron-electron interaction,in agreement with previous studies of the Peierls-Hubbard model [5–7].These results demonstrate that electronic correlations effects are important and should be taken into account in theoretical studies of doped polyacety-lene.They also confirm that a Mott-Peierls theory [4–6]could explain the insulator-metal transition observed in polyacetylene.The one-dimensional extended Peierls-Hubbard model is defined by the HamiltonianH =1πℓ(−1)ℓ∆ℓ− ℓσt −(−1)ℓ∆ℓTo determine the ground state of the Hamiltonian(1),one has to find both the lattice configuration {∆ℓ}and the electronic wavefunction which minimize the total ing a finite system DMRG algorithm [10],one can compute the electronic ground state,its energy and the gradient of this energy (thanks to the Hellmann-Feynman theorem)for any given lattice configuration,and thus perform the minimization of the total energy with respect to lattice degrees of freedom {∆ℓ}[6,8].In principle,direct electronic excitations can also be ob-tained by calculating excited states of the electronic part of the Hamiltonian (1)for a fixed lattice configuration.Unfortunately,while the DMRG method gives excellent results for ground states,it is more difficult to obtain results for specific excited states.Therefore,I have only calculated charge gaps,which can easily be obtained from ground state energies for different band fillings [6].The charge gap is believed to be equal to the lowest opti-cal absorption energy in the thermodynamic limit of the Peierls-Hubbard model (V =0).In the extended Peierls-Hubbard model (V =0)the relation between charge gap and optical gap is not known precisely but in this work I have assumed that both quantities are roughly equivalent in the thermodynamic limit.All calculations have been carried out for several chain lengths up to 200sites and results have always been ex-trapolated to an infinite chain.Only open chains are considered because the DMRG method performs much better in this case than for periodic boundary putations have been performed so that numerical er-rors on the ground state dimerization parameter ∆ℓare smaller than 10−3t .Numerical errors on gap values are estimated to be less than 10−2t at half filling and around 10−3t away from half filling.All these estimations of the accuracy are based on an analysis of the behavior of DMRG results as a function of the number m of quan-tum states kept per block.The largest value of m used in this work ranges from 80for short chains (50sites)at half filling to 400for long chains (200sites)away from half filling.Truncation errors are typically between 10−6and 10−7.I have also checked the accuracy of DMRG calculations against exact numerical results for long (up to 100sites)non-interacting (U =V =0)chains and against exact results for the one-dimensional Hubbard model (∆ℓ=0and V =0)[13].An excellent agreement has been found in both cases.My results at half filling are in good agreement with results obtained previously with DMRG [14]and other many-body techniques like exact diagonalizations,quan-tum Monte Carlo simulations,and variational meth-ods [3].They confirm that undoped polyacetylene is a Mott-Peierls insulator,which can be described with a reasonable accuracy by the extended Peierls-Hubbard models.I have determined appropriate parameters for polyacetylene by comparing model predictions to exper-imental values for the optical gap at half-filling,theDoping level (%)0.00.20.40.60.8G a p (u n i t s o f t )FIG.1.Optical gap (in units of t )of the Peierls-Hubbard (PH)model,extended Peierls-Hubbard (EPH)model and SSH model as a function of doping.optical transition energies induced by photo-generated neutral and charged solitons and the neutral soliton spin density obtained from magnetic resonance exper-iments [1,2].This comparison shows that λ=0.1,U =4V =2.5t and t =2.7eV seem to be appropriate for polyacetylene in agreement with previous studies [3,6].It is important to realize that in the Mott-Peierls regime the optical gap at half-filling is essentially a corre-lation gap as in the one-dimensional Hubbard model [13],although the electron-phonon coupling and the Peierls instability are responsible for features like the dimeriza-tion and the existence of solitons.For instance,DMRG calculations predict an optical gap E g =0.74t for the pa-rameters mentioned above.As the gap is only ∼0.04t for U =V =0and λ=0.1,electronic correlations account for at least 94%of the gap.Consequently,one expects this gap to be strongly reduced as soon as the system is doped because electronic correlations do not contribute to the formation of a gap away from half filling in the Hubbard model.A strong experimental evidence for this reduction is the difference between the gap at half filling (1.8eV )and the energy of the optical transition induced by photo-generated charged solitons (0.45eV )which cor-responds to the gap of a lightly doped chain in our sim-plified model.I have investigated the extended Peierls-Hubbard model at several dopant concentrations up to y =16%in the Mott-Peierls regime.In this regime the system evolves upon doping from the Mott-Peierls insulating state to a soliton lattice with a small gap.The evolu-tion of the optical gap upon doping is shown in Fig.1for the polyacetylene parameters mentioned previously.As expected,the gap is strongly reduced to ∼0.17t as soon as the system is doped.The amplitude of the lattice dis-tortion and the gap decrease with increasing doping but no transition to a metallic state is found up to the highest doping studied in this work (y =16%).It is possible that a transition occurs at a higher doping but this would not be relevant for the transition observed in polyacetylene21/ Chain length0.000.050.100.150.20C h a r g e g a p (u n i t s o f t )FIG.2.Charge gap (in units of t )of the Peierls-Hubbard model as a function of the inverse system size for two different doping levels.Lines are linear extrapolations.around y =6%.Quantum Monte Carlo simulations [7]have also shown that the lattice distortion survives at high doping for V =U/2.For y >4%,the amplitude of the lattice distortion ∆ℓcorresponds exactly to the value of the gap if both quantities are extrapolated to an in-finite chain.Therefore,away from half filling the gap is a band gap generated by the lattice modulation,though electronic correlations contribute indirectly to its forma-tion because they increase the amplitude of the lattice distortion [6].The soliton lattice found in the doped extended Peierls-Hubbard model is qualitatively similar to the soliton lat-tice predicted by simple electron-phonon models [12].However,it is important to realize that the evolution of the gap and lattice distortion amplitude upon dop-ing is different from the predictions of the Su-Schrieffer-Heeger [11](SSH)model (shown in Fig.1for λ=0.2).To reproduce the results obtained in the extended Peierls-Hubbard model with the SSH model one would need to use an effective electron-phonon coupling which de-creases with increasing doping.Moreover,such a doping-dependent parameter λ(y )should change abruptly at half-filling to reproduce the sudden disappearance of the correlation gap.Finally,one notes that the energy scales involved in both approaches differ by an order of mag-nitude.For instance,my calculations indicate a gap of about 0.07eV at y =8%while the SSH model pre-dicts 0.4eV [6].These results demonstrate that elec-tronic correlations effects in such one-dimensional sys-tems are not reproduced by simple single-electron mod-els with an effective electron-phonon coupling,contrary to a basic assumption of the SSH theory of conducting polymers [1,11].Thus,the electron-electron interaction and electronic correlations effects should be taken into account in theoretical studies of doped polyacetylene.In Fig.1one can also see that the gap of the Peierls-Hubbard model with λ=0.1and U =2.5t vanishes at a critical doping between 8and 12%.These results confirm the existence of a transition to a metallic state at a finiteBond index m−0.040.000.040.080.12∆m /tFIG. ttice dimerization parameter ∆m of the Peierls-Hubbard model at y =12%.doping [5–7].They also show that the electron-electron interaction can either support or oppose the Peierls in-stability away of half-filling depending on the parame-ters used,as predicted by the restricted Hartree-Fock approximation [5,9].It should be noted that my numer-ical results agree quantitatively with those presented in Ref.[8]but additional calculations and an analysis of finite size and chain edge effects lead to a different con-clusion.Fig.2shows the charge gap as a function of the system size for y =8%and 12%.The value of the gap extrapolated to an infinite chain is clearly finite for y =8%but vanishes for y =12%within numerical er-rors (∼10−3t ).The lattice dimerization parameter ∆ℓis shown in Fig.3for a 200-site chain at y =12%.The shape of ∆ℓlooks similar for y =8%except that the amplitude is smaller at higher doping.However,in the insulating phase (y ≤8%),the amplitude of the distor-tion in the middle of the chain tends to a finite value as the chain length increases.This confirms that this lattice modulation is a genuine Peierls distortion.On the other hand,in the metallic regime (y ≥12%)the amplitude de-creases as a power-law with an exponent −0.66as the sys-tem size increases.This behavior demonstrates that the lattice distortion is a chain edge effect with a very slow asymptotic decay.The amplitude of these Friedel oscilla-tions is known to decrease asymptotically as a power-law with an exponent -1in a one-dimensional Fermi liquid,but density fluctuations are strongly affected by electron-electron interaction and the exponent can be as small as −1acetylene.They also suggest that the primary mecha-nism of the insulator-metal transition in polyacetylene is the interplay between electron-phonon and electron-electron interactions,which induces a transition upon doping from an insulating state with a gap of∼1.8eV to a state with a gap which is two orders of magnitude smaller.Obviously,this theoretical investigation of the properties of an ideal,infinite and isolated chain cannot describe the properties of actual physical systems.Un-derstanding the insulator-metal transition of polyacety-lene will require the study of more realistic model in-cluding lattice dynamics,interchain couplings,interac-tion with dopant ions and disorder[16].In the future I plan to study such models with DMRG,particularly the effects of quantum lattice dynamics.I thank S.White and D.Baeriswyl for helpful com-ments and discussion.I wish to acknowledge support from the Swiss National Science Foundation.This work was also supported in part by the Campus Laboratory Collaborations Program of the University of California and by the NSF under Grant No.DMR-9509945.Some of the calculations were performed at the San Diego Su-percomputer Center.。
