Tavis-Cummings模型中三体纠缠态纠缠量的演化特性

合集下载

论文T-C模型

Tavis-Cummings模型中耦合双原子纠缠度的演化特性刘惠平（大连交通大学数理系，辽宁大连 116028）摘要：本文主要考虑了Tavis-Cummings(T-C)模型中有偶极-偶极相互作用的双原子和单模相干光场相互作用时原子间的纠缠演化规律。

文章给出了模型中系统时间演化算符的具体形式，并且给出了两原子初始均处于基态时不同耦合程度下原子之间的纠缠演化曲线和原子布居差演化曲线，发现原子之间始终是纠缠的，纠缠度随时间作周期性振荡，振荡幅度和周期都与原子间的耦合强度密切相关，同时原子间纠缠度的演化与原子布居差的坍塌-恢复有关联。

关键词：T-C模型；量子纠缠；布居差中图分类号：O431.2The Evolution Properties of Entanglement of Two Coupling Atoms in the Tavis-Cummings ModelLiu Huiping1，Cai Jinfang2(1.Dalian Jiaotong University Dlian 116028，China；2.Department of Physics,Beijing Institute of Technology,Beijing 100081，China)Abstract: Atomic evolution properties of entanglement are represented by concurrence and the effects of atoms-coupling on the properties are considered within the context of the Tavis-Cummings model. Two coupling two-level atoms interact with a quantized cavity field. The explicit forms of the evolution operator are given. We find atomic entanglement can always be kept though atoms are initially unentangled. The results also show that the amplitude of the entanglement evolution oscillation increases and the periods of the oscillation become longer with increasing atomic coupling effect. When atomic coupling is strong enough, atomic entanglement evolution behavior is similar to that of the Heisenberg model. A tomic entanglement evolution properties have relations with atomic population. Key words:T-C model；quantum entanglement；atomic population1引言T-C模型]1[是描述多个二能级原子和单模量子化光场相互作用的重要理论模型，从提出来就引起人们广泛的兴趣，国内外很多学者对这一模型及其推广作了大量的研究，并揭示出它们多种多样的非经典性质，如原子布居的周期坍塌与恢复、原子算符的压缩、光场的压缩性质等，但是对T-C模型中有关量子信息方面的研究还只是开始。

Tavis-Cummings模型中原子运动时三体纠缠量的演化特性

在量子隐行传态、子密集编码、子密钥分配量量以及在量子计算的加速、子纠错、错等方面量防
都起着关键作用。目前，们对Ｔｖｓｕｍｉｇ人ａｉ— ｍｎｓＣ括：场与纠缠双原子相互作用过程中的熵演化光中两两问的纠缠量的演化特性。
腔与腔场相互作用，而研究场与原子耦合产生体系三体纠缠量的影响。从的各种量子效应＿。原子运动和场模结构对原６］
子反转、的压缩、子力学通道与量子互熵以场量
ｌ原子运动时ＴＣ模型中态矢的演化 —
量子纠缠不仅对了解量子力学的基本概念有
重要意义，时它更是一种有用的信息 “ 源 ” 同资。
这里不考虑原子问的偶极．极相互作用。在偶偶
收稿日期：２０４２７０７４））
基金项目：江西省自然科学基金（３２１）０１０７
考虑两个全同的二能级原子处在单模腔场中，成双原子的ＴＣ模型。两原子以相同的速构 — 度ｖ同时出发沿腔轴Ｚ向运动。为简单起见，方
及光子的反聚束效应等诸多非经典特性都有着不可低估的影响。。 ’
研究中均得到十分有意义的结果。

耦合系数对Tavis—Cummings模型中三体纠缠态纠缠量的影响

求解该方程（考虑共振情况）并考虑初始条件，仅，
则得到：Ａ（）＝Ｔ（。１６）３ｒ１＂，ｏｏｋ
Ｔｎｏｇ等提出了用于表示三体及三体中两两间的
（分别为原子２的赝自旋算符，，口１口口分别为光
纠缠程度的物理量一纠缠量。Ｚｏ和Ｒｏ８ｕａ＿根据子的湮灭和产生算符，ｇ为原子一场间的耦合常光该纠缠量的定义，论了初始时两原子处于纠缠态量，为原子间偶极一讨偶极相互作用强度。初始时而演化成三体纠缠的纠缠度。本文从初始时两原子原子ｌ和原子２均处于基态，光场处于单光子状态，均处于基态与单模光场相互作用，而演化成三体纠可以得出初始时刻原子一光场体系的状态波函数缠态的情况，讨论了体系纠缠量随时间演化特征，以为：期对纠缠态的制备及量子纠缠的其他性质研究有一Ｉ（）Ｉ，ｂ，）０）＝，１ｂ（）２
日导。。÷ｌ宅｝＋：＋｝＋∞ 口： ∞ ｌ。口
ｇ（一ｈ口１＋口１＋）＋ｇ（一ｈ口２＋口２＋）＋｝．２＋＋２ｌ１（＋１）一（）１
域。一方面，量子纠缠体现了量子态的非定域性；另一方面，它在量子信息处理，例如量子隐形传态、式中‰ 为原子的本征跃迁频率，为腔场频率，口和）分别为原子激发态和基态，．＝量子编码及量子纠错、子密钥分配和量子计Ｉ）Ｉ６量口）口ＩＩ１（１，１＝１（１，１＝算＿中具有重要应用。因此，３研究多体系之间的Ｉ１（１－ｂ）ｂＩ＋Ｉ口）ｂＩ一）口１ｂ的赝自旋算符，＝Ｉ口）量子纠缠对量子信息处理具有重要的意义。对于三Ｉ，（，分别为原子ｌ口Ｉ— ｂ）ｂＩ２＝Ｉ２（２，．口）ｂＩ２＝Ｉ２）ｂ体或多体系统由于其复杂性一直处于探讨中。最近（２Ｉ２（２，＋

三量子比特纠缠态-概述说明以及解释

三量子比特纠缠态-概述说明以及解释1.引言1.1 概述概述量子比特是量子信息处理中的基本单元，而纠缠态是量子力学中一种特殊的量子态，其中量子比特之间存在非经典的相互关联。

在量子信息领域, 三量子比特纠缠态作为一种特殊形态的纠缠态，其研究具有重要意义。

本文旨在探讨三量子比特纠缠态的定义、性质以及在量子信息领域的应用，通过深入研究三量子比特纠缠态可以为量子信息处理提供新的思路和方法。

容1.2 文章结构:本文主要分为引言、正文和结论三个部分。

在引言部分，将介绍三量子比特纠缠态的概念和背景，以及阐明本文的结构和目的。

接着在正文部分，将详细探讨三量子比特纠缠态的定义、性质和在量子信息领域的应用。

最后在结论部分，将总结三量子比特纠缠态的重要性，展望未来研究方向并得出结论。

整个文章的结构清晰明了，旨在深入探讨三量子比特纠缠态在量子信息科学中的重要作用和应用。

1.3 目的：本文旨在深入探讨三量子比特纠缠态的定义、性质以及在量子信息领域的应用。

通过对三量子比特纠缠态的研究，我们希望能够更全面地理解量子纠缠在量子信息领域的重要性，并探讨其在量子计算、量子通信以及量子密钥分发等方面的潜在应用。

通过本文的阐述，我们也希望能够为未来的研究提供一定的参考和启发，推动量子信息领域的发展和应用。

分的内容2.正文2.1 三量子比特纠缠态的定义三量子比特纠缠态是指由三个量子比特共同组成的量子态，其中这三个量子比特之间存在着纠缠关系。

在量子力学中，纠缠是指两个或多个量子系统之间存在一种特殊的关联关系，使它们的状态无法被单独描述，而只能通过整体来描述这些系统的状态。

具体地说，三量子比特纠缠态可以用以下形式表示：\left \psi\right\rangle = a \left 000\right\rangle + b \left111\right\rangle其中，a和b为复数，且满足a ^2 + b ^2 = 1。

这个纠缠态表示了三个量子比特之间的纠缠关系，即它们的状态无论如何测量，都不会独立于其他量子比特的状态。

Tavis Cummings模型中原子的纠缠度

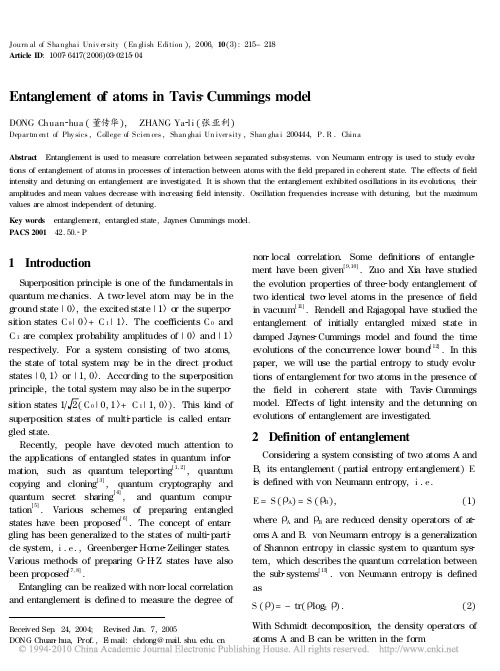
Journ al of Sha ngha i Univ er sity (En glish Edition ),2006,10(3):215-218Article ID :1007 6417(2006)03 0215 04Entanglement of atoms in Tavis Cummings modelDONG Chuan hua (董传华), ZHANG Ya li (张亚利)Depar tm ent of Phy sics ,College of Scien ces ,Shan ghai Un iver sity ,Shan gha i 200444,P .R .ChinaReceived Sep.24,2004; Revised Ja n.7,2005DO NG Chuan hua,Prof.,E mail:chdong@ma Abstract Entanglem ent is used to measure correlation between separated subsystems.von Neumann entropy is used to study evolu tions of entanglement of atoms in processes of interaction between atoms with the field prepared in coherent state.The effects of field intensity and detuning on entanglement a re investiga ted.It is shown that the entanglement exhibited osc illations in its evolutions,their amplitudes and mean values decrease with inc reasing field intensity.Oscillation frequenc ies increase with detuning,but the maximum values a re almost independent of detuning.Key words entanglem ent,entangled state,Jaynes Cummings model.PACS 2001 42.50. P1 IntroductionSuperposition principle is one of the fundamentals in quantum m echanics.A two level atom may be in the ground state |0 ,the excited state |1 o r the superpo sition states C 0|0 +C 1|1 .The coefficients C 0and C 1are complex probability amplitudes of |0 and |1 respectively.For a system consisting of two atoms,the state of total system may be in the direct p roduct states |0,1 or |1,0 .Acco rding to the superposition principle,the total system may also be in the superpo sition states 1 2(C 0|0,1 +C 1|1,0 ).This kind of superposition states of multi particle is called entan gled state.Recently,people have devoted much attention to the applications of entangled states in quantum information,such as quantum teleporting [1,2],quantumcopying and cloning [3],quantum cryptography andquantum secret sharing [4],and quantum computation [5].Various schemes of preparing entangledstates have been proposed [6].The concept of entan gling has been generalized to the states of multi parti cle system,i .e .,G reenberger Ho rne Zeilinger states.Various methods of preparing G H Z states have alsobeen proposed [7,8].Entangling can be realized with non local correlation and entanglement is defined to measure the degree ofnon local correlation.Some definitions of entanglement have been given [9,10].Zuo and Xia have studied the evolution properties of three body entanglement of two identical two level atoms in the presence of fieldin vacuum [11].Rendell and Rajagopal have studied the entanglement of initially entangled mixed state in damped Jaynes Cummings model and found the timeevolutions of the concurrence lower bound [12].In this paper,we will use the partial entropy to study evolu tions of entanglement for two atoms in the presence of the field in coherent state with Tavis Cummings model.Effects of light intensity and the detunning on evolutions of entanglement are investigated.2 Definition of entanglementConsidering a system consisting of two atoms A and B,its entanglement (partial entropy entanglement)E is defined with von Neumann entropy,i .e .E =S ( A )=S ( B ),(1)where A and B are reduced density operators of at oms A and B.von Neumann entropy is a generalization of Shannon entropy in classic system to quantum sys tem,which describes the quantum co rrelation betweenthe sub systems [13].von Neumann entropy is defined asS ( )=-tr( log 2 ).(2)With Schmidt decomposition,the density operato rs of atoms A and B can be written in the fo rmA=tr B( A B)=mj=12j|j A A!j|,B=tr A( A B)=mj=1 2j|j B B!j|,(3)where m∀min{dim H A,dim H B},H A and H B are the Hilbert subspaces of atoms A and B,respectively. It follows that A and B have m non zero eigenvalues j(j=1-m).The relation between E and j isE=-mj=1j log2 j.(4)For two atoms in the entangled states| AB=C1|1,1 +C2|1,0 +C3|0,1+C4|0,0 ,(5) the eigenvalues of A(or B)arej=121-(-1)j1-4|C1C4-C2C3|2,j=1or2.(6)The partial entropy entanglement can be calculated as follows.E=-( 1log2 1+ 2log2 2).(7) 3 Entanglement of two atoms in TavisCummings modelConsidering a system consisting of two level atoms A and B,the ground state and excited state are denot ed|0 and|1 respectively.The light field is initially prepared in the coherent state|! =nf n|n ,where f n=exp(- n 2)!n n!and n is mean photon num ber.Two atoms are in the entangled state|(0) ato m=1 2(|0,1 +|1,0 ).(8) This entangled state is one of Bell bases,which is maximally entangled state with entanglement E=1. The state evolves from the initial state to|(t) at time t>0|(t) =C1(t)|1,1;n-1 +C2(t)|1,0;n+C3(t)|0,1;n +C4(t)|0,0;n+1 ,(9) where the coefficients C j(t)(j=1-4)are to be de termined.In the interaction picture,the Hamiltonian of the system consisting of two atoms and a field in Tavis Cummings model is,H I=g(a+S-e i∀t+S+a e-i∀t),(10)where∀=#-#0,#0is the transiting frequency of at oms and#is the f requency of field,S+=S(A)++S(B)+, S-=S(A)-+S(B)-,and S(j)#(j=A or B)are the transit ing operato rs of atom A(or B).Substituting the inter action Hamiltonian Eq.(10)and the state vector Eq.(9)into the Schr dinger equation and solving it using a standard p rocedure with the initial condition Eq.(8), the coefficients C j(t),(j=1∃4)in Eq.(9)can be obtained as follows.C1(t)=-2n gm1∃1-∀[ei(∃1-∀)t-1]+m2∃2-∀[ei(∃2-∀)t-1]+m3∃3-∀[ei(∃3-∀)t-1],C2(t)=C3(t)=m1e i∃1t+m2e i∃2t+m3e i∃3t,C4(t)=-2n+1gm1∃1+∀[ei(∃1+∀)t-1]+m2∃2+∀[ei(∃2+∀)t-1]+m3∃3+∀[ei(∃3+∀)t-1],(11)wherem1=f n2∃2∃3+2(2n+1)g2(∃1-∃2)(∃1-∃3),m2=f n2∃1∃3+2(2n+1)g2(∃2-∃1)(∃2-∃3),m3=f n2∃1∃2+2(2n+1)g2(∃3-∃1)(∃3-∃2)(12)and∃1=2r1 3cos%,∃2=2r1 3cos(%+2& 3),∃3=2r1 3cos(%+4& 3),(13) r=[2(2n+1)g2+∀2]3 27,%=1 3arccos(-∀g2 r).(14) In the case of∀=0,C j(t)can be reduced toC1(t)=-i2nf n g sin(∋n t) ∋n,C2(t)=C3(t)=2-1 2f n cos(∋n t),C4(t)=-i2(n+1)f n g sin(∋n t) ∋n,(15) where Rabi frequency is2∋n and.(16)216J our nal of Shan gha i Univer sityThe reduced density operator of atom A can beobtainedA = n(|C 1|2+|C 2|2)|1 !1|+(|C 3|2+|C 4|2)|0 !0|+(C *2C 4+C *1C 3)|0 !1| +(C 2C *4+C 1C *3)|1 !0|,(17)its eigenvalues are =0.5(1#1-(),(18)where(=4[ n (|C 1|2+|C 2|2) n(|C 3|2+|C 4|2)- n(C 1C *3+C 2C *4)2].(19)Using Eq.(7),the evolutions of entanglement can be obtained,which are shown in Fig.1for the case of resonance.The atoms A and B are prepared initially in Bell base |1,0 +|0,1 ,so the initial entanglement is 1.In the processes of interaction between the atoms and the field,the state of the system consisting of at oms A and B will deviate from this Bell base.In case of resonance,the states will evolve to the state | (t ) at a time t >0,which can be rewritten as | (t ) =1 2 nf n {cos (∋n t )(|1,0;n +|0,1;n ) -i sin (∋n t )[1-1 (2n +1)|1,1n -1+1+1 (2n +1)|0,0;n +1 ]}=1 2 n f n {cos (∋n t )(|1,0;n +|0,1;n ) -i sin (∋n t )[(|1,1;n -1 +|0,0;n +1 )-1 (4n +2)(|1,1;n -1 -|0,0;n +1 )+%]}.(20)It can be seen from Eq.(20)that the other two Bellbases |1,1 #|0,0 appear in atom field interaction.These Bell bases evolve according to cosine and sine with frequency ∋n that is different for various pho ton numbers n .Superposing these Bell bases form the os cillations of entanglement in evolution. In a weaker field ( n <4),the valley values of oscil lations rise obviously with increasing field intensity so that the mean values of entanglements in the oscil lations increase (see Fig.1(a)-(c)).In a stronger field ( n >4),the mean values of entanglements de crease rapidly (see Fig.1(d)and (e)),and the am plitudes shrink sharply with enhancing the field.When n =15,entanglement is maintained roughly constant inevolution.Thus,enhancing the field makes the atomsdetangle.This can be explained by the fact that thequantum co rrelation is weakened due to enhancing the interaction of two atoms with the field respectively in a stronger field.Fig.1 Entanglement of two atoms in the case of resonanc e((a) n =0.2,(b) n =1.0,(c) n =4.0,(d) n =9.0,(e) n =15.0)Evolution of entanglement in off resonance are shown in Fig.2(for weaker field, n =0.2)and Fig.3(for stronger field, n =9).From these figures,it can be seen that oscillation frequencies increase and the amplitudes shrink with increasing detuning in a weaker field.Except these oscillations,there are slow varia tions with large amplitudes in evolution for a stronger field and large detuning.The minimum values rise in a weaker field (see Fig.2)and drop in a stronger field (see Fig.3)with increasing the detuning.The maxi mum values of entanglement in the evolution are inde pendent of detuning almost,while dependent only on the field intensity.In summary,in interactions between atoms and the field,entanglements of two atoms will oscillate due to the Rabi oscillations.The field intensity and the detun ing affect evolutions of atom entanglement.Entanglements of two atoms depend on the field in tensity.The mean entanglem ents increase slightly in a weak field and decrease obviously in a strong field217Vol.10 No.3 Jun.2006DONG C H,et al .: Entanglem ent of atom s in Tavis Cummings modelFig.2 Entanglement of two atoms in the case of off resonanc efor n =0.2((a)∀=0.5g ,(b)∀=2.0g ,(c)∀=4.0g ,(d)∀=10.0g ,(e)∀=20.5g)Fig.3 Entanglement of two atoms in the case of off resonanc efor n =9.0((a)∀=0,(b)∀=0.5g ,(c )∀=1.0g ,(d)∀=4.0g ,(e)∀=10g )with increasing field intensity.It follows that a strong field makes the atoms detangle.The amplitudes of oscillations of entanglements shrink sharply with in creasing field intensity.The maximum values of entan glement are independent of detuning but dependent on the field intensity.The oscillation frequencies increase and the amplitudes shrink in a weak field with the increasing detuning.References[1] Bennett C H,Wiesner S munication v ia one andtwo particle operators on Einstein Podolsky Rosen states [J].Phys .Rev .Lett .,1992,69(20):2881-2884.[2] Bennett C H,Brassard G,Cr peau C,et al .Teleportingan unknown quantum state via dual classical and Ein stein Podolsky Rosen c hannels [J].Phy s .Rev .Lett .,1993,70(13):1895-1898.[3] Buzek V,H illery M.Quantum copying:Beyond the nocloning theorem[J].Phys .Rev .,1996,A54(3):1844-1852.[4] Hillery M,Buzek V,Berthiaume A.Quantum secret sharing[J].Phys .Rev .,1999,A59(3):1829-1834.[5] Anders S rensen,Klaus M lme r.Entanglement and quantum computation with ions in the therm al motion [J ].Phys .Rev .,2000,A 62(2):022311 1-022311 11.[6] Cirace J I,Zoller P.Preparation of macroscopic superposition in many atom systems [J ].Phy s .Rev .,1994,A50(4):R2799-R2802.[7] Yao C M,Guo G C.Generation of spin type G HZ statesof the cavity field in squeezed coherent states[J].Acta Phy sica Sinica ,2001,50(1):59-62(in Chinese).[8] Liu X,Li H C.Prepa ration of multi atom GHZ states v iathe Raman interaction of V type three level atoms and one cavity field[J].Acta Phy sica Sin ica ,2001,50(9):1689-1692(in Chinese).[9] Vedral V,Plenio M B,Rippin M A,et al .Quantifyingentanglement[J].Phys .Rev .Lett .,1997,78(12):2275-2279.[10] Vedral V,Plenio M B.Entanglement measures and purification procedures[J].Phy s .Rev .,1998,A57:1619-1633.[11] Zhou Z C,Xia Y J.The evolution property of three bodyentanglement measure in Tavis Cumm ings model [J ].Acta Physica Sinica ,2003,52(11):2687-2693(in Chinese).[12] Rendell R W,Rajagopal A K.Revivals and entanglementfrom initially entangled mixed sta tes of a damped Jaynes Cumm ings model [J].Phys .Rev .,2003,A67(6):062110 1-062110 11.[13] von Neumann.Mathem atical F oundat ion s of Qua ntumMechan ics [M].Princeton University Press,Princeton,NJ,1955.(Editor YAO Yu e yuan )218J our nal of Shan gha i Univer sity。

三体系统量子纠缠度特性研究

三体系统量子纠缠度特性研究杨富社;王菊霞【摘要】Using the method of quantum calculation, the entanglement degrees of the three-body system, any two-body among the them, and the Tavis-Cummings model system are calculated in detail. The evolution property of three-body entangtement degree is showed via solving the Schrodinger equation of the atom-light field system. It is found that the entanglement degree of the atom-light field system shows the periodic oscillations with the time and the cycle is closely related to the initial state of the system. The entanglement degree in the three-body system has the same cycle as that of the any two-body in the three-body system. The system will evolve to a three-body maximum or partly entangled state from the initial non-entangled state.%应用量子计算方法,对三原子体系中三体问、两两间以及Tavis-Cummings模型系统的纠缠度进行了详细计算.通过求解原子与光场相互作用系统态矢满足的薛定谔方程,得出了该模型三体问纠缠度随时间的演化规律.结果表明:原子光场相互作用系统的纠缠度呈现随时问变化的周期振荡性,周期大小与系统初始状态有关.系统中三体间的纠缠度与三体中两两间纠缠度的振荡周期有关.随着时间的变化,体系由开始的非纠缠状态可演化为三体最大或部分纠缠态.【期刊名称】《陕西师范大学学报（自然科学版）》【年(卷),期】2011(039)001【总页数】5页(P33-37)【关键词】量子光学;纠缠态;三体纠缠;纠缠度【作者】杨富社;王菊霞【作者单位】长安大学,理学院,陕西,西安,710064;渭南师范学院,物理与电子工程系,量子光学与光子学研究所,陕西,渭南,714000【正文语种】中文【中图分类】O413.1自从纠缠态概念提出以来,人们已认识到纠缠态的重要性.为了定量描述纠缠的程度,引进纠缠度的概念,它是指纠缠态携带纠缠量的多少[1],从而可使不同纠缠态之间建立了可比关系.迄今,对纠缠度的具体量化定义形形色色,尚无普遍认可的统一化形式[2-11].1995年,Schlienz和M ahler提出了关于三体纠缠态纠缠量以及三体中两两间的纠缠量的计算公式[2];之后查新未[3]尝试给出相互独立的多体系统对应的量子纯态纠缠度定义;石名俊等人[4]又给出量子纯态纠缠度的几何解释;美国科学家Bennett提出了两种形式化但物理意义十分鲜明的定义[5],即生成纠缠EF(ρAB)与蒸馏纠缠ED(ρAB);波兰科学家 Horodecki和他的儿子提出了定义纠缠度应遵从的原则[6],即应遵从明显假定、基本假设、渐进性假设;Vedral等人给出几何意义非常鲜明的定义:纠缠度就是密度矩阵距离非纠缠集合的最小距离[7].关于量子纠缠态的度量虽然已提出一些相关描述的物理量,如 Von Neumann熵、纠缠相对熵[8]、密度算符之间的距离[9]、Rènyi纠缠度[10]和共生纠缠度(Concurrence)[11]等,但是人们仍未放弃寻找意义鲜明同时表达简单、易于求解的普遍的统一纠缠度定义.最先研究纠缠态判别问题并取得重要进展的是Peres[12],他给出了判别两个子系统的量子态为可分的必要条件;Ho rodecki还发现在束缚纠缠态中存在一种“纠缠激活”的有趣现象[13];Schlienz[14]和 Keller[15]也给出了不同的解释;阮曼奇、徐岗、曾谨言等提出[16]:对于二粒子体系而言,判断一个自旋态是否为纠缠态,最确切的判据是通过计算该自旋的单粒子约化密度的秩来确定.本文利用一种典型的纠缠度计算法,对三原子纠缠态、原子与光场相互作用系统的三体纠缠度进行较详细的计算,并定性分析纠缠度演化特性.1995年,Schlienz和M ahler提出的关于三体纠缠态纠缠量以及三体中两两间的纠缠量的计算公式[2]为可求解其相应状态的三原子间纠缠量以及三原子中任意两两间的纠缠量.在相互作用绘景中,T-C模型[17]中两个全同定态二能级原子与单模光场体系的相互作用哈密顿量为[18]从两种不同初态下的纠缠度的分析可以看出,原子与光场相互作用体系的纠缠量都呈现出明显的随时间变化的周期性振荡,周期的大小与体系的初始状态有关:初始状态不同,随时间变化过程中振荡的周期也不同.三体中两两间的纠缠量也呈现出周期性振荡,振荡周期与三体间纠缠量的振荡周期相等.随着时间的变化,体系由开始三体所处的非纠缠态(即t=0时,E3=0)可演化为三体最大纠缠态(即 E=1)以及部分纠缠态(即0＜E＜1).在计算三原子体系间的纠缠量以及三原子中任意两个原子间纠缠量大小的基础上,对原子与光场三体纠缠量的演化特性进行了分析.结果表明:无论初始原子处于EPR 纠缠态或非纠缠态,体系都将演化为三体最大或部分纠缠态,原子与光场体系的纠缠量呈现出明显的周期性振荡现象,周期的大小与光场及原子构成体系的状态有关,体系初始状态不同,演化过程中的周期大小不同.三体中两两间的纠缠量也呈现出周期性振荡,并且体系的三体纠缠量与三体中两两间的纠缠量的振荡周期相等.这对于多体纠缠程度量化问题起到了基础性指导作用,以便于有效的提高纠缠度,这将广泛应用于量子信息编码、量子信息传送和量子计算等量子信息光学和光场量子统计领域的研究之中.【相关文献】[1]Zhou Zhengwei,Guo Guangcan.Quantum entangled states[J].Physics,2000,29(11):695-699.[2]Schlienz J,Mahler G.Descrip tion of entanglement[J].Physical Review:A,1995,52(6):4396-4404.[3]Cha Xinwei.Entanglement of quantum pure states[J].西安邮电学院学报,2003,8(1):56-58.[4]Shi M ingjun,Du Jiangfeng,Zhu Dongpei.Entanglement of quantum pure states[J].物理学报,2000,49(5):825-829.[5]Bennett C H,Vincenzo D P,Smolin J A,et al.M ixedstate entanglement and quantum erro r correction[J].Physical Review:A,1996,54:3824-3851.[6]Horodecki M,Horodecki P,Horodecki R.Separability of mixed states:necessary and sufficient conditions[J].Physics Letters:A,1996,223(25):1-8.[7]Vedral V,Plenin M B,Rippin M A,et al.Quantifying entanglement[J].Physical Review Letters,1997,78:2275-2279.[8]Zheng Shibiao,Guo Guangcan.Efficient scheme for twoatom entanglement and quantum information p rocessing in cavity QED[J].Physical Review Letters,2000,85:2392-2395.[9]Knöll L,Orlow ski A.Distance between density operato r:App lication to the Jaynes-Commings model[J].Physical Review:A,1995,51:1622-1630.[10]Zheng Shibiao.One-step synthesis of multiatom Greenberger-Horne-Zeilinger states[J].Physical Review Letters,2001,87:2304041-2304044.[11]Christian F.R,Mark Riebe,Hartmut Häffner,et al.Control and Measurement of Three-Qubit Entangled States[J].Science,2004,304(4):1478-1480.[12]Peres A.Separability Criterion for Density Matrices[J].Physical ReviewLetters,1996,77:1413-1415.[13]Ho rodecki P,Ho rodecki M,Ho rodecki R.Bound Entanglement Can BeActivated[J].Physical Review Letters,1999,82:1056-1059.[14]Schlienz J,Mahler G.Descrip tion of entanglement[J].Physical Review:A,1995,52:4396-4404.[15]Keller T E,Rubin M H,Shih Y,et al.Theo ry of the three-photon entangledstate[J].Physical Review:A,1998,57:2076-2079.[16]Ruan Manqi,Xu Gang,Zeng Jinyan.The three kinds of types spin-maximum entangled state in two-particle system[J].中国科学:G辑,2003,33(5):411-415.[17]Peng Jinsheng,Li Gaoxiang.Introduction of Modern Quantum Op tics[M].北京:科学出版社,1996:81.[18]Zuo Zhanchun,Xia Yunjie.The evolution p roperty of three-body entanglement measure in Tavis-Cummings model[J].物理学报,2003,52(11):2687-2693.。

高斯型耦合Tavis-Cummings模型的场熵演化特性

邹艳
（．州学院物理系，山东德州２３２１德５０３；２山东省生物物理重点实验室，山东德州２３２）．５０３
摘要：利用全量子理论研究了高斯型耦合ＴｖｓＣｍｍｉｓ模型的量子纠缠特性，用数值计算方法讨论ａｉ — ｕｎｇ运
维普资讯
第２３卷第６期２００７年１２月
德州学院学报
ＪｕｎｌｏｚｏｉｅｓｔｏｒａｆＤｅｈｕＵｎｖｒｉｙ
ＶＯ．２３，Ｏ１Ｎ．６
Ｄｅ．．０７ｃ２０
高斯型耦合ＴｖｓＣｍｍｉｇ模型的场熵演化特性ａｉ－ｕｎｓ
的，而实际问题中，它可能是变化的，文献［０研究过原子沿具有驻波场的谐振腔腔轴运动时，１１研究过高斯型耦合Ｔ—ｃ模型的布居数反转和原子的偶极压缩特性；关于高文１］但
Ｐ— Ｐ２
．
１为腔内光场沿径向分布的有效半径，ｌ￣ｐ，（）Ｄ０当０一ｏ时，ｆ已减少为轴线处的１ｅ设￡／倍．一０时，原子位
于ｌ一２０，子运动的速度，０一Ｉ处原Ｄ —ｋｐ．ｇｏｋ为描述原子速度变化的常数．则
规律是相似的．
关键词：子光学；Ｔｖｓｕ量ａｉ—Ｃｍｍｉｇ模型；高斯型耦合系数；量子纠缠ｎｓ中图分类号：４１２０３．文献标识码：Ａ文章编号：１０９４（０７０ —０３Ｏ０４— ４４２０）６０３一４

Tavis—Cummings模型中原子间偶极相互作用对相对熵纠缠度的影响

摘要：用量子相对熵研究了耦合双原子与单模真空场相互作用系统的纠缠度，讨论了
原子间偶极相互作用对相对熵纠缠度的影响．究结果表明：研原子间纠缠与原子一间纠缠场
的时间演化相位相反．子间偶极一极相互作用的增强，原偶引起纠缠度的演化周期增大；子原
文章编号：００２７２０）２０７ —５１０ —４２（０７０ —０００
Ｔａｉ．ｍｍｉｓ模型中原子间偶极相互作用ｖｓＣｕｎｇ
对相对熵纠缠度的影响
曾可，双春，文
（．湖南大学计算机与通信学院，南长沙１湖４０８；．长沙学院应用物理与电子技术系，湖南长沙１０２２４００）１０３
维普资讯
第３４卷
第２期
湖
南科学版）
Ｖｏ．１３４．ＮＯ．２Ｆｅｂ．２００７
２７年２月００
ＪｕｎｌｆＨｕａｉｅｓｔ（ｔｒｌｃｎｅ）ｏｒａｏｎｎＵｎｖｒｉｙＮａｕａｉｃｓＳｅ
间纠缠度不变，原子一间纠缠度减少．而场
关键词：耦合双原子；单模真空场；纠缠度；对熵相
中图分类号：３．ｏ４１２文献标识码：Ａ
Ｉｆｕｎｅｏｈｐｌ— ｐｌｎｅａｔｏｅｗｅｎＡｔｍｓｏｈｎｌｅｃｆｔｅＤｉｏｅＤｉｏｅＩｔｒｃｉｎｂｔｅｏｎｔｅ

1、下载文档前请自行甄别文档内容的完整性，平台不提供额外的编辑、内容补充、找答案等附加服务。
2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
3、如文档侵犯您的权益，请联系客服反馈,我们会尽快为您处理(人工客服工作时间：9:00-18:30)。

# & " #:12 % ，（） ,9: ; ’% ! 1 ’) (! $ $! $ ’
(
)
（ 1 $! % " 1! " ） &（ 467 & # :12 ’% ，（<） $ % ）’ $ ’
$ （& "#） = !$ ! 其中’ ’ ! ) $
(
)
原子 ! 处于基 !"!" 初始状态为原子 # 处于激发态，态，光场为真空态初始时刻体系状态波函数为（%）〉’ " # ， #$ ， %〉 ) % 重复以上计算可得到（# 467 1 %） 1 & ! #） % "（ &（ ! 467 # % ）’ $ $ 8 （>）
第 ’" 卷第 %% 期 "(($ 年 %% 月（%%） %(((.$"U(V"(($V’" V"&I#.(#
物
理
学
报
W/)W PKXGY/W GYZY/W
[E84 ’"， ZE4 %%， ZE+?1F?L， "(($ !"(($ /N,24 PN9-4 GEA4
" " " " " " " " " " " " " " " " " " " " " " " " " " " " " " " " " " " " " " " " " " " " " " " " " " " " " " " " " " " " " " "
$
(
)
（$$） # （= &# * # （= &# *
$
&*
$
! #< &#
5
&*
5
），
（=）（?） )（ *）’ $ #，
$
（$*） &$
$
! #< &#
5
&$
5
），
（$5）
$$ 期
左战春等：012345678839:4 模型中三体纠缠态纠缠量的演化特性
!’%/
!（ "）# ! !，
$ （% " ! "
!
""
!
& $’ "!
(
""
(
） )
其中!% ，原子 ! 的约化密度算符 ) % 分别为原子 $、 $ !! 可以看出：原子反转粒子数呈现的振荡现象与原子间的耦合作用强度有关 ) 对于初始二原子处于纠缠态的情况，当原子间耦合作用与原子与场间耦合相比过弱时，原子反转粒子数呈现出周期振荡现象，但达不到最大反转数 ) 当耦合强度增大到一定值时，振荡消失，原子反转粒子数始终为零，原子相当于处在俘获状态；而对于初始二原子处于非纠缠态的情况，原子反转粒子数出现振荡幅度较大的周期振荡，并且反转数可达到最大和最小，即原子在激发态与基态间稳定跃迁 ) 这说明了原子间的耦合强度对原子的反转粒子数表现出非线性效应，另外也可得到原子反转粒子数与初始时原子与场体系的状态有关 )
!"#$%&’())$*+% 模型中三体纠缠态纠缠量的演化特性 !
左战春夏云杰!
（曲阜师范大学物理系，曲阜 "#$%&’）（"((" 年 %" 月 %" 日收到； "(($ 年 " 月 "’ 日收到修改稿）
研究两个全同二能级原子与单模场相互作用的 )*+,-./011,23- 模型中的量子纠缠 4 在场和两原子初始分别为真空场和纠缠或非纠缠状态下，得出体系状态将演化为三体近似 5 纠缠态 4 通过对纠缠量的计算得出：该三体近原子间及原子与场间的耦合系数、失谐程度、原子反转似 5 纠缠态纠缠量的演化特性将随三体所处的初始状态、粒子数的变化而变化 4 值得一提的是可得出原子间耦合作用强度对纠缠量的非线性效应，并且纠缠量展现出与原子反转粒子数一致的周期振荡现象 4
Байду номын сангаас
（!*）三体 + 纠缠态的纠缠量各种条件下随时间的演化行为如图 $ 至图 ( 所示 ) 由各图可以看出，该三体近似 + 纠缠态的纠缠量随时间的演化表现为周期性振荡行为，并且振荡特性与原子反转粒子数相一致 ) 为了与其进行比较，计算了原子 $ 和原子 ! 的反转粒子数并画出其随时间演化的曲线（如图 *） ) ）〈〉 #（ $ % % # $ !%$ $ ,〈 & $ !%$ & $ 〉 $
关键词：5 纠缠态，纠缠量，反转粒子数
,-’’：($&’，6"’(
缠程度问题已经得到了精确的描述和解决 4 但由于
%B 引
言
［%］
三体或多体系统的复杂性，至目前为止，人们对于三体或多体纠缠态的量化一直处于探讨之中 4 D?22?JJ 基础上发展而来的等人提出了多粒子纯态纠缠的精确和渐近测量方
条件给予证明 4 最近， MN?23 和 O0E 提出了一种产生两原子纠缠［%#］态的方案，并用来实现量子逻辑门和量子隐形传态 4 文献［%I］根据纠缠张量方法，提出了用于表示三体及三体中两两间的纠缠程度的物理量— — —纠缠量 4 本文根据该纠缠量的定义，对初始状态为二原子处于 7,2-J?,2.PE@E8-H9.QE-?1 （ 7PQ）纠缠态或非纠缠态两种情况，讨论了体系纠缠量随时间演化特性及其受原子的反转粒子数的影响 4
［%’］案 45E23 等人提出了潜在的多粒子纠缠测量4 ［%&］ KELE@?AH, 等人对于任意纠缠测量需满足的限定
在 C*92?-./011,23- 模型
（ )./）模型处理的是两个二能级原子 )*+,-./011,23与单模场相互作用的模型，目前对 )./ 模型的研究
［"，［6］ $］已经是硕果累累，黄春佳等人研究了定态双原
! ! ! （!’） # "（ $ $ ） , "（ ! $ ） , "（ " $），
〈 % ! !% % ! 〉,〈 & ! !% & ! 〉 #（ ! $ ）# ! !
! ! ! （!-） # "（ " $ ） , "（ ! $ ） , "（ $ $），
图$
第一种情况时体系的纠缠量随时间演化图! 第一种情况共振时体系的纠缠量随时间的演化
!
7.1*,8： 9:;,*< =>204 ?@04 A2
$<==
物
理
学
报
;$ 卷
（ " ! ""# ! " （ " ! ""$ ! " ! ! ! "!# ）! ! ! "!$ ）（，（#） !# ! ""#"!$ ! "!#""$ ）式中 $% 为原子的本征跃迁频率， $ 为腔场频率，和 & #〉分别为原子的激发态和基态， & "〉 "$# ’ & " # 〉〈 ## & ，〈 ## & ，〈 "# & " & ## 〉 "! # ’ & " # 〉 "" # ’ 〈 " # & 分别为原子 # 的赝自旋算符， & ## 〉 "$$ ’ & " $ 〉〈 #$ & ，〈 #$ & ，〈 "$ & 〈 "$ & " & #$ 〉 "! $ ’ & " $ 〉 "" $ ’ & # $ 〉分别为原子 $ 的赝自旋算符，" ， " ! 分别为光子的湮没和产生算符， ! 为原子( 光场间的耦合常量， # 为原子间偶极( 偶极相互作用强度 ) 光场 !"#" 初始状态为耦合双原子处于 $%& 纠缠态，为真空态初始时刻（ % ’ %）原子( 光场体系的状态波函数为 # （%）〉’ （ " # ，） #$ ， %〉! # # ， "$ ， %〉 ) （$） % $ ! 任意时刻的体系状态波函数为（ %）〉’ &（ #$ ， %〉! &（ #$ ， #〉 # % ） "# ， $ % ） ## ， % （*） "$ ， %〉 ) ! &（ * % ） ## ，在相互作用绘景中求解问题是方便的，将（ #）和（ *）式代入相互作用绘景中的 +,-./01234. 方程〉 ! （ %）（ %）〉， 1 ’ ’（ ! %% ( % ）% ! 求解该方程，并考虑到初始条件，则得到 1 & ! #） % "（ &（ # % ）’ &（ * % ）’ 467 $ 8 （5）
&（ * % ）’ " 8