Chapter 14 CHEMICAL EQUILIBRIUM - Glendale …
chemicalreactionengineering3ededition作者octavelevenspiel课后习题答案

Corresponding Solutions for Chemical Reaction EngineeringCHAPTER 1 OVERVIEW OF CHEMICAL REACTION ENGINEERING .......................................... 错误!未定义书签。
CHAPTER 2 KINETICS OF HOMOGENEOUS REACTIONS ........................................................ 错误!未定义书签。
CHAPTER 3 INTERPRETATION OF BATCH REACTOR DATA ..................................................... 错误!未定义书签。
CHAPTER 4 INTRODUCTION TO REACTOR DESIGN ............................................................... 错误!未定义书签。
CHAPTER 5 IDEAL REACTOR FOR A SINGLE REACTOR........................................................... 错误!未定义书签。
CHAPTER 6 DESIGN FOR SINGLE REACTIONS ....................................................................... 错误!未定义书签。
CHAPTER 10 CHOOSING THE RIGHT KIND OF REACTOR ....................................................... 错误!未定义书签。
Notes Equilibrium Le Châtelier’s Principle 注意

– An equlibrium position may be shown:
A
B or ABຫໍສະໝຸດ 1%99%99%
1%
✓Note the emphasis of the arrows direction
✓It depends on which side is favored; almost
all reactions are reversible to some extent
Reversible Chemical Reactions
• Reversible Reactions: Reactions that can occur forward & backward
• Forward: 2SO2(g) + O2(g) → 2SO3(g) • Reverse: 2SO2(g) + O2(g) ← 2SO3(g)
or
↔ 2SO2(g) + O2(g)
2SO3(g)
What is Chemical Equilibrium? • Chemical reactions are reversible:
–Reactants
Products
In a closed system, they reach equilibrium.
• so cooling an exothermic reaction will produce more product, and heating it would shift the reaction to the reactant side of the equilibrium: C + O2(g) → CO2(g) + 393.5 kJ
IG补课用Chapter 14 organic chemistry(完整版课件)

5.aliphatic(脂肪族的,无环的) alcohols and ketones (are named in a similar way to alkene)
CH3CH2CH2OH
propan-1-ol
H OH H H CCC H
H HH
propan-2-ol
CH3CH2COCH2CH3 pentan-3-one
Carboxylic acid 羧酸
ester 酯
benzene
-X,where X=F、Cl、 Br、I
chloromethane
-OH
methanol
ethanal
propanone
ethanoic
ethyl ethanoate
amine胺 nitrile腈
-NH2
methylamine
YCIC AS Organic Chemistry
2. π bond
① π bonds are formed by sideways overlap of p orbitals.原子轨道“肩 并肩”重叠。
② π bonds are not symmetrical about a line jointing the nuclei of the atoms forming the bond.
e.g ethane molecular formula: C2H6 Exercise: 1.propane 2.ethanoic acid
3.butanol
C3H8
C2H4O2
C4H10O
YCIC AS Organic Chemistry
3.Structural formula(结构式)
Denefition: the formula that tell us about the atoms bonded to each carbon atom in an
物理化学(傅献彩著)06章_化学平衡

若对Henry定律发生偏差,得
B ( T ,p ,x B )B * ( T ,p ) R T ln a x ,B
B* (T, p) 不是标准态化学势
B * (T ,p )B * (T ,p)p pV B d p B * (T ,p)
上一内容 下一内容 回主目录
返回
2021/5/27
6.2 化学反应的平衡常数和等温方程式
理想气体混合物反应系统
Kp
B
pB p
B e
rG mRTlnKp
K p 为理想气体混合物反应系统的标准平衡常数 它仅是温度的函数,压力已指定为标准压力
下标 “p” 表示是“压力商”,以区别于其他标 准平衡常数
rGm (T) 称为化学反应标准摩尔Gibbs自由能变化值, 仅是温度的函数。
上一内容 下一内容 回主目录
返回
2021/5/27
6.2 化学反应的平衡常数和等温方程式
对于任意反应
d D e E g G h H
rG m rG m ( T ) R T ln ( (f fG D / /p p) ) g d ( (f fH E / /p p) ) e h
溶液中反应的平衡常数
显然,
B ( T , p , x B ) B ( T , p , m B ) B ( T , p , c B )
但是
x,B (T )m ,B (T )c,B (T )
因为对数项中的数值也都不相等。
上一内容 下一内容 回主目录
返回
2021/5/27
溶液中反应的平衡常数
上一内容 下一内容 回主目录
返回
14 Chemical Equilibrium - Science:14化学平衡的科学
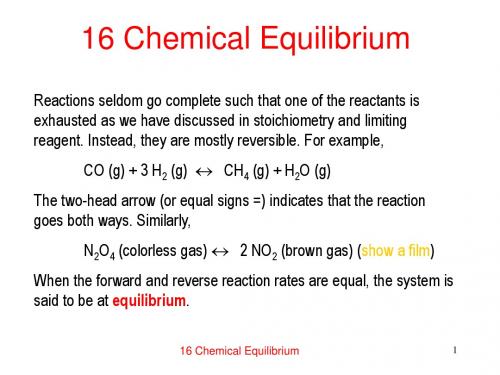
16 Chemical Equilibrium
6
The reaction quotients
For any chemical reaction in a system, aA+ b B c C + d D we can always write a quotient, [C]c [D]d Q = —————— [A]a [B]b for example, Know the definition of Q. Text: 16-5 defines Q & 16-1 defines K.
16 Chemical Equilibrium
5
Some Typical Equilibria
Macroscopic properties such as vapor pressure, solubility, distribution of a substance in two immiscible liquids are typical equilibria. Vapor pressure of a liquid is the pressure of the vapor in equilibrium with a liquid. It is a function of temperature. Solubility, amount in solution in equilibrium with solid, depends on the substance and the solvent, often also on temperature. Distribution (or equilibrium) coefficients of a substance in immiscible liquids are useful guides for the separation of mixtures into components.
有机化学 Chapter 14 organometal

§14.3 Preparation of organometallic compounds
A, Organolithium compounds
B, Organomagnesium compounds (Grignard reagent)
Aryl and vinyl chlorides don’t form Grignard reagents in diethyl ether. However, when THF is used as the solvent, they are okay.
2. Alkane synthesis
Primary alkyl halides, especially iodides, are the best substrates
Secondary and tertiary halides encounter elimination side reaction
§14.4 Organolithium & organomagnesium compounds as Brønsted bases
Organolithium and organomagnesium compounds are stable species They are strongly basic, and react instantly with proton donors even alcohol and water
Example:
环丙基锂
二乙基镁
When the metal bears a substituent other than carbon, the substituent is treated as if it were an anion and named separately:
合成治疗骨质疏松药物依普黄酮

O
f
Company Name
分布反应的机理讲解三
步 骤 ( 5) 烯 醚 化 反 应
LOGO
BocHN
OH
BocHN
O
O
f
步 骤 ( 6) Ring Closing Metathesis (RCM)反 应
O
g
BocHN
O
BocHN
O
O
g
O h
Company Name
O
H2O H
HO
O
O
O
j
步 骤 ( 10) : SN1取 代 反 应
k
HO
O
(CH3)2CHBr
O
O
O
O
k
l
Company Name
创新与突破
反应简单快捷
LOGO
有效实现了在空气中稳定, 并可以在常 温和常压下施催化的 体系
更加简单易行
无害溶剂 低毒废物
副产品更少
步 骤 ( 3) 醛 与 格 氏 试 剂 制 备 仲 醇 ( 亲 核 加 成 )
LOGO
BocHN
OH H O
b d
+
BocHN MgBr
OH
OH
e
步 骤 ( 4) 选 择 性 氧 化 β-不饱和醇
BocHN
OH
Acc(NH4Cl CrO3) BocHN
OH
OH
e
Ring Closing Metathesis(RCM)反应
——烯烃关环复分解反应 金属卡宾化合物
LOGO
Company Name
分布反应的机理讲解四
步 骤 ( 7) : 氨 基 保 护 基 的 脱 保 护
chemical_reaction_engineering(答案)

Corresponding Solutions for Chemical Reaction EngineeringCHAPTER 1 OVERVIEW OF CHEMICAL REACTION ENGINEERING ..................... 错误!未定义书签。
CHAPTER 2 KINETICS OF HOMOGENEOUS REACTIONS ............................. 错误!未定义书签。
CHAPTER 3 INTERPRETATION OF BATCH REACTOR DATA .......................... 错误!未定义书签。
CHAPTER 4 INTRODUCTION TO REACTOR DESIGN ................................ 错误!未定义书签。
CHAPTER 5 IDEAL REACTOR FOR A SINGLE REACTOR ............................ 错误!未定义书签。
CHAPTER 6 DESIGN FOR SINGLE REACTIONS ................................... 错误!未定义书签。
CHAPTER 10 CHOOSING THE RIGHT KIND OF REACTOR ........................... 错误!未定义书签。
CHAPTER 11 BASICS OF NON-IDEAL FLOW ..................................... 错误!未定义书签。
CHAPTER 18 SOLID CATALYZED REACTIONS .................................... 错误!未定义书签。
Chapter 1 Overview of Chemical Reaction Engineering1.1 Municipal waste water treatment plant. Consider a municipal water treatment plant for a small community Waste water, 32000 m 3/day, flows through the treatment plant with a mean residence time of 8 hr, air is bubbled through the tanks, and microbes in the tank attack and break down the organic material (organic waste) +O 2 −−−→−microbes CO 2 + H 2OA typical entering feed has a BOD (biological oxygen demand) of 200 mg O 2/liter, while the effluent has a megligible BOD. Find the rate of reaction, or decrease in BOD in the treatment tanks.FigureSolution:)/(1017.2)/(75.183132/100010001)0200()(313200031320001343333s m mol day m mol day molgm L mg g L mg day day m dayday m VdtdN r AA ⋅⨯=⋅=-⨯⨯⨯-⨯-=-=--1.2 Coal burning electrical power station. Large central power stations (about 1000 MW electrical) using fluiding bed combustors may be built some day (see These giants would be fed 240 tons of coal/hr (90% C, 10%H 2), 50% of whichWaste water3Clean water3200 mg O 2Zero O 2would burn within the battery of primary fluidized beds, the other 50% elsewhere in the system. One suggested design would use a battery of 10 fluidized beds, each 20 m long, 4 m wide, and containing solids to a depth of 1 m. Find the rate of reaction within the beds, based on the oxygen used.Solution:380010)1420(m V =⨯⨯⨯=)/(9000101089.05.01024033hr bed molc hrkgckgcoal kgc hr coal t N c ⋅-=⨯-=⨯⨯⨯-=∆∆ )/(25.111900080011322hr m kmolO t N V r r c c O ⋅=-⨯-=∆∆-=-=)/(12000412000190002hr bed mol dt dO ⋅=+⨯= )/(17.4800)/(105.113422s m mol hr bed mol dt dO V r O ⋅=⋅⨯==-Chapter 2 Kinetics of Homogeneous ReactionsA reaction has the stoichiometric equation A +B =2R . What is the order of reactionSolution: Because we don’t know whether it is an elementary reaction or not, we can’t tell the index of th e reaction.2.2 Given the reaction 2NO 2 + 1/2 O 2 = N 2O 5 , what is the relation between therates of formation and disappearance of the three reaction components Solution: 522224O N O NO r r r =-=-2.3 A reaction with stoichiometric equation A + B = R + S has the followingrate expression-r A = 2 AC BWhat is the rate expression for this reaction if the stoichiometric equation is written asA + 2B = 2R + SSolution: No change. The stoichiometric equation can’t effect the rate equation, so it doesn’t chang e.For the enzyme-substrate reaction of Example 2, the rate of disappearance ofsubstrate is given by-r A =A06]][[1760C E A + , mol/m 3·sWhat are the units of the two constants Solution: ][]6[]][][[][03A A C E A k s m mol r +=⋅=-3/][]6[m mol C A ==∴sm mol m mol m mol s m mol k 1)/)(/(/][3333=⋅⋅=2.5 For the complex reaction with stoichiometry A + 3B → 2R + S and with second-order rate expression-r A = k 1[A][B]are the reaction rates related as follows: r A = r B = r R If the rates are notso related, then how are they related Please account for the sings , + or - .Solution: R B A r r r 2131=-=-A certain reaction has a rate given by-r A = C2 A , mol/cm 3·minIf the concentration is to be expressed in mol/liter and time in hours,what would be the value and units of the rate constant Solution:min)()(3'⋅⨯-=⋅⨯-cm molr hr L mol r A A 22443'300005.0106610)(minAA A A A C C r r cm mol mol hr L r =⨯⨯=⋅⨯=-⋅⋅⋅=-∴ AA A A A C C cmmol mol L C cmmolC L mol C 33'3'10)()(=⋅⋅=∴⨯=⨯2'42'32'103)10(300300)(AA A A C C C r --⨯=⨯==-∴ 4'103-⨯=∴kFor a gas reaction at 400 K the rate is reported as-dtdp A= p2 A, atm/hr (a) What are the units of the rate constant(b) What is the value of the rate constant for this reaction if the rateequation is expressed as-r A = - dtdN V A1 = k C2 A , mol/m 3·sSolution:(a)The unit of the rate constant is ]/1[hr atm ⋅ (b)dtdN V r AA 1-=-Because it’s a gas reaction occuring at the fined terperatuse, so V=constant, and T=constant, so the equation can be reduced to22)(66.366.3)(1RT C RTP RT dt dP RT dt dP VRT V r A A A A A ==-=-=-22)66.3(A A kC C RT ==So we can get that the value of1.12040008205.066.366.3=⨯⨯==RT kThe pyrolysis of ethane proceeds with an activation energy of about 300 kJ/mol.How much faster the decomposition at 650℃ than at 500℃ Solution:586.7)92311731()10/(314.8/300)11(3211212=-⋅⋅=-==KK K mol kJ mol kJ T T R E k k Ln r r Ln7.197012=∴r rIn the mid-nineteenth century the entomologist Henri Fabre noted that Frenchants (garden variety) busily bustled about their business on hot days but were rather sluggish on cool days. Checking his results with Oregon ants, I findRunning speed, m/hr150160230295370Temperature, ℃1316222428What activation energy represents this change in bustliness Solution:RTE RTE RTE ek eak t cons ion concentrat f let ion concentrat f ek r ---=⋅⋅=⋅='00tan )()(RET Lnk Lnr A 1'-=∴ Suppose Tx Lnr y A 1,==, so ,REslope -= intercept 'Lnk =)/(1-⋅h m r A150 160 230 295 370 A LnrC T o /13 16 22 24 28 3101-⨯T-y = x +Also K REslope 9.5147-=-=, intercept 'Lnk == , mol kJ K mol J K E /80.42)/(3145.89.5147=⋅⨯-=Chapter 3 Interpretation of Batch Reactor DataIf -r A = - (dC A /dt) = mol/liter·sec when C A = 1 mol/liter, what is the rateof reaction when C A = 10 mol/liter Note: the order of reaction is not known.Solution: I nformation is not enough, so we can’t answer this kind of question.3.2 Liquid a sedomposes by first-order kinetics, and in a batch reactor 50%of A is converted in a 5-minute run. How much longer would it take to reach 75% conversionSolution: Because the decomposition of A is a 1st -order reaction, so we can express the rate equation as:A A kC r =-We know that for 1st -order reaction, kt C C LnAAo=, 11kt C C LnA Ao =, 22kt C CLn A Ao = Ao A C C 5.01=, Ao A C C 25.02=So 21)24(1)(11212Ln kLn Ln k C C Ln C C Ln k t t A Ao A Ao =-=-=- equ(1) min 521)(111===Ln kC C Ln k t A Ao equ(2) So m in 5112==-t t t3.3 Repeat the previous problem for second-order kinetics. Solution: We know that for 2nd -order reaction,kt C C A A =-011,So we have two equations as follow:min 511211101k kt C C C C C AoAo Ao A A ===-=-, equ(1) 2123)1(31411kt kt C C C C C AoAo Ao Ao A ===-=-, equ(2) So m in 15312==t t , m in 1012=-t tA 10-minute experimental run shows that 75% of liquid reactant is converted to product by a 21-order rate. What would be the fraction converted in a half-hour run Solution: In a-21order reaction: 5.0AA A kC dt dC r =-=-, After integration, we can get:5.015.02A Ao C C kt -=, So we have two equations as follow:min)10(5.0)41(15.05.05.05.015.0k kt C C C C C Ao Ao AoA Ao ===-=-, equ(1) min)30(25.025.0k kt C C A Ao ==-, equ(2)Combining these two equations, we can get:25.05.1kt C Ao =, but this means 05.02<A C ,which is impossible, so we can conclude that less than half hours, all the reactant is consumed up. So the fraction converted 1=A X .In a hmogeneous isothermal liquid polymerization, 20% of the monomer disappears in 34 minutes for initial monomer concentration of and also for mol/liter. What rate equation represents the disappearance of the monomer Solution: The rate of reactant is independent of the initial concentration of monomers, so we know the order of reaction is first-order,monomer monomer kC r =-And k C C Lnoomin)34(8.0= 1min 00657.0-=kmonomer monomer C r )min 00657.0(1-=-After 8 minutes in a batch reactor, reactant (C A0 = 1 mol/liter) is 80% converted; after 18 minutes, conversion is 90%. Find a rate equation to represent this reaction. Solution:In 1st order reaction, 43.1511111111212==--=Ln Ln X Lnk X Ln k t t A A , dissatisfied.In 2nd order reaction, 49/4/912.0111.01)11(1)11(11212==--=--=Ao Ao Ao Ao Ao Ao Ao A Ao A C C C C C C C C k C C k t t, satisfied.According to the information, the reaction is a 2nd -order reaction.nake-Eyes Magoo is a man of habit. For instance, his Friday evenings are all alike —into the joint with his week’s salary of $180, steady gambling at “2-up” for two hours, then home to his family leaving $45 behind. Snake Eyes’s betting pattern is predictable. He always bets in amounts proportional to his cash at hand, and his losses are also predictable —at a rate proportional to his cash at hand. This week Snake-Eyes received a raise in salary, so he played for three hours, but as usual went home with $135. How much was his raise Solution:180=Ao n , 13=A n , h t 2=,135'=A n , h t 3;=, A A kn r α-So we obtain kt n n LnAAo=, ''')()(tn n Ln t n n Ln AAo A Ao= 3135213180'Ao n Ln Ln =, 28'=AnThe first-order reversible liquid reaction A ↔ R , C A0 = mol/liter, C R0=0takes place in a batch reactor. After 8 minutes, conversion of A is % while equilibrium conversion is %. Find the equation for the this reaction. Solution: Liquid reaction, which belongs to constant volume system, 1st order reversible reaction, according to page56 eq. 53b, we obtain121112102110)(1)(-+-+=+-==⎰⎰AX A A tX k k k k Lnk k X k k k dX dt t Amin 8sec 480==t Θ, 33.0=A X , so we obtain eq(1)33.0)(1min8sec 480211121k k k k Ln k k +-+= eq(1) Ae AeAe c X X M C C k k K -+===1Re 21, 0==AoRo C C M , so we obtain eq(2) 232132121=-=-==AeAe c X X k k K ,212k k =∴ eq(2)Combining eq(1) and eq(2), we obtain1412sec 108.4m in 02888.0---⨯==k 14121sec 1063.9m in 05776.02---⨯===k kSo the rate equation is )(21A Ao A AA C C k C k dtdC r --=-=- )(sec 1063.9sec 108.401414A A A C C C -⨯-⨯=----Aqueous A reacts to form R (A→R) and in the first minute in a batch reactorits concentration drops from C A0 = mol/liter to C Af = mol/liter. Find the rate equation from the reaction if the kinetics are second-order with respect to A.Solution: It’s a irreversible second -order reaction system, according to page44 eq 12, we obtainmin 103.2197.111⋅=-k , so min015.01⋅=mol Lkso the rate equation is 21)min 015.0(A A C r -=-At room temperature sucrose is hydrolyzed by the catalytic action of the enzymesucrase as follows:Aucrose −−→−sucraseproductsStarting with a sucrose concentration C A0 = millimol/liter and an enzymeconcentration C E0= millimol/liter, the following kinetic data are obtained in a batch reactor (concentrations calculated from optical rotation measurements):Determine whether these data can be reasonably fitted by a knietic equationC A ,millimol/lite rt,hr1234567891011of the Michaelis-Menten type, or -r A =MA E A C C C C k +03 where C M = Michaelis constantIf the fit is reasonable, evaluate the constants k 3 and C M . Solve by the integral method.Solution: Solve the question by the integral method:AA M A A Eo A A C k Ck C C C C k dt dC r 5431+=+=-=-, M Eo C C k k 34=, MC k 15= AAo A AoA Ao C C C C Ln k k k C C t -⋅+=-4451hr t ,A C ,mmol/LA Ao AAo C C C C Ln-AAo C C t-1 2 3 4 5 6 7 8 9 10 11Suppose y=A Ao C C t-, x=AAo A Ao C C C C Ln-, thus we obtain such straight line graph9879.0134===Eo M C k C k Slope , intercept=0497.545=k k So )/(1956.00497.59879.015L mmol k C M ===, 14380.1901.09879.01956.0-=⨯==hr C C k k Eo MEnzyme E catalyzes the transformation of reactant A to product R as follows:A −−→−enzymeR, -r A = min22000⋅+liter molC C C A E AIf we introduce enzyme (C E0 = mol/liter) and reactant (C A0 = 10mol/liter) into a batch rector and let the reaction proceed, find the time needed for the concentration of reactant to drop to mol/liter. Note that the concentration of enzyme remains unchanged during the reaction.. Solution:510001.020021+=⨯+=-=-AA A A A C C C dC dt r Rearranging and integrating, we obtain:10025.0025.010)(510)510(⎥⎦⎤⎢⎣⎡-+=+-==⎰⎰A Ao A Ao A A t C C C C Ln dC C dt t min 79.109)(5025.01010=-+=A Ao C C Lnand . Jungers, Bull. soc. chim. France, 386(1957), present the data in Table on thereaction of sulfuric acid with diethylsulfate in a aqueous solutionat ℃:H 2SO 4 + (C 2H 5)2SO 4 → 2C 2H 5SO 4HInitial concentrations of H 2SO 4 and (C 2H 5)2SO 4 are each mol/liter. Find a rate equation for this reaction.Tablet, minC 2H 5SO 4H,mol/litert, minC 2H 5SO 4H,mol/liter0 0 180 41 194 48 212 55 267 75 318 96 368 127 379 146 410 162∞ Solution: It’s a constant -volume system, so we can use X A solving the problem: i) We postulate it is a 2nd order reversible reaction system R B A 2⇔+ The rate equation is: 221R B A A A C k C C k dtdC r -=-=- L mol C C Bo Ao /5.5==, )1(A Ao A X C C -=,A A Ao BoBC X C C C =-=, A Ao R X C C 2=When ∞=t , L mol X C C Ae Ao /8.52Re == So 5273.05.528.5=⨯=Ae X , L mol X C C C Ae Ao Be Ae /6.2)5273.01(5.5)1(=-⨯=-== After integrating, we obtaint C X k X X X X X LnAo AeA Ae A Ae Ae )11(2)12(1-=--- eq (1)The calculating result is presented in following Table.t ,min L mol C R /,L mol C A /,A XA Ae A Ae Ae X X X X X Ln---)12( )1(AeA X XLn -0 0 0 0 0 41 48 55 75 96 127 146 162 180 194 212 267 318 368 379410 ∞——Draw AAe AAe Ae X X X X X Ln---)12(~ t plot, we obtain a straight line:0067.0)11(21=-=Ao AeC X k Slope , min)/(10794.65.5)15273.01(20067.041⋅⨯=⨯-=∴-mol L kWhen approach to equilibrium, BeAe c C C C k k K 2Re 21==, so min)/(10364.18.56.210794.642242Re 12⋅⨯=⨯⨯==--mol L C C C k k Be Ae So the rate equation ism in)/()10364.110794.6(244⋅⨯-⨯=---L mol C C C r R B A Aii) We postulate it is a 1st order reversible reaction system, so the rate equation isR A AA C k C k dtdC r 21-=-=- After rearranging and integrating, we obtaint k X X X Ln AeAe A '11)1(=-eq (2) Draw )1(AeAX X Ln -~ t plot, we obtain another straight line:0068.0'1-==AeX k Slope ,So 13'1m in 10586.35273.00068.0--⨯-=⨯-=k133Re '1'2min 10607.18.56.210586.3---⨯-=⨯⨯-==C C k k AeSo the rate equation ism in)/()10607.110586.3(33⋅⨯+⨯-=---L mol C C r R A AWe find that this reaction corresponds to both a 1st and 2nd order reversible reaction system, by comparing eq.(1) and eq.(2), especially when X Ae = , the two equations are identical. This means these two equations would have almost the same fitness of data when the experiment data of the reaction show that X Ae =.(The data that we use just have X Ae = approached to , so it causes to this.)In the presence of a homogeneous catalyst of given concentration, aqueous reactant A is converted to product at the following rates, and C A alone determinesthis rate:C A ,mol/liter 1 2 4 6 7 9 12 -r A , mol/liter·hrWe plan to run this reaction in a batch reactor at the same catelyst concentration as used in getting the above data. Find the time needed to lower the concentration of A from C A0 = 10 mol/liter to C Af = 2 mol/liter.Solution: By using graphical integration method, we obtain that the shaped area is 50 hr.The thermal decomposition of hydrogen iodide 2HI → H 2 + I 2 is reported by [ as follows:T,℃ 508 427 393 356 283 k,cm 3/mol ·s×10-6×10-6Find the complete rate equation for this reaction. Use units of joules, moles, cm 3, and seconds. According to Arrhenius’ Law,k = k 0e -E/R Ttransform it,0 4 8 1216 20 02468101214Ca-1/Ra- In(k) = E/R·(1/T) -In(k)Drawing the figure of the relationship between k and T as follows:From the figure, we getslope = E/R = intercept = - In(k) =E = 60851 J/mol k= 105556 cm3/mol·sFrom the unit [k] we obtain the thermal decomposition is second-order reaction, so the rate expression is- rA = 105556e-60851/R T·CA2Chapter 4 Introduction to Reactor DesignGiven a gaseous feed, C A0 = 100, C B0 = 200, A +B→ R + S, X A = . Find X B ,C A ,C B . Solution: Given a gaseous feed, 100=Ao C , 200=Bo C , S R B A +→+0=A X , find B X , A C , B C0==B A εε, 202.0100)1(=⨯=-=A Ao A X C C4.02008.01001=⨯⨯==Bo A Ao B C X bC X 1206.0200)1(=⨯=-=B Bo B X C CGiven a dilute aqueous feed, C A0 = C B0 =100, A +2B→ R + S, C A = 20. Find X A , X B , C B .Solution: Given a dilute aqueous feed, 100==Bo Ao C C ,S R B A +→+2, 20=A C , find A X , B X , B CAqueous reaction system, so 0==B A εε When 0=A X , 200=V When 1=A X , 100=VSo 21-=A ε, 41-==Ao Bo A B bC C εε8.01002011=-=-=Ao A A C C X , 16.11008.010012>=⨯⨯=⋅=Bo A Ao B C X C a b X , which is impossible. So 1=B X , 100==Bo B C CGiven a gaseous feed, C A0 =200, C B0 =100, A +B→ R, C A = 50. Find X A , X B , C B .Solution: Given a gaseous feed, 200=Ao C , 100=Bo C ,R B A →+, 50=A C .find A X , B X , B C75.02005011=-=-=Ao A A C C X , 15.1>==BoAAo B C X bC X , which is impossible. So 100==Bo B C CGiven a gaseous feed, C A0 = C B0 =100, A +2B→ R, C B = 20. Find X A , X B , C A . Solution: Given a gaseous feed, 100=+Bo Ao C C ,R B A →+2, 20=Bo C , Find A X , B X , A C0=B X , 200100100=+=B A V ,1=B X 15010050=+=R A V25.0200200150-=-=B ε, 5.01002110025.0-=⨯⨯-=-A ε842.02025.010020100=⨯--=B X , 421.0100842.010021=⨯⨯=A X34.73421.05.01421.0110011=⨯--⨯=+-=A A A AoA X X C C εGiven a gaseous feed, T 0 =1000 K, π0=5atm, C A0=100, C B0=200, A +B→5R,T =400K, π=4atm, C A =20. Find X A , X B , C B .Solution: Given a gaseous feed, K T o 1000=, atm 50=π, 100=Ao C , 200=Bo CR B A 5→+, K T 400=, atm 4=π, 20=A C , find A X , B X , B C .1300300600=-=A ε, 2==Ao Bo AB bC C a εε, 5.0410********=⨯⨯=ππT T According to eq page 87,818.05.010020115.0100201110000=⨯⨯+⨯-=+-=ππεππT T C C T T C C X Ao A AAo A A409.0200818.0100=⨯==Bo A Ao B aC X bC X130818.011200)818.0100200(1)(0=⨯+⨯-=+-=A A Ao A Ao Bo B X C T T X a b C C C εππA Commercial Popcorn Popping Popcorn Popper. We are constructing a 1-literpopcorn to be operatedin steady flow. First tests in this unit show that 1 liter/min of raw corn feed stream produces 28 liter/minof mixed exit stream. Independent tests show that when raw corn pops its volumegoes from 1 to 31.With this information determine what fraction of raw corn is popped in theunit.Solution: 301131=-=A ε, ..1u a C Ao =, ..281281u a C C Ao A == %5.462813012811=⨯+-=+-=∴AA Ao A Ao A C C C C X εChapter 5 Ideal Reactor for a single ReactorConsider a gas-phase reaction 2A → R + 2S with unknown kinetics. If a spacevelocity of 1/min is needed for 90% conversion of A in a plug flow reactor, find the corresponding space-time and mean residence time or holding time of fluid in the plug flow reactor. Solution: min 11==sτ, Varying volume system, so t can’t be found.In an isothermal batch reactor 70% of a liquid reactant is converted in 13min. What space-time and space-velocity are needed to effect this conversion in a plug flow reactor and in a mixed flow reactor Solution: Liquid reaction system, so 0=A ε According to on page 92, min 130=-=⎰AX AAAo r dC C t , AAAo A A Ao R F M r X C r C C -=--=..τ, R F M ..τ can’t be certain. , ⎰-=AX AAAo R F P r dX C 0..τ, so m in 13...==R B R F P t τWe plan to replace our present mixed flow reactor with one having double thebolume. For the same aqueous feed (10 mol A/liter) and the same feed rate find the new conversion. The reaction are represented byA → R, -r A = ASolution: Liquid reaction system, so 0=A εA A Ao Ao r X C F V -==τ, 5.1)]1([)(A Ao A A Ao A Ao X C k X r C C C -=--Now we know: V V 2=', Ao Ao F F =', Ao Ao C C =', 7.0=A X So we obtain5.15.15.15.1)1()2)1(2A Ao A A Ao A Ao Ao X kC X X kC X F VF V -='-'==''52.8)7.01(7.02)1(5.15.1=-⨯='-'∴A AX X 794.0='A XAn aqueous feed of A and B (400liter/min, 100 mmol A/liter, 200 mmol B/liter)is to be converted to product in a plug flow reactor. The kinetics of the reaction is represented byA +B→ R, -r A = 200C A C Bmin⋅liter molFind the volume of reactor needed for % conversion of A to product. Solution: Aqueous reaction system, so 0=A εAccording to page 102 ,⎰⎰-=-==Af AfX AA X A A AoAo Ao r dX r dC C C t F V 001⎰-==AfX AAAo or dX C Vντ, m in /400liter o =ν, L r dX r dX C V AAX A A o Ao Af3.1244001.0999.000=-⨯=-=∴⎰⎰ν5.9 A specific enzyme acts as catalyst in the fermentation of reactant A. Ata given enzyme concentration in the aqueous feed stream (25 liter/min) find the volume of plug flow reactor needed for 95% conversion of reactant A (C A0 =2 mol/liter ). The kinetics of the fermentation at this enzyme concentration is given byA −−→−enzymeR , -r A = litermolC C A A ⋅+min 5.011.0Solution: according to page 102 , aqueous reaction, 0=ε⎰-=A X AA Ao r dX F V 0 )11(21251.05.010A AX A A A Ao X X Ln dX C C F V A+-⨯=+=∴⎰\L Ln4.986)95.005.01(125=+=Enzyme E catalyses the fermentation of substrate A (the reactant) to productR. Find the size of mixed flow reactor needed for 95% conversion of reactant in a feed stream (25 liter/min ) of reactant (2 mol/liter) and enzyme. The kinetics of the fermentation at this enzyme concentration are given byA −−→−enzymeR , -r A = litermolC C A A ⋅+min 5.011.0Solution: min /25L o =ν, L mol C Ao /2=, m in /50mol F Ao =, 95.0=A X Constant volume system, so we obtainmin 5.199205.05.01205.01.095.02=⨯⨯+⨯⨯⨯=-==AAAo or X C Vντ,39875.4min /25min 5.199m L V o =⨯==τν5.14 A stream of pure gaseous reactant A (C A0 = 660 mmol/liter) enters a plugflow reactor at a flow rate of F A0 = 540 mmol/min and polymerizes the as follows3A → R, -r A = 54min⋅liter mmolHow large a reactor is needed to lower the concentration of A in the exit stream to C Af = 330 mmol/literSolution: 321131-=-=A ε, 75.0660330321660330111=⨯--=+-=Ao A A Ao A A C C C C X ε 0-order homogeneous reaction, according to page 103A Ao AoAooX C F VC kV kk ===ντ So we obtainL X k C C F V A Ao Ao Ao 5.75475.05401=⨯==Gaseous reactant A decomposes as follows:A → 3 R, -r A = C AFind the conversion of A in a 50% A – 50% inert feed (υ0 = 180 liter/min, C A0 =300 mmol/liter) to a 1 m 3 mixed flow reactor. Solution: 31m V =, 1224=-=A ε According to page 91 , AAAoAAo AAAo oX X C X C r X C V+-=-==116.0ντmin/1801000)1(6.0)1(L LX X X A A A =-+=So we obtain 667.0=A XChapter 6 Design for Single ReactionsA liquid reactant stream (1 mol/liter) passes through two mixed flow reactorsin a series. The concentration of A in the exit of the first reactor is mol/liter. Find the concentration in the exit stream of the second reactor.The reaction is second-order with respect to A and V2/V1=2.Solution:V 2/V1= 2, τ1 =011υV=AAArCC--10 ,2τ =022υV=221AAArCC--CA0=1mol/l , CA1=l ,0201υυ=, -r A1=kC2 A1 ,-rA2=kC2 A2 (2nd-order) , 2×211AAAkCCC-=2221AAAkCCC-So we obtain 2×/=/(kCA22)CA2= mol/lWater containing a short-lived radioactive species flows continuously through a well-mixed holdup tank. This gives time for the radioactive material to decay into harmless waste. As it now operates, the activity of the exit stream is 1/7 of the fee d stream. This is not bad, but we’d like to lower it still more.One of our office secretaries suggests that we insert a baffle down the middle of the tank so that the holdup tank acts as two well-mixed tanks in series. Do you think this would help If not, tell why; if so calculate the expected activity of the exit stream compared to the entering stream. Solution: 1st-order reaction, constant volume system. From the information offered about the first reaction,we obtain1τ=01100117171A A A A A A C k C C kC C C V ⋅-=-=υ If a baffle is added,022220212122212υυτττV V +=+==011υV =2222221210A A A A A A kC C C kC C C -+-=007176A A kC C =6/k …… ① 02112121021υV kC C C A A A =-=3/k=222221A A A kC C C - …… ②Combining equation ① and ② we obtain:C A21= ; C A22==0161A C So it will help, and the expected activity of the exit stream is 1/16 of the feed.An aqueous reactant stream (4 mol A/liter) passes through a mixed flow reactorfollowed by a plug flow reactor. Find the concentration at the exit of the plug flow reactor if in the mixed flow reactor C A = 1 mol/liter. The reaction is second-order with respect to A, and the volume of the plug flow unit is three times that of the mixed flow unit. Solution: Constant volume system and 2nd-order reaction:υτmm V ==110A A A r C C --=2110A A A kC C C ->k 14-=3/k …… ① 03υυτmpp V V ===9/k= -⎰-fA A C C AA r dC 1= -⎰-Af C A AdC k C 12=)11(1-Af C k …… ②Combining equation. ① and ② we obtain:C Af = mol/literReactant A (A → R,C A0=26 mol/m 3) passes in steady flow through fourequal-size mixed flow reactors in series (τtotal=2 min). When steady stateis achieved the concentration of A is found to be 11, 5, 2, 1 mol/m 3 in the four units. For this reaction, what must be τplugso as to reduce C Afrom C A0 = 26 to C Af = 1 mol/m 3Solution:4321m m m m m τττττ=====110A A A r C C --=221A A A r C C --=332A A A r C C --=443A A A r C C --C A0=26mol/liter, C A1=11 mol/liter, C A2=5 mol/liter, C A3= 2mol/liter, C A4=1mol/liter So we abtain: 15/(-r A1) = 6/(-r A2) = 3/(-r A3) = 1/(-r A4) We postalate the reaction rate is 1 unit when C A4=1 mol/liter So we obtainC A , mol 11 5 2 1 -r A 30 12 6 2 1/(-r A )1/301/121/61/2⎰--=AfA C C A A p r dC 0τ=⎰-0A Af C C AAr dC So we obtain =p τ min.At 100℃ pure gaseous A reacts away with stoichiometry 2A → R + S in a constantvolume batch reactor as follows:t, sec 0 20 40 60 80 100 120 140 160 p A , atmWhat size of plug flow reactor operating at 100℃ and 1 atm can treat 100 moles A/hr in a feed consisting of 20% inserts to obtain 95% conversion of A Solution:。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
Chapter 14. CHEMICAL EQUILIBRIUM14.1 THE CONCEPT OF EQUILIBRIUM AND THE EQUILIBRIUM CONSTANTMany chemical reactions do not go to completion but instead attain a state of chemical equilibrium.Chemical equilibrium: A state in which the rates of the forward and reverse reactions are equal and the concentrations of the reactants and products remain constant.⇒Equilibrium is a dynamic process – the conversions of reactants to products and products to reactants are still going on, although there is no net change in the number of reactant and product molecules.concentrationFor a reaction:Thecoefficients.Law of mass action - The value of the equilibrium constant expression, K c, is constant for a given reaction at equilibrium and at a constant temperature.⇒The equilibrium concentrations of reactants and products may vary, but the value for K c remains the same.Other Characteristics of K c1) Equilibrium can be approached from either direction.2) K c does not depend on the initial concentrations of reactants and products.3) K c does depend on temperature.Magnitude of K c⇒If the K c value is large (K c >> 1), the equilibrium lies to the right and the reaction mixture contains mostly products.⇒If the K c value is small (K c <<1), the equilibrium lies to the left and the reaction mixture contains mostly reactants.⇒If the K c value is close to 1 (0.10 < K c< 10), the mixture contains appreciable amounts of both reactants and products.14.2 WRITING EQUILIBRIUM CONSTANT EXPRESSIONSCalculating Equilibrium Constants, K cK c values are listed without units ⇒ don't include units when calculating K c .If equilibrium concentrations are known, simply substitute the concentrations into the equilibrium constant expression: Example. For the reaction, CO + 3H 2 CH 4 + H 2O, calculate K c from the following equilibrium concentrations: [CO] = 0.0613 M; [H 2] = 0.1839 M; [CH 4] = 0.0387 M; [H 2O] = 0.0387 M.Homogeneous equilibria : reactants and products exist in a single phase.For the gas phase reaction: N 2O 4(g) 2NO 2(g)The equilibrium constant with the concentrations of reactants and products expressed in terms of molarity, K c , is:K c = ]O N []NO [4222 Gas Phase Expressions can also be expressed by K p⇒ The K p expression is written using equilibrium partial pressures of reactants & products. For the reaction given above, the K p expression is:K p = 422O N 2NO P PK p is related to K cSince pressure and molarity are related by the Ideal Gas Law, the following equation relates K p and K c :K p = K c (RT)∆nwhere R = 0.0821 mol K atm L ⋅⋅; T = temperature in Kelvin ∆n = moles of gaseous products – moles of gaseous reactants⇒ Note that K c = K p when the number of gas molecules are the same on both sides.Example. Does K c = K p for (a) H 2(g) + F 2(g) 2HF (g)? (b) 2SO 2(g) + O 2(g) 2SO 3(g)?Example. For the reaction, 2SO 2(g) + O 2(g) 2SO 3(g) (a) write the equilibrium constant expression, K p . (b) What is the value for K p if K c = 2.8x102 at 1000 K?Heterogeneous Equilibria and Solvents in Homogeneous EquilibriaHeterogeneous equilibria : reactants and products are present in more than one phase.pure solids and liquids : concentrations of pure solids and liquids are fixed by their density and molar mass (both constants) and do not vary with the amount.[]Mass Molar Density M == gmol L ml ml g L mol M ××==1103 ⇒ Thus, the concentrations of solids and liquids are incorporated in the K c value; they are not part of the variable K c expression: Example. Write the K c expression forCaCO 3(s) CaO (s) + CO 2(g)• Omit concentration terms for solids and liquids from K c and K p expressions; onlyinclude terms for gases (g) and aqueous substances (aq).Example. Write the K c expression for the following reaction:3Cu (s) + 2NO 3-(aq) + 8H +(aq) 3Cu 2+(aq) + 2NO (g) + 4H 2O (l)Modifying Equilibrium Constant Expressions:A. Changing stoichiometric coefficients:1) 2SO 2 + O 2 2SO 3 K 1 = 2) SO 2 + 21O 2 SO 3 K 2 = ⇒ If we multiply an equation by a factor, we must raise its K to that power to get the new K.B. Reversing the reaction:3) 2SO 3 2SO 2 + O 2 K 3 =⇒ K is the reciprocal of the K value for the reverse reaction.C. Adding Equations for Multiple Equilibrium Reactions:1. A + 2B C K 1 =2. A + D B K 2 =3. 2A + B + D C K 3 =⇒ When we add equations to get a new equation, the new K is the product of the other K’s. Example. 2H 2(g) + O 2(g) 2H 2O (g)K c = 1.7x1027N 2(g) + 3H 2(g) 2NH 3(g) K c = 1.5x102 Calculate the value of K c for 4NH 3(g) + 3O 2(g) 2N 2(g) + 6H 2O (g)14.3 THE RELATIONSHIP BETWEEN CHEMICAL KINETICS AND CHEMICAL EQUILIBRIUMFor the reaction N 2O 4(g) 2NO 2(g)Rate of forward reaction = Rate of reverse reaction k f [N 2O 4] = k r [NO 2]2Rearrange: ][][4222O N NO = rf k k = K c Thus, the equilibrium constant is simply the ratio of the forward and reverse rate constants which are both constant values at a given temperature.14.4 WHAT DOES THE EQUILIBRIUM CONSTANT TELL US?Predicting the Direction of ReactionThe reaction quotient, Q, is the resulting value when we substitute reactant and product concentrations into the equilibrium expression. 1. If Q > K, the reaction will go to the left.• The ratio of products over reactants is too large & the reaction will move toward equilibrium by forming more reactants.2. If Q < K, the reaction will go to the right.• The ratio of products over reactants is too small & the reaction will move towardequilibrium by forming more products.3. If Q = K, the reaction mixture is already at equilibrium, so no shift occurs.Example. For the reaction, B 2A, K c = 2. Suppose 3.0 moles of A and 3.0 moles of B are introduced into a 2.00 L flask. (a) In which direction will the reaction proceed to attain equilibrium? (b) Will the concentration of B increase, decrease or remain the same as the system moves towards equilibrium?Calculating Equilibrium ConcentrationsUse ICE Tables To Solve Equilibrium Problems For K c Or Equilibrium Amounts:1. I = initial concentration : Initial concentration of reactants are usually given; initial [Product]'s are assumed to be 0 unless otherwise specified.2. C = change in concentration : Assign change as the variable x; use the stoichiometry of the reaction to assign changes for all species.3. E = equilibrium concentration: E = I + C⇒ Note, values in ICE tables can be in terms of moles or Molarity (or atm for K p ), but values used in the K c expression must be in terms of Molarity (or atm for K p ).Example. When 0.250 mol SO2 and 0.200 mol O2 are placed in a 10.0 L vessel at 1000 K, the equilibrium mixture was found to contain 0.162 mol SO3. Calculate K c for this reaction:2SO2(g)+ O2(g)2SO3(g)Method to find equilibrium concentrations1) Set up the ICE table.2) Set up the K c expression; substitute equilibrium concentrations from the ICE table into K c.3) Solve for x; plug the solution for x back into the equilibrium concentration expressions. Perfect square problem to solve for x⇒Take the square root of both sides when the math expression is a perfect square. Example. At 430 °C, K p = 54.3 for the following reaction: H2(g)+ I2(g) 2HI(g)A mixture of H2 at a pressure of 0.500 atm and I2 at a pressure of 0.500 atm is placed in a container at 430 °C. Calculate the equilibrium partial pressures of HI, H2 and I2.Quadratic Formula ExampleFor this reaction, H2(g)+ F2(g)2HF(g), K c = 1.15x102 at a certain temperature.Suppose 3.000 moles of H2 and 6.000 moles of F2 are placed in a 3.000 L container. What are the equilibrium concentrations of H2, F2 and HF?14.5 FACTORS THAT AFFECT CHEMICAL EQUILIBRIUMLe Chatelier's Principle: If a system at equilibrium is disturbed by an external stress, the system adjusts to partially offset the stress as the system attains a new equilibrium position. Changes in Concentration⇒Adding a reactant or product, the equilibria shifts away from the increase in order to consume part of the added substance.⇒Removing a reactant or product, the equilibria shifts toward the decrease to replace part of the removed species.E.g. For H2+ I2 2HI, does the equilibria shift left or right if we: a) add H2? b) remove I2?a)b)Changes in Volume and PressureBecause the pressure of gases is related directly to the concentration by P = n/V, changing the pressure by increasing/decreasing the volume of a container will disturb an equilibrium system.⇒If P increases (V decreases), the system shifts to the side with a smaller number of gas molecules (this effectively reestablishes equilibrium by decreasing the pressure).⇒If P decreases (V increases), the system shifts to the side with a greater number of gas molecules.Example. For N2(g)+ 3H2(g) 2NH3(g), does the equilibrium shift left or right if the pressure is increased?Changes in TemperatureHeat can be considered a reactant in an endothermic rxn and a product in an exothermic rxn. Endothermic (∆H > 0) R + Heat ProductsExothermic (∆H < 0) R Products + HeatRecall that both K c and the position of the equilibrium system will vary with temperature: •K c is larger when the reaction shifts right. This occurs if T is increased for an Endothermic Reaction or T is decreased for an Exothermic reaction.•K c is smaller when the reaction shifts left. This occurs if T is decreased for an Endothermic Reaction or T is increased for an Exothermic reaction.Example. If the temperature is decreased for the reaction: 2CO22CO + O2, ∆H = 566 kJ. a) Will the equilibrium shift left or right? b) Does K c become larger or smaller?Effect of a Catalyst⇒Catalysts lower E a for the reaction, so a catalyst decreases the amount of time taken to reach equilibrium for both the forward and reverse reactions.⇒The catalyst does not affect the equilibrium concentrations of reactants and products in the equilibrium mixture; thus, the K c value does not change.Choosing Optimum ConditionsLe Chatelier’s principle can be used to select optimum conditions to form a substance.e.g. To form more NH3, predict the optimum conditions for temperature and pressure.N2(g) + 3H2(g) 2NH3(g)∆H = -91.8 kJ。
