模块测试_(人教B版必修一)(1)

合集下载

新教材2022版数学必修第一册(人教B版)课时作业-1.1.3.1交集与并集-含解析

课时作业(三)交集与并集一、选择题1．(多选)若集合M⊆N，则下列结论正确的是()A．M∩N＝M B．M∪N＝NC．M⊆(M∩N) D．(M∪N)⊆N2．已知集合A＝{x|x≥－3}，B＝{x|－5≤x≤2}，则A∪B＝()A．{x|x≥－5}B．{x|x≤2}C．{x|－3<x≤2}D．{x|－5≤x≤2}3．设集合A＝{1，2，3，4}，B＝{－1，0，2，3}，C＝{x∈R|－1≤x<2}，则(A∪B)∩C ＝()A．{－1，1}B．{0，1}C．{－1，0，1}D．{2，3，4}4．设集合A＝{x|－1≤x<2}，B＝{x|x<a}，若A∩B≠∅，则a的取值范围是()A．a<2B．a>－2C．a>－1D．－1<a≤2二、填空题5．定义A－B＝{x|x∈A，且x∉B}，若M＝{1，2，3，4，5}，N＝{2，3，6}，则N－M ＝________．6．设集合A＝{1，2，a}，B＝{1，a2}，若A∩B＝B，则实数a允许取的值有________个．7．已知集合A＝{x|x≤1}，B＝{x|x≥a}，且A∪B＝R，则实数a的取值范围为________．三、解答题8．设A＝{x|－1<x<2}，B＝{x|1<x<3}，求A∪B，A∩B.9．已知A＝{x|a<x≤a＋8}，B＝{x|x<－1或x>5}．若A∪B＝R，求a的取值范围．[尖子生题库]10．集合A＝{x|－1≤x<3}，B＝{x|2x－4≥x－2}．(1)求A∩B；(2)若集合C＝{x|2x＋a>0}，满足B∪C＝C，求实数a的取值范围．课时作业(三)交集与并集1．解析：∵集合M⊆N，∴在A中，M∩N＝M，故A正确；在B中，M∪N＝N，故B正确；在C中，M⊆(M∩N)，故C正确；在D中，(M∪N)⊆N，故D正确．答案：ABCD2．解析：结合数轴(图略)得A∪B＝{x|x≥－5}．答案：A3．解析：本题主要考查集合的运算．由题意得A∪B＝{1，2，3，4，－1，0}，∴(A∪B)∩C＝{1，2，3，4，－1，0}∩{x∈R|－1≤x<2}＝{－1，0，1}．故选C.答案：C4．解析：在数轴上表示出集合A，B即可得a的取值范围为a>－1.答案：C5．解析：关键是理解A－B运算的法则，N－M＝{x|x∈N，且x∉M}，所以N－M＝{6}．答案：{6}6．解析：由题意A∩B＝B知B⊆A，所以a2＝2，a＝±2，或a2＝a，a＝0或a＝1(舍去)，所以a＝±2，0，共3个．答案：37．解析：由A∪B＝R，得A与B的所有元素应覆盖整个数轴．如图所示：所以a必须在1的左侧，或与1重合，故a≤1.答案：(－∞，1]8．解析：如图所示：A ∪B ＝{x |－1<x <2}∪{x |1<x <3}＝{x |－1<x <3}． A ∩B ＝{x |－1<x <2}∩{x |1<x <3}＝{x |1<x <2}．9．解析：在数轴上标出集合A ，B ，如图．要使A ∪B ＝R ，则⎩⎪⎨⎪⎧a ＋8≥5，a <－1，解得－3≤a <－1. 综上可知，a 的取值范围为－3≤a <－1.10．解析：(1)∵B ＝{x |x ≥2}，∴A ∩B ＝{x |2≤x <3}．(2)C ＝⎩⎨⎧⎭⎬⎫x ⎪⎪x >－a 2，B ∪C ＝C ⇒B ⊆C ，∴－a 2<2，∴a >－4. 即a 的取值范围为a >－4.。

人教B版(2019)高中数学必修第一册第一章《集合与常用逻辑用语》检测卷(含答案)

人教B 版（2019）高中数学必修第一册第一章《集合与常用逻辑用语》检测卷一、单选题（本题有12小题，每小题5分，共60分）1．设全集为实数集R ，集合{}3,2,1,0,1,2,3A =---，{}2B x x =≥，则()RA B =（）A ．{}2,3B ．{}2,1,0,1--C ．{}3,2,1,0---D ．{}3,2,1,0,1---2．设x 、y R ∈，则“x y ≥”是“x y ≥”的（） A ．充分不必要条件 B ．必要不充分条件 C ．充要条件 D ．既不充分也不必要条件3．“1x >"是“11x<”的（） A ．充分不必要条件 B ．必要不充分条件 C ．充要条件D ．既不充分也不必要条件4．下列结论中，错误的是（） A ．“1x =”是“20x x -=”的充分不必要条件B ．已知命题2:,10p x R x ∀∈+>，则2:,10p x R x ⌝∃∈+≤C ．“220x x +->”是“1x >”的充分不必要条件；D ．命题：“x R ∀∈，sin 1x ≤”的否定是“0x R ∃∈，0sin 1x >”；5．已知：p ：1x ，2x 是方程2560x x +-=的两根，q ：126x x ⋅=-，则p 是q 的（） A ．充分不必要条件 B ．必要不充分条件 C ．充要条件D ．既不充分也不必要条件6．已知命题:1p x ∀>，4220212022x x +>，则p ⌝为（）A ．1x ∃≤，4220212022x x +≤B ．1x ∀>，4220212022x x +≤C ．1x ∃>，4220212022x x +≤D ．1x ∀≤，4220212022x x +>7．命题“()0,x ∀∈+∞，x 3＋3x ≥1”的否定是（）． A ．()0,x ∃∈+∞，x 3＋3x ＜1 B ．()0,x ∃∈+∞，x 3＋3x ≥1 C ．()0,x ∀∈+∞，x 3＋3x ＜1D ．x 3＋3x ≤18．已知集合{|25}M x x =-<<，{}33N x x =-≤≤，则M N ⋃=（） A ．{}3,2,1,0,1,2,3,4--- B ．{}1,0,1,2,3- C ．[)3,5-D ．(]2,3-9．设集合{0,1,2,3,4,5}U =，{0,2,3,5}M =，则UM =（）A ．{1,4}B ．{1,5}C ．{0,4,5}D ．{1,4,5}10．已知集合{}1,2A =，{},,B x x a b a A b A ==-∈∈，则集合B 中元素个数为（） A ．1B ．2C ．3D ．411．设a ∈R ，则“3a >”是“23a a >”的（） A ．充分不必要条件 B ．必要不充分条件 C ．充要条件D ．既不充分也不必要条件12．已知全集{}2,1,0,1U =--，集合{}220A x x x =+-=，{}0,1B =，则()U A B ⋃=（）A ．{}2,1,0--B ．{}2,1,1--C ．2,0,1D ．{}2,1,0,1--二、填空题（本题有4小题，每小题5分，共20分）13．命题“2000,230x x x ∃∈-+<R ”，此命题的否定是________命题．（填“真”或“假”）14．设命题:p n N ∀∈，22n n >，则p ⌝为________．15．,A B 是集合{}1,2,3,4的非空子集，则满足A B =∅的有序集合对(),A B 共有_______个． 16．设集合{}1,2,3,4A =，[)1,3B =，则A B =________.三、解答题（本题有6小题，共70分）17．（10分）已知集合{|2A x x =-或3}x ，{}B |05x x =<<，{}|12C x m x m =-≤≤ （1）求A B ，()R A B ；（2）若B C C ⋂=，求实数m 的取值范围．18．（12分）设全集为R ，集合P ＝{x |3＜x ≤13}，非空集合Q ＝{x |a ＋1≤x ＜2a －5}，（1）若a ＝10，求P ∩Q ； ()R P Q ；（2）若()Q P Q ⊆，求实数a 的取值范围19．（12分）设集合{}250A x x ax =-+>，{}25B x x =<<．（1）若集合R A =，求实数a 的取值范围；（2）若“x A ∈”是“x B ∈”的必要条件，求实数a 的取值范围．20．（12分）已知0m >，()():150p x x +-≤，:11q m x m -≤≤+. （1）若5m =，p q ∨为真命题，p q ∧为假命题，求实数x 的取值范围；（2）若p 是q 的充分不必要条件，求实数m 的取值范围.21．（12分）已知集合{|25},{|121}A x x B x m x m =-<<=+≤≤- （1）当3m =时，求()R A B ；（2）若A B A ⋃=，求实数m 的取值范围.22．（12分）设集合{}2=40A x R x x ∈+=，{}22=2(1)10,B x R x a x a a R ∈+++-=∈，若B A ⊆，求实数a 的值．参考答案1．D 【分析】先求得B R ，再根据交集运算即可得出结果. 【详解】 {}2B x x =≥,{}2B x x ∴=<R ,{}3,2,1,0,1,2,3A =---()RAB ∴={}3,2,1,0,1---.故选：D. 2．A 【分析】根据充要条件的定义，结合不等式的性质，举实例，可得答案．【详解】解：①若x y ，||x x ，||x y ∴成立，∴充分性成立，②当3x =-，2y =时，||x y 成立，但x y 不成立，∴必要性不成立，x y ∴是||x y 的充分不必要条件，故选：A ． 3．A 【分析】由11x<得10x x -<，即1x >或0x <可进行判断.【详解】由11x<得10xx -<，即1x >或0x <，所以1x >能够得到11x <，但是11x<不一定得到1x >， “1x >”是“11x<”成立的充分不必要条件. 故选：A.【点睛】结论点睛：本题考查充分不必要条件的判断，一般可根据如下规则判断：（1）若p 是q 的必要不充分条件，则q 对应集合是p 对应集合的真子集；（2）p 是q 的充分不必要条件，则p 对应集合是q 对应集合的真子集；（3）p 是q 的充分必要条件，则p 对应集合与q 对应集合相等；（4）p 是q 的既不充分又不必要条件，q 对的集合与p 对应集合互不包含 4．C 【分析】根据充分必要条件和全称量词的否定形式判断即可. 【详解】当1x =时，20x x -=.当20x x -=时，1x =或0x =.“1x =”是“20x x -=”的充分不必要条，A 对.对于含有一个量词的全称命题p ：“任意的”x M ∈，()p x 的否定，p ⌝是：“存在”x M ∈，()p x ⌝.B 对.同理，D 对.当220x x +->时，1x >或2x <-.当1x >时，220x x +->.“220x x +->”是“1x >”的必要不充分条件，C 错. 故选：C. 5．A 【分析】利用充分条件和必要条件的定义判断即可【详解】解：由2560x x +-=，得(1)(6)0x x -+=，解得1x =或6x =-，因为1x ，2x 是方程2560x x +-=的两根，所以126x x ⋅=-，当126x x ⋅=-时，1x ，2x 也可以不是方程260x x --=的两个根，所以p 是q 的充分不必要条件，故选：A 6．C 【分析】根据全称命题的否定为特称命题可得. 【详解】根据全称命题的否定为特称命题，可知命题p 的否定为1x ∃>，4220212022x x +≤. 故选：C. 7．A 【分析】将“任意”改为“存在”，只否定结论. 【详解】“()0,x ∀∈+∞，x 3＋3x ≥1”的否定是“()0,x ∃∈+∞，x 3＋3x ＜1”. 故选：A. 8．C 【分析】由已知集合，应用集合的并运算，求M N ⋃即可. 【详解】由题意，M N ⋃={}{|25}33{|35}x x x x x x -<<⋃-≤≤=-≤<， ∴M N ⋃=[)3,5-. 故选：C 9．A 【分析】根据补集的定义计算可得；【详解】解：因为{0,1,2,3,4,5}U =，{0,2,3,5}M =，所以{}1,4UM =故选：A 10．C 【分析】由集合B 的描述知{1,2}a ∈、{1,2}b ∈，可求出x a b =-，即得集合B 的元素个数. 【详解】解：由题意知：{1,2}a ∈，{1,2}b ∈，{}{}|,,0,1,1B x x a b a A b A ==-∈∈=-，∴集合B 中元素个数为3. 故选：C. 11．A 【分析】由23a a >，解得0a <或3a >．利用充分、必要条件的定义即可判断出．【详解】解：由23a a >，解得0a <或3a >． ∴ “3a >”是“23a a >”的充分不必要条件．故选：A ． 12．B 【分析】解一元二次方程用列举法表示集合A ，然后求出U B ，最后按集合的并集概念进行运算即可. 【详解】{}{}2201,2A x x x =+-==-，U{2,1}B =--，∴()U {2,1,1}A B ⋃=--.故选：B 13．真【分析】写出命题的否定形式，再判断真假即可. 【详解】命题“2000,230x x x ∃∈-+<R ”，此命题的否定为“2,230x x x ∀∈-+≥R ”，由()2223120x x x -+=-+≥，显然成立，所以命题的否定是真命题. 故答案为：真 14．2,2n n N n ∃∈≤【分析】根据命题的否定的定义求解．【详解】命题:p n N ∀∈，22n n >的否定是：2,2n n N n ∃∈≤．故答案为：2,2n n N n ∃∈≤． 15．50 【分析】根据题意可知{}1,2,3,4U =，当集合A 确定后，集合B 是UA 的非空子集，分别计算A 中有1、2、3个元素时有序集合对(),A B 的个数之和即可. 【详解】设{}1,2,3,4U =，因为A B =∅，所以B 是UA 的非空子集，当A 中只有一个元素时，(),A B 的个数为()342128⨯-=个，当A 中只有2个元素时，(),A B 的个数为()262118⨯-=个，当A 中只有3个元素时，(),A B 的个数为()14214⨯-=个，所以共有2818450++=个，故答案为：50. 16．[]{}1,34⋃ 【分析】直接根据并集的定义计算可得；【详解】解：因为{}1,2,3,4A =，[)1,3B = 所以[]{}1,34A B =⋃ 故答案为：[]{}1,34⋃17．（1）{}|35A B x x =≤<，(){25}R A B x x ⋃=-<<∣；（2）()5,11,2⎛⎫-∞- ⎪⎝⎭．【分析】（1）进行根据交集、并集和补集的定义运算即可；（2）根据BC C =可得出C B ⊆，然后讨论C 是否为空集：C =∅时，12m m ->；C ≠∅时得到不等式组，然后解出m 的范围即可．【详解】解：（1）因为{|2A x x =-或3}x ，{}B |05x x =<< 所以{}|35A B x x =≤<，{}|23RA x x =-<<(){}{}{}|23|05|25RA B x x x x x x =-<<<<=-<<（2）由B C C =，则C B ⊆ 当C =∅时，12m m ->，所以1m <- 当C ≠∅时，101225m m m m ->⎧⎪-≤⎨⎪<⎩，所以512m <<综上：实数m 的取值范围为()5,11,2⎛⎫-∞- ⎪⎝⎭18．（1）{|1113}x x ，{|1315}x x <<；（2） (]6,9. 【分析】（1）把a 的值代入求出集合Q ，再由交集、补集的运算求出P Q ，(R P Q ⋂；（2）由()Q P Q ⊆得Q P ⊆，再由子集的定义列出不等式组，求出a 的范围．【详解】（1）当10a =时，{|1115}Q x x =<，又集合{|313}P x x =<，所以{|313}{|1115}{|1113}P Q x x x x x x ⋂=<⋂<=，{|3RP x x =或13}x >，则(){|1315}R P Q x x ⋂=<<；（2）由()Q P Q ⊆得，Q P ⊆，因为Q φ≠，则125132513a a a a +<-⎧⎪+>⎨⎪-⎩，解得69a <，综上所述：实数a 的取值范围是(]6,9.19．（1）a -<；（2）a < 【分析】（1）由判别式小于0可得；（2）题意说明B A ⊆，即250x ax -+>在(2,3)上恒成立，分离参数后，由基本不等式求得函数的最小值可得结论．【详解】解：（1）∵{}250A x x ax R =-+>=，∴2200a ∆=-<，∴a -<（2）∵x A ∈是x B ∈的必要条件，∴B A ⊆，∵250x ax -+>，∴min 5a x x ⎛⎫<+ ⎪⎝⎭，()2,5x ∈，∵5x x +≥5x x+，即x =∴min 5x x ⎛⎫+= ⎪⎝⎭∴a <20．（1）{41x x -≤<-或}56x <≤；（2）[)4,+∞ 【分析】（1）由p q ∨为真命题，p q ∧为假命题，可得p 与q 一真一假，然后分p 真q 假、p 假q 真两种情况，分别列出关系式，求解即可；（2）由p 是q 的充分条件，可得[][]1,51,1m m -⊆-+，则有01115m m m >⎧⎪-≤-⎨⎪+≥⎩，从而可求出实数m的取值范围. 【详解】（1）当5m =时，:46q x -≤≤，由()()150x x +-≤，可得15x -≤≤，即P ：15x -≤≤. 因为p q ∨为真命题，p q ∧为假命题，故p 与q 一真一假，若p 真q 假，则1564x x x -≤≤⎧⎨><-⎩或，该不等式组无解；若p 假q 真，则1546x x x <->⎧⎨-≤≤⎩或，得41x -≤<-或56x <≤.综上所述，实数x 的取值范围为{41x x -≤<-或}56x <≤.（2）由题意，P ：15x -≤≤，:11q m x m -≤≤+，因为p 是q 的充分不必要条件，故[][]1,51,1m m -⊆-+，故111115m m m m -<+⎧⎪-≤-⎨⎪+≥⎩，得4m ≥，故实数m 的取值范围为[)4,+∞.21．（1）(){}5R A B =；（2）3m <.【分析】（1）根据集合的运算法则计算；（2）由A B A ⋃=得B A ⊆，然后分类B =∅和B ≠∅求解．【详解】（1）当3m =时，B 中不等式为45x ≤≤，即{}|45B x x =≤≤，∴{|2R A x x =≤-或5}x ，则(){}5R A B =（2）∵A B A ⋃=，∴B A ⊆，①当B =∅时，121m m +>-，即2m <，此时B A ⊆；②当B ≠∅时，12112215m m m m +≤+⎧⎪+>-⎨⎪-<⎩，即23m ≤<，此时B A ⊆.综上m 的取值范围为3m <.22．a ≤－1或a ＝1.【分析】先求出集合A ，当A ＝B 时，满足B A ⊆，再由根与系数的关系可求出实数a 的值；当B A ≠时，分B ≠∅和B =∅两种情况求解即可【详解】∵A ＝{0，－4}，B ⊆A ，于是可分为以下几种情况．(1)当A ＝B 时，B ＝{0，－4}，∴由根与系数的关系，得22(1)410a a -+=-⎧⎨-=⎩解得a ＝1. (2)当B A ≠时，又可分为两种情况． ①当B ≠∅时，即B ＝{0}或B ＝{－4}，当x ＝0时，有a ＝±1；当x ＝－4时，有a ＝7或a ＝1.又由Δ＝4(a ＋1)2－4(a 2－1)＝0，解得a ＝－1，此时B ＝{0}满足条件； ②当B =∅时，Δ＝4(a ＋1)2－4(a 2－1)<0，解得a <－1.综合(1)(2)知，所求实数a 的取值为a ≤－1或a ＝1.。

人教版高中数学必修一第一章单元测试(含答案)

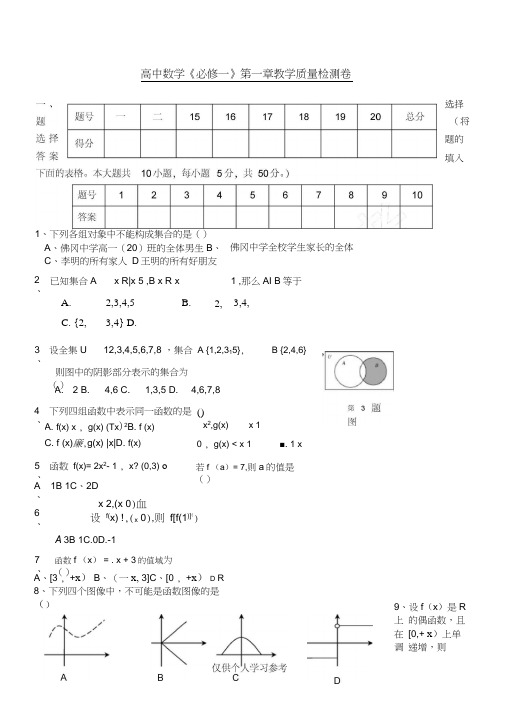
高中数学《必修一》第一章教学质量检测卷佛冈中学全校学生家长的全体 1、下列各组对象中不能构成集合的是（）A 、佛冈中学高一（20）班的全体男生B 、C 、李明的所有家人D 王明的所有好朋友选择（将题的填入2、已知集合A x R|x 5 ,B x R x 1 ,那么AI B 等于3、4、5、 A 、6、 7、 A. C. {2, 2,3,4,5 3,4} D.B.2, 3,4,12,3,4,5,6,7,8 ，集合 A {1,2,315}, 设全集U 则图中的阴影部分表示的集合为（）A. 2B. 4,6C. 1,3,5D. 4,6,7,8 下列四组函数中表示同一函数的是 A. f(x) x , g(x) (Tx )2B. f (x) C. f (x)廉,g(x) |x|D. f(x) 函数 f(x)= 2x 2- 1 , x? (0,3) o1B 1C 、2D B {2,4,6} ()x 2,g(x) x 1 0 , g(x) < x 1 ■. 1 x若f （a ）= 7,则a 的值是（） x 2,(x 0)血设f(x) !,(x 0),则f[f(1)]() A 3B 1C.0D.-1 函数f （x ） = . x + 3的值域为（） A 、[3 , +x ） B 、（一x, 3]C 、[0 , +x ）D R 8、下列四个图像中，不可能是函数图像的是（） 9、设f （x ）是R上的偶函数，且在 [0,+ x ）上单调递增，则f(-2),f(3),f(- )的大小顺序是：() A f(- )>f(3)>f(-2)B 、f(- )>f(-2)>f(3) C 、f(-2)>f(3)>f(- )D 、f(3)>f(-2)>f(- ) 10、在集合｛a , b , c , d ｝上定义两种运算和如下:那么 b (a c)() A. aB. bC. cD. d二、填空题(本大题共4小题，每小题5分，共20分) 11、函数y 1 (x 3)0的定义域为12、函数f(x) x 2 6x 10在区间［0,4］的最大值是Q I /'13、若 A { 2,2,3,4} , B {x|x t 2,t A}，用列举法表示 B 是.14、下列命题：①集合a,b,c,d 的子集个数有16个；②定义在R 上的奇函数f(x)必满足f (0) 0 ; ③f(x) 2x 1 2 2 2x 1既不是奇函数又不是偶函数；④偶函数的图像一定与y 轴相交；⑤f(x)」x在 ,0 U 0, 上是减函数。

新人教B版(2019新教材)高中数学必修第一册练习：(1)集合及其表示方法含答案

2019-2020学年人教B 版（2019）高中数学必修第一册同步学典（1）集合及其表示方法1、给出下列表述:①联合国常任理事国;③方程210x x +-=的实数根④全国著名的高等院校.以上能构成集合的是( )A.①③B.①②C.①③④D.①②③④2、下列各组对象中不能够成集合的是（）A.大通学校的全体学生B.2009年全国经济百强县C.2010年考入北京大学的全体学生D.美国NBA 的篮球明星3、给出以下五个对象,其中能构成集合的有( )①你所在班中身高超过1.75m 的同学;②所有平行四边形;③某数学教辅书中的所有习题;④所有有理数;⑤2016年高考试卷中的所有难题.A.1个B.2个C.3个D.4个4、设,a b 都是非零实数,由||||||a b ab y a b ab =++的可能取值组成的集合为( ) A. {}3B. {}3,2,1C. {}3,1,1-D. {}3,1-5、方程组31x y x y +=⎧⎨-=-⎩的解集不能表示为( ) A.3(,)|1x y x y x y ⎧+=⎫⎧⎪⎪⎨⎨⎬-=-⎪⎪⎩⎩⎭ B.1(,)|2x x y y ⎧=⎫⎧⎪⎪⎨⎨⎬=⎪⎪⎩⎩⎭C.{}1,2D.{}(,)|1,2x y x y ==6、下列集合中，不同于另外三个集合的是( )A.{}||1|0x x +=B.{}2|(1)0y y +=C.{}1x =-D.{}1-7、已知集合Ω中的三个元素,,l m n 分别是ABC △的三边长，则ABC △一定不是( )A.锐角三角形B.直角三角形C.钝角三角形D.等腰三角形 8、集合{}(,)|21x y y x =-表示( )A.方程21y x =-B.点(,)x yC.平面直角坐标系中的所有点组成的集合D.一次函数21y x =-图象上的所有点组成的集合9、已知集合{}{}|3,Z ,|31,Z M x x n n N x x n n ==∈==+∈,{}|31,Z P x x n n ==-∈，且,,a M b N c P ∈∈∈，若d a b c =-+，则( )A.d M ∈B.d N ∈C.d P ∈D.d M ∈且d N ∈10、已知,x y 为非零实数，则集合|x y xy M m m x y xy ⎧⎫⎪⎪==++⎨⎬⎪⎪⎩⎭为( ) A.{}0,3 B.{}1,3 C.{}1,3- D.{}1,3-11、由下列对象组成的总体属于集合的是__________(填序号).① 不超过3的正整数:② 高一数学课本中所有的难题;③ 中国的大城市;④ 平方后等于自身的数;⑤ 某校高一(2)班中考数学成绩在90分以上的学生.12、设集合{}52,n M m m n n *==+∈N，且100m <，则集合M 中所有元素的和为 .13、若{}20,2,m m m ∈-则实数m 的值为__________14、以方程2230x x --=和方程220x x --=的解为元素的集合中共有__________个元素.15已知集合,求的值.答案以及解析1答案及解析：答案：A解析：②④中元素不能确定.2答案及解析：答案：D解析：3答案及解析：答案：D解析：①②③④能构成集合.4答案及解析：答案：D解析：①当,a b 同正时, 1113y =++=;②当,a b 同负时, 1111y =--+=-;③当,a b 一正一负时, 1y =-,故D 正确.5答案及解析：答案：C解析：原方程组的解为12x y =⎧⎨=⎩，其解集中只含有一个元素，可表示为A,B,D,C 不符合，故选C.6答案及解析：答案：C解析：由集合的含义知{}{}{}2||1|0|(1)01x x y y +==+==-，而集合{}1x =-表示由方程1x =-组成的集合.故选C.7答案及解析：答案：D解析：因为集合中的元素是互异的，所以,,l m n 互不相等，即ABC △不可能是等腰三角形.故选D.8答案及解析：答案：D解析：本题中的集合是点集，其表示一次函数21y x =-图象上的所有点组成的集合.故选D.9答案及解析：答案：B解析：由题意，设3,Z,31,Z,31,Z a k k b y y c m m =∈=+∈=-∈,则3(31)313()2d k y m k y m =-++-=-+-，令t k y m =-+，则Z t ∈，则323313(1)1,Z d t t t t =-=-+=-+∈，则d N ∈，故选B.10答案及解析：答案：C解析：当0,0x y >>时，3m =；当0,0x y <<时，1111m =--+=-；若,x y 异号，不妨设0,0x y ><，则1(1)(1)1m =+-+-=-.综上，3m =或-1，即{}1,3M =-.11答案及解析：答案：①④⑤解析：②中“难题”标准不明确,不满足确定性; ③中“大城市”标准不明确,不满足确定性.12答案及解析：答案：231解析：1n =时，15127m =⨯+=，2n =时，252214m =⨯+=，3n =时，353223m =⨯+=,4n =时，454236m =⨯+=,5n =时，555257m =⨯+=，6n =时，656294m =⨯+=，当7n >时，100m ≥不合要求.故M 中所有元素的和为71423365794231+++++=.13答案及解析：答案：∵{}20,2,m m m ∈-∴0m =或220m m -=当0m =时, 220m m -=,这与集合元素的互异性矛盾, 当220m m -=时, 0m =或(舍去)或2m =故答案为: 2解析：14答案及解析：答案：3解析：因为方程2230x x --=的解是121,3x x =-=,方程220x x --=的解是,x x 3412=-=所以以这两个方程的解为元素的集合中的元素应为1,2,3,-共有3个元素.15答案及解析：答案：由有意义,得, 所以, 所以由得,故,于是有, ∴或. (1)当时,结合,知. 经检验,不符合题意.(2)当时,有或. 经检验,符合题意. 综上,知故。

人教版高中英语必修一Unit 1 单元测试题 1(含答案)

Unit 1 单元测试题第二部分：阅读理解（共两节，满分40分）第一节（共15小题；每小题2分，满分30分）阅读下列短文，从每题所给的四个选项（A、B、C和D）中，选出最佳选项。

A21. What’s worrying Anna?A. Her friend keeps telling lies.B. Her friend is not interesting.C. Her friend does not believe her.D. Her friend reads her terrible stories.22. Dani suggests that Tom _____.A. leave his two friendsB. tell his friends his thoughtsC. go on an outing with one of his friendsD. invite another to join his group of friends23. Whose friend has a big mouth?A. Tom.B. Mary.C. Anna.D. Daisy.24. The underlined word “teasing” in the last paragraph probably means “_____”.A. avoidingB. hatingC. making fun ofD. taking pity onBOn my first day of high school, I asked an eleventh-grader where my class was. And he told me it was “on the fourth floor, next to the pool.” I found out five minutes later th at we don’t even have a fourth floor and there’s no pool either! Besides that, I didn’t have any trouble with the older kid.I think the biggest difference between middle and high school is the homework load (工作量) and size of the school. I went from maybe fifteen minutes of homework a night to several hours, so I had to learn how to make full use of time! Our class size is around 550, but joining in clubs, sports, music, and other activities at school makes it easier to get to know people in every grade.The best advice I can give about the years you spend in high school is to learn things for yourself, not just to get a good grade. There have been so many tests that I’ve prepared for the night before, gotten an A, and not remembered anything later. I’ve changed that this year, and I enjoy school so much more. Don’t take easy classes just to have a simple year. If you have a choice between chemistry and sports, the first will prove to be a lot more useful!While drinking and smoking might be present i n some middle schools, they’re also around in high school. I have a lot of friends who promised they’d never drink or smoke, but are now partying every weekend. If you have “fun” and spend your nights wasted instead of studying, you will regret it when you’re applying for (申请) college. The “friends” who say you’re a loser for not partying are really not your friends at all. It’s hard to see your closest friends grow apart and go in different directions, but don’t follow their footsteps. Create your own path in life and make your own decisions.25. What happened to the author on his first day of high school?A. He had a fight with an eleventh-grader.B. He fell into a pool on the fourth floor.C. He was five minutes late for class.D. He was fooled by a schoolboy.26. The author advises high school students to _____.A. give up sportsB. choose useful classesC. try their best to get good gradesD. get ready for tests the night before27. The last paragraph mainly tells us _____.A. about the trouble caused by drinking and smokingB. about the importance of making friendsC. not to go to any party in high schoolD. not to lose ourselves in high schoolCIn the book The Best Little Girl in the World, Kessa has a serious eating disorder (进食障碍) called anorexia nervosa. But she is not alone. Many people have this eating disorder.In the beginning of her story, Kessa is a normal 15-year-old. She is good at many things, especially dancing. She has danced for many years and loves it. One day her dance teacher tells her to continue eating right, but maybe lose a few pounds. Once Kessa hears this, she takes things too far. Instead of cutting down on snacks and junk food, she decides not to eat at all. She does not eat breakfast, lunch, or dinner. She begins losing weight and becomes slimmer (更加苗条的) and slimmer. But she loses weight to a point where she is unhealthy.As her poor eating habits continue, her parents start getting as much help as possible to cure (治疗) their beautiful daughter. But it is just as hard for Kess a’s parents to deal with her disorder as it is for her. Everyday she exercises to lose more pounds and plans what and when she will eat. Her parents try everything, but Kessa decides not to have any fat on her body.Kessa’s doctor and parents finally take her to the hospital. She is now so thin that she can hardly walk. There, she is given good care.In the rest of the book, Kessa goes through a lot of trouble in order to cure her eating disorder. This book, I think, can help to prevent people from doing this to themselves. It shows the trouble that people go through just to be slimmer, and all theterrible things they must experience to be cured. It is a book I think every teenager should read.28. What does Kessa do to lose weight?A. She stops eating.B. She eats less junk food.C. She has three small meals a day.D. She dances many more hours a day.29. What do we know about Kessa’s eating disorder?A. It is caused by her dance teacher.B. It’s too serious to be cured.C. It makes her suffer a lot.D. It’s an unusual illness.30. The book mentioned in the text is mainly about _____.A. how to cure eating disordersB. the importance of eating rightC. a girl’s fight against an eating problemD. why so many people have eating disordersDTime for Kids (TFK) gets the great news on the coolest toys for 2014 at the 111th American International Toy Fair.What’s four days long, bigger than seven football fields, and filled with thousands of toys? It’s Toy Fair 2014!Each year, hundreds of toy companies from across the country come together in New York City. All kinds of products are shown as a way to know what’s to come in the new year. This year’s trends (款式) include oversize toys. “In 2014, everything is really big and really out there,” says Adrienne Appell, a toy-trends expert.Toy Fair is the largest toy trade show in the Western Hemisphere (西半球). Toy-store buyers go to the event to decide which toys they may want to sell during the holiday season. Members of the media, like TFK, go to the fair to report back on all the cool trends. Unfortunately, Toy Fair is not open to the public.This year’s Toy Fair was the biggest in its 111-year history. More than 1,000 toy companies showed products from simple card games to high-tech robots. “Larger Than Life” was a Toy Fair favorite. This trend is all about big toys with a big play value.Though it is all fun and games at Toy Fair, we saw lots of products making a push towards education, with STEAM. That stands for science, technology, engineering, art, and math. This trend includes word games. And of course, we saw some super cool, high-tech games. “Technology is always going to influence toys,” says Appell. “This year we saw lots of 3D games, and robots.”To know more information about the event, click (点击) here to watch the video.31. The American International Toy Fair _____.A. can be visited by everyoneB. attracts very few peopleC. is held every two yearsD. has a long history32. What can we infer from Toy Fair 2014?A. Big toys will be popular this year.B. Robots are great favorites of buyers.C. Toys are becoming more educational than fun.D. Simple card games will disappear on the market.33. What’s special about Toy Fair 2014?A. It is the largest.B. It is the longest.C. It sells all of its toys.D. It’s held in New York City.34. What’s the best title for the text?A. Kids love toysB. Time for toysC. Technology changes toysD. Toy-stores in New York City35. The text is taken from a _____.A. magazineB. newspaperC. websiteD. textbook第二节（共5小题；每小题2分，满分10分）根据短文内容，从短文后的选项中选出能填入空白处的最佳选项。

2021-2022学年新教材高中数学模块综合训练课后练习(含解析)新人教B版选择性必修第一册

模块综合训练一、单项选择题:本题共8小题,每小题5分,共40分.在每小题给出的四个选项中,只有一项是符合题目要求的.1.“ab=4”是“直线2x+ay-1=0与直线bx+2y-2=0平行”的( )A.充分不必要条件B.必要不充分条件C.充要条件D.既不充分也不必要条件两直线平行,∴斜率相等.即可得ab=4,又因为不能重合,当a=1,b=4时,满足ab=4,但是重合,故“ab=4”是“直线2x+ay-1=0与直线bx+2y-2=0平行”的必要不充分条件.2.如图,四面体S-ABC 中,D 为BC 中点,点E 在AD 上,AD=3AE ,则SE ⃗⃗⃗⃗⃗ =( ) A.13SA⃗⃗⃗⃗⃗ +12SB ⃗⃗⃗⃗⃗ +13SC ⃗⃗⃗⃗ B.23SA ⃗⃗⃗⃗⃗ +16SB ⃗⃗⃗⃗⃗ +16SC ⃗⃗⃗⃗ C.12SA ⃗⃗⃗⃗⃗ +14SB ⃗⃗⃗⃗⃗ +14SC ⃗⃗⃗⃗ D.12SA ⃗⃗⃗⃗⃗ +13SB ⃗⃗⃗⃗⃗ +16SC ⃗⃗⃗⃗S-ABC 中,D 为BC 中点,点E 在AD 上,AD=3AE ,∴SE ⃗⃗⃗⃗⃗ =SA ⃗⃗⃗⃗⃗ +13AD ⃗⃗⃗⃗⃗ =SA⃗⃗⃗⃗⃗ +13×12(AC ⃗⃗⃗⃗⃗ +AB ⃗⃗⃗⃗⃗ )=SA ⃗⃗⃗⃗⃗ +16AC ⃗⃗⃗⃗⃗ +16AB ⃗⃗⃗⃗⃗ =SA ⃗⃗⃗⃗⃗ +16(SC ⃗⃗⃗⃗ −SA ⃗⃗⃗⃗⃗ )+16(SB ⃗⃗⃗⃗⃗ −SA ⃗⃗⃗⃗⃗ )=23SA ⃗⃗⃗⃗⃗ +16SB ⃗⃗⃗⃗⃗ +16SC ⃗⃗⃗⃗ .3.圆P :(x+3)2+(y-4)2=1关于直线x+y-2=0对称的圆Q 的标准方程是( ) A.(x+2)2+(y-1)2=1 B.(x+2)2+(y-5)2=1 C.(x-2)2+(y+5)2=1 D.(x-4)2+(y+3)2=1P :(x+3)2+(y-4)2=1,圆心(-3,4),半径1,关于直线x+y-2=0对称的圆半径不变,设对称圆的圆心为(a ,b ),则{a -32+b+42-2=0,b -4a+3=1,解得{a =-2,b =5,所求圆Q 的标准方程为(x+2)2+(y-5)2=1.4.(2021新高考Ⅰ,5)已知F 1,F 2是椭圆C :x 29+y 24=1的两个焦点,点M 在C 上,则|MF 1|·|MF 2|的最大值为( )A.13B.12C.9D.6|MF 1|+|MF 2|=2a=6,则√|MF 1|·|MF 2|≤|MF 1|+|MF 2|2=3, 则|MF 1|·|MF 2|≤9,当且仅当|MF 1|=|MF 2|=3时,等号成立. 故|MF 1|·|MF 2|的最大值为9.故选C .5.坐标原点O(0,0)在动直线mx+ny-2m-2n=0上的投影为点P,若点Q(-1,-1),那么|PQ|的取值范围为()A.[√2,3√2]B.[√2,2√2]C.[2√2,3√2]D.[1,3√2]mx+ny-2m-2n=0,可化为m(x-2)+n(y-2)=0,故直线过定点M(2,2),坐标原点O(0,0)在动直线mx+ny-2m-2n=0上的投影为点P,故∠OPM=90°,所以P 在以OM为直径的圆上,圆的圆心N为(1,1),半径为√2,根据点与圆的关系,|NQ|=√(1+1)2+(1+1)2=2√2,故√2=2√2−√2≤|PQ|≤√2+2√2=3√2.6.正确使用远光灯对于夜间行车很重要.已知某家用汽车远光灯(如图)的纵断面是抛物线的一部分,光源在抛物线的焦点处,若灯口直径是20 cm,灯深10 cm,则光源到反光镜顶点的距离是()A.2.5 cmB.3.5 cmC.4.5 cmD.5.5 cmxOy ,如图所示,设对应抛物线的标准方程为y 2=2px ,由题意知抛物线过点(10,10),得100=2p×10,得p=5,则p 2=2.5,即焦点坐标为(2.5,0), 则光源到反光镜顶点的距离是2.5cm .7.如图,四棱锥S-ABCD 中,底面是正方形,各棱长都相等,记直线SA 与直线AD 所成角为α,直线SA 与平面ABCD 所成角为β,二面角S-AB-C 的平面角为γ,则( ) A.α>β>γ B.γ>α>β C.α>γ>β D.γ>β>αAC ,BD ,交于点O ,连接OS ,则OA ,OB ,OS 两两垂直,以O 为原点,OA 为x 轴,OB 为y 轴,OS 为z 轴,建立空间直角坐标系,设|AB|=2,则S (0,0,√2),A (√2,0,0),D (0,-√2,0),B (0,√2,0),SA ⃗⃗⃗⃗⃗ =(√2,0,-√2),AD ⃗⃗⃗⃗⃗ =(-√2,-√2,0),SB ⃗⃗⃗⃗⃗ =(0,√2,-√2),cos α=|SA ⃗⃗⃗⃗⃗ ·AD⃗⃗⃗⃗⃗⃗ ||SA ⃗⃗⃗⃗⃗ |·|AD⃗⃗⃗⃗⃗⃗ |=√4×√4=12,平面ABCD 的法向量n =(0,0,1),cos β=|n ·SA ⃗⃗⃗⃗⃗||n |·|SA ⃗⃗⃗⃗⃗ |=√2√4=√22,设平面SAB 的法向量m =(x ,y ,z ),则{m ·SA ⃗⃗⃗⃗⃗=√2x -√2z =0,m ·SB ⃗⃗⃗⃗⃗=√2y -√2z =0,取x=1,得m =(1,1,1),cos γ=|m ·n ||m |·|n |=√3=√33, ∵cos α<cos γ<cos β,∴α>γ>β.8.设F 1,F 2是双曲线C :x 2a 2−y 2b 2=1(a>0,b>0)的左、右焦点,O 是坐标原点,过F 2作C 的一条渐近线的垂线,垂足为P.若|PF 1|=√6|OP|,则C 的离心率为( ) A.√5 B.√3 C.2 D.√2|PF 2|=b ,|OF 2|=c ,∴|PO|=a.在Rt △POF 2中,cos ∠PF 2O=|PF 2||OF 2|=bc ,∵在△PF 1F 2中,cos ∠PF 2F 1=|PF 2|2+|F 1F 2|2-|PF 1|22|PF 2||F 1F 2|=bc ,∴b 2+4c 2-(√6a )22b ·2c=bc ⇒c2=3a 2,∴e=√3.二、多项选择题:本题共4小题,每小题5分,共20分.在每小题给出的四个选项中,有多项符合题目要求.全部选对的得5分,有选错的得0分,部分选对得3分.9.(2021新高考Ⅰ,11)已知点P 在圆(x-5)2+(y-5)2=16上,点A (4,0),B (0,2),则( ) A.点P 到直线AB 的距离小于10 B.点P 到直线AB 的距离大于2 C.当∠PBA 最小时,|PB|=3√2 D.当∠PBA 最大时,|PB|=3√2,记圆心为M ,半径为r ,则M (5,5),r=4.由条件得,直线AB 的方程为x 4+y2=1,整理得x+2y-4=0,过点M 作MN 垂直于直线AB ,垂足为N ,直线MN 与圆M 分别交于点P 1,P 2,圆心M (5,5)到直线AB 的距离|MN|=√12+22=√5,于是点P 到直线AB 的距离最小值为|P 2N|=|MN|-r=√5-4,最大值为|P 1N|=|MN|+r=√5+4. 又√5-4<2,√5+4<10,故A 正确,B 错误; 过点B 分别作圆的两条切线BP 3,BP 4,切点分别为点P 3,P 4,则当点P 在P 3处时∠PBA 最大,在P 4处时∠PBA 最小.又|BP 3|=|BP 4|=√|BM |2-r 2=√52+(5-2)2-42=3√2,故C,D 正确.故选A,C,D .10.若a =(-1,λ,-2),b =(2,-1,1),a 与b 的夹角为120°,则λ的值为( ) A.17 B.-17 C.-1 D.1a =(-1,λ,-2),b =(2,-1,1),a 与b 的夹角为120°,∴cos120°=a ·b |a |·|b |=√5+λ2·√6,解得λ=-1或λ=17.11.已知P 是椭圆C :x 26+y 2=1上的动点,Q 是圆D :(x+1)2+y 2=15上的动点,则( ) A.C 的焦距为√5B.C 的离心率为√306C.圆D 在C 的内部D.|PQ|的最小值为2√55c=√6-1=√5,则C的焦距为2√5,e=√5√6=√306.设P(x,y)(-√6≤x≤√6),则|PD|2=(x+1)2+y2=(x+1)2+1-x26=56(x+65)2+45≥45>15,所以圆D在C的内部,且|PQ|的最小值为√45−√15=√55.12.已知直线l过点P(1,0,-1),平行于向量a=(2,1,1),平面α过直线l与点M(1,2,3),则平面α的法向量可能是()A.(1,-4,2)B.(14,-1,12)C.(-14,1,-12) D.(0,-1,1),所研究平面的法向量垂直于向量a=(2,1,1)和向量PM⃗⃗⃗⃗⃗⃗ ,而PM⃗⃗⃗⃗⃗⃗ =(1,2,3)-(1,0,-1)=(0,2,4),选项A,(2,1,1)·(1,-4,2)=0,(0,2,4)·(1,-4,2)=0满足垂直,故正确;选项B,(2,1,1)·(14,-1,12)=0,(0,2,4)·(14,-1,12)=0满足垂直,故正确;选项C,(2,1,1)·(-14,1,-12)=0,(0,2,4)·(-14,1,-12)=0满足垂直,故正确;选项D,(2,1,1)·(0,-1,1)=0,但(0,2,4)·(0,-1,1)≠0,故错误.三、填空题:本题共4小题,每小题5分,共20分.13.过点(1,√2)的直线l将圆x2+y2-4x=0分成两段弧,当劣弧所对圆心角最小时,直线l 的斜率k= .(1,√2)的直线l将圆(x-2)2+y2=4分成两段弧,当劣弧所对的圆心角最小时,就是弦长最小,就是与圆心(2,0)和点(1,√2)的连线垂直的直线,连线的斜率是√2-01-2=-√2,直线l的斜率k=√22.14.(2021新高考Ⅰ,14)已知O为坐标原点,抛物线C:y2=2px(p>0)的焦点为F,P为C上一点,PF与x轴垂直,Q为x轴上一点,且PQ⊥OP.若|FQ|=6,则C的准线方程为.x=-32PF⊥x轴,∴x P=x F=p2,将x P=p2代入y2=2px,得y=±p.不妨设点P在x轴的上方,则P(p2,p),即|PF|=p.如图,由条件得,△PFO∽△QFP,∴|OF||PF|=|PF||QF|,即p2p=p6,解得p=3.故C的准线方程为x=-32.15.如图,在直三棱柱ABC-A1B1C1中,∠ACB=90°,AA1=AC=BC=1,则异面直线BC1与A1B1所成角为;二面角A-BC1-C的余弦值是.√33C 为原点建立如图空间直角坐标系,则A (0,1,0),B (1,0,0),C 1(0,0,1),A 1(0,1,1),B 1(1,0,1),BC 1⃗⃗⃗⃗⃗⃗⃗ =(-1,0,1),A 1B 1⃗⃗⃗⃗⃗⃗⃗⃗⃗ =(1,-1,0),AB⃗⃗⃗⃗⃗ =(1,-1,0).由cos <BC 1⃗⃗⃗⃗⃗⃗⃗ ,A 1B 1⃗⃗⃗⃗⃗⃗⃗⃗⃗ >=|√2×√2|=12,故异面直线BC 1与A 1B 1所成角为π3, 设平面ABC 1的一个法向量为m =(a ,b ,c ),由{m ·BC 1⃗⃗⃗⃗⃗⃗⃗ =-a +c =0,m ·AB⃗⃗⃗⃗⃗ =a -b =0,设a=1,得m =(1,1,1),平面BC 1C 的一个法向量n =(0,1,0),cos <m ,n >=√3=√33.16.已知抛物线的方程为x 2=2py (p>0),过抛物线的焦点,且斜率为1的直线与抛物线交于A ,B 两点,|AB|=8,则p= ,M 为抛物线弧AOB⏜上的动点,△AMB 面积的最大值是 .4√2抛物线的方程为x 2=2py (p>0),过抛物线的焦点F ,且斜率为1的直线与抛物线交于A ,B 两点,故直线AB 的方程为y-p 2=x-0,即y=x+p2,且直线AB 的倾斜角为45°. 代入抛物线的方程x 2=2py ,可得x 2-2px-p 2=0.设A ,B 两点的横坐标分别为m ,n ,m<n ,由根与系数的关系可得m+n=2p ,mn=-p 2.∵|AB|=|AF|+|BF|=(yA +p2)+y B+p2=(m+p2)+p2+(n+p2)+p2=8=m+n+2p=4p=8,∴p=2,故抛物线的方程为x2=4y,直线AB为y=x+1.设与直线AB平行且与抛物线相切的直线方程为y=x+m,代入抛物线方程,得x2-4x-4m=0.由Δ=42+16m=0,得m=-1.与直线AB平行且与抛物线相切的直线方程为y=x-1,两直线间的距离为d=√2=√2,∴△AMB面积的最大值为12·|AB|·d=12×8×√2=4√2.四、解答题:本题共6小题,共70分.解答应写出文字说明、证明过程或演算步骤.17.(10分)求分别满足下列条件的直线l的方程.(1)已知点P(2,1),l过点A(1,3),P到l距离为1;(2)l过点P(2,1)且在x轴,y轴上截距的绝对值相等.当l斜率不存在时,l的方程为x=1,满足条件.当l斜率存在时,设l:y-3=k(x-1),即kx-y+3-k=0,由d=√k2+1=1,得k=-34,即l:3x+4y-15=0.故直线l的方程为x=1或3x+4y-15=0.(2)当直线过原点时,直线的斜率为1-02-0=12,直线l的方程为x-2y=0.当直线截距相等时,设为xa +ya=1,代入(2,1),则a=3,即x+y-3=0.当直线截距互为相反数时,设为xa +y-a=1代入(2,1),则a=1,即x-y-1=0.综上,要求的直线l 的方程为x-2y=0或x+y-3=0或x-y-1=0. 18.(12分)(2021新高考Ⅰ,21)在平面直角坐标系xOy 中,已知点F 1(-√17,0),F 2(√17,0),点M 满足|MF 1|-|MF 2|=2.记M 的轨迹为C.(1)求C 的方程;(2)设点T 在直线x=12上,过T 的两条直线分别交C 于A ,B 两点和P ,Q 两点,且|TA|·|TB|=|TP|·|TQ|,求直线AB 的斜率与直线PQ 的斜率之和.∵|MF 1|-|MF 2|=2,且F 1(-√17,0),F 2(√17,0),∴点M的轨迹为双曲线的右支,且满足{2a =2,c =√17,c 2=a 2+b 2,∴{a 2=1,b 2=16,c 2=17.∴C 的方程为x 2-y 216=1(x ≥1).(2)设T (12,m),显然直线AB 的斜率与直线PQ 的斜率都存在.设直线AB 的方程为y=k 1(x -12)+m ,A (x 1,y 1),B (x 2,y 2), 由{y =k 1(x -12)+m ,16x 2-y 2=16,得16x 2-k 12(x 2-x +14)+2k 1m (x -12)+m2=16,即(16-k 12)x 2+(k 12-2k 1m )x-14k 12+k 1m-m 2-16=0. ∴|TA|·|TB|=(1+k 12)x 1-12x 2-12=(1+k 12)x 1x 2-12(x 1+x 2)+14=(1+k 12)k 1m -14k 12-m 2-1616-k 12−12·2k 1m -k 1216-k 12+14=(1+k 12)-m 2-1216-k 12=(1+k 12)·m 2+12k 12-16.设k PQ =k 2,同理可得|TP|·|TQ|=(1+k 22)·m 2+12k 22-16. ∵|TA|·|TB|=|TP|·|TQ|,∴(1+k 12)·m 2+12k 12-16=(1+k 22)·m 2+12k 22-16. ∴k 22-16k 12=k 12-16k 22.∴k 12=k 22.∵k 1≠k 2,∴k 1=-k 2. ∴k 1+k 2=0.19.(12分)已知椭圆C :x 2a 2+y 2b 2=1(a>b>0)过点A (-2,0),点B 为其上顶点,且直线AB 的斜率为√32.(1)求椭圆C 的方程;(2)设P 为第四象限内一点且在椭圆C 上,直线PA 与y 轴交于点M ,直线PB 与x 轴交于点N ,求证:四边形ABNM 的面积是定值.,设直线AB :y-0=√32(x+2),令x=0,则y=√3,于是B (0,√3), 所以a=2,b=√3, 故椭圆C 的方程为x 24+y 23=1.(2)设P (x 0,y 0)(x 0>0,y 0<0),且3x 02+4y 02=12,又A (-2,0),B (0,√3),所以直线AP :y -0y 0-0=x+2x 0+2,令x=0,y M =2y 0x 0+2,则|BM|=√3-y M =√3−2y 0x 0+2=√3x 0+2√3-2y 0x 0+2. 直线BP :√3y -√3=x -0x 0-0,令y=0,x N =√3x 0y -√3,则|AN|=2+x N=2+√3x0y-√3=0√3-√3x0y-√3.所以四边形ABNM的面积为S=12|BM|·|AN|=1 2×√3x0+2√3-2y0x0+2×0√3-√3x0y-√3=0202√3x000√3y02(x y-√3x+2y-2√3)=√3(00√3x00√3)2(λy-√3x+2y-2√3)=2√3,所以四边形ABNM的面积为定值2√3.20.(12分)如图,在四棱锥P-ABCD中,底面ABCD是菱形,∠ABC=120°,PA=PC,PB=PD,AC∩BD=O.(1)证明:PO⊥平面ABCD;(2)若PA与平面ABCD所成的角为30°,求二面角B-PC-D的余弦值.四边形ABCD是菱形,∴O为AC,BD的中点.又PA=PC,PB=PD,∴PO⊥AC,PO⊥BD.∵AC∩BD=O,且AC,BD⊂平面ABCD,∴PO⊥平面ABCD.ABCD的边长为2t(t>0).∵∠ABC=120°,∴∠BAD=60°,∴OA=√3t.由(1)知PO ⊥平面ABCD ,∴PA 与平面ABCD 所成的角为∠PAO=30°,得到PO=t ,建立如图所示的空间直角坐标系,则B (0,t ,0),C (-√3t ,0,0),P (0,0,t ),D (0,-t ,0),得到BP ⃗⃗⃗⃗⃗ =(0,-t ,t ),CP⃗⃗⃗⃗⃗ =(√3t ,0,t ). 设平面PBC 的法向量n 1=(x 1,y 1,z 1),平面PCD 的法向量n 2=(x 2,y 2,z 2).则{n 1·BP ⃗⃗⃗⃗⃗=0,n 1·CP ⃗⃗⃗⃗⃗=0,即{-ty 1+tz 1=0,√3tx 1+tz 1=0.令x=1,则y=z=-√3,得到n 1=(1,-√3,-√3). 同理可得n 2=(1,√3,-√3),所以|cos <n 1,n 2>|=|n 1·n 2||n 1||n 2|=17.因为二面角B-PC-D 为钝二面角,则余弦值为-17.21.(12分)在平面直角坐标系xOy 中,曲线Γ:y=x 2-mx+2m (m ∈R )与x 轴交于不同的两点A ,B ,曲线Γ与y 轴交于点C.(1)是否存在以AB 为直径的圆过点C ?若存在,求出该圆的方程;若不存在,请说明理由. (2)求证:过A ,B ,C 三点的圆过定点,并求出该定点的坐标.由曲线Γ:y=x 2-mx+2m (m ∈R ),令y=0,得x 2-mx+2m=0. 设A (x 1,0),B (x 2,0),则可得Δ=m 2-8m>0,x 1+x 2=m ,x 1x 2=2m. 令x=0,得y=2m ,即C (0,2m ).若存在以AB 为直径的圆过点C ,则AC⃗⃗⃗⃗⃗ ·BC ⃗⃗⃗⃗⃗ =0,得x 1x 2+4m 2=0,即2m+4m 2=0, 所以m=0或m=-12.由Δ>0,得m<0或m>8,所以m=-12,此时C (0,-1),AB 的中点M (-14,0)即圆心,半径r=|CM|=√174.故所求圆的方程为(x +14)2+y 2=1716. (2)设过A ,B ,C 的圆P 的方程为(x-a )2+(y-b )2=r 2满足{(x 1-a )2+b 2=r 2,(x 2-a )2+b 2=r 2,a 2+(2m -b )2=r 2,x 1x 2=2m ,x 1+x 2=m⇒{ a =m2,r 2=5m 24-m +14,b =m +12,代入P 得(x -m 2)2+y-m-122=5m 24-m+14,展开得(-x-2y+2)m+x 2+y 2-y=0, 当{-x -2y +2=0,x 2+y 2-y =0,即{x =0,y =1或{x =25,y =45时方程恒成立, ∴圆P 方程恒过定点(0,1)或(25,45).22.(12分)某高速公路隧道设计为单向三车道,每条车道宽4米,要求通行车辆限高5米,隧道全长1.5千米,隧道的断面轮廓线近似地看成半个椭圆形状(如图所示).(1)若最大拱高h 为6米,则隧道设计的拱宽l 至少是多少米?(结果取整数)(2)如何设计拱高h 和拱宽l ,才能使半个椭圆形隧道的土方工程量最小?(结果取整数) 参考数据:√11≈3.3,椭圆的面积公式为S=πab ,其中a ,b 分别为椭圆的长半轴和短半轴长.建立直角坐标系xOy如图所示,则点P(6,5)在椭圆x2a2+y2b2=1上,将b=h=6与点P(6,5)代入椭圆方程,得a=√11,此时l=2a=√11≈21.8,因此隧道设计的拱宽l至少是22米.(2)由椭圆方程x2a2+y2b2=1,得36a2+25b2≤1,因为1≥36a2+25b2≥2×6×5ab,即ab≥60,S=πab2≥30π,当且仅当6a=5b时,等号成立.由于隧道长度为1.5千米,故隧道的土方工程量V=1.5S≥45π,当V取得最小值时,有6a =5b,且ab=60,得a=6√2,b=5√2,此时l=2a=12√2≈16.97,h=b≈7.07.①若h=b=8,此时l=2a=17,此时V1=3πab4=3×17×8π8=51π,②若h=b=7,此时l=2a=18,此时V2=3πab4=3×9×7π4=47.25π,因为V1>V2,故当拱高为7米、拱宽为18米时,土方工程量最小.。

人教版高一英语必修一Unit1单元测试题(含答案)

人教版高一英语必修一单元测试Unit 1 FriendshipI.单项选择15分1 .You should have your hair cut; it’s getting_________.A. too much longB. much too longC. long too muchD. too long much2. It was in Shanghai _________I first met Mr. Smith.A. thatB. howC. whichD. when3 . ---I’m sorry. I _________ you last Sunday, but I forgot your telephone number.A. should inviteB. should have invitedC. must inviteD. must have invited4. ---Have you seen the film before? ---No. This is the first time that I ______ it.A. seeB. sawC. have seenD. had seen5. The thief was caught. The policeman ___________his bag and found the stolen wallet.A. looked upB. turned upC. searched forD. went through6. Please tell me _______ you are getting on with your new classmates.A. how B what C. where D. whether7. There was a time _________ I liked drawing.A. thatB. whenC. whileD. what8. The teacher said that practice ________ perfect.A. madeB. would makeC. will make D makes9. He asked ________ for the book.A. did I pay how muchB. I paid how muchC. how much I paidD. how much did I pay10. She decided to _________ the club to have dance training.A. join B join in C. take part in D. attend11. I find _________ difficult to translate this sentence into English.A. IB. thatC. her D it12. Today is my birthday, and his coming ________ my happiness.A. adds upB. adds toC. adds up toD. adds13. __________ wake his parents, the boy walked into the room quietly.A. In order not toB. In order to notC. So as not toD. So that not14. If ___________, you can use my car.A. it necessaryB. is necessaryC. necessary D, you are necessary15. ---Where is our head teacher? ---I saw him _____________ just now.A. went upstairsB. go upstairsC. go to upstairsD. go onto upstairsII、完形填空15分How do you repair a broken friendship? I will give you several tips below.To begin with, a __1__ friend is a treasure. You don’t throw a friend away because your feelings may get hurt or you are unwilling to __2__ a misunderstanding. Don’t let senseless things __3__ your friendship. To me, if it’s worth the fight, it’s worth the sacrifice(牺牲). If you can’t sacrifice to __4__the friendship, then maybe it isn’t a true friendship.Be willing to say__5__even if it isn’t your fault. The friendship is __6__ than who is at fault. If you can __7__the friendship with an apology, then do so. Don’t wait for your friends to realize __8__wrong they are. Go to them, make the first __9__. Raise the __10__ flag first. However you want to __11__ it, be willing to do what it may take to repair the relationship. Many friendships stay __12__ because both waited for the other to make the first move and neither did.Remind (提醒) each other that the friendship is __13__ important. Some simple words of how special the friendship is will go a long way to soften a __14__heart.When __15__ a friendship, you must remember that together you can do much more than either can do alone. A friendship is full of synergy (协同作用).1. A. health B. real C. playing D. well2. A. discuss B. tell C. speak D. say3. A. destroyed B. to destroy C. destroying D. destroy4. A. find B. like C. keep D. break5. A. good B. hello C. OK D. sorry6. A. larger B. large C. smaller D. small7. A. buy B. repair C. destroy D. stop8. A. where B. that C. how D. when9. A. talk B. laugh C. move D. go10. A. red B. white C. green D. blue11. A. look at B. look for C. look out D. look up12. A. broken B. awake C. normal D. good13. A. less B. little C. more D. much14. A. warm B. kind C. friendly D. hard15. A. repaired B. repairing C. repair D. to repairIII 阅读理解16分AOnce there was a poor farmer and his farm belonged to(属于) a rich man. One day he brought a basket of apples to the rich man’s house. On the doorsteps, he met two monkeys dressed like children. They jumped onto the basket to eat the apples and threw some on the ground. The farmer politely took off his hat and asked the monkeys to get off. They obeyed(服从) and the farmer went into the house. He asked to see the rich man. A servant took him to the room where the rich man was sitting."I have brought you the basket of apples you asked for," he said."But why have you brought a half-empty basket?" the rich man asked."I met your children outside, and they stole(偷) some of the apples."1. Why did the farmer bring apples to the rich man? BecauseA. he was poorB. he liked the rich manC. his farm belonged to the rich manD. the rich man’s children liked apples2. What did the monkeys do when the farmer was on the doorsteps?A. They jumped and jumped.B. They played.C. They ran away.D. They ate some of the apples.3. The monkeys left the basket because _______________.A. they had thrown apples on the groundB. the farmer had politely asked them to get offC. the famer would beat. themD. the farmer was angry with them4. How did the rich man feel when he saw the basket? He felt ________.A. pleasedB. unhappyC. excitedD. movedBWhen I was about 12, I had an enemy, a girl who liked to point out my shortcomings(缺点). Wee k by week her list grew: I was very thin, I wasn’t a good student, I talked too much, I was too proud, and so on. I tried to hear all this as long as I could. At last, I became very angry. I ran to my father with tears in my eyes.He listened to me quietly, then he asked. “Are the things she says true or not? Janet, didn’t you ever wonder what you’re really like ? Well, you now have that girl’s opinion. Go and make a list of everything she said and mark the points that are true. Pay no attention to the other things shesaid.” I did as he told me. To my great surprise, I discovered that about half the things were true. Some of them I couldn’t change (like being very thin), but a good number I could—and suddenly I wanted to change. For the first time I go to fairly clear picture of myself.I brought the list back to Daddy. He refused to take it. “That’s just for you,” he said.“You know better than anyone else the truth about yourself. But you have to learn to listen, not just close your ears in anger and feeling hurt. When something said about you is true, you’ll find it will be of help to you. Our world is full of people who think they know your duty. Don’t shut your ear s. Listen to them all, but hear the truth and do what you know is the right thing to do.”Daddy’s advice has returned to me at many important moments. In my life, I’ve never had a better piece of advice.5. What did the father do after he had heard his daughter’s complaint?A. He told her not to pay any attention to what her “enemy” had said.B. He criticized (批评) her and told her to overcome her shortcomings.C. He told her to write down all that her “enemy” had said about her and pay attention only to the things that were true.D. He refused to take the list and have a look at it.6. What does “Week by week her list grew” mean?A. Week by week she discovered more shortcomings of mine and pointed them out to me.B. She had made a list of my shortcomings and she kept on adding new ones to it so that it wasgrowing longer and longer.C. I was having more and more shortcomings as time went on.D. Week by week, my shortcomings grew more serious.7. Why did her father listen to her quietly?A. Because he believed that what her daughter’s “enemy” said was mostly true.B. Because he had been so angry with his daughter’s shortcomings that he wanted to show thisby keeping silent for a while.C. Because he knew that his daughter would not listen to him at that moment.D. Because he wasn’t quite sure which girl was telling the truth.8. Which do you think would be the best title for this passage?A. Not an Enemy, but the Best FriendB. The Best Advice I’ve Ever HadC. My FatherD. My Childhood单元测试Unit 1 Friendship班级__________ 姓名_______________________ 座号______ 得分______（满分100分）( 请把单选, 完型填空,和阅读题的答案填写在下面空格处)I.单选: 1___ 2___ 3___ 4___ 5___ 6___ 7___ 8___ 9___ 10___11___ 12___ 13___ 14___ 15___II.完型: 1___ 2___ 3___ 4___ 5___ 6___ 7___ 8___ 9___ 10___ 11___ 12___ 13___ 14___ 15___III. 阅读: A篇: 1___ 2___ 3___ 4___ B 篇: 5___ 6___ 7___ 8___ (请继续完成以下试题)IV单词拼写; 10分1She is __________ (心烦意乱) because she failed the examination yesterday.2He was punished by the school because he c____________(作弊) in the exam.3Many t_______________(青少年) are crazy about computer games and chatting on line.4I said hello to her, but she i____________________( 不理睬) me and walked on.5I’m very c______________________(担心) about your safety.6He has r_________________( 康复) from the illness.7I studied some Japanese at college, but I’ve ____________(完全地)forgot it now.8Don’t always stay indoors. You’d better go o_______________ for fun.9They left their hometown and s ______________(定居) in Fuzhou two years ago.10I’m afraid I d___________________( 不同意) with your view.V. 用所给词组的正确形式填空; 20分1 Every time he _________________ the figures(数据), he gets a different answer.2 Most of the bit cities of the world _________________________ traffic jam.3 The boys find it hard to ____________________________Tom, because he is often angryover little things.4 I will _______________ the story as it was told to me.5 I don’t think you broke my glasses __________________. Forget it!6 She said she _____________________ watching TV and she would go out for a walk.7 The girl hoped to see the famous film star ___________________________.8 The poor boy has just _____________________ a big earthquake and lost his family..9 When we are faced with danger, we should _____________________________.10 She reads more ______________________________ improve her English.VI. 句型转换24 分1 To improve safety, more high-speed trains slow down._____ ___________ _____ improve safety, more high-speed trains slow down.2 While I was doing my homework, I heard the door bell ring.__________ _______________ my homework, I heard the door bell ring.3 “ Can you swim, John?” he asked.( 改成间接引语)He asked John ____________ ___________ ___________ swim.4 “ Who will attend the meeting tomorrow?” she asked. ( 改成间接引语)She asked who _________ attend the meeting _____ _________ ________.5 Don’t open the window.” He said to me. ( 改成间接引语)He told me ______ ______ open the window.6 “Did you borrow the book yesterday?” Li Ming said to me. ( 改成间接引语)Li Ming asked me whether I ____________________ the book the day ___________.7 I lost my bicycle at the school gate. ( 改成强调句)______ _________ at the school gate __________ I lost my bicycle.8 The bad news upset her.She ______ __________ about the bad news.9 His long speech tired all of us.All of us were _________ _______ his long speech.参考答案:I. 单选: 1-5 B A B C D 6-10 A B D C A 11-15 D B A C BII. 完型: 1-5 B A D C D 6-10 A B C C B 11-15 A A C D BIII 阅读: 1-4 C D B B 5-8 C A A BIV 单词: 1 upset 2 cheated 3 teenagers 4 ignored 5 concerned6 recovered7 entirely8 outdoors9 settled 10 disagree V. 词组: 1 adds up 2 suffer from 3 get along with 4 set down5 on purpose6 was tired of7 face to face8 gone through9 calm down 10 in order toVI 句型; 1 In order to 2 While doing 3 if/whether he could4 would…the next day5 not to6 had borrowed…before7 It was …that 8 was upset 9 tired of。

(人教版B版)高中数学必修第一册第三章综合测试试卷01及答案

第三章综合测试一、选择题（本大题共12小题，每小题5分，共60分.在每小题给出的四个选项中，只有一项是符合题目要求的）1.已知2()1f x x =+，则[(1)]f f -的值等于（）A .2B .3C .4D .52.已知函数()1f x x =+，其定义域为{1,0,1,2}-，则函数的值域为（）A .[0,3]B .{0,3}C .{0,1,2,3}D .{|0}y y …3.函数y = ）A .{|01}x x ……B .{| 1 1}x x x --＜或＞C .{|01}x x x ¹-＜且D .{}|1 0x x x ¹-¹且4.已知二次函数()y f x =满足(2)(2)f x f x +=-，且函数图像截x 轴所得的线段长为8，则函数()y f x =的零点为（）A .2，6B .2，6-C .2-，6D .2-，6-5.若函数()y f x =的定义域是{|01}x x ≤≤，则函数()()(2)(01)F x f x a f x a a =+++＜＜的定义域是（）A .1|22a a x x -ìü-íýîþ≤B .|12a x x a ìü--íýîþ≤C .{|1}x a x a --≤≤D .1|2a x a x -ìü-íýîþ≤≤6.如图所示，可表示函数()y f x =的图像的只可能是（）A B C D7.已知函数2()1f x ax bx =++为定义在[2,1]a a -上的偶函数，则a b +的值是（）A .1B .1-C .1或1-D .0或18.若()f x 满足()()f x f x -=-，且在(,0)-¥上是增函数，(2)0f -=，则()0xf x ＜的解集是（）A .(2,0)(0,2)-UB .(,2)(0,2)-¥-UC .(,2)(2,)-¥-+¥U D .(2,0)(2,)-+¥U 9.设函数()f x 与()g x 的定义域是{|1}x x Î¹±R ，函数()f x 是一个偶函数，()g x 是一个奇函数，且1()()1f xg x x -=-，则()f x 等于（）A .2221x x -B .211x -C .221x -D .221x x -10.已知2()21(0)f x ax ax a =++＞，若()0f m ＜，则(2)f m +与1的大小关系式为（）A .(2)1f m +＜B .(2)1f m +=C .(2)1f m +＞D .(2)1f m +…11.函数()f x =（）A .是奇函数但不是偶函数B .是偶函数但不是奇函数C .既是奇函数又是偶函数D .既不是奇函数又不是偶函数12.已知2()2f x x x =+，若存在实数t ，使()3f x t x +…对[1,]x m Î恒成立，则实数m 的最大值是（）A .6B .7C .8D .9二、填空题（本大题共4小题，每小题5分，共20分.请把正确答案填在题中的横线上）13.已知1,[0,1],()2,[0,1],x f x x x Îì=í-Ïî，当[()]1f f x =时，x Î__________.14.关于x 的方程240x x a --=有四个不相等的实数根，则实数a 的取值范围为__________.15.已知函数719()1x f x x +=+，则()f x 的图像的对称中心是__________，集合{}*|()x f x Î=N __________.16.已知函数()f x 是定义在实数集R 上的不恒为零的偶函数，且对任意实数x 都有(1)(1)()xf x x f x +=+，则52f f æöæöç÷ç÷èøèø的值是__________.三、解答题（本大题共6小题，共70分，解答时写出必要的文字说明、证明过程或演算步骤）17.（本小题满分10分）已知函数2()2||1f x x x =--.（1）利用绝对值及分段函数知识，将函数()f x 的解析式写成分段函数；（2）在坐标系中画出()f x 的图像，并根据图像写出函数()f x 的单调区间和值域.18.（本小题满分12分）已知函数()f x 对任意实数x 均有()2(1)f x f x =-+，且()f x 在区间[0]1，上有解析式2()f x x =.（1）求(1)f -和(1.5)f 的值；（2）写出()f x 在区间[2,2]-上的解析式.19.（本小题满分12分）函数2()1ax bf x x +=+是定义在(,)-¥+¥上的奇函数，且1225f æö=ç÷èø.（1）求实数a ，b 的值.（2）用定义证明()f x 在(1,1)-上是增函数；（3）写出()f x 的单调减区间，并判断()f x 有无最大值或最小值.如有，写出最大值或最小值（无需说明理由）.20.（本小题满分12分）已知定义域为R 的单调函数()f x ，且(1)f x -的图像关于点(1,0)对称，当0x ＞时，1()3x f x x=-.（1）求()f x 的解析式；（2）若对任意的t ÎR ，不等式()()22220f t t f t k -+-＜恒成立，求实数k 的取值范围.21.（本小题满分12分）对于定义域为D 的函数()y f x =，若同时满足下列条件：①()f x 在D 内单调递增或单调递减；②存在区间[,]a b D Í，使()f x 在[,]a b 上的值域为[,]a b ，那么称()()x D y f x =Î为闭函数.（1）求闭函数3y x =-符合条件②的区间[,]a b .（2）判断函数31()(0)4f x x x x=+＞是否为闭函数？并说明理由；（3）判断函数y k =+是否为闭函数？若是闭函数，求实数k 的取值范围.22.（本小题满分12分）设函数()f x 的定义域为R ，当0x ＞时，()1f x ＞，对任意,x y ÎR ，都有()()()f x y f x f y +=g ，且(2)4f =.（1）求(0)f ，(1)f 的值.（2）证明：()f x 在R 上为单调递增函数.（3）若有不等式1()2f x f x x æö+ç÷èøg ＜成立，求x 的取值范围.第三章测试答案解析一、1.【答案】D【解析】由条件知(-1)2f =，(2)5f =，故选D .2.【答案】C【解析】将x 的值依次代入函数表达式可得0，1，2，3，所以函数的值域为{0,1,2,3}，故选C .3.【答案】C【解析】由条件知10x +¹且0x x -＞，解得0x ＜且1x ¹-.故选C 4.【答案】C【解析】由于函数()y f x =满足(2)(2)f x f x +=-，所以直线2x =为二次函数()y f x =图像的对称轴，根据二次函数图像的性质，图像与x 轴的交点必关于直线2x =对称.又两交点间的距高为8，则必有两交点的横坐标分别为1246x =+=，2242x =-=-.故函数的零点为2-，6.故选C .5.【答案】A【解析】由条件知01,021,x a x a +ìí+î…………，又01a ＜＜则122a ax --≤≤，故选A .6.【答案】D【解析】由函数定义可得，任意一个x 有唯一的y 与之对应，故选D .7.【答案】B【解析】因为函数2()1f x ax bx =++为定义在[2,1]a a -上的偶函数，所以21a a =-，1a =-，0b =，因此1a b +=-，故选B.8.【答案】A【解析】根据题意可知函数是奇函数，且在(,0)-¥，(0,)+¥上是增函数，对()0xf x ＜，分0x ＞，0x ＜进行讨论，可知解集为(2,0)(0,2)-U ，故选A.9.【答案】B【解析】1()()1f x g x x -=-∵，1()()1f x g x x ---=--∴，1()()1f xg x x +=--∴，21122()111f x x x x =-=-+-∴，21()1f x x =-，故选B .10.【答案】C【解析】因为2()21(0)f x ax ax a =++＞，所以其图像的对称轴为直线1x =-，所以()(2)0f m f m =--＜，又(0)1f =，所以(2)1f m +＞，故选C .11.【答案】A【解析】由定义城可知x ，因此原式化简为()f x =，那么根据函数的奇偶性的定义，可知该函数是奇函数不是偶函数，故选A .12.【答案】C【解析】由题意知，对任意[1,]x m Î，2()2()3x t x t x +++…恒成立，这个不等式可以理解为()f x t +的图像在直线3y x =的图像的下面时x 的取值范围.要使m 最大，需使两图像交点的横坐标分别为1和m .当1x =时，3y =，代入可求得4t =-（0t =舍去）.进而求得另一个交点为(8,24)，故8m =.故选C.二、13.【答案】[0,1][2,3]{5}U U 【解析】因为1,[0,1],()2,[0,1],x f x x x Îì=í-Ïî所以要满足元[()]1f f x =，需()[0,1]f x Î，[0,1]x Î或2[0,1]x -Î或5x =，这样解得x 的取值范围是[0,1][2,3]{5}U U .14.【答案】(0,4)【解析】原方程等价于24x x a -=，在同一坐标系内作出函数24y x x =-与函数y a =的图像，如图所示：平移直线y a =，可得当04a ＜＜时，两图像有4个不同的公共点，相应地方程240x x a --=有4个不相等的实数根，综上所述，可得实数a 的范围为04a ＜＜.15.(1,7)-{13,7,5,4,3,0,1,2,3,5,11}-----【解析】因为函数71912()711x f x x x +==+++，则()f x 的图像的对称中心为(1,7)-，集合{|()}{13,7,5,4,3,0,1,2,3,5,11}x f x *Î=-----N 16.【答案】0【解析】因为()f x 是定义在R 上的偶函数，因此令12x =-，可知11112222f f æöæö-=-ç÷ç÷èøèø，所以102f æö=ç÷èø，分别令32x =-，52x =-，可得302f æö=ç÷èø，502f æö=ç÷èø，令1x =-.得(0)0f =，因此可知502f f æöæö=ç÷ç÷èøèø.三、17.【答案】（1）22321,0()2||121,0x x x f x x x x x x ì--=--=í+-î＜….（2）图像如图所示.单调增区间为(1,0)-，(1,)+¥，单调减区间为(,1)-¥-，(0,1).值域为[2,)-+¥.18.【答案】（1）由题意知(1)2(11)2(0)0f f f -=--+=-=，1111(1,5)(10.5)(0.5)2248f f f =+=-=-´=-.（2）当[0,1]x Î时，2()f x x =；当(1,2]x Î时，1(0,1]x -Î，211()(1)(1)22f x f x x =--=--；当[1,0)x Î-时，1[0,1)x +Î，2()2(1)2(1)f x f x x =-+=-+；当[2,1)x Î--时，1[1,0)x +Î-，22()2(1)22(11)4(2)f x f x x x éù=-+=-´-++=+ëû.所以22224(2),[2,1),2(1),[1,0),(),[0,1],1(1),(1,2].2x x x x f x x x x x ì+Î--ï-+Î-ïï=íÎïï--Îïî19.【答案】（1）2()1ax bf x x +=+∵是奇函数()()f x f x -=-∴，2211ax b ax bx x -++=-++∴，0b =∴.故2()1axf x x =+，又1225f æö=ç÷èø∵，1a =∴（2）证明：由（1）知2()1xf x x =+，任取1211x x -＜＜＜，()()()()()()1212121222121211111x x x x x xf x f x x x x x ---=-=++++1211x x -∵＜＜＜，1211x x -∴＜＜，120x x -＜，1210x x -＞，2110x +＞，2210x +＞，()()120f x f x -∴＜，即()()12f x f x ＜，()f x ∴在(1,1)-上是增函数.（3）单调减区间为(,1),(1,)-¥-+¥.当1x =-时，min 1()2f x =-；当1x =时，max 1()2f x =.20.【答案】（1）由题意知()f x 的图像关于点(0,0)对称，是奇函数，∴(0)0f =当0x ＜时，0x -＞，1()3x f x x--=--∴，又∵函数()f x 是奇函数.∴()()f x f x -=-，1()3x f x x=-∴.综上所述，1(0),()30(0).x x f x xx ì-¹ï=íï=î（2）2(1)(0)03f f =-=∵＜，且()f x 在R 上单调.∴()f x 在R 上单调递减.由()()22220f t t f t k -+-＜，得()()2222f t t f t k ---＜.∵()f x 是奇函数，∴()()2222f t t f k t --＜，又∵()f x 是减函数，∴2222t t k t --＞即2320t t k --＞对任意t ÎR 恒成立，∴4120k D =+＜，得13k -＜.21.【答案】（1）由题意，3y x =-，在[,]a b 上单调递减，则33,,,b a a b b a ì=-ï=-íï>î解得1,1,a b =-ìí=î所以，所求区间为[1,1]-.（2）取11x =，210x =，则()()1273845f x f x ==，即()f x 不是(0,)+¥上的减函数.取，1110x -=，21100x =，()()12331010040400f x f x =++=＜，即()f x 不是(0,)+¥上的增函数.所以，函数在定义域内不单调递增或单调递减，从而该函数不是闭函数.（3）若y k =是闭函数，则存在区间[,]a b ，在区间[,]a b 上，函数()f x 的值域为[,]a b，即a kb k ì=ïí=+ïî∴a ，b为方程x k =+的两个实根，即方程22(21)20(2,)x k x k x x k -++-=-……有两个不等的实根，故两根均大于等于2-，且对称轴在直线2x =-的右边.当2k -…时，有220,(2)2(21)20,212,2k k k ìïD ï-+++-íï+ï-î＞…解得924k --….当2k -＞时，有220,(21)20,21,2k k k k k k ìïD ï-++-íï+ïî＞＞…无解.综上所述，9,24k æùÎ--çúèû.22.【答案】（1）因为(20)(2)(0)f f f +=g ，所以44(0)f =×，所以(0)1f =，又因为24(2)(11)(1)f f f ==+=，且当0x ＞时，()1f x ＞，所以(1)2f =.（2）证明：当0x ＜时，0x -＞，所以()1f x -＞，而(0)[()]()()f f x x f x f x =+-=-g ，所以1()()f x f x =-，所以0()1f x ＜＜，对任意的12,x x ÎR ，当12x x ＜时，有()()()]()()()1212222121f x f x f x x x f x f x f x x -=é-+-=--ë，因为120x x ＜＜，所以120x x -＜，所以()1201f x x -＜＜，即()1210f x x --＜，所以()()120f x f x -＜，即()()12f x f x ＜，所以()f x 在R 上是单调递增函数.（3）因为1()12f x f x æö+ç÷èøg ＜，所以11(1)f x f x æö++ç÷èø＜，而()f x 在R 上是单调递增函数，所以111x x ++，即10x x+＜，所以210x x +＜，所以0x ＜，所以x 的取值范围是(,0)-¥.。

1、下载文档前请自行甄别文档内容的完整性，平台不提供额外的编辑、内容补充、找答案等附加服务。
2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
3、如文档侵犯您的权益，请联系客服反馈,我们会尽快为您处理(人工客服工作时间：9:00-18:30)。

高中数学学习材料（灿若寒星精心整理制作）必修一模块测试试卷满分100分（附加题得分不计入总分）．考试时间：90分钟第Ⅰ卷（选择题，共40分）一、选择题：本大题共10小题，每小题4分，共40分．在每小题给出的四个选项中，只有一个是符合题目要求的．（1）设集合{}{}{}5,2,3,2,1,5,4,3,2,1===B A U ，则()UA B ð等于（）(A) {}2 (B) {}3,2 (C) {}3 ( D) {}3,1 （2）已知()⎪⎩⎪⎨⎧<=>=0,00,,20,2x x x x x f ，则()()()=-2f f f （）(A) 0 (B) 2 (C) 4 ( D) 8（3）函数()()3212++-=mx x m x f 为偶函数，则()x f 在区间()1,3-上（）（A ）单调递增（B ）单调递减（C ）先减后增（D ）先增后减（4）三个数3.07，73.0，3.0ln 的大小关系是（）（A ）3.0ln 3.0773.0>> （B ）73.03.03.0ln 7>>（C ）3.0ln 73.03.07>> （D ）73.03.073.0ln >>（5）若221xa=-，则33x xx x a a a a --++等于（）（A ）221- （B ）222- （C ）221+ （D ）21+（6）函数()23log 21-=x y 的定义域是(A)[)+∞,1 (B) ⎪⎭⎫⎝⎛+∞,32 （C ）⎥⎦⎤⎢⎣⎡1,32 （D ）⎥⎦⎤ ⎝⎛1,32 （7）函数()()1lg -=x x f 的图象为（）(A) (B) (C) ( D)（8）设1>a ，函数()log a f x x=在区间[]a a 2,上的最大值和最小值之差为21，则a 等于（）(A)2 (B)2 （C ）22 （D ）4（9）已知函数()x f 的图像是连续的，有如下的()x f x ,对应值如下表：x1 2 3 4 5 6 ()x f123.5621.45-7.8211.5753.76126.49则函数()x f 在区间[]6,1上的零点至少有（）（A ）2个（B ）3个（C ）4个（D ）5个（10）在同一个平面直角坐标平面内，函数()x g y =的图象与xe y =的图象关于直线xy =对称，而函数()x f y =的图象与()x g y =的图象关于y 轴对称，若()1-=m f ，则m 的值是（）（A ）e - （B ）e 1-（C ）e （D ）e 1第Ⅱ卷（非选择题，共60分）二、填空题：本大题共4小题，每小题5分，共20分．（11）函数()1,012≠>+=-a a a y x 且的图象必经过定点___________; （12）若()1212-=+x x f ，则()x f -1=____________; （13）已知,53m ba==且211=+b a ，则m 的值为_______；（14）已知()x f 是()()+∞∞-,00, 上的奇函数，当0<x 时，()x x x f +=2，则当0>x 时，()x f =______.三、解答题：本大题共4小题，共40分．（15）（本小题满分10分）已知集合{}24<<-=x x A ，{}15>-<=x x x B 或，{}R m m x m x C ∈+<<-=,11.（Ⅰ）若AC =∅，求实数m 的取值范围；（Ⅱ）若()C B A ⊆ ，求实数m 的取值范围.（16）（本小题满分10分）A 、B 两城相距100km ，在两地之间距A 城x km 处D 地建一核电站给A 、B 两城供电，为保证城市安全，核电站距城市的距离不得小于10km.已知供电费用刚好和供电距离的平方与供电量之积成正比，比例系数0.2λ=.若A 城供电量为20亿度/月，B 城为10亿度/月. （Ⅰ）写出x 的范围；（Ⅱ）把月供电总费用y 表示成x 的函数；（Ⅲ）核电站建在距A 城多远，才能使供电费用最小？（17）（本小题满分10分）已知函数()121+-=x a x f .（Ⅰ）若()210=f ，求a 的值；1234-1-2-3-4-4-3-2-14321Oy x（Ⅱ）求证：无论a 为何实数，()x f 总为增函数. （18）（本小题满分10分）已知函数()[]9,1,log 23∈+=x x x f ，求函数()[]()22x f x f y +=的最大值与最小值.（19）附加题（本小题满分10分，得分不计入总分）已知函数()1f x x ax=++ (a ∈R).（Ⅰ）试给出a 的一个值，并画出此时函数的图象；（Ⅱ）若函数 f (x) 在 R 上具有单调性，求a 的取值范围.参考答案一、选择题：（1）—（10） DCDAA DCDAB二、填空题：（11）()2,2 （12 ）122-x （13）15 （14）x x +-2三、解答题：（15）（本小题满分10分）解：（Ⅰ）,1412A C m m =∅∴+≤--≥或 ------------（3分）35≥-≤∴m m 或，故实数m 的取值范围是{}35≥-≤m m m 或. ------------（5分）（Ⅱ）由题意，得{},21<<=x x B A -------------（6分）(),C B A ⊆⎩⎨⎧≥+≤-∴2111m m ， -------------（8分）21≤≤∴m ，故实数m 的取值范围是{}21≤≤m m . ----------（10分）（16）（本小题满分10分）解：（Ⅰ）x 的取值范围为{}9010≤≤x x ； -----------（2分）（Ⅱ）依题意得221[2010(100)]5y x x =+- -------------（4分）（10≤x ≤90）； ------------（5分）（III ）由222110040000[2010(100)]6()533y x x x =+-=-+. -------------------（8分）则当x ＝1003千米时，y 最小. ---------------（9分）答：故当核电站建在距A 城1003千米时，才能使供电费用最小. ---------------（10分）（17）（本小题满分10分）解：（Ⅰ）1=a ; --------------------------（2分）（Ⅱ）()121x f x a =-+,设0,1221>-=∆<x x x x x 则，()()()()()()()2121121221212211121211122212121212122121x x x x x x x x x x x x x y f x f x a a -⎛⎫⎛⎫∆=-=--- ⎪ ⎪++⎝⎭⎝⎭-=-=+++⋅+-=+⋅+0,021,120,0212121>∆∴>-∴<<<---y x x x x x x∴无论a 为何实数，()x f 总为增函数. ----------------------（10------------（8分）分）（18）（本小题满分10分）解：()[],9,1,log 23x x x f +=()()6log 6log log 2log 23232323++=+++=∴x x x x y ----------（3分）[]2,0,log 3∈=t x t[]2,0,662∈++=∴t t t y -------------（5分）()332-+=t y ,图象对称轴3-=t--------------（7分）函数∴662++=t t y 在[]2,0上递增，0=∴t ，即1x =时，;6min =y 2t =，即9x =时，.22max =y -----------------------（10分）（19）附加题（本小题满分10分，得分不计入总分）（Ⅰ）解：略 ------------- （4分）（Ⅱ）解：化简()()()⎩⎨⎧-<---++=.1111 ≥11x x a x x a x f ，，， ① a >1时，当x ≥-1时，()(1)1f x a x =++是增函数，且()x f ≥()a f -=-1；当x < -1时，()(1)1f x a x =--是增函数，且()(1)f x f a <-=-. 所以，当a >1时，函数f (x) 在R 上是增函数.同理可知，当a <-1时，函数f (x) 在R 上是减函数. ② a =1或-1时，易知，不合题意.③ -1< a <1时，取x = 0，得f (0) =1，取x =21-a ，由21-a < -1，知f (21-a ) =1，所以f (0) = f (21-a ).所以函数f (x) 在R 上不具有单调性. 综上可知，a 的取值范围是(,1)(1,)-∞-+∞. ----------------------（10分）。