2016 AMC 10A
AMC 10A 2017 英语题目及答案

2017 AMC 10A ProblemsContents[hide]▪1 Problem 1▪2 Problem 2▪3 Problem 3▪4 Problem 4▪5 Problem 5▪6 Problem 6▪7 Problem 7▪8 Problem 8▪9 Problem 9▪10 Problem 10▪11 Problem 11▪12 Problem 12▪13 Problem 13▪14 Problem 14▪15 Problem 15▪16 Problem 16▪17 Problem 17▪18 Problem 18▪19 Problem 19▪20 Problem 20▪21 Problem 21▪22 Problem 22▪23 Problem 23▪24 Problem 24▪25 Problem 25▪26 See alsoProblem 1What is the value of ?SolutionProblem 2Pablo buys popsicles for his friends. The store sells single popsicles for each, -popsicle boxes for each, and -popsicle boxes for . What is the greatest number of popsicles that Pablo can buy with ?SolutionProblem 3Tamara has three rows of two -feet by -feet flower beds in her garden. The beds are separated and also surrounded by -foot-wide walkways, as shown on the diagram. What is the total area of the walkways, in square feet?Problem 4Mia is “helping” her mom pick up toys that are strewn on the floor. Mia’s mom manages to put toys into the toy box every seconds, but each time immediately after those seconds have elapsed, Mia takes toys out of the box. How much time, in minutes, will it take Mia and her mom to put all toysinto the box for the first time?Problem 5The sum of two nonzero real numbers is times their product. What is the sum ofthe reciprocals of the two numbers?SolutionMs. Carroll promised that anyone who got all the multiple choice questions right on the upcoming exam would receive an A on the exam. Which of of these statements necessarily follows logically?SolutionProblem 7Jerry and Silvia wanted to go from the southwest corner of a square field to the northeast corner. Jerry walked due east and then due north to reach the goal, but Silvia headed northeast and reached the goal walking in a straight line. Which of the following is closest to how much shorter Silvia's trip was, compared to Jerry's trip?SolutionProblem 8At a gathering of people, there are people who all know each other and people who know no one. People who know each other hug, and people who do not know each other shake hands. How many handshakes occur?SolutionProblem 9Minnie rides on a flat road at kilometers per hour (kph), downhill at kph, and uphill at kph. Penny rides on a flat road at kph, downhill at kph, and uphill at kph. Minnie goes from town to town , a distance of km all uphill, then from town to town , a distance of km all downhill, and then back to town , a distance of km on the flat. Penny goes the other way around using the same route. How many more minutes does it take Minnie to complete the -km ride than it takes Penny?SolutionJoy has thin rods, one each of every integer length from cm through cm. She places the rods with lengths cm, cm, and cm on a table. She then wants to choose a fourth rod that she can put with these three to form a quadrilateral with positive area. How many of the remaining rods can she choose as the fourth rod?SolutionProblem 11The region consisting of all points in three-dimensional space within units of line segment has volume . What is the length ?SolutionProblem 12Let be a set of points in the coordinate plane such that two of the three quantities and are equal and the third of the three quantities is no greater than this common value. Which of the following is a correct description forSolutionProblem 13Define a sequence recursively by and the remainder when is divided by for all Thus the sequence startsWhat isSolutionProblem 14Every week Roger pays for a movie ticket and a soda out of his allowance. Last week, Roger's allowance was dollars. The cost of his movie ticket was of the difference between and the cost of his soda, while the cost of his soda was of the difference between and the cost of his movie ticket. To the nearest whole percent, what fraction of did Roger pay for his movie ticket and soda?SolutionProblem 15Chloé chooses a real number uniformly at random from the interval . Independently, Laurent chooses a real number uniformly at random from the interval . What is the probability that Laurent's number is greater than Chloé's number?SolutionProblem 16There are 10 horses, named Horse 1, Horse 2, , Horse 10. They get their names from how many minutes it takes them to run one lap around a circular race track: Horse runs one lap in exactly minutes. At time 0 all the horses are together at the starting point on the track. The horses start running in the same direction, and they keep running around the circular track at their constant speeds. The least time , in minutes, at which all 10 horses will again simultaneously be at the starting point is . Let be the least time, in minutes, such that at least 5 of the horses are again at the starting point. What is the sum of the digits of ?SolutionProblem 17Distinct points , , , lie on the circle and have integer coordinates. The distances and are irrational numbers. What is the greatest possible value of the ratio ?SolutionProblem 18Amelia has a coin that lands heads with probability , and Blaine has a coin thatlands on heads with probability . Amelia and Blaine alternately toss their coins until someone gets a head; the first one to get a head wins. All coin tosses are independent. Amelia goes first. The probability that Amelia wins is , where and are relatively prime positive integers. What is ?SolutionProblem 19Alice refuses to sit next to either Bob or Carla. Derek refuses to sit next to Eric. How many ways are there for the five of them to sit in a row of 5 chairs under these conditions?SolutionProblem 20Let equal the sum of the digits of positive integer . For example,. For a particular positive integer , . Which of the following could be the value of ?SolutionProblem 21A square with side length is inscribed in a right triangle with sides of length , , and so that one vertex of the square coincides with the right-angle vertex of the triangle. A square with side length is inscribed in another right triangle with sides of length , , and so that one side of the square lies on the hypotenuseof the triangle. What is ?SolutionProblem 22Sides and of equilateral triangle are tangent to a circle at points and respectively. What fraction of the area of lies outside the circle?SolutionProblem 23How many triangles with positive area have all their vertices at points in the coordinate plane, where and are integers between and , inclusive?SolutionProblem 24For certain real numbers , , and , the polynomialhas three distinct roots, and each root of is also a root of the polynomial What isSolutionProblem 25How many integers between and , inclusive, have the property that some permutation of its digits is a multiple of between and For example, both and have this property.Solution2017 AMC 10A Answer Key1. C2. D3. B4. B5. C6. B7. A8. B9. C10. B11. D12. E13. D14. D15. C16. B17. D18. D19. C20. D21. D22. E23. B24. C25. A2017 AMC 10A Problems/Problem 1Contents[hide]▪1 Problem▪2 Solution 1▪3 Solution 2▪4 Solution 3▪5 Solution 4▪6 See AlsoProblemWhat is the value of ?Solution 1Notice this is the term in a recursive sequence, defined recursively asThus:Solution 2Starting to compute the inner expressions, we see the results are . This is always less than a power of . The only admissible answer choice by this rule is thus .Solution 3Working our way from the innermost parenthesis outwards and directly computing, we have .Solution 4If you distribute this you get a sum of the powers of . The largest power of in the series is , so the sum is .2017 AMC 10A Problems/Problem 2 ProblemPablo buys popsicles for his friends. The store sells single popsicles for each, -popsicle boxes for each, and -popsicle boxes for . What is the greatest number of popsicles that Pablo can buy with ?Solutionboxes give us the most popsicles/dollar, so we want to buy as many of those as possible. After buying , we have left. We cannot buy a third box, so we opt for the box instead (since it has a higher popsicles/dollar ratio than the pack). We're now out of money. We bought popsicles, so theanswer is .2017 AMC 10A Problems/Problem 3 ProblemTamara has three rows of two -feet by -feet flower beds in her garden. The beds are separated and also surrounded by -foot-wide walkways, as shown on the diagram. What is the total area of the walkways, in square feet?Finding the area of the shaded walkway can be achieved by computing the total area of Tamara's garden and then subtracting the combined area of her six flower beds.Since the width of Tamara's garden contains three margins, the total width isfeet.Similarly, the height of Tamara's garden is feet.Therefore, the total area of the garden is square feet.Finally, since the six flower beds each have an area of square feet, the area we seek is , and our answer is2017 AMC 10A Problems/Problem 4Contents[hide]▪1 Problem▪2 Solution▪3 Solution 2▪4 See alsoProblemMia is helping her mom pick up toys that are strewn on the floor. Mia’s mom manages to put toys into the toy box every seconds, but each time immediately after those seconds have elapsed, Mia takes toys out of the box. How much time, in minutes, will it take Mia and her mom to put all toys into the box for the first time?SolutionEvery seconds toys are put in the box, so after seconds there will be toys in the box. Mia's mom will then put toys into to the box and we have our total amount of time to be seconds, whichequals minutes.Solution 2Though Mia's mom places toys every seconds, Mia takes out toys right after. Therefore, after seconds, the two have collectively placed toy into the box. Therefore by minutes, the two would have placed toys into the box. Therefore, at minutes, the two would have placed toys into the box. Though Mia may take toys out right after, the number of toys in the box firstreaches by minutes.2017 AMC 10A Problems/Problem 5Contents[hide]▪1 Problem▪2 Solution▪3 Solution 2▪4 Solution 3▪5 See AlsoProblemThe sum of two nonzero real numbers is 4 times their product. What is the sum of the reciprocals of the two numbers?SolutionLet the two real numbers be . We are given that and dividing both sides by ,Note: we can easily verify that this is the correct answer; for example, 1/2 and 1/2 work, and the sum of their reciprocals is 4.Solution 2Instead of using algebra, another approach at this problem would be to notice the fact that one of the nonzero numbers has to be a fraction. See for yourself. Andby looking into fractions, we immediately see that and would fit the rule.Solution 3Notice that from the information given above,Because the sum of the reciprocals of two numbers is just the sum of the two numbers over the product of the two numbers orWe can solve this by substituting .Our answer is simply .Therefore, the answer is .2017 AMC 10A Problems/Problem 6 ProblemMs. Carroll promised that anyone who got all the multiple choice questions right on the upcoming exam would receive an A on the exam. Which one of these statements necessarily follows logically?SolutionRewriting the given statement: "if someone got all the multiple choice questions right on the upcoming exam then he or she would receive an A on the exam." If that someone is Lewis the statement becomes: "if Lewis got all the multiple choice questions right, then he got an A on the exam." The contrapositive: "If Lewis did not receive an A, then he got at least one of the multiple choice questions wrong (did not get all of them right)" must also be true leaving B as the correct answer. B is also equivalent to the contrapositive of the original statement, which implies that it must be true, so the answer is.2017 AMC 10A Problems/Problem 7 ProblemJerry and Silvia wanted to go from the southwest corner of a square field to the northeast corner. Jerry walked due east and then due north to reach the goal, but Silvia headed northeast and reached the goal walking in a straight line. Which ofthe following is closest to how much shorter Silvia's trip was, compared to Jerry's trip?SolutionLet represent how far Jerry walked, and represent how far Sylvia walked. Since the field is a square, and Jerry walked two sides of it, while Silvia walked the diagonal, we can simply define the side of the square field to be one, and find the distances they walked. Since Jerry walked two sides, Since Silvia walked the diagonal, she walked the hypotenuse of a 45, 45, 90 triangle with leglength 1. Thus, We can then take2017 AMC 10A Problems/Problem 8 Contents[hide]▪1 Problem▪2 Solution 1▪3 Solution 2▪4 Solution 3▪5 See AlsoProblemAt a gathering of people, there are people who all know each other and people who know no one. People who know each other a hug, and people who do not know each other shake hands. How many handshakes occur?Solution 1Each one of the ten people has to shake hands with all the other people they don’t know. So . From there, we calculate how many handshakes occurred between the people who don’t know each other. This is simply countinghow many ways to choose two people to shake hands, or . Thus the answer is .Solution 2We can also use complementary counting. First of all, handshakes or hugs occur. Then, if we can find the number of hugs, then we can subtract it from to find the handshakes. Hugs only happen between the 20 people whoknow each other, so there are hugs. . Solution 3We can focus on how many handshakes the 10 people get.The 1st person gets 29 handshakes.2nd gets 28......And the 10th receives 20 handshakes.We can write this as the sum of an arithmetic sequence.Therefore, the answer is2017 AMC 10A Problems/Problem 9 ProblemMinnie rides on a flat road at kilometers per hour (kph), downhill at kph, and uphill at kph. Penny rides on a flat road at kph, downhill at kph, and uphill at kph. Minnie goes from town to town , a distance of km all uphill, then from town to town , a distance of km all downhill, and then back to town , a distance of km on the flat. Penny goes the other way around using the same route. How many more minutes does it take Minnie to complete the -km ride than it takes Penny?SolutionThe distance from town to town is km uphill, and since Minnie rides uphill at a speed of kph, it will take her hours. Next, she will ride from town to town , a distance of km all downhill. Since Minnie rides downhill at a speed of kph, it will take her half an hour. Finally, she rides from town back to town , a flat distance of km. Minnie rides on a flat road at kph, so this will take her hour. Her entire trip takes her hours. Secondly, Penny will go from town to town , a flat distance of km. Since Penny rides on a flat road at kph,it will take her of an hour. Next Penny will go from town to town , which is uphill for Penny. Since Penny rides at a speed of kph uphill, and town and are km apart, it will take her hours. Finally, Penny goes from Townback to town , a distance of km downhill. Since Penny rides downhill atkph, it will only take her of an hour. In total, it takes her hours, which simplifies to hours and minutes. Finally, Penny's Hour Minute trip was minutes less than Minnie's Hour Minute Trip2017 AMC 10A Problems/Problem 10 ProblemJoy has thin rods, one each of every integer length from cm through cm. She places the rods with lengths cm, cm, and cm on a table. She then wants to choose a fourth rod that she can put with these three to form a quadrilateral with positive area. How many of the remaining rods can she choose as the fourth rod?SolutionThe triangle inequality generalizes to all polygons, so andto get . Now, we know that there are numbers between and exclusive, but we must subtract to account for the 2 lengthsalready used that are between those numbers, which gives2017 AMC 10A Problems/Problem 11Contents[hide]▪1 Problem▪2 Solution▪3 Diagram▪4 See AlsoProblemThe region consisting of all point in three-dimensional space within 3 units of line segment has volume 216. What is the length ?SolutionIn order to solve this problem, we must first visualize what the region contained looks like. We know that, in a three dimensional plane, the region consisting of all points within units of a point would be a sphere with radius . However, we need to find the region containing all points within 3 units of a segment. It can be seen that our region is a cylinder with two hemispheres on either end. We know the volume of our region, so we set up the following equation (the volume of our cylinder + the volume of our two hemispheres will equal ):, where is equal to the length of our line segment. Solving, we find that .Diagram/cwNt293.png2017 AMC 10A Problems/Problem 12ProblemLet be a set of points in the coordinate plane such that two of the three quantities and are equal and the third of the three quantities is no greater than this common value. Which of the following is a correct description forSolutionIf the two equal values are and , then . Also, because 3 is the common value. Solving for , we get . Therefore the portion of the line where is part of . This is a ray with an endpoint of .Similar to the process above, we assume that the two equal values are and . Solving the equation then . Also, because 3 is the common value. Solving for , we get . Therefore the portion of the line where is also part of . This is another ray with the sameendpoint as the above ray: .If and are the two equal values, then . Solving the equation for , we get . Also because is one way toexpress the common value. Solving for , we get . Therefore the portion of the line where is part of like the other two rays. Thelowest possible value that can be achieved is also .Since is made up of three rays with common endpoint , the answer is2017 AMC 10A Problems/Problem 13 ProblemDefine a sequence recursively by and the remainder when is divided by for all Thus the sequence startsWhat isSolutionA pattern starts to emerge as the function is continued. The repeating pattern isThe problem asks for the sum of eight consecutive terms in the sequence. Because there are eight numbers in the repeating sequence, we just need to find the sum of the numbers in the sequence, which is2017 AMC 10A Problems/Problem 14 ProblemEvery week Roger pays for a movie ticket and a soda out of his allowance. Last week, Roger's allowance was dollars. The cost of his movie ticket was of the difference between and the cost of his soda, while the cost of his soda was of the difference between and the cost of his movie ticket. To the nearest whole percent, what fraction of did Roger pay for his movie ticket and soda?SolutionLet = cost of movie ticketLet = cost of sodaWe can create two equations:Substituting we get:which yields:Now we can find s and we get:Since we want to find what fraction of did Roger pay for his movie ticket and soda, we add and to get:2017 AMC 10A Problems/Problem 15 Contents[hide]▪ 1 Problem▪ 2 Solution 1▪ 3 Solution 2▪ 4 Solution 3:▪ 5 See AlsoProblemChloé chooses a real number uniformly at random from the interval . Independently, Laurent chooses a real number uniformly at random from the interval . What is the probability that Laurent's number is greater than Chloé's number?Solution 1Denote "winning" to mean "picking a greater number". There is a chance that Laurent chooses a number in the interval . In this case, Chloécannot possibly win, since the maximum number she can pick is . Otherwise, if Laurent picks a number in the interval , with probability , then the two people are symmetric, and each has a chance of winning. Then,the total probability isSolution 2We can use geometric probability to solve this. Suppose a point lies in the-plane. Let be Chloe's number and be Laurent's number. Then obviously we want , which basically gives us a region above a line. We know thatChloe's number is in the interval and Laurent's number is in the interval , so we can create a rectangle in the plane, whose length is andwhose width is . Drawing it out, we see that it is easier to find the probability that Chloe's number is greater than Laurent's number and subtract this probability from . The probability that Chloe's number is larger than Laurent's number issimply the area of the region under the line , which is . Instead of bashing this out we know that the rectangle has area . Sothe probability that Laurent has a smaller number is . Simplifying the expression yields and so .Solution 3:Scale down by to get that Chloe picks from and Laurent picks from . There are an infinite number of cases for the number that Chloe picks, butthey are all centered around the average of . Therefore, Laurent has a range of to to pick from, on average, which is a length of out of a total length of . Therefore, the probability is2017 AMC 10A Problems/Problem 16 Contents[hide]▪ 1 Problem▪ 2 Solution 1▪ 3 Solution 2▪ 4 See AlsoProblemThere are 10 horses, named Horse , Horse , . . . , Horse . They get their names from how many minutes it takes them to run one lap around a circular race track: Horse runs one lap in exactly minutes. At time all the horses are together at the starting point on the track. The horses start running in the same direction, and they keep running around the circular track at their constant speeds. The least time , in minutes, at which all horses will again simultaneously be at the starting point is . Let be the least time, in minutes, such that at least 5 of the horses are again at the starting point. What is the sum of the digits ofSolution 1If we have horses, , then any number that is a multiple of the all those numbers is a time when all horses will meet at the starting point. The least of these numbers is the LCM. To minimize the LCM, we need the smallest primes, and we need to repeat them a lot. By inspection, we find that. Finally, .Solution 2We are trying to find the smallest number that has one-digit divisors. Therefore we try to find the LCM for smaller digits, such as ,, , or . We quickly consider since it is the smallest number that is the LCM of , , and . Since has single-digit divisors, namely , , , , and , our answer is2017 AMC 10A Problems/Problem 17 ProblemDistinct points , , , lie on the circle and have integer coordinates. The distances and are irrational numbers. What is thegreatest possible value of the ratio ?SolutionBecause , , , and are integers there are only a few coordinates that actually satisfy the equation. The coordinates areand We want to maximize and minimize They also have to bethe square root of something, because they are both irrational. The greatest value of happens when it and are almost directly across from each other and are in different quadrants. For example, the endpoints of the segmentcould be and because the two points are almost across from each other. The least value of is when the two endpoints are in the samequadrant and are very close to each other. This can occur when, for example,is and is They are in the same quadrant and no other point on the circle with integer coordinates is closer to the point than Using the distance formula, we get that is and that is2017 AMC 10A Problems/Problem 18 Contents[hide]▪ 1 Problem▪ 2 Solution▪ 3 Solution 2▪ 4 See AlsoProblemAmelia has a coin that lands heads with probability , and Blaine has a coin that lands on heads with probability . Amelia and Blaine alternately toss their coins until someone gets a head; the first one to get a head wins. All coin tosses are independent. Amelia goes first. The probability that Amelia wins is , whereand are relatively prime positive integers. What is ?SolutionLet be the probability Amelia wins. Note that, as if she gets to her turn again, she is back where she started with probability of winning . The chance she wins on her first turn is . The chance she makes itto her turn again is a combination of her failing to win the first turn - and Blaine failing to win - . Multiplying gives us . Thus, Therefore, , so the answer is .Solution 2Let be the probability Amelia wins. Note thatThis can be represented by an infinite geometric series,. Therefore, , so the answer isSolution by ktong2017 AMC 10A Problems/Problem 19 Contents[hide]▪ 1 Problem▪ 2 Solution 1▪ 3 Solution 2▪ 4 See AlsoProblemAlice refuses to sit next to either Bob or Carla. Derek refuses to sit next to Eric. How many ways are there for the five of them to sit in a row of 5 chairs under these conditions?Solution 1For notation purposes, let Alice be A, Bob be B, Carla be C, Derek be D, and Eric be E. We can split this problem up into two cases:A sits on an edge seat.Then, since B and C can't sit next to A, that must mean either D or E sits next to A. After we pick either D or E, then either B or C must sit next to D/E. Then, we can arrange the two remaining people in two ways. Since there are two different edge seats that A can sit in, there are a total of .A does not sit in an edge seat.In this case, then only two people that can sit next to A are D and E, and there are two ways to permute them, and this also handles the restriction that D can't sit next to E. Then, there are two ways to arrange B and C, the remaining people. However, there are three initial seats that A can sit in, so there areseatings in this case.Adding up all the cases, we have .Solution 2Label the seats through . The number of ways to seat Derek and Eric in the five seats with no restrictions is . The number of ways to seat Derek and Eric such that they sit next to each other is (which can be figure out quickly), so the number of ways such that Derek and Eric don't sit next to each other is . Note that once Derek and Eric are seated, there are three cases.The first case is that they sit at each end. There are two ways to seat Derek and Eric. But this is impossible because then Alice, Bob, and Carla would have to sit in some order in the middle three seats which would lead to Alice sitting next to Bob or Carla, a contradiction. So this case gives us ways.Another possible case is if Derek and Eric seat in seats and in some order. There are 2 possible ways to seat Derek and Eric like this. This leaves Alice, Bob, and Carla to sit in any order in the remaining three seats. Since no two of these three seats are consecutive, there are ways to do this. So the second case gives us total ways for the second case.The last case is if once Derek and Eric are seated, exactly one pair of consecutive seats is available. There are ways to seat Derek and Eric like this. Once they are seated like this, Alice cannot not sit in one of the two consecutive available seats without sitting next to Bob and Carla. So Alice has to sit in the other remaining chair. Then, there are two ways to seat Bob and Carla in the remaining two seats (which are consecutive). So this case gives us ways.So in total there are . So our answer is .。
2010 AMC 10A 试题及答案解析

2010 AMC 10A problems and solutions.The test was held on February 8, 2010. The first link contains the full set of test problems. The rest contain each individual problem and its solution.Problem 1Mary’s top book shelf holds five books with the follow ing widths, incentimeters: , , , , and . What is the average book width, in centimeters?SolutionTo find the average, we add up the widths , , , , and , to get a total sum of . Since there are books, the average book width isThe answer is .Problem 2Four identical squares and one rectangle are placed together to form one large square as shown. The length of the rectangle is how many times as large as its width?SolutionLet the length of the small square be , intuitively, the length of the big square is . It can be seen that the width of the rectangle is .Thus, the length of the rectangle is times large as the width. The answer is .Problem 3Tyrone had marbles and Eric had marbles. Tyrone then gave some of his marbles to Eric so that Tyrone ended with twice as many marbles as Eric. How many marbles did Tyrone give to Eric?SolutionLet be the number of marbles Tyrone gave to Eric. Then,. Solving for yields and . The answer is .Problem 4A book that is to be recorded onto compact discs takes minutes to read aloud. Each disc can hold up to minutes of reading. Assume that the smallest possible number of discs is used and that each disc contains the same length of reading. How many minutes of reading will each disc contain?SolutionAssuming that there were fractions of compact discs, it would take CDs to have equal reading time. However, since the number of discs can only be a whole number, there are at least 8 CDs, in which case it would have minutes on each of the 8 discs. The answer is .Problem 5The area of a circle whose circumference is is . What is the value of ?SolutionIf the circumference of a circle is , the radius would be . Since the area of a circle is , the area is . The answer is . Problem 6For positive numbers and the operation is defined asWhat is ?Solution. Then, is The answer isProblem 7Crystal has a running course marked out for her daily run. She starts this run by heading due north for one mile. She then runs northeast for one mile, then southeast for one mile. The last portion of her run takes her on a straight line back to where she started. How far, in miles is this last portion of her run?SolutionCrystal first runs North for one mile. Changing directions, she runs Northeast for another mile. The angle difference between North and Northeast is 45 degrees. She then switches directions to Southeast, meaning a 90 degree angle change. The distance now from travelling North for one mile, and her current destination is miles, because it is the hypotenuse of a 45-45-90 triangle with side length one (mile). Therefore, Crystal's distance from her starting position, x, is equal to , which is equal to . The answer isTony works hours a day and is paid $per hour for each full year of his age. During a six month period Tony worked days and earned $. How old was Tony at the end of the six month period?SolutionTony worked hours a day and is paid dollars per hour for each full year of his age. This basically says that he gets a dollar for each year of his age. So if he is years old, he gets dollars a day. We also know that he worked days and earned dollars. If he was years old at the beginning of his working period, he would have earned dollars. If he was years old at the beginning of his working period, he would have earned dollars. Because he earned dollars, we know that he was for some period of time, but not the whole time, because then the money earned would be greater than or equal to . This is why he was when he began, but turned sometime in the middle and earned dollars in total. So the answer is .The answer is . We could find out for how long he was and . . Then isand we know that he was for days, and for days. Thus, the answer is .Problem 9A palindrome, such as , is a number that remains the same when its digits are reversed. The numbers and are three-digit and four-digit palindromes, respectively. What is the sum of the digits of ?Solutionis at most , so is at most . The minimum value ofis . However, the only palindrome between and is , which means that must be .It follows that is , so the sum of the digits is .Marvin had a birthday on Tuesday, May 27 in the leap year . In what year will his birthday next fall on a Saturday?Solution(E) 2017There are 365 days in a non-leap year. There are 7 days in a week. Since 365 = 52 * 7 + 1 (or 365 is congruent to 1 mod 7), the same date (after February) moves "forward" one day in the subsequent year, if that year is not a leap year.For example: 5/27/08 Tue 5/27/09 WedHowever, a leap year has 366 days, and 366 = 52 * 7 + 2. So the same date (after February) moves "forward" two days in the subsequent year, if that year is a leap year.For example: 5/27/11 Fri 5/27/12 SunYou can keep count forward to find that the first time this date falls on a Saturday is in 2017:5/27/13 Mon 5/27/14 Tue 5/27/15 Wed 5/27/16 Fri 5/27/17 Sat Problem 11The length of the interval of solutions of the inequality is . What is ?SolutionSince we are given the range of the solutions, we must re-write the inequalities so that we have in terms of and .Subtract from all of the quantities:Divide all of the quantities by .Since we have the range of the solutions, we can make them equal to .Multiply both sides by 2.Re-write without using parentheses.Simplify.We need to find for the problem, so the answer isProblem 12Logan is constructing a scaled model of his town. The city's water tower stands 40 meters high, and the top portion is a sphere that holds 100,000 liters of water. Logan's miniature water tower holds 0.1 liters. How tall, in meters, should Logan make his tower?SolutionThe water tower holds times more water than Logan's miniature. Therefore, Logan should make his towertimes shorter than the actual tower. This ismeters high, or choice .Problem 13Angelina drove at an average rate of kph and then stopped minutes for gas. After the stop, she drove at an average rate of kph. Altogether she drove km in a total trip time of hours including the stop. Which equation could be used to solve for the time in hours that she drove before her stop?SolutionThe answer is ()because she drove at kmh for hours (the amount of time before the stop), and 100 kmh for because she wasn't driving for minutes, or hours. Multiplying by gives the total distance, which is kms. Therefore, the answer isProblem 14Triangle has . Let and be on and , respectively, such that . Let be the intersection of segments and , and suppose that is equilateral. What is ?SolutionLet .Since ,Problem 15In a magical swamp there are two species of talking amphibians: toads, whose statements are always true, and frogs, whose statements are always false. Four amphibians, Brian, Chris, LeRoy, and Mike live together in this swamp, and they make the following statements.Brian: "Mike and I are different species."Chris: "LeRoy is a frog."LeRoy: "Chris is a frog."Mike: "Of the four of us, at least two are toads."How many of these amphibians are frogs?SolutionSolution 1We can begin by first looking at Chris and LeRoy.Suppose Chris and LeRoy are the same species. If Chris is a toad, then what he says is true, so LeRoy is a frog. However, if LeRoy is a frog, then he is lying, but clearly Chris is not a frog, and we have a contradiction. The same applies if Chris is a frog.Clearly, Chris and LeRoy are different species, and so we have at least frog out of the two of them.Now suppose Mike is a toad. Then what he says is true because we already have toads. However, if Brian is a frog, then he is lying, yet his statement is true, a contradiction. If Brian is a toad, then what he says is true, but once again it conflicts with his statement, resulting in contradiction.Therefore, Mike must be a frog. His statement must be false, which means that there is at most toad. Since either Chris or LeRoy is already a toad, Brain must be a frog. We can also verify that his statement is indeed false.Both Mike and Brian are frogs, and one of either Chris or LeRoy is a frog, so we have frogs total.Solution 2Start with Brian. If he is a toad, he tells the truth, hence Mike is a frog. If Brian is a frog, he lies, hence Mike is a frog, too. Thus Mike must be a frog.As Mike is a frog, his statement is false, hence there is at most one toad.As there is at most one toad, at least one of Chris and LeRoy is a frog. But then the other one tells the truth, and therefore is a toad. Hence we must have one toad and three frogs.Problem 16Nondegenerate has integer side lengths, is an angle bisector, , and . What is the smallest possible value of the perimeter?SolutionBy the Angle Bisector Theorem, we know that . If we use the lowest possible integer values for AB and BC (the measures of AD and DC, respectively), then , contradicting the Triangle Inequality. If we use the next lowest values (and ), the Triangle Inequality is satisfied. Therefore, our answer is , or choice .Problem 17A solid cube has side length inches. A -inch by -inch square hole is cut into the center of each face. The edges of each cut are parallel to the edges of the cube, and each hole goes all the way through the cube. What is the volume, in cubic inches, of the remaining solid?SolutionSolution 1Imagine making the cuts one at a time. The first cut removes a box . The second cut removes two boxes, each of dimensions, and the third cut does the same as the second cut, on the last two faces. Hence the total volume of all cuts is .Therefore the volume of the rest of the cube is.Solution 2We can use Principle of Inclusion-Exclusion to find the final volume of the cube.There are 3 "cuts" through the cube that go from one end to the other. Each of these "cuts" has cubic inches. However, we can not just sum their volumes, as the central cube is included in each of these three cuts. To get the correct result, we can take the sum of the volumes of the three cuts, and subtract the volume of the central cube twice.Hence the total volume of the cuts is.Therefore the volume of the rest of the cube is.Solution 3We can visualize the final figure and see a cubic frame. We can find the volume of the figure by adding up the volumes of the edges and corners.Each edge can be seen as a box, and each corner can be seen as a box..Problem 18Bernardo randomly picks 3 distinct numbers from the setand arranges them in descending order to form a 3-digit number. Silvia randomly picks 3 distinct numbers from the set and also arranges them in descending order to form a 3-digit number. What is the probability that Bernardo's number is larger than Silvia's number?SolutionWe can solve this by breaking the problem down into cases and adding up the probabilities.Case : Bernardo picks . If Bernardo picks a then it is guaranteed that his number will be larger than Silvia's. The probability that he will pick a is .Case : Bernardo does not pick . Since the chance of Bernardo picking is , the probability of not picking is .If Bernardo does not pick 9, then he can pick any number from to . Since Bernardo is picking from the same set of numbers as Silvia, the probability that Bernardo's number is larger is equal to the probability that Silvia's number is larger.Ignoring the for now, the probability that they will pick the same number is the number of ways to pick Bernardo's 3 numbers divided by the number of ways to pick any 3 numbers.We get this probability to beProbability of Bernardo's number being greater isFactoring the fact that Bernardo could've picked a but didn't:Adding up the two cases we getProblem 19Equiangular hexagon has side lengthsand . The area of is of the area of the hexagon. What is the sum of all possible values of ?SolutionSolution 1It is clear that is an equilateral triangle. From the Law of Cosines, we get that . Therefore, the area of is .If we extend , and so that and meet at , and meet at , and and meet at , we find that hexagon is formed by taking equilateral triangle of side length and removing three equilateral triangles, , and , of side length . The area of is therefore.Based on the initial conditions,Simplifying this gives us . By Vieta's Formulas we know that the sum of the possible value of is .Solution 2As above, we find that the area of is .We also find by the sine triangle area formula that, and thusThis simplifies to.Problem 20A fly trapped inside a cubical box with side length meter decides to relieve its boredom by visiting each corner of the box. It will begin and end in the same corner and visit each of the other corners exactly once. To get from a corner to any other corner, it will either fly or crawl in a straight line. What is the maximum possible length, in meters, of its path?SolutionThe distance of an interior diagonal in this cube is and the distance of a diagonal on one of the square faces is . It would not make sense if the fly traveled an interior diagonal twice in a row, as it would return to the point it just came from, so at most the final sum can only have 4 as the coefficient of . The other 4 paths taken can be across a diagonal on one of the faces, so the maximum distance traveled is.Problem 21The polynomial has three positive integer zeros. What is the smallest possible value of ?SolutionBy Vieta's Formulas, we know that is the sum of the three roots of the polynomial . Also, 2010 factors into. But, since there are only three roots to the polynomial, two of the four prime factors must be multiplied so that we are left with three roots. To minimize , and should be multiplied, which means will be and the answer is .Problem 22Eight points are chosen on a circle, and chords are drawn connecting every pair of points. No three chords intersect in a single point insidethe circle. How many triangles with all three vertices in the interior of the circle are created?SolutionTo choose a chord, we know that two points must be chosen. This implies that for three chords to create a triangle and not intersect at a single point, six points need to be chosen. Therefore, the answer is which is equivalent to 28,Problem 23Each of 2010 boxes in a line contains a single red marble, and for , the box in the position also contains white marbles. Isabella begins at the first box and successively draws a single marble at random from each box, in order. She stops when she first draws a red marble. Let be the probability that Isabella stops afterdrawing exactly marbles. What is the smallest value of for which ?SolutionThe probability of drawing a white marble from box is . Theprobability of drawing a red marble from box is .The probability of drawing a red marble at box is thereforeIt is then easy to see that the lowest integer value of that satisfies the inequality is .Problem 24The number obtained from the last two nonzero digits of is equal to . What is ?SolutionWe will use the fact that for any integer ,First, we find that the number of factors of in is equal to. Let . The we want is therefore the last two digits of , or . Since there is clearly an excess of factors of 2, we know that , so it remains to find .If we divide by by taking out all the factors of in , we canwrite as where where every multiple of 5 is replaced by the number with all its factors of 5 removed. Specifically, every number in the form is replaced by , and every number in the form is replaced by .The number can be grouped as follows:Using the identity at the beginning of the solution, we can reducetoUsing the fact that (or simply the fact that if you have your powers of 2 memorized), we can deduce that . Therefore.Finally, combining with the fact that yields.Problem 25Jim starts with a positive integer and creates a sequence of numbers. Each successive number is obtained by subtracting the largest possible integer square less than or equal to the current number until zero is reached. For example, if Jim starts with , then his sequence contains numbers:Let be the smallest number for which Jim’s sequence has numbers. What is the units digit of ?SolutionWe can find the answer by working backwards. We begin with on the bottom row, then the goes to the right of the equal's sign in the row above. We find the smallest value for whichand , which is .We repeat the same procedure except with for the next row and for the row after that. However, at the fourth row, wesee that solving yields , in which case it would be incorrect since is not the greatest perfect square less than or equal to . So we make it a and solve . We continue on using this same method where we increase the perfect square until can be made bigger than it. When we repeat this until we have rows, we get:Hence the solution is the last digit of , which is .。
2010 AMC 10A 试题及答案解析

2010 AMC 10A problems and solutions.The test was held on February 8, 2010. The first link contains the full set of test problems. The rest contain each individual problem and its solution.Problem 1Mary’s top book shelf holds five books with the follow ing widths, incentimeters: , , , , and . What is the average book width, in centimeters?SolutionTo find the average, we add up the widths , , , , and , to get a total sum of . Since there are books, the average book width isThe answer is .Problem 2Four identical squares and one rectangle are placed together to form one large square as shown. The length of the rectangle is how many times as large as its width?SolutionLet the length of the small square be , intuitively, the length of the big square is . It can be seen that the width of the rectangle is .Thus, the length of the rectangle is times large as the width. The answer is .Problem 3Tyrone had marbles and Eric had marbles. Tyrone then gave some of his marbles to Eric so that Tyrone ended with twice as many marbles as Eric. How many marbles did Tyrone give to Eric?SolutionLet be the number of marbles Tyrone gave to Eric. Then,. Solving for yields and . The answer is .Problem 4A book that is to be recorded onto compact discs takes minutes to read aloud. Each disc can hold up to minutes of reading. Assume that the smallest possible number of discs is used and that each disc contains the same length of reading. How many minutes of reading will each disc contain?SolutionAssuming that there were fractions of compact discs, it would take CDs to have equal reading time. However, since the number of discs can only be a whole number, there are at least 8 CDs, in which case it would have minutes on each of the 8 discs. The answer is .Problem 5The area of a circle whose circumference is is . What is the value of ?SolutionIf the circumference of a circle is , the radius would be . Since the area of a circle is , the area is . The answer is . Problem 6For positive numbers and the operation is defined asWhat is ?Solution. Then, is The answer isProblem 7Crystal has a running course marked out for her daily run. She starts this run by heading due north for one mile. She then runs northeast for one mile, then southeast for one mile. The last portion of her run takes her on a straight line back to where she started. How far, in miles is this last portion of her run?SolutionCrystal first runs North for one mile. Changing directions, she runs Northeast for another mile. The angle difference between North and Northeast is 45 degrees. She then switches directions to Southeast, meaning a 90 degree angle change. The distance now from travelling North for one mile, and her current destination is miles, because it is the hypotenuse of a 45-45-90 triangle with side length one (mile). Therefore, Crystal's distance from her starting position, x, is equal to , which is equal to . The answer isTony works hours a day and is paid $per hour for each full year of his age. During a six month period Tony worked days and earned $. How old was Tony at the end of the six month period?SolutionTony worked hours a day and is paid dollars per hour for each full year of his age. This basically says that he gets a dollar for each year of his age. So if he is years old, he gets dollars a day. We also know that he worked days and earned dollars. If he was years old at the beginning of his working period, he would have earned dollars. If he was years old at the beginning of his working period, he would have earned dollars. Because he earned dollars, we know that he was for some period of time, but not the whole time, because then the money earned would be greater than or equal to . This is why he was when he began, but turned sometime in the middle and earned dollars in total. So the answer is .The answer is . We could find out for how long he was and . . Then isand we know that he was for days, and for days. Thus, the answer is .Problem 9A palindrome, such as , is a number that remains the same when its digits are reversed. The numbers and are three-digit and four-digit palindromes, respectively. What is the sum of the digits of ?Solutionis at most , so is at most . The minimum value ofis . However, the only palindrome between and is , which means that must be .It follows that is , so the sum of the digits is .Marvin had a birthday on Tuesday, May 27 in the leap year . In what year will his birthday next fall on a Saturday?Solution(E) 2017There are 365 days in a non-leap year. There are 7 days in a week. Since 365 = 52 * 7 + 1 (or 365 is congruent to 1 mod 7), the same date (after February) moves "forward" one day in the subsequent year, if that year is not a leap year.For example: 5/27/08 Tue 5/27/09 WedHowever, a leap year has 366 days, and 366 = 52 * 7 + 2. So the same date (after February) moves "forward" two days in the subsequent year, if that year is a leap year.For example: 5/27/11 Fri 5/27/12 SunYou can keep count forward to find that the first time this date falls on a Saturday is in 2017:5/27/13 Mon 5/27/14 Tue 5/27/15 Wed 5/27/16 Fri 5/27/17 Sat Problem 11The length of the interval of solutions of the inequality is . What is ?SolutionSince we are given the range of the solutions, we must re-write the inequalities so that we have in terms of and .Subtract from all of the quantities:Divide all of the quantities by .Since we have the range of the solutions, we can make them equal to .Multiply both sides by 2.Re-write without using parentheses.Simplify.We need to find for the problem, so the answer isProblem 12Logan is constructing a scaled model of his town. The city's water tower stands 40 meters high, and the top portion is a sphere that holds 100,000 liters of water. Logan's miniature water tower holds 0.1 liters. How tall, in meters, should Logan make his tower?SolutionThe water tower holds times more water than Logan's miniature. Therefore, Logan should make his towertimes shorter than the actual tower. This ismeters high, or choice .Problem 13Angelina drove at an average rate of kph and then stopped minutes for gas. After the stop, she drove at an average rate of kph. Altogether she drove km in a total trip time of hours including the stop. Which equation could be used to solve for the time in hours that she drove before her stop?SolutionThe answer is ()because she drove at kmh for hours (the amount of time before the stop), and 100 kmh for because she wasn't driving for minutes, or hours. Multiplying by gives the total distance, which is kms. Therefore, the answer isProblem 14Triangle has . Let and be on and , respectively, such that . Let be the intersection of segments and , and suppose that is equilateral. What is ?SolutionLet .Since ,Problem 15In a magical swamp there are two species of talking amphibians: toads, whose statements are always true, and frogs, whose statements are always false. Four amphibians, Brian, Chris, LeRoy, and Mike live together in this swamp, and they make the following statements.Brian: "Mike and I are different species."Chris: "LeRoy is a frog."LeRoy: "Chris is a frog."Mike: "Of the four of us, at least two are toads."How many of these amphibians are frogs?SolutionSolution 1We can begin by first looking at Chris and LeRoy.Suppose Chris and LeRoy are the same species. If Chris is a toad, then what he says is true, so LeRoy is a frog. However, if LeRoy is a frog, then he is lying, but clearly Chris is not a frog, and we have a contradiction. The same applies if Chris is a frog.Clearly, Chris and LeRoy are different species, and so we have at least frog out of the two of them.Now suppose Mike is a toad. Then what he says is true because we already have toads. However, if Brian is a frog, then he is lying, yet his statement is true, a contradiction. If Brian is a toad, then what he says is true, but once again it conflicts with his statement, resulting in contradiction.Therefore, Mike must be a frog. His statement must be false, which means that there is at most toad. Since either Chris or LeRoy is already a toad, Brain must be a frog. We can also verify that his statement is indeed false.Both Mike and Brian are frogs, and one of either Chris or LeRoy is a frog, so we have frogs total.Solution 2Start with Brian. If he is a toad, he tells the truth, hence Mike is a frog. If Brian is a frog, he lies, hence Mike is a frog, too. Thus Mike must be a frog.As Mike is a frog, his statement is false, hence there is at most one toad.As there is at most one toad, at least one of Chris and LeRoy is a frog. But then the other one tells the truth, and therefore is a toad. Hence we must have one toad and three frogs.Problem 16Nondegenerate has integer side lengths, is an angle bisector, , and . What is the smallest possible value of the perimeter?SolutionBy the Angle Bisector Theorem, we know that . If we use the lowest possible integer values for AB and BC (the measures of AD and DC, respectively), then , contradicting the Triangle Inequality. If we use the next lowest values (and ), the Triangle Inequality is satisfied. Therefore, our answer is , or choice .Problem 17A solid cube has side length inches. A -inch by -inch square hole is cut into the center of each face. The edges of each cut are parallel to the edges of the cube, and each hole goes all the way through the cube. What is the volume, in cubic inches, of the remaining solid?SolutionSolution 1Imagine making the cuts one at a time. The first cut removes a box . The second cut removes two boxes, each of dimensions, and the third cut does the same as the second cut, on the last two faces. Hence the total volume of all cuts is .Therefore the volume of the rest of the cube is.Solution 2We can use Principle of Inclusion-Exclusion to find the final volume of the cube.There are 3 "cuts" through the cube that go from one end to the other. Each of these "cuts" has cubic inches. However, we can not just sum their volumes, as the central cube is included in each of these three cuts. To get the correct result, we can take the sum of the volumes of the three cuts, and subtract the volume of the central cube twice.Hence the total volume of the cuts is.Therefore the volume of the rest of the cube is.Solution 3We can visualize the final figure and see a cubic frame. We can find the volume of the figure by adding up the volumes of the edges and corners.Each edge can be seen as a box, and each corner can be seen as a box..Problem 18Bernardo randomly picks 3 distinct numbers from the setand arranges them in descending order to form a 3-digit number. Silvia randomly picks 3 distinct numbers from the set and also arranges them in descending order to form a 3-digit number. What is the probability that Bernardo's number is larger than Silvia's number?SolutionWe can solve this by breaking the problem down into cases and adding up the probabilities.Case : Bernardo picks . If Bernardo picks a then it is guaranteed that his number will be larger than Silvia's. The probability that he will pick a is .Case : Bernardo does not pick . Since the chance of Bernardo picking is , the probability of not picking is .If Bernardo does not pick 9, then he can pick any number from to . Since Bernardo is picking from the same set of numbers as Silvia, the probability that Bernardo's number is larger is equal to the probability that Silvia's number is larger.Ignoring the for now, the probability that they will pick the same number is the number of ways to pick Bernardo's 3 numbers divided by the number of ways to pick any 3 numbers.We get this probability to beProbability of Bernardo's number being greater isFactoring the fact that Bernardo could've picked a but didn't:Adding up the two cases we getProblem 19Equiangular hexagon has side lengthsand . The area of is of the area of the hexagon. What is the sum of all possible values of ?SolutionSolution 1It is clear that is an equilateral triangle. From the Law of Cosines, we get that . Therefore, the area of is .If we extend , and so that and meet at , and meet at , and and meet at , we find that hexagon is formed by taking equilateral triangle of side length and removing three equilateral triangles, , and , of side length . The area of is therefore.Based on the initial conditions,Simplifying this gives us . By Vieta's Formulas we know that the sum of the possible value of is .Solution 2As above, we find that the area of is .We also find by the sine triangle area formula that, and thusThis simplifies to.Problem 20A fly trapped inside a cubical box with side length meter decides to relieve its boredom by visiting each corner of the box. It will begin and end in the same corner and visit each of the other corners exactly once. To get from a corner to any other corner, it will either fly or crawl in a straight line. What is the maximum possible length, in meters, of its path?SolutionThe distance of an interior diagonal in this cube is and the distance of a diagonal on one of the square faces is . It would not make sense if the fly traveled an interior diagonal twice in a row, as it would return to the point it just came from, so at most the final sum can only have 4 as the coefficient of . The other 4 paths taken can be across a diagonal on one of the faces, so the maximum distance traveled is.Problem 21The polynomial has three positive integer zeros. What is the smallest possible value of ?SolutionBy Vieta's Formulas, we know that is the sum of the three roots of the polynomial . Also, 2010 factors into. But, since there are only three roots to the polynomial, two of the four prime factors must be multiplied so that we are left with three roots. To minimize , and should be multiplied, which means will be and the answer is .Problem 22Eight points are chosen on a circle, and chords are drawn connecting every pair of points. No three chords intersect in a single point insidethe circle. How many triangles with all three vertices in the interior of the circle are created?SolutionTo choose a chord, we know that two points must be chosen. This implies that for three chords to create a triangle and not intersect at a single point, six points need to be chosen. Therefore, the answer is which is equivalent to 28,Problem 23Each of 2010 boxes in a line contains a single red marble, and for , the box in the position also contains white marbles. Isabella begins at the first box and successively draws a single marble at random from each box, in order. She stops when she first draws a red marble. Let be the probability that Isabella stops afterdrawing exactly marbles. What is the smallest value of for which ?SolutionThe probability of drawing a white marble from box is . Theprobability of drawing a red marble from box is .The probability of drawing a red marble at box is thereforeIt is then easy to see that the lowest integer value of that satisfies the inequality is .Problem 24The number obtained from the last two nonzero digits of is equal to . What is ?SolutionWe will use the fact that for any integer ,First, we find that the number of factors of in is equal to. Let . The we want is therefore the last two digits of , or . Since there is clearly an excess of factors of 2, we know that , so it remains to find .If we divide by by taking out all the factors of in , we canwrite as where where every multiple of 5 is replaced by the number with all its factors of 5 removed. Specifically, every number in the form is replaced by , and every number in the form is replaced by .The number can be grouped as follows:Using the identity at the beginning of the solution, we can reducetoUsing the fact that (or simply the fact that if you have your powers of 2 memorized), we can deduce that . Therefore.Finally, combining with the fact that yields.Problem 25Jim starts with a positive integer and creates a sequence of numbers. Each successive number is obtained by subtracting the largest possible integer square less than or equal to the current number until zero is reached. For example, if Jim starts with , then his sequence contains numbers:Let be the smallest number for which Jim’s sequence has numbers. What is the units digit of ?SolutionWe can find the answer by working backwards. We begin with on the bottom row, then the goes to the right of the equal's sign in the row above. We find the smallest value for whichand , which is .We repeat the same procedure except with for the next row and for the row after that. However, at the fourth row, wesee that solving yields , in which case it would be incorrect since is not the greatest perfect square less than or equal to . So we make it a and solve . We continue on using this same method where we increase the perfect square until can be made bigger than it. When we repeat this until we have rows, we get:Hence the solution is the last digit of , which is .。
AMC美国数学竞赛2003AMC10A试题及标准答案解析

2003 AMC 10A1、What is the difference between the sum of the first even counting numbers and the sum of the first odd counting numbers?SolutionThe first even counting numbers are .The first odd counting numbers are .Thus, the problem is asking for the value of..Alternatively, using the sum of an arithmetic progression formula, we can write .2、Members of the Rockham Soccer League buy socks and T-shirts. Socks cost per pair and each T-shirt costs more than a pair of socks. Each member needs one pair of socks and a shirt for home games and another pair of socks and a shirt for away games. If the total cost is , how many members are in the League?SolutionSince T-shirts cost dollars more than a pair of socks, T-shirts cost dollars.Since each member needs pairs of socks and T-shirts, the total cost for member is dollars.Since dollars was the cost for the club, and was the cost per member, the number of members in the League is .3、A solid box is cm by cm by cm. A new solid is formed by removing a cube cm on a side from each corner of this box. What percent of the original volume is removed?SolutionThe volume of the original box isThe volume of each cube that is removed isSince there are corners on the box, cubes are removed.So the total volume removed is .Therefore, the desired percentage is .4、It takes Mary minutes to walk uphill km from her home to school, but it takes her only minutes to walk from school to home along the same route. What is her average speed, in km/hr, for the round trip?SolutionSince she walked km to school and km back home, her total distance is km.Since she spent minutes walking to school and minutes walking back home, her total time is minutes = hours.Therefore her average speed in km/hr is5、Let and denote the solutions of . What is the value of ?SolutionUsing factoring:orSo and are and .Therefore the answer isOR we can use sum and product.6、Define to be for all real numbers and . Which of the following statements is not true?SolutionExamining statement C:when , but statement C says that it does for all . Therefore the statement that is not true is "for all " Alternatively, consider that the given "heart function" is actually the definition of the distance between two points. Examining all of the statements, only C is not necessarily true; if c is negative, the distance between and is the absolute value of , not itself, because distance is always nonnegative.7、How many non-congruent triangles with perimeter have integer side lengths?SolutionBy the triangle inequality, no one side may have a length greater than half the perimeter, which isSince all sides must be integers, the largest possible length of a side isTherefore, all such triangles must have all sides of length , , or . Since , at least one side must have a length of Thus, the remaining two sides have a combined length of . So, the remaining sides must be either and or and . Therefore, the number of triangles is .8、What is the probability that a randomly drawn positive factor ofis lessthan ?SolutionFor a positive number which is not a perfect square, exactly half of the positive factors will be less than .Since is not a perfect square, half of the positive factors of will be less than .Clearly, there are no positive factors of between and . Therefore half of the positive factors will be less than .So the answer is .9、SimplifySolution.Therefore:10、The polygon enclosed by t he solid lines in the figure consists of congruent squares joined edge-to-edge. One more congruent square is attached to an edge at one of the nine positions indicated. How many of the nine resulting polygons can be folded to form a cube with one face missing?SolutionLet the squares be labeled , , , and .When the polygon is folded, the "right" edge of square becomes adjacent to the "bottom edge" of square , and the "bottom" edge of square becomes adjacent to the "bottom" edge of square .So, any "new" square that is attached to those edges will prevent the polygon from becoming a cube with one face missing.Therefore, squares , , and will prevent the polygon from becoming a cube with one face missing.Squares , , , , , and will allow the polygon to become a cube with one face missing when folded.Thus the answer is .Another way to think of it is that a cube missing one face has of it's faces. Since the shape has faces already, we need another face. The only way to add anopther face is if the added square does not overlap any of the others. ,, and overlap, while 9 do not. The answer is11、The sum of the two -digit numbers and is . What is ?SolutionSince , , and are digits, , , .Therefore, .12、A point is randomly picked from inside the rectangle withvertices , , , and . What is the probability that ?SolutionThe rectangle has a width of and a height of .The area of this rectangle is .The line intersects the rectangle at and .The area which is the right isosceles triangle with side length that has vertices at , , and .The area of this triangle isTherefore, the probability that is13、The sum of three numbers is . The first is times the sum of the other two. The second is seven times the third. What is the product of all three?SolutionSolution 1Let the numbers be , , and in that order. The given tells us thatTherefore, the product of all three numbers is.Solution 2Alternatively, we can set up the system in matrix form:Or, in matrix formTo solve this matrix equation, we can rearrange it thus:Solving this matrix equation by using inverse matrices and matrix multiplication yieldsWhich means that , , and . Therefore,14、Let be the largest integer that is the product of exactly distinct prime numbers, , , and , where and are single digits. What is the sum of the digits of ?SolutionSince is a single digit prime number, the set of possible values of is .Since is a single digit prime number and is the units digit of the prime number , the set of possible values of is .Using these values for and , the set of possible values of isOut of this set, the prime values areTherefore the possible values of are:The largest possible value of is .So, the sum of the digits of is15、What is the probability that an integer in the set is divisible by and not divisible by ?SolutionThere are integers in the set.Since every 2nd integer is divisible by , there are integers divisible by in the set.To be divisible by both and , a number must be divisible by .Since every 6th integer is divisible by , there are integers divisible by both and in the set.So there are integers in this set that are divisible by and not divisible by .Therefore, the desired probability is16、What is the units digit of ?SolutionSince :Therefore, the units digit is17、The number of inches in the perimeter of an equilateral triangle equals the number of square inches in the area of its circumscribed circle. What is the radius, in inches, of the circle?SolutionLet be the length of a side of the equilateral triangle and let be the radius of the circle.In a circle with a radius the side of an inscribed equilateral triangle is .So .The perimeter of the triangle isThe area of the circle isSo:18、What is the sum of the reciprocals of the roots of the equationSolutionMultiplying both sides by :Let the roots be and .The problem is asking forBy Vieta's formulas:So the answer is .19、A semicircle of diameter sits at the top of a semicircle of diameter , as shown. The shaded area inside the smaller semicircle and outside the larger semicircle is called a lune. Determine the area of this lune.SolutionThe shaded area is equal to the area of the smaller semicircle minus the area of a sector of the larger circle plus the area of a triangle formed by two radii of the larger semicircle and the diameter of the smaller semicircle.The area of the smaller semicircle is .Since the radius of the larger semicircle is equal to the diameter of the smaller semicircle, the triangle is an equilateral triangle and the sector measures .The area of the sector of the larger semicircle is . The area of the triangle isSo the shaded area is20、A base-three-digit number is selected at random. Which of the following is closest to the probability that the base-representation and the base-representation of are both three-digit numerals?SolutionTo be a three digit number in base-10:Thus there are three-digit numbers in base-10To be a three-digit number in base-9:To be a three-digit number in base-11:So,Thus, there are base-10 three-digit numbers that are three digit numbers in base-9 and base-11.Therefore the desired probability is .21、Pat is to select six cookies from a tray containing only chocolate chip, oatmeal, and peanut butter cookies. There are at least six of each of these three kinds of cookies on the tray. How many different assortments of six cookies can be selected?SolutionSolution 1Let the ordered triplet represent the assortment of chocolate chip cookies, oatmeal cookies, and peanut butter cookies.Using casework:Pat selects chocolate chip cookies:Pat needs to select more cookies that are either oatmeal or peanut butter.The assortments are:assortments.Pat selects chocolate chip cookie:Pat needs to select more cookies that are either oatmeal or peanut butter.The assortments are:assortments.Pat selects chocolate chip cookies:Pat needs to select more cookies that are either oatmeal or peanut butter.The assortments are:assortments.Pat selects chocolate chip cookies:Pat needs to select more cookies that are either oatmeal or peanut butter.The assortments are:assortments.Pat selects chocolate chip cookies:Pat needs to select more cookies that are either oatmeal or peanut butter.The assortments are: assortments.Pat selects chocolate chip cookies:Pat needs to select more cookies that are either oatmeal or peanut butter.The assortments are: assortments.Pat selects chocolate chip cookies:Pat needs to select more cookies that are either oatmeal or peanut butter.The only assortment is: assortment.The total number of assortments of cookies that can be collected isSolution 2It is given that it is possible to select at least 6 of each. Therefore, we can make a bijection to the number of ways to divide the six choices into three categories, since it is assumed that their order is unimportant. Using the ball and urns formula, the number of ways to do this is22、In rectangle , we have , , is on with, is on with , line intersects line at , and is on line with . Find the length .SolutionS olution 1(Opposite angles are equal).(Both are 90 degrees).(Alt. Interior Angles are congruent).Therefore and are similar. and are also similar.is 9, therefore must equal 5. Similarly, must equal 3. Because and are similar, the ratio of and , must also hold true for and . , so is of . By Pythagorean theorem, ..So ..Therefore .Solution 2Since is a rectangle, .Since is a rectangle and ,.Since is a rectangle, .So, is a transversal, and .This is sufficient to prove that and .Using ratios:Since can't have 2 different lengths, both expressions for must be equal.Solution 3Since is a rectangle, , , and . From the Pythagorean Theorem, .LemmaStatement:Proof: , obviously.Since two angles of the triangles are equal, the third angles must equal each other. Therefore, the triangles are similar.Let .Also, , thereforeWe can multiply both sides by to get that is twice of 10, orSolution 4We extend BC such that it intersects GF at X. Since ABCD is a rectangle, it follows that CD=8, therefore, XF=8. Let GX=y. From the similarity of triangles GCH and GEA, we have the ratio 3:5 (as CH=9-6=3, and EA=9-4=5). GX and GF are the altitudes of GCH andGEA, respectively. Thus, y:y+8 = 3:5, from which we have y=12, thus GF=y+8=12+8=20. B.23、A large equilateral triangle is constructed by using toothpicks to create rows of small equilateral triangles. For example, in the figure we have rows of small congruent equilateral triangles, with small triangles in the base row. How many toothpicks would be needed to construct a large equilateral triangle if the base row of the triangle consists of small equilateral triangles?SolutionSolution 1There are small equilateral triangles.Each small equilateral triangle needs toothpicks to make it.But, each toothpick that isn't one of the toothpicks on the outside of the large equilateral triangle is a side for small equilateral triangles.So, the number of toothpicks on the inside of the large equilateral triangle isTherefore the total number of toothpicks isSolution 2We see that the bottom row of small triangles is formed from downward-facing triangles and upward-facing triangles. Since each downward-facing triangle uses three distinct toothpicks, andsince the total number of downward-facing triangles is, we have that the total number of toothpicks is24、Sally has five red cards numbered through and four blue cards numbered through . She stacks the cards so that the colors alternate and so that the number on each red card divides evenly into the number on each neighboring blue card. What is the sum of the numbers on the middle three cards?SolutionLet and designate the red card numbered and the blue card numbered , respectively.is the only blue card that evenly divides, so must be at one end of the stack and must be the card next to it.is the only other red card that evenly divides , so must be the other card next to .is the only blue card that evenly divides, so must be at the other end of the stack and must be the card next to it.is the only other red card that evenly divides , so must be the other card next to .doesn't evenly divide , so must be next to , must be next to , and must be in the middle.This yields the following arrangement from top to bottom:Therefore, the sum of the numbers on the middle three cards is.25、Let be a -digit number, and let and be the quotient and remainder, respectively, when is divided by . For how many values of is divisible by ?SolutionSolution 1When a -digit number is divided by , the first digits become the quotient, , and the last digits become the remainder, .Therefore, can be any integer from to inclusive, and can be any integer from to inclusive.For each of the possible values of , there are at least possible values of such that .Since there is "extra" possible value of that is congruent to , each of the values of that are congruent tohave more possible value of such that .Therefore, the number of possible values of such that is .Solution 2Let equal , where through are digits. Therefore,We now take :The divisor trick for 11 is as follows:"Let be an digit integer. Ifis divisible by , then is also divisible by ."Therefore, the five digit number is divisible by 11. The 5-digit multiples of 11 range from to . There aredivisors of 11 between those inclusive..- Solution 3Since q is a quotient and r is a remainder when n is divided by 100. So we have . Since we are counting choices where q+r is divisible by 11, we have for some k. This means that n is the sum of two multiples of 11 and would thus itself be a divisor of 11. Then we can count all the four digit divisors of 11 as in Solution 2. (This solution is essentially the same as solution 2, but it does not necessarily involve mods and so could potentially be faster.)NotesThe part labeled "divisor trick" actually follows from the same observation we made in the previous step: , therefore and for all . For a digit numberwe get, as claimed.Also note that in the "divisor trick" we actually want to assign the signs backwards - if we make sure that the last sign is a , the result will have the same remainder modulo as the original number.。
2007 AMC 10A 试题及答案解析

2007 AMC 10A1、One ticket to a show costs at full price. Susan buys 4 ticketsusing a coupon that gives her a 25% discount. Pam buys 5 tickets using a coupon that gives her a 30% discount. How many more dollars does Pam pay than Susan?Solution= the amount Pam spent = the amount Susan spent▪▪Pam pays 10 more dollars than Susan2、Define and . What is ?Solution3、An aquarium has a rectangular base that measures 100 cm by 40cm and has a height of 50 cm. It is filled with water to a height of 40 cm. A brick with a rectangular base that measures 40 cm by 20 cm and a height of 10 cm is placed in the aquarium. By how many centimeters does the water rise?SolutionThe volume of the brick is . Thus the water volume rose .4、The larger of two consecutive odd integers is three times thesmaller. What is their sum?SolutionLet the two consecutive odd integers be , . Then , so and their sum is .5、A school store sells 7 pencils and 8 notebooks for . It also sells 5 pencils and 3 notebooks for . How much do 16 pencils and 10 notebooks cost?SolutionWe let cost of pencils in cents, number of notebooks in cents. ThenSubtracting these equations yields . Backwards solving gives . Thus the answer is .6、At Euclid High School, the number of students taking the AMC10 was 60 in 2002, 66 in 2003, 70 in 2004, 76 in 2005, 78 and 2006, and is 85 in 2007. Between what two consecutive years was there the largest percentage increase?SolutionWe compute the percentage increases:1.2.3.4.5.The answer is .In fact, the answer follows directly from examining the differences between each year. The largest differences are , and it is easy to see that due to the decreased starting number of students in 2002 that that will be our answer.7、Last year Mr. John Q. Public received an inheritance. He paid 20% in federal taxes on the inheritance, and paid 10% of what he has left in state taxes. He paid a total of for both taxes. How many dollars was the inheritance?SolutionAfter paying his taxes, he has of the inheritance left. Since is of the inheritance, the whole inheritance is.8、Triangles and are isosceles with and . Point D is inside . , and . What is the degree measure of ?SolutionWe angle chase, and find out that:▪▪▪9、Real numbers and satisfy the equations and. What is ?SolutionAndSubstitution gives , and solving for yields . Thus .10、The Dunbar family consists of a mother, a father, and somechildren. The average age of the members of the family is , the father is years old, and the average age of the mother and children is . How many children are in the family?SolutionLet be the number of children. Then the total ages of the family is , and the total number of people in the family is . So11、The numbers from to are placed at the vertices of a cube in sucha manner that the sum of the four numbers on each face is the same.What is this common sum?SolutionThe sum of the numbers on the top face of a cube is equal to the sum of the numbers on the bottom face of the cube; these numbersrepresent all of the vertices of the cube. Thus the answer is.12、Two tour guides are leading six tourists. The guides decide to split up. Each tourist must choose one of the guides, but with the stipulation that each guide must take at least one tourist. How many different groupings of guides and tourists are possible?SolutionEach tourist has to pick in between the guides, so for tourists there are possible groupings. However, since each guide must take at least one tourist, we subtract the cases where a guide has no tourist. Thus the answer is .13、Yan is somewhere between his home and the stadium. To get to the stadium he can walk directly to the stadium, or else he can walk home and then ride his bicycle to the stadium. He rides times as fast as he walks, and both choices require the same amount of time. What is the ratio of Yan's distance from his home to his distance from the stadium?SolutionLet the distance from Yan's initial position to the stadium be and the distance from Yan's initial position to home be . We are trying to find , and we have the following identity given by the problem:Thus and the answer is14、A triangle with side lengths in the ratio is inscribed in a circle of radius . What is the area of the triangle?SolutionSince 3-4-5 is a Pythagorean triple, the triangle is a right triangle. Since the hypotenuse is a diameter of the circumcircle, the hypotenuse is . Then the other legs are and . The area is15、Four circles of radius are each tangent to two sides of a square and externally tangent to a circle of radius , as shown. What is the area of the square?SolutionThe diagonal has length . Therefore the sides have length , and the area isAlternate SolutionExtend two radii from the larger circle to the centers of the two smaller circles above. This forms a right triangle of sides . The length of the hypotenuse of the right triangle plus twice the radius of the smaller circle is equal to the side of the square. It follows, then16、Integers , , , and , not necessarily distinct, are chosen independently and at random from to , inclusive. What is the probability that is even?SolutionThe only times when is even is when and are of the same parity. The chance of being odd is , so it has a probability of being even. Therefore, the probability that will be even is.17、Suppose that and are positive integers such that . What is the minimum possible value of ?Solutionmust be a perfect cube, so each power of a prime in the factorization for must be divisible by . Thus the minimum value of is , which makes . These sum to .18、Consider the -sided polygon , as shown. Each of its sides has length , and each two consecutive sides form a right angle. Suppose that and meet at . What is the area of quadrilateral ?SolutionSolution 1We can obtain the solution by calculating the area of rectangleminus the combined area of triangles and .We know that triangles and are similar because. Also, since , the ratio of the distance from toto the distance from to is also . Solving with the fact that the distance from to is 4, we see that the distance from tois .The area of is simply , the area of is, and the area of rectangle is .Taking the area of rectangle and subtracting the combined area of and yields .Solution 2Extend and and call their intersection .The triangles and are clearly similar with ratio , hence and thus . The area of the triangle is . The triangles and are similar as well, and we now know that the ratio of their dimensions is .Draw altitudes from onto and , let their feet be and . We get that . Hence .Then the area of is , and the area ofcan be obtained by subtracting the area of , which is . Hence the answer is .19、A paint brush is swept along both diagonals of a square to produce the symmetric painted area, as shown. Half the area of the square is painted. What is the ratio of the side length of the square to the brush width?SolutionSolution 1Without loss of generality, let the side length of the square be unit. The area of the painted area is of the area of the larger square, sothe total unpainted area is also . Each of the unpainted triangle hasarea . It is easy to tell that these triangles are isosceles right triangles, so let be the side length of one of the smaller triangles:The diagonal of the triangle is . The corners of the painted areasare also isosceles right triangles with side length . Its diagonal is equal to the width of the paint, and is . The answer we are looking for is thus . Multiply the numerator and the denominator by to simplify, and you get orwhich is .Solution 2Again, have the length of the square equal to and let the width of each individual stripe be . Note that you can split each stripe into two rectangles and two isosceles right triangles at the corners. Thenthe area of each stripe is . The area covered by the two total stripes is twice the area of one stripe, minus the area in the intersection of the stripes, which is a square with side length . This area is equal to So:.By the quadratic formula,It's easy to tell that is too large, so . We want to find , and . Multiply the numerator and the denominator by ,20、Suppose that the number satisfies the equation . What is the value of ?SolutionSolution 1Notice that . Thus.Solution 2. We apply the quadratic formula to get.Thus (so it doesn't matter which root of we use). Using the binomial theorem we can expand this out and collect terms to get .Solution 3We know that . We can square both sides to get, so . Squaring both sides again gives, so . Q. E. D.Solution 4We let and be roots of a certain quadratic. Specifically. We use Newton's Sums given the coefficients to find .21、A sphere is inscribed in a cube that has a surface area of square meters. A second cube is then inscribed within the sphere. What is the surface area in square meters of the inner cube?SolutionSolution 1We rotate the smaller cube around the sphere such that two opposite vertices of the cube are on opposite faces of the larger cube. Thus the main diagonal of the smaller cube is the side length of the outer square.Let be the surface area of the inner square. The ratio of the areas of two similar figures is equal to the square of the ratio of their sides. As the diagonal of a cube has length where is a side of the cube, the ratio of a side of the inner square to that of the outer square (and the side of the outer square = the diagonal of the inner square), we have . Thus .Solution 2 (computation)The area of each face of the outer cube is , and the edge length of the outer cube is . This is also the diameter of the sphere, and thus the length of a long diagonal of the inner cube.A long diagonal of a cube is the hypotenuse of a right triangle with a side of the cube and a face diagonal of the cube as legs. If a side of the cube is , we see that .Thus the surface area of the inner cube is .22、A finite sequence of three-digit integers has the property that the tens and units digits of each terms are, respectively, the hundreds and tens digits of the next term, and the tens and units digits of the last term are, respectively, the hundreds and tens digits of the first term. For example, such a sequence might begin with terms , , and and end with the term . Let be the sum of all the terms in the sequence. What is the largest prime number that always divides ?SolutionA given digit appears as the hundreds digit, the tens digit, and the units digit of a term the same number of times. Let be the sum of theunits digits in all the terms. Then , so must be divisible by . To see that it need not be divisible by any larger prime, the sequence gives .23、How many ordered pairs of positive integers, with , have the property that their squares differ by ?SolutionFor every two factors , we have. Since ,, from which it follows that the number of ordered pairs is given by the number of ordered pairs. There are factors of , which give us six pairs . However, since are positive integers, we also need that are positive integers, so and must have the same parity. Thus we exclude the factors , and we are left with four pairs .24、Circles centered at and each have radius , as shown. Point is the midpoint of , and . Segments and are tangent to the circles centered at and , respectively, and is a common tangent. What is the area of the shaded region ?SolutionThe area we are trying to find is simply. Obviously, . Thus, is a rectangle, and so its area is.Since is tangent to circle , is a right . We knowand , so is isosceles, a -right , andhas with length . The area of . For obvious reasons, , and so the area of is also .(or , for that matter) is the area of its circle. Thusand both have an area of .Plugging all of these areas back into the original equation yields.25、For each positive integer , let denote the sum of the digits of For how many values of isSolutionSolution 1For the sake of notation let . Obviously . Then the maximum value of is when , and the sum becomes . So the minimum bound is . We do casework upon the tens digit:Case 1: . Easy to directly disprove.Case 2: . , and if and otherwise.Subcase a:.This exceeds our bounds, so no solution here.Subcase b:.First solution.Case 3: . , and if andotherwise.Subcase a:. Second solution.Subcase b: . Third solution.Case 4: . But , and the these clearly sum to .Case 5: . So and (recall that ), and . Fourth solution.In total we have solutions, which are and . Solution 2Clearly, . We can break this up into three cases:Case 1:Inspection gives .Case 2: , ,If you set up an equation, it reduces towhich has as its only solution satisfying the constraints , .Case 3: , ,This reduces to. The only two solutions satisfying the constraints for this equation are , and , .The solutions are thus and the answer is . Solution 3As in Solution 1, we note that and . Obviously, .As , this means that , or equivalently that .Thus . For each possible we get three possible .(E. g., if , then is a number such that and, therefore .)For each of these nine possibilities we compute asand check whether .We'll find out that out of the 9 cases, in 4 the value has the correct sum of digits.This happens for .。
2010AMC10A试题及答案解析

2010 AMC 10A problems and solutions.The test was held on February 8, 2010. The first link contains the full set of test problems. The rest contain each individual problem and its solution.Problem 1Mary’s top book shelf holds five books with the follow ing widths, incentimeters: , , , , and . What is the average book width, incentimeters?SolutionTo find the average, we add up the widths , , , , and , to geta total sum of . Since there are books, the average book width isThe answer is .Problem 2Four identical squares and one rectangle are placed together to form one large square as shown. The length of the rectangle is how many times as large as its width?SolutionLet the length of the small square be , intuitively, the length of the big square is . It can be seen that the width of the rectangle is .Thus, the length of the rectangle is times large as thewidth. The answer is .Problem 3Tyrone had marbles and Eric had marbles. Tyrone then gavesome of his marbles to Eric so that Tyrone ended with twice as many marbles as Eric. How many marbles did Tyrone give to Eric?SolutionLet be the number of marbles Tyrone gave to Eric. Then,. Solving for yields and . Theanswer is .Problem 4A book that is to be recorded onto compact discs takes minutes toread aloud. Each disc can hold up to minutes of reading. Assumethat the smallest possible number of discs is used and that each disc contains the same length of reading. How many minutes of reading will each disc contain?SolutionAssuming that there were fractions of compact discs, it would takeCDs to have equal reading time. However, since the number of discs can only be a whole number, there are at least 8 CDs,in which case it would have minutes on each of the 8 discs.The answer is .Problem 5The area of a circle whose circumference is is . What is thevalue of ?SolutionIf the circumference of a circle is , the radius would be . Sincethe area of a circle is , the area is . The answer is .Problem 6For positive numbers and the operation is defined asWhat is ?Solution. Then, is The answer isProblem 7Crystal has a running course marked out for her daily run. She starts this run by heading due north for one mile. She then runs northeast for one mile, then southeast for one mile. The last portion of her run takes her on a straight line back to where she started. How far, in miles is this last portion of her run?SolutionCrystal first runs North for one mile. Changing directions, she runs Northeast for another mile. The angle difference between North and Northeast is 45 degrees. She then switches directions to Southeast, meaning a 90 degree angle change. The distance now from travellingNorth for one mile, and her current destination is miles, because it is the hypotenuse of a 45-45-90 triangle with side length one (mile). Therefore, Crystal's distance from her starting position, x, is equal to, which is equal to . The answer isTony works hours a day and is paid $per hour for each full yearof his age. During a six month period Tony worked days and earned$. How old was Tony at the end of the six month period?SolutionTony worked hours a day and is paid dollars per hour for eachfull year of his age. This basically says that he gets a dollar for each year of his age. So if he is years old, he gets dollars a day. Wealso know that he worked days and earned dollars. If he wasyears old at the beginning of his working period, he would have earned dollars. If he was years old at the beginningof his working period, he would have earned dollars.Because he earned dollars, we know that he was for someperiod of time, but not the whole time, because then the money earned would be greater than or equal to . This is why he waswhen he began, but turned sometime in the middle and earneddollars in total. So the answer is .The answer is . We could findout for how long he was and . . Then isand we know that he was for days, and for days. Thus,the answer is .Problem 9A palindrome, such as , is a number that remains the same whenits digits are reversed. The numbers and are three-digit andfour-digit palindromes, respectively. What is the sum of the digits ofSolutionis at most , so is at most . The minimum value ofis . However, the only palindrome between and is ,which means that must be .It follows that is , so the sum of the digits is .Marvin had a birthday on Tuesday, May 27 in the leap year . Inwhat year will his birthday next fall on a Saturday?Solution(E) 2017There are 365 days in a non-leap year. There are 7 days in a week. Since 365 = 52 * 7 + 1 (or 365 is congruent to 1 mod 7), the same date (after February) moves "forward" one day in the subsequent year, if that year is not a leap year.For example: 5/27/08 Tue 5/27/09 WedHowever, a leap year has 366 days, and 366 = 52 * 7 + 2. So the same date (after February) moves "forward" two days in the subsequent year, if that year is a leap year.For example: 5/27/11 Fri 5/27/12 SunYou can keep count forward to find that the first time this date falls on a Saturday is in 2017:5/27/13 Mon 5/27/14 Tue 5/27/15 Wed 5/27/16 Fri 5/27/17 Sat Problem 11The length of the interval of solutions of the inequality is. What is ?SolutionSince we are given the range of the solutions, we must re-write the inequalities so that we have in terms of and .Subtract from all of the quantities:Divide all of the quantities by .Since we have the range of the solutions, we can make them equal to .Multiply both sides by 2.Re-write without using parentheses.Simplify.We need to find for the problem, so the answer isProblem 12Logan is constructing a scaled model of his town. The city's water tower stands 40 meters high, and the top portion is a sphere that holds 100,000 liters of water. Logan's miniature water tower holds 0.1 liters. How tall, in meters, should Logan make his tower?SolutionThe water tower holds times more water than Logan's miniature. Therefore, Logan should make his towertimes shorter than the actual tower. This ismeters high, or choice .Problem 13Angelina drove at an average rate of kph and then stoppedminutes for gas. After the stop, she drove at an average rate ofkph. Altogether she drove km in a total trip time of hoursincluding the stop. Which equation could be used to solve for the time in hours that she drove before her stop?SolutionThe answer is ()because she drove at kmh for hours (theamount of time before the stop), and 100 kmh for because shewasn't driving for minutes, or hours. Multiplying by gives thetotal distance, which is kms. Therefore, the answer isProblem 14Triangle has . Let and be on and ,respectively, such that . Let be the intersection ofsegments and , and suppose that is equilateral. What isSolutionLet .Since ,Problem 15In a magical swamp there are two species of talking amphibians: toads, whose statements are always true, and frogs, whose statements are always false. Four amphibians, Brian, Chris, LeRoy, and Mike live together in this swamp, and they make the following statements.Brian: "Mike and I are different species."Chris: "LeRoy is a frog."LeRoy: "Chris is a frog."Mike: "Of the four of us, at least two are toads."How many of these amphibians are frogs?SolutionSolution 1We can begin by first looking at Chris and LeRoy.Suppose Chris and LeRoy are the same species. If Chris is a toad, then what he says is true, so LeRoy is a frog. However, if LeRoy is a frog, then he is lying, but clearly Chris is not a frog, and we have a contradiction. The same applies if Chris is a frog.Clearly, Chris and LeRoy are different species, and so we have at least frog out of the two of them.Now suppose Mike is a toad. Then what he says is true because we already have toads. However, if Brian is a frog, then he is lying, yethis statement is true, a contradiction. If Brian is a toad, then what he says is true, but once again it conflicts with his statement, resulting in contradiction.Therefore, Mike must be a frog. His statement must be false, which means that there is at most toad. Since either Chris or LeRoy isalready a toad, Brain must be a frog. We can also verify that his statement is indeed false.Both Mike and Brian are frogs, and one of either Chris or LeRoy is afrog, so we have frogs total.Solution 2Start with Brian. If he is a toad, he tells the truth, hence Mike is a frog. If Brian is a frog, he lies, hence Mike is a frog, too. Thus Mike must be a frog.As Mike is a frog, his statement is false, hence there is at most one toad.As there is at most one toad, at least one of Chris and LeRoy is a frog. But then the other one tells the truth, and therefore is a toad. Hence we must have one toad and three frogs.Problem 16Nondegenerate has integer side lengths, is an anglebisector, , and . What is the smallest possible value ofthe perimeter?SolutionBy the Angle Bisector Theorem, we know that . If we use the lowest possible integer values for AB and BC (the measures of AD and DC, respectively), then ,contradicting the Triangle Inequality. If we use the next lowest values (and ), the Triangle Inequality is satisfied. Therefore,our answer is , or choice .Problem 17A solid cube has side length inches. A -inch by -inch square hole iscut into the center of each face. The edges of each cut are parallel to the edges of the cube, and each hole goes all the way through the cube. What is the volume, in cubic inches, of the remaining solid?SolutionSolution 1Imagine making the cuts one at a time. The first cut removes a box . The second cut removes two boxes, each of dimensions, and the third cut does the same as the second cut, on thelast two faces. Hence the total volume of all cuts is .Therefore the volume of the rest of the cube is.Solution 2We can use Principle of Inclusion-Exclusion to find the final volume of the cube.There are 3 "cuts" through the cube that go from one end to the other. Each of these "cuts" has cubic inches. However, we cannot just sum their volumes, as the central cube is included ineach of these three cuts. To get the correct result, we can take the sum of the volumes of the three cuts, and subtract the volume of the central cube twice.Hence the total volume of the cuts is.Therefore the volume of the rest of the cube is.Solution 3We can visualize the final figure and see a cubic frame. We can find the volume of the figure by adding up the volumes of the edges and corners.Each edge can be seen as a box, and each corner can beseen as a box..Problem 18Bernardo randomly picks 3 distinct numbers from the setand arranges them in descending order to form a 3-digit number. Silvia randomly picks 3 distinct numbers from the setand also arranges them in descending order to form a 3-digit number. What is the probability that Bernardo's number is larger than Silvia's number?SolutionWe can solve this by breaking the problem down into cases andadding up the probabilities.Case : Bernardo picks . If Bernardo picks a then it is guaranteedthat his number will be larger than Silvia's. The probability that he willpick a is .Case : Bernardo does not pick . Since the chance of Bernardopicking is , the probability of not picking is .If Bernardo does not pick 9, then he can pick any number from to .Since Bernardo is picking from the same set of numbers as Silvia, the probability that Bernardo's number is larger is equal to the probability that Silvia's number is larger.Ignoring the for now, the probability that they will pick the samenumber is the number of ways to pick Bernardo's 3 numbers divided by the number of ways to pick any 3 numbers.We get this probability to beProbability of Bernardo's number being greater isFactoring the fact that Bernardo could've picked a but didn't:Adding up the two cases we getProblem 19Equiangular hexagon has side lengthsand . The area of is of the area of thehexagon. What is the sum of all possible values of ?SolutionSolution 1It is clear that is an equilateral triangle. From the Law ofCosines, we get that . Therefore,the area of is .If we extend , and so that and meet at , andmeet at , and and meet at , we find that hexagonis formed by taking equilateral triangle of side lengthand removing three equilateral triangles, , and ,of side length . The area of is therefore.Based on the initial conditions,Simplifying this gives us . By Vieta's Formulas we knowthat the sum of the possible value of is .Solution 2As above, we find that the area of is .We also find by the sine triangle area formula that, and thusThis simplifies to.Problem 20A fly trapped inside a cubical box with side length meter decides torelieve its boredom by visiting each corner of the box. It will begin and end in the same corner and visit each of the other corners exactly once. To get from a corner to any other corner, it will either fly or crawl in a straight line. What is the maximum possible length, in meters, of its path?SolutionThe distance of an interior diagonal in this cube is and the distanceof a diagonal on one of the square faces is . It would not make sense if the fly traveled an interior diagonal twice in a row, as it would return to the point it just came from, so at most the final sum can onlyhave 4 as the coefficient of . The other 4 paths taken can be across a diagonal on one of the faces, so the maximum distance traveled is.Problem 21The polynomial has three positive integer zeros. What is the smallest possible value of ?SolutionBy Vieta's Formulas, we know that is the sum of the three roots ofthe polynomial . Also, 2010 factors into. But, since there are only three roots to the polynomial,two of the four prime factors must be multiplied so that we are left with three roots. To minimize , and should be multiplied, whichmeans will be and the answer is .Problem 22Eight points are chosen on a circle, and chords are drawn connecting every pair of points. No three chords intersect in a single point insidethe circle. How many triangles with all three vertices in the interior of the circle are created?SolutionTo choose a chord, we know that two points must be chosen. This implies that for three chords to create a triangle and not intersect at a single point, six points need to be chosen. Therefore, the answer iswhich is equivalent to 28,Problem 23Each of 2010 boxes in a line contains a single red marble, and for , the box in the position also contains white marbles.Isabella begins at the first box and successively draws a single marble at random from each box, in order. She stops when she first draws ared marble. Let be the probability that Isabella stops after drawing exactly marbles. What is the smallest value of for whichSolutionThe probability of drawing a white marble from box is . Theprobability of drawing a red marble from box is .The probability of drawing a red marble at box is thereforeIt is then easy to see that the lowest integer value of that satisfiesthe inequality is .Problem 24The number obtained from the last two nonzero digits of is equal to. What is ?SolutionWe will use the fact that for any integer ,First, we find that the number of factors of in is equal to. Let . The we want is thereforethe last two digits of , or . Since there is clearly anexcess of factors of 2, we know that , so it remains tofind .If we divide by by taking out all the factors of in , we canwrite as where whereevery multiple of 5 is replaced by the number with all its factors of 5 removed. Specifically, every number in the form is replaced by ,and every number in the form is replaced by .The number can be grouped as follows:Using the identity at the beginning of the solution, we can reducetoUsing the fact that (or simply the fact thatif you have your powers of 2 memorized), we can deducethat . Therefore.Finally, combining with the fact that yields.Problem 25Jim starts with a positive integer and creates a sequence of numbers. Each successive number is obtained by subtracting the largest possible integer square less than or equal to the current number until zero is reached. For example, if Jim starts with , then hissequence contains numbers:Let be the smallest number for which J im’s sequence has numbers.What is the units digit of ?SolutionWe can find the answer by working backwards. We begin withon the bottom row, then the goes to the right of the equal'ssign in the row above. We find the smallest value for whichand , which is .We repeat the same procedure except with for the next rowand for the row after that. However, at the fourth row, wesee that solving yields , in which case it would beincorrect since is not the greatest perfect square less than orequal to . So we make it a and solve . We continue onusing this same method where we increase the perfect square until can be made bigger than it. When we repeat this until we have rows,we get:Hence the solution is the last digit of , which is .。
2016年 AMC 10A真题含答案

2016AMC10AProblem1What is the value of?的值是多少?Problem2For what value does?,求x。
Problem3For every dollar Ben spent on bagels,David spent cents less.Ben paid more than David.How much did they spend in the bagel store together?Ben在硬面包上每花费1美元,David就比他少花25美分。
Ben总共比David多花了12.5美元,那么他们在面包店里一起总共花了多少钱?The remainder can be defined for all realnumbers and with by where denotes the greatest integerless than or equal to.What is the value of?对于所有实数x和y定义余数为:,其中,且表示取小于或等于的最大整数,求。
Problem5A rectangular box has integer side lengths in the ratio.Which of the following could be thevolume of the box?一个边长是整数的长方体盒子的三边之比为1:3:4。
下面哪个可能是盒子的体积?Problem6Ximena lists the whole numbers through once.Emilio copies Ximena's numbers,replacing eachoccurrence of the digit by the digit.Ximena adds her numbers and Emilio adds his numbers.Howmuch larger is Ximena's sum than Emilio's?Ximena把1到30的整数列成一列,Emilio拷贝了Ximena的数字,然后把所有的数字2替换成数字1。
2010 AMC10A试题(含答案)
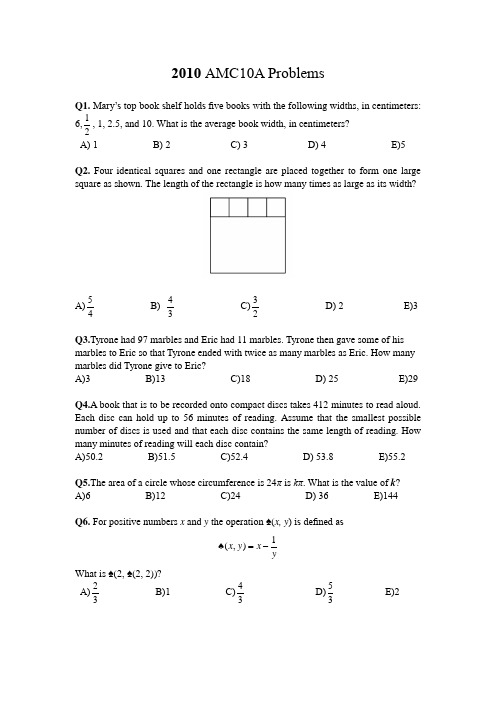
2010 AMC10A ProblemsQ1. Mary’s top book shelf holds five books with the follo wing widths, in centimeters:6,12, 1, 2.5, and 10. What is the average book width, in centimeters?A) 1 B) 2 C) 3 D) 4 E)5Q2. Four identical squares and one rectangle are placed together to form one large square as shown. The length of the rectangle is how many times as large as its width?A)54B)43C)32D) 2 E)3Q3.Tyrone had 97 marbles and Eric had 11 marbles. Tyrone then gave some of his marbles to Eric so that Tyrone ended with twice as many marbles as Eric. How many marbles did Tyrone give to Eric?A)3 B)13 C)18 D) 25 E)29 Q4.A book that is to be recorded onto compact discs takes 412 minutes to read aloud. Each disc can hold up to 56 minutes of reading. Assume that the smallest possible number of discs is used and that each disc contains the same length of reading. How many minutes of reading will each disc contain?A)50.2 B)51.5 C)52.4 D) 53.8 E)55.2Q5.The area of a circle whose circumference is 24π is kπ. What is the value of k?A)6 B)12 C)24 D) 36 E)144Q6. For positive numbers x and y the operation ♠(x, y) is defined as1(,)x y xy♠=-What is ♠(2, ♠(2, 2))?A)23B)1 C)43D)53E)2Q7.Crystal has a running course marked out for her daily run. She starts this run by heading due north for one mile. She then runs northeast for one mile, then southeast for one mile. The last portion of her run takes her on a straight line back to where she started. How far, in miles is this last portion of her run?A)1 B) D)2 E)Q8.Tony works 2 hours a day and is paid $0.50 per hour for each full year of his age. During a six month period Tony worked 50 days and earned $630. How old was Tony at the end of the six month period?A)9 B)11 C)12 D) 13 E)14Q9.A palindrome, such as 83438, is a number that remains the same when its digits are reversed. The numbers x and x + 32 are three -digit and four -digit palindromes, respectively. What is the sum of the digits of x ?A)20 B)21 C)22 D) 23 E)24Q11.The length of the interval of solutions of the inequality 23a x b ≤+≤is 10.What is b a -?A)6 B)10 C)15 D) 20 E)30Q12.Logan is constructing a scaled model of his town. The city’s water tower stands 40 meters high, and the top portion is a sphere that holds 100,000 liters of water. Logan’s miniature water tower holds 0.1 liters. How tall, in meters, should Logan make his tower?A)0.04 B)0.4π C)0.4 D) 4πE)4Q13.Angelina drove at an average rate of 80 kmh and then stopped 20 minutes for gas. After the stop, she drove at an average rate of 100 kmh. Altogether she drove 250 km in a total trip time of 3 hours including the stop. Which equation could be used to solve for the time t in hours that she drove before her stop? A)880100()2503t t +-= B)80250t = C)100250t = D)90250t = E)880()1002503t t -+=Q14.Triangle ABC has 2AB AC =⋅. Let D and E be on AB and BC , respectively, such that ∠BAE = ∠ACD . Let F be the intersection of segments AE and CD , and suppose that △CFE is equilateral. What is ∠ACB ?A)60° B)75° C)90° D) 105° E)120°Q15.In a magical swamp there are two species of talking amphibians: toads, whose statements are always true, and frogs, whose statements are always false. Four amphibians, Brian, Chris, LeRoy, and Mike live together in this swamp, and they make the following statements.Brian: “Mike and I are different species.”Chris: “LeRoy is a frog.”LeRoy: “Chris is a frog.”Mike: “Of the four of us, at least two are toads.”How many of these amphibians are frogs?A)0 B)1 C)2 D) 3 E)4Q16.Nondegenerate △ABC has integer side lengths, BD is an angle bisector, AD = 3, and DC = 8. What is the smallest possible value of the perimeter?A)30 B)33 C)35 D) 36 E)37 Q17.A solid cube has side length 3 inches. A 2-inch by 2-inch square hole is cut into the center of each face. The edges of each cut are parallel to the edges of the cube, and each hole goes all the way through the cube. What is the volume, in cubic inches, of the remaining solid?A)7 B)8 C)10 D) 12 E)15Q18.Bernardo randomly picks 3 distinct numbers from the set {1, 2, 3, 4, 5, 6, 7, 8, 9} and arranges them in descending order to form a 3-digit number. Silvia randomly picks 3 distinct numbers from the set {1, 2, 3, 4, 5, 6, 7, 8} and also arranges them in descending order to form a 3-digit number. What is the probability that Bernardo’s number is larger than Silvia’s number?A)4772B)3756C)23D)4972E)3956Q19.Equiangular hexagon ABCDEF has side lengths AB = CD = EF = 1 and BC = DE = F A = r. The area of △ACE is 70% of the area of the hexagon. What is the sum of all possible values of r?A)3B)103C)4 D)174E) 6Q20.A fly trapped inside a cubical box with side length 1 meter decides to relieve its boredom by visiting each corner of the box. It will begin and end in the same corner and visit each of the other corners exactly once. To get from a corner to any other corner, it will either fly or crawl in a straight line. What is the maximum possible length, in meters, of its path?A)4+B)2+C)2+D)E)Q21.The polynomial 322010-+-has three positive integer roots. What isx ax bxthe smallest possible value of a?A)78 B)88 C)98 D)108 E)118Q22.Eight points are chosen on a circle, and chords are drawn connecting every pair of points. No three chords intersect in a single point inside the circle. How many triangles with all three vertices in the interior of the circle are created?A) 28 B) 56 C) 70 D) 84 E) 140Q23.Each of 2010 boxes in a line contains a single red marble, and for 1 ≤k≤2010, the box in the k th position also contains k white marbles. Isabella begins at the first box and successively draws a single marble at random from each box, in order. She stops when she first draws a red marble. Let P(n) be the probability that Isabella stops after drawing exactly n marbles. What is the smallest value of n for which1()P n<?2010A) 45 B) 63 C) 64 D) 201 E) 1005Q24.The number obtained from the last two nonzero digits of 90! is equal to n. What is n?A) 12 B) 32 C) 48 D) 52 E) 68Q25.Jim starts with a positive integer n and creates a sequence of numbers. Each successive number is obtained by subtracting the largest possible integer square less than or equal to the current number until zero is reached. For example, if Jim starts with n = 55, then his sequence contains 5 numbers:Let N be the smallest number for which Jim’s sequence has 8 numbers. What is the units digit of N?A) 1 B) 3 C) 5 D) 7 E) 9AMC10A 2010中文解析Q1. Mary’s top book shelf holds five books with the follo wing widths, in centimeters:6,12, 1, 2.5, and 10. What is the average book width, in centimeters?A) 1 B) 2 C) 3 D) 4 E)5翻译:Mary最上面的书架上有五本书,宽度分别为: 6厘米、1厘米、2.5厘米和10厘米。
