《逻辑与计算机设计基础》(原书第五版)课后习题答案-chapter11_solutions-5th
《逻辑与计算机设计基础》(原书第五版)课后习题答案-chapter04_solutions-5th
X Y
DA
Clock C
D
BX
Z
Clock C
2
Present state
AB
00 00 00 00 01 01 01 01 10 10 10 10 11 11 11 11
Inputs
XY
00 01 10 11 00 01 10 11 00 01 10 11 00 01 10 11
Next state
Input
1 0 011 0 1
1
1
1
0
Output
0 1 000 1 0
0
0
0
1
Next State 01 00 00 01 11 00 01 11 10 10 00
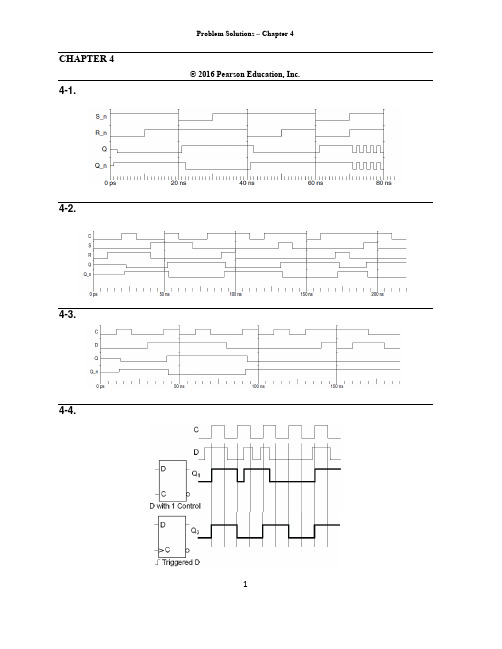
4-10.
00/0 11/1
01/0 10/1 11/0 0
00/0 01/1 10/0 11/1 01/0
00/1 1
01/1, 10/0
0
0
0 00
0 0
001
0
11
1 10
1 10
1 11
11
0
1
10
0
1
1 0
1
01
0
00
1
11
0
10
1
1
Nextt state state AB
A 0B 0
1
0
0 00 1
1 00 0
0 11 0
0 1
1
0 0
1
1 11 1
1 01 1
01
DA
B
1
A1 1
1
X
DDAA = AAXX+BBXX
计算机网络第五版课后习题问题详解
计算机网络第五版答案第一章概述1-01 计算机网络向用户可以提供那些服务？答：连通性和共享1-02 简述分组交换的要点。
答：（1）报文分组，加首部（2）经路由器储存转发（3）在目的地合并1-03 试从多个方面比较电路交换、报文交换和分组交换的主要优缺点。
答：（1）电路交换：端对端通信质量因约定了通信资源获得可靠保障，对连续传送大量数据效率高。
（2）报文交换：无须预约传输带宽，动态逐段利用传输带宽对突发式数据通信效率高，通信迅速。
（3）分组交换：具有报文交换之高效、迅速的要点，且各分组小，路由灵活，网络生存性能好。
1-04 为什么说因特网是自印刷术以来人类通信方面最大的变革？答：融合其他通信网络，在信息化过程中起核心作用，提供最好的连通性和信息共享，第一次提供了各种媒体形式的实时交互能力。
1-05 因特网的发展大致分为哪几个阶段？请指出这几个阶段的主要特点。
答：从单个网络APPANET向互联网发展；TCP/IP协议的初步成型建成三级结构的Internet；分为主干网、地区网和校园网；形成多层次ISP结构的Internet；ISP首次出现。
1-06 简述因特网标准制定的几个阶段？答：（1）因特网草案(Internet Draft) ——在这个阶段还不是 RFC 文档。
（2）建议标准(Proposed Standard) ——从这个阶段开始就成为 RFC 文档。
（3）草案标准(Draft Standard)（4）因特网标准(Internet Standard)1-07小写和大写开头的英文名字 internet 和Internet在意思上有何重要区别？答：（1） internet（互联网或互连网）：通用名词，它泛指由多个计算机网络互连而成的网络。
；协议无特指（2）Internet（因特网）：专用名词，特指采用 TCP/IP 协议的互联网络区别：后者实际上是前者的双向应用1-08 计算机网络都有哪些类别？各种类别的网络都有哪些特点？答：按范围：（1）广域网WAN：远程、高速、是Internet的核心网。
逻辑与计算机设计基础第五版
逻辑与计算机设计基础第五版逻辑与计算机设计是现代计算机科学的基础课程之一，它包含了逻辑学和计算机设计的基本原理与方法。
本文将介绍《逻辑与计算机设计基础第五版》这本教材的内容和意义。
逻辑学是研究思维规律和推理方法的学科，它对于计算机科学的发展具有重要的理论基础作用。
《逻辑与计算机设计基础第五版》从逻辑学的基本概念和原理入手，介绍了命题逻辑、谓词逻辑、命题演算与谓词演算等内容。
通过学习这些内容，读者可以了解逻辑学的基本知识，掌握逻辑推理的方法和技巧，从而提高自己的思维能力和解决问题的能力。
计算机设计是指设计和构造计算机硬件和软件的过程。
《逻辑与计算机设计基础第五版》以计算机硬件设计为主线，介绍了数字电路的基本原理和设计方法。
它从二进制系统、布尔代数和逻辑门开始，逐步讲解了组合逻辑电路和时序逻辑电路的设计与分析。
通过学习这些内容，读者可以了解计算机硬件的工作原理，掌握数字电路的设计和分析方法，从而能够设计和构造简单的计算机硬件系统。
除了逻辑学和计算机设计的基本原理和方法，本书还介绍了一些与计算机科学密切相关的内容，如数据表示与运算、存储器和输入输出设备、指令系统和汇编语言、计算机组成与体系结构等。
这些内容为读者进一步学习和研究计算机科学的相关领域奠定了坚实的基础。
《逻辑与计算机设计基础第五版》在编写过程中遵循了逻辑与计算机设计的基本原理，确保了内容的准确性和严谨性。
本书采用了简洁明了的语言，避免了晦涩难懂的数学公式和计算公式，使读者更好地理解和掌握相关知识。
此外，本书还配有丰富的例题和习题，供读者练习和巩固所学知识。
《逻辑与计算机设计基础第五版》是一本理论与实践相结合的教材，它既介绍了逻辑学和计算机设计的基本原理和方法，又通过实例和习题的方式帮助读者掌握相关技能。
对于计算机科学专业的学生和从事计算机相关工作的人员来说，它是一本必不可少的参考书。
阅读本书可以帮助读者建立起扎实的逻辑思维和计算机设计的基础，为进一步学习和研究计算机科学奠定坚实的基础。
最新最全标准C语言程序设计第五版课后基础练习题复习答案完整版.doc
课后练习题答案Chapter 11.1×√×√√×√××√1.2b c1.3ad1.4semicolon printf math.h \nChapter 22.1 ×√××√√×√××√×2.2 typedef 255 external constChapter 33.1 ×√××√√×××√√√3.2 integer modula 6 logical sizeof paratheses typeconversion precedence3.3 F F T F F F3.4 F T T T F3.5 (b) (c)3.6 0 -2 7 10.25 false 3 3 13.10 0 1 1 1 13.11d}1003.121101113.13 13.14 2003.15 x<=y3.16 TRUE3.192143.20-4040Chapter 44.1 ×√√√√√××√√√×4.2 %hd %x ctype.h %l %*d [^] blank 6 - %e4.4 (a) scanf(“%d %c %d”, &a, &b, &c); (b)scanf(“%d %f %s”, &a, &b, &c);(c) scanf(“%d-%d-%d”, &a, &b, &c); (d) scanf(“%d %s %d”, &a, &b, &c);4.5 (a)10x1.230000 (b)1234x 1.23 (c)1234 456.000000 (d) “123.40 ”(e) 1020 1222(乱码)4.7 （a）1988 x (b)乱码（c）120 乱码（d）乱码 x4.8 （a）1275 -235.740000(b) 1275-235.740000(c) 0 0.000000(d) 1275xxxx-235.74(e)Cambridge(f)1275 Cambridge4.10 1988 无无Chapter 55.1 √×√××××××√5.2 && switch break if-else x=y5.4 （a）x = 2; y = 0; (b) x = 1; y = 0;5.5 (a) if (grade <= 59)if (grade >= 50)second = second + 1;(b) if (number > 100) printf(“out of range”);else if (number < 0) printf(“out of range”);else sum = sum + number;(c)if (T > 200) printf(“admitted”);else if (M > 60){if (M > 60) printf(“admitted”);}else printf(“not admitted”);5.6 F T F T5.8 (a) x > 10 (b) (x != 10)||(y ! = 5) || (z >= 0)(c) (x + y != z) || (z > 5) (d) (x > 5) || (y != 10) || (z >= 5)5.9 (a) x = 5; y = 10; z = 1 (b) x = 5; y = 10; z = 1(c) x = 5; y = 0; z =0 (d) 无变化5.10 （a） x= 0; y = 2; z = 0; (b) x = 1; y = 2; z = 0;5.12 85.13 Delhi Bangalore END5.1424485.15 0 0 25.16 255.17 Number is negative5.18 ABC5.19 105.20 无输出Chapter 66.1 √√√××√×√√×6.2 n continue infinite indefinite-repetition-loop counter-variable 6.9 （a） 43210 (b)4321 (c)55555…55555(d)10 86.11 （a）无数次 x = 10; x = 5; x = 10; x = 5; x = 10;…(b)5次 m = 3, m = 5, m = 7, m = 9, m = 11(c)无数次, i 恒为0(d)4次 m = 11 n = 9; m = 12 n = 11; m = 13, n = 13; m = 14, n = 15;6.13（a）for (n = 1; n <= 32; n = n * 2) printf("%d ", n);（b）for (n = 1; n <= 243; n = n * 3) printf("%d ", n);（c）for (n = -4; n <= 4; n = n + 2) printf("%d ", n);(d)sum = 0;for (i = 2; i <= 16; i = sum){sum = sum + i;n = n - i;printf("%d %d %d \n", i, sum, n);}6.16 100 90 80 70 60 50 40 30 20 10 06.17 m = 20时陷入死循环，程序无输出6.18 16.19 16.20 死循环，延长时间用Chapter 77.1 √×√××√√√√××√7.2 index/subscript run-time dynamic multi-dimensional sorting7.14 157.15 HLOWRDChapter 88.1 ×√√√×√××√×××√×√8.2 %[ a-z,A-Z] strcpy 3 stdlib gets strlen strstr strcmp s1-s2 puts8.6 (a) The sky is the limit.(b) The sky is(c) T(d) The sky is the limit(e)8410410132115107121321051153211610410132108105109105116(f)输出1 2 3 4 5 6 7 8 9…21这些ASCII码对应的字符，无显示符号(g)%(h)U8.7 (d)8.8 78.9 (a)he(b)heorshe(c)5 78.11 puneChapter 99.1 √×××√×××√√√×××√×√√××9.2 actual-parameter local-variable int data-type variable-name scope recursive static type auto9.8 abceg9.9 abcdeg9.12 (a) 5 (b) 4 (c)3 (d)09.13 (a) 5 4 4 0 (b) 5.0 4.0 3.0 0.679.14 题目错，设z = 5；结果为：200 10009.15 1009.16 x = 3; y = 0Chapter 1010.1 ×√√√√×√√×√√√××√10.2 typedef union tag_name pointer memberChapter 1111.1 √×√√√√×√××11.2 address * * 0 null赠送以下资料5以内的加减法口算练习题姓名得分2+2= 3+2= 0+2= 0+1= 3-1= 2+1= 2+3= 1+4= 1-0= 2+2= 0-0= 3+2= 3-1= 2-1= 2+2= 4-3= 3+2= 2+2= 5-4= 3-1= 0+4= 4+1= 1+0= 0+0= 5-2= 3+2= 4-3= 2+2= 1+2= 5-2= 1+2= 2-0= 1+2= 4+1= 2+2= 2-0= 1-1= 2+2= 2-0= 1-0= 3+0= 4-2= 2-0= 3-0= 0+1= 4-1= 4+1= 3-1= 4-3= 2-0= 3-1= 1+3= 2-0= 1-0= 3+0= 1+2= 5-4= 1-1= 2+0= 3-1= 2-0= 0+1= 1+4= 2+3= 2-1= 3-1= 0+0= 2+2= 2-0= 3-1= 1+0= 1+2= 2+2= 1+3= 5-4= 0+2= 2+3= 1-0=5-2= 3-3= 1+2= 2-1= 3-3= 3-0= 4-4= 5-4= 2+2= 3-2= 3-0= 3+1= 2+1= 3-3= 4-4= 2-0= 4-0= 3-2= 3-0= 4-3= 5-2= 5+0=家长签名。
《逻辑与计算机设计基础》(原书第五版)课后习题答案-chapter01_solutions-5th
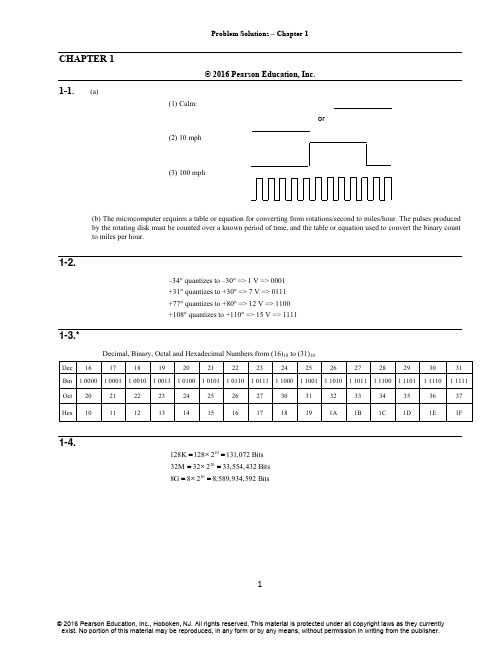
1CHAPTER 1© 2016 Pearson Education, Inc.1-1.(a)(1) Calm:(2) 10 mph(3) 100 mphor(b) The microcomputer requires a table or equation for converting from rotations/second to miles/hour. The pulses produced by the rotating disk must be counted over a known period of time, and the table or equation used to convert the binary count to miles per hour.1-2.–34° quantizes to –30° => 1 V => 0001+31° quantizes to +30° => 7 V => 0111 +77° quantizes to +80° => 12 V => 1100 +108° quantizes to +110° => 15 V => 11111-3.*Decimal, Binary, Octal and Hexadecimal Numbers from (16)to (31)1-4.1020301281282131,0723232233,554,4328828,589,934,592=⨯==⨯==⨯=K Bits M Bits G Bits21-5.220 = (1,000,00010 + d ) where d = 48,576 1Tb = 240 = (220)2 = (1,000,000 + d )2= (1,000,000)2 + 2(1,000,000) d + d 2 = 1,000,000,000,000 + 97,152,000,000 + 2,359,627,776 = 1,099,511,627,7761-6.112511212047252133,554,4311 Bits 1 Bits ⇒-=⇒-=1-7.*63202641013275321142(1001101)222277(1010011.101)22222283.625(10101110.1001)2222222174.5625----=+++==+++++==++++++=1-8.1 1 0 0 0 0 0 0 0 0 0 1 0 11-9.*1-10.*a)0.45 × 8 = 3.6 =>0.60 × 8 = 4.8 =>0.80 × 8 = 6.4 =>0.20 × 8 = 3.2 =>10= (16612.3463)8b) (1938.257)10 = (792.41CB)16c) (175.175)10 = (10101111.001011)21-11.*a) (673.6)8= (110 111 011.110)2= (1BB.C)16b) (E7C.B)16= (1110 0111 1100.1011)2= (7174.54)8c) (310.2)4= (11 01 00.10)2= (64.4)81-12.a) 1010 b) 0110 c) 1111001×1100 ×1001 ×0111010000 0110 11110010000 0000 0000001010 0000 11110011010 0110 11110011111000 0110110 11110010000000110110110101341-13.+1000110110101101010000000010000110001101011-----Quotient = 10001R emainder = 1 1-14.(a) 6 × 123 + 8 × 122 + 7 × 121 + 4 = 11608 (b)12 1-15.a)0 1 2 3 4 5 6 7 8 9 A BCDEFGHIJb) 20c) 21012010(.)1120122018201620(4658.8)BCI G -=⨯+⨯+⨯+⨯=1-16.*a) (BEE)r = (2699)1021021114142699111426850r r r r r ⨯+⨯+⨯=⨯+⨯-=By the quadratic equation: r = 15 or ≈ –16.27 ANSWER: r = 15 b) (365)r = (194)102102365194361890r r r r r ⨯+⨯+⨯=⨯+⨯-=By the quadratic equation: r = – 9 or 7 ANSWER: r = 751-17.Errata: The text has an error: 1480 should be 1460. This will be corrected in future printings.Noting the order of operations, first add (34)r and (24)r101010(34)34(24)24(34)(24)58r r r r r r r r r r =⨯+⨯=⨯+⨯+=⨯+⨯Now, multiply the result by (21)r101021(21)(58)10218⨯+⨯⨯⨯+⨯=⨯+⨯+r r r r r r Next, set the result equal to (1480)r and reorganize.21321321010218146161580⨯+⨯+=⨯+⨯+⨯⨯-⨯-⨯-⨯=r r r r r r r r r Finally, find the roots of this cubic polynomial. Solutions are: r = 8, – 1, – 1ANSWER: The chicken has 4 toes on each foot (half of 8).1-18.*a) (0100 1000 0110 0111)BCD = (4867)10= (1001100000011)2 b) (0011 0111 1000.0111 0101)BCD= (378.75)10=(101111010.11)21-19.*(694)10 = (0110 1001 0100)BCD (835)10=(1000 0011 0101)BCD1001 0100 +0011 +0101 1100 1001 +0110 +00001 001010011-20.*(a)101 1000111 1000Move R 011 1100 0 100 column > 0111Subtract 3 −0011011 1001 0Subtract 3 −001101 1001Move R 0 1100 110 100 column > 0111Subtract 3 −00110 1001 110Move R 0100 1110Move R 010 01110Move R 01 001110Move R 0 1001110 Leftmost 1 in BCD numbershifted out: Finished(b) 102101 1000011 1001 0111Move R 001 1100 1011 1 101 and 100 columns > 0111Subtract 3 −0011 -0011001 1001 1000 1Move R 00 1100 1100 01 101 and 100 columns > 0111Subtract 3 −0011 −001100 1001 1001 01Move R 0 0100 1100 101 100 column > 0111Subtract 3 −00110 0100 1001Move R 0010 0100 1101Move R 001 0010 01101Move R 00 1001 001101 100 column > 0111Subtract 3 −001100 0110 001101Move R 0 0011 0001101Move R 0001 10001101Move R 000 110001101 Leftmost 1 in BCDnumber shifted out: Finished 1-21.(a) 10210110011110001st Move L 1 1110002nd Move L 11 110003rd Move L 111 1000 100 column > 100Add 3 00111010 10004th Move L 1 0101 000 100 column > 100Add 3 00111 1000 0005th Move L 11 0000 006th Move L 110 00000 101 column > 100Add 3 00111001 0000 07th Move L 1 0001 00000 Least significant bit in binary number moved in:Finished(b) 103102101100011100101111st Move L 0 11100101112nd Move L 01 1100101113rd Move L 011 100101114th Move L 0111 0010111 100 column > 100Add 3 001161010 00101115th Move L 1 0100 0101116th Move L 10 1000 10111 100 column > 100Add 3 001110 1011 101117th Move L 101 0111 0111 101 & 100 columns > 100Add 3 0011 00111000 1010 01118th Move L 1 0001 0100 1119th Move L 10 0010 1001 11 100 column > 100Add 3 001110 0010 1100 1110th Move L 100 0101 1001 1 101 &100 columns > 100Add 3 0011 0011100 1000 1100 111th Move L 1001 0001 1001 Least significant bit in binary number moved in: Finished1-22.From Table 1-5, complementing the bit B6 will switch an uppercase letter to a lower case letter and vice versa.1-23.a) The name used is Brent M. Ledvina. An alternative answer: use both upper and lower case letters.0100 0010 B 0101 0010 R 0100 0101 E0100 1110 N 0101 0100 T 0010 0000 (SP)0100 1101 M 0010 1110 . 0010 0000 (SP)0100 1100 L 0100 0101 E 0100 0100 D0101 0110 V 0100 1001 I 0100 1110 N0100 0001 Ab) 0100 0010 1101 0010 1100 01010100 1110 1101 0100 1010 00000100 1101 0010 1110 1010 00001100 1100 1100 0101 0100 01000101 0110 1100 1001 0100 11100100 00011-24.1000111 G1101111 o01000001000011 C1100001 a1110010 r1100100 d1101001 i1101110 n1100001 a (Errata: This number appears as 110001, which would be “1”)1101100 l1110011 s0100001 !781-25.*a) (11111111)2b) (0010 0101 0101)BCD c) 011 0010 011 0101 011 0101ASCIId)0011 00101011 01011011 0101ASCII with Odd Parity1-26.a) U+0040 = 01000000b) U+00A2 = 11000010 10100010c) U+20AC = 11100010 10000010 10101100d)U+1F6B2 = 11110000 10011111 10011010 101100101-27.Binary Numbers from (32)to (47) with Odd and Even Parity1-28.Gray Code for Hexadecimal Digits1-29.(a) Wind Direction Gray CodeDirectionCode WordN 000 S 110 E 011 W 101 NW 100 NE 001 SW 111 SE0109(b) Wind Direction Gray Code (directions in adjacent order)DirectionCode WordN 000 NE 001 E 011 SE 010 S 110 SW 111 W 101 NW100As the wind direction changes, the codes change in the order of the rows of this table, as suming that the bottom row is “next to” the top row. From the table, the codes that result due to a wind direction change always change in a single bit.1-30.+The percentage of power consumed by the Gray code counter compared to a binary code counter equals:Number of bit changes using Gray code Number of bit changes using binary codeAs shown in Table 1-6, and by definition, the number of bit changes per cycle of an n-bit Gray code counter is 1 per count = 2n .Number of bit changes using Gray code = 2nFor a binary counter, notice that the least significant bit changes on every increment. The second least significant bit changes on every other increment. The third digit changes on every fourth increment of the counter, and so on. As shown in Table 1-6, the most significant digit changes twice per cycle of the binary counter.Number of bit changes using binary code 11222n n -+++(1)110221(21)122nni i n n i i ++==⎡⎤==-=--=-⎢⎥⎣⎦∑∑ % Power (1)210022n n +=⨯-。
数字逻辑(科学出版社第五版)课后习题答案综述
第一章开关理论基础1.将下列十进制数化为二进制数和八进制数十进制二进制八进制49 110001 6153 110101 65127 1111111 177635 1001111011 11737.493 111.1111 7.7479.43 10011001.0110111 231.3342.将下列二进制数转换成十进制数和八进制数二进制十进制八进制1010 10 12111101 61 751011100 92 1340.10011 0.59375 0.46101111 47 5701101 13 153.将下列十进制数转换成8421BCD码1997=0001 1001 1001 011165.312=0110 0101.0011 0001 00103.1416=0011.0001 0100 0001 01100.9475=0.1001 0100 0111 01014.列出真值表，写出X的真值表达式A B C X0 0 0 00 0 1 00 1 0 00 1 1 11 0 0 01 0 1 11 1 0 11 1 1 1 X=A BC+A B C+AB C+ABC5.求下列函数的值当A,B,C为0,1,0时：A B+BC=1(A+B+C)(A+B+C)=1(A B+A C)B=1当A,B,C为1,1,0时：A B+BC=0(A+B+C)(A+B+C)=1(A B+A C)B=1当A,B,C为1,0,1时：A B+BC=0(A+B+C)(A+B+C)=1(A B+A C)B=06.用真值表证明下列恒等式(1) (A⊕B)⊕C=A⊕(B⊕C)A B C (A⊕B)⊕C A⊕(B⊕C)0 0 0 0 00 0 1 1 10 1 0 1 10 1 1 0 01 0 0 1 11 0 1 0 01 1 0 0 01 1 1 1 1所以由真值表得证。
（2）A⊕B⊕C=A⊕B⊕CA B C A⊕B⊕C A⊕B⊕C0 0 0 1 10 0 1 0 0 0 1 0 0 0 0 1 1 1 1 1 0 0 0 0 1 0 1 1 1 1 1 0 1 1 1 1 1 0 07.证明下列等式（1） A+A B=A+B证明：左边= A+A B =A(B+B )+A B =AB+A B +A B =AB+A B +AB+A B =A+B =右边(2) ABC+A B C+AB C =AB+AC证明：左边= ABC+A B C+AB C = ABC+A B C+AB C +ABC =AC(B+B )+AB(C+C ) =AB+AC =右边（3） E D C CD A C B A A )(++++=A+CD+E 证明：左边=E D C CD A C B A A )(++++ =A+CD+A B C +CD E =A+CD+CD E =A+CD+E =右边（4） C B A C B A B A ++=C B C A B A ++证明：左边=C B A C B A B A ++=C B A C AB C B A B A +++)( =C B C A B A ++=右边8.用布尔代数化简下列各逻辑函数表达式9.将下列函数展开为最小项表达式 (1) F(A,B,C) = Σ(1,4,5,6,7)(2) F(A,B,C,D) = Σ(4,5,6,7,9,12,14) 10.用卡诺图化简下列各式（1）C AB C B BC A AC F +++=化简得F=C(2)C B A D A B A D C AB CD B A F++++=F=D A B A +（3） F(A,B,C,D)=∑m (0,1,2,5,6,7,8,9,13,14)化简得F=D BC D C A BC A C B D C ++++(4) F(A,B,C,D)=∑m (0,13,14,15)+∑ϕ(1,2,3,9,10,11)化简得F=AC AD B A ++11.利用与非门实现下列函数，并画出逻辑图。
第五版《大学计算机基础》思考题（含部分答案）
第五版《大学计算机基础》思考题（含部分答案）大学计算机基础思考题第一章计算机与信息社会1. 计算机的发展经历了哪几个阶段？各阶段的主要特征是什么？2. 按综合性能指标，计算机一般分为哪几类？3. 信息与数据的区别是什么？第二章计算机系统1. 简述计算机执行指令的过程。
1）取指令2）分析指令3）执行指令一条指令执行完成，程序计数器加1，然后回到1)。
2. 存储器的容量单位有哪些？若内存的大小为512MB，则它有多少个字节？容量单位有KB、MB、GB、TB 。
512MB等于512*220 个字节。
3. 指令和程序有什么区别？4. 简述机器语言、汇编语言、高级语言各自的特点。
5．计算机的硬件系统由哪几部分构成？主机主要包括了哪些部件？6．衡量CPU性能的主要技术指标有哪些？①CPU字长，CPU内部各寄存器之间一次能够传递的数据位，即在单位时间内能一次处理的二进制的位数。
②位宽，CPU通过外部总线与外部设备之间一次能够传递的数据位。
③x位CPU，通常用CPU的字长和位宽来称呼CPU。
④CPU外频，即CPU总线频率，是由主板为CPU提供的基准时钟频率。
⑤CPU主频，也叫工作频率，是CPU内核电路的实际运行频率。
来描述，精度越高表示生产工艺越先进。
⑥CPU的生产工艺技术，通常用m7. 内存和外存有什么区别？若内存大小为1G，则它有多少个字节？内存与外存有很多不同之处：①内存可以直接被CPU访问，外存不能直接被CPU访问；②内存信息是暂时的，外存信息可以永久保存的；③相对来说，内存容量小价格高，外存容量大价格低；④内存速度快，外存速度慢。
（1分）301G＝1024M＝1024×1024K＝1024×1024×1024B＝2B8. 系统主板主要包括了哪些部件？1）系统主板主要包括了控制芯片组、CPU插座、BIOS芯片、内存条插槽；2）系统主板也集成了软盘接口、硬盘接口、并行接口、串行接口、USB接口、AGP 总线扩展槽、PCI局部总线扩展槽、键盘和鼠标接口、多媒体和通信设备接口以及一些连接其他部件的接口等。
大学计算机第5版习题参考答案
《大学计算机》习题解答（2017.3）说明：1、部分思考题并无标准答案，需要学生在教材、校园网、因特网中查找相关资料；2、思考题能自圆其说者为“中”；言之有理者为“良”；举例说明者为“优”；3、思考题、简答题均以短小要点形式答题，不论有多少要点，答对3个均视为全部正确；各章习题参考答案第1章计算与计算思维1-1 简要说明计算机发展的三个历史阶段。
答：（1）古代计算机工具（2）中世纪计算机（3）现代计算机1-2 简要说明九九乘法口诀算法有哪些优点。
答：（1）建立了一套完整的算法规则；（2）具有临时存储功能，能连续运算；（3）出现了五进制；（4）制作简单，携带方便。
1-3 简要说明计算机集群系统有哪些特点。
答：（1）将多台计算机通过网络组成一个机群；（2）以单一系统模式管理；（3）并行计算；（4）提供高性能不停机服务；（5）系统计算能力非常高；（6）具有很好的容错功能。
1-4 简要说明各种类型计算机的主要特点。
答：（1）大型机计算机性能高。
（2）微机有海量应用软件，优秀的兼容能力，低价高性能。
（3）嵌入式计算机要求可靠性好。
1-5 简要说明图灵机的重要意义。
答：（1）图灵机证明了通用计算理论；（2）图灵机引入了读写、算法、程序、人工智能等概念；（3）复杂的理论问题可以转化为图灵机进行分析。
（4）图灵机可以分析什么是可计算的，什么是不可计算的。
1-6 简要说明冯诺依曼“存储程序”思想的重要性。
答：（1）为程序控制计算机提供了理论基础；（2）程序和数据的统一；（3）实现了程序控制计算机；（4）提高运算效率；（5）为程序员职业化提供了理论基础。
1-7 简要说明什么是计算思维。
答：周以真认为：计算思维是运用计算机科学的基础概念去求解问题、设计系统和理解人类行为，它涵盖了计算机科学的一系列思维活动。
1-8 举例说明计算思维的应用案例。
答：（1）复杂性分析：如战争分析、经济分析、算法分析等。
（2）抽象：如数据类型、数学公式等。
普通逻辑第5版考研课后题和答案
普通逻辑第5版考研课后题和答案普通逻辑第5版考研课后题和答案普通逻辑编写组《普通逻辑》（第5版）课后习题详解目录第1章引论第2章复合命题及其推理第3章命题的判定与自然推理第4章简单命题的基本要素——概念第5章性质命题及其推理第6章关系命题及其推理第7章谓词自然推理第8章模态命题及其推理第9章普通逻辑的基本规律第10章归纳推理第11章类比推理第12章假说第13章论证与谬误试看部分内容引论思考题1普通逻辑的对象是什么？答：从宏观上看，普通逻辑是研究思维的科学。
辩证唯物主义认为对客观事物的认识需经过从感性认识上升到理性认识，理性认识阶段即思维的阶段。
思维是人脑对客观世界的间接的、概括的反映，有抽象概括性和间接性两个最基本的特征。
普通逻辑主要研究思维的逻辑形式和逻辑规律。
2逻辑与语言有何关系？答：逻辑形式是指不同思维内容所具有的共同结构；语言形式是指某种语言的具体表达方式。
两者的关系如下：（1）联系逻辑与语言之间的联系是十分密切的，具体地表现为思维的逻辑形式与语言形式的紧密联系：概念、命题和推理的存在与表达要借助于语词和语句，否则就无法存在和表达。
（2）区别①同样一种逻辑形式可以用不同的语言形式来表达。
②同样一个语言形式在不同的场合能够表达不同的逻辑形式。
3学习普通逻辑有什么意义？答：学习普通逻辑的意义在于：（1）根本意义通过对普通逻辑的学习与应用，培养、训练和提高人们的逻辑思维能力，开发人们的智能，进而提高整个中华民族的科学文化水平与综合素质，促进知识创新、科技进步，促进和谐社会的构建。
（2）具体意义①学习普通逻辑能够给人们探求新知识提供必要的逻辑工具。
②学习普通逻辑能帮助人们准确、严密地表述和论证思想。
③学习普通逻辑对于人们反驳谬误、揭露诡辩十分必要。
④学习普通逻辑有利于人们学习、理解和掌握其他各门具体科学知识。
4怎样学好普通逻辑？答：学好普通逻辑需要：（1）提高学习自觉性和积极性学习普通逻辑可以把自发地运用逻辑变为自觉地运用逻辑，提高逻辑表达能力。
计算机网络第五版课后答案完整版
第一章概述1-2 试简述分组交换的特点答：分组交换实质上是在“存储——转发”基础上发展起来的。
它兼有电路交换和报文交换的优点。
分组交换在线路上采用动态复用技术传送按一定长度分割为许多小段的数据——分组。
每个分组标识后，在一条物理线路上采用动态复用的技术，同时传送多个数据分组。
把来自用户发端的数据暂存在交换机的存储器内，接着在网内转发。
到达接收端，再去掉分组头将各数据字段按顺序重新装配成完整的报文。
分组交换比电路交换的电路利用率高，比报文交换的传输时延小，交互性好。
1-5 因特网的发展大致分为哪几个阶段？请指出这几个阶段最主要的特点。
答：第一阶段是从单个网络ARPANRET 向互联网发展的过程。
最初的分组交换网ARPANET 只是一个单个的分组交换网，所有要连接在ARPANET 上的主机都直接与就近的结点交换机相连。
而后发展为所有使用TCP/IP 协议的计算机都能利用互联网相互通信。
第二阶段是1985-1993 年，特点是建成了三级结构的因特网第三阶段是1993 年至今，特点是逐渐形成了多层次ISP 结构的因特网。
1-24 试述五层协议的网络体系结构的要点，包括各层的主要功能。
答：所谓五层协议的网络体系结构是为便于学习计算机网络原理而采用的综合了OSI 七层模型和TCP/IP 的四层模型而得到的五层模型。
五层协议的体系结构见图1-1 所示。
应用层运输层网络层数据链路层物理层各层的主要功能：（1）应用层应用层确定进程之间通信的性质以满足用户的需要。
应用层不仅要提供应用进程所需要的信息交换和远地操作，而且还要作为互相作用的应用进程的用户代理（user agent),来完成一些为进行语义上有意义的信息交换所必须的功能。
(2）运输层任务是负责主机中两个进程间的通信。
因特网的运输层可使用两种不同的协议。
即面向连接的传输控制协议TCP 和无连接的用户数据报协议UDP。
面向连接的服务能够提供可靠的交付。
无连接服务则不能提供可靠的交付。
计算机应用基础(第五版)习题答案教材课后题
第1章计算机基础知识习题答案1.计算机系统由哪两部分组成？答案：计算机系统由硬件部分和软件部分组成。
2.什么是输入设备？什么是输出设备，说出另外几种输入、输出设备的名称。
答案：输入设备是完成信息输入到计算机的设备，输出设备则是完成把信息从计算机中输出的设备。
输入设备除了鼠标、键盘、扫描仪外，还有摄像头、话筒、光驱等。
输出设备除了显示器、打印机外，还有音响、绘图仪等。
3.什么是系统软件？什么是应用软件？答案：系统软件是使用计算机必不可少的，负责管理计算机系统中各种硬件，使得它们可以协调工作、并为用户使用计算机提供工作平台、维护计算机正常工作的一些软件。
系统软件包括操作系统、各种语言的编译程序、汇编程序、计算机的故障诊断程序、一些外设的驱动程序、数据库管理程序以及网络管理程序等。
应用软件是为了特定的用途或某种应用目的而编写的程序，例如字处理软件、表处理软件、银行管理软件、财务管理软件、教育软件等，都属于应用软件。
4.位、字节、字长的概念是什么？答案：位：又称bit，是计算机中最小的存储单位，只有0或者1两种状态。
字节：是存储器的基本计算单位，一个字节由8个bit组成。
字长：字是由若干个字节组成的一个存储单元，存放一条指令或一个数据，组成存储单元即字的位数称为字长。
5.1个G 是多少个字节？答案：1个G是1024M个字节，1M是1024K个字节，1K是1024个字节，因此1个G 是1024×1024×1024个字节。
6.将下面的十进制数转换为二进制数：（1）23 （2）376 （3）10581 （4）3777答案：（1）2 23 余 12 11 余 12 5 余 12 2 余 02 1 余 1即(23)10 = (10111)2（2）2 376 余 02 188 余 02 94 余 02 47 余 12 23 余 12 11 余12 5 余12 2 余02 1 余1即(376)10 = (101111000)2 （3）2 10581 余 12 5290 余 02 2645 余 12 1322 余 02 661 余 12 330 余02 165 余12 82 余02 41 余12 20 余02 10 余02 5 余12 2 余02 1 余1即(10581)10 = (101)2 （4）2 3777 余 12 1888 余 02 944 余 02 472 余 02 236 余 02 118 余02 59 余12 29 余12 14 余02 7 余12 3 余12 1 余1即(3777)10 27.将下面的二进制数转换为十进制数：答案：（1）(1101)2 =1×23＋1×22＋0×21＋1×20 =(13)10（2）(10011101)2 =1×27＋0×26＋0×25＋1×24＋1×23＋1×22＋0×21＋1×20 =(157)10（3）(11111111)2 =1×27＋1×26＋1×25＋1×24＋1×23＋1×22＋1×21＋1×20 =(255)10（4）()2 =0×215＋1×214＋0×213＋1×212＋1×211＋0×210＋1×29＋0×28＋1×27＋0×26＋1×25＋1×24＋0×23＋0×22＋1×21＋1×20=(23219)108.内存储器和外存储器的主要区别是什么？答案：主要区别是内存储器读写速度很快，外存储器读写速度相对较慢；内存储器数据在断电后将被清除，外存储器中的数据可以永久保存；内存储器的容量相对较小，而外存储器的容量可以很大。
《逻辑与计算机设计基础》(原书第五版)课后习题答案-chapter03_solutions-5th
Z X1 X 2 X3
X1 X2 X3 Z 0000 0011 0101 0110 1001 1010 1100 1111
3-7.+
ABCD 0000 0001 0011 0010 0110 0111 0101 0100 1100 1101 1111 1110 1010 1011 1001 1000
S0 BCD BCD AB ACD ABCD S1 AB AB ACD BCD S2 ABC ABD
A B C DWX Y Z
00000011
00010100
00100101
00110110
01000111
01011000
01101001
01111010
10001011
10011100
b) W = X1(X2 X3 X4 + X5 X9 X13 + X6 X11 X15) + X7(X5 X6 X8 + X3 X11 X15 + X4 X10 X13) + X9 X10 X 11 X12 + X13 X14 X15 X16 + X2 X6 X10 X14 + X4 X8 X12 X16 Gate Inpu11
F =FX=Z X+ZXY+ +XYYZ+ YZ
TfohriTcsthhaieisrsrftyiuhsletflhsoaaedrmdstaehemref.uefnufcultlinoacndt.iadosentrhaes tchaerry
Z
C
操作系统精髓与设计原理（第5版）课后习题答案
操作系统精髓与设计原理（第5版）课后习题答案第1章计算机系统概述1.1、图1.3中的理想机器还有两条I/O指令：0011 = 从I/O中载入AC0111 = 把AC保存到I/O中在这种情况下，12位地址标识一个特殊的外部设备。
请给出以下程序的执行过程（按照图1.4的格式）：1.从设备5中载入AC。
2.加上存储器单元940的内容。
3.把AC保存到设备6中。
假设从设备5中取到的下一个值为3940单元中的值为2。
答案：存储器（16进制内容）：300：3005；301：5940；302：7006步骤1：3005－>IR；步骤2：3－>AC步骤3：5940－>IR；步骤4：3＋2＝5－>AC步骤5：7006－>IR：步骤6：AC－>设备61.2、本章中用6步来描述图1.4中的程序执行情况，请使用MAR 和MBR扩充这个描述。
答案：1. a. PC中包含第一条指令的地址300，该指令的内容被送入MAR中。
b. 地址为300的指令的内容（值为十六进制数1940）被送入MBR，并且PC增1。
这两个步骤是并行完成的。
c. MBR中的值被送入指令寄存器IR中。
2. a. 指令寄存器IR中的地址部分（940）被送入MAR中。
b. 地址940中的值被送入MBR中。
c. MBR中的值被送入AC中。
3. a. PC中的值（301）被送入MAR中。
b. 地址为301的指令的内容（值为十六进制数5941）被送入MBR，并且PC增1。
c. MBR中的值被送入指令寄存器IR中。
4. a. 指令寄存器IR中的地址部分（941）被送入MAR中。
b. 地址941中的值被送入MBR中。
c. AC中以前的内容和地址为941的存储单元中的内容相加，结果保存到AC中。
5. a. PC中的值（302）被送入MAR中。
b. 地址为302的指令的内容（值为十六进制数2941）被送入MBR，并且PC增1。
c. MBR中的值被送入指令寄存器IR中。
数字逻辑课后习题答案（科学出版社_第五版）
第一章开关理论基础1.将下列十进制数化为二进制数和八进制数十进制二进制八进制49 110001 6153 110101 65127 1111111 177635 1001111011 11737.493 111.1111 7.7479.43 10011001.0110111 231.3342.将下列二进制数转换成十进制数和八进制数二进制十进制八进制1010 10 12111101 61 751011100 92 1340.10011 0.59375 0.46101111 47 5701101 13 153.将下列十进制数转换成8421BCD码1997=0001 1001 1001 011165.312=0110 0101.0011 0001 00103.1416=0011.0001 0100 0001 01100.9475=0.1001 0100 0111 01014.列出真值表，写出X的真值表达式A B C X0 0 0 00 0 1 00 1 0 00 1 1 11 0 0 01 0 1 11 1 0 11 1 1 1 X=A BC+A B C+AB C+ABC5.求下列函数的值当A,B,C为0,1,0时：A B+BC=1(A+B+C)(A+B+C)=1(A B+A C)B=1当A,B,C为1,1,0时：A B+BC=0(A+B+C)(A+B+C)=1(A B+A C)B=1当A,B,C为1,0,1时：A B+BC=0(A+B+C)(A+B+C)=1(A B+A C)B=06.用真值表证明下列恒等式(1) (A⊕B)⊕C=A⊕(B⊕C)A B C (A⊕B)⊕C A⊕(B⊕C)0 0 0 0 00 0 1 1 10 1 0 1 10 1 1 0 01 0 0 1 11 0 1 0 01 1 0 0 01 1 1 1 1所以由真值表得证。
（2）A⊕B⊕C=A⊕B⊕CA B C A⊕B⊕C A⊕B⊕C0 0 0 1 10 0 1 0 00 1 0 0 00 1 1 1 11 0 0 0 01 0 1 1 11 1 0 1 11 1 1 0 07.证明下列等式（1）A+A B=A+B证明：左边= A+A B=A(B+B)+A B=AB+A B+A B=AB+A B+AB+A B=A+B=右边(2)ABC+A B C+AB C=AB+AC证明：左边= ABC+A B C+AB C= ABC+A B C+AB C+ABC=AC(B+B)+AB(C+C)=AB+AC=右边（3）EDCCDACBAA)(++++=A+CD+E证明：左边=EDCCDACBAA)(++++=A+CD+A B C+CD E=A+CD+CD E=A+CD+E=右边（4）C B A C B A B A ++=CB C A B A ++证明：左边=CB AC B A B A ++=CB AC AB C B A B A +++)(=C B C A B A ++=右边8.用布尔代数化简下列各逻辑函数表达式(1) F=A+ABC+A C B +CB+C B = A+BC+C B(2) F ＝(A+B+C )(A+B+C) = (A+B)+C C = A+B(3) F ＝ABC D +ABD+BC D +ABCD+B C = AB+BC+BD(4) F=C AB C B BC A AC +++= BC(5) F=)()()()(B A B A B A B A ++++＝BA 9.将下列函数展开为最小项表达式(1) F(A,B,C) = Σ(1,4,5,6,7)(2) F(A,B,C,D) = Σ(4,5,6,7,9,12,14)10.用卡诺图化简下列各式（1）CAB C B BC A AC F +++=0ABC00 01 11 1011111化简得F=C(2)CB A D A B A DC AB CD B A F++++=111111ABCD 00 01 11 1000011110化简得F=DA B A +（3） F(A,B,C,D)=∑m (0,1,2,5,6,7,8,9,13,14)1111111111AB CD 00 01 11 1000011110化简得F=DBC D C A BC A C B D C ++++(4) F(A,B,C,D)=∑m (0,13,14,15)+∑ϕ(1,2,3,9,10,11)Φ1ΦΦ1ΦΦ1Φ1AB CD 00 01 11 1000011110化简得F=ACAD B A ++11.利用与非门实现下列函数，并画出逻辑图。
【免费下载】数字逻辑课后习题答案科学出版社 第五版
所以由真值表得证。
（2） A B C =A B C
X= A BC+A B C+AB C +ABC
A B+BC=1 (A+B+C)( A + B + C )=1 ( A B+A C )B=1 A B+BC=0 (A+B+C)( A + B + C )=1 ( A B+A C )B=1 A B+BC=0 (A+B+C)( A + B + C )=1 ( A B+A C )B=0
4.列出真值表，写出 X 的真值表达式
A BC X 0000 0010 0100 0111 1000
二进制 110001 110101 1111111 1001111011 111.1111 10011001.0110111
十进制 10 61 92 0.59375 47 13
对全部高中资料试卷电气设备，在安装过程中以及安装结束后进行高中资料试卷调整试验；通电检查所有设备高中资料电试力卷保相护互装作置用调与试相技互术关，系电，力根通保据过护生管高产线中工敷资艺设料高技试中术卷资，配料不置试仅技卷可术要以是求解指，决机对吊组电顶在气层进设配行备置继进不电行规保空范护载高与中带资负料荷试下卷高问总中题体资，配料而置试且时卷可，调保需控障要试各在验类最；管大对路限设习度备题内进到来行位确调。保整在机使管组其路高在敷中正设资常过料工程试况中卷下，安与要全过加，度强并工看且作护尽下关可都于能可管地以路缩正高小常中故工资障作料高；试中对卷资于连料继接试电管卷保口破护处坏进理范行高围整中，核资或对料者定试对值卷某，弯些审扁异核度常与固高校定中对盒资图位料纸置试，.卷保编工护写况层复进防杂行腐设自跨备动接与处地装理线置，弯高尤曲中其半资要径料避标试免高卷错等调误，试高要方中求案资技，料术编试交写5、卷底重电保。要气护管设设装线备备置敷4高、调动设中电试作技资气高，术料课中并3中试、件资且包卷管中料拒含试路调试绝线验敷试卷动槽方设技作、案技术，管以术来架及避等系免多统不项启必方动要式方高，案中为；资解对料决整试高套卷中启突语动然文过停电程机气中。课高因件中此中资，管料电壁试力薄卷高、电中接气资口设料不备试严进卷等行保问调护题试装，工置合作调理并试利且技用进术管行，线过要敷关求设运电技行力术高保。中护线资装缆料置敷试做设卷到原技准则术确：指灵在导活分。。线对对盒于于处调差，试动当过保不程护同中装电高置压中高回资中路料资交试料叉卷试时技卷，术调应问试采题技用，术金作是属为指隔调发板试电进人机行员一隔，变开需压处要器理在组；事在同前发一掌生线握内槽图部内 纸故，资障强料时电、，回设需路备要须制进同造行时厂外切家部断出电习具源题高高电中中源资资，料料线试试缆卷卷敷试切设验除完报从毕告而，与采要相用进关高行技中检术资查资料和料试检，卷测并主处且要理了保。解护现装场置设。备高中资料试卷布置情况与有关高中资料试卷电气系统接线等情况，然后根据规范与规程规定，制定设备调试高中资料试卷方案。
《逻辑与计算机设计基础》(原书第五版)课后习题答案-chapter01_solutions-5th
1CHAPTER 1© 2016 Pearson Education, Inc.1-1.(a)(1) Calm:(2) 10 mph(3) 100 mphor(b) The microcomputer requires a table or equation for converting from rotations/second to miles/hour. The pulses produced by the rotating disk must be counted over a known period of time, and the table or equation used to convert the binary count to miles per hour.1-2.–34° quantizes to –30° => 1 V => 0001+31° quantizes to +30° => 7 V => 0111 +77° quantizes to +80° => 12 V => 1100 +108° quantizes to +110° => 15 V => 11111-3.*Decimal, Binary, Octal and Hexadecimal Numbers from (16)to (31)1-4.1020301281282131,0723232233,554,4328828,589,934,592=⨯==⨯==⨯=K Bits M Bits G Bits21-5.220 = (1,000,00010 + d ) where d = 48,576 1Tb = 240 = (220)2 = (1,000,000 + d )2= (1,000,000)2 + 2(1,000,000) d + d 2 = 1,000,000,000,000 + 97,152,000,000 + 2,359,627,776 = 1,099,511,627,7761-6.112511212047252133,554,4311 Bits 1 Bits ⇒-=⇒-=1-7.*63202641013275321142(1001101)222277(1010011.101)22222283.625(10101110.1001)2222222174.5625----=+++==+++++==++++++=1-8.1 1 0 0 0 0 0 0 0 0 0 1 0 11-9.*1-10.*a)0.45 × 8 = 3.6 =>0.60 × 8 = 4.8 =>0.80 × 8 = 6.4 =>0.20 × 8 = 3.2 =>10= (16612.3463)8b) (1938.257)10 = (792.41CB)16c) (175.175)10 = (10101111.001011)21-11.*a) (673.6)8= (110 111 011.110)2= (1BB.C)16b) (E7C.B)16= (1110 0111 1100.1011)2= (7174.54)8c) (310.2)4= (11 01 00.10)2= (64.4)81-12.a) 1010 b) 0110 c) 1111001×1100 ×1001 ×0111010000 0110 11110010000 0000 0000001010 0000 11110011010 0110 11110011111000 0110110 11110010000000110110110101341-13.+1000110110101101010000000010000110001101011-----Quotient = 10001R emainder = 1 1-14.(a) 6 × 123 + 8 × 122 + 7 × 121 + 4 = 11608 (b)12 1-15.a)0 1 2 3 4 5 6 7 8 9 A BCDEFGHIJb) 20c) 21012010(.)1120122018201620(4658.8)BCI G -=⨯+⨯+⨯+⨯=1-16.*a) (BEE)r = (2699)1021021114142699111426850r r r r r ⨯+⨯+⨯=⨯+⨯-=By the quadratic equation: r = 15 or ≈ –16.27 ANSWER: r = 15 b) (365)r = (194)102102365194361890r r r r r ⨯+⨯+⨯=⨯+⨯-=By the quadratic equation: r = – 9 or 7 ANSWER: r = 751-17.Errata: The text has an error: 1480 should be 1460. This will be corrected in future printings.Noting the order of operations, first add (34)r and (24)r101010(34)34(24)24(34)(24)58r r r r r r r r r r =⨯+⨯=⨯+⨯+=⨯+⨯Now, multiply the result by (21)r101021(21)(58)10218⨯+⨯⨯⨯+⨯=⨯+⨯+r r r r r r Next, set the result equal to (1480)r and reorganize.21321321010218146161580⨯+⨯+=⨯+⨯+⨯⨯-⨯-⨯-⨯=r r r r r r r r r Finally, find the roots of this cubic polynomial. Solutions are: r = 8, – 1, – 1ANSWER: The chicken has 4 toes on each foot (half of 8).1-18.*a) (0100 1000 0110 0111)BCD = (4867)10= (1001100000011)2 b) (0011 0111 1000.0111 0101)BCD= (378.75)10=(101111010.11)21-19.*(694)10 = (0110 1001 0100)BCD (835)10=(1000 0011 0101)BCD1001 0100 +0011 +0101 1100 1001 +0110 +00001 001010011-20.*(a)101 1000111 1000Move R 011 1100 0 100 column > 0111Subtract 3 −0011011 1001 0Subtract 3 −001101 1001Move R 0 1100 110 100 column > 0111Subtract 3 −00110 1001 110Move R 0100 1110Move R 010 01110Move R 01 001110Move R 0 1001110 Leftmost 1 in BCD numbershifted out: Finished(b) 102101 1000011 1001 0111Move R 001 1100 1011 1 101 and 100 columns > 0111Subtract 3 −0011 -0011001 1001 1000 1Move R 00 1100 1100 01 101 and 100 columns > 0111Subtract 3 −0011 −001100 1001 1001 01Move R 0 0100 1100 101 100 column > 0111Subtract 3 −00110 0100 1001Move R 0010 0100 1101Move R 001 0010 01101Move R 00 1001 001101 100 column > 0111Subtract 3 −001100 0110 001101Move R 0 0011 0001101Move R 0001 10001101Move R 000 110001101 Leftmost 1 in BCDnumber shifted out: Finished 1-21.(a) 10210110011110001st Move L 1 1110002nd Move L 11 110003rd Move L 111 1000 100 column > 100Add 3 00111010 10004th Move L 1 0101 000 100 column > 100Add 3 00111 1000 0005th Move L 11 0000 006th Move L 110 00000 101 column > 100Add 3 00111001 0000 07th Move L 1 0001 00000 Least significant bit in binary number moved in:Finished(b) 103102101100011100101111st Move L 0 11100101112nd Move L 01 1100101113rd Move L 011 100101114th Move L 0111 0010111 100 column > 100Add 3 001161010 00101115th Move L 1 0100 0101116th Move L 10 1000 10111 100 column > 100Add 3 001110 1011 101117th Move L 101 0111 0111 101 & 100 columns > 100Add 3 0011 00111000 1010 01118th Move L 1 0001 0100 1119th Move L 10 0010 1001 11 100 column > 100Add 3 001110 0010 1100 1110th Move L 100 0101 1001 1 101 &100 columns > 100Add 3 0011 0011100 1000 1100 111th Move L 1001 0001 1001 Least significant bit in binary number moved in: Finished1-22.From Table 1-5, complementing the bit B6 will switch an uppercase letter to a lower case letter and vice versa.1-23.a) The name used is Brent M. Ledvina. An alternative answer: use both upper and lower case letters.0100 0010 B 0101 0010 R 0100 0101 E0100 1110 N 0101 0100 T 0010 0000 (SP)0100 1101 M 0010 1110 . 0010 0000 (SP)0100 1100 L 0100 0101 E 0100 0100 D0101 0110 V 0100 1001 I 0100 1110 N0100 0001 Ab) 0100 0010 1101 0010 1100 01010100 1110 1101 0100 1010 00000100 1101 0010 1110 1010 00001100 1100 1100 0101 0100 01000101 0110 1100 1001 0100 11100100 00011-24.1000111 G1101111 o01000001000011 C1100001 a1110010 r1100100 d1101001 i1101110 n1100001 a (Errata: This number appears as 110001, which would be “1”)1101100 l1110011 s0100001 !781-25.*a) (11111111)2b) (0010 0101 0101)BCD c) 011 0010 011 0101 011 0101ASCIId)0011 00101011 01011011 0101ASCII with Odd Parity1-26.a) U+0040 = 01000000b) U+00A2 = 11000010 10100010c) U+20AC = 11100010 10000010 10101100d)U+1F6B2 = 11110000 10011111 10011010 101100101-27.Binary Numbers from (32)to (47) with Odd and Even Parity1-28.Gray Code for Hexadecimal Digits1-29.(a) Wind Direction Gray CodeDirectionCode WordN 000 S 110 E 011 W 101 NW 100 NE 001 SW 111 SE0109(b) Wind Direction Gray Code (directions in adjacent order)DirectionCode WordN 000 NE 001 E 011 SE 010 S 110 SW 111 W 101 NW100As the wind direction changes, the codes change in the order of the rows of this table, as suming that the bottom row is “next to” the top row. From the table, the codes that result due to a wind direction change always change in a single bit.1-30.+The percentage of power consumed by the Gray code counter compared to a binary code counter equals:Number of bit changes using Gray code Number of bit changes using binary codeAs shown in Table 1-6, and by definition, the number of bit changes per cycle of an n-bit Gray code counter is 1 per count = 2n .Number of bit changes using Gray code = 2nFor a binary counter, notice that the least significant bit changes on every increment. The second least significant bit changes on every other increment. The third digit changes on every fourth increment of the counter, and so on. As shown in Table 1-6, the most significant digit changes twice per cycle of the binary counter.Number of bit changes using binary code 11222n n -+++(1)110221(21)122nni i n n i i ++==⎡⎤==-=--=-⎢⎥⎣⎦∑∑ % Power (1)210022n n +=⨯-。
逻辑与计算机设计基础课后答案及分析和实验
浙江大学计算机学院实验教学中心逻辑与计算机设计基础实验2010~2011秋冬9掌握二进制计数器/定时器的工作原理与设计方法掌握用计数器进行分频的概念和方法实验设备装有ISE的计算机系统1台Spartan III 实验板1套实验材料无设计一个数字钟，使用60进制和24(12)进制计数器，实现24小时内时间的实时显示。
60进制计数器用10进制与6进制计数器的组合来实现；24(12)进制可用类似方法实现。
采用4个计数器分别实现分钟的个位、分钟的十位、小时的个位、小时的十位计数。
数字钟的初值通过初始化语句来实现，用数码管前两位显示小时的十位和个位，后两位显示分钟的十位和个位。
把时钟加到多功能计算器中。
计数器是复杂数字系统和计算机硬件系统中的一个基本部件，是计数、分频、定时、同步和时基等电路的核心，在计算机、网络、通信等设备中经常使用到。
本实验以计数器为例，采用行为级描述的方法设计时序电路，实现各种常用的计数器和定时器。
•60进制计数器用10进制与6进制计数器的组合来实现•24进制计数器用4进制与6进制计数器的组合来实现module m_gen_min (clk_sec, clk_hour, min_low, min_high);input wire clk_sec;output reg clk_hour;output reg [3:0] min_low, min_high;reg [15:0] cnt;always @(posedge clk_sec) begin if (clk_hour == 1)clk_hour = 0;if (cnt == 59) begin cnt = 0;if (min_low == 9) begin min_low = 0; /* base 10 */if (min_high == 5) begin min_high = 0; /* base 6*/clk_hour = 1;end else min_high = min_high + 1;end else min_low = min_low + 1;end else cnt = cnt + 1;endendmodule分钟的生成以秒脉冲为时钟信号，将10进制和6进制组合成60进制，并产生小时脉冲信号。
逻辑与计算机设计基础第五版课后答案chapter06
逻辑与计算机设计基础第五版课后答案Chapter066.1 填空题1.逻辑电路中，5个逻辑门包括与门、或门、非门、与非门和或非门。
2.组合逻辑电路是由逻辑门组成的。
3.子电路是由节点和逻辑门组成的。
4.逻辑门的输入端数称为其阶数。
5.卡诺图的横纵坐标是逻辑变量。
6.卡诺图上的每个矩形对应一个最小项。
7.复杂逻辑函数可以用几个较小的逻辑单元构成。
8.门电路可不断扩展到更复杂的逻辑电路。
9.简化布尔函数是优化逻辑电路的一种方法。
10.简化布尔函数可以减少电路中的逻辑门数量。
6.2 选择题1.LogicWorks软件包含设计逻辑电路所需的全部功能，以下哪个是LogicWorks的主要特点？A. 用户界面简洁易用，适合初学者使用。
B. 提供了强大的绘图工具和仿真工具。
C. 方便学生理解和掌握基本逻辑电路的设计方法。
D. 以上三项都是。
答案：D2.LogicWorks 是一个集成功能强大的绘图工具和仿真工具于一身的逻辑设计软件，它具有如下特点，除了__B__之外全部是（）。
A. 用户界面简介易用，适合初学者使用。
B. 它可以为学生编写和作业设计电路图。
C. 提供由身强大的仿真工具。
D. 方便学生理解和掌握基本逻辑电路的设计方法。
答案：B3.在 LogicWorks 软件中，可以通过输入“ EN ” 来实现一个与逻辑电路的启停。
假设“ EN ” 通入值为高电位则电路启动，“ EN ” 通入值为低电位则电路停止。
A. 对B. 错答案：A4.LogicWorks 中连接点上的箭头表示其状态，仅当断头朝上是高电平表示 1，断头朝下是低电平表示 0。
A. 对B. 错答案：B5.以下哪一项是LogicWorks软件中可以直接编辑数字逻辑电路的操作？A. 点击元件图形中每个连接点上方那一根看不见的电线就可以显示与进一步编辑节点的名称。
B. 在每个连接点上方那根看不见的线上点击鼠标右键可以弹出含有描述节点名称的对话框。
C. 鼠标左键只点击连接点上方的连线，可以改变该位的电平状态。
- 1、下载文档前请自行甄别文档内容的完整性，平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益，请联系客服反馈,我们会尽快为您处理(人工客服工作时间：9:00-18:30)。
CHAPTER 11
Problem Solutions – Chapter 11
© 2016 Pearson Education, Inc.
11-1*
Heads × (cylinders/Head) × (sectors/cylinder) × (1 cylinder/track) × (bytes/sector)
11-6.
# Port A ADRS 1 0000 0001 2 0000 0010 3 0000 0100 4 0000 1000 5 0001 0000 6 0010 0000
Port B ADRS 0100 0001 0100 0010 0100 0100 0100 1000 0101 0000 0110 0000
Problem Solutions – Chapter 11
A given address can be shared by two registers if one is write only and one is read only. If a register is both written to and read from the bus, then it needs its own address. An 8-bit address provides 256 addresses. Suppose that the 50 % of registers requiring 1 address is X. Then the remaining 50 % of the registers, also X can share addresses requiring only 0.5 addresses. So 1.5 X = 256 and X = 170.67 registers for a total of 341.33 registers. To meet the original constraints exactly, the total number of registers must be divisible by 4, so 340 registers can be used, 170 of which are read/write, 85 of which are read only and 85 of which are write only. There is one more address available for either one read/write register or up to a pair with a read only register and a write only register.
RS0 A0, RS1 A1,(CDC)1S6 = A(71A160A05A1140A13)2(A2A1 RAS21A=1)0 RS1= 1
RS0 = A0, RS1 =A1, CS = A7A6A5A4A3(A2A1 + A2A1)
RS0
A0
RS1
A1
A7
A5
A6
A4
A3
CS
A2
A1
11-5.*
Control ADRS 1000 0001 1000 0010 1000 0100 1000 1000 1001 0000 1010 0000
Status ADRS 1100 0001 1100 0010 1100 0100 1100 1000 1101 0000 1110 0000
1
பைடு நூலகம் 11-7.*
111001((110CC010)))AB222))1166
= =
(110RR0 SS111010==2)
1 1
RSR0S1 ==1 0 RS0 RS0 = 1
=
0
(110R0S11011)=2 0 RSR0S1==1 0 RS0 = 1
(CD)16 = (1100 11(0C1C)2)16 = (1100RS11100)=2 0 RSR1S1==0 1 RS0= 0
11-8.
CPU
CPU Data Bus I/O Read Interface
I/O Data Bus STB IBF
for CS inputs permitting at most 14 I/O Interface Units to be supported.
b) Since two bits must be used to address the four registers, there are 14 bits remaining and 214 or 16,384 distinct I/O Interface Units can be supported.
a) 1 × 1023 × 63 × 512 =
32,224.5 Kbytes (K = 1024)
b) 4 × 8191 × 63 × 512 =
1,032,066 Kbytes
c) 16 × 16383 × 63 × 512 =
8,257,032 Kbytes
11-2.
8.5 msec + 4.17 msec + 0 msec + ((1 sec/(100 Mbytes)) × 1Mbytes)=22.67 ms
a) If each address line is used for a different CS input, there will be no way to address
the four registers so 2 bits are needed to address the registers. Only 14 lines can be used
11-3.
Pixels a) 1,310,720 b) 1,920,000 c) 1,764,000 d) 2,304,000
Subpixels 3,932,160 5,770,000 5,292,000 6,912,000
11-4.
(CA)16 = (CB)16 = (CC)16 =
(1100 (1100 (1100