2019AMC 8(美国数学竞赛)题目

合集下载

AMC8（美国数学邀请赛）

AMC8（美国数学邀请赛）AMC8(美国数学邀请赛）AMC8（American Mathematics Competition8）AMC8是美国初中数学竞赛，是针对八年级以下学生的数学科测试，有些小学四～六年级的优秀学生也可以参加，该竞赛开始于1985年，于每年11月中旬的一个星期二举行。

AMC8竞赛内容与美国7、8年级数学大纲相对应，包括（但不局限于）整数、分数、小数、百分数、比例、数论、日常的几何、面积、体积、概率及统计、逻辑推理等。

美国数学协会（MAA）组织AMC8竞赛的目的是通过这样一种对学生有吸引力的考试，增加学生在数学方面的兴趣及学习数学的热情，促进学生学习中学数学必修课程之外的数学内容，增强问题解决的能力，该考试给参加者提供了应用初中所学概念处理由易到难，并包含广泛应用的问题的机会，以使他们得到在初中数学课堂中所不能得到的解决问题的经验，获得高分的部分学生将受邀参加美国高中数学竞赛AMC10。

题数︰25题时间︰40分钟题型︰选择题满分︰25分成绩处理︰AMC总部计分方式︰答对一题一分，答错不倒扣美国数学竞赛amc8的常用数学英语单词美国数学竞赛amc8的常用数学英语单词数学mathematics, maths（BrE）, math（AmE）被除数dividend除数divisor 商quotient 等于equals, is equal to, is equivalent to 大于is greater than小于is lesser than大于等于is equal or greater than小于等于is equal or lesser than运算符operator数字digit数number自然数natural number公理axiom定理theorem计算calculation运算operation证明prove假设hypothesis, hypotheses（pl.）命题proposition算术arithmetic加plus（prep.）, add（v.）, addition（n.）被加数augend, summand加数addend和sum减minus（prep.）, subtract（v.）, subtraction（n.）被减数minuend减数subtrahend差remainder乘times（prep.）, multiply（v.）, multiplication（n.）被乘数multiplicand, faciend乘数multiplicator积product除divided by（prep.）, divide（v.）, division（n.）整数integer小数decimal小数点decimal point分数fraction分子numerator分母denominator比ratio正positive负negative零null, zero, nought, nil十进制decimal system二进制binary system十六进制hexadecimal system权weight, significance进位carry截尾truncation四舍五入round下舍入round down上舍入round up有效数字significant digit无效数字insignificant digit代数algebra公式formula, formulae（pl.）单项式monomial多项式polynomial, multinomial系数coefficient未知数unknown, x-factor, y-factor, z-factor 等式，方程式equation一次方程simple equation二次方程quadratic equation三次方程cubic equation四次方程quartic equation不等式inequation阶乘factorial对数logarithm指数，幂exponent乘方power二次方，平方square三次方，立方cube四次方the power of four, the fourth power n次方the power of n, the nth power开方evolution, extraction二次方根，平方根square root三次方根，立方根cube root四次方根the root of four, the fourth root n次方根the root of n, the nth root集合aggregate元素element空集void子集subset交集intersection并集union补集complement映射mapping函数function定义域domain, field of definition值域range常量constant变量variable单调性monotonicity奇偶性parity周期性periodicity图象image数列，级数series微积分calculus微分differential导数derivative极限limit无穷大infinite（a.）infinity（n.）无穷小infinitesimal积分integral定积分definite integral不定积分indefinite integral有理数rational number无理数irrational number实数real number虚数imaginary number复数complex number矩阵matrix行列式determinant几何geometry点point线line面plane体solid线段segment射线radial平行parallel相交intersect角angle角度degree弧度radian锐角acute angle直角right angle钝角obtuse angle平角straight angle周角perigon底base边side高height三角形triangle锐角三角形acute triangle直角三角形right triangle直角边leg斜边hypotenuse勾股定理Pythagorean theorem钝角三角形obtuse triangle不等边三角形scalene triangle等腰三角形isosceles triangle等边三角形equilateral triangle四边形quadrilateral平行四边形parallelogram矩形rectangle长length宽width菱形rhomb, rhombus, rhombi（pl.）, diamond 正方形square 梯形trapezoid直角梯形right trapezoid等腰梯形isosceles trapezoid五边形pentagon六边形hexagon七边形heptagon八边形octagon九边形enneagon十边形decagon十一边形hendecagon十二边形dodecagon多边形polygon正多边形equilateral polygon圆circle圆心centre（BrE）, center（AmE）半径radius 直径diameter圆周率pi弧arc半圆semicircle扇形sector环ring椭圆ellipse圆周circumference周长perimeter面积area轨迹locus, loca（pl.）相似similar全等congruent四面体tetrahedron五面体pentahedron六面体hexahedron平行六面体parallelepiped立方体cube七面体heptahedron八面体octahedron九面体enneahedron十面体decahedron十一面体hendecahedron 十二面体dodecahedron 二十面体icosahedron多面体polyhedron棱锥pyramid棱柱prism棱台frustum of a prism 旋转rotation轴axis圆锥cone圆柱cylinder圆台frustum of a cone球sphere半球hemisphere底面undersurface表面积surface area体积volume空间space坐标系coordinates坐标轴x-axis, y-axis, z-axis 横坐标x-coordinate纵坐标y-coordinate原点origin双曲线hyperbola抛物线parabola三角trigonometry正弦sine余弦cosine正切tangent余切cotangent正割secant余割cosecant反正弦arc sine反余弦arc cosine反正切arc tangent反余切arc cotangent反正割arc secant反余割arc cosecant相位phase周期period振幅amplitude内心incentre（BrE）, incenter（AmE）外心excentre（BrE）, excenter（AmE）旁心escentre（BrE）, escenter（AmE）垂心orthocentre（BrE）, orthocenter（AmE）重心barycentre（BrE）, barycenter（AmE）内切圆inscribed circle 外切圆circumcircle统计statistics平均数average加权平均数weighted average方差variance标准差root-mean-square deviation, standard deviation 比例propotion百分比percent百分点percentage百分位数percentile排列permutation组合combination概率，或然率probability分布distribution正态分布normal distribution非正态分布abnormal distribution 图表graph条形统计图bar graph柱形统计图histogram折线统计图broken line graph曲线统计图curve diagram扇形统计图pie diagram。

2019年美国数学竞赛8年级(AMC8)真题(附答案)(电脑版)

2019 年美国数学竞赛 8 年级(AMC8) 真题(附答案 )(电脑版 )
2019 年美国数学竞赛 8 年级(AMC8) 真题(附答案 )(电脑版 )
2019 年美国数学竞赛 8 年级(AMC8) 真题(附答案 )(电脑版 )
2019 年美国数学竞赛 8 年级(AMC8) 真题(附答案 )(电脑版 )
2019 年美国数学竞赛 8 年级(AMC8) 真题(附答案 )(电脑版 )
2019 年美国数学竞赛 8 年级(AMC8) 真题(附答案 )(电脑版 )
AMC 系列比赛一共有以下几个比赛：
American Mathematics Competition 8 - C 8
American Mathematics Competition 10/12 - AMC 10/12
American Invitational Mathematics Exam - AIME United States Mathematical Olympiad and Junior Mathematical Olympiad - USA(J)MO
2019 年美国数学竞赛 8 年级(AMC8) 真题(附答案 )(电脑版 )
2019 年美国数学竞赛 8 年级(AMC8) 真题(附答案 )(电脑版 )
2019 年美国数学竞赛 8 年级(AMC8) 真题(附答案 )(电脑版 )
2019 年美国数学竞赛 8 年级(AMC8) 真题(附答案 )(电脑版 )
2019 年美国数学竞赛 8 年级(AMC8) 真题(附答案 )(电脑版 )
美国数学竞赛 8 年级(AMC8)真题（附答案）
AMC 系列全称 American Mathematics Competitions 的（1950 年开始举办）的和最负盛名初高中生数学竞赛。日下午结束。

美国AMC8数学竞赛试题(含答案)

2001 年美国AMC8 (2001年月日时间40分钟)1. 卡西的商店正在制作一个高尔夫球奖品。

他必须给一颗高尔夫球面上的300个小凹洞着色，如果他每着色一个小凹洞需要2秒钟，试问共需多分钟才能完成他的工作。

(A) 4 (B) 6 (C) 8 (D) 10 (E) 12 。

2. 我正在思考两个正整数，它们的乘积是24且它们的和是11，试问这两个数中较大的数是什么。

(A) 3 (B) 4 (C) 6 (D) 8 (E) 12 。

3. 史密斯有63元，艾伯特比安加多2元，而安加所有的钱是史密斯的三分之一，试问艾伯特有元。

(A) 17 (B) 18 (C) 19 (D) 21 (E) 23 。

4. 在每个数字只能使用一次的情形下，将1，2，3，4及9作成最小的五位数，且此五位数为偶数，则其十位数字为。

(A) 1 (B) 2 (C) 3 (D) 4 (E) 9 。

5. 在一个暴风雨的黑夜里，史努比突然看见一道闪光。

10秒钟后，他听到打雷声音。

声音的速率是每秒1088呎，但1哩是5280呎。

若以哩为单位的条件下，估计史努比离闪电处的距离最接近下列何者。

(A) 1 (B) 121 (C) 2 (D) 221 (E) 3 。

6. 在一笔直道路的一旁有等间隔的6棵树。

第1棵树与第4棵树之间的距离是60呎。

试问第1 棵树到最后一棵树之间的距离是呎。

(A) 90 (B) 100 (C) 105 (D) 120 (E) 140 。

问题7、8、9请参考下列叙述：主题：竞赛场所上的风筝展览7. 葛妮芙为提升她的学校年度风筝奥林匹亚竞赛的质量，制作了一个小风筝与一个大风筝，并陈列在公告栏展览，这两个风筝都如同图中的形状，葛妮芙将小风筝张贴在单位长为一吋(即每两点距离一吋)的格子板上，并将大风筝张贴在单位长三吋(即每两点距离三吋)的格子板上。

试问小风筝的面积是平方吋。

(A) 21 (B) 22 (C) 23 (D) 24 (E) 25 。

美国数学竞赛AMC题目及答案

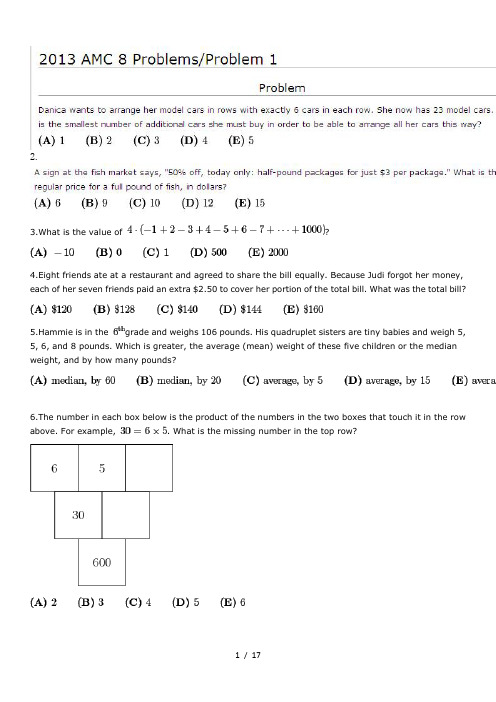
2.3.What is the value of ?4.Eight friends ate at a restaurant and agreed to share the bill equally. Because Judi forgot her money, each of her seven friends paid an extra $2.50 to cover her portion of the total bill. What was the total bill?5.Hammie is in the grade and weighs 106 pounds. His quadruplet sisters are tiny babies and weigh 5, 5, 6, and 8 pounds. Which is greater, the average (mean) weight of these five children or the median weight, and by how many pounds?6.The number in each box below is the product of the numbers in the two boxes that touch it in the row above. For example, . What is the missing number in the top row?7.Trey and his mom stopped at a railroad crossing to let a train pass. As the train began to pass, Trey counted 6 cars in the first 10 seconds. It took the train 2 minutes and 45 seconds to clear the crossing at a constant speed. Which of the following was the most likely number of cars in the train?8.A fair coin is tossed 3 times. What is the probability of at least two consecutive heads?9.The Incredible Hulk can double the distance he jumps with each succeeding jump. If his first jump is 1 meter, the second jump is 2 meters, the third jump is 4 meters, and so on, then on which jump will he first be able to jump more than 1 kilometer?10.What is the ratio of the least common multiple of 180 and 594 to the greatest common factor of 180 and 594?11. Ted's grandfather used his treadmill on 3 days this week. He went 2 miles each day. On Monday he jogged at a speed of 5 miles per hour. He walked at the rate of 3 miles per hour on Wednesday and at 4 miles per hour on Friday. If Grandfather had always walked at 4 miles per hour, he would have spent less time on the treadmill. How many minutes less?12. At the 2013 Winnebago County Fair a vendor is offering a "fair special" on sandals. If you buy one pair of sandals at the regular price of $50, you get a second pair at a 40% discount, and a third pair at half the regular price. Javier took advantage of the "fair special" to buy three pairs of sandals. What percentage of the $150 regular price did he save?13. When Clara totaled her scores, she inadvertently reversed the units digit and the tens digit of one score. By which of the following might her incorrect sum have differed from the correct one?14. Let the two digits be and .The correct score was . Clara misinterpreted it as . The difference between the two is which factors into . Therefore, since the difference is a multiple of 9, the only answerchoice that is a multiple of 9 is .15. If , , and , what is the product of , , and ?16. A number of students from Fibonacci Middle School are taking part in a community service project. The ratio of -graders to -graders is , and the the ratio of -graders to -graders is . What is the smallest number of students that could be participating in the project?17. The sum of six consecutive positive integers is 2013. What is the largest of these six integers?18. Isabella uses one-foot cubical blocks to build a rectangular fort that is 12 feet long, 10 feet wide, and 5 feet high. The floor and the four walls are all one foot thick. How many blocks does the fort contain?--Arpanliku 16:22, 27 November 2013 (EST) Courtesy of Lord.of.AMC19. Bridget, Cassie, and Hannah are discussing the results of their last math test. Hannah shows Bridget and Cassie her test, but Bridget and Cassie don't show theirs to anyone. Cassie says, 'I didn't get the lowest score in our class,' and Bridget adds, 'I didn't get the highest score.' What is the ranking of the three girls from highest to lowest?20. A rectangle is inscribed in a semicircle with longer side on the diameter. What is the area of the semicircle?21. Samantha lives 2 blocks west and 1 block south of the southwest corner of City Park. Her school is 2 blocks east and 2 blocks north of the northeast corner of City Park. On school days she bikes on streets to the southwest corner of City Park, then takes a diagonal path through the park to the northeast corner, and then bikes on streets to school. If her route is as short as possible, how many different routes can she take?22. Toothpicks are used to make a grid that is 60 toothpicks long and 32 toothpicks wide. How many toothpicks are used altogether?23. Angle of is a right angle. The sides of are the diameters of semicircles as shown. The area of the semicircle on equals , and the arc of the semicircle on has length .What is the radius of the semicircle on ?24. Squares , , and are equal in area. Points and are the midpoints of sidesand , respectively. What is the ratio of the area of the shaded pentagon to the sum of the areas of the three squares?25. A ball with diameter 4 inches starts at point A to roll along the track shown. The track is comprised of 3 semicircular arcs whose radii are inches, inches, and inches, respectively. The ball always remains in contact with the track and does not slip. What is the distance the center of theball travels over the course from A to B?1.2.The 50% off price of half a pound of fish is $3, so the 100%, or the regular price, of a half pound of fish is $6. Consequently, if half a pound of fish costs $6, then a whole pound of fish is dollars.3.Notice that we can pair up every two numbers to make a sum of 1:Therefore, the answer is .4.Each of her seven friends paid to cover Judi's portion. Therefore, Judi's portion must be . Since Judi was supposed to pay of the total bill, the total bill must be .5.The median here is obviously less than the mean, so option (A) and (B) are out.Lining up the numbers (5, 5, 6, 8, 106), we see that the median weight is 6 pounds.The average weight of the five kids is .Therefore, the average weight is bigger, by pounds, making the answer.6.Solution 1: Working BackwardsLet the value in the empty box in the middle row be , and the value in the empty box in the top row be . is the answer we're looking for.We see that , making .It follows that , so .Solution 2: Jumping Back to the StartAnother way to do this problem is to realize what makes up the bottommost number. This method doesn't work quite as well for this problem, but in a larger tree, it might be faster. (In this case, Solution 1 would be faster since there's only two missing numbers.)Again, let the value in the empty box in the middle row be , and the value in the empty box in the top row be . is the answer we're looking for.We can write some equations:Now we can substitute into the first equation using the two others:7.If Trey saw , then he saw .2 minutes and 45 seconds can also be expressed as seconds.Trey's rate of seeing cars, , can be multiplied by on the top and bottom (and preserve the same rate):. It follows that the most likely number of cars is . Solution 2minutes and seconds is equal to .Since Trey probably counts around cars every seconds, there are groups of cars that Trey most likely counts. Since , the closest answer choice is .8.First, there are ways to flip the coins, in order.The ways to get two consecutive heads are HHT and THH.The way to get three consecutive heads is HHH.Therefore, the probability of flipping at least two consecutive heads is .9.This is a geometric sequence in which the common ratio is 2. To find the jump that would be over a 1000 meters, we note that .However, because the first term is and not , the solution to the problem is10. To find either the LCM or the GCF of two numbers, always prime factorize first.The prime factorization of .The prime factorization of .Then, find the greatest power of all the numbers there are; if one number is one but not the other, use it (this is ). Multiply all of these to get 5940.For the GCF of 180 and 594, use the least power of all of the numbers that are in both factorizations and multiply. = 18.Thus the answer = = .We start off with a similar approach as the original solution. From the prime factorizations, the GCF is .It is a well known fact that . So we have,.Dividing by yields .Therefore, .11. We use that fact that . Let d= distance, r= rate or speed, and t=time. In this case, let represent the time.On Monday, he was at a rate of . So, .For Wednesday, he walked at a rate of . Therefore, .On Friday, he walked at a rate of . So, .Adding up the hours yields + + = .We now find the amount of time Grandfather would have taken if he walked at per day. Set up the equation, .To find the amount of time saved, subtract the two amounts: - = . To convert this to minutes, we multiply by .Thus, the solution to this problem is12. First, find the amount of money one will pay for three sandals without the discount. We have.Then, find the amount of money using the discount: .Finding the percentage yields .To find the percent saved, we have13. Let the two digits be and .The correct score was . Clara misinterpreted it as . The difference between the two is which factors into . Therefore, since the difference is a multiple of 9, the only answerchoice that is a multiple of 9 is .14. The probability that both show a green bean is . The probability that both show a red bean is . Therefore the probability is15.Therefore, .Therefore, .To most people, it would not be immediately evident that , so we can multiply 6's until we get the desired number:, so .Therefore the answer is .16. Solution 1: AlgebraWe multiply the first ratio by 8 on both sides, and the second ratio by 5 to get the same number for 8th graders, in order that we can put the two ratios together:Therefore, the ratio of 8th graders to 7th graders to 6th graders is . Since the ratio is in lowest terms, the smallest number of students participating in the project is .Solution 2: FakesolvingThe number of 8th graders has to be a multiple of 8 and 5, so assume it is 40 (the smallest possibility). Then there are 6th graders and 7th graders. The numbers of students is17. Solution 1The mean of these numbers is . Therefore the numbers are, so the answer isSolution 2Let the number be . Then our desired number is .Our integers are , so we have that.Solution 3Let the first term be . Our integers are . We have,18. Solution 1There are cubes on the base of the box. Then, for each of the 4 layers above the bottom (as since each cube is 1 foot by 1 foot by 1 foot and the box is 5 feet tall, there are 4 feet left), there arecubes. Hence, the answer is .Solution 2We can just calculate the volume of the prism that was cut out of the original box. Each interior side of the fort will be feet shorter than each side of the outside. Since the floor is foot, the height will be feet. So the volume of the interior box is .The volume of the original box is . Therefore, the number of blocks contained inthe fort is .19. If Hannah did better than Cassie, there would be no way she could know for sure that she didn't get the lowest score in the class. Therefore, Hannah did worse than Cassie. Similarly, if Hannah did worse than Bridget, there is no way Bridget could have known that she didn't get the highest in the class.Therefore, Hannah did better than Bridget, so our order is .20.A semicircle has symmetry, so the center is exactly at the midpoint of the 2 side on the rectangle, making the radius, by the Pythagorean Theorem, . The area is .21.The number of ways to get from Samantha's house to City Park is , and the number of ways toget from City Park to school is . Since there's one way to go through City Park (just walking straight through), the number of different ways to go from Samantha's house to City Park to school .22. There are vertical columns with a length of toothpicks, and there are horizontal rows with a length of toothpicks. An effective way to verify this is to try a small case, i.e. a grid of toothpicks.Thus, our answer is .23. Solution 1If the semicircle on AB were a full circle, the area would be 16pi. Therefore the diameter of the first circle is 8. The arc of the largest semicircle would normally have a complete diameter of 17. The Pythagoreantheorem says that the other side has length 15, so the radius is .Solution 2We go as in Solution 1, finding the diameter of the circle on AC and AB. Then, an extended version of the theorem says that the sum of the semicircles on the left is equal to the biggest one, so the area of thelargest is , and the middle one is , so the radius is .24.First let (where is the side length of the squares) for simplicity. We can extend until it hits theextension of . Call this point . The area of triangle then is The area of rectangle is . Thus, our desired area is . Now, the ratio of the shaded area to thecombined area of the three squares is .Let the side length of each square be .Let the intersection of and be .Since , . Since and are vertical angles, they are congruent. We also have by definition.So we have by congruence. Therefore, .Since and are midpoints of sides, . This combined with yields.The area of trapezoid is .The area of triangle is .So the area of the pentagon is .The area of the squares is .Therefore, .Let the intersection of and be .Now we have and .Because both triangles has a side on congruent squares therefore .Because and are vertical angles .Also both and are right angles so .Therefore by AAS(Angle, Angle, Side) .Then translating/rotating the shaded into the position ofSo the shaded area now completely covers the squareSet the area of a square asTherefore, .25. Solution 1The radius of the ball is 2 inches. If you think about the ball rolling or draw a path for the ball (see figure below), you see that in A and C it loses inches, and it gains inches on B.So, the departure from the length of the track means that the answer is . Solution 2The total length of all of the arcs is . Since we want the path from the center, the actual distance will be shorter. Therefore, the only answer choice less than is . Thissolution may be invalid because the actual distance can be longer if the path the center travels is on the outside of the curve, as it is in the middle bump.古希腊哲学大师亚里士多德说：人有两种，一种即“吃饭是为了活着”,一种是“活着是为了吃饭”.一个人之所以伟大，首先是因为他有超于常人的心。

amc82019年23题

AMC82019年第23题是关于篮球比赛得分的问题。

题目中提到，在Euclid高中的篮球赛后，发现队伍总分的4/5是由Alexa获得的，7/10是由Britany获得的，Chelsea得了15分。

队伍中的其他7个队员没有一个得分超过2分。

问其他7个队员获得的总分是多少分？
根据题目，我们可以进行以下分析：
计算Alexa的得分：Alexa的得分占总分的4/5，即(4/5) * 总分。

计算Britany的得分：Britany的得分占总分的7/10，即(7/10) * 总分。

计算Chelsea的得分：Chelsea得了15分。

计算其他7个队员的总得分：总得分- Alexa的得分- Britany的得分- Chelsea的得分。

具体计算过程如下：
总分= Alexa的得分+ Britany的得分+ Chelsea的得分+ 其他7个队员的总得分。

Alexa的得分= (4/5) * 总分。

Britany的得分= (7/10) * 总分。

Chelsea的得分= 15分。

其他7个队员的总得分= 总分- Alexa的得分- Britany的得分- Chelsea的得分。

通过以上计算，我们可以得到其他7个队员获得的总分为5分。

全国中学生生物学联赛试题及答案及美国数学竞赛8年级(AMC8)真题(附答案)(电脑版)

全国中学生生物学联赛试题注意事项：1．所有试题使用2B铅笔在机读卡上作答；2．试题按学科分类，单选和多选题混排，多选题答案完全正确才可得分3．纸质试卷72题，电子试卷48题，共计l20题；4．答题时间120分钟。

一、细胞生物学、生物化学、微生物学16题1．癌细胞与正常细胞的不同之处在于A．癌细胞不能合成DNA B．癌细胞被锁定在细胞周期中的S期C．癌细胞能持续分裂尽管彼此紧密相接D．癌细胞始终处于细胞周期中的分裂期2．人的肌肉组织分为快缩纤维和慢缩纤维两种，快缩纤维负责剧烈运动如举重，短跑，易产生酸痛感觉；慢缩纤维负责慢跑，游泳等有氧运动。

以下关于慢缩纤维和快缩纤维的描述，哪个是正确的A．快缩纤维含有的线粒体多，有氧呼吸能产生大量乳酸和ATP供能B．慢缩纤维含有的线粒体多，有氧呼吸不产生乳酸，产生的ATP也少C．快缩纤维含有的线粒体少，主要依靠糖酵解产生ATP供能，因此产生大量乳酸D．慢缩纤维含有的线粒体多，主要依靠糖酵解产生ATP供能3．在光合作用中参与电子传递的载体是A．叶绿素B．磷酸烯醇式丙酮酸C．NADH D．NADPH4．肽链生物合成时，信号肽A．是线粒体的定位信号B．将新生肽链导入内质网C．控制蛋白质分子的最终构象D．处于肽链的C末端5．原核细胞的特征包括A．无核糖体B．无转录后修饰C．无有氧呼吸D．无染色体6．以下糖类中属于寡糖的有(多选2分)A．甘露糖B．麦芽糖C．半乳糖D．蔗糖E．糖原7．以下关于蛋白质变性后所产生现象的描述不正确的是：A．溶解度降低B．疏水侧链基团被包裹在分子内部C．形成沉淀D．失去结晶能力8．真菌中的半知菌是指。

A．没有光合作用B．菌丝没有横隔C．没有发现有性生殖阶段D．不能运动。

9．关于维生素A的生理功能，下面的描述中哪个是错误的?A．抑制胆碱酯酶活性B．构成视觉细胞感光物质的成分C．参与上皮组织细胞膜糖蛋白合成D．严重缺乏时导致夜盲症10．磷酸戊糖途径的发生部位在A．细胞质B．线粒体C．叶绿体D．细胞膜11．在C4植物循环中，CO2进入叶肉细胞被固定的最初产物是A．甘油酸-3-磷酸B．苹果酸C．草酰乙酸D．丙酮酸12．在等电聚焦电泳过程中，随着蛋白质样品的迁移，电流的变化为A．越变越大，当样品到达其等电点位置时，电流达到最大值B．越变越小，当样品到达其等电点位置时，电流达到最小值，接近于零C．基本不变，为一恒定值D．不确定13．利用酵母菌进行乙醇发酵时若通入氧气，会导致菌株对葡萄糖利用速度降低，甚至停止生成乙醇，这种现象称为A．呼吸抑制效应B．巴斯德效应C．分子氧效应D．葡萄糖效应14．巴斯德设计的曲颈瓶实验，可以(单选1分)A．证明酒是酵母菌发酵获得B．否定自然发生学说的论点C．说明培养基灭菌后的作用D．验证某细菌是不能培养的15．营养缺陷型菌株是指(单选1分)A．不需要添加生长因子可在基础培养基上生长的菌株B．不需要添加生长因子可在丰富培养基上生长的菌株C．因突变需提供某种营养才能在基础培养基上生长的菌株D．因自发或诱发突变而导致的可抵抗环境不良因素的菌株16．以下哪类化合物属于微生物的次生代谢产物(多选2分)A．脂肪B．色素C．抗生素D．蛋白质E．毒素二、植物和动物的解剖、生理、组织和器官18题17．草履虫、水螅、乌贼、蟾蜍受到刺激后，均可从体内发出一些物质以攻击或防御敌害，在他们身体上，发出这些物质的结构是A．刺丝泡、刺细胞、墨囊、耳后腺B．刺丝泡、刺丝囊、外套腔、唾液腺C．表膜泡、刺丝囊、墨囊、唾液腺D．表膜泡、刺细胞、外套腔、耳后腺18．在动物卵裂时期，由于不同动物受精卵内卵黄多少及其在卵内分布的不同，卵裂方式也有很大差异，海胆、沙蚕、昆虫、乌贼的卵裂方式依次分别为(单选1分) A．完全均等卵裂(等裂)、表面卵裂、螺旋形卵裂、盘裂B．螺旋形卵裂、完全均等卵裂(等裂)、盘裂、表面卵裂C．螺旋形卵裂、完全均等卵裂(等裂)、表面卵裂、盘裂D．完全均等卵裂(等裂)、螺旋形卵裂、表面卵裂、盘裂19．不同动物类群具有独特的特征，现存棘皮动物、海绵动物、哺乳动物、鸟类所特有的特征依次为A．水管系、水沟系、下颌为单一齿骨、羽毛B．后口、水沟系、胎生、飞翔C．后口、骨针、胎生、羽毛D．水管系、骨针、下颌为单一齿骨、飞翔20．节肢动物类群很多，不同类群的排泄器官亦有差异，节肢动物门甲壳纲动物的排泄器官有(多选l分)A．基节腺B．触角腺C．颚腺D．马氏管21．家鸽的一侧体动脉弓退化，雌家鸽的一侧卵巢和输卵管也退化了，退化的这些器官是(单选1分)A．左体动脉弓和右侧的卵巢、输卵管B．左体动脉弓和左侧的卵巢、输卵管C．右体动脉弓和左侧的卵巢、输卵管D．右体动脉弓和右侧的卵巢、输卵管22．在海滨潮间带经常可以见到石鳖和沙蚕，以下不属于它们共同特征的是A．以裂体腔法形成真体腔B．后肾型排泄系统C．具有担轮幼虫期D．开管式循环系统23．以下哪项不是文昌鱼的特征A．具有脊索，背神经管，鳃裂B．有分节的肌肉，有哈氏窝C．有头，有心脏D．有特化的口器24．一家饭店涉嫌出售野生鸟类，检查人员在检查时发现了一种鸟类的足，三趾向前一趾向后，后趾与前面三趾在同一平面上，趾长，基部有蹼相连，这种鸟类是A．鹈形目B．鹳形目C．雁形目D．鹤形目25．以下哪组元素在植物体内参与氧化还原反应(单选2分)A．钼镍铜铁B．铁铜镁钼C．钙镁镍铜D．锰镍镁铜26．盐胁迫条件下，较耐盐的禾本科植物大麦可以通过将盐分局域于以下部位来缓解盐分对植物生长造成的危害(多选l分)A．根系B．幼叶C．叶鞘D．老叶27．关于植物的种子，下列描述正确的是(多选2分)A．种子由胚珠发育而来B．种子表面均有种孔、种脐和种脊的结构C．种子中的胚乳多来源于受精后的中央细胞，也有来自于雌配子体的细胞D．胚是休眠的幼小孢子体E．无胚乳种子在发育过程中没有胚乳形成28．有关被子植物花的叙述，下列哪一个是错误的(单选2分)A．花是适应于繁殖功能的变态短枝B．花托、花萼和花冠被认为是叶的变态C．雄蕊和雌蕊也被认为是叶的变态D．花托、花被、雄蕊和雌蕊均有茎的顶端分生组织产生29．玉米干旱缺水时叶片的内卷主要是失水造成的A．叶肉细胞B．叶表皮的毛状体C．位于上表皮的泡状(运动)细胞D．位于下表皮的泡状(运动)细胞30．有关C4植物，以下说法中正确的是(多选2分)A．叶解剖结构中可观察到“花环结构”B．光合作用CO2的初步固定和同化在不同细胞中进行C．光合作用CO2的初步固定和同化在同一细胞中进行D．在一定范围的强光、高温条件下光合效率高31．心肌细胞有效不应期的长短主要取决于A．静息电位水平B．0期去极化的速度C．阈电位水平D．平台期的长短32．血液中CO2分压升高使呼吸运动加强的最主要途径是(单选2分)A．直接刺激脑桥的呼吸相关神经元B．直接刺激延髓呼吸中枢的神经元C．刺激中枢化学感受器D．刺激颈动脉体和主动脉体感受器33．当去甲肾上腺素与β受体结合时，下列哪一种肌肉收缩或收缩加强(单选1分) A．心室肌B．子宫平滑肌C．小肠平滑肌D．血管平滑肌E．支气管平滑肌34．下列哪种因素可引起人尿量的明显增加的(多选2分)A．献血200ml后B．饮用清水1000ml后C．静脉注射神经垂体激素D．饮用生理盐水100ml后三、动物行为学、生态学15题35．如果一项研究，专注于了解不同生态因子对生物的影响，及生物对它们的耐受，那么这个研究属于哪一层次上的研究A．个体生态学B．种群生态学C．群落生态学D．生态系统生态学36．关于高等动物种群中性别比例，下面论述中错误的是A．大多数种群倾向于使出生性比趋近于l：1 B．老年组往往雌性多于雄性C．出生的时候，往往雄性多于雌性D．种群性比与世代长度直接相关37．社会性寄生是指A．寄生在动物社会中是普遍现象B．寄生只发生在特定社会等级的动物中C．社会性昆虫中发生的寄生行为D．强迫寄主动物为其提供食物或其他利益38．关于外来物种，以下论述错误的是A．所有的外来物种都是入侵种，都是有害的B．外来物种可以依靠风、鸟、昆虫等自然因素入侵C．有些外来物种是人类有意引入的D．入侵物种可能对生态系统造成长久的破坏39．适合度是指A．动物单一行为的适应性B．动物调整自己的行为以适合于生活在当时的环境C．动物的总体繁殖成功性D．最适合动物生活习性、满足营养需求的食物40．以下哪种情况不属于动物的行为节律A．候鸟随季节的迁徙B．哺乳动物晨昏活动习性C．细菌生长速度随营养物浓度起落而快慢变化D．招潮蟹的活动随潮汐变化而变化41．动物的生长和发育是需要一定温度的，下列哪个说法是正确的(单选2分) A．外界温度的高低直接决定了动物机体的体温，进而影响其生长发育B．当外界温度低于某一温度时，昆虫就停止生长发育，这一温度阈值称为发育起点温度C．动物的发育速度总是随环境温度的增高而加快的D．昆虫发育的有效积温是发育历期乘以发育期的平均温度，然后求和42．下列有关水生群落演替的说法中哪个是错误的A．水生群落的演替一般会依次经历裸底期、浮水植物期、沉水植物期、挺水植物期、湿生草本植物期等阶段B．在这一演替过程中池底逐渐变浅，最终向陆地变化C．挺水植物根系往往较发达，可以使水底迅速增高D．浮水植物的叶子漂浮在水面，影响到水下光照，不利于沉水植物生长43．关于固定行为型，下述论述正确的是(多选2分)A．固定行为型被特定的外部刺激所释放B．每一个物种都有物种特异的固定行为型C．固定行为型一旦释放就会持续到底D．固定行为型是一种先天行为44．在动物行为学研究中，严格定义行为类型是研究工作的基础。

AMC／AIME美国数学竞赛试题真题

AMC／AIME美国数学竞赛试题真题AMC／AIME美国数学竞赛试题真题考试信息AMC最新考试时间：●2010年第26届AMC8于11月16日，星期二●2011第12届AMC10A，第62届AMC12A 于2月8日，星期二●2011第12届AMC10B，第62届AMC12B 于2月23日，星期三●2011第29届AIME－1于3月17日，星期四2011第29届AIME－2于3月30日，星期三●2009年AMC8考试情况●2008年考试情况AMC/AIME中国历程：1983第1届AIME上海有76名同学获得参赛资格1984年第2届AIME有110人获得参赛资格1985年第3届AIME北京有118名同学获得参赛资格1986年第4届AIME上海有154名同学获得参赛资格，我国首次参加IMO的上海向明中学吴思皓就是在第四届AIME中获得满分1992年第10届AIME上海有一千多名同学获得参赛资格，其中格致中学潘毅明，交大附中张觉，上海中学葛建庆均获满分1993年第11届AIME上海有一千多名同学获得参赛资格，其中华东师大二附中高一王海栋，格致中学高二（女）黄静，市西中学高二张亮，复旦附中高三韩志刚四人获得满分，前三名总分排名复旦附中41分，华东师大二附中41分，上海中学40分。

北京地区参加2006年AMC的共有7所市重点学校的842名学生，有515名学生获得参加AIME资格，其中，清华附中有61名学生参加AMC，45名学生获得AIME资格，20名学生获得荣誉奖章据悉中国大陆以下地区可以报名参加考试：北京地区：中国数学会奥林匹克委员会负责组织实施长春地区、哈尔滨地区也有参加考试在华举办的美国人子弟学校也有参加考试广州地区：《数学奥林匹克报》负责组织实施。

在中国大陆报名者就在中国大陆考试。

考题采用英文版。

2009年AMC中国地区参赛学校一览表more info。

2019AMC8(美国数学竞赛)真题加详解

2019 AMC 8 Problems Problem 1Ike and Mike go into a sandwich shop with a total of to spend. Sandwiches cost each and soft drinks cost each. Ike and Mikeplan to buy as many sandwiches as they can and use the remaining money to buy soft drinks. Counting both soft drinks and sandwiches, how many items will they buy?Solution 1We maximize the number of sandwiches Mike and Ike can buy by finding the lowest multiple of that is less than This number isTherefore, they can buy sandwiches for They spend the remaining money on soft drinks, so they buy soft drinks. Combining the items, Mike and Ike buy soft drinks.The answer isProblem 2Three identical rectangles are put together to form rectangle , as shown in the figure below. Given that the length of the shorter side of each of the smaller rectangles is 5 feet, what is the area in square feet ofrectangle ?Solution 1We know that the length of the shorter side of the 3 identical rectangles are all 5 so we can use that by seeing that the longer side of the right rectangle is the same as 2 of the shorter sides of the other 2 left rectangles. This meansthat which is the longer side of the right rectangle, and because all the rectangles are congruent, we see that each of the rectangles have a longer side of 10 and a shorter side of 5. Now the bigger rectangle has a shorter length of 10(because the shorter side of the bigger rectangle is the bigger side of the shorter rectangle, which is 10) and so the bigger side of the bigger rectangle is the bigger side of the smaller rectangle + the smaller side of the smaller rectangle, which is . Thus, the area is forchoice ~~Saksham27Solution 2Using the diagram we find that the larger side of the small rectangle is 2 times the length of the smaller side. Therefore the longer side is . So the area of the identical rectangles is . We have 3 identical rectangles that form the large rectangle. Therefore the area of the large rectangleis . ~~fath2012Solution 3We see that if the short sides are 5, the long side has tobe because the long side is equal to the 2 short sides and because the rectangles are congruent. If that is to be, then the long side of the BIG rectangle(rectangle ) is because long side +short side of the small rectangle is . The short side ofrectangle is because it is the long side of the short rectangle. Multiplying and together gets us which is .~~mathboy282Problem 3Which of the following is the correct order of thefractions and from least to greatest?Solution 1Consider subtracting 1 from each of the fractions. Our new fractions would then be and . Since , it follows that the answeris-will3145Solution 2We take a commondenominator:Since it follows that the answeris .-xMidnightFirex~ dolphin7 - I took your idea and made it an explanation.Solution 3When and , . Hence, the answeris . ~ ryjsThis is also similar to Problem 20 on the AMC 2012.Problem 4Quadrilateral is a rhombus with perimeter meters. The length of diagonal is meters. What is the area in square meters ofrhombus ?Solution 1A rhombus has sides of equal length. Because the perimeter of the rhombusis , each side is . In a rhombus, diagonals are perpendicular and bisect each other, which means = = .Consider one of the right triangles:= , and = . Using Pythagorean theorem, we findthat = .Thus the values of the two diagonals are = and = . The area of a rhombus is = = =~phoenixfireSolution 2 (Heron's)Since a rhombus has sides ofequal length, . In triangle ABC, and . Using Heron's formula, we have. Simplifying, wehave so. ~~RWhiteProblem 5A tortoise challenges a hare to a race. The hare eagerly agrees and quickly runs ahead, leaving the slow-moving tortoise behind. Confident that he will win, the hare stops to take a nap. Meanwhile, the tortoise walks at a slow steady pace for the entire race. The hare awakes and runs to the finish line, only to find the tortoise already there. Which of the following graphs matches the description of the race, showing the distance traveled by the two animals over time from start to finish?Solution 1 (Using the answer choices)First, the tortoise walks at a constant rate, ruling out Second, when thehare is resting, the distance will stay the same, ruling out and . Third, the tortoise wins the race, meaning that the non-constant one should go off thegraph last, ruling out . Therefore, the answer is the only one left.Problem 6There are grid points (uniformly spaced) in the square shown in the diagram below, including the points on the edges. Point is in the center of the square. Given that point is randomly chosen among the other points, what is theprobability that the line is a line of symmetry for the square?Solution 1Lines of symmetry go through point , and there are directions the lines could go, and there are dots ateach direction.. ~heeeeeheeeeeeeProblem 7Shauna takes five tests, each worth a maximum of points. Her scores on the first three tests are , , and . In order to average for all five tests, what is the lowest score she could earn on one of the other two tests?Solution 1We should notice that we can turn the information we are given into a linear equation and just solve for our set variables. I'll use the variables and for thescores on the last two tests.We can now cross multiply to get rid of thedenominator. Now that we have this equation, we will assign as the lowest score of the two other tests, andso: Now we know that the lowest score on the two othertests is .~ aopsavSolution 2Right now, she scored and points, with a total of points. She wants her average to be for her tests so she needs to score points in total. She needs to score a total of points in her tests. So the minimum score she can get is when one of her scores is . So the least possible score she can get is . ~heeeeeeeheeeeeeNote: You can verify that is the right answer because it is the lowest answer out of the 5. Since it is possible to get 48, we are guaranteed that that is the right answer. ~~ gorefeebuddieSolution 3We can compare each of the scores with the averageof : , , , ;So the last one has to be (since all the differences have to sum to ), which corresponds to .Problem 8Gilda has a bag of marbles. She gives of them to her friend Pedro. Then Gilda gives of what is left to another friend, Ebony. Finally, Gildagives of what is now left in the bag to her brother Jimmy. What percentage of her original bag of marbles does Gilda have left for herself?Solution 1After Gilda gives % of the marbles to Pedro, she has % of the marbles left. If she then gives % of what's left to Ebony, she has = % of what she had at the beginning. Finally, she gives % of what's left to herbrother, so she has . of what she had in the beginning left.~heeeeeeeheeeeeeSolution 2Suppose Gilda has 100 marbles.Then she gives Pedro 20% of 100 = 20, she remains with 80 marbles.Out of 80 marbles she gives 10% of 80 = 8 to Ebony.Thus she remains with 72 marbles.Then she gives 25% of 72 = 18 to Jimmy, finally leaving her with 54.And =54%=~phoenixfireSolution 3 (Only if you have no time, do this method)Since she gave away 20% and 10% of what is left and then another 25% of what is actually left, we can do 20+10+25 or 55%. But it is actually going to be a bit more than 55% because 10% of what is left is not 10% of the total amount. Sothe only option that is greater than 100% - 55% is . ~~ gorefeebuddie Problem 9Alex and Felicia each have cats as pets. Alex buys cat food in cylindrical cans that are cm in diameter and cm high. Felicia buys cat food in cylindrical cans that are cm in diameter and cm high. What is the ratio of the volume one of Alex's cans to the volume one of Felicia's cans?Solution 1Using the formula for the volume of a cylinder, we get Alex, , and Felicia, . We can quickly notice that cancels out on both sides, and thatAlex's volume is of Felicia's leaving as the answer.~aopsavSolution 2Using the formula for the volume of a cylinder, we get that the volume of Alex's can is , and that the volume of Felicia's can is . Nowwe divide the volume of Alex's can by the volume of Felicia's can, so we get , which is ~~SmileKat32Solution 3The ratio of the numbers is . Looking closely at the formula , we see that the will cancel, meaning that the ratio of them willbeProblem 10The diagram shows the number of students at soccer practice each weekday during last week. After computing the mean and median values, Coach discovers that there were actually participants on Wednesday. Which of the followingstatements describes the change in the mean and median after the correction ismade?The mean increases by and the median does not change.The mean increases by and the median increases by .The mean increases by and the median increases by .The mean increases by and the median increases by .The mean increases by and the median increases by .Solution 1On Monday, people come. On Tuesday, people come. On Wednesday, people come. On Thursday, people come. Finally, onFriday, people come. , so themean is . The median is . The coach figures out that actually people come on Wednesday. The new mean is , while the new median is . The median and mean bothchange, so the answer is Another way to compute the change in mean is to notice that the sum increased by with the correction. So the average increased by .Problem 11The eighth grade class at Lincoln Middle School has students. Each student takes a math class or a foreign language class or both. There are eighth graders taking a math class, and there are eight graders taking a foreign language class. How many eighth graders take only a math class and not a foreign language class?Solution 1Let be the number of students taking both a math and a foreign language class.By P-I-E, we get = .Solving gives us .But we want the number of students taking only a math class.Which is .~phoenixfireSolution 2We have people taking classes. However we over-countedthe number of people who take both classes. If we subtract the original amount of people who take classes we get that people took the two classes. To find the amount of people who took only math class web subtract the people who didn'ttake only one math class, so we get -fath2012Solution 3We're basically just finding the number of students not taking a foreign languagesince all the rest would be taking only math.Problem12The faces of a cube are painted in six different colors: red , white ,green , brown , aqua , and purple . Three views of the cube are shown below. What is the color of the face opposite the aqua face?Solution 1is on the top, and is on the side, and is on the right side. That means that (image ) is on the left side. From the third image, you knowthat must be on the bottom since is sideways. That leaves us with the back, so the back must be . The front is opposite of the back, so the answeris .~heeeeeeeheeeeeSolution 2Looking closely we can see that all faces are connected with except for . Thus the answer is .Problem 13A palindrome is a number that has the same value when read from left to right or from right to left. (For example 12321 is a palindrome.) Let be the least three-digit integer which is not a palindrome but which is the sum of three distinct two-digit palindromes. What is the sum of the digits of ?SolutionNote that the only positive 2-digit palindromes are multiples of 11,namely . Since is the sum of 2-digit palindromes, is necessarily a multiple of 11. The smallest 3-digit multiple of 11 which is not a palindrome is 110, so is a candidate solution. We must check that 110 can be written as the sum of three distinct 2-digit palindromes; this sufficesas . Then , and the sum of the digits of is .Problem 14Isabella has coupons that can be redeemed for free ice cream cones at Pete's Sweet Treats. In order to make the coupons last, she decides that she will redeem one every days until she has used them all. She knows that Pete's is closed on Sundays, but as she circles the dates on her calendar, she realizes that no circled date falls on a Sunday. On what day of the week does Isabella redeem her first coupon?Solution 1Let to denote a day where one coupon is redeemed and the day when the second coupon is redeemed.If she starts on a she redeems her next coupon on .to .Thus is incorrect.If she starts on a she redeems her next coupon on .to .to .to .Thus is incorrect.If she starts on a she redeems her next couponon .to .to .to .to .And on she redeems her last coupon.No sunday occured thus is correct.Checking for the other options,If she starts on a she redeems her next coupon on . Thus is incorrect.If she starts on a she redeems her next coupon on .to .to .Checking for the other options gave us negative results, thus the answeris .~phoenixfireSolution 2LetWhich clearly indicates if you start form a you will not geta .Any other starting value may lead to a .Which means our answer is .~phoenixfireSolution 3Like Solution 2, let the days of the week be numbers. and arecoprime, so continuously adding to a number will cycle throughall numbers from to . If a string of 6 numbers in this cycle does not contain , then if you minus 3 from the first number of this cycle, it will always be . So, theanswer is . ~~SmileKat32Solution 4Since Sunday is the only day that has not been counted yet. We can just add the 3 days as it will become . ~~ gorefeebuddie Note: This only works when 7 and 3 are relatively prime.Problem 15On a beach people are wearing sunglasses and people are wearing caps. Some people are wearing both sunglasses and caps. If one of the people wearing a cap is selected at random, the probability that this person is is alsowearing sunglasses is . If instead, someone wearing sunglasses is selected at random, what is the probability that this person is also wearing acap?Solution 1The number of people wearing caps and sunglasses is . So then 14 people out of the 50 people wearing sunglasses also havecaps. ~heeeeeeheeeeeeProblem 16Qiang drives miles at an average speed of miles per hour. How many additional miles will he have to drive at miles per hour to average miles per hour for the entire trip?Solution 1(answer options)The only option that is easily divisible by is . Which gives 2 hours of travel. And by the formulaAnd =ThusBoth are equal and thus our answer is~phoenixfireSolution 2Note that the average speed is simply the total distance over the total time. Let the number of additional miles he has to drive be Therefore, the total distanceis and the total time (in hours) is We canset up the following equation: Simplifying the equation, we get Solving the equation yields so ouranswer is .~twinemmaSolution 3If he travels miles at a speed of miles per hour, he travels for 30 min. Average rate is total distance over total timeso , where d is the distance left to travel and t is the time to travel that distance. solve for to get . you also know that he has to travel miles per hour for some time, so plug that in for d to get and andsince , the answer is . Problem 17What is the value of theproductSolution 1We rewrite:The middle terms cancel, leaving us with~phoenixfireSolution 2If you calculate the first few values of the equation, all of the values tend to , but are not equal to it. The answer closest to but not equal to itis .~heeeeeeeheeeeeSolution 3Rewriting the numerator and the denominator, we get . We can simplify by canceling 99! on both sides, leaving us with: Werewrite as and cancel , which gets . Answer B. Problem 18The faces of each of two fair dice are numbered , , , , , and . When the two dice are tossed, what is the probability that their sum will be an even number?Solution 1The approach to this problem: There are two cases in which the sum can be an even number: both numbers are even and both numbers are odd. This results in only one case where the sum of the numbers are odd (one odd and one even in any order) . We can solve for how many ways the numbers add up to an odd number and subtract the answer from .How to solve the problem: The probability of getting an odd number firstis . In order to make the sum odd, we must select an even number next. The probability of getting an even number is . Now we multiply the twofractions: . However, this is not the answer because we could pick an even number first then an odd number. The equation is the same except backward and by the Communitive Property of Multiplication, the equations are itdoes not matter is the equation is backward or not. Thus we do . This is the probability of getting an odd number. In order to get the probability ofgetting an even number we do- ViratKohli2018 (VK18)Solution 2We have a die with evens and odds on both dies. For the sum to be even, the rolls must consist of odds or evens.Ways to roll odds (Case ): The total number of ways to roll oddsis , as there are choices for the first odd on the first rolland choices for the second odd on the second roll.Ways to roll evens (Case ): Similarly, we have ways toroll evens.Totally, we have ways to roll dies.Therefore the answer is , or .~A1337h4x0rSolution 3 (Complementary Counting)We count the ways to get an odd. If the sum is odd, then we must have an evenand an odd. The probability of an even is , and the probability of an odd is . We have to multiply by because the even and odd can be in any order. Thisgets us , so the answer is . - juliankuang Solution 4To get an even, you must get either 2 odds or 2 evens. The probability of getting 2 odds is . The probability of getting 2 evens is . If you add them together, you get =Problem 19In a tournament there are six teams that play each other twice. A teamearns points for a win, point for a draw, and points for a loss. After all the games have been played it turns out that the top three teams earned the same number of total points. What is the greatest possible number of total points for each of the top three teams?Solution 1After fully understanding the problem, we immediately know that the three top teams, say team , team , and team , must beat the other threeteams , , . Therefore, ,, must eachobtain points. However, they play against each teamtwice, for a total of points against , , and . For gamesbetween , , , we have 2 cases. In both cases, there is an equality of points between , , and .Case 1: A team ties the two other teams. For a tie, we have 1 point, so wehave points (they play twice). Therefore, this case brings a total of points.Case 2: A team beats one team while losing to another. This gives equality, as each team wins once and loses once as well. For a win, we have points, so a team gets points if they each win a game and lose a game. Thiscase brings a total of points.Therefore, we use Case 2 since it brings the greater amount of points, or , so the answer is .~A1337h4x0rNote that case 2 can be easily seen to be better as follows. Let be the number of points gets, be the number of points gets, and be the number of points gets. Since , to maximize , we can just maximize . But in each match, if one team wins then the total sum increases by points, whereas if they tie, the total sum increasesby points. So it is best if there are the fewest ties possible.Solution 2(1st match(3) + 2nd match(1)) * number of teams(6) = 24, .Explanation: So after reading the problem we see that there are 6 teams and each team versus each other twice. This means one of the two matches has to be a win, so 3 points so far. Now if we say that the team won again and make it 6 points, that would mean that team would be dominating the leader-board and the problem says that all the top 3 people have the same score. So that means the maximum amount of points we could get is 1 so that each team gets the same amount of matches won & drawn so that adds up to 4. 4 * the number of teams(6)= 24 so the answer isProblem 20How many different real numbers satisfy the equationSolutionWe have that if and only if .If , then , giving 2 solutions.If , then , giving 2 more solutions. All four of these solutions work, so the answer is . Further,the equation is a quartic in , so by the Fundamental Theorem of Algebra, there can be at most four real solutions.Problem 21What is the area of the triangle formed by the lines , , and ?Solution 1You need to first find the coordinates where the graphs intersect. ,and intersect at . , and intersect at . and intersect at . Usingthe Shoelace Theorem you get ==.~heeeeeeheeeeeSolution 2Graphing the lines, we can see that the height of the triangle is 4, and the base is 8. Using the formula for the area of a triangle, we get which is equalto . ~SmileKat32Problem 22A store increased the original price of a shirt by a certain percent and then lowered the new price by the same amount. Given that the resulting pricewas of the original price, by what percent was the price increased and decreased?Solution 1Suppose the fraction of discount is . Thatmeans ; so ,and , obtaining . Therefore, the price was increased and decreased by %, or .Solution 2(Answer options)Let the price be 100 and then trying for each option leads to .-phoenixfireSolution 3Let x be the discount. We can also work in reverse such as ()= .Thus = . Solving for givesus . But has to be positive. Thus = .Problem 23After Euclid High School's last basketball game, it was determined that of theteam's points were scored by Alexa and were scored by Brittany. Chelsea scored points. None of the other team members scored more than points What was the total number of points scored by the other team members?Solution 1Since and are integers, wehave . We see that the number of points scored by the other team members is less than or equal to and greater than or equal to . We let the total number of points be and the total number of points scored by the other team members, which meansthat, which means . The only value of that satisfies all conditions listed is , so . - juliankuang (lol im smart.) Solution 2Starting from the above equation where is the total number of points scored and is the number of points scored by the remaining 7 team members, we can simplify to obtain the Diophantineequation , or . Since is necessarily divisible by 28, let where and divide by 28 to obtain . Then it is easy to see () is the only candidate, giving . -scrabbler94Solution 3Fakesolve: We first start by setting the total number of points as ,since . However, we see that this does not work since we surpass the number of points just with the information given(). Next, we assume that the total number of points scored is . With this, we have that Alexa, Brittany, and Chelseascore: , and thus, the other seven players would have scored a total of (We see that this workssince we could have of them score points, and the other of themscore point) -aops5234Problem 24In triangle , point divides side sothat . Let be the midpoint of and let be thepoint of intersection of line and line . Given that the areaof is , what is the area of ?Solution 1Draw on such that is parallel to . That makestriangles and congruentsince . so .Since ( and ,so ), the altitude of triangle is equal to of the altitude of . The area of is , so the areaof ~heeeeeeeheeeee Solution 2 (Mass Points)First, when we see the problem, we see ratios, and we see that this triangle basically has no special properties (right, has medians, etc.) and this screams mass points at us.First, we assign a mass of to point . We figure out that has a massof since . Then, by adding , we get thatpoint has a mass of . By equality, point has a mass of also.Now, we add for point and for point .Now, is a common base for triangles and , so we figure out that the ratios of the areas is the ratios of the heights whichis . So, 's area is one third the area of , and weknow the area of is the area of since they have the same heights but different bases.So we get the area of as -BrudderNote: We can also find the ratios of the areas using the reciprocal of the product of the mass points of over the product of the mass pointsof which is which also yields -BrudderSolution 3is equal to . The area oftriangle is equal to because it is equal to on half of the area of triangle , which is equal to one third of the area of triangle , which is . The area of triangle is the sum of the areas oftriangles and , which is respectively and . So, is equal to =, so the area of triangle is . That minus the area of triangle is . ~~SmileKat32Solution 4 (Similar Triangles)Extend to such that asshown:Then and .Since , triangle has four times the area oftriangle . Since , we get .Since is also , we have becausetriangles and have the same height and same areas and so their bases must be the congruent. Thus triangle has twice the side lengths and therefore four times the area of triangle ,giving .(Credit to MP8148 for the idea)Solution 5 (Area Ratios)As before we figure out the areas labeled in the diagram. Then we notethat Solving gives . (Credit to scrabbler94 for the idea)Solution 6 (Coordinate Bashing)Let be a right triangle, andLetThe line can be described with the equationThe line can be described withSolving, we get andNow we can find-Trex4daysSolution 7Let =(the median divides the area of the triangle into two equal parts)Construction: Draw a circumcircle around with as is diameter. Extend to such that it meets the circle at . Draw line .(Since is cyclic)But is common in both with an area of 60.So, .\therefore (SAS Congruency Theorem).In , let be the median of .Which meansRotate to meet at and at . will fit exactlyin (both are radii of the circle). From the above solutions, .is a radius and is half of it implies = .Which meansThus~phoenixfire & flamewavelightSolution 8Using the ratio of and , we find the area of is and the areaof is . Also using the fact that is the midpoint of , we know . Let be a point such is parellel to . We immediatley know that by . Using that we can conclude has ratio .Using , we get . Therefore using the fact that is in , the area hasratio and we know hasarea so is . - fath2012Solution 9Labeling the areas in the diagram, we have:so .So our answer is . ~~RWhiteSolution 10 (Menelaus's Theorem)By Menelaus's Theorem on triangle , wehaveTherefore,Solution 11 (Graph Paper)Note: If graph paper isunavailable, this solution can still be used by constructing a small grid on a sheet of blank paper.As triangle is loosely defined, we can arrange its points such that the diagram fits nicely on a coordinate plane. By doing so, we can construct it on graph paper and be able to visually determine the relative sizes of the triangles. As point splits line segment in a ratio, we draw as avertical line segment units long. Point is thus unit belowpoint and units above point . By definition, Point splits linesegment in a ratio, so we draw units long directly leftof and draw directly between and , unit away from both.We then draw line segments and . We can easily tell thattriangle occupies square units of space. Constructing line and drawing at the intersection of and , we can easily see thattriangle forms a right triangle occupying of a square unit of space.。

美国数学竞赛AMC8 -- 2008年真题解析(英文解析+中文解析)

美国数学竞赛AMC8 – 2008年真题解析(英文解析+中文解析)Problem 1Answer: BSolution:50-12-24=14中文解析：总共花的钱是：12+12*2=36元。

剩余50-36=14元。

答案是BProblem 2Answer: ASolution:We can derive that c=8,L=6, U=7,and E=1. Therefore, the answer is 8671.中文解析：这10个字母的对应关系是： B -0；E-1; S-2; ......K -9. 按照这个对应关系：C-8，L-6，U-7，E-1. 即8671. 答案是A。

Problem 3Answer: ASolution:We can go backwards by days, but we can also backwards by weeks. If we go backwards by weeks, we see that February 6 is a Friday. If we now go backwards by days, February 1 is a Sunday.中文解析：13日是周五，则13-7=6，即6日也是周五，则倒推2月1日是周日。

答案是A。

Problem 4Answer: CSolution:The area outside the small triangle but inside the large triangle is 16-1=15. This is equally distributed between the three trapezoids. Each trapezoid has an area of 15/3=5.中文解析：大三角形的面积等于小的等边三角形的面积加上3个梯形的面积。

据此，三个梯形的面积是16-1=15. 每个梯形的面积是15/3=5. 答案是C。

2019年ACT数学真题

2019年ACT数学真题（正文）ACT（全称American College Testing，美国大学入学测试）是美国高中生们广泛参加，用于评估他们在英语、数学、阅读和科学分析等方面的学术能力。

本文将为您提供2019年ACT数学部分的真题，采用合适的格式以帮助您更好地理解和解决问题。

请注意，以下问题没有按照传统的小节和标题来组织。

而是根据每个问题的内容，逐一进行的解答。

希望这种形式对您的阅读体验有所帮助。

1. 第一道问题问题描述：在一个圆形游泳池中，雷切尔跳入跳板后以15米/秒的速度水平方向游过地面，最终在水中停下。

如果水中的阻力对她的影响可忽略不计，那么她跳下跳板时离地面多高？解答：由于雷切尔以水平方向从跳板的高度跳入水中，所以在垂直方向上没有受到阻力。

因此，她的垂直速度会保持不变。

我们可以利用这个概念，结合动能定理来解决这个问题。

假设跳板的高度为h，雷切尔在水中停下后，她的速度为0。

根据动能定理，可以得到以下等式：初速度的平方 + 2 * 加速度 * 距离 = 终速度的平方0 + 2 * 9.8 * h = 0由上式可得：19.6h = 0解得：h = 0因此，跳板离地面的高度为0米。

2. 第二道问题问题描述：一个等边三角形的边长为6个单位。

求其内切圆的半径。

解答：对于等边三角形来说，内切圆的半径等于三角形的高的一半。

我们可以利用三角形的特性来求解。

一个等边三角形的高可以通过连接三角形的顶点和底边的中点来得到。

由于这是一个等边三角形，所以这条线段是等边三角形的高，也是其内切圆的半径的垂直。

通过计算，我们可以得出垂直的长度为3√3。

因此，内切圆的半径为3√3/2个单位。

（在下面继续解答更多问题...）通过以上两个问题的解答，我们可以看到，ACT数学部分的真题在不同的领域（如几何、代数、数据分析等）都考察了不同的问题和解决方法。

这也是ACT数学部分考察学生的全面能力和解决问题的思维方式。

希望本文对您复习和理解2019年ACT数学真题有所帮助。

第19届美国数学奥林匹克试题解答

第19届美国数学奥林匹克试题解答单墫;刘亚强;学韩冰【期刊名称】《中等数学》【年(卷),期】1991(0)1【摘要】1.至多可以发行105个.我们可以造出105个互不相同的五位数证号a1a2a3a4a 5.第6位数字 a6规定为和a1+a2十a3+a4+a5的个位数字.这时,如果两个号码的前5位数字a1a2a3a4a 5与a1′a2′a3′a4′a5′中仅有一处不同,那么第6位【总页数】2页(P1-2)【作者】单墫;刘亚强;学韩冰【作者单位】[1]南京师范大学;[2]南京师范大学;[3]南开大学【正文语种】中文【中图分类】G633.6【相关文献】1.第四届女子数学奥林匹克试题解答 [J], 朱华伟（供稿）2.2006年中国数学奥林匹克（第21届全国中学生数学冬令营）试题解答 [J],3.第46届国际数学奥林匹克（IMO）试题解答 [J], 王建伟4.第2届中国东南地区数学奥林匹克试题解答 [J], 吴伟朝5.2004年中国数学奥林匹克冬令营试题解答 [J], 陶平生因版权原因，仅展示原文概要，查看原文内容请购买。

2019AMC8（美国数学竞赛）题目

2019AMC8（美国数学竞赛）题⽬2019 AMC 8 Problems Problem 1Ike and Mike go into a sandwich shop with a total of to spend. Sandwiches cost each and soft drinks cost each. Ike and Mike plan to buy as many sandwiches as they can and use the remaining money to buy soft drinks. Counting both soft drinks and sandwiches, how many items will they buy?Problem 2Three identical rectangles are put together to form rectangle , as shown in the figure below. Given that the length of the shorter side of each of the smaller rectangles is feet, what is the area in square feet ofrectangle ?Problem 3Which of the following is the correct order of the fractions , , and , from least to greatest?Problem 4Quadrilateral is a rhombus with perimeter meters. The length of diagonal is meters. What is the area in square meters of rhombus ?Problem 5A tortoise challenges a hare to a race. The hare eagerly agrees and quickly runs ahead, leaving the slow-moving tortoise behind. Confident that he will win, the hare stops to take a nap. Meanwhile, the tortoise walks at a slow steady pace for the entire race. The hare awakes and runs to the finish line, only to find the tortoise already there. Which of the following graphs matches the description of the race, showing the distance traveled by the two animals over time from start to finish?Problem 6There are grid points (uniformly spaced) in the square shown in the diagram below, including the points on the edges. Point is in the center of the square. Given that point is randomly chosen among the other points, what is theprobability that the line is a line of symmetry for the square?Problem 7Shauna takes five tests, each worth a maximum of points. Her scores on the first three tests are , , and . In order to average for all five tests, what is the lowest score she could earn on one of the other two tests?Problem 8Gilda has a bag of marbles. She gives of them to her friend Pedro. Then Gilda gives of what is left to another friend, Ebony. Finally, Gildagives of what is now left in the bag to her brother Jimmy. What percentage of her original bag of marbles does Gilda have left for herself?Problem 9Alex and Felicia each have cats as pets. Alex buys cat food in cylindrical cans that are cm in diameter and cm high. Felicia buys cat food in cylindrical cans that are cm in diameter and cm high. What is the ratio of the volume one of Alex's cans to the volume one of Felicia's cans?Problem 10The diagram shows the number of students at soccer practice each weekday during last week. After computing the mean and median values, Coach discovers that there were actually participants on Wednesday. Which of the followingstatements describes the change in the mean and median after the correction ismade?The mean increases by and the median does not change.The mean increases by and the median increases by .The mean increases by and the median increases by .The mean increases by and the median increases by .The mean increases by and the median increases by .Problem 11The eighth grade class at Lincoln Middle School has students. Each student takes a math class or a foreign language class or both. There are eighth graders taking a math class, and there are eight graders taking a foreign language class. How many eight graders take only a math class and not a foreign language class?The faces of a cube are painted in six different colors: red , white ,green , brown , aqua , and purple . Three views of the cube are shown below. What is the color of the face opposite the aqua face?Problem 13A palindrome is a number that has the same value when read from left to right or from right to left. (For example, 12321 is a palindrome.) Let be the least three-digit integer which is not a palindrome but which is the sum of three distinct two-digit palindromes. What is the sum of the digits of ?Problem 14Isabella has coupons that can be redeemed for free ice cream cones at Pete's Sweet Treats. In order to make the couponslast, she decides that she will redeem one every days until she has used them all. She knows that Pete's is closed on Sundays, but as she circles the dates on her calendar, she realizes that no circled date falls on a Sunday. On what day of the week does Isabella redeem her first coupon?On a beach people are wearing sunglasses and people are wearing caps. Some people are wearing both sunglasses and caps. If one of the people wearing a cap is selected at random, the probability that this person is is alsowearing sunglasses is . If instead, someone wearing sunglasses is selected at random, what is the probability that this person is also wearing a cap?Problem 16Qiang drives miles at an average speed of miles per hour. How many additional miles will he have to drive at miles per hour to average miles per hour for the entire trip?Problem 17What is the value of theproductProblem 18The faces of each of two fair dice are numbered , , , , , and . When the two dice are tossed, what is the probability that their sum will be an even number?Problem 19In a tournament there are six teams that play each other twice. A teamearns points for a win, point for a draw, and points for a loss. After all the games have been played it turns out that the top three teams earned the same number of total points. What is the greatest possible number of total points for each of the top three teams?Problem 20How many different real numbers satisfy the equationProblem 21What is the area of the triangle formed by the lines , , and ?Problem 22A store increased the original price of a shirt by a certain percent and then decreased the new price by the same amount. Given that the resulting pricewas of the original price, by what percent was the price increased and decreased?Problem 23After Euclid High School's last basketball game, it was determined that of theteam's points were scored by Alexa and were scored by Brittany. Chelsea scored points. None of the other team members scored morethan points. What was the total number of points scored by the other team members?Problem 24In triangle , point divides side sothat . Let be the midpoint of and let be thepoint of intersection of line and line . Given that the areaof is , what is the area of ?Problem 25Alice has apples. In how many ways can she share them with Becky and Chris so that each of the people has at least apples?。

AMC8(美国数学竞赛)历年真题、答案及中英文解析

AMC8（美国数学竞赛）历年真题、答案及中英文解析艾蕾特教育的AMC8 美国数学竞赛考试历年真题、答案及中英文解析：AMC8-2020年：真题 --- 答案---解析（英文解析+中文解析）AMC8 - 2019年：真题----答案----解析（英文解析+中文解析）AMC8 - 2018年：真题----答案----解析（英文解析+中文解析）AMC8 - 2017年：真题----答案----解析（英文解析+中文解析）AMC8 - 2016年：真题----答案----解析（英文解析+中文解析）AMC8 - 2015年：真题----答案----解析（英文解析+中文解析）AMC8 - 2014年：真题----答案----解析（英文解析+中文解析）AMC8 - 2013年：真题----答案----解析（英文解析+中文解析）AMC8 - 2012年：真题----答案----解析（英文解析+中文解析）析）AMC8 - 2010年：真题----答案----解析（英文解析+中文解析）AMC8 - 2009年：真题----答案----解析（英文解析+中文解析）AMC8 - 2008年：真题----答案----解析（英文解析+中文解析）AMC8 - 2007年：真题----答案----解析（英文解析+中文解析）AMC8 - 2006年：真题----答案----解析（英文解析+中文解析）AMC8 - 2005年：真题----答案----解析（英文解析+中文解析）AMC8 - 2004年：真题----答案----解析（英文解析+中文解析）AMC8 - 2003年：真题----答案----解析（英文解析+中文解析）AMC8 - 2002年：真题----答案----解析（英文解析+中文解析）AMC8 - 2001年：真题----答案----解析（英文解析+中文解析）AMC8 - 2000年：真题----答案----解析（英文解析+中文解析）析）AMC8 - 1998年：真题----答案----解析（英文解析+中文解析）AMC8 - 1997年：真题----答案----解析（英文解析+中文解析）AMC8 - 1996年：真题----答案----解析（英文解析+中文解析）AMC8 - 1995年：真题----答案----解析（英文解析+中文解析）AMC8 - 1994年：真题----答案----解析（英文解析+中文解析）AMC8 - 1993年：真题----答案----解析（英文解析+中文解析）AMC8 - 1992年：真题----答案----解析（英文解析+中文解析）AMC8 - 1991年：真题----答案----解析（英文解析+中文解析）AMC8 - 1990年：真题----答案----解析（英文解析+中文解析）AMC8 - 1989年：真题----答案----解析（英文解析+中文解析）AMC8 - 1988年：真题----答案----解析（英文解析+中文解析）析）AMC8 - 1986年：真题----答案----解析（英文解析+中文解析）AMC8 - 1985年：真题----答案----解析（英文解析+中文解析）◆AMC介绍◆AMC（American Mathematics Competitions) 由美国数学协会（MAA）组织的数学竞赛，分为 AMC8 、 AMC10、 AMC12 。

AMC8数学竞赛试题及详解

Problem 3What is the value of the expression ?化简的标准和顺序Problem 6If the degree measures of the angles of a triangle are in the ratio , what is the degree measure of the largest angle of the triangle?角度制与弧度制Problem 7Let be a 6-digit positive integer, such as 247247, whose first three digits are the same as its last three digits taken in the same order. Which of the following numbers must also be a factor of ?整除特征Problem 10A box contains five cards, numbered 1, 2, 3, 4, and 5. Three cards are selected randomly without replacement from the box. What is the probability that 4 is the largest value selected?离散概率Problem 11A square-shaped floor is covered with congruent square tiles. If the total number of tiles that lie on the two diagonals is 37, how many tiles cover the floor?tiles英[taɪlz]美[taɪlz]n. 瓦片，瓷砖( tile的名词复数); 扁平的小棋子;Problem 13Peter, Emma, and Kyler played chess with each other. Peter won 4 games and lost 2 games. Emma won 3 games and lost 3 games. If Kyler lost 3 games, how many games did he win?Problem 14Chloe and Zoe are both students in Ms. Demeanor's math class. Last night they each solved half of the problems in their homework assignment alone and then solved the other half together. Chloe had correct answers to only of the problems she solved alone, but overall of her answers were correct. Zoe had correct answersto of the problems she solved alone. What was Zoe's overall percentage of correct answers? C设数Problem 15In the arrangement of letters and numerals below, by how many different paths can one spell AMC8? Beginning at the A in the middle, a path allows only moves from oneletter to an adjacent (above, below, left, or right, but not diagonal) letter. One example of such a path is traced in the picture.乘法原理加法原理Problem 16In the figure below, choose point on so that and have equal perimeters. What is the area of ?D如果在AC上呢Problem 17Starting with some gold coins and some empty treasure chests, I tried to put 9 gold coins in each treasure chest, but that left 2 treasure chests empty. So instead I put 6 gold coins in each treasure chest, but then I had 3 gold coins left over. How many gold coins did I have?盈亏问题图示法Problem 18In the non-convex quadrilateral shown below, is a rightangle, , , , and .What is the area of quadrilateral ?勾股定理与逆定理“凸”定义Problem 19For any positive integer , the notation denotes the product of the integers through . What is the largest integer for which is a factor of the sum ?Problem 20An integer between and , inclusive, is chosen at random. What is the probability that it is an odd integer whose digits are all distinct?5*8*8*7Problem 21Suppose , , and are nonzero real numbers, and . What are the possible value(s) for ?可能性列全或者变个形Problem 22In the right triangle , , , and angle is a right angle. A semicircle is inscribed in the triangle as shown. What is the radius of the semicircle?相切，连接切点和圆心。

美国数学竞赛AMC8 -- 2008年真题解析(英文解析+中文解析)

美国数学竞赛AMC8 – 2008年真题解析(英文解析+中文解析)Problem 1Answer: BSolution:50-12-24=14中文解析：总共花的钱是：12+12*2=36元。

剩余50-36=14元。

答案是A。

据此，三个梯形的面积是16-1=15. 每个梯形的面积是15/3=5. 答案是C。

美国数学大联盟杯赛五年级试卷

2018-2019年度美国“数学大联盟杯赛”(中国赛区)初赛(五年级)(初赛时间：2018年11月14日,考试时间90分钟,总分200分)学生诚信协议：考试期间,我确定没有就所涉及的问题或结论,与任何人、用任何方式交流或讨论,我确定以下的答案均为我个人独立完成的成果,否则愿接受本次成绩无效的处罚。

如果您同意遵守以上协议请在装订线内签名选择题：每小题5分,答对加5分,答错不扣分,共200分,答案请填涂在答题卡上。

1. A 6 by 6 square has the same area as a 4 by ? rectangle.A) 3 B) 6 C) 8 D) 92.Every prime has exactly ? positive divisors.A) 1 B) 2 C) 3 D) 4 or more3.If I answered 34 out of 40 questions on my math test correctly, I answered ? % of thequestions correctly.A) 75 B) 80 C) 85 D) 904.120 ÷ 3 ÷ 4 × 12 =A) 1 B) 10 C) 12 D) 1205.10 × 20 × 30 × 40 = 24 ×?A) 1000 B) 10 000 C) 100 000 D) 1000 0006.One of my boxes contains 1 pencil and the others each contain 5 pencils. If there are 101pencils in my boxes, how many boxes do I have?A) 19 B) 20 C) 21 D) 227.An electrical company imports 2016 light bulbs. Unfortunately, 25%of those are damaged. How many light bulbs are not damaged?A) 25 B) 504 C) 1512 D) 20168.50 × (16 + 24) is the square ofA) -40 B) -4 C) 4 D) 809.Which of the following numbers has exactly 3 positive divisors?A) 49 B) 56 C) 69 D) 10010.Ten people stand in a line. Counting from the left, Jerry stands at the 5th position.Counting from the right, which position is he at?A) 4 B) 5 C) 6 D) 7 11.On a teamwork project, Jack contributed 2/7 of the total amount of work, Jill contributed1/4 of the work, Pat contributed 1/5 of the work, and Matt contributed the rest. Whocontributed the most toward this project?A) Jack B) Jill C) Pat D) Matt12.Which of the following numbers is a factor of 2016?A) 5 B) 11 C) 48 D) 9913.2 × 4 × 8 × 16 × 32 × 64 =A) 210B) 215C) 221D) 212014.On a game show, Al won four times as much as Bob, and Bob won four times as much asCy. If Al won $1536, how much did Al, Bob, and Cy win together?A) $96 B) $384 C) $1920 D) $201615.The sum of two composites cannot beA) odd B) even C) 11 D) 1716.If a and b are positive integers such that a/b = 5/7, then a + b isA) 12 B) 24 C) 36 D) not able to be determined17.What is the greatest odd factor of the number of hours in all the days of the year 2015?A) 3 B) 365 C) 1095 D) 328518.If the current month is February, what month will it be 1 199 999 months from now?A) January B) February C) March D) April19.Two angles are complementary. One of these angles is 36° less than the other. What is themeasure of the larger angle?A) 36°B) 54°C) 63°D) 72°20.(The square root of 16) + (the cube root of 64) + (the 4th root of 256) =A) 12 B) 24 C) 32 D) 6421.In ∆ABC, m∠A–m∠B = m∠B–m∠C. What is the degree measure of ∠B?A) 30 B) 60 C) 90 D) 12022.For every 3 math books I bought, I bought 2 biology books. I bought 55 books in all. Howmany of those are math books?A) 11 B) 22 C) 33 D) 4423.John wrote a number whose digits consists entirely of 1s. This number was a compositenumber. His number could contain exactly ? 1s.A) 17 B) 19 C) 29 D) 3224.Weird Town uses three types of currencies: Cons, Flegs, and Sels. If 3 Sels = 9 Cons and 2Cons = 4 Flegs, then 5 Sels = ? Flegs.A) 12 B) 24 C) 30 D) 36第1页,共4页25.If the length of a rectangular prism with volume V is doubled while the width and theheight are halved, the volume of the new prism will beA) 4V B) V/2 C) V D) 2V26.Rick and Roy each stands at different ends of a straight road that is 64 m long. They runtoward each other. Rick’s speed is 3 m/s and Roy’s speed is 5 m/s. They will meet in? seconds.A) 1 B) 2 C) 4 D) 827.If the area of a certain circle is 2016, its radius isA) sqrt(2016/π) B) sqrt(4032/π)C) 2016/πD) 1008/π28.In a toy shop, the cost of a Teddy Bear is 200% as much as that of a toy train. The cost ofa toy train is 6/5 the cost of a pack of the wooden blocks. The cost of a pack of woodenblocks is $50. What is the cost, in dollars, of the Teddy Bear?A) 60 B) 100 C) 120 D) 20029.In the sequence 2016, 225, 141, 66, 432, 99, 1458 …, each term after the first term is thesum of the cubes of the digits of the previous term. What is the 100th term of thissequence?A) 153 B) 351 C) 370 D) 37130.What is the sum of all the positive divisors of 210?A) 210 – 1 B) 211 – 1 C) 212 – 1 D) 213 – 131.It takes 4 hours for Mike and Lucy to finish a task. It takes Lucy and Jerry 5 hours tofinish the same task. And it takes 6 hours for Mike and Jerry to finish the same task. Lucy and Jerry first work on the task for 1 hour and 45 minutes. Then Mike takes over the task on his own. How many more hours does it take for Mike to finish the task?A) 3 B) 4 C) 5 D) 632.If you sell a cloth at its current price, you get $40 profit. The total profit you get selling 10clothes at 70% of its current price is equal to the total profit you get selling 20 clothes at $82 per cloth. What is the current price of a cloth?A) 80 B) 100 C) 120 D) 12533.There are 6 identical squares in the figure on the right. The side length of eachsquare is 1. Of all the triangles constructed by connecting three of the 18vertices in the figure, how many of them are triangles whose area is 2 andwhich has at least one vertical or horizontal side?A) 12 B) 16 C) 24 D) 2834.Pick up N numbers from 1 to 2015 inclusively, such that the sum of any three of the Nnumbers is divisible by 24. What is the maximum value of N?A) 83 B) 84 C) 168 D) 252 35.汤姆有一件花了64美金买来的衬衫,他打算以比原价高出25%的价格出售,他会卖出多少钱？A) $16 B) $32 C) $48 D) $8036.满足以下条件的最小整数是多少：“除以3余2,除以5余4,除以7余6。

AMC美国数学竞赛AMCB试题及答案解析

AMC美国数学竞赛AMCB试题及答案解析2003A M C10 B 1、Which of the following is the same as2、Al gets the disease algebritis and must take one green pill and one pink pill each day for two weeks. A green pill costs more than a pink pill, and Al’s pills cost a total of for the two weeks. How much does one green pill cost?3、The sum of 5 consecutive even integers is less than the sum of the ?rst consecutive odd counting numbers. What is the smallest of the even integers?4、Rose fills each of the rectangular regions of her rectangular flower bed with a different type of flower. The lengths, in feet, of the rectangular regions in her flower bed are as shown in the ?gure. She plants one flower per square foot in each region. Asters cost 1 each, begonias each, cannas 2 each, dahlias each, and Easter lilies 3 each. What is the least possible cost, in dollars, for her garden?5、Moe uses a mower to cut his rectangular -foot by -foot lawn. The swath he cuts is inches wide, but he overlaps each cut by inches tomake sure that no grass is missed. He walks at the rate of feet per hour while pushing the mower. Which of the following is closest to the number of hours it will take Moe to mow his lawn?.6、Many television screens are rectangles that are measured by the length of their diagonals. The ratio of the horizontal length to the height in a standard television screen is . The horizontal length of a “-inch” television screen is closest, in inches, to which of the following?7、The symbolism denotes the largest integer not exceeding . For example. , and . Compute.8、The second and fourth terms of a geometric sequence are and . Which of the following is a possible first term?9、Find the value of that satisfies the equation10、Nebraska, the home of the AMC, changed its license plate scheme. Each old license plate consisted of a letter followed by four digits. Each new license plate consists of three letters followed by three digits. By how many times is the number of possible license plates increased?11、A line with slope intersects a line with slope at the point . What is the distance between the -intercepts of these two lines?12、Al, Betty, and Clare split among them to be invested in different ways. Each begins with a different amount. At the end of one year they have a total of . Betty and Clare have both doubled their money, whereas Al has managed to lose . What was Al’s origin al portion?.13、Let denote the sum of the digits of the positive integer . For example, and . For how many two-digit values of is ?14、Given that , where both and are positive integers, find the smallest possible value for .15、There are players in a singles tennis tournament. The tournament is single elimination, meaning that a player who losesa match is eliminated. In the first round, the strongest players are given a bye, and the remaining players are paired off to play. After each round, the remaining players play in the next round. The match continues until only one player remains unbeaten. The total number of matches played is16、A restaurant offers three desserts, and exactly twice as many appetizers as main courses. A dinner consists of an appetizer, a main course, and a dessert. What is the least number of main courses that the restaurant should offer so that a customer could have a different dinner each night in the year ?.17、An ice cream cone consists of a sphere of vanilla ice cream and a right circular cone that has the same diameter as the sphere. If the ice cream melts, it will exactly ?ll the cone. Assume that the melted ice cream occupies of the volume of the frozen ice cream. What is the ratio of the cone’s height to its radius?18、What is the largest integer that is a divisor offor all positive even integers ?19、Three semicircles of radius are constructed on diameter of a semicircle of radius . The centers of the small semicircles divide into four line segments of equal length, as shown. What is the area of the shaded region that lies within the large semicircle but outside the smaller semicircles?20、In rectangle , and . Points and are on so that and . Lines and intersect at . Find the area of.21、A bag contains two red beads and two green beads. You reach into the bag and pull out a bead, replacing it with a red bead regardless of the color you pulled out. What is the probability that all beads in the bag are red after three such replacements?22、A clock chimes once at minutes past each hour and chimes on the hour according to the hour. For example, at 1 PM there is one chime and at noon and midnight there are twelve chimes. Starting at 11:15 AM on February , , on what date will the chime occur?23、A regular octagon has an area of one square unit. What is the area of the rectangle ?24、The ?rst four terms in an arithmetic sequence are , , , and, in that order. What is the ?fth term?25、How many distinct four-digit numbers are divisible by and have as their last two digits?。

1、下载文档前请自行甄别文档内容的完整性，平台不提供额外的编辑、内容补充、找答案等附加服务。
2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
3、如文档侵犯您的权益，请联系客服反馈,我们会尽快为您处理(人工客服工作时间：9:00-18:30)。