信号与系统 双语 奥本海姆 第二章PPT课件
合集下载
奥本海姆版信号与系统ppt

Instantaneous power: 1 2 R i (t ) p(t ) v(t ) i(t ) v (t ) R i 2 (t ) R _ v(t ) Let R=1Ω, so p(t ) i 2 (t ) v 2 (t ) x 2 (t )
+
Energy : t1 t t2
2
1
shift
f (t )
2 1
1 t
2
2
0
Scaling
Scaling
2
reversal
t
f (t )
2 1
shift
2 1
f (1 t )
f (1 3t )
1
t
0 1
1 0
1
2
2
1
0 1
t
1
2
1 3
0 2
t
3
f (3t )
f (1 3t )
Scaling
1
1 3
2
shift
1.2 Transformation of the Independent Variable
1.2.1 Examples of Transformations 1. Time Shift x(t-t0), x[n-n0]
t0<0
Advance
Time Shift
n0>0
Delay
x(t) and x(t-t0), or x[n] and x[n-n0]:
2. Time Reversal x(-t), x[-n]
——Reflection of x(t) or x[n]
2. Time Reversal x(-t), x[-n]
+
Energy : t1 t t2
2
1
shift
f (t )
2 1
1 t
2
2
0
Scaling
Scaling
2
reversal
t
f (t )
2 1
shift
2 1
f (1 t )
f (1 3t )
1
t
0 1
1 0
1
2
2
1
0 1
t
1
2
1 3
0 2
t
3
f (3t )
f (1 3t )
Scaling
1
1 3
2
shift
1.2 Transformation of the Independent Variable
1.2.1 Examples of Transformations 1. Time Shift x(t-t0), x[n-n0]
t0<0
Advance
Time Shift
n0>0
Delay
x(t) and x(t-t0), or x[n] and x[n-n0]:
2. Time Reversal x(-t), x[-n]
——Reflection of x(t) or x[n]
2. Time Reversal x(-t), x[-n]
奥本海姆信号与系统总结精品PPT课件

d
f1 (t) dt
d
yf 1 (t) dt
=
–3δ(t)
+
[4e-t
–πsin(πt)]ε(t)
根据LTI系统的时不变特性
f1(t–1) →y1f(t – 1) ={ –4e-(t-1) + cos[π(t–1)]}ε(t–1)
由线性性质,得:当输入f3(t) =
d
f1 (t dt
)
+2f1(t–1)时,
t
t
t
sin( x)[a
0
f1 ( x)
b
f2 (x)]d
x
a
0 sin(x) f1 (x) d x b
0 sin(x) f 2 (x) d x
= aT[{f1(t)}, {0}] +bT[{ f2(t) }, {0}],满足零状态线性;
T[{0},{ax1(0) + bx2(0)} ] = e-t[ax1(0) +bx2(0)] = ae-tx1(0)+ be-tx2(0) = aT[{0},{x1(0)}] +bT[{0},{x2(0)}], 满足零输入线性; 所以,该系统为线性系统。
Application Field
• 计算机、通信、语音与图像处理 • 电路设计、自动控制、雷达、电视 • 声学、地震学、化学过程控制、交通运输 • 经济预测、财务统计、市场信息、股市分析 • 宇宙探测、军事侦察、武器技术、安全报警 • 电子出版、新闻传媒、影视制作 • 远程教育、远程医疗、远程会议 • 虚拟仪器、虚拟手术 • 人体:
• 第6章 信号与系统的时域和频域特性 6 连续时间付里叶变换的极坐标表示;理想低通 滤波器;Bode图;一阶系统与二阶系统的分析 方法
信号与系统课件(奥本海姆+第二版)+中文课件.pdf

解:因为 x[n] = e jω0n = cos ω0n + j sin ω0n (欧拉公式)
则有 e jω0n = 1
∑ ∑ ∞
∞
E∞ = x[n] 2 = 1= ∞
n=−∞
n=−∞
∑ P∞
=
lim
N→∞
1N 2N +1n=−N
x[n] 2
= lim N→∞
1 ×(2N 2N +1
+1)
=1
所以是功率信号
控制
执行机构
网络
图 1 控制系统
R+
uc (t)
x (t)
C
uc (t)
-
t
图 2 RC电路
6 / 94
二、信号的分类 信号的分类方法很多。
1、确定性信号与随机信号 按信号与时间的函数关系来分,信号可分为确定性信号与随
机信号。 1)、确定性信号——指能够表示为确定的时间函数的信号。 当给定某一时间值时,信号有确定的数值。 例如:正弦信号、指数信号和各种周期信号等。 2)、随机信号——不是时间t的确定函数的信号。 它在每一个确定时刻的分布值是不确定的。 例如:电器元件中的热噪声等。
11 / 94
5、连续时间信号和离散时间信号——按自变量的取值是否连续来分。
1、连续时间信号——自变量是连续可变的,因此信号在自变量的连续值上 都有定义。我们用t表示连续时间变量,用圆括号(.)把自变量括在里面。例 如 图一的 x(t)。
x (t)
x [n]
X[1] X[-1]
0
t
图一 连续时间信号
1)、时间特性——波形、幅度、重复周期及信号变化的快慢等。 ω
2)、频率特性——振幅频谱和相位频谱。即从频域 来研究信号的变化情 况。
信号与系统 第二章ppt_part2
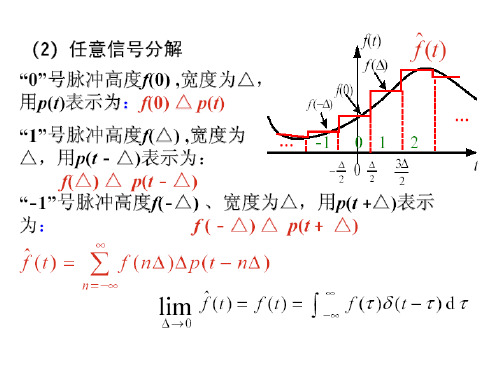
1
0 t 1
[1 e(t 1) ]
演示
[1 e(t 1) ]u(t 1) f1 (t ) f2 (t )
f1 (t )* f2 (t )
1
0
1
t
解法二:f 2 ( ) 不变,反褶 f1 ( ), f 2 ( ) f1 ( )
1 1 1
f1 (t ) f2 (t ) f 2 ( ) f1 (t )d
f
( 1) 2
t e d u ( ) e t u (t ) (1 e t )u (t ) (t ) e u ( )d 0
t
f1(t)*f2(t)=(1-e-t) u(t)- [(1-e-(t-2)] u(t-2)
n
即
y zs (t ) lim x(kt )h(t kt )t
t 0 k 0
y zs (t ) lim x(kt )h(t kt )t
t 0 k 0
n
当 t 0 时,t d , kt ,
t 0
t 0
lim
t k 0 0
s(t )
1 e
T
(t T )
e ]u(t T )
t
t
(t T )
]u(t T )
1
0
t
T
演示
例2-13 已知信号x(t)与h(t)如下图所示,求 h(t) x(t) 1 1
y(t ) x(t ) h(t )
-1/2 0 解:
1
t
0
2
t
y (t ) x( )h(t )d
h(t )
1
0 t 1
[1 e(t 1) ]
演示
[1 e(t 1) ]u(t 1) f1 (t ) f2 (t )
f1 (t )* f2 (t )
1
0
1
t
解法二:f 2 ( ) 不变,反褶 f1 ( ), f 2 ( ) f1 ( )
1 1 1
f1 (t ) f2 (t ) f 2 ( ) f1 (t )d
f
( 1) 2
t e d u ( ) e t u (t ) (1 e t )u (t ) (t ) e u ( )d 0
t
f1(t)*f2(t)=(1-e-t) u(t)- [(1-e-(t-2)] u(t-2)
n
即
y zs (t ) lim x(kt )h(t kt )t
t 0 k 0
y zs (t ) lim x(kt )h(t kt )t
t 0 k 0
n
当 t 0 时,t d , kt ,
t 0
t 0
lim
t k 0 0
s(t )
1 e
T
(t T )
e ]u(t T )
t
t
(t T )
]u(t T )
1
0
t
T
演示
例2-13 已知信号x(t)与h(t)如下图所示,求 h(t) x(t) 1 1
y(t ) x(t ) h(t )
-1/2 0 解:
1
t
0
2
t
y (t ) x( )h(t )d
h(t )
1
课件信号与系统奥本海姆.ppt

2. System a process of signals, in which input signals are transformed into output signals
4
Ch1. Signals and Systems
Signal:the carrier of information 信号:信息的载体
1
SIGNALS AND SYSTEMS
• 信号与系统
8
Main content : Ch1. Signals and Systems
• Continuous-Time and Discrete-Time Signals 〔连续时间与离散时间信号〕
• Transformations of the Independent Variable〔自变量的变换〕
信号是信息的具体物理表现形式,包含了信息的 具体内容。总是1个或多个独立变量的函数。
同一信息可以有不同的物理表现形式,因此对应 有不同的信号,但这些不同的信号都包含同一个信息。 这些不同的信号之间可以相互转换。
例如语音信息用声压表示,可用电压或电流信号 作为载体;也可以用一组数据(01)信号作载体。对应 模拟信号和数字信号,可以AD转换。
2
Ch1. Signals and Systems
控制论创始人维纳认为: 信息是人或物体与外部世界交换内容的名称。内 容是事物的原形,交换是信息载体[信号]将事物原形 [内容]映射到人或物体的感觉器官,人们把这种映射 的结果认为获得了信息。通俗地说,信息指人们得到 的消息。
信息多种多样、丰富多彩,具体的物理形态也千 差万别。
• Basic System Properties (根本系统性质) 9
Ch1. Signals and Systems
4
Ch1. Signals and Systems
Signal:the carrier of information 信号:信息的载体
1
SIGNALS AND SYSTEMS
• 信号与系统
8
Main content : Ch1. Signals and Systems
• Continuous-Time and Discrete-Time Signals 〔连续时间与离散时间信号〕
• Transformations of the Independent Variable〔自变量的变换〕
信号是信息的具体物理表现形式,包含了信息的 具体内容。总是1个或多个独立变量的函数。
同一信息可以有不同的物理表现形式,因此对应 有不同的信号,但这些不同的信号都包含同一个信息。 这些不同的信号之间可以相互转换。
例如语音信息用声压表示,可用电压或电流信号 作为载体;也可以用一组数据(01)信号作载体。对应 模拟信号和数字信号,可以AD转换。
2
Ch1. Signals and Systems
控制论创始人维纳认为: 信息是人或物体与外部世界交换内容的名称。内 容是事物的原形,交换是信息载体[信号]将事物原形 [内容]映射到人或物体的感觉器官,人们把这种映射 的结果认为获得了信息。通俗地说,信息指人们得到 的消息。
信息多种多样、丰富多彩,具体的物理形态也千 差万别。
• Basic System Properties (根本系统性质) 9
Ch1. Signals and Systems
《信号与系统》奥本海姆第二章

conditions ( 初始条件 ) : d y (t 0 ) , , dt d
N 1
y (t 0 )
N 1
dt
完全解:
y(t)=yh(t)+yp(t)
齐次解 特解
Generated by Foxit PDF Creator © Foxit Software For evaluation only.
当N=0时,即 ak 0, k 0 ,差分方程为:
M
a 0 y[ n ]
M
b
j0
j
x[ n j ]
y[ n ]
a
j 0
M
bj
0
x[ n j ]
h[ n]
j 0
bj a0
[n j ]
0nM
bn h[ n ] , a0
Generated by Foxit PDF Creator © Foxit Software For evaluation only.
w(n) bk x(n k ) b0 x(n) b1x(n 1) ... bM x(n M )
方框图为:
x n )) x(( n
D D
b0 1 / a 0
b1 a1
1/ a0y ( n ) y ( n) w(v n( )n ) w(n) b0
D D
D D
N k k 0
M k k 0
a y ( n k ) b x( n k )
N 1 M y(n) bk x(n k ) ak y(n k ) a0 k 0 k 1
令 w(n)
M
b x(n k )
奥本海姆信号与系统课件
More details on sampling will be given in a later chapter.
11
Notes: To distinguish CT signals from DT signals: • Variable notations: t, x, y, · · · for CT signals, n, m, k, · · · for DT signals. • More importantly, parentheses (.) are used for CT signals, while brackets [.] for DT signals.
9
How DT signals are generated ? There are signals of independent variables which • are inherently discrete (ex., no. of students in a class):
P [n]
3000 2800
s(t)
10 5 0 −5 −10 0 0.05 0. 1 0.15 0. 2 (a) 0.25 0. 3 0.35 0. 4
t
s[n]
10 5 0 −5 −10 0 5 10 (b) 15 20
n
Figure 10: (a) s(t) = 10cos(20πt − 0.5), t ∈ [0 0.4]. (b) s[n] = s(tn ) with tn = n/50.
p(t)dt =
t2 t1
v 2(t)dt
• Average power over (t1, t2): 1 t2 − t1
t2 t1
p(t)dt =
信号与系统 第二章

( x1 ( t ) + x2 ( t ))* h( t ) = x1 ( t )* h( t ) + x2 ( t )* h( t )
Application: Parallel system a common system Can break a complicated convolution into several simpler ones
Signals & Systems
Example 2.10
1 n x[n] = ( ) u[ n] + 2n u[− n] 2 h[n] = u[n]
Signals & Systems
2.3.3 The Associative Property
x[n]* ( h1 [n]* h2 [n]) = ( x[n]* h1 [n])* h2 [n] x ( t )*[h1 ( t )* h2 ( t )] = [ x ( t )* h1 ( t )]* h2 ( t )
1 h[n] = 0 n = 0,1 otherwise
Example 2.9
If the system is LTI,determine the relationship between input and output If the system is not LTI,determine the relationship between input and output
Signals & Systems
2.2 Continuous-Time LTI System: The Convolution Integral
2.2.1 The Representation ContinuousTime Signals In Term Of Impulse
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
10
Chapter 2 §2.3 卷积的计算 1. 由定义计算卷积积分
例2.6 xte au tt,a0htut
2. 图解法 例2.7 求下列两信号的卷积
xt 1 , 0tT ht
0 , 其余t 3. 利用卷积积分的运算性质求解
LTI Systems
yt
t , 0t2T 0 , 其余t
11
Chapter 2
in Terms of impulses
Example 2
3 xn
2
1
1 01 2
n
xknk
x n x 1 n 1 x 0 n x 1 n 1
xnxknk k 4
Chapter 2
LTI Systems
§2.1.2 The Discrete-Time Unit Impulse Responses and the
LTI Systems
§2.3 Properties of LTI Systems
xt ht ytxtht
xn hn ynxnhn
LTI系统的特性可由单位冲激响应完全描述
Example 2.9 ① LTI system
h n
1
0
n0,1 otherwise
② Nonlinear System
③ Time-variant System
a y n x n x n 1 2 aytco s3 txt
b y n m x n ,x a n 1 x b ytetxt 12
Chapter 2
LTI Systems
§2.3.1 Properties of Convolution Integral and Convolution Sum 1. The Commutative Property (交换律)
④ 多项式算法(适用于有限长度序列)
利用多项式算法求卷积和的逆运算
9
Chapter 2
LTI Systems
§2.2 Continuous-Time LTI Systems : The Convolution Integral (卷积积分)
§2.2.1 The Representation of Continuous-Time Signals in Terms of impulses
Convolution-Sum Representation of LTI Systems
1. The Unit Impulse Responses 单位冲激响应
hnL0,n
2. Convolution-Sum (卷积和)
yn xkhnkxnhn k k时刻的脉冲在n时刻的响应
系统在n时刻的输出包含所有时刻输入脉冲的影响
Chapter 2 Linear Time-invariant Systems
1
标题添加
点击此处输入相 关文本内容
标题添加
点击此处输入相 关文本内容
总体概述
点击此处输入 相关文本内容
点击此处输入 相关文本内容
Chapter 2
LTI Systems
Example 1 an LTI system
f1t
x n h 1 n h 2 n x n h 1 n x n h 2 n
xt
h1 t
yt
xt
yt
h1th2t
h2 t
14
Chapter 2 3. The Associative Property (结合律)
LTI Systems
x t h 1 t h 2 t x t h 1 t h 2 t
xt xtd ——Sifting Property
§2.2.2 The Continuous-Time Unit Impulse Response and the Convolution Integral Representation of LTI Systems
y t x t h t x h t d
5
Chapter 2 3. 卷积和的计算 ① 利用定义计算
例2.3 xnanun hnun
LTI Systems
xnhn?
② 图解法 Example 2.4
xn 1 , 0n4
hn
an
,
0n6
0a1
0 , otherwise
0 , otherwise
Determine the output signal yn
x n h 1 n h 2 n x n h 1 n h 2 n
xt h1 t
xt h2 t
yt
h2 t
yt
h1 t
xt h1th2t yt
x t h t h t x t x n h n h n x n
xt
xthtBiblioteka ht ht htxt
xt
13
Chapter 2
LTI Systems
2. The Distributive Property (分配律)
x t h 1 t h 2 t x t h 1 t x t h 2 t
6
xnxknk k
hnL0,n
yn xkhnkxnhn k
图解法步骤:
反折
㈠ hn hk
hk
㈡ 平移
hnk n
循 ㈢ 求乘积 xkhnk
环 ㈣ 对每一个n求和 xnhnxkhnk 7 k
Chapter 2
LTI Systems
Example 2.4
xn
1
,
0n4
hn
an
,
0n6
0a1
0 , otherwise
1
y1t
L
1
0
2t
0 1 2t
f2t f1 tf1 t 2
1
L
y2t y 1 ty 1 t 2
1
0
2
4
t
0
2
1
-1
4t
3
Chapter 2
LTI Systems
§2.1 Discrete-time LTI Systems : The Convolution Sum (卷积和)
§2.1.1 The Representation of Discrete-Time Signals
0 , otherwise
Determine the output signal yn
8
Chapter 2
LTI Systems
③ 不带进位的普通乘法 ——适用于因果序列或有限长度序列之间的卷积
Example 3
xn2,1,5 n0,1,2 h n 3,1,4,2 n0,1,2,3 Determine yn x n h n
