信号与系统-课件-(第三版)郑君里.ppt
合集下载
信号与系统第五章3郑君里ppt课件
c b a
完整O版PPT课件 a
b
c
25
复用收信端
收信端：带通滤波器，分开各路信号，解调。
带通
gt
带通
cos
ga
at
t
低通
fa t
cos bt 低通 fb t
带通
cosct 低通 fc t
G( )
c b a
O
a
b
c
完整版PPT课件
26
频分复用解调分析
先利用一个带通滤波器（带 a宽 m a m ）,
a
a
0
当 为 完整版其 PPT课他 件 值
12
系统功能
由Ha
图
形
可
见
，
此
系
统
功
能
1
a
Ha
是 理 想 带 通 滤 波 ，频中率心
2π
0
3 π， a
带
宽Bω
2π a
4π 2π O 2π 3π4π
aa
a aa
＊当参变量a改变时，可调节此带通滤波器中心频率
与带宽：
•增大a则中心频率降低、带宽变窄；
fs0 t
1
O Ts
t
完整版PPT课件
18
第十节 脉冲编码调制(PCM)
•脉冲幅度调制（PAM）：利用脉冲序列对连续信号 进行抽样产生的信号，这一过程的实质是把连续信号 转换为脉冲序列，而每个脉冲的幅度与各抽样点信号 的幅度成正比。 •脉冲编码调制（PCM）：把连续信号转换成数字 （编码）信号进行传输或处理，在转换过程中需要利 用PAM信号。
幅频特性在通带内为常数; 相频特性应为通过载频点的直线 •用带通系统传输调幅波的过程中，只关心包络波形 是否产生失真，并不注意载波相位如何变化，因为 在接收端经解调后得到所需的包络信号，载波本身 并未传递消息 。
《郑君里信号与系统》课件
离散时间信号的表示与性质
要点一
离散时间信号的表示
要点二
离散时间信号的性质
离散时间信号可以由离散的数值序列表示，这些数值在时 间上离散分布。常见的离散时间信号有单位阶跃信号、单 位冲激信号、正弦信号等。
离散时间信号具有周期性、稳定性、可重复性等性质。这 些性质对于信号处理和系统分析具有重要的意义。
离散时间系统的表示与性质
离散时间信号通过系统的响应表 示
当一个离散时间信号通过一个离散时间系统时，系统的 输出可以通过将输入信号与系统冲激响应相卷积得到。
离散时间信号通过系统的响应性 质
系统的输出响应具有与输入信号相同的周期性和稳定性 ，但可能发生幅度和相位的变化。此外，系统的输出响 应还受到系统稳定性和因果性的影响。
பைடு நூலகம்
PART 05
信号的变换域表示法
傅立叶变换的定义与性质
傅立叶变换的定义
将时间域信号转换为频率域信号的数学工具，通过将 信号分解为不同频率的正弦波和余弦波来描述信号的 频率特性。
傅立叶变换的性质
线性性、时移性、频移性、对称性、周期性和收敛性等 ，这些性质在信号处理中具有重要应用。
拉普拉斯变换的定义与性质
拉普拉斯变换的定义
极点影响系统的稳定性，决定了系统是否稳定以及系统的响应速度。
通过零极点分析系统稳定性
判断系统是否稳定
如果所有极点都位于复平面的左半部分，则系统是稳 定的。
计算系统的传递函数
通过求解系统函数的零极点，可以得到系统的传递函 数。
分析系统的动态特性
通过分析零极点的分布和位置，可以进一步分析系统 的动态特性和稳定性。
详细描述
信号可以根据其连续性与离散性分为连续时间信号和离散时间信号；根据确定 性可以分为确定信号和随机信号；根据周期性可以分为周期信号和非周期信号 ；根据能量与功率可以分为能量信号和功率信号。
信号与系统-课件-(第三版)郑君里-homework (8)
School of Computer Science and Information
Solution : (1) consider the zero - state response. y( n) 3 y( n 1) 2 y( n 2) x ( n) Y ( z ) 3 z 1Y ( z ) 2 z 2Y ( z ) X ( z ) Y (z) z2 H (z) ...... X (z) ( z 1)( z 2) ROC :| z | 2 The unit circle is not in ROC The system is not stable.
z X (z) z 1
1 z 3 z Y ( z ) ...... z 1 z 2 1 1 y( n) [ ( 2) n ] u( n) 6 3 1 6
School of Computer Science and Information
z 1 ( 3) H ( z ) ( z 1)( z 2) 1 3 z 1 2 z 2
School of Computer Science and Information
Solution : z x ( n) u( n) X ( z ) z 1 y( n) [2 2( 2) n 3( 3) n ] u( n) 2z 2z Y (z) z 1 z 2 Y (z) H (z) ...... X (z) 3z z3 3z 2z z2 z3
School of Computer Science and Information
( 2) consider the entire response. y( n)n) Y ( z ) 3[ z 1Y ( z ) y( 1)] 2[ z 2Y ( z ) z 1 y( 1) y( 2)] X ( z )
信号与系统 郑君里 第三版_课件
f (t) f1(t) f2 (t)
信号的数乘运算是指某信号乘以一实常数K，它是
将原信号每一时刻的值都乘以K ，即
2020/3/6
f (t) Kf (t)
30
1.3.3 信号的反褶、时移、尺度变换运算
（1）反褶运算 f (t) f (t) f(t) 1
以 t = 0为轴反褶 f(-t)
f (0)
综合式（2）和式（4），可得出如下结论： 冲激函数可以把冲激所在位置处的函数值抽取（筛选）出来。
2020/3/6
24
（2） (t) 是偶函数，即 (t) (t)
（3） t ( )d
0 t 0 1 t 0
u(t)
(t)
t
(
＋
E=1V －
C=1F
vc (t)
1
0
2
t
2020/3/6
例：图中假设S、E、C都是理
想元件（内阻为0），当 t = 0时 S闭合，求回路电流i（t）。
i(t) C dvC (t) dt
2 i(t)
1
0 2
0
t
i(t) (t)
(1)
0
t
演示 20
1. (t)的定义方法 （1）用表达式定义
R(t) t, (t 0)
R(t)
R(t t0 ) t t0 , (t t0 )
R(t-t0)
1
1
0 2020/3/6
1
t
0
t0
t0+1 t 14
二、单位阶跃信号
u(t) 0, (t 0) 1, (t 0) u(t)
信号与系统-课件-(第三版)郑君里-PPT课件
Example
f( t) f( t)
A … … 2 4 6 k
－ T
T 2
o
T 2 － A
T
t
－ 4 － 2 0
Periodic Signal
School of Computer Science and Information
3. Continuous-time Signal and Discrete-time Signal
Example
Noise Signal and Interfere Signal
School of Computer Science and Information
2. Periodic Signal and Aperiodic Signal
Periodic Signal — Has the property that it is
Random Signal — Can’t be represented mathematically as a function of certain time. We only know the probability of certain value.
School of Computer Science and Information
Vertical Wind Profile
School of Computer Science and Information
1.2 Systems
For the most part, our view of systems will be from an input-output perspective. A system responds to applied input signals, and its response is described in terms of one or more output signals.
郑君里信号与系统课件
2 an T1
T1 2 T 1 2
f ( t )dt
余弦分量 系数 正弦分量 系数
T1 2 T 1 2
f ( t ) cos(n1t )dt
2 bn T1
T1 2 T 1 2
f ( t ) sin( n1t )dt
注意！
傅立叶级数与傅立叶系数的联系与区别
指数形式傅立叶级数的傅里叶系数
尺度变换、初值、终值
卷积特性 拉氏逆变换
部分分式展开法（求系数）
系统函数H(s)
定义（两种定义方式）
求解（依据两种定义方式）
第四章 拉普拉斯变换、 连续时间系统的s域分析
收敛域：实际上就是拉氏变换存在的条件；
σ t
lim f (t ) e
t
0
σ σ0
三．一些常用函数的拉氏变换
t n st n n1 st e t e dt s 0 s 0
n n1 st t e dt s 0 n n 1 n 所以 L t L t s n1
Lt t e d t
st 0
1 1 st 1 e s2 s s 0 n2 2 2 1 2 2 L t Lt 2 3 s s s s n3 3 2 3 2 6 3 Lt Lt 3 4 s s s s
1 sin( t ) (e jt e jt ) 2j 1 cos(t ) (e jt e jt ) 2
推出 公式
第一章 绪论
关于冲激信号
(at )
1 (t ) a
尺度变换特性
(t ) f (t ) f (0) (t )
T1 2 T 1 2
f ( t )dt
余弦分量 系数 正弦分量 系数
T1 2 T 1 2
f ( t ) cos(n1t )dt
2 bn T1
T1 2 T 1 2
f ( t ) sin( n1t )dt
注意！
傅立叶级数与傅立叶系数的联系与区别
指数形式傅立叶级数的傅里叶系数
尺度变换、初值、终值
卷积特性 拉氏逆变换
部分分式展开法（求系数）
系统函数H(s)
定义（两种定义方式）
求解（依据两种定义方式）
第四章 拉普拉斯变换、 连续时间系统的s域分析
收敛域：实际上就是拉氏变换存在的条件；
σ t
lim f (t ) e
t
0
σ σ0
三．一些常用函数的拉氏变换
t n st n n1 st e t e dt s 0 s 0
n n1 st t e dt s 0 n n 1 n 所以 L t L t s n1
Lt t e d t
st 0
1 1 st 1 e s2 s s 0 n2 2 2 1 2 2 L t Lt 2 3 s s s s n3 3 2 3 2 6 3 Lt Lt 3 4 s s s s
1 sin( t ) (e jt e jt ) 2j 1 cos(t ) (e jt e jt ) 2
推出 公式
第一章 绪论
关于冲激信号
(at )
1 (t ) a
尺度变换特性
(t ) f (t ) f (0) (t )
信号与系统-课件-(第三版)郑君里-homework (10)
School of Computer Science and Information
Solution :
(1) y(n) 5 y( n 1) 6 y( n 2) 2 x(n)
Y ( z ) 5 z 1Y ( z ) 6 z 2Y ( z ) 2 F ( z ) Y (z) 2 2z 2 H (z) 2 1 2 X ( z ) 1 5z 6z z 5z 6
1 1 2z Y (z) ( ) 1 2 1 2 1 5z 6z 1 5z 6z z 1 2 1 3 z z z Y (z) 3 2 6 z 2 z 3 z 1
2 3 1 n n y( n) [ ( 2) ( 3) ]u( n) 3 2 6
( 2) z x( n) u( n) X ( z ) z 1
School of Computer Science and Information
( 2)
z x( n) u( n) X ( z ) z 1
z 2 ( z 3) Y (z) H (z) F (z) 2 ( z 1) ( z 2) 2z 3z 2z 2 ( z 1) z 1 z 2
0.2 cos j 0.2 sin H (e ) cos 0.8 j sin
j
School of Computer Science and Information
6. Consider a discrete system be described as : y( n) x ( n) y( n 1) (1) Determine h( n), and then draw the fram of system. ( 2) For the input x( n) 6u( n), determine the zero state response y( n) ?
信号与系统(郑君里)ppt
f(t)
O
t
f(n)
O 12
n
4．模拟信号，抽样信号，数字信号
•模拟信号：时间和幅值均为连续
f t
的信号。
抽
样
t
•抽样信号：时间离散的，幅值
O
量
连续的信号。
f n
化
•数字信号：时间和幅值均为离散 O
n
的信号。
f n
主要讨论确定性信号。 n
先连续，后离散；先周期，后非周期。O
时间轴 幅度轴
连续
连续 模拟信号
t
f(t)
t/2
f(t/2)
0
1
0
1
T
2
T
2
时间尺度压缩：t ห้องสมุดไป่ตู้ 2 ,波形扩展
求新坐标
t
f(t/2)
0
1
2T
2
f(t)f(2t)
f t
2 1
O
Tt
宗量相同，函数值相同
t
f(t)
2t
f(2t)
0
1
0
1
T
2
T
2
求新坐标
t
f(2t)
0
1
T/2
2
t2t，时间尺度增加，波形压缩。
比较
f t
2 1
O
Tt
O
t
二．单位阶跃信号
1. 定义
u(t )
0
u(t )
1
t 0 0点无定义或1
t 0
2
2. 有延迟的单位阶跃信号
1
O
t
u(t t0 )
0 u(t t0 ) 1
0 u(t t0 ) 1
- 1、下载文档前请自行甄别文档内容的完整性，平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益，请联系客服反馈,我们会尽快为您处理(人工客服工作时间：9:00-18:30)。
Continuous-time Signal — The independent variable is continuous, and thus these signals are defined for a continuum of values of the independent variable.
Periodic Signal — Has the property that it is unchanged by a time shift of T. For example, A periodic continuous-time or discrete-time signal can be represented as: f (t) f (t nT ) f (n) f (n kT )
School of Computer Science and Information
Example
Noise Signal and Interfere Signal
School of Computer Science and Information
2. Periodic Signal and Aperiodic Signal
School of Computer Science and Information
A Speech Signal
School of Computer Science and Information
பைடு நூலகம்
A Picture
School of Computer Science and Information
School of Computer Science and Information
Example
RLC circuit
School of Computer Science and Information
By interconnecting simpler subsystems. We can build more complex systems.
…… ……
School of Computer Science and Information
A Simple RC Circuit
The patterns of variation over time in the source voltage Vs and capacitor voltage Vc are examples of signals.
School of Computer Science and Information
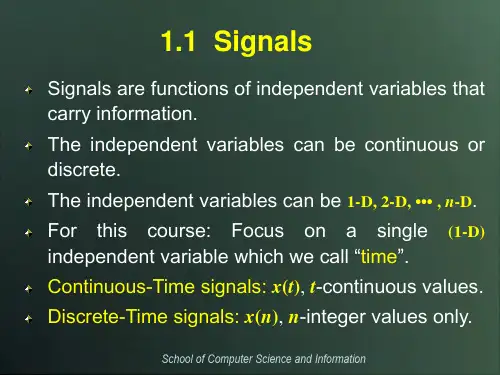
1.1 Signals
Signals are functions of independent variables that carry information. The independent variables can be continuous or discrete. The independent variables can be 1-D, 2-D, ••• , n-D. For this course: Focus on a single (1-D) independent variable which we call “time”. Continuous-Time signals: x(t), t-continuous values. Discrete-Time signals: x(n), n-integer values only.
School of Computer Science and Information
1.3 Types of Signals
1. Certain Signal and Random Signal
Certain Signal — Can be represented mathematically as a function of certain time. Random Signal — Can’t be represented mathematically as a function of certain time. We only know the probability of certain value.
Aperiodic Signal — Has not the property that it is unchanged by a time shift of T.
Notice:
When T→∞,then Periodic Signal → Aperiodic Signal.
School of Computer Science and Information
Vertical Wind Profile
School of Computer Science and Information
1.2 Systems
For the most part, our view of systems will be from an input-output perspective. A system responds to applied input signals, and its response is described in terms of one or more output signals.
Example
f (t) A
－ T T
oT
T
2
2
－A
f (t)
…
…
t
－4 －2 0
246
k
Periodic Signal
School of Computer Science and Information
3. Continuous-time Signal and Discrete-time Signal
School of Computer Science and Information
Examples
Electrical signals — voltages and currents in a circuit. Acoustic signals — audio or speech signals. Video signals — intensity variations in an image. Biological signals — sequence of bases in a gene.
Periodic Signal — Has the property that it is unchanged by a time shift of T. For example, A periodic continuous-time or discrete-time signal can be represented as: f (t) f (t nT ) f (n) f (n kT )
School of Computer Science and Information
Example
Noise Signal and Interfere Signal
School of Computer Science and Information
2. Periodic Signal and Aperiodic Signal
School of Computer Science and Information
A Speech Signal
School of Computer Science and Information
பைடு நூலகம்
A Picture
School of Computer Science and Information
School of Computer Science and Information
Example
RLC circuit
School of Computer Science and Information
By interconnecting simpler subsystems. We can build more complex systems.
…… ……
School of Computer Science and Information
A Simple RC Circuit
The patterns of variation over time in the source voltage Vs and capacitor voltage Vc are examples of signals.
School of Computer Science and Information
1.1 Signals
Signals are functions of independent variables that carry information. The independent variables can be continuous or discrete. The independent variables can be 1-D, 2-D, ••• , n-D. For this course: Focus on a single (1-D) independent variable which we call “time”. Continuous-Time signals: x(t), t-continuous values. Discrete-Time signals: x(n), n-integer values only.
School of Computer Science and Information
1.3 Types of Signals
1. Certain Signal and Random Signal
Certain Signal — Can be represented mathematically as a function of certain time. Random Signal — Can’t be represented mathematically as a function of certain time. We only know the probability of certain value.
Aperiodic Signal — Has not the property that it is unchanged by a time shift of T.
Notice:
When T→∞,then Periodic Signal → Aperiodic Signal.
School of Computer Science and Information
Vertical Wind Profile
School of Computer Science and Information
1.2 Systems
For the most part, our view of systems will be from an input-output perspective. A system responds to applied input signals, and its response is described in terms of one or more output signals.
Example
f (t) A
－ T T
oT
T
2
2
－A
f (t)
…
…
t
－4 －2 0
246
k
Periodic Signal
School of Computer Science and Information
3. Continuous-time Signal and Discrete-time Signal
School of Computer Science and Information
Examples
Electrical signals — voltages and currents in a circuit. Acoustic signals — audio or speech signals. Video signals — intensity variations in an image. Biological signals — sequence of bases in a gene.