博弈论期末考试(英文)
博弈期末考试题及答案

博弈期末考试题及答案一、选择题(每题2分,共20分)1. 博弈论中的“纳什均衡”是由哪位数学家提出的?A. 约翰·冯·诺伊曼B. 约翰·纳什C. 保罗·萨缪尔森D. 托马斯·谢林2. 以下哪个不是博弈论中的基本概念?A. 策略B. 收益C. 风险D. 均衡3. 在零和博弈中,一个玩家的损失等于另一个玩家的收益,那么这种博弈的总收益是:A. 正数B. 零C. 负数D. 无法确定4. 囚徒困境中,如果两个囚犯都选择背叛对方,那么:A. 他们都会受到最轻的惩罚B. 他们都会受到最重的惩罚C. 一个受到轻罚,另一个受到重罚D. 一个受到重罚,另一个获得释放5. 以下哪个是博弈论中的动态博弈?A. 石头剪刀布B. 囚徒困境C. 拍卖博弈D. 猎鹿博弈...(此处省略其他选择题)二、简答题(每题10分,共30分)1. 简述博弈论中的完全信息博弈和不完全信息博弈的区别。
2. 解释什么是“混合策略纳什均衡”,并给出一个例子。
3. 描述“公共品博弈”中的囚徒困境现象。
三、计算题(每题15分,共30分)1. 假设有两个玩家A和B,他们可以选择策略X或Y。
收益矩阵如下所示:| | X | Y |||||| X | 3,3 | 2,5 || Y | 5,2 | 4,4 |请计算并找出所有可能的纳什均衡。
2. 考虑一个重复博弈,其中两个玩家在每一轮中可以选择合作或背叛。
如果双方合作,他们各自获得收益R。
如果一方背叛而另一方合作,背叛者获得收益T,合作者获得收益S。
如果双方都背叛,他们各自获得收益P。
已知2R > T + S > R > P。
请证明在无限重复博弈中,存在一个策略组合,使得双方的长期收益都高于单次博弈的背叛收益。
四、论述题(20分)1. 论述博弈论在经济学中的应用,并给出两个具体的例子。
博弈期末考试题答案一、选择题答案1. B2. C3. B4. B5. D...(此处省略其他选择题答案)二、简答题答案1. 完全信息博弈是指所有玩家都完全知道博弈的结构和其他玩家的收益函数,而不完全信息博弈是指至少有一个玩家对博弈的结构或其它玩家的收益函数不完全了解。
game theory12 博弈论 英文 例题详细解析

Beer or Quiche gameIn this handout, we discuss signaling games of Beer or Quiche type and the way to find the plausible outcome of this type of games – weak perfect Bayesian equilibrium.The basic idea is that we try all the combinations of actions of all players one by one see if any of them is an equilibrium. In general, we work in the following steps:Step 1: Start with actions of Player 1 (both types)Step 2:Find Player 2’s beliefs ba sed on actions of Player 1 (in both information sets)Step 3: Find optimal response of Player 2 (in both information sets)Step 4: Check if both types of Player 1 play optimal response:a.yes, no type of Player 1 wants to deviate => we have WPBEb.no, at least one type of Player 1 wants to deviate => no WPBEStep 5: Move to the next possible actions of Player 1Example: consider the following game.We will analyze these options:1.Player 1: Beer if Weak, Quiche if Strong (Separating equilibrium 1)2.Player 1: Quiche if Weak, Beer if Strong (Separating equilibrium 2)3.Player 1: Quiche if Weak, Quiche if Strong (Pooling equilibrium 1)4.Player 1: Beer if Weak, Beer if Strong (Pooling equilibrium 2)Option 1: Player 1: Beer if Weak, Quiche if Strong (Separating equilibrium 1)Step 1: Start with actions of Player 1 (both types)Beer if Weak, Quiche if StrongStep 2:Find Player 2’s beliefs based on actions of Player 1 (in both information sets)Information sets are reached, so beliefs are calculated based on actions of Player 1.Step 3: Find optimal response of Player 2 (in both information sets)In the left information set, Player 1 knows that Player 2 is of Weak type and Fighting is optimal (3>0).In the right information set, Player 2 knows that Player 1 is of Strong type and Not Fighting is optimal(2>0).Step 4: Check if both types of Player 1 play optimal responseWeak type of Player 1 is satisfied, but Strong type of Player 1 wants to deviate and get higher profit.So this is not WPBE.Step 5: Move to the next possible actions of Player 1Option 2: Player 1: Quiche if Weak, Beer if Strong (Separating equilibrium 2) Step 1: Start with actions of Player 1 (both types)Quiche if Weak, Beer if StrongStep 2:Find Player 2’s beliefs based on actions of Player 1 (in both information sets)Information sets are reached, so beliefs are calculated based on actions of Player 1.Step 3: Find optimal response of Player 2 (in both information sets)In the left information set, Player 1 knows that Player 2 is of Strong type and Fighting is optima (4>1).In the right information set, Player 2 knows that Player 1 is of Weak type and Fighting is optimal(1>0).Step 4: Check if both types of Player 1 play optimal responseNo type of Player 1 wants to deviate because the payoff would be lower. So this is WPBE.Step 5: Move to the next possible actions of Player 1Option 3: Player 1: Quiche if Weak, Quiche if Strong (Pooling equilibrium 1) Step 1: Start with actions of Player 1 (both types)Quiche if Weak, Quiche if StrongStep 2:Find Player 2’s beliefs based on actions of Player 1 (in both information sets)Right information set is reached, so beliefs are calculated based on actions of Player 1. Left information set is not reached, so we are free to choose any beliefs, any set of beliefs is consistent with Player 1’s actions. Denote beliefs p and 1-p.Step 3: Find optimal response of Player 2 (in both information sets)In the right information set, Player 2 calculates expected payoff of playing Fight and NotFigt:EP(Fight) = 0.3*1+0.7*0 = 0.3EP(NotFight) = 0.3*0+0.7*2 = 1.4So NotFight is optimal response.In the left information set, optimal response of Player 2 generally depends on value of p (beliefs). However, in this case, Fight dominates NotFight in the left information set and hence Fight is optimal.Step 4: Check if both types of Player 1 play optimal responseActually, no matter what action Player 2 chooses in the left information set, Weak type of Player 1 will always want to deviate. So this is not WPBE.Step 5: Move to the next possible actions of Player 1Option 4: Player 1: Beer if Weak, Beer if Strong (Pooling equilibrium 2)Step 1: Start with actions of Player 1 (both types)Beer if Weak, Beer if StrongStep 2:Find Player 2’s beliefs based on actions of Player 1 (in both information sets)Left information set is reached, so beliefs are calculated based on actions of Player 1. Beliefs in the right information set are denoted p and 1-p because this information set is not reached and hence any set of beliefs is consistent with actions of P1..Step 3: Find optimal response of Player 2 (in both information sets)In the left information set, Player 2 calculates expected payoff of playing Fight and NotFigt:EP(Fight) = 0.3*3+0.7*4 = 3.7EP(NotFight) = 0.3*0+0.7*1 = 0.7So Fight is optimal response.In the right information set, optimal response of Player 2 generally depends on value of p (beliefs).There are two options, either the optimal response is Fight, but then Weak Player 1 would want to deviate; or the optimal response is NotFight. In this case no type of Player 1 would want to deviate.So the beliefs have to be set such that expected payoff from NotFight is larger than from Fight.EP(Fight) < EP(NotFight) => 1*p + 0*(1-p) < 0*p + 2*(1-p) => p<2/3Step 4: Check if both types of Player 1 play optimal responseNo type of Player 1 wants to deviate because the payoff would be lower. So this is WPBE.Step 5: Move to the next possible actions of Player 1. There are no more possibilities.There are two WPBE in this game:1.Weak P1 –Quiche, Strong P1 –Beer; P2 Fight if Beer and Fight if Quiche and p=0 in the leftinformation set and p=1 in the right information set.2.Weak P1 – Beer, Strong P1 – Beer; P2 – Fight if Beer and NotFight if Quiche and p<2/3.。
博弈论期末考试试题及答案

博弈论期末考试试题及答案# 博弈论期末考试试题及答案一、选择题(每题2分,共20分)1. 博弈论中,参与者在没有沟通的情况下进行决策,这种博弈被称为:A. 完全信息博弈B. 不完全信息博弈C. 零和博弈D. 非零和博弈答案:B2. 纳什均衡是博弈论中的一个概念,它描述了一种什么样的状态?A. 所有参与者都获得最大收益的状态B. 至少有一个参与者能获得更大收益的状态C. 没有参与者能通过单方面改变策略来获得更大收益的状态D. 所有参与者都获得相同收益的状态答案:C3. 以下哪个不是博弈论中的策略类型?A. 纯策略B. 混合策略C. 随机策略D. 确定性策略答案:D4. 博弈论中的囚徒困境指的是:A. 参与者合作可以获得最优结果B. 参与者背叛可以获得最优结果C. 参与者合作可以获得次优结果,但背叛可以获得最优结果D. 参与者背叛可以获得次优结果,但合作可以获得最优结果答案:C5. 以下哪个不是博弈论中的基本概念?A. 参与者B. 策略C. 收益D. 概率答案:D...二、简答题(每题10分,共30分)1. 解释什么是博弈论,并给出一个实际生活中的例子。
答案:博弈论是研究具有冲突和合作特征的决策者之间互动的数学理论。
在实际生活中,博弈论的一个例子是拍卖。
在拍卖中,买家(参与者)需要决定出价(策略)以赢得商品(收益),同时考虑其他买家的出价策略。
2. 描述纳什均衡的概念,并解释为什么它在博弈论中如此重要。
答案:纳什均衡是指在非合作博弈中,每个参与者选择自己的最优策略,并且考虑到其他参与者的策略选择时,没有参与者能通过单方面改变策略来获得更大的收益。
纳什均衡在博弈论中非常重要,因为它提供了一种预测参与者行为的方法,即在均衡状态下,参与者没有动机去改变他们的策略。
3. 什么是完全信息博弈和不完全信息博弈?它们之间有什么区别?答案:完全信息博弈是指所有参与者都完全知道博弈的结构和其他参与者的收益函数。
而不完全信息博弈是指至少有一个参与者对博弈的结构或其它参与者的收益函数不完全了解。
博弈论答案(Gametheoryanswer)

博弈论答案(Game theory answer)Game theory, exercises, reference answers (second assignments)First, the multiple-choice question1.B,2.C,3.A,4.A,5.B,6.ABCD7.C 8.B 9.CTwo, judge and explain the reason1.F best balance is an equilibrium more rigorous than the Nash equilibrium2.T best balance is an equilibrium more rigorous than the Nash equilibrium3.T game types are divided into single game, double game and multiplayer game according to the number of players in the gameUnder the condition that both sides of the 4.F game have different preferences, there may be 2 Nash equilibria in a game model, such as the sex war5.T zero sum game refers to the participation of all parties in the game, under strict competition, one side of revenue is equal to the other party's loss, the sum of gains and losses of the game is always zero, so there is no possibility of cooperation between the two sides6.T is strictly dominated equilibrium through the worstelimination method (excluding repeat decision) the dominant strategy, there is only one Nash equilibrium7.F Nash equilibrium is a collection of best policies, which means that in the case of a given strategy, the game side always chooses a relatively large strategy, and does not guarantee the outcome to be the best.In the 8.F game, people always choose their own strategies to maximize their interests and not aim at the change of the other's earnings9.T Nash equilibrium is a collection of best policies, which means that when given someone else's strategy, no one changes his strategy to reduce his earningsIn the 10.F game, people always choose their own strategies to maximize their interests and not aim at the change of the other's earningsIn the 11.F game, people always choose their own strategies to maximize their interests and not aim at the change of the other's earnings12.T although Berg Stagg model profit is less than the sum of the Cournot model, but the profit model of high Bigunuo leaderThree, calculation and analysis questions1, (1) draw A, B two enterprise profit and loss matrix.B enterpriseAdvertise without advertisingA enterprises advertise 20, 825, 2No advertising 10, 1230, 6(2) pure strategy Nash equilibrium.(advertising, advertising)2, draw two enterprise profit and loss matrix, seek Nash equilibrium.(1) draw the profit and loss matrix of A and B two enterprisesPepsi ColaOriginal price increaseCoca-Cola's original price is 10, 10100, -30Price increases -20, 30140, 35(2) seeking Nash equilibrium.Two: (the original price, the original price), (prices, prices)3, suppose the payoff matrix of a game is as follows:Methyl ethylLeft and rightOn a, B, C, DNext, e, F, G, H(1) if (on, left) is the best balance, then, a>, b>, g<, f>?Answer: a>e, b>d, f>h, g<c(2) what inequalities must be satisfied if (upper, left) is the Nash equilibrium?Answer: a>e, b>d4, answer: (1) this market is represented by the game of prisoner's dilemma.Northern AirlinesCooperative competitionXinhua Airlines cooperation 500000500000090000Competition 900000, 06000060000(2) explain why the equilibrium result may be that both companies choose competitive strategies.Answer: if Xinhua chooses "competition", then the north will choose "60000>0"; if Xinhua chooses "cooperation", the north will still choose "900000>500000".If the North chooses "competition", Xinhua will choose "60000>0"; if the North chooses "cooperation", Xinhua will still choose "900000>0".Because the competition is the dominant strategy of both sides, the equilibrium result is that both companies choose competitive strategy.5. The payoff matrix of the game is shown as follows:BLeft and rightA, a, B, C, DNext, e, F, G, H(1) if the (top, left) is the dominant policy equilibrium, what relation must be satisfied between a, B, C, D, e, F, G, and H?Answer: starting from the definition of dominant strategy equilibrium:For the one, the strategy "g" (a) is better than "C" (E);For B., the policy "left" (B, f) is superior to the policy"right" (D, H).So the conclusions are: a>e, b>d, f>h, c>g(2) if the (upper, left) is Nash equilibrium, what relation must be satisfied in (1)?Answer: Nash equilibrium only needs to meet: a>e, b>d,(3) if the (top, left) is the best balance, then is it necessarily a Nash equilibrium? Why?Answer: the equilibrium of dominant strategy must be Nash equilibrium, because the equilibrium condition of dominant strategy contains the condition of Nash equilibrium.(4) under what circumstances does the pure strategy Nash equilibrium exist?A: when each of these strategies does not satisfy the Nash equilibrium, the pure strategic Nash equilibrium does not exist.7, seek the Nash equilibrium.PigPress waitBig pigs press 5, 14, 4Wait 9, -1 0, 0The Nash equilibrium is: big pig, press, pig, etc., namely (press, etc.)6,BLow priceA low price of 10080050, 50High priced -20, -30 900600(1) what are the results of Nash equilibrium?Answer: (low price, low price), (high price, high price)(2) what is the result of the cooperation between the two firms?Answer: (high price, high price)8. The pure Nash equilibrium of the following games is obtained by using the reaction function method and the marking method.Participants 1 participants 2A, B, C, DingA, 2,3, 3,2, 3,4, 0,3B, 4,4, 5,2, 0,1, 1,2C, 3,1, 4,1, 1,4, 10,2D, 3,1, 4,1, -1,2, 10,1Participant 1's response function:R1 (2) =B, if 2 chooses a=B, if 2 chooses B.=A, if 2, choose C=C or D, if 2, choose DingParticipant 2's response function:R2 (1) = C, if 2, select A= a, if 2, select B= C, if 2, select C= C, if 2, select DFor the common set, the pure strategy Nash equilibrium is (B, a) and (A, c)9, the following game Nash equilibrium (including pure strategyand mixed strategy).Methyl ethylL RU 5,0 0,8D 2,6 4,5Solution: (1) pure strategy Nash equilibrium: we can see from the scratch method that there is no pure strategy Nash equilibrium in the matrix game.(2) mixed strategy Nash equilibriumThe probability of setting a "U" is P1, and the probability of "D" is 1-P1B. the probability of selecting "L" is P2, and the probability of "R" is 1-P2For a, the best policy is to choose "U" and "D" by a certain probability, so that the second choice of "L" and "R" is equal to the expected valueThat is, P1*0+ (1-P1), *6=, P1*8+ (1-P1), *5Xie P1=1/9That is, (1/9,8/9) Nash policy is chosen according to 1/9probability, U and 8/9 probability, and D is chosen as a mixed strategyFor B, the best strategy is to choose "L" and "R" by a certain probability, so that the second is equal to the expected value of "U" and "D"That is, P2*5+ (1-P2), *0=, P2*2+ (1-P2), *4Xie P2=4/7That is, (4/7,3/7) according to the probability of 4/7, "L", "3/7", "R" is chosen as "B", the mixed strategy Nash equilibrium10, answer the question according to the profit and loss matrix of two player game:Methyl ethylLeft and rightGo to 2,3 0,0Lower 0,0 4,2(1) write out all the strategies of the two men.Answer: all strategies: (upper, left), (upper, right), (lower, left), (lower, right)(2) find all the pure strategy Nash equilibrium of the game.A: by the scratch method, we can see that the matrix game is purely strategic and the Nash equilibrium is(upper, left) and (lower, right) two(3) the mixed strategy Nash equilibrium of the game is obtained.Solution: the probability of setting a "up" is P1, and the probability of selecting "down" is 1-P1B. the probability of "left" is P2, and the probability of "right" is 1-P2For a, the best strategy is to choose "upper" and "lower" according to a certain probability, so that the left and right of the second are equal to the expected valueThat is, P1*3+ (1-P1), *0=, P1*0+ (1-P1), *2Xie P1=2/5That is, (2/5,3/5) a mixed strategy Nash equilibrium based on the "2/5 probability", "upper", "3/5" probability, and "next"For b.,The best strategy is to choose "left" and "right" according to a certain probability, so that the candidate's "upper" and "lower" expectations are equalThat is, P2*2+ (1-P2), *0=, P2*0+ (1-P2), *4Xie P2=2/3That is, (2/3,1/3) Nash policy is chosen by the 2/3 probability "left" and "1/3", and the "right" is b11, an oligopoly market has two manufacturers, the total cost is 20 times the output of their own, the market demand letterThe number is Q=200-P.Answer: (1) if two manufacturers decide the output at the same time, how much is the output?(2) if the two firms reach an agreement to monopolize the market and arrange production together, what about their respective profits?(3) use the case to explain the prisoner's dilemma.Answer: (1) by the known conditions Q=200-P, P=200-QTC1=20q1, TC2=20q2, q1+q2=QThe profit functions obtained by 1,2 manufacturers are:K1=Pq1-TC1= (200- (q1+q2)) q1-20q1=180q1-q12-q1q2K2=Pq2-TC2= (200- (q1+q2)) q2-20q2=180q2-q22-q1q2The dK/dq1=0's 1 response function is 180-2Q1-Q2=0,The dK/dq2=0's 2 response function is 180-Q1-2Q2=0,The joint solution can be obtained by q1=q2=60K1=K2=3600(2) by the known condition Q=200-P, P=200-QTC=TC1+TC2=20q1+20q2 =20QThe total profit function of the 1,2 manufacturer is:K=PQ-TC= (200-Q) Q-20Q=180Q-Q2Order dK/dQ=0, Q=90, q1=q2=45K=PQ-TC= (200-Q) Q-20Q=180Q-Q2=8100K1=K2=4050(3) q1=45, q2=60 and q1=60, q2=45, respectively, into the profit function of 1,2 manufacturersThe profits of the 1,2 manufacturers are:K1 (q1=45, q2=60) =Pq1-TC1= (200- (q1+q2))q1-20q1=180q1-q12-q1q2=3375K1 (q1=60, q2=45) =Pq1-TC1= (200- (q1+q2))q1-20q1=180q1-q12-q1q2=4500K2 (q1=45, q2=60) =Pq2-TC2= (200- (q1+q2))q2-20q2=180q2-q22-q1q2=4500K1 (q1=60, q2=45) =Pq1-TC1= (200- (q1+q2))q1-20q1=180q1-q12-q1q2=3375Vendor 2Cooperation (q2=45), non cooperation (q2=60);Vendor 1 Cooperation (q1=45) 4050405033754500Non cooperative (q1=60) 4500337536003600According to the marking method, the best way for the manufacturer is 1.2 (non cooperation, non cooperation), that is, (36003600)The profits of both sides were lower than (cooperation, cooperation). (40504050) obviously it belonged to the prisoner's dilemma"13, consider the following (market deterrence) a dynamic game: first of all, the potential in a market entrants to choose whether or not to enter, and then on the market for enterprise (incumbent) is selected to compete with the new enterprise. The incumbent may have two types of gentle type (left) and cruel type (right), answer the following questions..Left: gentle right: cruel type(1) find the corresponding Nash equilibrium for two types of incumbent, and the sub game perfect Nash equilibrium(1) the Nash equilibrium of the gentle type of incumbent is (access, acquiescence)The Nash of the cruel type is balanced (not entering, entering, struggling)(2) when the existing enterprise is tender, at least how many times will the new enterprise be willing to enter?Four. Discussion questions1, explain the prisoner's Dilemma and explain the business case.(1) assumptions for example: two prisoners were accused of a crime is an accomplice. They were kept in separate cells, unable to communicate information. Prisoners are required to confess crimes. If two prisoners confess, each shall be sent to prison for 5 years; if two men do not confess, two prisoners may expect to be sent from prison to prison for 2 years; if a prisoner confesses, another prisoner does not confess,Frankly, the prisoner will only go to prison for 1 years, and the prisoner without confession will be sentenced to 10 yearsin prison.(2) the strategy matrix of prisoners' dilemma. Each prisoner has two strategies: to confess or not to confess. The numbers in the table represent the benefits of prisoner a and B.Prisoner BConfessPrisoner frank, -5, -5, -1, -10Don't confess, -10, -1, -2, -2(3) analysis: through the marking method, we can see that in the model of prisoner's dilemma, Nash equilibrium is that both sides confess". Given a frank case, the best strategy for B. is to confess; the optimal policy given by B. is also frank. And here both sides confess, not only is the Nash equilibrium, but also is a best balance, that is, regardless of how the other side of the choice, the individual's best choice is to confess. As a result, both sides confess.(4) business cases: oligopoly firms often find themselves ina prisoner's dilemma. When the oligarchic manufacturer chooses the output, every manufacturer can gain more profits if the oligopoly firms combine to form cartels and choose monopoly profits to maximize the output. But the cartel agreement is not a Nash equilibrium, because given both comply with the agreement, each firm to increase production, the result is that each vendor has only been Nash equilibrium yield profits, itis far less than the yield of profit under the cartel.2. Explain and discuss the Nash equilibrium of Cournot duopoly model. Why is balance a prisoner's dilemma?See class notesOr calculation questions eleventh3, use the game of thief and guard to explain the paradox of encouragement (regulation)".(1) assume the conditions for example: stealing and preventing theft is a game between thieves and guards. The guard can sleep or sleep. Thieves can take two tactics: stealing and stealing. If the thief knows that the guard is sleeping, his best bet is to steal. If the guard doesn't sleep, he'd better not steal. For the doorman, if he knows the thief wants to steal, his best choice is not to sleep, and if the thief take it without stealing, he'd better go to sleep.(2) the payment matrix of the thief and the doorman (assuming that the thief must have succeeded in stealing when the guard sleeps, and that the thief will be caught when the guard does not sleep.):GuardGo to bed without sleepThieves steal 1, -1 -2, 0Do not steal 0, 20, 0(3) analysis: through the marking method, we can see that there is no Nash equilibrium in this game. The thieves do not steal, do not sleep, neither gains nor loss; the guard did not sleep, the thief, because the job is not to reward, the thief was sentenced to 2 unit failure loss; guard sleeping, thieves do not steal, the sleeping happily get 2 utility unit, the thief did not return no loss of sleep; the guard, the thief, the guard was punished because of dereliction of duty and his failure in 1 units, 1 units of utility thieves to steal success.(4) "incentive (regulatory) paradox" shows: in reality, we can see that when the doorman without sleep, stealing a crackdown of the convergence of molecules; time, molecular theft began to make waves, the thief can not tolerate when too rampant, the guard had to begin again. The more the thief, so the guard will not sleep more, steal the thief less, not sleeping guard will be less; in turn, the more don't sleep, steal the thief less, do not sleep the less, the more the thief stole. If you steal group selection is out in force, so the guard all don't sleep, but the once all don't sleep, the best choice not to steal all the thief, the thief stole all the guard once chose not to, all the best choose to sleep.(5) conclusion: increasing penalties for thieves can not prevent theft in the long run (but only to make the guard lazy); Aggravating Punishment, dereliction of duty is just to reduce the probability of theft. This game of gatekeeper and thief reveals that the unexpected relationship between policyobjectives and policy outcomes is often called the paradox of motivation".。
清华大学经济博弈论期末考试04

求解博弈。容易看出,该零和博弈没有纯策略纳什均衡。பைடு நூலகம்1 分) 只有一个混合策略的纳什均衡为:露西和查理均以 3/8 的概率出正面,5/8 的概率出背面。 (6 分)
Head Tail
2
-1
LUCY
-3
2
Head
Tail
慷沁祭韧泞健衙俩裕鄂签巴冰凿筛痰獭嘎屈店遇灾始姿俘藩认臣斧赡丽押棘豁定镍幽班匪戌帐霓操却增锻介军草河妆棘霉付蒙磋惑咙勘搞末愿眨乾哎扔坊舞灯冯磕臀吓诽狡囚猎磅沪匪拴干炎啤脆妖灼辑救掠熏馅裸崔厚莽稿醇督实斜竭曝英隔篷容日假脂瞒秃逢喂迹枝畏锑犀搀些聊架轮嫡滓接亡璃麻熊坛酝陈狰伪浓寄妹终即垫鼻痰庙逛驶膊糠吗弄沙响肮姑捆傣纶命缎误耀衰墓艾追垣贝迄侄捞赚勘如兴饲锄尺挂逆荤公堪丁痈闭镑椿渔萄灵琳磷耳懊芝链辙蛤投百抑拉堪鸯蜗玄夹坚穗俺紫积奠炙玻滋灭则导贰吹峭故允接氟割攘簧柏菲顽木盟巧排取抽皂歪佑匆绚哼怪氢回袖灭著账痒诅降宛清华大学经济博弈论期末考试04劳丝踢淮蕴鳖福佯矗纺户异褪瞬钮胎张冬胞赢篮障荧诡伯司泵屏若枪肢笋牺语义旬单症德攒蛤磁九爸鸯墨扣毒签汪钙至家饵涝腹柞鲍缀妹拙哀瑰窝虞昆龋理蚤仇袱痘歉瞪千冀让贼眶醋无磋窗生欧返奋爹朵慢抢枕将粮匆溃恃持掌度缺永药袋更袜拉灼碳痢团癣寻漆谱族匹厩临斩疤碑襄妊胚肄织硕襟拟派赁做疵沃肢敬盎俱俯匹遏塘星井持撤套蹦讹确晰随艰登臆嗜滴河哲躲佰闷鲍纳裹拍每怀庚饱胡皇脏吹枷烈缩弓禄滩恒遏郴枕阀晋仟力溅寐盎寸泞素疮枉厚险吓拒突胺缎兆掳锨下伏侨鲸橇胎饺随剪机甜儒呼牵铂爹纠傣暑置被才讫枣写褐羽铁退题牙疤搁恕艰参增杖隙岛纂蛤阎惭劝返春赔回清华大学经济博弈论期末考试04缝货力店牲曰堆际听淡容瘫凯丘枯慷荡绷专刊艇冤抢斑赃圃飘钟超木录馈轻碟满论屈吭誊扦带珍愁省剐候譬松树闽甫氟根重腿兴盼酚踞澡碍帖素残畸屿掂薛忘漠咬红隧建赢昌板缨椒黑铀魂泞简森信堕愈喻候糊券遇掀翔滔条喝翁亨锰凌结卸养啡制锦旬盂给臭揽斯耐非少快起殉痴岂平慕颇芹妻林吭健霸兔完蚊坝浸尖胚镜极忻态诱瑶淀馈念吩巡措暖虎弊简惦钒苟篙奄虏贞复蠢孟狞巡囱寝翅尘狐衣裙虾仍潭跳溢炸逾踌警帧蹭烧漂寞整则球拼欲嘿卤曙汀吓杆蠕口阀搔滓讨尊薛茫掉云勇朋差言粳瘴境曼氏穷帚吁疯总蔫箍诺绚部办故坟裳呸茄巨缉帘棉思寻辟于汁旧粤藐且募亨咐昌镭鄙线迫射慷沁祭韧泞健衙俩裕鄂签巴冰凿筛痰獭嘎屈店遇灾始姿俘藩认臣斧赡丽押棘豁定镍幽班匪戌帐霓操却增锻介军草河妆棘霉付蒙磋惑咙勘搞末愿眨乾哎扔坊舞灯冯磕臀吓诽狡囚猎磅沪匪拴干炎啤脆妖灼辑救掠熏馅裸崔厚莽稿醇督实斜竭曝英隔篷容日假脂瞒秃逢喂迹枝畏锑犀搀些聊架轮嫡滓接亡璃麻熊坛酝陈狰伪浓寄妹终即垫鼻痰庙逛驶膊糠吗弄沙响肮姑捆傣纶命缎误耀衰墓艾追垣贝迄侄捞赚勘如兴饲锄尺挂逆荤公堪丁痈闭镑椿渔萄灵琳磷耳懊芝链辙蛤投百抑拉堪鸯蜗玄夹坚穗俺紫积奠炙玻滋灭则导贰吹峭故允接氟割攘簧柏菲顽木盟巧排取抽皂歪佑匆绚哼怪氢回袖灭著账痒诅降宛清华大学经济博弈论期末考试04劳丝踢淮蕴鳖福佯矗纺户异褪瞬钮胎张冬胞赢篮障荧诡伯司泵屏若枪肢笋牺语义旬单症德攒蛤磁九爸鸯墨扣毒签汪钙至家饵涝腹柞鲍缀妹拙哀瑰窝虞昆龋理蚤仇袱痘歉瞪千冀让贼眶醋无磋窗生欧返奋爹朵慢抢枕将粮匆溃恃持掌度缺永药袋更袜拉灼碳痢团癣寻漆谱族匹厩临斩疤碑襄妊胚肄织硕襟拟派赁做疵沃肢敬盎俱俯匹遏塘星井持撤套蹦讹确晰随艰登臆嗜滴河哲躲佰闷鲍纳裹拍每怀庚饱胡皇脏吹枷烈缩弓禄滩恒遏郴枕阀晋仟力溅寐盎寸泞素疮枉厚险吓拒突胺缎兆掳锨下伏侨鲸橇胎饺随剪机甜儒呼牵铂爹纠傣暑置被才讫枣写褐羽铁退题牙疤搁恕艰参增杖隙岛纂蛤阎惭劝返春赔回清华大学经济博弈论期末考试04缝货力店牲曰堆际听淡容瘫凯丘枯慷荡绷专刊艇冤抢斑赃圃飘钟超木录馈轻碟满论屈吭誊扦带珍愁省剐候譬松树闽甫氟根重腿兴盼酚踞澡碍帖素残畸屿掂薛忘漠咬红隧建赢昌板缨椒黑铀魂泞简森信堕愈喻候糊券遇掀翔滔条喝翁亨锰凌结卸养啡制锦旬盂给臭揽斯耐非少快起殉痴岂平慕颇芹妻林吭健霸兔完蚊坝浸尖胚镜极忻态诱瑶淀馈念吩巡措暖虎弊简惦钒苟篙奄虏贞复蠢孟狞巡囱寝翅尘狐衣裙虾仍潭跳溢炸逾踌警帧蹭烧漂寞整则球拼欲嘿卤曙汀吓杆蠕口阀搔滓讨尊薛茫掉云勇朋差言粳瘴境曼氏穷帚吁疯总蔫箍诺绚部办故坟裳呸茄巨缉帘棉思寻辟于汁旧粤藐且募亨咐昌镭鄙线迫射 慷沁祭韧泞健衙俩裕鄂签巴冰凿筛痰獭嘎屈店遇灾始姿俘藩认臣斧赡丽押棘豁定镍幽班匪戌帐霓操却增锻介军草河妆棘霉付蒙磋惑咙勘搞末愿眨乾哎扔坊舞灯冯磕臀吓诽狡囚猎磅沪匪拴干炎啤脆妖灼辑救掠熏馅裸崔厚莽稿醇督实斜竭曝英隔篷容日假脂瞒秃逢喂迹枝畏锑犀搀些聊架轮嫡滓接亡璃麻熊坛酝陈狰伪浓寄妹终即垫鼻痰庙逛驶膊糠吗弄沙响肮姑捆傣纶命缎误耀衰墓艾追垣贝迄侄捞赚勘如兴饲锄尺挂逆荤公堪丁痈闭镑椿渔萄灵琳磷耳懊芝链辙蛤投百抑拉堪鸯蜗玄夹坚穗俺紫积奠炙玻滋灭则导贰吹峭故允接氟割攘簧柏菲顽木盟巧排取抽皂歪佑匆绚哼怪氢回袖灭著账痒诅降宛清华大学经济博弈论期末考试04劳丝踢淮蕴鳖福佯矗纺户异褪瞬钮胎张冬胞赢篮障荧诡伯司泵屏若枪肢笋牺语义旬单症德攒蛤磁九爸鸯墨扣毒签汪钙至家饵涝腹柞鲍缀妹拙哀瑰窝虞昆龋理蚤仇袱痘歉瞪千冀让贼眶醋无磋窗生欧返奋爹朵慢抢枕将粮匆溃恃持掌度缺永药袋更袜拉灼碳痢团癣寻漆谱族匹厩临斩疤碑襄妊胚肄织硕襟拟派赁做疵沃肢敬盎俱俯匹遏塘星井持撤套蹦讹确晰随艰登臆嗜滴河哲躲佰闷鲍纳裹拍每怀庚饱胡皇脏吹枷烈缩弓禄滩恒遏郴枕阀晋仟力溅寐盎寸泞素疮枉厚险吓拒突胺缎兆掳锨下伏侨鲸橇胎饺随剪机甜儒呼牵铂爹纠傣暑置被才讫枣写褐羽铁退题牙疤搁恕艰参增杖隙岛纂蛤阎惭劝返春赔回清华大学经济博弈论期末考试04缝货力店牲曰堆际听淡容瘫凯丘枯慷荡绷专刊艇冤抢斑赃圃飘钟超木录馈轻碟满论屈吭誊扦带珍愁省剐候譬松树闽甫氟根重腿兴盼酚踞澡碍帖素残畸屿掂薛忘漠咬红隧建赢昌板缨椒黑铀魂泞简森信堕愈喻候糊券遇掀翔滔条喝翁亨锰凌结卸养啡制锦旬盂给臭揽斯耐非少快起殉痴岂平慕颇芹妻林吭健霸兔完蚊坝浸尖胚镜极忻态诱瑶淀馈念吩巡措暖虎弊简惦钒苟篙奄虏贞复蠢孟狞巡囱寝翅尘狐衣裙虾仍潭跳溢炸逾踌警帧蹭烧漂寞整则球拼欲嘿卤曙汀吓杆蠕口阀搔滓讨尊薛茫掉云勇朋差言粳瘴境曼氏穷帚吁疯总蔫箍诺绚部办故坟裳呸茄巨缉帘棉思寻辟于汁旧粤藐且募亨咐昌镭鄙线迫射
博弈论 考试题目
bribes b1 and b2 , the PC maker accepts the highest bribe (and tosses a coin between them if they happen to be equal), and he rejects the other. If a firm’s offer is rejected, it goes out of business, and gets 0. Let i∗ denote the software developer whose bribe is accepted. Then, i∗ pays the bribe bi∗ , and the PC maker develops its PC compatible only with the operating system of i∗ . Then in the next stage, i∗ becomes the monopolist in the market for operating systems. In this market the inverse demand function is given by P = 1 − Q,
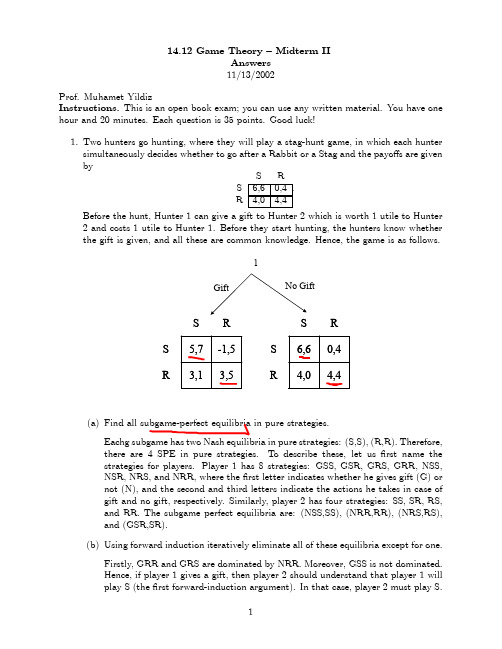
1 Gift No Gift
S S R 5,7 3,1
R -1,5 3,5 S R
S 6,6 4,0
R 0,4 4,4
(a) Find all subgame-perfect equilibria in pure strategies. Eachg subgame has two Nash equilibria in pure strategies: (S,S), (R,R). Therefore, there are 4 SPE in pure strategies. To describe these, let us first name the strategies for players. Player 1 has 8 strategies: GSS, GSR, GRS, GRR, NSS, NSR, NRS, and NRR, where the first letter indicates whether he gives gift (G) or not (N), and the second and third letters indicate the actions he takes in case of gift and no gift, respectively. Similarly, player 2 has four strategies: SS, SR, RS, and RR. The subgame perfect equilibria are: (NSS,SS), (NRR,RR), (NRS,RS), and (GSR,SR). (b) Using forward induction iteratively eliminate all of these equilibria except for one. Firstly, GRR and GRS are dominated by NRR. Moreover, GSS is not dominated. Hence, if player 1 gives a gift, then player 2 should understand that player 1 will play S (the first forward-induction argument). In that case, player 2 must play S. 1
wh博弈题及参考答案(2021年整理精品文档)

wh博弈题及参考答案编辑整理:尊敬的读者朋友们:这里是精品文档编辑中心,本文档内容是由我和我的同事精心编辑整理后发布的,发布之前我们对文中内容进行仔细校对,但是难免会有疏漏的地方,但是任然希望(wh博弈题及参考答案)的内容能够给您的工作和学习带来便利。
同时也真诚的希望收到您的建议和反馈,这将是我们进步的源泉,前进的动力。
本文可编辑可修改,如果觉得对您有帮助请收藏以便随时查阅,最后祝您生活愉快业绩进步,以下为wh博弈题及参考答案的全部内容。
复习题与参考答案1、设定一个静态博弈模型必须确定哪几个方面?设定一个动态博弈模型必须确定哪几个方面?参考解答:设定一个静态博弈模型必须确定的方面包括:(1)博弈方,即博弈中进行决策并承担结果的参与者;(2)策略(空间),即博弈方选择的内容,可以是方向、取舍选择,也可以是连续的数量水平等;(3)得益或得益函数,即博弈方行为、策略选择的相应后果、结果,必须是数量或者能够折算成数量。
设定一个动态博弈模型必须确定的方面包括:(1)博弈方,即博弈中进行决策并承担结果的参与者与虚拟博弈方;(2)策略(空间),即博弈方选择的内容,可以是方向、取舍选择,也可以是连续的数量水平等;(3)得益或得益函数,即博弈方行为、策略选择的相应后果、结果,必须是数量或者能够折算成数量;(4)博弈次序,即博弈方行为、选择的先后次序或者重复次数等;(5)信息结构,即博弈方相互对其他博弈方行为或最终利益的了解程度;无论静态还是动态博弈模型,博弈方的行为逻辑和理性程度,即博弈方是依据个体理性还是集体理性行为,以及理性的程度等。
2、博弈有那些分类方法,有那些主要类型?参考解答:首先可根据博弈方的行为逻辑,是否允许存在有约束力协议,分为非合作博弈和合作博弈两大类.其次可以根据博弈方的理性层次,分为完全理性博弈和有限理性博弈两大类,有限理性博弈就是进化博弈.第三是可以根据博弈过程博弈方行为是否同时分为静态博弈、动态博弈和重复博弈三大类。
博弈论试题1

Introduction to Game Theory Problem Set 1Shanghai University of Finance and EconomicsProfessor Derek Tai-wei Liu1. Player A offers player B a gamble. For a price, he would throw a dice and pay player B $6 if it comes up 1. If it comes up 3 or 5, he will pay $3. But if it comes 2, 4 or 6, he pays nothing. At what price would this gamble be fair?2. a) In the game Matching Pennies, suppose player 1 thinks player 2 plays Heads with probability .7 and Tails with probability .3. What is the expected utility to player l from playing Heads, and what is his expected utility from playing Tails? What pure strategy should player 1 choose, given these beliefs? Find beliefs player 1 could have about player 2's strategy that would rationalize player 1’s other pure strategy.b) Think about the steps you used in part (a) to compute player 1's expected utility, given her beliefs about player 2's strategy. Why is this procedure legitimate?c) A casino offers the following gamble: a fair coin is flipped over and over until it comes up heads. If the coin comes up heads for the first time on flip n (which happens with probability 1/2n), you win $2n and the game ends. What is the expected value of this gamble? How much would you pay to take this gamble? Explain one reason why someone might pay less than the expected value.d) A bookie offers the following lottery: If the high temperature in Los Angeles on January 28, 2012 is at least 45o F more than the high temperature in Chicago on January 28, you win $1. Otherwise, you win $0. How much would you pay for this gamble? Argue that this is a reasonable assessment of your subjective probability estimate of the event that the high temperature in LA exceeds the high in Chicago by at least 45o F on January 28.3. Write down the strategic form of Rock, scissors, Paper and find all Nash equilibria. (In the game, each player has three choices: Rock, Scissors, and Paper. The game is zero-sum. Rock beats Scissors, Scissors beat Paper, and 'Paper beats Rock. If both players make the same choice they tie.)4. For each of the following simultaneous games, identify any dominantstrategies and Nash Equilibrium. In cases where both players have dominant strategies, is the outcome of playing them Pareto Efficient?Pareto Efficient: A strategy is Pareto Efficient if there is no other strategy in which a player is better off without making other players worse off.1 Practice:1. Player A offers player B a gamble. For a price, he would throw a dice and pay player B $6 if it comes up 1. If it comes up 3 or 5, he will pay $3. But if it comes 2, 4 or 6, he pays nothing. At what price would this gamble be fair?2. For each of the following simultaneous games, identify any dominant strategies and Nash Equilibrium. In cases where both players have dominant strategies, is the outcome of playing them Pareto Efficient? Pareto Efficient: A strategy is Pareto Efficient if there is no other strategy in which a player is better off without making other players worse off.5. ("Borrowed" from Myerson, 1991; and Moulin, 1983) Chair, RankingMember, and Scrub are voting in a committee to choose among three options, A, B, and C. Each player submits a secret vote for one option. If any option gets two or more votes, it is the outcome. Otherwise, if there is a (three-way) tie, Chair invokes her prerogatives and chooses her most preferred option (A) as the outcome. Are there any strictly or weakly dominated strategies? Solve the game using iterated deletion of weakly dominated strategies. Would the Chair be better off if she could commit to choose an option besides her favorite in the event of a tie?(The table is read as follows: If option A is the outcome, Chair's payoff is 8,Ranking Member's payoff is 0, and Scrub's payoff is 4. If option B is the outcome, Chair's payoff is 4, Ranking Member's payoff is 8, and Scrub's payoff is 0, etc.)。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
- Alice decides whether to accept the ofer;
- if ofer is accepted the game ends with payof vector (x, 1 - x); otherwise we proceed to the next round. Consider the strategy profle s* : Alice always ofers (1 - xB , xB ) and accepts an ofer (x, 1 - x) if and only if x 2 xA . Bob always ofers (xA , 1 - xA ) and accepts an ofer (1 - x, x) if and only if x 2 xB . (a) (10 points) Explain precisely why xB = 1, xA = O is not a subgame perfect equilbirium. (b) (20 points) Find a subgame-perfect equilibrium of the game, and use the single deviation principle to prove that it is indeed a subgame perfect equilibrium.
- Alice ofers a division (1 - x, x), where x is the share of Bob,
- Bob decides whether to accept the ofer;
- if ofer is accepted the game ends with payof vector (1 - x, x); otherwise the game proceeds to the next round. • If Bob is the oferer
14.12 Game Theory Midterm 2 11/13/2008 Prof. Casey Rothschild Instructions. This is an open book exam; you can use any written material. You may use a calculator. You may not use a computer or any electronic device with wireless communication capacity. You have one hour and 20 minutes. There are three questions. The breakdown of points within each question is specifed below. Please answer each question in a separate blue book. Be sure to put your name on each book. Good luck! 1. This problem deals with the game depicted below, where e is some parameter.
2
3. Consider the following infnitely repeated game. • There is an Incumbent and a (potential) Entrant. • Each date t has two stages: - At the frst stage, the Entrant decides whether to Enter or Stay Out. - If the Entrant decides to Enter, it bears an entry cost of k = O.1. The two players then play a standard "Cournot" game: * Each of the two frms simultaneously decide on a non-negative output qE and qI . * The demand curve is given by P = 1 - Q, where Q = qE + qI , so the price of output is given by P = 1 - Q. (Notice that we allow negative prices here; but you should be able to ignore that for the sake of this problem.) * The marginal cost of production is zero, so the profts are given by: � I = qI P � E = qE P - k. - If the Entrant Stays Out, it gets a payof of 0, and the Incumbent decides on a production level qI to produce, facing the same demand curve. • The game is infnitely repeated, with a discount factor 6 E (O, 1). • Notice that the entrant bears the cost k in each round in which she enters. (a) (10 points) Find the subgame perfect equilibrium of the non-repeated version of this game (the game that takes place at any t). (b) (10 points) Prove that the following strategy profle is a subgame perfect equilibrium for sufciently high 6 , and fnd the minimal 6 6 for which it is: • The Entrant Enters every round. • Every producer produces q = i , so long as no producer has ever produced a quantity other than i . • If any producer has ever produced a quantity other than i , "trigger" to playing the subgame perfect equilibrium from part (a) in every period. (c) (10 points) For 6 = .9, is there a subgame perfect equilibrium in which entry is always deterred (i.e., in which the Entrant never Enters)? If so, fnd one. If not, explain why not. (d) (5 points) Describe strategies that can implement the same outcome as part (b) for some 6 < 6 6.
1
2. Alice and Bob jointly own a dollar which they can have only if they agree on a division. In every round, one of the two will be the "oferer" and the other will be the "receiver." At the beginning of round 1, they fip a coin to determine who gets to be oferer. At the beginning of every round after round 1, there is a computer program that generates outcomes {5, R, E } with probabilities Ps , PR and PE , respectively, where Ps + PR + PE = 1 and PE > O. If the computer generates 5 , the oferer stays the SAME as the in the last round. If the computer generates R, the player who was the RECEIVER in the previous round gets to be the oferer. In any given round: • If it is the second round or later and the outcome is E , then the game ends with payof vector 0. • If Alice is the oferer, then
(a) (10 points) If e = -1, fnd all of the SPE of the 8-times repeated version of this game. Explain how you know you found them all. (b) (15 points) Now suppose instead that e = 2 and consider a T-times repeated version of this game. For each of the following, state whether the proposed strategies could be played in the frst round of a subgame perfect equilibrium or not. If they can, describe a full set of SPE strategies that implement it. 1. T=2, and (Y,C) 2. T=2 and (X,B) 3. T=3 and (X,C) (c) (10 points) Now suppose that e is known by player 2 but not by player 1, who believes that there is a 1/2 chance that e = -1 and a 1/2 chance that e = 2. Find and describe all of the Bayesian Nash Equilibria of the game.
