压力容器ansys分析
压力容器及管道有限元分析(ANSYS,ABAQUS)
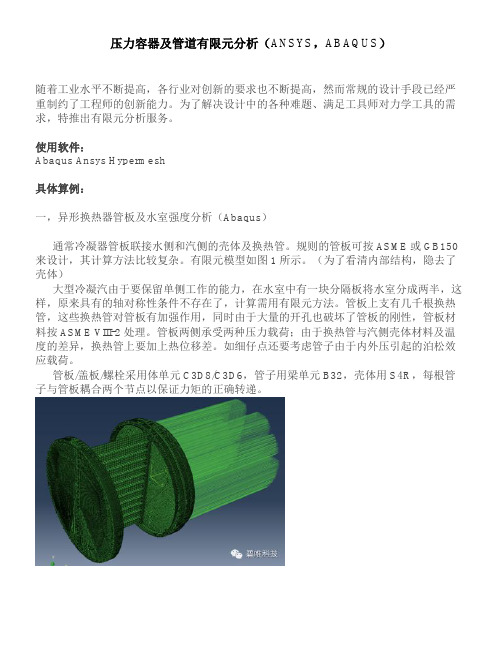
压力容器及管道有限元分析(ANSYS,ABAQUS)随着工业水平不断提高,各行业对创新的要求也不断提高,然而常规的设计手段已经严重制约了工程师的创新能力。
为了解决设计中的各种难题、满足工具师对力学工具的需求,特推出有限元分析服务。
使用软件:Abaqus Ansys Hypermesh具体算例:一,异形换热器管板及水室强度分析(Abaqus)通常冷凝器管板联接水侧和汽侧的壳体及换热管。
规则的管板可按ASME或GB150来设计,其计算方法比较复杂。
有限元模型如图1所示。
(为了看清内部结构,隐去了壳体)大型冷凝汽由于要保留单侧工作的能力,在水室中有一块分隔板将水室分成两半,这样,原来具有的轴对称性条件不存在了,计算需用有限元方法。
管板上支有几千根换热管,这些换热管对管板有加强作用,同时由于大量的开孔也破坏了管板的刚性,管板材料按ASME VIII-2处理。
管板两侧承受两种压力载荷;由于换热管与汽侧壳体材料及温度的差异,换热管上要加上热位移差。
如细仔点还要考虑管子由于内外压引起的泊松效应载荷。
管板/盖板/螺栓采用体单元C3D8/C3D6,管子用梁单元B32,壳体用S4R,每根管二,接管开口强度分析经常碰到容器上开口过大的问题,也常碰到奇形怪状的开口,或者其它一些附着物联接到容器上。
这类问题主要是建模的复杂。
图2,接管1三,异形的换热器壳体内压或外压分析通常换热器的壳子是很规则的,无论是管侧还是壳侧,都具有良好的轴对称性,即所谓的回转壳体。
回转壳体受压问题,可以用板壳理论来解,一般是有解的,这个解也正是ASMEVIII或GB150、 GB151这类规范的设计计算基础。
当壳体的轴对称性受到严重的破坏时,严格意义上来讲,原来的解是不适用了。
这时可采用数值方法来计算。
四,方形排汽管道(容器)的强度/刚性设计方形容的设计不及关心其强度,有时也要考虑其刚性,如图4所示,图4为一段排汽管道,上面还带有两组波纹管。
在工作过程,整过管道受内压或者外压,壳体会变形,有时会出现强度可以接受,但变形太大,太难看的情况,即刚度不太好。
基于ANSYS的压力容器疲劳分析与寿命预测

基于ANSYS的压力容器疲劳分析与寿命预测压力容器是工业生产中常见的设备之一,用于贮存和运输气体、液体或固体,承受着巨大的压力。
然而,由于长期的工作环境和作用力的影响,压力容器会出现疲劳现象,而疲劳失效可能导致严重事故甚至生命危险。
为了确保安全运行和提高使用寿命,进行压力容器疲劳分析与寿命预测是至关重要的。
压力容器的疲劳分析与寿命预测是一个复杂的工程问题,涉及多学科的知识。
在传统的方法中,工程师们通常依赖经验公式和试验数据进行分析,但这种方法存在一些不足之处。
首先,准确度受限于实验条件和试验数据的局限性。
其次,由于压力容器结构的复杂性,传统的方法难以考虑到各种工况变化以及应力分布的不均匀性。
因此,利用计算机辅助工程(CAE)软件进行压力容器的疲劳分析与寿命预测具有重要意义。
ANSYS作为一种强大的CAE软件,在压力容器疲劳分析的应用上已经被广泛认可。
它提供了多种分析模块,如有限元分析(FEA)、疲劳分析和寿命预测等,能够模拟复杂的结构和加载条件。
通过ANSYS的建模和分析工具,工程师们可以更加全面地了解压力容器的应力状态,并准确评估疲劳寿命。
在使用ANSYS进行压力容器疲劳分析时,首先需要进行几何建模和网格划分。
通过建模软件,可以创建一个精确的三维几何模型,并对其进行网格划分以获取一个合适的离散化模型。
然后,根据实际情况设置边界条件、加载条件和材料参数等。
在设定完成后,进行有限元分析,求解得到压力容器的应力分布。
接下来,进行疲劳分析和寿命预测。
ANSYS提供了多种疲劳分析模块,如低周疲劳、高周疲劳和疲劳寿命预测等。
根据所需分析的类型选择相应的模块,并输入相应的参数,如材料的SN曲线、载荷历程等。
通过对应力历程和SN曲线的相互作用进行计算,可以预测压力容器的疲劳寿命。
此外,还可以基于不同的疲劳损伤准则,如线性累积损伤准则、短模拟疲劳准则等,对容器的疲劳寿命进行评估和预测。
除了以上提到的分析方法,ANSYS还提供了一些辅助工具来进行压力容器的疲劳分析与寿命预测。
压力容器分析设计分析

1 问题描述利用ANSYS软件对压力容器用标准椭圆形封头和半球形封头进行应力分析,并沿着压力容器轴向方向绘制笛卡尔坐标系下X、Y、Z方向应力曲线,三个主应力曲线以及第一强度理论,第三强度理论、第四强度理论计算方法下的应力理论值和应力曲线。
相关参数:筒体内径:400mm,筒体长度为1000mm,筒体、封头厚度均为5mm,材料弹性模量为206GPa,泊松比为0.3,内压P=1MPa。
2 建模过程:单元选取:本题研究的是薄壁压力容器,对于薄壁结构,最好是选用shell单元,shell单元可以减少计算量。
而且,如果选实体单元,薄壁结构承受弯矩的时候,如果在厚度方向的单元层数太少,有时候计算结果误差比较大,反而不如shell单元计算准确。
材料特性:ANSYS 结构分析材料属性有线性 (Linear)、非线性 (Nolinear)、密度(Density)、热膨胀 (Thermal Expansion)、阻尼 (Damping)、摩擦系数 ( Friction Coefficient)、特殊材料 (Specialized Materials) 等七种。
本题选取材料模型为线弹性材料,材料参数E=206GPa,μ=0.3。
几何建模:本题采用实体建模,该方法适合于复杂模型,尤其适合于3D实体建模,需人工处理的数据量小,效率高。
允许对节点和单元实施不同的几何操作,支持布尔操作(相加、相减、相交等),支持ANSYS优化设计功能,可以进行自适应网格划分,可以进行局部网格划分,便于修正与改进。
本题采用的是从下往上的建模方式。
先建立点,再连线画圆,然后将线沿轴线旋转,得到压力容器模型,上封头为标准椭圆形封头,下封头为球形封头。
网格划分:对有限元分析,ANSYS有四种网格划分方法,自由网格划分、映射网格划分,延伸网格划分和自适应网格划分。
本题采用自由网格划分,自由网格划分功能十分强大,没有单元形状的限制,网格也不遵循任何的模式,因此适用于对复杂形状的面和体网格划分。
压力容器ansys有限元分析设计实例

ANSYS应力分析报告Stress Analysis Report学生姓名学号任课教师导师目录一. 设计分析依据 (2)1.1 设计参数 (2)1.2 计算及评定条件 (2)二. 结构壁厚计算 (3)三. 结构有限元分析 (4)3.1 有限元模型 (5)3.2 单元选择 (5)3.3 边界条件 (6)四. 应力分析及评定 (7)4.1 应力分析 (7)4.2 应力强度校核 (8)4.3疲劳分析校核 (11)五. 分析结论 (11)附录1设计载荷作用下结构应力沿路径线性化结果(A) (12)附录2设计载荷作用下结构应力沿路径线性化结果(B) (13)附录3设计载荷作用下结构应力沿路径线性化结果(C) (14)附录4设计载荷作用下结构应力沿路径线性化结果(D) (16)附录5设计载荷作用下结构应力沿路径线性化结果(E) (17)附录6设计载荷作用下结构应力沿路径线性化结果(F) (19)附录7设计载荷作用下结构应力沿路径线性化结果(G) (20)附录8设计载荷作用下结构应力沿路径线性化结果(H) (21)一. 设计分析依据(1)《压力容器安全技术监察规程》(2)JB4732-1995《钢制压力容器——分析设计标准》(2005确认版)1.1 设计参数表1 设备基本设计参数1.2 计算及评定条件(1) 静强度计算条件表2 设备载荷参数注:在计算包括二次应力强度的组合应力强度时,应选用工作载荷进行计算,本报告中分别选用设计载荷进行进行计算,故采用设计载荷进行强度分析结果是偏安全的。
(2) 材料性能参数材料性能参数见表3,其中弹性模量取自JB4732-95表G-5,泊松比根据JB4732-95的公式(5-1)计算得到,设计应力强度分别根据JB4732-95的表6-2和表6-6确定。
表3 材料性能参数性能(3) 疲劳计算条件此设备接管a 、c 上存在弯矩,接管载荷数据如表4所示。
表4 接管载荷数据表二. 结构壁厚计算按照静载荷条件,根据JB4732-95第七章(公式与图号均为标准中的编号)确定设备各元件壁厚,因介质密度较小,不考虑介质静压,同时忽略设备自重。
实例分析—运用有限元分析软件ANSYS对轴对称压力容器
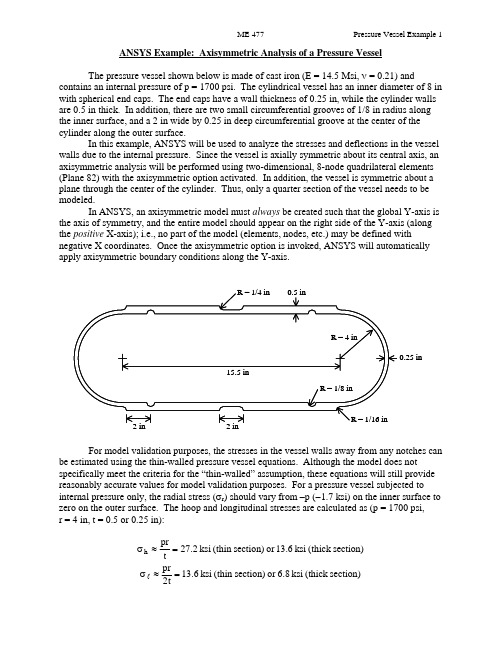
ANSYS Example: Axisymmetric Analysis of a Pressure VesselThe pressure vessel shown below is made of cast iron (E = 14.5 Msi, ν = 0.21) andcontains an internal pressure of p = 1700 psi. The cylindrical vessel has an inner diameter of 8 in with spherical end caps. The end caps have a wall thickness of 0.25 in, while the cylinder walls are 0.5 in thick. In addition, there are two small circumferential grooves of 1/8 in radius along the inner surface, and a 2 in wide by 0.25 in deep circumferential groove at the center of the cylinder along the outer surface.In this example, ANSYS will be used to analyze the stresses and deflections in the vessel walls due to the internal pressure. Since the vessel is axially symmetric about its central axis, an axisymmetric analysis will be performed using two-dimensional, 8-node quadrilateral elements (Plane 82) with the axisymmetric option activated. In addition, the vessel is symmetric about a plane through the center of the cylinder. Thus, only a quarter section of the vessel needs to be modeled.In ANSYS, an axisymmetric model must always be created such that the global Y-axis is the axis of symmetry, and the entire model should appear on the right side of the Y-axis (along the positive X-axis); i.e., no part of the model (elements, nodes, etc.) may be defined withnegative X coordinates. Once the axisymmetric option is invoked, ANSYS will automatically apply axisymmetric boundary conditions along the Y-axis.R = 1/16 inR = 1/8 inR = 1/4 in 0.5 in0.25 inR = 4 in2 in 2 in 15.5 inFor model validation purposes, the stresses in the vessel walls away from any notches can be estimated using the thin-walled pressure vessel equations. Although the model does notspecifically meet the criteria for the “thin-walled” assumption, these equations will still provide reasonably accurate values for model validation purposes. For a pressure vessel subjected to internal pressure only, the radial stress (σr ) should vary from –p (−1.7 ksi) on the inner surface to zero on the outer surface. The hoop and longitudinal stresses are calculated as (p = 1700 psi, r = 4 in, t = 0.5 or 0.25 in):section)(thick ksi 13.6or section)(thin ksi 27.2tpr h =≈σ section)(thick ksi 6.8or section)(thin ksi 6.31t2pr =≈σlANSYS Analysis:Start ANSYS Product Launcher, set the Working Directory to C:\temp, define Job Name as‘Pressure Vessel’, and click Run. Then define Title and Preferences.Utility MenuÆFileÆChange Jobname…Æ Enter ‘Pressure_Vessel’ Æ OKUtility MenuÆFileÆChange Title…Æ Enter ‘Stress Analysis of an Axisymmetric Pressure Vessel’ Æ OKANSYS Main MenuÆPreferencesÆ Preferences for GUI Filtering Æ Select ‘Structural’ and ‘h-method’ Æ OKEnter the Preprocessor to define the model geometry:Define Element Type (Axisymmetric Option) and Material Properties.ANSYS Main MenuÆPreprocessor ÆElement Type Æ Add/Edit/Delete Æ Add… ÆStructural Solid Quad 8 node 82 (PLANE82) (define ‘Element type reference number’ as 1) ÆOK Æ Click Options… Æ Select ‘Axisymmetric’ for K3 (Element behavior) Æ OK Æ Close ANSYS Main MenuÆPreprocessorÆMaterial PropsÆ Material Models Æ Double Click Structural Æ Linear Æ Elastic Æ Isotropic Æ Enter 14.5e6 for EX and 0.21 for PRXY Æ Click OK Æ Click Exit (under ‘Material’)Begin creating the geometry by defining two Circles for the spherical endcap, and Subtract Areas to create the vessel wall.ANSYS Main MenuÆPreprocessorÆModelingÆCreateÆAreasÆCircleÆ Solid Circle Æ Enter 0 for WP X, 0 for WP Y, and 4 for Radius Æ Apply Æ Enter 0 for WP X, 0 for WP Y, and 4.25 for Radius Æ OKANSYS Main MenuÆPreprocessorÆModelingÆOperateÆBooleansÆSubtractÆAreas Æ Select (with the mouse) Area 2 (bigger circle) Æ OK Æ Select Area 1 (smaller circle) Æ OKCreate Lines through the center of the Circles and Divide the Areas along these Lines.ANSYS Main MenuÆPreprocessorÆModelingÆCreateÆLinesÆLinesÆ Straight line Æ Click on the Keypoints on the outer circle which are on the X-axis to create a Line parallel to the X-axis (Circles are divided into four arcs by Ansys, with a Keypoint placed at the end of each arc). Similarly, click on the Keypoints on the outer circle which are on the Y-axis to create a Line parallel to the Y-axis Æ OKANSYS Main MenuÆPreprocessorÆModelingÆOperateÆBooleansÆDivideÆArea by Line Æ Select (with the mouse) the remaining Area (annulus)Æ OK Æ Select the two Lines that we have created Æ OKANSYS Main MenuÆPreprocessorÆModelingÆDeleteÆ Area and Below Æ Select the three Areas in the first, second, and third quadrants Æ OKDefine two Rectangles to create the walls of the cylindrical portion of the vessel (thick and thin sections). Define a Circle to create the circumferential groove on the inside of the vessel. ANSYS Main MenuÆPreprocessorÆModelingÆCreateÆAreasÆRectangleÆ By Dimensions Æ Enter 4 and 4.5 for X-coordinates and 0 and 7.75 for Y-coordinates Æ Click Apply Æ Enter 4.25 and 4.5 for X-coordinates and 6.75 and 7.75 for Y-coordinates Æ OK ANSYS Main MenuÆPreprocessorÆModeling ÆCreateÆAreasÆCircleÆ Solid Circle Æ Enter 4 for WP X, 2 for WP Y, and 1/8 for Radius Æ OKSubtract Areas to eliminate unused segments, and then Add all Areas to create a single Area for meshing.ANSYS Main MenuÆPreprocessorÆModelingÆOperateÆBooleansÆSubtractÆAreas Æ Select (with the mouse) the bigger rectangle Æ OK Æ Select the small rectangle and circle Æ OKANSYS Main MenuÆPreprocessorÆModelingÆOperateÆBooleansÆAddÆ Areas Æ Select ‘Pick All’ Æ OKCreate Line Fillets at the two transitions between the thick and thin sections.Utility Menu Æ Plot ÆLinesUtility Menu Æ Plot CtrlsÆNumbering…Æ Click ‘Line numbers’ On Æ OKANSYS Main MenuÆPreprocessorÆModelingÆCreateÆLinesÆ Line Fillet Æ Select (with the mouse) the two Lines near the lower Fillet Æ OK Æ Enter 1/16 for Fillet radius ÆApply Æ Select the two Lines near the upper Fillet Æ OK Æ Enter 1/4 for Fillet radius Æ OK Create Areas within the two Fillets and add these Areas to the main Area. First zoom in on the area of interest using the plot controls.ANSYS Main MenuÆPreprocessorÆModelingÆCreateÆAreasÆArbitraryÆ By Lines Æ Select (with the mouse) the Fillet and adjacent two Lines Æ OKRepeat for the other Fillet.ANSYS Main MenuÆPreprocessorÆModelingÆOperateÆBooleansÆAddÆ Areas Æ Select ‘Pick All’ Æ OKUtility Menu Æ Plot ÆLinesThe geometry should appear as shown below in the figure on the left.In this example, the irregular geometry will be Free Meshed with Quad Elements. Better control of Element sizing and distribution can be obtained with Mapped Meshing, but this would require that additional sub-Areas be defined within the main Area that have a regular (four-sided) geometry. Using Free Meshing, all Elements in the model will be approximately the same size. In the first run, we will choose a Global Size (approximate Element edge length) of 0.1 in. ANSYS Main MenuÆPreprocessorÆMeshingÆ MeshTool Æ Under ‘Size Controls: Global’ click Set Æ Enter 0.1 for ‘Element edge length’ ÆOK Æ Under ‘Mesh:’ select Areas, Quad and Free Æ Click Mesh Æ Select (with the mouse) the Area Æ OKEnter the Solution Menu to define boundary conditions and loads and run the analysis: ANSYS Main MenuÆSolutionÆAnalysis TypeÆ New Analysis Æ Select Static Æ OK The Boundary Conditions and Loads can now be applied. ANSYS will automatically apply the Axisymmetric Boundary Conditions along the Y-axis. However, we must apply the Symmetry Boundary Conditions along the upper edge of the model. Finally, the Pressure can be applied on all lines that make up the inner surface of the vessel. The magnitude should be input as the actual value – no reduction is needed to account for axisymmetry (ANSYS automatically makes the necessary adjustment of Loads in an Axisymmetric model).ANSYS Main MenuÆSolutionÆDefine LoadsÆApplyÆStructuralÆDisplacement ÆSymmetry B.C.Æ On Lines Æ Select the Line on top of the model (19) Æ OKANSYS Main MenuÆSolutionÆDefine LoadsÆApplyÆStructuralÆPressureÆ On Lines Æ Select (with the mouse) all the Lines on the inside of the vessel (20,12,16,17 and 2) ÆOK Æ Enter 1700 for ‘Load PRES value’ Æ OKThe pressure will be indicated by arrows, as shown above in the figure on the right.Save the Database and initiate the Solution using the current Load Step (LS).ANSYS Toolbar Æ SAVE_DBANSYS Main MenuÆSolutionÆSolveÆ Current LS Æ OK Æ Close the information window when solution is done Æ Close the /STATUS Command windowEnter the General Postprocessor to examine the results:First, plot the Deformed Shape.ANSYS Main MenuÆGeneral PostprocÆPlot ResultsÆ Deformed Shape Æ Select Def + undeformed Æ OKA Contour Plot of any stress component can be created. The radial, hoop (tangential), and longitudinal stresses should be checked to verify the model. Also, stress values at any particular node can be checked by using the “Query Results” command, selecting the desired component, and then picking the appropriate node. For this model, along the cylindrical portion of the vessel, x represents the radial direction, y represents the longitudinal direction, and z represents the hoop (tangential) direction. Powergraphics must be disabled to query results at nodes. ANSYS Toolbar Æ POWRGRPH Æ Select OFF Æ OKANSYS Main MenuÆGeneral PostprocÆPlot ResultsÆContour PlotÆ Nodal Solu ÆSelect ‘Stress’ and ‘X-Component of stress’ (or Y or Z) Æ OKANSYS Main MenuÆGeneral PostprocÆQuery ResultsÆ Nodal Solution Æ Select‘Stress’ and ‘X-direction SX’ (or SY or SZ) Æ OK Æ Select Nodes in the region of interest (may be helpful to zoom in on region)Compare the finite element stresses to the values calculated using the thin-wall equations. If the values are within reason (away from notches, etc.), proceed. For the purposes of failure analysis, we must select an appropriate failure theory. A plot of the von Mises stress is useful for identifying critical locations in the vessel. However, since the vessel is made of cast iron (brittle material), the “Maximum-Normal-Stress” failure criterion may be more appropriate (or Coulomb-Mohr or other similar failure theories). Create Contour Plots of the von Mises and 1st Principal stresses.ANSYS Main MenuÆGeneral PostprocÆPlot ResultsÆContour PlotÆ Nodal Solu ÆSelect ‘Stress’ and ‘von Mises stress’ Æ OKANSYS Main MenuÆGeneral PostprocÆPlot ResultsÆContour PlotÆ Nodal Solu ÆSelect ‘Stress’ and ‘1st Principal stress’ Æ OKThe plot of the model can be expanded around the axisymmetric axis to get a better view of the full model. For this plot, Powergraphics must be enabled.ANSYS Toolbar Æ POWRGRPH Æ Select ON Æ OKUtility Menu Æ PlotCtrlsÆStyleÆ Symmetry Expansion Æ 2-D Axi-Symmetric… Æ Select ‘Full expansion’ Æ OKNote the locations of the maximum stresses in the vessel. Are the critical locations where you would expect them to be? If not, why? Do you think the current model is accurate, or might there be some discretization error? Record the magnitudes and locations of the maximum stresses, and then refine the mesh and re-run the analysis to check for possible discretization error.。
压力容器管板的ANSYS有限元分析

用ANSYS软件进行压力容器管板的有限元分析序言压力容器管板是压力容器重要部件,根据管板结构的特点,它直接影响着管箱的承压能力。
它的变形情况及应力分析对整个箱管结构的应力分析起着决定性的作用。
然而J摺佣解析法对压力容器管板所受的应力和应变情况分析,解析误差太大。
采用ANSYS有限元分析软件建立压力容器管板的有限元模型,加载求解进行应力场分析对算出压力容器管板的最大应力泣变,利用ANSYS的有限元分析和计算机图形学功能显示三维应力等值面应移等值面,从而为压力容器管板机构的优化分析提供了充分的理论依据。
1基本分过程1.1创建有限元模型本文选用一种U型管式的压力容器来建模,管板材料选用20MuMo 锻件。
球形封头材料16MnR,材料的弹性模量E=20E+05MPa.泊松比为03,密度为7.8t/m3,设计压力P=31.4MPa,许用应力为196MPa。
在压力容器的应力的分析中,压力容器部件设计关心的是应力沿壁厚的分布规律及其大小,可采用沿壁厚方向的校核线代替校核面。
另外由于压力容器是轴对称结构,所以可选其一半结构来建模。
为了节省时间和存储空间,而又不影响分析结果,根据其结构,略去一些细节。
其中管孔对于管板强度的削弱,可以采用有效弹性模量E1和有效泊松比V1的概念将管板折算为同厚度的当量无孔圆平板,因此管板区域分为两大部分,1区按等效圆板来处理,而2区按实际悄况处理。
根据相关文献得到E1=054F,V1=0360综上所述,所得简化后有限元分析模型如图1所示:图1有限元分析模型1.2网格划分通常ANSYS的网格划分有两种方法,即自由划分和映射划分。
自由划分网格主要用于划分边界形状不规则的区域,分析稍度不够高,但要求划分的区域满足一定的拓补条件。
奕淞」分网格主要适合与敖钡臼形体,分析精度高。
鉴于压力容器管板的结构特点,本文同时采用了这两种方法。
在非边界区域采用醉编寸网格划分,在边界区域及梢度要求不是很高的区域采用自由网格划分。
压力容器ansys分析.

高压空气储气罐ANSYS 应力分析
压力容器是在冶金、化工、炼油、气体等工业生产中频繁使用,常常用来存储各类不同压力、温度、介质的气体,或被使用为干燥罐,蒸压釜、反应釜、缓冲罐、医用氧气瓶等等。
同时大部分罐都属于特种设备—压力容器,其制造和使用国家都有严格规范标准,特别是压力容器的疲劳强度和形体薄弱环节的研究对于特种设备的安全使用很重要,这里借助于ansys软件很直观精确地将其中一种压力容器—高压空气储气罐进行了疲劳分析之一—压力应力分析。
一、高压储气罐的设计条件:
①
建立几何模型
② 由于该容器形体的对称性,选择1/4 来分析:
三、加载求解
四、结果分析。
基于ANSYS的压力容器有限元分析及优化设计

317压力容器是一种能够承受压力的密闭容器,广泛应用于煤化工生产领域。
煤化工生产作业环境苛刻,需要其外壳具备较高的强度,保护内部电子元器件不被损坏。
为验证压力容器的耐压性能,需根据其工作条件设计压力容器,将机器人安装在压力容器内部,对压力容器进行加压以模拟其高压工作环境,检测外壳的耐压性能是否符合要求。
本文基于国标 GB150-2011中关于压力容器的规定,完成压力容器的各项参数的计算取值。
利用 ANSYS 有限元仿真软件对其进行校核,对该压力容器工作状态下的应力及变形情况进行分析,判断其结构强度及 O 形圈的密封效果是否符合要求[1]。
1 压力容器参数化设计 对实际工况进行分析,根据要求完成压力容器的初步设计,结构如图 1 所示。
图1 压力容器三维模型该压力容器主要由两部分组成:压力舱和平盖,两个部件通过螺栓连接,平盖挤压压力舱端面上的 O 形圈完成密封。
由于采用水作为介质进行加压维持压力舱内压力处于预定值,压力容器需经常浸泡在水环境中,容易腐蚀生锈,会对密封结构造成破坏,且存在安全隐患,因此采用不锈钢完成该压力容器的设计和制造。
平盖所承受的应力较大,工作时容易产生较大变形导致 O 形圈密封失效,因此平盖需采用高强度不锈钢材料。
20Cr13是一种常用的高强度马氏体不锈钢材料,具有高抗蚀性、高强度、高韧性和较强抗氧化性,被广泛应用于制造各种承受高应力的零件。
基于20Cr13的优良性能,选用该材料用于平盖的设计和制造[2]。
与平盖相比较,压力舱承受应力相对较小,选用 304 不锈钢用于压力舱的设计和制造。
基于国标 GB150-2011 关于压力容器的规定,对压力容器各部分的参数进行计算如下:(1)壳体厚度计算: 圆筒厚度计算公式如下:[]c ii c P D −=φσδ2P(1)式中,σ为圆筒壳体计算厚度(mm);p c 为计算压力(MPa);D i 为圆筒内直径(mm),[σ]i 为壳体材料的许用应力(MPa),φ为焊接接头系数。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
压力容器是在冶金、化工、炼油、气体等工业生产中频繁使用,常常用来存储各类不同压力、温度、介质的气体,或被使用为干燥罐,蒸压釜、反应釜、缓冲罐、医用氧气瓶等等。同时大部分罐都属于特种设备—压力容器,其制造和使用国家都有严格规范标准,特别是压力容器的疲劳强度和形体薄弱环节的研究对于特种设备的安全使用很重要,这里借助于ansys软件很直观精确地将其中一种压力容器—高压空气储气罐进行了疲劳分析之一—压力应力分析。
一、高压储气罐的设计条件:
①
②设计数据:
二、有限元模型建立:
1定义设计变量:
建立几何模型
2由于该容器形体的对称性,选择1/4来分析:
三、加
