2007.10 OE Jianping Yao Switchable optical UWB monocycle and doublet generation using a reconfig
16-100A 16-100电流重型,600V三极配置说明书
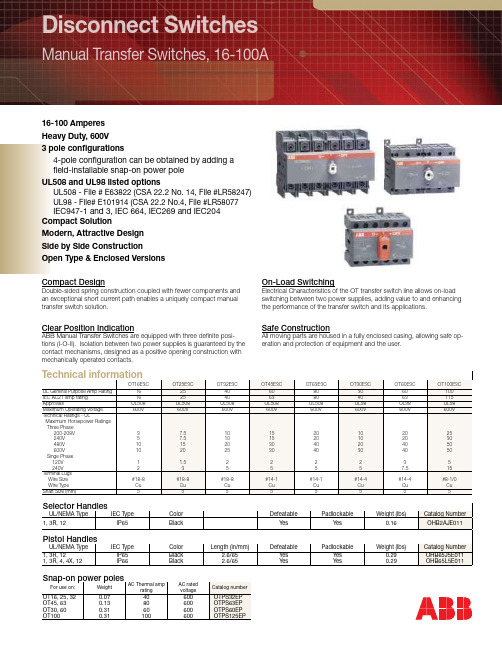
Technical information
OT16E3C
UL General Purpose Amp Rating IEC AC21 amp rating Approvals
Maximum Operating Voltage Technical Ratings - UL
Maximum Horsepower Ratings Three Phase
Defeatable
Yes Yes
Padlockable
Yes Yes
Weight (lbs)
0.29 0.29
Catalog Number
OHB65J5E011 OHB65L5E011
OT16, 25, 32E3C & E4C – Transfer switches
1.38 35
0.18 DIA. 4.5
On-Load Switching
Electrical Characteristics of the OT transfer switch line allows on-load switching between two power supplies, adding value to and enhancing the performance of the transfer switch and its applications.
Clear Position Indication
ABB Manual Transfer Switches are equipped with three definite positions (I-O-II). Isolation between two power supplies is guaranteed by the contact mechanisms, designed as a positive opening construction with mechanically operated contacts.
Instruction 说明书

Follow instruction manualThe instruction manual is part of the product and an important element within the safety concept.• Read and follow instruction manual.• A lways keep instruction manual available for the product.• P ass on instruction manual to all subsequent users of the product.1. Intended useThe product is applicable for liquids with specific conductivity over 10 µS/cm. Theswitching unit can sense the resistance between probes. Conductivity measurement is suitable only for detecting the presence of liquid at a given level of the tank. This level is represented by the length of the probe. The conductive switch is suitable for fìlling or emptying control with 2 to 4 relay outputs working simultaneously or for level detection of 2-4 independent levels (in 1 or 2 tanks) with 2 independent relay outputs.2. Safety and responsibilityIn order to provide safety in the plant, the operator is responsible for the following measures:• P roducts may only be used for its intended purpose, see intended use • N ever use a damaged or defective product. Immediately sort out damaged product.• M ake sure that the piping system has been installed professionally and serviced regularly.• P roducts and equipment shall only be installed by persons who have the required training, knowledge or experience.• R egularly train personnel in all relevant questions regarding locally applicableregulations, safety at work, environmental protection especially for pressurised pipes.The personnel is responsible for the following measures:• K now, understand and follow the instruction manual and the advices therein.3. FunctionThe level switch consists of 1 or 2 switching unit and the KLN-2 type probes. Probes are to be connected to the 2281 type probe socket head that can be screwed into the tank. lf the material of the tank or its internal insulation is not conductive then a reference probe should be used in addition to the one, two, three or four probe(s), if the material of the tank is conductive, the tank can be used as a reference probe.4. Technical Data4.1 Technical Data of the switching unitProbe Voltage 5 V AC Probe current < 1 mA ACSensitivityAdjustable: 5 kΩ ... 100 kΩMax. cable capacity 4 nF Response max. 400 ms Setting accuracy (mech.)± 5 %Delay Adjustable: 0.5 ... 10 s Relay output 2x SPDTSwitching voltage 250 V AC1, 24 V DC Switching current 16A AC1Switching power 4000 vA AC1. 384 W DC Electrical strength 4 kVMechanical life-span 3 x107 switches Electrical life-span 0,7x105 switches Power supply Un 24 V AC/DCVoltage range allowed nominal voltage -15 %...+100 %Power consumption max. 2.5 VA/ W Ambient temperature -20°C ... +55°CElectrical connection max. 2.5 mm 2 / with insulation 1.5 mm 2Electrical protection Class III Ingress protection IP 20Mechanical connection DIN EN 60715 rail Mass240 g6. Installation• Mount s witching unit on DIN EN 60715 rail.• Cut the KLN-2 type probes to the length required for level detection on site. • Screw probes into the sockets.• Tighten the probe with an M6 nut.•Use separators at every 0.5 m for multiple probe devices to keep the probes apart.7. Electrical Connectionlf the wall of the tank is conductive no reference probe is needed, ln this case terminal C is to be connected to the tank. On multiple probe units E1 and E2 are marked with 1,,.4, the reference probe is marked with C. Admissible length of cable between signal processor and probes depends on cable capacity and conductivity. Make sure E1 in upper level, E2 buttom level.8. Putting into operation8.1 AdjustmentThe green LED (U n ) shows that the unit is on, the energized state of the relays areindicated by the E1 respectively E2 LEDs. Operating mode, delay ON and delay OFF can be set with the DIP switch on the front panel. tE1(s) and tE2(s) potentiometers are for adjusting the delay time. The sensitivity setting (R potentiometer) should comply with lhe conductivity of the fluid. Do not set sensitivity higher than required because the vapour precipilation may lead to operation disturbance.Selection of delay type for input E1ta: delay OFF Georg Fischer Piping Systems Ltd CH-8201 Schaffhausen Phone +41(0)52 631 30 26 / info.ps @georgfischer .com / GFDO 6354_4 (07.14)© Georg Fischer Rohrleitungssysteme AG CH-8201 Schaffhausen/Schweiz, 2014Printed in SwitzerlandInstruction manual2281 Conductive multipoint switch with dual channel relayGF Piping SystemsGeorg Fischer Piping Systems Ltd CH-8201 SchaffhausenPhone +41(0)52 631 30 26 / info.ps@ /GFDO 6354_4 (07.14)© Georg Fischer Rohrleitungssysteme AGCH-8201 Schaffhausen/Schweiz, 2014Printed in Switzerland8.2 Level detectionThe relay allows level detection of 2 independent levels even in one tank or in two separated tanks.8.3 State of relaysE1 E2 E1 E2 E1 E2 E1 E2Function 2xDelay type E1 taFunction 2xDelay type E1 taFunction 2xDelay type E1 taFunction 2xDelay type E1 ta1xONtbtb1xONtbtb1xONtbtb1xONtbtb8.4 Level controlSelection of contacts depends on required function.9. Maintenance, RepairThe device does not require regular maintenance. Repair within and beyond the wanantyperiod is carried out at the manufacturer‘s location.10. Storage• Ambient temperature: -30 to +70 °C• Relative humidity: max. 85%11. DisclaimerThe technical data are not binding. They neither constitute expressly warranted cha-racteristics nor guaranteed properties nor a guaranteed durability.They are subject to modification. Our General Terms of Sale apply.。
Materials Studio 培训教程

Materials Studio 培训教目录Materials Studio 快速入门教程⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯2 Visualizer 模块快速入门教程⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯11用第一性原理预测AlAs 的晶格参数⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯36 CO 分子在Pd(110)表面的吸附⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯43Pd(110)面上的CO 分子电荷密度变化⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯55模拟CO_Pd(110)体系的STM 图⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯61使用DMol3 中的离域内坐标对固体进行几何优化⋯⋯⋯⋯⋯⋯64 用LST/QST 搜索过渡态⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯69气体在聚合体中扩散的测量⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯76聚合物与金属氧化物表面的相互作用⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯86计算共存相之间的界面张力⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯96运行简单的MesoDyn 模拟⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯99使用粉末衍射图进行分析⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯108指标化粉末衍射图⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯117无机物的Rietveld 精修⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯125使用Reflex Plus 来解析3-氯-反-苯乙烯酸的结构⋯⋯⋯⋯⋯⋯⋯133 无机化合物FIN31 的结构确定⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯⋯142创腾科技有限公司Neotrident Technology Limited 2Materials Studio 快速入门教程该教程将介绍Materials Studio 软件的基本功能,在这一部分,你将学到:1.生成Projects2.打开并且观察3D 文档3.绘制苯甲酰胺分子4.观察并且处理研究表格文档5.处理分子晶体:尿素6.建造Alpha 石英晶体7.建造多甲基异丁烯酸盐8.保存Project 并结束1. 生成Projects(1).运行Material Visualizer从运行菜单中运行或者在桌面点击快捷方式。
switch case语句java的用法

switch case语句java的用法
在Java中,switch-case语句用于多分支条件判断。
它的基本语法如下:
```java
switch (expression) {
case value1:
// 执行语句块1
break;
case value2:
// 执行语句块2
break;
case value3:
// 执行语句块3
break;
// 可以有更多的case
default:
// 默认情况下执行的语句块
break;
}
```
- `expression` 是一个表达式,用于进行条件判断。
-`value1`, `value2`, `value3` 等是每个case要与`expression`进行比较的值。
-当`expression`的值与某个`case`的值相等时,就会执行对应的语句块。
-如果没有匹配到任何的`case`,则会执行`default`语句块(可选的)。
- `break` 语句用于跳出switch-case 结构。
注意事项:
- `expression` 只能是byte、short、int、char和enum类型。
- `case`后面的值必须是常量或常量表达式,不能是变量或方法调用等。
-每个`case`后面需要使用`break`来结束语句块,否则会继续执行下一个`case`的语句块。
-如果省略了`break`,则会继续执行后面的`case`或`default`语句块,这种情况称为"case穿透"。
-如果不需要任何处理,可以省略`default`语句块。
Leshan Radio Company LBC807型号一般目的调制晶体管说明书

1General Purpose TransistorsLBC807-16LT1G LBC807-25LT1G LBC807-40LT1GSOT–231. FR–5 = 1.0 x 0.75 x 0.062 in.2. Alumina = 0.4 x 0.3 x 0.024 in. 99.5% alumina.PNP SiliconFEATUREƽCollector current capability I C =-500 mA.ƽCollector-emitter voltage V CEO (max) = -45 V.ƽGeneral purpose switching and amplification.ƽPNP complement: LBC807 Series.ƽDEVICE MARKING AND ORDERING INFORMATIONDeviceMarkingShipping LBC807-16LT1G 5A13000/Tape&Reel LBC807-16LT3G5A110000/Tape&Reel LBC807-25LT1G 5B13000/Tape&Reel LBC807-25LT3G5B110000/Tape&Reel LBC807-40LT1G 5C13000/Tape&Reel LBC807-40LT3G5C110000/Tape&ReelMAXIMUM RATINGSRatingSymbol Value Unit Collector–Emitter Voltage V CEO –45V Collector–Base Voltage V CBO –50V Emitter–Base VoltageVEBO–5.0V Collector Current — ContinuousI C–500mAdcTHERMAL CHARACTERISTICSCharacteristicSymbol MaxUnitTotal Device Dissipation FR– 5 Board, (1)P DT A = 25°C225mW Derate above 25°C1.8mW/°C Thermal Resistance, Junction to Ambient R θJA 556°C/WT otal Device DissipationP DAlumina Substrate, (2) T A = 25°C 300mW Derate above 25°C2.4mW/°C Thermal Resistance, Junction to Ambient R θJA 417°C/W Junction and Storage T emperatureT J , T stg–55 to +150°CEMITTER1We declare that the material of product compliance with RoHS requirements.23LBC807-16LT1G,LBC807-25LT1G,LBC807-40LT1GELECTRICAL CHARACTERISTICS(T A= 25°C unless otherwise noted.)Characteristic Symbol Min Typ Max UnitOFF CHARACTERISTICSCollector–Emitter Breakdown Voltage(I C= –10 mA)V(BR)CEO–45——VCollector–Emitter Breakdown Voltage(V EB= 0, I C = –10µA)V(BR)CES–50——VEmitter–Base Breakdown Voltage(I E= –1.0µA)V(BR)EBO–5.0——VCollector Cutoff Current I CBO(V CB= –20 V)——–100nA(V CB= –20 V, T J= 150°C)——–5.0µA ELECTRICAL CHARACTERISTICS(T A= 25°C unless otherwise noted)Characteristic Symbol Min Typ Max UnitON CHARACTERISTICSDC Current Gain h FE—(I C= –100 mA, V CE= –1.0 V) LBC807–16100—250LBC807–25160—400LBC807–40250—600(I C= –500 mA, V CE= –1.0 V) 40——Collector–Emitter Saturation VoltageV CE(sat)——–0.7 V(I C = –500 mA, I B = –50 mA)Base–Emitter On VoltageV BE(on) — — –1.2 V(I C = –500 mA, V CE= –1.0 V)SMALL–SIGNAL CHARACTERISTICSCurrent–Gain — Bandwidth Productf T100— — MHz(I C = –10 mA, V CE = –5.0 V dc, f = 100 MHz)Output Capacitance C—10—pFobo(V CB = –10 V, f = 1.0 MHz)LESHAN RADIO COMPANY, LTD.LBC807-16LT1G,LBC807-25LT1G,LBC807-40LT1GTYPICAL CHARACTERISTICS −LBC807−16LT1GFigure 1. DC Current Gain vs. CollectorCurrentFigure 2. Collector Emitter Saturation Voltagevs. Collector CurrentI C , COLLECTOR CURRENT (A)I C , COLLECTOR CURRENT (A)Figure 3. Base Emitter Saturation Voltage vs.Collector Current Figure 4. Base Emitter Voltage vs. CollectorCurrentI C , COLLECTOR CURRENT (A)I C , COLLECTOR CURRENT (A)h F E , D C C U R R E N T G A I NV C E (s a t ), C O L L E C T O R −E M I T T E RS A T U R A T I O N V O L T A G E (V )V B E (s a t ), B A S E −E M I T T E R S A T U R A T I O N V O L T A G E (V )V B E (o n ), B A S E −E M I T T E R V O L T A G E (V )LESHAN RADIO COMPANY, LTD.LBC807-16LT1G,LBC807-25LT1G,LBC807-40LT1GTYPICAL CHARACTERISTICS − LBC807−16LT1GI B , BASE CURRENT (mA)Figure 5. Saturation Region100101.0V R , REVERSE VOLTAGE (VOLTS)Figure 6. Temperature CoefficientsIC , COLLECTOR CURRENTFigure 7. CapacitancesV C E , C O L L E C T O R -E M I T T E R V O L T A G E (V O L T S )V , T E M P E R A T U R E C O E F F I C I E N T S (m V /C )°θC , C A P A C I T A N C E (p F )LESHAN RADIO COMPANY, LTD.LBC807-16LT1G,LBC807-25LT1G,LBC807-40LT1GTYPICAL CHARACTERISTICS − LBC807−25LT1GFigure 8. DC Current Gain vs. CollectorCurrentFigure 9. Collector Emitter Saturation Voltagevs. Collector CurrentI C , COLLECTOR CURRENT (A)I C , COLLECTOR CURRENT (A)100200300400500Figure 10. Base Emitter Saturation Voltage vs.Collector CurrentFigure 11. Base Emitter Voltage vs. CollectorCurrentI C , COLLECTOR CURRENT (A)I C , COLLECTOR CURRENT (A)0.20.30.50.60.70.81.01.1h F E , D C C U R R E N T G A I NV C E (s a t ), C O L L E C T O R −E M I T T E R S A T U R A T I O N V O L T A G E (V )V B E (s a t ), B A S E −E M I T T E R S A T U R A T I O N V O L T A G E (V )V B E (o n ), B A S E −E M I T T E R V O L T A G E (V )0.40.9Figure 12. Current Gain Bandwidth Productvs. Collector CurrentI C , COLLECTOR CURRENT (A)f T , C U R R E N T −G A I N −B A N D W I D T H P R O D U C T (M H z )LESHAN RADIO COMPANY, LTD.LBC807-16LT1G,LBC807-25LT1G,LBC807-40LT1GTYPICAL CHARACTERISTICS − LBC807−25LT1GI B , BASE CURRENT (mA)Figure 13. Saturation Region100101.0V R , REVERSE VOLTAGE (VOLTS)Figure 14. Temperature CoefficientsI C , COLLECTOR CURRENTFigure 15. CapacitancesV C E , C O L L E C T O R -E M I T T E R V O L T A G E (V O L T S )V , T E M P E R A T U R E C O E F F I C I E N T S (m V /C )°θC , C A P A C I T A N C E (p F )LBC807-16LT1G,LBC807-25LT1G,LBC807-40LT1GTYPICAL CHARACTERISTICS − LBC807−40LT1GFigure 16. DC Current Gain vs. CollectorCurrentFigure 17. Collector Emitter Saturation Voltagevs. Collector CurrentI C , COLLECTOR CURRENT (A)I C , COLLECTOR CURRENT (A)Figure 18. Base Emitter Saturation Voltage vs.Collector CurrentFigure 19. Base Emitter Voltage vs. CollectorCurrentI C , COLLECTOR CURRENT (A)I C , COLLECTOR CURRENT (A)h F E , D C C U R R E N T G A I NV C E (s a t ), C O L L E C T O R −E M I T T E R S A T U R A T I O N V O L T A G E (V )V B E (s a t ), B A S E −E M I T T E R S A T U R A T I O N V O L T A G E (V )V B E (o n ), B A S E −E M I T T E R V O L T A G E (V )Figure 20. Current Gain Bandwidth Productvs. Collector CurrentI C , COLLECTOR CURRENT (A)f T , C U R R E N T −G A I N −B A N D W I D T H P R O D U C T (M H z )LBC807-16LT1G,LBC807-25LT1G,LBC807-40LT1GTYPICAL CHARACTERISTICS − LBC807−40LT1GI B , BASE CURRENT (mA)Figure 21. Saturation RegionV R , REVERSE VOLTAGE (VOLTS)Figure 22. Temperature Coefficients +1.0I C , COLLECTOR CURRENTFigure 23. Capacitances-2.0-1.0V C E , C O L L E C T O R -E M I T T E R V O L T A G E (V O L T S )V , T E M P E R A T U R E C O E F F I C I E N T S (m V /C )°θC , C A P A C I T A N C E (p F )-1.0-0.8-0.6-0.4-0.2LESHAN RADIO COMPANY, LTD.LBC807-16LT1G,LBC807-25LT1G,LBC807-40LT1GTYPICAL CHARACTERISTICS −LBC807−16LT1G, LBC807−25LT1G, LBC807−40LT1GFigure 24. Safe Operating AreaV CE , COLLECTOR EMITTER VOLTAGE (V)I C , C O L L E C T O R C U R R E N T (A )LESHAN RADIO COMPANY, LTD.NOTES:1. DIMENSIONING AND TOLERANCING PER ANSIY14.5M, 1982.2. CONTROLLING DIMENSION: INCH.INCHES MILLIMETERSDIM MIN MAX MIN MAX A 0.11020.1197 2.80 3.04B 0.04720.0551 1.20 1.40C 0.03500.04400.89 1.11D 0.01500.02000.370.50G 0.07010.0807 1.78 2.04H 0.00050.00400.0130.100J 0.00340.00700.0850.177K 0.01400.02850.350.69L 0.03500.04010.89 1.02S 0.08300.1039 2.10 2.64V0.01770.02360.450.60SOT-23LBC807-16LT1G,LBC807-25LT1G,LBC807-40LT1G。
安捷伦产品目录

15
Real-Time PCR
16
Mx3000P QPCR System
17
Brilliant III Ultra-Fast SYBR Green QPCR and QRT-PCR Reagents
18
Brilliant III Ultra-Fast QPCR and QRT-PCR Reagents
Agilent / STRATAGENE
Agilent website: /genomics
Welgene | Agilent Stratagene
威健股份有限公司 | Stratagene 總代理
Table of Content
Table of Contents
/ XL1-Red Competent Cells SoloPack Gold Supercompetent Cells
/ TK Competent Cells Specialty Cells
/ Classic Cells / Fine Chemicals For Competent Cells
適用於 UNG 去汙染或 bisulphite
sequencing
適用於 TA Cloning
最高敏感性
取代傳統 Taq 的好選擇
-
2
威健股份有限公司 | Stratagene 總代理
PCR Enzyme & Instrument
Agilent SureCycler 8800
市場上領先的 cycling 速度和 sample 體積 10 ~ 100 μL 簡易快速可以選擇 96 well 和 384 well 操作盤 優秀的溫控設備讓各個 well 都能保持溫度的穩定 七吋的高解析度觸控螢幕讓操作上更為簡便 可以透過網路遠端操控儀器及監控儀器 Agilent 專業的技術支援可以幫助您應對各種 PCR 的問題
INTERNATIONAL JOURNAL OF CIRCUIT THEORY AND APPLICATIONS

INTERNATIONAL JOURNAL OF CIRCUIT THEORY AND APPLICATIONSInt.J.Circ.Theor.Appl.2006;34:559–582Published online in Wiley InterScience().DOI:10.1002/cta.375A wavelet-based piecewise approach for steady-state analysisof power electronics circuitsK.C.Tam,S.C.Wong∗,†and C.K.TseDepartment of Electronic and Information Engineering,Hong Kong Polytechnic University,Hong KongSUMMARYSimulation of steady-state waveforms is important to the design of power electronics circuits,as it reveals the maximum voltage and current stresses being imposed upon specific devices and components.This paper proposes an improved approach tofinding steady-state waveforms of power electronics circuits based on wavelet approximation.The proposed method exploits the time-domain piecewise property of power electronics circuits in order to improve the accuracy and computational efficiency.Instead of applying one wavelet approximation to the whole period,several wavelet approximations are applied in a piecewise manner tofit the entire waveform.This wavelet-based piecewise approximation approach can provide very accurate and efficient solution,with much less number of wavelet terms,for approximating steady-state waveforms of power electronics circuits.Copyright2006John Wiley&Sons,Ltd.Received26July2005;Revised26February2006KEY WORDS:power electronics;switching circuits;wavelet approximation;steady-state waveform1.INTRODUCTIONIn the design of power electronics systems,knowledge of the detailed steady-state waveforms is often indispensable as it provides important information about the likely maximum voltage and current stresses that are imposed upon certain semiconductor devices and passive compo-nents[1–3],even though such high stresses may occur for only a brief portion of the switching period.Conventional methods,such as brute-force transient simulation,for obtaining the steady-state waveforms are usually time consuming and may suffer from numerical instabilities, especially for power electronics circuits consisting of slow and fast variations in different parts of the same waveform.Recently,wavelets have been shown to be highly suitable for describingCorrespondence to:S.C.Wong,Department of Electronic and Information Engineering,Hong Kong Polytechnic University,Hunghom,Hong Kong.†E-mail:enscwong@.hkContract/sponsor:Hong Kong Research Grants Council;contract/grant number:PolyU5237/04ECopyright2006John Wiley&Sons,Ltd.560K.C.TAM,S.C.WONG AND C.K.TSEwaveforms with fast changing edges embedded in slowly varying backgrounds[4,5].Liu et al.[6] demonstrated a systematic algorithm for approximating steady-state waveforms arising from power electronics circuits using Chebyshev-polynomial wavelets.Moreover,power electronics circuits are piecewise varying in the time domain.Thus,approx-imating a waveform with one wavelet approximation(ing one set of wavelet functions and hence one set of wavelet coefficients)is rather inefficient as it may require an unnecessarily large wavelet set.In this paper,we propose a piecewise approach to solving the problem,using as many wavelet approximations as the number of switch states.The method yields an accurate steady-state waveform descriptions with much less number of wavelet terms.The paper is organized as follows.Section2reviews the systematic(standard)algorithm for approximating steady-state waveforms using polynomial wavelets,which was proposed by Liu et al.[6].Section3describes the procedure and formulation for approximating steady-state waveforms of piecewise switched systems.In Section4,application examples are presented to evaluate and compare the effectiveness of the proposed piecewise wavelet approximation with that of the standard wavelet approximation.Finally,we give the conclusion in Section5.2.REVIEW OF WA VELET APPROXIMATIONIt has been shown that wavelet approximation is effective for approximating steady-state waveforms of power electronics circuits as it takes advantage of the inherent nature of wavelets in describing fast edges which have been embedded in slowly moving backgrounds[6].Typically,power electronics circuits can be represented by a time-varying state-space equation˙x=A(t)x+U(t)(1) where x is the m-dim state vector,A(t)is an m×m time-varying matrix,and U is the inputfunction.Specifically,we writeA(t)=⎡⎢⎢⎢⎣a11(t)a12(t)···a1m(t)............a m1(t)a m2(t)···a mm(t)⎤⎥⎥⎥⎦(2)andU(t)=⎡⎢⎢⎢⎣u1(t)...u m(t)⎤⎥⎥⎥⎦(3)In the steady state,the solution satisfiesx(t)=x(t+T)for0 t T(4) where T is the period.For an appropriate translation and scaling,the boundary condition can be mapped to the closed interval[−1,1]x(+1)=x(−1)(5) Copyright2006John Wiley&Sons,Ltd.Int.J.Circ.Theor.Appl.2006;34:559–582A WA VELET-BASED PIECEWISE APPROACH FOR STEADY-STATE ANALYSIS561 Assume that the basic time-invariant approximation equation isx i(t)=K T i W(t)for−1 t 1and i=1,2,...,m(6) where W(t)is any wavelet basis of size2n+1+1(n being the wavelet level),K T i=[k i,0,...,k i,2n+1] is a coefficient vector of dimension2n+1+1,which is to be found.‡The wavelet transformedequation of(1)isKD W=A(t)K W+U(t)(7)whereK=⎡⎢⎢⎢⎢⎢⎢⎢⎣k1,0k1,1···k1,2n+1k2,0k2,1···k2,2n+1............k m,0k m,1···k m,2n+1⎤⎥⎥⎥⎥⎥⎥⎥⎦(8)Thus,(7)can be written generally asF(t)K=−U(t)(9) where F(t)is a m×(2n+1+1)m matrix and K is a(2n+1+1)m-dim vector,given byF(t)=⎡⎢⎢⎢⎢⎢⎢⎢⎢⎢⎢⎢⎣a11(t)W T(t)−W T(t)D T···a1i(t)W T(t)···a1m W T(t)...............a i1(t)W T(t)···a ii(t)W T(t)−W T(t)D T···a im W T(t)...............a m1(t)W T(t)···a mi(t)W T(t)···a mm W T(t)−W T(t)D T⎤⎥⎥⎥⎥⎥⎥⎥⎥⎥⎥⎥⎦(10)K=[K T1···K T m]T(11)Note that since the unknown K is of dimension(2n+1+1)m,we need(2n+1+1)m equations. Now,the boundary condition(5)provides m equations,i.e.[W(+1)−W(−1)]T K i=0for i=1,...,m(12) This equation can be easily solved by applying an appropriate interpolation technique or via direct numerical convolution[11].Liu et al.[6]suggested that the remaining2n+1m equations‡The construction of wavelet basis has been discussed in detail in Reference[6]and more formally in Reference[7].For more details on polynomial wavelets,see References[8–10].Copyright2006John Wiley&Sons,Ltd.Int.J.Circ.Theor.Appl.2006;34:559–582562K.C.TAM,S.C.WONG AND C.K.TSEare obtained by interpolating at2n+1distinct points, i,in the closed interval[−1,1],and the interpolation points can be chosen arbitrarily.Then,the approximation equation can be written as˜FK=˜U(13)where˜F= ˜F1˜F2and˜U=˜U1˜U2(14)with˜F1,˜F2,˜U1and˜U2given by˜F1=⎡⎢⎢⎢⎢⎢⎢⎢⎢⎢⎢⎢⎢⎢⎢⎣[W(+1)−W(−1)]T(00···0)···(00···0)(00···0)[W(+1)−W(−1)]T···(00···0)............(00···0)2n+1+1columns(00···0)···[W(+1)−W(−1)]T(2n+1+1)m columns⎤⎥⎥⎥⎥⎥⎥⎥⎥⎥⎥⎥⎥⎥⎥⎦⎫⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎬⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎭m rows(15)˜F2=⎡⎢⎢⎢⎢⎢⎢⎢⎢⎣F( 1)F( 2)...F( 2n+1)(2n+1+1)m columns⎤⎥⎥⎥⎥⎥⎥⎥⎥⎦⎫⎪⎪⎪⎪⎪⎪⎪⎪⎬⎪⎪⎪⎪⎪⎪⎪⎪⎭2n+1m rows(16)˜U1=⎡⎢⎢⎢⎣...⎤⎥⎥⎥⎦⎫⎪⎪⎪⎬⎪⎪⎪⎭m elements(17)˜U2=⎡⎢⎢⎢⎢⎢⎣−U( 1)−U( 2)...−U( 2n+1)⎤⎥⎥⎥⎥⎥⎦⎫⎪⎪⎪⎪⎪⎬⎪⎪⎪⎪⎪⎭2n+1m elements(18)Finally,by solving(13),we obtain all the coefficients necessary for generating an approximate solution for the steady-state system.Copyright2006John Wiley&Sons,Ltd.Int.J.Circ.Theor.Appl.2006;34:559–582A WA VELET-BASED PIECEWISE APPROACH FOR STEADY-STATE ANALYSIS5633.WA VELET-BASED PIECEWISE APPROXIMATION METHODAlthough the above standard algorithm,given in Reference[6],provides a well approximated steady-state solution,it does not exploit the piecewise switched nature of power electronics circuits to ease computation and to improve accuracy.Power electronics circuits are defined by a set of linear differential equations governing the dynamics for different intervals of time corresponding to different switch states.In the following,we propose a wavelet approximation algorithm specifically for treating power electronics circuits.For each interval(switch state),we canfind a wavelet representation.Then,a set of wavelet representations for all switch states can be‘glued’together to give a complete steady-state waveform.Formally,consider a p-switch-state converter.We can write the describing differential equation, for switch state j,as˙x j=A j x+U j for j=1,2,...,p(19) where A j is a time invariant matrix at state j.Equation(19)is the piecewise state equation of the system.In the steady state,the solution satisfies the following boundary conditions:x j−1(T j−1)=x j(0)for j=2,3,...,p(20) andx1(0)=x p(T p)(21)where T j is the time duration of state j and pj=1T j=T.Thus,mapping all switch states to the close interval[−1,1]in the wavelet space,the basic approximate equation becomesx j,i(t)=K T j,i W(t)for−1 t 1(22) with j=1,2,...,p and i=1,2,...,m,where K T j,i=[k1,i,0···k1,i,2n+1,k2,i,0···k2,i,2n+1,k j,i,0···k j,i,2n+1]is a coefficient vector of dimension(2n+1+1)×p,which is to be found.Asmentioned previously,the state equation is transformed to the wavelet space and then solved by using interpolation.The approximation equation is˜F(t)K=−˜U(t)(23) where˜F=⎡⎢⎢⎢⎢⎢⎢⎢⎢⎢⎢⎣˜F˜F1˜F2...˜Fp⎤⎥⎥⎥⎥⎥⎥⎥⎥⎥⎥⎦and˜U=⎡⎢⎢⎢⎢⎢⎢⎢⎢⎢⎢⎣˜U˜U1˜U2...˜Up⎤⎥⎥⎥⎥⎥⎥⎥⎥⎥⎥⎦(24)Copyright2006John Wiley&Sons,Ltd.Int.J.Circ.Theor.Appl.2006;34:559–582564K.C.TAM,S.C.WONG AND C.K.TSEwith ˜F0,˜F 1,˜F 2,˜F p ,˜U 0,˜U 1,˜U 2and ˜U p given by ˜F 0=⎡⎢⎢⎢⎢⎢⎢⎢⎢⎢⎢⎢⎢⎢⎢⎣F a 00···F b F b F a 0···00F b F a ···0...............00···F b F a (2n +1+1)×m ×p columns⎤⎥⎥⎥⎥⎥⎥⎥⎥⎥⎥⎥⎥⎥⎥⎦⎫⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎬⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎭m ×p rows (F a and F b are given in (33)and (34))(25)˜F 1=⎡⎢⎢⎢⎢⎢⎢⎢⎢⎢⎢⎢⎢⎢⎢⎣F ( 1)0 0F ( 2)0 0............F ( 2n +1) (2n +1+1)m columns 0(2n +1+1)m columns···0 (2n +1+1)m columns(2n +1+1)×m ×p columns⎤⎥⎥⎥⎥⎥⎥⎥⎥⎥⎥⎥⎥⎥⎥⎦⎫⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎬⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎭2n +1m rows(26)˜F 2=⎡⎢⎢⎢⎢⎢⎢⎢⎢⎣0F ( 1)···00F ( 2)···0............0(2n +1+1)m columnsF ( 2n +1)(2n +1+1)m columns···(2n +1+1)m columns⎤⎥⎥⎥⎥⎥⎥⎥⎥⎦(27)˜F p =⎡⎢⎢⎢⎢⎢⎢⎢⎢⎣0···0F ( 1)0···0F ( 2)...... 0(2n +1+1)m columns···(2n +1+1)m columnsF ( 2n +1)(2n +1+1)m columns⎤⎥⎥⎥⎥⎥⎥⎥⎥⎦(28)˜U0=⎡⎢⎢⎢⎣0 0⎤⎥⎥⎥⎦⎫⎪⎪⎪⎬⎪⎪⎪⎭m ×p elements(29)Copyright 2006John Wiley &Sons,Ltd.Int.J.Circ.Theor.Appl.2006;34:559–582A WA VELET-BASED PIECEWISE APPROACH FOR STEADY-STATE ANALYSIS565˜U1=⎡⎢⎢⎢⎢⎢⎣−U( 1)−U( 2)...−U( 2n+1)⎤⎥⎥⎥⎥⎥⎦⎫⎪⎪⎪⎪⎪⎬⎪⎪⎪⎪⎪⎭2n+1m elements(30)˜U2=⎡⎢⎢⎢⎢⎣−U( 1)−U( 2)...−U( 2n+1)⎤⎥⎥⎥⎥⎦(31)˜Up=⎡⎢⎢⎢⎢⎢⎣−U( 1)−U( 2)...−U( 2n+1)⎤⎥⎥⎥⎥⎥⎦(32)F a=⎡⎢⎢⎢⎢⎢⎢⎢⎢⎢⎢⎢⎢⎣[W(−1)]T0 00[W(−1)]T 0............00···[W(−1)]T(2n+1+1)m columns⎤⎥⎥⎥⎥⎥⎥⎥⎥⎥⎥⎥⎥⎦⎫⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎬⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎭m rows(33)F b=⎡⎢⎢⎢⎢⎢⎢⎢⎢⎢⎢⎢⎢⎣[−W(+1)]T0 00[−W(+1)]T 0............00···[−W(+1)]T(2n+1+1)m columns⎤⎥⎥⎥⎥⎥⎥⎥⎥⎥⎥⎥⎥⎦⎫⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎬⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎭m rows(34)Similar to the standard approach outlined in Section2,all the coefficients necessary for gener-ating approximate solutions for each switch state for the steady-state system can be obtained by solving(23).It should be noted that the wavelet-based piecewise method can be further enhanced for approx-imating steady-state solution using different wavelet levels for different switch states.Essentially, Copyright2006John Wiley&Sons,Ltd.Int.J.Circ.Theor.Appl.2006;34:559–582566K.C.TAM,S.C.WONG AND C.K.TSEwavelets of high levels should only be needed to represent waveforms in switch states where high-frequency details are present.By using different choices of wavelet levels for different switch states,solutions can be obtained more quickly.Such an application of varying wavelet levels for different switch intervals can be easily incorporated in the afore-described algorithm.4.APPLICATION EXAMPLESIn this section,we present four examples to demonstrate the effectiveness of our proposed wavelet-based piecewise method for steady-state analysis of switching circuits.The results will be evaluated using the mean relative error (MRE)and mean absolute error (MAE),which are defined byMRE =12 1−1ˆx (t )−x (t )x (t )d t (35)MAE =12 1−1|ˆx (t )−x (t )|d t (36)where ˆx (t )is the wavelet-approximated value and x (t )is the SPICE simulated result.The SPICE result,being generated from exact time-domain simulation of the actual circuit at device level,can be used for comparison and evaluation.In discrete forms,MAE and MRE are simply given byMRE =1N Ni =1ˆx i −x i x i(37)MAE =1N Ni =1|ˆx i −x i |(38)where N is the total number of points sampled along the interval [−1,1]for error calculation.In the following,we use uniform sampling (i.e.equal spacing)with N =1001,including boundary points.4.1.Example 1:a single pulse waveformConsider the single pulse waveform shown in Figure 1.This is an example of a waveform that cannot be efficiently approximated by the standard wavelet algorithm.The waveform consists of five segments corresponding to five switch states (S1–S5),and the corresponding state equations are given by (19),where A j and U j are given specifically asA j =⎧⎪⎪⎪⎪⎪⎪⎪⎪⎪⎨⎪⎪⎪⎪⎪⎪⎪⎪⎪⎩0if 0 t <t 10if t 1 t <t 21if t 2 t <t 30if t 3 t <t 40if t 4 t T(39)Copyright 2006John Wiley &Sons,Ltd.Int.J.Circ.Theor.Appl.2006;34:559–582A WA VELET-BASED PIECEWISE APPROACH FOR STEADY-STATE ANALYSIS567S1S2S3S4S50t1t2t3t4THFigure 1.A single pulse waveform consisting of 5switch states.andU j =⎧⎪⎪⎪⎪⎪⎪⎪⎨⎪⎪⎪⎪⎪⎪⎪⎩0if 0 t <t 1H /(t 2−t 1)if t 1 t <t 2−Hif t 2 t <t 3−H /(t 4−t 3)if t 3 t <t 40if t 4 t T(40)where H is the amplitude (see Figure 1).Switch states 2(S2)and 4(S4)correspond to the rising edge and falling edge,respectively.Obviously,when the widths of rising and falling edges are small (relative to the whole switching period),the standard wavelet method cannot provide a satisfactory approximation for this waveform unless very high wavelet levels are used.Theoretically,the entire pulse-like waveform can be very accurately approximated by a very large number of wavelet terms,but the computational efforts required are excessive.As mentioned before,since the piecewise approach describes each switch interval separately,it yields an accurate steady-state waveform description for each switch interval with much less number of wavelet terms.Figures 2(a)and (b)compare the approximated pulse waveforms using the proposed wavelet-based piecewise method and the standard wavelet method for two different choices of wavelet levels with different widths of rising and falling edges.This example clearly shows the benefits of the wavelet-based piecewise approximation using separate sets of wavelet coefficients for the different switch states.Copyright 2006John Wiley &Sons,Ltd.Int.J.Circ.Theor.Appl.2006;34:559–582568K.C.TAM,S.C.WONG AND C.K.TSE0−0.2−0.4−0.6−0.8−1−20−15−10−50.20.40.60.81−0.2−0.4−0.6−0.8−10.20.40.60.81(a)051015(b)Figure 2.Approximated pulse waveforms with amplitude 10.Dotted line is the standard wavelet approx-imated waveforms using wavelets of levels from −1to 5.Solid lines are the actual waveforms and the wavelet-based piecewise approximated waveforms using wavelets of levels from −1to 1:(a)switch states 2and 4with rising and falling times both equal to 5per cent of the period;and (b)switch states 2and 4with rising and falling times both equal to 1per cent of the period.4.2.Example 2:simple buck converterThe second example is the simple buck converter shown in Figure 3.Suppose the switch has a resistance of R s when it is turned on,and is practically open-circuit when it is turned off.The diode has a forward voltage drop of V f and an on-resistance of R d .The on-time and off-time equivalent circuits are shown in Figure 4.The basic system equation can be readily found as˙x=A (t )x +U (t )(41)where x =[i L v o ]T ,and A (t )and U (t )are given byA (t )=⎡⎢⎣−R d s (t )L −1L 1C −1RC⎤⎥⎦(42)U (t )=⎡⎣E (1−s (t ))+V f s (t )L⎤⎦(43)Copyright 2006John Wiley &Sons,Ltd.Int.J.Circ.Theor.Appl.2006;34:559–582Figure3.Simple buck convertercircuit.Figure4.Equivalent linear circuits of the buck converter:(a)during on time;and(b)during off time.Table ponent and parameter values for simulationof the simple buck converter.Component/parameter ValueMain inductance,L0.5mHCapacitance,C0.1mFLoad resistance,R10Input voltage,E100VDiode forward drop,V f0.8VSwitching period,T100 sOn-time,T D40 sSwitch on-resistance,R s0.001Diode on-resistance,R d0.001with s(t)defined bys(t)=⎧⎪⎨⎪⎩0for0 t T D1for T D t Ts(t−T)for all t>T(44)We have performed waveform approximations using the standard wavelet method and the proposed wavelet-based piecewise method.The circuit parameters are shown in Table I.We also generate waveforms from SPICE simulations which are used as references for comparison. The approximated inductor current is shown in Figure5.Simple visual inspection reveals that the wavelet-based piecewise approach always gives more accurate waveforms than the standard method.Copyright2006John Wiley&Sons,Ltd.Int.J.Circ.Theor.Appl.2006;34:559–582−0.5−10.51−0.5−10.51012345670123456712345671234567(a)(b)(c)(d)Figure 5.Inductor current waveforms of the buck converter.Solid line is waveform from piecewise wavelet approximation,dotted line is waveform from SPICE simulation and dot-dashed line is waveform using standard wavelet approximation.Note that the solid lines are nearly overlapping with the dotted lines:(a)using wavelets of levels from −1to 0;(b)using wavelets of levels from −1to 1;(c)using wavelets oflevels from −1to 4;and (d)using wavelets of levels from −1to 5.Table parison of MREs for approximating waveforms for the simple buck converter.Wavelet Number of MRE for i L MRE for v C CPU time (s)MRE for i L MRE for v C CPU time (s)levels wavelets (standard)(standard)(standard)(piecewise)(piecewise)(piecewise)−1to 030.9773300.9802850.0150.0041640.0033580.016−1to 150.2501360.1651870.0160.0030220.0024000.016−1to 290.0266670.0208900.0320.0030220.0024000.046−1to 3170.1281940.1180920.1090.0030220.0024000.110−1to 4330.0593070.0538670.3750.0030220.0024000.407−1to 5650.0280970.025478 1.4380.0030220.002400 1.735−1to 61290.0122120.011025 6.1880.0030220.0024009.344−1to 72570.0043420.00373328.6410.0030220.00240050.453In order to compare the results quantitatively,MREs are computed,as reported in Table II and plotted in Figure 6.Finally we note that the inductor current waveform has been very well approximated by using only 5wavelets of levels up to 1in the piecewise method with extremelyCopyright 2006John Wiley &Sons,Ltd.Int.J.Circ.Theor.Appl.2006;34:559–582123456700.10.20.30.40.50.60.70.80.91M R E (m e a n r e l a t i v e e r r o r )Wavelet Levelsinductor current : standard method inductor current : piecewise methodFigure parison of MREs for approximating inductor current for the simple buck converter.small MREs.Furthermore,as shown in Table II,the CPU time required by the standard method to achieve an MRE of about 0.0043for i L is 28.64s,while it is less than 0.016s with the proposed piecewise approach.Thus,we see that the piecewise method is significantly faster than the standard method.4.3.Example 3:boost converter with parasitic ringingsNext,we consider the boost converter shown in Figure 7.The equivalent on-time and off-time circuits are shown in Figure 8.Note that the parasitic capacitance across the switch and the leakage inductance are deliberately included to reveal waveform ringings which are realistic phenomena requiring rather long simulation time if a brute-force time-domain simulation method is used.The state equation of this converter is given by˙x=A (t )x +U (t )(45)where x =[i m i l v s v o ]T ,and A (t )and U (t )are given byA (t )=A 1(1−s (t ))+A 2s (t )(46)U (t )=U 1(1−s (t ))+U 2s (t )(47)Copyright 2006John Wiley &Sons,Ltd.Int.J.Circ.Theor.Appl.2006;34:559–582Figure7.Simple boost convertercircuit.Figure8.Equivalent linear circuits of the boost converter including parasitic components:(a)for on time;and(b)for off time.with s(t)defined earlier in(44)andA1=⎡⎢⎢⎢⎢⎢⎢⎢⎢⎢⎢⎢⎣−R mL mR mL m00R mL l−R l+R mL l−1L l1C s−1R s C s000−1RC⎤⎥⎥⎥⎥⎥⎥⎥⎥⎥⎥⎥⎦(48)A2=⎡⎢⎢⎢⎢⎢⎢⎢⎢⎢⎢⎢⎢⎣−R mR dL mR m R dL m0−R mL m d mR m R dL l−R mR d+R lL l−1L lR mL l d m1C s00R mC(R d+R m)−R mC(R d+R m)0−R+R m+R dC R(R d+R m)⎤⎥⎥⎥⎥⎥⎥⎥⎥⎥⎥⎥⎥⎦(49)Copyright2006John Wiley&Sons,Ltd.Int.J.Circ.Theor.Appl.2006;34:559–582U1=⎡⎢⎢⎢⎢⎢⎢⎢⎢⎢⎣EL m⎤⎥⎥⎥⎥⎥⎥⎥⎥⎥⎦(50)U2=⎡⎢⎢⎢⎢⎢⎢⎢⎢⎢⎢⎢⎢⎢⎣EL m−R m V fL m d mR m V fL l(R d+R m)−V f R mC(R d m⎤⎥⎥⎥⎥⎥⎥⎥⎥⎥⎥⎥⎥⎥⎦(51)Again we compare the approximated waveforms of the leakage inductor current using the proposed piecewise method and the standard wavelet method.The circuit parameters are listed in Table III.Figures9(a)and(b)show the approximated waveforms using the piecewise and standard wavelet methods for two different choices of wavelet levels.As expected,the piecewise method gives more accurate results with wavelets of relatively low levels.Since the waveform contains a substantial portion where the value is near zero,we use the mean absolute error(MAE)forTable ponent and parameter values for simulation ofthe boost converter.Component/parameter ValueMain inductance,L m200 HLeakage inductance,L l1 HParasitic resistance,R m1MOutput capacitance,C200 FLoad resistance,R10Input voltage,E10VDiode forward drop,V f0.8VSwitching period,T100 sOn-time,T D40 sParasitic lead resistance,R l0.5Switch on-resistance,R s0.001Switch capacitance,C s200nFDiode on-resistance,R d0.001Copyright2006John Wiley&Sons,Ltd.Int.J.Circ.Theor.Appl.2006;34:559–5820−0.2−0.4−0.6−0.8−1−50.20.40.60.815100(a)(b)−50.20.40.60.81510Figure 9.Leakage inductor waveforms of the boost converter.Solid line is waveform from wavelet-based piecewise approximation,dotted line is waveform from SPICE simulation and dot-dashed line is waveform using standard wavelet approximation:(a)using wavelets oflevels from −1to 4;and (b)using wavelets of levels from −1to 5.Table IV .Comparison of MAEs for approximating the leakage inductor currentfor the boost converter.Wavelet Number MAE for i l CPU time (s)MAE for i l CPU time (s)levels of wavelets(standard)(standard)(piecewise)(piecewise)−1to 3170.4501710.1250.2401820.156−1to 4330.3263290.4060.1448180.625−1to 5650.269990 1.6410.067127 3.500−1to 61290.2118157.7970.06399521.656−1to 72570.13254340.6250.063175171.563evaluation.From Table IV and Figure 10,the result clearly verifies the advantage of using the proposed wavelet-based piecewise method.Furthermore,inspecting the two switch states of the boost converter,it is obvious that switch state 2(off-time)is richer in high-frequency details,and therefore should be approximated with wavelets of higher levels.A more educated choice of wavelet levels can shorten the simulationCopyright 2006John Wiley &Sons,Ltd.Int.J.Circ.Theor.Appl.2006;34:559–582345670.050.10.150.20.250.30.350.40.450.5M A E (m e a n a b s o l u t e e r r o r )Wavelet Levelsleakage inductor current : standard method leakage inductor current : piecewise methodFigure parison of MAEs for approximating the leakage inductor current for the boost converter.time.Figure 11shows the approximated waveforms with different (more appropriate)choices of wavelet levels for switch states 1(on-time)and 2(off-time).Here,we note that smaller MAEs can generally be achieved with a less total number of wavelets,compared to the case where the same wavelet levels are employed for both switch states.Also,from Table IV,we see that the CPU time required for the standard method to achieve an MAE of about 0.13for i l is 40.625s,while it takes only slightly more than 0.6s with the piecewise method.Thus,the gain in computational speed is significant with the piecewise approach.4.4.Example 4:flyback converter with parasitic ringingsThe final example is a flyback converter,which is shown in Figure 12.The equivalent on-time and off-time circuits are shown in Figure 13.The parasitic capacitance across the switch and the transformer leakage inductance are included to reveal realistic waveform ringings.The state equation of this converter is given by˙x=A (t )x +U (t )(52)where x =[i m i l v s v o ]T ,and A (t )and U (t )are given byA (t )=A 1(1−s (t ))+A 2s (t )(53)U (t )=U 1(1−s (t ))+U 2s (t )(54)Copyright 2006John Wiley &Sons,Ltd.Int.J.Circ.Theor.Appl.2006;34:559–5820−0.2−0.4−0.6−0.8−1−6−4−20.20.40.60.81024680−0.2−0.4−0.6−0.8−1−6−4−20.20.40.60.81024680−0.2−0.4−0.6−0.8−1−6−4−20.20.40.60.81024680−0.2−0.4−0.6−0.8−1−6−4−20.20.40.60.8102468il(A)il(A)il(A)il(A)(a)(b)(c)(d)Figure 11.Leakage inductor waveforms of the boost converter with different choice of wavelet levels for the two switch states.Dotted line is waveform from SPICE simulation.Solid line is waveform using wavelet-based piecewise approximation.Two different wavelet levels,shown in brackets,are used for approximating switch states 1and 2,respectively:(a)(3,4)with MAE =0.154674;(b)(3,5)withMAE =0.082159;(c)(4,5)with MAE =0.071915;and (d)(5,6)with MAE =0.066218.Copyright 2006John Wiley &Sons,Ltd.Int.J.Circ.Theor.Appl.2006;34:559–582。
Huba Control Type 699 压力传感器说明书

1/5Huba Control Type 699 | Technical data subject to change | Edition 09/2020Pressure and fl owThe type 699 transmitters are available in switchable pressure ranges and with or without display. The full-version includes customer specific adjustment possibilities. Especially develo-ped sensors for each pressure range ensure accurate long term stable measurement and the large variety of options provide the perfect platform for use in air conditioning technology as well as for fine measurement in the industrial and medical environment.Relative and di ff erentialpressure transmitter -Type 699Differential pressure transmitter+ Available with or without LCD display + Adjustable measurement range + Switchable output signals+ Switchable response curve (linear or root-extracted)+ Resettable Zero Point (Reset button) + Full scale adjustable+ Attractive price / performance ratio+ Application at over and low pressure range possible + Fast, easy mounting. Housing incorporates integral bracket for wall or ceiling mountingPressure range -1 ... 1 mbar / 0 ... 0.3 – 50 mbar2/5Huba Control Type 699 | Technical data subject to change | Edition 09/2020Pressure and fl owPressure rangeRelative and differential-1 ... 1 mbar / 0 ... 0.3 – 50 mbar Operating conditions MediumAir and neutral gases TemperatureMedium / ambient 0 ... +70 ºCStorage-10 ... +70 ºCNo cond e nsationTolerable overload on one sideApplication at over pressure range< 3 mbar P1 = 50 mbar P2 = 4 mbar> 3 mbar P1 = 100 mbar P2 = 4 mbar Application at under pressure range< 3 mbar P1 = -4 mbar P2 = -50 mbar> 3 mbarP1 = -4 mbar P2 = -100 mbarRupture pressureambient temperature 2 x overload70 ºC1.5 x overloadMaterials in contact with mediumS e nsorC e ramic Al 2O 3 (96%)Diaphragm Silicon eHousingPolycarbonat PCElectrical overviewOutput 1) Pow e r supply 1) Load Curr e nt consumption 2)2 wire 4 ... 20 mA 8.0 ... 33 VDC < supply voltage - 8 V[Ohm] < 20 mA 3 wire 0 ... 10 V 13.5 ... 33 VDC / 24 VAC ±15% > 10 kOhm < 10 mA 0 ... 20 mA 13.5 ... 33 VDC / 24 VAC ±15% < 500 Ohm < 30 mA 4 ... 20 mA 13.5 ... 33 VDC / 24 VAC ±15% < 500 Ohm < 30 mA 0 ... 5 V 3) 6.5 ... 33 VDC / 24 VAC ±15% > 10 kOhm < 10 mA Filter Response time switchable by off / 0.2s / 1s / 5s / 20s Polarity reversal protection Short circuit proof and protected against polarity reversal. Each connection is protected against crossover up to max. supply voltage.Dynamic response Response time < 20 ms Load cycle< 10 HzProtection standard Without coverIP 00With coverIP 54IP 65DisplayLCD DisplayDouble spaced per 8 digit alphanumericAt additional backlight LCD-Display 30 mA current consumption Module MODBUSRTU RS-485Ranges of adjustmentThe zero piont is adjustable by reset button.The Full scale is adjustable by DIP-Switch and additional by the turbopoti.AdjustabilityOptional version with self configurable parameters (see order code selection table)Electrical connectionScrew terminals for wire and stranded conductors up to 1.5 mm 2Cable gland with built-in strain relief PG11Pressure connection Connection pipeØ 6.2 mmMounting instructions Installation arrangement Recommendation: Vertical, (Factory calibration) with pressure connections downwards MountingMounting bracket (integrated in case)Tests / Admissions ULElectromagnetic compatibilityCE-conformity acc. EN 61326-2-3 WeightWithout display ~ 90 g With display~ 100 gPackagingSingle packaging in cardboard Multiple packaging20 / 40 / 1200.02 A3/5Huba Control Type 699 | Technical data subject to change | Edition 09/2020Pressure and fl owOut100%a)Pressurec)Out b)Test conditions:25 ºC, 45% rF, Power supply 24 VDC TC z.p. / TC z.p. 0 … 70 ºC- no additional root-extracted errors - For changing diaphragm position, compensable with zero point reset ParameterUnit±0.5 mbar0 ... 1 mbar0 ... 3 mbar0 ... 5 mbar0 ... 10 - 50 mbarTolerance zero point max.% fs ±1.0±1.0±0.7±0.7±0.7Tolerance zero full scalemax.% fs ±1.0±1.0±0.7±0.7±0.7Resolution % fs0.2 0.2 0.1 0.1 0.1Total of linearity, hysteresis and repeatability max.% fs ±1.0±1.0±1.0±1.0±0.6Long therm stability acc. to DIN EN 60770% fs ±1.0±1.0±1.0±1.0±1.0TC zero point 1) typ.% fs/10K ±0.2±0.2±0.2±0.1±0.1TC zero point 1) max.% fs/10K ±1.0±1.0±0.5±0.4±0.4TC sensitivity 1) typ.% fs/10K ±0.3±0.3±0.2±0.1±0.1TC sensitivity 1)max.% fs/10K±0.6±0.6±0.5±0.5±0.24/5Huba Control Type 699 | Technical data subject to change | Edition 09/2020Pressure and flow9297EN 60 715-TH 35-7.54512835.2611.6Fig. 3Fig. 1Fig. 2Universal2 and3 wire3 wire2 wire Connection kit for vent duct (metal), 90º angled including tube 2 m long (Fig. 1) 104312Connection kit for vent duct (plastic), straight including tube 2 m long (Fig. 2) 100064DIN-rail mounting adaptor (Fig. 3) 112854Module MODBUS 117305Calibration certificate104551V rsion Variabl e param e t e rs Dual DIP-Switch Pressure ranges in grades Tenfold DIP-Switch Pressure ranges in grades; stepless adjustable with Turbo-Poti / output signals / fi lter (o ff / on) / response curve (linear / root extracted)Te nfold DIP-Switch with Display Pre ssure range s in grade s; ste ple ss adjustable with Turbo-Poti / pre ssure units / pre ssure range characte r / output signals; additional 0 ... 5 V / fi lter (o ff/ 0.2s / 1s / 5s / 20s) / response curve (linear / root extracted) / backlight (o ff / 5min / on)Huba Control AG Headquarters Schweiz Industriestrasse 17CH-5436 Würenlos Telefon +41 56 436 82 00Fax+41 56 436 82 82***********************Huba Control AGBranch O ff ice United KingdomUnit 13 Berkshire House, County Park Business Centre, Shrivenham Road Swindon Wiltshire SN1 2NR Phone +44 1993 77 66 67Fax+44 1993 77 66 71***********************Huba Control SA Succursale France Rue LavoisierTechnopôle Forbach-Sud F-57602 Forbach Cedex Téléphone +33 3 87 84 73 00Télécopieur +33 3 87 84 73 01***********************Huba Control AG Niederlassung Deutschland Schlattgrabenstrasse 24D-72141 Walddorfhäslach Telefon +49 7127 2393 00Fax+49 7127 2393 20***********************Huba Control USA, Inc.O ff ice United States of America 303 Wyman Street Suite #300Waltham MA 02451Tel: +1 866-6HUBACO (+1 866-648-2226)************************Huba Control AG Vestiging Nederland Hamseweg 20A NL-3828 AD-Hoogland Telefoon +31 33 433 03 66Telefax+31 33 433 03 77***********************。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
Switchable optical UWB monocycle anddoublet generation using a reconfigurable photonic microwave delay-line filterQing Wang and Jianping YaoMicrowave Photonics Research Laboratory, School of Information Technology and Engineering,University of Ottawa, Ottawa, ON, K1N 6N5, Canadajpyao@site.uOttawa.caAbstract: Optically switchable Ultra-Wideband (UWB) monocycle anddoublet pulse generation using an optically reconfigurable photonicmicrowave delay-line filter is proposed and demonstrated. The microwavefilter can be reconfigured as a two- or three-tap microwave filter withcoefficients of (1, -1) or (1, -2, 1). The function of the two- or three-tapmicrowave filter is equivalent to an operation of a first- or second-orderdifference, which can be approximated as a first- or second-order derivative.When a Gaussian pulse is inputted to the two- or three-tap microwavedelay-line filter, a Gaussian monocycle or doublet pulse is generated. Theproposed photonic microwave delay-line filter is implemented using apolarization modulator (PolM), a length of polarization maintaining fiber(PMF), and a balanced photo-detector (BPD). In the experiment, Gaussianmonocycle and doublet pulses with a fractional bandwidth of about 170%and 130% are generated. The switchability of the proposed UWB pulsegenerator in pulse shape and polarity is also experimentally demonstrated.©2007 Optical Society of AmericaOCIS codes: (060.0060) Fiber optics and optical communications; (070.1170) Analog opticalsignal processing; (060.2330) Fiber optics communications; (060.2310) Fiber optics.References and links1. D. Porcine, P. Research, and W. Hirt, “Ultra-wideband radio technology: Potential and challenges ahead,” IEEECommun. Mag. 41, 66-74 (2003).2.R. J. Fontana, “Recent System Applications of Short-Pulse Ultra-Wideband (UWB) Technology”, IEEE Trans.Microw. Theory Tech. 52, 2087-2104 (2004).3.M. Ghavami, L. B. Michael, and R. Kohno, Ultra wide-band signals and systems in communication engineering(Wiley, 2004)4.L. Zhu, S. Sun, and W. Menzel, “Ultra-wideband (UWB) bandpass filters using multiple-mode resonator,” IEEEMicrow. Wireless Compon. Lett. 15, 796-798 (2005).5.T. Kawanishi, T. Sakamoto, and M. Izutsu, “Ultra-wide-band signal generation using high-speed opticalfrequency-shift-keying technique,” IEEE International Topical Meeting on Microwave Photonics - Technical Digest, MWP’04 48-51 (2004).6.Q. Wang and J. P. Yao, “UWB doublet generation using a nonlinearly-biased electro-optic intensity modulator,”IEE Electron. Lett. 42, 1304-1305 (2006)7.Q. Wang, F. Zeng, S. Blais, and J. P. Yao, “Optical UWB monocycle pulse generation based on cross-gainmodulation in a semiconductor optical amplifier,” Opt. Lett. 31, 3083-3085 (2006).8. F. Zeng and J. P. Yao, “An approach to ultrawideband pulse generation and distribution over optical fiber,”IEEE Photon. Technol. Lett. 18, 823–825 (2006).9. C. Wang, F. Zeng, and J. P. Yao, “All-Fiber Ultrawideband Pulse Generation Based on Spectral Shaping andDispersion-Induced Frequency-to-Time Conversion,” IEEE Photon. Tech. Lett. 19, 137-139 (2007).10.I. S. Lin, J. D. McKinney, and A. M. Weiner, “Photonic synthesis of broadband microwave arbitrary waveformsapplicable to ultra-wideband communication,” IEEE Microw. Wireless Compon. Lett. 15, 226-228 (2005). 11. F. Zeng and J. P. Yao, “Ultrawideband impulse radio signal generation using a high-speed electrooptic phasemodulator and a fiber-Bragg-grating-based frequency discriminator,” IEEE Photon. Technol. Lett. 18, 2062- 2064 (2006).#86327 - $15.00 USD Received 10 Aug 2007; revised 13 Oct 2007; accepted 19 Oct 2007; published 23 Oct 2007 (C) 2007 OSA29 October 2007 / Vol. 15, No. 22 / OPTICS EXPRESS 1466712.J. P. Yao, F. Zeng, and Q. Wang, “Photonic generation of Ultra-Wideband signals,” J. Lightw. Technol. 25,(2007).13.J. D. Bull, N. A. F. Jaeger, H. Kato, M. Fairburn, A. Reid, and P. Ghanipour, “40 GHz electro-optic polarizationmodulator for fiber optic communications systems” Proc. SPIE 5577, 133-143 (2004).1. IntroductionUltra-Wideband (UWB) is considered as a promising technology for short-range high data-rate indoor wireless communications and high data-rate sensor networks, thanks to the many advantages, such as low power consumption, low spectral density, high immunity to multipath fading, enhanced capability to penetrate through obstacles, and coexistence with other conventional radio systems [1]-[3]. In 2002, the US Federal Communications Commission (FCC) approved the unlicensed use of the UWB spectrum from 3.1 to 10.6 GHz, with a power spectral density (PSD) lower than -41.3 dBm/MHz [1]. Based on the definition of FCC, a UWB signal should have a spectral bandwidth greater than 500 MHz or a fractional bandwidth greater than 20% [1].For UWB communications, one of the key challenges is the generation of UWB pulses that satisfy the FCC-specified spectrum mask. Several UWB pulse generation techniques have been proposed recently [4]-[11]. Among these techniques, the implementation of the first- or the second-order derivatives of a Gaussian pulse, to generate a Gaussian monocycle or a Gaussian doublet, is considered as a simple and efficient technique for UWB pulse generation [3]. UWB pulses can be generated in the electrical domain using electronic circuitry [4]. Recently, the generation of UWB pulses in the optical domain has been a topic of interest. The generation of UWB pulses in the optical domain provides a higher flexibility, which enables the generation of UWB pulses with switchable pulse shapes and polarities. In addition, the huge bandwidth offered by photonics enables the generation of UWB pulses to fully occupy the spectrum range specified by the FCC. Different approaches have been recently proposed and demonstrated [5]-[10]. The major limitation of the approaches in [5]-[9] is that each scheme can only generate one type of UWB pulse (Gaussian monocycle or doublet). For some applications, such as pulse shape modulation (PSM), it is desirable that both Gaussian monocycle and doublet can be generated in a single system. In [10], different waveforms can be obtained, but the switching speed between the waveforms is limited by the speed of the liquid crystal modulator. Very recently, a design was proposed to generate UWB monocycle and doublet pulses in one system [11], in which a fiber Bragg grating (FBG) was used to serve as a frequency discriminator, to perform phase modulation to intensity modulation (PM-IM) conversion. By locating the optical carrier at the linear or the quadrature region of the FBG reflection spectrum, UWB monocycle or doublet pulses were generated [11]. The main drawback of this scheme is the requirement for a high-speed tunable laser source (TLS) to realize the waveform switchability. In addition, the high sensitivity of the FBG to environmental changes would affect the stability of the system.It is known that a Gaussian monocycle or doublet pulse can be generated by implementing the first- or the second-order derivative of a Gaussian pulse. The first- or the second-order derivative can be approximated by the first- or the second-order difference. It is known that the first-order difference can be realized by using a two-tap microwave delay-line filter with coefficients of (1, -1) and a second-order difference can be realized using a three-tap microwave delay-line filter with coefficients of (1, -2, 1) [12]. In this paper, we propose an optically switchable UWB monocycle and doublet generation system based on a photonic microwave delay-line filter that can be reconfigured optically as a two- or three-tap microwave filter. A brief theoretical explanation of using a two- or three-tap microwave delay-line filter for UWB pulse generation is provided, and then the realization of the reconfigurable microwave delay-line filter with coefficients of (1, -1) or (1, -2, 1) is proposed. The microwave delay-line filter consists of a polarization modulator (PolM), a length of polarization maintaining fiber (PMF), and a balanced photo-detector (BPD). The use of the #86327 - $15.00 USD Received 10 Aug 2007; revised 13 Oct 2007; accepted 19 Oct 2007; published 23 Oct 2007 (C) 2007 OSA29 October 2007 / Vol. 15, No. 22 / OPTICS EXPRESS 14668reconfigurable microwave delay-line filter for UWB monocycle or doublet generation is demonstrated. Gaussian monocycles or doublets with fractional bandwidths of about 170% or 130% are experimentally obtained. The switchability of the proposed UWB generator in pulse shape and polarity is investigated and experimentally demonstrated.2. PrincipleMicrowaveFig. 1. The schematic diagram of an N-tap photonic microwave delay-line filterThe schematic diagram of a general N -tap photonic microwave delay-line filter is shown in Fig. 1. It consists of an optical source, an optical modulator, a delay tine device, and a photodetector (PD). The microwave signal to be filtered is modulated onto the lightwave generated from the optical source via the optical modulator. The modulated lightwave is then sent to an N -tap delay-line device to introduce different time delays with an identical time delay difference between two adjacent taps. The time-delayed signals are then applied to the PD. The time delay difference determines the free spectral range (FSR) and the coefficients determine the shape of the filter response. Mathematically, the frequency response of an N -tap microwave delay-line filter is given10()N jk N k k H a e ωτω−−==∑ (1)where τ is the time delay difference and k a is the coefficient of the k th tap. For a two-tapfilter with coefficients of (1, -1), the frequency response is given22()2sin 2j H j e ωτωτω−= (2)For a three-tap filter with coefficients of (1, -2, 1), the frequency response is given[]2232()()4sin 2j H H e ωτωτωω−==−(3) If /2ωτ is small, Eq. 2 and Eq. 3 can be approximated as22()j H j e ωτωωτ−≈ (4)223()j H e ωτωωτ−≈− (5) As can be seen, Eq. 4 and Eq. 5 have the same frequency responses as a first- and second-order differentiator except that a scaling factor and a linear phase shift are added, which will not affect the shape of the output microwave signal. The condition of small /2ωτ can be easily satisfied in the system by controlling the time-delay difference to be small. Therefore, we can conclude that a two- or a three-tap microwave delay-line filter with coefficients of (1, -1) or (1, -2, 1) and a small time-delay difference can be used to realize the first- or second-#86327 - $15.00 USD Received 10 Aug 2007; revised 13 Oct 2007; accepted 19 Oct 2007; published 23 Oct 2007(C) 2007 OSA 29 October 2007 / Vol. 15, No. 22 / OPTICS EXPRESS 14669order derivative. If the input microwave signal to the two- or three-tap microwave delay-line filter is a Gaussian pulse, a Gaussian monocycle pulse or doublet can be generated.3. Experiment and resultsτFig. 2. UWB monocycle and doublet pulse generation using a reconfigurable microwavephotonic delay-line filter; LD: laser diode, PC: polarization controller, PolM: polarizationmodulator, PMF: polarization maintaining fiber, BPD: balanced photo-detector.The microwave delay-line filter that can be reconfigured as a two- or three-tap photonic microwave delay-line filter with coefficients of (1, -1) or (1, -2, 1) is experimentally realized. The experiment setup is shown in Fig. 2. The filter consists of a PolM, a length of PMF and a BPD. The key components in the system are the PolM and the BPD. The PolM is a high-speed electrically tunable arbitrarily retarding, multiple-order wave plate [13], which can be used to generate the negative coefficient. When a linearly polarized incident light is oriented with an angle of 45o to one principal axis of the PolM, the polarization state of the output lightwave would change from linear polarization to orthogonal linear polarization, passing through elliptical and circular polarization states as the modulation voltage is varied by a half-wave , polarization modulation is thus achieved. The BPD (Discovery Semiconductors) voltage Vπconsists of two reversely-biased photo-detectors, with a tunable time-delay line incorporated in one branch of the BPD; therefore, it can realize time-delayed differential detection.In Fig. 2, the output lightwave from a laser diode (LD) with a power of about 8 dBm is sent to the PolM at an incident polarization angle of 45o for polarization modulation. Two complementary microwave signals modulated onto two orthogonally polarized optical carriers are obtained at the output of the PolM. The total optical power at the output of the PolM keeps constant. The two orthogonally polarized optical microwave signals are then sent to the PMF with their polarization directions aligned with the fast and the slow axes of the PMF. A time-delay difference τis introduced to the two signals due to the birefringence of PMF. The output optical microwave signals from the PMF is then equally split by a 50:50 fiber coupler and sent to the BPD. Two polarization controllers, PC1 and PC2, are incorporated at the input and the output of the PolM, with PC1 being used to adjust the polarization angles of the incident lightwave to have an angle of 45o to one principal axis of the PolM, and PC2 being used to align the polarization directions of the two complementary optical microwave signals with the fast and the slow axes of the PMF.The whole system in Fig. 2 is an optically reconfigurable photonic microwave delay-line filter. It is configured as a two-tap delay-line filter if one arm of the coupler is connected to only one input port of the BPD. In this case, only one PD in the BDP is used. The time delay difference between the two taps is generated due to the birefringence of the PMF. Since the two orthogonal polarization modes in the PMF are complementary in amplitude, the two-tap#86327 - $15.00 USD Received 10 Aug 2007; revised 13 Oct 2007; accepted 19 Oct 2007; published 23 Oct 2007 (C) 2007 OSA29 October 2007 / Vol. 15, No. 22 / OPTICS EXPRESS 14670microwave filter has opposite coefficients of (1, -1). The system in Fig. 2 can also be configured as a three-tap microwave delay-line filter with coefficients of (1, -2, 1) if the second arm of the coupler is connected to the other input port of the BPD, with an additional time-delay difference τ introduced between the two branches by adjusting the internal optical delay line in the BPD, as shown in Fig. 2. Note that the proposed reconfigurable photonic microwave delay-line filter is an incoherent filter as no optical interference occurs between the two orthogonally polarized lightwaves.The PolM (Versawave Technologies) used in the experiment can operate up to 40 GHz with a working wavelength range from 1530 to 1560 nm. The PMF (Corning PM1550) has a length of 42 m and a beat length of 3.75 mm. The time-delay difference τ between the fast and slow axes of the PMF is about 57 ps. 0510152025-60-50-40-30-20-10010H (f ) (d B ) f (GHz)(a) 0510152025-60-50-40-30-20-10010(b)H (f ) (d B ) f (GHz)Fig. 3. The frequency response of microwave filter configured as a two- or three-tap photonicmicrowave delay-line filter; (a) two-tap filter with coefficients of (1, -1) ; (b) three-tap filterwith coefficients of (1, -2, 1); solid line: experiment result; dashed line: simulation result.In the first experiment, the reconfigurability of the system in Fig. 2 as a two- and three-tap microwave filter is demonstrated. By setting the optical switch at the OFF and ON states, coefficients of (1, -1) and (1, -2, 1) are realized, respectively. The frequency responses, shown in Fig. 3(a) and 3(b), are measured using a vector network analyzer (VNA, Agilent 8364A). Comparing Fig. 3(a) and 3(b), we can find that the transmission lobe of the three-tap microwave filter is sharper than that of the two-tap microwave filter, this is because that the three-tap microwave filter has two degenerate zeros while the two-tap microwave filter has only one zero. The simulated frequency responses are also shown in Fig. 3(a) and 3(b). A good agreement is observed.02004006008001000202530354045(a)A m p l i t u d e (m V )Time (ps)02004006008001000-30-20-1001020 A m p l i t u d e (m V )Time (ps)(b)Fig. 4. The waveform of the generated Gaussian monocycle and doublet pulses; (a) Gaussian monocycle; (b) Gaussian doublet.In the second experiment, the switchability of the proposed UWB generator is demonstrated. To do this, a non-return-to-zero pulse train generated by a bit-error-rate tester (BERT, Agilent N4901B) is applied to the PolM via its RF input port. The Gaussian pulse #86327 - $15.00 USD Received 10 Aug 2007; revised 13 Oct 2007; accepted 19 Oct 2007; published 23 Oct 2007(C) 2007 OSA 29 October 2007 / Vol. 15, No. 22 / OPTICS EXPRESS 14671train has a data rate of 10 Gbit/s, a fixed pattern of “1000…” (one “1” in every 64 bits), which is equivalent to a pulse train with a repetition rate of 156 Mbit/s. The full-width at half-maximum (FWHM) of a Gaussian pulse is measured to be 80 ps. By configuring the system in Fig. 2 as a two- or three-tap microwave delay-line filter with coefficients of (1, -1) or (1, -2, 1), a Gaussian monocycle or doublet pulse is generated, with the waveforms shown in Fig. 4(a) and 4(b). Note that the time delay difference τ in the experiment is 57 ps, which is small enough to satisfy the condition at lower frequencies to get Eq. 4 and Eq. 5. For higher frequencies, the approximation error for Eq. 4 and Eq. 5 is larger. However, we realize that a larger time delay difference would bring a higher suppression at higher frequencies, which would make the generated UWB pulse spectrum better confined in the FCC mask.The polarity of the UWB monocycle and doublet can also be inverted by adjusting PC 2 to switch the slow and fast axes of the PMF. The pulse widths of the generated monocycle and doublet are measured to be about 200 ps and 258 ps. Their spectra are shown in Fig. 5(a) and 5(b). It is clearly seen that the spectrum of the Gaussian doublet is located at higher frequencies compared with that of the Gaussian monocycle, which is reasonable as the three-tap microwave filter has a sharper spectral response than that of the two-tap microwave filter. The -10 dB bandwidths of the monocycle and doublet pulses are measured to be 9.1 GHz and 8.0 GHz, with fractional bandwidths of about 170% and 130%. 5101520-100-90-80-70-60-50-40P o w e r (d B m ) f (GHz)(a)5101520-100-90-80-70-60-50-40 P o w e r (d B m ) f (GHz)(b)Fig. 5. The electrical spectra of the generated Gaussian monocycle and doublet pulses; (a) Gaussian monocycle; (b) Gaussian doublet.4. Conclusion An optically switchable UWB pulse generator using a reconfigurable photonic microwave delay-line filter was proposed and demonstrated. The filter could be easily reconfigured as a two- or three-tap delay-line filter. When the filter was configured as a two-tap microwave delay-line filter with coefficients of (1, -1), Gaussian monocycle pulses were generated. When the filter was configured as a three-tap delay-line filter with coefficients of (1, -2, 1), Gaussian doublet pulses were generated. The reconfigurability of the system could be easily realized by using an optical ON-OFF switch, which enables the system to operate at a very high speed. Therefore, the proposed system can be used to achieve high-speed pulse shape modulation (PSM). The system can also be used to achieve high-speed pulse polarity modulation (PPM) if PC 2 in the system is replaced by a high-speed electrically tunable half-wave plate, which can be another PolM. Therefore, the proposed UWB generation system has the capability in implementing both PSM and PPM at high speed, which is essential for a UWBcommunication system with different data modulation schemes. The generation of UWB pulses using the proposed reconfigurable photonic microwave delay-line filter was experimentally demonstrated, Gaussian monocycle and doublet pulses with a fractional bandwidth as high as 170% and 130% were obtained.AcknowledgementThe work was supported by The Natural Sciences and Engineering Research Council of Canada (NSERC).#86327 - $15.00 USD Received 10 Aug 2007; revised 13 Oct 2007; accepted 19 Oct 2007; published 23 Oct 2007(C) 2007 OSA 29 October 2007 / Vol. 15, No. 22 / OPTICS EXPRESS 14672。
