Recent status of polarized parton distributions

合集下载

polarization

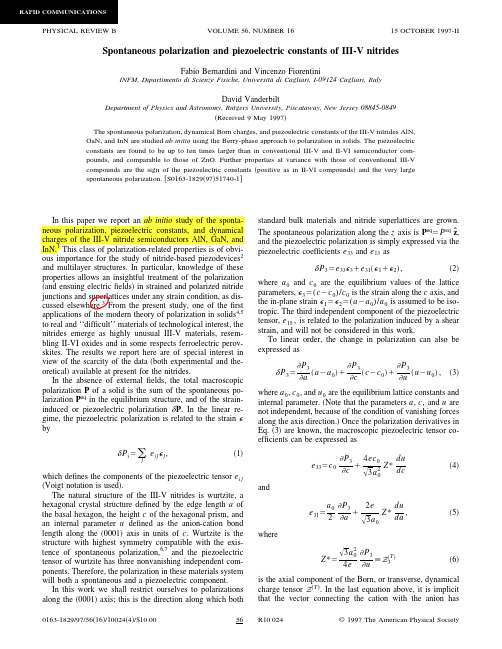
In this paper we report an ab initio study of the sponta-
neous polarization, piezoelectric constants, and dynamical
charges of the III-V nitride semiconductors AlN, GaN, and InN.1 This class of polarization-related properties is of obvious importance for the study of nitride-based piezodevices2
that the vector connecting the cation with the anion has
0163-1829/97/56͑16͒/10024͑4͒/$10.00
56 R10 024
© 1997 The American Physical Society
RAPID COMMUNICATIONS
In the absence of external ﬁelds, the total macroscopic
polarization P of a solid is the sum of the spontaneous polarization Peq in the equilibrium structure, and of the straininduced or piezoelectric polarization ␦P. In the linear regime, the piezoelectric polarization is related to the strain ⑀ by

Surface wave higher-mode phase velocity measurements using a roller- coaster-type algorithm

Geophys.J.Int.(2003)155,289–307Surface wave higher-mode phase velocity measurements usinga roller-coaster-type algorithm´Eric Beucler,∗´El´e onore Stutzmann and Jean-Paul MontagnerLaboratoire de sismologie globale,IPGP,4place Jussieu,75252Paris Cedex05,France.E-mail:beucler@ipgp.jussieu.frAccepted2003May20.Received2003January6;in original form2002March14S U M M A R YIn order to solve a highly non-linear problem by introducing the smallest a priori information,we present a new inverse technique called the‘roller coaster’technique and apply it to measuresurface wave mode-branch phase velocities.The fundamental mode and theﬁrst six overtoneparameter vectors,deﬁned over their own signiﬁcant frequency ranges,are smoothed averagephase velocity perturbations along the great circle epicentre–station path.These measurementsexplain well both Rayleigh and Love waveforms,within a maximum period range includedbetween40and500s.The main idea of this technique is toﬁrst determine all possibleconﬁgurations of the parameter vector,imposing large-scale correlations over the model space,and secondly to explore each of them locally in order to match the short-wavelength variations.Theﬁnal solution which achieves the minimum misﬁt of all local optimizations,in the least-squares sense,is then hardly inﬂuenced by the reference model.Each mode-branch a posteriorireliability estimate turns out to be a very powerful instrument in assessing the phase velocitymeasurements.Our Rayleigh results for the Vanuatu–California path seem to agree correctlywith previous ones.Key words:inverse problem,seismic tomography,surface waves,waveform analysis.1I N T R O D U C T I O NOver the last two decades,the resolution of global tomographic models has been greatly improved,because of the increase in the amount and the quality of data,and due to more and more sophisticated data processing and inversion schemes(Woodhouse&Dziewonski1984, 1986;Montagner1986;Nataf et al.1986;Giardini et al.1987;Montagner&Tanimoto1990;Tanimoto1990;Zhang&Tanimoto1991; Su et al.1994;Li&Romanowicz1995;Romanowicz1995;Trampert&Woodhouse1995;Laske&Masters1996;Ekstr¨o m et al.1997; Grand et al.1997;van der Hilst et al.1997;Liu&Dziewonski1998;Ekstr¨o m&Dziewonski1998;Laske&Masters1998;M´e gnin& Romanowicz2000;Ritsema&van Heijst2000,among others).These models are derived from surface wave phase velocities and/or body wave traveltimes(or waveforms)and/or free-oscillation splitting measurements.Body wave studies provide high-resolution models but suffer from the inhomogeneous distribution of earthquakes and recording stations,even when considering reﬂected or diffracted phases.On the other hand,the surface wave fundamental mode is mainly sensitive to the physical properties of the upper mantle.So,the investigation of the transition zone on a global scale,which plays a key role in mantle convection,can only be achieved by using higher-mode surface waves.Aﬁrst attempt at providing a global tomographic model using these waves has been proposed by Stutzmann&Montagner(1994),but with a limited amount of data.More recently,van Heijst&Woodhouse(1999)computed degree-12phase velocity maps of the fundamental mode and the fourﬁrst overtones for both Love and Rayleigh waves.These data have been combined with body wave traveltimes measurements and free-oscillation splitting measurements,to provide a global tomographic model with a high and uniform resolution over the whole mantle (Ritsema et al.1999;van Heijst et al.1999).The most recent S H model for the whole mantle was proposed by M´e gnin&Romanowicz (2000).This degree-24model results from waveform inversion of body and surface Love waves,including fundamental and higher modes and introducing cross-branch coupling.Extracting information from higher-mode surface waves is a difﬁcult task.The simultaneous arrivals(Fig.3in Section3)and the interference between the different mode-branches make the problem very underdetermined and non-linear.To remove the non-linearity,Cara &L´e vˆe que(1987)and L´e vˆe que et al.(1991)compute the cross-correlogram between the data and monomode synthetic seismograms and ∗Now at:´Ecole Normale Sup´e rieure,24rue Lhomond,75231Paris Cedex05,France.C 2003RAS289290´E.Beucler,´E.Stutzmann and J.-P.Montagnerinvert the amplitude and the phase of theﬁltered cross-correlogram.On the other hand,Nolet et al.(1986)and Nolet(1990)use an iterative inverse algorithm toﬁt the waveform in the time domain and increase the model complexity within the iterations.These two methods provide directly a1-D model corresponding to an average epicentre–station path.They wereﬁrst used‘manually’,which limited the amount of data that could be processed.The exponential increase in the amount of good-quality broad-band data has made necessary the automation of most parts of the data processing and an automatic version of these methods has been proposed by Debayle(1999)for the waveform inversion technique of Cara&L´e vˆe que(1987)and by Lebedev(2000)and Lebedev&Nolet(2003)for the partition waveform inversion.Stutzmann&Montagner(1993)split the inversion into two steps;at each iteration,a least-squares optimization to measure phase velocities is followed by an inversion to determine the1-D S-wave velocity model,in order to gain insight into the factors that control the depth resolution.They retrieve the phase velocity for a set of several seismograms recorded at a single station and originating from earthquakes located in the same area in order to improve the resolution.Another approach has been followed by van Heijst&Woodhouse(1997)who proposed a mode-branch stripping technique based on monomode cross-correlation functions.Phase velocity and amplitude perturbations are determined for the most energetic mode-branch,the waveform of which is then subtracted from the seismogram in order to determine the second most energetic mode-branch phase velocity and amplitude perturbations,and so on.More recently,Y oshizawa&Kennett(2002)used the neighbourhood algorithm(Sambridge1999a,b)to explore the model space in detail and to obtain directly a1-D velocity model which achieves the minimum misﬁt.It is difﬁcult to compare the efﬁciency of these methods because they all follow different approaches to taking account of the non-linearity of the problem.Up to now,it has only been possible to compare tomographic results obtained using these different techniques.In this paper,we introduce a new semi-automatic inverse procedure,the‘roller coaster’technique(owing to the shape of the misﬁt curve displayed in Fig.6b in Section3.4.1),to measure fundamental and overtone phase velocities both for Rayleigh and Love waves.This method can be applied either to a single seismogram or to a set of seismograms recorded at a single station.To deal with the non-linearity of the problem,the roller coaster technique combines the detection of all possible solutions at a large scale(which means solutions of large-wavelength variations of the parameter vector over the model space),and local least-squares inversions close to each of them,in order to match small variations of the model.The purpose of this article is to present an inverse procedure that introduces as little a priori information as possible in a non-linear scheme.So,even using a straightforward phase perturbation theory,we show how this algorithm detects and converges towards the best global misﬁt model.The roller coaster technique is applied to a path average theory but can be later adapted and used with a more realistic wave propagation theory.One issue of this study is to provide a3-D global model which does not suffer from strong a priori constraints during the inversion and which then can be used in the future as a reference model.We describe hereafter the forward problem and the non-linear inverse approach developed for solving it.An essential asset of this technique is to provide quantitative a posteriori information,in order to assess the accuracy of the phase velocity measurements.Resolution tests on both synthetic and real data are presented for Love and Rayleigh waves.2F O RWA R D P R O B L E MFollowing the normal-mode summation approach,a long-period seismogram can be modelled as the sum of the fundamental mode(n=0) and theﬁrst higher modes(n≥1),hereafter referred to as FM and HM,respectively.Eigenfrequencies and eigenfunctions are computed for both spheroidal and toroidal modes in a1-D reference model,PREM(Dziewonski&Anderson1981)in our case.Stoneley modes are removed,then the radial order n for the spheroidal modes corresponds to Okal’s classiﬁcation(Okal1978).In the following,all possible sorts of coupling between toroidal and spheroidal mode-branches(Woodhouse1980;Lognonn´e&Romanowicz1990;Deuss&Woodhouse2001) and off-great-circle propagation effects(Woodhouse&Wong1986;Laske&Masters1996)are neglected.For a given recorded long-period seismogram,the corresponding synthetic seismogram is computed using the formalism deﬁned by Woodhouse&Girnius(1982).In the most general case,the displacement u,corresponding of theﬁrst surface wave train,in the time domain, can be written asu(r,t)=12π+∞−∞nj=0A j(r,ω)exp[i j(r,ω)]exp(iωt)dω,(1)where r is the source–receiver spatial position,ωis the angular frequency and where A j and j represent the amplitude and the phase of the j th mode-branch,respectively,in the frequency domain.In the following,the recorded and the corresponding synthetic seismogram spectra (computed in PREM)are denoted by(R)and(S),respectively.In the Fourier domain,following Kanamori&Given(1981),a recorded seismogram spectrum can be written asA(R)(r,ω)expi (R)(r,ω)=nj=0B j(r,ω)expij(r,ω)−ωaCj(r,ω),(2)where a is the radius of the Earth, is the epicentral distance(in radians)and C(R)j(r,ω)is the real average phase velocity along the epicentre–station path of the j th mode-branch,which we wish to measure.The term B j(r,ω)includes source amplitude and geometrical spreading, whereas j(r,ω)corresponds to the source phase.The instrumental response is included in both terms and this expression is valid for bothRayleigh and Love waves.The phase shift due to the propagation in the real medium then resides in the term exp[−iωa /C(R)j(r,ω)].C 2003RAS,GJI,155,289–307The roller coaster technique291 Figure1.Illustration of possible2πphase jumps over the whole frequency range(dashed lines)or localized around a given frequency(dotted line).Thereference phase velocity used to compute these three curves is represented as a solid line.Considering that,toﬁrst order,the effect of a phase perturbation dominates over that of the amplitude perturbation(Li&Tanimoto 1993),and writing the real slowness as a perturbation of the synthetic slowness(computed in the1-D reference model),eq.(2)becomesA(R)(r,ω)expi (R)(r,ω)=nj=0A(S)j(r,ω)expij(r,ω)−ωaC(S)j(ω)−χ,(3) whereχ=ωa1C(R)j(r,ω)−1C(S)j(r,ω).(4) Let us now denote by p j(r,ω),the dimensionless parameter vector of the j th mode-branch deﬁned byp j(r,ω)=C(R)j(r,ω)−C(S)j(ω)Cj(ω).(5)Finally,introducing the synthetic phase (S)j(r,ω),as the sum of the source phase and the phase shift due to the propagation in the reference model,the forward problem can be expressed asd=g(p),A(R)(r,ω)expi (R)(r,ω)=nj=0A(S)j(r,ω)expi(S)j(r,ω)+ωaCj(ω)p j(r,ω).(6)For practical reasons,the results presented in this paper are computed following a forward problem expression based on phase velocity perturbation expanded to third order(eq.A5).When considering an absolute perturbation range lower than10per cent,results are,however, identical to those computed following eq.(6)(see Appendix A).Formally,eq.(6)can be summarized as a linear combination of complex cosines and sines and for this reason,a2πundetermination remains for every solution.For a given parameter p j(r,ω),it is obvious that two other solutions can be found by a2πshift such asp+j(r,ω)=p j(r,ω)+2πC(S)j(ω)ωa and p−j(r,ω)=p j(r,ω)−2πC(S)j(ω)ωa.(7) As an example of this feature,all the phase velocity curves presented in Fig.1satisfy eq.(6).This means that2πphase jumps can occur over the whole frequency range but can also be localized around a given frequency.Such an underdetermination as expressed in eq.(6)and such a non-unicity,in most cases due to the2πphase jumps,are often resolved by imposing some a priori constraints in the inversion.A contrario, the roller coaster technique explores a large range of possible solutions,with the smallest a priori as possible,before choosing the model that achieves the minimum misﬁt.3D E S C R I P T I O N O F T H E R O L L E R C O A S T E R T E C H N I Q U EThe method presented in this paper is a hybrid approach,combining detection of all possible large-scale solutions(which means solutions of long-wavelength conﬁgurations of the parameter vector)and local least-squares optimizations starting from each of these solutions,in order to match the short-wavelength variations of the model space.The different stages of the roller coaster technique are presented in Fig.2and described hereafter.Theﬁrst three stages are devoted to the reduction of the problem underdetermination,while the non-linearity and the non-unicity are taken into account in the following steps.C 2003RAS,GJI,155,289–307292´E.Beucler,´E.Stutzmann and J.-P.MontagnerStage1Stage2Stage3Stage4using least-squares2phasejumps?Stage5Stage6Figure2.Schematic diagram of the roller coaster technique.See Section3for details.3.1Selection of events,mode-branches and time windowsEvents with epicentral distances larger than55◦and shorter than135◦are selected.Thus,the FM is well separated in time from the HM(Fig.3), and theﬁrst and the second surface wave trains do not overlap.Since the FM signal amplitude is much larger than the HM amplitude for about 95per cent of earthquakes,each seismogram(real and synthetic)is temporally divided into two different time windows,corresponding to the FM and to the HM parts of the signal.An illustration of this amplitude discrepancy in the time domain is displayed in Fig.3(b)and when focusing on Fig.4(a),the spectrum amplitude of the whole real signal(FM+HM)is largely dominated by the FM one.Eight different pickings deﬁning the four time windows,illustrated in Fig.3(a),are computed using synthetic mode-branch wave trains and are checked manually.For this reason,this method is not completely automated,but this picking step is necessary to assess the data quality and the consistency between recorded and synthetic seismograms.In Appendix B,we show that the phase velocity measurements are not signiﬁcantly affected by a small change in the time window dimensions.An advantage of this temporal truncation is that,whatever the amplitude of the FM,the HM part of the seismograms can always be treated.Hence,the forward problem is now split into two equations,corresponding to the FM and to the HM parts,respectively.A(R) FM (r,ω)expi (R)FM(r,ω)=A(S)0(r,ω)expi(S)0(r,ω)+ωaC(ω)p0(r,ω)(8)andA(R) HM (r,ω)expi (R)HM(r,ω)=6j=1A(S)j(r,ω)expi(S)j(r,ω)+ωaC(S)j(ω)p j(r,ω).(9)Seismograms(real and synthetic)are bandpassﬁltered between40and500s.In this frequency range,only theﬁrst six overtone phase velocities can be efﬁciently retrieved.Tests on synthetic seismograms(up to n=15)with various depths and source parameters have shown that the HM for n≥7have negligible amplitudes in the selected time and frequency windows.C 2003RAS,GJI,155,289–307The roller coaster technique293Figure3.(a)Real vertical seismogram(solid line)and its corresponding synthetic computed in PREM(dotted line).The earthquake underlying this waveform occurred on1993September4in Afghanistan(36◦N,70◦E,depth of190km)and was recorded at the CAN GEOSCOPE station(Australia).The epicentral distance is estimated at around11340km.Both waveforms are divided into two time windows corresponding to the higher modes(T1–T2,T5–T6)and to the fundamental mode(T3–T4,T7–T8).(b)The contribution of each synthetic monomode shows the large-amplitude discrepancy and time delay between the fundamental mode and the overtones.The different symbols refer to the spectra displayed in Fig.4.3.2Clustering the eventsFollowing eq.(8),a single seismogram is sufﬁcient to measure the FM phase velocity,whereas for the HM(eq.9)the problem is still highly underdetermined since the different HM group velocities are very close.This can be avoided by a reduction of the number of independent parameters considering mathematical relations between different mode-branch phase velocities.The consequence of such an approach is to impose a strong a priori knowledge on the model space,which may be physically unjustiﬁed.Another way to reduce this underdetermination is to increase the amount of independent data while keeping the parameter space dimension constant.Therefore,all sufﬁciently close events are clustered into small areas,and each individual ray path belonging to the same box is considered to give equivalent results as a common ray path.This latter approach was followed by Stutzmann&Montagner(1993),but with5×5deg2boxes independently of epicentral distance and azimuth values,due to the limited number of data.Here,in order to prevent any bias induced by the clustering of events too far away from one to another,and to be consistent with the smallest wavelength,boxes are computed with a maximum aperture angle of2◦and4◦in the transverse and longitudinal directions,respectively(Fig.5),with respect to the great circle path.The boxes are computed in order to take into account as many different depths and source mechanisms as possible.The FM phase velocity inversion is performed for each path between a station and a box,whereas the HM phase velocities are only measured for the boxes including three or more events.Since only the sixﬁrst mode-branches spectra are inverted,the maximum number of events per box is set to eight.The use of different events implies average phase velocity measurements along the common ray paths which can be unsuitable for short epicentral distances,but increases the accuracy of the results for the epicentral distances considered.C 2003RAS,GJI,155,289–307294´E.Beucler,´E.Stutzmann and J.-P.MontagnerFigure4.(a)The normalized amplitude spectra of the whole real waveform(solid line)displayed in Fig.3(a).The real FM part of the signal(truncated between T3and T4)is represented as a dotted line and the real HM part(between T1and T2)as a dashed line.(b).The solid line corresponds to the normalized spectrum amplitude of the real signal truncated between T3and T4(Fig.3a).The corresponding synthetic FM is represented as a dotted line and only the frequency range represented by the white circles is selected as being signiﬁcant.(c)Selection of HM inversion frequency ranges using synthetic signiﬁcant amplitudes.The solid line corresponds to the real HM signal,picked between T1and T2(Fig.3a).For each mode-branch(dotted lines),only the frequency ranges deﬁned by the symbols(according to Fig.3b)are retained for the inversion.(d)Close up of the sixth synthetic overtone,in order to visualize the presence of lobes and the weak contribution frequency range in the spectrum amplitude.The stars delimit the selected frequency range.3.3Determination of the model space dimensionReal and synthetic amplitude spectra are normalized in order to minimize the effects due to the imprecision of source parameters and of instrumental response determination.As presented in Fig.4,a synthetic mode-branch spectrum is frequently composed by several lobes due to the source mechanism.Between each lobe and also near the frequency range edges due to the bandpassﬁlter,the amplitude strongly decreases down to zero,and therefore phase velocities are absolutely not constrained at these frequencies.It is around these frequencies that possible local2πphase jumps may occur(Fig.1).Then,we decide to reduce the model space dimension in order to take into account only well-constrained points.For each spectrum,the selection of signiﬁcant amplitudes,with a thresholdﬁxed to10per cent of the mean maximum spectra amplitude,deﬁnes the inverted frequency range.In the case of several lobes in a synthetic mode-branch amplitude spectrum,only the most energetic one is selected as shown in Figs4(c)and(d).For a given mode-branch,the simultaneous use of different earthquakes implies a discrimination criterion based upon a mean amplitude spectrum of all spectra,which tends to increase the dimensions of the signiﬁcant frequency range.The normalization and this selection of each mode-branch signiﬁcant amplitudes is also a way to include surface wave radiation pattern information in the procedure.Changes in source parameters can result in changes in the positions of the lobes in the mode-branch amplitude spectra over the whole frequency range(40–500s).In the future,it will be essential to include these possible biases in the scheme and then to simultaneously invert moment tensor,location and depth.C 2003RAS,GJI,155,289–307The roller coaster technique295Figure5.Geographical distribution of inversion boxes for the SSB GEOSCOPE station case.The enlarged area is deﬁned by the bold square in the inset (South America).Black stars denote epicentres and hatched grey boxes join each inversion group.Each common ray path(grey lines)starts from the barycentre (circles)of all events belonging to the same box.The maximum number of seismograms per box isﬁxed at eight.3.4Exploration of the model space at very large scaleThe main idea of this stage is to test a large number of phase velocity large-scale perturbations with the view of selecting several starting vectors for local inversions(see Section3.5).The high non-linearity of the problem is mainly due to the possible2πphase jumps.And,even though the previous stage(see Section3.3)prevents the shifts inside a given mode-branch phase velocity curve,2πphase jumps over the whole selected frequency range are still possible.For this reason a classical gradient least-squares optimization(Tarantola&Valette1982a)is inadequate.In a highly non-linear problem,a least-squares inversion only converges towards the best misﬁt model that is closest to the starting model and the number of iterations cannot change this feature.On the other hand,a complete exploration of all possible conﬁgurations in the parameter space is still incompatible with a short computation time procedure.Therefore,an exploration of the model space is performed at very large scale,in order to detect all possible models that globally explain the data set well.3.4.1Fundamental mode caseWhen considering a single mode-branch,the number of parameter vector components is rather small.The FM large-scale exploration can then be more detailed than in the HM case.Considering that,at low frequencies,data are correctly explained by the1-D reference model,the C 2003RAS,GJI,155,289–307296´E.Beucler,´E.Stutzmann and J.-P.MontagnerabFigure6.(a)Five examples of the FM parameter vector conﬁgurations during the exploration of the model space at large scale corresponding toαvalues equal to−5,−,0,+2.5and+5per cent.The selected points for which the phase velocity is measured(see Section3.3)are ordered into parameter vector components according to increasing frequency values.Theﬁrst indices then correspond to the low-frequency components(LF)and the last ones to the high-frequency(HF) components.Varying the exploration factorα,different perturbation shapes are then modelled and the misﬁt between data and the image of the corresponding vector is measured(represented in theﬁgure below).(b)The misﬁt in the FM case,symbolized by+,is the expression of the difference between data and the image of the tested model(referred to as pα)through the g function(eq.8).Theαvalues are expressed as a percentage with respect to the PREM.As an example,theﬁve stars correspond to the misﬁt values of theﬁve models represented in theﬁgure above.The circles represent the bestαvalues and the corresponding vectors are then considered as possible starting models for the next stage.dimensionless phase velocity perturbation(referred to as pα)can be modelled as shown in theﬁve examples displayed in Fig.6(a).Basically, the low-frequency component perturbations are smaller than the high-frequency ones.However,if such an assumption cannot be made,the simplest way to explore the model space is then byﬁxing an equalαperturbation value for all the components.The main idea is to impose strong correlations between all the components in order to estimate how high the non-linearity is.Varyingαenables one to compute different parameter vectors and solving eq.(8)to measure the distance between data and the image of a given model through the g function,integrated over the whole selected frequency range.Considering that only small perturbations can be retrieved,the exploration range is limited between−5and+5per cent,using an increment step of0.1per cent.The result of such an exploration is displayed in Fig.6(b)and clearly illustrates the high non-linearity and non-unicity of the problem.In a weakly non-linear problem,the misﬁt curve(referred to as||d−g(pα)||)should exhibit only one minimum.This would indicate that,whatever the value of the starting model,a gradient algorithm always converges towards the sameﬁnal model,the solution is then unique.In our case,Fig.6(b)shows that,when choosing the reference model(i.e.α=0per cent)as the starting model,a gradient least-squares optimization converges to the nearest best-ﬁtting solution(corresponding to the third circle),and could never reach the global best-ﬁtting model(in this example representedC 2003RAS,GJI,155,289–307The roller coaster technique 297by the fourth circle).Therefore,in order not to a priori limit the inversion result around a given model,all minima of the mis ﬁt curve (Fig.6b)are detected and the corresponding vectors are considered as possible starting models for local optimizations (see Section 3.5).3.4.2Higher-mode caseThe introduction of several mode-branches simultaneously is much more dif ﬁcult to treat and it becomes rapidly infeasible to explore the model space as accurately as performed for the FM.However,a similar approach is followed.In order to preserve a low computation time procedure,the increment step of αis ﬁxed at 1per cent.The different parameter vectors are computed as previously explained in Section3.4.1(the shape of each mode-branch subvector is the same as the examples displayed in Fig.6a).In order to take into account any possible in ﬂuence of one mode-branch on another,all combinations are tested systematically.Three different explorations of the model space are performed within three different research ranges:[−4.5to +1.5per cent],[−3to +3per cent]and [−1.5to +4.5per cent].For each of them,76possibilities of the parameter vector are modelled and the mis ﬁt between data and the image of the tested vector through the g function is computed.This approach is almost equivalent to performing a complete exploration in the range [−4.5to +4.5per cent],using a step of 0.5per cent,but less time consuming.Finally,all mis ﬁt curve minima are detected and,according to a state of null information concerning relations between each mode-branch phase velocities,all the corresponding vectors are retained as possible starting models.Thus,any association between each starting model subvectors is allowed.3.5Matching the short-wavelength variations of the modelIn this section,algorithms,notation and comments are identical for both FM and HM.Only the main ideas of the least-squares criterion are outlined.A complete description of this approach is given by Tarantola &Valette (1982a,b)and by Tarantola (1987).Some typical features related to the frequency/period duality are also detailed.3.5.1The gradient least-squares algorithmThe main assumption which leads us to use such an optimization is to consider that starting from the large-scale parameter vector (see Section 3.4),the non-linearity of the problem is largely reduced.Hence,to infer the model space from the data space,a gradient least-squares algorithm is performed (Tarantola &Valette 1982a).The expression of the model (or parameter)at the k th iteration is given by p k =p 0+C p ·G T k −1· C d +G k −1·C p ·G T k −1−1· d −g (p k −1)+G k −1·(p k −1−p 0) ,(10)where C p and C d are the a priori covariance operators on parameters and data,respectively,p 0the starting model,and where G k −1=∂g (p k −1)/∂p k −1is the matrix of partial derivatives of the g function established in eqs (8)and (9).The indices related to p are now expressing the iteration rank and no longer the mode-branch radial order.De ﬁning the k th image of the mis ﬁt function byS (p k )=12[g (p k )−d ]T ·C −1d ·[g (p k )−d ]+(p k −p 0)T ·C −1p ·(p k −p 0) ,(11)the maximum-likelihood point is de ﬁned by the minimum of S (p ).Minimizing the mis ﬁt function is then equivalent to ﬁnding the best compromise between decreasing the distance between the data vector and the image of the parameter vector through the g function,in the data space on one hand (ﬁrst part of eq.11),and not increasing the distance between the starting and the k th model on the other hand (second part of eq.11),following the covariances de ﬁned in the a priori operators on the data and the parameters.3.5.2A priori data covariance operatorThe a priori covariance operator on data,referred to as C d ,includes data errors and also all effects that cannot be modelled by the g function de ﬁned in eq.(8)and (9).The only way to really measure each data error and then to compute realistic covariances in the data space,would be to obtain exactly the corresponding seismogram in which the signal due to the seismic event is removed.Hence,errors over the data space are impossible to determine correctly.In order to introduce as little a priori information as possible,the C d matrix is computed with a constant value of 0.04(including data and theory uncertainties)for the diagonal elements and zero for the off-diagonal elements.In other words,this choice means that the phase velocity perturbations are expected to explain at least 80per cent of the recorded signal.3.5.3A priori parameter covariance operatorIn the model space,the a priori covariance operator on parameters,referred to as C p ,controls possible variations between the model vector components for a given iteration k (eq.10),and also between the starting and the k th model (eq.11).Considering that the phase velocity perturbation between two adjoining components (which are ordered according to increasing frequency values)of a given mode-branch do not vary too rapidly,C p is a non-diagonal matrix.This a priori information reduces the number of independent components and then induces smoothed phase velocity perturbation curves.A typical behaviour of our problem resides in the way the parameter space is discretized.In the matrix domain,the distance between two adjoining components is always the same,whereas,as the model space is not evenly spaced C 2003RAS,GJI ,155,289–307。

Search for a new state of matter – the Quark-Gluon Plasma一个物质–夸克胶子等离子体的新状态搜索 43

-- Fragmentation
-- multi-parton dynamics (recombination or coalescence or …)
-- Hydrodynamics (constituent quarks ? parton dynamics from gluons to constituent quarks? )
Nucleus-Nucleus Collisions and Volcanic Eruption
Volcanic high pT -- Strombolian eruption
Volcanic mediate pT – Spatter (clumps)
6
Volcanic low pT – Bulk matter flows
Evidence for Multi-parton Dynamics in Hadronization of Bulk Partonic Matter at RHIC
Huan Zhong Huang (黄焕中) Department of Physics and Astronomy
University of California Los Angeles
Rafelski+Danos, Molnar+Voloshin …..) Quark Recombination – (R.J. Fries et al, R. Hwa et al)
13
Constituent Quark Scaling
Constituent (n) Quark Scaling -- Meson n=2 and Baryon n=3 grouping
p td d td p N y d 2 1 π p td dtd p N 1 y i 12ic vo s ψ R () i)1( 0

O(alpha) QED Corrections to Polarized Elastic $mu e$ and Deep Inelastic l N Scattering

a r X i v :h e p -p h /9711228v 1 4 N o v 1997hep-ph/9711228October 1997O (α)QED Corrections to Polarized Elastic µe and Deep Inelastic lN ScatteringDima Bardin a,b,c ,Johannes Bl¨u mlein a ,Penka Christova a,d ,and Lida Kalinovskaya a,caDESY–Zeuthen,Platanenallee 6,D–15735Zeuthen,GermanybINFN,Sezione di Torino,Torino,ItalycJINR,ul.Joliot-Curie 6,RU–141980Dubna,RussiadBishop Konstantin Preslavsky University of Shoumen,9700Shoumen,BulgariaAbstractTwo computer codes relevant for the description of deep inelastic scattering oﬀpolarized targets are discussed.The code µe la deals with radiative corrections to elastic µe scattering,one method applied for muon beam polarimetry.The code HECTOR allows to calculate both the radiative corrections for unpolarized and polarized deep inelastic scattering,including higher order QED corrections.1IntroductionThe exact knowledge of QED,QCD,and electroweak (EW)radiative corrections (RC)to the deep inelastic scattering (DIS)processes is necessary for a precise determination of the nucleon structure functions.The present and forthcoming high statistics measurements of polarized structure functions in the SLAC experiments,by HERMES,and later by COMPASS require the knowledge of the RC to the DIS polarized cross-sections at the percent level.Several codes based on diﬀerent approaches for the calculation of the RC to DIS experiments,mainly for non-polarized DIS,were developped and thoroughly compared in the past,cf.[1].Later on the radiative corrections for a vast amount of experimentally relevant sets of kinematic variables were calculated [2],including also semi-inclusive situations as the RC’s in the case of tagged photons [3].Furthermore the radiative corrections to elastic µ-e scattering,a process to monitor (polarized)muon beams,were calculated [4].The corresponding codes are :•HECTOR 1.00,(1994-1995)[5],by the Dubna-Zeuthen Group.It calculates QED,QCD and EW corrections for variety of measuremets for unpolarized DIS.•µe la 1.00,(March 1996)[4],calculates O (α)QED correction for polarized µe elastic scattering.•HECTOR1.11,(1996)extends HECTOR1.00including the radiative corrections for polarized DIS[6],and for DIS with tagged photons[3].The beta-version of the code is available from http://www.ifh.de/.2The Programµe laMuon beams may be monitored using the processes ofµdecay andµe scattering in case of atomic targets.Both processes were used by the SMC experiment.Similar techniques will be used by the COMPASS experiment.For the cross section measurement the radiative corrections to these processes have to be known at high precision.For this purpose a renewed calculation of the radiative corrections toσ(µe→µe)was performed[4].The diﬀerential cross-section of polarized elasticµe scattering in the Born approximation reads,cf.[7],dσBORNm e Eµ (Y−y)2(1−P e Pµ) ,(1)where y=yµ=1−E′µ/Eµ=E′e/Eµ=y e,Y=(1+mµ/2/Eµ)−1=y max,mµ,m e–muon and electron masses,Eµ,E′µ,E′e the energies of the incoming and outgoing muon,and outgoing electron respectively,in the laboratory frame.Pµand P e denote the longitudinal polarizations of muon beam and electron target.At Born level yµand y e agree.However,both quantities are diﬀerent under inclusion of radiative corrections due to bremsstrahlung.The correction factors may be rather diﬀerent depending on which variables(yµor y e)are used.In the SMC analysis the yµ-distribution was used to measure the electron spin-ﬂip asymmetry A expµe.Since previous calculations,[8,9],referred to y e,and only ref.[9]took polarizations into account,a new calculation was performed,including the complete O(α)QED correction for the yµ-distribution,longitudinal polarizations for both leptons,theµ-mass eﬀects,and neglecting m e wherever possible.Furthermore the present calculation allows for cuts on the electron re-coil energy(35GeV),the energy balance(40GeV),and angular cuts for both outgoing leptons (1mrad).The default values are given in parentheses.Up to order O(α3),14Feynman graphs contribute to the cross-section forµ-e scattering, which may be subdivided into12=2×6pieces,which are separately gauge invariantdσQEDdyµ.(2) One may express(2)also asdσQEDdyµ+P e Pµdσpol kk=1−Born cross-section,k=b;2−RC for the muonic current:vertex+bremsstrahlung,k=µµ;3−amm contribution from muonic current,k=amm;4−RC for the electronic current:vertex+bremsstrahlung,k=ee;5−µe interference:two-photon exchange+muon-electron bremsstrahlung interference,k=µe;6−vacuum polarization correction,runningα,k=vp.The FORTRAN code for the scattering cross section(2)µe la was used in a recent analysis of the SMC collaboration.The RC,δA yµ,to the asymmetry A QEDµeshown inﬁgures1and2is deﬁned asδA yµ=A QEDµedσunpol.(4)The results may be summarized as follows.The O(α)QED RC to polarized elasticµe scattering were calculated for theﬁrst time using the variable yµ.A rather general FORTRAN codeµe la for this process was created allowing for the inclusion of kinematic cuts.Since under the conditions of the SMC experiment the corrections turn out to be small our calculation justiﬁes their neglection. 3Program HECTOR3.1Diﬀerent approaches to RC for DISThe radiative corrections to deep inelastic scattering are treated using two basic approaches. One possibility consists in generating events on the basis of matrix elements including the RC’s. This approach is suited for detector simulations,but requests a very hughe number of events to obtain the corrections at a high precision.Alternatively,semi-analytic codes allow a fast and very precise evaluation,even including a series of basic cuts andﬂexible adjustment to speciﬁc phase space requirements,which may be caused by the way kinematic variables are experimentally measured,cf.[2,5].Recently,a third approach,the so-called deterministic approach,was followed,cf.[10].It treats the RC’s completely exclusively combining features of fast computing with the possibility to apply any cuts.Some elements of this approach were used inµe la and in the branch of HECTOR1.11,in which DIS with tagged photons is calculated.Concerning the theoretical treatment three approaches are in use to calculate the radiative corrections:1)the model-independent approach(MI);2)the leading-log approximation(LLA); and3)an approach based on the quark-parton model(QPM)in evaluating the radiative correc-tions to the scattering cross-section.In the model-independent approach the QED corrections are only evaluated for the leptonic tensor.Strictly it applies only for neutral current processes.The hadronic tensor can be dealt with in its most general form on the Lorentz-level.Both lepton-hadron corrections as well as pure hadronic corrections are neglected.This is justiﬁed in a series of cases in which these corrections turn out to be very small.The leading logarithmic approximation is one of the semi-analytic treatments in which the diﬀerent collinear singularities of O((αln(Q2/m2l))n)are evaluated and other corrections are neglected.The QPM-approach deals with the full set of diagrams on the quark level.Within this method,any corrections(lepton-hadron interference, EW)can be included.However,it has limited precision too,now due to use of QPM-model itself. Details on the realization of these approaches within the code HECTOR are given in ref.[5,11].3.2O (α)QED Corrections for Polarized Deep Inelastic ScatteringTo introduce basic notation,we show the Born diagramr rr r j r r r r l ∓( k 1,m )l ∓( k 2,m )X ( p ′,M h )p ( p ,M )γ,Z ¨¨¨¨B ¨¨¨¨£¢ ¡£¢ ¡£¢ ¡£¢ ¡£¢ ¡£¢ ¡£¢ ¡£¢ ¡£¢ ¡£¢ ¡z r r r r r r r r r r r r r rr ¨¨¨¨B ¨¨¨¨r r r r j r r r r and the Born cross-section,which is presented as the product of the leptonic and hadronic tensordσBorn =2πα2p.k 1,x =Q 2q 2F 1(x,Q 2)+p µ p ν2p.qF 3(x,Q 2)+ie µνλσq λs σ(p.q )2G 2(x,Q 2)+p µ s ν+ s µ p νp.q1(p.q )2G 4(x,Q 2)+−g µν+q µq νp.qG 5(x,Q 2),(8)wherep µ=p µ−p.qq 2q µ,and s is the four vector of nucleon polarization,which is given by s =λp M (0, n )in the nucleonrest frame.The combined structure functions in eq.(8)F1,2(x,Q2)=Q2e Fγγ1,2(x,Q2)+2|Q e|(v l−p eλl a l)χ(Q2)FγZ1,2(x,Q2)+ v2l+a2l−2p eλl v l a l χ2(Q2)F ZZ1,2(x,Q2),F3(x,Q2)=2|Q e|(p e a l−λl v l)χ(Q2)FγZ3(x,Q2),+ 2p e v l a l−λl v2l+a2l χ2(Q2)F ZZ3(x,Q2),G1,2(x,Q2)=−Q2eλl gγγ1,2(x,Q2)+2|Q e|(p e a l−λl v l)χ(Q2)gγZ1,2(x,Q2),+ 2p e v l a l−λl v2l+a2l χ2(Q2)g ZZ1,2(x,Q2),G3,4,5(x,Q2)=2|Q e|(v l−p eλl a l)χ(Q2)gγZ3,4,5(x,Q2),+ v2l+a2l−2p eλl v l a l χ2(Q2)g ZZ3,4,5(x,Q2),(9) are expressed via the hadronic structure functions,the Z-boson-lepton couplings v l,a l,and the ratio of the propagators for the photon and Z-bosonχ(Q2)=Gµ2M2ZQ2+M2Z.(10)Furthermore we use the parameter p e for which p e=1for a scattered lepton and p e=−1for a scattered antilepton.The hadronic structure functions can be expressed in terms of parton densities accounting for the twist-2contributions only,see[12].Here,a series of relations between the diﬀerent structure functions are used in leading order QCD.The DIS cross-section on the Born-leveld2σBorndxdy +d2σpol Borndxdy =2πα2S ,S U3(y,Q2)=x 1−(1−y)2 ,(13) and the polarized partdσpol BornQ4λp N f p S5i=1S p gi(x,y)G i(x,Q2).(14)Here,S p gi(x,y)are functions,similar to(13),and may be found in[6].Furthermore we used the abbrevationsf L=1, n L=λp N k 12πSy 1−y−M2xy2π1−yThe O(α)DIS cross-section readsd2σQED,1πδVRd2σBorndx l dy l=d2σunpolQED,1dx l dy l.(16)All partial cross-sections have a form similar to the Born cross-section and are expressed in terms of kinematic functions and combinations of structure functions.In the O(α)approximation the measured cross-section,σrad,is deﬁne asd2σraddx l dy l +d2σQED,1dx l dy l+d2σpol radd2σBorn−1.(18)The radiative corrections calculated for leptonic variables grow towards high y and smaller values of x.Theﬁgures compare the results obtained in LLA,accounting for initial(i)andﬁnal state (f)radiation,as well as the Compton contribution(c2)with the result of the complete calculation of the leptonic corrections.In most of the phase space the LLA correction provides an excellent description,except of extreme kinematic ranges.A comparison of the radiative corrections for polarized deep inelastic scattering between the codes HECTOR and POLRAD[17]was carried out.It had to be performed under simpliﬁed conditions due to the restrictions of POLRAD.Corresponding results may be found in[11,13,14].3.3ConclusionsFor the evaluation of the QED radiative corrections to deep inelastic scattering of polarized targets two codes HECTOR and POLRAD exist.The code HECTOR allows a completely general study of the radiative corrections in the model independent approach in O(α)for neutral current reac-tions including Z-boson exchange.Furthermore,the LLA corrections are available in1st and2nd order,including soft-photon resummation and for charged current reactions.POLRAD contains a branch which may be used for some semi-inclusive DIS processes.The initial state radia-tive corrections(to2nd order in LLA+soft photon exponentiation)to these(and many more processes)can be calculated in detail with the code HECTOR,if the corresponding user-supplied routine USRBRN is used together with this package.This applies both for neutral and charged current processes as well as a large variety of diﬀerent measurements of kinematic variables. Aside the leptonic corrections,which were studied in detail already,further investigations may concern QED corrections to the hadronic tensor as well as the interference terms. References[1]Proceedings of the Workshop on Physics at HERA,1991Hamburg(DESY,Hamburg,1992),W.Buchm¨u ller and G.Ingelman(eds.).[2]J.Bl¨u mlein,Z.Phys.C65(1995)293.[3]D.Bardin,L.Kalinovskaya and T.Riemann,DESY96–213,Z.Phys.C in print.[4]D.Bardin and L.Kalinovskaya,µe la,version1.00,March1996.The source code is availablefrom http://www.ifh.de/~bardin.[5]A.Arbuzov,D.Bardin,J.Bl¨u mlein,L.Kalinovskaya and T.Riemann,Comput.Phys.Commun.94(1996)128,hep-ph/9510410[6]D.Bardin,J.Bl¨u mlein,P.Christova and L.Kalinovskaya,DESY96–189,hep-ph/9612435,Nucl.Phys.B in print.[7]SMC collaboration,D.Adams et al.,Phys.Lett.B396(1997)338;Phys.Rev.D56(1997)5330,and references therein.[8]A.I.Nikischov,Sov.J.Exp.Theor.Phys.Lett.9(1960)757;P.van Nieuwenhuizen,Nucl.Phys.B28(1971)429;D.Bardin and N.Shumeiko,Nucl.Phys.B127(1977)242.[9]T.V.Kukhto,N.M.Shumeiko and S.I.Timoshin,J.Phys.G13(1987)725.[10]G.Passarino,mun.97(1996)261.[11]D.Bardin,J.Bl¨u mlein,P.Christova,L.Kalinovskaya,and T.Riemann,Acta Phys.PolonicaB28(1997)511.[12]J.Bl¨u mlein and N.Kochelev,Phys.Lett.B381(1996)296;Nucl.Phys.B498(1997)285.[13]D.Bardin,J.Bl¨u mlein,P.Christova and L.Kalinovskaya,Preprint DESY96–198,hep-ph/9609399,in:Proceedings of the Workshop‘Future Physics at HERA’,G.Ingelman,A.De Roeck,R.Klanner(eds.),Vol.1,p.13;hep-ph/9609399.[14]D.Bardin,Contribution to the Proceedings of the International Conference on High EnergyPhysics,Warsaw,August1996.[15]M.Gl¨u ck,E.Reya,M.Stratmann and W.Vogelsang,Phys.Rev.D53(1996)4775.[16]S.Wandzura and F.Wilczek,Phys.Lett.B72(1977)195.[17]I.Akushevich,A.Il’ichev,N.Shumeiko,A.Soroko and A.Tolkachev,hep-ph/9706516.-20-18-16-14-12-10-8-6-4-200.10.20.30.40.50.60.70.80.91elaFigure 1:The QED radiative corrections to asymmetry without experimental cuts.-1-0.8-0.6-0.4-0.200.20.40.60.810.10.20.30.40.50.60.70.80.91elaFigure 2:The QED radiative corrections to asymmetry with experimental cuts.-50-40-30-20-100102030405000.10.20.30.40.50.60.70.80.91HectorFigure 3:A comparison of complete and LLA RC’s in the kinematic regime of HERMES for neutral current longitudinally polarized DIS in leptonic variables.The polarized parton densities [15]are used.The structure function g 2is calculated using the Wandzura–Wilczek relation.c 2stands for the Compton contribution,see [6]for details.-20-100102030405000.10.20.30.40.50.60.70.80.91HectorFigure 4:The same as in ﬁg.3,but for energies in the range of the SMC-experiment.-20-10010203040500.10.20.30.40.50.60.70.80.91HectorFigure 5:The same as in ﬁg.4for x =10−3.-200-150-100-5005010015020000.10.20.30.40.50.60.70.80.91HectorFigure 6:A comparison of complete and LLA RC’s at HERA collider kinematic regime for neutral current deep inelastic scattering oﬀa longitudinally polarized target measuring the kinematic variables at the leptonic vertex.。

2009PhysRevB_80_214110

Institute of Semiconductor Physics, 41 pr. Nauki, 03028 Kiev, Ukraine for Problems of Materials Science, National Academy of Science of Ukraine, 3 Krjijanovskogo, 03142 Kiev, Ukraine 3Department of Materials Science and Engineering, Pennsylvania State University, University Park, Pennsylvania 16802, USA 4 Oak Ridge National Laboratory, Oak Ridge, Tennessee 37831, USA 5Institute of Physics and Applied Mathematics, Ural State University, Ekaterinburg 620083, Russia ͑Received 24 September 2009; published 14 December 2009͒
2Institute 1
Thermodynamics of tip-induced nanodomain formation in scanning probe microscopy of ferroelectric ﬁlms and crystals is studied using the analytical Landau-Ginzburg-Devonshire approach and phase-ﬁeld modeling. The local redistribution of polarization induced by the biased probe apex is analyzed including the effects of polarization gradients, ﬁeld dependence of dielectric properties, intrinsic domain-wall width, and ﬁlm thickness. The polarization distribution inside a “subcritical” nucleus of the domain preceding the nucleation event is shown to be “soft” ͑i.e., smooth without domain walls͒ and localized below the probe, and the electrostatic ﬁeld distribution is dominated by the tip. In contrast, polarization distribution inside a stable domain is “hard” ͑i.e., sharp contrast with delineated domain walls͒ and the spontaneous polarization reorientation takes place inside a localized spatial region, where the absolute value of the resulting electric ﬁeld is larger than the thermodynamic coercive ﬁeld. The calculated coercive biases corresponding to formation of switched domains are in a good agreement with available experimental results for typical ferroelectric materials. The microscopic origin of the observed domain-tip elongation in the region where the probe electric ﬁeld is much smaller than the intrinsic coercive ﬁeld is the positive depolarization ﬁeld in front of the moving-counter domain wall. For inﬁnitely thin domain wall the depolarization ﬁeld outside the semiellipsoidal domain tip is always higher than the intrinsic coercive ﬁeld that must initiate the local domain breakdown through the sample depth while the domain length is ﬁnite in the energetic approach evolved by Landauer and Molotskii ͑we refer the phenomenon as Landauer-Molotskii paradox͒. Our approach provides the solution of the paradox: the domain vertical growth should be accompanied by the increase in the charged domain-wall width. DOI: 10.1103/PhysRevB.80.214110 PACS number͑s͒: 77.80.Fm, 77.22.Ej

The kinematic Sunyaev Zeldovich effect and transverse cluster velocities

Mon. Not. R. Astron. Soc. 000, 000–000 (1997)
Printed 1 February 2008
A (MN L TEX style ﬁle v1.4)
The kinematic Sunyaev Zeldovich eﬀect and transverse cluster veloc812310v1 16 Dec 1998
Accepted 199- Received 199- in original form 199-
ABSTRACT
The polarization of the CMBR scattered by galaxy clusters in the kinematic Sunyaev Zeldovich eﬀect depends on the transverse velocity of the cluster. This polarizing eﬀect is proportional to the transverse velocity squared, and so weaker that the change in intensity due to the radial motion in the kinematic eﬀect. The value given by Sunyaev and Zeldovich, and which is frequently cited, underestimates the polarizing eﬀect by a factor of ten. We show furthermore that the polarization has a strong frequency dependence. This means that the polarization should be detectable with the new generation of CMBR probes, at least for some clusters. Thus this eﬀect oﬀers, almost uniquely, a method of obtaining the vectorial velocity of clusters. Key words: Cosmology: theory – cosmic microwave – polarization

Carbon Fibers碳纤维

Vol.9CARBON FIBERS91CARBON FIBERSIntroductionCarbonﬁbers continue to be the main reinforcement materials in advanced com-posites(qv).The ability to manipulate their physical,chemical,electrical,and thermal properties makes carbonﬁbers suitable across a wide range of commer-cial applications,including military[aircraft and missiles(1)],structural[con-crete reinforcement(2,3)and automobile body panels],sports equipment(golf Encyclopedia of Polymer Science and Technology.Copyright John Wiley&Sons,Inc.All rights reserved.92CARBON FIBERS Vol.9Fig.1.Tensile strength versus modulus for some commercial carbonﬁbers.From Ref.8. To convert GPa to psi,multiply by145,000.clubs,skis,surfboards,and softball bats),energy storage[batteries and capaci-tors(4,5)],and activated carbons(catalysis,gas storage,and puriﬁcation)(6,7). The ultimate properties of the carbonﬁber directly depend on the selection and processing of the precursor materials,the formation of theﬁber,and subsequent processing of theﬁber.Figure1shows a comparison of carbonﬁber strengths and moduli as a function of precursor selection and treatment temperature.Carbonﬁbers are deﬁned by the International Union of Pure and Applied Chemistry(IUPAC)as“ﬁbers(ﬁlaments,tows,yarns,rovings)consisting of at least 92%(mass fraction)carbon,usually in a non-graphitic state(9).”Carbonﬁbers are produced either through carbonization(the controlled pyrolysis of aﬁber precur-sor in an inert atmosphere)or through growth from gaseous hydrocarbons.The carbonization process is preceded by a stabilization step in which precursorﬁbers are converted to thermally stable forms that will not melt during carbonization. During the carbonization process,inorganics and aliphatic carbons are driven off, leaving aﬁber consisting of graphene layer planes.An idealized carbonﬁber is shown in Figure2.Real carbonﬁbers cannot achieve the graphitic ideal.Instead the graphene planes are uneven and wavy,with a mean interplanar spacing signiﬁcantly greater than the0.335nm of graphite.This turbostratic structure of carbonﬁbers is shown in Figure3.The carbonﬁber production process involves a series of steps including spin-ning of the precursorﬁber,stabilization,carbonization,and post-carbonization treatments that include sizing and surface treatments.The speciﬁcs of these steps vary greatly,depending on the precursor material and the desired properties of the carbonﬁber.Figure4shows a schematic of the carbonﬁber production process.In the United States,carbonﬁbers are classiﬁed into two broad categories: high performance(HP)and general performance(GP)(12).High performance ﬁbers are further classiﬁed into high tensile(HT)and high modulus(HM). Table1summarizes the tensile properties of HT and HMﬁbers.Vol.9CARBON FIBERS93Fig.2.Idealized carbonﬁber structure.Adapted from Ref.10.Fig.3.The turbostratic structure of carbonﬁbers.Adapted from Ref.11.Fig.4.Schematic of carbonﬁber production.Adapted from Ref.11.94CARBON FIBERS Vol.9 Table1.Properties of High Performance Carbon Fibers aFiber type Tensile strength,GPa Young’s modulus,GPa High tensile(HT) 3.0–7.1150–300High modulus(HM)0.2–3.0400–900a Ref.12.This categorization of carbonﬁbers is not uniform.In the United Kingdom, theﬁber categories are Type I(HM),Type II(HS),and Type III(GP)(13).Some researchers refer toﬁbers at the higher end of the Young’s modulus range as ultra-high modulus(UHM)ﬁbers(14).Fibers are also distinguished as being graphi-tizable or nongraphitizable.Graphitizableﬁbers can,upon heat treatment,be converted to graphene layer planes with a mean interlayer spacing of less than 0.34nm(9),compared to the0.335-nm spacing for pure graphite.Although there is an objective standard for graphitizableﬁbers,many researchers incorrectly use the term to describe any carbonﬁber that develops measurable three-dimensional order.The Inorganic Chemistry Division Commission on High Temperature and Solid State Chemistry Subcommittee has developed recommended terminology to alleviate the confusion in nomenclature(9),but the multiple terminologies remain embedded in the culture of carbonﬁber research.The majority of commercial carbonﬁbers are produced from polyacryloni-trile(PAN)ﬁbers.In fact,HTA-12K PAN-based carbonﬁbers are the most com-monly used commercial carbonﬁber(15).PAN-basedﬁbers are the strongest commercially available carbonﬁbers and dominate structural applications. Mesophase pitch-based carbonﬁbers represent a smaller but signiﬁcant market niche.Theseﬁbers develop exceptional moduli and excel in lattice-based prop-erties,including stiffness and thermal conductivity(1).Rayon-basedﬁbers are used in heat shielding and in missile nosecones(16).Carbonﬁbers made from high performance polymers(17–19)or from chemical vapor deposition of hydro-carbons,such as benzene or methane,display unique properties that make them potentially attractive future alternatives(20–22).PAN-Based Carbon FibersPAN-based carbonﬁbers develop exceptional tensile strength[in excess of 7000MPa(23)]and are more resistant to compressive failure than high perfor-mance polymers(24).These factors combine to make PAN-based carbonﬁbers the ideal choice for applications requiring signiﬁcantﬁber strength.However, PAN-basedﬁbers are less appropriate than mesophase pitch-basedﬁbers for ap-plications in which molecular order-dependent properties are key.Commercial PAN is actually a copolymer of acrylonitrile and another monomer(vinyl acetate,methyl acrylate,or acrylic acid)that is added to lower the glass-transition temperature of the material and control its oxidation resis-tance(see A CRYLONITRILE AND A CRYLONITRILE P OLYMERS).The repeat unit of PAN is shown in Figure5.The copolymer is formed through a wet-spinning process in which the polymer solution is extruded directly into a liquid bath.A blend ofVol.9CARBON FIBERS95Fig.5.The molecular structure of PAN.phase separation and chemical reaction in the liquid results in solidiﬁcation of the polymer.A concentrated copolymer solution containing93–95wt%acrylonitrile is dissolved in a solvent(typically dimethylacetamide)to form a concentrated poly-mer solution that is fed to a storage tank(25).The solution isﬁltered to minimize impurities and passed through a spinnerette.Theﬁbers emerge through the small capillary holes directly into a coagula-tion bath containing ethylene glycol that extracts the solvent from theﬁber.The solvent extraction rate is governed by complex thermodynamic and kinetic rela-tionships but can be inﬂuenced by the selection of coagulation liquid,temperature, and the circulation rate of theﬂuid within the bath.Figure6shows a schematic representation of the wet spinning of PANﬁbers.The development of internal voids orﬂaws in theﬁber as well as the shape and texture of theﬁber are controlled by the solvent removal rate.Concentration gradients can occur during the solvent extraction process,resulting in a warping of theﬁber.For example,dog-bone–shapedﬁbers result from removing the sol-vent too rapidly(23).Theﬁber that emerges from the coagulation bath undergoes a series of post-spinning steps including washing,drawing,and drying during which theﬁber solidiﬁes into itsﬁnal form.These processes play a crucial role in developing the internal morphology of theﬁbers(26).Before the acrylicﬁbers(qv)produced in the wet-spinning process can be subjected to the elevated temperatures of carbonization,they must be converted to a thermally stable form that will not melt through oxidative stabilization.Sta-bilization is usually performed in air at temperatures between200and300◦C(27) and under tension to preventﬁber shrinkage(28).During the stabilization process,dehydrogenation,oxidation,and nitrile cy-clization reactions result in the formation of a condensed pyridine ring“ladder”structure that is suitable for subsequent carbonization(29).The general mecha-nism is shown in Figure7.The details of the stabilization mechanism are highlyFig.6.Wet spinning of PANﬁbers.Adapted from Ref.24.96CARBON FIBERS Vol.9Fig.7.General mechanism for the stabilization of PAN.Adapted from Ref.12. complex and have been the subject of many studies(30–33).All studies agree that control of the heating rate is essential because the reaction is highly exothermic. The stabilization time varies greatly with the size of theﬁber bundle,the tem-perature,and the oxidizing environment.Studies of stabilization at elevated tem-peratures(350–400◦C)show the development of additional intermolecular cross-linking,resulting in improved strengths(34,35).The stabilizedﬁber is carbonized in an inert atmosphere at temperatures ranging from1000to3000◦C,depending on the desired properties.During the pyrolysis,nearly all inorganic and nonaro-matic carbons are driven off.Primary off-gases include hydrogen cyanide,carbon dioxide,water,ammonia,methane,and hydrogen(35).The carbon yield during the process is generally in the range of40–45%(36).Heating rate plays a major role in controlling the release of gases.A decrease in tensile strength is generally observed at temperatures above1500◦C(37),which corresponds to theﬁnal major release of nitrogen(38).As a result,many of the highest strength PAN-based carbonﬁbers contain residual nitrogen.Although heat treatments of PAN-based carbonﬁbers above1700◦C are often referred to as graphitization,theseﬁbers are not graphitizable and do not develop highly ordered graphene planes.Because PAN-based carbonﬁbers are commonly used in applications requir-ing high tensile strength,great care must be taken to preserve the potential for high tensile strength during the spinning of the precursorﬁbers.Aﬁber is often viewed as a chain consisting of interlocking links.If there were no misalignments orﬂaws,each link would fail at essentially the same tensile load.However,ﬂaws in theﬁber act as weak links in the chain,lowering the observed tensile strength of theﬁber(39).As a result,the actual strength at which a givenﬁber will fail depends on both the frequency and severity of theseﬂaws(40,41).Macroscopic ﬂaws can result from inclusions,large voids,or damage from handling.Micro-scopicﬂaws can also lead to premature failure but are more difﬁcult to quantify. Johnson(42)attributed manyﬂaws to the misalignment of crystallites that in-terlink and result in voids.Highly orderedﬁbers are far more sensitive to these crystallite misalignments.As a result,ﬁbers with high moduli tend to have lower tensile strengths than less-ordered,less–ﬂaw-sensitive lower modulusﬁbers.Vol.9CARBON FIBERS97Fig.8.Schematic representation of a carbonized PANﬁber.Adapted from Ref.43.Diefendorf and Tokarsky(43)showed that carbonized PANﬁbers have aﬁb-rillar microstructure as shown in Figure8.This microstructure may be viewed as undulating ribbons and is highly resistant to premature tensile failure because of itsﬂexibility.Therefore,PAN-based carbonﬁbers are well suited to develop-ing high tensile strengths,but are not likely to develop the high level of three-dimensional order associated with high modulus precursor materials such as mesophase pitch.As such,PAN-based carbonﬁbers are considered nongraphitiz-ing and maintain a highly turbostratic organization of the graphene layer planes, even when exposed to very high treatment temperatures(42).Recent research in Poland is helping PAN-based carbonﬁbersﬁnd new appli-cations as biological implants.Low temperature carbonizedﬁbers have found use in ligament and tendon prostheses and in surgical sutures(44,45).Theseﬁbers are heat treated to temperatures below1300◦C and are far less crystalline than traditional carbonized PANﬁbers(46,47).Despite the promise of these studies, low temperature carbonized PANﬁbers do not share the commercial success of their high performance cousins.Mesophase Pitch-Based Carbon FibersPitch consists of a heavy fraction of predominantly aromatic hydrocarbons that is the residue of petroleum or coal tar distillation following the removal of creosote oil and anthracene(48).Table2provides a list of the major components in coal-tar fractions.The pitch consists of hundreds of thousands of different species with an average molecular weight of several hundreds.Many of the species are heterocyclic and are formed by a complex variety of chemical reactions,including thermal decompositions,hydrogen transfers,and oligomerization reactions(49). Pitch is a glassy solid at room temperature,but softens upon heating to form a98CARBON FIBERS Vol.9Table2.Major Components in Coal-Tar Fractions aBoiling Temperature,◦C<200200–250250–300300–350>350 Benzene Tar acids Methyl-naphthalene Phenathracene Chrysene Toluene Tar bases Acenapthene Anthracene Fluoranthene Xylene Naphthalene Fluorene Carbazole PyreneTar acids Diphenylene oxideTar basesSolventNaphthaa Ref.50.viscous liquid.Pitches are often characterized by determining their solubility in different organic solvents.Solvent fractionation also serves as a critical method of altering the composition of pitches prior toﬁber spinning(48).Certain pitches can be spun directly into isotropic pitchﬁbers with only minor devolitization.Carbonized isotropic pitchﬁbers cannot be graphitized and develop mechanical and thermal properties that are substantially inferior to those produced by other precursors.However,isotropic pitchﬁbers are very inexpensive and have found commercial applications in areas that do not require the excep-tional mechanical and thermal properties of mesophase pitch-based carbonﬁbers. Isotropic pitch-based carbonﬁbers are used inﬁlters,brake pads,activated car-bons,and as substrates for chemical vapor deposition(23).Table3summarizes properties of isotropic pitch-based carbonﬁbers.The physical explanation for why some precursors produced graphitizable carbons while others did not was developed by Brooks and Taylor(51).During thermal treatment of aromatic hydrocarbons between400and550◦C,an interme-diate liquid crystalline phase(mesophase)of spheroids with a mosaic structure formed within the continuous isotropic phase.Selected-area electron diffraction patterns show that each mesophase sphere has a single direction of preferred ori-entation as shown in Figure9.As the pyrolysis progresses,the spherules grow and coalesce.Ultimately,a phase inversion occurs and the mesophase becomes the continuous phase(52).There are no grain boundaries in the mesophase,though disclinations do exist.The composition of each phase changes continuously during the pyrolysis and when the entire material is mesophase,polymerization contin-ues with the release of volatiles(48).The formation of the mesophase can be Table3.Properties of Isotropic Pitch-Based Carbon Fibers aCarbon Density,Tensile strength,Young’s modulus, Fiber type wt%g/cm3MPa GPaT-101F>95 1.6579033T-101S>95 1.6572032T-201F>99 1.5769033T-201S>99 1.5759030a Ref.12.Vol.9CARBON FIBERS99mellar structure of mesophase spheruleinﬂuenced by numerous factors including the presence of insoluble particles,the presence of oxygen or sulfur,the applied pressure,the nature of the pitch itself, and the temperature of the heat-soak treatment.Partial oxidation of the pitch is particularly troubling because it results in the presence of inclusions of isotropic pitch which persist in the carbonized product and will not convert to mesophase at any treatment temperature(51).The two main processes for producing mesophase from pitch are heat soak-ing and solvent extraction.Theﬁrst heat-soaking process was developed at Union Carbide in1977to convert50wt%of low cost Ashland240isotropic pitch into a spinnable mesophase by heating the pitch for approximately40hours at400–410◦C(53).During this process,the higher density of the mesophase fraction caused it to collect on the bottom of the vessel.Agitating the pitch during pyrol-ysis led to a homogeneous emulsion of the mesophase and isotropic fraction that resulted in a more uniform and spinnable product(54).Chwastiak and Lewis(55) produced100%bulk mesophase by using an inert gas to agitate the mixture and remove volatile components.Subsequent research by Otani and Oya(56)produced a mesophase pitch with a lower softening temperature by adding a hydrogenation step either before or after the production of the mesophase.Figure10shows a typical molecule of mesophase pitch formed during the heat-soaking process.Mesophase is also produced through a solvent extraction technique.A por-tion of an isotropic pitch is extracted with an organic solvent such as benzene, hexane,or toluene.The remaining insoluble fraction is pyrolized for10min(as opposed to40h in the Lewis heat-soaking process)in a temperature range from 230◦C to400◦C,yielding a75–100wt%mesophase product(57).Both production strategies convert inexpensive pitch feedstocks to spinnable mesophases,but nei-ther can overcome some fundamental limitations of the feedstocks themselves. Pitch is a mixture of components reﬁned through a complex process of feedstocks that are inherently variable,making batch-to-batch consistency extremely difﬁ-cult.Further,during every step of pitch production and pyrolysis,impurities are concentrated in the retained fraction.These problems have spurred interest in alternative mesophase production strategies.Mochida(58)developed a process in which a synthetic mesophase is produced from the HF/BF3catalyzed polymerization of naphthalene or methyl naphthalene. HF/BF3is a Bronsted acid that has been used to catalyze coal liquifaction and aro-matic condensation.The aromatic resin(AR)-mesophase produced through this process is both more spinnable and more easily oxidized than that produced from100CARBON FIBERS Vol.9Fig.10.A typical heat-soaked mesophase molecule.Adapted from Ref.55. traditional pitch precursors.Mochida(59)reports that the thermal and mechani-cal properties of the carbonized AR-mesophaseﬁbers are comparable with those of the best commercialﬁbers.AR-mesophase has a much narrower molecular weight distribution than a mesophase formed from natural pitch and is free from sulfur and other impurities that present problems in traditional pitches.Figure11shows representative benzene-soluble fractions of AR-mesophase.Fig.11.Representative benzene-soluble fractions from AR-mesophase.Adapted from Ref.12.Vol.9CARBON FIBERS101Fig.12.The stacking behavior of mesophase pitch.Adapted from Ref.64.Another approach to improving the quality of the mesophase precursor in-volves supercriticalﬂuid extraction,which has been shown to fractionate isotropic petroleum pitches into fractions of different compositions,some of which contain 100%mesophase(60).When using supercriticalﬂuids,both temperature and pres-sure can be used to alter the strength of the solvent(61).Continuously varying the temperature and pressure allows selective fractions of relatively narrow molec-ular weight distributions to be isolated(62).Supercriticalﬂuid extraction offers several advantages in mesophase preparation including lower solvent require-ments and the ability to use a single,cost-effective solvent to produce a variety of pitch fractions(63).Once the mesophase pitch has been prepared,the next step in processing is spinning it intoﬁbers.This process is particularly important because when mesophase pitch is carbonized,the morphology of the pitch is the dominant fac-tor in determining the microstructure of the resulting graphiticﬁber.This re-sults from the stacking behavior of the mesophase molecules(64),as shown in Figure12.Mesophase pitchﬁbers are produced through melt spinning that is essen-tially the same as that used to spin commercial polymers.Figure13shows this process in which an extruder melts pitch particles and pumps the molten pitch through a multiholed spinnerette.The high extensional shear orients the liquid crystalline mesophase molecules as it approaches andﬂows through the spin-nerette.Theﬁbers emerging from the spinnerette are drawn by a windup spool.Although there are considerable similarities between melt spinning pitch and polymers,the rheological behavior of mesophase pitch is far more complex than that of most polymers.First,the viscosity of mesophase pitch displays an extreme sensitivity to temperature(65–67).This has direct impact onﬁber spin-ning because pitchﬁbers achieve theirﬁnal diameter within a few millimeters of exiting the spinnerette,unlike most polymeric materials.These data provide no insight into the texture in the developingﬁbers,but provide a basis for deter-mining appropriate spinning temperatures for pitches.Mesophase pitch exhibits shear-thinning behavior at low shear rates and becomes increasingly Newtonian102CARBON FIBERS Vol.9Fig.13.Melt spinning of mesophase pitchﬁbers.as shear rate increases(65).The pseudoplasticity of the mesophase likely relates to the alignment of the liquid crystalline mesophase domains at elevated shear (67).Mesophase pitch has been reported to display thixotropic behavior,but no clear basis for this behavior has been developed(68,69).McHugh and Edie(68)demonstrated that the microstructure of carbonized mesophase pitch-based carbonﬁbers can be predicted through the application of continuum theory.Leslie(69)and Ericksen(70)proposed a continuum theory that applies to theﬂow of nematics.In addition to the standard conservation equations (continuity and the equation of motion),a conservation of angular momentum equation(known as the balance of torques)is also considered.Unfortunately,the shear stress is not proportional to the shear rate.Instead,the viscous stress tensor is the sum of six terms,each with its own unique viscosity coefﬁcient.Although these equations are quite complex,they can be solved for a unique analytical solu-tion for a given geometry.When applied toﬁber spinning,the Leslie and Ericksen model can predict theﬂow-induced orientation of the aromatic planes in the as-spunﬁber.For example,the equations predict and explain the development of the radial texture observed for fully developedﬂow of discotic mesophase through a circular capillary(ie,a spinnerette hole).The balance of torques requires the mesophase toﬂow with a radial orientation as shown in Figure14.As a result of the ability to predictﬁber texture as a function of geometry, improved spinning geometries can be evaluated.McHugh and Edie(68)showed that a capillary with a tapered entry forms a more orderedﬁber as a result ofVol.9CARBON FIBERS103Fig.14.Origin of radial structure in mesophase pitchﬁbers.From Ref.23. creating less disruption to the fully organizedﬂow assumed by the Leslie and Ericksen model.Spinningﬁbers through a rectangular capillary generatesﬁber with a highly linear texture that results inﬁbers with higher thermal conductiv-ity(71).The line-origin texture of these“ribbonﬁbers”is shown in Figure15. Figure16shows the Leslie and Ericksen model solution toﬂow of discotic mesophaseﬂowing through a rectangular channel.The edges within the chan-nel represent the edges of the aromatic planes(68).Regardless of the shape and texture,the as-spunﬁbers must be stabilized prior to carbonization.During this process,oxygen reacts with side groups in the pitchﬁber,creating cross-linkages and adding weight.Typically,a6%weight gain is needed to completely stabilize mesophase pitchﬁbers(72),while an8%weight gain is more typical for PAN(73).Lower heating rates and lower temperaturesFig.15.The line-origin texture of ribbonﬁbers.104CARBON FIBERS Vol.9Fig.16.Orientation developed in discotic mesophaseﬂow through a rectangular channel. Adapted from Ref.68.during stabilization result in a more uniform stabilization,but also add to pro-cessing time(74).The carbonization of mesophase pitch-basedﬁbers closely resembles that which is previously described for PAN,but occurs in two stages.Typically,ﬁbers are heated to approximately1000◦C and held there allowing for the evolution of off-gases.The rate-limiting step in this low temperature carbonization is the free-radical breaking of carbon–hydrogen bonds.Following the release of gases, theﬁbers are heated to theirﬁnal treatment temperature,which may be as high as3000◦C.Unlike PAN-based carbonﬁbers,mesophase pitch-basedﬁbers experience signiﬁcant graphitization during which dislocations in the turbostratic carbon stacks are gradually annealed,resulting in the formation of a three-dimensional lattice.Fischbach(75)presented a detailed study of graphitization which charac-terizes the process as a combination of atomic diffusion and crystallite growth. Table4provides a comparison of properties for some commercial pitch-and PAN-based carbonﬁbers.Table4.Properties of Commercial Carbon Fibers aFiber type Precursor material Tensile strength,GPa Young’s modulus,GPa T-300PAN 3.66231T-650/35PAN 4.28241T-650/42Pitch 4.62290P-25Pitch 1.38159P-30X Pitch 2.76201P-55S Pitch 1.90379P-75S Pitch 2.07517P-100S Pitch 2.41759P-120S Pitch 2.41828K-1100Pitch 3.10931a Ref.76.Vol.9CARBON FIBERS105Fig.17.The repeat unit of Kevlar.Fig.18.The repeat unit of PBO.Carbon Fibers from High Performance PolymersThe primary factor limiting the increased commercial application of carbonﬁbers is their high production cost,a signiﬁcant portion of which is directly attributable to the lengthy stabilization process required by both pitch and PAN(77).The rigid-rod structure and high degree of aromaticity allow Kevlar(poly p-phenylene terephthalamide)and PBO(poly p-phenylene benzobisoxazole)ﬁbers to be con-verted directly to carbonﬁber without any stabilization(17–19).The repeat units of Kevlar and PBO are shown in Figures17and18(see P OLYAMIDES,A ROMATIC; R IGID-R OD P OLYMERS).Surprisingly,PBO-based carbonﬁbers also show some ca-pacity to graphitize(19).However,the commercial development of high perfor-mance polymer-based carbonﬁbers is impeded by their comparatively poor tensile strength.Table5compares the properties of PBO-and Kevlar-based carbonﬁbers with typical pitch-and PAN-basedﬁbers.In spite of the relatively poor tensile strengths of theﬁbers,carbonized Kevlar possesses characteristics that make it an ideal candidate as a substrate for chemical vapor deposition of silicon carbide including a compatible coefﬁcient of thermal expansion,sufﬁcient tensile strength to survive the coating process, availability from a reliable domestic supplier,and a smooth,even diameter of less than7µm(78).Table5.Properties of High Performance Polymer Based Carbon Fibers aTensile Young’s Electrical Precursor strength,modulus,Resistivity, material Product designation GPa GPaµ -m PBO Batch,1800◦C0.5224513 PBO Continuous,1800◦C0.8518310 Kevlar-291600◦C0.9414323 PAN T-300 3.6623118 Pitch P-55 1.904159a Refs.18,78.106CARBON FIBERS Vol.9 Rayon-Based Carbon FibersTheﬁrst carbonization of cellulose-basedﬁbers dates back to Thomas Edison,who carbonized a natural celluloseﬁlament for use as an incandescent lampﬁlament. In the mid-1950s,the Carbon Wool Corporation introduced theﬁrst commercial carbonized rayonﬁbers(79).PAN-and pitch-based carbonﬁbers have replaced rayon-basedﬁbers in most high performance applications;however,they continue toﬁnd use as ablative materials in missile nosecones and heat shielding(16).Ad-ditionally,the combination of low cost,ease of handling,and high natural porosity makes rayon an attractive precursor for activated carbonﬁbers(see C ELLULOSE F IBERS,R EGENERATED).Activated carbonﬁber(ACF)offers several advantages over traditional gran-ular and powdered carbons as an absorbent material.Rayon-based ACFs(80) possess(1)smallerﬁber diameters,which encourages rapid adsorption and desorptionby minimizing diffusion limitation;(2)more concentrated and controllable pore size distributions;and(3)excellent adsorption capacity at low adsorbate concentrations.Rayon-based ACFs are used in the adsorption of many volatile organic com-pounds including formaldehyde(80),methyl ethyl ketones(81),and benzene(81). ACFs are alsoﬁnding uses in natural gas storage(82),electrodes for batteries (83),catalyst supports(84),and NO x removal(85).Stabilized rayonﬁbers are carbonized and then activated with air(80),steam(86),or carbon dioxide(87), much as in granular carbon activation.The extent of pyrolysis governs the pore structure,carbon yield,and surface area of theﬁber,while activation impacts the presence of functional groups on the pore surface(12).Properties of some commercial ACFs are summarized in Table6.ACFs develop a pore structure with micropores(<60nm)open directly to the surface,unlike granular carbons where extensive surface oxidation results in macropores on the surface(88)with microporosity only in inner zone,as shown in Figure19.The presence of extensive surface microporosity has a signiﬁcant impact on the adsorption of volatile organic compounds.The overlap of attractive forces on opposite micropore walls are primarily responsible for the adsorption of gases at low concentrations(89).Carbonized rayonﬁbers present one drawback as an ACF precursor.Nearly 82%of the mass of the originalﬁber is evolved during pyrolysis,resulting in a Table6.Properties of Commercial Active Carbon Fibers aSurface Mean pore Benzene Tensile Fiber Precursor area,m2/g size,nm adsorption,wt%strength,MPa Toyobo KF Series Rayon1000–16000.5–1030–6060–130 Toho Finegard PAN500–12002–420–50196–490 Adol A Series Pitch1000–20001–2.520–4560–130a Ref.12.。

Anderson Localization of Polar Eigenmodes in Random Planar Composites

arXiv:physics/0602098v3 [physics.optics] 31 Oct 2006
Anderson Localization of Polar Eigenmodes in Random Planar Composites
Vadim A. Markel†
Departments of Radiology and Bioengineering, University of Pennsylvania, Philadelphia, PA 19104 Abstract. Anderson localization of classical waves in disordered media is a fundamental physical phenomenon that has attracted attention in the past three decades. More recently, localization of polar excitations in nanostructured metaldielectric ﬁlms (also known as random planar composite) has been subject of intense studies. Potential applications of planar composites include local near-ﬁeld microscopy and spectroscopy. A number of previous studies have relied on the quasistatic approximation and a direct analogy with localization of electrons in disordered solids. Here I consider the localization problem without the quasistatic approximation. I show that localization of polar excitations is characterized by algebraic rather than by exponential spatial conﬁnement. This result is also valid in two and three dimensions. I also show that the previously used localization criterion based on the gyration radius of eigenmodes is inconsistent with both exponential and algebraic localization. An alternative criterion based on the dipole participation number is proposed. Numerical demonstration of a localization-delocalization transition is given. Finally, it is shown that, contrary to the previous belief, localized modes can be eﬀectively coupled to running waves.

1、下载文档前请自行甄别文档内容的完整性，平台不提供额外的编辑、内容补充、找答案等附加服务。
2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
3、如文档侵犯您的权益，请联系客服反馈,我们会尽快为您处理(人工客服工作时间：9:00-18:30)。

a rXiv:h ep-ph/211190v113Nov22Recent status of polarized parton distributions M.Hirai (Asymmetry Analysis Collaboration )1Radiation Laboratory,RIKEN (The institute of Physics and Chemical Research),Wako,Saitama 351-0198,Japan Abstract.We study an inﬂuence of precise data on uncertainty of polarized parton distribution functions.This analysis includes the SLAC-E155proton target data which are precise measure-ments.Polarized PDF uncertainties are estimated by using the Hessian matrix.We examine cor-relation effect between the antiquark and gluon uncertainties.It suggests that reducing the gluon uncertainty is needed to determine the polarized antiquark distribution clearly.INTRODUCTION Polarized parton distribution functions (polarized PDF’s)have so far been optimized from polarized deep inelastic scattering (polarized DIS)world data [1,2].We could obtain only a slight piece of information about polarized antiquark and gluon distribu-tions.At this stage,the antiquark SU(3)f ﬂavor symmetry is assumed in most of the polarized PDF analyses.The SU(3)f symmetry breaking is already known as the Got-tfried sum rule violation in the unpolarized case.In principle,the polarized PDF analysis should take account of the symmetry breaking.However,we must not only determine a shape but also a sign of each polarized antiquark distribution.It needs more precise data to improve the current status.Semi-inclusive DIS experiments [3]are also expected to separate antiquark ﬂavor distributions.However,the separated distributions may not be credible due to ambiguity of the fragmentation functions.Then,antiquark ﬂavor dis-tributions cannot be decomposed clearly.The current knowledge of the polarized gluondistribution is still poor.The polarized gluon distribution is suggested as the positive distribution;however,there is large difference between various parameterization results.We would like to know ambiguity of polarized PDF’s quantitatively.PDF uncertainty plays an important role in illustrating the ambiguity.Furthermore,it is important to show the phenomenological uncertainty of predicted physical quantities (e.g.,scattering cross-sections and spin asymmetries)with parameterized PDF’s and their uncertainties in our work.A purpose of this analysis is to clarify the current knowledge about the polarized PDF’s from the polarized DIS world data by using their PDF uncertainty.In this analysis,the polarized PDF’s are optimized including precise SLAC-E155proton target data [4].Then,we examine an inﬂuence of the precise data on the polarized PDF uncertainty,which is estimated by the Hessian method.PARAMETERIZATION OF THE POLARIZED PDF’SThe polarized PDF’s are determined by using spin asymmetry A1of the polarized DIS experiments from the EMC,SMC,SLAC-E130,E142,E143,E154,E155,and HERMES:A1(x,Q2)=2x[1+R(x,Q2)]2n f∑i=1e2i ∆C q(x,αs)⊗[∆q i(x,Q2)+∆¯q i(x,Q2)]+∆C g(x,αs)⊗∆g(x,Q2) ,(2)where e i is the electric charge of quarks,and∆C q,∆C g are Wilson’s coefﬁcient functions. The convolution⊗is deﬁned by f(x)⊗g(x)= 1x dy/y f(x/y)g(y).The polarized PDF’s ∆f(≡f↑−f↓)are deﬁned as helicity distributions in the nucleon.In the AAC analysis, the polarized PDF∆f(x)is deﬁned at initial Q2by the weight function form:∆f(x)=Axα(1+λxγ)f(x),(3)where f(x)is the unpolarized PDF,and A,α,λ,andγare free parameters.Optimized PDF’s are four distributions;∆u v(x),∆d v(x),∆¯q(x),and∆g(x),and these are evolved from the initial Q2(=1GeV2)to the same Q2of experimental data by the DGLAP equation[7].In particular,the gluon distribution∆g(x)contributes to the structure function with the non-zero coefﬁcient function∆C g in the NLO case.This analysis uses two constraint conditions.First,the positivity condition is used to restrict large-x behavior of the polarized PDF’s.This condition corresponds to the probabilistic interpretation of the parton distributions in the LO:|∆f(x)|≤f(x).It needs not to be satisﬁed strictly in the NLO analysis.However,the polarized antiquark and gluon distributions tend to badly break the positivity limit:|∆f(x)|≫f(x).Such excessive behavior is due to the large experimental errors in the large-x region.Hence, this behavior should be limited by this condition.Next,the SU(3)fﬂavor symmetry is assumed:∆¯u(x)=∆¯d(x)=∆¯s(x)=∆s(x).Using this assumption,one canﬁx theﬁrst moments of the valence quarks with hyperon decay constants,then∆u v=0.926and∆d v=−0.341are obtained.Note that the Bjorken sum rule is satisﬁed automatically byﬁxingﬁrst moments.Furthermore,the spin content∆Σis obtained by∆ΣNf =3=∆u v+∆d v+6∆¯q.Since,the antiquark contribution isemphasized,then the spin content determination is susceptible to the antiquark behavior. In the analysis,we choose the modiﬁed minimal subtraction(UNCERTAINTY ESTIMATIONFortunately PDF uncertainty estimation method has been developed in the last several years(see a brief review[6]).The polarized PDF uncertainty comes from several error sources,e.g.,experimental errors,unpolarized PDF,ΛQCD,and so on.However,it is difﬁcult to incorporate these errors into uncertainty estimation simultaneously.In the present analysis,the polarized PDF uncertainty is estimated from experimental errors by using the Hessian matrix H i j which is deﬁned as a second order derivative matrix in the expandedχ2(a i)function around its minimum point.The PDF uncertaintyδ∆f(x) can be obtained easily by the inverse matrix of the Hessian and linear error propagation:[δ∆f(x)]2=∆χ2∑i,j ∂∆f(x)∂a j,(4)where∆χ2(=χ2(a i)−χ2min)is deﬁned as the difference from the minimumχ2.It deter-mines a conﬁdence level of the PDF uncertainty,and it depends on theχ2distributionK(s)with N degrees of freedom.Here,N is the number of optimized parameters.In our estimation,the value of∆χ2is obtained by the following equation: ∆χ20K(s)ds=σ, whereσ(=0.683)corresponds to1σerror of a standard distribution in order to com-pare with general experimental errors.The statistical and systematic errors are added in quadrature,so that it could be overestimation.The proper estimation exists between the overestimated uncertainty and the uncertainty from only the statistical error.RESULTS AND DISCUSSIONSThe bestﬁtting result isχ2(/d.o.f.)=346.33(0.90).Theﬁrst moments of new results and the AAC pervious results(AAC00,NLO set2)[1]are shown in Table.1.A correla-tion coefﬁcientρ¯qg between theﬁrst moment of the antiquark and gluon distributions is ρ¯qg=−0.836,and there is strong correlation between two distributions.The uncertain-ties of the new results become smaller than those of the previous results.The gluonﬁrst moment and spin content∆Σstill have large uncertainty.Theﬁxedﬁrst moments∆u v and ∆d v do not have uncertainty,then the∆Σuncertainty is six times as large as the antiquark uncertainty.Thus,the spin content is subject to the uncertainty of the antiquark distri-bution.Figure1shows the uncertainty of the new antiquark distribution.The antiquark uncertainty becomes rather large in the region x<0.01,however the experimental datascarcely exist.The polarized DIS spin asymmetries A p,d1(x)approaches rapidly to zeroin the rang x<0.004.It is insufﬁcient to clarify small-x behavior of the antiquark dis-tribution.Therefore,the antiquark determination has extrapolating ambiguity in small-x region.It is needed tight constraint condition or other experiment.In addition,Figure1shows comparison between the PDF uncertainties of new results and the previous results.There are no signiﬁcant improvements of the valence quark uncertainties.On the SU(3)f symmetry assumption,theﬁxingﬁrst moments strongly restricts the behavior of valence quark distributions.In contrast,the antiquark and gluon uncertainties are reduced in the range0.01<x<0.5,where the E155proton data exist.TABLE 1.First moments of the polarized antiquark,gluon,andspin content ∆Σwith their uncertainties at Q 2=1GeV 2.∆¯q ∆g ∆Σ−0.062±0.023,0.499±1.268,0.213±0.138AAC00The precise polarized DIS data can reduce the antiquark uncertainty mainly.On the other hand,the gluon uncertainty changes in response to antiquark uncertainty reduction due to a strong correlation between two distributions.Since the gluon contribution to the structure function g 1(x )is smaller than the quark and antiquark contributions,we can extract only a little information of the gluon distribution in spite of the NLO analysis.Actually,the gluon uncertainty is still large.It indicates the difﬁculty of determining the gluon distributions from the polarized DIS data.Therefore,the uncertainty reduction of the gluon distribution is due to the strong correlation rather than the NLO contribution.In order to examine the correlation effect on the parameterization,we re-analyzed the ∆g (x )=0case in which the ﬁxed gluon distribution does not have uncertainty.The polarized PDFuncertainties of the ∆g (x )=0case are compared to those of the ∆g (x )=0case in Figure 2.The gluon distribution slightly exists at high-Q 2due to Q 2evolution of the singlet type DGLAP equation.The valence quark uncertainties scarcely change.Drastic improvement of the antiquark uncertainty is due to vanished the large gluon uncertainty.the obscure gluon distribution brings about the larger antiquark uncertainty by the complementary relation.x x xx FIGURE 1.Polarized PDF’s with their uncertainties at Q 2=1GeV 2.Dashed curves are the uncertain-ties of previous results (AAC NLO-2)-0.3-0.2-0.1x Preliminary !-1.5-1-0.500.511.520.00010.0010.010.11x200 GeV 2∆g(x )=0 at 1 GeV 2FIGURE 2.Polarized antiquark and gluon distributions with their uncertainties at Q 2=1GeV 2.The shaded portion shows the uncertainty of ∆g (x )=0results,and the dashed curves are the uncertainties of new results (∆g (x )=0).SUMMARYBy this analysis,the polarized PDF’s were optimized from the polarized DIS world data which included the SLAC-E155proton target data.The polarized PDF uncertain-ties were estimated by the Hessian method.The E155precise measurements scarcely improve the valence quark uncertainties,but they can reduce the antiquark and gluon uncertainties.These,however,are still wrapped in large uncertainty.The SU(3)f sym-metry,which we are obliged to assume,restricts strongly the valence quark behavior by ﬁxing ﬁrst moments,and the spin content determination depends on the antiquark behavior.Additionally,there is the strong correlation between the antiquark and gluon distributions.If the gluon distribution is clariﬁed by RHIC-Spin at BNL,the uncertainty of the antiquark distribution can be reduced to some extent.Similarly,the complemen-tary relation can reduce the large uncertainty of the spin content which comes from the extrapolating issue of the antiquark behavior.Then,we will be able to investigate the antiquark ﬂavor dependence in detail.REFERENCES1.AAC,Y .Goto et al .,Phys.Rev.D62(2000)034017.2.De Florian and R.Sassot,Phys.Rev.D62(2000)094025;M.Glück,E.Reya,M.Stratmann,and W.V ogelsang,Phsy.Rev.D63(2001)09400;E.Leader,A.V .Sidorov,and D.B.Stamenov,Eur.Phys.J.C23(2002)479-485;J.Blümlein and H.Böttcher,Nucl.Phys.B B636(2002)225-263.Fortran pro-gram librarys of polarized PDF’s are available from /hepdata/pdf.html.3.SMC,B.Adeva et al .,Phys.Lett.B420,180(1998);HERMES,K.Ackerstaff et al .,Phys.Lett.B464123(1999).4.SLAC-E155,P.L.Anthony et al .,Phys.Lett.B493(2000)19.5.L.W.Whitlow,S.Rock,A.Bodek,S.Dasu and E.M.Riordan,Phys.Lett.B250,193(1990);SLAC-E143,K.Abe et al .,Nucl.Phys B452(1999)1946.M.Botje ,J.Phys.G 28(2002)779-790.7.V .N.Gribov and L.N.Lipatov,Sov.J.Nucl.Phys.15(1972)438and 675;G.Altarelli and G.Parisi,Nucl.Phys.B 126(1977)298;Yu.L.Dokshitzer,Sov.Phys.JETP 46(1977)641.8.M.Glück,E.Reya,and A.V ogt,Eur.Phys.J.C5(1998)461.。