TWI-JI-SCANJ-asy

合集下载

TWI工作教导方法

習 7. 有沒有將安全裝置、裝具交給學習的人，並且
準
加以說明，給予指示?
備 8. 是否將其工作與全體的關係加以說明?
9. 是否使學習的人進入正確的位置?
Paul Su
○ 記入欄
30
鄭記企管九、工作教導方法的自我反省表
沒有反省，就不會有進步
階段
項
目
○ 記入欄
第 1. 有沒有依照工作分解來教導?
NO.
工作物: 電話器
工具與材料: 號碼表、備忘錄用紙、鉛筆
主要步驟
要點
1.確認對方 2.拿起聽筒
電話號碼、所屬、姓名
用左手，備忘錄用紙置於右手邊
3.轉圓形數字盤
轉到底
4.說自己公司名
5.說出通話內容
確認對方，發言要清楚活用5WIH
6.放回聽筒
打個招呼，經過3秒
Paul Su
14
鄭記企管 6-5 工作分解表的好處
(2) 強調要點 (3) 清楚地、完整地、耐心地教導。 (4) 不要超過他的理解能力。
Paul Su
24
鄭記企管七、工作教導方法４階段
第３階段試作
(1) 讓他試作
改正錯誤。
(2) 讓他一面試作，一面說出主要步驟。
(3) 再讓他做一遍，同時說出要點。
(4) 教到確實了解為止。
Paul Su
25
Paul Su
○ 記入欄
29
鄭記企管九、工作教導方法的自我反省表
沒有反省，就不會有進步
階段
項
目
第 1. 是否造成輕鬆不拘束的氣氛?
1 2. 是否將學習的人介紹給附近的作業員?
階 3. 是否告知作業的名稱?

SMC EX260-SEC1 -SEC2 -SEC3 -SEC4 商品说明书

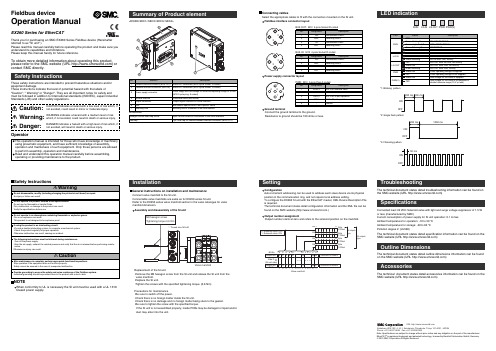
<EX260-SEC1/-SEC2/-SEC3/-SEC4>InstallationConfigurationAuto-increment addressing can be used to address each slave device via its physical position in the communication ring, and not require local address setting.To configure the EX260 SI unit with the EtherCAT master, XML Device Description File is required.The technical document states detail configuration information and the XML file can be found on the SMC website (.)Output number assignmentOutput number starts at zero and refers to the solenoid position on the manifold.General instructions on installation and maintenanceConnect valve manifold to the SI unit.Connectable valve manifolds are same as for EX250 series SI unit.Refer to the EX250 series valve manifold section in the valve catalogue for valvemanifold dimension.Power supply connector layoutGround terminalConnect the ground terminal to the ground.Resistance to ground should be 100 ohms or less.SettingReplacement of the SI unit•Remove the M3 hexagon screw from the SI unit and release the SI unit from the valve manifold.•Replace the SI unit.•Tighten the screws with the specified tightening torque. (0.6 Nm)Precautions for maintenance •Be sure to switch off the power.•Check there is no foreign matter inside the SI unit.•Check there is no damage and no foreign matter being stuck to the gasket.•Be sure to tighten the screw with the specified torque.If the SI unit is not assembled properly, inside PCBs may be damaged or liquid and/or dust may enter into the unit.Connecting cablesSelect the appropriate cables to fit with the connectors mounted on the SI unit.Fieldbus interface connector layoutTroubleshootingThe technical document states detail troubleshooting information can be found on the SMC website (URL )SpecificationsConnected load: 24VDC Solenoid valve with light and surge voltage suppressor of 1.5 W or less (manufactured by SMC)Current consumption of power supply for SI unit operation: 0.1 A max.Ambient temperature for operation: -10 to 50 ℃Ambient temperature for storage: -20 to 60 ℃Pollution degree 2: (UL508)The technical document states detail specification information can be found on the SMC website (URL )Akihabara UDX 15F, 4-14-1, Sotokanda, Chiyoda-ku, Tokyo 101-0021, JAPAN Phone: +81 3-5207-8249 Fax: +81 3-5298-5362URL Outline DimensionsThe technical document states detail outline dimensions information can be foundon the SMC website (URL )AccessoriesThe technical document states detail accessories information can be found on the SMC website (URL )*2: Single flash pattern*1: Blinking patternAssembly and disassembly of the SI unit*3: Flickering patternNOTEWhen conformity to UL is necessary the SI unit must be used with a UL 1310Class2 power supply.Fieldbus deviceOperation ManualEX260 Series for EtherCATThank you for purchasing an SMC EX260 Series Fieldbus device (Hereinafter referred to as "SI unit" ).Please read this manual carefully before operating the product and make sure you understand its capabilities and limitations.Please keep this manual handy for future reference.To obtain more detailed information about operating this product,please refer to the SMC website (URL ) or contact SMC directly.These safety instructions are intended to prevent hazardous situations and/or equipment damage.These instructions indicate the level of potential hazard with the labels of"Caution", " Warning" or "Danger". They are all important notes for safety and must be followed in addition to International standards (ISO/IEC), Japan Industrial Standards (JIS) and other safety regulations.OperatorNote: Specifications are subject to change without prior notice and any obligation on the part of the manufacturer.EtherCAT ®is registered trademark and patented technology, licensed by Beckhoff Automation GmbH, Germany.© 2010 SMC Corporation All Rights ReservedPWR: M12 5-pole Plug A-coded。

新版明亮黄色欧美公司企业介绍

TITLE HERE
TITLE HERE
TITLE HERE
Die Welt ist meine Vorstellung.
Die Welt ist meine Vorstellung.
ABOUT OUR ONLINE USERS
TITLE HERE
TITLE HERE
Die Welt ist meine Vorstellung.
Lorem ipsum dolor sit amet.
BUSINESS
INFOGRAPHIC
02
04
Lorem ipsum dolor sit amet
Lorem ipsum dolor sit amet.
Lorem ipsum dolor sit amet.
03
02
TITLE HERE
Lorem ipsum dolor sit amet, consectetur adipiscing elit.
03
TITLE HERE
Lorem ipsum dolor sit amet, consectetur adipiscing elit.
ABOUT OUR APPS
mangat pakek teurasisi tgkUt vestibuluat pakek teurasisi manuk le nyang beungek
Lorem ipsum dolor sit amet, consectetur adipiscing elit.
Lorem ipsum dolor sit amet, consectetur adipiscing elit.
Lorem ipsum dolor sit amet, consectetur adipiscing elit.

关于蜜蜂采蜜的100字英语作文五年级

关于蜜蜂采蜜的100字英语作文五年级全文共6篇示例，供读者参考篇1Bees and Their Sweet SecretsHave you ever looked closely at a bee? I mean really closely? They are such amazing little creatures! Their bodies are covered in fuzzy yellow and black stripes. They have big eyes that seem to look every which way at once. And of course, they have those neat little pouches on their legs to carry pollen and nectar.Bees are so interesting and important. Without them buzzing around collecting pollen and nectar from flowers, many plants wouldn't be able to make the fruits and seeds they need to grow the next year. Bees are like tiny airborne delivery workers, taking pollen from one plant to another so they can reproduce.But today I want to tell you all about how bees make one of the sweetest, most delicious foods on the planet - honey! It's honestly like liquid gold and bees are the master honey makers.It all starts when a worker bee lands on a flower. She uses her long, strawlike tongue to sip up the sugary nectar from deepinside the blossom. The nectar gets stored in a special honey stomach inside her little bee body. Once her stomach is full, she'll fly back to the beehive.At the hive, she passes the nectar to another bee. This bee takes the nectar and deposits it into one of the beeswax honeycombs that make up the hive. The nectar is pretty watery at first, so the bees have to work hard to thicken it up.They flap their wings really fast, creating a stream of air over the nectar. This evaporates a lot of the water from the nectar, making it thicker and stickier. As it thickens, the nectar starts turning into real honey! The bees also add some enzymes from their bodies that further break down the sugars and transform the nectar into honey.Once the honeycombs are nice and full of rich, gooey honey, the bees get to work capping each one with a thin layer of beeswax. This airtight seal protects the honey and allows it to "ripen" and achieve its final sweet, syrupy deliciousness.The whole process - from flower to sealed honeycomb - takes just a few weeks in the busy summer months. Pretty amazing for such tiny workers, right? Just one beehive can produce 60 pounds (27 kg) of honey each year! No wonder bees are cherished by beekeepers.While some of the honey gets raided by beekeepers (don't worry, they always leave plenty for the bees), most of it stays with the hive. The bees munch on honey all winter long to give them energy to keep warm and survive until spring blooms once again.When you think about all the work bees put into making honey - visiting thousands of flowers, regurgitating nectar, fanning it with their wings, capping the combs - you have a newfound appreciation for every golden spoonful! It's no wonder honey has been prized as a delicacy and medicine by cultures all around the world for thousands of years.Bees are simply amazing little creatures. Their dedication to collecting nectar, pollinating plants, and making store after store of life-sustaining honey is incredibly important. Just one colony can pollinate 300 million flowers each day! Imagine if bees didn't do their critical jobs - we wouldn't have many of the foods we love like apples, strawberries, cucumbers and almonds.So next time you tear open a packet of honey to drizzle over your yogurt or spread on a slice of fresh bread, remember to thank a bee. Their hard work and incredible honey-making skills allow us to enjoy one of nature's sweetest treats. Bees are the real busy workers behind that delicious taste of honey!篇2Buzzing Busy Bees and Their Sweet HoneyDid you know that bees are some of the hardest workers in nature? They fly from flower to flower, collecting a sweet liquid called nectar to make one of my favorite foods - honey! Let me tell you all about the amazing process of how bees make honey.Bees live together in a little house called a hive. The hive is made up of many bees - the queen bee, the worker bees, and some other bees. The queen is the most important one as she lays all the eggs. The worker bees are the ones that fly around collecting nectar and doing other jobs.When a worker bee finds an open flower full of nectar, it lands on the flower and uses its long, tubelike tongue to suck up the sugary liquid. It stores the nectar in a special honey stomach inside its body. Once that stomach is full, the bee flies back to the hive.At the hive, the bee regurgitates or throws up the nectar into a little wax room called a honeycomb. As more and more bees add their nectar, the honeycomb gets filled up. But it's not quite honey yet!You see, the nectar has too much water in it when the bees first put it in the honeycomb. So the bees have to dry it out to make real honey. They flutter their wings really fast, fanning the nectar and making the water evaporate from it. As the water goes away, the nectar starts getting thicker and stickier until finally it turns into that sweet, gooey honey we all love!Isn't that amazing? Those busy little bees worked so hard, visiting hundreds of flowers and doing a special honey dance to tell the other bees where the good flowers were. And their reward is making that delicious honey that tastes so yummy on toast or pancakes.Bees are just incredible little creatures. I'm glad my mom told me all about how they make honey, because now I have a whole new appreciation for the sticky sweet stuff. Whenever I see a bee buzzing around outside, I think about all the work it's doing to gather that nectar and make more honey for us. Thanks, bees!I hope you learned something new about how bees make honey. It's a really fascinating process that I didn't know much about until recently. Those bees sure are hard workers, having to fly from flower to flower, fill up their stomach sacs, go back tothe hive, and dry out the nectar into honey. No wonder it tastes so wonderfully sweet - it's the product of all their labor!The next time you put some honey on your food, I want you to think about the journey it went through to get there. Maybe you'll have a new appreciation for that little jar of golden sweetness, just like I do now. Who knew such small insects could create something so amazingly delicious through their dedication and hard work? Bees are pretty cool!篇3Bees are Amazing Honey Makers!Have you ever watched bees flying from flower to flower? It's so cool to see them hard at work gathering nectar and pollen. Bees are like tiny magic workers that turn flower nectar into one of the most delicious treats – honey!Inside the BeehiveA beehive is a very busy place, kind of like a mini city for bees. There are thousands of bees all working together. At the center is the queen bee. She is the largest bee and her job is to lay eggs to keep making more bees.The other bees have different jobs too. Some are worker bees who go out and collect nectar and pollen from flowers. When they find flowers, they use their long, tube-like tongues to suck up the nectar inside. They store this nectar in a special honey stomach inside their bodies.While out collecting, the bees also gather pollen by rubbing against the flowers' stamens. The pollen sticks to fuzzy hairs on their legs and bodies. This pollen will be used later as food for baby bees.Back at the hive, the worker bees pass the nectarmouth-to-mouth to other worker bees. As they pass it along, the nectar mixes with enzymes from their bodies which starts turning it into honey. The bees then deposit the nectar into the honeycomb cells and fan it with their wings to make it thicker.When the honey is just right, they cap the cell with a thin layer of beeswax. This sealed, perfect honey is saved to feed all the bees through the winter when no flowers are blooming.The Communication DanceBees have a very interesting way to tell the other bees where the best flowers are located. It's called the "waggle dance!" When a worker bee returns from collecting a great bunch offlowers, it does a little dance while shaking its body. The way it moves tells the others where to fly to find that flower patch.The other amazing bees in the hive are called "soldier bees." Their job is to guard and protect the hive from anything that might try to get the honey, like wasps or bears. They are ready to sting any intruders to keep their home safe.Making that Golden SweetnessWhile bees need to make and store lots of honey to get through winter, we humans are so lucky that they make extra for us to enjoy too! Beekeepers have special boxes called "supers" connected to the hive. The bees are allowed access and they fill these supers with honeycomb too.The beekeeper can then remove these frames of honey-filled comb, extracting all the golden, syrupy honey for us. Mmm..just thinking about bees hard at work makes honey taste even sweeter!Helping the BeesSadly, bees are having a tough time recently. Things like pesticides, diseases, and fewer wildflowers have caused many bee colonies to die off. We need to help protect these amazing pollinators.One way is to plant plenty of bright flowers, especially local wildflowers. Having more blooms gives bees more food sources. You can also put out a little dish of fresh water for thirsty bees. Another idea is to ask your parents about getting a backyard beehive! How cool would it be to have fresh honey right from your own yard?Bees are such hardworking buddies to have around. They help plants grow by pollinating. And they make that gooey, sweet treat we all love. Next time you taste honey, remember all the work the bees did to create that perfect spoonful of nature's candy! Three cheers for bees - hip hip hooray!篇4Bzzzzzz...The Wonder of Bees and HoneyHi there! My name is Sam and I'm a 5th grader who is totally fascinated by bees. I know a lot of kids get scared of bees because they can sting, but I think bees are amazing little creatures. Today, I'm going to tell you all about how bees make that delicious golden treat we call honey.First, we need to understand the different types of bees in a colony. There are thousands of bees living together, but they each have an important job. The queen bee is the only femalethat lays eggs. She's bigger than the other bees and can lay up to 2,000 eggs per day! The drone bees are the male bees and their main job is to mate with the queen.But the real stars of the show are the worker bees. These are all female bees that never get to have babies. Instead, they have vitally important roles like cleaning the hive, circulating air by beating their wings, gurading the entrance, and most crucially - collecting nectar to make honey!So how exactly do those hard-working bees go about making that sticky, sweet treat we love so much? It's a pretty incredible process when you break it down.It all starts when a worker bee visits a flower and uses its long, tubelike tongue to suck up the sugary nectar from the blossom. The bee stores this liquid gold in its extra stomach chamber called the honey stomach or crop. This self-storing nectar sac lets the bee collect much more nectar than it could hold in its regular digestive system.Once its crop is full, the worker bee heads back to the beehive to pass off its bounty. It regurgitates the nectar into the mouth of another worker bee back at the hive. This worker chews the liquid for awhile, breaking down the complex sugars intosimpler ones that are digested more easily by bees. This starts the process of turning the nectar into honey.The semi-digested nectar is then deposited into a honeycomb cell. To finish the process of turning nectar into honey, the bees need to reduce the moisture content. So dozens of workers endlessly fan the nectar-filled cells with their wings. This air circulation causes evaporation and thickens the liquid into that characteristic sticky honey texture we all know and love.When the honey is just right, the bees cap the cell with a thin layer of beeswax. This seals the honey in an air-tight environment to prevent any more moisture from getting in or out. And just like that - honey! The bees will repeat this process over and over, creating enough surplus honey to sustain the colony over winter when no flowers are blooming.The frames of honey are then taken to a warm room where a machine spins them around rapidly. The centrifugal force causes the honey to fly out of the cells and the empty honeycombs can go back to the bees so they don't have to make new ones from scratch. How cool is that?After being extracted from the frames, the honey goes through filters to remove any remaining wax particles or other debris. Finally, the pure liquid gold is bottled up for us topurchase at stores and farmer's markets. Mmmmm....nothing beats eating a spoonful of that sweet, all-natural treat straight from the bottle! Or putting it on toast, mixing it into tea, baking with it...the uses are endless.So there you have it - the amazing journey of how those busy little bees transform sugar-rich flower nectar into one of the world's most prized food items. I don't know about you, but I will never look at a jar of honey the same way again! All that hard work by篇5Bees and Their Amazing Honey HarvestingDid you know that bees are some of the most incredible little workers in nature? These fuzzy, flying insects are responsible for making one of the sweetest treats we enjoy - honey! I find it so fascinating to learn about how bees collect nectar from flowers and turn it into that sticky, golden syrup we love.A bee's life is busier than you can imagine. They are constantly on the move, flying from bloom to bloom in search of their favorite sugary liquids found inside flower blossoms. Using their long, tube-like tongues called proboscises, bees slurp up the nectar drops. But that's just the start of their amazing job!Once a bee has filled up her honey stomach with nectar, she zips back to the hive where the real magic happens. She passes the nectar onto other worker bees who chew it up and mix it with enzymes from glands in their heads. This breaks down the complex sugars in the nectar into simple sugars that make up honey.The bees then spread this honey mixture across the honeycomb cells and fan it with their wings to help evaporate the water from the nectar. As the water evaporates, the honey becomes thicker and stickier. Once it's just the right consistency, the bees cap the honeycomb cell with a thin layer of beeswax to seal it up tight. Now the tasty honey is ready for us to enjoy!s have been harvesting honey from bee hives for thousands of years. I think it's so neat that we get to take part in the bees' hard work and sample their sweet creation. Of course, beekeepers have to be very careful when collecting the honeycombs from the hive so they don't risk getting stung by the bees guarding their home.Once the honeycombs are gathered, the beeswax caps are sliced off and the honeycombs are placed in a machine called an extractor that spins them around rapidly. The force makes the honey drain out of the combs so it can be collected and bottledup. The empty honeycombs are usually returned to the hive so the industrious bees can refill them with more honey.Every batch of honey has a unique flavor that comes from the various nectars collected by the bees. Honeys can taste flavors like clover, orange blossom, buckwheat, and so many more depending on the flowers visited by those hard workers. My favorite is wildflower honey because it has a wonderfully rich, complex taste from all the different blooms the bees pollinated.I'm always blown away by how such tiny insects can work so tirelessly to make that sweet, sticky treat we all know and love. Just imagining the tens of thousands of flowers a honeybee must visit to fill her stomach, then repeat that same process over and over to fill an entire hive, makes me tired just thinking about it! But bees never stop because that's just their industrious nature.The next time you drizzle some honey over a piece of hot buttered toast or use it to sweeten your tea, I hope you'll pause and think about the amazing journey that amber liquid traveled to arrive at your plate. From flower to bee to beekeeper to you, that sticky treat is the ultimate example of sweet teamwork between humans and nature's hardest workers. Give it a taste and you'll surely agree - the bees' labor is worth savoring in every golden drop!篇6Bees and Their Honey HarvestDid you know that those little buzzing bees are some of the hardest workers in nature? They fly from flower to flower gathering sweet nectar to make a really special treat – honey! Let me tell you all about the amazing process of how bees collect nectar and turn it into that sticky, golden goodness we love.First, the lady bees called worker bees leave their hive in search of flowers loaded with nectar. Nectar is a sugary liquid inside the blossoms that attracts bees with its sweet smell. Using their long, tubular tongues, the bees slurp up the nectar drop by drop and store it in their extra honey stomach called a crop or honey stomach. This is separate from their regular digestion stomach where they store their own food. Pretty cool, right?Once her crop is full, the worker bee heads back to the hive. There, she passes the nectar onto another bee whose job is to handle and process the nectar into honey. This bee opens her mouth and lets the nectar drop onto her tongue. She moves her tongue around which adds enzymes from glands in her head to the nectar. These enzymes help break down the complex sugars in the nectar into simpler sugars that make up honey.The nectar-enzyme mix now gets deposited into the honeycomb cells in the hive. Bees use their wings to fan the liquid nectar, helping evaporate and thicken it. As more and more water evaporates from the nectar, it becomes stickier and thicker until it finally turns into honey! Once the cells are capped with a thin layer of beeswax, the honey is ready for eating.And the bees definitely eat a lot of their homemade honey to keep their energy up for foraging and making more honey. The excess honey is left in the hive over winter when no flowers are blooming. This stored honey feed allows the colony to survive until spring flowers bloom again.We humans are so lucky that bees make way more honey than they can eat themselves. Beekeepers are able to remove the excess combs full of honey from the hives, extracting and bottling the liquid gold for us to enjoy! Just imagine life without that sweet honey bear on your breakfast table. I can't!Not only is honey a delicious natural sweetener, but it's also thought to have antibacterial and anti-inflammatory properties that may help with coughs, sore throats and other ailments. Honey has been used for centuries as both food and medicine. Ancient Olympic athletes even ate honey as an energy booster before competitions!So the next time you have a spoonful of that sticky sweetness, remember the amazing journey it took getting from flower to hive to your kitchen table. Bees are true heroes of pollination and honey production. We definitely need to appreciate and protect these hard workers! Let's all do our part by planting bee-friendly flowers and avoiding pesticides that can harm bee colonies.I don't know about you, but I'm feeling really thankful for bees and their tasty honey. Pass the honeycomb, please! I'll take mine drizzled over a stack of fresh waffles. Mmmmm...doesn't get much better than that!。

TWI-JI培训课件

与其他人力资源管理方法的关系
TWI-JI与其他人力资源管理方法（如绩效管理、员工培训等）可以相互补充，共同提高组织的管理水平和员工的工作表现。
如何产生twi-ji
确定目标和期望
首先需要明确员工需要掌握的技能和知识，以及期望员工达到的工作表现。
设计学习计划
制定互动计划
实施指导
根据员工的特点和需要，设计相应的学习计划，包括学习目标、学习内容、学习方式、时间安排等。
借鉴经验
通过学习成功案例，可以总结出一些共性和成功的关键因素，为自己实施Towi-ji提供参考。
吸取教训
通过分析失败案例，可以总结出一些问题和教训，提醒自己在实施Towi-ji时需要注意和避免。
个性化定制
在借鉴他人经验教训的基础上，结合自己的实际情况和需求，制定个性化的Towi-ji实施方案。
02
twi-ji之基础原理
基础原理介绍
1
TW-I/J是日本安全工程协会（JSA）开发的安全管理方法，用于事故、灾害、风险的预防和应对。
2
TW-I/J基于PDCA循环，通过计划、实施、检查和改进四个阶段，实现安全管理的持续改进。
3
TW-I/J关注员工的安全意识、安全技能和安全行为的培训，以提高企业的安全管理水平。
针对不同应用场景的twi-ji实施方法
制造业
在制造业中，TWI-JI可用于操作员技能培训、设备维护和管理层领导力培训等方面。实施方法包括现场演示、角色扮演、小组讨论等。
服务业
在服务业中，TWI-JI可应用于服务人员技能培训、服务流程优化等方面。实施方法包括实地考察、案例分析、小组讨论等。
建筑业
twi-ji培训课件

TWI-JS(工作安全)ppt

Delta Confidential
事故防止之必要
---經濟上---
➢對工作場所或同事之影響
•影響生產
•增加成本
•影響工作情緒
•效率降低
•進度delay
• 其他
Delta Confidential
事故防止之必要
---公共上---
➢對社會之影響 •對公司形象破壞 •損壞公共設施 •阻礙經濟發展 •失業率增加 •環境污染 •其他
Delta Confidential
事故必有因, 應切斷連鎖
間接原因
直接原因 --不安全行動.狀態--
事故
➢慎思決定對策 ➢確實實施對策 ➢常常檢查結果
災
害
(人的方面&物
的方面)
Delta Confidential
安全第一, 預防為主.
佔事故總件數的
1
重傷
0.30%
希望籍此課程能給大家的工作帶來幫助. TWI 課程包含( JI 工作教導, JM 工作改善;JR 工
作關系和 JS 工作安全.)
Delta Confidential
何謂督導人員? >透過部屬完成工作的人.
• 督導人員必備之五條件
知
領導工作
技
教導
識
職責改善
能
Delta Confidential
在這工作場所發生了事故,運搬員的王先生受了裂傷與打撲傷,須一週的醫治.這時的情況說明如下,從約2周前拉門(1) 的情況已不太好,使用這拉門的清掃員黃先生將這件事報各陳領班,但並沒有採取任何措施,王先生受傷的當天,上司告知陳領班,廠長將會陪同客人來現場巡視.
陳領班當早上的工作告一段落,大約 10 點半時,到工作場所來巡視,這時發覺有垃圾堆,於是就吩咐黃先生(廠長也許現在馬上就會來,請你立刻清理垃圾).清掃員黃先生因為陳領班正在看著,所以顯得很緊張急欲求表現,想一次就把垃圾清理好,開始在手推車上堆積得像山一樣高.

Survey of Theory and Steering Laws of Single-Gimbal

Survey of Theory and Steering Laws of Single-GimbalControl Moment GyrosHaruhisa KurokawaNational Institute of Advanced Industrial Science and Technology,Tsukuba,Ibaraki 305-8564JapanDOI:10.2514/1.27316A geometric theory regarding singularity problems of single gimbal control moment gyrosystems is outlined.Most control moment gyrosystems have impassable and inescapable singular states that obstruct continuous changes of the total angular momentum vector.The remaining problem of the theory regarding degenerate singularity is described with an unproved conjecture.Based on the theory,various steering laws such as gradient methods and singularity robust methods for the pyramid-type control moment gyrosystem,and those for variable speed control moment gyros are surveyed and analyzed.Problems of the attitude control by these steering laws are examined by geometrical analyses without numerical simulation.NomenclatureC =Jacobian matrixc i =torque direction vector of the i th CMG unit ^c =unit eigenvector in H spaceg i =gimbal direction vector of the i th CMG unit H =total angular momentum vector of the system h i =normalized angular momentum vector of the i th CMG unitn =number of CMG units in the system p i =1=q iQ =quadratic form at a singular state q i =u h iT =output torque vector of the system t =timeu =singular vector,unit normal to all c i at a singular state"i =sign at a singular state=Gaussian curvature of a singular surface j =singular value of the matrix C =( 1;...; n ),a CMG statei =gimbal angle of the i th CMG uniti =orthonormal basis in tangent space of space !=gimbal rate vector obtained by a steering lawSuperscriptsN =null vectorS =vector at a singular state T =torque producing vectort =transpose of vector and matrixI.IntroductionASINGLE-GIMBAL control moment gyro (CMG)system is regarded as an effective torque generator for attitude control both for large space stations and small agile satellites because of its large torque ampli ﬁcation capability.Three or more single-gimbal CMG units are necessary for three-axis attitude control.A steering law gives each unit ’s motion,usually each unit ’s gimbal rate,to generate a required torque,which is provided by an attitude ing an appropriate steering law,the CMG system is expected to make a rapid change of its angular momentum vector to reach its maximum for maneuvering and to make a precise control of torque for pointing and tracking.Singularity is the most serious problem for these tasks.The possible output torque does not cover the 3-D space at a singular state.Points on the border of the range of the angular momentum vector are trivially singular.Other than those,any CMG system has singular states,which form surfaces inside the border,and such singular states must be avoided.Various singularity avoidance methods have been studied.The pseudoinverse solution has been tried as an exact method for steering law calculation [1];additional null solutions were used for singularity avoidance using a gradient method [2–4].Even with various objective functions as singularity measures,such gradient methods were insuf ﬁciently successful,especially for the pyramid-type (four-skew)system.Then,varieties of singularity robust (SR)inverse methods were proposed,which attempted singularity avoidance by allowing torque error [5–9].Recently,variable-speed CMGs (VSCMG),which were once studied as integrated power and attitude control devices [10],have been revived for their singularity avoidance capability [11–15].Mathematical studies of CMG singularity are essential for design and evaluation of a steering law,as well as for selection and evaluation of system con ﬁgurations.The work by Margulies and Aubrun [16],which has offered a general theory,has remained theReceived 15August 2006;accepted for publication 6March 2007.Copyright ©2007by the American Institute of Aeronautics and Astronautics,Inc.All rights reserved.Copies of this paper may be made for personal or internal use,on condition that the copier pay the $10.00per-copy fee to the Copyright Clearance Center,Inc.,222Rosewood Drive,Danvers,MA 01923;include the code 0731-5090/07$10.00in correspondence with the CCC.Haruhisa Kurokawa received B.E.and M.E.degrees in precision machinery engineering,and a Dr.Eng.degree in aeronautics and astronautics from the University of Tokyo,respectively,in 1978,1981,and 1997.He currently heads the Distributed System Design Research Group,Intelligent Systems Research Institute,National Institute of Advanced Industrial Science and Technology (AIST),Japan.His main research subjects are kinematics of mechanisms,distributed autonomous systems,and nonlinear control.His current research theme is a modular robotic system.J OURNAL OF G UIDANCE ,C ONTROL ,AND D YNAMICS Vol.30,No.5,September –October 20071331D o w n l o a d e d b y H U A Z H O N G U N I VE R S I T Y OF S C I E N C E o n A p r i l 1, 2015 | h t t p ://a r c .a i a a .o r g | D O I : 10.2514/1.27316most important and most referred paper.In contrast,works by Tokar published originally in Russian have been virtually ignored,even though they were published during the same year as [16]and presented various theoretical ideas and calculation results [17–20].About ten years were spent to catch up to the results presented by Tokar through studies following the paper by Margulies and Aubrun [21–27].Some confusion still remains in relation to the theory regarding terminology and interpretations;for this reason,theoretical studies have not been used effectively for analyses and evaluation of recent steering law studies.This paper is intended as a survey and analysis of steering laws based on the theory of CMG singularities.After a summary of basic terms and equations in Sec.II,the various research streams of theory will be brie ﬂy surveyed.Because theory itself is a basis of steering law analyses,a general and uni ﬁed theory will be outlined after this survey.A remaining problem regarding a degenerate state will be formulated.Section IV presents a survey of steering laws.Because all steering laws have been analyzed and evaluated using simulations and experiments in their original papers,geometric analyses and qualitative evaluations of those steering laws ’motions are provided in Sec.V.Section VI gives a guide for future studies.Mathematical details and remaining problems are brie ﬂy explained in the Appendix.II.Basic MathematicsVariables and equations,most of which are based on [16],are de ﬁned in this section.The same notation as those in [16]are used to the greatest extent possible,although most expressions are produced using vector algebra.Satellite dynamics and the effects of gimbal acceleration are not considered unless stated otherwise.A.General DescriptionA general system,especially a 3-D redundant system,is con-sidered consisting of n (>3)single-gimbal CMG units.The angular momentum of all CMG units is set to unity.The system state is de ﬁned by the set of all gimbal angles,each of which is denoted by i for i 1;...;n .No limits are assigned to the angles.Three mutually orthogonal unit vectors for each CMG unit are de ﬁned as a gimbal vector g i ,an angular momentum vector h i ,and a torque vector c i ,wherec i @h i =@ i g i h i(1)The gimbal vectors are ﬁxed and the others are dependent upon thegimbal angles.In this paper,the same symbol is used to represent a vector in the Euclidean space as well as its representation in a column vector based on the CMG system ’s coordinate basis.The system con ﬁguration is de ﬁned by the arrangement of the g i .Many con ﬁgurations have been investigated,most of which are categorized as follows:1)Independent-type con ﬁguration:all g i are different and no three of them are coplanar.2)Coplanar con ﬁguration:all g i are on the same plane.3)Multiparallel type con ﬁguration:g i are grouped sharing the same directions.As examples,a pyramid (four-skew)con ﬁguration is of the ﬁrst category and a roof-type (two-speed)con ﬁguration belongs to the latter two.The total angular momentum H and the output torque T are given byH HX n i 1h i(2)TXic id i =dt C d =dt(3)where is the state variable, 1;...; n ,and d =dt d 1=dt;...;d n =dt t is an n -dimensional column vector.The 3 n matrix C is the Jacobian of Eq.(2).Hereafter, and H are,respectively,called a state and a point.B.Steering LawA steering law obtains the gimbal rates,! !1;...;!n t ,with which the resulting torque in Eq.(3)is equal to the torque command T com .For n >3,the general solution is given as! C t CC t 1T com !N(4)The ﬁrst term in the right is the Moor –Penrose pseudoinverse solution,which gives the minimal norm solution.The second term,!N ,is a solution of the homogeneous equation as C !N 0;0;0 t ;it is called a null vector .(The term null motion is used as a ﬁnite change of the state,keeping H the same.)It is noteworthy that two kinds of torque are not shown in Eqs.(3)and (4),which must be included in the precise equation of the attitude control problem.One is the reaction torque by acceleration of gimbal rotation.It is usually ignored for steering laws in practice,as described in Sec.V.A.The other is the gyro-effect torque caused by the vehicle ’s rotation.This torque is usually included in the dynamics equation of the attitude control system;consequently,it is not ignored but included in T com in Eq.(4).C.Singular State and SurfaceWhen the system is singular,that is,det CC t 0,all torque vectors c i become coplanar and the possible outputs T of Eq.(3)do not span 3-D space.The rank of C can become one if all g i are on the same plane,as is true of a coplanar system.Hereinafter,an independent-type con ﬁguration is assumed.For that reason,the rank does not reduce to one.All singular H as points in 3-D space form a continuous and mostly smooth surface called a singular surface .It includes the angular momentum envelope (the envelope for short)and an internal singular surface.The singular surface and its corresponding are calculable using the methods described in [16,26].Let all variables at a singular state be superscripted by S .At a singular state S ,a unit vector u normal to all c S i along with related variables are de ﬁned asu c S i 0(5)q i u h S i(6)p i 1=q i (7)"i 8<: ;q i >00;q i 0 ;q i <0(8)These equations do not uniquely de ﬁne the variables,because there are two choices of u .Though unique de ﬁnition is not necessary for geometric analysis,a condition is assumed hereafter for simpler explanation in the next section so that the number of positive "i is not less than that of negative ones.Also see the footnote in Sec III.C.The Gaussian curvature of the singular surface is described as1= 1=2X iXjp i p j c S i c S ju 2(9)where a b c is a box product of three vectors such thata b c a b c [16,28].It is noteworthy that the range of H is assumed to be simply connected for any con ﬁguration without a hole or a tunnel.This is not trivial and has not been proved yet [16](see Appendix).The1332KUROKAWAD o w n l o a d e d b y H U A Z H O N G U N I VE R S I T Y OF S C I E N C E o n A p r i l 1, 2015 | h t t p ://a r c .a i a a .o r g | D O I : 10.2514/1.27316Gaussian curvature and the principal curvatures have an important meaning,as described later,but other properties of the surface described by differential geometric tools have not been related clearly to singularity analysis and steering laws.For such mathematics,[24,26]provide good explanations.D.Singular Value DecompositionThe matrix C can be represented using its singular values i and unitary transformations U and V ,as follows:C U 100000 200...000 3002435V t(10)Throughout this paper,the following is assumed:1 2 3 0(11)At a nonsingular state, 3≠0and the unitary matrices U and V canbe constructed byU ^c 1^c 2^c 3 ;V ’T 1’T 2’T 3’N 1’N 2...’N n 3(12)where three ^ci are the eigenvectors corresponding to the singular values i and which make up an orthonormal basis in the Euclidean space,and all ’i make up a corresponding orthonormal basis of the tangent space of .The three ’T i (i 1,2,3)are Moor –Penrosepseudoinverse solutions,and ’Ni (i 1;...;n 3)are null vectors in Eq.(4).At a singular state,the minimal singular value 3becomes 0and the two matrices in Eq.(12)become U ^c T 1^c T 2u ;V ’T 1’T 2’N1’N 2...’N n 2 (13)III.Theory of Singular SurfaceThe intended goal of the theory was ﬁrst to obtain the size andshape of the workspace of a CMG system for selection of a con ﬁguration.Margulies and Aubrun established a general method described in Sec.II.C,as well as a general formulation of a steering law and null vectors [16].They introduced a quadratic form,which this author followed to formulate a classi ﬁcation of a singular state according to whether it is escapable by a null motion [21].Bedrossian et al.[24]and Wie [26]pursued a more detailed investigation of the differential geometric properties of a singular surface related to escapability.During the same year as the work by Margulies and Aubrun,Tokar presented an impassable singularity theory based on the same quadratic form and evaluated various con ﬁgurations [17–20].Because the original paper of those were in Russian and their English translations used different terminology,such as a “gyrostabilizer ”or a “gyrodyne ”for a CMG,those works did not become well-known in the West.Nearly ten years were spent by the aforementioned researchers to match Tokar ’s results.Two terms,impassability and escapability,were used inde-pendently for similar properties of a singular state.In the following,their de ﬁnitions and the theory of CMG singularity will be summarized.Remaining problems regarding a degenerate state,which [24,26]emphasized as important for escapability analysis,will be formulated.A.Quadratic Form,Passability,and EscapabilityIn [16],a quadratic form Q d at a singular state is de ﬁned as a second-order in ﬁnitesimal change of H along the u direction by an in ﬁnitesimal displacement d (see Appendix):Q d 2 u H Xq i d i 2(14)An in ﬁnitesimal displacement d is an element of the n -dimensional tangent space of the space.Three special subspacesexist:the null space,its complementary space,and the singularly constrained space.(An expression of bases of the three spaces is shown in [23].)The null space f d N g is n 2-dimensional andspanned by f ’N i g in Eq.(13);its complementary space f d Tg is 2-D and spanned by f ’T i g .The singularly constrained space is 2-D and itselement,d S,keeps the system singular.Two spaces of d N and d T are suf ﬁcient to cover the whole d space,but two spaces of d N and d S are not always so.For the explanations in this section,we presume the following property at a singular state:Surface Regularity :The singular surface is regular,that is,smooth,at H S ,which implies that d H d S span the surface,and that d S and d N are independent,and that they all span n -dimensional space.This condition is assumed in [16]without any discussion.Actually,this holds at most singular states for an independent-type con ﬁguration (see Sec.III.D).By this condition,any d and the corresponding Q d are decomposable as [25,28]d d S d N(15)Q d Q d S Q d N(16)∗The ﬁrst term of the right-hand side in Eq.(16),Q d S ,is the second fundamental form of the singular surface (see Appendix).See [16]for another expression.The last term of Eq.(16),Q d N ,gives passability of the singular surface as follows:Because the ﬁrst term on the right is attributable to the curved singular surface,the last term represents second-order in ﬁnitesimal displacement of H to u direction from the singular surface.If this quadratic form is de ﬁnite,that is,if it is the same sign for any d N ,any motion is restricted to the same side of the surface in H space.Because the quadratic form and its derivatives are continuous with respect to S ,H S of de ﬁnite form make up a certain area of the surface,through which it is not possible to pass from one side to the other if is in the (in ﬁnitesimal)neighborhood of corresponding singular states.In this sense,such an area of the surface with a de ﬁnite form is termed impassable [20].Similarly,an area of the surface with an inde ﬁnite form is passable .Another aspect of this form category is escapability [21,24,26].There exist directions in the d N space (null space),along which the quadratic form is zero if a singular state is passable (i.e.,hyperbolic)having an inde ﬁnite Q d N .As the motion by d N keeps H on the singular surface and d N is independent from d S under surface regularity condition,escape from a passable singular state on a regular surface is always possible.In contrast,no escape is possible at an elliptic (i.e.,impassable)singular state.Therefore,passability and escapability are compatible where the surface is regular.Although other terms such as “elliptic/hyperbolic ”[16,21,24,26]and “de ﬁnite/inde ﬁnite ”[22,23]have also been used;the terms “impassable/passable ”are used in this paper because they were de ﬁned earliest.B.Passability and Surface CurvatureA relation is apparent between passability and the local shape of asingular surface.The signature of Q d is the sum of the signatures of the two quadratic forms Q d S and Q d N by Sylvester ’s law of inertia.Because the signature of Q d is f "i g and that of Q d S includes signs of two principal curvatures,there can be only three impassable surface types [23].Type 1:If all "i are positive,Q d N is always positive de ﬁnite.The H of this type is on the envelope and the Gaussian curvature is positive;consequently,the surface is convex to u .∗It is helpful to represent d S and d N ,respectively,as column vectors d S 2R 2and d N 2R n 2based on the orthonormal basis of the two subspaces.The two quadratic forms are expressed as d St Q S d S and d Nt Q N d N ,where Q S and Q N are 2 2and n 2 n 2 matrices.KUROKAWA 1333D o w n l o a d e d b y H U A Z H O N G U N I VE R S I T Y OF S C I E N C E o n A p r i l 1, 2015 | h t t p ://a r c .a i a a .o r g | D O I : 10.2514/1.27316Type 2:If all "i but one are positive and the Gaussian curvature is negative,Q d N is positive de ﬁnite.Part of this type surface is on the envelope,but the remaining part is internal.The surface is curved as a hyperbolic saddle surface.Type 3:If all "i but two are positive and the signature of the ﬁrst quadratic form is ; ,Q d N is positive de ﬁnite.The surface of this type is fully internal and is concave to u ,and its Gaussian curvature is positive.Note that a positive does not always imply impassability because the signature of Q d S can be ; with positive .†The preceding explanation ignores the case in which u is parallel to g i ,that is,"i q i 0.Equations (15)and (16)hold even in this case,and one signature of Q d S is 0,hence 0.Therefore,the signature of Q d N does not contain 0and a similar discussion is possible.C.Impassable Surface ExampleBased on this classi ﬁcation method and by various methods in [16],impassable surface regions are calculable.Any independent-type con ﬁguration has an internal impassable surface that is distinct from the envelope because a type 2surface extends smoothly inside from the envelope.The symmetric six-unit system has only type 2internal surfaces very near its envelope [20].However,systems with n 4or 5have both type 2and type 3surfaces extending far inside.For the symmetric pyramid con ﬁguration in Fig.1,impassable surface patches are obtained as in Fig.2.(A similar ﬁgure is shown in [20].)Impassable surfaces appear similar to connected strips forming a parallelepiped.A continuous curved line with analytical expressions is obtained to represent this framework of surface strips [25].Edges of the strip are shaped as folds;they are borders of impassable and passable regions (see a cross section in Fig.3a).On the folding border,1= in Eq.(9)is 0,and surface regularity does not hold.Figure 2a is a part of the internal impassable surfaces.All are obtained by successive 1=4rotations about the Z axis based on the system ’s symmetry (Fig.2b).As for multiparallel type con ﬁgurations,some differences exist in analysis,as described next.However,impassable surfaces of such systems are also de ﬁned by Q d N and can be calculated.Impassable surfaces of the roof-type system are the point at the origin and two unit circles [22].Unlike independent-type con ﬁgurations,there are systems with no internal impassable surface with n 6.‡D.Regularity Condition and Degenerate StateFor the examinations in Secs.III.A and III.B,an independent type of con ﬁguration and surface regularity are assumed.Although passability and escapability are de ﬁnable for any con ﬁguration,we require additional analysis for exceptional cases.Moreover,if the regularity condition does not hold,that is,one d S direction becomes included in the null space,and if a ﬁnite null motion along this d S maintains singularity,escape by this null motion is not possible.Bedrossian et al.[24]discussed this problem and termed this state a degenerate state.A degenerate state is a special case in which surface regularity breaks.Examples of degenerate states that have been identi ﬁed are all 2-D systems and multiparallel-type con ﬁgurations [24].Singularity and surface geometry of 2-D systems and multiparallel-type con ﬁgura-tions differ from those of independent-type con ﬁgurations.For example,a singular surface corresponding to u parallel to g i is a unit circle for an independent-type con ﬁguration,but it is a circular plate for a multiparallel-type con ﬁguration.Moreover,2-D systems withn 3and most multiparallel type systems with n 6have no internal impassable state.For an independent-type system,surface regularity breaks on the borderline of an impassable surface and a passable surface.Through calculations,no degenerate state has been found for various independent-type con ﬁgurations.This engenders the conjecture 1in the Appendix that no degenerate state exists for an independent-type con ﬁguration.Because most multiparallel type systems have no problem of impassability,it is expected that degenerate states cause a problem only for the roof-type system.To construct a comprehensive theory,these must be proved in a future study (see Appendix).E.Inverse Manifold and Topological AnalysisPassability is de ﬁned using in ﬁnitesimal analysis;it remains unclear how far the effect of an impassable surface extends.Inverse manifolds and their change clearly show the meaning of impassability and expected H deviation to avoid singularity [25,27].Through consideration of the topological connection of manifolds,a global problem of the pyramid-type system was found.1.Inverse ManifoldA steering law is a method to obtain gimbal rates that correspond to a given torque command:the change of H .A system ’s behaviorcanFig.1Symmetric pyramid-type system.The pyramid is half of a regular hexahedron for cos 2 1= 3.g 1g 4g 3g 2XY ZF QHGPW LV A a) Calculation resultsb) Total impassable surface structureFig.2Impassable surface of the symmetric pyramid-type system.a)Impassable singular points are calculated with " ; ; ; and ; ; ; for various u scattered on the Gaussian sphere.There is an analytical expression along line ABCDE.The surface patches around AB and inside BCDEB are of type 2,and that inside CFDC is of type 3.The cross section near *is shown in Fig.3a.b)All lines symmetrically transformed from the curved line ABCDE are shown with a half-cut envelope.†If n 4and the total signature is ; ; ; ,the surface with signature ( )is also impassable and positive is the suf ﬁcient condition for type 3.In this case,it is better for consistent de ﬁnition to rede ﬁne u by u and "by "so that u represents the direction in which H motion is impossible.‡The proofs of passability of multiparallel type con ﬁgurations in [23,28]were based on surface curvature,irrespective of surface regularity;hence they are not correct.An identical conclusion,however,is obtained by direct evaluation of Q d N .For example,a six-unit system as a pair of three-unit planar systems has no internal impassable surface because each planar subsystem has no internal impassable state as a 2-D system.1334KUROKAWAD o w n l o a d e d b y H U A Z H O N G U N I VE R S I T Y OF S C I E N C E o n A p r i l 1, 2015 | h t t p ://a r c .a i a a .o r g | D O I : 10.2514/1.27316be viewed by a path of that corresponds to a path of H if time is ignored.All possible paths of are visible by inverse images of the mapping of Eq.(2).The inverse image is a set of subspaces that are mutually disjoint.Each subspace is either an n 3-dimensional manifold or a similar complex,which is hereafter termed a null-motion manifold or a manifold for short.The shapes of manifolds in the neighborhood of a singular state are characterized by setting the quadratic form Q d N constant.It resembles either a superellipsoid or a superhyperbolic surface.The number of disjoint manifolds is held constant when H moves without encountering a singular surface.Figure 3a shows part of a cross section of the singular surface of the symmetric pyramid-type system in Fig.1.A manifold is topo-logically a loop in the 4-D torus space.Along with the H motion described by ABCDE,the manifold bifurcates at B,and one of them terminates at D (Fig.3b).Once the system state is on such a terminating manifold in the triangular region,there is no way to reach the other manifold by any null motion other than driving H out of the region through a passable surface.This example illustrates the effect of an impassable surface covering a region surrounded by singular surfaces.The shape and the size of an impassable surface are important for evaluation of possible error in the H path.2.Global ProblemTopological theory does not allow one-to-one continuous mappings from H to over the range of H [29].This fact is not always a problem for a steering law.If an H workspace without an impassable surface is considered,a gradient method works effec-tively.In this case,a discontinuous and one-to-multi mapping gives local maxima of the objective function for a gradient method.The question is the size of such workspace.A workspace without an impassable surface can be almost equal to the envelope in the case of the symmetric six unit system,but it must be reduced greatly in the case of the pyramid-type system.Apparently,the problem of impassability is not suf ﬁciently serious to exclude all impassable surfaces from the workspace when viewed with the aforementioned manifolds.Local avoidance of the impassable singularity shown in Fig.3b seems possible if an appropriate manifold is selected before entering the triangular region [27].However,if a reduced H workspace shown in Fig.4is considered,the aforementioned possibility was eliminated ﬁrst by simulation results [30],and subsequently by geometrical analysis [25].This problem,hereafter called a global problem,holds that no steering law for the symmetric pyramid-type system can continue to exactly generate arbitrary torque inside the workspace shown in Fig.4.IV.Survey of Steering LawsVarious steering laws have been proposed in the literature.Although most steering laws were formulated for a general system,the symmetric pyramid con ﬁguration is mainly assumed in the following unless stated otherwise.Steering laws are grouped as exact methods,of ﬂine planning,an SR inverse family,and methods for variable-speed CMGs.A.Steering Law by Exact Solution1.Moor –Penrose Pseudoinverse Steering LawThe pseudoinverse solution,which is the ﬁrst term on the right in Eq.(4),is orthogonal to the tangent space of the null-motion manifold.It has often been claimed that this method tends to drive the system to approach a singular state [3].There is actually no such tendency to approach or to escape from a singular state from a continuous control perspective because the motion of by the pseudoinverse solution remains on a curve that is normal to the manifolds.Discrete time control,however,induces the system to approach a passable state as follows:The shapes of manifolds near a passable singular state are hyperbolic;consequently,their normal curves are also hyperbolic and convex to the singular state.Stepwise change of i moves the system state away from the normal curve and nearer to the singular state.2.Gradient MethodVarious proposed steering laws with singularity avoidance are categorized as gradient methods [2–4].They use the solution of Eq.(4);a null motion is determined to increase (or decrease)an objective function,which represents distance (or similarity)to singular states such as det CC t ,the minimum eigenvalue of C ,or the condition number of C .Any passable singular state is avoidable and escapable by any such method,and no impassable one is escapable.Therefore,this method is effective for systems such as three double-gimbal CMGs or multiparallel type systems that have no internal impassable singularity.On the other hand,because of the global problem,no exact type steering law including a gradient method is effective for systems with a few CMG units,such as the pyramid-type system and the roof-type system.3.Steering Laws for the Roof-Type SystemStudies of the roof-type CMG system were made more than 15years ago [31,32].Those steering laws were based on decomposition of H into planar subsystems.Because singularity problems were better clari ﬁed for the roof-type system,those steering laws were designed to avoid most of the internal singular states through the use of exact methods.The remaining singular states were not escapable [31],or were avoided using a discontinuous motion [32].4.Preferred Angle Setting and Constrained Steering LawAfter simulation results by Bauer [30]indicating the global problem,Vadali et al.proposed preferred gimbal angles to assure singularity avoidance for command torque in a speci ﬁed direction [33].Such a method can be effective either for maneuvering or for pointing under the condition that the disturbance torque is roughly estimated along a certain direction.Even for maneuvering,its problem is that it is not always able to reach the preferred angles by null motion.For pointing,even when disturbance torque is in a certain direction,the CMG system controlled by a usual steeringlaw,AB Impassable surfaceb) Change of manifoldFig.3Path of H and change of inverse manifold.Manifolds are obtained by computer calculations and illustrated in 2-D space.XZYWorkspace candidateFig.4Spherical workspace candidate.The sphere entirely surrounds a hexahedral frame structure made of impassable surface strips.KUROKAWA 1335D o w n l o a d e d b y H U A Z H O N G U N I VE R S I T Y OF S C I E N C E o n A p r i l 1, 2015 | h t t p ://a r c .a i a a .o r g | D O I : 10.2514/1.27316。

lesson 10

SEC 4
SEC 5
马迪 2005年9月1日
SEC 6
返回

LOGO
Section 2
SEC 1
Sample Study
Sample 2 (1)
November 15, 2006 Mrs. Edith Hampton 4575 Village Drive Seattle, WA 98105-5032 Dear Mrs. Hampton: I was deeply saddened to learn of Frank’s death and I would like to express my sincere sympathy to you and your family on behalf of the senior management team here at Interconnect Corp. 惊悉弗兰克去世的消息不胜悲哀，我代表Interconnect高层管理人员向您及家人表示诚挚的慰问。
Ma Ji excelled at performing new items reflecting real life. Apart from satire, he pioneered new items praising the new life and new heroes and heroines. In addition to performing traditional items he started writing and performing cross-talk comic dialogues such as Ode to Friendship, Storm on the State and Multistory Restaurant, and solo comic dialogues like The Universal-Brand Cigarette. In Five Officers Contend for Fame, he was joined by several other comedians. Ma Ji also set great store by theoretical research into Xiangsheng so as to make his creations more socially relevant. His books include Selected Xiangsheng by Ma Ji and An Informal Discussion on the Art of Xiangsheng.

1、下载文档前请自行甄别文档内容的完整性，平台不提供额外的编辑、内容补充、找答案等附加服务。
2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
3、如文档侵犯您的权益，请联系客服反馈,我们会尽快为您处理(人工客服工作时间：9:00-18:30)。

SCA NJ
Company Profile
三、推行TWI-JI 后的效果
3、员工普遍反映：用工作指导方法来指导员工工作，学习领悟快，有效提高了工作效率；
4、员工对TWI-JI培训的效果评估反馈结果如下：
参加培训351人,共计有30人对26名培训讲师进行了效果评估,平均情况如下评价项目第一阶段:学习准备第二阶段：传授工作第三阶段：尝试练习第三阶段：检验成效优秀 48% 39% 40% 38% 良好 40% 35% 36% 37% 一般 6% 16% 14% 17% 有待提高 6% 10% 10% 8% 差 0% 0% 0% 0%
SCA NJ
Company Profile
二、 TWI-JI 三阶段培训和推广
6、利用公司宣传栏进行宣传，表彰TWI-JI工作推广较好的讲师。
SCA NJ
Company Profile
三、推行TWI-JI 后的效果
SCA NJ
Company Profile
三、推行TWI-JI 后的效果
1、生产现场管理水平明显提高，在公司每月的6S检查评分中，成绩提高明显；
运输和消费
品包装工业包装瓦楞纸板
家外用
卫生纸品、卫生理念包装产品纸巾产品
2008年销售额:110亿欧元,业务遍及全球90个国家
SCA NJ
Company Profile
一、SCA集团概况----主要品牌
个人护理用品失禁护理用品位列世界第一，占有全球27%的市场份额婴儿纸尿裤和女性卫生用品在区域市场占有领先地位卫生纸世界第三大、欧洲最大的家用卫生纸和家外卫生纸供应商包装产品欧洲领先的包装解决方案供应商之一欧洲第二大瓦楞纸板生产商林业产品欧洲最大森林面积所有者，拥有260万公顷的森林欧洲森林产品效益最好的生产厂商之一
成立时间 Established 股份所有 Shareholder 占地面积 Land Area 注册资本 Registered Capital 投资总额 Investment Amount
SCA NJ
1997.7 SCA 100% 53,669 sqm
USD 11,000K USD 22,000K
二、 TWI-JI 三阶段培训和推广
3、每一级担任讲师的学员必须每人提交两份作业分解培训案例；
作业分解案例
4、公司加强了培训的考勤管理，参加培训的学员均要在培训记录表中签到；
SCA NJ
Company Profile
二、 TWI-JI 三阶段培训和推广
5、每场培训均有未参加卓制培训的部门经理和公司领导参加，对讲师进行监督与督促；
零售和运输包装解决
方案消费品包装和工业包装代客包装服务 4,300名员工 15 家工厂
1,350名员工 2家工厂
总部设在中国上海在14个国家拥有约7,150名员工 2008年销售额约7.2亿欧元
SCA NJ
Company Profile
一、SCA集团概况----主要客户
SCA NJ
Company Profile
一、SCA集团概况----包装亚洲分布
2008年各区域销售比例
东南亚
中国
内蒙古呼和浩特北京河北香河天津河北保定上海闵行江苏苏州
17% 83%
中国
东南亚
马来西亚吉隆坡马来西亚柔佛新加坡 x 2 印尼巴淡岛
江苏南京湖北武汉广东广州
15家包装工厂，拥有4300名员工
SCA NJ
Company Profile
SCA NJ
Company Profile
一、SCA集团概况----包装亚洲产品
说明书 & 手册
全色印刷资料软/硬封面宣传册营销资料 CD组合
常规瓦楞包装
最多可达6色印刷针对商业用途的特制解决方案
消费类包装
最多可达6色印刷彩印裱贴纸板
附件类材料
缠绕膜托盘胶带
SCA NJ
Company Profile
二、 TWI-JI 三阶段培训和推广
公司开展了一系列配套工作，以确保TWI-JI的有效落实与推广：
1、专门制订了TWI-JI的培训计划；
TWI-JI培训计划及
实施情况
3、公司根据培训计划集中组织和安排每场培训，由上一级培训学员任讲师；
SCA NJ
Company Profile
Company Profile
一、SCA集团概况----南京公司厂区一览
正门 The Front gate
物流通道 Logistics & Loading Area
厂房 Workshops
两栋厂房&空中走廊
西门 The West Gate
员工餐厅 Staff Canteen
行政办公楼 Administration Office
部门 BOX厂 EPS厂物流部设备动力部人事行政部市场销售部商务部技术部质管部
08年6S月平均分 76 81 82 83 85 85 87 89 90
09年1至11月平均分 86 87 91 91 93 92 96 95 95
200912 91 91 91 96 96 96 98 95 97
TWI JI如何在工厂快速而有效的推广 How to implement TWI JI in the company efficiently within short time 南京爱生雅工业材料包装有限公司 Nan Jing SCA Industrial Material Packaging Co., Ltd
SCA NJ
Company Profile
二、 TWI-JI 三阶段培训和推广
第二阶段培训： 1、由第一阶段培训的8名学员作为讲师，对公司领班以上员工分批培训； 2、共计组织了7场培训、67人参加、合计接受培训103小时
第三阶段培训： 1、由第二阶段培训的领班以上员工作为讲师，对公司本班组成员分批培训； 2、共计组织了23场培训、284人参加、合计接受培训284小时
“成为领先的整合包装解决方案提供商，为客户、员工及股东创造更高的价值”
泡沫
EPS铸模 EPE转化
展示架
独立式展示架货架展示
工业类/重型瓦楞包装
大型箱重装箱
内衬
纸浆模瓦楞
特殊瓦楞包装
防腐蚀防静电
SCA NJ
Company Profile
一、SCA集团概况----南京公司
201001 91 92 92 94 95 96 95 96 97
SCA NJ
Company Profile
三、推行TWI-JI 后的效果
2、生产线上的操作失误、安全事故、产品质量等问题减少了；
纸箱月平均发生质量事故次数项目顾客投诉批量事故客户退货合计月平均产量(T) 吨产品质量事故率 08年 2 6 2 10 1309 0.73% 09年1至11月 3 5 2 10 1475 0.67% 0912 5 3 1 9 2039 0.44% 1001 0 3 3 6 2856 0.21%
SCA NJ
Company Profile
二、 TWI-JI 三阶段培训和推广
SCA NJ
Company Profile
二、 TWI-JI 三阶段培训和推广
第一阶段培训：由公司组织5名正式学员和3名旁听学员参加卓制公司TWI-JI培训

培训效果非常好，主要原因是： 1、集团及公司领导均高度重视； 2、培训方式新颖、讲解深入浅出、互动性强、使培训者能迅速学会如何指导部属很快地接受到正确、完整的技术或指令； 3、学员对于后续的第二阶段培训充满信息。
SCA NJ
Company Profile
一、SCA集团概况----主要工厂
卫生纸品澳洲

失禁护理用品婴儿纸尿裤女性卫生用品纸巾 1,500名员工 6家工厂

卫生纸品北亚
失禁护理用品纸巾
卫生纸品中南亚

失禁护理用品婴儿纸尿裤女性卫生用品纸巾
包装亚洲
SCA NJ
Company Profile
一、SCA集团概况
SCA NJ
Company Profile
一、SCA集团概况----主要产品
林业产品个人护理产品
印刷用纸实木产品纸浆和木材
失禁护理
用品婴儿纸尿裤女性卫生用品
15% 30%
21% 34%
厕卫纸，
厨卫卷纸，面巾纸，餐巾纸