lecture 3---

合集下载

【精选】Lecture-3---模型算法控制-MAC-幻灯片

u(k) dT Yr (k) G2U2(k) he(k)
模型匹配情况（即没有模型误差）
当e(k) 0(即gi gˆi ), u(k) dT Yr (k) G2U2(k)
y(1)h0u(1)h1u(0)
k
y(2)h0u(2)h1u(1)h2u(0)
k
y(k) hiu(ki)
y(k)h0u(k)h1u(k1) hku(0) hiu(ki)
i0
i0
5
预备知识
System
u(k)
y(k)
u { u ( 0 )u ( 1 ) u ( k ) } y { y ( 0 )y ( 1 ) y ( k )
3
预备知识
System
u(k)
y(k)
u ( k ): { 100} y { h ( k ) } { h 0h 1h 2 }
u { u (0 )00 }y { h 0 u ( 0 )h 1 u ( 0 )h 2 u ( 0 ) }
u { 010 }
y { 0h 0 h 1 h 2 }
u { 0u (1 )0 } y { 0 h 0 u ( 1 )h 1 u ( 1 )h 2 u ( 1 ) }
u { 0 0u ( k )0}y {0 h 0 u ( k )h 1 u ( k )h 2 u ( k ) }
u { u ( 0 )u ( 1 ) u ( k ) } y:{y(k)}?
4
预备知识
u { u(0 )00 } y { h 0 u (0 )h 1 u (0 )h 2 u (0 ) }
w
参考轨迹
yr (k i)
+
u (k )
y (k )
优化计算

lecture_3---耦合

以X的共振频率照射，使X( )与X( ) 之间快速相互迁移，从而使检测到的信号是二者的平均信号 A Notation: A{X}
A
J
A
X
irrad at X X
X( )
X( )
A( )
A( )
Decoupling of 13C NMR
更简单的谱线
更高的信噪比
OH
OH
O
Decoupling of 13C NMR
1H－1H三键耦合－－－Karplus
Ha
Equation
Jab Jad
Jac
远程耦合
跨越四根键及更远的偶合称为长程偶合，偶合常数一般小于1Hz。但是，对于连结偶合核的键在空间中呈固定‘ W ’构型的分子。
远程耦合
H
4
H
5
H
C
C
C
H
4J H1-H3 5J H1-H4
= 1.07 Hz = 1.21 Hz = 0.95 Hz
H－D一键耦合常数为6.5Hz，二、三键耦合常数为1－2Hz。 I＝1/2 可将质子裂分为N＋1个峰一键耦合常数为几十到一百几十，
19F
三氟丙酮中氟对甲基质子的四键耦合常数仍然有4.3Hz。
31P
I＝1/2 可将质子裂分为N＋1个峰一键耦合常数为200-700Hz，二键耦合常数为0.5-20Hz。
I
S
I
S
耦合常数 J （Hz）
耦合常数的值与谱仪频率无关
原子核间的耦合－－－N＋1（2I＋1）规律
b Ib S I aIbS S I S bIaS
aIaS
aa ab ba bb CH3
aaa aab aba baa abb bab bba bbb CH2

(英文)细胞生物学lecture3-生物膜的选择透过性

No ion flow
Membrane potential is 0
Ions would cross the membrane if allowed (if a channel was open) There is a membrane potential
13
Ion distribution across cell membranes
5
Carrier-mediated transport is saturable
When all substrate binding sites are filled: maximal transport rate (vmax) vmax depends on how fast the carrier can flip between its two conformational state
11
How can we test experimentally whether mutations in GLUT1 prevent glucose transport?
Step 1: Extract GLUT1 Step 2: Reconstitute in liposome from membrane 1. extract the transporter from the membrane using detergents
2
Which proteins mediate molecule transport across a membrane?
3
How does a carrier/transporter work?
• One or more specific binding sites for the solute • Ligand binding induces conformational change in the transporter conformational change is the rate limimation and conformational changes

lecture3

n-BuLi, then MeI (61%)
Me
Wojtkowski, JOC 1971, 1790.
Shanghai, China
• Base-induced Reactions
Aldol-type reactions:
O R N2
LDA is the optimal base for lithiation
Shanghai, China
§ 4-1 Some Reactions of Nitro Compounds
Stabilized carbanion Transformation of the -NO2 group
NO2 R
Other reactions
-NO2 as a Leaving Group
Me O
Me O
Me
Smith, JACS, 1981, 2009.
Me
Rearrangement:
OO N2
BF3 OEt 2
O N2
N2
O
R
Mander, Aust. J. Chem. 1979, 1975.
OBF 3 R
R
O
Shanghai, China
• Ring Expansion Reactions:
Shanghai, China
• Nucleophilic Addition
OH O R NO 2 + H R R3N EtOH NO 2 R
+ n
R R
O
R NO 2
H2N(CH 2)2NMe 2 Benzene, 80oC
NO 2
n NO 2
+ H2N(CH 2)2NMe 2 Benzene, 80oC

遗传学Lecture 3减数分裂

10
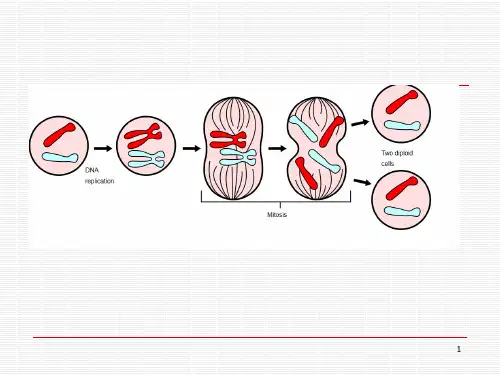
（二）减数分裂II
减数分裂I完成后进入间期，间期很短，相当于有丝分裂的间期，有的无间期，DNA不复制，很快进入第二次减数分裂，其过程与一般有丝分裂十分相似，可分前期、中期、后期、末期。
11
前期
中期
后期
末期四分孢子
四分孢子
性细胞形成过程中，每个性母细胞经过减数分裂形成4个子细胞，子细胞内的染色体数目只有原来性母细胞的一半，在植物中刚形成的细
15
非姐妹染色单体之间的交换
有
无
16
有丝分裂和减数分裂的对比视频网址
/v_show/id_XMTczNDk0OTQw.html?from=y1.7-1-87.4.16-1.8-1-2-15
18
19
20
21
22
遗传的染色体理论
The Boveri–Sutton chromosome theory (also known as the chromosome theory of inheritance or the Sutton–Boveri theory) is a fundamental unifying theory of genetics which identifies chromosomes as the carriers of genetic material （1902）.
1
减数分裂中染色体变化规律是1883年由 E.van贝内登在动物和1888年由E.A.施特拉斯布格在植物最初发现的。
二、减数分裂和遗传
减数分裂(meiosis)又称成熟分裂，是一特殊
的有丝分裂。特殊性表现于：仅发生于生殖细胞。包括两次连续分裂。
2
（一）减数分裂Ⅰ
前期、中期、后期、末期四个时期

稳定性与鲁棒性lecture3——鲁棒控制基础

u G(s)
y
N1 ( s ) G ( s) , D1 ( s )
N 2 ( s) H ( s) D2 ( s )
闭环传函
F ( s) G( s) H ( s) 1 G( s) H ( s)
通过F(s)的极点分布，判断系统的稳定性。也就是研究1+G(s)H(s)=0 的根，即 D1(s)D2(s)+N1(s) N2(s)=0 的根的情况
W(s) K(s) P(s)
w1
Ws(s) _ r
u
+
控制目标：尽量减少跟踪误差，即
由w1到e的传函
1 Ws ( s ) I [ P( s) ( s)W ( s)]K ( s)
1

确保鲁棒稳定性： W (s) P(s) K (s)S (s) 1 其中 S ( s)
1 I P( s) K ( s )
P( s)

1
(3) 反馈不确定性
ΔP(s) _ + P0(s) W(s)
P( s)
P ( s) 0 , 1 P( s )W ( s ) P ( s ) 0
ΔP(s) _
P( s )

1
W(s)
P0(s)
+
P ( s) 0 P( s ) , 1 P( s )W ( s )
所以标称模型只能是实际物理系统的不在外界干扰或系统模型发生变化时系统性能的保持能力；鲁棒控制：按照鲁棒性要求设计的控制方案叫做鲁棒控制; 鲁棒系统设计的目标：就是要在模型不精确和存在其他变化因素的条件下，使系统仍能保持预期性能。如果模型的变化和模型的不精确不影响系统的稳定性和其它动态性能，这样的系统我们称它为鲁棒控制系统。鲁棒控制理论：鲁棒性分析问题和鲁棒性综合问题

lecture3

Lecture3-Axioms of Consumer Preference and the Theory ofChoiceDavid Autor14.03Fall2004Agenda:1.Consumer preference theory(a)Notion of utility function(b)Axioms of consumer preference(c)Monotone transformations2.Theory of choice(a)Solving the consumer’s problemIngredientsCharacteristics of the solutionInterior vs corner solutions(b)Constrained maximization for consumer(c)Interpretation of the Lagrange multiplierRoad map:Theory1.Consumer preference theory2.Theory of choice3.Individual demand functions4.Market demandApplications1.Dead weight loss of Christmas2.Food stamps and other taxes and transfers3.Gi¤en goods:Theory and evidence1Consumer Preference TheoryA consumer’s utility from consumption of a given bundle“A”is determined by a personal utility function.1.1Cardinal and ordinal utilityCardinal Utility FunctionAccording to this approach U(A)is a cardinal number,that is:U:consumption bundle !R1measured in“utils”Ordinal Utility FunctionMore general than cardinal utility functionU provides a“ranking”or“preference ordering”over bundles.U:(A;B) !8<:A P B B P A A I BUsed in demand/consumer theoryCardinal vs Ordinal Utility FunctionsThe problem with cardinal utility functions comes from the di¢culty in…nding the appropriate measurement index(metric).Example:Is1util for person1equivalent to1util for person2?Or if we increase a person’s utility from1to2,is she twice as happy?By being unit-free ordinal utility functions avoid these problems.What’s important about utility functions is that it allows us to model how people make personal choices, that is,how they choose among competing alternatives.We do not’t need to know how many“utils”people experience from each choice to answer this question;we just need to know how they rank choices.Note:It’s much harder to model interpersonal comparisons of utility1.2Axioms of Consumer Preference TheoryCreated for purposes of:ing mathematical representation of utility functions2.Portraying rational behavior(rational in this case means‘optimizing’)3.Deriving“well-behaved”demand curves1.2.1Axiom1:Preferences are Complete(“Completeness”)For any two bundles A and B,a consumer can establish a preference ordering.That is,for any comparison of bundles,she will choose one and only one of the following:1.A P B2.B P A3.A I BWithout this property,preferences are unde…ned.1.2.2Axiom2:Preferences are Transitive(“Transitivity”)For any consumer if A P B and B P C then it must be that A P C:Consumers are consistent in their preferences.1.2.3Axiom3:Preferences are Continuous(“Continuity”)If A P B and C lies within an"radius of B then A P C.We need continuity to derive well-behaved demand curves.Given Axioms1-3are obeyed we can always de…ne a utility function.Any utility function that satis…es Axioms1-3cannot have indi¤erence curves that cross.Indi¤erence Curves De…ne a level of utility say U(x)=U then the indi¤erence curve for U,IC(U)is the locus of consumption bundles that generate utility level U for utility function U(x).An Indi¤erence Curve Map is a sequence of indi¤erence curves de…ned over every possible bundle and every utility level:f IC(0);IC(");IC(2");:::g with"=epsilonUtilsGood xGood yIC (2D)Utility function (3D)Graph 25Indi¤erence curves are level sets of this utility function.xyIC 3IC 2IC 1IC 3 !Utility level U 3IC 2 !Utility level U 2IC 1 !Utility level U 19=;U 3>U 2>U 1This is called an Indi¤erence Curve Map Properties:Every consumption bundle lies on some indi¤erence curve (by the completeness axiom) INDIFFERENCE CURVES CANNOT INTERSECT (by the transitivity axiom)Proof:say two indi¤erence curves intersect:Good yAccording to these indi¤erence curves:A P BB I CC P DD I ABy the above mentioned axioms:A P D and A I Dwhich is a contradiction.Axioms4.and5.are introduced to re‡ect observed behavior.They simplify problems greatly,but they are not necessary for a theory of rational choice.1.2.4Axiom4:Non-Satiation(Never Get Enough)Given two bundles,A and B,composed of two goods,X and Y.X A=amount of X in A,similarly X BY A=amount of Y in A,similarly Y BIf X A=X B and Y A>Y B(assuming utility is increasing in both arguments)then A P B(regardless of the levels of X A;X B;Y A;Y B)This implies that:1.The consumer always places positive value on more consumption2.Indi¤erence curve map stretches out endlessly(there is no upper limit to utility)1.2.5Axiom5:Diminishing Marginal Rate of SubstitutionIn order to de…ne this axiom we need to introduce the concept of Marginal Rate of Substitution and some further preliminary explanations.De…nition:MRS measures willingness to trade one bundle for another.Example:Bundle A=(6hours of sleep,50points on the problem set)Bundle B=(5hours of sleep,60points on the problem set)A andB lie on the same indi¤erence curveA student is willing to give up1more hour of sleep for10more points on the problem set.Her willingness to substitute sleep for grade points at the margin(i.e.for1fewer hours of sleep)is: 101= 10MRS(sleep for points)=j 10j=10MRS is measured along an indi¤erence curve and it may vary along the same indi¤erence curve.If so,we must de…ne the MRS relative to some bundle(starting point).dU=0along an indi¤erence curveTherefore:0=@U@xdx+@U@ydy0=MU x dx+MU y dydy dx=MU xMU y=MRS of x for yMRS must always be evaluated at some particular point(consumption bundle)on the indi¤erence curve.MRS of x for y decreases as we go down on the indi¤erence curve.This indi¤erence curve exhibits diminishing MRS:the rate at which(at the margin)a consumer is willing to trade x for y diminishes as the level of x consumed goes up.That is the slope of the indi¤erence curve between points B and C is less than the slope of the curve between points A and B.Diminishing MRS is a consequence of Diminishing Marginal Utility.A utility function exhibits diminishing marginal utility for good x when MU x decreases as consumption of x increases.A bow-shaped-to-origin(convex)indi¤erence curve is one in which utility function has diminishing MU fory Graph 29bothThis implies that consumer prefers diversity in consumption.An alternative de…nition of diminishing MRS can be given through the mathematical notion of convexity.De…nition:a function U(x;y)is convex if:U( x1+(1 )x2; y1+(1 )y2) U(x1;y1)+(1 )U(x2;y2)Suppose the two bundles,(x1;y1)and(x2;y2)are on the same indi¤erence curve.This property states that the convex combination of this two bundles is on higher indi¤erence curve than the two initial ones.Graph 30yx 2x 1y 2yx*y*where x = x 1+(1 )x 2and y = y 1+(1 )y 2.This is veri…ed for every 2(0;1).The following is an example of a non-convex curve:Graph 31y 2y 1x 2x 1y xIn this graph not every point on the line connecting two points above the curve is also above the curve,therefore the curve is not convex.Q:Suppose co¤ee and sushi have the same quality:the more you consume,the more you want.How do we1.3Cardinal vs Ordinal UtilityA utility function of the form U(x;y)=f(x;y)is cardinal in the sense that it reads o¤“utils”as a function of consumption.Obviously we don’t know what utils are or how to measure them.Nor do we assume that10utils is twice as good as5utils.That is a cardinal assumption.What we really care about is the ranking(or ordering)that a utility function gives over bundles of goods. Therefore we prefer to use ordinal utility functions.We want to know if A P B but not by how much.However we do care that the MRS along an indi¤erence curve is well de…ned,i.e.we do want to know precisely how people trade o¤among goods in indi¤erent(equally preferred)bundles.Q:How can we preserve properties of utility that we care about and believe in(1.ordering is unique and2. MRS exists)without imposing cardinal properties?A:We state that utility functions are only de…ned up to a“monotonic transformation”.De…nition:Monotonic TransformationLet I be an interval on the real line(R1)then:g:I !R1is a monotonic transformation if g is a strictly increasing function on I.If g(x)is di¤erentiable then g0(x)>08xInformally:A monotone transformation of a variable is a rank-preserving transformation.[Note:not all rank-preserving transformations are di¤erentiable.]Examples:Which are monotone functionsLet y be de…ned on R1:1.x=y+1[yes]2.x=2y[yes]3.x=exp(y)[yes]4.x=abs(y)[no]5.x=y2if y 0[yes]6.x=ln(y)if y>0[yes]7.x=y3if y 0[yes][yes–but unde…ned if y=0]8.x= 1y9.x=max(y2;y3)if y 0[yes]10.x=2y y2[no]Property:If U2(:)is a monotone transformation of U1(:),i.e.U2(:)=f(U1(:))where f(:)is monotone in U1as de…ned earlier,then:–U1and U2exhibit identical preference rankings–MRS of U1(U)and U2(U)=)U1and U2are equivalent for consumer theoryExample:U(x;y)=x y (Cobb-Douglas)U0U1U2xyWhat is the MRS along an indi¤erence curve U0?U0=x 0y 0dU0= x 10y 0dx+ x 0y 1dydydx U=U0= x 10y 0 x 0y 10= y0x0=@U=@x@U=@y Consider now a monotonic transformation of U:U1(x;y)=x yU2(x;y)=ln(U1(x;y))U2= ln x+ ln yWhat is the MRS of U2along an indi¤erence curve such that U2=ln U0?U20=ln U0= ln x0+ ln y0dU20=x0dx+y0dy=0dy U2=U20= y00=@U=@xwhich is the same as we derived for U1.How do we know that monotonic transformations always preserve the MRS of a utility function?Let U=f(x;y)be a utility functionLet g(U)be a monotonic transformation of U=f(x;y)The MRS of g(U)along an indi¤erence curve where U0=f(x0;y0)and g(U0)=g(f(x0;y0)) By totally di¤erentiating this equality we can obtain the MRS.dg(U0)=g0(f(x0;y0))f x(x0;y0)dx+g0(f(x0;y0))f y(x0;y0)dydy dx g(U)=g(U0)=g0(f(x0;y0))f x(x0;y0)g0(f(x0;y0))f y(x0;y0)=f x(x0;y0)f y(x0;y0)=@U=@x@U=@ywhich is the MRS of the original function U(x;y).。

Lecture 3 形式的复活

案例1
Dolphins were far hornier than Flipper ever let on; randy males had even been known to attempt couplings with human females.（Chromosome 8）
其实海豚很好色的，甚至比电视上的Flipper注还要过分，据说好色的雄性海豚还想和女人结合呢。
文学性
文学性是语言通过一定的排列组合造就的。这种排列组合是有规律的，这规律就是对语言运用的常规的故意违反，造成形式上的变异，从而形成陌生化或反常化（defamiliarization）的形式特征
读者在解读这些新颖乃至费解的形式特征进而获得理解的过程就是诗学所追求的审美或诗学效果。
三、“亡者”归来
后结构主义更加强调形式
案例3
The lip of the sun curled over the horizon to blow a red glow across the island.
太阳从天边升起，小岛沐浴在一片一片红色的朝霞之中
改译
太阳在地平线上撅起一弯唇瓣，向着小岛吹了一口红色的光。
案例4
The fault-finder will find faults in paradise.（Love Your Life）
（注：Flipper是1964年在美国播出的一套长篇电视剧，剧中的主角是一条名叫Flipper的海豚。）
改译
海豚其实是很好色的，比那个费利波[注略]好色多了，据说那些雄性的海豚骚劲上来了连雌性的人都想勾搭呢。
案例2
You hear it all along the river. You hear it, loud and strong, from the rowers as they urge the junk with its high stern, the mast lashed alongside, down the swift running stream. You hear it from the trackers, a more breathless chant, as they pull desperately against the current…（The Song of the River)

商务英语课程讲义 - Lecture 3-打印50

Lecture 3ReadingArticle A - Company structure[1]The chain of commandTraditionally, organizations have had a hierarchical or pyramidal structure, with one person or a group of people at the top, and an increasing number of people below them at each successive level. This is sometimes called line structure. There is a clear chain of command running down the pyramid. All the people in the organization know what decisions they are able to make, who their line manager (or boss) is(to whom they report), and who their immediate subordinates are (over whom they have line authority, and can give instructions to).[2]Functional structureYet the activities of most organizations are too complicated to be organized in a single hierarchy. Most large manufacturing companies, for example, have a functional structure, including, among others, specialized production, finance, marketing, sales, and human resources departments. This means, for instance, that the production and marketing departments can not take financial decisions without consulting the finance department. Large organizations making a range of products are often further divided into separate operating divisions.A disadvantage of functional organization is that people are often more concerned with the success of their own department than that of the company as a whole, so there are permanent conflicts between, say, finance and marketing or marketing and production over what the objectives are.[3]Flattening hierarchies and delegating responsibilityA problem with very hierarchical organizations is that people at lower levels can’t take important decisions, but have to pass on responsibility to their boss. However, the modern tendency is to reduce the chain of command, take out layers of management, and make the organization much flatter. Advanced IT systems have reduced the need for administrative staff and enabled companies to remove layers of workers from the structure. Many companies have also been forced to cut back and eliminate jobs in recessions. Typically, the owners of small firms want to keep as much control over their businesses who want to motivate their staff often delegate decision making and responsibilities to other people.[4]Matrix managementAnother way to get round hierarchies is to use matrix management, in which people report to more than one superior. For example, a product manager with an idea could deal directly with the managers responsible for a certain market segment and for a geographical region, as well as managers inthe finance, sales and production departments. Matrices involving several departments can become quite complex, so it is sometimes necessary to give one department priority in decision making.[5]TeamsA further possibility is to have wholly autonomous, temporary groups or teams that are responsible for an entire project, and are split up as soon as it is successfully completed. But teams are not always very good at decision making, and usually requires a strong leader.Article B - Hard Sell around the Photocopier[1]Sociologists have long recognized that businesses of less than 200 individuals can operate through the free flow of information among the members. Once their size exceeds this figure, however, some kind of hierarchical structure or line management system is necessary to prevent total chaos resulting from failures of communication. Imposing structures of this kind has its costs: information can only flow along certain channels because only certain individuals contact each other regularly;moreover, the lack of personalized contacts means that individuals lack that sense of personal commitment that makes the world of small groups go round. Favors will only be done when there is a clear quid pro quo, an immediate return to the giver, rather than being a matter of communal obligation. Large organizations are less flexible.[2]One solution to this problem would, of course, be to structure large organizations into smaller units of a size that can act as a cohesive group. By allowing these groups to build reciprocal alliances with each other, larger organizations can be built up. However, merely having groups of say, 150 will never of itself be a panacea to the problems of the organization. Something else is needed: the people (involved) must be able to build direct personal relationships. To allow free flow of information, they have to be able to interact in a casual way. Maintaining too formal a structure of relationships inevitably inhibits the way a system works.[3]The importance of this was drawn to my attention a couple of years ago by a TV producer. The production unit for which she worked produced all the educational output for a particular TV station.Whether by chance or by design, it so happened that there were almost exactly 150 people in the unit. The whole process worked very smoothly as an organiztion for many years until they were moved into purpose-built accommodation. Then for no apparent reason, everything started to fall apart. The work seemed to be more difficult to do, not to say less satisfying.[4]It was some time before they worked out what the problem was. It turned out that, when the architects were designing the new building, they decided that the coffee room where everyone ate their sandwiches at lunch times was an unnecessary luxury and so dispensed with it.The logic seemed to be that if people were encouraged to eat their sandwiches at their desks, then they weremore likely to get on with their work and less likely to idle time away. And with that, they inadvertently destroyed the intimate social networks that empowered the whole organization. What had apparently been happening was that as people gathered informally over their sandwiches in the coffee room, useful snippets of information were casually being exchanged. Someone had a problem they could not solve, and began to discuss it over lunch with a friend from another section. The friend knew just the person to ask. Or someone overhearing the conversation would have a suggestion, or would go away and happen to bump into someone who knew the answer a day or so later; a quick phone call and the problem was resolved. Or a casual comment sparked an idea for a new program.[5]It was these kinds of chance encounter in the coffee room, idle chatter around the photocopier, that made the difference between a successful organization and a less successful one.Business Vocabulary in Use- Voc Exercises3.1 Look at the executives and managers listed in A above. Match each task(1-6) with the particular person most likely to be responsible for doing it.1.Meet with advertising agency to discuss new advertisements for thecompany’s holidays.2. Study possible new holiday destinations in detail.3. Analyze last year’s profits in relation to the previous year’s.4. Contact newspaper to advertise new jobs.5. Deal with complaints from customers.6. Discuss sales figures with sales team.3.2 Who’s who on this company board? Look at B above and complete theDiagram.My name’s Maria Montebello, and I’m president and CEO. We have some excellent people on our board, including two who are not involved in day-to-day running of the company: George Gomi and Julia Jones.My name’s Stan Smith and it’s my job to look after the accounts. I work closely with Clarissa Chang and Richard Roberts, as they tell me what their departments need for marketing and research, and I allocate them an annual budget.My name’s Deirdre Dawes and I head up personnel, on the same level in the company as Clarissa Chang and Richard Roberts.1Maria Montebello 2Non-executive director35 6 7。

Lecture 3-final

确实，吸烟危害人们的健康，并且它侵犯了不吸烟者的权力和自由。然而，我认为不应该完全禁止吸烟，原因如下。
We must admit that smoking is detrimental to people’s health. However, the harm that smoking brings to people’s health is exaggerated. 我们必须承认吸烟对人们的健康是有害的。然而，吸烟给人们健康带来的伤害是被夸大了。
结尾段
To sum up, I do not think that smoking should be completely banned. Tobacco industry makes much contribution to the national economy. Smoking brings many psychological benefits to smokers. Moreover, the complete ban of smoking will give rise to many social problems.
理由段 3
We must admit that smoking is detrimental (有害的) to people’s health. However, the harm that smoking brings to people’s health is exaggerated (夸张, 夸大). As long as we smoke in moderation (适度的, 适量的), it will not do great harm to our health. In history, many famous people, such as Sir Winston Churchill, smoked and enjoyed a long life expectancy (长寿).

托福听力TPO11原文 Lecture 3-智课教育旗下智课教育

智课网托福备考资料托福听力TPO11原文 Lecture 3-智课教育旗下智课教育以下是小编为大家整理的托福听力TPO11原文中Lecture 3的文本内容，希望大家能够认真阅读，不懂的地方可以参考翻译，相信会有所收获。

下面就让小编来为大家介绍一下托福听力TPO11原文中Lecture 3的文本内容吧，大家要好好把握，这些都是非常有价值的材料，同时，大家也可以登录智课教育论坛进行TPO练习辅导，希望能够给准备托福听力的同学带来帮助。

TPO11 Lecture 3 Environmental scienceProfessor：When land gets develop for human use, the landscape changes. We don’t see as many types of vegetation, trees, grasses and so forth. This in turn leads to other losses: the loss of animal that once lived there. Err…but these are the obvious changes, but there are also less obvious changes like the climate.One interesting case of this…of…of changes in the local land use causing changes in climate, specifically the temperature is in Florida. Now what comes to mind when you think of the state of Florida?Student A：Sunshine, beaches.Student B：Warm weather, oranges…Professor：Yes, exactly. Florida has long had a great citric industry; large growth of oranges, lemons and the like. Florida’s winter is very mild; the temperature doesn’t often get below freezing. But there are some areas in Florida that do freeze. So in the early 1900s, farmers moved even further south in Florida, to areas that were even less likely to freeze. Obviously, freezing temperatures are danger to the crops. A bad barrier of cold weather, a long spell of frosts could ruin a farm and the entire crop, anyway, before these citric growers moved south, much of the land in south Florida, was what we called wetlands.Wetlands are areas of marshy, swampy land, areas where water covers thesoil, or is present either at or near the surface of the soil for large part of the year. Wetlands have their own unique ecosystem, with plants and animals with special an interesting adaptation. Very exciting, but it’s not what we are talking about today. Emm…where was I?Student A：Farmers moved south?Professor：Oh, yes. Farmers moved south. But the land was not suitable for farming. You can’t grow orange in wetland, so farmers had to transform the wetlands into lands suitable for farming. To do that, you have to drain the water from theland,move the water elsewhere, and divert to the water sources such as rivers. Hundreds of miles of drainage canals were built in the wetlands. Now these areas, the new areas the farmers moved to, used to be warm and unlikely to freeze, however, recently the area has become susceptible to freezes. And we are trying to understand why.Student B：Is it some global temperature change or weather pattern like El Ni?o or something?Professor：Well, there are two theories. One idea is as you suggested that major weather patterns, something like El Ni?o, are responsible. But the other idea and this is the one that I personally subscribe to, is of the changes in the temperature pattern had been brought about by the loss of wetlands.Student A：Well, how would the loss of wetlands make a difference?Professor：Well, think about what we’ve been studying so far. We discussed the impact of landscapes on temperature, right? What affects does the body of water have on an area?Student A：Oh, yeah. Bodies of water tend to absorb the heat during the day, and then they release the heat at night.Professor：Yes, exactly. What you just said is what I want you all to understand. Bodies of water release heat and moisture backinto the environment. So places near large bodies of water are generally milder, err...slightly warmer than those without water. And what I, another think is that the loss of the wetlands has created the situation where the local temperatures in the area are not slightly different, slightly colder than they were 100 years ago, before the wetland were drained.Student B：Emm…do we know what the temperature was like back then?Professor：Well, we were able to estimate this. We have data about South Florida’s current landscape, emm…the plant cover. And we were able to reconstruct data about the landscape prior to 1900. Then we enter those data, information about what the landscape look like before and after the wetlands weredrained.We enter the data into a computer weather model. This model can predict temperatures. And when all the data were entered, an overall cooling trend was predicted by the model.Student B：How much colder does it get now?Professor：Well, actually the model shows a drop of only a few degrees Celsius. But this is enough to cause dramatic damage to crops. If temperatures over night are already very close to the freezing point, then this drop of just a few degrees can take the temperature below freezing. And freezing causes frosts, which kill crops. These damaging frosts wouldn’t happen if the wetlands were still in existence, just as the tiny temperature difference can have major consequences.《环境科学》教授：当土地发展到适应人类使用，景色就发生了变化。

托福听力TPO3学习笔记之lecture3-智课教育旗下智课教育

智课网托福备考资料托福听力TPO3学习笔记之lecture3-智课教育旗下智课教育以下是小编在学习托福听力TPO3中的lecture3时做的学习笔记，针对难词注解、长难句分析以及考题对应考点这三大方面展开，仅供参考。

下面就让小编来为大家介绍一下这篇托福听力TPO3学习笔记的内容吧，这是小编的一些见解，以便帮助大家更加充分的理解TPO真题，希望能够给正在准备托福听力的考友们带来帮助。

一、难词注解preserved adj. 保藏的;腌制的;喝醉的primitive adj. 原始的，远古的;简单的，粗糙的masterpieces n. 杰作;绝无仅有的人speculate vi. 推测;投机;思索rhinoceros n. [脊椎] 犀牛torch n. 火把，火炬;手电筒;启发之物二、长难句分析That would make them not just older than any other cave art, but about twice as old as the art in the caves at Altamira or Lascaux, which you may have heard of.这使得这些壁画不仅比其他洞窟艺术更为古远，更是尔塔米粒和拉斯科洞窟中的艺术作品年代的两倍。

你可能听过这两个洞窟的名字。

阿难词注解：Altamira or Lascaux 尔塔米粒和拉斯科难句类型：than 比较级，以及定语从句难句拆分：than 比较级的运用，twice as old as句式的运用，最后是which引导的定语从句修饰Altamira or Lascaux三、考题对应考点6.开头原则之直入主题式，(原文中)Some of the world’s oldest preserved art is the cave art of Europe, most of it in Spain andFrance. And the earliest cave paintings found to date are those of the Chauvet Cave in France discovered in 1994.Pro的第一句话就揭示了他这节课的主题是ealiestcave也就是A选项中的同义转换，所以答案选A7.举例原则，提到他女儿的例子是要引起大家的注意，(原文中)And you know, I remember when I heard about the results of the dating of the Chauvet paintings, I said to my wife, ―Can you believethese paintings are over 30,000 years old?‖ And my3-yearold daughter piped up and said, ―Is that older than my great-grandmother?‖ That was the oldest age she knew.那么从pro 描述他听到dating的消息以及家人的反映可以看出，这是为了体现这cave painting的年代久远,所以选D8.转折原则，but后面的是重点I mean, we tend to think that people who lived at that time must have been pretty primitive. But I’m gonna show you some slides in a few minutes and I think you will agree with me that this art is anything but primitive. They are masterpieces. Pro先说人们对于那时的人的映像是十分原始的，从but后转折的内容anything but primitive. They are masterpieces. 可以看出PRO给予了极高的肯定也就是It is extremely well done.所以选择A9.结果原则And people did go see the art. There are charcoal marks from their torches on the cave walls clearly dating from thousands of years after the paintings were made. So we can tell people went there.也就是说这些marks意味着人们在这些作品完成很久之后，去看了这些洞内的作品,所以答案选B10.转折原则，"But earlier at Chauvet, there is a significant interest in large dangerous animals, lots of rhinoceros, lions, mammoth, bears." 问的是作品中涉及的动物具有怎样的特别之处，在听的时候要特别注意but后面的内容，所以答案是"Many of them are dangerous"，也就是B11.问答原则，D选项出自"Deep inside a cave like that is not really a place you’d want to stay, so, why? What inspired thePaleolithic artists to make such beautiful art in such inaccessible places? "C选项出自"why these preciseanimals?"，根据同义转换，可知答案为CD。

托福听力TPO12原文 Lecture 3-智课教育旗下智课教育

智课网托福备考资料托福听力TPO12原文 Lecture 3-智课教育旗下智课教育下面就让小编来为大家介绍一下托福听力TPO12原文中Lecture 3的文本内容吧，大家要好好把握，这些都是非常有价值的材料，同时，大家也可以登录智课教育论坛进行TPO练习辅导，希望能够给准备托福听力的同学带来帮助。

TPO 12 Lecture 3 Music historyProfessor：The word opera means work, actually it means works. It’s the plural of the word opus from the Latin. And in Italian it refers in general to works of art. Opera Lyric or lyric of opera refers to what we think of as opera, the musicaldrama.Opera was commonplace in Italy for almost thousands of years before it became commercial as a venture. And during those years, several things happened primarily linguistic or thematic and both involving secularization.Musical drama started in the churches. It was an educational tool. It was used primarily as a vehicle for teaching religion and was generally presented in the Latin, the language of the Christian Church which had considerable influence in Italy at that time. But the language of everyday life was evolving in Europe and at a certain point in the middle ages it was really only merchants, Socratics and clergy who can deal with Latin. The vast majority of the population used their own regional vernacular in all aspects to their lives. And so in what is now Italy, operas quit being presented in Latin and started being presented in Italian. And once that happened, the themes of the opera presentations also started to change. And musical drama moved from the church to the plaza right outside the church. And the themes again, the themes changed. And opera was no longer about teaching religion as it was about satire and about expressing the ideas of society your government without committing yourself to writing and risking imprisonment or persecution, or what have you.Opera, as wethink of it, is of course a rather restive form. It is the melodious drama of ancient Greek theater, the term ‘melodious drama’being shortened eventually to ‘melodrama’ because operas frequently are melodramatic, not to say unrealistic. And the group that put the first operas together that we have today even, were, they were…well…it was a group of men that included Gallo Leo’s father Venchesil, and they met in Florence he and a group of friends of the counts of the party and they formed what is called the Camarola Dayir Bardy. And they took classical theater and reproduced it in the Renaissance’s time. This…uh…this produced some of the operas that we have today.Now what happened in the following centuries is very simple. Opera originated in Italy but was not confined to Italy any more than the Italians were. And so as the Italians migrated across Europe, they carried theater with them and opera specifically because it was an Italian form. What happened is that the major divide in opera that endures today took place. The French said opera auto-reflect the rhythm and Kevin of dramatic literature, bearing in mind that we are talking about the golden age in French literature. And so the music was secondary, if you will, to the dramatic Kevin of language, to the way the rhythm of language was used to express feeling and used to add drama and of course as a result instead of arias or solos, which would come to dominated Italian opera. The French relied on that what is the Italian called French Word 1 or French Word 2 in English. The lyrics were spoken, frequently to the accomp**nt of a harpsichord.The French said you really cannot talk about real people who lived in opera and they relied on mythology to give them their characters and their plots, mythology, the past old traditions, the novels of chivalry or the epics of chivalry out of the middle Ages. The Italian said, no this is a great historical tool and what a better way to educate the public about Neo or Attallaor any number of people than to put them into a play they can see and listen to. The English appropriated opera after the French. Opera came late to England because alltheaters, public theaters were closed, of course, during their civil war. And it wasn’t until the restoration in 1660 that public theaters again opened and opera took off. The English made a major adjustment to opera and exported what they had done to opera back to Italy. So that you have this circle of musical influences, the Italians invented opera, the French adapted it, the English adopted it, and the Italians took it back.It came to America late and was considered to elites for the general public. But Broadway musicals fulfilled a similar function for a great long while. George Champon wrote about opera, “If an extraterrestrial being or two appear before us and say, what is your society like, what is this Earth thing all about, you could do worse than take that creature to an opera.” Because opera does, after all, begin with a man and a woman and any motion.《音乐史》教授：“歌剧”这个词的意思是“作品”。

lecture 3佛经翻译理论下

11
❖之谦 ❖“恭明前译，颇丽其辞，仍迷其
旨，是使宏标乖于谬文，至味淡于华艳。”
12
彦琮其人
❖彦琮，俗姓李，出身士族，赵郡柏人。（今河南）十岁出家，通梵语。隋初开皇三年（583），开始参加译经。开皇十二年，奉诏入京，居大兴善寺主持译经。至大业二年，又去东都洛阳，进上林园翻译馆，仍从事整理与翻译佛经。
9
❖胡音失者，正之以天竺。秦名谬者，定之以字义。不可变者，即而书之。是以异名斌然，胡音殆半。斯实匠者之公谨，笔受之重慎也。幸冀遵实崇本之贤，推而体之，不以文朴见答、烦异见慎也
10
对于前人的批评 ❖鸠摩罗什
❖“法师于秦语大格，唯译一往；方言殊好，犹隔而未通。苟言不相喻，则情无由比；不比之情，则不可以托悟怀于文表；不喻之言，亦何得委殊于一致？理固然矣！”
”若玄奘者，则意译直译，圆满调和，斯道之极轨也。“－－梁启超
27
玄奘译场的翻译职司
译主
证义
证文
全场主脑
译主助手
或称证梵本
28
玄奘译场的翻
整理译文
29
玄奘译场的翻译职司
参译
校勘
刊定
润文
润饰译文
30
玄奘译场的翻译职司
梵呗
唱念
监护大使
监阅译经
31
玄奘之五不翻
❖其一为秘密故，如“陀罗尼”（直言，咒语）。其二为含多义故，如“薄伽”，梵具六义（自在，炽盛，端严，名称，吉祥，尊贵）。其三为无此故，如“阎浮树 ”（胜金树），中夏实无此木。其四为顺古故，如“阿耨nòu菩提” （正偏知），非不可翻，而摩腾以来，常存梵音。其五为生善故，如“般若” 尊重，“智慧”迷浅。
wwwthemegallerycom彦琮的翻译思想?宁贵朴而近理?宁贵朴而近理不用巧而背源不用巧而背源wwwthemegallerycom辩证论之八备1诚心爱法志愿益人不惮久时2将践觉场先牢戒足不染讥恶3筌qun晓三藏义贯两乘不苦暗滞wwwthemegallerycom辩证论之八备4旁涉坟史工缀典词不过鲁拙5襟抱平恕器重虚融不好专执6耽dn于道术澹于名利不欲高衒wwwthemegallerycom辩证论之八备7要识梵言乃闲正译不坠彼学8薄阅苍雅粗谙篆隶不昧此文wwwthemegallerycom佛经翻译第二阶段特点翻译理翻译理论和技论和技巧研究上有所进步单单一原本转为本转为多种便于相互校勘原私译转私译转为官译为官译个人翻译转为集体翻译123wwwthemegallerycom进步互校勘译wwwthemegallerycom佛经翻译第三阶段618906背景介绍1阶段特点阶段特点2wwwthemegallerycom代表人物3玄奘其人?玄奘通称三藏法师姓陈名祎洛州人今河南

1《口译》课件--Lecture 3--熊萍萍

E
C
Checking the Previewing (3)
• I‟d like to have a 7 o‟clock morning call, breakfast sent up to my room, laundry done, some documents photocopied, an express mail sent out, and something like that. • 我要早上7点钟叫醒，早餐送到我房间，衣服要洗熨，文件要复印，邮件要快递，诸如此类的事情需要服务。 • 我在你们的酒店预定了一间双人间，有确认函。 • I have made a reservation for a double room with your hotel. And here‟s the confirmation letter. • 提前10天预定房间可以享受8折优惠价。 • You will have a good rate with 20% off when you make a reservation 10 days in advance. • 我们有许多现代化的健身设备可满足客人的不同需求。 • We have many modern fitness facilities catering to the different needs of our guests.
美国第四大投资银行雷曼兄弟公司宣布破产而美国第三大投资银行美林公司则被美国银行收购这些都引发了投资者的强烈担忧全球主要股市因此纷纷大跌
Interpreting Course
Lecture 3
Ⅰ. Active Listening (2) Ⅱ. Interpreting for Reception Service (2)