Temporal Correlation of Hard X-rays and MeterDecimeter Radio Structures in Solar Flares
南方平差易闭合导线数据处理流程

南方平差易闭合导线数据处理流程1.首先,需要将导线的测量数据输入计算机软件中进行处理。
First, the measurement data of the closed-loop traverse needs to be input into the computer software for processing.2.然后,对测量数据进行精确的检查和校正,确保数据的准确性和完整性。
Next, the measurement data needs to be carefully checked and corrected to ensure the accuracy and completeness of the data.3.接着,需要进行数据的平差计算,利用最小二乘法等数学方法对数据进行处理。
Then, the data needs to be adjusted through the least squares method and other mathematical techniques.4.在进行平差计算时,需要考虑各种误差来源,包括观测误差、仪器误差和环境条件等因素。
During the adjustment process, various sources of errors need to be taken into account, including observation errors, instrument errors, and environmental conditions.5.进行平差计算后,需要进行闭合差检查,确保闭合差符合规定的精度要求。
After the adjustment, it is necessary to check theclosing error to ensure that it meets the required accuracy.6.如果闭合差超出了允许的范围,需要进行进一步的调整和处理,直至闭合差满足要求。
The Infrared-X-ray continuum correlation in Active Galactic Nuclei
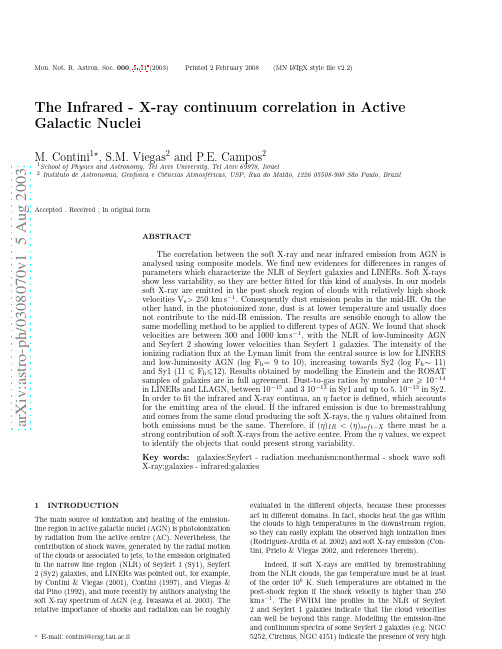
a r X i v :a s t r o -p h /0308070v 1 5 A u g 2003Mon.Not.R.Astron.Soc.000,1–11(2003)Printed 2February 2008(MN L A T E X style file v2.2)The Infrared -X-ray continuum correlation in ActiveGalactic NucleiM.Contini 1⋆,S.M.Viegas 2and P.E.Campos 21School of Physics and Astronomy,Tel Aviv University,Tel Aviv 69978,Israel 2Instituto de Astronomia,Geof ´isica e Ciˆe ncias Atmosf´e ricas,USP,Rua do Mat˜a o,122605508-900S˜a o Paulo,BrazilAccepted .Received ;In original formABSTRACTThe correlation between the soft X-ray and near infrared emission from AGN isanalysed using composite models.We find new evidences for differences in ranges of parameters which characterize the NLR of Seyfert galaxies and LINERs.Soft X-rays show less variability,so they are better fitted for this kind of analysis.In our models soft X-ray are emitted in the post shock region of clouds with relatively high shock velocities V s >250km s −1.Consequently dust emission peaks in the mid-IR.On the other hand,in the photoionized zone,dust is at lower temperature and usually does not contribute to the mid-IR emission.The results are sensible enough to allow the same modelling method to be applied to different types of AGN.We found that shock velocities are between 300and 1000km s −1,with the NLR of low-luminosity AGN and Seyfert 2showing lower velocities than Seyfert 1galaxies.The intensity of the ionizing radiation flux at the Lyman limit from the central source is low for LINERS and low-luminosity AGN (log F h =9to 10),increasing towards Sy2(log F h ∼11)and Sy1(11 F h 12).Results obtained by modelling the Einstein and the ROSAT samples of galaxies are in full agreement.Dust-to-gas ratios by number are 10−14in LINERs and LLAGN,between 10−15and 310−13in Sy1and up to 5.10−13in Sy2.In order to fit the infrared and X-ray continua,an ηfactor is defined,which accounts for the emitting area of the cloud.If the infrared emission is due to bremsstrahlung and comes from the same cloud producing the soft X-rays,the ηvalues obtained from both emissions must be the same.Therefore,if (η)IR <(η)soft −X there must be a strong contribution of soft X-rays from the active centre.From the ηvalues,we expect to identify the objects that could present strong variability.Key words:galaxies:Seyfert -radiation mechanism:nonthermal -shock wave soft X-ray:galaxies -infrared:galaxies1INTRODUCTIONThe main source of ionization and heating of the emission-line region in active galactic nuclei (AGN)is photoionization by radiation from the active centre (AC).Nevertheless,the contribution of shock waves,generated by the radial motion of the clouds or associated to jets,to the emission originated in the narrow line region (NLR)of Seyfert 1(Sy1),Seyfert 2(Sy2)galaxies,and LINERs was pointed out,for example,by Contini &Viegas (2001),Contini (1997),and Viegas &dal Pino (1992),and more recently by authors analysing the soft X-ray spectrum of AGN (e.g.Iwasawa et al.2003).The relative importance of shocks and radiation can be roughly⋆E-mail:contini@ccsg.tau.ac.ilevaluated in the different objects,because these processes act in different domains.In fact,shocks heat the gas within the clouds to high temperatures in the downstream region,so they can easily explain the observed high ionization lines (Rodriguez-Ardila et al.2002)and soft X-ray emission (Con-tini,Prieto &Viegas 2002,and references therein).Indeed,if soft X-rays are emitted by bremsstrahlung from the NLR clouds,the gas temperature must be at least of the order 106K.Such temperatures are obtained in the post-shock region if the shock velocity is higher than 250km s −1.The FWHM line profiles in the NLR of Seyfert 2and Seyfert 1galaxies indicate that the cloud velocities can well be beyond this range.Modelling the emission-line and continuum spectra of some Seyfert 2galaxies (e.g.NGC 5252,Circinus,NGC 4151)indicate the presence of very high2M.Contini,S.M.Viegas,and P.E.Camposshock velocities which can provide a thermal origin for the observed soft X-rays.Based on early observations of different galaxies by EX-OSAT,the nature and variability of soft X-rays was investi-gated,revealing a low and long term variability compatible with bremsstrahlung emission from a hot gas.For instance, Pounds et al.(1986)showed the existence of a soft X-ray spectral component,distinct from the main(nuclear)X-ray emission of NGC4151by a lack of variability.This compo-nent is shown to be consistent with thermal emission from a hot,confining medium in the region of the narrow emission-line clouds.Perhaps NGC4151is an exception to the general rule that soft X-ray emission from AGNs is variable,because most of its soft X-rayflux is absorbed by a high intrinsic column density,so that its faint,diffuse X-rayflux can be detected.This effect should not appear in most objects.The correlation between the soft X-ray and infrared lu-minosities in AGN has been studied by several authors and is controversial.One important issue being the source of vari-ability in the various frequency ranges.In particular,the correlations of L X(0.5-4.5keV)versus L IR for the IRAS 12µm,25µm,60µm,and100µm bands have been exam-ined by Green et al.(1992).They claim that L X versus L25µm and L X versus L100µm resemble very closely the L X versus L60µm correlation and that the plot of L X versus L12is considerably better defined,yet,just as in the L60µm plot,”Seyfert galaxies still show no significant correlation”(Green et al.,1992,Fig.3)On the other hand,the correlation between the 10µm and the hard X-ray emission showed opposite results (Carleton et al.1987and Giuiricin et al.1994)and was reanalysed by Giuricin,Mardirossian,&Mezzetti(1995). They obtained a weak correlation with a harder(2to10 keV)X-ray emission,mainly induced by Seyfert2data. They also found that the10µm emission correlates well with the12µm and25µm,and less well with the far-infrared emis-sion at60µm and100µm.These authors favor thermal emis-sion as the origin of the mid-infrared emission of Seyfert galaxies.Dust is present in the NLR clouds and re-emission by grains may dominate the IR domain.If the intense UV-optical central radiation heating the nuclear dust has an X-ray counterpart,a correlation between the infrared and X-ray emission should be expected(Barvainis1990).Be-cause in shocked zones the dust grains are usually heated to higher temperatures than in photoionized regions,the con-tribution from the shocked NLR clouds probably dominate the near infrared emission.A contribution to the IR from bremsstrahlung emission from a cooler gas is not excluded and would result in a correlation between the the soft X-ray and the near IR continua.Nevertheless,in the former case the mutual heating of dust and gas(Viegas&Contini1994) can also lead to a correlation between those two continua. Therefore,in both cases a correlation is expected.Notice that the contribution of a dusty torus to the IR emission suggested by the AGN unified model(Antonucci&Miller 1985)will give poor or no correlation with the soft X-ray emission as pointed out by Green et al.(1992)(see also Con-tini,Viegas,&Prieto2003and references therein).A major problem concerning correlations between X-ray and IR emission is X-ray variability.It is well established that soft X-rays from low luminosity AGN(LLAGN)and LINERs show less and/or longer variability than the hard X-rays(Halderson,Moran,&Filippenko2001,Terashima, Ho&Ptak2000).Following Green et al.(1992),we will, therefore,analyse the possible correlation between mid-IR ( L12)and X-rays for these galaxies,which present a bolo-metric luminosity that can be several orders of magnitude lower than the brightest AGN.Notice that10µm falls in the absorption trough of the spectral energy distribution due to silicate grains.On the other hand,PAH emission bands dominate the infrared SED of galaxies at6.2,7.7,8.6,11.3,and12.7µm(Spoon et al 2002).However,PAH grains are small compared with silicate grains and easily sputtered in AGN,where shock velocities are high enough( 300km s−1).Therefore,we refer in this paper to the12µm emission in the mid-IR.It was found in previous investigations on the Seyfert galaxies,NGC5252(Contini,Prieto,&Viegas1998a),Circi-nus(Contini,Prieto,&Viegas1998b)and NGC4151(Con-tini,Viegas,&Prieto2002),that the critical shock velocity (V s)to produce dust emission in the mid-IR is of the order of500km s−1(Viegas&Contini1994),and that the crit-ical V s for soft X-ray emission,fitting most of our sample for Seyfert2galaxies,is about1000km s−1(Contini&Vie-gas2000).However,the dust-to-gas ratio,d/g,may change from galaxy to galaxy,as well as in the different regions of a single galaxy.Therefore a scattering of the infrared data for a sample of galaxies is expected.The FWHM of the line profiles is lower in LINERs(< 300km s−1)than in Seyfert galaxies(300to500km s−1). Notice,however,that broad Hαlines have been observed from LINERs(Ho,Filippenko,&Sargent1997),indicating that clouds with higher velocities may also contribute to the spectra.Therefore,in these objects,characterized by a low ionizingflux from the AC,shocks are relatively important and may contribute to both the near-IR and soft X-rays emission.The observed data will be confronted to model results obtained by numerical simulations with the SUMA code(Viegas&Contini1994,Contini&Viegas2001),which accounts for both the shock and photoionization effects in the NLR clouds.Once tested for the low-luminosity objects,in the sec-ond part of this paper we will apply the same kind of anal-ysis to Seyfert galaxies.We will restrict the data to the soft X-ray part of the spectrum,where the variability is not as strong as in the hard X-rays.From the comparison with model results we expect to select those galaxies where the contribution to soft-X rays from the AC prevails,indicating in this way the objects of the sample which should show a higher degree of variability.The spectral energy distribution(SED)of dust and gas emissions obtained from the models,as well as the corre-sponding temperatures of the dust grains are discussed in Sect.2.The L X- L12correlation for LLAGN and LINERs is investigated in Sect.3,while that for Seyfert galaxies is discussed in Sect.4.Concluding remarks appear in Sect.5. 2GAS AND DUST EMISSIONS2.1The modelIn our model the nuclear clouds are moving outward from the AC.A shock front appears at the cloud outer edge,whileThe Infrared -X-ray continuum correlation in AGN3Figure 1.The SED of the continua emitted by bremsstrahlung and by dust from the clouds.Long-dashed lines refer to V s =500km s −1,logF h =12;short dashed lines to V s =300km s −1,logF h =11.Solid lines refer to V s =1000km s −1.Thick lines rep-resent RD models,thin lines the SD models.The vertical lines correspond to the main IR observed wavelengths.radiation from the AC reaches the inner edge.The physical conditions of a emitting cloud due to the coupled effect of shock and photoionization by a radiation source are obtained with the SUMA code assuming a plan-parallel symmetry.The calculations start at the shocked edge.In the first run,only the shock effect is accounted for,and the physical con-ditions are obtained for several ( 300)slabs before reaching the opposite edge.In this first run the results correspond to a shock-dominated model.Iterative runs alternatively start at the photoionized and shocked edges,accounting for both effects,until convergence of the physical conditions in the cloud is achieved.The input parameters for a single-cloud model are the shock velocity,V s ,the preshock density,n 0,the preshock magnetic field,B 0,the ionizing radiation spectrum,the chemical abundances,the dust-to-gas ratio by number,d/g,and the geometric thickness of the clouds,D.A power-law is adopted for the central ionizing radiation spectrum reach-ing the cloud,characterized by the flux F h ,at the Lyman limit (in units of cm −2s −1eV −1)and the power law index α(F h ∝ν−α).For all the models,B 0=10−4gauss,αUV =1.5and αX =0.4,and cosmic abundances (Allen 1973)are adopted.The model is either radiation dominated (RD)or shock dominated (SD)depending on the intensity of the ionizing flux and on the shock velocity (Viegas-Aldrovandi &Contini 1989).For SD models F h =0.The gas entering the shock front is thermalized and heated.Depending on the shock velocity,a high tempera-ture zone can be produced where soft X-rays are generated by bremsstrahlung.A lower temperature zone may produce near infrared emission by the same mechanism.The tem-perature of the photoionized zone is never too high (<4.×104K),thus its bremsstrahlung emission is mainly in the IR-optical range,depending on the intensity of the ioniz-Table 1.Model results :the temperature of grains-100010000.5318.-113003000.5154.55.2-100010000.2147.-113003000.2165.55.8-100010000.01--113003000.01168.69.712.73003000.01168.112.4M.Contini,S.M.Viegas,and P.E.CamposFigure2.The ratio of the12µluminosity to the soft X-ray luminosity versus the soft X-ray luminosity for the low-luminosity active galaxies of the Halderson et al(2001)sample.than in the SD zone,even for small grains(a gr=0.01µm) and high F h(51012cm−2s−1eV−1).On the other hand, dust is destroyed by sputtering if the initial grain radius is low and V s is high,as exemplified by the model with a gr= 0.01µm V s=1000km s−1.In brief,if the mid-infrared emission of AGN,particu-larly the12µemission,is thermal emission due to grains, the grains must be heated in a shocked zone.3LOW LUMINOSITY AGNThe correlation between the near IR and the soft X-rays for LLAGN is analysed using36nearby objects from the Halderson et al.(2001)sample.Most of the X-rays data come from observations with ROSAT while the infrared data from IRAS.Some of the objects are also included in the Terashima et al.(2002)sample of LLAGN observed with ASCA.Short term variability( 1day)was found in NGC 3031,NGC4258and NGC5033.Long-term variability is observed in NGC3031,NGC4258and NGC4579,while no significant variability was found in NGC404,NGC4111, NGC4438,NGC4579and NGC4639.The correlation between the12µm and soft X-rays for the LLAGN is shown in Fig.2,where we plot the ratio of the IR and X-rays observedfluxes versus the X-ray luminosity. The choice of the vertical axis is explained below,where we compare the observational data with the results of numerical simulations.3.1Modelling the sampleThe single-cloud model provides the continuum and emission-linefluxes calculated at the cloud.Thus,in order to obtain the luminosities,the model results must be mul-tiplied by a factorηaccounting for the emitting area.As-suming that the single cloud conditions correspond totheFigure3.The comparison of model calculations with the obser-vational data.The results of the models with different values of V s and the same log F h are joined by dotted(log F h=9),short-dashed log F h=10),long-dashed(logF h=11)and dash-dotted (logF h=12)lines.Solid lines correspond to shock dominated(SD) models calculated with F h=0Thin lines refer to bremsstrahlung emission at12µm,while thick lines refer to dust emission at12µm.The values of V s increases from left to right.gas around the central source,the emitting area can be re-lated to the distance of the cloud to the central source and to the covering factor(see,for instance,Contini&Viegas 2000).Plotting aflux ratio versus a luminosity,as in Fig.2, this factor will affect only the horizontal axis,and the match of the models to the data would be easier,if IR and X-ray emission come from the same cloud and there is a negligible contribution of X-rays from the central source.Since we assume that the soft X-rays come from the shocked edge of the NLR clouds,the post-shocked zone must reach temperatures higher than105K and a mini-mum shock velocity of300km s−1is required.Based on previous works,a grid of models with V s=300km s−1and n0=300cm−3,V s=500km s−1and n0=300cm−3,V s=700 km s−1and n0=500cm−3,and log F h=9,10,11,12was ob-tained for clouds with a dust-to-gas ratio of10−14,a geo-metrical width of D=1019cm,a pre-shock magneticfield of B0=10−4gauss and initial grain radius of0.2µm.Notice that log F h=12is an upper limit since a low radiationflux from the AC is characteristic of LLAGN.Model results and observational data are compared in Figure3.The lines join results of models with different val-ues of V s but the same F h.The observations of the galaxies NGC4486,NGC4579, and NGC4051are better explained by SD models(thin solid line joining the results for V s=900,1000,and1100 km s−1and d/g=10−13).Only the bremsstrahlung is shown in this case,because at these high velocities the grains are mostly destroyed by sputtering.The F12µm/F X ra-tios for SD models calculated with V s=300,500,and700 km s−1and d/g=10−14are too low.Higher values(thick solid line)are obtained assuming d/g=2×10−13.The Infrared-X-ray continuum correlation in AGN5 Table2.The characteristics of LLAGN404L250030011 1. 1.51052L1.9500-700300-50010 1.15.51068S1.970050011 1.23.62841L25003009 1.15.53031S1.5700500SD20. 2.43379L23003009br.15.53982S1.9500-700300-50010-12 1.-br.15.54051S1.21000500SD br.15.54168S1.950030010br.15.54203L1.9500-700300-5009br.>15.54258S1.950030012 1.8.94278L1.9500-700300-50010br.15.54293L2300-50030010 1.15.54314L2300-50030010br.15.54374L2500-700300-5009 1.15.54388S1.9500-700300-50010 1.15.54395S1.8500-700300-50011 1. 1.554438L1.9500-700300-5009 1.15.54450L1.9500-700300-50010br.15.54472S2500-700300-5009 1.15.54477S2500-700300-5009 1.15.54486L2900500SD br.8.94494L250030010br.15.54501S2500-700300-50010 1.15.54550L2>500>3009br.15.54565S1.9>500>30010-12 1.15.5-8.94579S1.91000500SD br.8.94636L1.9<700<5009 1.15.54639S1.0500-700300-5009 1.15.54651L250030012br.15.54698S2>500>3009 1.15.54725S2>500>3009 1.15.54736L2>500>30012 1.8.94762L2500-700300-5009 1.15.55194S2>500>30012br15.55195L250030012 1.8.96M.Contini,S.M.Viegas,and P.E.Campos Figure 5.The calculated radio continuum for NGC4374, NGC4278,and NGC4258.The two components are shown: the power-law due to Fermi mechanism(thin solid line)and the bremsstrahlung from a cool gas(dotted line)The thick solid line corresponds to the sum of the two components.chrotron radiation created by the Fermi mechanism at the shock front and the bremsstrahlung from the cooler cloud zones.A few examples appear in Fig.5,showing model re-sults and observational data for the galaxies NGC4374 (top panel,NGC4278(middle panel),and NGC4258(bot-tom panel).The model input parameters are listed in Table 2.The observational data were found in the NASA Extra-galactic Data(NED))and come from Laing&Peakock1980, Kuhr et al.1981,Kellermann et al1969,Gower et al.1967, Wright&Otrupcek1990),Douglas et al.1996,Ekers1969, Large et al.1981,Heeschen&Wade1964,White&Becker 1992,Waldram et al.1996,Becker et al.1995,Condon et al. 1983,Dressel&Condon,1978,Becker et al.1991,Gregory &Condon1991,Israel&Mahoney1990,Ficarra et al.1985. 4THE L X- L12CORRELATION FORSEYFERT GALAXIESIn this section the correlation between the soft X-ray lumi-nosity(L X)and the12µm luminosity( L12)is investigated for a relatively large sample of Seyfert galaxies which in-cludes objects with higher luminosities relative to those of LINERS and LLAGNs studied in the previous sections.We have collected a sample of31Sy1and19Sy2ob-served with the Einstein(0.5-4keV band)and IRAS satel-lites.Another sample includes26Sy1,8Sy1.5and10Sy2 observed with ROSAT(0.5-2.4keV).The Einstein data come from Green,Anderson&Ward(1992),the ROSAT data were taken from Moran,Halpern&Helfand(1996).The IRAS data come from the NED.The luminosities are calcu-lated assuming a Hubble constant equal to50km s−1Mpc−1.The correlation between L12/L X and L X for Sy1and Sy2galaxies are shown in Fig.6using Einstein(top panel) and ROSAT data(bottom panel),respectively.For com-parison,the LLAGN data(Helderson et al.2001)are also plotted.As expected the LLAGn are fainter in X-rays than the Seyfert galaxies.As expected,NGC1068,included in the Halderson et al.sample,is located in the Seyfert galaxy zone.The separation between the two classes of objects is more clearly marked for ROSAT data.The L12/L X ratios are roughly of the same order.These ratios depend mainly on the dust-to-gas ratio and on the physical conditions in the NLR,indicating that the conditions are similar in the two classes of galaxies.The same series of models(same notations)applied to the LLAGN in Fig.3are used to roughly model the Seyfert galaxies.In order tofit the data for the Einstein sample,we adopted anηcorresponding to an average distance of the clouds from the AC of∼460pc for Sy1and∼90pc for Sy2.This corresponds to shifting the model ensemble to the right hand side in the diagram.However,tofind the best fit for Sy2we had to shift the models upwards by a factor of30.The vertical-axis corresponds to the L12/L X ratio, where L12represents dust emission.Therefore,the bestfit corresponds to dust-to-gas ratios higher by a factor of30 (d/g=3×10−13)and already suggests that the NLR of Sy2 is dustier than that of Sy1.The same parameters used for the Einstein sample are adopted tofit the ROSAT sample (Fig.6bottom).Notice that Mrk335,NGC1365,and NGCThe Infrared-X-ray continuum correlation in AGN7 Table3.The logarithm of theηfactors using the Einstein dataSy1RD43.844.2-747.-thick long-dashedSy1SD42.6-46.0187.6251.thin short-dashedSy1RD44.1-45.21055. 1.26thick short-dashedSy2SD40.1-44.510.52512.thin short-dashedSy2SD40.1-45.310.5 1.58(4)thin dottedSy2RD43.3-44.8420. 3.16thick short-dashedSy2RD43.3-45.3420.10.0thick dottedtype model X-ray1IR(br.)IR(dust)r(pc)d/g(10−14)line type1ROSAT data1566from the Einstein sample,showing low L X,should be classified as Sy2rather than Sy1.Thefit shown in Fig.6is reasonable,although more refined modelling is necessary.This will be done in the next section,where the NLR physical conditions,as well as the evaluation of the distances of the Sy1and Sy2emitting clouds to the center,are discussed.For this,the L12ver-sus L X correlation is used for both Sy1and Sy2galaxies (Fig.7and8,respectively,corresponding to the Einstein and ROSAT data).4.1Modelling the correlationSingle-cloud models that better reproduce the L IR-L X cor-relation shown in Figs.7and8are chosen from a grid of models.All models are calculated with d/g=10−15by num-ber,B0=10−4gauss,and n0=300,500,and1000cm−3for models with V s=300,500,and1000km s−1,respectively (cf Fig.1).Usually in the NLR the decreasing radiationflux with the distance to the AC is followed by a decreasing ve-locityfield.Moreover,we adopted a low dust-to-gas ratio as a lower limit,in order tofind out the whole range of d/g values for both Sy1and Sy2.The theoretical results are represented by lines,labelled by the input parameters V s for SD models(thin lines),and the pair(V s,log F h)in the case of RD models(thick lines). Short dashed lines refer to dust emission in the IR,while long dashed lines to IR bremsstrahlung.Fitting of the observed correlation with our models is not so simple.First,recall that models correspond to single clouds(see Sect.3.2).Thus,the comparison between the theoretical and observational data can only give a rough indication of the physical conditions of the gas and of the velocityfield of the clouds emitting soft X-rays.Second,as already commented above(Sect.3.1),in order to derive the luminosities,thefluxes calculated by the models must be multiplied by a factor(η)which represents the fraction of the observed luminosity coming from a specific type of cloud, characterized by V s,n0and F h.If the infrared emission is due to dust,this factor must also account for the differences of the dust-to-gas ratio assumed in the calculations and the real value for each galactic nucleus.A higher d/g value leads to an enhancement of the L IR emission.Thus,we expect that theηfactor is not constant but may have different values for different galaxies.It could be empirically obtained byfitting the observed emission-line and continuum spectra with a multi-cloud model,as already presented for Circinus and NGC5252(Contini et al.1988b,a).Since our goal is to analyse the L12-L X correlation we try to obtain a rough picture of the physical processes in the active nuclei with a minimum number of input parameters. First we constrain the models by considering the main trends shown by the data,because these trends define which type of models must be adopted(RD,SD,IR from dust or IR from bremsstrahlung).Then,we shift the model results in order to cover the largest number of data by changing theηfactor.Recall that in the SED presented in Fig.1,the high8M.Contini,S.M.Viegas,and P.E.CamposFigure 6.Top panel : L 12/L X versus L X for Sy1(filled cir-cles)and Sy2(filled triangles)galaxies using Einstein data.Bot-tom panel : L 12/L X versus L X for Sy1(open circles)and Sy2(open triangles)galaxies using ROSAT data.In both panels,filled squares represent the LLAGN and the lines correspond to model results with the same notation used in Fig.3.frequency domain corresponds to the hot gas emission,i.e.,even in radiation dominated models it depends on the shock velocity.So,the soft X-ray emission is due to bremsstrahlung in the shocked zone of high velocity clouds while its infrared counterpart can contribute to the mid-or near-IR emission.In this case,since both continua come from the same cloud,the corresponding ηshould be the same.The logarithm of ηfor the bremsstrahlung and for dust emission,adopted for modelling the correlations presented in Figs.7and 8,are given in Tables 3and 4for Einstein and ROSAT data,respectively.In the first column of Tables 3and 4the type of the galaxy is given.In the ROSAT sample,Sy1includes the Sy1.5data.The model type (SD or RD)isindicated in the second column.The logarithm of the ηvalue which provides the best fit of the data by the corresponding model (see line type in column 8)are given in columns 3,4,and 5for L X , L 12generated by bremsstrahlung,and L 12by dust,respectively.In columns 6and 7the calculated distance of the emitting clouds from the AC (assuming a covering factor equal to unity)and the d/g ratio by number are given,respectively.The values of d/g by number which better fit the data are calculated from the difference between ηcorresponding to IR(dust)and ηcorresponding to X-ray for each type of galaxies in Tables 3and 4.The line type used to draw the curves corresponding to the models (Figs.7and 8)are listed in the last column.4.2Seyfert 1galaxiesTwo clear trends are present in Fig.7(top panel)corre-sponding to the Sy1Einstein sample:(a)Sy1with L X 3×1043show a larger scatter of the L 12values;(b)the ob-jects with higher L X values are mainly located between RD (thick short-dashed line)and SD (thin short-dashed line)models both with IR due to dust emission .The results cor-responding to L 12due to bremsstrahlung (thick long-dashed line)could eventually explain some Sy1and Sy2data.Regarding the ROSAT data (Fig.7,bottom panel),most of the data are also inside a sector defined by RD mod-els (the low edge)and SD models (upper edge),as found for the Einstein sample.High L 12Sy1galaxies are bet-ter fitted by dust emission from SD models with 300<V s 1000km s −1(thin line),whereas those with low L 12would be dominated by dusty RD clouds with F h =1011−12(cm −2s −1eV −1)and V s in the range 300-500km s −1.Also for the ROSAT data,the line corresponding to the IR bremsstrahlung emission fits some Sy1and Sy2data,but not the trend of the Sy1complex.4.3Seyfert 2galaxiesThe sample of Sy2is shown in Fig.8both for Einstein (top panel)and ROSAT data (bottom panel).In both samples,the data are better explained by RD or SD models with L 12produced by dust thermal emission.The notation is the same as in Fig.7.Notice that thin short-dash lines refer to SD models and thick short-dash to RD models with IR from dust,while dotted lines correspond to the same mod-els shifted in the diagrams toward higher L IR ,in order to explain the high IR luminosity of objects which are rich in dust.Except for four objects in the top panel and three in the bottom panel,most of the data points are located between two SD curves,characterized by log η=40.1and 43.3On the other hand,the objects on right of the the SD curves are close to the RD results (thick short-dash)with log F h =11,V s between 300and 500,and a relatively low d/g (see Tables 3and 4).4.4DiscussionThe first results of our investigation show that the X-ray and near-IR correlation for Sy1can be explained by the composite models of clouds producing both bremsstrahlung。
辐射成像原理-第3章-投影X射线成像(2)

Scintillator for a-Si based DR
CsI(T1): Best candidate as a screen material coupled with a-Si photodiodes
Advantages:
Good emission spectrum matching to a-Si photodiode quantum efficiency
0.9~1.3 1.0~1.5 1.1~1.6 1.3~1.8 1.4~2.0 1.6~2.2 1.7~2.4
焦点标称值 1.3 1.4 1.5 1.6 1.7 1.8 1.9 2.0 2.2 2.4 2.6 2.8 3.0
宽度 1.3~1.8 1.4~1.9 1.5~2.0 1.6~2.1 1.7~2.2 1.8~2.3 1.9~2.4 2.0~2.6 2.2~2.9 2.4~3.1 2.6~3.4 2.8~3.6 3.0~3.9
3、影像的失真
三、散射线
1、散ห้องสมุดไป่ตู้线引起照片对比度损失
2、被照体厚度与散射线
3、照射野和散射线
4、散射线的抑制
5、散射线的排除
• Groedel法
• 应用滤线栅法
栅比
R h
D
栅密度
n 1 dD
• 应用滤线栅法
焦距f0 界限f1、f2
• 滤线栅的物理性能
一次X线透过率
Tp
I
'' p
I
' p
R
0
D
2
2
D
四、Wiener频谱
Wiener spectrum
Ws S
R
ei2 d
S F 2 L
Hard X-ray and Infrared Emission from Apparently Single White Dwarfs

1
2
Chu et al.
Figure 1. ROSAT PSPC observations of KPD 0005+5106, PG 1159, and WD 2226−210. The top two rows show soft (0.1–0.4band images. The poor point-spread-function in the soft band is caused by an electronic ghost image at energies below 0.2 keV. The bottom row shows the PSPC spectra. To show the hard counts of PG 1159, its spectrum above 0.6 keV is scaled up by a factor of 50 as marked and plotted in open symbols.
arXiv:astro-ph/0701025v1 1 Jan 2007
**FULL TITLE** ASP Conference Series, Vol. **VOLUME**, **YEAR OF PUBLICATION** **NAMES OF EDITORS**
介导紧张性抑制的GABAA受体与颞叶癫痫

介导紧张性抑制的GABA A受体与颞叶癫痫癫痫是脑内兴奋性活动增强和抑制性活动相对低下的结果。
γ氨基丁酸(GABA)是脑内的主要抑制性递质。
在癫痫病人及实验性动物模型的癫痫病灶中均发现GABA介导的抑制功能低下1,2,如果升高脑内GABA浓度或应用GABA 受体的激动剂则可以发挥治疗作用3,4。
GABA A受体是GABA的离子型受体,激活后可产生两种截然不同的抑制形式:相性抑制和紧张性抑制5。
自从发现GABAA受体以来,早期的大多数研究关注于其介导的相性抑制,即抑制性突触后电流/电位。
后来发现GABA可以作用于某些GABA A受体产生持续的抑制性电导,而且这种持续电导所携带的电荷远远大于相性抑制携带的电荷6。
这种紧张性抑制在调节膜电位和神经网络兴奋性中起重要作用。
颞叶癫痫是最常见的局灶性癫痫,约有30%的患者对抗癫痫药物耐药,很多病人需要手术切除治疗。
在颞叶癫痫的形成过程中,海马结构中的紧张性抑制也扮演了重要角色,同时也成为潜在的治疗靶点。
本文将阐述生理状态下海马结构中GABA A受体介导的紧张性抑制及在颞叶癫痫中发生的变化,讨论通过调节紧张性抑制来治疗癫痫的策略。
1、紧张性抑制紧张性抑制是一种持续性的抑制信号。
与相性抑制不同的是,紧张性抑制并不与突触前动作电位形成点对点的锁时信号通讯。
介导紧张性抑制的GABA A受体存在于突触周围或突触外,对GABA亲和力高,且脱敏缓慢或不完全脱敏7。
这样的特点使它们可以结合周围环境中较低浓度的GABA并维持通道持续开放。
海马结构中的齿状回颗粒细胞、海马锥体细胞及中间神经元均存在这种信号8,9,10。
此外,在脑内其他神经元中也可检测到GABA A受体介导的紧张性抑制,例如:小脑颗粒细胞、丘脑神经元、皮层2/3层锥体神经元、皮层神经胶质样细胞、纹状体11,12,13,14,15。
2、海马结构中介导紧张性抑制的GABA A受体及分布目前已知有20种不同的GABA A受体亚基,包括α1–6,β1–4,γ1–3,ε,δ,θ,π和ρ1–3。
基于时空相关性的LSTM算法及PM_(2.5)浓度预测应用

第38卷第6期 计算机应用与软件Vol 38No.62021年6月 ComputerApplicationsandSoftwareJun.2021基于时空相关性的LSTM算法及PM2.5浓度预测应用赵彦明(河北民族师范学院数学与计算机科学学院 河北承德067000)收稿日期:2019-09-12。
河北省社科基金项目(HB18TJ004)。
赵彦明,副教授,主研领域:脑计算理论,图像分析,生物特征识别。
摘 要 现阶段空气污染物粒子浓度演进过程模拟与预测算法忽视了粒子浓度的空间相关性,且没有实现粒子浓度的时间依赖性与空间相关性融合。
对此,提出基于时空相关性的LSTM算法(TS_LSTM)并应用于PM2.5浓度预测。
该算法提出空间相关性及其相关因子计算方法;将局部区域相关性因子与LSTM算法的遗忘门和记忆门融合,建立基于局部地理信息的LSTM算法(LTS_LSTM);融合LTS_LSTM算法学习结果与全局空间相关性因子,构造基于全局地理信息时空相关的LSTM算法(GTS_LSTM)。
模拟全局与局部的空气污染物粒子浓度演进过程,并实现离子浓度预测。
在全局与局部数据集上,将该算法与回归算法、支持向量机、模糊神经网络、LSTM神经网络、GC LSTM神经网络、DL LSTM神经网络比较研究,结果表明:在空气粒子浓度预测上,该算法的预测性能优于各种传统预测算法,接近深度LSTM算法。
关键词 长短时记忆网络 空气污染物浓度预测 循环神经网络 时空相关性 PM2.5中图分类号 TP3 文献标志码 A DOI:10.3969/j.issn.1000 386x.2021.06.040LSTMALGORITHMBASEDONSPATIO TEMPORALCORRELATIONANDITSAPPLICATIONOFPM2.5CONCENTRATIONPREDICTIONZhaoYanming(SchoolofMathematicsandComputerScience,HebeiNormalUniversityforNationalities,Chengde067000,Hebei,China)Abstract Atpresent,thesimulationandpredictionalgorithmoftheevolutionprocessofairpollutantparticleconcentrationignoresthespatialcorrelationofparticleconcentration,anddoesnotrealizethefusionoftime dependentandspatialcorrelationofparticleconcentration.Basedonthis,aLSTMalgorithmbasedonspatio temporalcorrelation(TS_LSTM)anditsapplicationofPM2.5concentrationpredictionisproposed.Itproposedthecalculationmethodofcorrelationfactor;thefactoroflocalspatialinformationcorrelationwasfusedwithforgettinggateandmemorygateofLSTMalgorithmtoestablishtheLSTMalgorithmofthelocalgeographicinformation(LTS_LSTM);thealgorithmfusedthelearningresultofLTS_LSTMalgorithmwiththeglobalspatialcorrelationfactortoestablishaglobalspatio temporalcorrelationLSTMalgorithm(GTS_LSTM).Itsimulatedglobalandlocalevolutionprocessofairpollutantparticleconcentration,andrealizedionconcentrationprediction.Onthelocalandglobaldataset,thisalgorithmiscomparedwithregressionalgorithm,supportvectormachine,fuzzyneuralnetwork,LSTMneuralnetwork,GC LSTMneuralnetworkandDL LSTMneuralnetwork.Theresultsshowthat:intheairparticleconcentrationprediction,thepredictionperformanceofthisalgorithmisbetterthanvarioustraditionalpredictionalgorithms,closetothedepthLSTMalgorithm.Keywords LSTM Predictionofairpollutantconcentration Recurrentneuralnetwork Spatio temporalcorrelation PM2.5250 计算机应用与软件2021年0 引 言空气污染物对人类健康的威胁与日俱增。
(完整版)医学影像专业英语

(1)To prospectively evaluate the effect of heart rate, heart rate variability, and calcification dual-source computed tomography (CT) image quality and to prospectively assess diagnostic accuracy of dual-source CT for coronary artery stenosis. by using invasive coronary angiography as the reference standard.前瞻性评价心率、心率变异性及钙化双源计算机断层扫描成像质量的影响及对冠状动脉狭窄的双源性冠状动脉狭窄诊断的准确性评价。
以侵入性冠状动脉造影为参照标准。
(2)Chest radiography plays an essential role in the diagnosis of thoracic disease and is the most frequently performed radiologic examination in the United States. Since the discovery of X rays more than a century ago, advances in technology have yieled numerous improvements in thoracic imaging. Evolutionary progress in film-based imaging has led to the development of excellent screen-film systems specifically designed for chest radiography.胸部X线摄影中起着至关重要的作用在胸部疾病的诊断,是最常用的影像学检查在美国。
50-110nm高反射率多层膜的设计、制备与检测

第1章绪论1.1引言第1章绪论自20世纪50年代以来,人们开始了对光学多层膜的研究【“。
经过几代人的不懈努力,多层膜的研究与应用几乎遍布了整个电磁波谱[25/,如图1.1所示。
从红外到软x射线以至于波长更短的硬x射线波段,多层膜都以其特有的优势在科学研究与技术应用领域发挥着不可替代的作用。
然而,电磁波谱中,在极紫外与真空紫外约t0-200加1波段,人们的研究并不深入。
主要是因为材料在这一波段具有不同于其他波段的吸收特性,研制符合应用要求的多层膜光学元件有一定困难。
即便如此,人们还是可以采用常规的多层膜结构在小于50nl"n和大于110am波段实现了光学元件的反射率增强。
然而在50—110nna强吸收波段,长期的研究工作却难有突破。
主要是因为所有材料在这一波段的吸收特性尤其明显,几乎可以吸收全部辐射光。
正是这种强吸收特性,使得常规的多层膜难以产生适合的光学特性。
近年来,随着空间科学与技术的发展,真空紫外与极紫外波段光谱在天体物理,大气物理,太阳光谱学以及卫星表面膜层的温度控制等众多领域有着迫切的应用需要【4】,同时在同步辐射光学系统以及皿微米光刻技术【5l中也突显出重要的研究价值。
要在这些领域进行研究工作,性能良好的50-110姒波段高反射镜是必备的光学元件。
因此,科学技术的进步迫切需要人们致力于50.110nln强吸收波段高反射镜的研究。
图1.150.110nlm波段在电磁波谱中的位置Figure1.1ThepositionofS0·110minthewavelengthrangeoflightl3.2磁控溅射3.2.1磁控溅射原理磁控溅射法是在与靶表面平行的方向上施加磁场,利用电场和磁场相互垂直的磁控管原理.使靶表面发射的二次电子只能在靶附近的封闭等离子体内作螺旋式运动,电子在阴极区的行程增加,造成电子与气体分子碰撞几率增加,电离效率提高,同时减少了电子对基片的轰击降低7基扳温度,实现低温高速溅射,如图3.1所示。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
a rXiv:as tr o-ph/53145v17Mar25Temporal Correlation of Hard X-rays and Meter/Decimeter Radio Structures in Solar Flares Kaspar Arzner 1and Arnold O.Benz 21Paul Scherrer Institut,CH-5232Villigen PSI,Switzerland 2Institute of Astronomy,ETH Z¨u rich,CH-8092Zurich,Switzerland February 2,2008Abstract.We investigate the relative timing between hard X-ray (HXR)peaks and structures in metric and decimetric radio emissions of solar flares using data from the RHESSI and Phoenix-2instruments.The radio events under consideration are predominantly classified as type III bursts,decimetric pulsations and patches.The RHESSI data are demodulated using special techniques appropriate for a Phoenix-2temporal resolution of 0.1s.The absolute timing accuracy of the two instruments is found to be about 170ms,and much better on the average.It is found that type III radio groups often coincide with enhanced HXR emission,but only a relatively small fraction (∼20%)of the groups show close correlation on time scales <1s.If structures correlate,the HXRs precede the type III emissions in a majority of cases,and by 0.69±0.19s on the average.Reversed drift type III bursts are also delayed,but high-frequency and harmonic emission is retarded less.The decimetric pulsations and patches (DCIM)have a larger scatter of delays,but do not have a statistically significant sign or an average different from zero.The time delay does not show a center-to-limb variation excluding simple propagation effects.The delay by scattering near the source region is suggested to be the most efficient process on the average for delaying type III radio emission.1.Introduction A large fraction of the energy released in solar flares first appears in accelerated particles.Energetic electrons generate both impulsive radio emissions and hard X-rays (HXR),which are often closely associated.The HXRs,emitted by electron-ion bremsstrahlung,were occasionally found to have temporal fine structures down to several 10ms (Den-nis,1985),but more often (in some 10%of all ≥M class events)to the order of several 100ms (Kiplinger et al.1984).A very tight correlation is regularly observed between the radio emission produced incoherently by the synchrotron mechanism in centimeter wavelengths and bremsstrahlung X-rays as high-energy electrons are involved in both.Less correlation or even no association has been reported for the coherent radio emissions by electron beams,trapped electrons and from less-known mechanisms involving probably also non-thermal electrons (Benz et al.,2005).This is likely to be caused by the limited sensitivity of present HXR observations.c 2008Kluwer Academic Publishers.Printed in the Netherlands.2Kaspar Arzner and Arnold BenzAmong the different types of coherent radio emission in the meter and decimeter ranges,type III bursts,narrowband spikes and pulsa-tions often concur with HXRs.As both HXR and radio emissions are emitted presumably by nonthermal electrons,the question arises whether both originate from the same electron population.More than in a general association,e.g.with type III groups,such an identity would be manifest in correlations of individual structures.Indeed,earlier observations revealed occasional correlations between individual type III bursts and HXR pulses(e.g.,at meter waves Kane et al.,1982,and at decimeter waves Benz et al.,1983,Aschwanden et al.,1995a)at a timing accuracy of a few0.1seconds.Type III bursts are caused by electron beams exciting Langmuir waves in the coronal plasma.Dennis et al.(1984)found coincidences of reversed-slope type III bursts drifting downward in the corona and HXR peaks.A linear relation between the rate of type III bursts per second and the HXR emission has been reported for a case including a rich group of radio bursts(Aschwanden et al.,1995b).The frequency range of the above comparisons was limited to less than1GHz,and photon energies to more than25keV.None of the reported coincidences were without time shifts of the order of a few0.1s.As there are many reasons for delays of one or the other emission,including clock errors,the small difference did not cause much concern.Good correlation between the integratedflux of narrowband spikes in decimeter radio waves and the HXRflux has been reported by Benz &Kane(1986)and G¨u del(1991).However,Aschwanden&G¨u del (1992)noted that the integrated spike radiation is delayed by1-2 seconds.Decimetric pulsations are more frequent than narrowband spikes.Some correlation with hard X-rays has been noticed by Benz et al.(1983)and Aschwanden et al.(1985).Kliem et al.(2000)and Farnik et al.(2003)reported a detailed anti-correlation or a delay of thefine structures in pulsating radio emission.Narrowband spikes and pulsations are generally believed to originate from velocity-space instabilities,such as caused by a loss-cone distribution.However,there is no confirmed theory at present.The present investigation searches systematically for possible radio-HXR correlations on sub-second time scales using data from the Phoe-nix-2(Benz et al.,1991,Messmer et al.,1999)and RHESSI(Lin et al. 2002)experiments.The availability of this new radio data set allows us to extend existing studies towards higher frequencies that include coherent emissions of denser sources and that are possibly more relevant forflare physics.The greatly enhanced spectral resolution of RHESSI allows including lower energy photons,selecting the most relevant en-ergy range,and separating it from thermal emissions.As RHESSI isCorrelation of HXR and m/dm Radio Structures3 spin modulated,time resolution below the spin period requires careful demodulation,described in the second chapter.The demodulation in-troduces a relative timing uncertainty,which is however offset by the order of magnitude gain in absolute accuracy compared to previous observations.2.Data selection,reduction,and preprocessingFrom the period of February2002to March2003,a set of events was selected according to the following criteria(in this order):(i)Presence of distinct radio structures on time scales below1s.(ii)A minimum simultaneously observed HXRflux of50ct/s/subcollimator above6 keV.(iii)Absence of RHESSI attenuator movements during the inves-tigated contiguous time range(typically,about one minute),and(iv) the availability of an approximate source position,which is needed for demodulation(see below).Out of the40initial candidates,only22 revealed HXR-radio correlation on inspection by eye,and were further investigated.The investigated events are compiled in Table I.2.1.Radio and hard X-ray observationsPhoenix-2is a full-sun polarization-sensitive radio spectrometer located at Bleien(8o6’44”E,47o20’26”N),Switzerland.In its present configu-ration,it has a7m dish and200narrowband frequency channels(∆ν= 1,3,or10MHz)covering the range from0.1to4GHz.Detection(after two IF stages)is logarithmic,and the nominal radiometric noise is1-5%.The radio data were calibrated and cleaned from telecommunica-tion artifacts using gliding background subtraction,and bad channels were eliminated.Only radioflux density is considered for correlation with RHESSI data,but circular polarization was consulted for radio type identification.The radio spectrogram is integrated over afinite bandwidth in order to obtain a single time profile.The RHESSI satellite detects individual photons between3keV and17MeV in4096energy channels with a time resolution of2−20s (≈1µs).Although the full energy response matrix is available for solar sources,we use here only the diagonal response because as our emphasis is on time structures in rather broad energy bands,and not on exact spectroscopy.RHESSI is designed as a HXR imager,with two-dimensional imaging achieved by rotational modulation(Schnopper, 1968;Skinner and Ponman,1995).In this technique,the spatial infor-mation is encoded in temporal modulation of the observed HXRflux when the source becomes shadowed by linear grids which arefixed on4Kaspar Arzner and Arnold BenzTable I.Thefinal record of correlated events.A negative time delayindicates that HXR comes before radio.The windows for correlationare given in center time,radio frequency and HXR energy.The labelsin the last column refer to(reversed)type III(RS),U bursts,anddecimetric pulsations or patches(DCIM).Time Frequency Energy Delay Radio[UTC][MHz][keV][s]Typethe rotating(T S∼4s)spacecraft(Hurford et al.,2002).The RHESSI optics consists of9pairs of linear grids(‘subcollimators’)with angular pitches p i=2.61×3i/2arc seconds(i=1..9),and instantaneous mod-ulation periods range from5·10−4s to2s,depending on subcollimator and source location.2.2.Instrumental timing accuracyThe Phoenix-2clock is locked to GPS timing,accurate to one millisec-ond.The spectrometer uses UTC,derived from GPS time having no leap seconds.The timing of the n th frequency channel is shifted byCorrelation of HXR and m/dm Radio Structures5 (n−1)×0.5ms relative to thefirst channel,for which there is timestamp available on each data record.The time stamp in Phoenix-2data has been measured by receiving the DCF77time signal transmittedfrom Mainflingen near Frankfurt/Main(Germany)at77.5kHz.Theabsolute timing of DCF77at emission is of the order of one millisecond.After correcting for propagation time,the Phoenix-2time stamp wasfound delayed by22±2ms.This systematic radio delay is caused bysignal propagation in the spectrometer and has been stable over time.It is corrected in the following where relevant,making the absolutePhoenix-2timing accurate to within about5ms.The RHESSI time stamp of each photon in UTC is set withinapproximately oneµs of its arrival time.RHESSI timing is also synchro-nized with GPS and accurate within one millisecond(J.McTiernan,m.).The observations of more than a dozen non-solar gamma-ray bursts and soft X-ray repeaters have allowed to verify the RHESSItiming accuracy(K.Hurley,m.).A further systematic effect arises from the light travel time between RHESSI and Phoenix-2.Theextreme cases arise when Phoenix-2is at dusk/dawn and RHESSI isat noon,or vice versa.The maximum possible delay is thus±r E/c=±20ms.It can go in both ways.The light travel time from RHESSI (altitude600km)to ground is only2ms,and always negative(radiodelayed).Time differences due to effects in the solar corona will bediscussed later.2.3.Visibility-based HXR demodulationSince the RHESSI modulation interferes with temporal structures be-low the spin period,the RHESSI light curves must be demodulated prior to comparison with radio observations.Demodulation is an inverse problem,and is here accomplished by the method of(Arzner2002, 2004).In this‘visibility-based’method,the solar HXR distribution during some10T S in afixed energy band is assumed to be of the form B(x,y,t)= k r t,k B k(x,y),and the binned time profile r t,k of the k-th source component is estimated by penalized maximum-likelihood(Yu et al.1994).The goal quantity is the Bayesian probability P tot=L×P a, where L is the posterior probability(likelihood)that the data are ob-served if the model was true,and P a is the a priori probability assigned to the model.The likelihood is calculated assuming Poisson statistics, and the a priori probability is chosen asP a=exp −16Kaspar Arzner and Arnold BenzThe form of Eq.(1)favors smooth time profiles without imposing a bias.The larger the value ofαk,the smoother is r t,k as a function of t;as a rule-of-thumb,the autocorrelation time1of the maximum-P tot solution isτk∼max(∆t,√1defined byτ−2= S(ω)ω2dω S(ω)dωwith S(ω)the power spectral density. 2Projections of B k(x,y)on the sine and cosine components of the modulation pattern(Hurford et al.,2002).Correlation of HXR and m/dm Radio Structures7 nal solver.Convergence is controlled by monitoring the goal function log P tot and its contributions log L and log P a.The solution usually becomes stable after a ing the Portland Group Fortran90compiler with standard optimization on a medium-size work station,execution time for500iterations is about1second for typical time bins∆t=0.12s,and scales linearly with1/∆t.While we make here no explicit use of the visibilities,they absorb, unavoidably,observational information and thereby degrade the quality of r t,k.An estimate on the error of r t,k can be obtained by perturbing the solution r t,k until log L deviates by more than18Kaspar Arzner and Arnold BenzFigure1.Simulation of the intrinsic timing accuracy of the demodulation.a)–true time profile(black)and demodulation(gray)of a multicomponent Gaussian source(inlet).b)–cross-correlation between true and demodulated time profiles.c)–simulated delays of104samples;the shaded range contains70%probability mass. See text.active region and the impulsive source are modeled as Gaussians in space and time.The active region is placed at random on the solar disc,and the impulsive source is placed at random within the active ing a simulated aspect solution and simulated data gaps(rate 1s−1,mean duration0.25s),the Poisson intensity is computed for each subcollimator,and a sequence of binned counts is generated.From these,the demodulation is calculated(Fig.1a gray line)and compared to the true spatially integrated profile(Fig.1a black line).The role of the active region is to provide a time-dependent background with a generally non-vanishing slope,which may,potentially,bias the tim-ing.Such a slope-induced bias is thus included in the simulation.TheCorrelation of HXR and m/dm Radio Structures9 simulated average count rate of Fig.1is350counts/s/subcollimator,which is representative for an M classflare.The reconstructed curve has a time resolution∆t=0.062s and smoothing parametersα1=10−1andα2=3·10−4.The cross-correlation with the original,in the dashedinterval of panel a),is presented in panel b).Panel c)shows the distribu-tion of104simulated delays between the original and the reconstructedcurves,as given by the peak cross-correlation coefficient.The delayshave a standard deviationσintr=0.031s,where the subscript refersto the intrinsic accuracy of the demodulation method.Alternatively,we may consider the shaded region in Fig.1c,which contains70%probability mass and has widthτ70=0.060s.For Gaussian statistics,one expectsτ70/2=σ;this is well fulfilled for the simulation of Fig.1,but deviations may occur for real data(Sect.2.4.2).In this case,τ70isthe more significant quantity.classflares with larger count rates allowfiner time bins,smallersmoothness parametersαk,and better intrinsic timing accuracyσintr. From simulations similar to Fig.1we deduce the estimateσintr≃0.3s c if c >∼100s−1(3) where c is the average count rate in ct/s/subcollimator in the wholetime interval(∼10T S)under consideration.(It is not the peak intensityalone which matters because modulation is to be identified from thewhole time interval.)The limit c >∼100s−1in Eq.(3)is understood in the sense that above this value,σintr becomes weakly dependent onthe model characteristics such as the total signal-to-background countratio or the instantaneous signal-to-background intensity;below100ct/s/subcollimator,the achievable accuracy depends on the model char-acteristics and is typically lower(σintr∼0.1s).We may thus interpret a count rate of100ct/s/subcollimator as a minimum requirement for reliable use of the visibility-based demodulation method.It should be recalled that Eq.(3)refers to the timing accuracy as measured in the numerical experiment of Fig.1,and does not include any systematic (instrumental)errors.A similar error asσintr must be expected from a cross-correlationwith a radio signal assumed to be without noise.Although the errorintroduced by demodulation exceeds the instrumental timing errors(Sect.2.2),it is symmetric and thus cancels out on the average.2.4.2.Systematic errorsWe turn now to the second strategy,involving real data.An estimatefor the robustness of demodulated features may be gained by varying the subsets of subcollimators and smoothness parametersαk,and by10Kaspar Arzner and Arnold BenzFigure2.Top panel:different solutions of the demodulation problem,using differ-ent subcollimator(SC)sets and smoothness parametersαk.Subsequent graphs are offset by100ct/s/subcollimator for better clarity,with the bottom graph having zero offset.Middle panel:an alternative demodulation method(private comm.G. Hurford).Bottom panel:raw data,in time bins of one spin period. comparison with other demodulation methods.Figure2(top panel) shows the demodulation results from different sets of subcollimators andαk parameters using data of theflare of April15,2002,08:51:30. Different curves are offset by100ct/s for better clarity,with the bot-tom curve having zero offset.The non-solar background is forced hereto zero in order to facilitate the comparison with an alternative de-modulation method(middle panel)which does not include non-solar background.The top curve of Fig.2is obtained from thefinest two subcollimators under the assumption of a single source component.The next two curves represent odd-numbered(3,5,7,9)and even-numbered (1,4,6,8)subcollimators,assuming two source components in both cases (collimator#2is replaced by#1due to increased background).Both subcollimator sets cover the full range of angular resolutions and grid orientations.The bottom curve involves all subcollimators except#2. Generally,more subcollimators contain more information and there-fore should give better estimates.However,pieces of information from different subcollimators may sometimes be contradictory.This is,for instance,the case at08:51:34for even and odd subcollimators(top panel,middle curves).It may also happen that only one single subcol-limator sees a short intense peak.The global demodulation then barely responds,since the likelihood cost for all other subcollimators would be too high.Erratic contributions from cosmic rays are(hopefully) suppressed in this way.The middle panel of Figure2shows the result of an alternative demodulation method proposed by G.Hurford(priv. comm.).This method removes sinusoidal contributions at the instan-taneous modulation frequency,inferred from the source centroid,and corrects for time-dependent grid transmission and detector lifetime. The algorithm acts locally in time in intervals of duration0.3s,which were chosen to be commensurate with the time resolution(Eq.2)of the visibility-based demodulation.The middle panel is to be compared to the bottom curve of the top panel,as both represent the true incoming counts per second and subcollimator.Finally,the bottom panel presents the raw counts in bins of one spin period(T S=4.1428s).This may serve as a very coarse but trustworthy benchmark.Table II.Above diagonal:standard deviation andτ70/2(in brackets)of the delay between different demodulations.Below diagonal:corresponding mean values.All values are in seconds.The labels O and E refer to even and odd-numbered subcol-limators,and the subscripts denote the number of assumed source components.A refers to all subcollimators except#2.Each entry is computed over a sample of54HXR peaks.O1-0.00070.365(0.348)0.392(0.404)0.034(0.031) E10.0430.0110.00080.033(0.022)A2The top panel of Figure2suggests a method to determine the total(intrinsic+systematic)timing accuracy of the visibility-based demodulation.By selecting manifest peaks like at08:51:23UTC,and correlating the demodulations of different subsets of subcollimators and varyingαparameters,an estimate on the effective timing accuracy can be obtained.This procedure gives an upper bound;the timing error with respect to the relatively more accurate radio signal should be smaller by a factor∼2−1/2.We have carried out the above programme for a total of54identified HXR peaks during the events listed in Tab.I,employing5different combinations of subcollimator sets and αparameters.The result is summarized in Table II.The label O1 denotes the odd-numbered subcollimators(3,5,7,9)with a single source component;O2the odd-numbered subcollimators with two source com-ponents;E1the even-numbered subcollimators(1,4,6,8)with one source component,E2similarly with two components.Finally,A2involves all subcollimators(except#2)and two source components;it is A2which is normally used for the correlation with the radio data.The smoothness parametersαk were chosen such that single and double sources had a similar time resolution,which also corresponds to the one used in the HXR-radio correlation study.The entries of Tab.II above the diagonal are the standard deviation andτ70/2(in brackets)in seconds,whereτ70 is defined empirically as in Fig.1c.Below the diagonal is the mean delay in seconds.There are two major features which become apparent from Tab.II:first,the introduction of additional source components(O1→O2and E1→E2)has only a minor effect on the demodulation solution, and the resulting delays are within the intrinsic accuracy(Sect.2.4.1). Secondly,the use of disjoint observational data sets(O k↔E k)leads to much larger discrepancies in the order of0.4s.Not surprisingly,A2 agrees better with each of its subsets O2and E2than these do among each other.The mean values(lower triangle)are of the order of the√corresponding standard deviations divided by3.Radio-HXR Comparison3.1.ProcedureOnce the radio and HXR data are calibrated and demodulated,respec-tively,they are confronted with each other and searched for commonfine structures.We illustrate our procedure in Figure3,consideringas example the GOES C classflare of April15,2002,08:51UTC.In the top and middle panels of Figure3,the calibrated Phoenix-2spectrogram is displayed against the RHESSI raw counts.Grayscalerepresents histogram-equalized logarithmic radioflux.Dots represent asubset of all observed RHESSI counts,selected at random to show theraw distribution.In afirst step,we select a HXR energy band and timerange containing the bulk offlare-associated counts(middle panel,solid line;Table I).The average count rate in this energy band is then used tochoose time bins(see Arzner,2004for details;here:∆t=122ms),anda binned event list is generated.This,together with the aspect solution(Fivian et al.,2002,Hurford and Curtis,2002)and grid transmission(Hurford et al.,2002),forms the input to the demodulation code.Byvarying the subset of subcollimators,the reliability of temporal struc-tures is tested,and different choices of smoothness constraintsαk areexplored(Section2.4,2).The demodulation r t,k adjudged optimum isshown in the bottom panel of Figure3.It has two source componentswith time scalesτ1∼8.7s(light gray)andτ2∼0.5s(dark gray), which add up to the total solar HXRflux(black).All subcollimatorswere used,except for#2,suffering enhanced background(Smith et al.,2002).For comparison,the binned(∆t=0.2s)raw counts of thefinestsubcollimator#1are also shown(Fig.2bottom,histogram style).Since 0.2s exceeds the modulation frequency of subcollimator#1(exceptat glancing source passages of the RHESSI grids),this is expected toyield a noisy proxy for the true time profiles.Data gaps(Smith etal.,2002),where the count rate drops to zero,are not corrected inthe binned raw counts,but are incorporated–via detector lifetime–in the demodulation.With some100ct/s/subcollimator,the event isamong the weaker ones considered in this study.Contrary to Fig.2,the non-solar is not kept zero butfitted as35%.In a next step,we select a time-frequency box(Fig.3top panel,solid line;Table I)containing the radio emission to be correlated withthe HXR.In doing so we attempt to capture the onset of type IIIssuch as to minimize delays due to the radio drift.The RHESSI lightcurve is the sum of all source components if several are used.The radiospectrogram inside the selected time-frequency box is then frequency-integrated to obtain the radio light curve shown in Fig.4(top left).Figure 3.Top:radio spectrogram,and time-frequency box selected for cross-correlation.Middle:RHESSI raw counts(all subcollimators;only a random subset of50.000is shown)and time-energy window(solid line)selected for de-modulation.Bottom:subcollimator#1,binned in0.2s bins(histogram style)and demodulated time profiles(light gray:slow component;dark gray:fast component; black:slow+fast components).From this,a linear trend is subtracted(Fig.4top right),and the result is cross-correlated with a similarly de-trended RHESSI light curve(Fig. 4middle row).The HXR de-trending amounts to a removal of slow vari-ations,which typically arise from low-energy(thermal)contributions. The cross-correlation is shown in the bottom panel of Fig.4.The time delay is defined from the peak of the cross-correlation,and indicated by dashed line.The gray shaded region in the left middle panel is anFigure4.Close-up of Fig.3:time profiles of Phoenix-2(top line)and demodulated RHESSI(middle line).The RHESSI signal represents the totalflux summed over both source components.A linear trend(left column,dashed)is subtracted to obtain de-trended light curves(right column),the cross-correlation of which is shown in the bottom panel.Negativeτindicates that HXR comesfirst.The error of the demodulation,obtained by numerical perturbation of the solution,is shaded gray (left column,middle row).error estimate of the demodulation(see Sect.2.3).By comparing the lightcurves in the right column of Fig.4we conclude that a chance coincidence of the two major peaks is unlikely,but a one-to-one cor-respondence of all structures is certainly contestable.With a leap of imagination one may draw associations between individual RHESSI and Phoenix-2peaks,and investigate their relative timing.Averaging would then yield a mean delay which is compatible with the maximum of the cross-correlation of the full time series.Figure5.The event of August31,2002,14:20UTC.3.2.SurveyAll events listed in Table I have been processed in the way described above.The radio burst types have been classified according to Isliker& Benz(1994).They include type III bursts and DCIM,an abbreviation used in Solar and Geophysical Data for events in the decimetric range that do notfit the metric classification,such as pulsations,patches and narrowband spikes.The delays were determined by cross-correlation as described above.A total of24bursts has been analyzed.Their average delay is−0.85±0.28s,thus the HXRs are generally leading.The time delay is plotted in Fig.12versus the maximum frequency used in the analysis(Fig.3top,rectangle).The17groups of correlating type III bursts are delayed by−0.69±0.19 s on average.The distribution is broad,having a standard deviation of0.79s.Only3out of15have a positive delay.The8groups of type III bursts with maximum frequency above1GHz are delayed less onaverage(−0.45s)than the groups below1GHz(−0.96s).Although theFigure6.The event of June3,2002,17:13UTC.standard deviations are so large that these trends are not statistically significant,they exist independently and are significant within groups (e.g.Fig.6).In particular,it can be seen from Fig.12that the type III delays(diamonds)below1GHz are consistently negative,while at higher frequencies positive delays occur as well.Surprisingly,the4 groups of reversed slope type III bursts(triangles)are also delayed(av-erage−1.2s).It should be pointed out that not all of the investigated type III events have clear HXR correlations on the level of individual peaks.Judged by eye,we estimate that only some20%of the XHR-associated type III bursts correlate peakwise;this estimate is limited by the small statistics and by the sensitivity of the HXR demodulation.The average delay of the7DCIM bursts(Fig.12,crosses;the outlier at−6.01s is not shown)is−1.25±0.86s,but the distribution is broad, having a standard deviation of2.27s.Thus2out of7DCIM have positive delay,and the mean delay of DCIM is statistically not different from zero.The order of magnitude of the delays is similar to the onereported by Aschwanden&G¨u del(1992)for decimetric narrowband。
