Positivity of Chern Classes for Reflexive Sheaves on P^N

合集下载

学术英语(第二版)综合教师手册unit3

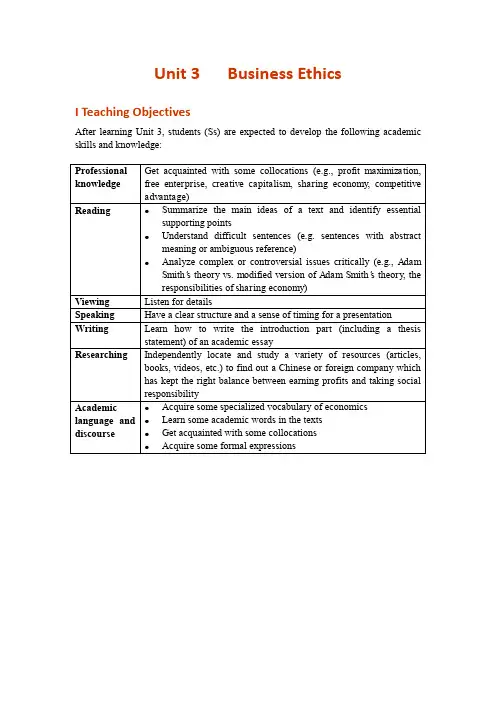
Unit 3 Business EthicsI Teaching ObjectivesAfter learning Unit 3, students (Ss) are expected to develop the following academic skills and knowledge:II Teaching Activities and ResourcesReadingText ALead-inTeaching StepsAsk Ss to work in groups and complete the task in Lead-in. Then choose some groups to share their answers with the whole class.Answer Keys (Suggested Answers)1.Corporate Social Responsibility (CSR) can be defined as a self-regulatingbusiness model that helps a company be socially accountable —to itself, its stakeholders, and the public. In other words, it is the continuing commitment by business to behave ethically and contribute to economic development while improving the quality of life of the workforce and their families as well as of the local community and society at large.2.Text AnalysisTeaching Steps1.OverviewAsk Ss to preview Text A before class. Or, allocate some time for Ss to read the text quickly in class. Then invite several Ss to summarize the main idea of Text A.2.In-Depth Analysis1)Show Ss the following words and ask them to contribute to the class as muchas possible with what they know about these words. Provide additional information in Supplementary Information when necessary.•economic system•free enterprise2)Explain some important language points in Language Support to Ss.3)Discuss with Ss the features of the two versions of Adam Smith’s theory bydoing Task 1 in Critical reading and thinking.4)Organize a group discussion about the questions from Task 2 in Criticalreading and thinking. Encourage Ss to think independently, critically and creatively and share their ideas with each other.Supplementary Information1.economic systemAn economic system is a system for producing, distributing and consuming goods and services, including the combination of the various institutions, agencies, consumers, entities that comprise the economic structure of a given society or community. It also includes how these various agencies and institutions are linked to one another, how information goes between them, and the social relations within the system.Two of the basic and general economic systems are market economy and planned economy. Market economy is an economic system in which businesses and individuals decide what to produce and buy, and the market determines quantities sold and prices, whereas planned economy is an economic system in which decisions regarding production and investment are embodied in a plan formulated by a central authority, usually by a public body such as a government agency.Although a planned economy may be based on either centralized or decentralized forms of economic planning, it usually refers to a centrally planned economy.2.free enterpriseFree enterprise refers to the system of business in which individuals are free to decide what to produce, how to produce, and at what price to sell.Language Support1.Most academics today subscribe to the following modified version of AdamSmith’s theory:…(Para. 2)In the text, the phrase “subscribe to”means “agreeing with or supporting (an opinion, theory, etc.)”（同意；赞成）.e.g. I subscribe to the idea that voting is my civic duty.She subscribes to the theory that some dinosaurs were warm-blooded andothers were cold-blooded.It can also refer to “paying money to get (a publication or service) regularly”（订阅）.e.g. I subscribe to several magazines.We have subscribed to an evening newspaper.2.These constraints take a number of different forms. …Another set ofconstraints …(Para. 3)Two recent examples dramatically illustrate these points. First, …(Para. 3) Classification is employed to organize things into categories with a single organizing principle, and give examples of things that fit into each category. Here are other commonly-used words and expressions for classification:•The first (kind/type/group/class/etc.) …; the second …; the third …•sort, classify, categorize …•category, group, class, kind …3.Saving the financial system required a bailout of the banks and otherfinancial companies, and that bailout imposed huge costs on the taxpayers, and also helped push the economy into a deep recession. (Para. 5)impose sth. on sb.:i) force something on someone把......强加于e.g. He always imposes his opinion on others.ii) levy a tax or duty征税e.g. It is therefore necessary for governments to impose a higher tax on this kindof food.Here is another usage of the word “impose”:impose on sb.:i) force oneself on others强加于人e.g. Thanks for your offer to help, but I did not want to impose on you.ii) take unfair advantage of 利用e.g. Don’t you think you are imposing on your neighbor when you use histelephone for half an hour?4.be true to one’He is always true to his word and focused on the big picture.Ninety days later, true to his word, the goldsmith brought the crown.5.To date, Buffett has contributed more than $6 billion, and over time he isscheduled to contribute additional stock that is now worth in excess of $40 billion. (Para. 7)in excess of: greater than, more than 超过e.g. Luggage in excess of 100 kg will be charged extra.This plane can reach speeds in excess of 1000 kilometers an hour.Answer KeysCritical reading and thinkingTask 1 / Overview1The original version：The invisible hand; Profit maximization; Free enterprise systemThe modified version:the wealth of its stockholders; the best economic system; constraints2Argument 11) a wide range of regulations are imposed2) assessing costs on companies; BP oil spillArgument 21) behave ethically; value and reputation2) Don’t Be Evil3) creative capitalism4) contributed more than $6 billionTask 2 / Points for discussion (Suggested Answers)11) Yes, I agree. Because:• Earning profit is the main aim of every economic activity. A business is an economic activity and hence, its main aim should be to earn profit.• Profit is a measure of eff iciency and economic prosperity of the business. This justifies the concept of profit maximization.• Economic conditions do not remain the same all the time. A business can retain its profit so that it can survive the adverse situations in the future. Profit gives protection against future risks and uncertainties.• Profit is the main source of finance for growth and expansion. Profit is essential for the business in order to invest in new business propositions.• Profitability is essential for fulfilling the social goals of the business. With profit maximization a business can do socio-economic welfare and follow its policies of corporate social responsibility.2) No, I don’t agree. Because:Business should do anything they can to make a profit, but never at the cost of human welfare and natural environment. Human welfare must be considered as important as profit making. A business is successful to the extent that it provides a product that contributes to happiness in all forms. As a matter of fact, the great value of a business should be morally associated with consumer’s satisfaction and safety. On the other hand, businesses of great value should not be blind to their moral obligations of protecting the natural environment.2 Yes, it is a very effective way for society to impose such regulations to prevent companies from engaging in practices that hurt society. For most businesses their ultimate goal is to make profits. It is true that most businessmen try to abide by the laws while making profits. However we have to admit that there are some who use every means to make as much money as possible, even regardless of the interests ofemployees, communities, and environment. Therefore, the regulations such as penalties and suspension for internal rectification should be imposed to deter companies from unlawful practices.3 Yes, having a good reputation with customers, suppliers, employees and regulators is essential if value is to be maximized. Business success is associated with many entities, such as customers, suppliers, employees and regulators. If customers are satisfied with your products and service, they will be loyal to your company. If suppliers provide you with qualified products, your business will operate well. If employees are treated well, they will work very hard and are willing to be part of your company. If regulators are certain that your company acts fairly and follows rules, your company will develop sustainably. All above depends on whether the business is continually operating with society’s interests in mind and maintaining a good reputation.Language building-upAnswer keysTask 1 / Specialized vocabulary11 纳税人2 自由企业制度3 股东4 经济体制5 市场力量；市场调节作用6 金融机构7 stock price 8 mission9 corporate motto 10 assets11 profit maximization 12 financial system21 financial institutions2 free enterprise system3 assets4 Taxpayers5 stockholder6 profit maximization7 economic system 8 market forcesTask 2 / Academic vocabulary1. principal2. dramatically3. academics4. maximization5. framework6. range7. compensate 8. transfer9. inadequate 10. constraintsTask 3 / Collocations1. subscribe to2. striving to3. imposing …on4. go out of business5. as a whole6. engaged in7. go hand in hand with 8. in excess ofTask 4 / Formal English1 insights2 subscribe to3 imposes4 adverse5 mission6 philanthropic7 donated 8 famedTranslation of Text A达到适当的平衡在1776年，亚当·斯密描述了一只“看不见的手”是如何引导企业追求利润的，而这只手会引导企业做出有利于社会的决策。

Shiing-Shen Chern

Shiing-Shen Chern (1911–2004)Shing-Tung Yau is professor of mathemat-ics at Harvard University.HIs email address is yau@.This is the speech given at the Harvard Memorial Conference for S.-S.Chern.geometric intuition will perhaps never be cleared up.…Whatever the truth of the matter,mathe-matics in our century would not have made such impressive progress without the geometric sense ofÉlie Cartan,Heinz Hopf,Chern and a very few more.It seems safe to predict that such men will always be needed if mathematics is to go on as before.”Chern:Father of Global Intrinsic Geometry Both Cartan and Chern saw the importance ofﬁber bundles to problems in diﬀerential geometry.It is certainly true that global diﬀerential geometry was studied by other great mathe-maticians:Cohn-Vossen,Minkowski,Hilbert,and Weyl,among others.However,most of their works focused on global surfaces in three-dimensional Euclidean space.Chern was theﬁrst who succeeded in building a bridge between intrinsic geometry and algebraic topology for manifolds of all dimensions,not just surface.(Cartan’s work in diﬀerential geometry was more local in nature,except his investigation of Lie groups and symmetric spaces.)The theory ofﬁber bundles was his major tool.Reﬂecting on intrinsic geometry,Chern com-mented:“Riemannian geometry and its generaliza-tion in diﬀerential geometry are local in character. It seems a mystery to me that we do need a whole1226Notices of the AMS Volume58,Number9space to piece the neighborhood together.This is achieved by topology.”Equivalence ProblemMost of the works of Chern were related to the problem of equivalence.In1869E.Christoﬀel and R.Lipschitz solved the fundamental problem in Riemannian geometry—the form problem:To determine when two ds2’s dif-fer by a change of coordinates,Christoﬀel introduced covariantdiﬀerentiation,which is now calledthe Levi-Civita connection.Cartan generalized this problem to a much more general setting;it is called the equivalence prob-lem.Given a Lie group G∈GL(n,R),given two sets of linearly indepen-dent linear diﬀerential formsθiandθ∗j in the coordinates x k andx∗l respectively,1≤i,j,k,l≤n,ﬁnd conditions under which thereare functionsx∗i=x∗i(x1,x2,...,x n)such thatθ∗j,after the substitu-tion of these functions,diﬀer fromθi by a transformation of G.This problem involves local invariants.Cartan provided a procedure to generate such invariants. Almost all of the work of Chern is related to this problem.Chern(1932–1943)During the eleven-year period from1932to1943, Chern studied web geometry,projective line geom-etry,invariants of the contact of pairs of subman-ifolds in projective space,and transformations of surfaces related to the Bäcklund transform in soli-ton theory.Chern continued this line of research in his collaborations with Griﬃths and Terng later.Between1940and1942,Chern[5]started to generalize the theory of integral geometry,an area initially developed by Crofton and Blaschke.He ob-served that this theory can best be understood in terms of two homogeneous spaces with the same Lie group G(see also Weil’s review[20]).This leads to the diagram associated to two sub-groups H and K of G:+QQQ Qs GG/H G/KTwo cosets aH and bK are incident to each other if they intersect in G.This notionﬁts nicely in mod-ern investigations of the Funk-Radon problem of determining a function on a manifold in terms of its integrals over certain submanifolds.Although the focus of this work is“classical”integral geom-etry,the doubleﬁbration diagrams depicted above preﬁgure in an uncanny way a diﬀerent,more mod-ern type of integral geometry:the theory of gener-alized Radon transforms developed by Gelfand and Graev in the lateﬁfties and early sixties.Using this approach,Chern generalized many important formulas of Crofton.In1952he[8]also generalized the kinematic formula of Poincaré, Santaló,and Blaschke.On the impact of these generalizations by Chern,Weil commented:“It lifted the whole sub-ject at one stroke to a higher plane than where Blaschke’s school had lifted it.I was impressed by the unusual talent and depth of understanding that shone through it.”Chern’s Visit to Princeton(1943–1945)In1943Chern left Kunming for Princeton.He ar-rived at the moment whenﬁber bundle theory was beginning to evolve from the works of Cartan and Whitney.When he arrived at Princeton,Weil had just pub-lished his work with Allendoerfer on the Gauss-Bonnet formula.Weil directed Chern toward the works of Todd and Eger on“canonical classes”in algebraic geometry.He pointed out to Chern that their work on characteristic classes for complex manifolds had only been completed in the spirit of Italian geometry and rested upon some unproved assumptions.Theﬁrst fundamental work Chern did on global intrinsic geometry was his intrinsic proof of the Gauss-Bonnet formula[6],re-proving the recent work of Allendoerfer and Weil[2]for general closed Riemannian manifolds.The proof of Allendoerfer-Weil rested on the use of local embeddings of the manifold in Euclidean space,isometric for an approximating real analytic metric.(The existence of local isometric embeddings for real analytic metrics had been established earlier by Burstin, Cartan,and Janet.)Weil commented on Chern’s proof of the Gauss-Bonnet formula:“Following the footsteps of H.Weyl and other writers,the latter proof, resting on the consideration of‘tubes’,did de-pend(although this was not apparent at that time) on the construction of a sphere-bundle,but of a nonintrinsic one,viz.the transversal bundle for a given immersion.…Chern’s proof operated ex-plicitly for theﬁrst time with an intrinsic bundle, the bundle of tangent vectors of length one,thus clarifying the whole subject once and for all.”A century earlier,Gauss established the concept of intrinsic geometry.Chern’s proof of Gauss-Bonnet opened up a new horizon.Global topology was now linked with intrinsic geometry through the concept ofﬁber bundle and transgression onOctober2011Notices of the AMS1227the intrinsic tangent sphere bundle.A new era of global intrinsic geometry arrived.While Hopf’s vectorﬁeld theorem had clearly in-ﬂuenced Chern’s thinking,the proof was a tour de force based on Chern’s insights and powerful al-gebraic manipulation.Hopf declared that Chern’s proof had brought diﬀerential geometry into a new era.In particular,it gave rise to the idea of trans-gression.Chern’s proof remains one of the most admired proofs in the modern history of mathe-matics.Chern ClassesReﬂecting upon his early encounter with Chern classes,Chern said:“My introduction to the char-acteristic class was through the Gauss-Bonnet formula,known to every student of surface the-ory.Long before1943,when I gave an intrinsic proof of the n-dimensional Gauss-Bonnet formula, I knew,by using orthonormal frames in surface theory,that the classical Gauss-Bonnet is but a global consequence of the Gauss formula which expresses the‘theorima egregium’.The algebraic aspect of this proof is theﬁrst instance of a con-struction later known as a transgression,which was destined to play a fundamental role in the homology theory ofﬁber bundles and in other problems.”Cartan’s work on frame bundles and de Rham’s theorem always stayed close to Chern’s thinking. The concept ofﬁber bundles stands at the very heart of modern mathematics.It’s a central uni-fying notion for many important objects in math-ematics and physics.It isﬁtting to give a brief de-scription of its history.In1937 E.Stiefel(1936)andH.Whitney(1937)introducedStiefel-Whitney classes;they wereonly deﬁned modulo integer two.From1939to1944J.Feldbau(1939),C.Ehresmann(1941,1942,1943),Chern(1944,1945)andN.Steenrod(1944)made a system-atic study of the topology ofﬁberbundles.In1942L.Pontryagin intro-duced the Pontryagin class.In1944he associated topologicalinvariants using the curvature ofRiemannian manifolds.His paperswere published in the Dokladyjournal.He was short in provingthat these curvature invariants arethe same as Pontryagin classes.Let me describe what is needed to prove the equivalence of the curvature and topological def-initions of characteristic classes.Recall that,in the proof of the Gauss-Bonnet for-mula,one uses vectorﬁelds s1,...,s k in general position.In his thesis in1936Stiefel proved that the locus at which the vectorﬁelds are linearly de-pendent forms a(k−1)-dimensional cycle whose homology class is independent of the choice of s i.In1937Whitney[21]considered sections for more general sphere bundles from the point of view of obstruction theory.Whitney noticed the importance of the universal bundle over the Grass-mannian G(q,N)of q planes in R N.He showed that any rank q bundle over the manifold is iso-morphic to the pullback of the universal bundle under a map f:M→G(q,N).Pontryagin(1942)and Steenrod[19]observed that,when N is large,the map f is unique up to homotopy.The characteristic classes of the bundle are given byf∗H∗(Gr(q,N))⊂H∗(M)In1934Ehresmann[17]studied the cohomology of H∗(Gr(q,N))and showed that it is generated by Schubert cells.Atﬁrst,Chern tried to prove the equivalence of the Pontryagin classes deﬁned using Schubert cells and classes deﬁned using the curvature form. The diﬃculty was how to integrate these diﬀeren-tial forms deﬁned by the curvature form over the Schubert cells.Chern quickly realized that it was easier to handle the case over complex numbers.Speaking of this discovery,Chern said:“It was a trivial ob-servation and a stroke of luck,when I saw in1944 that the situation for complex vector bundles is far simpler,because most of the classical complex spaces,such as the classical complex Grassmann manifolds,the complex Stiefel manifolds,etc., have no torsion.”For a complex vector bundle E,the Chern classes c i(E)belong to H2i(M,Z).By his discovery, Chern proved the equivalence of three deﬁnitions of characteristic classes:one using obstruction theory,one using Schubert cells,and the third using curvature forms of a connection on the bundle.The Fundamental Paper of Chern(1946)In the paper[7],Chern laid the foundation of Her-mitian geometry on complex manifolds.In this paper,Chern introduced the concept of Hermitian connections;using the curvature form Ωof the Hermitian connection,Chern deﬁneddet I+√2πΩ =1+c1(Ω)+···+c q(Ω).The importance of deﬁning Chern classes by dif-ferential forms cannot be overstated in mathemat-ics.It also provides fundamental tools in modern physics.An example is the concept of transgres-sion introduced by Chern.Letϕbe the connection form deﬁned on the frame bundle associated with the vector bundle.1228Notices of the AMS Volume58,Number9The curvature form isΩ=dϕ−ϕ∧ϕand c 1(Ω)=√2πTr Ω=√2πd(Tr ϕ)Tr (Ω∧Ω)=d(Tr (dϕ∧ϕ)+1−1This theorem of Chern is the only known general statement of the“Hodge conjecture”besides the case of dimension one and codimension one cycles, which is a relatively easy consequence of the Lef-schetz(1,1)theorem.Chern’s theorem also pro-vided a direct link between holomorphic K-theory and algebraic cycles.In Chicago,Chern and Lashof[12]studied the concept of tight embedding of hypersurfaces in Euclidean spaces.This work was generalized and continued by Kuiper and Banchoﬀ.Berkeley Days and Return to ChinaChern moved to Berkeley in1961.He spent all his years in Berkeley until his retirement in1979; he served at the math department for three more years after his retirement.The arrival of Chern and Smale in Berkeley coincided with the period when the mathematics department at Berkeley rose to become a major leading department in the world. Building on the strength of the existing faculties hired by Evans,Tarski,Morrey,Kelly,and others, Chern had hired many outstanding geometers and topologists who set up Berkeley to be the center in geometry and topology.Berkeley in the1960s was an extraordinarily exciting place for people interested in geometry:students,faculty,and vis-iting mathematicians alike.The graduate students felt as if they were in the center of the universe of geometry.Everyone else in the world of geometry came to visit.Chern trained many outstanding students in the period when he was in Berkeley.This group includes Garland,do Carmo,Shiﬀman,Weinstein, Banchoﬀ,Millson,S.Y.Cheng,Peter Li,Web-ster,Donnelly,and Wolfson,not counting myself. Chern organized the training of his Ph.D.students through the help of some of his older students and his friends.For example,Garland got advice from H.C.Wang,Millson from Jim Simons.The charm of Chern was essential to keeping this large group of outstanding geometers working together in Campbell Hall and in Evans Hall in Berkeley.The geometry seminar and the colloquium in Berkeley were always packed with students,faculty mem-bers,and visitors.It is well known that Chern treated every visitor with a splendid dinner in a Chinese restaurant or else an elegant party in his house.His wife was able to entertain everybody with grace and nice Chinese food.This period of Berkeley days is unforgettable to two generations of geometers.In Berkeley,Chern studied minimal surface the-ory along with Calabi and Osserman[14].He also tried to generalize Nevanlinna theory to a broader setting;his eﬀort led to the discovery of the Bott-Chern form and the Chern-Levine-Nirenberg intrinsic norms;all of these have had much impact in complex geometry beyond the original purpose. His work with Simons has had deep inﬂuence in geometry and physics,including knot theory. The work with Moser[13]on local invariants of real hypersurfaces in complex Euclidean spaces is fundamental in several complex variables.He and Griﬃths[11]generalized his old work on web geometry.In the early eighties,along with Singer and Moore,Chern founded MSRI at ter,he retired and returned to China.In China he formed a mathematics center in Nankai.That center is considered to be successful and inﬂuential. ConclusionChern’s ability to create invariants for impor-tant geometric structures was unsurpassed by any mathematician whom I have ever known.His works on the Gauss-Bonnet formula,on Chern classes,on projective diﬀerential geometry,on aﬃne geometry,and on Chern-Moser invariants for pseudo-convex domains demonstrate his strength. Before he died,he had embarked on the major un-dertaking of applying the Cartan-Kähler system to a more general geometric setting.Chern once said:“The importance of complex numbers in geometry is a mystery to me.It is well organized and complete.”Chern always regretted that ancient Chi-nese mathematicians never discovered complex numbers.Chern’s everlasting works in complex geometry made up for the earlier losses by Chinese mathematics over the last two thousand years.The Chinese astronomers named a star after Chern.May his accomplishments always shine on the future generations of mathematicians. References[1]C.B.Allendoerfer,The Euler number of aRiemann manifold,Amer.J.Math.62(1940),243–248.[2]C.B.Allendoerfer and A.Weil,The Gauss-Bonnet theorem for Riemannian polyhedra,Trans.Amer.Math.Soc.53(1943),101–129.[3]O.Bonnet,Mémoire sur la théorie généraledes surfaces,J.Ecole Polytech.19(1848),1–146.[4]R.Bott and S.-S.Chern,Hermitian vectorbundles and the equidistribution of the zeroesof their holomorphic sections,Acta Math.114(1965),71–112.[5]S.-S.Chern,On integral geometry in Kleinspaces,Ann.of Math.43(1942),178–189.[6],Characteristic classes of Hermitian manifolds,Ann.of Math.47(1946),85–121.[8],On the characteristic classes of com-plex sphere bundles and algebraic varieties,Amer.J.Math.75(1953),565–597.1230Notices of the AMS Volume58,Number9[10]F.Hirzebruch is professor emeritus at the Universität Bonn and director emeritus of the Max-Planck-Institut für Mathematik in Bonn.His email address is hirzebruch@mpim-bonn.mpg.de.c i=0for i≥n−r+1.For real diﬀerentiable man-ifolds such questions are treated in the disserta-tion of Hopf’s student Stiefel[4],later a well-known computer scientist.For a compact complex mani-fold X of dimension n,the n-dimensional products of the Chern classes of the tangent bundle(all di-mensions complex)give the Chern numbers,when integrated over X,for example c n[X]is the Euler-Poincarécharacteristic(Poincaré-Hopf theorem).From1950to1952I was scientiﬁc assistant in Erlangen and wrote the paper[6]where ideas of Hopf entered[2].Some of the results could have been generalized to higher dimensions.But the so-called“duality formula”was not yet proved. This formula says that the total Chern class1+c1+ c2+···of the direct sum of two complex vector bundles equals the product of the total Chern classes of the summands.The paper[6]has a remark written during proofreading that Chern and Kodaira told me that the“duality formula”is proved in a forthcoming paper of Chern[7].In the commentary to my paper[6]in volume1of my Collected Papers(Springer1987),I write that my knowledge about Chern classes increased with the speed of aﬂash when I came to Princeton in August1952as a member of the Institute for Advanced Study and talked with K.Kodaira,D.C. Spencer,and,a little later,with A.Borel,who told me about his thesis containing his theory about the cohomology of the classifying spaces of com-pact Lie groups.For the unitary group U(n),this implies that the Chern class c i can be considered in a natural way as the i th elementary symmetric function in certain variables x1,x2,...,x n.My two years(1952–54)at the Institute for Ad-vanced Study were formative for my mathematical career([8],[9]).I had to study and develop funda-mental properties of Chern classes,introduced the Chern character,which later(joint work with M.F. Atiyah)became a functor from K-theory to rational cohomology.I began to publish my results in1953. The main theorem is announced in[10].It concerns the Euler number of a projective algebraic variety V with coeﬃcients in the sheaf of holomorphic sec-tions of a complex analytic vector bundle W over V. Chern classes everywhere!I quote from[10]:“The main theorem expresses this Euler-Poincaréchar-acteristic as a polynomial in the Chern classes of the tangential bundle of V and in the Chern classes of the bundle W.”The Chern classes accompanied me throughout all my mathematical life;for example:In2009I gave the annual Oberwolfach lecture about Chern classes[11].Myﬁancée joined me in Princeton in November 1952.We married.A“marriage tour”was orga-nized,for which Spencer gave me some support from his Air Force project.I lectured in seven places during this trip,including Chicago,where we met the great master Shiing-Shen Chern and hisOctober2011Notices of the AMS1231charming wife.He was forty-one,I was twenty-ﬁve. For me he was a gentleman advanced in age.But all shyness disappeared.He was interested in my progress in Princeton about which I also talked in my lecture.We must have spoken about his papers[3]and[7].Chern begins in[3]with a study of the Grassmannian H(n,N)of linear subspaces of dimension n in the complex vector space of dimension n+N.He deﬁnes the Chern classes of the n-dimension tautological bundle over the Grassmannian in terms of Schubert calculus.From here Chern comes to the deﬁnition using r-tuples of sections.For N→∞,the Grassmannian be-comes the classifying space of U(n),and we are close to what I learned from Borel.For Hermit-ian manifolds Chern shows how to represent the Chern classes by diﬀerential forms.The paper[7]has the following deﬁnition of Chern classes:Let E be a complex vector bundle of dimension n over the base B.Let P be the associated projective bundle withﬁber P n−1(C). Let L be the tautological line bundle over P and g=−c1(L).Then g restricted to theﬁber of P is the positive generator of H2(P n−1(C),Z).Integra-tion of g n−1+m over theﬁber in P gives¯c m,the m th “dual”Chern class of E.The total“dual”Chern class¯c=1+¯c1+¯c2+···is deﬁned byc·¯c=1.If B=H(n,N),then¯c is the total Chern class of the complementary N-dimensional tautological bundle over B.Chern uses this to prove that the Chern classes are represented by algebraic cycles if everything happens in the projective algebraic category.The Cherns invited my wife and me for dinner in their home.For theﬁrst time we enjoyed the cook-ing of Mrs.Chern.Many meals in Berkeley would follow.The Chern family,with their two children in1950,can be seen in the photograph on page XX of his Selected Papers(Springer1978).Chern presented me a copy of this book with the dedica-tion“To Fritz.Warmest regards.June1979”.The signature is in Chinese characters.During1955–56I was an assistant professor at Princeton University.I gave a course on my book [12].Chern and Serre attended at least occasion-ally.Chern,Serre,and I wrote a paper,“On the index of aﬁbered manifold”,which was submitted in September1956[13].There the multiplicativity of the signature[=index]is proved forﬁbrations of compact connected oriented manifolds provided the fundamental group of the base acts trivially on the rational cohomology of theﬁber.In1960Chern became a professor in Berkeley.I visited him there in1962,1963,1967,1968,1973, 1974,1979,1983,1986,and1998,always with part of my family.Chern inspired an oﬃcial oﬀer to me by the University of California(November1968). He wrote to me:“We all hope that you willﬁnd Berkeley suﬃciently attractive to deserve your seri-ous consideration.Some disturbances are expected but they need not concern you.I am going to sub-mit to the NSF a new proposal for research support and will be glad to include you in the proposal.”In Bonn I was very involved in discussions with the protesting students and expected to have a qui-eter life in Berkeley as a new faculty member with more time for mathematics.Finally I decided to stay in Bonn.Chern was very disappointed.But the invitations to Berkeley continued.The Cherns were always very helpful in many practical problems: picking us up at the airport,ﬁnding a house,lend-ing us things useful for housekeeping,even lend-ing us a car,depositing items in their house we had bought to be used during the next visit….We enjoyed the Cherns’hospitality in their beautiful home in El Cerrito,overlooking the Bay with the famous Bay Bridge,or in excellent Chinese restau-rants in Berkeley and Oakland where the Cherns were highly respected guests.There were always interesting conversations with the Cherns and the other dinner guests.In1979there was a conference,“The Chern Symposium”,on the occasion of Chern’s retire-ment as a professor of the university.In the Proceedings[14]I.M.Singer writes:“The confer-ence also reﬂected Professor Chern’s personality, active yet relaxed,mixed with gentleness and good humor.We wish him good health,a long life,hap-piness,and a continuation of his extraordinary deep and original contributions to mathematics.”This came also from my heart.Chern did not really retire.In1981he became theﬁrst director of the Mathematical Sciences Re-search Institute in Berkeley.When the MSRI build-ing was ready,I sometimes used Chern’s beautiful oﬃce with a wonderful view.In1981I nominated Chern for the“Alexander von Humboldt-Preis”.He received it and spent part of the summers of1982and1984in Bonn. He talked at the Arbeitstagungen of these years on the topics“web geometry”and“some applications of the method of moving frames”.In1998I was invited to be one of theﬁrst Chern professors in Berkeley.These visiting professor-ships areﬁnanced by Robert G.Uomini,a former student of Chern,who had won an enormous sum in the lottery.In my case a one-day Chern sympo-sium was held,followed by a four-week course.The title of my Chern lecture in the symposium was “Why do I like Chern classes?”I gave four answers:(1)The Chern classes remind me of my youth.I hope this became clear in the beginningof this contribution.(2)The Chern classes have so many diﬀerentdeﬁnitions.As a joke I added:I especiallylike that all these deﬁnitions are equivalent.There are the deﬁnitions in Chern’s pa-pers[3]and[7].The statement in the joke1232Notices of the AMS Volume58,Number9needed some work,which was carriedout by Borel and me and perhaps by oth-ers,too.The diﬃculty consisted in signquestions:Are we dealing with a complexvector bundle V or its dual V∗?(3)“Chern has a beautiful character.”There was the story that during a lectureabout K-theory and its functor ch to ra-tional cohomology I cried out,“Chern hasa beautiful character!”Chern was presentand smiled.(4)Chern classes have so many applications.In1998Chern was eighty-seven years old.He did not appear so old to me.He came to my Chern lecture and also to some lectures in my four-week course.The Cherns came to an oﬃcial dinner.They invited us to a Chinese restaurant.Berkeley1998!The last time I saw Chern.The Cherns gradually moved to China.But we stayed in contact.We edited the two vol-umes on the Wolf Prize winners in mathematics (published by World Scientiﬁc in2000and2001).My retirement as director of the Max Planck In-stitute for Mathematics in Bonn in1995was cele-brated by a“party”with informal lectures,perfor-mances,music,lunches and dinners organized by Don Zagier.It lasted two or three days.Zagier had the idea to produce a book with essays or short statements by the participants and by some other people who could not attend.Chern did not come. But one page is by him(see Figure1).In2005the School of Mathematics of the In-stitute for Advanced Study in Princeton had its seventy-ﬁfth anniversary.Of the older members Chern,Bott,Hirzebruch,and Atiyah were invited to present to the inner circle how the time at the Institute was formative for their careers,Chern by television.But he died in2004.I also gave a math-ematical lecture in which Borel and Chernﬁgured prominently.Chern classes everywhere!Borel and I had shown in the1950s how to calculate the Chern classes and the Chern numbers of compact complex homogeneous spaces.An example(in a formulation by E.Calabi):Let X be the projective contravari-ant tangent bundle of P3(C)andY the projective covariant tangentbundle.Then the Chern number c51of theseﬁve-dimensional complexhomogeneous spaces X and Y,re-spectively,equals4500and4860.This is interesting because X andY are diﬀeomorphic(compare[11]and the work of D.Kotschick men-tioned there).Remark.It is unavoidable that this contribution has some overlap with[15]and with myinterviewFigure1.about Chern of December6,2010,here in Bonn [Zala Films with George Csicsery for MSRI]. References[1]F.Hirzebruch,Berichtüber meine Zeit in derSchweiz in den Jahren1948–1950,In:math.ch/100.Schweizerische Mathematische Gesellschaft1910–2010.EMS Publishing House,2010,303–315.[2]H.Hopf,Zur Topologie der komplexen Mannig-faltigkeiten,In:Studies and Essays presented to R.Courant.New York1948,167–185.[3]S.S.Chern Characteristic classes of Hermitianmanifolds,Amer.J.Math.47(1946),85–121.[4]E.Stiefel,Richtungsfelder und Fernparallelis-mus in n-dimensionalen Mannigfaltigkeiten,Comm.Math.Helvetici8(1935/36),305–353.[5]N.Steenrod,The topology ofﬁbre bundles,Princeton Math.Ser.14,Princeton Univ.Press,1951.[6]F.Hirzebruch,Übertragung einiger Sätze ausder Theorie der algebraischen Flächen auf kom-plexe Mannigfaltigkeiten von zwei komplexen Dimensionen,J.Reine Angew.Math.191(1953), 110–124.[7]S.-S.Chern,On the characteristic classes of com-plex sphere bundles and algebraic varieties,Amer.J.Math.75(1953),565–597.[8]F.Hirzebruch,The signature theorem:Reminis-cences and recreation.In:Prospects in Mathematics.Ann.Math.Stud.70(1971),3–31.[9],Arithmetic genera and the theorem of Riemann-Roch for algebraic varieties,PNAS40 (1954),110–114.October2011Notices of the AMS1233。

[美]R·格伦·哈伯德《宏观经济学》R.GlennHubbard,AnthonyP

Macroeconomics R. GLENN HUBBARD COLUMBIA UNIVERSITY ANTHONY PATRICK O’BRIEN LEHIGH UNIVERSITY MATTHEW RAFFERTY QUINNIPIAC UNIVERSITY Boston Columbus Indianapolis New York San Francisco Upper Saddle RiverAmsterdam Cape Town Dubai London Madrid Milan Munich Paris Montreal Toronto Delhi Mexico City So Paulo Sydney Hong Kong Seoul Singapore Taipei TokyoAbout the AuthorsGlenn Hubbard Professor Researcher and Policymaker R. Glenn Hubbard is the dean and Russell L. Carson Professor of Finance and Economics in the Graduate School of Business at Columbia University and professor of economics in Columbia’s Faculty of Arts and Sciences. He is also a research associate of the National Bureau of Economic Research and a director of Automatic Data Processing Black Rock Closed- End Funds KKR Financial Corporation and MetLife. Professor Hubbard received his Ph.D. in economics from Harvard University in 1983. From 2001 to 2003 he served as chairman of the White House Council of Economic Advisers and chairman of the OECD Economy Policy Commit- tee and from 1991 to 1993 he was deputy assistant secretary of the U.S. Treasury Department. He currently serves as co-chair of the nonpar-tisan Committee on Capital Markets Regulation and the Corporate Boards Study Group. ProfessorHubbard is the author of more than 100 articles in leading journals including American EconomicReview Brookings Papers on Economic Activity Journal of Finance Journal of Financial EconomicsJournal of Money Credit and Banking Journal of Political Economy Journal of Public EconomicsQuarterly Journal of Economics RAND Journal of Economics and Review of Economics and Statistics.Tony O’Brien Award-Winning Professor and Researcher Anthony Patrick O’Brien is a professor of economics at Lehigh University. He received a Ph.D. from the University of California Berkeley in 1987. He has taught principles of economics money and banking and interme- diate macroeconomics for more than 20 years in both large sections and small honors classes. He received the Lehigh University Award for Distin- guished Teaching. He was formerly the director of the Diamond Center for Economic Education and was named a Dana Foundation Faculty Fel- low and Lehigh Class of 1961 Professor of Economics. He has been a visit- ing professor at the University of California Santa Barbara and Carnegie Mellon University. Professor O’Brien’s research has dealt with such issues as the evolution of the U.S. automobile industry sources of U.S. economiccompetitiveness the development of U.S. trade policy the causes of the Great Depression and thecauses of black–white income differences. His research has been published in leading journals in-cluding American Economic Review Quarterly Journal of Economics Journal of Money Credit andBanking Industrial Relations Journal of Economic History Explorations in Economic History andJournal of PolicyHistory.Matthew Rafferty Professor and Researcher Matthew Christopher Rafferty is a professor of economics and department chairperson at Quinnipiac University. He has also been a visiting professor at Union College. He received a Ph.D. from the University of California Davis in 1997 and has taught intermediate macroeconomics for 15 years in both large and small sections. Professor Rafferty’s research has f ocused on university and firm-financed research and development activities. In particular he is interested in understanding how corporate governance and equity compensation influence firm research and development. His research has been published in leading journals including the Journal of Financial and Quantitative Analysis Journal of Corporate Finance Research Policy and the Southern Economic Journal. He has worked as a consultantfor theConnecticut Petroleum Council on issues before the Connecticut state legislature. He has alsowritten op-ed pieces that have appeared in several newspapers including the New York Times. iii Brief Contents Part 1: Introduction Chapter 1 The Long and Short of Macroeconomics 1 Chapter 2 Measuring the Macroeconomy 23 Chapter 3 The Financial System 59 Part 2: Macroeconomics in the Long Run: Economic Growth Chapter 4 Determining Aggregate Production 105 Chapter 5 Long-Run Economic Growth 143 Chapter 6 Money and Inflation 188 Chapter 7 The Labor Market 231 Part 3: Macroeconomics in the Short Run: Theory and Policy Chapter 8 Business Cycles 271 Chapter 9 IS–MP: A Short-Run Macroeconomic Model 302 Chapter 10 Monetary Policy in the Short Run 363 Chapter 11 Fiscal Policy in the Short Run 407 Chapter 12 Aggregate Demand Aggregate Supply and Monetary Policy 448 Part 4: Extensions Chapter 13 Fiscal Policy and the Government Budget in the Long Run 486 Chapter 14 Consumption and Investment 521 Chapter 15 The Balance of Payments Exchange Rates and Macroeconomic Policy 559 Glossary G-1 Index I-1ivContentsChapter 1 The Long and Short of Macroeconomics 1WHEN YOU ENTER THE JOB MARKET CAN MATTER A LOT ........................................................ 11.1 What Macroeconomics Is About........................................................................... 2 Macroeconomics in the Short Run and in the Long Run .................................................... 2 Long-Run Growth in the United States ............................................................................. 3 Some Countries Have Not Experienced Significant Long-Run Growth ............................... 4 Aging Populations Pose a Challenge to Governments Around the World .......................... 5 Unemployment in the United States ................................................................................. 6 How Unemployment Rates Differ Across Developed Countries ......................................... 7 Inflation Rates Fluctuate Over Time and Across Countries................................................. 7 Econo mic Policy Can Help Stabilize the Economy .. (8)International Factors Have Become Increasingly Important in Explaining Macroeconomic Events................................................................................. 91.2 How Economists Think About Macroeconomics ............................................. 11 What Is the Best Way to Analyze Macroeconomic Issues .............................................. 11 Macroeconomic Models.................................................................................................. 12Solved Problem 1.2: Do Rising Imports Lead to a Permanent Reductionin U.S. Employment. (12)Assumptions Endogenous Variables and Exogenous Variables in EconomicModels ........................................................................................................ 13 Forming and Testing Hypotheses in Economic Models .................................................... 14Making the Connection: What Do People Know About Macroeconomicsand How Do They KnowIt .............................................................................................. 151.3 Key Issues and Questions of Macroeconomics ............................................... 16An Inside Look: Will Consumer Spending Nudge Employers to Hire................................ 18Chapter Summary and Problems ............................................................................. 20 Key Terms and Concepts Review Questions Problems and Applications Data Exercise Theseend-of-chapter resource materials repeat in all chapters.Chapter 2 Measuring the Macroeconomy 23HOW DO WE KNOW WHEN WE ARE IN ARECESSION ........................................................... 23Key Issue andQuestion .................................................................................................... 232.1 GDP: Measuring Total Production and Total Income ..................................... 25 How theGovernment Calculates GDP (25)Production and Income (26)The Circular Flow of Income (27)An Example of Measuring GDP (29)National Income Identities and the Components of GDP (29)vvi CONTENTS Making the Connection: Will Public Employee Pensions Wreck State and Local Government Budgets.................................................................... 31 The Relationship Between GDP and GNP........................................................................ 33 2.2 Real GDP Nominal GDP and the GDP Deflator.............................................. 33 Solved Problem 2.2a: Calculating Real GDP . (34)Price Indexes and the GDP Deflator (35)Solved Problem 2.2b: Calculating the Inflation Rate ..........................................................36 The Chain-Weighted Measure of Real GDP ....................................................................37 Making the Connection: Trying to Hit a Moving Target: Forecasting with “Real-Time Data” .................................................................................. 37 Comparing GDP Across Countries................................................................................... 38 Making the Connection: The Incredible Shrinking Chinese Economy ................................ 39 GDP and National Income .............................................................................................. 40 2.3 Inflation Rates and Interest Rates ....................................................................... 41 The Consumer Price Index .............................................................................................. 42 Making the Connection: Does Indexing Preserve the Purchasing Power of Social Security Payments ................................................................ 43 How Accurate Is theCPI ............................................................................................... 44 The Way the Federal Reserve Measures Inflation ............................................................ 44 InterestRates .................................................................................................................. 45 2.4 Measuring Employment and Unemployment .. (47)Answering the Key Question ............................................................................................ 49 An Inside Look: Weak Construction Market Persists.......................................................... 50 Chapter 3 The Financial System 59 THE WONDERFUL WORLD OFCREDIT ................................................................................... 59 Key Issue and Question .................................................................................................... 59 3.1 Overview of the Financial System ...................................................................... 60 Financial Markets and Financial Intermediaries ................................................................ 61 Making the Connection: Is General Motors Making Cars or Making Loans .................... 62 Making the Connection: Investing in the Worldwide Stock Market . (64)Banking and Securitization (67)The Mortgage Market and the Subprime Lending Disaster (67)Asymmetric Information and Principal–Agent Problems in Financial Markets...................68 3.2 The Role of the Central Bank in the Financial System (69)Central Banks as Lenders of Last Resort ..........................................................................69 Bank Runs Contagion and Asset Deflation ....................................................................70 Making the Connection: Panics Then and Now: The Collapse of the Bank of United States in 1930 and the Collapse of Lehman Brothers in2008 (71)3.3 Determining Interest Rates: The Market for Loanable Funds and the Market forMoney .......................................................................................... 76 Saving and Supply in the Loanable Funds Market ........................................................... 76 Investment and the Demand for Loanable Funds ............................................................ 77 Explaining Movements in Saving Investment and the Real Interest Rate (78)CONTENTS .。

Asymptotic Theory for Quantum Bose Systems with Many Degrees of Freedom

s s s
=
i
ℓ,s,m,q
∂ ∂α
m
− α∗m αs ∂ ∂α |α|2
ℓ
∂ ∂α∗ α∗m αq
q
∂ ∂α |α|2
ℓ
exp
|α|2
=
i
ℓ,s,m,q
Hℓs Fmq α∗ℓ
α∗m αq − αs ∂ ∂α
ℓ
exp
=
i
ℓ,s
Hℓs
− αs
exp
α|F (t)|α .
In a slightly more general setting, let H = opWick H(z ∗ , z ) For H as in (1.1) this means H(z ∗ , z ) = Hℓs z ∗ℓ z s
+
d Φα |F Φα dt i = Φα |(HF − F H )Φα = = i
ℓ,s,m,q
(1.7)
Hℓs Fmq (t)
Φα |a†ℓ as a†m aq Φα − Φα |a†m aq a†ℓ as Φα
i
ℓ,s,m,q
Hℓs Fmq α∗ℓ αq a†s Φα |a†m Φα − α∗m αs a†q Φα |a†ℓ Φα Hℓs Fmq α∗ℓ αq α∗ℓ ∂ ∂α∗ ∂ ∂α∗ ∂ ∂α∗
∗
For the purpose of the present paper it will be suﬃcient to treat the case when H is a polynomial in z ∗ , z . We will address the mathematical problems related to a more general H in future publications. In case H(z ∗ , z ) is a polynomial, the equation (1.9) has the following explicit form: d α|F |α = dt i =

学术英语（第二版）医学教师用书Unit8

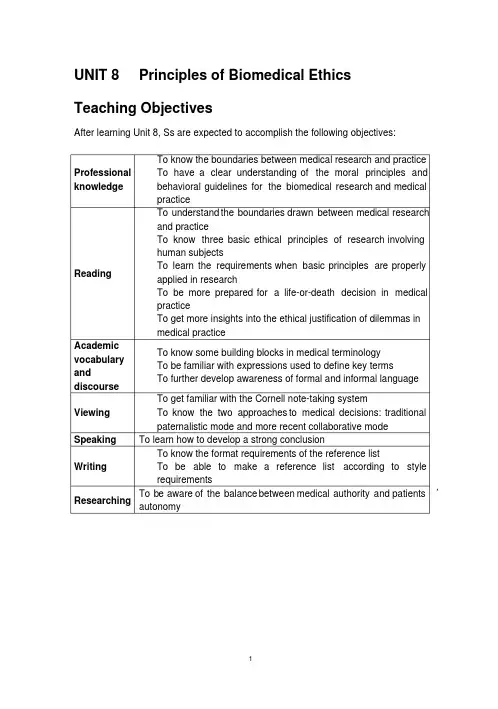
UNIT 8 Principles of Biomedical Ethics Teaching ObjectivesAfter learning Unit 8, Ss are expected to accomplish the following objectives:Professional knowledge To know the boundaries between medical research and practice To have a clear understanding of the moral principles and behavioral guidelines for the biomedical research and medical practiceReading To understand the boundaries drawn between medical research and practiceTo know three basic ethical principles of research involving human subjectsTo learn the requirements when basic principles are properly applied in researchTo be more prepared for a life-or-death decision in medical practiceTo get more insights into the ethical justification of dilemmas in medical practiceAcademic vocabulary and discourse To know some building blocks in medical terminologyTo be familiar with expressions used to define key termsTo further develop awareness of formal and informal languageViewing To get familiar with the Cornell note-taking systemTo know the two approaches to medical decisions: traditional paternalistic mode and more recent collaborative modeSpeaking To learn how to develop a strong conclusionWriting To know the format requirements of the reference listTo be able to make a reference list according to style requirementsResearching To be aware of the balance between medical authority and patients’　autonomyTeaching Activities and ResourcesPart 1 ReadingText ALead-inSuggested teaching plan1.To draw Ss’　a ttention and to raise their awareness of the importance ofbiomedical ethics, T is advised to relate the discussion of this unit to the real-world happenings. Before starting the class, search the media for the latest news reports, either at home or abroad, about controversial events in medicine community or healthcare settings.2.Start the class by doing T ask / Lead-in and relate the content of the video clip toyour findings in the pre-class searching.Key to the task2) Death4) Patient rightsScriptWell, advancements in medical science have afforded us the opportunity to live decades l onger than in previous generations. For every new possibility offered, we now face an equal number of challenges and we find ourselves confronting decisions that are unprecedented in human history. When does life begin? When should life end? How do we define death when we have the ability to keep people technically alive, or we should say, technologically alive long after their discrete.”I’m your host body parts no longer function? Welcome to “Matter and Beyondaround medical MaryLynn Schiavi. In this program we’re going to explore issuesscience that are forcing us to define life, death, quality of life, patient rights, and confront the moral and ethical questions that arise when facing critical healthcare decisions.3.Introduce the topic of Text A as a natural continuum of Lead-in.Text ComprehensionSuggested teaching plan1.Make good use of Lead-in video clip as it serves as a perfect introduction to thetopic of this unit. Elaborate on the connection of its content with the latest events in the real world. Naturally, ask Ss how medicine differs from other branches of natural science, especially when human subjects are involved in the research.Here are some hints:Medicine Other branches of science Health as the ultimate goal Knowledge and truth as the ultimate goal Morality and ethics as priority factors Honesty as a prime virtue of scientistsDoctor as a teacher, counselor, friend,advocate, protector, and healer all atthe same timeScientist as an explorerGood doctor: empathic, sympathetic, caring, patient, considerate Good scientist: inquisitive, persistent, perseverant, creative2.Analyze the text and lead Ss to discuss, integrating Task 2 / Critical reading andthinking / Text A into analysis and discussion. The presentation topics should be assigned to individual Ss for preparation at least one week in advance. Ask other Ss to preview the text with the guidance of presentation topics.3.Integrate Task 2 / Language building-up / Text A when a careful definition ofkey terms is covered.4. When analyzing the text, ask Ss to pay special attention to the sentences listed inLanguage focus below.5.If time allows, ask Ss to do Task 1 / Critical reading and thinking / Text A inabout five minutes. Check out the task by asking one or two Ss to read their answers. This is done to get an overview about the text.Language focus1.… described in a formal protocol that sets forth an objective … (P185, Para.2)set forth是动词词组，表示用清晰、具体的方式解释或描述，多用于正式的书面语中。

心理学专业外语：The behaviourist approach to psychology

The behaviourist approach to psychology心理学的行为主义研究方法J.B.Watson：‘Give me a dozen healthy infants……and my own specified world to bring them up in and I’ll guarantee to take any one at random and train him to become any type of specialist I might select-doctor, lawyer…and yes, even beggarman and thief. ’华生：“给我一打健全的婴儿和我可用以培育他们的特殊世界，我就可以保证随机选出任何一个，我都可以把他训练成为我所选定的任何类型的特殊人物如医生、律师……或甚至乞丐、小偷。

”Origins and history1.起源与历史The behaviourist approach was influenced by the philosophy of empiricism （which argues that knowledge comes from the environment via the senses,since humans are like a ‘tabula rasa’,or blank slate,at birth）and the physical sciences（which emphasise scientific and objective methods of investigation）.行为主义的研究方法受到经验主义哲学和物理科学的影响。

前者主张知识来自于感官接受的环境信息，认为人类在出生时像一块白板或空白黑板，后者强调研究方法的客观性和科学性。

Watson started the behaviourist movement in 1913 when he wrote an article entitled ‘Psychology as the behaviourist views it’,which set out its main principles and assumptions.Drawing on earlier work by Pavlov,behaviourists such as Watson,Thorndike and Skinner proceeded to develop theories of learning（such as classical and operant conditioning）that they attempted to use to explain virtually all behaviour.华生在1913年发表的论文《一个行为主义研究者眼中的心理学》标志着行为主义运动的开始，文中阐述了行为主义的主要原理和假设。

中英文论文写作(摘要)

· Write the paper before you write the abstra
1 基本特性 2 时态 3 语态 4 语法修辞 5 一个典型示例 6 化学常用句式
2 时态
以一般现在时为主, 也使用一般过去时和现在完成时从理论上讲: 说法一: 一般现在时
通过科学实验取得的研究结果、结论，揭示自然界的客观规律一般过去时
Objective 动词不定式开头 To investigate … To study … To explore … To examine … To determine … To report … To review … 使用第一人称时，用凡指的we, the author, the authors ,不用I。如: In this paper we conclude …
Result: The contents of the components of midecamyc A1 and leucomycin A6 was 30%～ 50% and 10%～20% respectively, the contents of the rest components w lower, different manufactures produces have differ components. Conclution: To revise the specificati meleumycin for quality control.
指示性文摘（indicative abstract）
This type of abstract is designed to ind the subject of a paper, making it easy fo potential readers to decide whether to r the paper.

reflexives提纲

Chapter 11. Purpose of the study2. Generalization3. Method of studyChapter21. Standard of identifying the reflexives1.1 Incomplete definition of reflexive不完全的反身代词定义1.2 Definitions, discrimination of reflexives in English and In ChineseChapter 3 Analyze the reflexives1)Under the constrains theory (under the jurisdiction, out of jurisdiction)( method of usage)（semantics）2）Under the recognition theory-self, intensive reflexives（logics of recognition, syntactic demonstration and semantic quality）3）Under the practical acquisition theoryExperiment procedureDiscussionSummaryChapter 4 ConclusionChapter 11. Purpose of the studyThe restriction relationship between the anaphora and antecedent has long been a touchy issue in the study of linguistics. We have known that Chomsky erected and developed his theory –constraint theory-based on the conception of governing that defines the anaphora must be constrained in the governing category. And, this theory is commonly called the first constrain theory, which arose a great repercussion in the world wide. We, naturally, did not want to fall behind of this trend of academics and have begun to study it . However, there was a misconception that the Chinese “ziji”should be identified as a reflexive after our making our first step to this area of the study, for the reason that it is constrained in its own governing category.But, after a gradual study, we have found so many exceptions to this identification that enough to overthrow the absolute ascription. Chinese, interestingly, have both similarities and differences between English and, without doubt, have its own characteristics of the reflexives and some of them can hardly been ascribe into the absolute reflexives in English context. In another word, it has some characteristic differs from normal definition of the reflexives. Give those differences; it is understandable that the practical acquisition study is being explored by academicians. We hoped that we can derive objective and accurate from this perspective.This article will aim at analyzing the reflexives in constrains theory, primarily focusing on the sentence construction, syntactic, semantic perspectives. Furthermore, it goes on to illustratesome cases of the practical acquisition to fuller the analysis of the reflexives on both side .Within this exploration, we might able to get relatively more accurate and comprehensive, objective understanding of the reflexives, which help us to further our understanding of mother tongue language and the international English.2 The generalizations on the present study of Reflexive.2.1 Present study of reflexives on sentence structure2.1 .1Present English reflexives study on sentences structureWe are primarily focusing on the Chomsky’s governing conception. He used conception of governing category to constrain the action, distribution and role of reflexives in the sentences. His idea can be briefly summarized as following points:A: Anaphora is constrained by government category.B: general pronoun is not constrained by governing category.Antecedent is the one that command the anaphora. The principle of command usually can be described as following:2) X commands Y, only when Y is ascribed and belong to the first divergent point, as well as the X and Y do not belong to each other.Figure 1 S represents Sentence.N represents a single word class, such as N, A, V, and P, and can also represent the phrase language class, such as the NP, AP, the VP, and PP. In the way to S, N1 and, N2 are nodes. Principle: each node commands the same level node; it cannot overreach their right and control their superiors or his immediate subordinates, so S cannot control NI, N2, and N3 .second. In Figure 1 the N1 is subject, commanding the entire predicate phrase.In the example of “Sara’s mother hates herself” the restriction is commanded by “Sara’s mother”, not “Sara’s”.So anaphora must be restricted in the governing category, and according to this quality, Zribi-hertz defined principle to following test of distributions (T)that can used to test the anaphora is restricted in or out of category.T1 anaphora has no ability of discriminate.Wash him （*himself）T2 Anaphora must have antecedent in the governing category.Sara has just finished, but you may catch her up tomorrow.T3 Anaphora must by command by its antecedent.Sara’s mother hates her （*himself）Sara’s mother hates herself.T4 only anaphora can be restricted in the governing category.Sara likes herself.T5 Anaphora can only be restricted in the governing category.a. Sara, insists that Judy likes her; （*herself）b. Sara insists that Judy likes herself.T6 Anaphora cannot have Split antecedents.Sara, said to Mary about them. (* themselves)According to these, an agreement has been achieved that the anaphora cannot fall into normal category, they seek and find antecedents out of governing category. This is totally different with normal understanding of English anaphora.2.1.2 Present state of the Chinese reflexives’ study on sentence structure1) Pronoun analysisWang Jia Ninng Believed that Chinese reflexives have its own character and cannot be easily ascribed to any given existent category. Linguistic follow this opinion want to s eek for another new constrain principle to define reflexives.2) Parameter analysisMazzini and Wexler and yang is the representatives of this method, who insist that governing category can be described through subset principle that has been illustrated by parameter.3) Logic shifting analysisAlthough academician haven’t reach a consensus about how shifting happened, they agree that the long distance constraint “-self” is the result of covert and cyclic shifting under the abstract and logic level. In word, it still has a local constraining relationship.4) Relative subject analysisProgovac and Frank come up with an idea that is non-shifting theory that lately (1992) be revised within the context of Chomsky principles.2.2 .Present reflexives study on semantic level2.2.1English reflexive on semantic levelAcademicians who focused on semantic level using natural language study of systemic-functional grammar ,that is the structure of the language demonstrate the objective Heraclitus combining the prediction quality of semantic, markedness of structure sign, economy in expression, redundancy of semantic and sentence structure, clarity in message transmission to illustrate the usage of English reflexive.2.2.2Chinese reflexive on semantic level(1)Theory of structuralismChen ping , A Chinese scholar believes that the base point and high subjectivity demonstration of antecedent in the sentence actually play an important role in understanding the Chinese reflexive.(2) Thematic hierarchyXu Lie Jiong thinks that the constraint theory cannot solve the “-self” pro blem. Thus, he come up with a new theory that point out that the “-self” plays a thematic roles in constrain function. (3)Pure semantic theoryThis idea, based on Huang Yan’s Neo-Gricean Theory, suggested the pure semantic level of solving the corresponding phenomenon in language. He believes that the corresponding should be explained between DRP and two usage principle of language—information and method.3.Overview of present study on contrast between English and Chinese reflexiveProfessions primarily focus on four perspectives to illustrate the difference between the English and Chinese reflexive.(1)language difference, which means the difference in entirely two languages hold theresponsibility for explaining the English and Chinese reflexive.(2)Parameter difference, which means the various parameter results the different reflexive inChinese and English. Parameterizing led the Chinese and English has various requisite, which make the “-self” have no need to be restricted in that region’s governing categ ory.(3)Shifting difference, which regards the difference as the normal syntactic principle in differentlevel that has constrained many kind of language,” the difference happened in shifting. (4)Vocabulary differences, which attribute “-self” quality is decided by vocabulary differences.“-self” demonstrate the characteristic that have been decided by its born quality that can seldom changed.4.content and analysis method1. content of the studyAccording to the present standard identification method for reflexives, the article wants to give the relatively comprehensive definition of English and Chinese reflexives definition as well as its characteristics. Within this character, we might have the ability to find their similarities and differences. This article also wants to find the origins of the differences without staying in the apparent level of finding those differences.2 analysis method研究方法This article focuses on the theory of reflexives in English and Chinese. We know that any comparison should be built on a level of relatively common level of the two parts, or the comparison will lose its significance. It also has been known that the two comparing part should be stayed in the same level, that is the reason that they can be compared. So, based on the same level of sentence structure, syntactic, semantic analysis we get great abundance of theory analysis. Within this context, the article also lends structuralism theory to analyze and define the reflexives. Moreover, based on the experiment conducted, we derived the practical acquisition theory of both English and Chinese reflexives usage.。

1、下载文档前请自行甄别文档内容的完整性，平台不提供额外的编辑、内容补充、找答案等附加服务。
2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
3、如文档侵犯您的权益，请联系客服反馈,我们会尽快为您处理(人工客服工作时间：9:00-18:30)。

2
Notation and preliminary results
In what follows, we consider an algebraically closed ﬁeld k of characteristic 0; PN is the projective space of dimension N over k. As usual, if F is a coherent sheaf on PN , we will denote by hi (F ) the dimension of the i-th cohomology module H i (F ) as a k-vector space i F the direct sum ⊕ i 0 and by H∗ n∈Z H F (n); in particular H∗ OPN = k [X0 , . . . , Xn ] and for F 0 F has a natural structure of H 0 O coherent sheaf, H∗ ∗ PN -module; if Y is a subvariety (that is N a closed subscheme) in P , we will denote by deg2 (Y ) the degree of the codimension 2 (may be reducible or not reduced) component of Y . We recall some basic properties of Chern classes and reﬂexive sheaves. 1. For every coherent sheaf F on PN , we denote by ci (F ) or simply ci (i = 1, . . . N ) its
Positivity of Chern classes for Reﬂexive Sheaves in Pn
Cristina Bertone-Margherita Roggero Dipartimento di Matematica dell’Universit` a Via Carlo Alberto 10 10123 Torino, Italy
So, we can not expect to control the positivity of the i-th Chern class for every reﬂexive sheaf when i ≥ 4, even if i is lower than the rank. The diﬀerent behavior of a general reﬂexive sheaf F with respect to a vector bundle is clearly due to the presence of its “singular locus” S , that is the set of points where F is not locally free; S is a closed subset of codimension ≥ 3, so that if i > 3 the ith Chern class ci (F ) can be a too little object with respect to it. We might think to apply the same argument also to the third Chern class c3 and even to the lower ones c1 and c2 in case the reﬂexive sheaf (or even the bundle) is not globally generated on some closed subset of small codimension. Thus, it is a little surprising to discover that, on the contrary, c1 , c2 and c3 are positive under the above weaker conditions. In fact, in §3 we obtain the above quoted positivity results for the ﬁrst and second Chern classes of a rank n reﬂexive sheaf F , generated by global sections at least on some (non empty) open subset of PN , as a consequence of more general inequalities involving c1 and c2 . More precisely, for every subsheaf of F isomorphic to a “free” bundle of the type ⊕n i=1 OPN (αi ), we prove sharp lower bounds for c1 and c2 depending on the integers αi and completely classify sheaves for which either c1 or c2 reaches the minimum allowed or some value close to it: they are either free bundles or reﬂexive sheaves having homological dimension ≤ 1 and a very short free resolution (Corollary 3.8). In §4 we obtain similar results on c1 and c2 using a slightly diﬀerent set of hypotheses, also involving the general splitting type of F . Finally in §5 we prove that also the third Chern class c3 cannot be negative if F is generated by global sections “almost everywhere”(Theorem 5.3) and that (under some additional condition on the homological dimension of F ) c3 can vanish only if F is a bundle (Corollary 5.4); this extend to sheaves of any rank on projective spaces of any dimension, a well known property for rank 2 reﬂexive sheaves on P3 (see [3], Proposition 2.6).0 01 Nhomakorabea2
n−2 The sheaf F = G ⊕ OP N is reﬂexive too, it is generated by global sections and it has the same Chern classes as G , so the “even” ones are negative from the fourth on. ′ = G ⊕O For the “odd” Chern classes, we can start from the rank 3 reﬂexive sheaf G1 PN (a) ′ ′ (where G is as above and a ≫ 0) such that c4 (G1 ) > 0. Again, if l ≫ 0, G1 = G1 (l) and n−3 F1 = G1 ⊕ OP N are generated by global sections, their ﬁrst four Chern classes are positive, while the ﬁfth and following “odd” ones are negative.
cristina.bertone@unito.it margherita.roggero@unito.it
arXiv:0709.3218v1 [math.AG] 20 Sep 2007
Abstract It is well known that the Chern classes ci of a rank n vector bundle on PN , generated by global sections, are non negative if i ≤ n and vanish otherwise. This paper deals with the following question: does the above result hold for the wider class of reﬂexive sheaves? We show that the answer is “No ” when i ≥ 4 (no matter of rank): ci can in fact be as negative as we want; on the contrary if i ≤ 3 we are able to prove and extend that property. We obtain sharp, lower bounds for c1 , c2 and c3 for every reﬂexive sheaf F which is generated by global sections on some (non empty) open subset and completely classify sheaves for which either of them reach the minimum allowed, or some value close to it.