Instability criterion for oblique modes in stratified circular Couette flow
REFORMULATIONS OF A BICRITERION EQUILIBRIUM MODEL Patrice
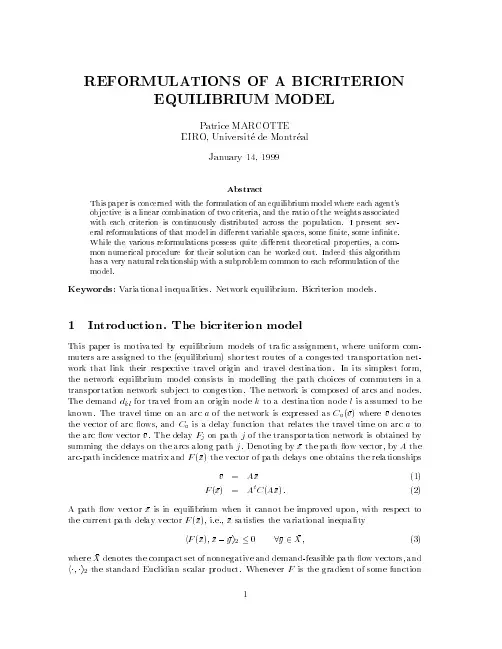
(4)
Various extensions of this basic model have been proposed since its introduction, and the interested reader could do much worse than consult the monograph by Patriksson 9] or the survey by Florian and Hearn 3]. In this paper, I focus on a variant of the tra c assignment model that involves two criteria, travel delay F (x) and out-of-pocket travel cost G(x), to x ideas. Let be a parameter that converts one time unit into one money unit. If this parameter, whose inverse is the monetary value of one time unit, is uniform among the users of the network, then a time-money equilibrium corresponds to the solution of the variational inequality
f , x is a solution of the variational inequality (3) if and only if it satis es the rst-order
optimality conditions associated with the mathematical program min f (y ):
伍德里奇计量经济学名词解释

伍德里奇计量经济学名词解释伍德里奇计量经济学(WoodridgeEconometrics):伍德里奇计量经济学是一种应用数学和统计学方法来分析经济现象和经济数据的学科。
它结合了经济学理论和数学统计学的工具,旨在提供经验性经济分析的定量解释和预测。
一阶自相关(First-orderAutocorrelation):一阶自相关是指一个时间序列中当前观测值与前一个观测值之间的相关性。
在计量经济学中,一阶自相关是对时间序列数据的经济模型进行估计和推断时的一个重要考虑因素。
误差项(ErrorTerm):误差项是指在经济模型中无法被观测到或测量到的影响因素,它代表了模型中未被考虑的其他影响因素对观测结果的影响。
误差项通常假设为随机变量,其期望值为零。
多重共线性(Multicollinearity):多重共线性指的是经济模型中自变量之间存在高度相关性或线性相关性的情况。
多重共线性可能导致模型估计的不稳定性,使得对自变量系数的解释变得困难。
假设检验(HypothesisTesting):假设检验是用于验证经济模型中假设是否成立的统计方法。
通过收集样本数据并进行统计推断,假设检验可以帮助我们判断经济模型中的假设是否支持或拒绝。
平稳性(Stationarity):平稳性是指时间序列数据的统计性质在时间上保持不变的特性。
对于经济数据的分析,平稳性假设是许多计量经济模型的基础之一,它要求数据的均值、方差和协方差不随时间发生显著变化。
识别(Identification):识别是指在经济模型中确定模型参数的唯一性和可估计性。
在伍德里奇计量经济学中,识别是一个重要的问题,它要求我们通过模型设定和数据的限制来确保模型参数能够被准确估计。
异方差性(Heteroscedasticity):异方差性指的是在经济数据中,随着自变量的变化,误差项的方差也发生变化的现象。
酒店运营管理知到章节答案智慧树2023年上海商学院

酒店运营管理知到章节测试答案智慧树2023年最新上海商学院第一章测试1.行业结构不合理造成酒店业过度竞争的现象不存在。
参考答案:错2.对于单体酒店来说,立根的基础是当地的人文和区域特色。
参考答案:错3.完善服务体系,实现“线上+线下”完美的双模式在明确酒店定位后,重要的是提升酒店的软实力,即是酒店的服务水准和附加价值参考答案:对4.互联网思维非常注重人的价值,尤其是对酒店行业来说,抓住接触、沟通和服务客户的各种方式,就是“以人为本”宗旨的最重要体现。
参考答案:对5.未来中高端酒店产品需要考虑的是特色和品质,而不是规模。
参考答案:对6.跨界合作可以为酒店投资人创造更多共赢的市场机会。
参考答案:对7.酒店与互联网的冲突就在于关联性,即是酒店的宣传印象和真实体验的完整度和期望度是否维持在理想的落差之中。
参考答案:错8.互联网+计划的目的在于充分发挥互联网的优势,将互联网与传统产业深入融合,以产业升级提升(),最后实现社会财富的增加。
参考答案:经济生产力9.当前,以( )为标志的民宿民俗风酒店盛行。
参考答案:风景旅游名胜;异国风情;鲜明地域特色10.对于单体酒店来说,立根的基础更多的是(),很少能扩大范围跨越地域传播。
参考答案:当地的人文和区域特色第二章测试1.酒店投资成本不可逆性是指由于投资失败导致投资成本部分或全部变成沉没成本,使得无法收回成本。
参考答案:对2.酒店投资的不确定性是指投资者可以相对清楚的知道未来投资收益状况。
参考答案:错3.酒店投资类型可以多样化,如可以进行酒店的产权投资,也可以非产权投资(租赁)。
参考答案:对4.酒店业主可以通过酒店折旧为酒店收入提供税收庇护。
参考答案:对5.一个酒店项目的开发除了开发商,还需要酒店管理公司负责对项目的论证、建筑及内装设计等工作。
参考答案:错6.为使酒店开发更为科学合理,下列哪个公司应尽早参入酒店项目的规划及建设( )参考答案:酒店咨询及管理公司7.请将下列酒店开发的基本步骤按顺序排列()参考答案:项目运营阶段;项目报批阶段;概念化设计阶段;可行性分析阶段;设计建造阶段8.酒店投资的非系统风险不包括()参考答案:经济风险;市场风险9.酒店投资是一种实物投资,下列哪项描述是正确的的()参考答案:最大投资在其建设期;投资周期长;期望收益高10.下列哪个地方属于酒店的创利面积()参考答案:酒店大堂;酒店餐厅;酒店客房第三章测试1.针对负需求而言,当某地区顾客不需要某种餐饮产品时,餐饮管理人员采取措施,扭转这种趋势称为参考答案:扭转式营销2.菜肴销售分析是通过()和()指数进行参考答案:顾客满意和销售额3.影响餐饮产品的价格因素不包括参考答案:地域4.顾客满意程度高,营业收入水平高的菜品属于参考答案:明星5.以下应该删除的菜品是参考答案:销售额小于 1,顾客满意指数小于 16.以下业务能力属于餐饮部门高层管理能力的范围的是参考答案:市场营销策划;菜单设计与定价;目标预算制定7.企业地理位置、交通条件和就餐环境均属于餐饮部门的可控因素参考答案:错8.餐饮部门是五星级酒店中带来收益最高的部门。
材料、结构力学名词英文

力学 mechanics 牛顿力学 Newtonian mechanics 经典力学 classical mechanics 静力学 statics 运动学 kinematics 动力学 dynamics子波 wavelet 次级子波 secondary wavele 驻波 standing wave声强 intensity of sound 声强计 phonometer 声调 intonation音色 musical quality 音调 pitch 声级 sound level声压[强] sound pressure 声源 sound source 声阻抗 acoustic impedance声抗 acoustic reactance 声阻 acoustic resistance 声导纳 acoustic admittance声导 acoustic conductance 声纳 acoustic susceptance 声共振 acoustic resonance声波 sound wave 超声波 supersonic wave 声速 sound velocity次声波 infrasonic wave 亚声速 subsonic speed又称“亚音速”。
超声速 supersonic speed又称“超音速”。
声呐 sonar 共鸣 resonance回波 echo 回声 echo 拍 beat 拍频 beat frequency群速 group velocity 相速 phase velocity 能流 energy flux能流密度 energy flux density 材料力学 mechanics of materials, strength of materials 应力 stress 法向应力 normal stress 剪[切]应力 shear stress单轴应力 uniaxial stress 双轴应力 biaxial stress 拉[伸]应力 tensile stress压[缩]应力 compressive stress 周向应力 circumferential stress纵向应力 longitudinal stress 轴向应力 axial stress弯[曲]应力 bending stress, flexural stress 扭[转]应力 torsional stress局部应力 localized stress 残余应力 residual stress 热应力 thermal stress最大法向应力 maximum normal stress 最小法向应力 minimum normal stress最大剪应力 maximum shear stress 主应力 principal stress主剪应力 principal shear stress 工作应力 working stress 许用应力 allowable stress应力集中 stress concentration 应力集中系数 stress concentration factor应力状态 state of stress 应力分析 stress analysis结构[强度]分析 structured analysis 应变 strain 剪[切]应变 shear strain法向应变 normal strain 拉[伸]应变 tensile strain 压[缩]应变 compressive strain 体积应变 volumetric strain 残余应变 residual strain 热应变 thermal strain最大法向应变 maximum normal strain 主应变 principal strain主剪应变 principal shear strain 名义应变 nominal strain应变状态 state of strain 载荷 load又称“荷载”。
Gamma1=4 Gamma1=2

In this paper we have described the evolution of the inviscid modes found by Hall and Horseman (1991); this has done using viscous{critical{layer and di usion{layer theories in the context of a weakly nonlinear instability theory. In particular, we have considered the evolution of a mode near the critical streamwise location where the vortex structure has developed su ciently to ( rst) render the now three{dimensional boundary{layer ow unstable to inviscid modes. At such a location, the ow is marginally unstable and we can consider the evolution of the most dangerous (important) mode. We note that our theory is not directly applicable to modes excited at streamwise locations where the ow supports a band of unstable modes (i.e. at an O(1) distance downstream from the critical x{location); in such cases the most dangerous mode has too large a growth rate and the wavenumber will not be close enough to a \neutral' value for weakly{nonlinear theory to be immediately applicable. However, it can be argued that viscous spreading e ects (or some other external e ect) will reduce the growth rates to a size where a weakly nonlinear theory (based on unsteady critical{layer theory rather than viscous critical{layer theory) is appropriate. The papers by, for example, Michalke (1964); Crighton & Gaster (1976) and Hultgren (1992) support such an argument, which has been used in many recent papers concerned with ow stability eg. Goldstein & Leib (1988); Goldstein & Hultgren (1988); Goldstein & Leib (1989); Hultgren (1992); Wu, Lee & Cowley (1993). The evolution of the Hall{Horseman{modes for the non{marginal stability case is the subject of current study by the authors and will be reported on in due course.
船舶专业英语词汇(按英文排列)
螺旋桨整流帽 开槽,开凹口 橹,桨 斜式双柱系缆桩 远洋船 偏离中心的装载 型值 离岸钻井 离岸工程结构物 加油点 浮油回收船 钻油架 甲板上桁架 敞水 最优性准则 矿砂船 矩形的 正交的 外列板 舷外机 侧视图 外首帆 舾装 舾装工 舷外吊杆叉头 总体稳性 外悬 桨 明轮推进的 巴拿马运河 强胸结构,抗拍击结构 强胸横梁 抗拍击纵材 平行中体 局部舱壁 有效载荷 柱,垂直的,正交的 投影照相测量法 打桩船 支柱 限位胎架 销,枢轴 管装工 铺管驳船 活塞 螺距 纵摇
纵剖图 船壳板 船舶装配工 船舶水动力学 船台 船厂 有套罩螺旋桨,导管螺旋桨 舷边肋骨 旁内龙骨 舷侧外板 甲板边板 单缸引擎 升沉 六自由度 表面摩擦力 (气垫船)围裙 砰击 套管,套筒,套环 回转液压马达 一部分,薄片 有坡度船台 底边舱斜顶板 定边舱斜底板 圆舭 声纳 悬挂舵 眼睛型骨架 速长比 舷伸甲板 颤振 稳性 稳定平衡 右舷 静平衡 汽轮船 操纵装置,舵机 船艏 艏柱型线 船艉 尾拖网滚筒 尾突体 尾滑道,尾跳板 尾封板 艉波 加劲,加强 扶强材,加劲杆 跨立,外包式叶片
机械(主机)卖方 磁力式龙门吊 处女航 主推叶轮 主轴系 大型船舶 操纵性 人孔 边板 海事的,海运的,靠海的 速度调整标度盘 桅杆 桅座 矩阵 商船 商船建造统计表 稳心 稳心高 金属板电镀槽 金属工 公制单位 中线面 舯横剖面 中横剖面系数 物资清单,物料表 船模试验水池 主机操作监视台 螺旋桨运转监视屏 船壳板的形状复杂 放样间 多体船 多用途船 多种船型建造规划 蘑菇形通风桶 相互排它性的属性 数值控制 海里 造船学 航区 航海甲板 准万向舵机,准万向齿轮 静载荷曲线 中性轴,中和轴 中性平衡 不可收放式减摇鳍 法向的,正交的 常规运作状况
machinery vendor magnet gantry maiden voyage main impeller main shafting major ship maneuverability manhole margin plate maritime mark disk of speed adjusting mast mast clutch matrix merchant ship Merchant Shipbuilding Return metacenter metacentric height metal plate path metal worker metric unit middle line plane midship section midship section coefficient ML model tank monitoring desk of main engine operation monitoring screen of screw working condition more shape to the shell mould loft multihull vessel multi-purpose carrier multi-ship program mushroom ventilator mutually exclusive attribute N/C nautical mile naval architecture navigation area navigation deck near-universal gear net-load curve neutral axis neutral equilibrium non-retractable fin stabilizer normal normal operating condition
欧洲保险公司的整合风险监管框架
No 4/2006 Solvfor European insurers
3 Executive summary 5 The reform explained 14 Solvency II in comparison 19 To what extent might Solvency II change the industry’s solvency position? 24 The impact of Solvency II on European insurance markets 39 Appraisal of Solvency II 40 Appendices
Published by: Swiss Reinsurance Company Economic Research & Consulting P.O. Box 8022 Zurich Switzerland Telephone +41 43 285 2551 Fax +41 43 285 4749 E-mail: sigma@ New York Office: 55 East 52nd Street 40th Floor New York, NY 10055 Telephone +1 212 317 5135 Fax +1 212 317 5455 Hong Kong Office: 18 Harbour Road, Wanchai Central Plaza, 61st Floor Hong Kong, SAR Telephone +852 2582 5691 Fax +852 2511 6603 Authors: Patrizia Baur Telephone +41 43 285 3153 Rudolf Enz Telephone +41 43 285 2239 sigma co-editor: Aurelia Zanetti Telephone +41 43 285 2544 Managing editor: Thomas Hess, Head of Economic Research & Consulting, is responsible for the sigma series. The editorial deadline for this study was 12 May 2006. sigma is available in English (original language), German, French, Italian, Spanish, Chinese and Japanese. sigma is available on Swiss Re’s website: /sigma The internet version may contain slightly updated information. Translations: Swiss Re Group Language Services Graphic design and production: Swiss Re Logistics/Media Production © 2006 Swiss Reinsurance Company Zurich All rights reserved. The entire content of this sigma edition is subject to copyright with all rights reserved. The information may be used for private or internal purposes, provided that any copyright or other proprietary notices are not removed. Electronic reuse of the data published in sigma is prohibited. Reproduction in whole or in part or use for any public purpose is permitted only with the prior written approval of Swiss Re Economic Research & Consulting and if the source reference “Swiss Re, sigma No 4/2006” is indicated. Courtesy copies are appreciated. Although all the information used in this study was taken from reliable sources, Swiss Reinsurance Company does not accept any responsibility for the accuracy or comprehensiveness of the information given. The information provided is for informational purposes only and in no way constitutes Swiss Re’s position. In no event shall Swiss Re be liable for any loss or damage arising in connection with the use of this information.
可信度风险生成式大模型
可信度风险生成式大模型下载温馨提示:该文档是我店铺精心编制而成,希望大家下载以后,能够帮助大家解决实际的问题。
文档下载后可定制随意修改,请根据实际需要进行相应的调整和使用,谢谢!并且,本店铺为大家提供各种各样类型的实用资料,如教育随笔、日记赏析、句子摘抄、古诗大全、经典美文、话题作文、工作总结、词语解析、文案摘录、其他资料等等,如想了解不同资料格式和写法,敬请关注!Download tips: This document is carefully compiled by the editor. I hope that after you download them, they can help yousolve practical problems. The document can be customized and modified after downloading, please adjust and use it according to actual needs, thank you!In addition, our shop provides you with various types of practical materials, such as educational essays, diary appreciation, sentence excerpts, ancient poems, classic articles, topic composition, work summary, word parsing, copy excerpts,other materials and so on, want to know different data formats and writing methods, please pay attention!可信度风险生成式大模型是一种用于评估信息可信度的复杂系统,它结合了大数据分析和机器学习技术,可以帮助我们更准确地判断一个信息源或信息内容的可信程度。
航海英语词汇
metacenter 稳心metacentric height 稳心高metal plate path 金属板电镀槽metal worker 金属工metric unit 公制单位middle line plane 中线面midship section 舯横剖面midship section coefficient 中横剖面系数ML 物资清单,物料表model tank 船模试验水池monitoring desk of main engine operation 主机操作监视台monitoring screen of screw working condition 螺旋桨运转监视屏more shape to the shell 船壳板的形状复杂mould loft 放样间multihull vessel 多体船multi-purpose carrier 多用途船multi-ship program 多种船型建造规划mushroom ventilator 蘑菇形通风桶mutually exclusive attribute 相互排它性的属性N/C 数值控制nautical mile 海里naval architecture 造船学navigation area 航区navigation deck 航海甲板near-universal gear 准万向舵机,准万向齿轮net-load curve 静载荷曲线neutral axis 中性轴,中和轴neutral equilibrium 中性平衡non-retractable fin stabilizer 不可收放式减摇鳍normal 法向的,正交的normal operating condition 常规运作状况nose cone 螺旋桨整流帽notch 开槽,开凹口oar 橹,桨oblique bitts 斜式双柱系缆桩ocean going ship 远洋船off-center loading 偏离中心的装载offsets 型值offshore drilling 离岸钻井offshore structure 离岸工程结构物oil filler 加油点oil skimmer 浮油回收船oil-rig 钻油架on-deck girder 甲板上桁架open water 敞水optimality criterion 最优性准则ore carrier 矿砂船orthogonal 矩形的orthogonal 正交的out strake 外列板outboard motor 舷外机outboard profile 侧视图outer jib 外首帆outfit 舾装outfitter 舾装工outrigger 舷外吊杆叉头overall stability 总体稳性overhang 外悬paddle 桨paddle-wheel-propelled 明轮推进的Panama Canal 巴拿马运河panting arrangement 强胸结构,抗拍击结构panting beam 强胸横梁panting stringer 抗拍击纵材parallel middle body 平行中体partial bulkhead 局部舱壁payload 有效载荷perpendicular 柱,垂直的,正交的photogrammetry 投影照相测量法pile driving barge 打桩船pillar 支柱pin jig 限位胎架pintle 销,枢轴pipe fitter 管装工pipe laying barge 铺管驳船piston 活塞pitch 螺距pitch 纵摇plan views 设计图planning hull 滑行船体Plimsoll line 普林索尔载重线polar-exploration craft 极地考察船poop 尾楼port 左舷port call 沿途到港停靠positive righting moment 正扶正力矩power and lighting system 动力与照明系统precept 技术规则preliminary design 初步设计pressure coaming 阻力式舱口防水挡板principal dimensions 主尺度Program Evaluation and Review Technique 规划评估与复核法progressive flooding 累进进水project 探照灯propeller shaft bracket 尾轴架propeller type log 螺旋桨推进器测程仪PVC foamed plastic PVC泡沫塑料quadrant 舵柄quality assurance 质量保证quarter 居住区quarter pillar 舱内侧梁柱quartering sea 尾斜浪quasi-steady wave 准定长波quay 码头,停泊所quotation 报价单racking 倾斜,变形,船体扭转变形radiography X射线探伤rake 倾斜raked bow 前倾式船首raster 光栅refrigerated cargo ship 冷藏货物运输船Register (船舶)登录簿,船名录Registo Italiano Navade 意大利船级社regulating knob of fuel pressure 燃油压力调节钮reserve buoyancy 储备浮力residuary resistance 剩余阻力resultant 合力reverse frame 内底横骨Reynolds number 雷诺数right-handed propeller 右旋进桨righting arm 扶正力臂,恢复力臂rigid side walls 刚性侧壁rise of floor 底升riverine warfare vessel 内河舰艇rivet 铆接,铆钉roll 横摇roll-on/roll-off (Ro/Ro) 滚装rotary screw propeller 回转式螺旋推进器rounded gunwale 修圆的舷边rounded sheer strake 圆弧舷板rubber tile 橡皮瓦rudder 舵rudder bearing 舵承rudder blade 舵叶rudder control rod 操舵杆rudder gudgeon 舵钮rudder pintle 舵销rudder post 舵柱rudder spindle 舵轴rudder stock 舵杆rudder trunk 舵杆围井run 去流段sag 中垂salvage lifting vessel 救捞船scale 缩尺,尺度schedule coordination 生产规程协调schedule reviews 施工生产进度审核screen bulkhead 轻型舱壁Sea keeping performance 耐波性能sea spectra 海浪谱sea state 海况seakeeping 适航性seasickness 晕船seaworthness 适航性seaworthness 适航性section moulus 剖面模数sectiongs 剖面,横剖面self-induced 自身诱导的self-propulsion 自航semi-balanced rudder 半平衡舵semi-submersible drilling rig 半潜式钻井架shaft bossing 轴榖shaft bracket 轴支架shear 剪切,剪力shear buckling 剪切性屈曲shear curve 剪力曲线sheer 舷弧sheer aft 艉舷弧sheer drawing 剖面图sheer forward 艏舷弧sheer plane 纵剖面sheer profile 总剖线sheer profile 纵剖图shell plating 船壳板ship fitter 船舶装配工ship hydrodynamics 船舶水动力学shipway 船台shipyard 船厂shrouded screw 有套罩螺旋桨,导管螺旋桨side frame 舷边肋骨side keelson 旁内龙骨side plate 舷侧外板side stringer 甲板边板single-cylinder engine 单缸引擎sinkage 升沉six degrees of freedom 六自由度skin friction 表面摩擦力skirt (气垫船)围裙slamming 砰击sleeve 套管,套筒,套环slewing hydraulic motor 回转液压马达slice 一部分,薄片sloping shipway 有坡度船台sloping top plate of bottom side tank 底边舱斜顶板slopint bottom plate of topside tank 定边舱斜底板soft chine 圆舭sonar 声纳spade rudder 悬挂舵spectacle frame 眼睛型骨架speed-to-length ratio 速长比sponson deck 舷伸甲板springing 颤振stability 稳性stable equilibrium 稳定平衡starboard 右舷static equilibrium 静平衡steamer 汽轮船steering gear 操纵装置,舵机stem 船艏stem contour 艏柱型线stern 船艉stern barrel 尾拖网滚筒stern counter 尾突体stern ramp 尾滑道,尾跳板stern transom plate 尾封板stern wave 艉波stiffen 加劲,加强stiffener 扶强材,加劲杆straddle 跨立,外包式叶片strain 应变strake 船体列板streamline 流线streamlined casing 流线型套管strength curves 强度曲线strength deck 强力甲板stress concentration 应力集中structural instability 结构不稳定性strut 支柱,支撑构型subassembly 分部装配subdivision 分舱submerged nozzle 浸没式喷口submersible 潜期suction back of a blade 桨叶片抽吸叶背Suez Canal tonnage 苏伊士运河吨位限制summer load water line 夏季载重水线superintendent 监督管理人,总段长,车间主任superstructure 上层建筑Supervision of the Society’s surveyor船级社验船师的监造书supper cavitating propeller 超空泡螺旋桨surface nozzle 水面式喷口surface piercing 穿透水面的surface preparation and coating 表面加工处理与喷涂surge 纵荡surmount 顶上覆盖,越过swage plate 压筋板swash bulkhead 止荡舱壁SWATH (Small Waterplane Area Twin Hull) 小水线面双体船sway 横荡tail-stabilizer anchor 尾翼式锚talking paper 讨论文件tangential 切向的,正切的tangential viscous force 切向粘性力tanker 油船tee T型构件,三通管tender 交通小艇tensile stress 拉(张)应力thermal effect 热效应throttle valve 节流阀throughput 物料流量thrust 推力thruster 推力器,助推器timber carrier 木材运输船tip of a blade 桨叶叶梢tip vortex 梢涡toed towards amidships 趾部朝向船舯tonnage 吨位torpedo 鱼雷torque 扭矩torque 扭矩trailing edge 随边transom stern 方尾transverse bulkhead plating 横隔舱壁板transverse section 横剖面transverse stability 横稳性trawling 拖网trial 实船试验trim 纵倾trim by the stern/bow 艉艏倾trimaran 三体的tripping bracket 防倾肘板trough 波谷tugboat 拖船tumble home (船侧)内倾tunnel wall effect 水桶壁面效应turnable blade 可转动式桨叶turnable shrouded screw 转动导管螺旋桨tweendeck cargo space 甲板间舱tweendedk frame 甲板间肋骨two nodded frequency 双节点频率ULCC 超级大型原油轮ultrasonic 超声波的underwriter (海运)保险商unsymmetrical 非对称的upright position 正浮位置vapor pocket 气化阱ventilation and air conditioning diagram 通风与空调铺设设计图Venturi section 文丘里试验段vertical prismatic coefficient 横剖面系数vertical-axis(cycloidal)propeller 直叶(摆线)推进器vessel component vender 造船部件销售商viscosity 粘性VLCC 巨型原油轮Voith-Schneider propeller 外摆线直翼式推进器v-section v型剖面wake current 伴流,尾流water jet 喷水(推进)管water plane 水线面watertight integrity 水密完整性wave pattern 波形wave suppressor 消波器,消波板wave-making resistance 兴波阻力weather deck 露天甲板web 腹板web beam 强横梁web frame 腹肋板weler 焊工wetted surface 湿表面积winch 绞车windlass 起锚机wing shaft 侧轴wing-keel 翅龙骨(游艇)working allowance 有效使用修正量worm gear 蜗轮,蜗杆yacht 快艇yard issue 船厂开工任务发布书yards 帆桁yaw 首摇afaired set of lines 经过光顺处理的一套型线a stereo pair of photographs 一对立体投影相片abaft 朝向船体abandonment cost 船舶废置成本费用accommodation 居住(舱室)accommodation ladder 舷梯adjust valve 调节阀adjustable-pitch 可调螺距式admiralty 海军部advance coefficient 进速系数aerostatic 空气静力学的aft peak bulkhead 艉尖舱壁aft peak tank 艉尖舱aileron 副鳍air cushion vehicle 气垫船air diffuser 空气扩散器air intake 进气口aircraft carrier 航空母舰air-driven water pump 气动水泵airfoil 气翼,翼剖面,机面,方向舵alignment chock 组装校准用垫楔aluminum alloy structure 铝合金结构American Bureau of Shipping 美国船级社amidships 舯amphibious 两栖的anchor arm 锚臂anchor chain 锚链anchor crown 锚冠anchor fluke 锚爪anchor mouth 锚唇anchor recess 锚穴anchor shackle 锚卸扣anchor stock 锚杆angle bar 角钢angle of attack 攻角angle plate 角钢angled deck 斜角甲板anticipated loads encountered at sea 在波浪中遭遇到的预期载荷anti-pitching fins 减纵摇鳍antiroll fins 减摇鳍anti-rolling tank 减摇水舱appendage 附体artisan 技工assembly line 装配流水线at-sea replenishment 海上补给augment of resistance 阻力增额auxiliary systems 辅机系统auxiliary tank 调节水舱axial advance 轴向进速backing structure 垫衬结构back-up member 焊接垫板balance weight 平衡锤ball bearing 滚珠轴承ball valve 球阀ballast tank 压载水舱bar 型材bar keel 棒龙骨,方龙骨,矩形龙骨barge 驳船baseline 基线basic design 基本设计batten 压条,板条beam 船宽,梁beam bracket 横梁肘板beam knee 横梁肘板bed-plate girder 基座纵桁bending-moment curves 弯矩曲线Benoulli’s law伯努利定律berth term 停泊期bevel 折角bidder 投标人bilge 舭,舱底bilge bracket 舭肘板bilge radius 舭半径bilge sounding pipe 舭部边舱水深探管bitt 单柱系缆桩blade root 叶跟blade section 叶元剖面blast 喷丸block coefficient 方形系数blue peter 出航旗boarding deck 登艇甲板boat davit 吊艇架boat fall 吊艇索boat guy 稳艇索bobstay 首斜尾拉索body plan 横剖面图bolt 螺栓,上螺栓固定Bonjean curve 邦戎曲线boom 吊杆boss 螺旋桨轴榖bottom side girder 旁底桁bottom side tank 底边舱bottom transverse 底列板boundary layer 边界层bow line 前体纵剖线bow wave 艏波bowsprit 艏斜桅bow-thruster 艏侧推器box girder 箱桁bracket floor 框架肋板brake 制动装置brake band 制动带brake crank arm 制动曲柄brake drum 刹车卷筒brake hydraulic cylinder 制动液压缸brake hydraulic pipe 刹车液压管breadth extreme 最大宽,计算宽度breadth moulded 型宽breakbulk 件杂货breasthook 艏肘板bridge 桥楼,驾驶台bridge console stand 驾驶室集中操作台BSRA 英国船舶研究协会buckle 屈曲buffer spring 缓冲弹簧built-up plate section 组合型材bulb plate 球头扁钢bulbous bow 球状船艏,球鼻首bulk carrier 散货船bulk oil carrier 散装油轮bulkhead 舱壁bulwark 舷墙bulwark plate 舷墙板bulwark stay 舷墙支撑buoy tender 航标船buoyant 浮力的buoyant box 浮箱Bureau Veritas 法国船级社butt weld 对缝焊接butterfly screw cap 蝶形螺帽buttock 后体纵剖线by convention 按照惯例,按约定cable ship 布缆船cable winch 钢索绞车CAD(computer-aided design) 计算机辅助设计CAE(computer-aided manufacturing) 计算机辅助制造CAM(computer-aided engineering) 计算机辅助工程camber 梁拱cant beam 斜横梁cant frame 斜肋骨cantilever beam 悬臂梁capacity plan 舱容图CAPP(computer –aided process planning) 计算机辅助施工计划制定capsize 倾覆capsizing moment 倾覆力臂captain 船长captured-air-bubble vehicle 束缚气泡减阻船cargo cubic 货舱舱容,载货容积cargo handling 货物装卸carriage 拖车,拖架cast steel stem post 铸钢艏柱catamaran 高速双体船catamaran 双体的cavitation 空泡cavitation number 空泡数cavitation tunnel 空泡水筒center keelson 中内龙骨centerline bulkhead 中纵舱壁centroid 型心,重心,质心,矩心chain cable stopper 制链器chart 海图charterer 租船人chief engineer 轮机长chine 舭,舷,脊chock 导览钳CIM(computer integrated manufacturing) 计算机集成组合制造circulation theory 环流理论classification society 船级社cleat 系缆扣clipper bow 飞剪型船首clutch 离合器coastal cargo 沿海客货轮cofferdam 防撞舱壁combined cast and rolled stem 混合型艏柱commercial ship 营利用船commissary spaces 补给库舱室,粮食库common carrier 通用运输船commuter 交通船compartment 舱室compass 罗经concept design 概念设计connecting tank 连接水柜constant-pitch propeller 定螺距螺旋桨constraint condition 约束条件container 集装箱containerized 集装箱化contract design 合同设计contra-rotating propellers 对转桨controllable-pitch 可控螺距式corrosion 锈蚀,腐蚀couple 力矩,力偶crane 克令吊,起重机crank 曲柄crest (of wave) 波峰crew quarters 船员居住舱criterion 判据,准则Critical Path Method 关键路径法cross-channel automobile ferries 横越海峡车客渡轮cross-sectional area 横剖面面积crow’s nest桅杆瞭望台cruiser stern 巡洋舰尾crussing range 航程cup and ball joint 球窝关节curvature 曲率curves of form 各船形曲线cushion of air 气垫damage stability 破损稳性damper 缓冲器damping 阻尼davit arm 吊臂deadweight 总载重量de-ballast 卸除压载deck line at side 甲板边线deck longitudinal 甲板纵骨deck stringer 甲板边板deck transverse 强横梁deckhouse 舱面室,甲板室deep v hull 深v型船体delivery 交船depth 船深derrick 起重机,吊杆design margin 设计余量design spiral 设计螺旋循环方式destroyer 驱逐舰detachable shackle 散合式连接卸扣detail design 详细设计diagonal stiffener 斜置加强筋diagram 图,原理图,设计图diesel engine 柴油机dimensionless ratio 无量纲比值displacement 排水量displacement type vessel 排水型船distributed load 分布载荷division 站,划分,分隔do work 做功dock 泊靠double hook 山字钩double iteration procedure 双重迭代法double roller chock 双滚轮式导览钳double-acting steam cylinder 双向作用的蒸汽气缸down halyard 降帆索draft 吃水drag 阻力,拖拽力drainage 排水draught 吃水,草图,设计图,牵引力dredge 挖泥船drift 漂移,偏航drilling rig 钻架drillship 钻井船drive shaft 驱动器轴driving gear box 传动齿轮箱driving shaft system 传动轴系dry dock 干船坞ducted propeller 导管螺旋桨dynamic supported craft 动力支撑型船舶dynamometer 测力计,功率计e.h.p 有效马力eccentric wheel 偏心轮echo-sounder 回声探深仪eddy 漩涡eddy-making resistance 漩涡阻力efficiency 供给能力,供给量electrohydraulic 电动液压的electroplater 电镀工elevations 高度,高程,船型线图的侧面图,立视图,纵剖线图,海拔empirical formula 经验公式enclosed fabrication shop 封闭式装配车间enclosed lifeboat 封闭式救生艇end open link 末端链环end shackle 末端卸扣endurance 续航力endurance 续航力,全功率工作时间engine room frame 机舱肋骨engine room hatch end beam 机舱口端梁ensign staff 船尾旗杆entrance 进流段erection 装配,安装exhaust valve 排气阀expanded bracket 延伸肘板expansion joint 伸缩接头extrapolate 外插fair 光顺faised floor 升高肋板fan 鼓风机fatigue 疲劳feasibility study 可行性研究feathering blade 顺流变距桨叶fender 护舷ferry 渡轮,渡运航线fillet weld connection 贴角焊连接fin angle feedback set 鳍角反馈装置fine fast ship 纤细高速船fine form 瘦长船型finite element 有限元fire tube boiler 水火管锅炉fixed-pitch 固定螺距式flange 突边,法兰盘flanking rudders 侧翼舵flap-type rudder 襟翼舵flare 外飘,外张flat of keel 平板龙骨fleets of vessels 船队flexural 挠曲的floating crane 起重船floodable length curve 可进长度曲线flow of materials 物流flow pattern 流型,流线谱flush deck vessel 平甲板型船flying bridge 游艇驾驶台flying jib 艏三角帆folding batch cover 折叠式舱口盖folding retractable fin stabilizer 折叠收放式减摇鳍following edge 随边following ship 后续船foot brake 脚踏刹车fore peak 艏尖舱forged steel stem 锻钢艏柱forging 锻件,锻造forward draft mark 船首水尺forward/afer perpendicular 艏艉柱forward/after shoulder 前/后肩foundry casting 翻砂铸造frame 船肋骨,框架,桁架freeboard 干舷freeboard deck 干舷甲板freight rate 运费率fresh water loadline 淡水载重线frictional resistance 摩擦阻力Froude number 傅汝德数fuel/water supply vessel 油水供给船full form 丰满船型full scale 全尺度fullness 丰满度funnel 烟囱furnishings 内装修gaff 纵帆斜桁gaff foresail 前桅主帆gangway 舷梯gantt chart 甘特图gasketed openings 装以密封垫的开口general arrangement 总布置general cargo ship 杂货船generatrix 母线geometrically similar form 外形相似船型girder 桁梁,桁架girder of foundation 基座纵桁governmental authorities 政府当局,管理机构gradient 梯度graving dock 槽式船坞Green Book 绿皮书,19世纪英国另一船级社的船名录,现合并与劳埃德船级社,用于登录快速远洋船gross ton 长吨(1.016公吨)group technology 成祖建造技术GT 成组建造技术guided-missile cruiser 导弹巡洋舰gunwale 船舷上缘gunwale angle 舷边角钢gunwale rounded thick strake 舷边圆弧厚板guyline 定位索gypsy 链轮gyro-pilot steering indicator 自动操舵操纵台gyroscope 回转仪half breadth plan 半宽图half depth girder 半深纵骨half rounded flat plate 半圆扁钢hard chine 尖舭hatch beam sockets 舱口梁座hatch coaming 舱口围板hatch cover 舱口盖hatch cover 舱口盖板hatch cover rack 舱口盖板隔架hatch side cantilever 舱口悬臂梁hawse pipe 锚链桶hawsehole 锚链孔heave 垂荡heel 横倾heel piece 艉柱根helicoidal 螺旋面的,螺旋状的hinge 铰链hinged stern door 艉部吊门HMS 英国皇家海军舰艇hog 中拱hold 船舱homogeneous cylinder 均质柱状体hopper barge 倾卸驳horizontal stiffener 水平扶强材hub 桨毂,轴毂,套筒hull form 船型,船体外形hull girder stress 船体桁应力HVAC(heating ventilating and cooling) 取暖,通风与冷却hydraulic mechanism 液压机构hydrodynamic 水动力学的hydrofoil 水翼hydrostatic 水静力的IAGG(interactive computer graphics) 交互式计算机图像技术icebreaker 破冰船icebreaker 破冰船IMCO(Intergovernmental Maritime ConsultativeOrganization) 国际海事质询组织immerse 浸水,浸没impact load 冲击载荷imperial unit 英制单位in strake 内列板inboard profile 纵剖面图incremental plasticity 增量塑性independent tank 独立舱柜initial stability at small angle of inclination 小倾角初稳性inland waterways vessel 内河船inner bottom 内底in-plane load 面内载荷intact stability 完整稳性intercostals 肋间的,加强的International Association of Classification Society (IACS) 国际船级社联合会International Towing Tank Conference (ITTC) 国际船模试验水池会议intersection 交点,交叉,横断(切)inventory control 存货管理iterative process 迭代过程jack 船首旗jack 千斤顶joinery 细木工keel 龙骨keel laying 开始船舶建造kenter shackle 双半式连接链环Kristen-Boeing propeller 正摆线推进器landing craft 登陆艇launch 发射,下水launch 汽艇launching equipmeng (向水中)投放设备LCC 大型原油轮leading edge 导缘,导边ledge 副梁材length overall 总长leveler 调平器,矫平机life saving appliance 救生设备lifebuoy 救生圈lifejacket 救生衣lift fan 升力风扇lift offsets 量取型值light load draft 空载吃水lightening hole 减轻孔light-ship 空船limbers board 舭部污水道顶板liner trade 定期班轮营运业lines 型线lines plan 型线图Linnean hierarchical taxonomy 林式等级式分类学liquefied gas carrier 液化气运输船liquefied natural gas carrier 液化天然气船liquefied petroleum gas carrier 液化石油气船liquid bulk cargo carrier 液体散货船liquid chemical tanker 液体化学品船list 倾斜living and utility spaces 居住与公用舱室Lloyd’s Register of shipping劳埃德船级社Lloyd’s Rules劳埃德规范Load Line Convention 载重线公约load line regulations 载重线公约,规范load waterplane 载重水线面loft floor 放样台longitudinal (transverse) 纵(横)稳心高longitudinal bending 纵总弯曲longitudinal prismatic coefficient 纵向菱形系数longitudinal strength 纵总强度longitudinally framed system 纵骨架式结构luffing winch 变幅绞车machinery vendor 机械(主机)卖方magnet gantry 磁力式龙门吊maiden voyage 处女航main impeller 主推叶轮main shafting 主轴系major ship 大型船舶maneuverability 操纵性manhole 人孔margin plate 边板maritime 海事的,海运的,靠海的mark disk of speed adjusting 速度调整标度盘mast 桅杆mast clutch 桅座matrix 矩阵merchant ship 商船。
膝关节的康复治疗 英文
KNEE REHABILITATION膝关节的康复治疗主讲: 高信拱美国物理治疗博士Introduction of the KneeThree “sub joint” articulation surfaces:The multiple functions of the normal kneeTwo Most Important Characteristics of the Knee1. The stability in full extension is provided by the soft tissues2. the mobility/flexibility is provided by: bony structurecertain range of flexion forADL/runningoptimal orientation of the foot relative to the irregularities of the groundKnee works by axial compression under the action of gravityFunctionally, the knee can support the body weight in the erect position without muscle contractionIt participates in lowering and elevating body weight (up to 0.5 m ) in sitting, squatting, or climbingIn walking, the normal knee reduces energy expenditureThe first degree of freedomThe first degree of freedom - movements of flexion and extension The second degree of freedom accessory movement, medial or lateral rotation of the tibia on the femurhighly ingenious mechanical knee structureshave poor degree of interlocking of the surfacesknee gains mobility but losses stability Functional Anatomy and Mechanics of the KneeJoint Structure and Function / Palpable Joint Structures To palpate the superficial structures of the knee - recommended to test in sitting position - knee relaxed at 90 d Joint Structure and Function / Nonpalpable StructuresKnee Joint Complex and FunctionVery complex and unstable joint rather than the classic anatomical description of the knee as a simple hinge jointtwo degrees of freedom: flexion-extension and axial rotation.The knee is a composite of three “subjointsAll these structures are enclosed within a single joint capsule.Tibiofemoral JointPhysiologic (Normal) Valgus Tibiofemoral Alignment andWeight-Bearing ForcesGenu Valgum (“Knock Knees”)Genu Varum (“Bow Legs”):In either genu valgum or genu varum: Constant overloading:lateral or medial articular cartilagemay result in damage to the cartilage The menisci of the knee are important indistributingabsorbingAngulation and compression of the knee:Elimination of any angulation between the femur and tibia or a mild genu varum will increase the compression on the medial meniscus by25 %Five degrees of genu varum (medial Tibiofemoral angle of 175°)will increase the forces by50 %Tibial Articular SurfaceThe two concave (in frontal plane), asymmetric plateausMenisciTwo joint discs - attached to the articulating surfaces on the tibial condylesAn accessory joint structureenhances congruenceassists in the balancebetween mobilityand stability needed by the joint The lateral meniscus is attached to the: posterior cruciate ligament (PCL)popliteus musclevia the coronary ligaments and posterior capsulethe connections of the lateral meniscus are:fairly loosemore mobility on the lateral tibial condylenot easy to be injuredThe connections of the medial meniscus are:fairly tightlack of mobilityone of several reasonswhy torn more frequently than the lateral meniscusMenisciVascularized only on the periphery by: capillaries fromthe joint capsulethe synovial membraneThe pattern of vascularity may account forthe low incidence of meniscal injuries in young childrenmenisci have ample blood supplythe ability of the menisci in the adult to regenerateonly in the vascularized peripheral regionKnee Joint CapsuleSynovial Lining:the ACLs and PCLs being contained outside the synovial sleeve within the fibrous capsule Tibiofemoral JointFlexion/ExtensionRotationRange of motionArthokinematicsIncongruenceIn weight-bearing flexion:The femoral condyles roll posteriorly and slide anteriorlyThe menisci follow the roll of the condyles by distorting posteriorly in flexion.In weight-bearing extension:The femoral condyles roll anteriorly and slide posteriorlyIn the last portion of extension, motion stops at the lateral femoral condyle, but sliding continues on the medial femoral condyle to produce locking of the knee LigamentsGiven the lack of bony restraint to virtually any of the knee motionsthe ligaments are credited with resisting or controlling:Excessive knee extensionVarus and valgus stresses at the knee attempted adduction or abduction of the tibia, respectivelyAnterior or posterior displacement of the tibia beneath the femurMedial or lateral rotation of the tibia beneath the femurCombinations of AP displacements and rotations of the tibia, known as rotatory stabilizationLigamentsthe ligamentous function can change depending on the position of the knee joint:on how the stresses are appliedon whatactivepassivestructures are concomitantly intact Collateral ligamentsMedial Collateral ligamentLateral Collateral ligamentCruciate LigamentsBoth cruciate ligaments appear to play a rolein producing and controlling rotation of the tibiaThe ACL appears to twist around the PCL in medial rotation of the tibiachecking excessive medial rotationThe primary function of the ACL:Resist anterior translation of the tibia on the femurTwo types of instability can occur at the knee: straight and combined Patellofemoral JointThe PatellaAs an anatomic pulleyFollowing Removal of the PatellaThe Contribution of the Patella Patellofemoral Joint Reaction Forces Increase PatellofemoralJoint Reaction Force:In gaitWith stair climbing or with running hills Superior Tibiofibular Joint Neuromuscular Components Movements and Range of Motion at the KneeRotationThe axis of motion for rotation at the Tibiofemoral jointin open kinematic chainin closed kinematic chainMuscular StructuresExtensorsThe quadriceps muscles:rectus femorisvastus medialis, including the vastus medialis obliquus (VMO)vastus lateralisvastus intermediusThe only portion of the quadriceps that crosses two joints is the rectus femoris which originates on the Anterior Inferior Iliac Spine The pull of the vastus medialis:depended on which segment of the muscle was assessedThe proximal (upper) fibers - Vastus Medialis Longus (VML)were angulated 15 to 18°mediallythe distal (lower) fibers - Vastus Medialis Oblique (VMO)were angulated 50 to 55°mediallyThe Iliotibial Tract (Bend)The Popliteus MuscleThe Gastrocnemius MuscleThe SartoriusThe GracilisThe Hamstrings andRectus Femoris Muscleshave combined functions:Both cross the hip and knee jointRectus femoris:open kinematic chain:•extends the knee•flexes the hipclosed kinematic chain:•control knee flexion•control hip extensionsummaryMuscles of the knee:dual roles:1 ) controlling motion2) proving stabilityfor the joint that has little inherent bony stabilityNeural Structures at the knee continuations of the proximal nerves: posteriorly - the sciatic nerveanteriorly - the femoral nerve Posteriorly, the sciatic nerve distally sciatic notch hamstring compartment between the biceps femoris underlying adductor magnus superior - popliteal fossa divides into1) tibial branch2) peroneal branchNeural Structures at the kneeThe following nerves can be compressed or entrapped:A) the tibial nerve as it enters the fibrous arch at the origin of the soleus muscleB) the common peroneal nerve at the neck of the fibulaC) the infrapatellar branch of the saphenous nerve as it pierces the sartorius muscleEvaluationSubjective ExaminationPrinciples of Extremity Evaluation:* main complaints:- valuable clues about problems- help plan the objective examination - the mechanism of injury determine the structures - may be injured (acute trauma)EvaluationSubjective ExaminationAcute knee injuries can range from:* patellofemoral dislocation* ligamentous ruptures* meniscal tearsRepetitive trauma can affect (around the knee ):LigamentsTendonsburse.Patellofemoral dysfunction (common) The patterns of symptoms and mechanism ofinjury will help guide the objective examination ofthe knee.Planning the Objective Examination Based on the subjective cluesA well-planned examination:will limit the possibility of inadvertently aggravating the problem Tests should be prioritized to avoid excessive symptom provocation - may interfere with a complete and accurate assessment Suspected contributing areas such as the hip, ankle, and lumbar spine should be evaluated as needed to implicate or rule out their involvementThe following problems should be considered when planning the objective examination:severityIrritabilityNatureObjective ExaminationPrinciples of Extremity Evaluation areas - contribute to symptoms - be checked first- include:Lumbar spine, especially if movement of the low back provokes pain in the knee areaHipPelvisAnkleshould be all considered in a thorough examination if these structures are thought to contribute to the clinical problemsEvaluation / Objective Examination Structural ObservationStructural observation of the knee includes looking for alignment problems at:spinePelvisHipsentire lower extremityStructural Observation ANTERIORFirst be observedStand comfortably - feet no farther than shoulder width apart and pointed straight aheadThis posture allows comparison form: * side to side* patient to patientThe quadriceps angle (Q-angle):the angle between:ASIS middle / patella tibial tuberositytraditionally measurement:supine / knee fully extended / relaxed however, the position of the feet and hips can alter the Q-angle, especially in weight bearingClinically:the Q-angle should be measured with the patient standing (if it is possible) so it represents normal weight bearing functionNormal Q-angles measureMen: < 10 – 12 dWomen: < 15 dThe Q-angle should be measured and compared bilaterallyLATERALalignment be noted from the sides and compared bilaterally:Patella alta / Patella BajaStructural Observation POSTERIORTo compare the heights of:The popliteal crease and fibular head Any asymmertry in the calf musculature abnormal finding such as swelling from a popliteal cyst (Baker's Cyst)MobilityPhysiological MobilityRefers to the movement of the knee in:1) standard planar movements2) functional combined motions Physiological mobility is tested:1) actively2) passivelyPhysiological MobilityAccessory MobilityRefers to:1) joint play2) component motionsWhen to test accessory mobility:1) physiological mobility is limited2) abnormal responses to physiological mobilityGliding testsBy joint, the following glides should be tested:Tibiofemoral joint1. anterior2. posterior3. medial4. lateralPatellofemoral joint1. superior2. inferior3. medial4. lateralSuperior tibiofibular joint1. anterior2. posteriorPertinent findings include:available range of motionend-feelany crepitusany symptoms provokedAll findings should be compared to:the contralateral limbnormal accessory mobilitySpecific Manual Muscle Testing (MMT):Assesses the status of the contractile elements of the kneePerformed in the mid-range of motion to avoid stress on the noncontractile structuresPatient gradually increases effort to a voluntary maximum contraction StrengthNeurological ExaminationSpecial TestsSpecial tests:Gather more information about the knee problemIdentify the contributing factors of the patient's problemThe Special Tests Classified as Four Types:1) musculotendinous test,2) effusion test3) joint and ligamentous test4) functional test Musculotendinous TestsHamstring TightnessEly's TestOber's TestNoble's Compression TestEffusion TestStroke TestFluctuation TestPatellar Tap TestJoint/Ligamentous TestsPatellar Tilt TestLateral Pull TestMcConnell TestApprehension TestVarus StressTestLachman's TestAnterior Drawer TestPosterior Sag SignPosterior Drawer TestQuadriceps Active TestSquat Walk TestSteinman's SignHyperflexion-Hyperextension TestMcMurray-Anderson Test FUNCTIONAL TESTSHelp the clinician gain valuable informationThese are:•Two-legged full squat•One-legged half squat•Circular one-leg hoppinga) clockwiseb) counterclockwiseShould be compared bilaterally / normal Should be noted:Willingness to perform the testthe symptom responseSigns of apprehensionObjective Evaluation Summaryobjective - subjective examination findings:should be reviewed and correlated many structures can contribute to knee symptomsThe contribution of all knee tissues: LigamentsMenisciTendonsMusclesBurse Nervesjoints themselves should be considered Objective Evaluation Summaryother sources of knee complaints:Hipfoot and anklelumbar spineThe initial evaluation must be geared toward screening these jointsRe-assessments should include reconsideration of these areas, especially if the patient is not benefiting from treatmentObjective Evaluation SummaryAt the conclusion of the evaluation, the clinician determines what problems the patient has and develops a problem listthis list helps the clinician set goalsand plan treatmentsKnee Joint InjuryThe interest and participation in sports activities currently in vogue among all age groups and both sexes is subjecting the knee complex to an increased risk of injurySports may cause either direct or indirect injuryLigamentous Injuries:May occur as a result of a force that causes the joint to exceed its normal ROMACL TearsConservative ManagementTo rehabilitate the ACL-injured knee through non-operative meansMany complications with chronic ACL insufficiency:•Chronic synovitis•recurrent meniscal tears •progressive joint laxity •advanced joint surface erosion•These changes can be accelerated in the ACL deficient knee that has undergone medical meniscectomyIn the ACL deficient knee:decrease in proprioceptionincreased threshold for movement detectionThe goal of rehabilitation is to maintain the functional stability of the knee by compensating for the ligament injury with neuromuscular mechanisms Successful treatment appears to be related to:the level of proprioception losshamstring functionthe use of bracesactivity modification after injury History – Acute ACL Injury •history of acute trauma •"snap" or "pop“•rapid onset of a large effusion •Hemarthrosis•unable to bear weight normally •range of motion will be limited •70% with this history have injury to the ACL•may also have a history of the knee giving way.ACL TearsHistory – Chronic ACL Injury •Episodic giving way•Effusion•PainThe specific activities that irritate the condition give clues to the mechanical instability presentACL TearsSigns / symptomsacute ACL sprain complains of: •Pain•Swelling•Limited weight wearingchronic ACL sprain complain of: •positional apprehension •intermittent pain•effusion with specific activities ACL TearsExamination reveals:positive Lachman's testanterior drawer testThese tests may be difficult to perform in the acutely injured knee due to:Swellingmuscle guardingACL TearsDiagnosticsPhysical examination and historyoften sufficient to confirm an ACL injuryMRIdefinitive diagnostic test for evaluating ACL integrityIt can pickup subtle changes in the ligamentous response to injury that may not even be evident to a surgeon probing an apparently intact ACL during diagnostic arthroscopic surgeryACL TearsProblem ListMay present with any of the following clinical problems:•Knee instability with weight bearing activity•Recurrent episodes of pain and swelling at the knee•Decreased proprioception•Pain on active movement of the knee •Altered gait patternConservative TreatmentACL ReconstructionACL Tear (Surgical)ACL ReconstructionNon-operative treatment is still popular and very commonhowever, patients whose functional goal is:•stressful work•Sportare prime candidates for a reconstructive procedureRecent advances in:InstrumentationGraft fixationHave facilitatedMore accurate graft placement Stronger graft fixationArthoscopically assisted surgery has allowed:Earlier rehabilitationMore aggressive rehabilitation Physical Examination Diagnostic Arthroscopy Harvesting The Graft Preparing The GraftNotchplastyDrilling The Tibial Tunnel Drilling The Femoral Tunnel Passing The Graft Inserting The Graft Femoral FixationTibial FixationRehabilitation/Physical Therapy S/P ACL Reconstruction Traditional Rehabilitation: Aggressive rehabilitation now:•The most common intra-articular graft is the vascularized patellar tendon graft (bone-tendon-bone)•Other grafts are sometimes used Gore-Tex graftfrozen cadaver allograftscombined techniques - the ligament augmentation device (LAD) - combines an autograft with polyethyleneS/P ACL Reconstruction - Problem List post-operative ACL repairs present with some of the following problems: 1. Moderate joint effusion2. Poor quadriceps contractions3. Healing (unstable) ACL graft4. Poor weightbearing tolerance5. Decreased knee proprioceptionSix Stages in the Rehabilitation Process 1. Immediate post-operative phase (day 1-7) goals:prevent an infection of the surgical woundprevent deleterious immobilization gain full passive knee extension activate the quadricep and hamstring musclesafely ambulate with crutches and an immobilizer (brace)2. Maximum protection phase (week 2-6) goals:improve muscle strength Improve endurancegradually increase motionambulate independently without an assistive devicecontrol external forces to protect the graftProprioception exercises can be initiated in this phase3. Controlled ambulation phase (week 6-9)•D/C knee brace for ambulation (using the brace in full extensionweightberaring for up to six weeks can cause an infrapatellar catching problem near the graft site) •proper gait mechanics•Other protocols do not use the brace for this long in weightbearing4. Moderate protection phase ( week 9-14)•Continue activity modification to protect the knee•Exercises are accelerated•Isokinetic testing can be considered, with certain limitations:(A) The arc of motion should be limited to 45-90 degrees(B) An anti-shear device should be used - helps decrease the anterior shear forces generated with open kinematic chain quad contractions5. Light activity phase (12-16 weeks) - may begin: •Running•cutting ( changing direction while running)•agility drills•Strengthening exercises should continue6. Return to activity phase ( 5-6 months) •progress through a gradual return to normal vigorous activities •Functional strengthening•continue proprioception exercises •There are many different pos-operative protocols for ACL reconstructions •the clinician should work closely with the referring surgeon to develop guidelines for patients with these problemsSUMMARY•The knee is placed between the body's two longest bony segments•The knee must withstand considerable loads in daily activities•Its stability is balanced by an intricate relationship of:• ligamentous restraints• muscular controlAccelerated Rehabilitation for Anterior Cruciate Ligament Reconstruction0-1 days:Exercise / Activitystart physical therapy for gait training and pain controlRehabilitation/Physical TherapyS/P ACL Reconstruction Accelerated Rehabilitation for Anterior Cruciate Ligament Reconstruction1-7 days:inspect wound dailycryo-cuff and frequent PRICEimmobilizer in full extension for sleeping and weightbearingweightbearing as tolerated with crutchesRehabilitation/Physical TherapyS/P ACL Reconstruction Accelerated Rehabilitation for Anterior Cruciate Ligament Reconstruction1-7 days (cont’s)patellofemoral mobilizations as toleratedwall slide exercise for flexion (< 120 degrees as tolerated)full terminal knee extension ASAP Rehabilitation/Physical TherapyS/P ACL Reconstruction Accelerated Rehabilitation for Anterior Cruciate Ligament Reconstruction7-14 daysclosed kinematic chain strengthening stationary bike for ROM and strengtheningpool exercise if wound healedstart incisional mobilization ASAP Rehabilitation/Physical TherapyS/P ACL Reconstruction Accelerated Rehabilitation for Anterior Cruciate Ligament Reconstruction7-14 days (cont’s)progressive weightbearing to tolerancePRICE as neededelectrical muscle stimulation as neededproprioceptive balance board activitiesRehabilitation/Physical TherapyS/P ACL Reconstruction Accelerated Rehabilitation for Anterior Cruciate Ligament Reconstruction2-6 weeksprogress flexion to full as tolerated continue weightbearing to full as toleratedprogressive closed kinematic chain strengtheningprevent excessiveinflammation/edema with PRICEpost-exerciseRehabilitation/Physical Therapy S/P ACL ReconstructionAccelerated Rehabilitation for Anterior Cruciate Ligament Reconstruction 6-12 weeksprogress flexion to full as tolerated pool running as tolerated light jogging permittedcontinue closed kinematic chain strengtheningRehabilitation/Physical Therapy S/P ACL ReconstructionAccelerated Rehabilitation for Anterior Cruciate Ligament Reconstruction 12-24 weeksincrease closed kinematic chain exercise Agilityfunctional activities astolerated( running, jumping, cutting) as needed depending upon goalfunctional brace optional for sports orworkMeniscal Tears•1. The menisci act as: •mechanical spacers•prevent contact between the a) femoral condyles b) tibial plateausin non-weight bearing situationsc) After a total meniscectormy small amount of joint space narrowing •2. The menisci normally distribute greater than 50% of the total forces across the knee in weight bearing situations•capabilities of the menisci are lost with total meniscectomy•3. The menisci normally help the ligaments limit joint translation Signs / SymptomsVariety of complaints, including:•joint line pain •joint locking•giving way of the knee •limited ROM•swelling around the joint•frequently concurrent injury to a) the ligamentous strctures b) the patellofemoral joint Symptoms usually occur during weightbearing Diagnostics •MRI•Arthrogram – invasive – no more •Radiographs:sometimes used for adolescentrule out a growth plate fracture in an acute injurybut do not provide information about the menisci Problem ListPatients with meniscal tears may present with any of the following problems: •Knee joint line pain•Catching or locking of the knee •Painful, limited knee flexion or extension•Intolerance of weightbearing activities •Antalgic gait pattern Treatment vary from: •non-operative•partial meniscectomy •meniscal repairRehabilitation guidelines for meniscus injury are based on multiple factors, including: •the location•extent of the lesion•weightbearing surface degeneration •duration of injury •joint stabilityGenerally, rehabilitation of non-operative meniscal injury and partial meniscectomy patients requires 6-12 weeksRehabilitation after meniscal repair can take up to six monthsShort Term Treatment•For both the non-operative and post-operative patient includes:•symptom relief measures•joint effusion control•using assistive devices to: •decrease weightbearing load •facilitate a normal gait pattern •Partial meniscectomy patients:can begin FWB as early as 4-7 days after surgery•Meniscal repair patients:usually NWB for 3-6 weeks after surgeryOpen kinematic chain exercises should be used early in rehabilitation:•help build strength•increase range of motion •controlling weightbearing forces•be gradually progressed to closed kinematic chain exercisesShort Long Term TreatmentClosed kinematic chain exercises: •Should be carefully controlled, avoiding: •unnecessary joint pain•Effusion•Reinjury•Should be gradually returned to FWB •Gradually increasing weightbearing forces:•Pool therapy•Underwater treadmillsBalance / Proprioceptive training should be gradually introduced, especially when the patient has a history of recurrent ligamentous injuries髌骨软化症的运动康复疗法Chondromalacia PatellaSports Rehabilitation •Common problem, 25-34% population •Second most common •Affects: athletes and sedentary individuals•Softening/fissuring of the undersurface of the patellaPatellofemoral Joint Reaction Forces Increase PatellofemoralJoint Reaction ForcePatellar Alignment•Tight lateral structures •Excessive pronation–Tightness of gastrocnemius and hamstrings•VMO insufficiency•Patella Alta•Increased Q-AngleReasons for Increased Q-Angle •Femoral neck anteversion •External tibial torsion•Lateral displacement of the tibial tubercleTightness in MusclesVastus Medialis Oblique(VMO) InsufficiencyRealigns the patella mediallythe only dynamic medial stabilizer Active through the whole ROMArises from the adductor magnus tendon Innervated from a branch of the femoral nerve can be activated as a single motor unit.VMO Studies Indicate:EMG recordings in normal subjects: VMO : VL ratio (1 : 1)VMO is tonically activeEMG recordings in patellofemoral sufferers:VMO : VL ratio (< 1 : 1)VMO is phasically active (Segmental activation)Selective Quadriceps Inhibition•Pain reflex inhibitory effect •Knee Joint Effusion 20-30 mls of saline in the knee joint inhibits the VMO50-60 mls requires to inhibit the VL & RFEvaluationSubjective ExaminationPrinciples of the EvaluationPatient HistoryEvaluationPlanning the Objective Examination Based on the subjective cluesA well-planned examination:will limit the possibility of aggravating the problemTests should be prioritized to avoid excessive symptom provocation - may interfere with a complete and accurate assessmentStatic Structural Observation ANTERIORLATERALPOSTERIORDynamic Functional Observation •Help the clinician gain valuable information•These are:•Two-legged full squat•One-legged half squat•Circular one-leg hoppinga) clockwiseb) counterclockwiseShould be compared bilaterally / normal Should be noted:Willingness to perform the testthe symptom responseSigns of apprehensionRange of MotionOpen Chain & Closed Chain Physiological MobilityAccessory Mobility - Gliding tests •Patellofemoral joint•1. superior•2. inferior •3. medial•4. lateralPatellar OrientationSpecific Manual Muscle Testing (MMT):Clinician resists the contraction isometricallyStrength should be:* grad strength* note any symptom responseShould be included all the muscle:* acting on the knee* acting across the knee* influencing the knee •Especially:–VMO–Quads–Hamstrings–Hip Abd ControlMuscle Length•Hamstrings•Gastrocs•Hip IR•Hip ERSpecial Tests•Clarke’s Test•Patella Apprehension Test •Patella Tilt Test•Ober’s Test•VMO Test•OthersLigamentsMenisciThe connections of the lateral meniscus are:•fairly loose–more mobility on the lateral tibial condyle•not easy to be injuredThe connections of the medial meniscus are:•fairly tight–lack of mobility。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
a r X i v :0807.0702v 1 [p h y s i c s .f l u -d y n ] 4 J u l 2008Instability criterion for oblique modes in stratified circular Couette flow C.Normand Institut de Physique Th´e orique,CNRS,URA 2306CEA,IPhT,91191Gif-sur-Yvette Cedex,France.contact:christiane.normand@cea.fr July 4,2008Abstract An analytical approach is carried out that provides an inviscid stability criterion for the strato-rotational instability (in short SRI)occurring in a Taylor-Couette system.The control parameters of the problem are the rotation ratio µand the radius ratio η.The study is motivated by recent numerical [1]and experimental [2]results reporting the existence of unstable modes beyond the Rayleigh line for centrifugal instability (µ=η2).While the modified Rayleigh criterion for stably stratified flows provides the instability condition,µ<1,unstable modes were never found beyond the line µ=η.Taking into account finite gap effects and considering non axisymmetric perturbations in the small azimuthal wavenumber limit,we derive the instability condition :µ<2η2/(1+η2).For a thin gap,η=1−ε,this is in agreement with the condition µ<ηfound numerically for η=0.78and experimentally for η=0.8.For a wide gap and ηsmall the instability condition is approximated by µ<2η2.Whatever the gap size,instability is predicted for values of µlarger than the critical one,µc =η2,corresponding to centrifugal instability.1IntroductionDespite its long-lasting study since the work of Taylor[3],the stability of cylindrical Couette flow remains a vivid research area attracting many investigators.A survey of the literature on the topic can be found in[4].Theflow occurs in the annular gap between two concen-tric cylinders of radii R1<R2rotating independently at the angular velocitiesΩ1andΩ2, respectively.The control parameters are the radius ratioη=R1/R2and the rotation ratio µ=Ω2/Ω1.Inside the gap,the angular velocity profile of the laminar steadyflow isΩ(r).Its linear stability with respect to axisymmetric perturbations is governed,in the inviscid limit, by the Rayleigh criterion[5]:d(r2Ω)2/dr>0.A generalized Rayleigh criterion for non-axisymmetric centrifugal instabilities has been derived for a free axisymmetric vortex using a large axial wavenumber WKB approximation[6].The instability takes the form of a spatially oscillating mode localized between two turning points where it matches with the exponentially decaying solutions outside.The generalization to take into account a background rotation and a stable stratification did not reveal fundamental changes in the Rayleigh criterion.This contrasts with what was found for boundedflows[7,8,9].In these studies,it was shown that a stable stratification drastically changes the stability criterion,showing a strong analogy with the modified Rayleigh criterion obtained when a magneticfield parallel to the cylinders’axis is present.In the hydromagnetic case[10,11]the condition for stability with respect to axisymmetric perturbations is:d(Ω2)/dr>0.The presence of a stable stratification has a similar effect on the Rayleigh criterion though its validity is in that case restricted to non axisymmetric perturbations[7,8,9].In the astrophysical context,the magneto-rotational instability(MRI)was recognized as a potential source of turbulence in accretion disks[12],especially Keplerian disks,with angular velocityΩ(r)∼r−3/2,unable to sustain centrifugal instability according to the standard Rayleigh criterion.The MRI mechanism is robust,it occurs in bounded as well as unbounded flows and for compressible or incompressiblefluid.The capacity of the strato-rotational instability(SRI)to trigger turbulence in Keplerian disks raises the open question of what are the appropriate radial boundary conditions at the edges of the disk.The existence of SRI has been demonstrated in the inviscid limit for perturbations that satisfy no normal flow conditions on the channel walls.In the viscous case,both the no-slip[1]and stress-free [8]conditions give rise to instability.Mixed boundary conditions in which no normalflowis imposed on one side of the channel and zero pressure on the other side were not able to sustain growing modes[9].The effect of a vertically varying stratification was also examined [9]showing that SRI persists in that case.Beside possible applications in geophysics and astrophysics,SRI was studied in the labo-ratory.Until very recently the conclusion of experimental studies was that a stable vertical stratification stabilizes theflow[13,14,15].The experimental evidence of the strato-rotational instability(SRI)in a Taylor-Couette system was definitely assessed in[2].Moreover,the val-ues of the control parameters for which instability occurs were found in good agreement with numerical predictions[1]that yields the condition:µ<η,for instability.The aim of the present contribution is to derive an instability criterion by an entirely ana-lytical analysis that improves previous studies achieved in the small gap limit[7,8,9].When curvature effects are neglected the problem under consideration reduces to the stability of a stratified plane Couetteflow rotating at constant angular velocityΩ.In cartesian coordinates (x,y,z)theflow velocity is V=(0,Sx,0)where S is the constant shear.The condition for instability in the stratified case is S/2Ω<0while the standard Rayleigh-Pedley criterion gives:S/2Ω<−1,when there is no stratification.The substitution S→rΩ′,is often used to deduce the modified Rayleigh criterion for stratifiedflows with curved streamlines :d(Ω2)/dr<0.When applied to the circular Couetteflow,the instability criterion simply yields:µ<1.However,in numerical and experimental studies[1,2]achieved forfinite values of the gap,unstables modes were never found beyond the lineµ=η.The narrowing of the instability range is possibly due to curvature effects that were not taken into account with sufficient accuracy in the transposition S→rΩ′.In the present contribution a more appropriate treatement of curvature effects is carried out leading to an instability criterion that involves the two parametersµandη.Our stability results will be compared to the experimental ones[2]obtained for a value of the radius ratioη=0.8,and to the numerical ones[1]forη=0.78andη=0.3corresponding to a wider gap.2Stratified circular CouetteflowIn cylindrical coordinates(r,ϕ,z)the velocityfield in the basic state is V=(0,rΩ(r),0)with the angular velocity given by[1]Ω(r)=A1−η2,and B=Ω1µ−η2rZu=−iGrΩp=−iσDp(3)where D≡∂/∂r and D∗=D+1/r.Here,σ=ω−lΩ,and Z=2Ω+rΩ′.The quantities K and G are given belowK=σ2−N2,and G=σ2m2+l2withσ1=ω1−l1Ω.It must be stressed that the generalization of the Rayleigh criterion[6] performed in the limit m>>1,with a different scaling for the frequency assumed of order unity,is not equivalent to our approach.This could explain why SRI was not found in[6].The perturbations u and p are expanded according top=p0+εp1+...and u=εu1+ (5)and substituted in(2)-(3)to gives at the leading order inεthe set of equations:l1σ1D∗u1+Ωp0=iσ1Dp0(7)rWe have introduced the Rayleigh discriminantΦ=2ΩZ and the function g(r)=ˆm2σ21−l21/r2 withˆm=m/N.Eqs.(6)-(7)are the circular version of those obtained for a plane Couette flow[9],they constitute a set of coupled equations providedΦ=0.It was shown in[9] that elimination of u1leads to a simple equation for p0,its two independent solutions being exponential functions.Then substituting p0in(7)gives immediately the expression for u1. Finally,satisfaction of the conditions u1=0,on the two boundaries confining theflow leads to the instability criterion.The same procedure is applied here to the cylindrical case.After some calculations,thefirst step gives the equation satisfied by p0σ1 D2p0+ 1Φ Dp0−ˆm2Φp0 +2l1Ω∂r Log ΦΩ′r−Φx)found in[9]for the stratified rotating plane Couetteflow when the Rayleigh criterion for stability,Φ>0,is√satisfied.For circular Couetteflow andΦ=4BΩ,we shall introduceˆp=p0/Ωr4 2−3Awith the differential operator L0=D∗D accounting for curvature effects.We shall assume thatˆm is large enough so that the term inˆm2dominates in Eq.(10).Our analysis differs from previous studies that linearized the angular velocity:Ω(r)=Ω(r0)+(r−r0)Ω′+···around a mean radius r0as in the thin gap limit.Here,the exact expression ofΩ(r)given in (1)is substituted in(10)that becomesL0ˆp−4ˆm2B AAB andα=2ˆmB.The expression forˆp isˆp=A0I n(αr)+B0K n(αr)(12) where the unknown coefficients A0and B0will be determined by the satisfaction of the boundary conditions on u1.Substituting(12)in(7)and using the relation between the√Bessel functions and their derivatives,one gets u1=ˆu/Ψn+σ1 n2Ω Ψn∓αΨn−1 (14)rIn the following,we shall neglect the terms inΩ′/Ωthat appear in factor ofσ1,which are small compared to the terms proportional to n orαwhich behave likeˆm.Satisfaction of the boundary conditions u1(R1)=u1(R2)=0provides the algebraic systemMA=0(15) with the vector A=(A0,B0)and the elements of the matrix M given by M ij=U j(R i). Their expressions are easily deduced fromλiU(R i)=2.3Dispersion relationThe vanishing of the determinant associated to(15)leads to the dispersion relationλ1R2S n−α2ˆσ1ˆσ2S n−1+αˆσ2λ1R2C21=0(17)where we have introduced the quantitiesS n=I(1)n K(2)n−I(2)n K(1)n and C ij=I(i)n K(j)n−1+I(j)n−1K(i)n for(i,j)∈(1,2)(18) To simplify the calculations we shall use the asymptotic expansions at large arguments of the modified Bessels functions I and K.At the lowest order,the asymptotic behaviors of expressions(18)are:S n=S n−1≈sinh(α1−α2)α1α2and C12=C21≈cosh(α1−α2)α1α2(19)With q=α(R2−R1),the dispersion relation(17)readsλ1λ2R1−ˆσ1λ2R1R2−X+Ω1Ω2l21 X−4(n−1)R1(1−η) nω21−nl1(Ω1+Ω2)ω1+(n−2)l21Ω1Ω2 (22) where a term of order of magnitude smaller than n and n−2has been neglected in T2. The quantity X involved in expression(21)for T1can be expressed in terms of the control parameters,µandη,as followsX=n21−η(23)2.4Instability criterionIt is worth while noticing that the sign of the quantityη−µwhich appears in expression(23) for X seems determinant for the stability of the system according to what has been observed both in experiments[2]and in numerical computations[1].To check whether our calculations support thisfinding we shall determine the roots of the dispersion relation(20).Instabilitycould occur if there is a pair of complex conjugate roots,one with negative imaginary part corresponding to instability growth,the other to decay.The calculation of the discriminant and the determination of its sign is facilitated by introducing the quantities Y,Z and Q= Q0coth q defined as follows:Y=2nR1R2and Q0=nα1−η(1−µ)(µ−η2)(R1R2)2(Ω1+Ω2)2−4(X−Q)1+η1+η2(28)For small gap size we can write:η=1−ε.Expansion of(28)tofirst order inεgives the approximated conditionµ<η,in agreement with numerical[1]and experimental[2]results found forη=0.78andη=0.8respectively.For these two values of the gap the exact condition (28)predicts instability forµ<0.756andµ<0.78,well beyond the corresponding Rayleigh linesµ=0.6andµ=0.64.For larger gap size,consideringηas a small expansion parameter and neglecting terms of orderη4,the condition(28)becomesµ<2η2.This confirms that the frontier for instability is beyond the Rayleigh line which is exceeded by a factor two.However,the instability condition(28)do notfit with accuracy the numerical results found in[1]for η=0.3.The discrepancy could be explained by the behavior of the numerical neutral curves (Reynolds number versusµ)that strongly depend on the azimuthal wavenumber[1].The instability condition(28)which is independent of l cannot reproduce this feature.However, the instability boundary:µ=0.165,deduced from(28)forη=0.3,fits surprisingly well with the numerical results obtained for the azimuthal mode with wavenumber l=3,while it is not the case for the smaller values l=1,2.3ConclusionWe performed an inviscid stability analysis of SRI in a Taylor-Couette system.Finite gap effects were taken into account more appropriately than in previous inviscid approaches.The assumptions that have been made never concerned the angular velocity profile which is kept equal toΩ=A/r2+B.Thus,gap size effects manifest themselves through the quantities A and B which depend onµandη,the control parameters of the system.For intermediate values of the gap(η≈0.8),the stability boundary:µ=ηwas recovered with a good accuracy in agreement with numerical[1]and experimental[2]results.For a wider gap(η=0.3)the agreement with numericalfindings is better for the mode l=3than for the modes l=1,2, though the reason of such a behavior is not yet clearly understood.The angular velocity profile of circular Couetteflow is peculiar since it allows an analytical resolution for the pressure perturbations in terms of Bessel functions.This is an essential step in the above derivation of the stability criterion for SRI in incompressiblefluid.A slight change inΩ(r)can lead to completely different stability results.Aflow with constant angular momentum(Ω∼r−2)obtained when B=0in(1),was considered in a thin cylindrical shell [16].This type offlow is generally assumed centrifugally stable,its Rayleigh discriminant being equal to zero.WhenΦ=0,Eqs.(6)and(7)are decoupled and the above analysis for SRI cannot be applied.In that case,the existence of nonaxisymmetric unstable modes was proved for unstratifiedflow in a compressiblefluid[16]with an equation of state of the type p∼ργ.It will be interesting in future work to examine if the present analytical approach could be extended to compressiblefluids for appropriate angular velocity profiles.References[1]D.Shalybkov and G.Rudiger,Stability of density-stratified viscous Taylor-Couetteflows, Astronom and Astrophys.438,411-417(2005).[2]M.Le Bars and P.Le Gal,Experimental analysis of the Strato-Rotational Instability ina cylindrical Couetteflow,Phys.Rev.Lett.99,064502(2007).[3]G.I.Taylor,Stability of viscousfluid contained between two rotating cylinders,Phil. Trans.Roy.Soc.London A223,289-343(1923).[4]R.Tagg:A guide to literature related to the Taylor-Couette problem,in Ordered and turbulent patterns in Taylor-Couetteflow,edited by C.D.Andereck and F.Hayot(Plenum, New York,1992).[5]Lord Rayleigh,On the dynamics of revolvingfluids,Proc.Roy.Soc.Cambridge,A93, 148-154(1916).[6]P.Billant and F.Gallaire,Generalized criterion for non-axisymmetric centrifugal insta-bilities,J.Fluid Mech.542,365(2005).[7]I.Yavneh,J.C.McWilliams and M.J.Molemaker,Non-axisymmetric instability of centrifugally-stable stratified Taylor-Couetteflow,J.Fluid Mech.448,1-21(2001).[8]B.Dubrulle,L.Mari´e,C.Normand,D.Richard,F.Hersant and J.-P.Zahn,A hydrody-namic shear instability in stratified disks,Astronom and Astrophys.429,1-13(2005).[9]O.M.Umurhan,On the stratorotational instability in the quasi-hydrostatic semi-geostrophic limit,Mon.Not.R.Astron.Soc.365,85-100(2006).[10]S.Chandrasekhar,The stability of non-dissipative Couetteflow in hydromagnetics,Proc. Nat.Acad.Sci.46,253(1960).[11]E.P.Velikhov,Stability of an ideally conducting liquidflowing between cylinders rotating in a magneticfield,J.Exp.Theoret.Phys.36,1398-1404(1959).[12]S.A.Balbus and J.F.Hawley,Instability,turbulence and enhanced transport in accretion disk.Rev.Mod.Phys.70,1-53(1998).[13]E.M.Withjack and C.F.Chen,An experimental study of Couette instability of stratified fluids,J.Fluid Mech.66,725(1974).[14]B.M.Boubnov,E.B.Gledzer and E.J.Hopfinger,Stratified circular Couetteflow: instability andflow regimes,J.Fluid Mech.292,333-358(1995).[15]F.Caton,B.Janiaud and E.J.Hopfinger,Stability and bifurcations in stratified Taylor-Couetteflow,J.Fluid Mech.419,93-124(2000).[16]J.C.B.Papaloizou and J.E.Pringle,The dynamical stability of differentially rotating discs with constant specific angular momentum,Mon.Not.R.Astronom.Soc.208,721-750 (1984).11。
