A note on D-branes in group manifolds flux quantisation and D0-charge

合集下载

On a Unified Theory of Generalized Branes Coupled to Gauge Fields, Including the Gravitatio

a r X i v :h e p -t h /0605126v 2 7 M a y 2007On a Uniﬁed Theory of Generalized Branes Coupled to Gauge Fields,Including the Gravitational and Kalb-Ramond Fields M.Pavˇs iˇc Joˇz ef Stefan Institute,Jamova 39,SI-1000Ljubljana,Slovenia;E-mail:matej.pavsic@ijs.si ABSTRACT We investigate a theory in which fundamental objects are branes described in terms of higher grade coordinates X µ1...µn encoding both the motion of a brane as a whole,and its volume evolution.We thus formulate a dynamics which generalizes the dynamics of the usual branes.Geometrically,coordinates X µ1...µn and associated coordinate frame ﬁelds {γµ1...µn }extend the notion of geometry from spacetime to that of an enlarged space,called Cliﬀord space or C -space.If we start from 4-dimensional spacetime,thenthe dimension of C -space is 16.The fact that C -space has more than four dimensions suggests that it could serve as a realization of Kaluza-Klein idea.The “extra dimensions”are not just the ordinary extra dimensions,they are related to the volume degrees of freedom,therefore they are physical,and need not be compactiﬁed.Gauge ﬁelds are due to the metric of Cliﬀord space.It turns out that amongst the latter gauge ﬁelds there also exist higher grade,antisymmetric ﬁelds of the Kalb-Ramond type,and their non-Abelian generalization.All those ﬁelds are naturally coupled to the generalized branes,whose dynamics is given by a generalized Howe-Tucker action in curved C -space.1IntroductionPoint particle is an idealization never found in nature.Physical objects are extended and possess in principle inﬁnitely many degrees of freedom.It is now widely accepted that even at the“fundamental”level objects are extended.Relativistic strings and higher dimensional extended objects,branes,have attracted much attention during last three decades[1,2,3,4].An extended object,such as a brane,during its motion sweeps a worldsheet,whose points form an n-dimensional manifold1V n embedded in a target space(time)V N.World-sheet is usually considered as being formed by a set of points,that is,with a worldsheet we associate a manifold of points,V n.Alternatively,we can consider a worldsheet as being formed by a set of closed(n−1)-branes(that we shall call“loops”).For instance,a string world sheet V2can be considered as being formed by a set of1-loops.In particular such a 1-loop can be just a closed string which in the course of its evolution sweeps a worldsheet V2which,in this case,has the form of a world tube.But in general,this need not be the case.A set of1-loops on V2need not coincide with a family of strings for various values a time-like parameter.Thus even a worldsheet swept by an open string can be considered as a set of closed loops.The ideas that we pursue here are motivated and based to certain extent by those developed in refs.[6]–[8].We shall employ the very powerfull geometric language based on Cliﬀord algebra[9,10],which has turned out to be very suitable for an elegant formulation of p-brane theory and its generalization[11]–[16].We will employ the property that multivectors of various deﬁnite grades,i.e.,R-vectors,since they represent oriented lines,areas,volumes,...,shortly,R-volumes(that we will also call R-areas),can be used in the description of branes.With a brane one can associate an oriented R-volume (R-area).Superpositions of R-vectors are generic Cliﬀord numbers,that we call polyvec-tors.They represent geometric objects,which are superposition of oriented lines,areas, volumes,....,that we associate,respectively,with point particles,closed strings,closed 2-branes,...,or alternatively,with open strings,open2-branes,open3-branes,etc..A polyvector is thus used for description of a physical object,a generalized brane,whose components are branes of various dimensionalities.We thus describe branes by means of higher grade coordinates xµ1...µR,R= 0,1,2,...,N,corresponding to an oriented R-area associated with a brane,where N is the dimension of the spacetime V N we started from.The latter coordinates are collec-tive coordinates,[13,14],analogous to the center of mass coordinates[11].They do not provide a full description of an extended object,they merely sample it.Nevertheless,ifhigher grade coordinates xµ1...µR are given,then certainly we have more information about an extended object than in the case when only its center of mass coordinates are given. By higher grade coordinates we no longer approximate an extended object with a point like object;we take into account its extra structure.We associate all those higher grade coordinates with points of an2N-dimensionalspace,called Cliﬀord space,shortly C-space,denoted C VN .Every point of C VNrepresentsa possible extended event,associated with a generalized brane.In order to consider an object’s dynamics,one has to introduce a continuous parameter, sayτ,and consider a mappingτ→xµ1...µR=Xµ1...µR(τ).So functions Xµ1...µR(τ)describe a curve in an2N-dimensional space C VN.This generalizes the concept of worldline Xµ(τ)in spacetime V N.The action principle is given by the minimal length action in C VN.That the objetcs,sampled by Xµ1...µR satisfy such dynamics is our postulate[11,12,16,15],we do not derive it.The intersection of a C-space worldline Xµ1...µR(τ)with an underlying spacetime V N(which is a subspace of C VN)gives,in general an extended event2.Therefore,what we observe in spacetime are“instantonic”extended objects that are localized both in space-like and time-like directions3.According to this generalized dynamics,worldlines areinﬁnitely extended in C VN,but in general,their intersections with subspace V N areﬁnite. In spacetime V N we observeﬁnite objects whose time like extension may increase with evolution,and so after a while they mimic the worldlines of the usual relativity theory. This has been investigated in refs.[11,15].We have also found that such C-space theory includes the Stueckelberg theory[17]–[24]as a particular case,and also has implications for the resolution of the long standing problem of time in quantum gravity[25,12,26].Objects described by coordinates xµ1...µR are points in Cliﬀord space C VN,also calledextended events.Objects given by functions Xµ1...µR(τ)are worldlines in C VN.A further possibility is to consider,e.g.,continuous sets of extended events,described by functions Xµ1...µR(ξA),A=1,2,...,2n,n<N,whereξA≡ξa1...a r,r=0,1,2,...,n<N,are2n higher grade coordinates denoting oriented r-areas in the parameter space R n.FunctionsXµ1...µR(ξA)describe a2n-dimensional surface in C VN.This generalizes the concept of worldsheet or world manifold Xµ(ξa),a=1,2,...,n,i.e.,the surface that an evolving brane sweeps in the embedding spacetime V N.A C-space worldline Xµ1...µR(τ)does not provide a“full”description of an extended object,because not“all”degrees of freedom are taken into account;Xµ1...µR(τ)only pro-vides certain“collective”degrees of freedom that sample an extended object.On the contrary,a C-space worldsheet Xµ1...µR(ξA)provides much more detailed description,be-cause of the presence of2n continuous parametersξA,on which the generalized coordinatefunctions Xµ1...µR(ξA)depend.In particular,the latter functions can be such that they describe just an ordinary worldsheet,swept by an ordinary brane.But in general,they describe more complicated extended objects,with an extra structure.We equip our manifold C VNwith metric,connection and curvature.In the case ofvanishing curvature,we can proceed as follows.We choose in C VNan origin E0withcoordinates Xµ1...µR(E0)=0.This enables us to describe points E of C VNwith vectors pointing from E0to E.Since those vectors are Cliﬀord numbers,we call them polyvectors.So points of ourﬂat space C VN(i.e.,with vanishing curvature)are described by polyvectorsxµ1...µRγµ1...µR ,whereγµ1...µRare basis Cliﬀord numbers,that span a Cliﬀord algebra C NSo our extended objects,the events E in C VN,are described by Cliﬀord numbers.This actually brings spinors into the description,since,as is well known,the elements of left (right)ideals of a Cliﬀord algebra represent spinors[27].So one does not need to postulate spinorial variables separately,as is usually done in string and brane theories.Our model is an alternative to the theory of spinning branes and supersymmetric branes,including spinning strings and superstrings[1].In refs.[16]it was shown that the16-dimensional Cliﬀord space provides a framework for a consistent string theory.One does not need to postulate extra dimensions of spacetime.One can start from4-dimensional spacetime, andﬁnds that the corresponding Cliﬀord space provides enough degrees of freedom for a string,so that the Virasoro algebra has no central charges.According to this theory all16 dimensions of Cliﬀord space are physical and thus observable[11,28,29],because they are related to the extended nature of objects.Therefore,there is no need for compactiﬁcation of the extra dimensions of Cliﬀord space.As a next step it was proposed[28,29]that curved16-dimensional Cliﬀord space can provide a realization of Kaluza-Klein theory.Gravitational as well as other gauge interactions can be uniﬁed within such a framework.In ref.[28,29]we considered Yang-Mills gaugeﬁeld potenitals as components of the C-space connection,and Yang-Mills gaugeﬁeld strengths as components of the curvature of that connection.It was also shown[29]that in a curved C-space which admits K isometries,Yang-Mills gauge potentials occur not only in the connection,but also in the metric,or equivalently,in the vielbein.In this paper we concentrate on the latter property,and further investigate it by studying the brane action in curved background C-space.So we obtain the minimal coupling terms in the classical generalized brane action,and we show that the latter coupling terms contain the ordinary4-dimensional gravitationalﬁelds,Yang-Mills gauge ﬁelds Aαµ,α=1,2,...,K,and also the higher grade,in general non-Abelian,gaugeﬁeldsAαµ1...µR of the Kalb-Ramond type.We thus formulate an elegant,uniﬁed theory for theclassical generalized branes coupled to all those variousﬁelds.2On the description of extended objects2.1Worldsheet described by a set of point eventsAs an example of a relativistic extended object,let usﬁrst consider the string.An evolving string sweeps a worldsheet,a physical object in the embedding spacetime.Worldsheet can be considered as being formed by a set of point events.So with a worldsheet we can asso-ciate a2-dimensional manifold V2,called world manifold,embedded in an N-dimensional target space V N.With every point on V2we associate two parameters(coordinates)ξa,a=0,1which are arbitrary(like“house numbers”).The embedding of V2into V N is described by the mappingξa→xµ=Xµ(ξa),ξa∈R n⊂R n(1)where xµ,µ=0,1,2,...,N−1are coordinates describing position in V N,whilst Xµ(ξa)are embedding functions(Fig.1),deﬁned over a domain R n within a set R n of real numbers.T©x1x2x0ξaV2·V Na point......................................................................................................................................................................................................................................................................................................................................................................................................................................................Figure1:A worldsheet can be considered as being formed by a set of point events as-sociated with points of a world manifold V2embedded in V N,described by the mapping ξa→xµ=Xµ(ξa).With our worldsheet we thus associate a2-parameter set of points described by func-tions Xµ(ξ0,ξ1).We distinguish here the physical object,the worldsheet,from the corre-sponding mathematical object,the manifold(world manifold)V2.Strictly,we should use two diﬀerent symbols for those diﬀerent objects.In practice,we will not be so rigorous, and we will simply denote worldsheet by the symbol V2(in general V N).2.2Worldsheet described by a set of loopsIn previous section a worldsheet was described by a2-parameter set of points described by functions Xµ(ξ0,ξ1)Alternatively,instead of points we can consider closed lines,loops, each being described by functions Xµ(s),where s∈[s1,s2]⊂R is a parameter along a loop.A1-parameter family of such loops Xµ(s,α),α∈[α1,α2]⊂R sweeps a worldsheet V2.This holds regardless of whether such worldsheet is open or closed.However,in the case of an open worldsheet,the loops are just kinematically possible objects,and they cannot be associated with physical closed strings.In the case of a closed worldsheet,a world tube,we can consider it as being swept by an evolving closed string.We will now demonstrate,how with every loop one can associate an oriented area, whose projections onto the coordinate planes are Xµν.The latter quantities are func-tionals of a loop Xµ(s).If we consider not a single loop,but a family of loops Xµ(s,α),α∈[α1,α2],then Xµνare functions of parameterα,besides being functionals of a loop.So we obtain a1-parameter family of oriented areas described by functions Xµν(α).Let us stress again that for everyﬁxedα,it holds,of course,that Xµνare functionals of Xµ(s,α).If we choose a loop B on V2,i.e.,a loop from a given family{Xµ(s,α),α∈[α1,α2]}, then we obtain the corresponding components Xµνof the oriented area by performing the integration of inﬁnitesimal oriented area elements over a chosen surface whose boundary is our loop B.Given a boundary loop B,it does not matter which surface we choose.In the following,for simplicity,we will choose just our worldsheet V2for the surface.Let us now consider a surface element on V2.Let dξ1=dξa1e a and dξ2=dξa2e a be two inﬁnitesimal vectors on V2,expanded in terms of basis vectors e a,a=0,1.An inﬁnitesimal oriented area is given by the wedge productdΣ=dξ1∧dξ2=dξa1dξb2e a∧e b=1space basis vectors γµ:e a =∂a X µγµ(4)In the following we will adopt the geometric calculus in which basis vectors are Cliﬀord numbers satisfyingγµ·γν≡12(γµγν−γνγµ)(6)Analogous relations we have for the worldsheet basis vectors e a :e a ·e b ≡12(e a e b −e b e a )(8)where γab is the metric on V 2which,according to eq.(4),canbeconsidered as being induced from the target space.If we insert the relation (4)into eq.(2)we havedΣ=12X µνγµ∧γν=12ΣBd ξab 12 ΣB d ξab (∂a X µ∂b X ν−∂a X ν∂b X µ)(11)By Stokes theorem this is equal toX µν[B ]=1∂s −X ν∂X µ4Such integration poses no problem in ﬂat V N .In curved space we may still use the same expression(10)which then deﬁnes such integral that all vectors are carried together by means of parallel transport along geodesics into a chosen point of V N where the integration is actually performed [33].where Xµ(s)describes a boundary loop B,s being a parameter along the loop.Eq.(12)demonstrates that Xµνare components of the bivector,determining an ori-ented area,associated with a surface enclosed by a loop Xµ(s)on the worldsheet V2. Hence there is a close correspondence between surfaces and the boundary loops.The components Xµνcan be therefore be considered as bivector coordinates of a loop.These are collective coordinates,since the detailed shape(conﬁguration)of the loop is not de-termined by Xµν.Only the oriented area associated with a surface enclosed by the loop is determined by Xµν.Therefore Xµνrefers to a class of loops,from which we may choose a representative loop,and say that Xµνare its coordinates.From now on,‘loop’we will be often a short hand expression for a representative loop in the sense above.By means of eqs.(10)–(12)we have performed a mapping from an inﬁnite dimensional space of loops Xµ(s)into aﬁnite dimensional space of oriented areas Xµν.Instead of describing loops by inﬁnite dimensional objects Xµ(s),we can describe them byﬁnite dimensional objects,oriented areas,with bivector coordinates Xµν.We have thus arrived at aﬁnite dimensional description of loops(in particular,closed strings),the so called quenched minisuperspace description suggested by Aurilia et al.[13].When we consider not a single loop Xµ(s),but a1-parameter family of loops Xµ(s,α), we have a worldsheet,considered as being formed by a set of loops.By means of eqs.(10)–(12),with every loop within such a family,i.e.,for aﬁxedα,we can associate bivector coordinates Xµν.For variableαwe then obtain functions Xµν(α).This is a quenched minisuperspace description of a worldsheet.A full description is in terms of embedding functions Xµ(ξ0,ξ1),or a family of loops Xµ(s,α).In the following we will consider two particular choices for parameterα.In eq.(10)we have the expression for an oriented area associated with a loop B.It has been obtained upon the integration of the inﬁnitesimal oriented surface elements(2). Besides the oriented area we can associate with our loop on V2also a scalar quantity, namely the scalar area A which we obtain according toA= ΣB√γ(dξabǫab)2(14)4i.e.A= √2 |γ|dξ12(15) If we choose dξa1=(dξ1,0),dξa2=(0,dξ2),then dξ12=dξ1dξ2.We imagine that our surface V 2is covered by a family of loops X µ(α,s )(Fig.2),such that the totality of points of all those loops is in one-to-one correspondence with the points of the manifold V 2,and that for parameter αwe take the scalar area A .To every loop there belong bivector coordinates X µν(calculated according to eq.(10))and a scalar parameter A (calculated according to eq.(15)),determining the the scalar area.Dependence of X µνon A is characteristic for a given class of surfaces V 2.If we consider a diﬀerent class of surface V 2,then functions X µν(A )are in general diﬀerent (Fig.3)T©x 1x 2x 0A V 2V N A a 1-loop..................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................Figure 2:We consider a loop on V 2.It determines an oriented area whose extrinsic 2-vector coordinates are X µν.The scalar area of the surface element enclosed by the loop is A .Given an initial loop,functions X µν(A )are characteristic for a class of the surface V 2...............................................................................................................................................................................................................................................................................................................................................................................................................................................................................................(a)(b)Figure 3:(a)Examples of two diﬀerent surfaces belonging to a class of surfaces that all satisfy equation X 12(A )=k .Constant k diﬀers from zero,if a cillindric surface has only one boundary loop,so that,e.g.,the upper part is open,whilst the lower part is closed.(b)Example of surfaces belonging to a class of surfaces that satisfy equation X 12(A )=k A .Instead of starting with a given surface V 2on which we determine a family of loops and calculate the functions X µν(A ),we can start from the other end.We may assume that all what is known are functions X µν(A ).From those functions we do not know whatthe surface(worldsheet)V2exactly is,but we have some partial information(see Fig.3), up to a class of surfaces(worldsheets).Functions Xµν(A)provide a means of describing a surface V2,although not in all details.Instead of the scalar area A we may take as the parameter any other parameter5.We may take,for instance,just the integral6in the parameter space R2ξab(B)= ΣB dξ′ab(16) taken over a domain corresponding to a surfaceΣB with boundary B.So we have a mapping B→ξab(B),such that to any boundary loop B of our family there correspond parametersξab.Because of the propertyξab=−ξba,(where a,b=0,1if V2is time like),there is in fact a single parameterξ12.The extrinsic2-vector coordinates Xµνare functions ofξab.The mappingξab→xµν=Xµν(ξab)(17) determines a class of surfaces V2,embedded in V N,which are all in accordance with eq.(17).Knowing the functions Xµν(ξab)means knowing a class{V2},but not a particular V2∈{V2}.The mappingτ→xµ=Xµ(τ),involving vector coordinates,describes a curve(a “worldline”)in the space spanned by vectorsγµ.The derivative of Xµwith respect toτ, i.e.,˙Xµ=d Xµ/dτ,is the tangent vector to the worldline,or velocity.Similarly,the mapping(17),involving bivector coordinates,describes a curve(a “worldline”)in the space spanned by the bivectorsγµ∧γν,and we can calculate the derivative∂ab Xµν≡∂Xµνn!dξa1...a n e a1∧...∧e a n(19)whered ξa 1...a n =d ξ[a 11d ξa 22...d ξa n ]n (20)If we consider the basis vectors e a on V n as being induced from the basis vectors γµof the embedding space V N according to the relation (4),we havedΣ=1n !d ξa 1...a n ∂a 1X µ1...∂a n X µn γµ1∧...∧γµn (21)After the integration over a ﬁnite n -surface ΣB with boundary B we obtain a ﬁnite n -vector ΣBdΣ=1n ! ΣB d ξa 1...a n ∂a 1X µ1...∂a n X µn γµ1∧...∧γµn =1n !∂[a 1X µ1...∂a n ]X µn γµ1∧...∧γµn (22)Its n -vector components areX µ1...µn [B ]= ΣBd ξa 1...a n 1family is such that the totality of the points of the (n −1)-loops belonging to the family is in one-to-one correspondence with the points of the worldsheet V n .In other words,by mapping (26)we have aquenchedminisuperspace description of worldsheet .We started from a brane described by the embedding functions X µ(ξa ),and derived the expression (23)and functions (26).Once we have X µ1...µ2as functions of a parameter ξa 1...a n ,we may forget about the embedding x µ=X µ(ξa )that we started from.We may assume that all the information available to us are just functions X µ1...µn (ξa 1...a n )given by mapping (26).Then we do not have knowledge of a particular worldshet’s manifold V n ,but of a class {V n }of worldsheet’s manifolds that all satisfy eq.(26)for given functions X µ1...µn (ξa 1...a n ).So we calculate the derivative∂a 1...a n X µ1...µn ≡∂X µ1...µn2d n ξ|e |(e a ∂a X µe b ∂b X µ+2−n )(28)where |e |≡ 7Their inner products e a ·e b =ηab gives the Minkowski metric.weﬁnd the following relations8∂e a∂e b c=nδa b(30)∂|e|∂γcd∂γcd2e c(e a∂a Xµ∂b Xµ+2−n)+∂c Xµ∂d Xµe d=0(32) Performing the inner product with e c and using e c·e c=n weﬁnde a∂a Xµe b∂b Xµ=n(33) and eq.(32)becomese c=∂c Xµ∂d Xµe d(34) This is the equation of“motion”for the Lagrange multipliers e a.In order to understand better the meaning of eq.(34)let us perform the inner product with e a:e c·e a=∂c Xµ∂d Xµe d·e a(35) Since e c·e a=γca and e d·e a=δd a we obtain after renaming the indicesγab=∂a Xµ∂b Xµ(36) This is the relation for the induced metric on the worldsheet.On the other hand,eq.(35) can be written ase a·e b=(∂a Xµγµ)·(∂a Xνγν)(37) from which we have that basis vectors e a on the worldsheet V n are expressed in terms of the embedding space basis vectorsγµ:e a=∂a Xµγµ(38) With our procedure we have thus derived eq.(4)as a solution to our dynamical sytem.Using eq.(36)weﬁnd that the action(28)is equivalent to the well known Howe–Tucker action which is a functional of Xµ(ξ)andγab:I[Xµ,γab]=κ|γ|(γab∂a Xµ∂b Xµ+2−n)(39)3.2Objects described in terms of X µ1...µn (ξa 1...a n )In Sec.2.3we have seen that an alternative description of extended objects,up to a class in which all objects have the same coordinates X µ1...µn ,is given by the mapping(26).Let us assume that such objects are described by the following actionI [X µ1,...,µn ,e ]=κn ! 1∂ξa 1...a n‡× 1∂ξb 1...b n+1 (40)Factor 1/n !inside the bracket comes from the deﬁnition of the worldsheet n -vector (1/n !)e a 1∧...∧e a n ∂X µ1...µn /∂ξa 1...a n .The extra factor 1/n !in front of the bracket comes from the square of the target space n -vector (1/n !)(∂X µ1...µn /∂ξa 1...a n )γµ1∧...∧γµn .The operation ‡reverses the order of vectors.Let us take into account the following relations:e a 1∧...∧e a n =e ǫa 1...a n(41)e a 1∧...∧e a n =e −1ǫa 1...a n (42)e −1=e e ‡·e = n !ǫa 1...a n ξa 1...a n (45)and rewrite the n -area velocity according to∂X µ1...µn∂˜ξ∂˜ξ∂˜ξǫc 1...c n (46)where we have used ∂ξa 1...a n ∂˜ξ=1∂ξc 1...c n(48)By using eqs.((33),(35)and (48)we can rewrite the action (40)asI [X µ1...µn ,λ]=κλn !∂X µ1...µn ∂˜ξ+λ (49)whered˜ξ=1n!ǫa1...a ndξ[a1a...dξa n]n=ǫa1...a ndξa1a...dξa n n=dξ1dξ2...dξn≡d nξ(50)The last step in eq.(50)holds in a coordinates system in which dξa11=dξ1,dξa22=dξ2, ...,dξa n n=dξn.The action(49)is a functional of the n-area variables Xµ1...µn(˜ξ)and a Lagarange multiplierλ,deﬁned in eq.(43).Variation of eq.(49)with respect toλand Xµ1...µn, respectively,givesδλ:1∂˜ξ∂Xµ1...µnd˜ξ 1∂˜ξ=0(52)These are the equations of motion for the n-area variables.Inserting eq.(51)into(49)we obtain the action which is a functional of Xµ1...µn solely: I[Xµ1...µn]=κ d˜ξ 1∂˜ξ∂Xµ1...µnIn the compact notation we setX M≡Xµ1...µR,µ1<µ2<...<µRξA≡ξa1...a r,a1<a2<...<a rand write the mapping(54)asξA→x M=X M(ξA)(55) This is the parametric equation of our generalized extended object.Such object lives in atarget space which is now generalized to Cliﬀord space(shortly C-space).The worldsheet associated with the extended object is also generalized to a Cliﬀord space.In the following we will explain this in more detail.In eq.(54)or(55)we have a generalization of the usual relationξa→xµ=Xµ(ξa),a=1,2,...,n;µ=0,1,2,...,N−1(56) that describes an n-dimensional surface,called worldsheet or world manifold,V n,em-bedded in N-dimensional target space V N.In eq.(56)the space R n of parametersξa is isomorphic to an n-dimensional vector space V n,spanned by an orthonormal basis{h a}. The vector space V n should not be confused with the worldsheet V n,which is a manifold (embedded in a higher dimensional manifold V N).Instead of V n we can consider the corresponding Cliﬀord algebra C n which is itself a vector space.Amongst its elements are r-vectors associated with(r−1)-loops,r= 0,1,2,...,n.A generic object is a superposition of r-vectors for diﬀerent grades,and it isdescribed by a Cliﬀord number,a polyvector,ξa1...a r h a1∧...∧h a r∈C n Our objects are now extended events E[29],superpositions of(r−1)-loops,to which we assign a set of2n parameters(coordinates)ξA≡ξa1...a r,r=0,1,2,...,n according to the mappingE→ξA(E)(57) The assignment is arbitrary.We may choose an object E0to which we assign coordinates ξA(E)=0.This is a coordinate origin.Choosing an origin E0,the polyvectorsξA h A pointing from E0to any E are in one-to-one correspondence with extended events E.The space of extended events is then isomorphic to Cliﬀord algebra C n,and the latter algebra, in turn,is isomorphic to the space of parameter{ξA}=R2n.Therefore we will speak about C n as the parametric Cliﬀord algebra or parametric polyvector space.The parametric space C n is by deﬁnition a(poly)vector space,spanned by a basis h a1∧,r=0,1,2,...,n,formed by the orthonormal basis{h a},a=1,2,...,n.This implies ...∧h arthat C n is a metric space,but its metric is just formal,without any physical content.generated by a Now let us consider the mapping(55)from C n into a Cliﬀord space C VN。

Brauer-Manin obstructions to integral points

a rXiv:079.1154v1[math.A G]7S ep27BRAUER–MANIN OBSTRUCTIONS TO INTEGRAL POINTS ANDREW KRESCH AND YURI TSCHINKEL Abstract.We study Brauer–Manin obstructions to integral points on open sub-sets of the projective plane. 1.Introduction Let k be a number ﬁeld and X a smooth projective geometrically irreducible variety over k .It is well known that the existence of points of X over all completions k v of k does not imply the existence of a k -rational point on X ,in general.This phenomenon is referred to as the failure of the Hasse principle.Examples of failure of the Hasse principle are known for genus 1curves,cubic surfaces,etc.Even when the Hasse principle holds,rational points need not be dense in the set of adelic points of X .This phenomenon,the failure of weak approximation,also is known in many examples.For instance,weak approximation always fails when X (k )=∅and X ⊗¯k (the variety obtained by base change to the algebraic closure ¯k of k )has nontrivial algebraic fundamental group [Min89].Often (e.g.,conjecturally for rational surfaces)the failure of the Hasse principle and weak approximation is explained by the Brauer–Manin obstruction [Man71],[Man74].By the exact sequence from class ﬁeld theory 0→Br(k )−→ v Br(k v )P inv v −→Q /Z →0(where inv v denotes the sum of local invariants)we have the constraint X (k )⊂X (A k )Br :={(x v )∈X (A k )| inv v (α|x v )=0∀α∈Br(X )}on the set X (A k )of adelic points on X .When X (A k )Br =X (A k ),then we say there is a Brauer–Manin obstruction to the Hasse principle,respectively to weak approximation,in case X (A k )Br =∅,respectively,X (A k )Br=∅.For a thorough introduction to the subject,see [Sko01].For a survey,see [Pey05].The study of rational points on projective hypersurfaces is equivalent to the study of integral solutions to homogeneous Diophantine equations f (x 0,...,x n )=0.Many interesting Diophantine problems involve non-homogeneous equations.Their solu-tions can be interpreted as integral points on quasi-projective varieties.Let o k be the ring of integers of k .Let X be an integral model for X ,i.e.,a scheme,projective and ﬂat over Spec(o k )having general ﬁber X .Let Z be a reduced closed subscheme of X ,and set U =X Z .Let Z be an integral model for Z (which is uniquely determined by Z and X ).An integral point of U is a k -rational point on X whose unique extension to an o k -point of X has image disjoint from Z .One can2ANDREW KRESCH AND YURI TSCHINKELalso speak of S-integral points on U by requiring the intersection with the Z to be supported on theﬁbers above aﬁnite collection S of non-archimedean places of k. (The notion of integral and S-integral points on U depends on the choice of integral model X.)One can speak of the Hasse principle,weak approximation,and strong approxi-mation for U,or more accurately,for U=X Z.(For smooth projective varieties, weak and strong approximation are the same.)Strong approximation concerns the approximation of adelic points by integral(or S-integral)points.So,the failure of strong approximation can translate as the statement at a given(non-homogeneous) Diophantine equation has no integral solutions with prescribed congruence conditions. We want to illustrate a failure of strong approximation–concretely realized as the insolubility of Diophantine equations that admit rational solutions as well as v-adic integer solutions for all v–which is explained by the Brauer–Manin obstruction:U(o k)⊂ v∤∞U(o v)× v|∞U(k v) Br(U),where the set on the right is deﬁned to be the tuples of adelic points,integral at all non-archimedean places,whose sum of local invariants is zero with respect to every element of Br(U).The Brauer–Manin obstruction has been extensively studied in the setting of pro-jective varieties;see,e.g.,[CTKS87].But it has only recently begun to be looked at for open varieties;see[CTX].In the known examples,X is a quadric surface, D is a conic,and the relevant Brauer group element is algebraic,i.e.,lies in the kernel of Br(U)→Br(U⊗¯k).In this paper we use transcendental Brauer group elements.Transcendental Brauer–Manin obstructions have previously been exhibited on projective varieties[Wit04],[Har96].We take U to be the complement of a geometrically irreducible smooth divisor D on X.Then,to have Br(X)=Br(U)we must have dim(X)≥2,so the simplest examples occur with X=P2.Over an algebraically closedﬁeld,[AM92]relates the Brauer group of the complement of a smooth divisor to unramiﬁed cyclic coverings of the divisor,by means of an exact sequence:0→Br(X⊗¯k)→Br(U⊗¯k)→H1(D⊗¯k,Q/Z)→0.(1.1) We select D⊂P2for which there are nontrivial unramiﬁed coverings deﬁned over k, and for which(known)constructions of algebras representing ramiﬁed Brauer group elements can be carried out over k.We work over the groundﬁeld k=Q.This note is inspired by lectures of J.-L.Colliot-Th´e l`e ne on his joint work with F.Xu[CTX],in which they use the Brauer–Manin obstruction to give a new ex-planation of the failure of Hasse principle,exhibited in[BR95]and[SX04],in the problem of representing integers by quadratic forms in three variables.2.QuarticConsider the Diophantine equation−2x4−y4+18z4=1.(2.1) We claim that(i)There are solutions in p-adic integers for all p.(ii)There are solutions in Q.(iii)There are no solutions in Z.BRAUER–MANIN OBSTRUCTIONS3Indeed,there is the rational solution(1/2,0,1/2),which is a p-adic integer solution√for p=2.A2-adic integer solution is(0,44ANDREW KRESCH AND YURI TSCHINKELThe algebra(2.2)deﬁnes an element A∈Br(U).This extends to an element of Br(U⊗Z[1/2]),where U=P2Z D.Hence,A is unramiﬁed at all points of U(Z p)for p≥3.According to a2-adic analysis,at Z2-points(X:Y:Z)of P2withX≡0(mod2),Y≡1(mod2),Z≡1(mod2),(2.3) we havef(X,Y,Z)h(X,Y,Z)≡3(mod4),−g(X,Y,Z)h(X,Y,Z)≡3(mod4),hence A is ramiﬁed at all such2-adic points.We leave it to the reader to check that A is unramiﬁed at real points of U.So,for any integers X,Y,Z satisfying(2.3)there must exist a prime divisor p≥3of f(X,Y,Z).Examining reductions modulo16, any integer solution to(2.1)would have to satisfy(2.3),hence(2.1)has no integer solutions.Notice that there is an obvious integer point(0:1:0)on U.So,this example constitutes a Brauer–Manin obstruction to strong approximation on U.3.CubicConsider the Diophantine equationy2z−(4x−z)(16x2+20xz+7z2)=1.(3.1) We claim,again,that there are solutions in Z p for all p,in Q,but not in Z.A rational solution is(1/4,1,1),and a2-adic integer solution is(0,0,3BRAUER–MANIN OBSTRUCTIONS5 [Har96] D.Harari,Obstructions de Brauer–Manin transcendantes,in S.David,ed.,Number theory(Paris,1993–1994)London Math.Soc.Lecture Note Ser.235,Cambridge Univ.Press,Cambridge,1996,75–87.[Jac01] B.Jacob,Division algebras which only ramify along a hyperelliptic curve in P2,J.Algebra 242(2001),720–728.[KT04] A.Kresch and Yu.Tschinkel,On the arithmetic of del Pezzo surfaces of degree2,Proc.London Math.Soc.(3)89(2004),545–569.[KRTY06]B.`E.Kunyavskii,L.H.Rowen,S.V.Tikhonov,and V.I.Yanchevskii,Division algebras that ramify only on a plane quartic curve,Proc.Amer.Math.Soc.134(2006),921–929. [Man71]Yu.I.Manin,Le groupe de Brauer-Grothendieck en g´e om´e trie diophantienne,in Actes du Congr`e s International des Math´e maticiens(Nice,1970),Tome1,Gauthier-Villars,Paris,1971,401–411.[Man74]Yu.I.Manin,Cubic forms:algebra,geometry,arithmetic,North-Holland Publishing Co., Amsterdam,1974.[Min89]Kh.P.Minchev,Strong approximation for varieties over an algebraic numberﬁeld,Dokl.Akad.Nauk BSSR33(1989),5–8,92.[Pey05] E.Peyre,Obstructions au principe de Hasse et`a l’approximation faible,S´e minaire Bour-baki,2003-04,Ast´e risque299(2005),Exp.No.931,165–193.[SX04]R.Schulze-Pillot and F.Xu,Representations by spinor genera of ternary quadratic forms, in R.Baeza et al,eds.,Algebraic and arithmetic theory of quadratic forms(Talca,2002),Contemp.Math.344,Amer.Math.Soc.,Providence,2004,323–337.[Sko01] A.N.Skorobogatov,Torsors and rational points,Cambridge University Press,Cam-bridge,2001.[Wit04]O.Wittenberg,Transcendental Brauer-Manin obstruction on a pencil of elliptic curves, in B.Poonen and Yu.Tschinkel,eds.,Arithmetic of higher-dimensional algebraic varieties(Palo Alto,CA,2002),Progr.Math.226,Birkh¨a user Boston,Boston,MA,2004,259–267.Institut f¨u r Mathematik,Universit¨a t Z¨u rich,Winterthurerstrasse190,CH-8057Z¨u rich, SwitzerlandE-mail address:andrew.kresch@math.unizh.chMathematisches Institut,Georg-August-Universit¨a t G¨o ttingen,Bunsenstrasse3-5,D-37073G¨o ttingen,Germany and Courant Institute,251Mercer Street,New York,NY 10012E-mail address:tschinkel@。

2023-2024学年上海市浦东新区东昌中学高一下学期期末考试英语试题

2023-2024学年上海市浦东新区东昌中学高一下学期期末考试英语试题1. The manager had fallen asleep where he __________, without undressing.A．had laid B．was lying C．was laying D．had lied 2. Mr Zhang is said __________ in American now, but I don’t know which state he is in.A．to have studied B．having studied C．to be studying D．to study 3. The visitors came to the railway station, only __________ that the train had left.A．to tell B．to be told C．told D．being told 4. He was speaking to his chemistry teacher __________ I saw him.A．for the first time B．the first time C．the first timewhen D．when the first time5. You’d better take something to read when you go to see a doctor __________ you have to wait.A．as if B．even if C．so that D．in case6. She is no longer the sweet girl __________ she used to be.A．what B．who C．that D．when7. Their school is built __________ there was a big rice field.A．what B．where C．in which D．which8. With the fast development of agriculture, the people ________ village I taught before had lived a happy life.A．who B．whose C．in whose D．in which9. The president stood up, __________ a friendly smile and began to make a speech.A．to give B．giving C．gave D．given10. There are cases __________ speech has started late in a child who eventually turns out to be of high IQ.A．why B．which C．where D．as Directions: After reading the passage below, fill in the blanks to make the passage coherent and grammatically correct. For the blanks with a given word, fill in each blank with the proper form of the given word; for the other blanks, use one word that best fits each blank.Number of Steps a Day It Takes to Cut Risk of Early DeathNew research suggests exactly how many steps you need to take each day to reduce the risk of heart disease and early death. If you 11 (fail) in your pursuit of walking 10,000 steps a day —researchers have some good news for you.They found walking up to 10,000 steps a day reduces these risks. The lowest risk of early death was 12 people who took 9,000 to 10,500 steps a day. When it came to avoiding heart attack, people 13 (manage) around 9,700 steps a day had the lowest risks.Experts have previously found people who spend a lot of time sitting while awake are more likely 14 (suffer) an early death and develop heart disease. It has been unclear 15 walking can balance out the effects of sitting down for most of the day — until now.A study, published in the British Journal of Sports Medicine, analysed data from 72,174 people 16 (age) around 61 from UK. Participants wore a device for seven days to measure their exercise levels. After a seven-year follow-up, 1,633 deaths and 6,190 cardiovascular (心脏血管的) events, such as heart attack, were recorded. The results showed any amount of daily steps above 2,200 a day were linked to lower death and heart disease — 17 the rest of the day was spent being inactive.Julie Ward, a senior nurse in the U.K., said, “We encourage people to stay active for 18 heart and circulatory health by doing 150 minutes of moderate exercise a week. “This can be any activity 19 fits into your lifestyle, such as taking regular walking breaks away from your computer screen, going to the gym, enjoying exercise classes, or even getting off the bus one stop 20 (early) to get more steps in.”Directions: Fill in each blank with a proper word chosen from the box. Each word can only be used once. Note that there is one word more than you need.A. topicB. developedC. arguablyD. increasinglyE. repeatedF. eventsG. shares H. individual I. influenced J. appeals K. literaryPoetry is a kind of writing in which the sound and meaning of groups of words express ideas or emotion in addition to the experiences or strong feelings the writer 21 . Unlike most other forms of writing, poetry is often written in lines, rather than paragraphs. Poetry also sounds different from other forms of writing, often using rhythm and rhyme to create an interesting sound when read aloud. Poetry catches the attention of a reader because it 22 to both emotions and senses.Sound is 23 the single most important aspect of any poem. The sound that any given word makes, or the sounds that come from specific groups of words used together, are what make poetry so unique as a form of writing. A typical story or report does not focus on the sounds that each 24 word makes when read. But poems generally contain few words, so it is important that each word plays a role in making an impact on the reader. Rhythm is the flow of sounds created by successive words in a poem. When you read a poem you can often hear this 25 pattern, or “beat” in the sounds. This is called meter.Some of the oldest and best-known poetry in the world came from Ancient Greece. As far back as 700 BCE, poets there recited their work at public 26 and religious ceremonies. The great epic poems The Iliad and The Odyssey by Homer came from Greece. The Greeks eventually 27 Roman poets, such as Virgil, who wrote the Aeneid 30 BCE. In medieval times, poems such as Beowulf, The Divine Comedy by Dante. and The Canterbury Tales by Chaucer were written. Religion and romance became the 28 of choice for many poets at that time.Poetry 29 even more during the Renaissance period of history, an era of many great cultural achievements. This was the period during which Shakespeare, the most well-known poet, was making his mark! Needless to say, a trend had started. Poetry has continued to grow and change as a form of 30 expression in modern times.Once upon a time there lived in Germany two brothers who loved a good story — one with magic and danger, royalty and villains (恶棍). At school they met a wise man who led them to a treasure — a library of old books with tales more fascinating than any they had ever heard. _________, the brothers began collecting their own stories, listening to the folktales people told them. Soon they_________ their own treasure — a book of fairy tales that would charm millions in faraway lands for generations to come.The brothers Grimm, Jacob and Wilhelm, named their story collection Children’s and Household Tales and published it in Germany in 1812. The collection which has been translated into more than 160 languages up to now is a publishing _________. The stories and their characters have appeared in theatre, opera, comic books, movies, paintings, rock music, advertising and even fashion.Such _________ would have shocked the modest Grimms. During their lifetimes the book sold few copies in Germany. The early editions were not even _________ children. They had no illustrations, and scholarly footnotes took up almost as much space as the tales themselves. Jacob and Wilhelm Grimm viewed themselves as _________ students of folklore. They began their work at a time when Germany had been occupied by the French under Napoleon. As young scholars, the brothers Grimm began to work on the fairy tale collection in order to save the endangered oral storytelling tradition of Germany.Long before the Grimms’ time, _________ developed in inns, barns, and peasant homes. During winter nights, as they sat spinning wood, women kept each other company and entertained each other with tales of adventure, romance and magic. _________, 40 such storytellers delivered tales to the Grimms, many of them coming to their house in Kassel. Although the brothers implied that they were just _________ the tales, Wilhelm polished and reshaped the stories up to the final edition of 1857. In an effort to make them more __________ to children and their parents, he stressed the moral of each tale and emphasized gender roles. According to the Grimms, the collection served as “a manual of __________.” To this day, parents read them to their children be cause they approve of the lessons in the stories: keep your promises, don’t talk to strangers, work hard, obey your parents.So what __________ their popularity? Some have suggested that it is because the characters are always striving for happiness. But the truth probably lies in their __________. The Grimms’ tales were born out of a storytelling tradition without __________ of age or culture. The brothers’ skill was to translate these into a universal style of writing that seems to mirror whatever moods or interests we bring to our __________ of them. And so it was that the Grimms’ fairy tales lived happily ever after.31.A．Inspired B．Disappointed C．Discouraged D．Relieved32.A．estimated B．produced C．sacrificed D．stocked33.A．medium B．partnership C．finding D．wonder34.A．quality B．wealth C．fame D．benefit35.A．marked as B．robbed of C．aimed at D．prevented from 36.A．intelligent B．hardworking C．peculiar D．patriotic37.A．collection B．storytelling C．entertainment D．meeting38.A．Besides B．Altogether C．However D．Similarly39.A．creating B．developing C．reviewing D．recording40.A．accustomed B．acceptable C．cruel D．compared41.A．manners B．parentship C．publishing D．adaptation42.A．results from B．depends on C．accounts for D．responds to43.A．appeal B．flexibility C．availability D．origin44.A．boundaries B．influences C．indications D．distributions 45.A．writing B．sharing C．reading D．beginning Charles Robert Darwin was born on 12 February 1809 in Shropshire, England. Darwin’s childhood passion was science, and his interest in chemistry, however, was clear; he was even nicknamed‘Gas’ by his classmates.In 1825, his father sent him to study medicine at Edinburgh University, where he learned how to classify plants. Darwin became passionate about natural history and this became his focus while he studied at Cambridge. Darwin went on a voyage together with Robert Fitzroy, the captain of HMS Beagle, to South America to facilitate British trade in Patagonia. The journey was life-changing. Darwin spent much of the trip on land collecting samples of plants, animals and rocks, which helpedhim to develop an understanding of the processes that shape the Earth’s surface. Darwin’s analysis of the plants and animals that he gathered led him to express doubts on former explanations about how species formed and evolved over time.Darwin’s work convinced him that natural selection was key to understanding the development of the natural world. The theory of natural selection says that individuals of a species are more likely to survive when they inherit(经遗传获得) characteristics best suited for that specific environment. These features then become more widespread and can lead eventually to the development of a new species. With natural selection, Darwin argued how a wide variety of life forms developed over time from a single common ancestor.Darwin married his cousin, Emma Wedgwood, in 1839. When Darwin’s eldest daughter, Annie, died from a sudden illness in 1851, he lost his belief in God. His tenth and final child, Charles Waring Darwin, was born in 1856. Significantly for Darwin, this baby was disabled, altering how Darwin thought about the human species. Darwin had previously thought that species remained adapted until the environment changed; he now believed that every new variation was imperfect and that a struggle to survive was what drove species to adapt.Though rejected at the beginning, Darwin’s theory of evolution by natural selection is nowadays well accepted by the scientific community as the best evidence-based explanation for the diversity and complexity of life on Earth. The Natural History Museum’s library alone has 478 editions of his On the Origin of Species in 38 languages.46. What made Darwin reconsider the origin and development of species?A．Examining plants and animals collected.B．His desire for a voyage to different continents.C．Classifying samples in a journey to South America.D．His passion for natural history at Edinburgh University.47. We can learn from paragraphs 1 to 3 that Darwin ________.A．used natural selection to develop new speciesB．enjoyed being called nicknames related to scienceC．learned some knowledge about plants when studying medicineD．argued with others over the diversity of life forms for a long period48. Which of the following changed Darwin’s view on the human species?A．That he had ten children in all. B．His youngest son’s being disabled.C．That he lost his eldest daughter. D．His marriage with Emma Wedgwood.49. This passage is mainly about ________.A．Darwin’s passion for medical science B．Darwin’s theory and experimentsC．Charles Darwin’s changing interest D．Charles Darwin’s life and workA．adventure lovers B．foreign travellers C．creativeteenagers D．professional sportsmen51. Catherine would like to develop her confidence in a safe and positive atmosphere, so she can call ________ for more information.A．(800) 2223-3595 B．(888) 4209-7855 C．(886) 6817-2173 D．(212) 6743-4300 52. Lucy’s dream is to be a film actress, so she’d probably go to ________ to attend a camp.A．Colorado B．Oregon C．Texas D．New York“Choose your friends wisely” may not only be good parental advice but also a way to d o better in college, a research study finds.The group of three researchers put that advice to the test at Berea College, a small liberal arts school in Kentucky, by looking at how much friends actually influence study habits and grades. They found that students who befriended studious (勤奋的) peers spent more hours studying themselves and posted higher grades during their freshman year.“It’s no fun to study by yourself,” said Nirav Mehta, one of the study’s authors, explaining the intuition behind the study. “If you want to goof off, and your friends are at the library, then you’re going to go to the library, too. And while you’re there, you’re probably going to get some studying done too.”Of course, it’s possible that studious people gravitate toward other studious people. They might have hit the books and got as many A’s no matter who their friends were. So the researchers checked to see if randomly assigned roommates also have a positive influence on study habits and grades. They found almost the same results: students who were assigned a studious roommate freshman year also studied more each day and had higher grade-point averages.Unfortunately, the opposite is also true, the researchers found. If you have friends and roommates who don’t study a lot, you’re likely to get dragged down by their poor habits, studying less and earning lower grades.Analyzing friends and study habits is usually difficult for researchers. But students at Berea College were asked to list their four best friends at the end of each semester and they kept careful daily logs of their time, including time spent studying. At the beginning of freshman year, the students were surveyed on their high school study habits. The researchers also had access to roommate assignments, high school grades and college grades.From this information, the economists calculated the average amount of time each student’s college friends had reported studying in high school. They found that for every additional 10 hours a week that a student’s friends had spent studying, on average, the student’s own study time in college would likely increase by almost 25 minutes a day, and the student’s own GPA would likely rise by almost a tenth of a point during freshman year.53. The phrase “goof off” (paragraph 3) most probably means ________.A．achieve higher grades B．choose your friendsC．go to the library D．be lazy about studying54. Why did the researchers also study the randomly assigned roommates?A．To further test the theory. B．To figure out more study habits.C．To put forward a new theory. D．To get more students to work hard.55. To carry out their research, what information did the researchers collect from students at Berea College?A．How many studious friends they have.B．How they comment on their friends’ grades.C．How much time they spent studying each day.D．How they thought of their own college grades.56. What suggestion would the researchers most likely give college students?A．If you want to do well in study, you’d better pick a hardworking friend.B．If you want to get on well with your roommates, you’d better work hard.C．If you want to raise your GPA, you’d better keep track of your study time.D．If you want to have a happy freshman year, you’d better care less about peer effects.A Recipe for Avoiding DisasterEvery log cabin homeowner has had those “what if” thoughts. What if conditions become so dry that a wildfire starts near my cabin? What if the rain is so relentless that the lake outside my doorstep begins to overflow its banks? What if tornado-fueled winds threaten to destroy everything I’ve created?57 . That’s the bad news. The good news? There are ways you can use your landscaping to minimize the damage that extreme weather can cause and still have an attractive yard all at the same time. Here are a few tips for using your outdoor resources in a way that can lessen the impact of a natural disaster on your log home:WILDFIRES: The key to keeping fires from damaging your home is regular maintenance with a focus on how fires spread. Begin by removing dead plants like trees and shrubs promptly and trimming (修剪) any branches that overhang the property or have close contact with the log walls.58 . It’s also a good idea to install irrigation in a 50-foot radius around your home to help create a fireproofing perimeter.FLOODS: A massive amount of flooding that leaves your house and yard in standing water is a tall order in terms of prevention strategies, but more moderate flooding can be stopped with a few simple landscaping tricks. 59 . You can create extra drainage (排水系统) through the use of strategically placed stones and bushes that direct water away from the foundation.TORNADOES: To minimize the damage caused by the sudden gusts tornado weather brings, be sure to keep trees and shrubs trimmed. 60 .61. 栖息地 ________62. 非凡的；显著的 ________63. 精致的；精细的 ________64. 恐龙 ________65. 简图；图解 ________66. 计算；核算 ________汉译英67. 整容手术 ________68. 全新的；崭新的 ________69. 正在运行；正在操作 ________70. 在校园内 ________71. 试穿 ________72. 他总是对自己的工作充满了热情。

Calibrated Manifolds and Gauge Theory

a rX iv:mat h /42368v9[mat h.GT]19A ug27CALIBRATED MANIFOLDS AND GAUGE THEORY SELMAN AKBULUT AND SEMA SALUR Abstract.By a theorem of Mclean,the deformation space of an associative submanifold Y of an integrable G 2-manifold (M,ϕ)can be identiﬁed with the kernel of a Dirac operator D /:Ω0(ν)→Ω0(ν)on the normal bundle νof Y .Here,we generalize this to the non-integrable case,and also show that the defor-mation space becomes smooth after perturbing it by natural parameters,which corresponds to moving Y through ‘pseudo-associative’submanifolds.Inﬁnitesi-mally,this corresponds to twisting the Dirac operator D /→D /A with connections A of ν.Furthermore,the normal bundles of the associative submanifolds with Spin c structure have natural complex structures,which helps us to relate their deformations to Seiberg-Witten type equations.If we consider G 2manifolds with 2-plane ﬁelds (M,ϕ,Λ)(they always exist)we can split the tangent space T M as a direct sum of an associative 3-plane bun-dle and a complex 4-plane bundle.This allows us to deﬁne (almost)Λ-associative submanifolds of M ,whose deformation equations,when perturbed,reduce to Seiberg-Witten equations,hence we can assign local invariants to these ing this we can assign an invariant to (M,ϕ,Λ).These Seiberg-Witten equations on the submanifolds are restrictions of global equations on M .We also discuss similar results for the Cayley submanifolds of a Spin (7)manifold.0.Introduction We ﬁrst study deformations of associative submanifolds Y 3of a G 2manifold (M 7,ϕ),where ϕ∈Ω3(M )is the G 2structure.We prove a generalized version of the McLean’s theorem where integrability condition of the underlying G 2structure is not necessary.This deformation space might be singular,but by perturbing it with some natural parameters it can be made smooth.This amounts to deforming Y through the associatives in (M,ϕ)with varying ϕ,or alternatively deforming Y throughthe pseudo-associative submanifolds (Y ’s whose tangent planes become associative after rotating by a generic element of the gauge group of T M ).Inﬁnitesimally,these perturbed deformations correspond to the kernel of the twisted Dirac operator D /A :Ω0(ν)→Ω0(ν),twisted by some connection A in ν(Y ).2SELMAN AKBULUT AND SEMA SALURThe associative submanifolds with Spin c structures in(M,ϕ)are useful objects to study,because their normal bundles have natural complex structures.Also we can view(M,ϕ)as an analog of a symplectic manifold,and view a non-vanishing 2-planeﬁeldΛon M as an analog of a complex structure tamingϕ.Note that 2-planeﬁelds are stronger versions of Spin c structures on M7,and they always exist by[T].The data(M7,ϕ,Λ)determines an interesting splitting of the tangent bundle T M=E⊕V,where E is the bundle of associative3-planes,and V is the complementary4-plane bundle with a complex structure,which is a spinor bundle of E.Then the integral submanifolds Y3of E,which we callΛ-associative submanifolds,can be viewed as analogues of J-holomorphic curves;because their normal bundles come with an almost complex structure.Even if they may not always exist,their perturbed versions,i.e.almostΛ-associative submanifolds,always do. AlmostΛ-associative submanifolds are the transverse sections of the bundle V→M. We can deform such Y by using the connections in the determinant line bundle of ν(Y)and get a smooth deformation space,which is described by the twisted Dirac equation.Then by constraining this new variable with another natural equation we arrive to Seiberg-Witten type equations for Y.So we can assign an integer to Y, which is invariant under small isotopies through almostΛ-assocative submanifolds. In fact it turns out that(M7,ϕ,Λ)gives aﬁner splitting T M=¯E⊕ξ,where¯E is a6-plane bundle with a complex structure,andξis a real line bundle.In a way this structure of(M,ϕ)mimics the structure of(Calabi-Yau)×S1manifolds,and by‘rotating’ξinside of T M we get a new insight for so-called“Mirror manifolds”which is investigated in[AS1].There is a similar process for the deformations of Cayley submanifolds X4⊂N8of a Spin(7)manifold(N8,Ψ),which we discuss at the end.So in a wayΛ-associative(or Cayley)manifolds in a G2(or Spin(7))manifold,behave much like higher dimensional analogue of holomorphic curves in a Calabi-Yau manifold.We would like to thank MSRI,IAS,Princeton and Harvard Universities for pro-viding a stimulating environment where this paper is written,and we thank R.Kirby and G.Tian for continuous encouragement.Theﬁrst named author thanks to R. Bryant and C.Taubes for stimulating discussions and useful suggestions.31.PreliminariesHere weﬁrst review basic properties of the manifolds with special holonomy(most material can be found in[B2],[B3],[H],[HL]),and then proceed to prove some new results.Recall that the set of octonions O=H⊕l H=R8is an8-dimensional division algebra generated by<1,i,j,k,l,li,lj,lk>.On the set of the imaginary octonions im O=R7we have the cross product operation×:R7×R7→R7,deﬁned by u×v=im(¯v.u).The exceptional Lie group G2can be deﬁned as the linear automorphisms of im O preserving this cross product operation,G2=Aut(R7,×). There is also another useful description in terms of the orthogonal3-frames in R7: (1)G2={(u1,u2,u3)∈(im O)3|<u i,u j>=δij,<u1×u2,u3>=0} Alternatively,G2can be deﬁned as the subgroup of the linear group GL(7,R) whichﬁxes a particular3-formϕ0∈Ω3(R7).Denote e ijk=dx i∧dx j∧dx k∈Ω3(R7), thenG2={A∈GL(7,R)|A∗ϕ0=ϕ0}(2)ϕ0=e123+e145+e167+e246−e257−e347−e356Deﬁnition1.A smooth7-manifold M7has a G2structure if its tangent frame bundle reduces to a G2bundle.Equivalently,M7has a G2structure if there is a 3-formϕ∈Ω3(M)such that at each x∈M the pair(T x(M),ϕ(x))is isomorphic to(T0(R7),ϕ0).Here are some useful properties,discussed more fully in[B2]:Any G2structure ϕon M7gives an orientationµ∈Ω7(M)on M,and thisµdetermines a metric g= , on M,and a cross product structure×on its tangent bundle of M as follows:Let i v denote the interior product with a vector v then(3) u,v =[i u(ϕ)∧i v(ϕ)∧ϕ]/6µ(4)ϕ(u,v,w)= u×v,wTo emphasize the dependency onϕsometimes g is denoted by gϕ.In particular,the 14-dimensional Lie group G2imbeds into SO(7)subgroup of GL(7,R).Note that because of the way we deﬁned G2=Gϕ02,this imbedding is determined byϕ0. Since GL(7,R)acts onΛ3(R7)with stabilizer G2,its orbitΛ3+(R7)is open for dimension reasons,so the choice ofϕ0in the above deﬁnition is generic(in fact it has two orbits containing±ϕ0).G2has many copies Gϕ2inside GL(7,R),which are all conjugate to each other,since G2has only one7dimensional representation.Hence the space of G2structures on M7are identiﬁed with the sections of the bundle: (5)RP7≃GL(7,R)/G2→Λ3+(M)−→M4SELMAN AKBULUT AND SEMA SALURwhich are called the positive3-forms,these are the set of3-formsΩ3+(M)that can be identiﬁed pointwise byϕ0.Each Gϕ2imbeds into a conjugate of one standard copy SO(7)⊂GL(7,R).The space of G2structuresϕon M,which induce the same metric on M,that is allϕ’s for which the corresponding Gϕ2lies in the standard SO(7),are the sections of the bundle(whoseﬁber is the orbit ofϕ0under SO(7)): (6)RP7=SO(7)/G2→˜Λ3+(M)−→Mwhich we will denote by˜Ω3+(M).The set of smooth7-manifolds with G2-structures coincides with the set of7-manifolds with spin structure,though this correspondence is not1−1.This is because Spin(7)acts on S7with stabilizer G2inducing the ﬁbrationsG2→Spin(7)→S7→BG2→BSpin(7)and so there is no obstruction to lifting maps M7→BSpin(7)to BG2,and there are many liftings.Cotangent frame bundle P∗(M)→M of a manifold with G2 structure(M,ϕ)can be expressed as P∗(M)=∪x∈M P∗x(M),where eachﬁber is: P∗x(M)={u∈Hom(T x(M),R7)|u∗(ϕ0)=ϕ(x)}Throughout this paper we will denote the cotangent frame bundle by P∗(M)→M and its adapted frame bundle by P(M).They can be G2or SO(7)frame bundles; to emphasize it sometimes we will specify them by the notations P SO(7)(M)or P G2(M).Also we will denote the sections of a bundleξ→Y byΩ0(Y,ξ)or simply byΩ0(ξ),and the bundle valued p-forms byΩp(ξ)=Ω0(Λp T∗Y⊗ξ),and the sphere bundle ofξby S(ξ).There is a notion of a G2structureϕon M7being integrable, which corresponds toϕbeing an harmonic form:Deﬁnition2.A manifold with G2structure(M,ϕ)is called a G2manifold if the holonomy group of the Levi-Civita connection(of the metric gϕ)lies inside of G2. Equivalently(M,ϕ)is a G2manifold ifϕis parallel with respect to the metric gϕi.e.∇gϕ(ϕ)=0;this condition is equivalent to dϕ=0=d(∗gϕϕ).In short one can deﬁne a G2manifold to be any Riemannian manifold(M7,g) whose holonomy group is contained in G2,thenϕand the cross product×come as a consequence.It turns out that the conditionϕbeing harmonic is equivalent to the condition that at each point x0∈M there is a chart(U,x0)→(R7,0)on which ϕequals toϕ0up to second order term,i.e.on the image of U(7)ϕ(x)=ϕ0+O(|x|2)Remark1.For example if(X6,ω,Ω)is a complex3-dimensional Calabi-Yau man-ifold with K¨a hler formω,and a nowhere vanishing holomorphic3-formΩ,then X×S1has holonomy group SU(3)⊂G2,hence is a G2manifold.In this case (8)ϕ=ReΩ+ω∧dt.5 Deﬁnition 3.Let(M,ϕ)be a manifold with a G2structure.A4-dimensional submanifold X⊂M is called an co-associative ifϕ|X=0.A3-dimensional submanifold Y⊂M is called an associative ifϕ|Y≡vol(Y);this condition is equivalent toχ|Y≡0,whereχ∈Ω3(M,T M)is the tangent bundle valued3-form deﬁned by the identity:(9) χ(u,v,w),z =∗ϕ(u,v,w,z)The equivalence of these conditions follows from the‘associator equality’of[HL] (10)ϕ(u,v,w)2+|χ(u,v,w)|2/4=|u∧v∧w|2In general,if{e1,e2,..,e7}is any orthonormal coframe on(M,ϕ),then the expres-sion(2)forϕhold on a chart.By calculation∗ϕ,and using(9)we can calculate the expression ofχ(note the error in the the second term of6th line of the corresponding formula(5.4)of[M]):(11)∗ϕ=e4567+e2367+e2345+e1357−e1346−e1256−e1247χ=(e256+e247+e346−e357)e1+(−e156−e147−e345−e367)e2+(e245+e267−e146+e157)e3+(−e567+e127+e136−e235)e4+(e126+e467−e137+e234)e5+(−e457−e125−e134−e237)e6+(e135−e124+e456+e236)e7Alsoχcan be expressed in terms of cross product operation(c.f.[H],[HL],[K]): (12)χ(u,v,w)=−u×(v×w)− u,v w+ u,w vWhen dϕ=0,the associative submanifolds are volume minimizing submanifolds of M(calibrated byϕ).Even in the general case of a manifold with a G2structure (M,ϕ),the formχimposes an interesting structure near associative submanifolds: Notice(9)implies that,χmaps every oriented3-plane in T x(M)to the orthogonal subspace T x(M)⊥,so if we choose local coordinates(x1,...,x7)for M7we get (13)χ= aαJ dx J⊗∂6SELMAN AKBULUT AND SEMA SALURFrom(9)it is easy to calculate aαijk=∗ϕijks g sα,where g−1=(g ij)is the inverse of the metric g=(g ij),and of course the metric g can be expressed in terms ofϕ.By evaluatingχon the orientation form of Y we get a normal vectorﬁeld so: Lemma1.To any3-dimensional submanifold Y3⊂(M,ϕ),χassociates a normal vectorﬁeld,which vanishes when Y is associative.Henceχdeﬁnes an interestingﬂow on3dimensional submanifolds of(M,ϕ),ﬁxing associative submanifolds.On the associative submanifolds with a Spin c structure,χrotates their normal bundles and imposes a complex structure on them: Lemma2.To any associative manifold Y3⊂(M,ϕ)with a non-vanishing oriented 2-planeﬁeld,χdeﬁnes an almost complex structure on its normal bundleν(Y) (notice that in particular any coassociative submanifold X⊂M has an almost complex structure if its normal bundle has a non-vanishing section).Proof.Let L⊂R7be an associative3-plane,that isϕ|L=vol(L).Then to every pair of orthonormal vectors{u,v}⊂L,the formχdeﬁnes a complex structure on the orthogonal4-plane L⊥,as follows:Deﬁne j:L⊥→L⊥by(15)j(X)=χ(u,v,X)This is well deﬁned i.e.j(X)∈L⊥,because when w∈L we have:<χ(u,v,X),w>=∗ϕ(u,v,X,w)=−∗ϕ(u,v,w,X)=<χ(u,v,w),X>=0Also j2(X)=j(χ(u,v,X))=χ(u,v,χ(u,v,X))=−X.We can check the last equality by taking an orthonormal basis{X j}⊂L⊥and calculating<χ(u,v,χ(u,v,X i)),X j>=∗ϕ(u,v,χ(u,v,X i),X j)=−∗ϕ(u,v,X j,χ(u,v,X i))=−<χ(u,v,X j),χ(u,v,X i)>=−δijThe last equality holds since the map j is orthogonal,and the orthogonality can be seen by polarizing the associator equality(10),and by noticingϕ(u,v,X i)=0. Observe that the map j only depends on the oriented2-plane l=<u,v>generated by{u,v}.So the result follows.In fact,for any unit vectorﬁeldξon an associative Y(i.e.a Spin c structure) deﬁnes a complex structure Jξ:ν(Y)→ν(Y)by Jξ(z)=z×ξ,and the complex structure deﬁned in Lemma2corresponds to J u×v,because from(12):χ(u,v,z)=χ(z,u,v)=−z×(u×v)− z,u v+ z,v u=J v×u(z).Also recall that the complex structures on any SO(4)bundle such asν→Y are given by the unit sections of the associated SO(3)bundleλ+(ν)→Y,which is induced by the left reductions SO(4)=(SU(2)×SU(2))/Z2→SU(2)/Z2=SO(3).7 Deﬁnition4.A Riemannian8-manifold(N8,g)is called a Spin(7)manifold if the holonomy group of its Levi-Civita connection lies in Spin(7)⊂GL(8,R). Equivalently a Spin(7)manifold(N,Ψ)is a Riemannian8-manifold with a triple cross product×on its tangent bundle,and a harmonic4-formΨ∈Ω4(N)withΨ(u,v,w,z)=g(u×v×w,z)It is easily checked that if(M,ϕ)is a G2manifold,then(M×S1,Ψ)is a Spin(7) manifold whereΨ=ϕ∧dt−∗ϕ.Deﬁnition 5.A4-dimensional submanifold X of a Spin(7)manifold(N,Ψ)is called Cayley ifΨ|X≡vol(X).This is equivalent toτ|X≡0whereτ∈Ω4(N,E) is a certain vector-bundle valued4-form deﬁned by the“four-fold cross product”of the imaginary octonionsτ(v1,v2,v3,v4)=v1×v2×v3×v4(see[M],[HL]).2.Grassmann BundlesLet G(3,7)be the Grassmann manifold of oriented3-planes in R7.Let M7be an oriented smooth7-manifold,and let˜M→M be the bundle oriented3-planes in T M,which is deﬁned by the identiﬁcation[p,L]=[pg,g−1L]∈˜M:(16)˜M=P SO(7)(M)×SO(7)G(3,7)→M.This is just the bundle˜M=P SO(7)(M)/SO(3)×SO(4)→P SO(7)(M)/SO(7)=M. Letξ→G(3,7)be the universal R3bundle,andν=ξ⊥→G(3,7)be the dual R4 bundle.Therefore,Hom(ξ,ν)=ξ∗⊗ν−→G(3,7)is the tangent bundle T G(3,7).ξ,νextendﬁberwise to give bundlesΞ→˜M,V→˜M respectively,and letΞ∗be the dual ofΞ.Notice that Hom(Ξ,V)=Ξ∗⊗V→˜M is the bundle of vertical vectors T v(˜M)of T(˜M)→M,i.e.the tangents to theﬁbers ofπ:˜M→M,hence (17)T˜M∼=T v(˜M)⊕π∗T M=(Ξ∗⊗V)⊕Ξ⊕V.That is,T˜M is the vector bundle associated to principal SO(3)×SO(4)bundle P SO(7)→˜M by the obvious representation of SO(3)×SO(4)to(R3)∗⊗R4+R3+R4. The identiﬁcation(17)is deﬁned up to gauge automorphisms of bundlesΞand V. Note that the bundle V=Ξ⊥depends on the metric,and hence it depends onϕwhen metric is induced from a G2structure(M,ϕ).To emphasize this fact we can denote it by Vϕ→˜M.But when we are considering G2structures coming from G2 subgroups of aﬁxed copy of SO(7)⊂GL(7,R),they induce the same metric and so this distinction is not necessary.8SELMAN AKBULUT AND SEMA SALURLet P(V)→˜M be the SO(4)frame bundle of the vector bundle V,identify R4 with the quaternions H,and identify SU(2)with the unit quaternions Sp(1)=S3. Recall that SO(4)is the equivalence classes of pairs[q,λ]of unit quaternionsSO(4)=(SU(2)×SU(2))/Z2Hence V→˜M is the associated vector bundle to P(V)via the SO(4)representation (18)x→qxλ−1There is a pair of R3=im(H)bundles over˜M corresponding to the left and right SO(3)reductions of SO(4),which are given by the SO(3)representations(19)λ+(V):x→qx q−1λ−(V):y→λyλ−1The map x⊗y→xy gives actionsλ+(V)⊗V→V and V⊗λ−(V)→V;by combining we can think of them as one conjugation action(20)(λ+(V)⊗λ−(V))⊗V→VIf the SO(4)bundle P(V)→˜M lifts to a Spin(4)=SU(2)×SU(2)bundle (locally it does),we get two additional bundles over˜M(21)S:y→qy E:y→yλ−1They identify V as a tensor product of two quaternionic line bundles V=S⊗H E.In particular,λ+(V)=ad(S)andλ−(V)=ad(E),i.e.they are the SO(3)reductions of the SU(2)bundles S and E.Also there is a multiplication map S⊗E→V.Recall the identiﬁcations:Λ2(V)=Λ2+(V)⊕Λ2−(V)=λ−(V)⊕λ+(V)=λ(V)=gl(V)=ad(V).2.1.Associative Grassmann Bundles.Now consider the Grassmannian of associative3-planes Gϕ(3,7)in R7,con-sisting of elements L∈G(3,7)with the propertyϕ0|L=vol(L)(or equivalently χ0|L=0).G2acts on Gϕ(3,7)transitively with the stabilizer SO(4),so it gives the identiﬁcation Gϕ(3,7)=G2/SO(4).If we identify the imaginary octonions by R7=Im(O)∼=im(H)⊕H,then the action of the subgroup SO(4)⊂G2on R7is (22) ρ(A)00Awhereρ:SO(4)=(SU(2)×SU(2))/Z2→SO(3)is the projection of theﬁrst factor ([HL]),that is for[q,λ]∈SO(4)the action is given by(x,y)→(qxq−1,qyλ−1).So the action of SO(4)on the3-plane L=im(H)is determined by its action on L⊥. Now let M7be a G2manifold.Similar to the construction before,we can construct the bundle of associative Grassmannians over M(which is a submanifold of˜M):(23)˜Mϕ=P G2(M)×G2Gϕ(3,7)→M9which is just the quotient bundle˜Mϕ=P G2(M)/SO(4)−→P G2(M)/G2=M.Asin the previous section,the restriction of the universal bundlesξ,ν=ξ⊥→Gϕ(3,7) induce3and4plane bundlesΞ→˜Mϕand V→˜Mϕ(by restricting from˜M).Also (24)T˜Mϕ∼=T v(˜Mϕ)⊕Ξ⊕VFrom(22)we see that in the associative case,we have an important identiﬁcation:Ξ=λ+(V)(as bundles over˜Mϕ),and the dual of the actionλ+(V)⊗V→V givesa Cliﬀord multiplication:(25)Ξ∗⊗V→VIn fact this is just the map induced from the cross product operation[AS2].Recall that T v(˜M)=Ξ∗⊗V→˜M is the subbundle of vertical vectors of T(˜M)→M. The total space E(νϕ)of the normal bundle of the imbedding˜Mϕ⊂˜M should be thought of an open tubular neighborhood of˜Mϕin˜M,and it has a nice description: Lemma3.([M])Normal bundleνϕof˜Mϕ⊂˜M is isomorphic to V,and the bundle of vertical vectors T v(˜Mϕ)is the kernel of the Cliﬀord multiplication c:Ξ∗⊗V→V. We have T v(˜M)|˜Mϕ=T v(˜Mϕ)⊕νϕ,and the following exact sequence over˜MϕT v(˜Mϕ)→Ξ∗⊗V|˜Mϕc−→V|˜Mϕ→0Hence the quotient bundle,T v(˜M)/T v(˜Mϕ)is isomorphic to V.Proof.This is because the Lie algebra inclusion g2⊂so(7)is given byaβ−βtρ(a)where a∈so(4)is y→qy−yλ,andρ(a)∈so(3)is x→qx−xq.So the tangent space inclusion of G2/SO(4)⊂SO(7)/SO(4)×SO(3)is given by the matrix β∈(im H)∗⊗H.Therefore,if we writeβas column vectors of three queternions β=(β1,β2,β3)=i∗⊗β1+j∗⊗β2+k∗⊗β3,thenβ1i+β2j+β3k=0([M],[Mc]). The reader can consult Lemma5of[AS2]for a more self contained proof of this fact,where the Cliﬀord multiplication is identiﬁed with the cross product operation.3.Associative SubmanifoldsAny imbedding of a3-manifold f:Y3֒→M7induces an imbedding˜f:Y֒→˜M:(26)˜M⊃˜Mϕ˜fր↓Y f−→M10SELMAN AKBULUT AND SEMA SALURand the pull-backs˜f∗Ξ=T(Y)and˜f∗V=ν(Y)give the tangent and normal bundles of Y.Furthermore,if f is an imbedding of an associative submanifold into a G2manifold(M,ϕ),then the image of˜f lands in˜Mϕ.We will denote this canonical lifting of any3-manifold Y⊂M by˜Y⊂˜M.Also since we have the dependency V=Vϕ,we can denoteν(Y)=ν(Y)ϕ=νϕwhen needed.˜Mϕcan be thought of as a universal space parameterizing associative submani-folds of M.In particular,if˜f:Y֒→˜Mϕis the lifting of an associative submanifold, by pulling back we see that the principal SO(4)bundle P(V)→˜Mϕinduces an SO(4)-bundle P(Y)→Y,and gives the following vector bundles via the represen-tations:(27)ν(Y):y→qyλ−1 T(Y):x→qx q−1where[q,λ]∈SO(4),ν=ν(Y)and T(Y)=λ+(ν).Also we can identify T∗Y with T Y by the induced metric.From above we have the action T∗Y⊗ν→νinducing actionsΛ∗(T∗Y)⊗ν→ν.Let L=Λ3(Ξ)→˜M be the determinant(real)line bundle.Recall that the deﬁnition(9)implies thatχmaps every oriented3-plane in T x(M)to its comple-mentary subspace,soχgives a bundle map L→V over˜M,which is a section of L∗⊗V→˜M.SinceΞis oriented L is trivial,soχactually gives a section(28)χ=χϕ∈Ω0(˜M,V)Clearly˜Mϕ⊂˜M is the codimension4submanifold which is the zeros of thissection.Associative submanifolds Y⊂M are characterized by the conditionχ|˜Y =0,where˜Y⊂˜M is the canonical lifting of Y.Similarlyϕdeﬁnes a mapϕ:˜M→R.3.1.Pseudo-associative submanifolds.Here we generalize associative submanifolds to a moreﬂexible class of submani-folds.To do this weﬁrst generalize the notion of imbedded submanifolds.Deﬁnition 6.A Grassmann-framed3-manifold in(M,ϕ)is a triple(Y3,f,F), where f:Y֒→M is an imbedding,F:Y→˜M,such that the following commute(29)˜M Fր↓Y f−→MWe call(Y,f,F)a pseudo-associative submanifold if in addition Image(F)⊂˜Mϕ. So a pseudo-associative submanifold(Y,f,F)with F=˜f is associative.11 Remark2.The bundle˜M→M always admits a section,in fact the subbundle ˜Mϕ→M has a section.This is because by[T]every orientable7-manifold admits a non-vanishing linearly independent2-frameﬁeldΛ={v1,v2}1.By Grahm-Schmidt process with metric gϕ,we can assume thatΛis orthonormal.The cross product assignsΛto an orthonormal3-frameﬁeld{v1,v2,v1×ϕv2}on M,then3-plane gen-erated by{v1,v2,v1×ϕv2}:=<v1,v2,v1×ϕv2>gives a section ofλϕ:M→˜Mϕ.LetFigure1.Z(M)and Zϕ(M)denote the set of Grassmann-framed and the pseudo-associative submanifolds,respectively,and let Aϕ(M)be the set of associative submanifolds. We have inclusions Aϕ(M)֒→Zϕ(M)֒→Z(M),where theﬁrst map is given by (Y,f)→(Y,f,˜f).So there is an inclusion Im(Y,M)֒→Z(M),where Im(Y,M)is the space of imbeddings.This inclusion can be thought of the canonical sections ofa bundle(30)Z(Y)π−→Im(Y,M)withﬁbersπ−1(f)=Ω0(Y,f∗˜M).We also have the subbundle Zϕ(Y)π−→Im(Y,M) withﬁbersπ−1(f)=Ω0(Y,f∗˜Mϕ).So Z(Y)is the set of triples(Y,f,F)(in short just set of F’s),where F:Y→˜M is a lifting of the imbedding f:Y֒→M. Also Zϕ(Y)⊂Z(Y)is a smooth submanifold,since˜Mϕ⊂˜M is smooth.There is the canonical sectionΦ:Im(Y,M)→Z(Y)given byΦ(f)=˜f.Therefore,Φ−1Zϕ(Y):=Imϕ(Y,M)is the set of associative imbeddings Y⊂M.Also,any 2-frameﬁeldΛas above gives to a sectionΦΛ(f)=λϕ◦f.To make these deﬁnitions parameter free we also have to divide Im(Y,M)by the diﬀeomorphism group of Y.12SELMAN AKBULUT AND SEMA SALURThere are also the vertical tangent bundles of Z(Y)and Zϕ(Y)T v Z(Y)π−→Z(Y)∪∪T v Zϕ(Y)π|−→Zϕ(Y)withﬁbersπ−1(F)=Ω0(Y,F∗(Ξ∗⊗V)).By Lemma3theﬁbers of T v(Zϕ)can be identiﬁed with the kernel of the map induced by the Cliﬀord multiplication (31)c:Ω0(Y,F∗(Ξ∗⊗V))→Ω0(Y,F∗(V))One of the nice properties of a pseudo-associative submanifold(Y,f,F)is that there is a Cliﬀord multiplication action(by pull back)(32)F∗(Ξ∗)⊗F∗(V)→F∗(V)If F is close to˜f,by parallel translating theﬁbers over F(x)and˜f(x)along geodesics in˜M we get canonical identiﬁcations:(33)F∗(Ξ)∼=T Y F∗(V)∼=νfinducing Cliﬀord multiplication between the tangent and the normal bundles.So if ∀x∈Y the distance between F(x)and˜f(x)is less then the injectivity radius j(˜M), there is a Cliﬀord multiplication between the tangent and normal bundles of Y.3.2.Dirac operator.The normal bundleν=ν(Y)of any orientable3-manifold Y in a G2manifold (M,ϕ)has a Spin(4)structure(e.g.[B2]).Hence we have SU(2)bundles S and E over Y such thatν=S⊗H E(18),with SO(3)reductions adS=λ+(ν),and adE=λ−(ν)which is also the bundle of endomorphisms End(E).If Y is associative, then the bundle ad(S)becomes isomorphic to T Y,i.e.S becomes the spinor bundle of Y,soν(Y)becomes a twisted spinor bundle.The Levi-Civita connection of the G2metric of(M,ϕ)induces connections on the associated bundles V andΞon˜M.In particular,it induces connections on the tangent and normal bundles of any submanifold Y3⊂M.We will call these connections the background connections.Let A0be the induced connection on the normal bundleν=S⊗E.From the Lie algebra decomposition so(4)=so(3)⊕so(3),we can write A0=B0⊕A0,where B0and A0are connections on S and E, respectively.Let A(E)and A(S)be the set of connections on the bundles E and S.Hence A∈A(E),B∈A(S)are in the form A=A0+a,B=B0+b,where a∈Ω1(Y,ad E) and b∈Ω1(Y,ad S).SoΩ1(Y,λ±(ν))parametrizes connections on S and E,and the connections onνare in the form A=B⊕A.To emphasize the dependency on b and a we sometimes denote A=A(b,a),and A0=A(0,0)=A0.13 Now,let Y3⊂M be any smooth manifold.We can ex press the covariant derivative∇A:Ω0(Y,ν)→Ω1(Y,ν)onνby∇A= e i⊗∇e i,where{e i}and{e i} are orthonormal tangent and cotangent frameﬁelds of Y,respectively.Furthermore, if Y is an associative submanifold,we can use the Cliﬀord multiplication of(25)(i.e. the cross product)to form the twisted Dirac operator D/A:Ω0(Y,ν)→Ω0(Y,ν) (34)D/A= e i.∇e iThe sections lying in the kernel of this operator are usually called harmonic spinors twisted by(E,A).Elements of the kernel of D/Aare called the harmonic spinors twisted by E,or just the twisted harmonic spinors.4.DeformationsIn[M],McLean showed that the space of associative submanifolds of a G2mani-fold(M,ϕ),in a neighborhood of aﬁxed associative submanifold Y,can be identiﬁed with the harmonic spinors on Y twisted by E.Since the cokernel of the Dirac op-erator can vary,the dimension of its kernel is not determined(it has zero index since Y is odd dimensional).We will remedy this problem by deforming Y in a larger class of submanifolds.To motivate our aproach we willﬁrst sketch a proof of McLean’s theorem(adapting the explanation in[B3]).Let Y⊂M be an associative submanifold,Y will determine a lifting˜Y⊂˜Mϕ.Let us recall that the G2structure ϕgives a metric connection on M,hence it gives a connection A0and a covariant diﬀerentiation in the normal bundleν(Y)=ν∇A:Ω0(Y,ν)→Ω1(Y,ν)=Ω0(Y,T∗Y⊗ν)Recall that we identiﬁed T∗y(Y)⊗νy(Y)by the tangent space of the Grassmannian of3-planes T G(3,7)in T y(M).So the covariant derivative lifts normal vectorﬁeldsv of Y⊂M to vertical vectorﬁelds˜v in T(˜M)|˜Y .We want the normal vectorﬁelds v of Y to move Y in the class of associative submanifolds of M,i.e.we want the liftings˜Y v of the nearby copies Y v of Y(pushed oﬀby the vectorﬁeld v) to lie in˜Mϕ⊂˜M upstairs,i.e.we want the component of˜v in the direction of the normal bundle˜Mϕ⊂˜M to vanish.By Lemma3,this means∇A(v)should be in the kernel of the Cliﬀord multiplication c=cϕ:Ω0(T∗(Y)⊗ν)→Ω0(ν),i.e.D/A0(v)=c(∇A(v))=0,where D/Ais the Dirac operator induced by thebackground connection A0,i.e.the composition(35)Ω0(Y,ν)∇A0−→Ω0(Y,T∗Y⊗ν)c→Ω0(Y,ν)The condition D/A(v)=0impliesϕmust be integrable at Y,i.e.the so(7)-metric connection∇Aon Y coincides with G2-connection(c.f.[B2]).Now we give a general version of the McLean’s theorem,without integrability assumption onϕ:Recall from(Section3.1)thatΦ−1Zϕ(Y)is the set of associative14SELMAN AKBULUT AND SEMA SALURsubmanifolds Y ⊂M ,where Φ:Im (Y,M )→Z (Y )is the canonical section (Gauss map)given by Φ(f )=˜f.Therefore,if f :Y ֒→M is the above inclusion,then Φ(f )∈Z ϕ.So this moduli space is smooth if Φwas transversal to Z ϕ(Y ).MM ~~G (3,7)Figure 2.Theorem 4.Let (M 7,ϕ)be a manifold with a G 2structure,and Y 3⊂M be an associative submanifold.Then the tangent space of associative submanifolds of M at Y can be identiﬁed with the kernel of a Dirac operator D /A :Ω0(Y,ν)→Ω0(Y,ν),where A =A 0+a ,and A 0is the connection on νinduced by the metric g ϕ,and a ∈Ω1(Y,ad (ν)).In the case ϕis integrable a =0.In particular,the space of associative submanifolds of M is smooth at Y if the cokernel of D /A is zero.Proof.Let f :Y ֒→M denote the imbedding.We consider unparameterized deformations of Y in Im (Y,M )along its normal directions.Fix a trivialization T Y ∼=im (H ),by (17)we have an identiﬁcation ˜f ∗(T v ˜M )∼=T Y ∗⊗ν+T Y +ν.We ﬁrst claim Π◦d Φ(v )=∇A (v ),where d Φis the induced map on the tangent space and Πis the vertical projection.Ω0(Y,ν)=T f Im (Y,M )d Φ−→T ˜f Z (Y )=Ω0(Y,˜f ∗(T v ˜M ))Π→Ω0(Y,T ∗Y ⊗ν)↓exp↓exp Im (Y,M )Φ−→Z (Y )。

A note on the Bruhat decomposition of semisimple Lie groups

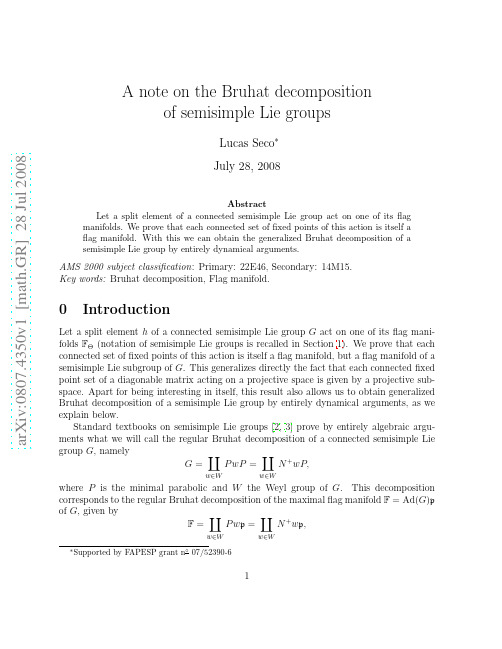
a r X i v :0807.4350v 1 [m a t h .G R ] 28 J u l 2008A note on the Bruhat decompositionof semisimple Lie groupsLucas Seco ∗July 28,2008Abstract Let a split element of a connected semisimple Lie group act on one of its ﬂag manifolds.We prove that each connected set of ﬁxed points of this action is itself a ﬂag manifold.With this we can obtain the generalized Bruhat decomposition of a semisimple Lie group by entirely dynamical arguments.AMS 2000subject classiﬁcation :Primary:22E46,Secondary:14M15.Key words:Bruhat decomposition,Flag manifold.0Introduction Let a split element h of a connected semisimple Lie group G act on one of its ﬂag mani-folds F Θ(notation of semisimple Lie groups is recalled in Section 1).We prove that each connected set of ﬁxed points of this action is itself a ﬂag manifold,but a ﬂag manifold of a semisimple Lie subgroup of G .This generalizes directly the fact that each connected ﬁxed point set of a diagonable matrix acting on a projective space is given by a projective sub-space.Apart for being interesting in itself,this result also allows us to obtain generalized Bruhat decomposition of a semisimple Lie group by entirely dynamical arguments,as we explain below.Standard textbooks on semisimple Lie groups [2,3]prove by entirely algebraic argu-ments what we will call the regular Bruhat decomposition of a connected semisimple Liegroup G ,namely G = w ∈W P wP = w ∈WN +wP,where P is the minimal parabolic and W the Weyl group of G .This decomposition corresponds to the regular Bruhat decomposition of the maximal ﬂag manifold F =Ad(G )p of G ,given by F = w ∈W P w p = w ∈WN +w p ,07/52390-61which can be seen as the decomposition of F into unstable manifolds of the action of a split-regular element h∈A+:see Section3of[1]for a proof of this by entirely dynamical arguments(this is recalled at the end of Section1).From this regular Bruhat decomposition on the maximalﬂag manifold F one readily obtains the regular Bruhat decomposition on the partialﬂag manifolds FΘ=Ad(G)pΘgiven byFΘ= w∈W/WΘP w pΘ= w∈W/WΘN+w pΘ,the argument goes as follows.Projecting the regular Bruhat decomposition of F onto FΘone needs only to show the disjointedness of the above decomposition.If the unstable manifolds N+w pΘand N+s pΘmeet,for s,w∈W,then there exists n∈N+such that w pΘ=ns pΘ.Taking the regular element h∈A+we have for k∈Z that w pΘis aﬁxed point so thatw pΘ=h−k w pΘ=h−k ns pΘ→s pΘ,when k→+∞.It follows that w pΘ=s pΘ,so that s−1w pΘ=pΘwhich,by the Iwasawa decomposition of PΘ,implies that s−1w∈KΘ∩M∗so that s−1w∈WΘ,that is,w∈sWΘ, as claimed.The corresponding decomposition in G is the regular Bruhat decompositionG= w∈W/WΘP wPΘ= w∈W/WΘN+wPΘ.Usually much harder to obtain is what we will call the generalized Bruhat decomposition of G,given byG=w∈W∆\W/WΘP∆wPΘ.To show this[3]appeals to the use of Tits Systems(see Section1.2of[3]).Dynamically, this corresponds to the decomposition of theﬂag manifold FΘinto unstable manifolds of the action of an irregular split-element h∈cl A+,h=exp(H),FΘ=w∈W H\W/WΘP H w pΘ=w∈W H\W/WΘN+HZ H w pΘ,where∆is the set of simple roots which annihilate H.In this case theﬁxed points of h degenerate intoﬁxed point manifolds Z H w pΘ.To show that these unstable manifolds aredisjoint we can argue as above to get rid of the unstable part N+H so that the only diﬃcultyis to show thatﬁxed point manifolds are disjoint when we take w∈W H\W/WΘ.At this point of the argument[1]appeals to a general theorem of Borel-Tits(see Proposition1.3 of[1]).In this note we show the disjointedness of the aboveﬁxed point manifolds as a byproduct of showing that each of theseﬁxed point manifold is itself equivariantly diﬀeomorphic to aﬂag manifold.With this,one can obtain the generalized Bruhat decomposition of a semisimple Lie group by entirely dynamical arguments:one follows Section3of[1]to2prove the regular Bruhat decomposition and then uses the result of this article to provethe generalized Bruhat decomposition.In theﬁrst section we recall notation and preliminar results on semisimple Lie theory.In the second section we prove the main result Theorem2.2and deduce from it that theﬁxed point manifolds are disjoint.1Preliminaries on Semi-simple Lie TheoryFor the theory of semi-simple Lie groups and theirﬂag manifolds we refer to Duistermat-Kolk-Varadarajan[1],Helgason[2]and Warner[3].To set notation let G be a connectednoncompact semi-simple Lie group with Lie algebra g.We assume throughout that G hasﬁnite center.Fix a Cartan involutionθof g with Cartan decomposition g=k⊕s.The form Bθ(X,Y)=− X,θY ,where ·,· is the Cartan-Killing form of g,is an inner product.Fix a maximal abelian subspace a⊂s and a Weyl chamber a+⊂a.We letΠbe theset of roots of a,Π+the positive roots corresponding to a+,Σthe set of simple roots in Π+andΠ−=−Π+the negative roots.For a rootα∈Πwe denote by Hα∈a its corootso that Bθ(Hα,H)=α(H)for all H∈a.The Iwasawa decomposition of the Lie algebra g reads g=k⊕a⊕n±with n±= α∈Π±gαwhere gαis the root space associated toα. As to the global decompositions of the group we write G=KS and G=KAN±withK=exp k,S=exp s,A=exp a and N±=exp n±.The Weyl group W associated to a is theﬁnite group generated by the reﬂections overthe root hyperplanesα=0in a,α∈Π.W acts on a by isometries and can be alternatively be given as W=M∗/M where M∗and M are the normalizer and the centralizer of A in K,respectively.We write m for the Lie algebra of M.There is an unique element w−∈W which takes the simple rootsΣto−Σ,w−is called the principal involution of W.Associated to a subset of simple rootsΘ⊂Σthere are several Lie algebras and groups(cf.[3],Section1.2.4):We write g(Θ)for the(semi-simple)Lie subalgebra generated bygα,α∈Θ,and put k(Θ)=g(Θ)∩k,a(Θ)=g(Θ)∩a,and n±(Θ)=g(Θ)∩n±.The simple roots of g(Θ)are given byΘ,more precisely,by restricting the functionals ofΘto a(Θ).The coroots Hα,α∈Θ,form a basis for a(Θ).Let G(Θ)and K(Θ)be the connected groups with Lie algebra g(Θ)and k(Θ),respectively.Then G(Θ)is a connected semisimple Lie group withﬁnite center.Let A(Θ)=exp a(Θ),N±(Θ)=exp n±(Θ).We have the Iwasawa decomposition G(Θ)=K(Θ)A(Θ)N±(Θ).Let aΘ={H∈a:α(H)= 0,α∈Θ}be the orthocomplement of a(Θ)in a with respect to the Bθ-inner product and put AΘ=exp aΘ.The subsetΘsingles out the subgroup WΘof the Weyl group which acts trivially aΘ.Alternatively WΘcan be given as the subgroup generated by the reﬂections with respect to the rootsα∈Θ.The restriction of w∈WΘto a(Θ)furnishes an isomorphism between WΘand the Weyl groyp W(Θ)of G(Θ)The standard parabolic subalgebra of typeΘ⊂Σwith respect to chamber a+is deﬁnedbypΘ=n−(Θ)⊕m⊕a⊕n+.The corresponding standard parabolic subgroup PΘis the normalizer of pΘin G.It has3the Iwasawa decomposition P Θ=K ΘAN +.The empty set Θ=∅gives the minimal parabolic subalgebra p =m ⊕a ⊕n +whose minimal parabolic subgroup P =P ∅has Iwasawa decomposition P =MAN +.Let ∆⊂Θ⊂Σ.Then P ∆⊂P Θ,also we denote by P (Θ)∆the parabolic subgroup of G (Θ)of type ∆.We let Z Θbe the centralizer of a Θin G and K Θ=Z Θ∩K .We have that K Θdecomposes as K Θ=MK (Θ)and that Z Θdecomposes as Z Θ=MG (Θ)A Θwhich implies that Z Θ=K ΘAN (Θ)is the Iwasawa decomposition of Z Θ(which is a reductive Lie group).Let ∆⊂Σ,then 1a Θ∩∆=a Θ+a ∆.Thus it follows that Z Θ∩∆=Z Θ∩Z ∆,K Θ∩∆=K Θ∩K ∆and P Θ∩∆=P Θ∩P ∆.For H ∈a we denote by Z H ,W H etc.the centralizer of H in G ,W etc.,respectively.When H ∈cl a +we putΘ(H )={α∈Σ:α(H )=0},and we have that Z H =Z Θ(H ),K H =K Θ(H ),N +H =N +(Θ(H ))and W H =W Θ(H ).Let n ±Θ= α∈Π±− Θ g αand N ±Θ=exp(n ±Θ).Then N ±decomposes as N ±=N (Θ)±N ±Θwhere N (Θ)±normalizes N ±Θand N (Θ)±∩N ±Θ=1.We have that g =n −Θ⊕p Θ,that N −Θ∩P Θ=1and also that P Θis the normalizer of n +Θin G .P Θdecomposes as P Θ=Z ΘN +Θ,where Z Θnormalizes N +Θand Z Θ∩N +Θ=1.We write p −Θ=θ(p Θ)for the parabolicsubalgebra opposed to p Θ.It is conjugate to the parabolic subalgebra p Θ∗where Θ∗=−(w −)Θis the dual to Θand w −is the principal involution of W .More precisely,p −Θ=k p Θ∗where k ∈M ∗is a representative of w −.If P −Θis the parabolic subgroup associated to p −Θthen Z Θ=P Θ∩P −Θand P −Θ=Z ΘN −Θ,where Z Θnormalizes N −Θand Z Θ∩N −Θ=1.The ﬂag manifold of type Θis the orbit F Θ=Ad(G )p Θ,which identiﬁes with the homogeneous space G/P Θ.Since the center of G normalizes p Θ,the ﬂag manifold depends only on the Lie algebra g of G .The empty set Θ=∅gives the maximal ﬂag manifold F =F ∅.If ∆⊂Θthen there is a G -equivariant projection F ∆→F Θgiven by g p ∆→g p Θ,g ∈G .Some subalgebras of g which are deﬁned by the choice of a Weyl chamber of a and a subset of the associated simple roots can be deﬁned alternatively by the choice of an element H ∈a as follows.First note that the eigenspaces of ad(H )in g are the weight spaces g α,and that the centralizer of H in g is given by z H = {g α:α(H )=0},where the sum is taken over α∈a ∗.Now deﬁne the negative and positive nilpotent subalgebras of type H given by n −H = {g α:α(H )<0},n +H = {g α:α(H )>0},and the parabolic subalgebra of type H which is given by p H = {g α:α(H )≥0},where in all cases αruns through all the roots Π.Then we have thatg =n −H ⊕z H ⊕n +H and p H =z H ⊕n +H ,and thatn±wH =w n±Hp wH=w p H.Deﬁne theﬂag manifold of type H given by the orbitF H=Ad(G)p H.Now choose a chamber a+of a which contains H in its closure,consider the simple rootsΣassociated to a+and takeΘ(H)⊂Σ.Since a rootα∈Θ(H)if,and only if,α|aΘ(H)=0,we have thatz H=zΘ(H),n±(H)=n±Θ(H),p H=pΘ(H).So it follows thatF H=FΘ(H),and that the isotropy of G in p H is PΘ(H)=KΘ(H)AN+=K H AN+,since KΘ(H)=K H. In particular we have thatw pΘ(H)=w p H=p wH.We note that we can proceed reciprocally.That is,if a+andΘare given,we can choose an H∈cl a+such thatΘ(H)=Θand describe the objects that depend on a+andΘby H(clearly,such an H is not unique.)We recall the dynamics of a split element of G acting in theﬂag manifold FΘ(see Section3of[1]).Let the split element H∈cl a+act in FΘbyt·b=exp(tH)b,b∈FΘ.(1) The connected sets ofﬁxed point of the H-action are parametrized by w∈W and are given byﬁx(H,w)=Z H w pΘ=K H w pΘ,(2) so that they are in bijection with the double coset W H\W/WΘ.It follows from Z H=ZΘ(H) and from the decomposition ZΘ=G(Θ)MAΘthat we haveﬁx(H,w)=G(Θ(H))w pΘ.Each w-ﬁxed point set has stable/unstable manifold given respectively byst(H,w)=N±Θ(H)ﬁx(H,w)=P±Θ(H)w pΘ.(3)This H-action decomposes FΘin the disjoint union of stable/unstable manifoldsFΘ=W H\W/WΘP±Θ(H)w pΘ,(4)this is known as the Bruhat decomposition of FΘ.It follows from these considerations that the dynamics of the H-action in FΘdepends only on the set of rootsΘ(H)which annihilate H.52Fixed points as ﬂag manifoldsLet πΘ:a →a (Θ)be the orthogonal projection parallel to a Θ.Lemma 2.1The following assertions are true.1.The projection by πΘof a regular element of a is a regular element of a (Θ).2.The projection by πΘof a chamber in a is contained inside a chamber of a (Θ).3.For w ∈W denote by a (Θ)w the chamber of a (Θ)which contains the projection by πΘof the chamber w a +.Then the nilpotent subalgebras n +e n (Θ)w w.r.t.to the chambers a +and a (Θ)w satisfyn (Θ)w ⊂w n +.Proof:We ﬁrst observe that for α∈Θ,we have that α|a Θ=0so that for H ∈a we have α(πΘ(H ))=α(H ).From this it follows that if H is regular in a ,then πΘ(H )is regular in a (Θ),which proves the ﬁrst item.For the second item we observe that the projection of a chamber of a is a convex set of a (Θ)which,by the ﬁrst item,consists of regular elements of a (Θ),and hence it is contained in a chamber of a (Θ).For the third item let α∈Θ.If α>0in a (Θ)w then α>0in πΘ(w a +)and hence,by the ﬁrst remark of the proof,we have that α>0in w a +.It follows thatn (Θ)w = {g α:α|a (Θ)w >0,α∈ Θ }⊂{g α:α|w a +>0,α∈Π}=w n +,as desired.Theorem 2.2Let X ∈cl a +,Θ⊂Σ,w ∈W .Consider ∆=Θ(X )and H Θ∈cl a +such that Θ(H Θ)=Θ.Then the mapﬁx(X,w )Θ→F π∆(wH Θ)(g (∆)),g p wH Θ→g p π∆(wH Θ),g ∈G (∆)is a well deﬁned G (∆)-equivariant diﬀeomorphism.Proof:Since we have ﬁx(X,w )Θ=G (∆)p wH Θit follows that the above map,let us call it ψ,is deﬁned in all of its domain and it is G (∆)-equivariant.It remains to prove that ψis well deﬁned and is injective both of which will follow if we show that the isotropy of p wH Θin G (∆)coincides with the isotropy of p π∆(wH Θ)in G (∆).For this,let a (∆)w the chamber of a (∆)which contains the projection by π∆of the chamber w a +.Consider the Iwasawa decomposition of G and P wH Θw.r.t.to the chamber w a +,and the Iwasawa decomposition of G (∆)w.r.t.to the chamber a (∆)wG =KAwN +w −1,P wH Θ=K wH ΘAwN +w −1,G (∆)=K (∆)A (∆)N (∆)w ,6where,by item(3)of the previous Lemma,we have thatN(∆)w⊂wN+w−1.Thus,by the uniqueness of the Iwasawa decomposition of G,it follows that the isotropy of p wHΘin G(∆)is given byG(∆)∩P wHΘ=(K(∆)∩K wHΘ)A(∆)N(∆)w.Theﬁrst term in the right hand side can be written asK(∆)∩K wHΘ=K(∆)wHΘ=K(∆)π∆(wHΘ),where in the last equality we used that K(∆)already centralizes a∆.Sinceπ∆(wHΘ)lies in the closure of the chamber a(Θ)w,it follows thatG(∆)∩wPΘw−1=K(∆)π∆(wHΘ)A(∆)N(∆)w=Pπ∆(wHΘ)(∆),which is precisely the isotropy of pπ∆(wHΘ)in G(∆).It is then immediate that the inverseofψis given by g pπ∆(wHΘ)→g p wHΘ,g∈G(∆),which shows thatψis a diﬀeomorphism.Corollary2.3Ifﬁx(X,w′)Θ∩ﬁx(X,w)Θ=∅then w′∈W X wWΘ.Proof:Here we will adopt the notation of Theorem2.2,denoting byψthe diﬀeomorphism of that theorem.Ifﬁx(X,w′)Θ∩ﬁx(X,w)Θ=∅then there exists g∈G(∆)such that w′pΘ=gw pΘ.Take a regular h∈A(∆),using the G(∆)-equivariance ofψfor k∈Z we have thatw′pΘ=h k w′pΘ=h k gw pΘ=h k g p wHΘ=ψ−1(h k g pπ∆(wHΘ))=(∗).By the regular Bruhat decomposition of theﬂag manifold Fπ∆(wHΘ)(g(∆))(cf.Theorem??),letting k→∞we have that there exists s∈W(∆)=W∆=W X such that(∗)→ψ−1(s pπ∆(wHΘ))=s p wHΘ=sw pΘ.It follows that w−1s−1w′pΘ=pΘ,so that w−1s−1w′∈M∗∩KΘ,which implies that w−1s−1w∈WΘ.Hence w∈swWΘ⊂W X wWΘ,as desired.References[1]Duistermat,Kolk,Varadarajan,Functions,ﬂows and oscilatory integral onﬂag man-ifolds and(...),Compositio Math.49,309-398,(1983)[2]Helgason,S.Diﬀerential Geometry,Lie Groups and Symmetric Spaces,AcademicPress,(1978).[3]Warner,G.Harmonic analysis on semi-simple Lie groups I.Springer-Verlag(1972).7。

医学英语文献阅读-2022年学习资料

Brachiocephalic artery-Lelt common carotid artery-Pul onic valve-Left subclavian artery-Superior vena cavaortic arch-Pulmonary artery-Right pulmonary-Left pulm nary-artery branches-Ascending-aorta-veins-Left atriu -Aortic valve-Mitral-Right--bicuspid-Tricuspid-ventri le-Right ventricle-Inferior-Endocardium-Apex-Blood hi h in oxygen-Myocardium-Interventricular-Blood low in xygen-Epicardium-septum-FIGURE 9-2.The heart and grea vessels.Reprinted with permission from Cohen BJ,Wood DL.Memmler's The-Human Body in Health and Disease.9th Ed.Philadelphia:Lippincott williams wilkins,2000.
Frontal-Sagittal-Transverse-coronal-plane-horizontalIGURE 5-2.Planes of division.Reprinted with permissio from Cohen BJ,Wood DL.Memmler's The Human-Body in He lth and Disease.9th Ed.Philadelphia:Lippincott Willia s Wilkins,2000.

an overweights men阅读理解

an overweights men阅读理解Like a needle climbing up a bathroom scale, the number keeps rising. In 1991, 15% of Americans were obese(肥胖的); by 1999, that proportion had grown to 27%. Youngsters, who should have age and activity on their side, are growing larger as well: 19% of Americans under 17 are obese. Waistbands have been popping in other western countries too, as physical activity has declined and diets have expanded. By and large, people in the rich world seem to have lost the fight against flab(松弛).Meanwhile, poorer nations have enjoyed some success in their battles against malnutrition and famine. But, according to research presented at the annual meeting of the American Association for the Advancement of Science, it is more a case of being out of the frying pan and into the fire. The most striking example actually in the poor world comes from the Pacific islands, home of the world's most obese communities. In 1966, 14% of the men on this island were obese while 100% of men under the age of 30 in 1996 were obese.This increase in weight has been uneven as well as fast. As a result, undernourished and over-nourished people frequently live cheek by jowl(面颊). The mix can even occurwithin a single household. A study of families in Indonesia found that nearly 10% contained both the hungry and the fat. This is a mysterious phenomenon, but might have something to do with people of different ages being given different amounts of food to eat.The prospect of heading off these problems is bleak. In many affected countries there are cultural factorsto contend with, such as an emphasis on eating large meals together, or on food as a form. of hospitality.Moreover, there is a good measure of disbelief on the part of policymakers that such a problem Could existin their countries. Add to that reluctance on the part of governments to spend resources on promoting dietand exercise while starvation is still a real threat, and the result is a recipe for inaction. Unless something is done soon, it might not be possible to turn the clock back.练习题：Choose correct answers to the question:1.The first sentence of the passage most probably implies that ______.A.many Americans are obsessed with the rising temperature in their bathroomB.more people are overweighed in the United StatesC.people are doing more physical exercises with the help of scalesD.youngsters become taller and healthier thanks to more activities2.As physical exercise declines and diet expands, ______.A.other western countries has been defeated by fatB.obesity has become an epidemic(流行病)of the rich worldC.waistbands begin to be popular in other western countriesD.western countries can no longer fight against obesity3.Which is NOT the point of the example of the Pacific Islands?A.The poor community has shaken off poverty and people are well-fed now.B.Obesity is becoming a problem in the developing world too.C.Excessive weight increase will cause no less harm than the food shortage.D.The problem of overweight emerges very fast.4.Of tackling obesity in the poor world, we can learn from the passage that____A.the matter is so complex as to go beyond our capacityB.no matter what we do, the prospect will always be bleakC.it is starvation, the real threat, that needs to be solvedD.we should take immediate actions before it becomes incurable5.What is the main idea of this passage?A.Obesity is now a global problem that needs tackling.B.The weights increase fast throughout the whole world.C.Obesity and starvation are two main problems in the poor world.D.Obesity has shifted from the rich world to the poor world.。

天线选择题

Chapter11.An antenna, in addition to radiating or receiving energy, it is also used to ___ energy in some directions and suppress in others.A.dissipating 发散B.generate noiseC.Accentuate 突出2.An antenna is a device that, in the transmitting mode, converts ___ waves to ___ waves.A.Traveling, guidedB.Free-space, travelingC.Guided, free-space3.An antenna is a device that, in the transmitting mode, converts ___ waves to ___ waves.A.Traveling, guidedB.Free-space, travelingC.Free-space, guided4.Constructive and destructive interference patterns inside transmission lines connected to antenna, formed by reflections from impedance mismatches between the transmission lines and the antenna, are referred to as:A.Traveling wavesB.Free-space wavesC.Standing waves5.An aggregate集合of individual discrete radiating elements(antenna) in an electrical and geometrical arrangement is referred to as an:A.continues sourceB.arrayC.waveguide6.To create a radiation, there must be a ___A.direct currentB.time-varying currentC.accelerating current7.To create a radiation, there must be a ___A.direct chargeB.time-varying chargeC.accelerating charge8.To create a radiation, there must be a ___A.direct chargeB.time-varying chargeC.decelerating charge9.Radiation can be created by ___A.smooth wiresB.discontinuous wiresC.infinite length wires10.Radiation can be created by ___A.smooth wiresB.bent wiresC.infinite length wires11.The distance between the two half-period free-space waves is ___mbda/4mbda/2mbda12.The length L(in wavelength) of a wire/dipole antenna fed at the center whose current maximum occurs at the feed is equal to ___ . Assume 0<L<lambda.A.0.25 lambdaB.0.5 lambdaC.1.0 lambdaCCCCB BCCBB BBChapter21.A radiator having equal radiation in all direction is referred to as a:A.Directional radiatorB.Isotropic radiatorC.Guided radiator2.A pattern having an essentially nondirectional pattern in a given plane and a directional pattern in any orthogonal plane is referred to as:A.DirectionalB.OmnidirectionalC.Isotropic3.A radiator having the property of radiating or receiving electromagnetic waves more effectively in some directions than in other is referred to as a:A.Directional antennaB.Omnidirectional antennaC.Isotropic antenna4.The plane that contains the electric-field vector and the direction of maximum radiation is referred to as the:A.E-planeB.H-planeC.D-plane5.The plane that contains the magnetic-field vector and the direction of maximum radiation is referred to as the:A.E-planeB.H-planeC.D-plane6.The portion of the radiation pattern bounded by regions of relatively weak radiation is to as radiation ___A.EfficiencyB.LobeC.Impedance7.The radiation lobe containing the direction of maximum radiation is referred to as the:A.MinorB.MajorC.Lobe8.The region mostimmediate to antenna where the radiative field predominates is referred to as the:A.Far-field regionB.Radiating near-field regionC.Reactive near-field region9.The region of an antenna where the angular field distribution is essentially independent of the distance from the antenna and where the real power density predominates is referred to as the:A.Far-field regionB.Radiating near-field regionC.Reactive near-field region10.The region of an antenna between the reactive near-field is referred to as the:A.Radiating near-field regionB.Fraunhofer regionC.Friis region11.In MKS, the units of power density are:A.Watts/meterB.Watts/meter squaredC.Watts/unit solid angle12.In MKS, the units of power intensity are:A.Watts/meterB.W atts/meter squaredC.Watts/unit solid angleBBAAB BBCAA BC13.The radiation intensity is related to the radiation by:A.RB.R^2C.1/r^214.The ratio of the radiation intensity of an antenna to the radiation intensity of an isotropic source is defined as the:A.GainB.DirectivityC.Efficiency15.The ratio of 4pi over the beam solid angle is defined as the:A.GainB.DirectivityC.Efficiency16.The ratio of the gain to the directivity of an antenna is defined as the:A.Aperture efficiencyB.Reflection efficiencyC.Radiation efficiency17.The directivities D_theta and D_phi are referred to as the:A.Theta directivitiesB.Phi directivitiesC.Partial directivities18.The total maximum directivity is also defined as Do=4*Pi/WA where WA is referred to as the:A.Beam efficiencyB.Beam bandwidthC.Beam solid angle19.The angular separation between two identical point on an amplitude pattern of an antenna is referred to as the:A.BandwidthB.Beam widthC.Lobe20.The range of frequencies within which the performance of an antenna, with respect to some characteristics, conform to a specific standard is referred to as the:A.BandwidthB.Beam widthC.LobeBBBCCCBA21.The figure traced, as a function of time, of the extremity of a time-varying electric field vector at a fixed location in space on a plane perpendicular to the direction of propagation is referred to as the:A.GainB.DirectivityC.Polarization22.The polarization of an electric field with two components orthogonal to each other and to the direction of propagation, of the same amplitude, and with a 90 deg phase difference is:A.LinearB.CircularC.Elliptical23.An electric field that has two orthogonalcomponents transverse to the direction of propagation and a 0 deg or multiples of 180 deg phase difference is:A.LinearB.CircularC.Elliptical24.The polarization of an electromagnetic wave two orthogonal components transverse to the direction of propagation, the components are of equal magnitude and the phase difference between the two field components is 45 deg is:A.LinearB.CircularC.Elliptical25.The factor that accounts for the polarization mismatches between an incident wave and a receiving antenna is referred to as the:A.Antenna efficiencyB.Polarization loss factorC.Polarization ellipse26.The maximum effective area of an small finite length dipole, with a idealized triangular currentdistribution, is typically ___ than the physical area of its cross section.A.EqualB.GreaterC.Smaller27.Same as 26B28.The real pats of the input impedance of an antenna are referred to as the:A.Radiation resistanceB.Radiation reactanceC.Reflection resistance29.The ratio of the radiation resistance over the sum of the radiation resistance and loss resistance is defined as the:A.Antenna efficiencyB.Radiation efficiencyC.Reflection efficiency30.For a linear wire antenna, its maximum effective length is typically ___ than the physical length.A. GreaterB.SmallerC.Equal31.For an aperture antenna, its maxmum effective area is typically ___ than the physical area.A.GreaterB.SmallerC.Equal32.The ratio of the maximum effective area to the physical area of an antenna is defined as the:A.Antenna efficiencyB.Reflection efficiencyC.Aperture efficiency33.The ratio of the available power at the terminals ofa receiving antenna to the power density of a plane wave incident on the antenna is referred to as the:A.Effective lengthB.Effective areaC.Directivity34.The scattering characteristics of a radar target are usually represented by the:A.GainB.DirectivityC.Radar cross section35.The equation that relates the received power tothe transmitted power of the two antennas, separated by a large distance R, is referred to as the:A.Radar rangeB.Friis transmissionC.Antenna temperature36.The equation that relates the received power to the transmitted power of the two antennas, after the signal has been scattered by a radar target, is referred to as the:A.Radar rangeB.Friis transmissionC.Antenna temperatureCBACB BBABB BCBCB A。

1、下载文档前请自行甄别文档内容的完整性，平台不提供额外的编辑、内容补充、找答案等附加服务。
2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
3、如文档侵犯您的权益，请联系客服反馈,我们会尽快为您处理(人工客服工作时间：9:00-18:30)。

∗
Spin-00/17, hep-th/0006145.
1
2
at the two elements in the centre of SU(2), and k − 1 spherical D2branes [10]. A closer look at this quantisation condition suggests a natural deﬁnition for the D0-brane charge of a given D-brane, which reads 1 1 Q0 = 2 4π T 2π g ˜∗ ω −
˜ (g ) with A Dirichlet direction is determined by an eigenvector of R eigenvalue −1, whereas all the other eigenvectors correspond to Neumann directions, that is, directions tangent to the worldvolume of the D-brane. If we parametrise the worldsheet of the string by (σ, τ ) we can rewrite the above boundary conditions in the following form ˜ )∂σ X = (½ − R ˜ )∂τ X , i(½ + R (6)
1. Introduction Recently the issues of D0 charge and U(1) ﬂux quantisation for a class of D-branes in the SU(2) group manifold have attracted a great deal of attention [1, 2, 3, 4, 5, 6]. Our aim here is to show, using a somewhat diﬀerent line of argument, that the same basic results can be obtained without any reference to a hypothetical U(1) gauge ﬁeld or, indeed, of its ﬂux. In this letter we show, using the formalism developed in [7], that a D-brane in a group manifold sitting on a (twisted) conjugacy class C, and described, in the framework of the boundary state approach, by the matrix R of gluing conditions is characterised by a gauge invariant two-form ﬁeld ω deﬁned on the worldvolume of the D-brane whose components are determined by R. By comparing the boundary conditions coming from the gluing conditions with the ones deduced from the classical sigma model action, we are able to identify this two-form ﬁeld ω with the gauge-invariant combination B + 2πα′F . In order to write the boundary WZW action in terms of the threeform H and the two-form ω one is forced to introduce, much as in the case of the standard WZW model, a certain ﬁeld extension g ˜. The requirement that the quantum theory be independent of the choice of ﬁeld extension imposes two quantisation conditions [8, 9]: the ﬁrst one, imposed on H alone and similar to the closed string case, is an integrality condition on the cohomology class of H in H ∗ (G); whereas the second is that the periods of (H, ω ) over cycles in the relative homology H3 (G, C) take integer values. In the case of SU(2) at level k , these conditions yield k + 1 D-branes: two point-like D-branes situated
A NOTE ON D-BRANES IN GROUP MANIFOLDS: FLUX QUANTISATION AND D0-CHARGE
SONIA STANCIU
arXiv:hep-th/0006145v2 31 Jul 2000
Abstract. We show that a D-brane in a group manifold given by a (twisted) conjugacy class is characterised by a gauge invariant two-form ﬁeld determined in terms of the matrix of gluing conditions. Using a quantisation argument based on the path integral one obtains the known quantisation condition for the corresponding D-branes. We ﬁnd no evidence for the existence of a quantised U(1) gauge ﬁeld ﬂux. We propose an expression for the D0 charge of such D-branes.
∂B B
g ˜∗ H
(mod k ) ,
(1)
where B is a three-manifold such that g (∂ B) = C. This quantity is naturally gauge invariant and quantised (with integer values), independently of any assumption regarding the existence of a U(1) gauge ﬁeld on the brane. In the particular case of the D2-branes in SU(2), the H ﬁeld contribution is similar to the Poynting-type bulk contribution advocated in [3], and is valid also when H belongs to a nontrivial cohomology class. This quantity can be thought of as a generalisation of the U(1) ﬂux in the case where the three-form ﬁeld H belongs to a nontrivial cohomology class. 2. Semi-classical analysis 2.1. Boundary conditions from the boundary state approach. We consider D-branes in a group G which preserve conformal invariance and the inﬁnite-dimensional symmetry of the current algebra of the bulk theory. They are described in terms of the following gluing conditions: ¯, J = RJ (2) where the matrix of gluing conditions R : g → g is a metric-preserving automorphism of the Lie algebra g; that is, [R(Ta ), R(Tb )] = R([Ta , Tb ]) , and RT ΩT = Ω , (4) in the obvious notation. This type of gluing conditions describe [10, 11, 7] D-branes whose worldvolumes lie on twisted conjugacy classes. More precisely, D-branes in a WZW model with group G come in several types, classiﬁed [12] by the group Outo (G) of metric-preserving outer automorphisms of G, which is deﬁned as the quotient Auto (G)/Inno (G) of the group of metric-preserving automorphisms by the invariant subgroup of inner automorphisms. In [7] it was shown that the above gluing conditions give rise, at a given point g in G, to the following boundary conditions ¯ , ∂g = R(g )∂g where the map R(g ) : Tg G → Tg G is deﬁned as