An automata-theoretic approach to reasoning about infinite-state systems
氢键的应用
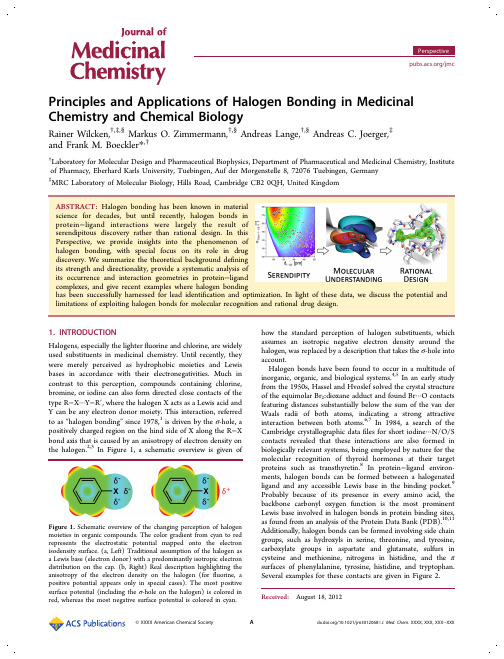
how the standard perception of halogen substituents, which assumes an isotropic negative electron density around the halogen, was replaced by a description that takes the σ-hole into account. Halogen bonds have been found to occur in a multitude of inorganic, organic, and biological systems.4,5 In an early study from the 1950s, Hassel and Hvoslef solved the crystal structure of the equimolar Br2:dioxane adduct and found Br···O contacts featuring distances substantially below the sum of the van der Waals radii of both atoms, indicating a strong attractive interaction between both atoms.6,7 In 1984, a search of the Cambridge crystallographic data files for short iodine···N/O/S contacts revealed that these interactions are also formed in biologically relevant systems, being employed by nature for the molecular recognition of thyroid hormones at their target proteins such as transthyretin.8 In protein−ligand environments, halogen bonds can be formed between a halogenated ligand and any accessible Lewis base in the binding pocket.9 Probably because of its presence in every amino acid, the backbone carbonyl oxygen function is the most prominent Lewis base involved in halogen bonds in protein binding sites, as found from an analysis of the Protein Data Bank (PDB).10,11 Additionally, halogen bonds can be formed involving side chain groups, such as hydroxyls in serine, threonine, and tyrosine, carboxylate groups in aspartate and glutamate, sulfurs in cysteine and methionine, nitrogens in histidine, and the π surfaces of phenylalanine, tyrosine, histidine, and tryptophan. Several examples for these contacts are given in Figure 2.
英语科普环保类阅读试题答案及解析

英语科普环保类阅读试题答案及解析1. February 16, 2014(VOA)JAKARTA — The United States and China say they recognize the need for "urgent action" to address the twin challenges of climate change and the air pollution caused by burning fossil fuels. U.S. Secretary of State John Kerry called for greater political will to address a warming planet.China is the leading producer of greenhouse gases and joins the United States as the largest consumers of energy. So U.S. officials say the world's two biggest economies agreeing to limit emissions and promote energy efficiency in buildings and industry "sends a strong message to the world" that this is an issue that needs to be addressed now.Following that agreement with Chinese leaders, Secretary Kerry told an audience in Indonesia that Washington hopes this "unique partnership" with Beijing will help set an example for global leadership and global seriousness on climate change.Kerry Warns About Global Climate Change"Together we account for roughly 40 percent of the world’s emissions. But this is not just about China and the United States," Kerry said. "It is about every country on Earth doing whatever it can to pursue cleaner and healthier energy sources.Kerry said scientific evidence compels the world to act."It is not a lack of ability that is the problem," Kerry said. "It is a lack of political resolve that is standing in our way.The European Union is calling for a 40 percent cut in carbon emissions by 2030. European Commission President Jose Manuel Barroso says that target is ambitious and affordable."It shows that we are beyond debate where we either have to be green or a defender of industry," Barroso said. "We believe these two issues are not contradictory, but can perfectly go together if handled smartly.Some European parliamentarians and some environmentalists believe the carbon reduction goals do not do enough to encourage cleaner energy. Jason Anderson heads European climate and energy policy for the World Wildlife Fund."We want to make sure that they do not put the brakes on the energy transition that we are seeing now," Anderson said. "Their ambitions for renewable energy would actually slow down the pace of change, which it is completely senseless as it is one of those areas of the economy that is growing right now.Kerry's climate speech was the first in a series of events meant to focus on cutting carbon emissions before talks in Paris next year on coming up with a successor to the 1997 Kyoto Protocol, which was never ratified by the United States. ()419【1】European Commission President Jose Manuel Barroso believes that________.A.The European Union accounts for 40 percent of the world’s emissions.B.we either have to be green or a defender of industry.C.we have to be green and a defender of industry if handled smartly.D.the carbon reduction goals do not do enough to encourage cleaner energy.【答案】C【解析】考查细节理解。
Journal of Loss Prevention in the Process Industries

HAZOP e Local approach in the Mexican oil &gas industryM.Pérez-Marín a ,M.A.Rodríguez-Toral b ,*aInstituto Mexicano del Petróleo,Dirección de Seguridad y Medio Ambiente,Eje Central Lázaro Cárdenas Norte No.152,07730México,D.F.,Mexicob PEMEX,Dirección Corporativa de Operaciones,Gerencia de Análisis de Inversiones,Torre Ejecutiva,Piso 12,Av.Marina Nacional No.329,11311México,D.F.,Mexicoa r t i c l e i n f oArticle history:Received 3September 2012Received in revised form 26March 2013Accepted 27March 2013Keywords:HAZOPRisk acceptance criteria Oil &gasa b s t r a c tHAZOP (Hazard and Operability)studies began about 40years ago,when the Process Industry and complexity of its operations start to massively grow in different parts of the world.HAZOP has been successfully applied in Process Systems hazard identi fication by operators,design engineers and consulting firms.Nevertheless,after a few decades since its first applications,HAZOP studies are not truly standard in worldwide industrial practice.It is common to find differences in its execution and results format.The aim of this paper is to show that in the Mexican case at National level in the oil and gas industry,there exist an explicit acceptance risk criteria,thus impacting the risk scenarios prioritizing process.Although HAZOP studies in the Mexican oil &gas industry,based on PEMEX corporate standard has precise acceptance criteria,it is not a signi ficant difference in HAZOP applied elsewhere,but has the advantage of being fully transparent in terms of what a local industry is willing to accept as the level of risk acceptance criteria,also helps to gain an understanding of the degree of HAZOP applications in the Mexican oil &gas sector.Contrary to this in HAZOP ISO standard,risk acceptance criteria is not speci fied and it only mentions that HAZOP can consider scenarios ranking.The paper concludes indicating major implications of risk ranking in HAZOP,whether before or after safeguards identi fication.Ó2013Elsevier Ltd.All rights reserved.1.IntroductionHAZOP (Hazard and Operability)studies appeared in systematic way about 40years ago (Lawley,1974)where a multidisciplinary group uses keywords on Process variables to find potential hazards and operability troubles (Mannan,2012,pp.8-31).The basic prin-ciple is to have a full process description and to ask in each node what deviations to the design purpose can occur,what causes produce them,and what consequences can be presented.This is done systematically by applying the guide words:Not ,More than ,Less than ,etc.as to generate a list of potential failures in equipment and process components.The objective of this paper is to show that in the Mexican case at National level in the oil and gas industry,there is an explicit acceptance risk criteria,thus impacting the risk scenarios priori-tizing process.Although HAZOP methodology in the Mexican oil &gas industry,based on PEMEX corporate standard has precise acceptance criteria,it is not a signi ficant difference in HAZOP studies applied elsewhere,but has the advantage of being fullytransparent in terms of what a local industry is willing to accept as the level of risk acceptance criteria,also helps to gain an under-standing of the degree of HAZOP applications in the Mexican oil &gas sector.Contrary to this in HAZOP ISO standard (ISO,2000),risk acceptance criteria is not speci fied and it only mentions that HAZOP can consider scenarios ranking.The paper concludes indicating major implications of risk prioritizing in HAZOP,whether before or after safeguards identi fication.2.Previous workHAZOP studies include from original ICI method with required actions only,to current applications based on computerized documentation,registering design intentions at nodes,guide words,causes,deviations,consequences,safeguards,cause fre-quencies,loss contention impact,risk reduction factors,scenarios analysis,finding analysis and many combinations among them.In the open literature there have been reported interesting and signi ficant studies about HAZOP,like HAZOP and HAZAN differences (Gujar,1996)where HAZOP was identi fied as qualitative hazard identi fication technique,while HAZAN was considered for the quantitative risk determination.This difference is not strictly valid today,since there are now companies using HAZOP with risk analysis*Corresponding author.Tel.:þ525519442500x57043.E-mail addresses:mpmarin@imp.mx (M.Pérez-Marín),miguel.angel.rodriguezt@ ,matoral09@ (M.A.Rodríguez-Toral).Contents lists available at SciVerse ScienceDirectJournal of Loss Prevention in the Process Industriesjou rn al homepage :/locate/jlp0950-4230/$e see front matter Ó2013Elsevier Ltd.All rights reserved./10.1016/j.jlp.2013.03.008Journal of Loss Prevention in the Process Industries 26(2013)936e 940and its acceptance criteria(Goyal&Kugan,2012).Other approaches include HAZOP execution optimization(Khan,1997);the use of intelligent systems to automate HAZOP(Venkatasubramanian,Zhao, &Viswanathan,2000);the integration of HAZOP with Fault Tree Analysis(FTA)and with Event Tree Analysis(ETA)(Kuo,Hsu,& Chang,1997).According to CCPS(2001)any qualitative method for hazard evaluation applied to identify scenarios in terms of their initial causes,events sequence,consequences and safeguards,can beextended to register Layer of Protection Analysis(LOPA).Since HAZOP scenarios report are presented typically in tabular form there can be added columns considering the frequency in terms of order of magnitude and the probability of occurrence identified in LOPA.There should be identified the Independent and the non-Independent Protection Layers,IPL and non-IPL respec-tively.Then the Probability of Failure on Demand(PFDs)for IPL and for non-IPL can be included as well as IPL integrity.Another approach consists of a combination of HAZOP/LOPA analysis including risk magnitude to rank risk reduction actions (Johnson,2010),a general method is shown,without emphasizing in any particular application.An extended HAZOP/LOPA analysis for Safety Integrity Level(SIL)is presented there,showing the quan-titative benefit of applying risk reduction measures.In this way one scenario can be compared with tolerable risk criteria besides of being able to compare each scenario according to its risk value.A recent review paper has reported variations of HAZOP methodology for several applications including batch processes, laboratory operations,mechanical operations and programmable electronic systems(PES)among others(Dunjó,Fthenakis,Vílchez, &Arnaldos,2010).Wide and important contributions to HAZOP knowledge have been reported in the open literature that have promoted usage and knowledge of HAZOP studies.However,even though there is available the IEC standard on HAZOP studies,IEC-61882:2001there is not a worldwide agreement on HAZOP methodology and there-fore there exist a great variety of approaches for HAZOP studies.At international level there exist an ample number of ap-proaches in HAZOP studies;even though the best advanced prac-tices have been taken by several expert groups around the world, there is not uniformity among different consulting companies or industry internal expert groups(Goyal&Kugan,2012).The Mexican case is not the exception about this,but in the local oil and gas industry there exist a national PEMEX corporate standard that is specific in HAZOP application,it includes ranking risk scenarios (PEMEX,2008),qualitative hazard ranking,as well as the two ap-proaches recognized in HAZOP,Cause by Cause(CÂC)and Devia-tion by Deviation(DÂD).Published work including risk criteria include approaches in countries from the Americas,Europe and Asia(CCPS,2009),but nothing about Mexico has been reported.3.HAZOP variationsIn the technical literature there is no consensus in the HAZOP studies procedure,from the several differences it is consider that the more important are the variations according to:(DÂD)or (CÂC).Table1shows HAZOP variations,where(CQÂCQ)means Consequence by Consequence analysis.The implications of choosing(CÂC)are that in this approach there are obtained unique relationships of Consequences,Safeguards and Recommendations,for each specific Cause of a given Deviation. For(DÂD),all Causes,Consequences,Safeguards and Recommenda-tions are related only to one particular Deviation,thus producing that not all Causes appear to produce all the Consequences.In practice HAZOP approach(DÂD)can optimize analysis time development.However,its drawback comes when HAZOP includes risk ranking since it cannot be determined easily which Cause to consider in probability assignment.In choosing(CÂC)HAZOP there is no such a problem,although it may take more time on the analysis.The HAZOP team leader should agree HAZOP approach with customer and communicate this to the HAZOP team.In our experience factors to consider when choosing HAZOP approach are:1.If HAZOP will be followed by Layers of Protection Analysis(LOPA)for Safety Integrity Level(SIL)selection,then choose (CÂC).2.If HAZOP is going to be the only hazard identification study,it isworth to make it with major detail using(CÂC).3.If HAZOP is part of an environmental risk study that requires aConsequence analysis,then use(DÂD).4.If HAZOP is going to be done with limited time or becauseHAZOP team cannot spend too much time in the analysis,then use(DÂD).Although this is not desirable since may compro-mise process safety.Regarding risk ranking in HAZOP,looking at IEC standard(IEC, 2001)it is found that HAZOP studies there are(DÂD)it refers to (IEC,1995)in considering deviation ranking in accordance to their severity or on their relative risk.One advantage of risk ranking is that presentation of HAZOP results is very convenient,in particular when informing the management on the recommendations to be followedfirst or with higher priority as a function of risk evaluated by the HAZOP team regarding associated Cause with a given recommendation.Tables2and3are shown as illustrative example of the convenience of event risk ranking under HAZOP,showing no risk ranking in Table2and risk ranking in Table3.When HAZOP presents a list of recommendations without ranking,the management can focus to recommendations with perhaps the lower resource needs and not necessarily the ones with higher risk.Table1Main approaches in HAZOP studies.Source HAZOP approach(Crowl&Louvar,2011)(DÂD)(ABS,2004)(CÂC)&(DÂD)(Hyatt,2003)(CÂC),(DÂD)&(CQÂCQ) (IEC,2001)(DÂD)(CCPS,2008);(Crawley,Preston,& Tyler,2008)(DÂD),(CÂC)Table2HAZOP recommendations without risk ranking.DescriptionRecommendation1Recommendation2Recommendation3Recommendation4Recommendation5Table3HAZOP recommendations with risk ranking.Scenario risk DescriptionHigh Recommendation2High Recommendation5Medium Recommendation3Low Recommendation1Low Recommendation4M.Pérez-Marín,M.A.Rodríguez-Toral/Journal of Loss Prevention in the Process Industries26(2013)936e940937As can be seen in Tables 2and 3,for the management there will be more important to know HAZOP results as in Table 3,in order to take decisions on planning response according to ranking risk.4.HAZOP standard for the Mexican oil &gas industryLooking at the worldwide recognized guidelines for hazard identi fication (ISO,2000)there is mentioned that when consid-ering scenarios qualitative risk assignment,one may use risk matrix for comparing the importance of risk reduction measures of the different options,but there is not a speci fic risk matrix with risk values to consider.In Mexico there exist two national standards were tolerable and intolerable risk is de fined,one is the Mexican National Standard NOM-028(NOM,2005)and the other is PEMEX corporate standard NRF-018(PEMEX,2008).In both Mexican standards the matrix form is considered for relating frequency and consequences.Fig.1shows the risk matrix in (NOM,2005),nomenclature regarding letters in this matrix is described in Tables 4e 6.It can be mentioned that risk matrix in (NOM,2005)is optional for risk management in local chemical process plants.For Mexican oil &gas industry,there exist a PEMEX corporate standard (NRF),Fig.2,shows the corresponding risk matrix (PEMEX,2008).Nomenclature regarding letters in this matrix is described in Tables 7e 9for risk concerning the community.It is important to mention that PEMEX corporate standard considers environmental risks,business risks,and corporate image risks.These are not shown here for space limitations.The Mexican National Standard (NOM)as being of general applicability gives the possibility for single entities (like PEMEX)to determine its own risk criteria as this company opted to do.PEMEX risk matrix can be converted to NOM ’s by category ’s grouping infrequency categories,thus giving same flexibility,but with risk speci fic for local industry acceptance risk criteria.One principal consideration in ranking risk is to de fine if ranking is done before safeguards de finition or after.This de finition is relevant in:HAZOP kick-off presentation by HAZOP leader,explaining im-plications of risk ranking.HAZOP schedule de finition.Risk ranking at this point takes shorter time since time is not consumed in estimating risk reduction for each safeguard.If after HAZOP a LOPA is going to be done,then it should be advisable to request that HAZOP leader considers risk ranking before safeguards de finition,since LOPA has established rules in de fining which safeguards are protections and the given risk reduction.Otherwise if for time or resource limitations HAZOP is not going to be followed by LOPA,then HAZOP should consider risk ranking after safeguards de finition.Therefore,the HAZOP leader should explain to the HAZOP team at the kick-off meeting a concise explanation of necessary considerations to identify safeguards having criteria to distinguish them as Independent Protection Layers (IPL)as well as the risk reduction provided by each IPL.In HAZOP report there should be make clear all assumptions and credits given to the Protections identi fied by the HAZOP team.Figs.3and 4,shows a vision of both kinds of HAZOP reports:For the case of risk ranking before and after safeguards de finition.In Figs.3Fig.1.Risk matrix in (NOM,2005).Table 5Probability description (Y -axis of matrix in Fig.1)(NOM,2005).Frequency Frequency quantitative criteria L41in 10years L31in 100years L21in 1000years L1<1in 1000yearsTable 6Risk description (within matrix in Fig.1)(NOM,2005).Risk level Risk qualitative descriptionA Intolerable:risk must be reduced.B Undesirable:risk reduction required or a more rigorous risk estimation.C Tolerable risk:risk reduction is needed.DTolerable risk:risk reduction not needed.Fig.2.Risk matrix as in (PEMEX,2008).Table 7Probability description (Y -axis of matrix in Fig.2)(PEMEX,2008).Frequency Occurrence criteria Category Type Quantitative QualitativeHighF4>10À1>1in 10yearsEvent can be presented within the next 10years.Medium F310À1À10À21in 10years e 1in 100years It can occur at least once in facility lifetime.LowF210À2À10À31in 100years e 1in 1000years Possible,it has never occurred in the facility,but probably ithas occurred in a similar facility.Remote F1<10À3<1in 1000years Virtually impossible.It is norealistic its occurrence.Table 4Consequences description (X -axis of matrix in Fig.1)(NOM,2005).Consequences Consequence quantitative criteriaC4One or more fatalities (on site).Injuries or fatalities in the community (off-site).C3Permanent damage in a speci fic Process or construction area.Several disability accidents or hospitalization.C2One disability accident.Multiple injuries.C1One injured.Emergency response without injuries.M.Pérez-Marín,M.A.Rodríguez-Toral /Journal of Loss Prevention in the Process Industries 26(2013)936e 940938and4“F”means frequency,C means consequence and R is risk as a function of“F”and“C”.One disadvantage of risk ranking before safeguards definition is that resulting risks usually are found to be High,Intolerable or Unacceptable.This makes difficult the decision to be made by the management on what recommendations should be carried outfirst and which can wait.One advantage in risk ranking after safeguards definition is that it allows to show the management the risk scenario fully classified, without any tendency for identifying most risk as High(Intolerable or Unacceptable).In this way,the management will have a good description on which scenario need prompt attention and thus take risk to tolerable levels.There is commercial software for HAZOP methodology,but it normally requires the user to use his/her risk matrix,since risk matrix definition represents an extensive knowledge,resources and consensus to be recognized.The Mexican case is worldwide unique in HAZOP methodology, since it uses an agreed and recognized risk matrix and risk priori-tizing criteria according to local culture and risk understanding for the oil&gas sector.The risk matrix with corresponding risk levels took into account political,economical and ethic values.Advantages in using risk matrix in HAZOP are:they are easy to understand and to apply;once they are established and recognized they are of low cost;they allow risk ranking,thus helping risk reduction requirements and limitations.However,some disad-vantages in risk matrix use are:it may sometimes be difficult to separate frequency categories,for instance it may not be easy to separate low from remote in Table7.The risk matrix subdivision may have important uncertainties,because there are qualitative considerations in its definition.Thus,it may be advantageous to update Pemex corporate HAZOP standard(PEMEX,2008)to consider a6Â6matrix instead of the current4Â4matrix.5.ConclusionsHAZOP studies are not a simple procedure application that as-sures safe Process systems on its own.It is part of a global design cycle.Thus,it is necessary to establish beforehand the HAZOP study scope that should include at least:methodology,type(CÂC,DÂD, etc.)report format,acceptance risk criteria and expected results.Mexico belongs to the reduced number of places where accep-tance risk criteria has been explicitly defined for HAZOP studies at national level.ReferencesABS.(2004).Process safety institute.Course103“Process hazard analysis leader training,using the HAZOP and what-if/checklist techniques”.Houston TX:Amer-ican Bureau of Shipping.CCPS(Center for Chemical Process Safety).(2001).Layer of protection analysis: Simplified process risk assessment.New York,USA:AIChE.CCPS(Center for Chemical Process Safety).(2008).Guidelines for hazard evaluation procedures(3rd ed.).New York,USA:AIChE/John Wiley&Sons.CCPS(Center for Chemical Process Safety).(2009).Guidelines for Developing Quan-titative Safety Risk Criteria,Appendix B.Survey of worldwide risk criteria appli-cations.New York,USA:AIChE.Crawley,F.,Preston,M.,&Tyler,B.(2008).HAZOP:Guide to best practice(2nd ed.).UK:Institution of Chemical Engineers.Crowl,D.A.,&Louvar,J.F.(2011).Chemical process safety,fundamentals with ap-plications(3rd ed.).New Jersey,USA:Prentice Hall.Table8Consequences description(X-axis of matrix in Fig.2)(PEMEX,2008).Event type and consequence categoryEffect:Minor C1Moderate C2Serious C3Catastrophic C4 To peopleNeighbors Health and Safety.No impact on publichealth and safety.Neighborhood alert;potentialimpact to public health and safety.Evacuation;Minor injuries or moderateconsequence on public health and safety;side-effects cost between5and10millionMX$(0.38e0.76million US$).Evacuation;injured people;one ormore fatalities;sever consequenceon public health and safety;injuriesand side-consequence cost over10million MX$(0.76million US$).Health and Safetyof employees,serviceproviders/contractors.No injuries;first aid.Medical treatment;Minor injurieswithout disability to work;reversible health treatment.Hospitalization;multiple injured people;total or partial disability;moderate healthtreatment.One o more fatalities;Severe injurieswith irreversible damages;permanenttotal or partial incapacity.Table9Risk description(within matrix in Fig.2)(PEMEX,2008).Risk level Risk description Risk qualitative descriptionA Intolerable Risk requires immediate action;cost should not be a limitation and doing nothing is not an acceptable option.Risk with level“A”represents an emergency situation and there should be implements with immediate temporary controls.Risk mitigation should bedone by engineered controls and/or human factors until Risk is reduced to type“C”or preferably to type“D”in less than90days.B Undesirable Risk should be reduced and there should be additional investigation.However,corrective actions should be taken within the next90days.If solution takes longer there should be installed on-site immediate temporary controls for risk reduction.C Acceptablewith control Significant risk,but can be compensated with corrective actions during programmed facilities shutdown,to avoid interruption of work plans and extra-costs.Solutions measures to solve riskfindings should be done within18months.Mitigation actions should focus operations discipline and protection systems reliability.D ReasonablyacceptableRisk requires control,but it is of low impact and its attention can be carried out along with other operations improvements.Fig.3.Risk ranking before safeguard definition.Fig.4.Risk ranking after safeguards definition.M.Pérez-Marín,M.A.Rodríguez-Toral/Journal of Loss Prevention in the Process Industries26(2013)936e940939Dunjó,J.,Fthenakis,V.,Vílchez,J.A.,&Arnaldos,J.(2010).Hazard and opera-bility(HAZOP)analysis.A literature review.Journal of Hazardous Materials, 173,19e32.Goyal,R.K.,&Kugan,S.(2012).Hazard and operability studies(HAZOP)e best practices adopted by BAPCO(Barahin Petroleum Company).In Presented at SPE middle east health,safety,security and environment conference and exhibition.Abu Dhabi,UAE.2e4April.Gujar,A.M.(1996).Myths of HAZOP and HAZAN.Journal of Loss Prevention in the Process Industry,9(6),357e361.Hyatt,N.(2003).Guidelines for process hazards analysis,hazards identification and risk analysis(pp.6-7e6-9).Ontario,Canada:CRC Press.IEC.(1995).IEC60300-3-9:1995.Risk management.Guide to risk analysis of techno-logical systems.Dependability management e Part3:Application guide e Section 9:Risk analysis of technological systems.Geneva:International Electrotechnical Commission.IEC.(2001).IEC61882.Hazard and operability studies(HAZOP studies)e Application guide.Geneva:International Electrotechnical Commission.ISO.(2000).ISO17776.Guidelines on tools and techniques for hazard identification and risk assessment.Geneva:International Organization for Standardization.Johnson,R.W.(2010).Beyond-compliance uses of HAZOP/LOPA studies.Journal of Loss Prevention in the Process Industries,23(6),727e733.Khan,F.I.(1997).OptHAZOP-effective and optimum approach for HAZOP study.Journal of Loss Prevention in the Process Industry,10(3),191e204.Kuo,D.H.,Hsu,D.S.,&Chang,C.T.(1997).A prototype for integrating automatic fault tree/event tree/HAZOP puters&Chemical Engineering,21(9e10),S923e S928.Lawley,H.G.(1974).Operability studies and hazard analysis.Chemical Engineering Progress,70(4),45e56.Mannan,S.(2012).Lee’s loss prevention in the process industries.Hazard identifica-tion,assessment and control,Vol.1,3rd ed.,Elsevier,(pp.8e31).NOM.(2005).NOM-028-STPS-2004.Mexican National standard:“Norma Oficial Mexicana”.In Organización del trabajo-Seguridad en los procesos de sustancias químicas:(in Spanish),published in January2005.PEMEX.(2008).Corporate Standard:“Norma de Referencia NRF-018-PEMEX-2007“Estudios de Riesgo”(in Spanish),published in January2008. Venkatasubramanian,V.,Zhao,J.,&Viswanathan,S.(2000).Intelligent systems for HAZOP analysis of complex process puters&Chemical Engineering, 24(9e10),2291e2302.M.Pérez-Marín,M.A.Rodríguez-Toral/Journal of Loss Prevention in the Process Industries26(2013)936e940 940。
高三英语学术研究方法创新不断单选题30题

高三英语学术研究方法创新不断单选题30题1.In academic research, a thorough literature review is ______ essential step.A.anB.aC.theD./答案:A。
本题考查冠词的用法。
“essential”是以元音音素开头的单词,所以用“an”。
“a”用于辅音音素开头的单词前;“the”表示特指;“/”即零冠词,此处需要一个不定冠词来表示“一个”的意思,且“essential”以元音音素开头,所以选“A”。
2.______ successful academic research requires careful planning and dedication.A.AB.AnC.TheD./答案:D。
本题考查零冠词的用法。
“successful academic research”在此处是泛指学术研究,不是特指某一项学术研究,也不是可数名词单数需用不定冠词修饰的情况,所以用零冠词“/”。
3.At the heart of academic research is ______ pursuit of knowledge.A.aC.theD./答案:C。
本题考查定冠词的用法。
“the pursuit of knowledge”表示“对知识的追求”,是特指的概念,所以用“the”。
4.Researchers need ______ accurate data to draw valid conclusions.A.anB.aC.theD./答案:D。
本题考查零冠词的用法。
“data”在此处是不可数名词,且不是特指某一特定的数据,所以用零冠词“/”。
5.______ innovation is crucial in academic research.A.AnB.AC.TheD./答案:D。
本题考查零冠词的用法。
“innovation”在此处是泛指创新,不是特指某一个创新,也不是可数名词单数需用不定冠词修饰的情况,所以用零冠词“/”。
Timed games with branching-time winning conditions

Timed games with branching-time winning conditionsAniello MuranoDipartimento di Informatica ed Applicazioni,Universit`a degli Studi di Salerno,Via S.Allende,84081Baronissi(SA){murano}@dia.unisa.itThe theory of games,traditionally related to the economic-theoretic environment(see for instance [19]),has been attracting the interest of many researchers in both computer science and control theory. The notion of a game naturally arises in the verification of reactive systems and program synthesis [4,21].In the compositional approach,a reactive system is seen as a set of interacting components, each of them is modelled as an open system(that is,a system whose behaviour depends on the current state as well as the behaviour of the environment in which it is embedded).The interaction between a component and the rest of the system,or the interaction between a controller and the related plant, both can be modeled as a two-player game.For verifying closed systems,where a system behaviour is completely determined by the current state,model-checking is a very successful technique[6,7,8]. The equivalent of model-checking for open systems is checking for the existence of a winning strategy, according to a given winning condition,in a two-player game[15].In this paper we focus on the verification of open real-time systems,modeled as nondeterministic timed automata[2]:finite automata augmented with afinite set of real-valued clocks.The transitions of a timed automaton are enabled according to the current state,that is,the current location and the current clock values.In a transition,clocks can be instantaneously reset.The value of a clock is exactly the time elapsed since the last time it was reset.A clock constraint(guard)is associated to each transition with the meaning that a transition can be taken only if the associated guard is enabled.A clock constraint(invariant)can also be associated to each location with the meaning that the automaton can stay in a location as long as the corresponding invariant remains true.When interpreting a nondeterministic timed automaton as a(timed)game graph,we capture the choices of the protagonist by the symbols associated with the transitions and nondeterminism is used to model the possible choices of the antagonist.To model the case that the protagonist stays idle and the antagonist is moving,we use a special symbol denoted byξ.The case that both players stay idle is captured by letting time elapse in a location.A play of a timed game is thus constructed in the following way.At each time,a player declares the time it will wait idling,along with its next choice. At the time one of the players or both move,both players are allowed to redeclare their next move and the time they will issue it.Technically,a play is a run of the automaton modeling the game.A game is given by a game graph along with a winning condition that establishes which computations are winning for the protagonist.In this paper we solve Ctl and Tctl games,that is,timed games with1a winning condition given,respectively,by a formula of the branching-time temporal logic Ctl[6],or its real-time extension Tctl[1].C tl Timed Games.A way to decide discrete games is to reduce them to the emptiness problem for tree automata(see,for example[22]).Here,we extend the automata-theoretic approach to solve Ctl timed games.Given a timed game(G,ϕ),where G is a game graph andϕa Ctl formula, we construct a tree automaton A G that accepts all theω–trees corresponding to a strategy of the protagonist.We construct this automaton exploiting the clock region relation[2].Then,we construct another tree automaton Aϕaccepting all the trees satisfying the property expressed byϕ.Since strategies of the protagonist correspond to trees accepted by A G,to construct Aϕwe only need to capture models with arity bounded above by the arity of A G(i.e.the maximum branching degree of A G transitions).Thus,there exists a winning strategy of the protagonist in the game(G,ϕ) if and only if the intersection between the languages accepted by A G and Aϕis not empty.Since the size of Aϕis exponential in the size ofϕ[25],checking the emptiness for a B¨u chi tree automaton is polynomial in the number of its states[26],and the intersection of the languages accepted by A G and Aϕis accepted by a B¨u chi tree automaton whose number of states is exponential in the number of states of A G and Aϕ[23],we obtain an algorithm that takes exponential time.Since timed reachability games are known to be Exptime-hard[14],we have that our result is complete.T ctl Timed Games.The syntax of Tctl is given by augmenting the temporal operators of Ctl(except for the“next”,which is discarded since it does not have a clear meaning in a dense time domain)with a timing constraint of type≈c,where≈is one among<,≤,>,≥,and=,and c is a natural number.While Ctl allows to express qualitative temporal requirements on the occurrence of events,with Tctl it is also possible to express quantitative requirements.For example,using Ctl we can express assertion like“p1is true until p2is true”,but we cannot express assertion like“p1is true until p2is true within time2”,which can be expressed in Tctl.In Tctl timed games,thus,we allow dense real-time in both the game graph and the winning condition.Model-checking of Tctl-formulas is Pspace-complete while checking for their satisfiability is undecidable[1].In a recent paper,the satisfiability of Tctl is proved to be Exptime-complete if equality is not allowed in timing constraints of the formulas[16].Here we obtain the same complex-ity/decidability result for games.We prove that Tctl games are Exptime-complete,if equality is not allowed in timing constraints.Our approach to solve Tctl games relies on a reduction to the emptiness problem of timed B¨u chi tree automata.Given a timed automaton G and a Tctl–formula ϕ,we solve the game(G,ϕ)by constructing two timed B¨u chi tree automata:A G and Aϕ.The auto-maton A G accepts,for each strategy F of the protagonist,a timedω-tree which is“embedded”into F.The automaton Aϕaccepts instead all the models ofϕwith arity bounded above by the arity of G.By the known results on timed B¨u chi tree automata[17],the intersection of A G and Aϕis still a timed B¨u chi tree automaton,and accepts all the trees that satisfyϕand are embedded into a strategy.Thus,a timed game G admits a winning strategy with respect to a formulaϕif and only if the language accepted by the intersection automaton is not empty.Denoting the product of the sizes ofϕand G by K,we have that the number of states of the intersection automaton is exponential in K while the size of its clock constraints is linear in K.Since checking the emptiness for a timed B¨u chi tree automaton is polynomial in the number of states and exponential in clock size[17],we obtain an algorithm that takes exponential time.The result we obtain is tight,in the sense that Tctl games2are Exptime-complete(hardness is a consequence of the Exptime-hardness of the reachability timed games[14]).Finally,by allowing equality in the timing constraints,Tctl games turns out to be undecidable. It is possible to prove this result by reducing the satisfiability problem of Tctl–formulas,which is known to be undecidable[1]to our problem.Another approach to prove this result is to introduce the module-checking problem of Tctl–formulas in dense real-time,and prove that this problem is undecidable when equality is allowed in the timing constraints[18].Here,the proof is obtained by reducing the problem of deciding whether a nondeterministic two-counter machine has a recurring computation(this problem is known to beΣ11-hard[12]).Games have been studied in the context of discrete,timed,and hybrid systems(see[5,13,21,24]. In literature,different formulations of games with winning conditions expressed by temporal logics have been considered.In[3],alternating temporal logic is introduced.In[15]the model checking of open systems(module checking)is studied.This problem turns out to be Exptime-compete for specification given by Ctl-formulas and2Exptime-complete for specification given by Ctl*-formulas. Rectangular hybrid games with winning conditions expressed by Ltl formulas were solved in[13].In [9],the controller synthesis of timed automata with linear-time specifications given also as timed automata is addressed.Finally,in[10]an automata-theoretic approach to solve Ltl timed games is also considered.Acknowledgments.This abstract contains results obtained during my Ph.D.studies and have been obtained in collaboration with other researchers that I would like to thank:Moshe Vardi, Marherita Napoli,Salvatore La Torre,Marco Faella.References[1]R.Alur,C.Courcoubetis,and D.L.Dill.Model-checking in dense rmation andComputation,104(1):2–34,1993.[2]R.Alur and D.L.Dill.A theory of timed automata.Theoretical Computer Science,126:183–235,1994.[3]R.Alur,T.A.Henzinger,and O.Kupferman.Alternating-time temporal logic.In Proc.of the38thIEEE Symposium on Foundations of Computer Science,pages100–109,1997.[4]M.Abadi,mport,and P.Wolper.Realizable and unrealizable specifications of reactive systems.In Proc.of the16th Intern.Colloquium on Automata,Languages and Programming,ICALP’89,LNCS372,pages1–17,1989.[5]E.Asarin,O.Maler,A.Pnueli,and J.Sifakis.Controller synthesis for timed automata.In Proc.IFAC Symposium on System Structure and Control,pages469–474.Elsevier,1998.[6]E.A.Emerson and ing branching-time temporal logic to synthesize synchronizationskeletons.Science of Computer Programming,2:241–266,1982.[7]E.M.Clarke,E.A.Emerson,A.P.Sistla.Automatic verification offinite-state concurrent systemsusing temporal-logic specifications.ACM Transactions on Programming Languages and Systems,v.8,2:244-263,19863[8]E.M.Clarke,puter-aided verification.IEEE Spectrum,v.33,6:61–67,1996[9]D.D’Souza,P.Madhusudan.Timed control synthesis for external specifications.In Proc.of the19th Annual Symposium on Theoretical Aspects of Computer Science,(STACS’02),LNCS2285:571–582,2002.[10]M.Faella, Torre,and A.Murano.Automata-theoretic decision of timed games.In Proc.of the3rd International Workshop on Verification,Model Checking,and Abstract Interpretation,VMCAI 2002,LNCS2294,pages94–108.Springer-Verlag,2000.[11]M.Faella, Torre and A.Murano.Dense Real-Time Games.Proc.of the Seventeenth AnnualIEEE Symposium on Logic in Computer Science(LICS02),pages167–176,2002.[12]D.Harel,A.Pnueli,J.Stavi.Propositional Dynamic Logic of Regular Programs”,In Journal ofComputer and System Sciences,26:222-243,1983[13]T.A.Henzinger,B.Horowitz,and R.Majumdar.Rectangular hybrid games.In Proc.of the10thInternational Conference on Concurrency Theory,CONCUR’99,LNCS1664,pages320–335,1999.[14]T.Henzinger and P.Kopke.Discrete-time control for rectangular hybrid automata.TheoreticalComputer Science,221(1–2):369–392,1999[15]O.Kupferman and M.Y.Vardi.Module checking.In Computer Aided Verification,Proc.Eighth Int.Workshop,LNCS1102,pages75–86.Springer-Verlag,1996.[16] Torre and M.Napoli.A decidable dense branching-time temporal logic.In Proc.of the20th Conference on the Foundations of Software Technology and Theoretical Computer Science, FSTTCS’00,LNCS1974,pages139–150.Springer-Verlag,2000.[17] Torre and M.Napoli.Timed tree automata with an application to temporal logic.ActaInformatica,38(2):89–116,2001.[18]A.Murano and M.Vardi.Real-time module checking.Work in progress.[19]J.Von Neumann and O.Morgenstern.Theory of Games and Economic Behavior.Princeton Uni-versity Press,1944.[20]A.Pnueli.The temporal logic of programs.In Proc.of the18th IEEE Symposium on Foundationsof Computer Science,pages46–77,1977.[21]A.Pnueli and R.Rosner.On the synthesis of a reactive module.In Proc.of the16th ACM Symposiumon Principles of Programming Languages,pages179–190,1989.[22]M.O.Rabin.Automata on infinite objects and Church’s problem.Trans.Amer.Math.Soc.,1972.[23]W.Thomas.Automata on infinite objects.In J.van Leeuwen,editor,Handbook of TheoreticalComputer Science,volume B,pages133–191.Elsevier Science Publishers,1990.[24]W.Thomas.On the synthesis of strategies in infinite games.In Ernst W.Mayr and Claude Puech,editors,12th Annual Symposium on Theoretical Aspects of Computer Science,STACS’95,LNCS 900,pages1–13.Springer-Verlag,1995.[25]M.Y.Vardi and P.Wolper.Automata-theoretic techniques for modal logics of programs.Journal ofComputer and System Sciences,32:182–211,1986.[26]M.Y.Vardi and P.Wolper.Reasoning about infinite rmation and Computation,115:1–37,1994.4。
高三英语学术道德单选题20题

高三英语学术道德单选题20题1. In academic research, one should always be honest. If you copy someone else's work without giving credit, it's considered ______.A. plagiarismB. innovationC. inspirationD. creation答案:A。
本题考查学术道德中的概念。
选项A“plagiarism”意为“抄袭”,符合不诚实的行为描述。
选项B“innovation”指“创新”,与抄袭相反。
选项C“inspiration”意为“灵感”,并非抄袭行为。
选项D“creation”指“创造”,也与抄袭无关。
2. When conducting a scientific experiment, it's crucial to report the results truthfully. Falsifying data is a serious violation of ______.A. academic integrityB. academic freedomC. academic successD. academic difficulty答案:A。
此题重点在学术道德的概念。
选项A“academic integrity”即“学术诚信”,伪造数据违反了学术诚信。
选项B“academic freedom”是“学术自由”,与题目不符。
选项C“academic success”指“学术成功”,并非重点。
选项D“academic difficulty”意为“学术困难”,与题目无关。
3. In an academic paper, if you claim someone else's ideas as yourown, this is a form of ______.A. citationB. referenceC. dishonestyD. honesty答案:C。
阿列纽斯定律 Activation energy, Arrhenius law

On this page:Reaction mechanisms, molecularity Collision theory of chemical changeAnatomy of moleclar collisions Activation energy CatalystsTemperature and kinetic energyThe Arrhenius lawDeterining the activation energy The pre-exponential factorWhat you should be able to do Concept mapGeneral Chemistry Virtual Textbook → kinetics/dynamics → collision /activatonCollision and activationthe Arrhenius Lawindex | rate laws 1 | rate laws 2 | activation | mechanisms | solutions | catalysis | experimentalNote: this document will print in an appropriately modified format (15 pages)Why are some reactions so much faster than others, and why are reaction rates independent of the thermodynamictendency of the reaction to take place?These are the central questions weaddress in this unit. In doing so, we open the door to the important topic ofreaction mechanisms : what happens at the microscopic level when chemical reactions take place? We can thank Prof. Svante Arrhenius for unlocking this door!To keep things as simple as possible, we will restrict ourself to reactions that take place in the gas phase.The same principles will apply to reactions in liquids and solids, but with addedcomplications that we will discuss in a later unit.1 Reaction mechanismsThe mechanism of a chemical reaction is the sequence of actual events that take place as reactant molecules are converted into products. Each of these events constitutes an elementary step that can be represented as a coming-together of discrete particles ("collison") or as the breaking-up of a molecule ("dissociation") into simpler units. The molecular entity that emerges from each step may be a final product of the reaction, or it might be an intermediate — a species that is created in one elementary step and destroyed in a subsequent step, and therefore does not appear in the net reaction equation.Step by step...A reaction mechanism must ultimately be understood as a "blow-by-blow"description of the molecular-level events whose sequence leads from reactants to products. These elementary steps (also called elementary reactions ) are almost always very simple ones involving one, two, or [rarely] three chemical species which are classified, respectively, asunimolecular A →by far the most common bimolecular A + B →termolecularA +B +C →very rareIn a gas at roomtemperature and normal atmospheric pressure,there will be about 1033collisions in each cubic centimetre every second.If every collision between two reactant molecules yielded products, all reactions would becomplete in a fraction of a second.2 Collision theory of chemical changeMolecules must collide before they can reactThis fundamental rule must guide any analysis of an ordinary chemical reaction mechanism.This explains why termolecular processes are so uncommon. The kinetictheory of gases tells us that for every 1000 binary collisions, there will be only one event in which three molecules simultaneously come together. Four-way collisions are so improbable that this process has never been demonstrated in an elementary reaction.Consider a simple bimolecular stepA +B → productsClearly, if two molecules A and B are to react, they must approach closely enough to disrupt some of their existing bonds and to permit the creation of any new ones that are needed in the products. We call such an encounter a collision .The frequency of collisions between A and B in a gas will be proportional to the concentration of each; if we double [A], the frequency of A-Bcollisions will double, and doubling [B] will have the same effect. So if all collisions lead to products, than the rate of a bimolecular process will be first-order in A and B, or second-order overall:rate = k [A][B]but...Not all collisions are equalWhen two billiard balls collide, they simply bounce off of each other. This is also the most likely outcome if the reaction between A and B requires a significant disruption or rearrangement of the bonds between their atoms.In order to effectively initiate a reaction, collisions must be sufficiently energetic (kinetic energy) to bring about this bond disruption. More about this further on.And there is often one additional requirement. In many reactions,especially those involving more complex molecules, the reacting species must be oriented in amanner that is appropriate for the particular process. For example, in the gas-phase reaction ofdinitrogen oxide with nitric oxide,the oxygen end of N 2O must hit thenitrogen end of NO; reversing theorientation of either molecule prevents the reaction.Owing to the extensive randomization of molecular motions in a gas or liquid,there are always enough correctly-oriented molecules for some of the molecules to react. But of course, the more critical this orientational requirement is, the fewer collisions will be effective.Anatomy of a collisionEnergetic collisions between molecules cause interatomic bonds to stretch and bend farther, temporarily weakening them so that they become moresusceptible to cleavage. Distortion of the bonds can expose their associated electron clouds to interactions with other reactants that might lead to the formation of new bonds.Chemical bonds have some of the properties ofmechanical springs, whose potential energydepends on the extent to which they arestretched or compressed. Each atom-to-atombond can be described by a potential energydiagram that shows how its energy changeswith its length. When the bond absorbs energy(either from heating or through a collision), it iselevated to a higher quantized vibrational state(indicated by the horizontal lines) that weakensthe bond as its length oscillates between theextended limits corresponding to the curve.A particular collision will typically excite a number of bonds in this way. Within about 10–13 second this excitation gets distributed among the other bonds in the molecule in rather complex and unpredictable ways that can concentrate the added energy at a particularly vulnerable point. The affected bond can stretch and bend farther, making it more susceptible to cleavage. Even if the bond does not break by pure stretching, it can become distorted or twisted so as to expose nearby electron clouds to interactions with other reactants that might encourage a reaction.Consider, for example, the isomerization of cyclopropane to propene which takes place at fairly high temperatures in the gas phase.We can imagine the collision-to-product sequence in the following [grossly oversimplified] way:Note thatTo keep things simple, we do not show the hydrogen atoms here. This isreasonable because C–C bonds are weaker then C–H bonds and thus less likely to be affected.The collision at will usually be with another cyclopropane molecule, butbecause no part of the colliding molecule gets incorporated into the product, it can in principle be a noble gas or some other non-reacting species;Although the C–C bonds in cyclopropane are all identicial, the instantaneouslocalization of the collisional energy can distort the molecule in various ways (), leading to a configuration sufficiently unstable to initiate the rearrangement to the product.Unimolecular processes also begin with a collisionThe cyclopropane isomerization described above is typical of many decomposition reactions that are found to follow first-order kinetics, implying that the process is unimolecular. Until about 1921, chemists did not understand the role of collisions in unimolecular processes. It turns out that the mechanisms of such reactions are really rather complicated, andThe chemical reactions associated with most food spoilage are catalyzed by enzymes produced by the bacteria which mediatethese processes.Here is a short YouTube video on activation energy.The "reaction coordinate " plotted along the abscissa represents the changes in atomic coordinates as thesystem progresses from reactants to products. In the very simplest elementary reactions it might correspond to the stretching or twisting of a particular bond, and be shown to a scale. In general, however, the reaction coordinate is a rather abstract concept that cannot be tied to any single measurable and scaleable quantity.The activated complex (also known as the transition state ) represents the structure of the system as it exists at the peak of the activation energy curve. It does not correpond to an identifiable intermediate structure (which would more properly be considered the product of a separate elementary process), but rather to whatever configuration of atoms exists during the collision, which lasts for only about 0.1 picosecond.Activation energy diagrams always incorporate the energetics (ΔU or ΔH ) of the net reaction, but it isimportant to understand that the latter quantities depend solely on the thermodynamics of the process which are always independent of the reaction pathway. This means that the same reaction can exhibit different activation energies if it can follow alternative pathways.With a few exceptions for very simple processes,activation energy diagrams are largely conceptual constructs based on our standard collision model forchemical reactions. It would be unwise to read too much into them.that at very low pressures they do follow second-order kinetics. Suchreactions are more properly described as pseudounimolecular . The details are beyond the scope of this course, but a good introduction can be found on this U. Arizona page .Activation energyHigher temperatures, faster reactionsIt is common knowledge that chemical reactions occur more rapidly at higher temperatures. Everyone knows that milk turns sour much more rapidly if stored at room temperature rather than in a refrigerator, butter goes rancid more quickly in the summer than in the winter, and eggs hard-boil more quickly at sea level than in the mountains. For the same reason, cold-blooded animals such as reptiles and insects tend to be noticeably more lethargic on cold days.It is not hard to understand why this should be. Thermal energy relates direction to motion at the molecular level. As the temperature rises,molecules move faster and collide more vigorously, greatly increasing the likelyhood of bond cleavages and rearrangemens as described above.Activation energy diagramsMost reactions involving neutral molecules cannot take place at all until they have acquired the energy needed to stretch, bend, or otherwisedistort one or more bonds. This critical energy is known as the activation energy of the reaction. Activation energy diagrams of the kind shown below plot the total energy input to a reaction system as it proceeds from reactants to products.In examining such diagrams, take special note of the following:Gallery of activation energy plotsActivation energy diagrams can describe both exothermic and endothermic reactions:... and the activation energies of the forward reaction can be large, small, or zero (independently, of course, of the value of ΔH):Processes with zero activation energy mostcommonly involve the combination ofoppositely-charged ions or the pairing up ofelectrons in free radicals, as in thedimerization of nitric oxide (which is an odd-electron molecule).In this plot for the dissociation ofbromine, the E a is just the enthalpyof atomizationBr2(g)→ 2 Br· (g)and the reaction coordinatecorresponds roughly to thestretching of the vibrationally-excited bond. The "activatedcomplex", if it is considered toexist, is just the last, longest"stretch". The reverse reaction,being the recombination of tworadicals, occurs immediately oncontact.Where does the activation energy come from?In most cases, the activation energy issupplied by thermal energy, eitherthrough intermoleculr collisions or (inthe case of thermal dissocation) bythermal excitation of a bond-stretchingA review of the principles of gas molecular velocities and the Boltzmann distribution can be found on the "KMT-classic" page .vibration to a sufficiently high quantum level.As products are formed, the activation energy is returned in the form of vibrational energy which is quickly degraded to heat.It's worth noting, however, that other sources of activation energy are sometimes applicable:Absorption of light by a molecule (photoexcitation) can be a very clean and efficient, but it doesn't always work. It's not enough that the wavelength of the light correspond to the activation energy; it must also fall within the absorption spectrum of the molecule, and (in a complex molecule) enough of it must end up in the right part of the molecule, such as in a particular bond.Electrochemical activation . Molecules capable of losing or gaining electrons at the surface of an electrode can undergo activation from an extra potential (known as the overvoltage ) between the electrode and the solution. The electrode surface often plays an active role, so the process is also known as electrocatalysis .Catalysts can reduce activation energyA catalyst is usually defined as a substance that speeds up a reaction without being consumed by it. More specifically, a catalyst provides an alternative, lower activation energypathway between reactants and products. As such, they are vitally important to chemical technology; approximately 95%of industrial chemical processes involve catalysts of various kind. In addition, most biochemical processes that occur inliving organisms are mediated by enzymes , which are catalysts made of proteins.It is important to understand that a catalyst affects only the kinetics of a reaction; it does not alter the thermodynamictendency for the reaction to occur. Thus there is a single value of ΔH for the two pathways depicted in the plot on the right.Temperature and kinetic energyIn the vast majority of cases, we depend on thermal actvation, so the major factor we need to consider is what fraction of the molecules possess enough kinetic energy to react at a given temperature.According to kinetic molecular theory, a population of molecules at a given temperature is distributed over a variety of kinetic energies that is described by the Maxwell-Boltzman distribution law.The two distribution plots shown here are for a lower temperature T 1 and a higher temperature T 2. The areaunder each curve represents the total number ofmolecules whose energies fall within particular range.The shaded regions indicate the number of moleculeswhich are sufficiently energetic to meet the requirements dictated by the two values of E a that are shown.It is clear from these plots that the fraction of moleculeswhose kinetic energy exceeds the activation energyincreases quite rapidly as the temperature is raised. Thisthe reason that virtually all chemical reactions (and allelementary reactions) are more rapid at highertemperatures.2 The Arrhenius lawBy 1890 it was common knowledge that highertemperatures speed up reactions, often doubling the ratefor a 10-degree rise, but the reasons for this were notclear. Finally, in 1899, the Swedish chemist SvanteArrhenius (1859-1927) combined the concepts of activationenergy and the Boltzmann disribution law into one of themost important relationships in physical chemistry:Take a moment to focus on the meaning of this equation, neglecting the A factorfor the time being.First, note that this is another form of the exponential decay law we discussed inthe previous section of this series. What is "decaying" here is not the concentrationof a reactant as a function of time, but the magnitude of the rate constant as afunction of the exponent –E a /RT. And what is the significance of this quantity? Ifyou recall that RT is the average kinetic energy, it will be apparent that theexponent is just the ratio of the activation energy E a to the average kinetic energy.The larger this ratio, the smaller the rate (hence the negative sign.) This meansthat high temperature and low activation energy favor larger rate constants, andthus speed up the reaction. And because these terms occur in an exponent, theireffects on the rate are quite substantial.The two plots below show the effects of the activation energy (denotedhere by E‡) on the rate constant. Even a modest activation energy of50 kJ/mol reduces the rate by a factor of 108.= (8.314)(0.693) / (.00339 - 0.00328)0.00366)= (3.37 J mol –1K –1) / (0.000923 K –1) = 3650 J mol –1 = 3.65 kJ mol –1Comment : This rather low value seems reasonable because protein denaturation involves the disruption of relatively weak hydrogen bonds; no covalent bonds are broken.The pre-exponential factorIt is now time to focus in on the pre-exponential term A in the Arrhenius equation. We have been neglecting it because it is not directly involved in relating temperature andactivation energy, which is the main practical use of the equation. Butsince A multiplies the exponential term, its value clearly contributes to the value of the rate constant and thus of the rate.Recall that the exponential part of the Arrhenius equation expresses the fraction of reactant molecules that possess enough kinetic energy to react,as governed by the Maxwell-Boltzmann law. This fraction can run from zero to nearly unity, depending on the magnitudes of E a and of the temperature.If this fraction were unity, the Arrhenius law would reduce tok = AIn other words, A is the fraction of molecules that would react if either the activation energy were zero, or if the kinetic energy of all molecules exceeded E a — admittedly, an uncommon scenario.It's all about collisionsSo what would limit the rate constant if there were no activation energy requirements? The most obvious factor would be the rate at whichreactant molecules come into contact. This can be calculated from kinetic molecular theory and is known as the frequency- or collision factor Z .In some reactions, the relative orientation of the molecules at the point of collision is important, so we can also define a geometrical or steric factor (commonly denoted by ρ (Greek lower case rho ).In general, we can express A as the product of these two factors:A = Z ρValues of ρ are generally very difficult to assess; they are sometimeCrickets and popcornMany biological processes exhibit a temperature dependence that follows the Arrhenius law, and can thus be characterized by an activation energy. See this interesting Dartmouth U.page that looks at the kinetics of cricket chirps .In an article on the Kinetics of Popping of Popcorn (Cereal Chemisty 82(1): 53-59), J. Byrd and M. Perona found that popping follows a first-order rate law with an activation energy of 53.8 kJ/mol.This kind of electrophilic addition reaction is well-known to all students of organic chemistry. A very nice (but not-for-beginners) discussion of such reactions and their mechanisms can be found here .estimated by comparing the observed rate constant with the one in which A is assumed to be the same as Z .Direction makes a differenceThe more complicated the structures of the reactants, the more likely that the value of the rate constant will depend on the trajectories at which the reactants approach each other.We showed one example of this near the top of the page, but for another,consider the addition of a hydrogen halide such as HCl to the double bond of an alkene, converting it to a chloroalkane.Experiments have shown that the reaction only takes place when the HCl molecule approaches the alkene with its hydrogen-end, and in a direction that is approximately perpendicular to the double bond, as shown at below.The reason for this becomes apparent when we recall that HCl is highly polar owing to the high electronegativity of chlorine, so that the hydrogen end of the molecule is slightly positive.The double bond of ethene consists of two clouds of negative charge corresponding to the σ (sigma ) and π (pi )molecular orbitals. The latter, which extends above and below the plane of the C 2H 4 molecule, interacts with and attracts the HCl molecule.If, instead, the HCl approaches with its chlorine endleading as in , electrostatic repulsion between the like charges causes the two molecules to bounce away from each other before any reaction can takeplace. The same thing happens in ; the electronegativity difference between carbon and hydrogen is too small to make the C–H bond sufficiently polar to attract the incoming chlorine atom.Now that you know what it takes to get a reaction started, you are ready for the next lesson that describes their actual mechanisms .What you should be able to doThe lesson you should take from this example is that once you start combining a variety of chemical principles, you gradually develop what might be called "chemical intuition" which you can apply to a wide variety of problems. This is far more important than memorizing specific examples.Make sure you thoroughly understand the following essential ideas whichhave been presented above. It is especially imortant that you know theprecise meanings of all the green-highlighted terms in the context of thistopic.Explain the meaning of a reaction mechanism and define elementary step andintermediate.Describe the role of collisions in reaction mechanisms, and explain why not allcollisions lead to the formation of products.Sketch out activation energy diagrams for simple reactions that areendothermic or exothermic,Explain how an activated complex differs from an intermediate.Define catalyst, and sketch out an activation energy diagram that illustrateshow catalysts work.Explain the significance of the various terms that appear in the Arrhenius Law.Sketch out a typical Arrhenius Law plot for a hypothetical reaction at higher andlower temperatures.Explain how the activation energy of a reaction can be determinedexperimentally.Explain the significance of the various terms that appear in the pre-exponentialfactor of the Arrhenius equation.Concept Mapindex| rate laws 1 | rate laws 2 | activation | mechanisms | solutions | catalysis| experimental© 2009 by Stephen Lower - last modified 2011-07-29For information about this Web site or to contact the author,please see the Chem1 Virtual Textbook home page.This work is licensed under a Creative Commons Attribution-Share Alike 3.0 License.。
Can the maturity concept be used to separate the autogenous shrinkage and
Can the maturity concept be used to separate the autogenous shrinkage andthermal deformation of a cement paste at early age?Philippe Turcry a ,Ahmed Loukili a,*,Laurent Barcelo b ,Jean Michel Casabonne baLaboratoire Ge´nie Civil de Nantes St-Nazaire,Ecole Centrale de Nantes,BP 92101,44321Nantes Cedex,France bLAF ARGE,Laboratoire Central de Recherche,95rue du Montmurier,38290St-Quentin Fallavier,FranceReceived 3January 2001;accepted 18March 2002AbstractThe influence of temperature on the autogenous shrinkage of cement paste has been studied using the maturity approach based on Arrhenius’law.Application of this law requires knowledge of the apparent activation energy,E a ,of cement.In this work,E a has been determined by the ‘‘setting time method.’’The external volume change of cement paste was measured by hydrostatic weighing.In order to separate the thermal and autogenous deformations,the thermal dilation coefficient (TDC)was determined at both 20and 30°C.Investigations have shown that maturity can be used to predict autogenous shrinkage under isothermal and realistic conditions as long as temperatures remain between 10and 40°C.Outside of this temperature range,the calculated autogenous deformation and measured isothermal shrinkage are quite different and,as a result,autogenous shrinkage appears to be dependent on more than hydration advancement alone.D 2002Elsevier Science Ltd.All rights reserved.Keywords:Autogenous shrinkage;Temperature;Cement paste;Early age;Maturity concept1.IntroductionAt early age,thermal deformations and autogenous shrinkage occur simultaneously and may,if restrained,lead to the cracking of cementitious materials.These thermal deformations,e th ,result from the temperature rise caused by hydration reactions and are proportional to the thermal dilation coefficient (TDC)of the cement paste:e TH =TDC ÂD T .Autogenous shrinkage,on the other hand,is a consequence of chemical shrinkage:hydration products are denser than the unhydrated compounds.In the plastic state,this contraction is converted into settlement.Once the solid skeleton has been formed and in the absence of an external source of water,hydration reactions continue through the consumption of capillary water.This phenomenon is called ‘‘self-desiccation.’’According to the Kelvin equation,this self-desiccation gradually increases the tensile stress in pore water,which then leads to a global compressive stress on thesolid skeleton.A deformation,called autogenous shrinkage,e as ,thereby occurs.For the calculation of stresses at early age,autogenous shrinkage is assumed to be dependent solely on the degree of hydration,a ,with e as =e as (a ),and total deformation is taken as equal to the sum of thermal and autogenous deformations,i.e.,e total =e as +e th [1].However,recent studies [2,3]have shown that the autogenous shrinkage amplitude of concrete is influenced by the temperature history.It seems that for a given degree of hydration,concretes with different temperature histories do not develop the same autogenous shrinkage.These results question the applicabil-ity of the maturity concept.This paper focuses on the influence of temperature on the autogenous shrinkage of cement paste by use of the maturity concept [4].The rate of chemical reactions is affected by temperature,since cement hydration is thermally activated.Moreover,the microstructure itself will undergo change when formed at different temperatures.The maturity con-cept serves to predict the effect of temperature on certain material properties with respect to the degree of hydration (e.g.,compressive strength).This concept is based on *Corresponding author.Tel.:+33-2-40-37-16-67;fax:+33-2-40-37-25-35.Cement and Concrete Research 32(2002)1443–1450sensitivity of hydration reactions,called the apparent activa-tion energy,E a.This study deals first with the determination of apparent activation energy by using the setting time method[5,6]. Deformations of the cement paste at early age were meas-ured for different thermal conditions,both isothermal and ‘‘realistic.’’The method chosen to assess volume change was the measurement of buoyancy variations for a sample in a flexible latex mold through hydrostatic weighing,as presented in detail elsewhere in the literature[3].With this method,we also measured the TDC,which has allowed calculating thermal deformations in the realistic cases. Lastly,using the maturity approach,we have attempted to separate thermal deformation from autogenous shrinkage.2.Materials and experimental methods2.1.MaterialsAn ordinary type I Portland cement(CPA CEM I52.5 CP2),containing60%C3S,12%C2S,9%C3A,and8% C4AF,was used.The Blaine specific surface was332m2/kg. The water-to-cement(W/C)ratio was0.25.Cement and water were mixed for3min in order to ensure a homogen-eous mix.2.2.Experimental methods2.2.1.Determination of E a by means of the setting times methodThe maturity concept determines the time required at a reference temperature for the cement paste to achieve the same level of development as that under the influence of the actual time–temperature history.This time is called the ‘‘equivalent age’’and can be calculated by a function based on Arrhenius’law(Eq.(1)):t equ¼Z t0expE aR1273þq refÀ1273þqðtÞd tð1Þwhere t equ is the equivalent age at the reference temper-ature,q ref(h),t is the paste age(h),q(t)is the paste temperature at time t(°C),q ref is the reference temperature (°C),E a is the apparent activation energy(J/mol),and R is the universal gas constant(J/mol/K).The following proposed relation has been demonstrated theoretically[5]:lnD aD t¼ÀE aR1T0þconstantð2Þwhere T0is the temperature of the paste(K)under isothermal conditions and D t is the time required to increase the degree of hydration by an increment D a at T0.By deduce E a.Nevertheless,a way must be found to character-ize the degree of hydration a.The setting time method presumes that both the initial set time,t is,and final set time, t fs,are reached for definite levels of hydration(at a given W/ C ratio).This method therefore consists of measuring these specific times(t is and t fs)for several isothermal conditions and,in the subsequent step,calculating an activation energy for each period,initial setting and setting(t fsÀt is).Initial set time and setting time of cement pastes were measured using the Vicat needle apparatus,which operates in accordance with European Standard EN196-3.The samples were placed in a water bath at various temperatures (10,20,30,and40°C).The temperature inside the samples was measured through embedded thermocouples.2.2.2.External volume changeMeasurements of the external volume change of cement paste were conducted through hydrostatic weighing.Imme-diately after casting,a prophylactic without lubricant was filled with cement paste in order to form a spherical sample. The latex mold was closed using a thin stainless steel wire. The excess latex was then cut and the sample container was cleaned and weighed.The sample was hung under a balance and immediately immersed in the water bath at the chosen temperature.The sample mass was approximately90g.The bath temperature was controlled by a‘‘cryo-thermostat’’(Fig.1)with an accuracy of0.1°C.The external volume change results from a change in the buoyancy force and serves to alter the weight reading on the balance.Both the bath temperature and measured mass were continuously logged on the computer at5-min intervals for a period exceeding24h.In realistic tests,the bath temperature is imposed by a cement paste sample placed in a quasi-adiabatic enclosure after casting.The temperature is measured by a ther-mocouple introduced into the cement paste.The weighed sample therefore undergoes the same temperature history.A detailed description of this system is provided in Ref.[3].In order to obtain different temperature histories(Fig.2),the mass of the sample placed in the enclosure ischanged.P.Turcry et al./Cement and Concrete Research32(2002)1443–1450 14442.3.Calculation of the deformationsThe external shrinkage,expressed in cubic millimeters per gram cement,was obtained from Eq.(3)as a result of the calculation already discussed in detail [3]:D VM c¼r iw r w ðT ÞÀ1ÀM r V i r w ðT ÞV iM cð3Þwhere T is the bath temperature (°C),r iw is the initial density of water (g/cm 3),r w (T )is the density of water as a function of temperature (g/cm 3),V i is the initial sample volume (cm 3),D V is the volume change (cm 3),M r is the mass reading on the balance (g),and M c the cement mass in the sample (g).3.Results and discussion3.1.Determination of E a by the setting times method The results related to the initial set and setting times determined by the Vicat needle device have been listed in Table 1.Both the initial set and setting time increase with decreasing temperature.This finding has been previ-ously reported.Figs.3and 4were drawn by assuming isothermal con-ditions until the initial set;a linear curve was fitted to the data points.According to Eq.(2),the computed apparent activa-tion energies were 29kJ/mol for the time periods until theinitial set and 39kJ/mol between the initial and final sets.This procedure yields two activation energies,which is not unrealistic since E a reflects more of a mathematical than a chemical tool,thereby making it possible to estimate the global thermal sensitivity of all reactions taking place in the hydrating cement paste.3.2.Isothermal tests at 10,20,30,and 40°CExposed to each temperature,the external shrinkage was determined as the mean value of three measurementsandFig.2.Different realistic temperature histories.Table 1Initial set and setting time at different temperatures Averagetemperature (°C)Initial set time,t is (min)Setting time,t fs Àt is (min)121501382011090347050Fig.3.Arrhenius plot for initial set times.P .Turcry et al./Cement and Concrete Research 32(2002)1443–14501445expressed in cubic millimeters per gram of cement,with a variation of less than 8%.The external shrinkage at different temperatures versus real time has been plotted in Fig.5.It can be observed that temperature has an unsystematic effect on the autogenous deformation of cement paste.This result,which has also been reported by Bjøntegaard [2],is not surprising since the comparison was conducted in an inconsistent manner by virtue of not incorporating the temperature effect on the rate of cement hydration,which is responsible for auto-genous paring autogenous shrinkage curves versus real age is similar to comparing different materials;in this case,we need to apply the maturity concept.Fig.6shows deformation curves versus equivalent age,which has been calculated for each temperature using Eq.(1).E a is taken as equal to 29kJ/mol before the initial setand 39kJ/mol thereafter.The reference temperature is 20°C (as results obtained at 20°C do not change with time transformation).It is important to note that deformations must be initialized at the same equivalent age,i.e.,for the same theoretical degree of hydration.The selected equival-ent time origin was 3.25h,in correspondence with the final set time (Fig.6).It can be seen that the four curves follow similar trends with just little scatter,which is caused by measurement accuracy levels.The maturity concept there-fore leads to predicting with reasonable accuracy the auto-genous shrinkage of cement paste under isothermal conditions over the 10–40°C range.3.3.External volume change in realistic casesBy considering the behavior of material to be isotropic,linear deformation represents one-third of thevolumetricparison of deformation for different temperatures versus pasteage.P .Turcry et al./Cement and Concrete Research 32(2002)1443–14501446deformation calculated in Eq.(3).In this part,deformations are expressed in micrometers per meter.3.3.1.Deformation kineticsThree realistic temperature tests with initial temperatures of 10and 20°C were performed.A typical curve of a realistic temperature history (20–60°C)is illustrated in Fig.7.The realistic deformation curve can be divided into four phases:AB,BC,CD,and DE.In the first phase,it is observed that the isothermal and realistic deformations display the same shape since the temperature is constant in both tests.When temperature increases in the realistic test (B),the curves diverge;during BC,no ‘‘realistic’’deforma-tion is recorded.It seems that thermal deformations com-pensate for autogenous shrinkage.In the case of the ‘‘realistic’’test,thermal expansion occurs until the temper-ature peak (CD),while the rate of autogenous shrinkage at 20°C is decreasing.During the cooling period (DE),a large deformation caused by both thermal contraction and auto-genous shrinkage can be observed.Moreover,when the temperature returned to 20°C,the magnitude of the ‘‘real-istic’’deformation corresponds to the autogenous shrinkage recorded under isothermal conditions.It can thereby bestated that the total measured deformation under realistic conditions is equal to the sum of the measured deformation in the isothermal test and a thermal component related to temperature history.In order to separate thermal and auto-genous deformations,we have determined the evolution of the TDC with respect to time.3.4.TDC measurementsThe TDC was measured with the method of spontaneous heating by using hydrostatic weighing [3].Tests were carried out under two isothermal conditions:20and 30°C.Every hour,the bath temperature was increased by 4°C within a period of about 7min and then immediately decreased to the isothermal temperature.Fig.8presents the evolution in weight given by the balance without any water density correction.Note that the 4°C peak effect is different at very early age and after 2h,which reveals an evolution in the TDC.By using this method for the TDC calculation,it was assumed that the temperature rise was sufficiently fast for the deformation measured during heating to beconsid-parison of the isothermal test at 20°C and the 20–60°C realistictest.P .Turcry et al./Cement and Concrete Research 32(2002)1443–14501447ered only from a thermal origin.In spite of the disper-sion of experimental points (Fig.9),TDC seems to depend solely on equivalent age (with q ref =20°C).A TDC curve may be interpolated from our results in accordance with Eq.(4)below:TDC ðt equ Þ¼137*exp ð1:44Àt equ Þþ28:ð4ÞIt is observed in Fig.9that the TDC decreases sharply during the first few hours after casting and then remains constant from 8h onwards.Its value is high (28m m/m °C),yet in agreement with other cement paste TDC reported by other researchers [7].Miao et al.[8]reported a TDC of about 24m m/m °C at 7h after casting for a concrete with a W/C ratio of 0.28.It should be noted that the TDC value is very sensitive to the experimental measurement procedure used.3.5.Separation of thermal and autogenous deformations Interpolated TDC allows determining the thermal de-formation for any temperature history.We can also deduce autogenous deformation from total deformation by assum-ing that total deformation is the sum of the two other components.Moreover,deformation separation has to be performed by application of the maturity concept.Fig.10presents an example of separation in the case of the 10–40°C realistic test.3.6.Maturity concept approach results and discussion Figs.11and 12compare isothermal shrinkage at 10and 20°C,respectively,and calculate autogenous deformation.It can be observed that although the determined TDC is rather inaccurate,both the calculated and measureddefor-Fig.10.Separation of thermal and autogenous deformations for the 10–40°C temperaturehistory.P .Turcry et al./Cement and Concrete Research 32(2002)1443–14501448mations are nearly identical.The maturity approach pro-vides a good estimate of the autogenous shrinkage of cement paste.However,the method used to separate thermal and autogenous deformations yields a different result for the 20–60°C test (Fig.13).In this instance,the final amplitude of the calculated autogenous deformation is about 700m m/m higher than that measured in the isothermal test at 20°C.Moreover,the calculated deformation displays a significant dilation peak.In other words,the usually expected kinetics of autogenous shrinkage is not present herein.In Fig.13,it is observed that the final amplitude of the calculated autogenous deformation is nearly equal to the amplitude of isothermal shrinkage plus the final amplitude of thermal deformation.In fact,thermal deformation is not reversible because of TDC evolution:during the heating period,TDC is higher than the cooling phase and,con-sequently,temperature variations induce dilation.In the 10–40°C test (Fig.11),no dilation can be detected;paste heating and cooling must therefore occur at a time when TDC is constant.At this juncture,two assumptions can be made.Firstly,the maturity concept cannot predict autogenous deformation evolution and amplitude when the thermal history lies beyond a temperature field between 10and 40°C.The maturity concept is a mathematical tool that may be too simplistic for describing microstructural changes.For example,the hydration product form,and therefore chem-ical shrinkage,may be different at 20°C than at 60°C.The validity of the determined TDC can also be ques-tioned.Indeed,this TDC does not allow entirely separating thermal and autogenous deformations in the 20–60°C test.For example,dilation observed in the calculated autogenous deformation may be from a thermal origin.Afterwards,we determined a theoretical TDC that allows obtaining the same autogenous shrinkage by means of calculation as by iso-thermal measurement.This method has been developed by Laplante [1].Thermal deformation is presumed to be obtained by subtracting the measured isothermal deforma-tion from the measured total deformation.TDC is equal to the ratio of this deformation to the temperature difference between isothermal and realistictests.parison of the measured autogenous shrinkage at 20°C and the calculated autogenous deformation for the 20–30°Ctest.parison of Laplante method TDC and experimentalTDC.P .Turcry et al./Cement and Concrete Research 32(2002)1443–14501449Fig.14compares experimental TDC from isothermal tests and TDC calculated using the Laplante method.It is shown that the calculated coefficient does not present a typical evolution.TDC may be dependent on thermal history when the temperature of realistic history exceeds a certain limit.This result reveals the need for further work in TDC measurement,for example,in the realistic case.4.Conclusions and subsequent workBased on the assumption that the maturity concept is applicable throughout the hydration process,the following conclusions can be drawn:ÁBetween10and40°C,the maturity concept allowspredicting the isothermal autogenous shrinkage of cement paste measured using the volumetric method:autogenous shrinkage thus seems to be solely dependent on the degree of hydration.ÁIn realistic tests,total deformation is shown in aninitial analysis to be the sum of autogenous and thermal components.Separation of thermal and autogenous defor-mations is therefore made possible by use of a valuable TDC.ÁThe TDC is linked to the degree of hydration onlybetween20and30°C.ÁThe maturity concept allows estimating the autogen-ous deformation amplitude for thermal conditions between 10and40°C by measuring the isothermal shrinkage.ÁFor the20–60°C realistic test,both the calculated autogenous deformation and isothermal measured shrinkage differ.Autogenous deformation therefore seems to be affec-ted by thermal history above40°C.Nonetheless,a process must be developed in the future to accurately measure TDC under different thermal conditions:both isothermal and realistic.These results also need to be verified by means of linear tests.References[1]plante,Proprie´te´s Me´caniques des be´tons durcissants:AnalyseCompare´e des Be´tons Classiques et a`Tre`s Hautes Performances,PhD thesis,Ecole Nationale des Ponts et Chausse´es,Paris,1993(in French).[2]Ø.Bjøntegaard,Thermal dilation and autogenous deformation as driv-ing forces to self-induced stresses in high performance concrete,PhD thesis,The Norwegian University of Science and Technology,N-7491 Trondheim,1999.[3]A.Loukili,D.Chopin,A.Khelidj,J.Y.Le Touzo,A new approach todetermine autogenous shrinkage of mortar at early age considering temperature history,Cem.Concr.Res.30(6)(2000)915–922. [4]plante,S.Roussel,S.Lecrux,Technique maturome´trique:la loid’Arrhe´nius au service des chantiers,Proceedings of the Internation-al RILEM Conference,RILEM Publications,Arles,France,1998, pp.323–342(in French).[5]L.D’Aloia,De´termination de l’e´nergie d’activation apparente du be´tondans le cadre de l’application de la me´thode du temps e´quivalent a`la pre´vision au jeune aˆge:approches expe´rimentales me´caniques et calo-rime´trique,simulations nume´riques,PhD thesis,Institut National des Sciences Applique´es de Lyon,1998(in French).[6]R.C.A.Pinto,K.C.Hover,Application of maturity approach to settingtimes,ACI Mater.J.96(6)(1999)686–691.[7]N.Bouzoubaa,Thermal dilation coefficient of concrete,University ofSherbrooke,Canada,1992(in French).[8]B.Miao,P.C.Aı¨tcin,W.D.CooK,D.Mitchell,Influence of concretestrength on in situ properties of large columns,ACI Mater.J.90(3) (1993)214–219.P.Turcry et al./Cement and Concrete Research32(2002)1443–1450 1450。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
An Automata-Theoretic Approach to Reasoning aboutInfinite-State SystemsOrna Kupferman and Moshe Y.VardiHebrew University,The institute of Computer Science,Jerusalem91904,Israel Email:orna@cs.huji.ac.il,URL:http://www.cs.huji.ac.il/ornaRice University,Department of Computer Science,Houston,TX77251-1892,U.S.A.Email:vardi@,URL:/vardiAbstract.We develop an automata-theoretic framework for reasoning about infinite-state sequential systems.Our framework is based on the observation that statesof such systems,which carry afinite but unbounded amount of information,canbe viewed as nodes in an infinite tree,and transitions between states can be simu-lated byfinite-state automata.Checking that the system satisfies a temporal prop-erty can then be done by an alternating two-way tree automaton that navigatesthrough the tree.As has been the case withfinite-state systems,the automata-theoretic framework is quite versatile.We demonstrate it by solving several ver-sions of the model-checking problem for-calculus specifications and prefix-recognizable systems,and by solving the realizability and synthesis problems for-calculus specifications with respect to prefix-recognizable environments.1IntroductionOne of the most significant developments in the area of formal design verification is the discovery of algorithmic methods for verifying temporal-logic properties offinite-state systems[CES86,LP85,QS81,VW86].In temporal-logic model checking,we verify the correctness of afinite-state system with respect to a desired behavior by checking whether a labeled state-transition graph that models the system satisfies a temporal logic formula that specifies this behavior(for a survey,see[CGP99]).Symbolic methods that enable model checking of very large state spaces,and the great ease of use of fully algorithmic methods,led to industrial acceptance of temporal model checking [BBG94].An important research topic over the past decade has been the application of model checking to infinite-state systems.Notable successes in this area has been the appli-cation of model checking to real-time and hybrid systems(cf.[HHWT95,LPY97]). Another active thrust of research is the application of model checking to infinite-state sequential systems.These are systems in which a state carries afinite,but unbounded, amount of information,e.g.,a pushdown store.The origin of this thrust is the impor-tant result by M¨u ller and Schupp that the monadic second-order theory of context-free graphs is decidable[MS85].As the complexity involved in that decidability result is nonelementary,researchers sought decidability results of elementary complexity.Thisstarted with Burkart and Steffen,who developed an exponential-time algorithm for model-checking formulas in the alternation-free-calculus with respect to context-free graphs[BS92].Researchers then went on to extend this result to the-calculus, on one hand,and to more general graphs on the other hand,such as pushdown graphs [BS99a,Wal96],regular graphs[BQ96],and prefix-recognizable graphs[Cau96].The most powerful result so far is an exponential-time algorithm by Burkart for model checking formulas of the-calculus with respect to prefix-recognizable graphs[Bur97b]. See also[BCMS00,BE96,BEM97,BS99b,Bur97a,FWW97].In this paper we develop an automata-theoretic framework for reasoning about infinite-state sequential systems.The automata-theoretic approach uses the theory of automata as a unifying paradigm for system specification,verification,and synthesis [WVS83,EJ91,Kur94,VW94,KVW00].Automata enables the separation of the logical and the algorithmic aspects of reasoning about systems,yielding clean and asymptoti-cally optimal algorithms.The automata-theoretic framework for reasoning aboutfinite-state systems has proven to be very versatile.Automata are the key to techniques such as on-the-fly verification[GPVW95],and they are useful also for modular verifica-tion[KV98],partial-order verification[GW94,WW96],verification of real-time and hybrid systems[HKV96,DW99],and verification of open systems[AHK97,KV99]. Many decision and synthesis problems have automata-based solutions and no other solution for them is known[EJ88,PR89,KV00].Automata-based methods have been implemented in industrial automated-verification tools(c.f.,COSPAN[HHK96]and SPIN[Hol97,VB99]).The automata-theoretic approach,however,has long been thought to be inapplica-ble for effective reasoning about infinite-state systems.The reason,essentially,lies in the fact that the automata-theoretic techniques involve constructions in which the state space of the system directly influences the state space of the automaton(e.g.,when we take the product of a specification automaton with the graph that models the system). On the other hand,the automata we know to handle havefinitely many states.The key insight,which enables us to overcome this difficulty,and which is implicit in all pre-vious decidability results in the area of infinite-state sequential systems,is that in spite of the somewhat misleading terminology(e.g.,“context-free graphs”and“pushdown graphs”),the classes of infinite-state graphs for which decidability is known can be de-scribed byfinite-state automata.This is explained by the fact the the states of the graphs that model these systems can be viewed as nodes in an infinite tree and transitions be-tween states can be expressed byfinite-state automata.As a result,automata-theoretic techniques can be used to reason about such systems.In particular,we show that various problems related to the analysis of such systems can be reduced to the emptiness prob-lem for alternating two-way tree automata,which was recently shown to be decidable in exponential time[Var98].Wefirst show how the automata-theoretic framework can be used to solve the-calculus model-checking problem with respect to context-free and prefix-recognizable systems.While our framework does not establish new complexity results for model checking of infinite-state sequential systems,it appears to be,like the automata-theoretic framework forfinite-state systems,very versatile,and it has further potential applica-tions.We demonstrate it by showing how the-calculus model-checking algorithm can be extended to graphs with regular state properties,to graphs with regular fairnessconstraints,to-calculus with backwards modalities,and to checking realizability of -calculus formulas with respect to infinite-state sequential environments.In each of these problems all we have to demonstrate is a(fairly simple)reduction to the empti-ness problem for alternating two-way tree automata;the(exponentially)hard work is then done by the emptiness-checking algorithm.2Preliminaries2.1Labeled rewrite systemsA labeled transition graph is quadruple,where is a(possibly infinite)set of states,is afinite set of actions,is a labeled transition relation,and is an initial state.When,we say that is an-successor of,and is an-predecessor of.For a state,we denote by,the graph with as its initial state.A rewrite system is a quadruple,where is afinite alphabet,is afinite set of actions,maps each action to afinite set of rewrite rules,to be defined below,and is an initial word.Intuitively,describes the possible rules that can be applied by taking the action.We consider here two types of rewrite systems.In a context-free rewrite system,each rewrite rule is a pair.In a prefix-recognizable rewrite system,each rewrite rule is a triple of regular expressions over,each defining a subset of.We refer to rewrite rules in as-rules.The rewrite system induces the labeled transition graph, where if there is a rewrite rule in whose application on re-sults in.Formally,if is a context-free rewrite system,then if .If is a prefix-recognizable system,then if there are regular expressions,,and such that,,,and.A labeled transition graph that is induced by a context-free rewrite system is called a context-free graph.A labeled transition system that is induced by a prefix-recognizable rewrite system is called a prefix-recognizable graph.Note that in order to apply an-transition in state of a context-free graph,we only need to match thefirst latter of with thefirst element of an-rule.On the other hand,in an application of an-transition in a prefix-recognizable graph,we shouldfind an-rule and a partition of to a pre-fix that belongs to thefirst element of the rule and a suffix that belongs to the second element.Example1.The context-free rewrite sys-tem,withand, induces the labeled transition graph on the right.a ab b bb b bA AB ABB ABBBB BB BBBabWe define the size of as the space required in order to encode the rewrite rules in.Thus,in the case of a context-free rewrite system,, and in a prefix-recognizable rewrite system,,where is the size of a nondeterministic automaton provided for the regular ex-pression.2.2-calculusThe-calculus is a modal logic augmented with least and greatestfixpoint operators [Koz83].Given afinite set of actions and afinite set of variables,a-calculus formula(in a positive normal form)over and is one of the following:–,,or for all;–or,for-calculus formulas and;–or,for and a-calculus formula;–or,for and a-calculus formula.A sentence is a formula that contains no free variables from(that is,all the variables are in a scope of somefixed-point operator).We define the semantics of-calculus with respect to a labeled transition graph and a valuation for its free variables.Each formula and valuation then define a setof states of that satisfy the formula.For a valuation,a variable, and a set,we denote by the valuation obtained from by assigning to.The mapping is defined inductively as follows:–and;–For,we have;–;–;–for all such that we have;–there is such that and;–;–.Note that cares only about the valuation of free variables in.In particular,no valuation is required for a sentence.For a state and a sentence,we say that holds at in,denoted iff.Also,iff.2.3Alternating two-way automataGiven afinite set of directions,an-tree is a set such that if, where and,then also.The elements of are called nodes,and the empty word is the root of.For every and,the node is the parent of .Each node of has a direction in.The direction of the root is the symbol (we assume that).The direction of a node is.We denote by the direction of node.An-tree is a full infinite tree if.A path of a tree is a set such that and for every there exists a unique such that.Note that our definitions here reverse the standard definitions(e.g.,when ,the successors of the node are and(rather than and)1.Given twofinite sets and,a-labeled-tree is a pair where is an -tree and maps each node of to a letter in.When and arenot important or clear from the context,we call a labeled tree.We say that an -labeled-tree is-exhaustive if for every node,we have.Alternating automata on infinite trees generalize nondeterministic tree automata and werefirst introduced in[MS87].Here we describe alternating two-way tree automata. For afinite set,let be the set of positive Boolean formulas over(i.e., boolean formulas built from elements in using and),where we also allow the formulas and,and,as usual,has precedence over.For a set and a formula,we say that satisfies iff assigning to elements in and assigning to elements in makes true.For a set of directions,the extension of is the set(we assume that).An alternating two-way automaton over-labeled-trees is a tuple, where is the input alphabet,is afinite set of states,is the transition function,is an initial state,and specifies the acceptance condition.A run of an alternating automaton over a labeled tree is a labeled treein which every node is labeled by an element of.A node in,labeled by,describes a copy of the automaton that is in the state and reads the node of.Note that many nodes of can correspond to the same node of;there is no one-to-one correspondence between the nodes of the run and the nodes of the tree.The labels of a node and its successors have to satisfy the transition function.Formally,a run is a-labeled-tree,for some set of directions,whereand satisfies the following:1.and.2.Consider with and.Then there is a(possiblyempty)set,such that satisfies,and for all,there is such that and the following hold:–If,then.–If,then.–If,then,for some and,and. Thus,-transitions leave the automaton on the same node of the input tree,and-transitions take it up to the parent node.Note that the automaton cannot go up the root of the input tree,as whenever,we require that.A run is accepting if all its infinite paths satisfy the acceptance condition. We consider here parity acceptance conditions[EJ91].A parity condition over a state set is afinite sequence of subsets of,where.The number of sets is called the index of.Given a run and an infinite path,let be such that if and only if there are infinitely many for which.That is,contains exactly all the states that appear infinitely often in.A path satisfies the condition if there is an even for which and.An automaton accepts a labeled tree if and only if there exists a run that accepts it.We denote by the set of all-labeled trees that accepts.The automaton is nonempty iff.Theorem2.Given an alternating two-way parity tree automaton with states and index,we can construct an equivalent nondeterministic one-way parity tree automa-ton whose number of states is exponential in and whose index is linear in[Var98], and we can check the nonemptiness of in time exponential in[EJS93].2.4Alternating automata on labeled transition graphsConsider a labeled transition graph.For the set of actions,let.An alternating automaton on labeled transition graphs(graph automaton,for short)[JW95]2is a tuple,where ,,and are as in alternating two-way automata,is a set of actions,andis the transition function.Intuitively,when is in state and it reads a state of,fulfilling an atom(or,for short)requires to send a copy in state to some-successor of.Similarly,fulfilling an atom requires to send copies in state to all the-successors of.Thus,like symmetric automata [DW99,Wil99],graph automata cannot distinguish between the various-successors of a state and treat them in an existential or universal way.Like runs of alternating two-way automata,a run of a graph automaton over a labeled transition graph is a labeled tree in which every node is labeled by an element of.A node labeled by,describes a copy of the automaton that is in the state of and reads the state of.Formally,a run is a -labeled-tree,where is an arbitrary set of directions,,and satisfies the following:1.and.2.Consider with and.Then there is a(possibly empty)set,such that satisfies,and for all,the following hold:–If,then there is such that and.–If,then for every-successor of,there is such thatand.–If,then there is an-successor of and such thatand.A run is accepting if all its infinite paths satisfy the acceptance condition.The graph is accepted by if there is an accepting run on it.We denote by the set of all graphs that accepts.We denote by the automaton with as its initial state.We use graph automata as our specification language.We say that a labeled transi-tion graph satisfies a graph automaton,denoted,if accepts.It is shown in[JW95]that graph automata are as expressive as-calculus.In particular,we have the following.Theorem3.Given a-calculus formula,of length and alternation depth,we can construct a graph parity automaton such that is exactly the set of graphs satisfying.The automaton has states and index.3Model Checking of Context-free GraphsIn this section we present an automata-theoretic approach to model-checking of context-free transition systems.Consider a labeled transition graph, induced by a rewrite system.Since the state space of is the full -tree,we can think of each transition as a“jump”that is activated by the action from the node of the-tree to the node.Thus,if is a context-free rewrite system and we are at node of the-tree,an application of the action takes us to nodes,for.Technically,this means that wefirst move up to the parent of,and then move down along.Such a navigation through the -tree can be easily performed by two-way automata.Theorem4.Given a context-free rewrite system and a graph automaton,we can construct an alternating two-way parity automaton over-labeled-trees such that is not empty iff satisfies.The automaton has states,and has the same index as. Proof:The automaton checks that the input tree is-exhaustive(that is,each node is labeled by its direction).As such,can learn from labels it reads the state in that each node corresponds to.The transition function of then consults the rewrite rules in in order to transform an atom in to a chain of transitions that spread copies of to the corresponding nodes of the full-tree.We define as follows.–,where is the set of all suffixes of words for which there are and such that.Intuitively,when visits a node in state,it checks that with initial state is accepted by.In particular,when,then with initial state(the node currently being visited)needs to be accepted by.In addition, if,then also checks that.States of the form are called action states.From these states consults and in order to impose new requirements on the exhaustive-tree.States of the form,for,are called navigation states.From these states only navigates downwards to reach new action states.On its way,also checks the-exhaustiveness of the input tree.–In order to define,wefirst define the function.Intuitively, transforms atoms participating in,together with a letter, which stands for the direction of the current node,to a formula that describes the requirements on when the rewrite rules in are applied to words of the form .For,,and,we defineIf.If.If.Note that may contain no pairs in(that is,the transition relation of may not be total).In particular,this happens when(that is,the stateof has no successors).Then,we take empty conjunctions as,and take empty disjunctions as.In order to understand the function,consider the case.When reads the state of the input graph,fulfilling the atom requires to send copies in state to all the-successors of.The automaton then sends to the node copies that check whether all the states,with,are accepted by with initial state.Now,for a formula,the formulais obtained from by replacing an atom by the atom.We can now define for all as follow...Thus,in action states,reads the direction of the current node and applies the rewrite rules of in order to impose new requirements according to.In navigation states,needs to go downwards and check that the nodes it comes across on its way are labeled by their direction.For that,proceeds only with the direction of the current node(maintained as the third element of the state),and sends to direction a state whose third element is.Note that since we reach states with only with upward transitions,visits these states only when it reads nodes that have already been read by a copy of that does check whether is labeled by its direction.–.Thus,in its initial state checks that with initial state is accepted by with initial state.It also checks that the root of the input tree is labeled with.–is obtained from by replacing each set by the set.Context-free rewrite systems can be viewed as a special case of prefix-recognizable rewrite systems.In the next section we describe how to extend the construction de-scribed above to prefix-recognizable graphs,and we also analyze the complexity of the model-checking algorithm that follows for the two types of systems.4Model Checking of Prefix-Recognizable GraphsIn this section we extend the construction described in Section3to prefix-recognizable transition systems.The idea is similar:two-way automata can navigate through the full -tree and simulate transitions in a system induced by a rewrite system by a chain of transitions in the tree.While in context-free transition systems the application of rewrite rules involved one move up the tree and then a chain of moves down,here things are a bit more involved.In order to apply a rewrite rule,the automaton has to move upwards along a word in,check that the remaining word leading to the root is in, and move downwards along a word in.As we explain below,does so by simulating automata for the regular expressions participating in.Theorem5.Given a prefix-recognizable rewrite system and a graph automaton,we can construct an alternating two-wayparity automaton over-labeled-trees such that is not empty iff satisfies.The automaton has states,and has the same index as.Proof:For a regular expression on,let be a non-deterministic word automaton with.Let there is such that be the set of all triples in,for some,and let be the union of all the state spaces of the automata associated with regular expressions that participate in.As in the case of context-free rewrite systems,checks that the input tree is the -exhaustive tree and then uses its labels in order to learn the state in that each node corresponds to.As there,applies to the transition function of the rewrite rules of .Here,however,the application of the rewrite rules on atoms of the form and is more involved,and we describe it below.Assume that wants to check whether accepts,and it wants to proceed with an atom in.The automaton needs to check whether accepts for some state reachable from by applying an-rule.That is,a state for which there is and partitions and ,of and,respectively,such that is accepted by,is accepted by, and is accepted by.The way detects such a state is the following.From the node,the automaton simulates the automaton upwards(that is,guesses a run of on the word it reads as it proceeds on direction from towards the root of the -tree).Suppose that on its way up to the root,encounters a state in as it reads the node.This means that the word read so far is in,and can serve as the prefix above.If this is indeed the case(and may also continue as if a state in has not been encountered;thus guess that the word read so far is not),then it is left to check that the word is accepted by,and that there is a state that is obtained from by prefixing it with a word that is accepted by.To check thefirst condition, sends a copy in direction that simulates a run of,hoping to reach a state inas it reaches the root(that is,guesses a run of on the word it reads as it proceeds from up to the root of the-tree).To check the second condition,simulates the automaton downwards.A node that reads as it encounters a state in can serve as the state we are after.The case for an atom is similar,only that here needs to check whether accepts for all states reachable from by applying an-rule,and thus the choices made by for guessing the partition of and the prefix of are now treated dually.In order to follow the above application of rewrite rules,the state space of is.Thus,a state is a6-tuple,where is the expected direction of the current node(needed in order to check the-exhaustiveness),is the current simulation mode(states in mode are action states,where we apply on the transitions in,and states in modes and are states where we simulate automata for, and,respectively),is the simulating mode(depending on whether we are applying to an or an atom),is the rewrite rule in we areapplying,and is the current state of the simulated automaton3.The formal definition of the transition function of follows quite straightforwardly from the definition of the state space and the explanation above.The acceptance condition of is the adjustment of to the new state space.That is,it is obtained from by replacing each set by the set.Considering only action states excludes runs in which the simulation of the automata for the regular expressions continues forever.Indeed,as long as a copy of simulates an automaton,,or,it stays in simulation mode,or, respectively.The constructions described in Theorems4and5reduce the model-checking prob-lem to the nonemptiness problem of an alternating two-way parity tree automaton.By Theorem2,we then have the following.Theorem6.The model-checking problem for a context-free or a prefix recognizable rewrite system and a graph automaton, can be solved in time exponential in,where and is the index of .Together with Theorem3,we can conclude with an EXPTIME bound also for the model-checking problem of-calculus formulas matching the lower bound in[Wal96]. Note that the fact the same complexity bound holds for both context-free and prefix-recognizable rewrite systems stems from the different definition of in the two cases.5ExtensionsThe automata-theoretic approach offers several extensions to the model-checking set-ting.We describe some of these extensions below.5.1Regular state propertiesThe systems we want to reason about often have,in addition to a set of actions,also a set of state properties.In the case offinite-state systems,these are described by a mapping that associates with each state of the labeled transition graph that models the system,the property that is true in it(for simplicity,we assume that exactly one property holds in each state).In our case,of infinite-state graphs induced by rewrite systems,we consider regular state properties,where each property is associated with a regular expression over,describing the set of states(words in)in which holds.Again,we assume that for each,there is a single such that .In order to specify behaviors of labeled transition graphs with regular state proper-ties in,we consider an extension of graph automata with the alphabet.The tran-sition function of an extended automaton,is;thus it reads from the input graph both the state properties,in order to know with which transition to proceed,and the actions,in order to know to which successors to proceed.The formal definition of a run of an extended graph automa-ton on a labeled transition graph with state properties is the straightforward extension of the definition given in Section2.4for the graph automata described there.Alterna-tively,one can consider a-calculus with both state properties and actions[Koz83]. Theorem3holds also for formulas in such a-calculus.Having our solution to the model-checking problem based on two-way automata,it is simple to extend it to graphs and specifications with state properties.Indeed,when-ever the automaton from Theorems4and5reads the state and takes a transition from an action state,it should now also guess the property that holds in and proceed according to the transition function of the specification automaton with in-put letter.In order to check that the guess is correct,the automaton simulates the word automaton upwards,hoping to visit an accepting state when the root is reached.The complexity of the model-checking algorithm stays the same.5.2FairnessThe systems we want to reason about are often augmented with fairness constraints. Like state properties,we can define a regular fairness constraint by a regular expression ,where a computation of the labeled transition graph is fair iff it contains infinitely many states in(this corresponds to weak fairness;other types of fairness can be defined similarly).It is easy to extend our model-checking algorithm to handle fairness (that is,let the path quantification in the specification range only on fair paths4):the automaton can guess whether the state currently visited is in,and then simulate the word automaton upwards,hoping to visit an accepting state when the root is reached.When checks an existential property,it has to make sure that the property is satisfied along a fair path,and it is therefore required to visit infinitely many states in .When checks a universal property,it may guess that a path it follows is not fair,in which case eventually always send copies that simulate the automaton for.The complexity of the model-checking algorithm stays the same.5.3Backward modalitiesAnother extension is the treatment of specifications with backwards modalities.While forward modalities express weakest precondition,backward modalities express strongest postcondition,and they are very useful for reasoning about the past[LPZ85].In order to adjust graph automata to backward reasoning,we add to the“directions”and.This enables the graph automata to move to-predecessors of the cur-rent state.More formally,if a graph automaton reads a state of the input graph,then fulfilling an atom requires to send a copy in state to some-predecessor of, and dually for.Theorem3can then be extended to-calculus formulas and graph automata with both forward and backward modalities[Var98].。
