湘潭大学 工程数学 2012年博士研究生考博考研真题
湘潭大学2012年推免生复试科目(笔试)

科目名称
考试大纲
(提纲式列举本科目须考查的知识要点,纸张不够可附页)
541
英语语言文学(文学70分、语言学30分)
语言学部分
一、考试目的
考察考生掌握语言学基础知识、基本理论和基本分析方法的程度及水平,重点考察考生分析解决问题的能力。
二、考试内容:
考生应掌握以下方面的内容:
1.语言学导论
语言的定义、起源、特征、功能。语言学的主要分支学科。宏观语言
593
翻译实务
贾文波.《汉英时文翻译》,中国对外翻译出版公司,2000年版
仲伟合.《英语口译教程》(上、下),高等教育出版社,2006年版
240
英语(二外)
《新视野大学英语读写教程》1-4册,郑树棠等,外语教育与研究出版社,2007年版
241
德语(二外)
《大学德语》(修订版)1-3册,赵仲、顾士渊等,高等教育出版社,2002年版
复试包括口试和笔试两部分。
二、考试形式及比例
按照学校通用评分要求执行。
三、考试内容及时间
口试内容包含以下几点:
1、本科学习情况(含本科学习成绩、获奖情况);
2、基础知识、专业知识的掌握情况,对报考专业的了解程度;
3、综合知识应用能力;
4、语言表达能力、思维的敏锐性及逻辑思维能力。
笔试主要内容是阅读、理解、诠释、翻译(参照初试形式,适当增加深化理解内容)及命题作文。作文:根据所给题目及要求用德语撰写规定体裁的文章,长度为300-350个单词。做到内容充实、语言通顺、用词恰当、表达得体。
04日语教学
复试专业课
544日语写作:日语小论文(内容与报考方向一致)
二外口语、听力测试
240 英语
博士研究生考试试题

博士研究生考试试题一、名词解释1、professional paper(第一页)2、subjective description(77页)3、technical report(166页)二、简答题1、写出确定题目中的5个基本原则(第5页)2、P21第一题;3、P40页第二题;4、P55第二题;5、P69第一题;6、P88第三题;7、P125第二题;三、论述题1、P49,根据这个例子,分析5A步骤。
(首先写5A指的是什么,然后再写上P49这一段)。
2、P155如何看待剽窃问题。
(可加上自己的观点)名词解释1.Professional paper:A professional paper is a formal printed document in which professional present their views and research finding on any deliberately chosen topic. It is variously known as “research paper”, “course paper”, “thesis paper” or “library paper”.2.Subjective description:subjective description shows the author’s impressions of or responses to what they see. The author does not only want of describe the object itself but also express directly or indirectly his impressions or opinions of what he is describing.3. Technical report: technical report refers to the papers whose contents involve certain unpublicized key technologies, technical know-how, or traditional Chinese technological processes, special raw materials, new technologies or methods that may bring about greater economic benefit, as well as technical renovation and transformation of relevant devices, instrument, prototypes, etc.简答题1.Finding topics: (1) It should be a topic within the reach of the author and capable of being finished within the assigned or suggested time limited. (2) It should be a topic of practical value for the specialty or the development of economy or science in general. (3) It should be a topic for which sufficient materials and documents can be made available either through readings or through investigations. Research work is by nature discovery and creation. (4) It should bear being tested theoretically and experimentally if his research is experimentation and investigation in nature as science demands thoroughness, accuracy and objectivity. (5) It should be a topic free from the author’s personal bias or preference even if it may be a topic of humanistic nature.2. What are the general functions of a title in a professional paper?(1) Generalizing the text; By glancing at the title, the reader will immediately know, incorporating with the abstract, what is mainly dealt with in the paper.(2) Attracting the reader; Ideally, the title should be such that, by reading it, any professional reader can see whether the paper is worth reading at all.(3) Facilitating the retrieval. A good title can help the readerin his search for information.3. What are the writing requirements for an abstract?(1) Integrity; An abstract should include what the writer has done and what he has achieved within the scope of the topic, such as the research theories, research methods, investigations and experimental results and conclusions. To differentiate his paper work from others, the writer needs to stress his own contribution.(2) Concise; The writer’s abstract is a miniature version of the body of his paper, self-contained and unambiguous.(3) Consistency; As a mini-paper, an abstract should be consistent with the other parts of the whole paper.(4) Concentration; An evaluation of a new technique or a discussion of the validity of the new results should properly appear in the conclusive section.4. What are the “5 step” in abstract writing?(1) Underlining key words and sentences; Underline the key words and sentences which are often signaled by transitional devices.(2) Listing essential point of the paper; Try to put the same emphasis onto the points of your paper as you did with the original paper.(3) Boiling down each section to a sentence or two; This is especially important for a descriptive (or indicative) abstract.(4) Drafting the abstract; Use your own words wherever possible.(5) Checking the final draft. Firstly, it should be shorten further to a minimum length. Secondly recheck the abstract until you are satisfied with it. Finally, the abstract should be read critically by your colleagues for objectivity.5. What is your understanding of the structural features of the introduction?(1)Introducing the subject; Since the topic in this section is what the paper is going to deal with, the readers, then, can get a preliminary but overall impression before going on with the full text of the paper.(2) Limiting the research scope; Only when an introduction clearly defines the limits of the research scope can readers retrieve the information efficiently provided that the subject is introduced correctly.(3) Stating the general purpose; The function of stating the general purpose is aimed at telling the reader of the general purpose of the why to where to start or where to guide.(4) Showing the writing arrangement. The logical arrangement of the writing enables the reader to understand the paper more easily when further reading is necessary.6. What are the main methods of logical development?(1) Developing by chronological sequence; (2) Developing by investigation procedures;(3) Developing the research “from abstract to concrete”; (4) Developing the research “from concrete to abstract”; (5) Developing by using other logical sequencing.7. Give your understanding of the writing requirements for results with your own example?(1) Any data shown in this section must be meaningful. (2) The presentation of results should be short without verbiage and be of crystal clarity.论述题1. P49,根据这个例子,分析5A步骤。
湘潭大学832高等代数2012-2017年考研专业课真题试卷

湘潭大学2017年考研专业课真题试卷(原版)
精都教育——全国 100000 考生的选择
我们的梦想,为成就更多人的梦想
湘 潭 大 学 研 究 生 入 学 考 试 试 题
原版考研真题试卷
更多真题、笔记、模拟试卷、题库、讲义资料,就上精都考研网 /
湘潭大学2016年考研专业课真题试卷(原版)
湘潭大学2016年考研专业课真题试卷(原版)
精都教育——全国 100000 考生的选择
我们的梦想,为成就更多人的梦想
湘 潭 大 学 研 究 生 入 学 考 试 试 题
原版考库、讲义资料,就上精都考研网 /
2012年湘潭大学考研专业课真题试卷(原版)
2012年湘潭大学考研专业课真题试卷(原版)
2015年湘潭大学考研专业课真题试卷(原版)
2015年湘潭大学考研专业课真题试卷(原版)
精都教育——全国 100000 考生的选择
我们的梦想,为成就更多人的梦想
湘 潭 大 学 研 究 生 入 学 考 试 试 题
原版考研真题试卷
更多真题、笔记、模拟试卷、题库、讲义资料,就上精都考研网 /
2014年湘潭大学考研专业课真题试卷(原版)
2014年湘潭大学考研专业课真题试卷(原版)
精都教育——全国 100000 考生的选择
我们的梦想,为成就更多人的梦想
湘 潭 大 学 研 究 生 入 学 考 试 试 题
原版考研真题试卷
更多真题、笔记、模拟试卷、题库、讲义资料,就上精都考研网 /
2013年湘潭大学考研专业课真题试卷(原版)
2013年湘潭大学考研专业课真题试卷(原版)
精都教育——全国 100000 考生的选择
2012年考研数学真题(完整版)

2012年全国硕士研究生入学统一考试数学一试题一、选择题:1:8小题,每小题4分,共32分.下列每题给出的四个选项中,只有一个选项符合题目要求的,请将所选项前的字母填在答题纸...指定位置上. (1) 曲线221x x y x +=-渐近线的条数 ( )(A) 0 (B) 1 (C) 2 (D) 3 (2) 设函数2()(1)(2)()xxnx y x e ee n =---L ,其中n 为正整数,则(0)y '= ( )(A) 1(1)(1)!n n --- (B) (1)(1)!n n -- (C) 1(1)!n n -- (D) (1)!n n -(3) 如果函数(,)f x y 在(0,0)处连续,那么下列命题正确的是 ( )(A) 若极限00(,)limx y f x y x y→→+存在,则(,)f x y 在(0,0)处可微(B) 若极限2200(,)limx y f x y x y→→+存在,则(,)f x y 在(0,0)处可微 (C) 若(,)f x y 在(0,0)处可微,则 极限00(,)limx y f x y x y →→+存在(D) 若(,)f x y 在(0,0)处可微,则 极限2200(,)limx y f x y x y→→+存在 (4)设2sin (1,2,3)k x K e xdx k π==⎰I 则有 ( )(A)123I I I << (B) 321I I I << (C) 231I I I << (D)213I I I <<(5)设1100C α⎛⎫ ⎪= ⎪ ⎪⎝⎭,2201C α⎛⎫ ⎪= ⎪ ⎪⎝⎭ ,3311C α⎛⎫ ⎪=- ⎪ ⎪⎝⎭ ,4411C α-⎛⎫ ⎪= ⎪ ⎪⎝⎭,其中1234,,,C C C C 为任意常数,则下列向量组线性相关的为( )(A)123,,ααα (B) 124,,ααα (C)134,,ααα (D)234,,ααα(6) 设A 为3阶矩阵,P 为3阶可逆矩阵,且1100010002p AP -⎛⎫⎪= ⎪ ⎪⎝⎭.若P=(123,,ααα),1223(,,)ααααα=+,则1Q AQ -= ( )(A) 100020001⎛⎫ ⎪ ⎪ ⎪⎝⎭(B) 100010002⎛⎫ ⎪ ⎪ ⎪⎝⎭(C) 200010002⎛⎫ ⎪ ⎪ ⎪⎝⎭(D)200020001⎛⎫ ⎪ ⎪ ⎪⎝⎭(7)设随机变量X 与Y 相互独立,且分别服从参数为1与参数为4的指数分布,则{}p X Y <=( )(A)15 (B) 13(C) 25 (D) 45 (8)将长度为1m 的木棒随机地截成两段,则两段长度的相关系数为 ( )(A) 1 (B) 12 (C) 12- (D)1-二、填空题:9:14小题,每小题4分,共24分.请将答案写在答题纸...指定位置上. (9)若函数()f x 满足方程'''()()2()0f x f x f x +-=及''()()2f x f x e +=,则()f x =(10)2x =⎰(11)(2,1,1)()|zgrad xy +y=(12)设(){},,1,0,0,0x y z x y z x y z ∑=++=≥≥≥,则2y ds ∑=⎰⎰(13)设X 为三维单位向量,E 为三阶单位矩阵,则矩阵T E XX -的秩为 (14)设A ,B ,C 是随机变量,A 与C 互不相容,()()()11,,23p AB P C p AB C === 三、解答题:15~23小题,共94分.请将解答写在答题纸...指定位置上.解答应写出文字说明、证明过程或演算步骤.(15)证明21ln cos 1(11)12x x x x x x ++≥+-<<-(16)求函数222(,)x y f x y xe +-=的极值(17)求幂级数22044321nn n n x n ∞=+++∑的收敛域及和函数 (18)已知曲线(),:(0),cos 2x f t L t y tπ=⎧≤<⎨=⎩其中函数()f t 具有连续导数,且'(0)0,()0(0).2f f t t π=><<若曲线L的切线与x 轴的交点到切点的距离恒为1,求函数()f t 的表达式,并求此曲线L 与x 轴与y 轴无边界的区域的面积。
2012考研数学二真题及答案解析

dy
,所以
=
2x
dx ey +1
(10)计算
lim
x→∞
n
⎛ ⎜⎝
1
1 +n
2
+
22
1 +
n2
+…+
n2
1 +
n2
⎞ ⎟⎠
= ________。
3
π
【答案】:
4
∑ ∫ 【解析】:原式 = lim 1
n→∞ n
n
1
i=1
1
+
⎛ ⎜⎝
i n
⎞2 ⎟⎠
=
1 dx 0 1+ x2
=
arctan x 1 0
三、解答题:15—23 小题,共 94 分.请将解答写在答.题.纸.指定位置上.解答应写出文字说明、证明过程或 演算步骤. (15)(本题满分 10 分)
已知函数 f (x) = 1+ x − 1 ,记 a = lim f (x)
sin x x,
x→0
(1)求 a 的值
(2)若当 x → 0 时, f (x) − a 是 xk 的同阶无穷小,求 k
D
∫∫ ∫ ∫ 【解析】:
xydσ = π dθ 1+cosθ r cosθ ⋅ r sin θ ⋅ rdr
0
0
D
∫ = 1 π sin θ ⋅ cosθ ⋅ (1 + cosθ )4 dθ 40
6
∫ = 16
πθ sin
cos θ
(2 cos2
θ
− 1) cos8
θ
dθ
022
2
22
π
湘潭大学数据库原理2012年试题

制卷人签名:制卷日期:审核人签名::审核日期:………………………………………………………………………………………………………………………………………………………………………………………………………………………………………………装…………………… 订……………………线…………………………………………………………………湘潭大学20 年下学期20 级《数据库原理》课程考试试卷(卷)适用年级专业计算机科学与技术、软件工程考试方式闭卷考试时间 120 分钟学院专业班级学号姓名………………………………………………………………………………………………………………一、选择题1.关系R(A,B)和S(B,C)中分别有10个和15个元组,属性B是R的主码,则R?S中元组数目的范围是( A )。
A.(0,15)B.(10,15)C.(10,25)D.(0,150)2. 下列聚合函数中不忽略空值 (null) 的是( B )。
A. SUM(列名)B. COUNT(*)C. MAX(列名)D. AVG(列名)二、填空题1. 有一个关系:学生(学号,姓名,系别),规定学号的值域是8个数字组成的字符串,这一规则属于___用户自定义完整性__约束。
2. 为了确保数据的完整性,要求数据库系统维持事务的四个性质:_______原子性_______、一致性、______隔离性_________和持久性,即ACID 特性。
得分三、For the following relations R and S:R A B C S A B D2 4 4 5 1 92 3 4 3 2 82 4 2 1 1 73 2 3 2 3 51.List all nontrivial functional dependencies satisfied by relation S.A->B A->D AB->D D->A D->B DB->A AD->B2.Given the result of πA,B, D(R?S).四、某汽车运输公司数据库中有一个记录司机运输里程的关系模式:R(司机编号,汽车牌照,行驶公里,车队编号,车队主管)此处每个汽车牌照对应一辆汽车。
2013年-2014年、2016年-2017年湘潭大学854材料科学基础(二)考研真题试题试卷汇编
第 4 页,共 9 页
芝士传媒 × 题源库
页,共 9 页
芝士传媒 × 题源库
...让知识更美味...
第 6 页,共 9 页
芝士传媒 × 题源库
...让知识更美味...
芝士传媒 × 题源库
...让知识更美味...
目 录
2013 年湘潭大学 833 材料科学基础(二)考研真题试题试卷·····································2 2014 年湘潭大学 852 材料科学基础(二)考研真题试题试卷·····································4 2016 年湘潭大学 852 材料科学基础(二)考研真题试题试卷·····································5 2017 年湘潭大学 854 材料科学基础(二)考研真题试题试卷·····································8
第 7 页,共 9 页
芝士传媒 × 题源库
...让知识更美味...
第 8 页,共 9 页
芝士传媒 × 题源库
...让知识更美味...
第 9 页,共 9 页
第 1 页,共 9 页
芝士传媒 × 题源库
...让知识更美味...
第 2 页,共 9 页
芝士传媒 × 题源库
...让知识更美味...
第 3 页,共 9 页
芝士传媒 × 题源库
...让知识更美味...
数2--12真题答案
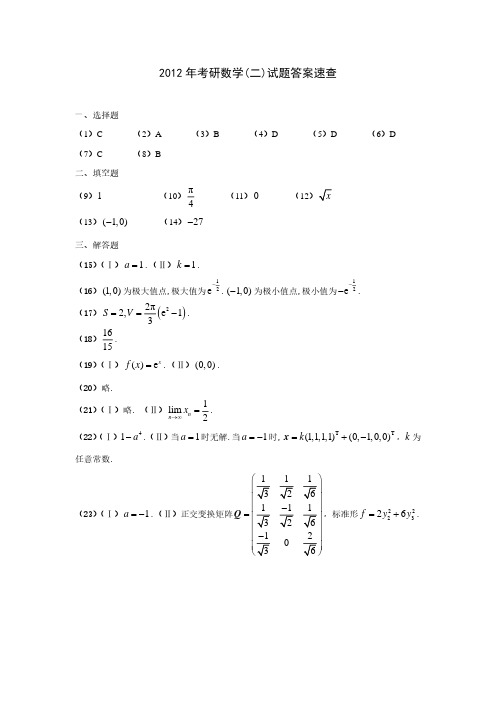
2012年考研数学(二)试题答案速查一、选择题(1)C (2)A (3)B (4)D (5)D (6)D (7)C (8)B 二、填空题(9)1 (10)π4(11)0 (12)x (13)(1,0)− (14)27− 三、解答题(15)(Ⅰ)1a =.(Ⅱ)1k =. (16)(1,0)为极大值点,极大值为12e −.(1,0)−为极小值点,极小值为12e−−.(17)()22π2,e 13S V ==−. (18)1615. (19)(Ⅰ)()e xf x =.(Ⅱ)(0,0). (20)略.(21)(Ⅰ)略. (Ⅱ)1lim 2n n x →∞=. (22)(Ⅰ)41a −.(Ⅱ)当1a =时无解.当1a =−时,TT(1,1,1,1)(0,1,0,0)k =+−x ,k 为任意常数.(23)(Ⅰ)1a =−.(Ⅱ)正交变换矩阵11132611132612036⎛⎫ ⎪ ⎪−⎪=⎪ ⎪− ⎪ ⎪⎝⎭Q ,标准形222326f y y =+.2012年全国硕士研究生入学统一考试数学(二)参考答案一、选择题:1~8小题,每小题4分,共32分.下列每题给出的四个选项中,只有一个选项是符合题目要求的.请将所选项前的字母填在答题纸...指定位置上. (1)【答案】C .【解答】由题可知,22(1)1(1)(1)x x x x y x x x ++==−−+,故1lim ,x y →=∞所以1x =为垂直渐近线; 又由lim 1x y →∞=,故1y =为水平渐近线,无斜渐近线,故曲线渐近线的条数为2.(2)【答案】A.【解答】因为2100()(0)(e 1)(e 2)(e )(0)lim lim(1)(1)!x x nx n x x f x f n f n x x−→→−−−−'===−−,所以选A. (3)【答案】B.【解答】因为0(1,2,)n a n >=,所以数列{}n S 单调递增.如果{}n S 有界,由单调有界收敛准则知数列{}n S 极限存在,而1n n n a S S −=−,则1lim lim()0n n n n n a S S −→∞→∞=−=,即数列{}n a 收敛. 由此可知数列{}n S 有界是数列{}n a 收敛的充分条件. 反之,若{}n a 收敛,{}n S 未必收敛,例如,取1n a =(1,2,)n =,n S n =无上界,故选B. (4)【答案】D. 【解答】因为22π21πe sin d 0,x I I x x −=<⎰故21I I <;222π3π31π2πe sin d e sin d x x I I x x x x −=+⎰⎰22ππ(π)(2π)0esin d e sin d 0x x x x x x ++=−+>⎰⎰,故31I I >.所以选D.(5)【答案】D. 【解答】因为(,)0,f x y x∂>∂所以,固定y 值由12>x x 得1121(,)(,)>f x y f x y ,同理当(,)0,f x y y∂<∂固定x 值由12<y y 得2122(,)(,)>f x y f x y ,所以有答案D.(6)【答案】D.【解答】由二重积分的区域对称性可知π1552πsin 2(1)d d d (1)d πDxx y x y x x y y −−=−=−⎰⎰⎰⎰.(7)【答案】C.【解答】由已知可得134,,0,=ααα所以134,,ααα线性相关,选C. (8)【答案】B.【解答】1223123100(,,)(,,)110001Q ααααααα⎛⎫ ⎪=+= ⎪ ⎪⎝⎭,故11100110001−−⎛⎫ ⎪= ⎪ ⎪⎝⎭Q AQ ,1100100100100100110110010110010001001002001002−⎛⎫⎛⎫⎛⎫⎛⎫⎛⎫⎪ ⎪⎪⎪ ⎪=−= ⎪ ⎪⎪⎪ ⎪ ⎪ ⎪⎪⎪ ⎪⎝⎭⎝⎭⎝⎭⎝⎭⎝⎭P AP ,所以选B.二、填空题:9~14小题,每小题4分,共24分.请将答案写在答题纸...指定位置上. (9)【答案】1.【解答】方程21e yx y −+=两边分别对x 求导,得d d 2e d d y y yx x x−= ①, 由0=x ,0=y ,得d 0d x yx==. 对①式两边再对x 求导,得22222d d d 2e e d d d y y y y y x x x ⎛⎫−=+ ⎪⎝⎭, 由0=x ,0=y ,d 0d x yx==,得22d 1d x yx==.(10)【答案】π4. 【解答】2222111lim ()12n n n n n n →∞++++++122222*********πlim ...lim d 14121111n n n i x n n x n i n n n n →∞→∞=⎛⎫ ⎪ ⎪=++=== ⎪+⎛⎫⎛⎫⎛⎫⎛⎫++++ ⎪ ⎪ ⎪ ⎪ ⎪⎝⎭⎝⎭⎝⎭⎝⎭⎝⎭∑⎰. (11)【答案】0. 【解答】因为1(ln ),z f x y =+所以211,z z f f x x y y ∂∂−''==⇒∂∂20z zx y x y∂∂+=∂∂.(12)【答案】x .【解答】由题知该方程可化为d 3d x xy y y+=,为一阶线性微分方程,带入公式求解可得 3xy y C =+,带入初始条件可得0C =,最终可得结果.(13)【答案】(1,0)−. 【解答】由曲率公式()3/221y k y ''='+,曲线方程代入公式可得.(14)【答案】27−.【解答】由初等矩阵的性质可知010100001B PA A ⎛⎫⎪== ⎪ ⎪⎝⎭,所以,**27BA PAA ==−.三、解答题:15~23小题,共94分.解答应写出文字说明、证明过程或演算步骤.请将答案写在答题纸...指定位置上. (15)(本题满分10分) 解:(Ⅰ)0011sin lim 1lim 1sin sin x x x x x x x x x →→+−⎛⎫−=−=⎪⎝⎭,1a =.(Ⅱ)221000sin 1()sin sin sin lim lim lim sin k k k x x x x x xf x a x x x x x x x x x x x+→→→+−−−+−−== 22110001(sin )(1)1cos 2lim lim lim (2)(2)k k k x x x xx x x x x k x k x +++→→→−+−===++, 因为它们为同阶无穷小量,所以1k =.(16)(本题满分10分)解:()()22222221e0,e0x y x y ffx xy xy++−−∂∂=−==−=∂∂,可解得1,0.x y =⎧⎨=⎩或1,0.x y =−⎧⎨=⎩. 因为22222222222222222(3)e,(1)e ,(1)e xy x y x y f f f x x x y y x xyx y+++−−−∂∂∂=−=−=−∂∂∂∂,所以当1,0.x y =⎧⎨=⎩时,11222e ;0;e A B C −−=−==−.又因为20,0AC B A −><,所以(1,0)为极大值点,极大值为12e−.同理当1,0.x y =−⎧⎨=⎩时,验证可得其为极小值点,极小值为12e −−.(17)(本题满分12分)解:设切点(,)A a b ,切线方程斜率为k ,则1k a=,ln b a =,并且(0,1)与A 两点共线,直线方程为1b ka −=,由此解得221e ,2,ea b k ===.切线方程:211,e y x =+与x 轴交于B 坐标为(1,0),直线AB 的方程22:(1)e 1AB l y x =−−,则 区域D 的面积22e 2e2222112(1)2ln d ln e 1e 12e 1e 1D x x x S x x x x x ⎛⎫−−⎡⎤=−=−−=+−+= ⎪⎢⎥−−⎣⎦⎝⎭⎰区域D 绕x 轴旋转一周所得旋转体的体积()()22e 22212(1)2ππln d e 1e 13x V x x ⎡⎤−⎛⎫=−=−⎢⎥⎪−⎝⎭⎢⎥⎣⎦⎰.(18)(本题满分10分)解:如图,利用极坐标计算,由cos ,sin .x r r r θθ=⎧⎨=⎩,得π1cos 0d d cos sin d Dxy r r r r θσθθθ+=⎰⎰⎰⎰π401sin cos (1cos )d 4θθθθ=+⎰ π401cos (1cos )d cos 4θθθ=−+⎰141116cos (1)d 415t t t t θ−=+=⎰.(19)(本题满分10分)解:(Ⅰ)由()()2()0,f x f x f x '''+−=可知特征方程为220λλ+−=,通解为yxO2πD1cos r θ=+212e e x x y C C −=+,将其带入方程()()2e f x f x ''+=,可得2122e 5e 2e x x x C C −+=, 121,0C C ==.所以()e x f x =.(Ⅱ)由220()()d xy f x f t t =−⎰,得22'2e e d 1,xxt y x t −=+⎰2222202e e d 4e e d 2xxxt xt y t x t x −−''=++⎰⎰,令0,0y x ''==,当0x >时,0y ''>;当0x <时,0y ''<. 所以(0,0)为其拐点.(20)(本题满分11分)证明:令21()ln cos 1(11)12x x f x x x x x +=+−−−<<−,则有()()f x f x =−,为偶函数.所以只需讨论0x >即可.()2211212()lnsin ln sin 11111x x x x f x x x x x x x x x x x +−+'=+−−=+−−−+−−−, ()()22422416(1)()cos 1,()sin 11x x f x x f x x x x −'''''=−−=+−−.当01x <<时,()0f x '''>,则()f x ''单调递增,且(0)2f ''=,所以()0f x ''>. 所以,当01x <<时,()f x '单调递增,且(0)0f '=,所以()f x 递增,且(0)0f =, 所以,当01x <<时,结论成立.同理,在10x −<<时,结论成立.(21)(本题满分11分) 解: (Ⅰ)令1()1,nn n F x x x x −=+++−则12()(1)21n n n F x nx n x x −−'=+−+++,所以该函数在1,12⎛⎫⎪⎝⎭内单调递增.因为1111()102222n n n F =++−=−<, (1)10n F n =−>,所以有零点定理可知方程在1,12⎛⎫⎪⎝⎭内至少有一个实根.又函数单调,所以有且仅有一个实根. (Ⅱ)先证明单调性.()()11111111(1)(1)00n n n n n n n n n n n n n n n n F x F x x x x x x x x −−++++++−=++−−++−=+>,而函数()n F x 单调,所以1n n x x +>,所以数列{}n x 单调递减.又1,12n x ⎛⎫∈⎪⎝⎭,所以数列是有界的.因此数列收敛,且lim 0n n n x →∞=.所以由1(1)1101nn n n n n nn nx x x xx x −−++−=−=−,两端取极限可得1lim 2n n x →∞=.(22)(本题满分11分)解:(Ⅰ)4221(1)(1)A =−=−+a a a ;(Ⅱ)由题可知当0A =时,解得1=a 或1=−a .当1a =时,增广矩阵作初等变换得,()1100101101|0011000002⎛⎫⎪−⎪→ ⎪⎪−⎝⎭A β,()()|r r <A A β,故方程组无解;当1a =−时,增广矩阵作初等变换得,()1001001011|0011000000−⎛⎫⎪−−⎪→ ⎪− ⎪⎝⎭A β,()()|3r r <=A A β,方程组有解,并可求得通解为T T (1,1,1,1)(0,1,0,0)x =+−k ,其中k 为任意常数.(23)(本题满分11分)解: (Ⅰ)由二次型的秩为2,知T()2r =A A ,故()2r =A ,对A 作初等变换,1011010110111000101000a a a ⎛⎫⎛⎫⎪ ⎪⎪ ⎪=→⎪ ⎪−+ ⎪ ⎪−⎝⎭⎝⎭A ,可得1a =−.(Ⅱ)当1a =−时,得T202022224⎛⎫ ⎪= ⎪ ⎪⎝⎭A A .()()T 2020*******λλλλλλλ−−⎛⎫ ⎪−=−−=−− ⎪ ⎪−−−⎝⎭E A A ,可得T A A 的特征值1230,2,6λλλ===.当10λ=时,解方程组T(0)−E A A x =0,得相应的特征向量()T11,1,1=−α;当22λ=时,解方程组T(2)−E A A x =0,得相应的特征向量()T21,1,0=−α;当36λ=时,解方程组T(6)−E A A x =0,得相应的特征向量()T31,1,2=α.因为特征值各不相等,所以特征向量相互正交,故只需单位化,得()T111,1,13=−β,()T 211,1,02=−β,()T 311,1,26=β.于是得到正交矩阵11132611132612036⎛⎫ ⎪ ⎪−⎪=⎪ ⎪− ⎪ ⎪⎝⎭Q . 在正交变换=x Qy 下,二次型的标准型为222326f y y =+.。
