美国高中学生数学竞赛题

合集下载

amc12数学题

amc12数学题篇一：AMC12数学题是指美国数学竞赛（AMC）12级的数学题目。

AMC12是一项面向高中生的数学竞赛，旨在鼓励学生对数学的深入学习和探索。

这些数学题目涵盖了广泛的数学概念和技巧，并要求学生通过逻辑推理和解题技巧来解决问题。

AMC12数学题的难度较高，常常需要学生具备扎实的数学基础和解题能力。

这些题目涉及的数学领域包括代数、几何、数论和概率等。

许多题目都要求学生运用多个数学概念进行综合分析和解答。

因此，参加AMC12数学竞赛是考验学生数学能力和解题能力的良好机会。

解答AMC12数学题需要学生具备良好的数学思维和解题技巧。

学生需要能够正确理解问题并找出解决问题的方法。

在解答过程中，学生需要通过分析和推理来得出结论，同时要注意细节和逻辑的严谨性。

有时候，解答AMC12数学题还需要一些创造性思维和巧妙的数学变换。

参加AMC12数学竞赛对学生有很多好处。

首先，它可以提高学生的数学能力和解题能力，培养学生的数学思维和分析能力。

其次，参加竞赛可以增强学生对数学的兴趣和热爱，激发学生对数学的探索欲望。

此外，竞赛还可以培养学生的竞争意识和团队合作精神。

最重要的是，参加AMC12数学竞赛可以为学生在大学申请中增加一份优势，显示出学生在数学方面的才能和成就。

总之，AMC12数学题是一系列挑战性的数学问题，要求学生具备扎实的数学基础和解题能力。

通过参加AMC12数学竞赛，学生能够提高数学能力，培养数学思维，并为将来的学术和职业发展奠定坚实的基础。

篇二：AMC12（美国数学竞赛12级）是由美国数学协会举办的一项数学竞赛，面向高中学生。

这项竞赛旨在鼓励学生培养解决复杂数学问题的能力，并提供一个展示自己数学才能的平台。

AMC12数学题目通常涵盖广泛的数学领域，包括代数、几何、概率与统计、数论等。

这些题目要求学生在有限的时间内思考、分析和解决问题，考察他们的数学思维能力、推理能力和解决问题的能力。

举一个例子，假设有一道AMC12的几何问题：如图所示，一个直径为12的圆与一个半径为8的圆相切。

美国数学竞赛试题-部分

2000AMC12ProblemsProblem1In the year,the United States will host the International Mathematical Olympiad.Let and be distinct positive integers such that the product.What is the largest possible value of the sum?Problem2Problem3Each day,Jenny ate of the jellybeans that were in her jar at the beginning of that day. At the end of the second day,remained.How many jellybeans were in the jar originally?Problem4The Fibonacci sequence starts with two1s,and each term afterwards is the sum of its two predecessors.Which one of the ten digits is the last to appear in the units position of a number in the Fibonacci sequence?Problem5If where thenProblem6Two different prime numbers between and are chosen.When their sum is subtracted from their product,which of the following numbers could be obtained?Problem7How many positive integers have the property that is a positive integer?Problem8Figures,,,and consist of,,,and non-overlapping squares.If the pattern continued,how many non-overlapping squares would there be in figure?Problem9Mrs.Walter gave an exam in a mathematics class of five students.She entered the scores in random order into a spreadsheet,which recalculated the class average after each score was entered.Mrs. Walter noticed that after each score was entered,the average was always an integer.The scores (listed in ascending order)were71,76,80,82,and91.What was the last score Mrs.Walters entered?Problem10The point is reflected in the-plane,then its image is rotatedby about the-axis to produce,and finally,is translated by5units in the positive-direction to produce.What are the coordinates of?Problem11Two non-zero real numbers,and satisfy.Which of the following is a possible value of?Problem12Let A,M,and C be nonnegative integers such that.What is the maximum value of+++?Problem13One morning each member of Angela’s family drank an8-ounce mixture of coffee with milk.The amounts of coffee and milk varied from cup to cup,but were never zero.Angela drank a quarter of the total amount of milk and a sixth of the total amount of coffee.How many people are in the family?Problem14When the mean,median,and modeof the listare arranged in increasing order,they form a non-constant arithmetic progression.What is the sum of all possible real values of?Problem15Let be a function for which.Find the sum of all values of for which.Problem16A checkerboard of rows and columns has a number written in each square,beginning in the upper left corner,so that the first row is numbered,the second row, and so on down the board.If the board is renumbered so that the left column,top to bottom, is,the second column and so on across the board,some squares have the same numbers in both numbering systems.Find the sum of the numbers in these squares (under either system).Problem17A centered at has radius and contains the point.The segment is tangent to the circle at and.If point lies on and bisects,thenProblem18In year,the day of the year is a Tuesday.In year,the day is also a Tuesday.On what day of the week did th day of year occur?Problem19triangle,,,.Let denote the midpointof and let denote the intersection of with the bisector of angle.Which of the following is closest to the area of the triangle?Problem20If and are positive numbers satisfyingThen what is the value of latex?Problem21Through a point on the hypotenuse of right triangle,lines are drawn parallel to the legs of the triangle so that the triangle is divided into asquare and two smaller right triangles.The area of one of the two small right triangles times the area of the square.The ratio of the area of the other small right triangle to the area of the square isProblem22The graph below shows a portion of the curve defined by the quarticpolynomial.Which of the following is the smallest?Problem23Professor Gamble buys a lottery ticket,which requires that he pick six different integers from through,inclusive.He chooses his numbers so that the sum of the base-ten logarithms of his six numbers is an integer.It so happens that the integers on the winning ticket have the same property—the sum of the base-ten logarithms is an integer.What is the probability that Professor Gamble holds the winning ticket?Problem24If circular arcs and centers at and,respectively,then there exists a circletangent to both and,and to.If the length of is,then the circumference of the circle isProblem25Eight congruent Equilateral triangle each of a different color,are used to construct a regular octahedron.How many distinguishable ways are there to construct the octahedron?(Two colored octahedrons are distinguishable if neither can be rotated to look just like the other.)Problem6For how many positive integers does there exist at least one positive integer n such that?infinitely manyProblem7A arc of circle A is equal in length to a arc of circle B.What is the ratio of circle A's area and circle B's area?Problem8Betsy designed a flag using blue triangles,small white squares,and a red center square,as shown. Let be the total area of the blue triangles,the total area of the white squares,and the area of the red square.Which of the following is correct?Problem9Jamal wants to save30files onto disks,each with1.44MB space.3of the files take up0.8MB, 12of the files take up0.7MB,and the rest take up0.4MB.It is not possible to split a file onto2different disks.What is the smallest number of disks needed to store all30files?Problem10Sarah places four ounces of coffee into an eight-ounce cup and four ounces of cream into a second cup of the same size.She then pours half the coffee from the first cup to the second and,after stirring thoroughly,pours half the liquid in the second cup back to the first.What fraction of the liquid in the first cup is now cream?Problem11Mr.Earl E.Bird gets up every day at8:00AM to go to work.If he drives at an average speed of40miles per hour,he will be late by3minutes.If he drives at an average speed of60milesper hour,he will be early by3minutes.How many miles per hour does Mr.Bird need to drive to get to work exactly on time?Problem12Both roots of the quadratic equation are prime numbers.The number of possible values of isProblem13Two different positive numbers and each differ from their reciprocals by.What is?Problem14For all positive integers,let.Let. Which of the following relations is true?Problem15The mean,median,unique mode,and range of a collection of eight integers are all equal to8. The largest integer that can be an element of this collection isProblem16Tina randomly selects two distinct numbers from the set{1,2,3,4,5},and Sergio randomly selects a number from the set{1,2,...,10}.What is the probability that Sergio's number is larger than the sum of the two numbers chosen by Tina?Problem17Several sets of prime numbers,such as use each of the nine nonzero digits exactly once.What is the smallest possible sum such a set of primes could have?Problem18Let and be circles definedby and respectively.What is the length of the shortest line segment that is tangent to at and to at?Problem19The graph of the function is shown below.How many solutions does theequation have?Problem20Suppose that and are digits,not both nine and not both zero,and the repeatingdecimal is expressed as a fraction in lowest terms.How many different denominators are possible?Problem21Consider the sequence of numbers:For,the-th term of the sequence is the units digit of the sum of the two previous terms.Let denote the sum of the first terms of this sequence.The smallest value of for which is:Problem22Triangle is a right triangle with as its right angle,, and.Let be randomly chosen inside,and extend to meet at. What is the probability that?Problem23In triangle,side and the perpendicular bisector of meet in point,and bisects.If and,what is the area of triangle?SAT II数学词汇表代数部分1.基础add，plus加subtract减difference差multiply times乘product积divide除divisible可被整除的divided evenly被整除dividend被除数divisor因子，除数quotient商remainder余数factorial阶乘power乘方radical sign,root sign根号round to四舍五入to the nearest四舍五入2.有关集合union并集proper subset真子集solution set解集3.有关代数式、方程和不等式algebraic term代数项like terms,similar terms同类项numerical coefficient数字系数literal coefficient字母系数inequality不等式triangle inequality三角不等式range值域original equation原方程equivalent equation同解方程等价方程linear equation线性方程(e.g.5x+6=22)4.有关分数和小数proper fraction真分数improper fraction假分数mixed number带分数vulgar fraction，common fraction普通分数simple fraction简分数complex fraction繁分数numerator分子denominator分母(least)common denominator(最小)公分母quarter四分之一decimal fraction纯小数infinite decimal无穷小数recurring decimal循环小数tenths unit十分位5.基本数学概念arithmetic mean算术平均值weighted average加权平均值geometric mean几何平均数exponent指数，幂base乘幂的底数,底边cube立方数，立方体square root平方根cube root立方根common logarithm常用对数digit数字constant常数variable变量inverse function反函数complementary function余函数linear一次的，线性的factorization因式分解absolute value绝对值，e.g.｜-32｜=32round off四舍五入6.有关数论natural number自然数positive number正数negative number负数odd integer奇整数,odd number奇数even integer,even number偶数integer,whole number整数positive whole number正整数negative whole number负整数consecutive number连续整数real number,rational number实数,有理数irrational(number)无理数inverse倒数composite number合数e.g.4,6,8,9,10,12,14,15……reciprocal 倒数common divisor公约数multiple倍数(least)common multiple(最小)公倍数(prime)factor(质)因子common factor公因子prime number质数e.g.2,3,5,7,11,13,15……ordinary scale,decimal scale十进制nonnegative非负的tens十位units个位mode众数median中数common ratio公比7.数列arithmetic progression(sequence)等差数列geometric progression(sequence)等比数列8.其它approximate近似(anti)clockwise(逆)顺时针方向cardinal基数ordinal序数direct proportion正比distinct不同的estimation估计，近似parentheses括号proportion比例permutation排列combination组合table表格trigonometric function三角函数unit单位,位几何部分1.所有的角alternate angle内错角corresponding angle同位角vertical angle对顶角central angle圆心角interior angle内角exterior angle外角supplementary angles补角complementary angle余角adjacent angle邻角acute angle锐角obtuse angle钝角right angle直角round angle周角straight angle平角included angle夹角2.所有的三角形equilateral triangle等边三角形scalene triangle不等边三角形isosceles triangle等腰三角形right triangle直角三角形oblique斜三角形inscribed triangle内接三角形3.有关收敛的平面图形，除三角形外semicircle半圆concentric circles同心圆quadrilateral四边形pentagon五边形hexagon六边形heptagon七边形octagon八边形nonagon九边形decagon十边形polygon多边形parallelogram平行四边形equilateral等边形plane平面square正方形，平方rectangle长方形regular polygon正多边形rhombus菱形trapezoid梯形4.其它平面图形arc弧line,straight line直线line segment线段parallel lines平行线segment of a circle弧形5.有关立体图形cube立方体，立方数rectangular solid长方体regular solid/regular polyhedron正多面体circular cylinder圆柱体cone圆锥sphere球体solid立体的6.有关图形上的附属物altitude高depth深度side边长circumference,perimeter周长radian弧度surface area表面积volume体积arm直角三角形的股cross section横截面center of acircle圆心chord弦radius半径angle bisector角平分线diagonal对角线diameter直径edge棱face of a solid立体的面hypotenuse斜边included side夹边leg三角形的直角边median of a triangle三角形的中线base底边，底数(e.g.2的5次方，2就是底数) opposite直角三角形中的对边midpoint中点endpoint端点vertex(复数形式vertices)顶点tangent切线的transversal截线intercept截距7.有关坐标coordinate system坐标系rectangular coordinate直角坐标系origin原点abscissa横坐标ordinate纵坐标Number line数轴quadrant象限slope斜率complex plane复平面8.其它plane geometry平面几何trigonometry三角学bisect平分circumscribe外切inscribe内切intersect相交perpendicular垂直Pythagorean theorem勾股定理congruent全等的multilateral多边的其它相关词汇cent美分penny一美分硬币nickel5美分硬币dime一角硬币dozen打(12个)score廿(20个)Centigrade摄氏Fahrenheit华氏quart夸脱gallon加仑(1gallon=4quart)yard码meter米micron微米inch英寸foot英尺minute分(角度的度量单位，60分=1度) square measure平方单位制cubic meter立方米pint品脱(干量或液量的单位)。

美国数学竞赛AMC题目及答案

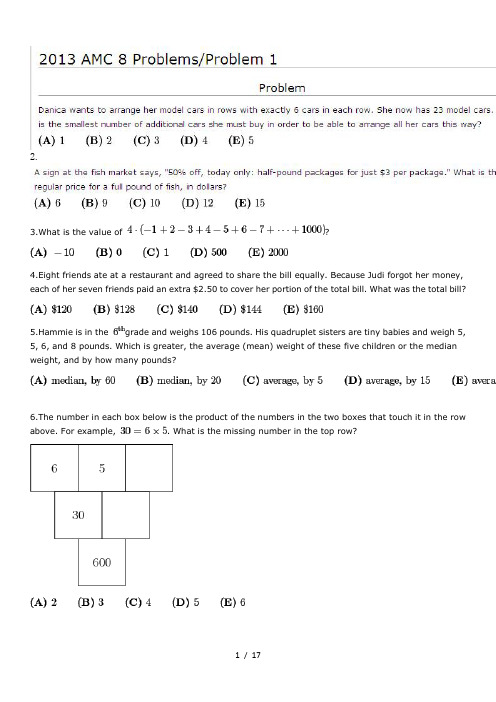
2.3.What is the value of ?4.Eight friends ate at a restaurant and agreed to share the bill equally. Because Judi forgot her money, each of her seven friends paid an extra $2.50 to cover her portion of the total bill. What was the total bill?5.Hammie is in the grade and weighs 106 pounds. His quadruplet sisters are tiny babies and weigh 5, 5, 6, and 8 pounds. Which is greater, the average (mean) weight of these five children or the median weight, and by how many pounds?6.The number in each box below is the product of the numbers in the two boxes that touch it in the row above. For example, . What is the missing number in the top row?7.Trey and his mom stopped at a railroad crossing to let a train pass. As the train began to pass, Trey counted 6 cars in the first 10 seconds. It took the train 2 minutes and 45 seconds to clear the crossing at a constant speed. Which of the following was the most likely number of cars in the train?8.A fair coin is tossed 3 times. What is the probability of at least two consecutive heads?9.The Incredible Hulk can double the distance he jumps with each succeeding jump. If his first jump is 1 meter, the second jump is 2 meters, the third jump is 4 meters, and so on, then on which jump will he first be able to jump more than 1 kilometer?10.What is the ratio of the least common multiple of 180 and 594 to the greatest common factor of 180 and 594?11. Ted's grandfather used his treadmill on 3 days this week. He went 2 miles each day. On Monday he jogged at a speed of 5 miles per hour. He walked at the rate of 3 miles per hour on Wednesday and at 4 miles per hour on Friday. If Grandfather had always walked at 4 miles per hour, he would have spent less time on the treadmill. How many minutes less?12. At the 2013 Winnebago County Fair a vendor is offering a "fair special" on sandals. If you buy one pair of sandals at the regular price of $50, you get a second pair at a 40% discount, and a third pair at half the regular price. Javier took advantage of the "fair special" to buy three pairs of sandals. What percentage of the $150 regular price did he save?13. When Clara totaled her scores, she inadvertently reversed the units digit and the tens digit of one score. By which of the following might her incorrect sum have differed from the correct one?14. Let the two digits be and .The correct score was . Clara misinterpreted it as . The difference between the two is which factors into . Therefore, since the difference is a multiple of 9, the only answerchoice that is a multiple of 9 is .15. If , , and , what is the product of , , and ?16. A number of students from Fibonacci Middle School are taking part in a community service project. The ratio of -graders to -graders is , and the the ratio of -graders to -graders is . What is the smallest number of students that could be participating in the project?17. The sum of six consecutive positive integers is 2013. What is the largest of these six integers?18. Isabella uses one-foot cubical blocks to build a rectangular fort that is 12 feet long, 10 feet wide, and 5 feet high. The floor and the four walls are all one foot thick. How many blocks does the fort contain?--Arpanliku 16:22, 27 November 2013 (EST) Courtesy of Lord.of.AMC19. Bridget, Cassie, and Hannah are discussing the results of their last math test. Hannah shows Bridget and Cassie her test, but Bridget and Cassie don't show theirs to anyone. Cassie says, 'I didn't get the lowest score in our class,' and Bridget adds, 'I didn't get the highest score.' What is the ranking of the three girls from highest to lowest?20. A rectangle is inscribed in a semicircle with longer side on the diameter. What is the area of the semicircle?21. Samantha lives 2 blocks west and 1 block south of the southwest corner of City Park. Her school is 2 blocks east and 2 blocks north of the northeast corner of City Park. On school days she bikes on streets to the southwest corner of City Park, then takes a diagonal path through the park to the northeast corner, and then bikes on streets to school. If her route is as short as possible, how many different routes can she take?22. Toothpicks are used to make a grid that is 60 toothpicks long and 32 toothpicks wide. How many toothpicks are used altogether?23. Angle of is a right angle. The sides of are the diameters of semicircles as shown. The area of the semicircle on equals , and the arc of the semicircle on has length .What is the radius of the semicircle on ?24. Squares , , and are equal in area. Points and are the midpoints of sidesand , respectively. What is the ratio of the area of the shaded pentagon to the sum of the areas of the three squares?25. A ball with diameter 4 inches starts at point A to roll along the track shown. The track is comprised of 3 semicircular arcs whose radii are inches, inches, and inches, respectively. The ball always remains in contact with the track and does not slip. What is the distance the center of theball travels over the course from A to B?1.2.The 50% off price of half a pound of fish is $3, so the 100%, or the regular price, of a half pound of fish is $6. Consequently, if half a pound of fish costs $6, then a whole pound of fish is dollars.3.Notice that we can pair up every two numbers to make a sum of 1:Therefore, the answer is .4.Each of her seven friends paid to cover Judi's portion. Therefore, Judi's portion must be . Since Judi was supposed to pay of the total bill, the total bill must be .5.The median here is obviously less than the mean, so option (A) and (B) are out.Lining up the numbers (5, 5, 6, 8, 106), we see that the median weight is 6 pounds.The average weight of the five kids is .Therefore, the average weight is bigger, by pounds, making the answer.6.Solution 1: Working BackwardsLet the value in the empty box in the middle row be , and the value in the empty box in the top row be . is the answer we're looking for.We see that , making .It follows that , so .Solution 2: Jumping Back to the StartAnother way to do this problem is to realize what makes up the bottommost number. This method doesn't work quite as well for this problem, but in a larger tree, it might be faster. (In this case, Solution 1 would be faster since there's only two missing numbers.)Again, let the value in the empty box in the middle row be , and the value in the empty box in the top row be . is the answer we're looking for.We can write some equations:Now we can substitute into the first equation using the two others:7.If Trey saw , then he saw .2 minutes and 45 seconds can also be expressed as seconds.Trey's rate of seeing cars, , can be multiplied by on the top and bottom (and preserve the same rate):. It follows that the most likely number of cars is . Solution 2minutes and seconds is equal to .Since Trey probably counts around cars every seconds, there are groups of cars that Trey most likely counts. Since , the closest answer choice is .8.First, there are ways to flip the coins, in order.The ways to get two consecutive heads are HHT and THH.The way to get three consecutive heads is HHH.Therefore, the probability of flipping at least two consecutive heads is .9.This is a geometric sequence in which the common ratio is 2. To find the jump that would be over a 1000 meters, we note that .However, because the first term is and not , the solution to the problem is10. To find either the LCM or the GCF of two numbers, always prime factorize first.The prime factorization of .The prime factorization of .Then, find the greatest power of all the numbers there are; if one number is one but not the other, use it (this is ). Multiply all of these to get 5940.For the GCF of 180 and 594, use the least power of all of the numbers that are in both factorizations and multiply. = 18.Thus the answer = = .We start off with a similar approach as the original solution. From the prime factorizations, the GCF is .It is a well known fact that . So we have,.Dividing by yields .Therefore, .11. We use that fact that . Let d= distance, r= rate or speed, and t=time. In this case, let represent the time.On Monday, he was at a rate of . So, .For Wednesday, he walked at a rate of . Therefore, .On Friday, he walked at a rate of . So, .Adding up the hours yields + + = .We now find the amount of time Grandfather would have taken if he walked at per day. Set up the equation, .To find the amount of time saved, subtract the two amounts: - = . To convert this to minutes, we multiply by .Thus, the solution to this problem is12. First, find the amount of money one will pay for three sandals without the discount. We have.Then, find the amount of money using the discount: .Finding the percentage yields .To find the percent saved, we have13. Let the two digits be and .The correct score was . Clara misinterpreted it as . The difference between the two is which factors into . Therefore, since the difference is a multiple of 9, the only answerchoice that is a multiple of 9 is .14. The probability that both show a green bean is . The probability that both show a red bean is . Therefore the probability is15.Therefore, .Therefore, .To most people, it would not be immediately evident that , so we can multiply 6's until we get the desired number:, so .Therefore the answer is .16. Solution 1: AlgebraWe multiply the first ratio by 8 on both sides, and the second ratio by 5 to get the same number for 8th graders, in order that we can put the two ratios together:Therefore, the ratio of 8th graders to 7th graders to 6th graders is . Since the ratio is in lowest terms, the smallest number of students participating in the project is .Solution 2: FakesolvingThe number of 8th graders has to be a multiple of 8 and 5, so assume it is 40 (the smallest possibility). Then there are 6th graders and 7th graders. The numbers of students is17. Solution 1The mean of these numbers is . Therefore the numbers are, so the answer isSolution 2Let the number be . Then our desired number is .Our integers are , so we have that.Solution 3Let the first term be . Our integers are . We have,18. Solution 1There are cubes on the base of the box. Then, for each of the 4 layers above the bottom (as since each cube is 1 foot by 1 foot by 1 foot and the box is 5 feet tall, there are 4 feet left), there arecubes. Hence, the answer is .Solution 2We can just calculate the volume of the prism that was cut out of the original box. Each interior side of the fort will be feet shorter than each side of the outside. Since the floor is foot, the height will be feet. So the volume of the interior box is .The volume of the original box is . Therefore, the number of blocks contained inthe fort is .19. If Hannah did better than Cassie, there would be no way she could know for sure that she didn't get the lowest score in the class. Therefore, Hannah did worse than Cassie. Similarly, if Hannah did worse than Bridget, there is no way Bridget could have known that she didn't get the highest in the class.Therefore, Hannah did better than Bridget, so our order is .20.A semicircle has symmetry, so the center is exactly at the midpoint of the 2 side on the rectangle, making the radius, by the Pythagorean Theorem, . The area is .21.The number of ways to get from Samantha's house to City Park is , and the number of ways toget from City Park to school is . Since there's one way to go through City Park (just walking straight through), the number of different ways to go from Samantha's house to City Park to school .22. There are vertical columns with a length of toothpicks, and there are horizontal rows with a length of toothpicks. An effective way to verify this is to try a small case, i.e. a grid of toothpicks.Thus, our answer is .23. Solution 1If the semicircle on AB were a full circle, the area would be 16pi. Therefore the diameter of the first circle is 8. The arc of the largest semicircle would normally have a complete diameter of 17. The Pythagoreantheorem says that the other side has length 15, so the radius is .Solution 2We go as in Solution 1, finding the diameter of the circle on AC and AB. Then, an extended version of the theorem says that the sum of the semicircles on the left is equal to the biggest one, so the area of thelargest is , and the middle one is , so the radius is .24.First let (where is the side length of the squares) for simplicity. We can extend until it hits theextension of . Call this point . The area of triangle then is The area of rectangle is . Thus, our desired area is . Now, the ratio of the shaded area to thecombined area of the three squares is .Let the side length of each square be .Let the intersection of and be .Since , . Since and are vertical angles, they are congruent. We also have by definition.So we have by congruence. Therefore, .Since and are midpoints of sides, . This combined with yields.The area of trapezoid is .The area of triangle is .So the area of the pentagon is .The area of the squares is .Therefore, .Let the intersection of and be .Now we have and .Because both triangles has a side on congruent squares therefore .Because and are vertical angles .Also both and are right angles so .Therefore by AAS(Angle, Angle, Side) .Then translating/rotating the shaded into the position ofSo the shaded area now completely covers the squareSet the area of a square asTherefore, .25. Solution 1The radius of the ball is 2 inches. If you think about the ball rolling or draw a path for the ball (see figure below), you see that in A and C it loses inches, and it gains inches on B.So, the departure from the length of the track means that the answer is . Solution 2The total length of all of the arcs is . Since we want the path from the center, the actual distance will be shorter. Therefore, the only answer choice less than is . Thissolution may be invalid because the actual distance can be longer if the path the center travels is on the outside of the curve, as it is in the middle bump.古希腊哲学大师亚里士多德说：人有两种，一种即“吃饭是为了活着”,一种是“活着是为了吃饭”.一个人之所以伟大，首先是因为他有超于常人的心。

2019年美国数学竞赛(AMC10B)的试题与解答

这时第二个容器里充满了 3 的水. 问小容器与大容器的容积 4
之比是多少?
解: 设第一、二个容器的容积分别为 V1 和 V2, 依题意有
5 6 V1 =
3 4 V2,
从而
V1 V2
=
3 4 5 6
=
9 , 故 (D) 正确. 10
2. Consider the statement, ”If n is not prime, then n − 2 is
√ 由垂线段最短知, m2 + n2
|√am + bn|
=
√ 5.
当且仅当
a2 + b2
OA 与直线 ax + by = 5 垂直即 an = bm 时取最小值.
解法 2 由柯西不等式, (a2 +b2)(m2 +n2) (am+bn)2,
即 5(m2 + n2) 等号成立.
√ 25, m2 + n2
6 ter and the second was empty. She poured all the water from the
first container into the second container, at which point the sec3
ond container was full of water. What is the ratio of the volume 4
解: 反例是指符合命题的条件但不符合命题的结论的例
子, 即满足“若 n 不是素数, 则 n − 2 是素数. ”选项中只有 27
满足, 故 (E) 正确.
评注运用柯西不等式关键是“配凑”形式, 充分利用已
知条件. 当出现分式和的形式时, 一般构造分式分母和作为

美国高中学生数学竞赛题

美国高中学生数学竞赛题1.(1995年文理)设(3x-1)6=a6x6+a5x5+a4x4+a3x3+a2x2+a1x+a0，求a6+a5+a4+a3+a2+a1+a0的值。

答案：64。

2.(1989年文)如果(1-2x)7=a0+a1x+a2x2+…+a7x7，那么a1+a2+…+a7的值等于()A.-2B.-1C.0D.2答案：(A)3.(1989年理)已知(1-2x)7=a0+a1x+a2x2+…+a7x7，那么a1+a2+…+a7=____。

答案：-2。

题源：(美28届10题)若(3x-1)7=a7x7+a6x6+…+a0，那么a7+a6+…+a0等于()A.0B.1C.64D.-64E.128答案：(E)改编点评：1题将指数7改为6，改为简答题；2题将底数(3x-1)改为(1-2x)，展开式改为x的升幂排列，所求结论中去掉了常数项a0，3题改编方法同2题，改为填空题。

4.(1990年文)已知f(x)=x5+ax3+bx-8，且f(-2)=10，那么f(2)等于()A.-26B.-18C.-10D.10答案：(A)题源：(美33届12题)设f(x)=ax7+bx3+cx-5，其中a.b和c是常数，如图f(-7)=7，那么f(7)等于()A.-17B.-7C.14D.21E.不能唯一确定答案：(A)改编点评：降低了次数，减少了一个字母系数，降低了难度。

5.(1990年文理)如果实数x、y满足等式(x-2)2+y2=3，那么的最大值是()A. B. C. D.答案：(D)题源：(美35届29题)在满足方程(x-3)2+(y-3)2=6的实数对(x，y)中，的最大值是()A.3+2B.2+C.3D.6E.6+2答案：(A)改编点评：圆方程中的圆心坐标、半径作了改变，题设的叙述方式也作了变化。

6.(1990年文理)函数y=+++的值域是()A.{-2，4}B.{-2，0，4}C.{-2，0，2，4}D.{-4，-2，0，4}答案：(B)题源：(美28届8题)非零实数的每一个三重组(a，b，c)构成一个数。

2019美国数学联赛高三组试题(2019AMC12A)

1、一个半径为4英尺的披萨的面积比一个半径为3英尺披萨大N ％，那个整数最接近于N ？ A 、25 B 、33 C 、44 D 、66 E 、782、如果a 是b 的150％，那么b 3是a 的百分之几？ A 、50B 、3266C 、150D 、200E 、4503、一个盒子里由28个红球，20个绿球，19个黄球，13个蓝球，11个白球，和9个黑球。

至少要从盒子里一次性取出多少个球，才能确保有其中有15个颜色相同的球。

A 、75 B 、76 C 、79 D 、84 E 、914、若干连续整数的和为45，这些连续整数中，最大的整数为n ，则n 的最大值是什么？ A 、9 B 、25 C 、45 D 、90 E 、1205、两条直线的斜率分别为2和21，相交于点()2,2，两条直线和直线10=+y x 所围成三角形的面积是多少？ A 、4B 、24C 、6D 、8E 、266、如下图所示，直线l 上有着有一个正方形和一条线段构成的无限多个一样的花纹。

下面四种平面变换中，有多少个是变换后所得的图形和原来图形相同的变换。

（1）绕着某个直线l 上的点的旋转变换；（2）某个沿着于直线l 平行方向的平移变换；（3）以直线l 为对称轴的反射变换；（4）以垂直于直线l 的直线为对称轴的反射变换 A 、0 B 、1 C 、2 D 、3E 、47、Melenie 计算2019年365天的日期的平均数μ，中位数M ，和众数。

记12月1日为121，12月2日为122，12月28日为1228，7月31日为731。

设所有众数的平寻书为d ，下面那个选项是对的？ A 、M d <<μB 、μ<<d MC 、μ==M dD 、μ<<M dE 、M d <<μ8、在平面有四条不同的直线，这些直线恰好有N 个交点，那么N 所有可能取值之和是多？ A 、14 B 、16 C 、18 D 、19 E 、21 9、已知一个数列{}n a 满足11=a ，732=a ，并且12122-----⋅=n n n n n a a aa a 对于任意3≥n 恒成立，而且2019a 可以写成()*,N q p qp∈。

精选美国amc中文版试题及答案

2000到2012年A M C10美国数学竞赛P 0 A 0B 0 C0 D 0 全美中学数学分级能力测验(AMC 10)2000年第01届美国AMC10 (2000年2月日时间75分钟)1. 国际数学奥林匹亚将于2001年在美国举办，假设I 、M 、O 分别表示不同的正整数，且满足I ?M ?O =2001，则试问I ?M ?O 之最大值为。

(A) 23 (B) 55 (C) 99 (D) 111 (E) 6712. 2000(20002000)为。

(A) 40 (B) 50 (C) 55 (D) 60 (E) 754. Candra 每月要付给网络公司固定的月租费及上网的拨接费，已知她12月的账单为12.48元，而她1月的账单为17.54元，若她1月的上网时间是12月的两倍，试问月租费是元。

(A) 2.53 (B) 5.06 (C) 6.24 (D) 7.42 (E) 8.775. 如图M ，N 分别为PA 与PB 之中点，试问当P 在一条平行AB 的直在线移动时，下列各数值有项会变动。

(a) MN 长 (b) △PAB 之周长 (c) △PAB 之面积 (d) ABNM 之面积 (A) 0项 (B) 1项 (C) 2项 (D) 3项 (E) 4项6. 费氏数列是以两个1开始，接下来各项均为前两项之和，试问在费氏数列各项的个位数字中，最后出现的阿拉伯数字为。

(A) 0 (B) 4 (C) 6 (D) 7 (E) 97. 如图，矩形ABCD 中，AD =1，P 在AB 上，且DP 与DB 三等分 ?ADC ，试问△BDP 之周长为。

高中数学竞赛典型题目

数学竞赛典型题目（一）1.(美国数学竞赛)设n a a a ,,,21是整数列,并且他们的最大公因子是1.令S 是一个整数集,具有性质:（1）),,2,1(n i S a i （2）}),,2,1{,(n ji S a a ji,其中j i,可以相同（3）对于S y x,，若S yx，则Syx证明：S 为全体整数的集合。

2．(美国数学竞赛)c b a ,,是正实数，证明：3252525)()3)(3)(3(c b a ccbbaa3．(加拿大数学竞赛)T 为1002004的所有正约数的集合，求集合T 的子集S 中的最大可能的元素个数。

其中S 中没有两个元素，一个是另一个的倍数。

4．(英国数学竞赛)证明：存在一个整数n 满足下列条件：（1）n 的二进制表达式中恰好有2004个1和2004个0；（2）2004能整除n .5．(英国数学竞赛)在0和1之间，用十进制表示为21.0a a 的实数x 满足：在表达式中至多有2004个不同的区块形式，)20041(20031ka a a kkk ，证明：x 是有理数。

6．(亚太地区数学竞赛)求所有由正整数组成的有限非空数集S ，满足：如果S nm,，则Sn m n m),(7．(亚太地区数学竞赛)平面上有2004个点，并且无三点共线，S 为通过任何两点的直线的集合。

证明：点可以被染成两种颜色使得两点同色当且仅当S 中有奇数条直线分离这两点。

8．(亚太地区数学竞赛)证明：)()!1(*2N n nnn 是偶数。

9．(亚太地区数学竞赛)z y x ,,是正实数，证明：)(9)2)(2)(2(222zx yz xy zyx10．（越南数学竞赛）函数f 满足)0(2sin 2cos )(cot xx xx f ，令)11)(1()()(xx f x f x g ，求)(x g 在区间]1,1[的上最值。

11．（越南数学竞赛）定义17612)(,91524)(2323x xxx q x xxx p ，证明：（1）每个多项式都有三个不同的实根；（2）令A 为)(x p 的最大实根，B 为)(x q 的最大实根，证明：4322B A 12．（越南数学竞赛）令F 为所有满足R R f :且x x f f x f )]2([)3(对任意R x成立的函数f 的集合。

AMC8(美国数学竞赛)历年真题、答案及中英文解析

AMC8（美国数学竞赛）历年真题、答案及中英文解析艾蕾特教育的AMC8 美国数学竞赛考试历年真题、答案及中英文解析：AMC8-2020年：真题 --- 答案---解析（英文解析+中文解析）AMC8 - 2019年：真题----答案----解析（英文解析+中文解析）AMC8 - 2018年：真题----答案----解析（英文解析+中文解析）AMC8 - 2017年：真题----答案----解析（英文解析+中文解析）AMC8 - 2016年：真题----答案----解析（英文解析+中文解析）AMC8 - 2015年：真题----答案----解析（英文解析+中文解析）AMC8 - 2014年：真题----答案----解析（英文解析+中文解析）AMC8 - 2013年：真题----答案----解析（英文解析+中文解析）AMC8 - 2012年：真题----答案----解析（英文解析+中文解析）析）AMC8 - 2010年：真题----答案----解析（英文解析+中文解析）AMC8 - 2009年：真题----答案----解析（英文解析+中文解析）AMC8 - 2008年：真题----答案----解析（英文解析+中文解析）AMC8 - 2007年：真题----答案----解析（英文解析+中文解析）AMC8 - 2006年：真题----答案----解析（英文解析+中文解析）AMC8 - 2005年：真题----答案----解析（英文解析+中文解析）AMC8 - 2004年：真题----答案----解析（英文解析+中文解析）AMC8 - 2003年：真题----答案----解析（英文解析+中文解析）AMC8 - 2002年：真题----答案----解析（英文解析+中文解析）AMC8 - 2001年：真题----答案----解析（英文解析+中文解析）AMC8 - 2000年：真题----答案----解析（英文解析+中文解析）析）AMC8 - 1998年：真题----答案----解析（英文解析+中文解析）AMC8 - 1997年：真题----答案----解析（英文解析+中文解析）AMC8 - 1996年：真题----答案----解析（英文解析+中文解析）AMC8 - 1995年：真题----答案----解析（英文解析+中文解析）AMC8 - 1994年：真题----答案----解析（英文解析+中文解析）AMC8 - 1993年：真题----答案----解析（英文解析+中文解析）AMC8 - 1992年：真题----答案----解析（英文解析+中文解析）AMC8 - 1991年：真题----答案----解析（英文解析+中文解析）AMC8 - 1990年：真题----答案----解析（英文解析+中文解析）AMC8 - 1989年：真题----答案----解析（英文解析+中文解析）AMC8 - 1988年：真题----答案----解析（英文解析+中文解析）析）AMC8 - 1986年：真题----答案----解析（英文解析+中文解析）AMC8 - 1985年：真题----答案----解析（英文解析+中文解析）◆AMC介绍◆AMC（American Mathematics Competitions) 由美国数学协会（MAA）组织的数学竞赛，分为 AMC8 、 AMC10、 AMC12 。

2021年全美数学竞赛108Problems

10
Problem (2021 AMC 10B Problem 11) Grandma has just ﬁnished baking a large rectangular pan of brownies. She is planning to make rectangular pieces of equal size and shape, with straight cuts parallel to the sides of the pan. Each cut must be made entirely across the pan. Grandma wants to make the same number of interior pieces as pieces along the perimeter of the pan. What is the greatest possible number of brownies she can produce? (A) 24 (B) 30 (C) 48 (D) 60 (E) 64
16
Problem (2021 AMC 10B Problem 17) Raven, Oscar, Aditi, Tyrone, and Kim play a card game. Each person is given 2 cards out of a set of 10 cards numbered 1, 2, 3, . . . , 10. The score of of a player is the sum of the numbers of their cards. The scores of the players are as follows: Ravon-11, Oscar-4, Aditi-7, Tyrone-16, Kim-17. Which of the following statements is true? (A) Ravon was given card 3. (B) Aditi was given card 3. (C) Ravon was given card 4. (D) Aditi was given card 4. (E) Tyrone was given card 7.

2020年美国数学竞赛(AMC12A)的试题与解答

2020年美国数学竞赛(AMC12A)的试题与解答华南师范大学数学科学学院(510631)李湖南1.Carlos took 70%of a whole pie.Maria took one third of the remainder.What portion of the original pie was left?(A)10%(B)15%(C)20%(D)30%(E)35%译文卡洛斯取走了一整块派的70%,玛利亚取走了剩余的三分之一.问这块派还剩下多少?解(1−70%)×(1−13)=20%,故(C)正确.2.The acronym AMC is shown in the rectangular grid be-low with grid lines spaced 1unit apart.In units,what is the sum of the lengths of the line segments that form the acronym AMC?(A)17(B)15+2√2(C)13+4√2(D)11+6√2(E)21译文下图是矩形格子中的首字母缩写AMC ,其中小正方形格子的边长为1.则字母AMC 的线段长度之和是多少?解观察图形可知,直线段长度和为13,而小正方形对角线长度为√2,共4条,故总和为13+4√2,(C)正确.3.A driver travels for 2hours at 60miles per hour,during which her car gets 30miles per gallon of gasoline.She is paid $0.50per mile,and her only expense is gasoline at $2.00per gal-lon.What is her net rate of pay,in dollars per hour,after this expense?(A)20(B)22(C)24(D)25(E)26译文一位司机以60英里/小时的速度驾车2小时,她的车每跑30英里需要消耗1加仑汽油.她能获得0.50美元/英里的报酬,唯一的花费就是2美元/加仑的汽油.问她每小时除去消耗之后的净收益是多少美元?解1个小时她能跑60英里,获得60×0.50=30美元,汽油费为60÷30×2=4美元,故净收益为26美元,(E)正确.4.How many 4-digit positive integers (that is,integers be-tween 1000and 9999,inclusive)having only even digits are di-visible by 5?(A)80(B)100(C)125(D)200(E)500译文有多少个四位的正整数(也就是在1000和9999之间的整数)能被5整除且所有数字均为偶数?解依题意,符合条件的四位数的个位数只能是0,十位数和百位数可以是0,2,4,6,8,千位数只能是2,4,6,8,共有1×5×5×4=100种选择,故(B)正确.5.The 25integers from -10to 14,inclusive,can be arranged to form a 5-by-5square in which the sum of the numbers in each row,the sum of the numbers in each column,the sum of the num-bers along each of the main diagonals are all the same.What is the value of this common sum?(A)2(B)5(C)10(D)25(E)50译文将25个整数分别是从-10到14,放入5×5的格子中,使得格子里的每行、每列和两条对角线的数字和均相等.问这个数字和是多少?解这是一个5阶幻方问题,25个数字之和是(−10)+(−9)+···+13+14=50,分别放入5行,故每行的数字和是10,(C)正确.6.In the place figure shown below,3of the unit squares have been shaded.What is the least number of additional unit squares that must be shaded so that the resulting figure has two lines ofsymmetry?(A)4(B)5(C)6(D)7(E)8译文如下图所示,3个单元格被涂成阴影部分.问至少还需要把多少个单元格涂成阴影才能使整个图有两条对称轴?解由于这是一个4×5的矩形,两条对称轴只可能是长和宽两条边的中垂线,从而至少有7个单元格需要填涂,如右图所示.故(D)正确.7.Seven cubes,whose volumes are 1,8,27,64,125,216,and 343cubic units,are stacked vertically to form a tower in which the volumes of the cubes decrease from bottom to top.Ex-cept for the bottom cube,the bottom face of each cube lies com-pletely on top of the cube below it.What is the total surface area of the tower (including the bottom)in square units?(A)644(B)658(C)664(D)720(E)749译文七个立方体,体积分别是1,8,27,64,125,216,343个立方单位,依次按照体积大小由底到顶垂直地堆积成一座塔.除了最底部的立方体,每个立方体的底面都完全被下面的立方体的顶面覆盖.问这座塔的表面积(包括底面)是多少个平方单位?解这七个数都是立方数,则这七个立方体的棱长分别是1,2,3,4,5,6,7,从而塔的侧面积为4×(12+22+...+72)=560,而上、下底面积之和为2×72=98,共658,故(B)正确.8.What is the median of the following list of 4040numbers?1,2,3,...,2020,12,22,32,...,20202(A)1974.5(B)1975.5(C)1976.5(D)1977.5(E)1978.5译文下列4040个数:1,2,3,...,2020,12,22,32,...,20202的中位数是多少?解由于442=1936,452=2025,从而以上数列按递增排列的话,就成为:1,12,...,4,22,...,1936,442,...,1976,1977,...,2020,452,462,...,20202此时,1976成为第2020个数,所求中位数为1976+19772=1976.5,故(C)正确.9.How many solutions does the equation tan (2x )=cos (x 2)have on the interval [0,2π]?(A)1(B)2(C)3(D)4(E)5译文方程tan (2x )=cos (x 2)在区间[0,2π]上有多少个解?解y =tan 2x 是一个周期为π2、值域为R 的函数,在一个周期(−π4,π4)内严格单调递增,y =cos x2是一个周期为4π、值域为[−1,1]的函数,在区间[0,2π]上严格单调递减.如图示,在区间(π4,3π4),(3π4,5π4),(5π4,7π4)内,这是y =tan 2x 完整的周期,两条曲线均有一个交点;在区间[0,π4),(7π4,2π]上,这是y =tan 2x 的半周期,两条曲线刚好也有一个交点.故共有5个交点,(E)正确.10.There is a unique integer n such that log 2(log 16n )=log 4(log 4n ).What is the sum of the digits of n ?(A)4(B)7(C)8(D)11(E)13译文存在唯一的整数n 使得log 2(log 16n )=log 4(log 4n )成立,则n 的各个数位上的数字之和是多少?解log 4(log 4n )=log 2(log 16n )=log 22(log 16n )2,可得log 4n =(log 16n )2,使用换底公式有ln n ln 4=(ln n ln 16)2=ln 2n4ln 24,从而ln n 4ln 4=1⇒n =44=256.故(E)正确.11.A frog sitting at the point (1,2)begins a sequence of jumps,where each jump is parallel to one of the coordinate ax-es and has length 1,and the direction of each jump (up,down,left,right)is chosen independently at random.The sequence ends when the frog reaches a side of the square with vertices (0,0),(0,4),(4,0),and (4,4).What is the probability that the sequence ofjumps ends on a vertical side of the square?(A)12(B)58(C)23(D)34(E)78译文一只青蛙坐在点(1,2)上,开始一系列的跳跃,每次跳跃都平行于坐标轴且长度为1,方向(上、下、左、右)是随机的且独立,当青蛙到达由点(0,0),(0,4),(4,0),(4,4)构成的正方形的一条边的时候,跳跃终止.问跳跃终止于正方形竖直的两条边上的概率是多少?解如图示,青蛙在点F 1处,它可以向四个方向跳跃,概率均为14,向左跳跃,立刻达成目标;向上、向右、向下分别跳跃到点A 1,C,A 3处,再通过其它跳跃达成目标.根据对称性,青蛙由点A 1,A 2,A 3,A 4出发达成目标的概率是一样的,设为a ;青蛙由点B 1,B 2出发达成目标的概率是一样的,设为b ;青蛙由点F 1,F 2出发达成目标的概率是一样的,设为x ;青蛙由点C 出发达成目标的概率设为c .因此,P (青蛙由F 1出发达成目标)=P (青蛙向左)+P (青蛙向上)×P (青蛙由A 1出发达成目标)+P (青蛙向右)×P (青蛙由C 出发达成目标)+P (青蛙向下)×P (青蛙由A 3出发达成目标),即有x =14+a 2+c 4;同理,可得方程组x =14+a 2+c 4a =14+x 4+b 4b =a 2+c 4c =x 2+b 2成立,解得(x,a,b,c )=(58,12,38,12).故(B)正确.12.Line l in the coordinate plane has equation 3x −5y +40=0.This line is rotated 45◦counterclockwise about the point (20,20)to obtain line k .What is the x –coordinate of the x –intercept of line k ?(A)10(B)15(C)20(D)25(E)30译文坐标平面上的直线l 的方程为3x −5y +40=0,其绕点(20,20)作逆时针旋转45◦后得到直线k .则直线k 与x 轴交点的横坐标是多少?解如图示,直线k 与l 的斜率分别为tan ∠1和tan ∠2,依题意有∠1=∠2+45◦,tan ∠2=35,于是tan ∠1=tan (∠2+45◦)=tan ∠2+tan 45◦1−tan ∠2·tan 45◦=4,从而得到直线k 的方程为y −20=4(x −20),当y =0时,求得x =15.故(B)正确.13.There are integer a,b and c ,each greater than 1,such that a √N b √N c √N =36√N 25for all N >1.What is b ?(A)2(B)3(C)4(D)5(E)6译文设a,b,c 均是大于1的整数,且式子a √N b √N c √N =36√N 25对于N >1均成立.问b 是多少?解a √Nb √N c√N =N 1a N 1ab N 1abc =N bc +c +1abc 36√N 25=N 2536,依题意可得,bc +c +1abc =2536,解得a =2,b =3,c =6.故(B)正确.14.Regular octagon ABCDEF GH has area n .Let m bethe area of quadrilateral ACEG .What is mn?(A)√24(B)√22(C)34(D)3√25(E)2√23译文设正八边形ABCDEF GH 的面积为n ,四边形ACEG 的面积为m .则m n是多少?解如图,取正八边形的中心点O ,连OA ,OB .令OA =a ,则AC=√2a,∠AOB =45◦,于是m =S ACEG =(√2a )2=2a 2,n =S ABCDEF GH =8S ∆AOB =8×12a 2sin 45◦=2√2a 2,从而得m n =2a 22√2a 2=√22.故(B)正确.15.In the complex plane,let A be the set of solutions toz 3−8=0and let B be the set of solutions to z 3−8z 2−8z +64=0.What is the greatest distance between a point of A and a point of B ?(A)2√3(B)6(C)9(D)2√21(E)9+√3译文在复平面上,设A 是方程z 3−8=0的解集,B 是方程z 3−8z 2−8z +64=0的解集.问A 中一点到B 中一点的最远距离是多少?解解方程z 3−8=(z −2)(z 2+2z +4)=0,得A ={2,−1+√3i,−1−√3i };解方程z 3−8z 2−8z +64=(z −8)(z 2−8)=0,得B ={8,2√2,−2√2}.容易看出A 到B 的最远距离为d = (−1+√3i )−8 =√(−9)2+√32=2√21.故(D)正确.16.A point is chosen at random within the square in the co-ordinate plane whose vertices are (0,0),(2020,0),(2020,2020),and (0,2020).The probability that the point lies within d units ofa lattice point is 12.(A point (x,y )is a lattice point if x and y areboth integers.)What is d to the nearest tenth?(A)0.3(B)0.4(C)0.5(D)0.6(E)0.7译文坐标平面上有一个以(0,0),(2020,0),(2020,2020)和(0,2020)为顶点的正方形.在正方形内随机选择一个点,该点位于格点的d 个单位内的概率是12.(点(x,y )称为格点,若x 和y 均为整数.)则d 精确到十分位是多少?解如图示,以格点为圆心,d 为半径作一些圆,则正方形内的圆内部分就是符合条件的点集.因此,该点落在此区域的概率为P=该区域的面积正方形面积,即12=(20192+2019×2+1)πd220202=πd2,求得d=√12π≈0.4,故(B)正确.17.The vertices of a quadrilateral lie on the graph of y= ln x,and the x-coordinates of these vertices are consecutive pos-itive integers.The area of the quadrilateral is ln 9190.What is thex-coordinate of the leftmost vertex?(A)6(B)7(C)10(D)12(E)13译文一个四边形的顶点均在y=ln x的图像上,且它们的横坐标是连续正整数.该四边形的面积为ln 9190,则最左边顶点的横坐标是多少?解如图示,ABCD 是y=ln x上的四边形,过A作x轴的平行线,过C作x轴的垂线,交于点P,连结P B,P D,设点A坐标为(x,ln x),则有B(x+1,ln(x+1)),C(x+2,ln(x+2)),D(x+3,ln(x+3)),P(x+2,ln x),于是S ABCD=S∆P AB+S∆P BC+S∆P CD−S∆P AD=12×2×[ln(x+1)−ln x]+2×12×[ln(x+2)−ln x]×1−12×2×[ln(x+3)−ln x]=ln (x+1)(x+2)x(x+3)=ln9190.可得(x+1)(x+2)x(x+3)=9190,解得x=12或x=−15(舍去).故(D)正确.18.Quadrilateral ABCD satisfies∠ABC=∠ACD= 90◦,AC=20and CD=30.Diagonals AC and BD inter-sects at point E and AE=5.What is the area of Quadrilateral ABCD?(A)330(B)340(C)350(D)360(E)370译文四边形ABCD满足∠ABC=∠ACD=90◦, AC=20,CD=30.对角线AC和BD交于点E,且AE=5.求四边形ABCD 的面积是多少?解如图示,以AC为直径作一个圆,交BD与点F,依题意可得EC=15,ED=√EC2+CD2=15√5.设BE=x,依据相交弦定理AE·EC=BE·EF,则得EF=75x,DF=15√5−75x,DB=15√5+x;再由切割线定理DC2=DF·DB,得900=(15√5−75x)·(15√5+x),解得x=3√5或x=−5√5(舍去).而S∆ACD=12×20×30=300,S∆ABCS∆ACD=BEED=15,可得S∆ABC=60,故S ABCD=360,(D)正确.19.There exists a unique strictly increasing sequence of nonnegative integers a1<a2<···<a k such that2289+1217+1= 2a1+2a2+···+2a k.What is k?(A)117(B)136(C)137(D)273(E)306译文存在唯一严格递增的非负整数列a1<a2<···< a k使得2289+1217+1=2a1+2a2+···+2a k,则k是多少?解令217=x,则2289+1217+1=x17+1x+1=x16−x15+x14−x13+...+x2−x+1,而x16−x15=2272−2255=2271+2270+...+2255,同理x14−x13=2238−2221=2237+2236+ (2221)...,x2−x=234−217=233+232+ (217)从而2289+1217+1=20+(217+···+232+233)+···+(2255+···+2270+2271),共8×17+1=137项,故(C)正确.20.Let T be the triangle in the coordinate plane with vertices (0,0),(4,0),and(0,3).Consider the following five isometries (rigid transformations)of the plane:rotation of90◦,180◦,and 270◦counterclockwise around the origin,reflection across the x-axis,and reflection across the y-axis.How many of the125 sequences of three of these transformations(not necessarily dis-tinct)will return T to its original position?(For example,a180◦rotation,followed by a reflection across the x-axis,followed by a reflection across the y-axis will return T to its original position, but a90◦rotation,followed by a reflection across the x-axis,fol-lowed by another reflection across the x-axis will not return T to its original position.)(A)12(B)15(C)17(D)20(E)25译文设T是坐标平面上以(0,0),(4,0)和(0,3)为顶点的三角形.考虑以下五种平面上的等距变换(刚体变换):绕原点作90◦,180◦和270◦的逆时针旋转,关于x轴或y轴的反射.任选三种变换(不必不同)可以组成125种组合,有多少种组合将使得T 变回起始位置?(例如,一个关于y 轴的反射,接着一个关于x 轴的反射,再接着一个180◦的旋转,将会使得T 变回起始位置;但一个关于x 轴的反射,接着另一个关于x 轴的反射,再接着一个90◦的旋转,将不会使得T 变回起始位置.)解分两种情况:(1)全部由旋转组成:只要三次旋转的角度和为360◦或720◦即可满足要求,因此有90◦+90◦+180◦,90◦+180◦+90◦,180◦+90◦+90◦,270◦+270◦+180◦,270◦+180◦+270◦,180◦+270◦+270◦共6种组合;(2)由旋转和反射组合而成:有y 轴+x 轴+180◦,y 轴+180◦+x 轴,180◦+x 轴+y 轴,180◦+y 轴+x 轴,x 轴+180◦+y 轴,x 轴+y 轴+180◦,也是6种组合.故(A)正确.21.How many positive integers n are there such that n is a multiple of 5,and the least common multiple of 5!and n equals 5times the greatest common divisor of 10!And n ?(A)12(B)24(C)36(D)48(E)72译文有多少个正整数n ,使得n 是一个5的倍数,且n 与5!的最小公倍数是n 与10!的最大公因数的5倍?解由题意,[n,5!]=5×(n,10!),而5!=23×3×5,10!=28×34×52×7,可知n 不含除2,3,5,7以外的素因子,可设n =2a ×3b ×5c ×7d ,其中a,b,c,d ∈N ,且c 1.根据[2a ×3b ×5c ×7d ,23×3×5]=5×(2a ×3b ×5c×7d,28×34×52×7),以及最大公因数和最小公倍数的取法,可得3 a 8,1 b 4,c =3,0 d 1.故n 有6×4×1×2=48种取法,(D)正确.22.Let (a n )and (b n )be the sequence of real numbers suchthat (2+i )n =a n +b n i for all integers n 0,where i =√−1.What is ∞∑n =0a n b n7n?(A)38(B)716(C)12(D)916(E)47译文设(a n )和(b n )是使(2+i )n =a n +b n i 对所有的整数n 0均成立的实数列,其中i =√−1,则∞∑n =0a n b n7n是多少?解由(2+i )n =a n +b n i ,可得(2−i )n =a n −b n i,两式相加减得(2+i )n +(2−i )n =2a n ,(2+i )n −(2−i )n =2b n i从而a nb n =(2+i )n +(2−i )n 2·(2+i )n −(2−i )n2i =(3+4i )n −(3−4i )n4i 于是∞∑n =0a n b n 7n =∞∑n =017n ·(3+4i )n −(3−4i )n4i =14i ·∞∑n =0(3+4i )n −(3−4i )n 7n=14i·11−3+4i 7−11−3−4i 7=14i ·(74−4i −74+4i )=716故(B)正确.23.Jason rolls three fair standard six-sided dice.Then he looks at the rolls and chooses a subset of the dice (possibly emp-ty,possibly all three dice)to reroll.After rerolling,he wins if and only if the sum of the numbers faces up on the three dice is exactly 7.Jason always plays to optimize his chances of winning.What is the probability that he chooses to reroll exactly two of the dice?(A)736(B)524(C)29(D)1772(E)14译文詹森掷3颗标准、均匀的骰子,他看了结果之后会选择若干(可能是0,也可能是3)颗重掷.当3颗骰子正面朝上的数字和为7点的时候,他就赢了.詹森总是按照朝着他赢的最优策略去掷.问他刚好选择2颗骰子重掷的概率是多少?解掷1颗骰子得1,2,3,4,5,6点的概率均为16;掷2颗骰子得3点只有两种情况:12和21,概率为236,···;掷3颗骰子得7点有15种情况:115,151,511,124,142,214,241,412,421,133,313,331,223,232,322,概率为15216,···.经过计算,所有结果如下表所示:分类/概率/结果1234567掷1颗161616161616掷2颗136236336436536掷3颗1216321632161021615216因此,詹森要选择2颗骰子重掷,则上次掷的结果中,任意两颗骰子的数字和不能小于7点,否则他将选择重掷1颗骰子;且不能3颗骰子都是4点或者以上,要不然他将选择2019年全国高中数学联赛一试A 卷第10题的探究广东省佛山市乐从中学(528315)林国红一、题目呈现题目(2019年全国高中数学联赛一试(A 卷)第10题)在平面直角坐标系xoy 中,圆Ω与抛物线Γ:y 2=4x 恰有一个公共点,且圆Ω与x 轴相切于抛物线Γ的焦点F ,求圆Ω的半径.二、解法探究解法1(利用均值不等式)由题可知抛物线Γ的焦点为F (1,0),由对称性,不妨设圆Ω在x 轴上方与x 轴相切于F ,设圆Ω的半径为r .故圆Ω的方程为(x −1)2+(y −r )2=r 21⃝将x =14y 2代入1⃝并化简,得(y 24−1)2+y 2−2ry =0.显然y >0,故r =y 4+8y 2+1632y =(y 2+4)232y2⃝根据条件,2⃝恰有一个正数解y ,该y 值对应Ω与Γ的唯一公共点.考虑f (y )=(y 2+4)232y(y >0)的最小值.重掷3颗骰子.根据以上分析,满足条件的情况有:(1)掷出1点、6点、6点,3种情况;(2)掷出2点、5点、5点,3种情况;(3)掷出2点、5点、6点,6种情况;(4)掷出2点、6点、6点,3种情况;(5)掷出3点、4点、4点,3种情况;(6)掷出3点、4点、5点,6种情况;(7)掷出3点、4点、6点,6种情况;(8)掷出3点、5点、5点,3种情况;(9)掷出3点、5点、6点,6种情况;(10)掷出3点、6点、6点,3种情况.由加法原理,共42种情况,故所求概率为42216=736,(A)正确.24.Suppose that ∆ABC is an equilateral triangle of side length s ,with the property that there is a unique point P insidethe triangle such that AP =1,BP =√3,CP =2.What is s ?(A)1+√2(B)√7(C)83(D)√5+√5(E)2√2译文设∆ABC 是一个边长为s 的正三角形,内部有一点P ,使得AP =1,BP =√3,CP =2.问s 是多少?解如图,将∆AP C 绕点A 逆时针旋转60◦,得到∆ADB ,连结DP ,则AD =AP =1,DB =P C =2,∠DAP =60◦,因而∆ADP 是一个正三角形,可得DP =1,进而DP 2+BP 2=DB 2,所以∆DP B 是一个直角三角形,∠DP B =90◦,因此∠AP B =150◦.根据余弦定理,s 2=AB 2=AP 2+P B 2−2AP ·P B ·cos ∠AP B =1+3−2√3cos 150◦=7,即得s =√7.故(B)正确.25.The number a =pq,where p and q are relatively prime positive integers,has the property that the sum of all real num-bers x satisfying ⌊x ⌋·{x }=a ·x 2is 420,where ⌊x ⌋denotes the greatest integer less than or equal to x and {x }=x −⌊x ⌋denotes the dfractional part of x .What is p +q ?(A)245(B)593(C)929(D)1331(E)1332译文数a =pq满足性质:符合方程⌊x ⌋·{x }=a ·x 2的所有实数x 之和为420,其中p ,q 是互素的正整数,⌊x ⌋表示小于等于x 的最大整数,{x }=x −⌊x ⌋表示x 的小数部分.则p +q 是多少?解设⌊x ⌋=n ,{x }=r ,则x =n +r,0 r <1,代入方程⌊x ⌋·{x }=a ·x 2,整理得ar 2+(2a −1)nr +an 2=0,解得r =(1−2a )n ±√(1−4a )n 22a ,可知0<a 14.再由0 r =(1−2a )n −√(1−4a )n 22a<1,解得0 n <2a (1−2a )−√1−4ac .若c 是整数,则∑x =∑(n +r )=∑(n +nc)=c −1∑n =0(n +n c )=c 2−12=420,解得c =29,从而a =29900;若c 不是整数,则∑x =⌊c ⌋∑n =0(n +n c )=⌊c ⌋(⌊c ⌋+1)2·c +1c=420c 无解.故a =29900,p +q =929,(C)正确.。

赏析几道美国AMC12数学竞赛题

－ ABy0 A2 + B2
－
AC
时
f'（
x）
＞ 0．
f（ x） min
=
f（ B2 x0
－ ABy0 A2 + B2
－
AC ）
=
（
B2 x0
－ ABy0 － A2 + B2
AC
－
x0 ）
2
+（
A
B2 ·
x0
B
－ ABy0 A2 + B2
－
AC
+
C B
+ y0 ） 2
=
A2 （
Ax0
+
By0
+ C） 2 + B2 （ Ax0 （ A2 + B2 ） 2
can be written in the form of r1槡n1 + r2槡n2 + r3 ，where
r1 ，r2 ，and r3 are rationalnumbers and n1 and n2 are positive integers not divisible by the square of a prime． What is the greatest integer less than or equal to r1 + r2 + r3 + n1 + n2 ？
= 2·（ x － x0）
+
2·（
Ax + B
C
+
y0 ）
·A B
=
2·（
A2
+ B2
B2
）
·x
－ 2·（

AMT美国数学竞赛微积分真题

AMT美国数学竞赛微积分真题AMT美国数学竞赛微积分真题如下：
1.设f(x)为定义在区间[a,b]上的连续函数，证明：
若f(x)在区间[a,b]上单调递增，则∫f(x)dx在区间[a,b]上有f(b)-f(a)。

证明：
根据单调性，可知f(x)在[a,b]上是连续的，则可以用定积分定理证明：∫f(x)dx=F(b)-F(a)
其中F(x)是f(x)的原函数。

由于f(x)在[a,b]上单调递增，则F(x)也在[a,b]上单调递增，因此：
F(b)-F(a)=f(b)-f(a)
即证明了定理。

2.设f(x)为定义在区间[a,b]上的连续函数，证明：
若f(x)在区间[a,b]上单调递减，则∫f(x)dx在区间[a,b]上有f(a)-f(b)。

证明：
根据单调性，可知f(x)在[a,b]上是连续的，则可以用定积分定理证明：∫f(x)dx=F(b)-F(a)
其中F(x)是f(x)的原函数。

由于f(x)在[a,b]上单调递减，则F(x)也在[a,b]上单调递减，因此：
F(b)-F(a)=f(a)-f(b)
即证明了定理。

【最新】美国高中数学试题-word范文模板 (11页)

本文部分内容来自网络整理，本司不为其真实性负责，如有异议或侵权请及时联系，本司将立即删除！== 本文为word格式，下载后可方便编辑和修改！ ==美国高中数学试题篇一：高中一年级美国数学竞赛试题(简称AMC10)201X年B卷Problem 1Each third-grade classroom at Pearl Creek Elementary has 18 students and 2 pet rabbits. How many more students than rabbits are there in all 4 of the third-grade classrooms?SolutionProblem 2A circle of radius 5 is inscribed in a rectangle as shown. The ratio of the length of the rectangle to its width is 2:1. What is the area of the rectangle?SolutionProblem 3The point in the xy-plane with coordinates (1000, 201X) is reflected across the line y=201X. What are the coordinates of the reflected point?SolutionProblem 4When Ringo places his marbles into bags with 6 marbles per bag, he has 4 marbles left over. When Paul does the same with his marbles, he has 3 marbles left over. Ringo and Paul pool their marbles and place them into as many bags as possible, with 6 marbles per bag. How many marbles will be left over?SolutionProblem 5Anna enjoys dinner at a restaurant in Washington, D.C., where the sales tax on meals is 10%. She leaves a 15% tip on the price of her meal before the sales tax is added, and the tax is calculated on the pre-tip amount. She spends a total of 27.50 dollars for dinner. What is the cost of her dinner without tax or tip in dollars?SolutionProblem 6In order to estimate the value of x-y where x and y are real numbers with x > y > 0, Xiaoli rounded x up by a small amount, rounded y down by the same amount, and then subtracted her rounded values. Which of the following statements isnecessarily correct?A) Her estimate is larger than x-y B) Her estimate is smaller than x-y C) Her estimate equals x-y D) Her estimate equals y - x E) Her estimate is 0SolutionProblem 7For a science project, Sammy observed a chipmunk and a squirrel stashing acorns in holes. The chipmunk hid 3 acorns in each of the holes it dug. The squirrel hid 4 acorns in each of the holes it dug. They each hid the same number of acorns, although the squirrel needed 4 fewer holes. How many acorns did the chipmunk hide?SolutionProblem 8What is the sum of all integer solutions toSolution ?Problem 9Two integers have a sum of 26. When two more integers are added to the first two integers the sum is 41. Finally when two more integersare added to the sum of the previous four integers the sum is 57. What is the minimum number of even integers among the 6 integers?SolutionProblem 10How many ordered pairs of positive integers (M,N) satisfy the equationSolution=Problem 11A dessert chef prepares the dessert for every day of a week starting with Sunday. The dessert each day is either cake, pie, ice cream, or pudding. The same dessert may not be served two days in a row. There must be cake on Friday because of a birthday. How many different dessert menus for the week are possible?SolutionProblem 12Point B is due east of point A. Point C is due north of point B. The distance between points A and C is , and = 45 degrees. Point D is 20 meters due North of point C. The distance AD is between which two integers?SolutionProblem 13It takes Clea 60 seconds to walk down an escalator when it is not operating, and only 24 seconds to walk down the escalator when it is operating. How many seconds does it take Clea to ride down the operating escalator when she just stands on it?SolutionProblem 14Two equilateral triangles are contained in square whose side length isa rhombus. What is the area of the rhombus? . The bases of these triangles are the opposite side of the square, and their intersection isSolutionProblem 15In a round-robin tournament with 6 teams, each team plays one game against each other team, and each game results in one team winning and one team losing. At the end of the tournament, the teams are ranked by the number of games won. What is the maximum number of teams that could be tied for the most wins at the end on the tournament?SolutionProblem 16Three circles with radius 2 are mutually tangent. What is the total area of the circles and the region bounded by them, as shown in the figure?SolutionProblem 17Jesse cuts a circular paper disk of radius 12 along two radii to form two sectors, the smaller having a central angle of 120 degrees. He makes two circular cones, using each sector to form the lateral surface of a cone. What is the ratio of the volume of the smaller cone to that of the larger?SolutionProblem 18Suppose that one of every 500 people in a certain population has a particulardisease, which displays no symptoms. A blood test is available for screening for this disease. For a person who has this disease, the test always turns out positive. For a person who does not have the disease, however, there is a。

2021年美国数学竞赛(AMC10A)的试题与解答

2021年美国数学竞赛(AMC10A)的试题与解答华南师范大学数学科学学院(510631)李湖南1.算式(22−2)−(32−3)+(42−4)的值是多少?(A)1(B)2(C)5(D)8(E)12解直接计算,原式=2−6+12=8,故(D)正确.2.Portia高中的学生人数是Lara高中的3倍,这两所高中总共有2600名学生.问Portia高中有多少名学生?(A)600(B)650(C)1950(D)2000(E)2050解依题意可知,Portia高中占总人数的34,即有2600×34=1950名学生,故(C)正确.3.两个自然数之和是17402.这两个数中的一个可以被10整除,如果去掉该数的个位数字则得到另外一个数.问这两个数的差是多少?(A)10272(B)11700(C)13362(D)14238(E)15426解设第一个数为x,则第二个数为x10,已知x+x10=17402,解得x=15820,于是两数之差为x−x10=910x=14238,故(D)正确.4.一辆小车冲下山坡,它第一秒移动了5英寸,并且速度不断加快.在每个连续的1秒时间间隔内,它都比前1秒多移动7英寸.小车用了30秒到达山脚.问它一共行进了多少英寸?(A)215(B)360(C)2992(D)3195(E)3242解小车每秒移动的距离是个等差数列,首项为5,公差为7,第30秒移动了208英寸,从而距离和为(5+208)×30÷2=3195英寸,故(D)正确.5.一个共有k>12名学生的班级进行测验的平均分为8分,其中12名学生的测验平均分是14分.问其余学生的测验平均分如何用k来表示?(A)14−8k−12(B)8k−168k−12(C)1412−8k(D)14(k−12)k2(E)14(k−12)8k解所有学生的分数总和为8k,其中12名学生的分数和为168,剩下k−12名学生的分数和为8k−168,故平均分为8k−168k−12,(B)正确.6.Chantal和Jean从山路的起点开始向消防塔徒步旅行.Jean背着一个沉重的背包,走得较慢.Chantal开始以每小时4英里的速度行走,走到路程一半,山路变得非常陡峭,Chantal的速度减慢到每小时2英里.到达塔后,她立即掉头,以每小时3英里的速度沿着陡峭的山路向下走,她在路程的一半处遇见了Jean.从出发到他们相遇,Jean的平均速度是每小时多少英里?(A)1213(B)1(C)1312(D)2413(E)2解设半程为x英里,Jean所用的时间与Chantal一样,均为x4+x2+x3=13x12小时,于是Jean的平均速度为x(13x)/12=1213英里/小时,故(A)正确.7.汤姆有13条蛇,其中4条是紫色的,5条是快乐的.他观察发现:他的所有快乐的蛇都能做加法,他的紫色的蛇不会做减法,而且他所有不会做减法的蛇也不会做加法.关于汤姆的蛇,可以得出以下哪个结论?(A)紫色的蛇可以做加法(B)紫色的蛇是快乐的(C)能做加法的蛇是紫色的(D)快乐的蛇不是紫色的(E)快乐的蛇不能做减法解紫色的蛇不会做减法,从而也不会做加法,因此也不是快乐的.故快乐的蛇都不是紫色的,(D)正确.8.一名学生在用66乘以如下的循环小数时, 1.abab···=1.˙a˙b,其中a和b是数字.他没有注意到循环小数的标识,而只是做了66乘以1.ab.后来他发现他的答案比正确答案小0.5.问两位整数ab是多少?(A)15(B)30(C)45(D)60(E)75解由题意可得,0.5=66×(1.˙a˙b−1.ab)=66×0.00˙a˙b= 0.66×ab99,解得ab=75,故(E)正确.9.对于实数x和y,(xy−1)2+(x+y)2的最小可能值是多少?(A)0(B)14(C)12(D)1(E)2解(xy−1)2+(x+y)2=x2y2+x2+y2+1 1,此时x=y=0,故(D)正确.10.算式(2+3)(22+32)(24+34)(28+38)(216+ 316)(232+332)(264+364)与下面哪个表达式相等?(A)3127+2127(B)3127+2127+2×363+3×263(C)3128−2128(D)3128+2128(E)5127解连续使用平方差公式,可得原式=(3−2)(3+2)(32+22)(34+24)(38+28)(316+216)(332+232)(364+264)=3128−2128,故(C)正确.11.选择下面哪个整数b 为基数,可以使得b 进制数2021b −221b 不能被3整除?(A)3(B)4(C)6(D)7(E)8解由于2021b −221b =2×b 3−2×b 2=2b 2(b −1),当b =3,4,6,7时,该数均能被3整除,故(E)正确.12.如图所示,两个顶点朝下的正圆锥包含相同量的液体.液体顶部表面的半径分别为3厘米和6厘米.在每个圆锥体中放入一个半径为1厘米的球形弹子,它沉入底部,完全浸没,没有任何液体溢出.问窄圆锥内液面上升的高度与宽圆锥内液面上升的高度之比是多少?(A)1:1(B)47:43(C)2:1(D)40:13(E)4:1解设液体的体积为V ,左右两边液面高度分别为h 1,h 2,则V =13π32·h 1=13π62·h 2,因而h 1=4h 2.设放入弹子的体积为V 0=43π,左右两边液面上升高度分别为∆h 1,∆h 2,顶部液面的半径分别为r 1,r 2,则有V +V 0=13πr 21·(h 1+∆h 1)=13πr 22·(h 2+∆h 2),3r 1=h 1h 1+∆h 1,6r 2=h 2h 2+∆h 2,解得r 1r 2=12,∆h 1∆h 2=41,故(E)正确.13.四面体ABCD 中,各边长为AB =2,AC =3,AD =4,BC =√13,BD =2√5和CD =5,问它的体积是多少?(A)3(B)2√3(C)4(D)3√3(E)6解如图所示,由于AB 2+AC 2=BC 2,AB 2+AD 2=BD 2,AC 2+AD 2=CD 2,可得AB,AC,AD 互相垂直,即ABCD 是个直四面体.故V ABCD =13S ∆ABD ·AC =13·12×2×4·3=4,(C)正确.14.多项式z 6−10z 5+Az 4+Bz 3+Cz 2+Dz +16的根都是正整数,有可能重复.问B 的取值是多少?(A)−88(B)−80(C)−64(D)−41(E)−40解设多项式的根为x 1,x 2,x 3,x 4,x 5,x 6,其中均为正整数,则z 6−10z 5+Az 4+Bz 3+Cz 2+Dz +16=(z −x 1)(z −x 2)···(z −x 6),可得 x 1+x 2+x 3+x 4+x 5+x 6=10,x 1x 2x 3x 4x 5x 6=16,−∑i<j<kx i x j x k =B,解得x 1=x 2=1,x 3=x 4=x 5=x 6=2,于是B =−(C 14·12·2+C 12·C 24·1·22+C 34·23)=−88,故(A)正确.15.A,B,C 和D 的值从{1,2,3,4,5,6}中不重复地选取(即没有两个字母的取值相同),使得两条曲线y =Ax 2+B 和y =Cx 2+D 相交的不同取值方式有多少种?(不考虑曲线列出的顺序,例如,A =3,B =2,C =4,D =1与A =4,B =1,C =3,D =2被认为是相同的)(A)30(B)60(C)90(D)180(E)360解要使得y =Ax 2+B 和y =Cx 2+D 相交,则方程Ax 2+B =Cx 2+D 有解,即有(A −C )x 2=D −B ,此时A −C,D −B 的符号一样.任取A,C ∈{1,2,3,4,5,6},则D,B ∈{1,2,3,4,5,6}−{A,C },大小关系须一致,则有2C 26C 24=180种选择;另外不考虑曲线的顺序,故不同的取值方式共有180÷2=90种,(C)正确.16.在下面的数据列表中,对于1 n 200,整数n 出现了n 次.1,2,2,3,3,3,4,4,4,4,···,200,200,···,200.问这组数据列表中的中位数是多少?(A)100.5(B)134(C)142(D)150.5(E)167解这列数共有1+2+3+ (200)12×200×201=20100个,中位数是第10050与第10051个数的平均值.先求得12n (n +1) 10050的最大值为n max =141,且12n max (n max +1)=10011.这说明第10050和第10051个数均为142,故中位数为142,(C)正确.17.在梯形ABCD 中,AB //CD ,BC =CD =43,并且AD ⊥BD .设O 是对角线AC 和BD 的交点,P 是BD的中点.已知OP =11,AD 的长度可以表示成m √n ,其中m 和n 是正整数,并且n 不能被任何质数的平方所整除.问m +n 的值是多少?(A)65(B)132(C)157(D)194(E)215解如图所示,延长CP 交AB 于点E ,由于∆CBD 是等腰三角形,P 是BD 中点,从而CP⊥BD ,进而CE //DA ,于是AECD 是个平行四边形,得EA =CD =43.又∠CBD =∠CDB =∠ABD ,可得∆CBP =∆EBP ,即得BE =BC =43,于是AB =86.再根据∆CDO ∆ABO ,有OD OB =CD AB =12,即OD OB =BP −11BP +11=12,解得BP =33,从而AD =CE =2CP =2√BC 2−BP 2=2√432−332=4√190,故m +n =194,(D)正确.18.令f 是一个定义在正有理数集合上的函数,它具有性质:对于所有的正有理数a 和b ,f (ab )=f (a )+f (b ).假设f 还具有性质:对于每一个质数p ,f (p )=p .问以下哪个数x 满足f (x )<0?(A)1732(B)1116(C)79(D)76(E)2511解f (1)=f (1·1)=2f (1)⇒f (1)=0,f (1)=f (b ·1b )=f (b )+f (1b )=0⇒f (1b)=−f (b ),对于任意正有理数b ;f (a b )=f (a ·1b )=f (a )+f (1b)=f (a )−f (b ),对于任意正有理数a 和b ;f (p n )=f (p ·p ·····p n 个)=nf (p ),对于任意质数p ,n ∈N .因此,f (2511)=f (25)−f (11)=2f (5)−f (11)=2·5−11=−1<0,故(E)正确.19.由x 2+y 2=3|x −y |+3|x +y |的图像所界定的图形的面积是m +nπ,其中m 和n 是整数.问m +n 是多少?(A)18(B)27(C)39(D)45(E)54解如图所示,对点(x,y )的位置进行讨论:(1)在第一象限:当y x 时,方程为x 2+y 2=3(x −y )+3(x +y ),化简可得(x −3)2+y 2=9,它是一个八分之一圆;当y >x 时,方程可化为x 2+(y −3)2=9,也是一个八分之一圆;(2)在第二象限:当y −x 时,方程可化为x 2+(y −3)2=9;当y <−x 时,方程可化为(x +3)2+y 2=9;(3)在第三象限:当y x 时,方程可化为(x +3)2+y 2=9;当y <x 时,方程可化为x 2+(y +3)2=9;(4)在第四象限:当y −x 时,方程可化为(x −3)2+y 2=9;当y <−x 时,方程可化为x 2+(y +3)2=9.综上可得,该图像由四个半径为3的半圆封闭而成,面积为62+2·π·32=36+18π,故m +n =36+18=54,(E)正确.20.数列1,2,3,4,5有多少种重新排列的方式,使得没有连续三项是递增的,也没有连续三项是递减的?(A)10(B)18(C)24(D)32(E)44解由题意可知,符合条件的数列中,连续两项的单调性只能是:增减增减,或减增减增.用排列表示即有:13254,14253,14352,15243,15342;21435,21534,23154,24153,24351,25143,25341;31425,31524,32415,32514,34152,34251,35142,35241.如果对集合{1,2,3,4,5}做一个置换1234554321 ,所得排列仍然符合条件,即以5,4开头的排列和以1,2开头的排列一样多.故所有符合条件的数列有(5+7)×2+8=32个,(D)正确.21.设ABCDEF 是等角六边形,由直线AB ,CD 和EF 所组成的三角形面积为192√3,由直线BC ,DE 和F A 所组成的三角形的面积是324√3.六边形ABCDEF 的周长可用m +n √p 表达,其中m ,n 和p 是正整数,并且p不能被任何质数的平方整除.问m +n +p 的值是多少?(A)47(B)52(C)55(D)58(E)63解如图所示,分别向两边延长各边,交于点G ,H ,I ,J ,K ,L ,由题意可得,S ∆GHI =192√3,S ∆JKL =324√3.由于六边形的每个内角都是120◦,因此图中所有三角形的内角都是60◦,即所有三角形都是等边三角形.于是,S ∆GHI =√34IG 2=√34(a +b +f )2=192√3,解得a +b +f =16√3;同理S ∆JKL =√34(c +d +e )2=324√3,得c +d +e =36.故六边形的周长为a +b +c +d +e +f =36+16√3,即m +n +p =36+16+3=55,(C)正确.22.Hiram 的代数笔记有50页,打印在25张纸上;第一张纸包括第1和第2页,第二张纸包括第3和第4页,以此类推.有一天,他去午餐前把笔记本放在桌子上,室友决定从笔记中间借几页.当Hiram回来时,他发现他的室友从笔记中拿走了连续的若干张纸,并且所有剩余纸张上页码的平均值正好是19.问有多少张纸被借走了?(A)10(B)13(C)15(D)17(E)20解设笔记被借走了x张纸,分别是从第a张纸到第a+x−1张纸,其中a+x−1 25,页码正好是从2a−1到2(a+x−1),页码和为(2a−1)+2a+···+2(a+x−1)=(2a−1)+2(a+x−1)2·2x=(4a+2x−3)x,而所有页码和为1+2+···+50=1275,从而剩下的页码和为19(50−2x)=1275−(4a+2x−3)x,整理得(4a+2x−41)x=325,解得x=13,a=10,故(B)正确.23.青蛙Frieda在一个3×3的方格表上开始一系列跳跃,每次跳跃都随机选择一个方向—–向上、向下、向左或向右,从一个方格移动到旁边的方格.她不能斜着跳,当跳跃的方向会使得Frieda离开方格表时,她会“绕个圈”,跳到相对的另一边.例如,如果Frieda从中心方格开始,向上跳跃两次,第一次跳跃后她将位于最上面一行的中间方格,第二次跳跃将使得Frieda跳到相对的边,落在最下面一行的中间方格.假设Frieda从中心方格出发,最多随机跳跃四次,并且当到达角落方格时就停止跳跃.问她在四次跳跃中到达角落方格的概率是多少?(A)916(B)58(C)37(D)2532(E)1316解记P(n)为第n次到达角落方格的概率,显然P(1)=0;第一次跳跃可朝四个方向,不妨设第一次跳跃向右,则当第二次跳跃向上或向下时,可到达角落方格,概率为24,即P(2)=12;若第二次跳跃向左,概率为14,即回到出发点S,此时第四次跳跃到达角落方格的概率为1 4×P(2)=18;若第二次跳跃向右,概率为14,此时到了S左边的方格,则第三次跳跃向上或向下可到达角落方格,即P(3)=14×12=18;若第三次跳跃向左,概率为14,则回到S右边的方格,第四次跳跃向上或向下可到达角落方格,概率为14×14×12=132,从而P(4)=18+132=532.而其它情况均四次到达不了角落方格.故所求概率为4∑i=1P(i)=0+12+18+532=2532,(D)正确.24.设a是正实数,考虑由(x+ay)2=4a2和(ax−y)2=a2组成的四边形的内部.对所有的a>0而言,这个区域的面积怎样用a来表示?(A)8a2(a+1)2(B)4aa+1(C)8aa+1(D)8a2a2+1(E)8aa2+1解如图所示,四边形由四条直线y=−1ax±2,y=ax±a围成,由于斜率之积为−1,从而四边形是个矩形.设直线y=−1ax+2与x轴负方向的夹角为θ,则tanθ=1a,矩形的长为4cosθ,宽为2cosθ,即所求面积为S=8cos2θ.于是S=4·2cos2θ=4(1+cos2θ)=4(1+1−tan2θ1+tan2θ)=4·21+tan2θ=8·11+1a2=8a21+a2.故(D)正确.25.将3枚不可区分的红色筹码,3枚不可区分的蓝色筹码和3枚不可区分的绿色筹码分别放入3×3方格表的各个小方格中,使得无论是垂直方向还是水平方向,都没有两个相同颜色的筹码相邻,问共有多少种放法?(A)12(B)18(C)24(D)30(E)36解首先考虑中心方格,不妨设放入红色筹码,分两种情况:(1)其余两个红色筹码在一边,四个方向均可:那剩下6个方格只能按以下方式放入,∆放一种颜色,空格放另一种颜色.此时有4×2=8种放法;(2)其余两个红色筹码各在一边,即在对角线上,两个方向均可:那剩下6个方格也只能按以下方式放入,∆放一种颜色,空格放另一种颜色.此时有2×2=4种放法.综上,中心方格放入红色筹码,共有12种放法.如果放入蓝色筹码或绿色筹码,也是一样,故所有的放法有3×12=36种,(E)正确.。

1、下载文档前请自行甄别文档内容的完整性，平台不提供额外的编辑、内容补充、找答案等附加服务。
2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
3、如文档侵犯您的权益，请联系客服反馈,我们会尽快为您处理(人工客服工作时间：9:00-18:30)。

美国高中学生数学竞赛题
1.(1995年文理)设(3x-1)6=a6x6+a5x5+a4x4+a3x3+a2x2+a1x+a0，求a6+a5+a4+a3+a2+a1+a0的值。

答案：64。

2.(1989年文)如果(1-2x)7=a0+a1x+a2x2+…+a7x7，那么a1+a2+…+a7的值等于()
A.-2
B.-1
C.0
D.2
答案：(A)
3.(1989年理)已知(1-2x)7=a0+a1x+a2x2+…+a7x7，那么a1+a2+…+a7=____。

答案：-2。

题源：(美28届10题)若(3x-1)7=a7x7+a6x6+…+a0，那么a7+a6+…+a0等于()
A.0
B.1
C.64
D.-64
E.128
答案：(E)
改编点评：1题将指数7改为6，改为简答题；2题将底数(3x-1)改为(1-2x)，展开式改为x的升幂排列，所求结论中去掉了常数项a0，3题改编方法同2题，改为填空题。

4.(1990年文)已知f(x)=x5+ax3+bx-8，且f(-2)=10，那么f(2)等于()
A.-26
B.-18
C.-10
D.10
答案：(A)
题源：(美33届12题)设f(x)=ax7+bx3+cx-5，其中a.b和c是常数，如图f(-7)=7，那么f(7)等于()
A.-17
B.-7
C.14
D.21
E.不能唯一确定
答案：(A)
改编点评：降低了次数，减少了一个字母系数，降低了难度。

5.(1990年文理)如果实数x、y满足等式(x-2)2+y2=3，那么的最大值是()
A. B. C. D.
答案：(D)
题源：(美35届29题)在满足方程(x-3)2+(y-3)2=6的实数对(x，y)中，的最大值是()
A.3+2
B.2+
C.3
D.6
E.6+2
答案：(A)
改编点评：圆方程中的圆心坐标、半径作了改变，题设的叙述方式也作了变化。

6.(1990年文理)函数y=+++的值域是()
A.{-2，4}
B.{-2，0，4}
C.{-2，0，2，4}
D.{-4，-2，0，4}
答案：(B)
题源：(美28届8题)非零实数的每一个三重组(a，b，c)构成一个数。

如此构成的所有数的集是()
A.{0}
B.{-4，0，4}
C.{-4，-2，0，2，4}
D.{-4，-2，2，4}
E.这些都不对
答案：(B)
改编点评：将a、b、c改为三角函数，考查的知识面更广。

7.(1992年文理)长方体的全面积为11，十二条棱长度之和为24，则这个长方体的一条对角线长为()
A.2
B.
C.5
D.6
答案：(C)
题源：(美35届25题)一个长方体的表面积为22cm2，并且它的所有棱的总长度为24cm，那么它的对角线的长度(按cm计)是()
A. B. C. D. E.不能被唯一确定
答案：(D)
改编点评：作了两个方面的变化：将全面积22cm2改为11，去掉单位。

8.(1992年文理)如果函数f(x)=x2+bx+c对任意实数t都有f(2+t)=f(2-t)，那么()
A.f(2)＜f(1)＜f(4)
B.f(1)＜f(2)＜f(4)
C.f(2)＜f(4)＜f(1)
D.f(4)＜f(2)＜f(1)
题源：(美35届16题)函数f(x)对于一切实数x都满足f(2+x)=f(2-x)，如果方程f(x)=0恰好有四个不同的实根，那么这些根的和是()
A.0
B.2
C.4
D.6
E.8
答案：(E)
改编点评：题源是一个抽象函数问题，有一些难度，作为高考题是不合适的，8题主要采用题源中对称轴的表达方式，通过设计一个二次函数，结合对称性考查函数的单调性。

9.(1994年文理)已知sinθ+cosθ=，θ∈(0，π)，则ctgθ的值是____。

答案：-。

题源：(美29届15题)若sinx+cosx=，且0≤x≤π，那么tgx是()
A.-
B.-
C.
D.
答案：(A)
改编点评：x改为θ，将求正切值改为求余切值，改成填空题。

10.(1995年文理)等差数列{an}，{bn}的前n项和分别为Sn和Tn，若=，则等于()
A.1
B.
C.
D.
答案：(C)
题源：(美20届32题)设Sn和Tn分别为两个等差数列的前n项和，如果对所有的n，有=，则第一个数列与第二个数列的第十一项的比是()
A. B. C. D. E.不能确定
答案：(A)
改编点评：改变的比值，设问方式作了较大变化，将求某一项的比值改为求比值的极限。

11.(2002年文理)不等式(1+x)(1-|x|)＞0的解集是()
A.{x|0≤x＜1}
B.{x|x＜0且x≠-1}
C.{x|-1＜n＜1}
D.{x|x＜1且x≠-1}
答案：(D)
题源：(美27届7题)若x是实数，那么(1-|x|)(1+x)是正数的充分必要条件是() A.|x|＜1 B.x＜1 C.|x|＞1 D.x＜-1 E.x＜-1或-1＜x＜1
答案：(E)
改编点评：改变了设问方式，将求充要条件改为求不等式解集。