AMC 美国数学竞赛 2001 AMC 10 试题及答案解析
美国数学竞赛AMC题目及答案
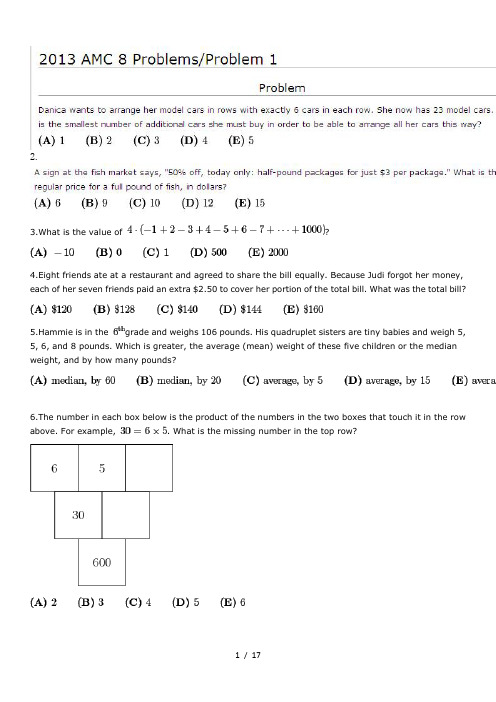
2.3.What is the value of ?4.Eight friends ate at a restaurant and agreed to share the bill equally. Because Judi forgot her money, each of her seven friends paid an extra $2.50 to cover her portion of the total bill. What was the total bill?5.Hammie is in the grade and weighs 106 pounds. His quadruplet sisters are tiny babies and weigh 5, 5, 6, and 8 pounds. Which is greater, the average (mean) weight of these five children or the median weight, and by how many pounds?6.The number in each box below is the product of the numbers in the two boxes that touch it in the row above. For example, . What is the missing number in the top row?7.Trey and his mom stopped at a railroad crossing to let a train pass. As the train began to pass, Trey counted 6 cars in the first 10 seconds. It took the train 2 minutes and 45 seconds to clear the crossing at a constant speed. Which of the following was the most likely number of cars in the train?8.A fair coin is tossed 3 times. What is the probability of at least two consecutive heads?9.The Incredible Hulk can double the distance he jumps with each succeeding jump. If his first jump is 1 meter, the second jump is 2 meters, the third jump is 4 meters, and so on, then on which jump will he first be able to jump more than 1 kilometer?10.What is the ratio of the least common multiple of 180 and 594 to the greatest common factor of 180 and 594?11. Ted's grandfather used his treadmill on 3 days this week. He went 2 miles each day. On Monday he jogged at a speed of 5 miles per hour. He walked at the rate of 3 miles per hour on Wednesday and at 4 miles per hour on Friday. If Grandfather had always walked at 4 miles per hour, he would have spent less time on the treadmill. How many minutes less?12. At the 2013 Winnebago County Fair a vendor is offering a "fair special" on sandals. If you buy one pair of sandals at the regular price of $50, you get a second pair at a 40% discount, and a third pair at half the regular price. Javier took advantage of the "fair special" to buy three pairs of sandals. What percentage of the $150 regular price did he save?13. When Clara totaled her scores, she inadvertently reversed the units digit and the tens digit of one score. By which of the following might her incorrect sum have differed from the correct one?14. Let the two digits be and .The correct score was . Clara misinterpreted it as . The difference between the two is which factors into . Therefore, since the difference is a multiple of 9, the only answerchoice that is a multiple of 9 is .15. If , , and , what is the product of , , and ?16. A number of students from Fibonacci Middle School are taking part in a community service project. The ratio of -graders to -graders is , and the the ratio of -graders to -graders is . What is the smallest number of students that could be participating in the project?17. The sum of six consecutive positive integers is 2013. What is the largest of these six integers?18. Isabella uses one-foot cubical blocks to build a rectangular fort that is 12 feet long, 10 feet wide, and 5 feet high. The floor and the four walls are all one foot thick. How many blocks does the fort contain?--Arpanliku 16:22, 27 November 2013 (EST) Courtesy of Lord.of.AMC19. Bridget, Cassie, and Hannah are discussing the results of their last math test. Hannah shows Bridget and Cassie her test, but Bridget and Cassie don't show theirs to anyone. Cassie says, 'I didn't get the lowest score in our class,' and Bridget adds, 'I didn't get the highest score.' What is the ranking of the three girls from highest to lowest?20. A rectangle is inscribed in a semicircle with longer side on the diameter. What is the area of the semicircle?21. Samantha lives 2 blocks west and 1 block south of the southwest corner of City Park. Her school is 2 blocks east and 2 blocks north of the northeast corner of City Park. On school days she bikes on streets to the southwest corner of City Park, then takes a diagonal path through the park to the northeast corner, and then bikes on streets to school. If her route is as short as possible, how many different routes can she take?22. Toothpicks are used to make a grid that is 60 toothpicks long and 32 toothpicks wide. How many toothpicks are used altogether?23. Angle of is a right angle. The sides of are the diameters of semicircles as shown. The area of the semicircle on equals , and the arc of the semicircle on has length .What is the radius of the semicircle on ?24. Squares , , and are equal in area. Points and are the midpoints of sidesand , respectively. What is the ratio of the area of the shaded pentagon to the sum of the areas of the three squares?25. A ball with diameter 4 inches starts at point A to roll along the track shown. The track is comprised of 3 semicircular arcs whose radii are inches, inches, and inches, respectively. The ball always remains in contact with the track and does not slip. What is the distance the center of theball travels over the course from A to B?1.2.The 50% off price of half a pound of fish is $3, so the 100%, or the regular price, of a half pound of fish is $6. Consequently, if half a pound of fish costs $6, then a whole pound of fish is dollars.3.Notice that we can pair up every two numbers to make a sum of 1:Therefore, the answer is .4.Each of her seven friends paid to cover Judi's portion. Therefore, Judi's portion must be . Since Judi was supposed to pay of the total bill, the total bill must be .5.The median here is obviously less than the mean, so option (A) and (B) are out.Lining up the numbers (5, 5, 6, 8, 106), we see that the median weight is 6 pounds.The average weight of the five kids is .Therefore, the average weight is bigger, by pounds, making the answer.6.Solution 1: Working BackwardsLet the value in the empty box in the middle row be , and the value in the empty box in the top row be . is the answer we're looking for.We see that , making .It follows that , so .Solution 2: Jumping Back to the StartAnother way to do this problem is to realize what makes up the bottommost number. This method doesn't work quite as well for this problem, but in a larger tree, it might be faster. (In this case, Solution 1 would be faster since there's only two missing numbers.)Again, let the value in the empty box in the middle row be , and the value in the empty box in the top row be . is the answer we're looking for.We can write some equations:Now we can substitute into the first equation using the two others:7.If Trey saw , then he saw .2 minutes and 45 seconds can also be expressed as seconds.Trey's rate of seeing cars, , can be multiplied by on the top and bottom (and preserve the same rate):. It follows that the most likely number of cars is . Solution 2minutes and seconds is equal to .Since Trey probably counts around cars every seconds, there are groups of cars that Trey most likely counts. Since , the closest answer choice is .8.First, there are ways to flip the coins, in order.The ways to get two consecutive heads are HHT and THH.The way to get three consecutive heads is HHH.Therefore, the probability of flipping at least two consecutive heads is .9.This is a geometric sequence in which the common ratio is 2. To find the jump that would be over a 1000 meters, we note that .However, because the first term is and not , the solution to the problem is10. To find either the LCM or the GCF of two numbers, always prime factorize first.The prime factorization of .The prime factorization of .Then, find the greatest power of all the numbers there are; if one number is one but not the other, use it (this is ). Multiply all of these to get 5940.For the GCF of 180 and 594, use the least power of all of the numbers that are in both factorizations and multiply. = 18.Thus the answer = = .We start off with a similar approach as the original solution. From the prime factorizations, the GCF is .It is a well known fact that . So we have,.Dividing by yields .Therefore, .11. We use that fact that . Let d= distance, r= rate or speed, and t=time. In this case, let represent the time.On Monday, he was at a rate of . So, .For Wednesday, he walked at a rate of . Therefore, .On Friday, he walked at a rate of . So, .Adding up the hours yields + + = .We now find the amount of time Grandfather would have taken if he walked at per day. Set up the equation, .To find the amount of time saved, subtract the two amounts: - = . To convert this to minutes, we multiply by .Thus, the solution to this problem is12. First, find the amount of money one will pay for three sandals without the discount. We have.Then, find the amount of money using the discount: .Finding the percentage yields .To find the percent saved, we have13. Let the two digits be and .The correct score was . Clara misinterpreted it as . The difference between the two is which factors into . Therefore, since the difference is a multiple of 9, the only answerchoice that is a multiple of 9 is .14. The probability that both show a green bean is . The probability that both show a red bean is . Therefore the probability is15.Therefore, .Therefore, .To most people, it would not be immediately evident that , so we can multiply 6's until we get the desired number:, so .Therefore the answer is .16. Solution 1: AlgebraWe multiply the first ratio by 8 on both sides, and the second ratio by 5 to get the same number for 8th graders, in order that we can put the two ratios together:Therefore, the ratio of 8th graders to 7th graders to 6th graders is . Since the ratio is in lowest terms, the smallest number of students participating in the project is .Solution 2: FakesolvingThe number of 8th graders has to be a multiple of 8 and 5, so assume it is 40 (the smallest possibility). Then there are 6th graders and 7th graders. The numbers of students is17. Solution 1The mean of these numbers is . Therefore the numbers are, so the answer isSolution 2Let the number be . Then our desired number is .Our integers are , so we have that.Solution 3Let the first term be . Our integers are . We have,18. Solution 1There are cubes on the base of the box. Then, for each of the 4 layers above the bottom (as since each cube is 1 foot by 1 foot by 1 foot and the box is 5 feet tall, there are 4 feet left), there arecubes. Hence, the answer is .Solution 2We can just calculate the volume of the prism that was cut out of the original box. Each interior side of the fort will be feet shorter than each side of the outside. Since the floor is foot, the height will be feet. So the volume of the interior box is .The volume of the original box is . Therefore, the number of blocks contained inthe fort is .19. If Hannah did better than Cassie, there would be no way she could know for sure that she didn't get the lowest score in the class. Therefore, Hannah did worse than Cassie. Similarly, if Hannah did worse than Bridget, there is no way Bridget could have known that she didn't get the highest in the class.Therefore, Hannah did better than Bridget, so our order is .20.A semicircle has symmetry, so the center is exactly at the midpoint of the 2 side on the rectangle, making the radius, by the Pythagorean Theorem, . The area is .21.The number of ways to get from Samantha's house to City Park is , and the number of ways toget from City Park to school is . Since there's one way to go through City Park (just walking straight through), the number of different ways to go from Samantha's house to City Park to school .22. There are vertical columns with a length of toothpicks, and there are horizontal rows with a length of toothpicks. An effective way to verify this is to try a small case, i.e. a grid of toothpicks.Thus, our answer is .23. Solution 1If the semicircle on AB were a full circle, the area would be 16pi. Therefore the diameter of the first circle is 8. The arc of the largest semicircle would normally have a complete diameter of 17. The Pythagoreantheorem says that the other side has length 15, so the radius is .Solution 2We go as in Solution 1, finding the diameter of the circle on AC and AB. Then, an extended version of the theorem says that the sum of the semicircles on the left is equal to the biggest one, so the area of thelargest is , and the middle one is , so the radius is .24.First let (where is the side length of the squares) for simplicity. We can extend until it hits theextension of . Call this point . The area of triangle then is The area of rectangle is . Thus, our desired area is . Now, the ratio of the shaded area to thecombined area of the three squares is .Let the side length of each square be .Let the intersection of and be .Since , . Since and are vertical angles, they are congruent. We also have by definition.So we have by congruence. Therefore, .Since and are midpoints of sides, . This combined with yields.The area of trapezoid is .The area of triangle is .So the area of the pentagon is .The area of the squares is .Therefore, .Let the intersection of and be .Now we have and .Because both triangles has a side on congruent squares therefore .Because and are vertical angles .Also both and are right angles so .Therefore by AAS(Angle, Angle, Side) .Then translating/rotating the shaded into the position ofSo the shaded area now completely covers the squareSet the area of a square asTherefore, .25. Solution 1The radius of the ball is 2 inches. If you think about the ball rolling or draw a path for the ball (see figure below), you see that in A and C it loses inches, and it gains inches on B.So, the departure from the length of the track means that the answer is . Solution 2The total length of all of the arcs is . Since we want the path from the center, the actual distance will be shorter. Therefore, the only answer choice less than is . Thissolution may be invalid because the actual distance can be longer if the path the center travels is on the outside of the curve, as it is in the middle bump.古希腊哲学大师亚里士多德说:人有两种,一种即“吃饭是为了活着”,一种是“活着是为了吃饭”.一个人之所以伟大,首先是因为他有超于常人的心。
2001年AMC10测验试题

2001年AMC10測驗試題1.設下列資料:n,n+3,n+4,n+5,n+6,n+8,n+10,n+12,n+15的中位數是10,試問它們的平均數為何?(A)4 (B)6 (C)7 (D)10 (E)112.一數x比它的倒數與它的加法反元素(即相反數)的乘積多2,試問此數在下列那個區間中?(A)-4≦X≦-2 (B)-2<X≦0 (C)0<X≦2 (D)2<X≦4 (E)4<X≦63.二數之和為S,假設將每個數加3後均再2倍,試問最後二個新數之和為何?(A)2S+3 (B)3S+2 (C)3S+6 (D)2S+6 (E)2S+124.一個圓和一個三角形最多有幾個交點?(A)2 (B)3 (C)4 (D)5 (E)65.下列十二個不同圖形(每一個圖形均由5個小正方形所構成)中,有多少個圖形至少存在一條對稱軸?(A)3 (B)4 (C)5 (D)6 (E)76.設P(n)及S(n)分別表示正整數n的每一個位數其數字之乘積及和。
譬如P(23)=6,S(23)=5。
若N為二位數使得N=P(N)+S(N)時,則N的個位數字為何?(A)2 (B)3 (C)6 (D)8 (E)97.當一個正小數的小數點向右移動四位後,所得的新數是原數倒數的四倍,試問原數為下列何者?(A)0.0002 (B)0.002 (C)0.02 (D)0.2 (E)28.甲、乙、丙、丁四個人是學校數學實驗室的小老師,他們值班的日程表如下:甲每隔3天值班一次,乙每隔4天值班一次,丙每隔6天值班一次,丁每隔7天值班一次。
若今天他們四個人同時在實驗室值班,則最少須幾天後,他們會再度一起值班?(A)42 (B)84 (C)126 (D)178 (E)2529.克莉絲汀所居住的州所得稅之徵收辨法如下:年所得$28000(含)以下部分課以p%的稅,超出%28000部分則課以(p+2)%的稅,克莉絲汀發現他所付出的州所得稅等於他年所得的(p+0.25)%,試問她的全年所得是多少?(A)$28000 (B)$32000 (C)$35000 (D)$42000 (E)$5600010.設x,y,z都是正數,且xy=24, xz=48, yz=72,則x+y+z=?(A)18 (B)19 (C)20 (D)22 (E)2411.設想由單位正方形所構成之大正方形,右圖所示為其中的一部分,圍繞中心黑色正方形的第一圈共有8個單位正方形,第二圈共有16個單位正方形,依此類推,第100圈中的單位正方形的個數是?(A)396 (B)404 (C)800 (D)10,000 (E)10,40412.設n為三個連續整數的乘積並設n可被7整除,試問下列各數中那一個未必是n的因數?(A)6 (B)14 (C)21 (D)28 (E)4213.一個由不同數字所組成之電話號碼呈現ABC-DEF-GHIJ的形式,此號碼中的每一組數字皆成遞減之順序,即A>B>C, D>E>F, G>H>I>J。
2001年美国大学生数学建模大赛赛题

2001 Mathematical Contest in ModelingThe ProblemsProblem A: Choosing a Bicycle WheelProblem B: Escaping a Hurricane's Wrath (An Ill Wind...)Problem A: Choosing a Bicycle WheelCyclists have different types of wheels they can use on their bicycles. The two basic types of wheels are those constructed using wire spokes and those constructed of a solid disk (see Figure 1) The spoked wheels are lighter, but the solid wheels are more aerodynamic. A solid wheel is never used on the front for a road race but can be used on the rear of the bike.Professional cyclists look at a racecourse and make an educated guess as to what kind of wheels should be used. The decision is based on the number and steepness of the hills, the weather, wind speed, the competition, and other considerations. The director sportif of your favorite team would like to have a better system in place and has asked your team for information to help determine what kind of wheel should be used for a given course.Figure 1: A solid wheel is shown on the left and a spoked wheel is shown on theright.The director sportif needs specific information to help make a decision and has asked your team to accomplish the tasks listed below. For each of the tasks assume that the same spoked wheel will always be used on the front but there is a choice of wheels for the rear.Task 1. Provide a table giving the wind speed at which the power required for a solid rear wheel is less than for a spoked rear wheel. The table should include the windspeeds for different road grades starting from zero percent to ten percent in onepercent increments. (Road grade is defined to be the ratio of the total rise of a hilldivided by the length of the road. If the hill is viewed as a triangle, the grade is the sine of the angle at the bottom of the hill.) A rider starts at the bottom of the hill at a speedof 45 kph, and the deceleration of the rider is proportional to the road grade. A riderwill lose about 8 kph for a five percent grade over 100 meters.∙Task 2. Provide an example of how the table could be used for a specific time trial course.∙Task 3. Determine if the table is an adequate means for deciding on the wheel configuration and offer other suggestions as to how to make this decision.Problem B: Escaping a Hurricane's Wrath (An Ill Wind...)Evacuating the coast of South Carolina ahead of the predicted landfall of Hurricane Floyd in 1999 led to a monumental traffic jam. Traffic slowed to a standstill on Interstate I-26, which is the principal route going inland from Charleston to the relatively safe haven of Columbia in the center of the state. What is normally an easy two-hour drive took up to 18 hours to complete. Many cars simply ran out of gas along the way. Fortunately, Floyd turned north and spared the state this time, but the public outcry is forcing state officials to find ways to avoid a repeat of this traffic nightmare.The principal proposal put forth to deal with this problem is the reversal of traffic on I-26, so that both sides, including the coastal-bound lanes, have traffic headed inland from Charleston to Columbia. Plans to carry this out have been prepared (and posted on the Web) by the South Carolina Emergency Preparedness Division. Traffic reversal on principal roads leading inland from Myrtle Beach and Hilton Head is also planned.A simplified map of South Carolina is shown. Charleston has approximately 500,000 people, Myrtle Beach has about 200,000 people, and another 250,000 people are spread out along the rest of the coastal strip. (More accurate data, if sought, are widely available.)The interstates have two lanes of traffic in each direction except in the metropolitan areas where they have three. Columbia, another metro area of around 500,000 people, does not have sufficient hotel space to accommodate the evacuees (including some coming from farther north by other routes), so some traffic continues outbound on I-26 towards Spartanburg; on I-77 north to Charlotte; and on I-20 east to Atlanta. In 1999, traffic leaving Columbia going northwest was moving only very slowly. Construct a model for the problem to investigate what strategies may reduce the congestion observed in 1999. Here are the questions that need to be addressed:1.Under what conditions does the plan for turning the two coastal-bound lanes of I-26into two lanes of Columbia-bound traffic, essentially turning the entire I-26 intoone-way traffic, significantly improve evacuation traffic flow?2.In 1999, the simultaneous evacuation of the state's entire coastal region was ordered.Would the evacuation traffic flow improve under an alternative strategy that staggers the evacuation, perhaps county-by-county over some time period consistent with the pattern of how hurricanes affect the coast?3.Several smaller highways besides I-26 extend inland from the coast. Under whatconditions would it improve evacuation flow to turn around traffic on these?4.What effect would it have on evacuation flow to establish more temporary shelters inColumbia, to reduce the traffic leaving Columbia?5.In 1999, many families leaving the coast brought along their boats, campers, andmotor homes. Many drove all of their cars. Under what conditions should there berestrictions on vehicle types or numbers of vehicles brought in order to guaranteetimely evacuation?6.It has been suggested that in 1999 some of the coastal residents of Georgia and Florida,who were fleeing the earlier predicted landfalls of Hurricane Floyd to the south, came up I-95 and compounded the traffic problems. How big an impact can they have on the evacuation traffic flow?Clearly identify what measures of performance are used to comparestrategies. Required: Prepare a short newspaper article, not to exceed two pages, explaining the results and conclusions of your study to the public.Clearly identify what measures of performance are used to compare strategies.Required: Prepare a short newspaper article, not to exceed two pages, explaining the results and conclusions of your study to the public.。
2001AIME美国数学邀请赛

2001 AIME11、 求所有这样的两位数之和,它们满足能被其中任意数码整除.2、 不同实数的有限集S 满足如下性质:{}1S 的平均数比S 的平均数小13,且{}2001S 的平均数比S 的平均数大27,求S 的平均数.3、 找出方程200120011()02xx +-=的所有根,包括实根与虚根,假定方程没有复根.4、 在A B C ∆中,60,45A B ︒︒∠=∠=.A ∠的角平分线交B C 于点T ,且24A T =.A B C∆的面积可以写成a +其中a b c ,,都是正整数,且c 不能被任何素数的平方整除.求a b c ++.5、 椭圆2244x y +=中内接一等边三角形,它的一个顶点坐标是(0,1),一个高在y 轴上,m n 、是互质的正整数.求m n +.6、 掷筛子四次,后三次的点数总不比前面的点数小的概率可以表示为/m n ,其中m n 、是互质的正整数.求m n +.7、 在A B C ∆中,21,22A B A C ==和20B C =.点D 和点E 分别在A B 与A C ,使得D E平行于B C 且D E 通过A B C ∆的内心.此时/D E m n =,其中m n 、是互质的正整数.求m n +.8、 如果一个十进制数N 在七进制下的结果是N 的2倍,就把它叫做7-10双倍数.例如51是7-10双倍数,因为它在七进制下的结果是102.求最大的7-10双倍数.9、 在A B C ∆中,13,15A B B C ==和17C A =.点D 、点E 和点F 分别在A B 、B C 和C A .设,A D p A B B E q B C =⋅=⋅和C F r C A =⋅,其中p q r 、、都是正数,且满足23p q r ++=,22225p q r++=.D E F ∆与A B C ∆的面积之比可以写成/m n 的形式,其中m n 、是互质的正整数.求m n +.10、设点集S 的坐标x y z 、、都是整数且满足02,03,04x y z ≤≤≤≤≤≤.从S 中随机抽取两个不同点,则二者的中点仍在S 中的概率为/m n ,其中m n 、是互质的正整数.求m n +.11、在5N ⨯点阵中,这些点从左到右、从上到下依次编号(如第一行的序号是从1到N ,第二行的序号是从1N +到2N ,等等).从第i 行中任意抽取一点i P ,这样我们选择了5个点1234,,,P P P P 和5P .设i P 对应数i x .现在从第一列开始重新对点进行从左到右的编序,这时设i P 对应数是i y .我们发现规律12213445,,,x y x y x y x y ====且53x y =.求N 的最小可能值.12、一个球内切于顶点分别是(6,0,0),(0,4,0),(0,0,2)A B C ===和(0,0,0)D =的四面体.球的半径是/m n ,其中m n 、是互质的正整数.求m n +.13、在某个特定的圆中,角度为d 的弧对应的弦长是22厘米,角度为2d 的弧对应的弦长比角度为3d 的弧对应的弦长大20厘米,其中120d <.角度为3d 的弧对应的弦长可以表示为m -+m n 、是互质的正整数.求m n +.14、邮递员要给街道上的19个房子送邮件.他发现没有两个相邻的房子在同一天都收到邮件,且连续多于两个的房子必定会有一个房子收到邮件.求有多少种可能邮递的方法? 15、数1,2,3,4,5,6,7和8随机的写在正八面体的8个面上,使得每个面上的数都不相同.则不相邻的两数共用一个边的概率是/m n ,其中m n 、是互质的正整数.求m n +. 答案 1、 答案 630用a 表示满足题目条件性质的十位数,b 表示个位数,故|10,|10a a b b a b ++,推出|,|10a b b a .前一个条件成立须满足b ka =,其中k 是某一正整数.后一条件暗含着1,2,5k k k ===.因此满足条件的两位数是11,22,33,...,90,12,24,36,48和15.求和结果为1145121015630⋅+⋅+=. 2、 答案 651设含有n 个元素的集合S 的平均值是x .故1131n x x n +=-+ 和2001271n x x n +=++即:1(1)13(1)n x n x n +=+-+ 和 2001(1)27(n x n x n +=+++.二者相减得200040(1)n =+,故49n =.因此651x =.3、 答案 500 应用二项式定理20012001200120012200120012000199920001999110()()2212001200012001...22220012001250...2xx xx xxxx xx=+-=--⋅⎛⎫⎛⎫=-+⋅-+ ⎪ ⎪⎝⎭⎝⎭=-⋅+根据根的和的公式得到220012505002001⋅⋅=.4、 答案291注意到角C 与角A T C 都是75︒,因此24A C A T ==。
2000年-2002 美国AMC10

PABCD2000年 第01届 美国AMC10 (2000年2月 日 时间75分钟)1. 国际数学奥林匹亚将于2001年在美国举办,假设I 、M 、O 分别表示不同的正整数,且满足I ⨯M ⨯O =2001,则试问I +M +O 之最大值为 。
(A) 23 (B) 55 (C) 99 (D) 111 (E) 671 。
2. 2000(20002000)为 。
(A) 20002001 (B) 40002000 (C) 20004000 (D) 40000002000 (E) 20004000000 。
3. Jenny 每天早上都会吃掉她所剩下的聪明豆的20%,今知在第二天结束时,有32颗剩下,试问一开始聪明豆有 颗。
(A) 40 (B) 50 (C) 55 (D) 60 (E) 75 。
4. Candra 每月要付给网络公司固定的月租费及上网的拨接费,已知她12月的账单为12.48元,而她1月的账单为17.54元,若她1月的上网时间是12月的两倍,试问月租费是 元。
(A) 2.53 (B) 5.06 (C) 6.24 (D) 7.42 (E) 8.77 。
5. 如图M ,N 分别为PA 与PB 之中点,试问当P 在一条平行AB 的直在线移动时,下列各数值有 项会变动。
(a)MN长 (b) △PAB 之周长 (c) △PAB 之面积 (d) ABNM 之面积(A) 0项 (B) 1项 (C) 2项 (D) 3项 (E) 4项 。
6. 费氏数列是以两个1开始,接下来各项均为前两项之和,试问在费氏数列各项的个位数字中,最后出现的阿拉伯数字为 。
(A) 0 (B) 4 (C) 6 (D) 7 (E) 9 。
7. 如图,矩形ABCD 中,AD =1,P 在AB 上,且DP 与DB 三等分∠ADC ,试问△BDP 之周长为 。
(A) 3+33 (B) 2+334 (C) 2+2(D)2533+ (E) 2+335 。
amc数学竞赛试题

amc数学竞赛试题考试时间:2小时本试卷共分为两部分,共计10道题目。
请仔细阅读题目并按照要求作答。
第一部分:选择题从每道题的四个选项中选择一个正确答案,并将其标记在答题卡上。
1. 下列哪个数是一个有理数?A. √2B. πC. -1.5D. e2. 设P(x)为多项式(x+1)(x+2)(x+3),则P(1)的值为?A. 0B. 6C. -6D. 123. 若a, b, c满足a + b + c = 0,下列哪个等式成立?A. a^2 + b^2 + c^2 = 0B. a^3 + b^3 + c^3 = 0C. a^2 + b^2 + c^2 = 1D. a^3 + b^3 + c^3 = -3abc4. 几何数列的前两项为3和6,且每一项等于前一项与后一项的乘积。
则该数列的第四项为?A. 108B. 72C. 54D. 365. 设a, b, c为正整数,且满足a^2 + b^2 = c^2,则下列哪组数满足题设条件?A. (3, 4, 2)B. (4, 5, 6)C. (5, 12, 13)D. (6, 8, 9)第二部分:解答题请详细解答以下两道题目,并将解答过程和答案写在答题卡上。
6. 已知直角三角形ABC,∠ACB = 90°,AB = 5,BC = 12,请计算∠BAC的正弦值。
7. 给定一个集合S,包含n个不同的正整数。
如果从集合S中选择两个数,它们的和恰好等于100,有多少种不同的选择方法?请用组合数学的方法解决此问题。
答题注意事项:1. 答题前请仔细审题,理解题意;2. 解答题请写清楚解题过程,逐步推导;3. 如果需要用到公式或定理,请正确运用并说明原理;4. 请使用规定的答题卡进行作答,不要在试卷上直接书写。
考试结束后,请将答题卡交给监考人员。
祝你考试顺利!。
美国AMC10中文版试题及答案

2000到20XX年AMC10美国数学竞赛0 0P 0 A 0 B 0 C 0D 0 全美中学数学分级能力测验(AMC 10)2000年 第01届 美国AMC10 (2000年2月 日 时间75分钟)1. 国际数学奥林匹亚将于 在美国举办,假设I 、M 、O 分别表示不同的正整数,且满足I ⨯M ⨯O =2001,则试问I +M +O 之最大值为 。
(A) 23 (B) 55 (C) 99 (D) 111 (E) 6712. 2000(20002000)为 。
(A) 20002001 (B) 40002000 (C) 20004000 (D) 40000002000 (E) 200040000003. Jenny 每天早上都会吃掉她所剩下的聪明豆的20%,今知在第二天结束时,有32颗剩下,试问一开始聪明豆有 颗。
(A) 40 (B) 50 (C) 55 (D) 60 (E) 754. Candra 每月要付给网络公司固定的月租费及上网的拨接费,已知她12月的账单为12.48元,而她1月的账单为17.54元,若她1月的上网时间是12月的两倍,试问月租费是 元。
(A) 2.53 (B) 5.06 (C) 6.24 (D) 7.42 (E) 8.775. 如图M ,N 分别为PA 与PB 之中点,试问当P 在一条平行AB 的直 在线移动时,下列各数值有 项会变动。
(a) MN 长 (b) △P AB 之周长 (c) △P AB 之面积 (d) ABNM 之面积(A) 0项 (B) 1项 (C) 2项 (D) 3项 (E) 4项 6. 费氏数列是以两个1开始,接下来各项均为前两项之和,试问在费氏数列各项的个位数字中, 最后出现的阿拉伯数字为 。
(A) 0 (B) 4 (C) 6 (D) 7 (E) 97. 如图,矩形ABCD 中,AD =1,P 在AB 上,且DP 与DB 三等分∠ADC ,试问△BDP 之周长为 。
AMC10美国数学竞赛讲义

AMC 中的数论问题1:Remember the prime between 1 to 100:2 3 5 7 11 13 17 19 23 29 31 37 41 43 47 53 59 61 67 71 73 79 83 89 91 2:Perfect number: Letis the prime number.if21p - is also the prime number. then1(21)2p p --is the perfect number. For example:6,28,496.3: Let ,0n abc a =≠ is three digital integer .if 333n a b c =++Then the number n is called Daffodils number . There are only four numbers: 153 370 371 407 Let ,0n abcd a =≠ is four digital integer .if 4444d n a b c +=++Then the number n is called Roses number . There are only three numbers: 1634 8208 94744:The Fundamental Theorem of ArithmeticEvery natural number can be written as a product of primes uniquely up to order.5:Suppose that a and b are integers with b =0. Then there exists unique integers q and r such that 0 ≤ r< |b| and a = bq + r.6:(1)Greatest Common Divisor: Let gcd (a, b) = max {d ∈ Z: d | a and d | b}. For any integers a and b, we havegcd(a, b) = gcd(b, a) = gcd(±a, ±b) = gcd(a, b − a) = gcd(a, b + a). For example: gcd(150, 60) = gcd(60, 30) = gcd(30, 0) = 30(2)Least common multiple:Let lcm(a,b)=min{d∈Z: a | d and b | d }. (3)We have that: ab= gcd(a, b) lcm(a,b) 7:Congruence modulo nIf ,0a b mq m -=≠,then we call a congruence b modulo m and we rewritemod a b m ≡.(1)Assume .Ifthen we havemod a c b d m ±≡± , mod ac bd m ≡ , mod k k a b m ≡(2) The equation ax ≡ b (mod m) has a solution if and only if gcd(a, m) divides b.8:How to find the unit digit of some special integers (1)How many zero at the end of !nFor example, when 100n =, Let N be the number zero at the end of 100!then10010010020424525125N ⎡⎤⎡⎤⎡⎤=++=+=⎢⎥⎢⎥⎢⎥⎣⎦⎣⎦⎣⎦(2) ,,a n Z ∈Find the unit digit na . For example, when 100,3n a ==9:Palindrome, such as 83438, is a number that remains the same when its digits are reversed.Therearesomenumber not only palindrome but(1)Some special palindrome n that 2n is also palindrome. For example :222221111121111123211111123432111111111112345678987654321=====(2)How to create a palindrome? Almost integer plus the number of its reverseddigits and repeat it again and again. Then we get a palindrome. For example:87781651655617267266271353135335314884+=+=+=+=But whether any integer has this Property has yet to prove(3) The palindrome equation means that equation from left to right and right to left it all set up.For example :1242242112231132211121241388888831421211⨯=⨯⨯=⨯⨯==⨯Let ab and cde are two digital and three digital integers. If the digits satisfy the,,9a c b e d c e d ⨯=⨯=+≤, then ab cde edc ba ⨯=⨯.10: Features of an integer divisible by some prime number If n is even ,then 2|n一个整数n 的所有位数上的数字之和是3(或者9)的倍数,则n 被3(或者9)整除一个整数n 的尾数是零, 则n 被5整除一个整数n 的后三位与截取后三位的数值的差被7、11、13整除, 则n 被7、11、13整除一个整数n 的最后两位数被4整除,则n 被4整除 一个整数n 的最后三位数被8整除,则n 被8整除一个整数n 的奇数位之和与偶数位之和的差被11整除,则n 被11整除 11. The number Theoretic functions If 312123t rr rrt n p p p p =(1) {}12()#0:|(1)(1)(1)t n a a n r r r χ=>=+++(2)12222111222|()(1)(1)(1)t r r r t t t a nn a p p p p p p p p p δ==+++++++++∑(3){}11221111122()#:,gcd(,)1()()()ttr r r r r r t t n a N a n a n p p p p p p φ---=∈≤==---For example: 2(12)(23)(21)(11)6χχ=⋅=++= 22(12)(23)(122)(13)28δδ=⋅=+++=22(12)(23)(22)(31)4φφ=⋅=--=Exercise1. The sums of three whole numbers taken in pairs are 12, 17, and 19. What is the middle number?(A) 4 (B) 5 (C) 6 (D) 7 (E) 83. For the positive integer n, let <n> denote the sum of all the positive divisors of n with the exception of n itself. For example, <4>=1+2=3 and <12>=1+2+3+4+6=16. What is <<<6>>>?(A) 6 (B) 12 (C) 24 (D) 32 (E) 368. What is the sum of all integer solutions to 21<(x-2)<25? (A) 10 (B) 12(C) 15(D) 19(E) 510 How many ordered pairs of positive integers (M,N) satisfy the equation6=6M N(A) 6(B) 7(C) 8(D) 9(E) 101. Let a and b be relatively prime integers with >>0a b and 333-73=(-)3a b a b . What is -a b ?(A) 1 (B) 2(C) 3(D) 4(E) 515.The figures 123,,F F F and 4F shown are the first in a sequence of figures. For 3n ≥, n F is constructed from -1n F by surrounding it with a square and placing one more diamond on each side of the new square than -1n F had on each side of its outside square. For example, figure 3F has 13 diamonds. How many diamonds are there in figure 20F ?18. Positive integers a, b, and c are randomly and independently selected withreplacement from the set {1, 2, 3,…, 2010}. What is the probability thatabc ab a ++ is divisible by 3?(A)13(B)2981(C)3181(D)1127(E)132724. Let ,a b and c be positive integers with >>a b c such that 222-b -c +=2011a ab and 222+3b +3c -3-2-2=-1997a ab ac bc . What is a ?(A) 249 (B) 250 (C) 251 (D) 252 (E)2535. In multiplying two positive integers a and b, Ron reversed the digits of the two-digit number a. His erroneous product was 161. What is the correct value of the product of a and b?(A) 116 (B) 161 (C) 204 (D) 214 (E) 22423. What is the hundreds digit of 20112011?(A) 1 (B) 4 (C) 5 (D) 6 (E) 9 9. A palindrome, such as 83438, is a number that remains the same when its digits are reversed. The numbers x and x+32 are three-digit and four-digit palindromes, respectively. What is the sum of the digits of x?(A) 20 (B) 21 (C) 22 (D) 23 (E) 2421. The polynomial 322010x ax bx -+- has three positive integer zeros. What is the smallest possible value of a?(A) 78 (B) 88 (C) 98 (D) 108 (E) 11824. The number obtained from the last two nonzero digits of 90! Is equal to n. What is n?(A) 12 (B) 32 (C) 48 (D) 52 (E) 6825. Jim starts with a positive integer n and creates a sequence of numbers. Each successive number is obtained by subtracting the largest possible integer square less than or equal to the current number until zero is reached. For example, if Jim starts with n=55, then his sequence contain 5 numbers:55 55-72= 66-22= 22-12= 11-12= 0Let N be the smallest number for which Jim’s sequence has 8 numbers. What is the units digit of N?(A) 1 (B) 3 (C) 5 (D) 7 (E) 921.What is the remainder when 01220093+3+3++3is divided by 8?(A) 0(B) 1(C) 2(D) 4(E) 65.What is the sum of the digits of the square of 111,111,111? (A) 18 (B) 27(C) 45(D) 63(E) 8110064(A) 6 (B) 7 (C) 8 (D) 9 (E) 1024. Let 2200820082k =+. What is the units digit of 222k +? (A) 0(B) 1(C) 4(D) 6(E) 8AMC about algebraic problems一、Linear relations(1) Slope y-intercept form: y kx b =+ (k is the slope, b is the y-intercept) (2)Standard form: 0Ax By C ++=(3)Slope and one point 0000(,),()()P x y k slope y y k x x -=- (4) Two points 1122(,),(,)P x y P x y12121212y y y y y y x x x x x x ---==--- (5)x,y-intercept form: (,0),(0,),(0,0)1x y P a Q b a b a b≠≠+= 二、the relations of the two lines 111222:0,:0l A x B y C l A x B y C ++=++= (1) 1l ∥2l 122112210,0A B A B C B C B ⇔-=-≠ (1) 1l ⊥2l 12120A A B B ⇔-=三、Special multiplication rules:222223322332212211222112222()()()2()()()()()()(2)()((1)(1))(1)()n n n n n n n n n n n n n n a b a b a b a b a ab b a b a b a ab b a b a b a ab b a b a b a a b ab b n a b a b a a b ab b n is odd n a b c ab bc ac a b -----------=-+±=±+-=-+++=+-+-=-++++≥+=+-++-+->++=++⇔-22()()0b c c a a b c+-+-=⇔==四、quadratic equations and PolynomialThe quadratic equations 2(0)y ax bx c a =++≠ has two roots 12,x x then we has1212b c x x x x a a+=-=More generally, if the polynomial 121210nn n n n x a x a x a x a ---+++++=has n roots 123,,,,n x x x x ,then we have:1231122312123(1)n n n n n nx x x x a x x x x x x a x x x x a -++++=-++==-开方的开方、估计开方数的大小 绝对值方程Arithmetic Sequence123(1)(2)(3)()n m a a n d a n d a n d a n m d =+-=+-=+-==+-121321()()()()2222n n n m n m n n a a n a a n a a n a a s ---+++++=====1(1)2n n n ds na -=+If n=2k, then we have 1()n k k s k a a +=+ If n=2k+1, then we have 1n k s na += Geometric sequence123123n n n n m n m a a q a q a q a q ----=====1(1)1,1n n a q q s q-≠=-Some special sequence 1, 1, 2, 3, 5, 8,… 9,99,999,9999,… 1,11,111,1111,… Exercise4 .When Ringo places his marbles into bags with 6 marbles per bag, he has 4 marbles left over. When Paul does the same with his marbles, he has 3 marbles left over. Ringo and Paul pool their marbles and place them into as many bags as possible, with 6 marbles per bag. How many marbles will be left over?7 For a science project, Sammy observed a chipmunk and a squirrel stashing acorns in holes. The chipmunk hid 3 acorns in each of the holes it dug. The squirrel hid 4 acorns in each of the holes it dug. They each hid the same number of acorns, although the squirrel needed 4 fewer holes. How many acorns did the chipmunk hide?21. Four distinct points are arranged on a plane so that the segments connecting them have lengths ,,,,, and . What is the ratio of to ?6. The product of two positive numbers is 9. The reciprocal of one of these numbers is 4 times the reciprocal of the other number. What is the sum of the two numbers?8. In a bag of marbles,35of the marbles are blue and the rest are red. If the number of red marbles is doubled and the number of blue marbles stays the same, what fraction of the marbles will be red?13. An iterative average of the numbers 1, 2, 3, 4, and 5 is computed the following way. Arrange the five numbers in some order. Find the mean of the first two numbers, and then find the mean of that with the third number, then the mean of that with the fourth number, and finally the mean of that with the fifth number. What is the difference between the largest and smallest possible values that can be obtained using this procedure?16. Three runners start running simultaneously from the same point on a 500-meter circular track. They each run clockwise around the course maintaining constant speeds of 4.4, 4.8, and 5.0 meters per second. The runners stop once they are all together again somewhere on the circular course. How many seconds do the runners run?24. Let ,a b and c be positive integers with >>a b c such that 222-b -c +=2011a ab and 222+3b +3c -3-2-2=-1997a ab ac bc . What is a ?(A) 249 (B) 250(C) 251(D) 252(E) 2531. What is 246135135246++++-++++? (A) -1(B) 536(C) 712(D)14760(E)43310. Consider the set of numbers {1, 10, 102, 103 (1010)}. The ratio of the largest element of the set to the sum of the other ten elements of the set is closest to which integer?(A) 1 (B) 9 (C) 10 (D) 11 (E) 10125816x x +=-(A) -64 (B) -24 (C) -9 (D) 24 (E) 5764. Let X and Y be the following sums of arithmetic sequences: X= 10 + 12 + 14 + …+ 100. Y= 12 + 14 + 16 + …+ 102. What is the value of Y X -?(A) 92 (B) 98 (C) 100 (D) 102 (E) 1127. Which of the following equations does NOT have a solution? (A) 2(7)0x +=(B) -350x += (C) 20x --=(D) 80x -= (E) -340x -=16. Which of the following in equal to 962962-++? (A) 32(B) 26(C)722(D) 33 (E) 613. What is the sum of all the solutions of 2602x x x =--?(A) 32 (B) 60 (C) 92 (D) 120 (E) 12414. The average of the numbers 1, 2, 3… 98, 99, and x is 100x. What is x?(A)49101 (B)50101(C)12 (D)51101(E)509911. The length of the interval of solutions of the inequality 23a x b ≤+≤ is10. What is b-a?(A) 6 (B) 10 (C) 15 (D) 20 (E) 3013. Angelina drove at an average rate of 80 kph and then stopped 20 minutes for gas. After the stop, she drove at an average rate of 100 kph. Altogether she drove 250 km in a total trip time of 3 hours including the stop. Which equation could be used to solve for the time t in hours that she drove before her stop?(A) 880100()2503t t +-=(B) 80250t = (C) 100250t =(D) 90250t =(E) 880()1002503t t -+=21. The polynomial 32-2010x ax bx +- has three positive integer zeros. What is the smallest possible value of a?(A) 78 (B) 88 (C) 98 (D) 108 (E) 11815.When a bucket is two-thirds full of water, the bucket and water weigh kilograms. When the bucket is one-half full of water the total weight is kilograms. In terms of and , what is the total weight in kilograms when the bucket is full of water?13.Suppose thatand. Which of the following is equal tofor every pair of integers?16.Let ,,, and be real numbers with,, and. What is the sum of all possible values of?5. Which of the following is equal to the product?81216442008............481242004n n + (A) 251(B) 502(C) 1004(D) 2008 (E) 40167. The fraction 20082200622007220052(3)(3)(3)(3)-- simplifies to which of the following?(A) 1 (B) 9/4 (C) 3 (D) 9/2 (E) 913. Doug can paint a room in 5 hours. Dave can paint the same room in 7 hours. Doug and Dave paint the room together and take a one-hour break for lunch. Let t be the total time, in hours, required for them to complete the job working together, including lunch. Which of the following equations is satisfied by t ?(A) 11()(1)157t ++=(B) 11()1157t ++= (C) 11()157t +=(D) 11()(1)157t +-=(E) (57)1t +=15. Yesterday Han drove 1 hour longer than Ian at an average speed 5 miles per hour faster than Ian. Jan drove 2 hours longer than Ian at an average speed 10 miles per hour faster than Ian. Han drove 70 miles more than Ian. How many more miles did Jan drive than Ian?(A) 120 (B) 130 (C) 140 (D) 150 (E) 160AMC 中的几何问题一、三角形有关知识点1.三角形的简单性质与几个面积公式①三角形任何两边之和大于第三边; ②三角形任何两边之差小于第三边; ③三角形三个内角的和等于180°; ④三角形三个外角的和等于360°;⑤三角形一个外角等于和它不相邻的两个内角的和; ⑥三角形一个外角大于任何一个和它不相邻的内角。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
USA AMC 10 20011The median of the listis . What is the mean?Solution2A number is more than the product of its reciprocal and its additive inverse. In which interval does the number lie?Solution3The sum of two numbers is . Suppose 3 is added to each number and then each of the resulting numbers is doubled. What is the sum of the final two numbers?Solution4What is the maximum number of possible points of intersection of a circle and a triangle?Solution5How many of the twelve pentominoes pictured below have at least one line of symettry?Solution6Let and denote the product and the sum, respectively, of thedigits of the integer . For example, and . Supposeis a two-digit number such that . What is the units digit of ?Solution7When the decimal point of a certain positive decimal number is moved four places to the right, the new number is four times the reciprocal of the original number. What is the original number?Solution8Wanda, Darren, Beatrice, and Chi are tutors in the school math lab. Their schedule is as follows: Darren works every third school day, Wanda works every fourth school day, Beatrice works every sixth school day, and Chi works every seventh school day. Today they are all working in the math lab. In how many school days from today willthey next be together tutoring in the lab?Solution9The state income tax where Kristin lives is levied at the rate of of the first of annual income plus of any amount above . Kristin noticed that the state income tax she paid amounted to of her annual income. What was her annual income?Solution10If , , and are positive with , , and , then isSolution11Consider the dark square in an array of unit squares, part of which is shown. The first ring of squ ares around this center square contains unit squares. The second ring contains unit squares. If we continue this process, the number of unit squares in the ring isSolution12Suppose that is the product of three consecutive integers and that is divisible by . Which of the following is not necessarily a divisor of Solution13A telephone number has the form , where each letter represents a different digit. The digits in each part of the numbers are in decreasing order; that is, , , and . Furthermore, , , and are consecutive even digits; , , , and are consecutive odd digits; and . Find .Solution14A charity sells 140 benefit tickets for a total of . Some tickets sell for full price (a whole dollar amount), and the rest sells for half price. How much money is raised by the full-price tickets?Solution15A street has parallel curbs feet apart. A crosswalk bounded by two parallel stripes crosses the street at an angle. The length of the curb between the stripes is feet and each stripe is feet long. Find the distance, in feet, between the stripes.Solution16The mean of three numbers is 10 more than the least of the numbers and 15 less than the greatest. The median of the three numbers is 5. What is their sum?Solution17Which of the cones listed below can be formed from a sector of a circle of radius by aligning the two straight sides?A cone with slant height of and radiusA cone with height of and radiusA cone with slant height of and radiusA cone with height of and radiusA cone with slant height of and radiusSolution18The plane is tiled by congruent squares and congruent pentagons as indicated. The percent of the plane that is enclosed by the pentagons is closest toSolution19Pat wants to buy four donuts from an ample supply of three types of donuts: glazed, chocolate, and powdered. How many different selections are possible?Solution20A regular octagon is formed by cutting an isosceles right triangle from each of the corners of a square with sides of length . What is the length of each side of the octagon?Solution21A right circular cylinder with its diameter equal to its height is inscribed in a right circular cone. The cone has diameter and altitude , and the axes of the cylinder and cone coincide. Find the radius of the cylinder.Solution22In the magic square shown, the sums of the numbers in each row, column, and diagonal are the same. Five of these numbers are represented by , , , , and . Find .Solution23A box contains exactly five chips, three red and two white. Chips are randomly removed one at a time without replacement until all the red chips are drawn or all the white chips are drawn. What is the probability that the last chip drawn is white?Solution24In trapezoid , and are perpendicular to , with, , and . What is ?Solution25How many positive integers not exceeding are multiples of or but not ?。
