2012、11、10、09年电子科技大学研究生数理方程期末试卷
11年研究生试卷(答案)

五.(10分)今有赵、钱、孙、李、周五位教师,要承担语文、数学、物理、化学、英语五门课程。已知赵熟悉数学、物理、化学三门课程,钱熟悉语文、数学、物理、英语四门课程,孙、李、周都只熟悉数学、物理两门课程。问能否安排他们都只上他们熟悉的一门课程,使得每门课程都有人教(用图论方法求解)。
3.设 是图 的推广邻接矩阵,则 的 行 列元 等于由 中顶点 到顶点 的长度为_n_途径数目。
4.完全图 的邻接矩阵的最大特征值为_n_。
5.不同构的3阶单图共有___4___个。
6.设 阶图 是具有 个分支的森林,则其边数 。
7. 阶树( )的点连通度为___1___;边连通度为____1___;点色数为__2___; 若其最大度为 ,则边色数为___ __。
8.图 是 连通的,则 中任意点对间至少有_k__条内点不交路。
9.5阶度极大非哈密尔顿图族为___ ___和__ _____。
10.完全图 能够分解为 个边不相交的一因子之并。
11. 设连通平面图 具有5个顶点,9条边,则其面数为__6_; ( )阶极大平面图的面数等于__ ___; ( )阶极大外平面图的顶点都在外部面边界上时,其内部面共有 个。
A1: LA, S ; A2: MA, LA, G ; A3: MA, G, LA;
A4: G, LA, AC ; A5: AC, LA, S ; A6: G, AC;
A7: GT, MA, LA ; A8: LA,GT, S ; A9: AC, S, LA;
A10: GT, S。人只上一门自己所熟悉的课程。
六.(6分)设 是赋权完全偶图G=(V,E)的可行顶点标号,若标号对应的相等子图 含完美匹配 ,则 是G的最优匹配。
2011-2012-2电子科大《数字逻辑设计及应用》期末考试题参考解答
………密………封………线………以………内………答………题………无………效……电子科技大学2011 -2012学年第 二 学期期 末 考试 A 卷课程名称:_数字逻辑设计及应用__ 考试形式: 闭卷 考试日期: 20 12 年 07 月 02 日 考试时长:_120___分钟课程成绩构成:平时 30 %, 期中 30 %, 实验 0 %, 期末 40 % 本试卷试题由___六__部分构成,共__6___页。
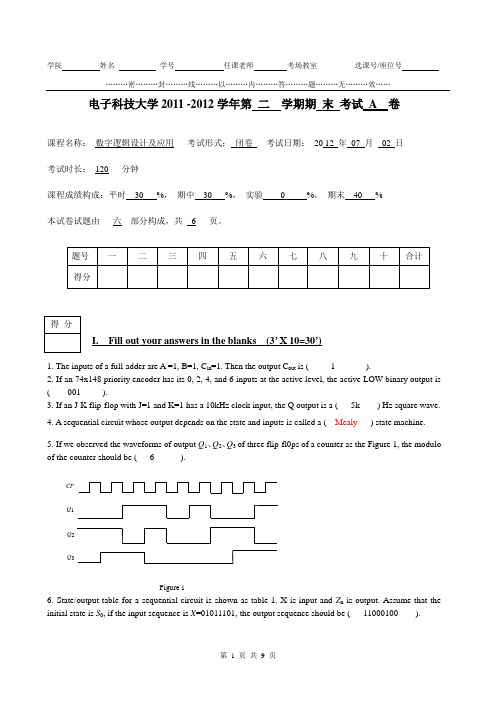
I. Fill out your answers in the blanks (3’ X 10=30’)1. The inputs of a full-adder are A =1, B=1, C in =1. Then the output C out is ( 1 ).2. If an 74x148 priority encoder has its 0, 2, 4, and 6 inputs at the active level, the active LOW binary output is ( 001 ).3. If an J-K flip-flop with J=1 and K=1 has a 10kHz clock input, the Q output is a (5k ) Hz square wave. 4. A sequential circuit whose output depends on the state and inputs is called a ( Mealy ) state machine. 5. If we observed the waveforms of output Q 1、Q 2、Q 3 of three flip-fl0ps of a counter as the Figure 1, the modulo of the counter should be ( 6 ).Figure 16. State/output table for a sequential circuit is shown as table 1. X is input and Z n is output. Assume that the initial state is S 0, if the input sequence is X =01011101, the output sequence should be ( 11000100 ).CP Q 1 Q 2 Q 3………密………封………线………以………内………答………题………无………效……7.Transition/output table for a sequential circuit is shown in Table 2, X is input and Y is output, the sequential circuit is a modulus ( 3 ) up/down counter.8. A 4-bit linear feedback shift-register (LFSR) counter with no self-correction can have ( 15 ) normal states. 9. A serial sequence generator by feedback shift registers 74x194 is shown in Figure 2, assume the initial state is Q 2Q 1Q 0=110, the feedback function LIN = Q 2/Q 1/ + Q 2Q 0/, the output sequence in Q 2 is ( 110100 ).Figure 210. When the input is 01000000 of an 8 bit DAC, the corresponding output voltage is 2V . The output voltage range for the DAC is ( 0 ~ 7.97 )V .II. Please select the only one correct answer in the following questions.(2’ X 5=10’)1. If a 74x85 magnitude comparator has ALTBOUT=AGTBOUT=0, AEQBOUT=1 on its outputs, the cascading inputs are ( B ).A) ALTBIN=0, AEQBIN=0, AGTBIN=0 B) ALTBIN=0, AEQBIN=1, AGTBIN=0 C) ALTBIN=1, AEQBIN=0, AGTBIN=1 D) ALTBIN=1, AEQBIN=1, AGTBIN=1 2. For an edge-triggered D flip-flop, ( D) is correct.A) a change in the state of the flip-flop can only at a clock pulse edge B) the state that the flip-flop goes to depends on the D inputn+1n 21………密………封………线………以………内………答………题………无………效……C) the output follows the input at each clock pulse D) all of these answers3. An asynchronous counter differs from a synchronous in ( B ). A) the number of states in its sequence B) the method of clocking C) the type of flip-flop used D) the value of the modulus4. A modulus-10 Johnson counter requires ( C ).A) ten flip-flopB) four flip-flopC) five flip-flopD) twelve flip-flop5. The capacity of a memory that has 10 bits address bus and can store 8 bits at each address is ( B ). A) 1024 B) 8192 C) 80 D) 256III. A D Latch and a D Flip-flop are shown in Figure 3. The waveforms of a clock CP and aninput D are shown in Figure 4. Assume the initial state of Q is 1. Try to draw the waveforms of Q of the D Latch and the D Flip-flop. Ignore the delay of the circuit. (15’ )Figure 3CP DttFigure 4Answer:………密………封………线………以………内………答………题………无………效……CP D 锁锁锁锁锁锁QQIn the waveform of the output, the delay of the gate circuit has been taken into consideration. 评分标准:1.锁存器和触发器Q 端波形上升、下降沿正确,得12分;每错一处扣1分,扣完12分为止;2.判断上升沿和高电平状态有效,得3分;错一个扣1分,扣完3分为止。
电子科技大学期末数字电子技术考试题a卷-参考答案

电子科技大学二零零九至二零一零学年第 二 学期期 末 考试数字逻辑设计及应用 课程考试题 A 卷(120分钟)考试形式:闭卷 考试日期2010年7月12日课程成绩构成:平时 20 分, 期中 20 分, 实验 0 分, 期末 60 分一、To fill your answers in the blanks (1’×25)1. If [X]10= - 110, then [X]two's-complement =[ ]2,[X]one's-complement =[ ]2. (Assumed the number system is 8-bit long) 2. Performing the following number system conversions: A. [10101100]2=[ 0 ]2421B. [1625]10=[01001 ]excess-3C. [ 1010011 ]GRAY =[10011000 ]8421BCD3. If ∑=C B A F ,,)6,3,2,1(, then F D ∑=C B A ,,( 1,4,5,6 )=C B A ,,∏(0,2,3,7 ).4. If the parameters of 74LS-series are defined as follows: V OL max = 0.5 V , V OH min = 2.7 V , V IL max = 0.8 V , V IH min = 2.0 V , then the low-state DC noise margin is 0.3V ,the high-state DC noise margin is 0.7V .5. Assigning 0 to Low and 1 to High is called positive logic. A CMOS XOR gate in positive logic is called XNOR gate in negative logic.6. A sequential circuit whose output depends on the state alone is called a Moore machine.7. To design a "001010" serial sequence generator by shift registers, the shift register should need 4 bit as least.8. If we use the simplest state assignment method for 130 sates, then we need at least8state variables.9. One state transition equation is Q*=JQ'+K'Q. If we use D flip-flop to complete the equation, the D input terminal of D flip-flop should be have the function D= JQ'+K'Q.10.Which state in Fig. 1 is ambiguous D11.A CMOS circuit is shown as Fig. 2, its logic function z= A’B’+ABFig. 1 Fig. 212.If number [A]two's-complement =01101010 and [B]one's-complement =1001, calculate [A-B]two's-complement and indicate whether or not overflow occurs.(Assumed the number system is 8-bit long)[A-B]two's-complement = 01110000, overflow no13. If a RAM’s capacity is 16K words × 8 bits, the address inputs should be 14bits; We need 8chips of 8K ⨯8 bits RAM to form a 16 K ⨯ 32 bits ROM..14. Which is the XOR gate of the following circuit A .15.There are 2n-n invalid states in an n-bit ring counter state diagram.16.An unused CMOS NOR input should be tied to logic Low level or 0 .17.The function of a DAC is translating the Digital inputs to the same value of analogoutputs.二、Complete the following truth table of taking a vote by A,B,C, when more than two of A,B,C approve a resolution, the resolution is passed; at the same time, the resolution can’t go through if A don’t agree.For A,B,C, assume 1 is indicated approval, 0 is indicated opposition. For the F,A B C F三、The circuit to the below realizes a combinational function F of four variables. Fill in the Karnaugh map of the logic function F realized by the multiplexer-based circuit. (6’)四、(A) Minimize the logic function expressionF = A·B + AC’ +B’·C+BC’+B’D+BD’+ADE(H+G) (5’)F = A·B + AC’ +B’·C+BC’+B’D+BD’ = A·(B ’C )’ +B’·C+BC’+B’D+BD’= A +B’·C+BC’+B’D+BD’+C ’D (或= A +B’·C+BC’+B’D+BD’+CD ’)= A +B’·C+BD’+C ’D (或= A + BC’+B’D +CD ’)(B) To find the minimum sum of product for F and use NAND-NAND gates to realize it (6’)),,,(Z Y X W F Π(1,3,4,6,9,11,12,14)------3分 F= X ’Z ’+XZ -----2分 =( X ’Z ’+XZ)’’=(( X ’Z ’)’(XZ)’)’ ------1分五、Realize the logic function using one chip of 74LS139 and two NAND gates.(8’)∑=)6,2(),,(C B A F ∑=)3,2,0(),,(E D C GF(A,B,C)=C’∑(1,3) ---- 3分 G(C,D,E)=C’∑(0,2,3) ----3分-六、Design a self-correcting modulo-6 counter with D flip-flops. Write out the excitation equations and output equation. Q2Q1Q0 denote the present states, Q2*Q1*Q0* denote the next states, Z denote the output. The state transition/output table is as following.(10’)Q2Q1Q0Q2*Q1*Q0*Z000 100 0100 110 0110 111 0111 011 0011 001 0001 000 1激励方程式:D2=Q0’(2分,错-2分)D1=Q2 (2分,错-2分)D0=Q1 (2分,错-2分)修改自启动:D2=Q0 +Q2Q1’(1分,错-1分)D1=Q2+Q1Q0’(1分,错-1分)D0=Q1+Q2Q0 (1分,错-1分)输出方程式:Z=Q1’Q0 (1分,错-1分)得分七、Construct a minimal state/output table for a moore sequential machine, that will detect the input sequences: x=101. If x=101 is detected, then Z=1.The input sequences DO NOT overlap one another. The states are denoted with S0~S3.(10’)For example:X:0 1 0 1 0 0 1 0 1 0 1 1 0 1 1 0 0 0 1 1 ……Z:0 0 0 1 0 0 0 0 1 0 0 0 0 1 0 0 0 0 0 0 ……state/output table八、Please write out the state/output table and the transition/output table and theexcitation/output table of this state machine.(states Q2 Q1=00~11, use the state name A~D )(10’)Transition/output table State/output table Excitation/output table(4分) (3分) (3分)评分标准:转移/输出表正确,得4分;每错一处扣0.5分,扣完4分为止;由转移/输出表得到状态/输出表正确,得3分;每错一处扣0.5分,扣完3分为止;激励/输出表正确,得3分;每错一处扣0.5分,扣完3分为止。
电子科技大学《数学实验》2008-2009学年期末试题(含答案)
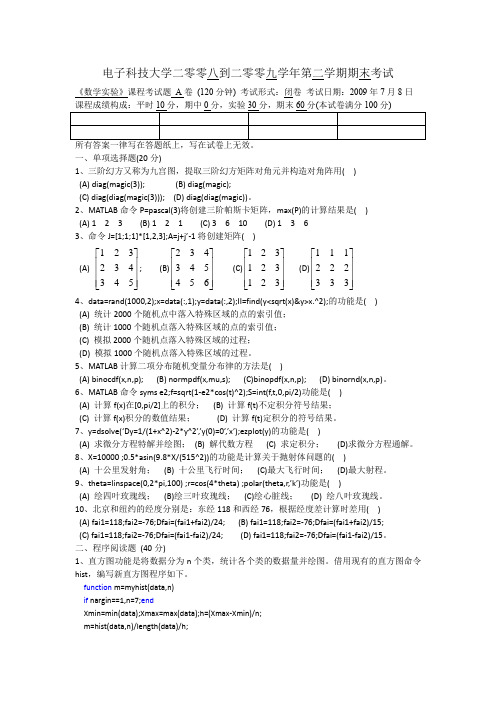
电子科技大学二零零八到二零零九学年第二学期期末考试《数学实验》课程考试题A卷(120分钟) 考试形式:闭卷考试日期:2009年7月8日一、单项选择题(20分)1、三阶幻方又称为九宫图,提取三阶幻方矩阵对角元并构造对角阵用( )(A) diag(magic(3)); (B) diag(magic);(C) diag(diag(magic(3))); (D) diag(diag(magic))。
2、MATLAB命令P=pascal(3)将创建三阶帕斯卡矩阵,max(P)的计算结果是( )(A) 1 2 3 (B) 1 2 1 (C) 3 6 10 (D) 1 3 63、命令J=*1;1;1+**1,2,3+;A=j+j’-1将创建矩阵( )(A)123234345⎡⎤⎢⎥⎢⎥⎢⎥⎣⎦; (B)234345456⎡⎤⎢⎥⎢⎥⎢⎥⎣⎦(C)123123123⎡⎤⎢⎥⎢⎥⎢⎥⎣⎦(D)111222333⎡⎤⎢⎥⎢⎥⎢⎥⎣⎦4、data=rand(1000,2);x=data(:,1);y=data(:,2);II=find(y<sqrt(x)&y>x.^2);的功能是( )(A) 统计2000个随机点中落入特殊区域的点的索引值;(B) 统计1000个随机点落入特殊区域的点的索引值;(C) 模拟2000个随机点落入特殊区域的过程;(D) 模拟1000个随机点落入特殊区域的过程。
5、MATLAB计算二项分布随机变量分布律的方法是( )(A) binocdf(x,n,p); (B) normpdf(x,mu,s); (C)binopdf(x,n,p); (D) binornd(x,n,p)。
6、MATLAB命令syms e2;f=sqrt(1-e2*cos(t)^2);S=int(f,t,0,pi/2)功能是()(A) 计算f(x)在[0,pi/2]上的积分;(B) 计算f(t)不定积分符号结果;(C) 计算f(x)积分的数值结果;(D) 计算f(t)定积分的符号结果。
电子科技大学数学物理方法研究生试题09-14

x R, t 0 utt uxx t sin x 4.求下面的定解问题: (15 分) . u ( x, 0) 0, u ( x, 0) sin x t
x2 4 a 2t
5.求证 F
1
e
a 2 2t
e 2a t
1
,其中 F (?) 表示 Fourier 逆变换.(15 分)
将该定解问题化成可直接分离变量求解的问题(不需要求出解的具体形式)。 3.长为 l 的均匀细杆,其侧面与左端保持零度,右端绝热,杆内初始温度分布为 ( x ) ,求杆内温 度分布 u ( x, t ) .(20 分)
utt 9u xx x 2 et x R, t 0 4.求下面的定解问题: (10 分) . u x, 0 x 2 x 18, u x, 0 sin x 18 t
7.写出球形域的 Dirichlets 问题对应的 Green 函数及其定解问题.(10 分)
8.证明:
d xJ1 ( x) xJ 0 ( x) . (10 分) dx
9. (1)写出 Legendre 方程和 Legendre 多项式; (2)将函数 f ( x) 2 3x, x 1 用 Legendre 多项式展开.(10 分)
1.化方程 x2uxx 2 xyuxy y 2u yy xux yu y 0 为标准形. (10 分)
utt a 2u xx 0 0 xl 2. 把定解问题: u x (0, t ) h1 (t ), u x (l , t ) h2 (t ) 的非齐次边界条件化为齐次边界条 u ( x, 0) ( x), u ( x, 0) ( x) 0 x l t
电子科技大学数值分析研究生期末考试习题二
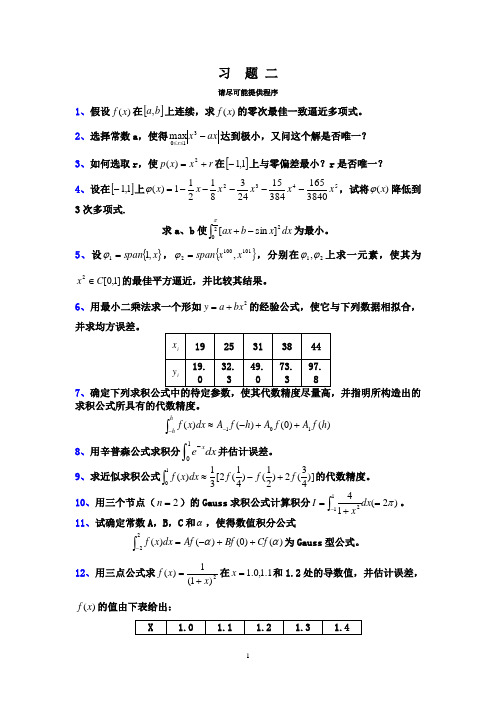
习 题 二请尽可能提供程序1、假设)(x f 在[]b a ,上连续,求)(x f 的零次最佳一致逼近多项式。
2、选择常数a ,使得ax x x -≤≤310max 达到极小,又问这个解是否唯一?3、如何选取r ,使r x x p +=2)(在[]1,1-上与零偏差最小?r 是否唯一?4、设在[]1,1-上543238401653841524381211)(x x x x x x -----=ϕ,试将)(x ϕ降低到3次多项式.求a 、b 使⎰-+202]sin [πdx x b ax 为最小。
5、设{}x span ,11=ϕ,{}1011002,x x span =ϕ,分别在21,ϕϕ上求一元素,使其为]1,0[2C x ∈的最佳平方逼近,并比较其结果。
6、用最小二乘法求一个形如2bx a y +=的经验公式,使它与下列数据相拟合,并求均方误差。
i x 19 25 31 38 44i y19.0 32.3 49.0 73.3 97.87、确定下列求积公式中的待定参数,使其代数精度尽量高,并指明所构造出的求积公式所具有的代数精度。
)()0()()(101h f A f A h f A dx x f hh++-≈--⎰8、用辛普森公式求积分1x e dx -⎰并估计误差。
9、求近似求积公式)]43(2)21()41(2[31)(10f f f dx x f +-≈⎰的代数精度。
10、用三个节点(2=n )的Gauss 求积公式计算积分)2(14112π=+=⎰-dx x I 。
11、试确定常数A ,B ,C 和α,使得数值积分公式)()0()()(22ααCf Bf Af dx x f ++-=⎰-为Gauss 型公式。
12、用三点公式求2)1(1)(x x f +=在1.1,0.1=x 和1.2处的导数值,并估计误差,)(x f 的值由下表给出:X1.0 1.11.2 1.3 1.4)(x f0.2500 0.2268 0.2066 0.1890 0.173613、就初值问题0)0(,=+='y b ax y 分别导出欧拉方法和改进的欧拉方法的近似解的表达式,并与准确解bx ax y +=221相比较。
成都电子科技大学概率论与数理统计2012-2016年考研初试真题+答案

第 1 页 共 4 页电子科技大学2016年攻读硕士学位研究生入学考试试题考试科目857 概率论与数理统计注:所有答案必须写在答题纸上,写在试卷或草稿纸上均无效。
一、 填空题(每题3分,共15分)1、任取一正整数,该数的平方的末位数是1的概率是__________.2、 设随机变量123,,X X X 相互独立,其中1X 在区间[0,6]上服从均匀分布,2X 服从正态分布2(0,2)N ,3X 服从参数为3λ=的泊松分布,记12323Y X X X =-+,则D (Y )=___________.3、 设随机变量X 服从参数为2的泊松分布,且Y =3X -2,则E (3Y +2)=__________.4、 设随机变量,X Y 相互独立且都服从正态分布2(0,3)N ,而129,,,X X X ⋅⋅⋅和129,,,Y Y Y ⋅⋅⋅为分别来自总体X 和Y 的简单随机样本,则统计量U =服从 ,参数为 . 5、 假设一批产品中一,二,三等品各占60%,30%,10%,从中随意取出一件,结果不是三等品,则取得的是一等品的概率为 .二、 单项选择题(每题3分,共15分)1、设当事件A 与B 同时发生时,事件C 必发生,则( )(A)()()()1P C P A P B ≤+- (B) ()()()1P C P A P B ≥+- (C) ()()P C P AB = (D) ()()P C P A B =2、设随机变量,X Y 均服从正态分布,2(,4)X N μ,2(,5)YN μ,记1{4}p P X μ=≤-,2{5}p P Y μ=≥+,则()第 2 页 共 4 页(A)对任何实数μ,都有12p p =(B )对任何实数μ,都有12p p < (C) 只对μ的个别值,才有12p p = (D )对任何实数μ,都有12p p > . 3、如果,ξη满足()()D D ξηξη+=-,则必有 ( ) (A)ξ与η独立 (B) ξ与η不相关 (C) 0D η=(D) 0D D ξη= 4、若设随机变量X 和Y 都服从标准正态分布,则( )(A) X +Y 服从正态分布 (B)22X Y +服从2χ分布 (C)2X 和2Y 都服从2χ分布 (D)22/X Y 服从F 分布 5、设12,,X X ⋅⋅⋅为独立同分布序列,且(1,2,)i X i =⋅⋅⋅均服从参数为4的指数分布,当n 比较大时,11ni i X n =∑近似服从 ( ). (A) 4(4,)N n(B) 11(,)416N n (C)11(,)416N (D) (4,)16n N 三、简答题(每题10分,共30分)1、 有两个口袋,甲袋中盛有两个白球,一个黑球,乙袋中盛有一个白球,两个黑球,由甲袋中任取一个球放入乙袋,再从乙袋中取出一个球,求取得白球的概率。
西安电子科技大学 2009年攻读硕士学位研究生入学考试试题

西安电子科技大学2009年攻读硕士学位研究生入学考试试题考试科目代码及名称822电磁场与微波技术考试时间2009年 1月 10日下午( 3小时)答题要求:所有答案〈填空题按照标号写〉必须写在答题纸上,写在试卷上一律作废,准考证号写在指定位置!一、(15分) z=0平面将无限大空间分为两个区域:z<0区域为空气,z>0区域为相对磁导率μr =1,相对介电常数εr =4的理想介质,若知空气中的电场强度为14x z E a a =+V/m ,试求:(1)理想介质中的电场强度E 2;(2)理想介质中电位移矢量D 2与界面间的夹角α;(3) z=0平面上的极化面电荷密度ρsp .二、(15分)均匀平面电磁波在相对磁导率μr =1的理想介质中传播,其电场强度的瞬时值为88(,)5sin[2(10)]5cos[2(10)]x v E r t a t z a t z ππ=-+-(mV/m ),试求:(1)该理想介质的相对介电常数εr ;(2)平面电磁波在该理想介质中的相速度V p ;(3)平面电磁波的极化状态。
三、(15分)空气中传播着磁场复矢量振幅(0.80.6)1()(34)12j x z x z H r a a e ππ-+=-mA/m ,的均匀平面电磁波,试求:(1)该平面电磁波的波长λ;(2)该平面电磁波传播方向的单位矢n ;(3)该平面电磁波电场的复振幅矢量 E®。
四、(15分)电场强度复振幅矢量2()24j z i x E r a e ππ-=(mA/m )的均匀平面电磁波由空气垂直入射到相对介电常数εr =2.25,相对磁导率μr =1的半无限大理想介质的界面(z=0平面),试求:(1)反射波电场强度的振幅E rm ;(2)反射波磁场的复振幅矢量H r (r);(3)透射波电场的复振幅矢量E t (r)。
五、(20分)己知无耗传输线电长度为θ,特性阻抗Z 0=1。
第五题用图(a )(1)已知负载阻抗L l l Z r jx =+,求负载驻波比ρL ;(2)求输入驻波比ρin ;(3)求负载反射系数ΓL 。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
2012、11、10、09年电子科技大学研究生数理方程期末试卷
电子科技大学研究生试卷
(考试时间: 14点 至 16 点 ,共 2小时)
课程名称 数理方程与特殊函数 教师 学时60 学分 3 教学方式 闭卷 考核日期 2012年 12 月 28 日 成绩 考核方式: (学生填写)
1.把方程
22222320u u u
x x y y
∂∂∂++=∂∂∂∂化为标准型,指出其
类型,求出其通解. (10分)
2.
设定解问题:(10分)
2000(),0,0,,0(),(),0.
tt xx x x l t t t u a u f x x l t u A u B t u x u x x l ϕψ====⎧-=<<>⎪⎪
==>⎨⎪==≤≤⎪⎩
将该定解问题化成可直接分离变量求解的问题(不需要求出解的具体形式)。
学 号 姓 学 院 教 座位
……………………密……………封……………线……………以……………
第 1页
3.
长为l 的均匀细杆,其侧面与左端保持零度,右端绝热,杆内初始温度分布为()x ϕ,求杆内温度分布
(,)u x t .
(20分)
4.求下面的定解问题:(10分)
22
009,(,0)18,sin 18
t
tt
xx t t t u u x e x R t u x x u x ==⎧-=∈>⎪⎨=++=+⎪⎩.
第2页
5.求22
cos()a e x d ϖτϖϖ+∞-⎰.(10分)
6. 222
23()(22)(25)
s s F s s s s s ++=++++,求Laplace 逆变换1
(())L F s -.(10分)
第3页
7.写出球形域的Dirichlets 问题对应的:(1) Green 函数及其定解问题. (2) Green 函数相对于边界外侧的方向导数.(10分)
8.设n ϖ(n=1,2,…)是0()0J x =的所有正根,将函数2()1(01)f x x x =-<<展开为Bessel 函数0()n J x ϖ的级数.(10分)
9.(1)写出Legendre 多项式的一般形式或罗德利克表示形式; (2)将函数2()23,1f x x x x =++≤用Legendre 多项式展开.(10分)
第4页。
