期权期货及其衍生产品约翰赫尔官方课件

合集下载

[考研专业课课件] 赫尔《期货、期权及其他衍生产品》课件第34章实物期权

常数）。定义V为两年后所报出的每平方英尺写字
楼空间的费用。假设每年都是预先交付租金。期权的收益是 100000 Amax（V-35，0）
其中A是由下式所给出的摊还因子
A 1 1 e 0.051 1 e 0.052 1 e 0.053 1 e 0.054 4.5355
ˆ V 30e 0.062 33.82 E
在风险中性世界里收益的期望值是110.15万
美元期权的价值为150.14e-0．05×2=135.86万美元结论：为这个期权支付100万美元是划算的。
34．3 估计风险市场价格
当一个特定变量存在历史数据时，可以用资本资产定价模型来估计它的风险市场价格。考虑一个仅依赖于这个变量的投资资产。定义： μ——投资资产收益率的期望； σ——投资资产收益的波动率； λ——变量的风险市场价格； ρ——变量的百分比变化与一个包含广泛股
考虑一项费用为1亿美元而延续5年的投资。
预期每年的现金流入估计为2500万美元。如果经过风险调整后的贴现率是12％（连续复利），那么投资的净现值（以百万计）是
100 25e 0.121 25e 0.122 25e 0.123 25e 0.124 25e 0.125 11.53
2．如何计算适用于基本项目（即没有隐含期
权的项目）贴现率。
34．2 风险中性定价的推广
在27．1节里，定义一个变量θ的风险市场价格为
r
（34-1）
对任何仅依赖于θ的可交易证券都会得到同样的风险市场价格λ。假设某个实物资产依赖于一些变量 θi（i=l，2，…）。令mi和σi分别为θi的增长
率期望和波动率，于是
d i
i
mi dt si dzi

期权期货及其衍生产品约翰赫尔官方课件97522共21页

Notation
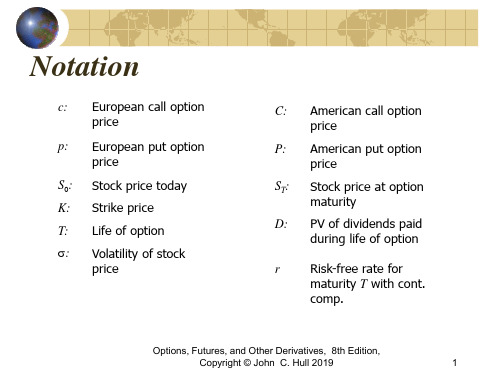
c: European call option price
p: European put option price
S0: Stock price today K: Strike price T: Life of option s: Volatility of stock
price
C: American call option price
ST > K ST − K
K ST 0 ST ST
ST < K 0 K K
K− ST ST K
Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
9
The Put-Call Parity Result (Equation
P: American put option price
ST: Stock price at option maturity
D: PV of dividends paid during life of option
r Risk-free rate for maturity T with cont. comp.
(Equation 10.5, page 221)
p Ke -rT–S0
Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
7
Put-Call Parity: No Dividends
Cc Pp
Options, Futures, and Other Derivatives, 8th Edition,

期权期货及其衍生产品约翰赫尔官方课件共29页

Copyright © John C. Hull 2019
2
Mechanics of Put Futures Option
When a put futures option is exercised the holder acquires
A short position in the futures A cash amount equal to the excess of the strike price over the futures price at the time of the most recent settlement
Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
1
Mechanics of Call Futures Options
When a call futures option is exercised the holder acquires
A long position in the futures A cash amount equal to the excess of the futures price at the time of the most recent settlement over the strike price
Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
6
Potential Advantages of Futures Options over Spot Options

[考研专业课课件] 赫尔《期货、期权及其他衍生产品》课件第28章利率衍生产品：标准市场模型

B T
和
d2 d1 B T
又如5．5节所述，FB可以利用以下公式来计算
B0 I FB P 0, T
（28-3）
B0——债券在0时刻的价格
I——期权有效期内债券所支付息票的贴现值
注意：公式中，即期债券价格与远期债券价
格均为现金价格（cash price），而非报价（ quoted price）。式（28-1）和式（28-2）中的执行价格K是现金价格，实际中通常执行价格为报价，所以K应等于执行价格+在到期日时的应计利息。
【例28-2】考虑一个10个月期的欧式看涨期
权，标的资产是期限为9.75年，面值为1 000美元的债券（当期权到期时，该债券的剩余期限为8年零11个月）。假设，债券的当前现金价格为960美元执行价格为1 000美元 10个月期的无风险利率为每年10％债券远期价格的波动率为每年9％
债券券息率为10％，每半年支付一次
2．对于债券持有人而言，由于看跌期权增加
了债券本身的价值，附有可退还条款的债券比没有这种条款的债券收益率要低。
应用：贷款、储蓄、贷款许诺
1．假定某金融机构的5年定期存款可以被提前提取而没有任何惩罚，那么它就包含一个债券美式看跌期权（存款是一个投资者有权在任何时刻以其面值卖给金融机构的债券）。 2．在贷款和有抵押贷款中可以提前付清的权利也是关于债券的看涨期权。
=0.9200，σB=0.09及T=10/12，由（28-1），看
涨期权的价格为9.49美元。
（b）如果执行价格是报价
因为期权的到期日是息票支付后的一个月，K 中必须再加上一个月的累计利息， K=1000+100×0.08333=1008.33 FB =939.68，P（0，T）=0.9200，σB=0.09， T=0.8333）由式（28-1），看涨期权价格为7.97美元。

期权期货及其衍生产品,约翰赫尔官方课件

Options, Futures, and Other Derivatives, 8th Edition, Copyright © John C. Hull 2012
14
Final Tree; Fig 33.2
E 44.35 B 30.49 A 20.00 C 21.56 D 15.25 F 31.37 G 22.18 H 15.69 I 11.10 J 45.68 K 32.30 L 22.85 M 16.16 N 11.43
dS q* (t ) a ln S dt sdz S

Assume a = 0.1, s = 0.2, and Dt = 1 year
Options, Futures, and Other Derivatives, 8th Edition, Copyright © John C. Hull 2012
H 0.2217 0.6566 0.1217
I 0.0867 0.0266 0.8867
Options, Futures, and Other Derivatives, 8th Edition, Copyright © John C. Hull 2012
13
Determining q(t)
The nodes on the tree are moved so that the expected commodity price equals the futures price Assume that the one-year, two-year and three-years futures price for the commodity are $22, $23, and $24, respectively

期权期货及其衍生产品约翰赫尔官方课件-PPT精选文档

Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
2
Day Count Conventions in the U.S. (Page 129)
Treasury Bonds: Actual/Actual (in period)
Bond: 8% Actual/ Actual in period.
4% is earned between coupon payment dates. Accruals on an Actual basis. When coupons are paid on March 1 and Sept 1, how much interest is earned between March 1 and April 1?
Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
10
Conversion Factor
Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
4
Examples continued
T-Bill: 8% Actual/360:
8% is earned in 360 days. Accrual calculated by dividing the actual number of days in the period by 360. How much interest is earned between March 1 and April 1?

期权期货及其衍生产品约翰赫尔官方课件97522PPT精品文档21页

Copyright © John C. Hull 2019
2
Effect of Variables on Option Pricing (Table 10.1, page 215)
Variable
c
p
C
P
S0
+
−
+
−
K
−
+
−
+
T
?
?
+
+
s
+
+
+
+
r
+
−
+
Байду номын сангаас
−
D
−
+
−
+
Options, Futures, and Other Derivatives, 8th Edition,
Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
11
Arbitrage Opportunities
Suppose that
c= 3 T = 0.25 K =30
Copyright © John C. Hull 2019
5
Lower Bound for European Call Option Prices; No Dividends (Equation 10.4, page 220)
c S0 –Ke -rT
Options, Futures, and Other Derivatives, 8th Edition,

期货期权及其衍生品配套课件(全34章)Ch03.ppt

Cost of Asset=S2 – (F2 – F1) = F1 + Basis
Options, Futures, and Other Derivatives, 7th International
Edition, Copyright © John C. Hull 2008
7
Short Hedge
Options, Futures, and Other Derivatives, 7th International
Edition, Copyright © John C. Hull 2008
8
Choice of Contract
Choose a delivery month that is as close as possible to, but later than, the end of the life of the hedge When there is no futures contract on the asset being hedged, choose the contract whose futures price is most highly correlated with the asset price. This is known as cross hedging.
Options, Futures, and Other Derivatives, 7th International
Edition, Copyright © John C. Hull 2008
6
Long Hedge
We define
F1 : Initial Futures Price F2 : Final Futures Price S2 : Final Asset Price If you hedge the future purchase of an asset by entering into a long futures contract then

1、下载文档前请自行甄别文档内容的完整性，平台不提供额外的编辑、内容补充、找答案等附加服务。
2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
3、如文档侵犯您的权益，请联系客服反馈,我们会尽快为您处理(人工客服工作时间：9:00-18:30)。

Bond: 8% Actual/ Actual in period.
4% is earned between coupon payment dates. Accruals on an Actual basis. When coupons are paid on March 1 and Sept 1, how much interest is earned between March 1 and April 1?
Chapter 6 Interest Rate Futures
Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
1
Day Count Convention
Defines:
the period of time to which the interest rate applies The period of time used to calculate accrued interest (relevant when the instrument is bought of sold
P 360 (100 Y ) n
Y is cash price per $100 P is quoted price
Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
7
Treasury Bond Price Quotes in the U.S
Cash price received by party with short position = Most recent settlement price × Conversion factor + Accrued interest
Options, Futures, and Other Derivatives, 8th Edition,
Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
4
Examples continued
T-Bill: 8% Actual/360:
8% is earned in 360 days. Accrual calculated by dividing the actual number of days in the period by 360. How much interest is earned between March 1 and April 1?
Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
5
The February Effect (Business Snapshot 6.1)
How many days of interest are earned between February 28, 2019 and March 1, 2019 when
Bond: 8% 30/360
Assumes 30 days per month and 360 days per year. When coupons are paid on March 1 and Sept 1, how much interest is earned between March 1 and April 1?
day count is Actual/Actual in period? day count is 30/360?
Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
6
Treasury Bill Prices in the US
Corporate Bonds: 30/360
Money Market Instruments:
Actual/360
Options, Futures, and Othopyright © John C. Hull 2019
3
Examples
Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
10
Conversion Factor
Cash price = Quoted price + Accrued Interest
Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
8
Treasury Bond Futures
Pages 132-136
Copyright © John C. Hull 2019
9
Example
Most recent settlement price = 90.00 Conversion factor of bond delivered = 1.3800 Accrued interest on bond =3.00 Price received for bond is 1.3800×90.00+3.00 = $127.20 per $100 of principal
Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
2
Day Count Conventions in the U.S. (Page 129)
Treasury Bonds: Actual/Actual (in period)