On Witt vector cohomology for singular varieties
小鼠脑电波周期节律与呼吸过程相关性分析

• 94•由于外界刺激引起大脑兴奋,产生脑电波信号,而脑电波的信号可以通过局部场电位信号来表示。
本文对局部场电位信号进行小波分解,分解成五个频率段的波形,分别对应脑电波中的五个波段,即δ 波段、θ 波段、α 波段、β 波和 γ 波段。
通过相关性分析方法,计算出分解之后的δ波段与呼吸过程平均发生率之间的相关性系数。
根据小鼠平均呼吸发生率与脑电波中δ 波段的积差相关系数,推断在睡眠状态下的小鼠脑电波的周期节律与呼吸过程具有一定的相关性。
1 引言脑电波来自于大脑内部,一般认为大脑在活动时,脑皮质细胞群之间就会形成电位差,从而在大脑皮质的细胞外产生电流。
而局部场电位(Local Field Potential ,LFP )则反映来自神经元网络局部神经核团的活动状态,它也是一种神经集合的协同行为。
所以LFP 信号是脑内某局部大量神经元树突电位和的综合反映。
对应于不同行为或思维的脑电波,称之为脑电波成份。
如果能分离出与行为相关联的脑电波,将有助于对大脑疾病的诊断。
由于人的大脑非常复杂,研究人的思维也相对困难。
由于人脑的复杂性,在研究脑电波形成机理时,科学工作者大多采用动物实验,比如小鼠等。
呼吸系统是人体直接与外界接触最密切的子系统。
人体通过呼吸系统从外界空气中摄取氧气,同时排出二氧化碳,维持人体基本代谢的平衡。
呼吸运动是有节律的,在中枢神经系统支配下呼吸肌可以自律性收缩,通过调节呼吸的幅度和频率能使肺泡通气量适应机体新陈代谢的需要。
而呼吸的节律受中枢调节控制。
脑电波是一种自发的有节律的神经电活动,可划分为五个波段,即δ 波段(1~3Hz )、θ 波段(4~7Hz )、α 波段(8~13Hz )、β 波段(14~30Hz )和 γ 波段(30~35Hz )(J.Ito,S.Roy,Y.Liu,M.Fletcher,L.Lu,J.D.Boughter,S.Grun,D.H.Heck,Whisker barrel cortex delta oscillations and gamma power in the awake mouse are linked to respiration,Nature Communication,2014)。
单位球上加权Bergman空间上加权复合算子的本性范数英文
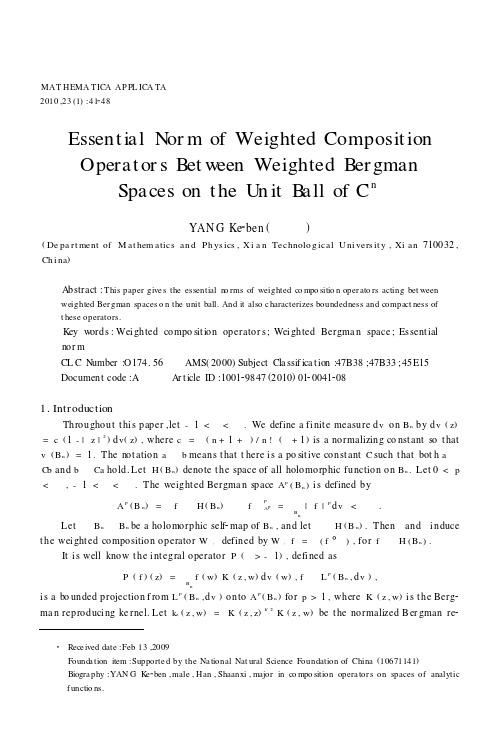
give s the essential no rms of weighted co mpo sitio n oper ato rs acting bet ween
weighted Ber gman spaces o n the unit ball. And it also c haracterizes boundedness and compact ness of
42
MAT HEMA TICA AP PL ICA TA
2010
2 (Bn ) . produci ng kernel ,which is unit vector i n Aα [1 ] Riedl charact erized boundednes s a nd compact nes s of compo si tion ope rator s bet ween p q ( p ≤ q) on Hardy spaces H p and Hq a nd Smit h [ 2] between wei ghte d Bergman spaces Aα and Aβ t he unit di sk ,in t erms of t he Neva nlinna and generalized Nevanli nna counti ng f unct io ns . The compact ne ss cri teria for t he case q < p were done by J archow [ 3 ] for t he Hardy space s and by Smit h and Yang[ 4] for t he weight ed Bergma n spaces . Esse ntial norm est imat es of composition operators f rom H p to Hq ( q < p ) on t he unit ball in C n were recentl y obt ai ned by Gorki n and MacClue r in [5 ]. p q In t hi s paper ,we give es sential norm esti mates for Wψ,φ f ro m Aα to Aβ , 0 < q ≤ p < ∞, - 1 < α,β < ∞ . The boundednes s and compact nes s of t he se operators a re al so charact erized.
非对称自变量线性各向同性张量函数的表示

非对称自变量线性各向同性张量函数的表示
郭仲衡
【期刊名称】《应用数学和力学》
【年(卷),期】1981(0)6
【摘要】本文给出了非对称自变量的线性各向同性标量值和张量值张量函数表示定理的数学证明.
【总页数】8页(P613-620)
【关键词】函数;量值;非对称;张量;自变量
【作者】郭仲衡
【作者单位】北京大学数学系
【正文语种】中文
【中图分类】O3
【相关文献】
1.非对称格林函数的指数梯度横向各向同性基体涂层系统 [J], F. Akbari;A. Khojasteh;M. Rahimian
2.鼓励学术争论,推动学术合作--张量和张量函数表示学术争论研讨会后记 [J], 黄克智;黄永念;郑泉水
3.关于各向同性张量函数的表示定理 [J], 梁浩云
4.非对称格林函数的指数梯度横向各向同性基体涂层系统 [J], F. Akbari;A. Khojasteh;M. Rahimian;
5.一类非对称各向同性张量函数导数的不变表示 [J], 王志乔;兑关锁
因版权原因,仅展示原文概要,查看原文内容请购买。
二维ising模型低温关联函数

二维ising模型低温关联函数摘要:1.引言2.二维ising 模型的概述3.低温关联函数的定义和性质4.二维ising 模型在低温关联函数中的应用5.结论正文:【引言】在统计力学中,ising 模型是一个自发磁化的二维晶格模型,由L.Onsager 于1944 年首次提出。
在这个模型中,晶格上的每个点上都有一个自旋变量,这些自旋变量可以是+1 或-1。
在低温下,ising 模型表现出一些有趣的物理性质,如磁化强度、相变等现象。
本文主要讨论二维ising 模型在低温关联函数中的应用。
【二维ising 模型的概述】二维ising 模型是一个自发磁化的二维晶格模型,其中晶格上的每个点上都有一个自旋变量,这些自旋变量可以是+1 或-1。
在这个模型中,自旋变量之间的相互作用是通过一个哈密顿量来描述的。
二维ising 模型的哈密顿量可以表示为:H = -J ∑<i,j> [S_i·S_j - n_i·n_j]其中,J 是相互作用强度,S_i 和S_j 分别是晶格点i 和j 上的自旋变量,n_i 和n_j 分别是晶格点i 和j 上的自旋数。
在这个模型中,自旋变量之间的相互作用是短程的,并且是异号的。
【低温关联函数的定义和性质】在二维ising 模型中,低温关联函数是用来描述自旋变量之间在低温下的相关性的重要物理量。
低温关联函数可以定义为:C(r) = S_i·S_j - n_i·n_j其中,S_i 和S_j 分别是晶格点i 和j 上的自旋变量,n_i 和n_j 分别是晶格点i 和j 上的自旋数,表示期望值。
在二维ising 模型中,低温关联函数C(r) 随着距离r 的增大而衰减,表现出短程相关的特性。
【二维ising 模型在低温关联函数中的应用】二维ising 模型在低温关联函数中的应用主要体现在以下几个方面:1.磁化强度:在低温下,二维ising 模型的磁化强度可以描述为:M = S_i = ∑_i S_i其中,S_i 是晶格点i 上的自旋变量。
k接近一致凸空间的对偶空间

为主要结果 ,得到了这两类 B n c a ah空 间的特征刻 画及 一些性质 ,弄清了 一致光滑 空间、
k接 近 一 致 光 滑 空 间 、
系.
接 近 一致 光 滑 空 间, 完全 k光 滑 空 间和 接 近 一 致 光 滑 空 间 的 蕴 涵关 接 近 一 致光 滑 空 间; 完全 光滑 空 间;
一
致 凸和 接近 一致 凸空间 的对偶 空 间,本 文将 引入 k接近 一致 光滑 和 接 近 一致光 滑 空
间.
本文 中, 表 示 实 B n c 间, a ah空 表 示 它的共 轭 空间 . S ) z: ∈X, l 1 ( ={ I ) = 和 ( ={ ∈X, I 1 ) : )分别 表示 的 单位球 和单 位球 面 . c ( ) B) 味着 和 o{ U 意 B 所 成集 合 的 凸包 ,这 里 B 对 序 列 { 令 spx ) n { 一 I: c X. z )c X, e ( =if『 l n≠ m} I . 对 序列 { )c X 及 X ∈X, 一l 叫 i m : X意 味着 X 弱收 敛于 X .对 序列 { }c X 及 ^
数
学
物
理
学
报
V 1l 03 _. A
17 99年, FS lvn2 引入了 忌一致 凸空间 (U ) . la [ ui ] k R 的概念 , 其对偶 空间 一致 光滑空 间 (US k )由苏雅 拉 图和吴 【 于 19 。 】 9 8年 引入 ,并证 明: k一致光 滑空 间蕴含 自反性 ,且 ( 或
足条件 s ( ) 的任何序列 { } ( , {d 】使 1l +… + l ~ e x >e p c ) 存在 n L , l X X l <1
高分子物理名词解释

高分子物理名词解释Θ溶剂(Θ solvent):链段-溶剂相互吸引刚好抵消链段间空间排斥的溶剂,形成高分子溶液时观察不到远程作用,该溶剂中的高分子链的行为同无扰链2.7Θ温度(Θ temperature):溶剂表现出Θ溶剂性质的温度2.7Argon理论(Argon theory):一种银纹扩展过程的模型,描述了分子链被伸展将聚合物材料空化的过程5.3Avrami方程(Avrami equation):描述物质结晶转化率与时间关系的方程:--α,α为转化率,K与n称Avrami常数(Avrami constants) 4.8 =Kt1n)ex p(Bingham流体(Bingham liquid):此类流体具有一个屈服应力σy,应力低于σy时不产生形变,当应力大于σy时才发生流动,应力高于σy的部分与应变速率呈线性关系3.13 Boltzmann叠加原理(Blotzmann superposition principle):Boltzmann提出的粘弹性原理:认为样品在不同时刻对应力或应变的响应各自独立并可线性叠加 3.8Bravais晶格(Bravais lattice):结构单元在空间的排列方式4.1Burger's模型(Burger's model):由一个Maxwell模型和一个Kelvin模型串联构成的粘弹性模型3.7Cauchy应变(Cauchy strain):拉伸引起的相对于样品初始长度的形变分数,又称工程应变3.16Charpy冲击测试(Charpy impact test):样品以简支梁形式放置的冲击强度测试,测量样品单位截面积的冲击能5.4Considère构图(Considère construction):以真应力对工程应作图以判定细颈稳定性的方法5.2Eyring模型(Eyring model):一种描述材料形变过程的分子模型,认为形变是结构单元越过能垒的跳跃式运动5.2Flory-Huggins参数(Flory-Huggins interaction parameter):描述聚合物链段与溶剂分子间相互作用的参数,常用χ表示,物理意义为一个溶质分子被放入溶剂中作用能变化与动能之比2.11.2Flory构图(Flory construction):保持固定拉伸比所需的力f对实验温度作图得到,由截距确定内能对拉伸力的贡献,由斜率确定熵对拉伸力的贡献2.16.2Flory特征比(characteristic ratio):无扰链均方末端距与自由连接链均方末端距的比值2.4 Griffith理论(Griffith theory):一种描述材料断裂机理的理论,认为断裂是吸收外界能量产生新表面的过程5.4Hencky应变(Hencky strain):拉伸引起的相对于样品形变分数积分,又称真应变3.16 Hermans取向因子(Hermans orientation factor):描述结构单元取向程度的参数,是结构单元与参考方向夹角余弦均方值的函数4.8, 4.10Hoffman-Weeks作图法(Hoffman-Weeks plot):一种确定平衡熔点的方法。
大学物理中矢量微积分的计算

0前言大学物理与中学物理相比,最显著的区别就应用矢量、导数和微积分来分析和求解生活实践中更一般的实际问题,微积分思想和方法的运用,使大学物理相比于中学物理有质的飞跃。
相对于高等数学只注重代数形式的导数和微积分性质和计算,大学物理中几乎全是矢量的导数和微积分模型的建立和求解[1],如果没有掌握矢量的导数和微积分的处理方法,对于解物理问题,往往会觉得无从下手。
本文就大学物理中矢量的导数和微积分的求解问题提出自己的一点见解,以期对初学者有所帮助。
1矢量和微积分思想矢量是既有大小又有方向的量。
大学物理中很多物理量都是用矢量的乘法来表示,这就涉及矢量的点积与叉积,如功W =F →·r →=Fr cosθ结果为标量,力矩M →=r →×F →结果为矢量,其中θ为两矢量之间的夹角。
与中学物理研究的大都是“常量”、“标量”,用代数和平面几何去解决生活实践中某个特殊类型的问题不同,大学物理中的研究的大都是“变量”、矢量”,用矢量和微积分来解决生活实践中更一般的实际问题。
对于一般物理实际问题,常常需要应用微积分来解决,其基本思想是先“微”后“积”。
由于物理量对时间或者空间分布不均,因而需要把研究物理量在时间或者空间范围内进行无限次分割,分割后的物理量在这些足够小的时空区域(即微元区域)就变成了均匀分布,这时恰当的选取微元,写出元过程或者元贡献的表达式,然后把所有有限小的过程累加求和[2],再应用定积分,确定积分上、下限,然后求得计算结果。
大学物理中的矢量求解,不管是微分还是积分,首先要将矢量标量化运算,也就是说先要把矢量向某一方向或者坐标系进行投影,然后再进行微积分运算。
大体可以归纳为两类,一类是矢量的微分或求导问题,一类是矢量的积分问题。
2矢量的求导问题这类问题在大学物理中比较简单,一般就是先把矢量在坐标系进行投影,然后再在各个分量方向上求导。
例如由位矢r →(t )求速度v →(t )和加速度a →(t ),则先对r →(t )“矢量标量化运算”,即把r →(t )向直接坐标系进行x ,y ,z 方向进行投影,即有r →(t )=x (t )i ^+y (t )j ^+z (t )k ^,然后在个方向上进行求导,如v →(t )=dr →dt =dx dt i ^+dy dt j ^+dz dt k^,同样的,求加速度也是先投影后求导,a →(t )=dv →dt =dvx dt i ^+dv y dt j ^+dv z dt k ^=d 2x dt 2i ^+d 2y dt 2j ^+d 2z dt2k ^。
薛定谔—麦克斯韦尔方程径向解的存在性和多重性(英文)

In 1887, the German physicist Erwin Schrödinger proposed a radial solution to the Maxwell-Schrödinger equation. This equation describes the behavior of an electron in an atom and is used to calculate its energy levels. The radial solution was found to be valid for all values of angular momentum quantum number l, which means that it can describe any type of atomic orbital.The existence and multiplicity of this radial solution has been studied extensively since then. It has been shown that there are infinitely many solutions for each value of l, with each one corresponding to a different energy level. Furthermore, these solutions can be divided into two categories: bound states and scattering states. Bound states have negative energies and correspond to electrons that are trapped within the atom; scattering states have positive energies and correspond to electrons that escape from the atom after being excited by external radiation or collisions with other particles.The existence and multiplicity of these solutions is important because they provide insight into how atoms interact with their environment through electromagnetic radiation or collisions with other particles. They also help us understand why certain elements form molecules when combined together, as well as why some elements remain stable while others decay over time due to radioactive processes such as alpha decay or beta decay.。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
a rX iv:mat h /51349v1[mat h.AG ]17Oct25ON WITT VECTOR COHOMOLOGY FOR SINGULAR VARIETIES PIERRE BERTHELOT,SPENCER BLOCH,AND H ´EL `ENE ESNAULT Abstract.Over a perfect field k of characteristic p >0,we con-struct a “Witt vector cohomology with compact supports”for sep-arated k -schemes of finite type,extending (after tensorisation with Q )the classical theory for proper k -schemes.We define a canonical morphism from rigid cohomology with compact supports to Witt vector cohomology with compact supports,and we prove that it provides an identification between the latter and the slope <1part of the former.Over a finite field,this allows one to compute con-gruences for the number of rational points in special examples.In particular,the congruence modulo the cardinality of the finite field of the number of rational points of a theta divisor on an abelian variety does not depend on the choice of the theta divisor.This answers positively a question by J.-P.Serre.Contents 1.Introduction 12.Witt vector cohomology with compact supports 63.A descent theorem 174.Witt vector cohomology and rigid cohomology 215.Proof of the main theorem 286.Applications and examples 33References 411.IntroductionLet k be a perfect field of characteristic p >0,W =W (k ),K =Frac(W ).If X is a proper and smooth variety defined over k ,the theory of the de Rham-Witt complex and the degeneration of the slope2PIERRE BERTHELOT,SPENCER BLOCH,AND H´EL`ENE ESNAULT spectral sequence provide a functorial isomorphism([6,III,3.5],[20, II,3.5])(1.1)H∗crys(X/K)<1∼−−→H∗(X,W O X)K,where H∗crys(X/K)<1is the maximal subspace on which Frobenius acts with slopes<1,and the subscript K denotes tensorisation with K.If we only assume that X is proper,but maybe singular,we can use rigid cohomology to generalize crystalline cohomology while retaining all the standard properties of a topological cohomology theory.Thus, the left hand side of(1.1)remains well defined,and,when k isfinite, the alternated product of the corresponding characteristic polynomials of Frobenius can be interpreted as the factor of the zeta functionζ(X,t)“of slopes<1”(see6.1for a precise definition).On the other hand,the classical theory of the de Rham-Witt complex can no longer be directly applied to X,but the sheaf of Witt vectors W O X is still available. Thus the right hand side of(1.1)remains also well defined.As in the smooth case,this is afinitely generated K-vector space endowed with a Frobenius action with slopes in[0,1[(Proposition2.10),which has the advantage of being directly related to the coherent cohomology of X.It is therefore of interest to know whether,when X is singular, (1.1)can be generalized as an isomorphism(1.2)H∗rig(X/K)<1∼−−→H∗(X,W O X)K,where H∗rig(X/K)<1denotes the subspace of slope<1of H∗rig(X/K). Our main result gives a positive answer to this question.More gener-ally,we show that a“Witt vector cohomology with compact supports”can be defined for separated k-schemes offinite type,giving cohomology spaces H∗c(X,W O X,K)which arefinite dimensional K-vector spaces, endowed with a Frobenius action with slopes in[0,1[.Then,for any such scheme X,the slope<1subspace of the rigid cohomology with compact supports of X has the following description:Theorem1.1.Let k be a perfectfield of characteristic p>0,X a sep-arated k-scheme offinite type.There exists a functorial isomorphism (1.3)−−→H∗c(X,W O X,K).H∗rig,c(X/K)<1∼This is a striking confirmation of Serre’s intuition[24]about the relation between topological and Witt vector cohomologies.On the other hand,this result bears some analogy with Hodge theory.Recall from[8](or[12])that if X is a proper scheme defined over C,then its Betti cohomology H∗(X,C)is a direct summand of its de Rham cohomology H∗(X,Ω•X).Coherent cohomology H∗(X,O X)does not exactly compute the corner piece of the Hodgefiltration,as would beWITT VECTOR COHOMOLOGY OF SINGULAR VARIETIES3 an exact analogy with the formulation of Theorem1.1,but it gives an upper bound.Indeed,by[14],Proposition1.2,and[15],Proof of Theorem1.1,one has a functorial surjective map H∗(X,O X)։gr0F H∗(X,C).The construction of these Witt vector cohomology spaces is given in section2.If U is a separated k-scheme offinite type,U֒→X an open immersion in a proper k-scheme,and I⊂O X any coherent ideal such that V(I)=X\U,we define W I=Ker(W O X→W(O X/I)),and we show that the cohomology spaces H∗(X,W I K)actually depend onlyon U(Theorem2.4).This results from an extension to Witt vectors of Deligne’s results on the independence on the compactification for the construction of the f!functor for coherent sheaves[18].When U varies,these spaces are contravariant functors with respect to proper maps,and covariant functors with respect to open immer-sions.In particular,they give rise to the usual long exact sequence relating the cohomologies of U,of an open subset V⊂U,and of the complement T of V in U.Thus,they can be viewed as providing a notion of Witt vector cohomology spaces with compact support for U. We defineH∗c(U,W O U,K):=H∗(X,W I K).Among other properties,we prove in section3that these cohomology spaces satisfy a particular case of cohomological descent(Theorem3.2) which will be used in the proof of Theorem1.1.The construction of the canonical homomorphism between rigid and Witt vector cohomologies is given in section4(Theorem4.5).First, we recall how to compute rigid cohomology for a proper k-scheme X when there exists a closed immersion of X in a smooth formal W-scheme P,using the de Rham complex of P with coefficients in an appropriate sheaf of O P-algebras A X,P.When P can be endowed with a lifting of the absolute Frobenius endomorphism of its specialfibre,a simple construction(based on an idea of Illusie[20])provides a func-torial morphism from this de Rham complex to W O X,ingˇCech resolutions,this morphism can still be defined in the general case as a morphism in the derived category of sheaves of K-vector spaces.Then we obtain(1.3)by taking the morphism induced on cohomology and restricting to the slope<1subspace.By means of simplicial resolu-tions based on de Jong’s theorem,we prove in section5that it is an isomorphism.The proof proceeds by reduction to the case of proper and smooth schemes,using Theorem3.2and the descent properties of rigid cohomology proved by Chiarellotto and Tsuzuki([10],[27],[28]).4PIERRE BERTHELOT,SPENCER BLOCH,AND H´EL`ENE ESNAULTWe now list some applications of Theorem1.1,which are developed in section6.Wefirst remark that it implies a vanishing theorem.If X is smooth projective,and Y⊂X is a divisor so that U=X\Y is affine,then Serre vanishing says that H i(X,O X(−nY))=0for n large and i<dim(X).If k has characteristic0,then we can take n=1by Kodaira vanishing.One then has H i(X,O X(−Y))=0for i<dim(X). It is tempting to view the following corollary as an analogue when k has characteristic p:Corollary1.2.Let X be a proper scheme defined over a perfectfield k of characteristic p>0.Let I⊂O X be a coherent ideal defining a closed subscheme Y⊂X such that U=X\Y is affine,smooth and equidimensional of dimension n.ThenH i c(U,W O U,K)=H i(X,W I)K=H i(X,W I r)K=0for all i=n and all r≥1.Indeed,when U is smooth and affine,H i rig(U/K)can be identified with Monsky-Washnitzer cohomology[4],and therefore vanishes for i>n=dim(U).If moreover U is equidimensional,it follows by Poincar´e duality[5]that H i rig,c(U/K)=0for i<n.So(1.3)implies that H i(X,W I)K=0for i<n.On the other hand,the closureU.Therefore H i(X,W I)K=0for i>n.When k is afinitefield F q,with q=p a,Theorem1.1implies a statement about zeta functions.By([16],Th´e or`e me II),the Lefschetz trace formula provides an expression of theζ-function of X as the alternating productζ(X,t)= i P i(X,t)(−1)i+1,where P i(X,t)=det(1−tφ|H i rig,c(X/K)),andφ=F a denotes theF q-linear Frobenius endomorphism of X.On the other hand,we define(1.4)P W i(X,t)=det(1−tφ|H i c(X,W O X,K)),ζW(X,t)= i P W i(X,t)(−1)i+1.If we denote byζ<1(X,t)the“slope<1factor”ofζ(X,t)(cf.6.1),we get formally from(1.3):WITT VECTOR COHOMOLOGY OF SINGULAR VARIETIES5 Corollary1.3.Let X be a separated scheme offinite type over afinite field.Then one has(1.5)ζ<1(X,t)=ζW(X,t).This result can be used to prove congruences mod q on the number of F q-rational points of certain varieties.The following theorem answers a question of Serre,and was the initial motivation for this work.Recall that an effective divisor D on an abelian variety A is called a theta divisor if O A(D)is ample and defines a principal polarization. Theorem1.4.LetΘ,Θ′be two theta divisors on an abelian variety defined over afinitefield F q.Then:(1.6)|Θ(F q)|≡|Θ′(F q)|mod q.Actually,Serre’s original formulation predicts that,on an abelian variety defined over afield,the difference of the motives ofΘandΘ′is divisible by the Lefschetz motive.Our Theorem1.4answers the point counting consequence of it.We also have more elementary point counting consequences. Corollary1.5(Ax[1],Katz[21]).Let D1,...,D r⊂P n be hypersur-faces of degrees d1,...,d r,defined over thefinitefield F q.Assume that j d j≤n.Then(1.7)|(D1∩...∩D r)(F q)|≡1mod q.We observe here that this congruence is the best approximation of the results of Ax and Katz that can be obtained using Witt vector cohomology,since this method only provides information on the slope <1factor of the zeta function.It would be worthwhile to have for higher slopes results similar to Theorem1.1which might give the full Ax-Katz congruences.We also remark that Ax’s theorem has a motivic proof[7],which of course is more powerful than this slope proof.Yet it is of interest to remark that Theorem1.1applies here as well.As for Theorem1.4,it seems more difficult to formulate a motivic proof,as it would have to deal with non-effective motives(see the discussion in6.6).Let us mentionfinally the following general consequence of Theorem 1.1,which for example can be applied in the context of the work of Fu and Wan on mirror congruences for Calabi-Yau varieties([29],[30]): Corollary1.6.Let f:X→Y be a morphism between two proper F q-−−→H i(X,O X) schemes.If f induces isomorphisms f∗:H i(Y,O Y)∼for all i≥0,then(1.8)|X(F q)|≡|Y(F q)|mod q.6PIERRE BERTHELOT,SPENCER BLOCH,AND H´EL`ENE ESNAULT Acknowledgements:It is a pleasure to thank Jean-Pierre Serre for hisstrong encouragement and his help.Theorem1.4was the main moti-vation for this work.We thank Luc Illusie for useful discussions.The third named author thanks Eckart Viehweg for his interest and en-couragement.Corollary1.2is inspired by the analogy on the relation between de Rham and coherent cohomology,which has been developed jointly with him.Notations and conventions:Throughout this article,k denotes a per-fectfield of characteristic p,W=W(k),σ:W→W the Frobenius automorphism of W,K=Frac(W).The subscript K will denote ten-sorisation with K over W.We recall that,on any noetherian topo-logical space,taking cohomology commutes with tensorisation by Q. Therefore the subscript K will be moved inside or outside cohomology or direct images without further justification.We denote by D b(K)(resp.D b(X,K))the derived category of bound-ed complexes of K-vector spaces(plexes of sheaves of K-vector spaces on a topological space X).All formal schemes considered in this article are W-formal schemes for the p-adic topology.2.Witt vector cohomology with compact supports We give in this section some properties of Witt vector cohomology which are a strong indication of its topological nature,and will be used later in our proof of Theorem1.1.In particular,we show how to attach Witt vector cohomology spaces with compact supports to any separated k-scheme offinite type.If X is a scheme,A a sheaf of rings on X,and n≥1,we denote by W n A,or W n(A)if confusion may arise,the sheaf of Witt vectors of length n with coefficients in A,and by W A=lim←−n W n A,or W(A), the sheaf of Witt vectors of infinite length.If I⊂A is an ideal,we denote by W n I=Ker(W n A→W n(A/I)),or W n(I),the sheaf of Witt vectors(a0,a1,...,a n−1)such that a i is a section of I for all i,and we define similarly W I.Note that,when I is quasi-coherent,the canonicalmorphism W I→R lim←−n W n I is an isomorphism,as H1(U,W n I)=0 for any affine open subset U⊂X and any n,and the projective system Γ(U,W n I)has surjective transition maps.For any X,any sheaf of rings A on X,and any ideal I⊂A,we use the notations RΓ(X,W I)and H∗(X,W I)to denote the ZariskiWITT VECTOR COHOMOLOGY OF SINGULAR VARIETIES7 cohomology of the sheaf W I.Thus,when I is quasi-coherent,the canonical morphismRΓ(X,W I)→R lim←−n RΓ(X,W n I)is an isomorphism.When X is a proper k-scheme and I⊂O X is a coherent ideal,the cohomology modules H∗(X,W n I)are artinian W-modules;then it follows from the Mittag-Leffler criterium that the morphismH∗(X,W I)→lim←−n H∗(X,W n I)is an isomorphism.We will shorten notations by writing W O X,K,W I K for(W O X)K, (W I)K.We recall again that,when X is noetherian,there is a canon-ical isomorphism−−→RΓ(X,W I K).RΓ(X,W I)K∼In this article,we will be particularly interested in the K-vector spaces H∗(X,W O X,K),and in their generalizations H∗(X,W I K).Our main observation is that,in contrast to the W-modules H∗(X,W O X), which are sensitive to nilpotent sections of O X,they behave like a topological cohomology theory for separated k-schemes offinite type. The following easy proposition is somehow a key point.Proposition2.1.Let X be a k-scheme offinite type.(i)The canonical homomorphismW O X,K→W O X red,Kis an isomorphism,and induces a functorial isomorphism−−→H∗(X red,W O X red,K),H∗(X,W O X,K)∼compatible with the action of F and V.√J,i.e.(ii)Let I,J⊂O X be coherent ideals,and assumethere exists N≥1such that I N⊂J and J N⊂I.Then there is a canonical identificationW I K∼=W J K,inducing a functorial isomorphismH∗(X,W I K)∼=H∗(X,W J K),compatible with the action of F and V.8PIERRE BERTHELOT,SPENCER BLOCH,AND H´EL`ENE ESNAULT Proof.Let N=Ker(O X→O X red).To prove thefirst claim of assertion (i),it suffices to show that W N K=0.But the action of p is invertible, and p=V F=F V,so it suffices to show F is nilpotent.This is clear since F acts on W N by raising coordinates to the p-th power.Taking cohomology,the second claim follows.Assertion(ii)follows from assertion(i)applied to O X/I and O X/J.We now prove the existence of Mayer-Vietoris exact sequences for Witt vector cohomology.Proposition2.2.Let X be a k-scheme offinite type,X1,X2⊂X two closed subschemes such that X=X1∪X2,and Z=X1∩X2.There is an exact sequence(2.1)0→W O X,K→W O X1,K⊕W O X2,K→W O Z,K→0, providing a Mayer-Vietoris long exact sequence···→H i(X,W O X,K)→H i(X1,W O X1,K)⊕H i(X2,W O X2,K)→H i(Z,W O Z,K)→H i+1(X,W O X,K)→···. Proof.Let I1,I2be the ideals of O X defining X1and X2.Thanks to 2.1,we may assume that O Z=O X/(I1+I2).It is easy to check that W(I1+I2)=W(I1)+W(I2).From the exact sequence0→O X/(I1∩I2)→O X/I1⊕O X/I2→O X/(I1+I2)→0,we can then deduce an exact sequence0→W(O X/(I1∩I2))→W O X1⊕W O X2→W O Z→0. Since X=X1∪X2,I1∩I2is a nilpotent ideal,and Proposition2.1 implies that W O X,K∼−−→W(O X/(I1∩I2))K.This gives the short exact sequence of the statement.The long one follows by taking coho-mology. Corollary2.3.Let X be a k-scheme offinite type,X1,...,X r⊂X closed subschemes such that X=X1∪···∪X r.For each sequence 1≤i0<···<i n≤r,let X i0,...,i n=X i0∩···∩X i n.Then the sequence(2.2)0→W O X,K→ri=1W O Xi,K→···→W O X1,...,r,K→0is exact.WITT VECTOR COHOMOLOGY OF SINGULAR VARIETIES9 Proof.The statement being true for r=2,we proceed by induction on r.Let X′=X2∪···∪X r.Up to a shift,the complex(2.2)is the coneof the morphism of complexes(2.3)0W O X2,...,r,K0 0 r i=2W O X1,i,K W O X1,...,r,KW O X,K W O X′,K00W O X1∩X′,K10PIERRE BERTHELOT,SPENCER BLOCH,AND H´EL`ENE ESNAULT Lemma 2.5.Let I⊂A be an ideal in a ring A.For all integers n≥2,r,s∈N,defineW r,s n(I)={(a0,a1,...)∈W n(A)|a0∈I r,a i∈I s for all i≥1}.(i)If s≤pr,the subset W r,s n(I)is an ideal of W n(A),which sits in the short exact sequence of abelian groups−−−→I r→0.(2.7)0→W n−1(I s)V−→W r,s n(I)R n−1(ii)If r≤s≤pr,the sequence(2.7)sits in a commutative diagram of short exact sequences(2.8)0W n(I s)I s00V R n−100W n(I r)R n−10where the vertical arrows are the natural inclusions.Proof.Assumefirst that s≤pr.The subset W r,s n(I)can be described as the set of Witt vectors of the form adenotes the Teichm¨u ller representative of a.To prove that it is an additive subgroup,it suffices to verify that if a,a′∈I r,then a′=a+a′(x0,x1,x2,...)=(ax0,a p x1,a p2x2,...),V(b)x=V(bF(x)).If we assume in addition that r≤s,then the vertical inclusions of the diagram are defined,and its commutativity is obvious.We will use repeatedly the following elementary remark.WITT VECTOR COHOMOLOGY OF SINGULAR VARIETIES11 Lemma2.6.Let C be an abelian category,and let(2.9)EvGG′′wFbe a commutative diagram of morphisms of C such that the horizontal sequence is exact.If u′=0and u′′=0,then w◦v=0.Proof.Exercise. Lemma2.7.(i)Under the assumptions of Theorem2.4,(i)there exists an integer a≥0such that,for all q≥1,all n≥1and all r≥0, the canonical morphism(2.10)R q f∗(W n(I′r+a))→R q f∗(W n(I′r))is the zero morphism.−−→U,and define (ii)Assume that f induces an isomorphism U′∼K r n=Ker W n(I r)→f∗(W n(I′r)) ,C r n=Coker W n(I r)→f∗(W n(I′r)) .Then there exists an integer a≥0such that,for all n≥1and all r≥0,the canonical morphisms(2.11)K r+an→K r n,C r+a n→C r n,are the zero morphisms.Proof.Wefirst prove assertion(i).We mayfix q≥1,since R q f∗=0 for q big enough.When n=1,we can apply Deligne’s result[18,App., Prop.5]to O X′,and we obtain an integer b≥0such that,for all r≥0, the canonical morphismu1:R q f∗(I′r+b)→R q f∗(I′r)is0(note that,for this result,Deligne’s argument only uses that f is finite above U).Let us prove by induction on n that,for all n≥1and all r≥b,the canonical morphismu n:R q f∗(W n(I′r+b))→R q f∗(W n(I′r))is also0.The condition r≥b implies that the couple(r,r+b)is such that r≤r+b≤pr.Therefore,we may use Lemma2.5to define ideals (I′)⊂W n(O X′),and,for n≥2,we obtain a commutative W r,r+bn12PIERRE BERTHELOT,SPENCER BLOCH,AND H´EL`ENE ESNAULT diagram(2.8)relative to I′and(r,r+b).Since the middle row of (2.8)is a short exact sequence,the diagramR q f∗(W n(I′r+b))R q f∗(I′r+b)u1V R n−1R q f∗(W n(I′r))obtained by applying the functor R q f∗to(2.8)has an exact middle row.As u1=0,and the composition of the middle vertical arrows is u n,Lemma2.6shows by induction that u n=0for all n≥1.If we set a=2b,then assertion(i)holds.Assertion(ii)can be proved by the same method.Let R= r≥0I r. It follows from[17,3.3.1]that the quasi-coherent graded R-modules K1= r≥0K r1and C1= r≥0C r1arefinitely generated.As the restric-tion of f to U′is an isomorphism,they are supported in Y.Therefore, there exists an integer m such that I m K r1=I m C r1=0for all r≥0. Moreover,there exists an integer d such that,for all r≥d the canonical morphismsI⊗O X K r1→K r+11,I⊗O X C r1→C r+11,are surjective.It follows that the morphismsK r+m1→K r1,C r+m1→C r1are0for r≥d.Replacing m by b=d+m,the corresponding mor-phisms are0for all r≥0,which proves assertion(ii)when n=1. As K r1⊂O X for all r,this implies that K r1=0for r≥b.Thanks to the exact sequences−−−→K r1,0→K r n−1V−→K r n R n−1it follows that K r n=0for all n≥1and all r≥b.Thus assertion(ii) holds for the modules K r n,with a=b.To prove it for the modules C r n,we introduce for r≥b the modulesC r,r+b n=Coker W r,r+b n(I)→f∗(W r,r+b n(I′)) .WITT VECTOR COHOMOLOGY OF SINGULAR VARIETIES13 The snake lemma applied to the diagramsf∗(W n−1(I′r+b))V f∗(I′r)gives exact sequences−−−→C r1.C r+b n−1V−→C r,r+b n R n−1The functoriality of the diagrams(2.8)imply that these exact sequences sit in commutative diagramsC r+b n C r+b1u1V R n−1C r n,where the morphisms u i are the canonical morphisms,and the com-position of the middle vertical arrows is u n.As u1=0,Lemma2.6 implies by induction that u n=0for all n.This proves assertion(ii) for C r n,with a=2b.2.8.Proof of Theorem2.4.Under the assumptions of2.4,let q≥1be an integer,and let a be an integer satisfying the conclusion of Lemma2.7(i)for the family of sheaves R q f∗(W n(I′r)),for all n≥1 and r≥0.Let c be such that p c>a.Since the Frobenius map F c:R q f∗(W n(I′r))→R q f∗(W n(I′r))factors through R q f∗(W n(I′p c r)), it follows from Lemma2.7that F c acts by zero on R q f∗(W n(I′r)),for all n≥1and all r≥1.Therefore,for all r≥1,F c acts by zero onR lim←−n(R q f∗(W n(I′r))).In particular,F c acts by zero on the sheaves lim←−n R q f∗(W n I′)and R1lim←−n R q f∗(W n I′).As the inverse system(W n I′)n≥1has surjective transition maps,and terms with vanishing cohomology on affine open subsets,it is lim←−n-acyclic,and we obtainR f∗(W I′)=R f∗(R lim←−n W n I′)∼=R lim←−n R f∗(W n I′).14PIERRE BERTHELOT,SPENCER BLOCH,AND H´EL`ENE ESNAULTThis isomorphism provides a biregular spectral sequenceE i,j2=R i lim←−n R j f∗(W n I′)⇒R i+j f∗(W I′),in which thefiltration of the terms R i+j f∗(W I′)is of length≤2sincethe functors R i lim←−n are zero for i≥2.As F c acts by zero on the terms E i,j2for j≥1,F2c acts by zero on the terms R i+j f∗(W I′)for i+j≥2.On the other hand,the term E1,02=R1lim←−n f∗(W n I′)is0,be-cause the morphisms f∗(W n+1I′)→f∗(W n I′)are surjective(since f∗(W n I′)∼=W n(f∗(I′))),and the cohomology of the terms f∗(W n I′) vanishes on any open affine subset.Therefore,F c acts by zero on R1f∗(W I′).Thus the action of F on R q f∗(W I′)is nilpotent for all q≥1.Since this action becomes an isomorphism after tensorisation with K,we obtain that R q f∗(W I′K)=R q f∗(W I′)K=0for all q≥1,which proves assertion(i)of Theorem2.4.Let us assume now that f:U′→U is an isomorphism.It follows from Lemma2.7(ii)that there exists an integer a such that,for alln≥1and all r≥0,the morphisms K r+an→K r n and C r+a n→C r n are zero. Taking c such that p c>a,it follows that F c acts by zero on K r n and C r n for all n and all r.Therefore,F c acts by zero on lim←−n K1n and lim←−n C1n.It is easy to check that these two inverse systems and the inverse system Im W n I→f∗(W n I′) all have surjective transition maps.As theirterms have vanishing cohomology on affine open subsets,they are lim←−-acyclic,and we obtainKer W I→f∗(W I′) =lim←−n K1n,Coker W I→f∗(W I′) =lim←−n C1n.After tensorisation with K,F becomes an isomorphism on lim←−n K1n and lim←−n C1n,and assertion(ii)of Theorem2.4follows.2.9.We now observe that the previous results imply that the coho-mology spaces H∗(X,W I K)only depend on the k-scheme U,and have the same functoriality properties with respect to U than cohomology with compact supports.Indeed,suppose U isfixed,and let U֒→X1,U֒→X2be two open immersions into proper k-schemes.Let X⊂X1×k X2be the scheme theoretic closure of U embedded diagonally into X1×k X2.The two projections induce proper maps p1:X→X1,p2:X→X2.If I⊂O X,I1⊂O X1,I2⊂O X2are the ideals defining Y=(X\U)red,WITT VECTOR COHOMOLOGY OF SINGULAR VARIETIES15 Y1=(X1\U)red and Y2=(X2\U)red,we deduce from2.1and2.4that the homomorphismsRΓ(X2,W I2,K)p∗2−→RΓ(X,W I K)p∗1←−RΓ(X1,W I1,K)are isomorphisms.If we defineε12=p∗−11◦p∗2:RΓ(X2,W I2,K)∼−→RΓ(X1,W I1,K),it is easy to check that the isomorphismsεij satisfy the transitivity condition for a third open immersion U֒→X3into a proper k-scheme. Therefore,they provide canonical identifications between the cohomol-ogy complexes RΓ(X,W I K)defined by various open immersions of U into proper k-schemes X.Assume U is a separated k-scheme offinite type.Since,by Nagata’s theorem,there exists a proper k-scheme X and an open immersion U֒→X,this independence property allows to define the Witt vector cohomology with compact supports of U by setting(2.12)RΓc(U,W O U,K):=RΓ(X,W I K)∼=RΓ(X,W I)K,(2.13)H∗c(U,W O U,K):=H∗(X,W I K)∼=H∗(X,W I)K, where I⊂O X is any coherent ideal defining the closed subset X\U. As the restriction of W I to U is W O U,there is a canonical morphism RΓc(U,W O U,K)→RΓ(U,W O U,K),which is an isomorphism when U is proper.These cohomology groups have the following functoriality properties:(i)They are contravariant with respect to proper maps.Let f:U′→U be a proper k-morphism of separated k-schemes, and let U′֒→X′,U֒→X be open immersions into proper k-schemes. Replacing if necessary X′by the scheme theoretic closure of the graph of f in X′×k X,we may assume that there exists a k-morphism g: X′→X extending f,and that U′is dense in X′.As f is proper,it follows that U′=g−1(U).If I⊂O X is any coherent ideal such that V(I)=X\U,then I′=IO X′is such that V(I′)=X′\U′,and we can define the homomorphismf∗:RΓc(U,W O U,K)→RΓc(U′,W O U′,K)as beingg∗:RΓ(X,W I K)→RΓ(X′,W I′K).We leave as an exercise to check that,up to canonical isomorphism,f∗does not depend on the choices.(ii)They are covariant with respect to open immersions.16PIERRE BERTHELOT,SPENCER BLOCH,AND H´EL`ENE ESNAULTLet j:V֒→U be an open immersion,let U֒→X be an open immersion into a proper k-scheme,and let I,J⊂O X be the ideals of Y=(X\U)red and Z=(X\V)red.Then the homomorphismj∗:RΓc(V,W O V,K)→RΓc(U,W O U,K)is defined as beingRΓ(X,W J K)→RΓ(X,W I K).If T=U\V=Z∩U,then T is open in the proper k-scheme Z,and the ideal I/J⊂O Z defines the complement of T in Z.Thus,the usual distinguished triangle(2.14)RΓc(V,W O V,K)→RΓc(U,W O U,K)→RΓc(T,W O T,K)+1−→is obtained by tensoring the short exact sequence0→W J→W I→W(I/J)→0,with K,and taking its cohomology on X.Proposition2.10.For any separated k-scheme offinite type U,the co-homology spaces H∗c(U,W O U,K)arefinite dimensional K-vector spaces, on which the Frobenius endomorphism has slopes in[0,1[.Proof.It suffices to prove the statement when U is a proper k-scheme X.Then the statement reduces to thefiniteness of the usual cohomol-ogy spaces H∗(X,W O X)K.This is a well-known result(cf.[6,III,Th.2.2],which is valid for W O X without the smoothness assumption).For the sake of completeness,we give a proof here.Write M:=H i(X,W O X).As X is proper,the groups H i(X,W n O X) satisfy the Mittag-Leffler condition,so that the homomorphismH i(X,W O X)→lim←−n H i(X,W n O X)is an isomorphism.Therefore,the Verschiebung endomorphism endows M with a structure of module over the non necessarily commutative ring R:=Wσ[[V]],where the indexσrefers to the commutation rules aV=Vσ(a)for a∈W.Then M is separated and complete for the V-adic topology.On the other hand,M/V M֒→H i(X,O X)is a finite dimensional k-vector space.Since M/V M isfinitely generated over W,it follows that M isfinitely generated over R.Moreover, the fact that M/V M hasfinite length implies that M is a torsion R-module.Thus,there exists afinite number of non zero elements a i(V)=a i,0+a i,1V+a i,2V2+...∈R,i=1,...,r,and a surjection(2.15)ri=1R/Ra i(V)։M.WITT VECTOR COHOMOLOGY OF SINGULAR VARIETIES17 Fix some i,1≤i≤r.We are interested in the module structure after inverting p,so we may assume that a i,ℓ∈W(k)×for someℓ.Letℓbeminimal,so p|a i,j,0≤j<ℓ.Even if the ring R is not commutative, we can get a factorizationja i,j V j= c i,0+c i,1V+...b i,0+...+b i,ℓ−1Vℓ−1+Vℓ ;p|b i,j. To see this,we factorj a i,j V j≡c(s)i,0+c(s)i,1V+... b(s)i,0+...+b(s)i,ℓ−1Vℓ−1+Vℓ mod p s+1,where b(s+1)i,j ,c(s+1)i,j≡b(s)i,j,c(s)i,j mod p s+1,starting with b(0)i,j=0andc(0)i,j=a i,j+ℓ.Note that c(s)i,0∈W(k)×.Details are standard and are left for the reader.Writing b i(V)=b i,0+b i,1V+...+Vℓwith b i,j=lim b(s)i,j, it follows that R/Ra i(V)∼=R/Rb i(V)∼=W(k)⊕ℓ.Thefiniteness now follows from(2.15).As H∗(X,W O X)is afinitely generated W-module modulo torsion, the slopes of the Frobenius endomorphism are positive.On the other hand,the existence of a Verschiebung endomorphism V such that F V=V F=p implies that all slopes are≤1.As V is topologi-cally nilpotent,there cannot be any non zero element of slope1in H∗(X,W O X,K),and all its slopes belong to[0,1[.3.A descent theoremWe will need simplicial resolutions based on de Jong’s fundamental result on alterations.In this section,we briefly recall some related definitions,and how to construct such resolutions.We then prove for Witt vector cohomology with compact supports a particular case of ´e tale cohomological descent which will be one of the main ingredients in our proof of Theorem1.1.If X is a scheme,and n≥−1an integer,we denote as usual by sk X n the truncation functor from the category of simplicial X-schemes to the category of n-truncated simplicial X-schemes,and by cosk X n its right adjoint[12,5.1].Definition3.1.Let X be a reduced k-scheme offinite type,and letX•be a simplicial k-scheme(resp.N-truncated simplicial scheme,forsome N∈N).a)A k-augmentation f•:X•→X is called a proper hypercovering(resp.N-truncated proper hypercovering)of X if,for all n≥0(resp.。
