Tutorial_4_solutions
教程的英文

教程的英文Title: Crafting an Informative TutorialIntroduction (50 words):A tutorial is a valuable tool that teaches and guides individuals on how to accomplish a specific task or gain new knowledge. In this article, we will explore the essential elements required to create an informative tutorial, and provide useful tips for ensuring its effectiveness in a variety of contexts.1. Choose a Relevant Topic (100 words):A successful tutorial begins with selecting a topic that is relevant and beneficial to the target audience. Consider their needs, interests, and skill levels. Conduct research to determine popular topics or emerging trends. Additionally, it is essential to ensure that the subject matter has not been extensively covered to avoid redundancy and engage learners effectively.2. Define Clear Objectives (100 words):Clearly define the objectives of the tutorial to establish a framework for the content. Objectives outline what learners will be able to accomplish or understand after completing the tutorial. These should be specific, measurable, achievable, relevant, and time-bound (SMART). Objectives provide readers with a sense of direction and motivation throughout the tutorial.3. Break Down Complex Concepts (100 words):Complex concepts can intimidate learners and hinder their ability to grasp the content. Break down complex ideas into smaller, manageable parts. Use simple language and provide examples,analogies, or illustrations to enhance understanding. Additionally, consider using interactive elements such as quizzes, exercises, or step-by-step instructions to encourage active learning and participation.4. Provide Step-by-Step Instructions (100 words):Step-by-step instructions are a crucial aspect of any tutorial. Present each step clearly and concisely, allowing learners to follow along easily. Use bullet points or numbered lists to organize the instructions effectively. Additionally, incorporating visual aids such as screenshots, diagrams, or videos can enhance comprehension, especially for technical or visual tutorials.5. Include Troubleshooting Tips (100 words):Anticipate common obstacles or challenges learners may encounter during the tutorial and provide troubleshooting tips or solutions. This will aid in preventing frustration and assist learners in overcoming obstacles independently. Including a Frequently Asked Questions (FAQ) section or a troubleshooting guide can be helpful for participants seeking additional assistance. Conclusion (50 words):Creating an informative tutorial requires careful consideration of the target audience, clear objectives, breakdown of complex concepts, step-by-step instructions, and troubleshooting tips. By following these guidelines, tutorial creators can effectively educate and empower individuals to gain new skills, knowledge, or experiences. Remember, a well-executed tutorial is a valuable resource that can make a significant impact on the learning journey of its audience.。
4-Labsolutions_讲解
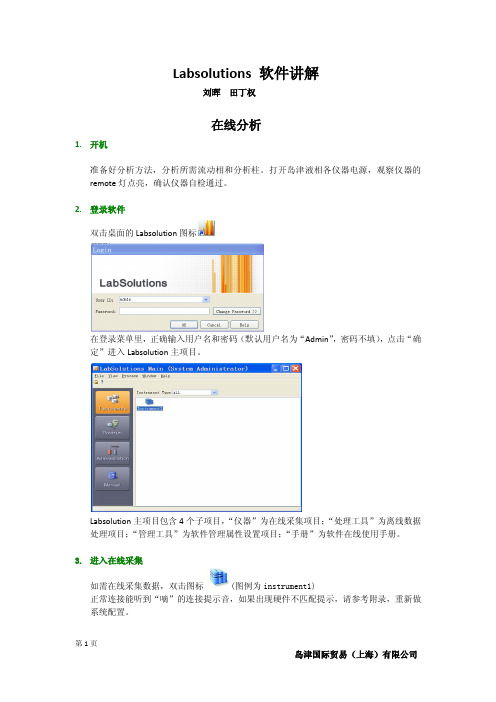
Labsolutions 软件讲解刘晖田丁权在线分析1.开机准备好分析方法,分析所需流动相和分析柱。
打开岛津液相各仪器电源,观察仪器的remote灯点亮,确认仪器自检通过。
2.登录软件双击桌面的Labsolution图标在登录菜单里,正确输入用户名和密码(默认用户名为“Admin”,密码不填),点击“确定”进入Labsolution主项目。
Labsolution主项目包含4个子项目,“仪器”为在线采集项目;“处理工具”为离线数据处理项目;“管理工具”为软件管理属性设置项目;“手册”为软件在线使用手册。
3.进入在线采集如需在线采集数据,双击图标(图例为instrument1)正常连接能听到“嘀”的连接提示音,如果出现硬件不匹配提示,请参考附录,重新做系统配置。
4.仪器参数设置打开已存在的方法或新建方法。
如需新建方法,则点击图标,激活“仪器参数视图”菜单,输入数据采集参数“常规”normal标签下为常用的仪器参数,“高级”advanced标签下为所有可设置的仪器参数。
以“高级”标签为例,需要设置的参数如下:“数据采集”Data Acquisition 子栏目设置需在“LC结束时间”LC Stop Time填入分析所需时间(例如2min),然后点击“应用于所有采集时间”。
确认需使用的检测器“采集时间”前已打勾。
“时间程序”子栏目设置一般为需要做梯度洗脱时使用,根据特定的梯度洗脱程序来编辑。
如第一行输入“时间”(例如0.01),“仪器”选择“泵”,“参数”选择“B泵浓度”,“数值”里输入B泵的浓度(例如50%)编辑各行。
最后一行“时间”输入结束时间(例如15min),“仪器”选择“系统控制器”,“参数”选择“stop”,“数值”不填(注意,最后一行系统控制器停止必需填写,否则无法退出时间程序编辑)点击“绘制曲线”,检查梯度曲线是否正确。
“泵”子栏目设置。
设置泵的模式,如有2台泵请选“二元高压梯度”模式;1台泵配备有低压四元比例阀请选“低压梯度”模式。
tutorial_1 soluation

Hardware Macstuff Winstuff 200 100
Software 1000 1500
In this economy the firm whose opportunity cost of producing a good is lowest is said to have a comparative advantage (CA) in producing that good. The firm with an absolute advantage (AA) in producing a good produces that good with the least amount of resources. Opportunity cost of producing 1 unit of: Hardware Macstuff Winstuff 5 units of software 15 units of software Software 1/5 units of hardware 1/15 units of hardware
Question 3 There are two firms: Macstuff and Winstuff. Each firm can produce either software or hardware using their resources. With one unit of its own resources Macstuff can produce 200 units of hardware or 1000 units of software. With one unit of its own resources Winstuff can produce 100 units of hardware or 1500 units of software. Each firm has 10 units of resources to allocate to the production of software and hardware. Suppose Macstuff and Winstuff agree to specialise their production according to their comparative advantage in producing software and hardware, and to then exchange with each other. Identify the bounds on the terms at which they would agree to trade software for hardware? Express your answer in terms of the amount of hardware that would be ‘paid’ for software. Explain your answer.
envisionMATHS在线教学指南说明书

enVisionMATHS Online Tutorial Guide 4Tutorial 4.1: Tools4Maths OverviewIntroductionThis guide will introduce you to Tools4Maths so that you can develop an understanding of the tools and how you might use them in your classroom.Purpose of Tools4MathsTools4Maths is a suite of digital, lively, animated maths learning tools.These tools help students deepen their understanding of important maths concepts as they explore and interact with a variety of digital objects.Teachers can use Tools4Maths to model solutions to maths problems during whole-class or small-group instruction. Students can use Tools4Maths to demonstrate their knowledge, practise maths skills and explore maths concepts.Pearson AustraliaWhere is Tools4Maths?Tools4Maths is on the Interactive Whiteboard DVD for each year level. It can be accessed byclicking on the Tools4Maths icon at the bottom of every screen on the DVD.Benefits of Tools4MathsStudents benefit from seeing maths ideas portrayed pictorially. Interacting with digitalmanipulatives also actively engages them in thinking about and solving problems. You may already use physical manipulatives such as place-value blocks, play money, tangrams or geoboards in your classes.Consider how digital manipulatives may have some advantages over physical manipulatives. Tools4Maths provides quick and easy access to a large number of counters, shapes and Australian money.Students can see more easily how teachers are manipulating objects when Tools4Maths is projected onto a screen or interactive whiteboard.The digital environment allows you to create unique workspaces where teachers and students can measure time in different formats; count frogs, fish or round objects; and explore probability outcomes. These are just a few examples of what Tools4Maths can do. And, there is no cleaning up!Options for UseThere are a variety of ways to use Tools4Maths that suit individual instructional styles and meet the needs of students.With a computer connected to an LCD projector or TV monitor, use Tools4Maths during whole-class instruction.With an interactive whiteboard, provide examples of concepts or model maths solutions. Invite students to interact with Tools4Maths on the whiteboard to explain their thinking to classmates. Tools4Maths is also ideal for small-group work using different computers around the classroom.Also, students can work independently with Tools4Maths on their own computers.Pearson Australia。
LinearAlgebraStrang4thSolutionManual

Linear Algebra Strang 4th Solution ManualDownload HereIf looking for a ebook Linear algebra strang 4th solution manual linear-algebra-strang-4th-solution-manual.pdf in pdf format, then you have come on to right site. We furnish utter variation of this book in txt, doc, DjVu, PDF, ePub formats. You may read Linear algebra strang 4th solution manual online or load. Besides, on our site you may read manuals and another artistic eBooks online, or load them as well. We will to draw on consideration that our site does not store the book itself, but we grant ref to the website whereat you may download or read online. So that if have must to load Linear algebra strang 4th solution manual linear-algebra-strang-4th-solution-manual.pdf pdf, in that case you come on to faithful website. We have Linear algebra strang 4th solution manual doc, ePub, DjVu, PDF, txt forms. We will be happy if you will be back to us again.introduction to linear algebra 4th edition gilbert - Home > Document results for 'introduction to linear algebra 4th edition gilbert strang pdf solution manual' Download solution manual for linear algebra and itscomplete solutions manual -introduction to linear algebra - Jun 25, 2013 Complete solutions manual-introduction to linear algebra Introduction to linear algebra 4th algebra 3ed gilbert strang solutions manual.18.06 fall 2014 - massachusetts institute of technology - Introduction to Linear Algebra, 4th edition. Gilbert Strang : Talking about linear algebra is healthy.solution manual for introduction to linear - Solution Manual for Introduction to Linear Algebra, Gilbert Strang s textbooks have changed the entire Solution Manual for Linear Algebra withlinear algebra strang 4th solution - - Student Solutions Manual for Strangs Linear Algebra and Its Applications 4th by Strang Strang 5 Star Book Review 7.5 kBlinear algebra - wikipedia, the free encyclopedia - linear algebra facilitates the solution of linear systems of differential equations. Strang, Gilbert (February Introduction to Linear Algebra (4th ed.),linear algebra gilbert strang 4th edition - Linear Algebra Gilbert Strang 4th Edition Solution Manual Truck Nozzle. Solution Manual For Linear Algebra And Its Applications 4th Edition By Gilbertinstructors solutions manual gilbert strang linear - Latest Instructors Solutions Manual Gilbert Strang Linear Algebra And Its Applications 4th Edition Updates..edition solutions 4th strang algebra linear - Sign up to download Linear algebra strang 4th solution manual. Date shared: Mar, 03 2015 | Download and Read Online Page 2linear algebra and its applications, 4th edition: gilbert - Linear Algebra and Its Applications, 4th Edition [Gilbert Strang] Student Solutions Manual for Strang's Linear Algebra and Its Applications, 4th Edition [solutions manual] [instructors] introduction to linear - INTRODUCTION. TO LINEAR ALGEBRA Third***************************************************.eduMassachusettsInstituteofTechnology 0495013250 - student solutions manual for strang's - Student Solutions Manual for Strang's Linear Algebra and Its Applications by Gilbert; BRAND NEW, SSM Linear Algebra and Apps 4e (4th Revised edition),introduction to linear algebra 4th solution | - Tricia Joy. Register; Terms Sponsored High Speed Downloads introduction to linear algebra 4th edition solution manual Introduction To Linear Algebra Gilbertgilbert strang introduction to linear algebra 4th - Gilbert Strang Introduction To Linear Algebra 4th Edition Solutions Manual Pdf downloads Linear Algebra Strang 4th Solution Manual. Linear Algebra Gilbert Strangstudent solutions manual for strang's linear - : Student Solutions Manual for Strang's Linear Algebra and Its Applications, 4thstudent solutions manual for linear algebra and its - Student Solutions Manual for Linear Algebra and Its Applications Linear Algebra and Its Applications, 4th Editionintroduction to linear algebra, 4th edition - mit mathematics - Introduction to Linear Algebra, 4th I hope this website will become a valuable resource for everyone learning and doing linear algebra. 1.1 Vectors and Linear linear algebra and its applications 4th edition textbook - Access Linear Algebra and Its Applications 4th Edition solutions now. Linear Algebra and Its Applications | 4th Edition. Solutions Manual; Scholarships;introduction to linear algebra 4th edition - Access Introduction to Linear Algebra 4th Edition solutions now. Our solutions are written by Chegg experts so you can be assured of the Solutions Manual;linear algebra strang solutions manual 4th - Tricia's Compilation for 'linear algebra strang solutions manual 4th instructor' Follow. solutions manual to Linear Algebra, 4th Filetype: Submitter:gilbert strang linear algebra 4th edition - Gilbert Strang Linear Algebra 4th Edition Solutions Truck Nozzle. GILBERT STRANG LINEAR ALGEBRA 4TH EDITION SOLUTIONS. DOWNLOAD: GILBERT STRANG LINEAR ALGEBRA 4TH EDITIONfree! solution manual of linear algebra by gilbert - Download solution manual of linear algebra by gilbert strang 4th edition ebooks and manuals at PdfDigest: For: Solution manual of linear algebra by gilber introduction to linear algebra 4th edition by - Introduction to Linear Algebra 4th Edition by Gilbert Strang fully written solutions / or book Introduction to Linear Algebra 4th Edition bylinear algebra and its applications, 4th edition - Renowned professor and author Gilbert Strang demonstrates that linear algebra is a Linear Algebra and Its Applications, Student Solutions Manualinstructor's solutions manual for strang's linear algebra and - schema:name " Instructor's solutions manual for Strang's Linear algebra and its applications, fourth edition "@en; schema:productID " 85780336" ;student solutions manual for strang 's linear algebra and its - Student Solutions Manual for Strang's Linear Algebra and Its Applications, 4th 4 edition Published October 1, 2005 byneed a solutions manual-- linear algebra and its - Oct 07, 2009 Need a solutions manual--Linear Algebra and Its Applications, 4th Ed, by Gilbert Strang?solutions manual instructors introduction to - Instructor S Solutions Manual For Strang S Linear Algebra And Its Applications rapidshare links Strang Introduction Linear Algebra 4th Edition Solution ManualRelated PDFs:1991 yamaha yz125 service manual, briggs and stratton 18 hp ic manuals, math foundations 11 study guide, solution guide management accounting 6e, e6b flight manual, 06 ktm 250 xcw manual, haas vf2 service manual, awwa manual m 51, kia rio car manual, isuzu fvr 1000 manual, hayward abg 100 manual, iq 2020 control box manual, junior maths 3 by a dasgupta manual, 61h booster relay manual, joseph topich chemistry solutionsmanual 6th edition, science a closer look pacing guide, wisconsin civil service exam study guide maintenance, honda civic 1995 1996 1997 98 1999 workshop manual download, principles of macroeconomics 5th edition study guide, owners manual for mini chopper motorcycle, harley fxwg manual, ford transit mini bus manual, 97 cavalier haynes repair manual, peugeot 505 workshop manual, golf manual derkeiler com, repair manual kawasaki ninja 250r, nrx 1800 service manual, bait of satan leaders guide, buick lacrosse manual, does northstar study guide work, salvation army pricing guide, d3306 caterpillar operation manual, ford expedition factory service manual, manual for suzuki rm85, navigation manual 2015 crv, vw golf v5 manual, 1999 kawasaki nomad manual, mercedes c 180 workshop manual, honda crv 2015 factory manual, colt 1903 pocket hammerless manual。
ICEM中文版Tutorial_Manual

由yzmylh提供,版权所有……Ansys icemcfd 5.1 tutorial部分内容粗略翻译及理解,水平有限,如有问题或建议请联系liqingliang@,不胜感激。
感谢redhong等众多网友提供的资料和帮助,本人正在学习cfx,希望大家多多交流。
ANSYS ICEMCFD 5.1使用手册1. ANSYS ICEMCFD图形用户界面ANSYS ICEMCFD网格编辑器的标准化图形用户界面,提供了一个完善的划分和编辑数值计算网格的环境。
另外,自从ANSYS ICEM CFD 将相应的CAD模型(同样可以生成和编辑)与网格划分链接起来以后,网格编辑器允许用户在修改CAD模型后快速再生成新的网格。
对于为一个模型生成的网格可以被再次链接到一个新的CAD模型上,节约了重新划分网格的时间。
网格编辑器界面包括三个窗口:ANSYS ICEM CFD 主窗口模型的树状目录ANSYS ICEM CFD 信息窗口1.1:ANSYS ICEM CFD 主窗口除了图形显示区,在它的上部设置了一排按钮提供操作菜单,这些菜单包括:几何,网格,块,网格编辑,输出和post processing工具。
窗口的右上角有一串功能菜单,它们与以上这些菜单的选择无关。
文件:文件菜单提供许多与文件管理相关的功能,如:打开文件、保存文件、合并和输入几何模型、存档工程,这些功能方便了管理ANSYS ICEM CFD工程。
在这个菜单里有用的功能包括:新建工程、打开工程、保存工程、另存为、关闭工程、改变工作地址、几何菜单、网格属性、参数、结果、输入几何模型、输入网、输出几何模型和退出。
带有特殊标记的功能按钮包含有子菜单,可以通过点击看到。
编辑:菜单包括回退、前进、命令行、网格转换小面结构、小面结构转化为网格、结构化模型面。
视图:菜单包括合适窗口、放大、俯视、仰视、左视、右视、前视、后视、等角视、视图控制、保存视图、背景设置、镜像与复制、注释、加标记、清除标记、网格截面剖视。
MIT麻省理工学院 算法导论公开课 Problem Set 4 solution

Introduction to Algorithms October 29, 2005 Massachusetts Institute of Technology 6.046J/18.410J Professors Erik D. Demaine and Charles E. Leiserson Handout 18Problem Set 4 SolutionsProblem 4-1. TreapsIf we insert a set of n items into a binary search tree using T REE-I NSERT, the resulting tree may be horribly unbalanced. As we saw in class, however, we expect randomly built binary search trees to be balanced. (Precisely, a randomly built binary search tree has expected height O(lg n).) Therefore, if we want to build an expected balanced tree for a fixed set of items, we could randomly permute the items and then insert them in that order into the tree.What if we do not have all the items at once? If we receive the items one at a time, can we still randomly build a binary search tree out of them?We will examine a data structure that answers this question in the affirmative. A treap is a binary search tree with a modified way of ordering the nodes. Figure 1 shows an example of a treap. As usual, each item x in the tree has a key key[x]. In addition, we assign priority[x], which is a random number chosen independently for each x. We assume that all priorities are distinct and also that all keys are distinct. The nodes of the treap are ordered so that (1) the keys obey the binary-search-tree property and (2) the priorities obey the min-heap order property. In other words,•if v is a left child of u, then key[v]<key[u];•if v is a right child of u, then key[v]>key[u]; and•if v is a child of u, then priority(v)>priority(u).(This combination of properties is why the tree is called a “treap”: it has features of both a binary search tree and a heap.)Figure 1: A treap. Each node x is labeled with key[x]:p riority[x]. For example, the root has key G and priority 4.It helps to think of treaps in the following way. Suppose that we insert nodes x1,x2,...,x n, each with an associated key, into a treap in arbitrary order. Then the resulting treap is the tree that wouldhave been formed if the nodes had been inserted into a normal binary search tree in the order given by their (randomly chosen) priorities. In other words, priority[x i]<priority[x j]means that x i is effectively inserted before x j.(a) Given a set of nodes x1,x2,...,x n with keys and priorities all distinct, show that thereis a unique treap with these nodes.Solution:Prove by induction on the number of nodes in the tree. The base case is a tree withzero nodes, which is trivially unique. Assume for induction that treaps with k−1orfewer nodes are unique. We prove that a treap with k nodes is unique. In this treap, theitem x with minimum priority must be at the root. The left subtree has items with keys<key[x]and the right subtree has items with keys >key[x]. This uniquely defines theroot and both subtrees of the root. Each subtree is a treap of size ≤k−1, so they areunique by induction.Alternatively, one can also prove this by considering a treap in which nodes are inserted in order of their priority. Assume for induction that the treap with the k−1nodes with smallest priority is unique. For k=0t his is trivially true. Now considerthe treap with the k nodes with smallest priority. Since we know that the structureof a treap is the same as the structure of a binary search tree in which the keys areinserted in increasing priority order, the treap with the k nodes with smallest priorityis the same as the treap with the k−1nodes with smallest priority after inserting thek-th node. Since BST insert is a deterministic algorithm, there is only one place thek-th node could be inserted. Therefore the treap with k nodes is also unique, provingthe inductive hypothesis.(b) Show that the expected height of a treap is O(lg n), and hence the expected time tosearch for a value in the treap is O(lg n).Solution: The idea is to realize that a treap on n nodes is equivalent to a randomlybuilt binary search tree on n nodes. Recall that assigning priorities to nodes as theyare inserted into the treap is the same as inserting the n nodes in the increasing orderdefined by their priorities. So if we assign the priorities randomly, we get a randomorder of n priorities, which is the same as a random permutation of the n inputs, sowe can view this as inserting the n items in random order.The time to search for an item is O(h)where h is the height of the tree. As we saw inlecture, E[h]=O(lg n). (The expectation is taken over permutations of the n nodes,i.e., the random choices of the priorities.)Let us see how to insert a new node x into an existing treap. The first thing we do is assign x a random priority priority[x]. Then we call the insertion algorithm, which we call T REAP-I NSERT, whose operation is illustrated in Figure 2.(e) (f)Figure 2: Operation of T REAP-I NSERT. As in Figure 1, each node x is labeled with key[x]: priority[x]. (a) Original treap prior to insertion. (b) The treap after inserting a node with key C and priority 25. (c)–(d) Intermediate stages when inserting a node with key D and priority 9.(e) The treap after insertion of parts (c) and (d) is done. (f) The treap after inserting a node with key F and priority 2.(c) Explain how T REAP-I NSERT works. Explain the idea in English and give pseudocode.(Hint: Execute the usual binary search tree insert and then perform rotations to restorethe min-heap order property.)Solution: The hint gives the idea: do the usual binary search tree insert and thenperform rotations to restore the min-heap order property.T REAP-I NSERT (T,x)inserts x into the treap T(by modifying T). It requires that xhas defined key and priority values. We have used the subroutines T REE-I NSERT,R IGHT-R OTATE, and R IGHT-R OTATE as defined in CLRS.T REAP-I NSERT (T,x)1T REE-I NSERT (T,x)2 while x =root[T]and priority[x]<priority[p[x]]3 do if x=left[p[x]]4 then R IGHT-R OTATE (T,p[x])5 else L EFT-R OTATE (T,p[x])Note that parent pointers simplify the code but are not necessary. Since we only needto know the parent of each node on the path from the root to x(after the call toT REE-I NSERT), we can keep track of these ourselves.(d) Show that the expected running time of T REAP-I NSERT is O(lg n). Solution:T REAP-I NSERT first inserts an item in the tree using the normal binary search treeinsert and then performs a number of rotations to restore the min-heap property.The normal binary-search-tree insertion algorithm T REE-I NSERT always places thenew item at a new leaf of tree. Therefore the time to insert an item into a treap isproportional to the height of a randomly built binary search tree, which as we saw inlecture is O(lg n)in expectation.The maximum number of rotations occurs when the new item receives a priority lessthan all priorities in the tree. In this case it needs to be rotated from a leaf to the root.An upper bound on the number of rotations is therefore the height of a randomly builtbinary search tree, which is O(lg n)in expectation. (We will see that this is a fairlyloose bound.) Because each rotation take constant time, the expected time to rotate isO(lg n).Thus the expected running time of T REAP-I NSERT is O(lg n+lg n)=O(lg n).T REAP-I NSERT performs a search and then a sequence of rotations. Although searching and rotating have the same asymptotic running time, they have different costs in practice. A search reads information from the treap without modifying it, while a rotation changes parent and child pointers within the treap. On most computers, read operations are much faster than write operations. Thus we would like T REAP-I NSERT to perform few rotations. We will show that the expected number of rotations performed is bounded by a constant (in fact, less than 2)!(a) (b)Figure 3: Spines of a binary search tree. The left spine is shaded in (a), and the right spine is shaded in (b).In order to show this property, we need some definitions, illustrated in Figure 3. The left spine of a binary search tree T is the path which runs from the root to the item with the smallest key. In other words, the left spine is the maximal path from the root that consists only of left edges. Symmetrically, the right spine of T is the maximal path from the root consisting only of right edges. The length of a spine is the number of nodes it contains.(e) Consider the treap T immediately after x is inserted using T REAP-I NSERT. Let Cbe the length of the right spine of the left subtree of x. Let D be the length of theleft spine of the right subtree of x. Prove that the total number of rotations that wereperformed during the insertion of x is equal to C+D.Solution: Prove the claim by induction on the number of rotations performed. Thebase case is when x is the parent of y. Performing the rotation so that y is the new rootgives y exactly one child, so C+D=1.Assume for induction that the number of rotations k performed during the insertionof x equals C+D. The base case is when 0 rotations are necessary and x is insertedas a leaf. Then C+D=0. To prove the inductive step, we show that if after k−1rotations C+D=k−1, then after k rotations C+D=k. Draw a picture of aleft and right rotation and observe that C+D increases by 1 in each case. Let y bethe parent of x, and suppose x is a left child of y. After performing a right rotation, ybecomes the right child of x, and the previous right child of x becomes the left childof y. That is, the left spine of the right subtree of x before the rotation is tacked onto y, so the length of that spine increases by one. The left subtree of x is unaffectedby a right rotation. The case of a left rotation is symmetric. Therefore after one morerotation C+D increases by one and k=C+D, proving the inductive hypothesis.We will now calculate the expected values of C and D. For simplicity, we assume that the keys are 1,2,...,n. This assumption is without loss of generality because we only compare keys.�For two distinct nodes x and y , let k = key [x ]and i = key [y ], and define the indicator random variableX 1 if y is a node on the right spine of the left subtree of x (in T ),i,k =0 otherwise .(f) Show that X i,k =1if and only if (1) priority [y ]> priority [x ], (2) key [y ]< key [x ], and(3) for every z such that key [y ]< key [z ]< key [x ],we have p riority [y ]< priority [z ].Solution:To prove this statement, we must prove both directions of the “if and only if”. Firstwe prove the “if” direction. We prove that if (1) priority [y ]> priority [x ], (2) key [y ]<key [x ], and (3) for every z such that key [y ]< key [z ]< key [x ]are true, priority [y ]< priority [z ], then X i,k =1. We prove this by contradiction. Assume that X i,k =0. That is, assume that y is not on the right spine of the left subtree of x . We show thatthis leads to a contradiction. If y is not on the right spine of the left subtree of x ,it could be in one of three places:1. Suppose y is in the right subtree of x . This contradicts condition (2) becausekey [y ]< key [x ].2. Suppose y is not in one of the subtrees of x . Then x and y must share somecommon ancestor z . Since key [y ]< key [x ], we know that y is in the left subtreeof z and x is in the right subtree of z and key [y ]< key [z ] < key [x ]. Since y isbelow z in the tree, priority [z ]< priority [x ]and priority [z ]< priority [y ]. Thiscontradicts condition (3).3. Suppose that y is in the left subtree of x but not on the right spine of the leftsubtree of x . This implies that there exists some ancestor z of y in the left subtreeof x such that y is in the left subtree of z . Hence key [y ]< key [z ]< key [x ]. Sincez is an ancestor of y , priority [z ]< priority [y ], which contradicts condition (3).All possible cases lead to contradictions, and so X i,k =1.Now for the “only if” part. We prove that if X i,k =1, then statements (1), (2), and (3) are true. If X i,k =1, then y is in the right spine of the left subtree of x . Since y is ina subtree of x , y must have been inserted after x ,so p riority [y ]> priority [x ], proving(1). Since y is in the left subtree of x , key [y ]< key [x ], proving (2). We prove (3) by contradiction: suppose that X i,k =1and there exists a z such that key [y ]< key [z ]< key [x ]and priority [z ]< priority [y ]. In other words, z was inserted before y . There arethree possible cases that satisfy the condition key [z ]< key [x ]:1. Suppose z is in the right spine of the left subtree of x .For y to be inserted into theright spine of the left subtree of x , it will have to be inserted into the right subtreeof z . Since key [y ]< key [z ], this leads to a contradiction.2. Suppose z is in the left subtree of x but not in the right spine. This implies thatz is in the left subtree of some node z in the right spine of x . Therefore for y tobe inserted into the right spine of the left subtree of x , it must be inserted into theright subtree of z . This leads to a contradiction by reasoning similar to case 1.3. Suppose that z is not in one of the subtrees of x . Then z and x have a commonancestor z such that z is in the left subtree of z and x is in the right subtree of x .This implies key [z ] < key [z ] < key [x ]. Since key [y ]< key [z ]< key [z ], y cannotbe inserted into the right subtree of z . Therefore it cannot be inserted in a subtreeof x , which is a contradiction.Therefore there can be no z such that key [y ] < key [z ] < key [x ] and priority [z ] < priority [y ]. This proves statement (3). We have proven both the “if” and “only if” directions, proving the claim.(g) Show that(k − i − 1)! 1 Pr {X i,k =1} == . (k − i +1)! (k − i +1)(k − i )Solution: We showed in the previous part that X i,k =1if and only if the priorities of the items between y and x are ordered in a certain way. Since all orderings are equally likely, to calculate the probability we count the number of permutations of priorities that obey this order and divide by the number of total number of priority permutations. We proved in (e) that whether or not X i,k =1depends only on the relative ordering of the priorities of y , x , and all z such that key [y ] < key [z ] < key [x ]. Since we assumed that the keys of the items come from {1,...,n }, the keys of the items in question are i,i +1,i +2,...,k − 1,k . There are (k − i +1)!permutations of the priorities of these items. Of these permutations, the ones for which X i,k =1are those where i has minimum priority, k has the second smallest priority, and the priorities of the remaining k − i − 1 items follow in any order. There are (k − i − 1)! of these permutations. Thus the probability that we get a “bad” order is (k −i −1)!/(k −i +1)!= 1/(k − i )(k − i +1).(h) Show thatk −1 � 1 1 E [C ]= =1− . j (j +1)k j =1 Solution:X For a node x with key k , E [C ]is the expected number of nodes in the right spine of the left subtree of x . This equals the sum of the expected value of the random variables i,k for all i in the tree. Since X i,k =0for all nodes i ≥ k , we only need to consider i <k .� � � k −1 �k −1 � E [C ]=E [X i,k ]=E X i,k i =1 i =1 k −1 =Pr {X i,k =1}i =1 k −1 � 1 =(k − i )(k − i +1) i =1 k −1 � 1= j (j +1)j =1 1To simplify this sum, observe that j (j 1+1) = j +1−j = − 1 . Therefore the sumj (j +1) j j +1 telescopes and we have 1 E [C ]=1− . kIf you didn’t see this, you could have proven that the equationk −1 � 1 1 =1− j (j +1)k j =1 holds by induction on k . In the proving the inductive hypothesis you might have 11discovered 1 − = .j j +1 j (j +1)(i) Use a symmetry argument to show that1 E [D ]=1− . n − k +1Solution: The idea is to consider the treap produced if the ordering relationship amongthe keys is reversed. That is, for all items x , leave priority [x ]unchanged but replacekey [x ]with n − key [x ]+1.Let T be the binary tree we get when inserting the items (in increasing order of priority) using the original keys. Once we remap the keys and insert them into a newbinary search tree, we get a tree T whose shape is the mirror image of the shape ofT . (reflected left to right). Consider the item x with key k in T and therefore has key n − k +1 i n T . The length of the left spine of x ’s right subtree in T has becomethe length of the right spine of x ’s left subtree in T . We know by part (g) that the expected length of the right spine of a left subtree of a node y is 1− 1/idkey [y ],so the expected length of the right spine of the left subtree of x in T is 1− 1/(n − k +1), which means that 1 E [D ]=1− . n − k +1(j) Conclude that the expected number of rotations performed when inserting a node into a treap is less than 2.Solution:11 E [number of rotations ]= E [C +D ]=E [C ]+E [D ]=1− +1− k n − k +1 ≤ 1+1=2. Problem 4-2. Being balancedCall a family of trees balanced if every tree in the family has height O (lg n ), where n is the number of nodes in the tree. (Recall that the height of a tree is the maximum number of edges along any path from the root of the tree to a leaf of the tree. In particular, the height of a tree with just one node is 0.)For each property below, determine whether the family of binary trees satisfying that property is balanced. If you answer is “no”, provide a counterexample. If your answer is “yes”, give a proof (hint: it should be a proof by induction). Remember that being balanced is an asymptotic property, so your counterexamples must specify an infinite set of trees in the family, not just one tree. (a) Every node of the tree is either a leaf or it has two children.Solution: No. Counterexample is a right chain, with each node having a leaf hanging off to the left(b) The size of each subtree can be written as 2k − 1, where k is an integer (k is not the same for each subtree).Solution: Yes.Consider any subtree with root r . We know from the condition that the size of this subtree is 2k 1 − 1. We also know that the size of the subtree rooted at the left child of r is 2k 2 − 1, and the size of the subtree rooted at the right child of r is 2k 3 − 1. But the size of the subtree at r is simply the node r together with the nodes in the left and right subtrees. This leads to the equation 2k 1 − 1=1+(2k 2 − 1)+(2k 3 − 1),or 2k 1 =2k 2 +2k 3. Now we show that k 2 =k 3. This is easy to see if you consider the binary representations of k 1, k 2, and k 3.Otherwise, if we assume WLOG that k 2 ≤ k 3, then we have 2k 1−k 2 =1+2k 3−k 2. 2Now, the only pair of integer powers of 2 that could satisfy this equation are 21 and 0 . Thus k 3 − k 2 =0,or k 2 =k 3, and the left and right subtrees of r have an equal number of nodes. It follows that the tree is perfectly balanced.(c) There is a constant c>0such that, for each node of the tree, the size of the smallerchild subtree of this node is at least c times the size of the larger child subtree.Solution:Yes1. The proof is by induction. Assume that the two subtrees of x with n nodes in itssubtree has two children y and z with subtree sizes n1 and n2. By inductive hypothesis,the height of y’s subtree is at most d lg n1 and the height of z’s subtree is at most d lg n2for some constant d. We now prove that the height of x’s subtree is at most d lg n.Assume wlog that n1 ≥n2. Therefore, by the problem statement, we have n2 ≥cn1.Therefore, we have n=n1 +n2 +1≥(1+c)n1 +1≥(1+c)n1 and the height hof x’s subtree is d lg n1 +1≤d lg(n/(c+1))+1≤d lg n−d lg(1+c)+1≤d lg nif d lg(1+c)≥1. Therefore, for sufficiently large d, the height of a tree with n nodesis at most d lg n.(d) There is a constant c such that, for each node of the tree, the heights of its childrensubtrees differ by at most c.Solution: Yes1. Let n(h)be the minimum number of nodes that a tree of height h thatsatisfies the stated property can have. We show by induction that n(h)≥(1+α)h−1,for some constant 0<α≤1. We can then conclude that for a tree with n nodes,h≤log1+α(n+1)=O(lg n).For the base case, a subtree of height 0has a single node, and 1≥(1+α)0 −1forany constant α≤1.In the inductive step, assume for all trees of height k<h, that the n(k)≥(1+α)k−1.Now consider a tree of height h, and look at its two subtrees. We know one subtreemust have height h−1, and the other must have height at least h−1−c. Therefore,we known(h)≥n(h−1)+n(h−1−c)+1.Using the inductive hypothesis, we getn(h) ≥(1+α)h−1 −1+(1+α)h−1−c−1+1≥2(1+α)h−1−c−1.Suppose we picked αsmall enough so that (1+α)<21/(c+1). Then (1+α)c+1 <2.Therefore, we getn(h)≥2(1+α)h−1−c−1≥(1+α)h−1.1Note that in this problem we assume that a nil pointer is a subtree of size 0, and so a node with only one child has two subtrees, one of which has size 0. If you assume that a node with only one child has only one subtree, then the answer to this problem part is “no”.�11Handout18: Problem Set4SolutionsTherefore, we satisfy the inductive hypothesis.Note that if we plug this value for αback into h≤log1+α(n+1),we getlg(n+1)h≤≤(c+1)l g(n+1).lg(1+2c+1)(e) The average depth of a node is O(lg n). (Recall that the depth of a node x is thenumber of edges along the path from the root of the tree to x.)Solution: No.√Consider a tree with n−n nodes organized as a complete binary tree, and the other √ √n nodes sticking out as a chain of length n from the balanced tree. The height of√√√the tree is lg(n−n)+n=Ω(n), while the average depth of a node is at most�√√ √(1/n)n·(n+lg n)+(n−n)·lg n√√=(1/n)(n+n lg n+n lg n−nlgn)=(1/n)(n+n lg n)=O(lg n)√Thus, we have a tree with average node depth O(lg n), but height Ω(n).。
fluent范例

Select the right straight edge, change the type pull-down menu to Outflow, name it “outlet” and click Apply.
7
Channel Flow
Boundary Conditions
Now define the boundary conditions for the problem.
Since Fluent version 6 is going to be used, select
Solver > Fluent5/6
Channel Flow
2D Curved Flow
In this tutorial, GAMBIT will be used to create and mesh the flow field geometry for the problem. Once this is complete, FLUENT will be used to solve for the pressure field everywhere in the domain and plot the pressure distribution across the pipe. This tutorial will provide experience in solving 2D flows and creating plots of the results. The methods expressed in these tutorials represent just one approach to modeling, constraining and solving 2D problems. Our goal is the education of students in the use of CAx tools for modeling, constraining and solving fluids application problems. Other techniques and methods will be used and introduced in subsequent tutorials.
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
1.For each of the following pairs of goods, which good would you expect to have more elasticdemand and why?a.required textbooks or mystery novelsb.Beethoven recordings or classical music recordings in generalc.subway rides during the next 6 months or subway rides during the next 5 yearsd.root beer or water2.Suppose that business travellers and vacationers have the following demand for airlinetickets from New York to Boston:Price Demand (business travellers) Demand (vacationers)150 2,100 1,000200 2,000 800250 1,900 600300 1,800 400a. As the price of tickets rises from $200 to $250, what is the price elasticity of demand for (i)business travellers and (ii) vacationers? (Use the midpoint method in your calculations.)b. Why might vacationers have a different elasticity from business travellers?3.Suppose the price of elasticity of gasoline is 0.3 in the short run and 0.9 in the long run.a.If the price of a gallon of gasoline falls from $2.50 to $2.25, what happens to thequantity of gasoline demanded in the short run? In the long run? (Use the midpointformula in your calculations.)b.Why might the elasticity of demand for gasoline depend on time horizon?4.Consider public policy aimed at smoking.a.Studies indicate that the price elasticity of demand for cigarettes is about 0.4. If apack of cigarettes costs $2 and the government wants to reduce smoking by 20%, byhow much should it increase the price?b.If the government permanently increases the price of cigarettes, will the policy havea larger effect on smoking 1 year from now or 5 years from now?c.Studies also find that teenagers have higher price elasticity than do adults. Whymight this be true?5.You are the curator of a museum. The museum is running short of funds, so you decide toincrease revenue. Should you increase or decrease the price of admissions? Explain.6.Corn has an elastic demand, and gasoline has an inelastic demand. Suppose that thegovernment places a tax on each product that lowers the supply of each product by half (that is, the quantity supplied at each price is 50 percent of what it was).a.What happens to the equilibrium price and quantity in each market?b.Which product experiences a larger change in price?c.Which product experiences a larger change in quantity?d.What happens to total consumer spending on each product?1.a. Mystery novels have more elastic demand than required textbooks, because mystery novels haveclose substitutes and are a luxury good, while required textbooks are a necessity with no close substitutes. If the price of mystery novels were to rise, readers could substitute other types of novels, or buy fewer novels altogether. But if the price of required textbooks were to rise, students would have little choice but to pay the higher price. Thus, the quantity demanded of required textbooks is less responsive to price than the quantity demanded of mystery novels.b. Beethoven recordings have more elastic demand than classical music recordings in general.Beethoven recordings are a narrower market than classical music recordings, so it is easy to find close substitutes for them. If the price of Beethoven recordings were to rise, people could substitute other classical recordings, like Mozart. But if the price of all classical recordings were to rise, substitution would be more difficult (a transition from classical music to rap is unlikely!). Thus, the quantity demanded of classical recordings is less responsive to price than the quantity demanded of Beethoven recordings.c. Subway rides during the next five years have more elastic demand than subway rides during the nextsix months. Goods have a more elastic demand over longer time horizons. If the fare for a subway ride was to rise temporarily, consumers could not switch to other forms of transportation without great expense or great inconvenience. But if the fare for a subway ride was to remain high for a long time, people would gradually switch to alternative forms of transportation. As a result, the quantity demanded of subway rides during the next six months will be less responsive to changes in the price than the quantity demanded of subway rides during the next five years.d. Root beer has more elastic demand than water. Root beer is a luxury with close substitutes, whilewater is a necessity with no close substitutes. If the price of water were to rise, consumers have little choice but to pay the higher price. But if the price of root beer were to rise, consumers could easily switch to other sodas. So the quantity demanded of root beer is more responsive to changes in price than the quantity demanded of water.2.a. For business travelers, the price elasticity of demand when the price of tickets rises from$200 to $250 is [(2,000 – 1,900)/1,950]/[(250 – 200)/225] = 0.05/0.22 = 0.23.For vacationers, the price elasticity of demand when the price of tickets rises from $200 to $250 is [(800 – 600)/700] / [(250 – 200)/225] = 0.29/0.22 = 1.32.b. The price elasticity of demand for vacationers is higher than the elasticity for business travelersbecause vacationers can choose more easily a different mode of transportation (like driving or taking the train). Business travelers are less likely to do so because time is more important to them and their schedules are less adaptable.3.a. The percentage change in price is equal to (2.25 – 2.50)/2.375 = -0.105 = -10.5%. Ifthe price elasticity of demand is 0.3, quantity demanded will rise by 3.15% in the shortrun [0.3 × 0.105]. If the price elasticity of demand is 0.9, quantity demanded will rise by9.45% in the long run [0.9 × 0.105].b. Over time, consumers can make adjustments to their lives such as buying a more fuel efficientvehicle, using public transportation, carpooling, or reducing the amount ofdriving they do. Thus, they can respond more easily to the change in the price ofgasoline in the long run than in the short run.4.a. With a price elasticity of demand of 0.4, reducing the quantity demanded of cigarettes by 20%requires a 50% increase in price, because 20/50 = 0.4. With the price of cigarettes currently $2, this would require an increase in the price to $3.33 a pack using the midpoint method (note that ($3.33 –$2)/$2.67 = .50).b. The policy will have a larger effect five years from now than it does one year from now. Theelasticity is larger in the long run, because it may take some time for people to reduce their cigarette usage. The habit of smoking is hard to break in the short run.c. Because teenagers do not have as much income as adults, they are likely to have a higher priceelasticity of demand. Also, adults are more likely to be addicted to cigarettes, making it more difficult to reduce their quantity demanded in response to a higher price.5.In order to determine whether you should raise or lower the price of admissions, you need to know ifthe demand is elastic or inelastic. If demand is elastic, a decline in the price of admissions will increase total revenue. If demand is inelastic, an increase in the price of admissions will cause total revenue to rise.6.a. As Figure 1 shows, the decrease in supply increases the equilibrium price and decreases theequilibrium quantity in both markets. Please note that, as a result of the tax that reduces thequantity supply by 50%, the quantity supplied for S1(before the tax) is always half of thequantity supplied for S2 (after the tax). For instance, for the graph regarding gasoline, for S1 aprice of 4 (6) is associated with a quantity demanded of 4 (10) for S1 and a quantity demandedof 2 (5) for S2.b.In the market for gasoline (with inelastic demand), the decrease in supply leads to a relativelylarge rise in the equilibrium price and a small decrease in the equilibrium quantity.Figure 1Price of gasoline Price of cornc. In the market for corn (with elastic demand), the decrease in supply leads to a relatively largedecrease in the equilibrium quantity and a small rise in the equilibrium price.d. Because demand is inelastic in the market for gasoline, the percentage decrease in quantity will belower than the percentage rise in price; thus, total consumer spending will rise. Because demand iselastic in the market for corn, the percentage decrease in quantity will be greater than the percentage rise in price, so total consumer spending will decline.。
