2004-A-Input-to-state stability of networked control systems

合集下载

Stability and stabilization of nonlinear system-Chapter 8非线性系统稳定性和稳定化

Chapter8Open ProblemsIn this chapter,we would like to give a list of open and unanswered problems in Mathematical Control Theory.The solutions of these open problems will be very important for the development of modern nonlinear control theory.Expectedly novel mathematical analysis and synthesis tools need to be developed to address these challenging problems.The interested reader should also consult the book[3]for other signiﬁcant and important open problems in Mathematical Control Theory.Open Problem#1Under what conditions WIOS implies IOS?A qualitative characterization of the IOS property for abstract control systems as discussed in this book has not been available yet.For systems described by ODEs, many qualitative characterizations of the ISS and IOS properties are provided in [21–23].Moreover,Theorem4.1in Chap.4gives a complete qualitative character-ization of the WIOS property:“0-GAOS”+“RFC”+“the continuity with respect to initial conditions and external inputs”implies WIOSA similar qualitative characterization for the IOS property in a general context of abstract dynamical systems as discussed in this book will be very important for control designs and applications.Open Problem#2Development of small-gain techniques for dynamical systems described by Partial Differential Equations(PDEs).Small-gain results have been well studied forﬁnite-dimensional nonlinear sys-tems described by ordinary differential,or difference,equations(see,e.g.,[8–10] and references therein).However,as of today,there is little research devoted to the development of small-gain techniques for nonlinear systems described by Partial Differential Equations(PDEs).We believe that the small-gain results provided in the present book(Theorems5.1and5.2in Chap.5)will pave the road for the appli-cation of small-gain results to systems described by PDEs.I.Karafyllis,Z.-P.Jiang,Stability and Stabilization of Nonlinear Systems,381 Communications and Control Engineering,DOI10.1007/978-0-85729-513-2_8,©Springer-Verlag London Limited2011Open Problem#3Formulas for the Coron–Rosier methodology.Theorem6.1in Chap.6is an existence-type result.Although its proof is con-structive,it cannot be easily applied for feedback design purposes.The creation of formulas for the Coron–Rosier approach will be very signiﬁcant for control pur-poses,since the Coron–Rosier approach can allow nonconvex control sets and does not require additional properties for the Control Lyapunov Function.The signiﬁ-cance of the solution of this open problem is also noted in[5].Open Problem#4When is a nonlinear,time-varying,time-delay system stabiliz-able?We have recently provided a positive answer to the above question when the sys-tem only involves state-delay[13].A complete answer to the question of when the nonlinear time-varying system with both state and input delays is stabilizable re-mains open and requires deeper investigation.Nonetheless,it should be mentioned that sufﬁcient,but not necessary,conditions for the solution of the stabilization prob-lem with input delays are proposed in the recent work of Krsti´c[14–16](also see [11]).To our knowledge,a necessary and sufﬁcient condition for stabilizability is missing even for linear time-varying systems with input delays.Open Problem#5Application of small-gain results for distributed feedback design of large-scale nonlinear systems.Large-scale systems are abundant in variousﬁelds of science and engineering and have gained increasing attention due to emerging engineering and biomedical applications.Examples of these applications are from smart grids with green and re-newable energy sources,modern transportation networks,and biological networks. There has been some success with the use of decentralized control strategy for both linear and nonlinear large-scale systems;see[7,19]and many references therein. Clearly more remains to be accomplished in this excitingﬁeld.We feel that small-gain is a very appropriate tool for addressing some of these modern-day challenges. The small-gain results of the present book(Theorems5.1and5.2in Chap.5)make a preliminary step forward toward studying some complex large-scale systems be-yond the past literature of decentralized systems and control.Open Problem#6Extension of the discretization approach for autonomous sys-tems.The discretization approach for Lyapunov functionals was described in Chap.2 (Propositions2.4and2.5).However,as remarked in Chap.2,the discretization ap-proach requires good knowledge of some approximation of the solution map,and its use has been restricted to time-varying systems with special structure(see[1,17, 18]).An extension of the discretization approach for autonomous systems wouldbe an important contribution in stability theory because such a result would al-low the use of positive deﬁnite functions with non sign-deﬁnite derivative.The re-quired extension of the discretization approach must utilize appropriate differential inequalities in the same spirit as the classical Lyapunov’s approach(without requir-ing knowledge of the solution map or a system with special structure).The recent work in[12]is an attempt in this research direction(see also references therein). However,the problem is still completely“untouched.”Open Problem#7Application of feedback design methodologies to other mathe-matical problems.In this book,we have seen the applications of certain tools of modern nonlinear control theory to problems arising from mathematics and economics.Particularly, we have seen•applications of small-gain results to game theory(see Sect.5.5in Chap.5),•applications to numerical analysis(see Sect.7.3).We believe that feedback design methodologies can be applied with success to other areas of mathematical sciences.Fixed Point Theory(see[6])and Optimization Theory can be beneﬁted by the application of certain tools of modern nonlinear con-trol theory.Corollary5.4in Chap.5already shows that small-gain results can have serious consequences in Fixed Point Theory.Further connections between Fixed Point Theory and Stability Theory are provided by the work of Burton(see[4]and references therein)but are in the opposite direction from what we propose,that is, the work of Burton applies results from Fixed Point Theory to Stability Theory.The efforts for the solution of problems in Game Theory,Numerical Analysis, Fixed Point Theory,and Optimization Theory will necessarily demand the creation of novel results in stability theory and feedback stabilization theory.Therefore,the application of modern nonlinear control theory to other areas of applied mathe-matics will result to a“knowledge feedback mechanism”between Mathematical Control Theory and other areas in mathematics!Open Problem#8Integral input-to-state stability(for short,iISS)in complex dy-namical systems.The external stability results of this book are exclusively targeted at extensions of Sontag’s ISS property and its variants to a very general context of complex dynamic systems.That is,we want to address a wide class of dynamical systems which may not satisfy the semigroup property,motivated by important examples of hybrid sys-tems,switched systems,and time-delay systems.It remains an open and important, but interesting,question to know how much we could do with the iISS property introduced in[2,20].References1.Aeyels,D.,Peuteman,J.:A new asymptotic stability criterion for nonlinear time-variant dif-ferential equations.IEEE Transactions on Automatic Control43(7),968–971(1998)2.Angeli,D.,Sontag,E.D.,Wang,Y.:A characterization of integral input-to-state stability.IEEETransactions on Automatic Control45(6),1082–1097(2000)3.Blondel,V.D.,Megretski,A.(eds.):Unsolved Problems in Mathematical Systems and ControlTheory.Princeton University Press,Princeton(2004)4.Burton,T.A.:Stability by Fixed Point Theory for Functional Differential Equations.Dover,Mineola(2006)5.Coron,J.-M.:Control and Nonlinearity.Mathematical Surveys and Monographs,vol.136.AMS,Providence(2007)6.Granas,A.,Dugundji,J.:Fixed Point Theory.Springer Monographs in Mathematics.Springer,New York(2003)7.Jiang,Z.P.:Decentralized control for large-scale nonlinear systems:A review of recent results.Dynamics of Continuous,Discrete and Impulsive Systems11,537–552(2004).Special Issue in honor of Prof.Siljak’s70th birthday8.Jiang,Z.P.:Control of interconnected nonlinear systems:a small-gain viewpoint.In:deQueiroz,M.,Malisoff,M.,Wolenski,P.(eds.)Optimal Control,Stabilization,and Nonsmooth Analysis.Lecture Notes in Control and Information Sciences,vol.301,pp.183–195.Springer, Heidelberg(2004)9.Jiang,Z.P.,Mareels,I.M.Y.:A small-gain control method for nonlinear cascaded systems withdynamic uncertainties.IEEE Transactions on Automatic Control42,292–308(1997)10.Jiang,Z.P.,Teel,A.,Praly,L.:Small-gain theorems for ISS systems and applications.Mathe-matics of Control,Signals,and Systems7,95–120(1994)11.Karafyllis,I.:Stabilization by means of approximate predictors for systems with delayed in-put.To appear in SIAM Journal on Control and Optimization12.Karafyllis,I.:Can we prove stability by using a positive deﬁnite function with non sign-deﬁnite derivative?Submitted to Nonlinear Analysis Theory,Methods and Applications 13.Karafyllis,I.,Jiang,Z.P.:Necessary and sufﬁcient Lyapunov-like conditions for robustnonlinear stabilization.ESAIM:Control,Optimization and Calculus of Variations(2009).doi:10.1051/cocv/2009029,pp.1–42,August200914.Krsti´c,M.:Delay Compensation for Nonlinear,Adaptive,and PDE Systems.Systems&Con-trol:Foundations&Applications.Birkhäuser,Boston(2009)15.Krsti´c,M.:Input delay compensation for forward complete and feedforward nonlinear sys-tems.IEEE Transactions on Automatic Control55,287–303(2010)16.Krsti´c,M.:Lyapunov stability of linear predictor feedback for time-varying input delay.IEEETransactions on Automatic Control55,554–559(2010)17.Peuteman,J.,Aeyels,D.:Exponential stability of slowly time-varying nonlinear systems.Mathematics of Control,Signals and Systems15,42–70(2002)18.Peuteman,J.,Aeyels,D.:Exponential stability of nonlinear time-varying differential equationsand partial averaging.Mathematics of Control,Signals and Systems15,202–228(2002)19.Siljak,D.:Decentralized Control of Complex Systems.Academic Press,New York(1991)20.Sontag,E.D.:Comments on integral variants of ISS.Systems Control Letters3(1–2),93–100(1998)21.Sontag,E.D.,Wang,Y.:On characterizations of the input-to-state stability property.Systemsand Control Letters24,351–359(1995)22.Sontag,E.D.,Wang,Y.:New characterizations of the input-to-state stability.IEEE Transac-tions on Automatic Control41,1283–1294(1996)23.Sontag,E.D.,Wang,Y.:Lyapunov characterizations of input to output stability.SIAM Journalon Control and Optimization39,226–249(2001)。

AP2004

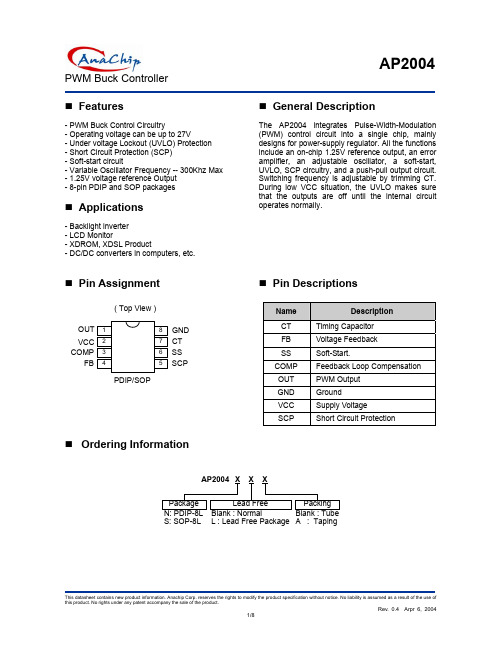
PWM Buck ControllerFeatures- PWM Buck Control Circuitry- Operating voltage can be up to 27V- Under voltage Lockout (UVLO) Protection - Short Circuit Protection (SCP) - Soft-start circuit- Variable Oscillator Frequency -- 300Khz Max - 1.25V voltage reference Output - 8-pin PDIP and SOP packagesApplications- Backlight inverter - LCD Monitor- XDROM, XDSL Product- DC/DC converters in computers, etc.General DescriptionThe AP2004 integrates Pulse-Width-Modulation (PWM) control circuit into a single chip, mainly designs for power-supply regulator. All the functions include an on-chip 1.25V reference output, an error amplifier, an adjustable oscillator, a soft-start, UVLO, SCP circuitry, and a push-pull output circuit. Switching frequency is adjustable by trimming CT. During low VCC situation, the UVLO makes sure that the outputs are off until the internal circuit operates normally.Pin AssignmentOUT GND SCPSS FBVCC CT COMP( Top View )PDIP/SOPPin DescriptionsNameDescriptionCT TimingCapacitor FB Voltage Feedback SS Soft-Start. COMPFeedback Loop CompensationOUT PWM OutputGND Ground VCC Supply Voltage SCP Short Circuit ProtectionOrdering InformationS: SOP-8LA : TapingL : Lead Free PackagePWM Buck ControllerBlock DiagramOUTFBVCCSCP CTCOMPGNDSSAbsolute Maximum RatingsSymbol ParameterRating Unit V CC Supply voltage 28 V V I Amplifier input voltage 20 V V O Collector output voltage V CC -1.0VVI SOURCE Source current 200 mA I SINK Sink current200 mA T OP Operating temperature range -20 to +85 o C T ST Storage temperature range-65 to +150o C T LEAD Lead temperature 1.6 mm(1/16 inch) from case for 10 seconds260oCPWM Buck ControllerRecommended Operating ConditionsSymbolParameterMin. Max. Unit V CC Supply voltage 3.6 27V V I Amplifier input voltage 1.05 1.45V V O Collector output voltage Vcc-1.5 VI FBCurrent into feedback terminal45 µA R F Feedback resistor 100 k Ω C T Timing capacitor 100 6800pF F OSC Oscillator frequency 10 300 KHz T OPOperating free-air temperature-2085ºCElectrical Characteristics (T A=25ºC, VCC=6V, f=200 Khz)Reference (REF) Symbol Parameter Conditions Min.Typ. Max.UnitComp connect to FB 1.225 1.25 1.275VT A = -20ºC ~ 25ºC -0.1 ±1 % V REF Output voltage change withtemperature T A = 25ºC ~ 85ºC -0.2 ±1 %Under voltage lockout (UVLO) Symbol Parameter Conditions Min.Typ. Max.Unit V UT Upper threshold voltage (V CC ) 2.9 VV LWT Lower threshold voltage (V CC ) 2.4 VV HT Hysteresis (V CC ) I O(REF) = 0.1mAT A = 25ºC500 mV Short-circuit protection (SCP) control Symbol Parameter Conditions Min.Typ. Max.Unit V IT Input threshold voltage T A = 25ºC 0.600.67 0.75V V STB Standby voltage No pull up 100 130 160 mV V LT Latched input voltage No pull up 50 100 mV I SCP Input (source) current V I = 0.7V, T A = 25ºC -10 -15 -20 µAV CT Comparator threshold voltage(COMP)1.5 VOscillator (OSC) Symbol Parameter Conditions Min.Typ. Max.Unit F OSC Frequency C T =270 pF 200 KHzStandard deviation of frequency C T =270 pF 10∆F OSC Frequency change with voltage V CC =3.6V ~ 20V 1%PWM Buck ControllerElectrical Characteristics (Continued) (T A=25ºC, VCC=6V, f=200 Khz)Error-amplifierSymbol Parameter ConditionsMin.Typ. Max. Unit V IO Input offset voltage V O (FB)=1.25V ±6 mV I IO Input offset current V O (FB)=1.25V ±100 nA I IB Input bias currentV O (FB)=1.25V 160 500 nA V CMCommon-mode input voltagerangeV CC =3.6V ~ 20V1.051.45VAVOpen-loop voltageamplificationR F =200 k Ω 70 80 dBGBW Unity-gain bandwidth1.5 MHz CMRR Common-mode rejection ratio 60 80 dB V OH Max. output voltage V ref -0.1 VV OL Min. output voltage1 V I OI Output (sink) current (COMP)V ID = -0.1V, V O = 1.25V 0.5 1.6 mA I OOOutput (source) current(COMP)V ID = 0.1V, V O = 1.25V-45 -70 µA Output sectionSymbol ParameterConditions Min.Typ. Max. Unit I LEAK Leakage current V O = 25V 10 µA Sink current V IN = 20V 200 mA I DRV Source currentV IN = 20V 200 mA V SATOutput saturation voltageI O = 10 mA 1.0 1.5 V I SC Short-circuit output currentV O = 6V120 mA PWM comparatorSymbol ParameterConditionsMin.Typ.Max.UnitV T0 CT 0.6 0.7 V V T100 Input threshold voltage at f =10 KHz (COMP) Maximum duty cycle 1.2 1.3 V Total deviceSymbolParameterConditionsMin.Typ. Max. Unit I CCA Average supply current C T = 270pF6 10 mA Soft StartSymbol Parameter ConditionsMin.Typ. Max. Unit V SS Soft-start Voltage 2.3 VI SSConstant Charge Current20µAPWM Buck ControllerTypical Application CircuitC3Step-Down DC/DC converterTypical CharacteristicsPWM Buck ControllerTypical Characteristics (Continued)Marking Information(Top View)PDIP/SOPLogo ID codeYear: "01" =2001 "02" =2002Xth week: 01~52~PWM Buck ControllerPackage Information(1) PDIP-8L (Plastic Dual-in-line Package )E-PIN O0.118 inchDimensions in millimeters Dimensions in inchesSymbolMin. Nom. Max. Min. Nom. Max.A - - 5.33 - - 0.210A1 0.38 - - 0.015 - - A2 3.1 3.30 3.5 0.122 0.130 0.138B 0.36 0.46 0.56 0.014 0.018 0.022B1 1.4 1.52 1.65 0.055 0.060 0.065 B2 0.81 0.99 1.14 0.032 0.039 0.045C 0.20 0.25 0.36 0.008 0.010 0.014D 9.02 9.27 9.53 0.355 0.365 0.375E 7.62 7.94 8.26 0.300 0.313 0.325E1 6.15 6.35 6.55 0.242 0.250 0.258e - 2.54 - - 0.100 -L 2.92 3.3 3.81 0.115 0.130 0.150 eB 8.38 8.89 9.40 0.330 0.350 0.370 S 0.71 0.84 0.97 0.028 0.033 0.038PWM Buck ControllerPackage Information (Continued)(2) SOP- 8L(JEDEC Small Outline Package)Dimensions In Millimeters Dimensions In InchesSymbolMin. Nom. Max. Min. Nom. Max.A 1.40 1.60 1.75 0.055 0.063 0.0690.040 - 0.100A1 0.10 - 0.25A2 1.30 1.45 1.50 0.051 0.057 0.059B 0.33 0.41 0.51 0.013 0.016 0.020C 0.19 0.20 0.25 0.0075 0.008 0.010D 4.80 5.05 5.30 0.189 0.199 0.209E 3.70 3.90 4.10 0.146 0.154 0.161e - 1.27 - - 0.050 -H 5.79 5.99 6.20 0.228 0.236 0.244L 0.38 0.71 1.27 0.015 0.028 0.050y - - 0.10 - - 0.004θ0O - 8O0O - 8O。

输入-状态稳定性 input-to-state stability 非线性控制英文材料

1 α− 1 ◦ α2 ◦ X 1 α− 2 (α1 (r )) X −1 (min{k1 , X (ru )}). r , u < ru
i.e
(6) (7) (8) (9)
Theorem 2 : (Global ISS Theorem) If the preceeding conditions are satisﬁed with D = Rn and Du = Rm, and if α1 , α2 , α3 ∈ K∞, then the system (1) is globally input-to-state stable.
x ≤d L∞ ). L∞
< ru will
Once inside this region, it is trapped inside Ωd, because of the condition on ˙. V
L∞ )},
∀t ≥ 0, 0 ≤ T ≤ t.
(3)
Deﬁnition 2 : A continuously diﬀerentiable function V : D → R is said to be an ISS Local Lyapunov function on D for the system (1) if there exist class K functions α1 , α2 , α3 , and X such that: ∀x ∈ D, t > 0 (4) α1( x ) ≤ V (x(t)) ≤ α2 ( x ) ∂V (x) f (x, u) ≤ −α3( x ) u ∈ Du : x ≥ X ( u ). (5) ∂x V is said to be an ISS Global Lyapunov function if D = Rn , Du = Rm, and α1 , α2, α3 ∈ K∞. Remarks: this means that V is an ISS Lyapunov function if (a) It is positive deﬁnite in D. (b) It is negative deﬁnite along the trajectories of (1) whenever the trajectories are outside of the ball deﬁned by x∗ = X ( u ).

三亚2024年08版小学4年级下册第10次英语第一单元期中试卷

三亚2024年08版小学4年级下册英语第一单元期中试卷考试时间：90分钟（总分:140）A卷一、综合题(共计100题共100分)1. 填空题：A _____ is a natural formation that rises prominently.2. 选择题：What do you call a place where you can borrow books?A. LibraryB. BookstoreC. SchoolD. Office3. 填空题：The __________ (历史的展望未来) influences policies.4. 填空题：I enjoy playing ______ with my siblings.5. 填空题：The hedgehog curls into a _______ (球).6. se invented ________ around 100 AD. 填空题：The Civi7. 听力题：A chemical reaction may produce ______.8. 填空题：I like to build a __________ when it snows. (雪人)9. 填空题：Planting trees can help combat ______ (气候变化).10. 选择题：What do you call the practice of growing crops?A. AgricultureB. HorticultureC. FarmingD. Gardening11. 选择题：Which planet has the longest day?A. EarthB. VenusC. MarsD. Mercury12. 填空题：My dad is really _______ (形容词) when it comes to fixing things. 他总是 _______ (动词).13. 选择题：How do you say "good night" in French?A. Bonne nuitB. Buenas nochesC. BuonanotteD. Dobranoc14. 听力题：My aunt is a ______. She helps organize events.15. 填空题：________ (植物保护活动) raise awareness.16. 选择题：What do we call a traditional story that explains something in nature?A. MythB. FableC. LegendD. Tale17. 听力题：A compound that has both acidic and basic properties is called an ______.18. 填空题：My dog loves to play with his ________.19. 填空题：She is a _____ (科学家) who studies the ocean.20. 小猫) likes to play with balls of yarn. 填空题：The ___21. 填空题：The butterfly emerges from its _________ (蛹).22. 选择题：What do we call the act of promoting teamwork?A. CollaborationB. CooperationC. PartnershipD. All of the Above答案：D23. 听力题：She is ___ her homework now. (doing, done, do)24. 选择题：What is the color of bananas?A. RedB. YellowC. GreenD. Blue答案: B25. 填空题：The _______ (猴子) eats bananas and berries.26. 听力题：In space, there is no air or ______.27. 选择题：What is the capital of Grenada?a. St. George'sb. Gouyavec. Grenvilled. Carriacou答案:a28. 听力题：I like to ___ puzzles. (solve)29. 听力题：A _______ can measure the pressure of liquids in a container.30. 选择题：What do we call the process of learning through experience?A. EducationB. TrainingC. PracticeD. Apprenticeship答案:A31. 听力题：The _______ of a wave can be visualized with a diagram.32. 听力题：Every planet in our solar system orbits the ______.33. 听力题：My aunt lives in a _____ (city/country).34. 选择题：What is the season after winter?A. FallB. SummerC. SpringD. Autumn答案:C35. 填空题：The _____ (青蛙) has a unique way of communicating.36. 选择题：What is the opposite of 'old'?A. YoungB. MatureC. AgedD. Elderly答案:A37. 听力题：There are _____ states of matter: solid, liquid, and gas.38. 填空题：My __________ (玩具名) is really __________ (形容词) to play with.39. 选择题：How many players are on a baseball team?A. NineB. TenC. ElevenD. Twelve40. 选择题：What is the term for a young female horse?A. ColtB. FillyC. FoalD. Mare答案:B41. 填空题：The __________ (古代文明的遗迹) are found all over the world.42. 填空题：We observed a ________ growing.43. 听力题：We will go _____ (shopping/working) tomorrow.44. 听力题：The clock ticks _____ (slowly/quickly).45. 填空题：My favorite board game is _______ (大富翁).46. 选择题：What is the color of a typical blueberry?A. GreenB. BlueC. RedD. Yellow答案:B47. 选择题：What is the name of the famous volcano in Italy?A. Mount EtnaB. Mount VesuviusC. Mount St. HelensD. Mount Fuji48. 填空题：I have a ________ that helps me learn.49. 选择题：What is the capital of the Republic of the Congo?A. BrazzavilleB. KinshasaC. Pointe-NoireD. Ouesso答案:A. Brazzaville50. 听力题：My mom loves to do ____ (yarn crafts).51. 填空题：The sun is _______ in the sky.52. 填空题：A ______ (蜗牛) carries its home with it wherever it goes.53. 选择题：What do we call a baby dog?A. KittenB. PuppyC. CalfD. Chick54. 听力题：My dad loves to go fishing at the ____ (lake).55. 填空题：I dream of becoming a ______ (艺术家) one day. I want to create beautiful pieces that inspire others.56. 听力题：The Earth's crust contains many valuable ______ resources.57. 填空题：My brother has a knack for __________ (解决问题).58. 听力题：The _______ can be used for decoration.59. 选择题：Which shape is round?A. SquareB. TriangleC. CircleD. Rectangle答案:CA rabbit's foot is considered a ______ (好运) charm.61. 选择题：What is the capital of France?A. BerlinB. LondonC. ParisD. Madrid答案:C62. 选择题：Which planet is known for its blue color?A. EarthB. NeptuneC. UranusD. Both B and C答案: D63. 填空题：A duck's quack can be quite ________________ (响亮).64. 填空题：Many fruits grow from _____ (树) or bushes.65. 选择题：What is the opposite of dark?A. BrightB. LightC. DullD. Shadow66. 选择题：What do we call the first month of the year?A. FebruaryB. MarchC. AprilD. January答案:D67. 填空题：A ladybug is often seen on ______ (绿叶).68. 填空题：The __________ is a major river that flows through Nigeria. (尼日尔河)Many _______ have beautiful flowers.70. 听力题：The Industrial Revolution started in the ________.71. 选择题：What is the term for a young shark?a. Pupb. Kitc. Calfd. Chick答案:a72. 填空题：__________ (化学制剂) can enhance the effectiveness of medications and treatments.73. 选择题：What do you wear on your feet?A. HatB. GlovesC. ShoesD. Scarf答案:C74. 选择题：What is the name of the sweet treat made from sugar and gelatin?A. Gummy BearsB. Candy CornC. Jelly BeansD. Marshmallows答案: D75. 听力题：The ______ helps us see light.76. 选择题：What do bees produce?A. MilkB. HoneyC. SilkD. Wool答案: B77. 填空题：The penguin waddles _______ (走路) on ice.Many cultures use _____ (植物药) for healing.79. 听力题：The cake is ________ and sweet.80. 填空题：The _______ (昆虫) crawls on the ground.81. 填空题：Planting _____ (本地树种) contributes to ecological stability.82. 选择题：What is the main language spoken in the USA?A. SpanishB. FrenchC. EnglishD. German答案:C83. 听力题：The chemical formula for potassium hydrogen phthalate is _______.84. 选择题：What is the opposite of loud?A. QuietB. SoftC. SilentD. Mute答案:A85. 选择题：What is the capital of India?A. DelhiB. MumbaiC. KolkataD. Bangalore86. 听力题：The cat is very ___. (playful)87. 选择题：What do you call the person who teaches students?A. DoctorB. TeacherC. EngineerD. Artist88. 听力题：A reaction that requires energy input is called an ______ reaction.89. 选择题：What is the tallest mountain in the world?A. K2B. Mount EverestC. KilimanjaroD. Denali答案：B90. 听力题：The capital of Papua New Guinea is __________.91. 听力题：The pH scale measures how _______ or basic a solution is.92. 填空题：The ancient city of __________ (雅典) is known for its democracy.93. 填空题：The ancient Egyptians used _____ for mummification.94. 选择题：What do we call a young fish?A. FryB. FingerlingC. LarvaD. Pup95. 选择题：What is the capital of the USA?A. New YorkB. Los AngelesC. Washington,D.C.D. Chicago答案:C96. 选择题：What is the capital of Ecuador?A. QuitoB. GuayaquilC. CuencaD. Loja答案: A97. 选择题：What is the term for a young snake?A. HatchlingB. PupC. KitD. Calf答案:A. Hatchling98. 听力题：My uncle is a fantastic ____ (chef).99. 选择题：Which animal can fly?A. FishB. BirdC. DogD. Cat100. 填空题：We have a ______ (精彩的) event planned for next week.。

Formation input-to-state stability

FORMATION INPUT-TO-STATE STABILITYHerbert G.Tanner and George J.PappasDepartment of Electrical EngineeringUniversity of PennsylvaniaPhiladelphia,PA19102tanner@,pappasg@Abstract:This paper introduces the notion of formation input-to-state stability in order to characterize the internal stability of leader-follower formations,with respect to inputs received by the formation leader.Formation ISS is a weaker form of stability than string stability since it does not require inter-agent communication.It relates group input to internal state of the group through the formation graph adjacency matrix.In this framework,different formation structures can be analyzed and compared in terms of their stability properties and their robustness.Keywords:Formations,graphs,interconnected systems,input-to-state stability.1.INTRODUCTIONFormation control problems have attracted increased attention following the advances on communication and computation technologies that enabled the de-velopment of distributed,multi-agent systems.Direct ﬁelds of application include automated highway sys-tems(Varaiya,1993;Swaroop and Hedrick,1996; Yanakiev and Kanellakopoulos,1996),reconnais-sance using wheeled robots(Balch and Arkin,1998), formationﬂight control(Mesbahi and Hadaegh,2001; Beard et al.,2000)and sattelite clustering(McInnes, 1995).For coordinating the motion of a group of agents,three different interconnection architectures have been con-sidered,namely behavior-based,virtual structure and leader-follower.In behavior based approach(Balch and Arkin,1998;Lager et al.,1994;Yun et al.,1997), several motion premitives are deﬁned for each agent and then the group behavior is generated as a weighted sum of these primary behaviors.Behavior based con-trol schemes are usually hard to analyze formally, although some attempts have been made(Egerstedt, 2000).In leader-follower approaches(Beard et al., 2000;Desai and Kumar,1997;Tabuada et al.,2001;Fierro et al.,2001),one agent is the leader of the formation and all other agents are required to fol-low the leader,directly or indirectly.Virtual structure type formations(Tan and Lewis,1997;Egerstedt and Hu,2001),on the other hand,usually require a cen-tralized control architecture.Balch and Arkin(1998)implement behavior-based schemes on formations of unmanned ground vehicles and test different formation types.Yun et al.(1997) develop elementary behavior strategies for maintain-ing a circular formation using potentialﬁeld meth-ods.Egerstedt and Hu(2001)adopt a virtual struc-ture architecture in which the agents follow a vir-tual leader using a centralized potential-ﬁeld control scheme.Fierro et al.(2001)develop feedback lineariz-ing controllers for the control of mobile robot forma-tions in which each agent is required to follow one or two leaders.Tabuada et al.(2001)investigate the conditions under which a set of formation constraints can be satisﬁed given the dynamics of the agents and consider the problem of obtaining a consistent group abstraction for the whole formation.This paper focuses on a different problem:given a leader-follower formation,investigate how the leader input affects the internal stability of the overall for-mation.Stability properties of interconnected systems have been studied within the framework of string stability(Swaroop and Hedrick,1996;Yanakiev and Kanellakopoulos,1996).String stability actually re-quires the attenuation of errors as they propagate in the formation.However,sting stability conditions are generally restrictive and generally require inter-agent communication.It is known,for instance(Yanakiev and Kanellakopoulos,1996)that string stability in au-tonomous operation of an AHS with constant interve-hicle spacing,where each vehicle receives information only with respect to the preceding vehicle,is impos-sible.We therefore believe that a weaker notion of stability of interconnected system that relates group objectives with internal stability would be useful. Our approach is based on the notion of input-to-state stability(Sontag and Wang,1995)and exploits the fact that the cascade interconnection of two input-to-state stable systems is itself input-to-state stable(Khalil, 1996;Krsti´c et al.,1995).This property allows the propagation of input-to-state gains through the for-mation structure and facilitates the calculation of the total group gains that characterize the formation per-formance in terms of stability.Formation ISS is a weaker form of stability than string stability,in the sense that it does not require inter-agent communica-tion and relies entirely on position feedback only(as opposed to both position and velocity feedback)from each leader to its follower.We represent the formation by means of a formation graph(Tabuada et al.,2001). Graphs are especially suited to capture the intercon-nections(Tabuada et al.,2001;Fierro et al.,2001) and informationﬂow(Fax and Murray,2001)within a formation.The proposed approach provides a means to link the formation leader’s motion or the external input to the internal state and the adjacency matrix of the formation.It establishes a method for comparing stability properties of different formation schemes. The rest of the paper is organized as follows:in sec-tion2the deﬁnitions for formation graphs and for-mation input-to-state stability(ISS)are given.Section 3establishes the ISS properties of an leader-follower interconnection and in section4it is shown how these properties can be propagated from one formation graph edge to another to cover the whole formation. Section5provides examples of two stucturally differ-ent basic formation conﬁgurations and indicates how interconnection differences affect stability properties. In section6results are summarized and future re-search directions are highlighted.2.FORMATION GRAPHSA formation is being modeled by means of a formation graph.The graph representation of a formation allows a uniﬁed way of capturing both the dynamics of each agent and the inter-agent formation speciﬁcations.All agent dynamics are supposed to be expressed by lin-ear,time invariant controllable systems.Formation speciﬁcations take the form of reference relative posi-tions between the agents,that describe the shape of the formation and assign roles to each agent in terms of the responcibility to preserve the speciﬁcations.Such an assignment imposes a leader-follower relationship that leads to a decentralized control architecture.The assignment is expressed as a directed edge on the formation graph(Figure1).Fig.1.An example of a formation graphDeﬁnition2.1.(Formation Graph).A formation graph F=(V,E,D)is a directed graph that consists of:•Aﬁnite set V={v1,...,v l}of l vertices and amapping v i→T R n that assignes to each verticean LTI control system describing the dynamicsof a particular agent:˙x i=A i x i+B i u iwhere x i∈R n is the state of the agent accociatedwith vectice v i,u i∈R m is the agent control inputand A i∈R n×n,B i∈R m×m is a controllable pairof matrices.•A binary relation E⊂V×V representing aleader-follower link between agents,with(v i,v j)∈E whenever the agent associated with vectice v iis to follow the agent of v j.•Aﬁnite set of formation speciﬁcations D indexedby the set E,D={d i j}(vi,v j)∈E.For each edge (v i,v j),d i j∈R n,denotes the desired relativedistance that the agent associated with vectice v ihas to maintain from the agent associated withagent v j.Our discussion specializes in acyclic formation graphs. This implies that there can be at least one agent v L that can play the role of a leader(i.e.a vectice with no outgoing arrow).The input of the leader can be used to control the evolution of the whole formation.Thegraph is ordered starting from the leader and following a breadth-ﬁrst numbering of its vertices.For every edge (v i ,v j )we associate an error vector that expresses the deviation from the speciﬁcation prescribed for that edge:z i j x j −x i −d i j ∈R ni jThe formation error z is deﬁned as the augmented vector formed by concatenating the error vectors for all edges (v i ,v j )∈E :z z e e ∈E A natural way to represent the connectivity of the graph is by means of the adjacency matrix,A .We will therefore consider the mapping E →R l ×l that assigns to the set E of ordered vertice pairs (v i ,v j )the adjacency matrix A E ∈R l ×l .Our aim is to investigate the stability properties of the formation with respect to the input u L of the formation leader.We thus need to deﬁne the kind of stability in terms of which the formation will be analyzed:Deﬁnition 2.2.(Formation Input-to-State Stability).A formation is called input-to-state stable iff there isa classfunction βand a class function γsuch that for any initial formation error z (0)and for any bounded inputs of the formation leader u L (·)the evolution of the formation error satisﬁes:z (t ) ≤β( z (0) ,t )+γsup τ≤tu L(1)By investigating the formation input-to-state stabilitywe establish a relationship between the amplitude of the input of the formation leader and the evolution of the formation errors.This will provide upper bounds for the leaders input in order for the formation shape to be maintained inside some desired speciﬁcations.Further,it will allow to characterize and compare formations according to their stability properties.3.EDGE INPUT-TO-STATE STABILITY In the leader-follower conﬁguration,one agent is re-quired to follow another by maintaining a constant distance,x j −x i =d i j .If agent i is required to follow agent j ,then this objective is naturally pursued by applying a follower feedback control law that depends on the relative distance between the agents.For x i =x j −d i j to be an equilibrium of the closed loop control system:˙x i =A i x i +B i u iit should hold that A i (x j −d i j )∈(B i );otherwise the follower cannot be stabilized at that distance from its leader.Suppose that there exists an e i j such that B i e i j =−A i (x j −d i j ).Then the following feedback law can be used for the follower:u i =K i (x j −x i −d i j )+e i jleading to the closed loop dynamics:˙x i =(A i −B i K i )(x i −x j +d i j )Then the error dynamics of the i -j pair of leader-follower becomes:˙z i j =(A i −B i K i )z i j +˙xj which can be written,assuming that agent j followsagent k :˙z i j =(A i −B i K i )z i j +g i j (2)where g i j −(A j −B j K j )z jk .The stability of the follower is thus directly dependenton the matrix (A i −B i K i ),the eigenvalues of which can be arbitrarily chosen,and the interconnection term g i j .The interconnection term can be bounded as follows:g i j ≤λM (A j −B j K j ) z jkwhere λM (·)is the maximum eigenvalue of a given matrix.If K i is chosen so that A i −B i K i is Hurwitz,then the solution of the Lyapunov equation:P i (A i −B i K i )+(A i −B i K i )T P i =−Iprovides a symmetric and positive deﬁnite matrix P i and a natural Lyapunov function candidate V i =x T i P i x i for the interconnection dynamics (2)that satisﬁes:λm (P i ) x i ≤V i ≤λM (P i ) x iwhere λm (·)and λM (·)denote the minimum and max-imum eigenvalue of a given matrix,respectively.Forthe derivative of V i :˙V i ≤− x i 2+2λM (P i )λM (A j −B j K j ) x i z jk≤−(1−θ) x i 2≤0for all x i ≥2λM (P i )λM (A j −B j K j )λm (P i )12λM (P i )t+2(λM (P i ))3(λm (P i ))12λM (P i )t(3)γi (r )=¯γi r (4)where¯βi =λM (P i )2γi =2(λM (P i ))3(λm (P i ))14.FROM EDGE STABILITY TO FORMATIONSTABILITYAn important property of input-to-state stability is that it is preserved in cascade connections.The property allows propagation of ISS properties from one agent to another,all the way up to the formation leader. This procedure will yield the global input gains of the leader and give a measure of the sensitivity of the formation shape with respect to the input applied at the leader.In the previous section it was shown that under the assumption of pure state feedback,a formation graph edge is input-to-state stable.The gain functions for the cascade interconnection˙x1=f1(t,x1,x2,u)˙x2=f2(t,x2,u)are given as:β(r,t)=β1(2β1(r,t2)+γ1(2β2(r,t2λM(P i)t+4¯γi¯βi¯βje−1−θ4λM(P j)t+¯βj e−1−θ{ζz 1=x 1z 2=x 2−x 1−d z 3=x 3−x 2−dthe formation equations can be written as:˙z 1=u ˙z 2=−kz 2−u ˙z 3=−kz 3+kz 2For the 1−2interconnection,a Lyapunov functioncandidate could be:V 2(z 2)12|z 2|2≤V 2(z 2)≤1k θ,θ∈(0,1).Then it follows that,|z 2|≤|z 2(0)|e−√k θ=β2(|z 2(0)|,t )+γ2(sup τ≤t|u |)The ISS input-gain function for agent v 2isγ2=sup τ≤t |u |∈z 23and its time derivative would then be˙V 3(z 3)=−kz 23+kz 3z 2For |z |3>sup τ≤t |z 2(τ)|θThen the formation,as a cascade connection of the subsystems of agents v 2and v 3,is input-to-state stable withγ(sup τ≤t|u |)=6+6k θ+θ2(1−θ)t,γ2=12(1−θ)t,γ3=1k θsup τ≤t|u |It can be shown analytically that the second formation can outperform the ﬁrst in terms of the magnitude of relative errors with respect to the leader’s velocity.Speciﬁcally,if we denote by γs the input-to-state gain of the ﬁrst interconnection connection and by γp the input-to-state gain of the second interconnection,γsk θ2≥6θ+6k θ+θk θ2=6k +7k θ≥2sup τ≤t |u |6.CONCLUSIONSIn this paper,the notion of formation input-to-state stability has been introduced.This form of stability can be used to characterize the internal state of a formation that has a leader-follower achitecture,and establishes a link between the motion of the leader of the formation or its external input and the shape of the formation.Formation ISS is a weaker form of stability than string stability,in the sense that it does not require inter-agent communication and relies entirely on position feedback only (as opposed to both position and velocity feedback)from each leader to its follower.Moreover,it establishes a link between the formation internal state and the outside world.In the proposed framework,different formation struc-tures can be analyzed and compared in terms of their stability properties.Future work is directed towards investigating the ef-fect of (limited)inter-agent communication on forma-tion stability and consistent ways of group abstrac-tions that are based on the formation ISS properties.Acknowledgment:This research is partially sup-ported by the University of Pennsylvania Research Foundation.7.REFERENCESBalch,T.and R.Arkin(1998).Behavior-based forma-tion control for multirobot systems.IEEE Trans-actions on Robotics and Automation.Beard,R.W.,wton and F.Y.Hadaegh(2000).A coordination architecture for spacecraft forma-tion control.IEEE Transactions on Control Sys-tems Technology.To appear.Desai,J.and V.Kumar(1997).Motion planning of nonholonomic cooperating mobile manipulators.In:IEEE International Conference on Robotics and Automation.Albuquerque,New Mexico. Egerstedt,Magnus(2000).Behavior based robotics using hybrid automata.In:Hybrid Systems:Com-putation and Control.Lecture Notes in Computer Science.Springer-Verlag.Egerstedt,Magnus and Xiaoming Hu(2001).Forma-tion constrained multi-agent control.In:Proceed-ings of the IEEE Conference on Robotics and Au-tomation.Seoul,Korea.pp.3961–3966.Fax,J.Alexander and Richard M.Murray(2001).Graph laplacians and vehicle formation stabiliza-tion.Technical Report01-007.CDS,California Institute of Technology.Fierro,R.,A.Das,V.Kumar,and J.P.Ostrowski (2001).Hybrid control of formations of robots.In:Proceedings of the IEEE International Con-ference on Robotics and Automation.Seoul,Ko-rea.pp.157–162.Khalil,Hassan,K.(1996).Nonlinear Systems.Pren-tice Hall.Krsti´c,Miroslav,Ioannis Kanellakopoulos and Petar Kokotovi´c(1995).Nonlinear and Adaptive Con-trol Design.John Willey and Sons.Lager,D.,J.Rosenblatt and M.Hebert(1994).A behavior-based systems for off-road navigation.IEEE Transaction on Robotics and Automation 10(6),776–783.McInnes, C.R.(1995).Autonomous ring forma-tion for a planar constellation of satellites.AIAA Journal of Guidance Control and Dynamics 18(5),1215–1217.Mesbahi,M.and F.Hadaegh(2001).Formationﬂying of multiple spacecraft via graphs,matrix inequal-ities,and switching.AIAA Journal of Guidance, Control and Dynamics24(2),369–377. Sontag,Eduardo D.and Yuan Wang(1995).On char-acterizations of the input-to-state stability prop-erty.Systems&Control Letters(24),351–359. Swaroop,D.and J.K.Hedrick(1996).Sting stability of interconnected systems.IEEE Transactions on Automatic Control41(3),349–357. Tabuada,Paulo,George J.Pappas and Pedro Lima (2001).Feasible formations of multi-agent sys-tems.In:Proceedings of the American Control Conference.Arlington,V A.pp.56–61.Tan,Kar-Han and M.Anthony Lewis(1997).Virtual structures for high-precision cooperative mobile robot control.Autonomous Robots4(4),387–403. Varaiya,P.(1993).Smart cars on smart roads:prob-lems of control.IEEE Transactions on Automatic Control38(2),195–207.Yanakiev,Diana and Ioannis Kanellakopoulos(1996).A simpliﬁed framework for string stability anal-ysis in AHS.In:Proceedings of the13th IFAC World Congress.San Francisco,CA.pp.177–182.Yun,Xiaoping,Gokhan Alptekin and Okay Albayrak (1997).Line and circle formations of distributed physical mobile robots.Journal of Robotic Sys-tems14(2),63–76.。

INTERNATIONAL JOURNAL OF ROBUST AND NONLINEAR CONTROL Int. J. Robust Nonlinear Control 8, 4

SING KIONG NGUANG AND MINYUE FU *
The Department of Electrical and Electronic Engineering, The University of Auckland, Private Bag 92019, Auckland, New Zealand Department of Electrical and Computer Engineering, University of Newcastle, N.S.W. 2308, Australia
1. INTRODUCTION Robust stabilization of nonlinear systems has been an important research problem in recent years. Its origin can be traced back to Leitmann’s paper [1] who introduced the matching conditions and a technique for robust stabilization of systems under these conditions. Subsequently, a great deal of work has been done to study various robust stabilization issues for matched nonlinearity and uncertainty; see References 2 — 4 for example. Most recently, the generalized matching conditions, also known as the triangular structure, have been used to capture a much larger class of nonlinearities and uncertainties using the so-called back-stepping design approach; see e.g., References 5 — 13. A main drawback of the aforementioned results is that the closed-loop system may not be very robust against additional mismatched nonlinearity and/or uncertainty. Although there are a number of papers dealing with mismatched uncertainties (see, e.g., References 14 — 16) the results are not quite satisfactory in the sense that the additional uncertainty is not taken into account in the control design. That is, the controller is designed based on the matched uncertainty only, then the size of the allowable mismatched uncertainty is calculated depending on the robustness margin of the resulting closed-loop system. Also, this method works only for linear systems with suﬃciently

H∞ gearshift control of a dual clutch based on uncertain TS models

2013 American Control Conference (ACC) Washington, DC, USA, June 17-19, 2013
H∞ gearshift control of a dual clutch based on uncertain TS models
Van-Nhu Trmbrine1
1 LAMIH, UMR CNRS 8201, University of Valenciennes and of Hainaut-Cambrésis, Le Mont Houy, 59300 Valenciennes, France (e-mail: {vannhu.tran, uber, michel.dambrine}@univ-valenciennes.fr) 2 Department of Mechanical Engineering, University of Transport and Communication, Dong-da, Hanoi, Vietnam (e-mail: nhutrv@)
as minimizing the slipping energy and preserving the driving comfort. To achieve these goals, in the literature on the clutch management, many different approaches have been proposed: optimal control [1], [2], [3], [4], [5], ﬂatness control [6], model predictive control [7], sliding mode control [8], [9], [10], PID control [11]. Most approaches are based on a linear model of the powertrain. In [11], the authors have developed a smooth control law for gear shifting of an DDCT based on the use of a PID controller to track the engine and on-coming clutch slipping speed desired reference signals. The estimation torque of the off-going clutch is considered as an input signal for the controller. The gearshift controller output signals are the engine and on-coming clutch torques. The normal force is generated through the clutch characteristic which depends on the coefﬁcient of friction. In [7], a model predictive control is proposed to manage launch, gearshift and idle modes of an AMT. A cost function deﬁned by the tracking reference requirement for the engine and the clutch slipping speeds is considered. The engine torque which is needed for calculating control law is estimated by an observer. The control law takes into account the engine no-stall and no-lurch conditions. The clutch slipping time in the simulation is rather too long: approximately 5 seconds for a launch and 2.5 seconds for a gearshift. In our work [10], a launch controller based on a DDCT model taking into account the dual clutch actuator is developed according to sliding mode control theory. In [12], the authors used a backstepping technique and an ISS (Input-to-State Stability) methodology to carry out the clutch slip control problem during the shifting with an automatic transmission type clutch-to-clutch1 . The authors focus on the "inertia phase"2 . In [13], the authors have developed a dynamic model and a logic shift for a DCT. The normal force proﬁles for oncoming and off-going clutches have been created in order to upgrade as much as possible the shift performance based on simulation trials. In the dual clutch model, the friction coefﬁcient is the difﬁcult parameter to identify. The vehicle mass is variable. The load torque is unknown and depends on the road conditions, the road slope, the tires states and the vehicle mass. Thus, the aim of this paper is to develop a robust control law based on the use of a Takagi-Sugeno (TS) fuzzy model to

小学上册第2次英语第一单元全练全测

小学上册英语第一单元全练全测英语试题一、综合题(本题有100小题，每小题1分，共100分.每小题不选、错误，均不给分)1.This toy is _______ (破了).2.The beauty of floral displays can enhance any ______ or event. (花卉展示的美丽可以提升任何场合或活动的氛围。

)3.The ________ is a small bird that sings sweetly.4.Which animal is known for its ability to swim fast?A. DogB. DolphinC. CatD. ElephantB5.The _____ (train) goes very fast.6.How do you say "good afternoon" in Spanish?A. Buenos díasB. Buenas tardesC. Buenas nochesD. Adiós7.My pet rabbit has soft _______ (毛) that I like to pet.8.How many players are in a basketball team?A. 5B. 6C. 7D. 8A9.What do we call a large body of fresh water surrounded by land?A. OceanB. SeaC. LakeD. RiverC10. A _______ (小狼) learns to hunt from its parents.11.I think it’s fun to go ________ (赶集) on weekends.12.I like to _______ in the evening.13.The _______ (蝙蝠) flies at night.14.The __________ River flows through London.15.My friend is very ________.16.The __________ (历史的复杂性) demands thorough examination.17.This girl, ______ (这个女孩), loves to play the flute.18.What is 15 + 10?A. 20B. 25C. 30D. 3519.The kitten is ______ with a ball of yarn. (playing)20.What do we call an animal that eats only plants?A. CarnivoreB. HerbivoreC. OmnivoreD. Insectivore21.The weather is _____ (sunny/cloudy) today.22.In a reaction, the total mass of the reactants equals the total mass of the _____.23. A ______ (仙人掌) can store water for long periods.24.The dog is ______ with its ball. (playing)25.What is the capital of Egypt?A. CairoB. AlexandriaC. LuxorD. GizaA26.The __________ is a famous river in South America. (亚马逊河)27. A __________ is a mixture of liquids that do not mix.28.climate adaptation) prepares for climate impacts. The ____29.Which animal is known for its long neck?A. ElephantB. GiraffeC. LionD. Tiger30.What do you call the person who flies an airplane?A. PilotB. DriverC. CaptainD. Engineer31.n rainforest is rich in ______ (生物多样性). The Amer32.I have a _____ (compass) for navigation.33.The chemical formula for barium sulfate is __________.34.The ancient Egyptians made ______ (木乃伊) as part of their burial practices.35.What is the opposite of busy?A. FreeB. OccupiedC. EngagedD. Both A and BA36.I have a funny ________ (笑话) to tell you.37.The ______ (根系) plays a key role in stability.38. A ____(public forum) invites community input.39.Which animal is known for its ability to change color?A. ChameleonB. TigerC. ElephantD. Panda40.I like to draw pictures of ________.41.The flowers in the garden are _______ and cheerful, spreading happiness.42.The ________ is a bird that can talk.43.I always try to be ______ (诚实) and tell the truth. Honesty is very ______ (重要的) in friendships.44.The _____ (狮子) is a symbol of strength and bravery.45.Water is made up of hydrogen and ______.46.What is the capital of Egypt?A. CairoB. AlexandriaC. LuxorD. GizaA47. A _______ helps us understand how energy is transferred from one form to another.48.My mom loves __________ (知识分享).49.In a solution, the solvent is the substance that dissolves the _____.50.My favorite animal is the ______ (大象). It is very ______ (聪明) and gentle.51.The ________ was a famous document that outlined human rights.52.She has long ___. (hair)53.I can make my own ________ (玩具) from recycled materials.54.The direction of a force is called its ______.55.I want to grow a ________ for my mom's birthday.56.Which instrument has strings and is played by plucking?A. HarpB. FluteC. TrumpetD. TromboneA57.What is the term for a young horse?A. CalfB. PuppyC. FoalD. KittenC58.I like to ride my _______ (我喜欢骑我的_______).59.The __________ (植物的分类) system is complex and varied.60.The main gas released by burning fossil fuels is ______ dioxide.61. A _____ (植物适应性训练) can prepare plants for environmental changes.62.She speaks ________ languages.63.The ______ (植物的生态功能) is vital for balance.64.The smell of certain flowers can evoke strong ______. (某些花的香气可以引发强烈的情感。

1、下载文档前请自行甄别文档内容的完整性，平台不提供额外的编辑、内容补充、找答案等附加服务。
2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
3、如文档侵犯您的权益，请联系客服反馈,我们会尽快为您处理(人工客服工作时间：9:00-18:30)。

Automatica 40 (2004) 2121 – 2128 /locate/automatica
Brief paper
Input-to-state stability of networked control systemsଁ
D. Neši´ ca , ∗ , A.R. Teelb
ଁ The work of ﬁrst author was supported by the Australian Research Council under the discovery grants scheme. The work of second author was supported by the AFOSR under Grant F49620-03-1-0203, the NSF under Grant ECS-0324679 and ARO under Grant DAAD19-03-1-0144. Part of this research was carried out while the second author was visiting the Mittag-Lefﬂer Institute, Sweden in March 2003, during its emphasis on Mathematical Control and Systems Theory. This paper was not presented at any IFAC meeting. This paper was recommended for publication in revised form by Associate Editor Jessey W. Grizzle under the direction of Editor Hassan Khalil. ∗ Corresponding author. Tel.: +61-3-8344-5167; fax: +61-3-8344-6678. E-mail addresses: d.nesic@ee.mu.oz.au (D. Neši´ c), teelrnet congestion protocols to “drive-by-wire” systems. NCS are currently receiving considerable attention in the literature as illustrated by recent articles (Walsh, Beldiman, & Bushnell, 2001; Walsh, Ye, & Bushnell, 2002; Neši´ c & Teel, 2004a; Zhang & Branicky, 2001) and references listed therein. The area of NCS is still in its infancy and existing results can be improved in at least two directions. First, most existing literature considers only stabilization of linear NCS whereas nonlinear NCS have received little attention (with few exceptions, such as and Neši´ c & Teel, 2004a; Walsh et al., 2001). Second, most results treat NCS without disturbances and we are aware only of limited results on stability of NCS with disturbances, such as the L∞ to rootmean-square stability of a class of NCS considered Hassibi, Boyd, and How (1999); Lp stability of NCS considered Neši´ c and Teel (2004a); results on input-output stability of linear jump parameter systems Dullerud and Lall (1999a) that can be exploited for certain NCS with static protocols. Also, in some cases it is possible to use tools for linear sampled-data systems (Dullerud & Lall, 1999b) for analysis and design of certain classes of linear NCS. In this paper,
a Department of Electrical and Electronic Engineering,The University of Melbourne, Parkville, 3052, Vic., Australia b CCEC, Electrical and Computer Engineering Department, University of California, Santa Barbara, CA 93106-9560, USA
Received 4 March 2004; received in revised form 15 June 2004; accepted 5 July 2004 Available online 11 September 2004
Abstract A new class of Lyapunov uniformly globally asymptotically stable (UGAS) protocols in networked control systems (NCS) is considered. It is shown that if the controller is designed without taking into account the network so that it yields input-to-state stability (ISS) with respect to external disturbances (not necessarily with respect to the error that will come from the network implementation), then the same controller will achieve semi-global practical ISS for the NCS when implemented via the network with a Lyapunov UGAS protocol. Moreover, the ISS gain is preserved. The adjustable parameter with respect to which semi-global practical ISS is achieved is the maximal allowable transfer interval (MATI) between transmission times. ᭧ 2004 Elsevier Ltd. All rights reserved.
we consider input-to-state stability (ISS) of nonlinear NCS with disturbances. We follow the method proposed in Walsh et al. (2001, 2002), in which one ﬁrst designs the controller without taking into account the network and then in the second step one determines a design parameter called the maximum allowable transfer interval (MATI) so that the closed loop remains stable when some control and sensor signals are transmitted via the network. This approach was shown to produce stabilizing controllers for linear NCS in Walsh et al. (2001) and nonlinear NCS in Walsh et al. (2002). Moreover, Lp stability of nonlinear systems with a large class of uniformly globally exponentially stable (UGES) protocols was investigated in Neši´ c and Teel (2004a). It was shown in Neši´ c and Teel (2004a) that several common static and dynamic protocols investigated in Walsh et al. (2001, 2002) belong to the class of UGES protocols. We consider Lyapunov uniformly globally asymptotically stable (UGAS) protocols that generalize uniformly globally exponentially stable (UGES) protocols considered in Neši´ c and Teel (2004a). We show that if the controller is designed without taking into account the network so that it yields input-to-state stability (ISS) of the closed loop system (see Sontag, 1989), then the same controller will achieve semiglobal practical ISS of NCS when implemented via the network with a Lyapunov UGAS protocol. The parameter that can be adjusted in the protocol and that is used to achieve semi-global practical ISS is MATI (see Walsh et al., 2001, 2002).