The Two-Phase Pair Corona Model for Active Galactic Nuclei and X-ray Binaries How to Obtain
美赛 数学建模 埃博拉

For office use only T1________________ T2________________ T3________________ T4________________Team Control Number39595Problem ChosenAFor office use onlyF1________________F2________________F3________________F4________________2015 Mathematical Contest in Modeling (MCM) Summary SheetEradicating EbolaSummaryWith a high risk of death, Ebola virus disease (EDV), or simple Ebola, is a horrible disease which has caused great amount of death. In this paper, we mainly build two mathematical model to help eradicate Ebola, including a Virus Propagation Model based on BA scale-free network and SIRED, a delivery system model base on local optimization.For the former part, we firstly establish a BA scale-free network to simulate the realistic interpersonal network. Basing on this network, we set up a series rules to describe the procedure of Ebola propagation, which can be refined as the “Susceptible-Exposed- Infective-Removal-Death” (SEIRD) model. By combining this two model toget her via stimulation, we, using the variation of infective number and death number to reflect the procedure of Ebola spread, successfully restore the propagation of Ebola and predict the variation trend of them. Both the infective number and death number have a high agreement with the report from WHO. Basing on the infective number curve, we easily gain the quantity of the medicine needed and the speed of manufacturing of the vaccine and drug.For the latter part, we use a local optimization method to establish a feasible delivery system. Firstly, we choose Representative points in the map and make clustering analysis based on Euclidean distance, to classify points into three area parts. Then, we select delivery centers based on Analytic Hierarchy Process (AHP) and Principal Component Analysis (PCA) in each part. Besides, routes are designed according to prim algorithm, aiming at minimum the cost in every part. In this way, we build a delivery system. By comparing the results with treatment Centers distribution which has been built, the effectiveness of the model could be examined.Besides, we also discuss other critical factors, such as isolation measures, in the further discussion part. We conclude that isolation measures play a significant role thought the entire process of eradicating Ebola.Above all, our models are both scientific and reliable. They can be applied further to other relative problems.Key Words:SIRED, Complex Network, Cluster Analysis, Analytic Hierarchy Process (AHP) Delivery Systems Model (DSM), Principal Component Analysis (PCA)Table of Content1.Introduction (1)1.1.Background (1)1.2.Restatement of the Problem (1)2.Assumptions and Notions (1)2.1.Assumptions and Justifications (1)2.2.Notions (2)3.The Virus Propagation Model Based on Complex Networks and SEIRD Model (3)3.1.Model Overview (3)plex Network Model (3)3.2.1.Small-World Network Model (3)3.2.2.BA Scale-Free Network Model (4)3.3.SIR-Based SEIRD Model (5)3.3.1.SIR Model (5)3.3.2.SEIRD Model (6)3.4.The Study of Infection Rate, Recovery Rate and Death Rate Based on the LeastSquare Method (6)3.4.1.The Relevant Calculation about Infection Rate (6)3.4.2.The Relevant Calculation about the Recovery Rate (7)3.4.3.The Relevant Calculation of Death Rate (7)3.5.The Simulation of the Transmission of Ebola Virus (8)3.5.1.The Simulation of Complex Network Model (9)3.5.2.The Simulation of Virus Transmission (10)3.6.Results and Result Analysis (11)3.6.1. A Complex Network Simulation Results Model (11)3.6.2.The Spread of the Virus the Simulation Results (12)4.Delivery Systems Model(DSM) Based on Local Optimization (13)4.1.Model Overview (13)4.2.Cluster Division Based on Cluster Analysis (14)4.3.Delivery Centers and Routes Planning Based on AHP and PCA (17)4.3.1The Three-hierarchy Structure (18)4.3.2Analytic Hierarchy Process and Principal Component Analysis for DSM .. 194.3.3Obtain the Centers (21)4.3.4Obtain the Routes (22)4.4.Results and Analysis (23)5.Other Critical Factors for Eradicating Ebola (24)5.1The Effect of the Time to Isolate Ebola on Fighting against Ebola (24)5.2The Effect of Timely Medical Treatment to Isolate Ebola on Fighting against Ebola (25)6.Results and results analysis (26)6.1.The virus propagation model based on complex networks (26)6.1.1.The contrast and analysis concerning the results of simulation and thereality (26)6.1.2.Forecast for the future (28)6.2.Delivery Systems Model Based on Local Optimization (28)7.Strengths and Weaknesses (29)7.1.Strengths (29)7.2.Weaknesses (29)8.Conclusion (30)9.Reference (30)10.Appendix (1)1.Introduction1.1.BackgroundWith a high risk of death, Ebola virus disease (EDV), or simple Ebola, is a disease of humans and other primates. Since its first outbreak in March 2014, over 8000 people have lost their lives. And till 3 February 2015, 22,495 suspected cases and 8,981 deaths had been reported. [1] However, this disease spreads only by direct contact with the bold or body fluids of a person who has developed symptoms of the disease. Following infection, patients will typically remain asymptomatic for a period of 2-21 days. During this time, tests for the virus will be negative, and patients are not infectious, posing no public health risk.[2] And recently, the world medicine association has announced that their new medication could stop Ebola and cure patients whose disease is not advanced. Thus, a feasible delivery system is in great demand and measures to eradicating Ebola should be taken immediately.1.2.Restatement of the ProblemWith the background mentioned above, we are required to build a model to help eradicate Ebola, which can be decomposed as:●Build a model, which can estimate the suspects number, exposed number,infect number, death number and recover numbers, to describe the spreadprocedure of the Ebola from its very beginning to the future.●Build an optimized model to help establish a possible and feasible deliverysystem including selecting delivery location and delivery system networkdesign.●Estimate of the quantity of the needed medicine and manufacturing speed ofvaccine or drug, based on the results of our models.●Discuss other critical factors which help eradicate Ebola.2.Assumptions and Notions2.1.Assumptions and JustificationsTo simplify the problem, we make the following basic assumptions, each of which is properly justified.●Assume that there is no people flow between countries after outbreak ofdisease in the country.After the outbreak, countries usually will ban thecontact between locals and foreigners to minimize the incoming of the virus.●Assuming that virus infection rate and fatality rate will not change bythe change of regions.Virus infection rate and fatality rate are largelydetermined by the nature of the virus itself. The different between differentregions just have a little effect and it will be ignored.●Assume that there are only rail, road and aircraft for transportation. Inthe West Africa, waterage is rare. Rail, and road are for nearby transportationwhile aircraft is for faraway.2.2. NotionsAll the variables used in this paper are listed in Table 2.1 and Table 2.2.Table 2.1 Symbols for Virus Propagation Model(VPM)SymbolDefinition Units βInfection Rate for the Susceptible in SIR Model or SEIRD Model unitless γRecovery Rate for the Infective in SIR Model unitless rRateRecovery Rate for the Infective in SEIRD Model unitless dRateDeath Rate for the Infective in the SIR Model or SEIRD Model unitless ∆N iNew Patients on a Daily Basis person N i−1The Total Number of Patients in the Previous Day person nThe Average Degree of Each Node in the Network unitless tTime S ∆D iThe New Death Toll on a Daily Basis person D i−1The Total Number of Patients in the Previous Day person moThe Initial Number of Nodes in the BA Scale-Free Network node mThe Number of Added Sides from One New Node in the BA Scale-Free Network side ∏iThe Probability for the Connection between New Nodes and the Existing Node I in the BA Scale-Free Network unitless NThe Total Number of Nodes in the BA Scale-Free Network node k iThe Degree of Node I in the BA Scale-Free Network unitless eThe Number of Sides in the BA Scale-Free Network side n SThe Number of the Susceptible in the SEIRD Model person n EThe Number of the Exposed in the SEIRD Model person n IThe Number of the Infective in the SEIRD Model person n RThe Number of the Removal in the SEIRD Model person n DThe Number of the Dead in the SEIRD Model person RThe Probability of Virus Propagation from the Recovered unitless RandomE The Number of the Exposed Who Have Reached the Exposed Time Limit Ranging from 2 to 21 Days at the Current Momentperson n E(一天)t The Number of the Exposed Who Have Reached the Final Day of the ExposedTime Limit yet not quarantine personTable 2.2 Symbols for Delivery Systems Model(DSM)SymbolDefinition Units Athe judging matrix unitless a ijThe element of judging matrix unitless λmaxthe greatest eigenvalue of matrix A unitless CI the indicator of consistency check unitlessCR the consistency ratio unitlessRI the random consistency index unitlessCW the weight vector for criteria level unitlessAW the weight vector for alternatives level unitlessY the evaluation grade unitlessV A set of points unitlessV i,V j the point of V unitlessE A set of edges unitlessDis ij The real distance of i and j unitless Arrive ij Judging for whether there is an side between i and j unitless3.The Virus Propagation Model Based on Complex Networks and SEIRD Model3.1.Model OverviewWe aim to build a Susceptible-Exposed-Infective-Removal-Death (SEIRD) virus propagation model which is based on Susceptible-Infective-Removal (SIR) model. The aimed model is featured by complex networks, which exhibit two statistical characteristics, including the Small-World Effect and the Scale-Free Effect. These characteristics could produce relatively real person-to-person and region-to-region networks. Through the statistics of the existing patients and deaths, we will try to find the relationship among the infection rate, the recovery rate and the death rate with the change of time. Then, with the help of SEIRD model, these statistics would be used to simulate the current situation concerning the number of the susceptible, the exposed, the infective, the recovered and the dead and conduct the prediction of the future.plex Network ModelResearches have shown that the person-to-person networks in real life exhibit the Small-World Effect and Scale-Free Effect. Here we will introduce the Small-World Network Model by Watts and Strogatz, and the Scale-Free Network Model by Barabdsi and Albert [3].3.2.1.Small-World Network ModelSince random network and regular network could neither properly present some important characteristics of real network, Watts and Strogatz proposed a new network model between the random network and regular network in 1998, namely WS Small-World Network Model, the construction algorithm of which is as follows.Start from a regular network: consider a regular network which contains N nodes, and these nodes form a ring. Each node is linked with its adjacent nodes, the number of which is K/Z on both left side and right side. Also, K is an even number.Randomized re-connection: the probability P will witness a random re connection with each side in the network. In other words, an endpoint of a certain side will remain unchanged and the other endpoint would be the node in the random selection.There are two rules. The first is two different nodes will at most have one side. The second is every node cannot have a side which is connected with this node [4].Randomized re-connection in construction algorithm of the WS Small-World Model may damage connectivity of network, so Newman and Watts improved this model in 1999. The new one is called NW Small-World Model, the construction algorithm of which is as follows.Start from a regular network: consider a regular network which contains N nodes, and these nodes form a ring. Each node is linked with its adjacent nodes, the number of which is K/Z on both left side and right side. Also, K is an even number.Randomized addition of sides: the probability P will witness the random selection of two nodes and the subsequent addition of a side between these two nodes. There are two rules. The first is two different nodes will at most have one side. The second is every node cannot have a side which is connected with this node[4].The network constructed by the two models are shown in Fig 3.1.Fig 3. 1 WS Small-World network and NW Small-World network3.2.2.BA Scale-Free Network ModelIn October, 1999, Barabdsi and Albert published article in Science called "Emergence of Scaling in Random Networks" [5], which proposed an important discovery that the distribution function of connectivity for many complex networks exhibit a form of power laws. Since no obvious length characteristics of connectivity could be seen among nodes in these networks, so they are called scale-free networks.As for the cause of power laws distribution, Barabasi and Albert believe that many previous network models did not take into account two important characteristics of actual networks: the consistent expanding of network and the nature of new nodes’ prior connection in the network. These characteristics will not only make node degrees which are relatively larger increase much faster, but also produce more new nodes, thus node degrees will become even larger. Then we could see the Matthew Effect. [4]Based on the Scale-Free Network, Barabasi and Albert proposed a scale-free network model, called BA Model, the construction algorithm of which is as followsi.The expanding of network: start from a network which has Mo nodes, thenintroduce a new node after each time interval and connect this node with mnodes. The prerequisite is m≤m0.ii.Prior connection: the probability between a new node and an existing node iis ∏i, the node degree of i is k i, and the node degree of j is k j. These threefactors should satisfy the following equation.∏i=k i/∑k jj(3-1) After t steps,this algorithm could lead to a network featured by m0+t nodes and m×t sides.The network of BA Model is shown in Fig 3.2.Fig 3.2 BA Scale-Free Network3.3.SIR-Based SEIRD Model3.3.1.SIR ModelSIR is the most classic model in the epidemic models, in which S represents susceptible, I represents infective and R represents removal. Specifically, the susceptible are those who are not infected, yet vulnerable to be infected after contact with the confirmed patients. The infective are those who have got the disease and could pass it to the susceptible. As for the removal, it refers to those who are quarantined or immune to a certain disease after they have recovered.In the disease propagation, SIR Model is built with the infection rate as β, the recovery rate as γ, which is shown in Fig 3.3:Fig 3.3 SIR propagation modelThis model is suitable epidemics which have the following features: no latency, only propagated by the patients, difficult to cause death, patients are immune to this disease after recovery once and for all. As for the Ebola virus, this model is insufficient to present the propagation process. Therefore, we propose the SEIRD model based on the SIR model and overcome the defects of the SIR model, thus making the SEIRD model more suitable for the research of Ebola virus.3.3.2.SEIRD ModelThe characteristics of Ebola virus are shown in Table 3.1:Table 3.1 The characteristics of Ebola virus characteristicsDetailsLatency Exposed period ranging from 2 to 21days with no infectivity during this stage[2]Retention After the recovery, there is still a certain chance of propagation[6]Immunity Recovery is accompanied with lifelong immunityTherefore, it is needed to add E (the exposed) and D (the dead) in the SIR model.E represents those who have been infected, have no symptoms, and not contagious. But within 2-21 days, the exposed will become contagious. D represents those who are dead and not contagious.From Table 3.1, we know that Ebola virus has the feature of retention, because even when they are in the state of removal, it is still possible these recovered will be infectious.In the process of disease propagation, SEIRD has witnessed the infection rate as β and the recovery rate as rRate and dRate,which is shown as follows.Fig 3.4SEIRD propagation model3.4.The Study of Infection Rate, Recovery Rate and Death RateBased on the Least Square MethodAs for the calculation of infection rate, recovery rate and death rate, we could make use of the least square method to match the daily confirmed patients and the dead toll, thus getting the function about the relationship with the passage of time.And we choose the relevant data from Guinea since it is in severely hit by the Ebola outbreak in West Africa. The variance regarding the total number of patients and the total death toll could be seen in Appendix 11.1.3.4.1.The Relevant Calculation about Infection RateThe infection rate refers to the probability that the susceptible are in contact (here it refers to the contact with body fluids) with un-isolated patients and infected with the virus. For each infective patient, the number of side is the node degree, namely the number whom he or she could infect. Therefore, the infection rate could be calculatedin this way: the number of new patients each day divides the possible number of whom each confirmed patient could infect. The number of new patients is ∆N i. The total number of patients in the previous day is N i−1. The average node degree in the network is n. The equation is as follows.β=∆N i/(N i−1×n)(3-2) The β could be calculated based on the total number o f patients in Guinea (see Appendix 11.1). Then with time data and the method of least square method, we could do data fitting and calculate the time-dependent equation. The fitting image is listed in Fig 3.5.Fig 3.5 The fitting result image of β with the passage of timeThe result of fitting curve is:β=0.0367×t−0.3189/n(3-3) And n is the average degree of person-to-person network in the process of simulation.3.4.2.The Relevant Calculation about the Recovery RateThe recovery rate refers to the probability of recovery for those who have been infected. Since at present, few instances of recovery from Ebola disease could be witness in the world (probability is almost close to zero), so the rRate here is set to be 0.001.3.4.3.The Relevant Calculation of Death RateThe death rate refers to the probability that patients become dead in process of treatment. And the death rate is calculated in the following way: the total number of new deaths every day divides the total number of patients in the previous day. The total number of new deaths in a new day is ∆D i. The total number of patients in the previous day is D i−1. The equation isdRate=∆D i/D i−1(3-4) The dRate could be calculated based on the total number of dead patients and the total number of patients in Guinea (see Appendix 11.1). Then with time data and the method of least square method, we could do data fitting and calculate the time-dependent equation. The fitting image is listed in Fig 3.6.Fig 3.6 The fitting result image of dRate with the passage of time The result of fitting curve is:dRate=(−6.119e−07)×t2 −0.0001562×t + 0.01558(3-5) 3.5.The Simulation of the Transmission of Ebola VirusThe simulation for Ebola will mainly be divided into two aspects, namely the simulation of complex network model and that of virus spread. The related flow chart will be shown in Fig 3.7.Fig 3.7 Flow chat of Stimulation of Ebola Virus Transmission3.5.1.The Simulation of Complex Network ModelFrom the previous introduction about complex network model, BA Scale-Free Network Model has displayed the Matthew Effect, which means the stronger would be much stronger and the weaker would be much weaker. In social networks, this effect is also widely seen. Take one person who just joins in a group for an example, he would normally contact with those who have the largest circle of friends. Therefore, those who get the least friends can hardly know more new friends. This finally leadsto a phenomenon that the person who is most acquainted will have more and more friends and vice versa.Based on this, the BA Scale-Free Network Model is apparently superior to that of Small-World Model. As a result of that,we would use the former to simulate the interpersonal network.According to the rules of BA network model, we should start from a network which has Mo nodes, then introduce a new node after each time interval and connect this node with m nodes. The prerequisite is m≤m0.During the connection process, the probability between a new node and an existing node i is ∏i, the node degree of i k i, and the node degree of j is k j. These three factors should satisfy the following equation.∏i=k i/∑k jj(3-6) Specifically, when the existing nodes have larger node degrees, it would be much more easier for the new ones to connect with the existing ones.After t steps, there would be a BA Scale-Free Network Model. The number of its nodes is expressed as N and the number of its sides is expressed as e:N=m0+t(3-7)e=m×t(3-8) The population of Sierra Leone now is 6.1 million and we would use this datum to produce its interpersonal network. For more details, please refer to Appendix 11.2.3.5.2.The Simulation of Virus TransmissionIn the transmission process, we assume the infection rate is β, the recovery rate is rRate, and the death rate is dRate. Based on the fitting results we previously get, we can simulate the virus transmission situation as time goes.Here comes the details.In the first place, there would be one patient who initiates the epidemic. Every single day, the virus would transmit to others among the main network and the probability of one-time propagation is β. Also, the patients would have rRate of recovery and dRate of death. Meanwhile, if the patient has been infected for 30 days, he or she would die anyway. The exposed would be in a latent period, during which they are not infectious and asymptomatic. In 2 to 21 days, these exposed ones would become infectious.n S、n E、n I、n R、n D represent the 5 different numbers of people in the SEIRD Model. t means time step (or a day),R represents the probability that those who have recovered patients would infect others. RandomE denotes the number of exposed patients who have reached the period of 2 to 21 days at the current moment.Here is the formula showing the changes in the numbers of those five types of people.n S t+1=n S t−n I t×n×β−n R t×n×β×R(3-9) n E t+1=n E t+n I t×n×β+n R t×n×β×R−RandomE(3-10) n I t+1=n I t+RandomE−n I t×rRate−n I t×dRate(3-11)n R t+1=n R t+n I t×rRate(3-12)n D t+1=n D t+n I t×dRate(3-13) After that, when the transmission has reached a certain scale (20 days after the transmission), the international organizations would adopt the measure of quarantine towards infective patients to avoid further contagion. As for the exposed patients, since they could not be quarantined immediately, so they have one day to infect others and in the next day, they would be quarantined at once.Finally, for those who have recovered, there is still a certain chance that they will propagate the disease within their networks.n Ed t on behalf of the moments lurk in reaching the last day with infectious but has not yet been isolated number. The process of five types of personnel number change:It represents the number of the exposed who have reached their last day of latency, begin to be contagious and have not yet been quarantined at the current moment. During this process, the formula exhibiting changes for these five categories of people could be listed as follows.n S t+1=n S t−n Ed t×n×β−n R t×n×β×R(3-14) n E t+1=n E t+n Ed t×n×β+n R t×n×β×R−RandomE(3-15)n I t+1=n I t+RandomE−n I t×rRate−n I t×dRate(3-16)n R t+1=n R t+n I t×rRate(3-17)n D t+1=n D t+n I t×dRate(3-18)3.6.Results and Result Analysis3.6.1. A Complex Network Simulation Results ModelPersonnel network is illustrated as Fig 3.8. Because of the population is too large so it is difficult to figure out. We use a red point to represent 10000 persons.Fig 3.8 Personnel relation network diagramThe probability distribution of nodes in a network of degrees is illustrated as Fig 3.9.The same node degrees set in 2-4, in it with degree of 4most.On behalf of each person every day in the network average fluid contact with 2-4 people.Fig 3.9 The probability of the node degree distribution map network3.6.2.The Spread of the Virus the Simulation ResultsThe number of every kind of the curveof the change over time in SEIRD model is illustrated in Fig 3.10. Due to the large population, the graph is local amplification. It is unable to find the number of susceptible people in the picture. For the rest of the curve, black represents the exposed, red represents the sufferer and pink for the removed.Fig 3.10 The number of SEIR model with the change of time4.Delivery Systems Model(DSM) Based on Local Optimization4.1.Model OverviewOptimized distributing is the most significant problem while building Delivery Systems, and it is a NP (nondeterministic polynomial) problem. In order to studied the problem, Li Zong-yong, Li Yue and Wang Zhi-xue organized an optimized distributing algorithm based on genetic algorithm in 2006[7]. In addition, Liu Hai-yan, Li Zong-ping, Ye Huai-zhen[8]discussed logistics distribution center allocation problem based on optimization method. With the help of current literature, we build a Delivery Systems Model (DSM) for drug and vaccine delivery, based on Local Optimization.In this topic, in order to establish the feasible delivery systems for WesternAfrica, we take Sierra Leone as an example. There is 14 Districts in Sierra Leone.In this model, we choose points based on Sierra Leone politics. Representative point in every District is selected. The points are located by longitude and latitude. We use Euclidean distance-based clustering analysis to process the data, so that the point set will be classified into three sub-set. Every sub-set is a part. Then, one point in every sub-set will be selected as a delivery center based on Analytic Hierarchy Process (AHP) and Principal Component Analysis (PCA). Besides, we will design the routes based on Prim algorithm, aiming at minimum the cost in every sub-set. In this way, we will build a delivery system.In addition, we will compare the results with Treatment Centers distribution which has been built, to analyze the model.4.2. Cluster Division Based on Cluster AnalysisThere are 14 districts in Sierra Leone. In every district, we choose the center position as a point. In this model, the first step is to cluster. Cluster Analysis is based on similarity. In this model, the similarity could be measured by geography distance. There is different method for cluster.Suppose there are n variable, and the objects are x and y1212(,,,),(,,,),n n x x x x y y y y ==By Euclidean distance, the distance can be calculated by (4-1)(,)d x y =(4-1)By Cosine Similarity distance, the distance can be calculated by (4-2)2(,)ni ix yd x y =∑(4-2)In this model, we use Euclidean distance. We cluster 14 districts into three Parts. First of all, we need to know about the distances between districts. The 14 Districts of Sierra Leone are located by longitude and latitude. Establish a right angle coordinate system. Set the longitude as the abscissa and latitude as ordinate. Set the Greenwich meridian and the Equator as 0 degree. The West and the South are regarded as negative while the East and the North are regarded as positive. In addition, the data related to the latitude and longitude would be converted to standard decimal form. For example, point (1330',830'W N ︒︒) is located as (-13.5, 8.5).According to World Health Organization (WHO )[9]statistics data, the basic data of Sierra Leone’ Districts are obtained, which is shown in Table 4.1 .。
维克トン能源(Victron Energy)AC耦合和因子1.0规则说明书
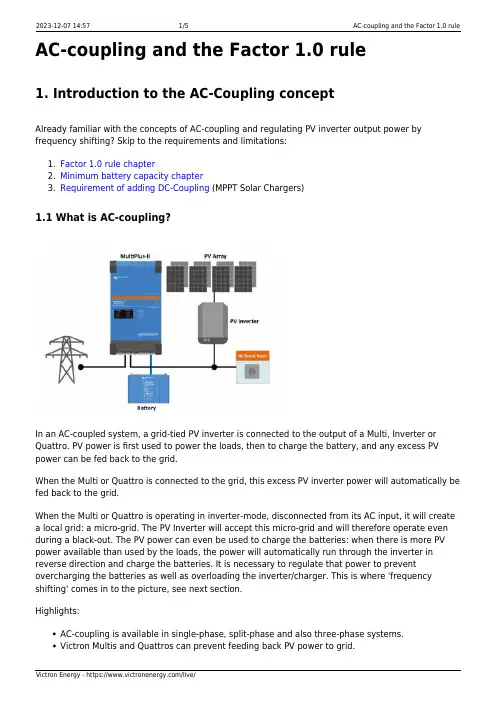
AC-coupling and the Factor 1.0 rule1. Introduction to the AC-Coupling conceptAlready familiar with the concepts of AC-coupling and regulating PV inverter output power by frequency shifting? Skip to the requirements and limitations:Factor 1.0 rule chapter1.2.Minimum battery capacity chapter3.Requirement of adding DC-Coupling (MPPT Solar Chargers)1.1 What is AC-coupling?In an AC-coupled system, a grid-tied PV inverter is connected to the output of a Multi, Inverter or Quattro. PV power is first used to power the loads, then to charge the battery, and any excess PV power can be fed back to the grid.When the Multi or Quattro is connected to the grid, this excess PV inverter power will automatically be fed back to the grid.When the Multi or Quattro is operating in inverter-mode, disconnected from its AC input, it will create a local grid: a micro-grid. The PV Inverter will accept this micro-grid and will therefore operate even during a black-out. The PV power can even be used to charge the batteries: when there is more PV power available than used by the loads, the power will automatically run through the inverter in reverse direction and charge the batteries. It is necessary to regulate that power to prevent overcharging the batteries as well as overloading the inverter/charger. This is where 'frequency shifting' comes in to the picture, see next section.Highlights:AC-coupling is available in single-phase, split-phase and also three-phase systems.Victron Multis and Quattros can prevent feeding back PV power to grid.Systems with only a grid-tied PV inverter will fail when there is a grid black-out. A micro-grid system will continue to operate, and even keep using solar power.It is also possible to run a AC-coupled micro-grid on a generatorMost brands of PV inverters can be used for these systems, they need to be setup to support frequency shifting, often called the island-mode or micro-grid mode. For SolarEdge settings, see Integrating with SolarEdge, for Fronius settings, see AC-coupled PV with Fronius PV Inverters & for reading and controling ABB/Fimer inverters, see AC-coupled PV with ABB/Fimer PV Inverters.To read out SMA inverters, see AC-coupled PV with SMA PV Inverters.If power will be fed back into the grid an anti-islanding device may have to be added to thesystem, depending on local regulations.1.2 What is frequency shifting?Frequency shifting is used to regulate the output power of a Grid-tie PV Inverter, or Grid-tie Wind inverter, by changing the frequency of the AC. The MultiPlus (or Quattro) will automatically control the frequency to prevent over charging the battery. See also the chapter 'Example & background'.For how to configure, see chapter 4.2. The Factor 1.0 ruleThe max PV power must be equal or less than the VA rating of the inverter/charger2.1 Rule definitionIn both grid-connected and off-grid systems with PV inverters installed on the output of a Multi, Inverter or Quattro, there is a maximum of PV power that can be installed. This limit is called the factor 1.0 rule: 3.000 VA Multi >= 3.000 Wp installed solar power. So for a 8.000 VA Quattro the maximum is 8.000 Wp, for two paralleled 8000 VA Quattros the maximum is 16.000 Wp, etc.2.2 Example and backgroundTo understand the background, consider the following situation: the PV inverter is at full power, supplying a big load. The Multi is in inverter mode. Then, suddenly and at once, this load is switched off. At that moment the PV inverter will continue operating at full power until the AC frequency has been increased. Increasing this frequency will take a very short time, but during that time all power will be directed into the batteries as there is no other place for it to go. This causes the following: When batteries are (nearly) full, the battery voltage will spike, possibly causing the Multi toswitch off in DC over-voltage alarm.The same spike will cause the AC output voltage of the Multi to spike, as these two are directly related, and when the spike on the battery voltage is high and fast enough, the Multi can never regulate its PWMs down fast enough to prevent the spike on AC. This spike can damage the PV inverter, the Multi and also any connected loads and other equipment.Another problem is that the Multi starts charge current protection.In the best case it might switch the grid inverter off immediately by setting the AC frequency tothe disconnect frequency as configured in the assistant.It is no problem to overpower the grid inverter by installing more solar panels. Some people do this to increase the generated solar power in winter time or rainy weather. Refer to the PV Inverter datasheet to maximum allowed installed PV power. Two times the inverter nameplate rating or even more is not uncommon!2.3 Charge current limitAnother question frequently asked is how can this factor be 1.0? Since the charger inside a 3000 VA Multi is not 3000 VA but closer to 2000 VA? The explanation lies in the fact that it will regulate. In other words: when there is too much power coming in, causing the charge current to exceed the limit, it will increase the output frequency again and will keep regulating the AC output frequency to charge with the limit.An example, a 3000 VA Multi, with 3000 W of solar power coming out of a PV inverter: 1.When the Multi is connected to the grid, all 3000 W can be fed back to the grid through theMulti, no problem.2.In case the Multi is not connected to the grid, the 3000 Wp is more than the charger in a Multi 3000 VA can handle. The charger is around 2000 W. Therefore the grid inverter assistant will automatically increase the frequency to reduce the output of the grid inverter, to matchmaximum charge current.2.4 Should you look at the total PV array, or the PV inverter rating?The mentioned 3000 Wp and 8000 Wp is the Watt-peak which can be expected from the solar system. So for an oversized PV array, where the total Watt-peak installed PV panels exceeds the power of the PV Inverter, you take the Wp from the inverter. For example 7000 Wp of solar panels installed, with an 6000 Watt PV grid inverter, the figure to be used in the calculations is 6000 Wp.And for an undersized PV array, where the total Wp of installed PV panels is less than the installed PV grid inverter, you use the Wp from the PV panels in your calculation.3. Minimum battery capacityBesides the relation between installed PV Power and the inverter/charger VA rating, it is also important to have a sufficiently sized battery. The minimum battery capacity depends on the type of battery, lead or lithium.Note that, besides the minimum battery capacity, the mentioned sizes are often also the most economical battery size. In case used for self-consumption purposes that is. In case the goal is to increase autonomy, of course installing a large battery increases the system autonomy in case of a grid failure.3.1 Lead batteries1 kWp installed PV power requires approximately 5kWh of lead acid battery:100 Ah at 48 Vdc200 Ah at 24 Vdc400 Ah at 12 VdcEach additional 1 kWp of AC PV will require an additional proportional 5 kWh increase in lead acid battery storage.3.2 Lithium batteries1,5 kWp installed AC PV power requires 4.8 kWh of battery storage:100 Ah at 48 Vdc200 Ah at 24 Vdc400 Ah at 12 VdcEach additional 1.5 kWp of AC PV will require an additional proportional 4.8 kWh increase in battery storage.4 Requirement of adding DC-Coupling - MPPT Solar Chargers Not required for Energy Storage Systems in Germany or other reliable grid situations.Required for offgrid systems as well as backup systems that need to overcome extended grid failures. Reason: recover from deadlock situation of AC-Coupling only situation.There is no Factor 1.0 limit that applies for DC coupled PV through a Victron MPPT. Nor is there a specific minimum amount of battery storage capacity, though please follow battery manufacture specifications for maximum charge rates. A rule of thumb is C10 (10% of Ah capacity in A) for lead acid batteries, and C2 (50% of Ah capacity in A) for lithium batteries.5 Software configurationMultis and Quattros with factory settings will not shift the AC output frequency to regulate charge current. When setting up an AC Coupled system, install either the ESS Assistant (for grid-connected systems) or the PV Inverter Support Assistant (for off-grid systems).The Inverter RS will automatically shift frequency without any additional configuration required when a surplus/back-feed of AC is detected on the AC-output.Other options, all deprecated, are:1.Self-consumption Hub-2 v3 assistantHub-4 Assistant in combination with the PV Inverter support Assistant 2.3.Use the Inverter period time settings on the Virtual switch tab. MonitoringSee Connecing a PV inverter section of the GX manual.DISQUSView the discussion thread.。
GSK ZJY series spindle servo motor user 说明书

In this manual, we have tried as much as possible to describe all the various matters about the spindle servo motor. However, we can not describe all the matters which must not be done or which can not be done because there are so many possibilities. Therefore, matters which are not especially described in this manual should be regarded as “impossible” or “forbidden”.The copyright of the user manual is owned by GSK CNC Equipment Co., Ltd (Hereinafter referred to as GSK).It is against the law for any organization or individual to reproduce this manual in any form without the permission of GSK and GSK reserves the right to investigate its law duty.User Manual GSK ZJY Series Spindle Servo MotorIIPREFACEDear user:It’s our honor that you select GSK ZJY serial spindle servo motor (Hereinafter referred to as the motor).For safety of the motor and the product and for the normal and effective running, please read the manual carefully before installing and using the product.SAFETY PRECAUTIONThe incorrect connection and operation may cause the accident, so before using and operating the motor, please read the manual carefully!1. The motor is installed with the photoelectric encoder, and it’s not allowed to hit themotor. And the user can’t disassemble the photoelectric encoder by himself;otherwise, once the encoder is damaged, it may cause the motor out of running!2. In the normal climate, measure the insulation resistance, which the motor winding isagainst with the case, by 1000V megameter, and the value should NOT be less than20 MΩ.3. The motor and the drive should be connected correctly based on the manual toguarantee the protective grounding stable and reliable.4. The motor can run with load only after the motor is free of noise and vibration duringrunning from zero speed to the maximum speed in the dry run mode.5. During the motor running, it’s not allowed to touch the motor shaft and case.6. Only the qualified person can adjust and maintain the motor.7. It is forbidden to move the motor by dragging the wire (cable), the motor shaft or theencoder.8. GSK does NOT take any responsibility for any change on the product by the user, andthe warranty bill becomes invalid.All specifications and designs are subject to change without notice.IIIUser Manual GSK ZJY Series Spindle Servo MotorIVRESPONSIBILITYResponsibility of the manufacturer——The manufacturer should be in charge of the design and the structure of the motor and its accessories.——The manufacturer should be responsible for the safety of the motor and its accessories.——The manufacturer should be in charge of the provided information and suggestion for the user.Responsibility of the end user——The user should be very familiar with the safety operation through learning the motor safety operation or participating in the training session.——The user should be responsible for the safety after adding, changing or modifying the original motor and its accessories by himself.——The user should be in charge of the danger resulted from the operation, adjusting, maintenance, installation and storage which are not complied with the manual regulation.The manual is kept by the end user .Thank you for your corporation during using GSK product.ContentⅠ PRODUCT CHARACTERISTICS (1)Ⅱ RUNNING CONDITIONS (1)ⅢMODELS of the MACHINE (2)Ⅳ MAIN TECHNICAL PARAMETERS and OVERALL DIMENSION of the MOTOR (3)Ⅴ MECHANICAL CHARACTERISTICS CURVE of the MOTOR (11)ⅥCONNECTION and INSTALLATION of the MOTOR (21)ⅦSTORAGE of the MOTOR (23)ⅧTRANSPORTATION of the MOTOR (23)Ⅸ WARRANTY (23)VUser Manual GSK ZJY Series Spindle Servo Motor VI1Ⅰ PRODUCT CHARACTERISTICSGSK ZJY spindle servo motor is a new type of three-phase inductive motor with highperformance and adopts insulation structure of F level, corona resistance enameled wire dedicated for the frequency conversion motor and the encoder with high speed andprecision, and the motor is researched, developed and manufactured by GSK. The product is with thecharacteristics of the compact structure, high rotation precision, low noise, high reliability and high capability with low cost, etc, which can widely satisfy the requirements of the CNC machine tool and the automation.Ⅱ RUNNING CONDITIONS2.1 The height above sea level should NOT exceed 1000m.2.2 The environment temperature should be in the range of -10℃ ~ +40℃. 2.3 The relative air humidity is ≤90% (without the condensation).2.4AC voltage value of steady state is :(0.9 ~1.1)multiplies AC rated voltage value .User Manual GSK ZJY Series Spindle Servo Motor2ⅢMODELS of the MACHINE Example: ZJY208A-5.5BH-B5A1LY1-LZJY 208 A - 5.5B H -B5A1L Y1(**) - L ⑴ ⑵ ⑶ ⑷ ⑸⑹⑺ ⑻⑼⑽⑾ ⑿SR.NO MEANING ⑴ The spindle servo motor ⑵ Motor width (182, 208, 265) ⑶ Design sequence number (None: Original A ,B ,C……:design sequence number) ⑷ Rated power (Unit: kW ) ⑸ Rated speed (T: 300 r/min, U: 450 r/min, V: 600 r/min, W: 750 r/min,A: 1000 r/min, B:1500 r/min, C: 2000 r/min, D: 2500 r/min, E: 3000 r/min )⑹ Max. speed (F: 12000 r/min, H:10000 r/min, M:7000 r/min, L:4500 r/min ) ⑺ Structure installation type:(B5 flange installation, B3 footing installation, B35 flange footing installation ) ⑻ Encoder type (None: Incremental 1024 p/r, A: Incremental 2500p/r, A1: Incremental 4096 p/r, A2: Incremental 5000 p/r, A4: Absolute 17 bit, A8: Absolute 19 bit )⑼ Look the terminal box position in view from the shaft end (None: on the top, R: on the right, L: on the left).⑽ Shaft end (None: Optic axis, Y1: with the standard key slot) ⑾ Customer special order numbers are bracketed in two capitals.⑿Power supply voltage (none: three-phase 380~440V, L: three-phase 220V)Note: ZJY182-3.7BM, ZJY208A-5.5BL and ZJY208A-7.5BL encoder types are only the incremental 1024 p/r.Product characteristics:Adopt the totally enclosed air cooling structure without the case, good shape and compactstructure.Employ the optimized electromagnetic design with the characters of the low noise, smoothrunning and high efficiency.Introduce the imported bearing in high precision, and the rotor reaches the high precisionwith the dynamic balance process, which can ensure the motor running stable and reliable with small vibration and low noise in the maximum rotational speed range.Adopt the enameled wire of corona resistance, the motor can be driven reliably at theambient temperature of -15℃~40 and ℃in the environment with the dust and oil mist. Employ the encoder at high speed and in high precision, and it can be incorporated into thedrive with high performance for controlling the speed and the position in high precision. The overload capacity is strong and the motor is reliably running at rated power of 30min150% or 5min 300%.The speed regulation range is wide and the maximum speed can reach 12000r/min. Impact resistance, long lifetime and high cost performance. Protection level: IP54(GB/T 4942.1—2006) Insulation grade: Grade F (GB 755—2008) Vibration grade: Grade B (GB 10068—2008)Ⅳ MAIN TECHNICAL PARAMETERS and OVERALL DIMENSION of the MOTOR4.1 Refer to list 1 about the main technical parameters of three-phase 380~440V spindle motor and its overall dimension.List 1SPECITEMZJY182-1.5BH ZJY182-2.2BH ZJY182-2.2CF ZJY182-3.7BHZJY182-3.7DF ZJY182-5.5CF ZJY182-7.5EH ZJY182-3.7BM ZJY208A-3.7WLRated power (kW ) 1.5 2.2 2.2 3.7 3.7 5.5 7.5 3.7 3.7 Adapted GS drive type GS3048Y GS3048Y GS3050Y GS3050Y GS3050Y GS3075Y GS3100Y GS3050Y GS3050YDrive power supply (V ) Three-phase AC 380~440V 50/60Hz Rated current(A ) 7.3 7.5 9 15.5 13 19 21 10.4 11.3 Rated frequency (Hz ) 50 50 69 50 87 70 100 50 25 Rated torque (N·m ) 9.5 14 10.5 24 14 26 24 24 47 30min power (kW ) 2.2 3.7 3.7 5.5 5.5 7.5 11 5.5 5.5 30min current(A )9.3 11 14.6 19.6 19 25 30 14.8 16 30min torque (N·m )14 24 17.7 35 21 37 35 35 70 Rated speed(r/min ) 1500 1500 2000 1500 2500 2000 3000 1500 750Constant power range (r/min ) 1500~8000 1500~80002000~100001500~8000 2500~100002000~100003000~90001500~5000 750~3000Max. speed (r/min ) 10000 10000 12000 10000 12000 12000 10000 7000 4500Moment of inertia (kg·m 2) 0.0056 0.00740.0056 0.0115 0.00740.0115 0.0115 0.00930.0309Weight (kg ) 27 32 27 43 32 43 43 3777Installation typeIM B5 or B35Cooling fanpower supply Three phase AC 380~440V 50/60Hz 37W 0.1A Threephase AC 380~440V50/60Hz 40W 0.14AA 182 182 182 182 182 182 182 182 208B 91 91 91 91 91 91 91 91 104C 126 126 126 126 126 126 126 126 160D 185 185 185 185 185 185 185 185 215E 60 60 60 60 60 60 60 60 80F 324 351 324 406 351 406 406 376 524G 198 225 198 280 225 280 280 250 395H150h7 150h7 150h7 150h7 150h7 150h7 150h7 150h7 180h7 I 12 12 12 12 12 12 12 12 14 J28h6 28h6 28h6 28h6 28h6 28h6 28h6 28h6 38h6 K 184 184 184 184 184 184 184 184 212L 93 93 93 93 93 93 93 93 106 N 156 156 156 156 156 156 156 156 180 P 32 32 32 32 32 32 32 32 40 Q 132 159 132 214 159 214 214 184 320 S 60 60 60 60 60 60 60 60 80 T 4 4 4 4 4 4 4 4 5 Overall dimen-sion(refer to figures) Z 12 12 12 12 12 12 12 12 12User Manual GSK ZJY Series Spindle Servo Motor4List 1 (Continued)SPECITEMZJY208A-2.2AM ZJY208A-3.7AM ZJY208A-5.5AM ZJY208A-2.2BH ZJY208A-3.7BH ZJY208A-5.5BH ZJY208A-7.5BH ZJY208A-3.7BM ZJY208A-5.5BMRated power (kW ) 2.2 3.7 5.5 2.2 3.7 5.5 7.5 3.7 5.5 Adapted GS drive type GS3048Y GS3050Y GS3075Y GS3048Y GS3050Y GS3075Y GS3100Y GS3050Y GS3050YDrive power supply (V ) Three phase AC 380~440V 50/60Hz Rated current(A ) 6.7 10.2 16.3 8.9 12.6 18.4 22.4 8.6 13 Ratedfrequency (Hz ) 33.3 33.3 33.3 50 50 50 50 50 50 Rated torque (N·m ) 21 35 53 14 24 35 48 24 35 30min power (kW ) 3.7 5.5 7.5 3.7 5.5 7.5 11 5.5 7.5 30min current(A )10.6 14.2 20.5 13.8 18 24 32.2 12.7 16.9 30min torque (N·m )37 53 72 24 35 48 70 35 48 Rated speed (r/min ) 1000 1000 1000 1500 1500 1500 1500 1500 1500 Constant power range (r/min ) 1000~4000 1000~4000 1000~4000 1500~8000 1500~8000 1500~8000 1500~8000 1500~5000 1500~5000Max. speed (r/min ) 7000 7000 7000 10000 10000 10000 10000 7000 7000Moment of inertia (kg·m 2)0.0168 0.0238 0.0309 0.0116 0.0168 0.0238 0.0309 0.0168 0.0238Weight (kg ) 51 66 77 49 51 66 77 51 66 Installation type IM B5 or B35 Cooling fanpower supplyThree phase AC 380~440V 50/60Hz 40W 0.14A A 208 208 208 208 208 208 208 208 208 B 104 104 104 104 104 104 104 104 104 C 160 160 160 160 160 160 160 160 160 D 215 215 215 215 215 215 215 215 215 E 60 80 80 60 60 80 80 60 80 F 414 469 524 364 414 469 524 414 469 G 285 340 395 235 285 340 395 285 340H180h7 180h7 180h7 180h7 180h7 180h7 180h7 180h7 180h7 I 14 14 14 14 14 14 14 14 14 J28h6 38h6 38h6 28h6 28h6 38h6 38h6 28h6 38h6 K 212 212 212 212 212 212 212 212 212L 106 106 106 106 106 106 106 106 106 N 180 180 180 180 180 180 180 180 180 P 40 40 40 40 40 40 40 40 40 Q 210 265 320 160 210 265 320 210 265 S 60 80 80 53 60 80 80 60 80 T 5 5 5 5 5 5 5 5 5 Overall dimen-sion(refer to figures) Z 12 12 12 12 12 12 12 12 12List 1 (Continued)SPECITEMZJY208A-7.5BM ZJY208A-5.5BL ZJY208A-7.5BL ZJY208A-11CM ZJY208A-11EHZJY265A-5.5WL ZJY265A-7.5WL ZJY265A-11WL ZJY265A-7.5AM ZJY265A-11AMRated power (kW ) 7.5 5.5 7.5 11 11 5.5 7.5 11 7.5 11 Adapted GS drive type GS3075Y GS3050Y GS3075Y GS3148Y GS3100Y GS3075Y GS3100Y GS3148Y GS3100Y GS3148YDrive power supply (V ) Three phase AC 380~440V 50/60Hz Rated current(A ) 17 12.9 17.9 28.3 25.2 16.3 21.4 30 21.5 30.9 Ratedfrequency (Hz ) 50 50 50 69 100 25 25 25 33.3 33.3 Rated torque (N·m ) 48 35 48 52.6 35 70 95.5 140 72 105 30min power (kW ) 11 7.5 11 15 15 7.5 11 15 11 15 30min current(A )24.6 16.8 24 37 31.6 20.8 30.1 41 29 40.2 30min torque (N·m )704870 71.6 48 95.5 140 191 105 145Rated speed (r/min ) 1500 1500 1500 2000 3000 750 750 750 1000 1000 Constant power range (r/min ) 1500~5000 1500~4500 1500~4500 2000~7000 3000~9000 750~3000750~3000750~30001000~4000 1000~4000Max. speed (r/min ) 7000 4500 4500 7000 100004500 4500 4500 7000 7000Moment of inertia (kg·m 2) 0.0309 0.0168 0.0238 0.03090.03090.07440.08260.086 0.04130.0826Weight (kg )775266 77.8 66 107 125 143 89 125Installation type IM B5 or B35 IM B3 or B5Cooling fanpower supply Three phase AC 380~440V 50/60Hz 40W 0.14A Three phase AC 380~440V 50/60Hz 70W 0.21AA 208 208 208 208 208 265 265 265 265 265B 104 104 104 104 104 132 132 132 132 132C 160 160 160 160 160 185 185 185 185 185D 215 215 215 215 215 265 265 265 265 265E 80 80 80 110 80 110 110 110 110 110F 524 414 469 524 469 488 533 578 443 533G 395 285 340 395 340 347 392 437 302 392H180h7 180h7 180h7 180h7180h7230h7230h7230h7 230h7230h7I 14 14 14 14 14 14 14 14 14 14 J38h6 38h6 38h6 48h6 38h6 48h6 48h6 55h6 48h6 48h6 K 212 212 212 212 212 256 256 256 256 256L 106 106 106 106 106 135 135 135 135 135 N 180 180 180 180 180 230 230 230 230 230 P 40 40 40 40 40 40 40 40 40 40 Q 320 210 265 320 265 270 315 360 225 315 S 80 80 80 110 80 110 110 110 110 110 T 5 5 5 5 5 5 5 5 5 5 Overall dimen-sion(refer to figures) Z 12 12 12 12 12 15 15 15 15 15List 1 (continued)SPEC ITEMZJY265A-15AM ZJY265A-5.5BM ZJY265A-7.5BM ZJY265A-11BMZJY265A-15BMZJY265A-18.5BMZJY265A-22BM ZJY265A-7.5BH ZJY265A-11BHZJY265A-15BHRated power (kW ) 15 5.5 7.5 11 15 18.5 22 7.5 11 15 Adapted GS drive type GS3150Y GS3050Y GS3075Y GS3100Y GS3150Y GS3150Y GS3200Y GS3100Y GS3148Y GS3150YDrive power supply (V ) Three phase AC 380~440V 50/60Hz Rated current(A ) 48.3 15 18 26 35 48.7 58 21 30 40.7 Ratedfrequency (Hz ) 33.3 50 50 50 50 50 50 50 50 50 Rated torque (N·m ) 143 35 49 72 98 118 140 48 70 95 30min power (kW ) 18.5 7.5 11 15 18.5 22 30 11 15 18.5 30min current(A )56 18.7 26 34 42 54.7 73 28.5 38.3 42.7 30min torque (N·m )177 48 74 100 123 140 191 70 95 118 Rated speed (r/min ) 1000 1500 1500 1500 1500 1500 1500 1500 1500 1500 Constant power range (r/min ) 1000~4000 1500~5000 1500~5000 1500~5000 1500~5000 1500~5000 1500~5000 1500~8000 1500~8000 1500~8000 Max. speed (r/min ) 7000 7000 7000 7000 7000 7000 7000 10000 1000010000Moment of inertia (kg·m 2)0.086 0.0205 0.0413 0.07440.08260.086 0.102 0.0413 0.07440.0826Weight (kg ) 143 62 89 107 125 143 162 89 107 125 Installation type IM B3 or B5 Cooling fanpower supplyThree phase AC 380~440V 50/60Hz 70W 0.21A A 265 265 265 265 265 265 265 265 265 265 B 132 132 132 132 132 132 132 132 132 132 C 185 185 185 185 185 185 185 185 185 185 D 265 265 265 265 265 265 265 265 265 265 E 110 110 110 110 110 110 110 110 110 110 F 578 383 443 488 533 578 633 443 488 533 G 437 242 302 347 392 437 492 302 347 392H230h7 230h7 230h7 230h7230h7230h7230h7230h7 230h7230h7I 14 14 14 14 14 14 14 14 14 14 J48h6 48h6 48h6 48h6 48h6 55h6 55h6 48h6 48h6 48h6 K 256 256 256 256 256 256 256 256 256 256L 135 135 135 135 135 135 135 135 135 135 N 230 230 230 230 230 230 230 230 230 230 P 40 40 40 40 40 40 40 40 40 40 Q 360 165 225 270 315 360 415 225 270 315 S 110 110 110 110 110 110 110 110 110 110 T 5 5 5 5 5 5 5 5 5 5 Overall dimen-sion(refer to figures) Z 15 15 15 15 15 15 15 15 15 154.2 Refer to list 2 about the main technical parameters of three-phase 220V spindle motor and its overall dimension.List 2SPECITEMZJY182-1.5BH ZJY182-2.2BH ZJY182-2.2CFZJY182-3.7BH ZJY182-3.7DF ZJY182-5.5CF ZJY208A-3.7WL ZJY208A-2.2AMRated power (kW ) 1.5 2.2 2.2 3.7 3.7 5.5 3.7 2.2 Adapted GS drive type GS2050Y GS2050Y GS2075Y GS2100Y GS2100Y GS2100Y GS2075Y GS2050YDrive power supply (V ) Three phase AC 220V 50/60Hz Rated current(A ) 10.7 12.9 14.5 23.5 22.9 32.5 19.6 11.6 Ratedfrequency (Hz ) 50 50 69 50 87 70 25 33.3 Rated torque (N·m ) 9.5 14 10.5 24 14 26 47 21 30min power (kW ) 2.2 3.7 3.7 5.5 5.5 7.5 5.5 3.7 30min current(A )17.6 20 23 36.4 33.8 47.6 27.3 18.4 30min torque (N·m )14 24 17.7 35 21 37 70 37 Rated speed (r/min ) 1500 1500 2000 1500 2500 2000 750 1000 Constant power range (r/min ) 1500~8000 1500~80002000~100001500~80002500~100002000~10000750~30001000~4000 Max. speed (r/min ) 10000 10000 12000 10000 12000 12000 45007000Moment of inertia (kg·m 2)0.0056 0.0074 0.0056 0.0115 0.00740.0115 0.0309 0.0168Weight (kg ) 27 32 27 43 32 43 77 51 Installation type IM B5 or B35Cooling fanpower supply Three phase AC 220V 50/60Hz 37W 0.1A Three phase AC 220V 50/60Hz 40W 0.14AA 182 182 182 182 182 182 208 208B 91 91 91 91 91 91 104 104C 126 126 126 126 126 126 160 160D 185 185 185 185 185 185 215 215E 60 60 60 60 60 60 80 60F 324 351 324 406 351 406 524 414G 198 225 198 280 225 280 395 285H150h7 150h7 150h7 150h7 150h7 150h7 180h7 180h7 I 12 12 12 12 12 12 14 14 J28h6 28h6 28h6 28h6 28h6 28h6 38h6 28h6 K 184 184 184 184 184 184 212 212L 93 93 93 93 93 93 106 106 N 156 156 156 156 156 156 180 180 P 32 32 32 32 32 32 40 40 Q 132 159 132 214 159 214 320 210 S 60 60 60 60 60 60 80 60 T 4 4 4 4 4 4 5 5 Overall dimen-sion(refer to figures) Z 12 12 12 12 12 12 12 12List 2 (Continued)SPECITEMZJY208A-3.7AM ZJY208A-5.5AM ZJY208A-2.2BH ZJY208A-3.7BH ZJY208A-5.5BH ZJY208A-3.7BM ZJY208A-5.5BM ZJY208A-7.5BMRated power (kW ) 3.7 5.5 2.2 3.7 5.5 3.7 5.5 7.5 Adapted GS drive type GS2075Y GS2100Y GS2075Y GS2100Y GS2100Y GS2075Y GS2100Y GS2100YDrive power supply (V ) Three phase AC 220V 50/60Hz Rated current(A ) 17.7 28.2 15.3 21.8 31.8 14.9 22.5 29.4 Ratedfrequency (Hz ) 33.3 33.3 50 50 50 50 50 50 Rated torque (N·m ) 35 53 14 24 35 24 35 48 30min power (kW ) 5.5 7.5 3.7 5.5 7.5 5.5 7.5 11 30min current(A )24.6 35.5 23.9 31.2 41.6 22 29.3 42.6 30min torque (N·m )53 72 24 35 48 35 48 70 Rated speed (r/min ) 1000 1000 1500 1500 1500 1500 1500 1500 Constant power range (r/min ) 1000~4000 1000~4000 1500~8000 1500~8000 1500~8000 1500~5000 1500~5000 1500~5000Max. speed (r/min ) 7000 7000 10000 10000 10000 7000 7000 7000Moment of inertia (kg·m 2)0.0238 0.0309 0.0116 0.0168 0.0238 0.0168 0.0238 0.0309Weight (kg ) 66 77 49 51 66 51 66 77 Installation type IM B5 or B35 Cooling fanpower supplyThree phase AC 220V 50/60Hz 40W 0.14A A 208 208 208 208 208 208 208 208 B 104 104 104 104 104 104 104 104 C 160 160 160 160 160 160 160 160 D 215 215 215 215 215 215 215 215 E 80 80 60 60 80 60 80 80 F 469 524 364 414 469 414 469 524 G 340 395 235 285 340 285 340 395H180h7 180h7 180h7 180h7 180h7 180h7 180h7 180h7 I 14 14 14 14 14 14 14 14 J38h6 38h6 28h6 28h6 38h6 28h6 38h6 38h6 K 212 212 212 212 212 212 212 212L 106 106 106 106 106 106 106 106 N 180 180 180 180 180 180 180 180 P 40 40 40 40 40 40 40 40 Q 265 320 160 210 265 210 265 320 S 80 80 53 60 80 60 80 80 T 5 5 5 5 5 5 5 5 Overall dimen-sion(refer to figures) Z 12 12 12 12 12 12 12 124.3 About the outline drawings of the motors of various installation types please referto the following figures.Flange installation type(B5)Footing installation type (B3) and left & right outlet method Flange & footing installation type(B35)and left & right outlet method4.4 Dimension of the Standard Key Slot4.4.1 ZJY182-3.7BM, ZJY208A-3.7BM, ZJY208A-2.2AMThe configuration keys: GB/T 1096 Key: 8×7×50About the dimension of the shaft end key slot, refer to the following left figure; And the central screw hole dimension on the end face of the rotary axis is M10×20.4.4.2 ZJY208A-5.5BM, ZJY208A-7.5BM, ZJY208A-5.5BL, ZJY208A-7.5BL,ZJY208A-3.7AM, ZJY208A-3.7WL, ZJY208A-5.5AMThe configuration keys: GB/T 1096 Key: 10×8×70About the dimension of the shaft end key slot, refer to the following figure; and the central screw hole dimension on the end face of the rotary axis is M10×20.ZJY265A-11BM, ZJY265A-15BM, ZJY265A-7.5AM, ZJY265A-11AM,ZJY265A-15AM, ZJY208A-11CMThe configuration keys: GB/T 1096 Key: 14×9×90About the dimension of the shaft end key slot, refer to the following figure; and the central screw hole dimension on the end face of the rotary axis is M10×20.The configuration keys: GB/T 1096 Key: 16×10×90About the dimension of the shaft end key slot, refer to the following figure; and the central screw hole dimension on the end face of the rotary axis is M10×20.ⅤMECHANICAL CHARACTERISTICS CURVE of the MOTOR Figure:Power or torque in the continuous working state;Power or torque in 30min working state.Motor type Power curve Torque curveZJY182-1.5BH ZJY182-2.2BH ZJY182-2.2CF15002.210000115001.580001.552002.20.511.522.50500010000Speed (r/min)Power (kW)15001480001.7100000.915009.5520042468101214160500010000Speed (r/min)Torque (N·m)15003.7100001.515002.280002.237143.70.511.522.533.540500010000Speed(r/min)Power (kW)20003.720002.2120001100002.275003.70.511.522.533.540500010000Speed(r/min)Power (kW)150023.580002.6100001.415001437149.55101520250500010000Speed (r/min)Torque (N· m)200017.6100002.1120000.7200010.575004.751015200500010000Speed (r/min)Torque (N· m)Motor typePower curveTorque curveZJY182-3.7BHZJY182-3.7DFZJY182-5.5CFZJY182-7.5EH1500 5.5100002.215003.780003.756005.50 1 2 3 4 5 6 0500010000Speed (r/min)Power (kW)1500358000 4.4100002.1150023.55600 9.30510********35400500010000Speed (r/min)Torque (N· m)25005.525003.7120002.2100003.776005.50 1 2 3 4 5 6 0500010000Speed (r/min)Power (kW)250021100003.5120001.7250014.17600 6.95101520250500010000Speed (r/min)Torque (N·m)2000 7.5120003.720005.5100005.577777.50 1 2 3 4 5 6 7 8 0500010000Speed (r/min)Power (kW)200035.8100005.2120002.9200026.277779.20510********35400500010000Speed (r/min)Torque (N· m)30001130007.5100005.590007.57250110 2 4 6 8 10 12 0500010000Speed (r/min)Power (kW)3000353000 23.890007.9100005.27250 14.405101520253035400500010000Speed (r/min)Torque (N· m)Motor type Power curve Torque curveZJY182-3.7BM ZJY208A-3.7WL ZJY208A-2.2AM ZJY208A-3.7AM15005.570002.215003.750003.726005.51234560200040006000Speed (r/min)Power (kW)1500355000770003150023.5260020.25101520253035400200040006000Speed (r/min)Torque (N· m)7505.545002.27503.730003.712005.5123456020004000Speed (r/min)Power (kW)75070300011.745004.675047.1120043.71020304050607080020004000Speed (r/min)Torque (N· m)10003.770000.4510002.240002.214283.70.511.522.533.540200040006000Speed (r/min)Power (kW)100035.340005.270000.6100021142824.75101520253035400200040006000Speed (r/min)Torque (N· m)10005.570001.510003.740003.715455.51234560200040006000Speed (r/min)Power (kW)100052.540008.870002100035.3154533.91020304050600200040006000Speed (r/min)Torque (N· m)Motor typePower curveTorque curveZJY208A-5.5AMZJY208A-2.2BHZJY208A-3.7BHZJY208A-5.5BH1000 7.570003.210005.540005.513917.50 1 2 3 4 5 6 7 8 0200040006000Speed (r/min)Power (kW)100071.64000 13.170004.3100052.51391 51.40102030405060708002000 40006000Speed (r/min)Torque (N· m)15003.7100001.515002.280002.237143.70 0.5 1 1.5 2 2.5 3 3.5 4 0500010000Speed (r/min)Power (kW)150023.58000 2.6100001.41500143714 9.55101520250500010000Speed (r/min)Torque (N· m)1500 5.5100002.215003.780003.756005.50 1 2 3 4 5 6 0500010000Speed (r/min)Power (kW)1500358000 4.4100002.1150023.55600 9.30510********35400500010000Speed (r/min)Torque (N· m)1500 7.5100003.715005.580005.557777.50 1 2 3 4 5 6 7 8 0500010000Speed (r/min)Power (kW)150047.78000 6.5100003.51500355777 12.3010********600500010000Speed (r/min)Torque (N· m)Motor type Power curve Torque curveZJY208A-7.5BH ZJY208A-3.7BM ZJY208A-5.5BM ZJY208A-7.5BM150011100005.515007.580007.5450011246810120500010000Speed(r/min)Power (kW)15007080008.9100005.2150047.7450023.310203040506070800500010000Speed (r/min)Torque (N· m)15005.570002.215003.750003.726005.51234560200040006000Speed (r/min)Power (kW)1500355000770003150023.5260020.25101520253035400200040006000Speed (r/min)Torque (N· m)15007.570003.715005.550005.527777.5123456780200040006000Speed (r/min)Power (kW)150047.7500010.570005150035277725.71020304050600200040006000Speed (r/min)Torque (N· m)1500117000515007.550007.5220011246810120200040006000Speed (r/min)Power (kW)150070500014.370006.8150047.7220047.710203040506070800200040006000Speed (r/min)Torque (N· m)Motor typePower curveTorque curveZJY208A-5.5BLZJY208A-7.5BLZJY208A-11CMZJY208A-11EH15007.515005.545005.50 1 2 3 4 5 6 7 8 020004000Speed (r/min)Power (kW)1500 47.7150035450011.60102030405060020004000Speed (r/min)Torque (N· m)150********.545007.50 2 4 6 8 10 12 020004000Speed (r/min)Power (kW)1500701500 47.7450015.901020304050607080020004000Speed (r/min)Torque (N· m)2000152000117000110 2 4 6 8 10 12 14 16 0200040006000Speed (r/min)Power (kW)2000 71.62000 52.5700015010203040506070800200040006000Speed (r/min)Torque (N· m)300015300011100007.59000117857150 2 4 6 8 10 12 14 16 0500010000Speed (r/min)Power (kW)3000 47.7300035900011.6100007.1785718.21020304050600500010000Speed (r/min)Torque (N· m)。
comsol等离子体放电一维模型

3 |
ATMOSPHERIC PRESSURE CORONA DISCHARGE
Solved with COMSOL Multiphysics 5.1
The space charge density ρ is automatically computed based on the plasma chemistry specified in the model using the formula N ρ = q Z n – n k k e k = 1
For detailed information about electrostatics see Theory for the Electrostatics Interface in the Plasma Module User’s Guide.
1 |
ATMOSPHERIC PRESSURE CORONA DISCHARGE
Solved with COMSOL Multiphysics 5.1
cathode (-Vin)
simulated domain (1D)
anode
ri
ro
Figure 1: Not-to-scale cross section of the co-axial configuration. The negative potential (-Vin) is applied at the inner conductor (cathode) and the outer electrode is grounded (anode). The shaded area represents the ionization region created by the positive space charge distribution generated in the vicinity of the cathode.
杰克森(JEKSON)高频测试仪模型U9825、U9845、U9805X用户手册说明书

U9825/U9845/U9805X Impulse Winding Tester P21Features● Delta and star connection of three-phase machine, Primary and secondary windings, primary and secondary secondary windings, primary and secondary + primary and secondary windings of single-phase machine can be tested; satisfying motor interturn testing of all series (U9845, all-powerful motor test)● Provide 8-channel sweep test at most (U9805X)● Two-channel comparison test, dispense with standard waveform acquisition ● 65k color 7’’ TFT high definition display screen, selectable Chinese and English interfaces● Waveform sampling rate:100Msps; memory depth: 6500bytes ● Max. Test speed: 12 meas/sec● Four waveform comparison methods: Area, differential area, corona and differential phase● Strong corona analysis and extraction function (several corona modes and corona display function) to excavate potential defective insulation of products● Excellent test repeatability to guarantee stable measurement● Instrument parameters automatic storage and boot-up file load function ● Vertical exaggeration, horizontal zoom and movement of waveforms for detail observation● Sample average function, average processing of 32 standard waveforms ● Automatic standard acquisition mode to select suitable sampling rate ● Destructive testing bring the right test voltage for you● Fast test mode can make real-time change of impulse voltage and sampling rate● Imposed demagnetized impulse to ensure the conformity of the tested waveforms ● 20000 pieces of historical measured data storage and discrimination statistics function; can be saved to the U-disk● Directly save the screen pictures (BMP , GIF, PNG) or waveform data to U-disk through SAVE key● System firmware upgrade through U-disk● Foot switch interface for easy and fast measurements● Handler, RS232C, USB Device, USB Host and GPIB (option)Brief Introduction● U9825/U9845/U9805X series impulse winding tester adopts high stability & high-voltage impact power and thyristor module controlled high-voltage switching devices, which bring a substantial increase in the stability and reliability of the product.With advanced 32 bit CPU and high speed FPGA, 100Msps sampling rate and memory depth of 6500 bytes make the test more precise; the use of high speed testing technique make the maximum test speed up to 12 meas/sec. ● Comparison test function W i t h o u t c o l l e c t i n g standard waveforms,the consistency of two tested coils can be compared rapidly.● Corona display functionUp to 100Msps sampling rate ensure precise extraction of corona information. The equipped corona display function make corona information and product performance clear and visualized.Corona display in normal waveform display Corona display in magnified waveform displaySpecificationsU9825/U9845/U9805XProvided by: (800) 404-ATECAdvanced Test Equipment Rentals®。
持续性心房颤动“2C3L”策略联合Marshall静脉无水乙醇化学消融疗效随访

DOI:10.3969/j.issn.1007-5062.2021.06.001•临床论著•持续性心房颤动“2C3L”策略联合Marshall静脉无水乙醇化学消融疗效随访左嵩桑才华赖一炜薄小雯蒋晨曦汤日波龙德勇杜昕董建增马长生[摘要]目的:评价持续性心房颤动“2C3L”策略联合Marshall静脉无水乙醇化学消融(EIVOM)术后随访1年的疗效。
方法:纳入2019年5月至2019年12月期间,于北京安贞医院接受持续性心房颤动“2C3L”消融策略联合Marshall静脉无水乙醇化学消融方案的患者48例,观察术后12个月有无心房颤动(AF)/心动过速(AD)发作。
结果:纳入的48例患者平均年龄(61.4±10.4)岁,其中36例男性(75.0%),25例长程持续性心房颤动(52.1%)。
43例(89.6%)成功完成了EIVOM,未完成的5例全部因未发现Marshall静脉。
所有患者均实现肺静脉隔离,二尖瓣峡部线、左心房顶部线及三尖瓣峡部线阻滞。
经过12个月的随访,42例(87.5%)患者在接受单次消融治疗后(无抗心律失常药物)未发作AF/AT,6例复发患者中的4例在随访期间行再次消融。
经过“2C3L”联合EIVOM策略治疗后,单次/多次消融1年无心房颤动/房性心动过速生存率为85.4%及93.5%。
所有病例均无严重并发症发生。
结论:持续性心房颤动“2C3L”联合EIVOM策略安全、有效,并能使持续性心房颤动患者在术后1年保持较高的窦性律维持。
[关键词]持续性心房颤动;Marshall静脉;导管消融;复发[中图分类号]R54[文献标志码]A[文章编号]1007・5062(2021)06・523・06One-year follow-up analysis on"2C3L”approach plus ethanol infusion into the vein of Marshall forpersistent atrial fibrillation ZUO Song,SANG Caihua,LAI Yiwei,BO Xiaowen,JIANG Chenxi,TANG Ribo,LONG Deyong,DU Xin,DONG Jianzeng,MA Changsheng Department of Cardiology,Beijing AnzhenHospital,Capital Medical University,Beijing Institute of Heart,Lung and Blood Vessel Diseases,Beijing100029,China[Abstract]Objective:To summarize the experience on the catheter ablation of persistent atrial fibrillation using the“2C3L”strategy combined with ethanol infusion in the vein of Marshall(EIVOM)after one-year follow-up.Methods:48patients with persistent atrial fibrillation using"2C3L"strategy combined withEIVOM were enrolled in Beijing Anzhen Hospital from May2019to December2019.Observe the occurrence ofatrial fibrillation or atrial tachycardia after a12-month follow-up.Results:The average age of the enrolled48patients was(61.4±10.4)years,the male ratio was75.0,and long-term persistent atrial fibrillation accountedfor about52.1.Among them,43cases(89.6%)completed EIVOM,and the remaining5cases were all due tothe absence of vein of Marshall.All patients achieved pulmonary vein isolation,mitral valve isthmus line,leftatrial roofline,and tricuspid isthmus line block.After12months of follow-up,42patients(87.5%)received asingle ablation treatment(without antiarrhythmic drugs)without AF/AT,and4of the six relapsed patients underwent reoperation during the follow-up period.After"2C3L"combined with EIVOM strategy treatment,the1year survival rate without atrial fibrillation/tachycardia after single/multiple ablation was85.4%and93.5%.Conclusions:Persistent atrial fibrillation"2C3L"strategy combined with EIVOM strategy is effective and feasible.It can maintain a high sinus maintenance rate in patients with persistent atrial fibrillation after a12-monthfollow-up.No serious complications occurred in all cases.[Keywords]Persistent atrial fibrillation;Catheter ablation;Vein of Marshall;Recurrence基金项目:国家重点研发计划课题(2016YFC1301002,2016YFC0900901,2020YFC2004803);国家自然科学基金(82000322)作者单位:100029首都医科大学附属北京安贞医院-北京市心肺血管疾病研究所心血管内科通信作者:马长生,主任医师,教授,博士生导师,研究方向:心脏电生理和心律失常。
Influence of interband interaction on Isotope Effect Exponent of MgB2 Superconductors
P.Udomsamuthirun(1),C.Kumvongsa (2) ,A.Burakorn(1), P.Changkanarth(1)
2
1.Introduction The isotope effect exponent, α , is one of the most interesting properties of superconductors. In the conventional BCS theory α = 0 .5 for all element. In high- Tc superconductors , experimenter found that α is smaller than 0.5[1-3]. This unusual small value leads to suggestion that the pairing interaction might be predominantly of electronic origin with a possible small phononnic contribution[4]. To explain the unusual isotope effect in high- Tc superconductors, many models have been proposed such as the van Hove singularity[5-7], anharmonic phonon[8,9], pairing-breaking effect[10], and pseudogap[11,12]. The discovery of [13] of superconductivity in MgB2 with a high critical temperature , Tc ≈ 39 K, has attracted a lot of considerable attention. Various experiments [14-21] suggest the existence of mutiband in MgB2 superconductors.The gap values ∆( k ) cluster into two groups at low temperature, a small value of ≈ 2.5 meV and a large value of of ≈ 7 meV. The calculation of the electron structure [2226] support this conclusion. The Fermi surface consists of four sheets : two threedimensional sheets from the π bonding and antibonding bands ( 2p z ) , and two nearly cylindrical sheets from the two-dimensional σ band ( 2p x , y ) [24,27]. There is a large difference in the electron-phonon coupling on different Fermi surface sheet and this fact leads to multiband description of superconductivity. The average electronphonon coupling strength is found to be small values[14-16].Ummarino et al.[28] proposed that MgB2 is the weak coupling two band phononic system where the Coulomb pseudopotential and the interchannel paring mechanism are key terms to interpret the superconductivity state. Garland[29] has shown that Coulomb potential in the d-orbitals of transition metal reduce the isotope exponent whereas sp-metals generally shown a nearly full isotope effect. So for sp- metal as MgB2 , the Coulomb effect could not be account to explain the reduced of isotope exponent. Budko et al.[30] and Hinks et al.[31] measured the boron isotope exponent and estimated as α B = 0 . 26 ± 0 .03 and nearly zero magnesium isotope effect. The boron isotope exponent is closed to that obtained for the YNi2 B2 C and LuNi2 B2 C borocarbideds [32,33] where theoretical work[34] suggested that the phonons responsible for the superconductivity are high- frequency boron optical modes. This observation is consistent with a phonon- mediated BCS superconducting mechanism that boron phonon modes are playing an important role. The theory of thermodynamic and transport properties of MgB2 was made in the framework of the two band BCS model [35-43]. Zhitomirsky and Dao[44] derive the Ginzburg-Landau functional for two gap superconductors from the microscopic BCS model and then investigate the magnetic properties . The concept of multiband superconductors was first introduced by Suhl[45] and Moskalenke[46] in case of large disparity of the electron-phonon interaction for different Fermi-surface sheets. The purpose of this paper is to derive the exact formula of Tc ‘s equation and the isotope effect exponent of two-band superconductors in weak-coupling limit by considering the influence of interband interaction . The paring interaction in each band consisted of 2 parts : a attractive electron-phonon interaction and a attractive non-electron-phonon interaction are included in our model. 2. Model and calculation The properties of MgB2 suggest the two-band s-wave superconductors(σ-band and π -band). And in each band ,it may have two energy
整体立铣刀圆弧刃前刀面的磨削轨迹算法
机械设计与制造Machinery Design & Manufacture147第6期2021年6月整体立铳刀圆弧刃前刀面的磨削轨迹算法张潇然,罗斌,陈思远,程雪峰(西南交通大学机械工程学院,四川成都610031)摘要:针对圆弧立铳刀磨削中周齿前刀面与端齿前刀面的过渡问题,提出磨削圆弧刃前刀面的砂轮轨迹算法,以此实现 周齿与端齿前刀面的光滑连接。
定义了一种切深磨削点轨迹曲线,可以同时约束圆弧前刀面的宽度和前角;定义了圆弧刃在平面中的瞬时前刀面,计算在瞬时前刀面中的砂轮磨削轨迹和姿态,再经过空间坐标变换,得出砂轮实际加工轨迹。
通过C++将算法编写为相应程序,进行仿真和实际加工验证,所得验证结果证明了该方法的正确性和可行性。
关键词:立铳刀;磨削加工;端齿圆弧刃;前刀面中图分类号:TH16;TH161 文献标识码:A 文章编号:1001-3997(2021)06-0147-03The Grinding Algorithm for the Rake Face of the Arc Edge of the Integral End MillZHANG Xiao-ran, LUO Bin, CHEN Si-yuan, CHENG Xue-feng(School of Mechanical Engineering , Southwest Jiaotong University, Sichuan Chengdu 610031, China)Abstract :A iming at the transition problem between the rake f ace of p eripheral f lank and the rake f ace of e nd tooth in the circulararc end mill, proposing a grinding algorithm f or the rake face of the arc edge that can achieve smooth connection between thetwo. Defines a depth-of-depth curve that can simultaneously constrain the width and rake angle of t he arc rake f ace. Defines theinstantaneous rake f ace of the arc edge and calculates the grinding path and attitude of t he grinding wheel in it. After the space coordinate transformation, the actual machining track of t he grinding wheel is obtained. Programming the algorithm into corre sponding p rogram by C++, and p erformming the simulation and p rocessing verification. The obtained results prove the correctnessand f easibility of t he algorithm.Key Words :End Mill ; Grinding ; Arc Edge ; Rake Face1引言圆弧头立铳刀是目前常见的高速切削刀具,具有制造成本低、材料切除率大等特点。
[资料]电力专业英语
[资料]电力专业英语(1)元件设备三绕组变压器:three-column transformer ThrClnTrans 双绕组变压器:double-column transformer DblClmnTrans 电容器:Capacitor并联电容器:shunt capacitorshunt 分流器,闪开,断开,并励电抗器:Reactor母线:Bus bar输电线:Transmission Line发电厂:power plant断路器:Breaker刀闸(隔离开关):Isolator分接头:tap电动机:motor(3) 状态参数有功:active power无功:reactive power电流:current容量:capacity电压:voltage档位:tap position有功损耗: active loss无功损耗:reactive loss功率因数:power-factor 要素,因素,代理人 factor 功率:power功角:power-angle电压等级:voltage grade空载损耗:no-load loss铁损:iron loss铜损:copper loss空载电流:no-load current(4) 电路阻抗:impedance正序阻抗:positive sequence impedance负序阻抗:negative sequence impedance零序阻抗:zero sequence impedance电阻:resistor电抗:reactance电导:conductance电纳:susceptance无功负载:reactive load 或者QLoad有功负载: active load或者 PLoad遥测:YC(telemetering)遥信:YX励磁电流(转子电流):magnetizing current定子:stator功角:power-angle上限:upper limit下限:lower limit并列的:apposable高压: high voltage低压:low voltage中压:middle voltage电力系统 power system发电机 generator励磁 excitation励磁器 excitermain exciter主励磁机电动励磁机motor-driven exciter副励磁机导频激励器pilot exciter;整流器式励磁机rectifier exciter旋转磁场励磁机rotating-field exciter发电机励磁机 generator exciter 电压 voltage电流 current母线 bus变压器 transformer升压变压器 step-up transformer 高压侧 high side 输电系统 power transmission system输电线 transmission line 固定串联电容补偿fixed series capacitor compensation稳定 stability电压稳定 voltage stability功角稳定 angle stability暂态稳定 transient stability 电厂 power plant能量输送 power transfer交流 AC装机容量 installed capacity 电网 power system落点 drop point开关站 switch station双回同杆并架 double-circuit lines on the same tower变电站 transformer substation 补偿度 degree of compensation 高抗high voltage shunt reactor 无功补偿 reactive power compensation 故障 fault调节 regulation裕度 magin三相故障 three phase fault 故障切除时间 fault clearing time 极限切除时间 critical clearing time 切机 generator triping高顶值 high limited value 强行励磁 reinforced excitation 线路补偿器LDC(line drop compensation)机端 generator terminal 静态 static (state)动态 dynamic (state)单机无穷大系统 one machine - infinity bus system机端电压控制 AVR电抗 reactance电阻 resistance功角 power angle有功(功率) active power无功(功率) reactive power 功率因数 power factor无功电流 reactive current 下降特性 droop characteristics 斜率 slope 额定 rating变比 ratio参考值 reference value电压互感器 PT分接头 tap下降率 droop rate仿真分析 simulation analysis 传递函数 transfer function 框图 block diagram受端 receive-side裕度 margin同步 synchronization 失去同步 loss of synchronization阻尼 damping摇摆 swing保护断路器 circuit breaker电阻:resistance电抗:reactance阻抗:impedance电导:conductance电纳:susceptance导纳:admittance电感:inductance电容: capacitance二极管:diodehydraulic power plant 水力发电厂Remote control 远控HVAC(Heating, Ventilating, and Air-Conditioning)abbreviate 缩写~缩写为 abscissa axis 横坐标absolute encoder 绝对编码器 ac squirrel cage induction motor 交流笼型感应电动机ac motor 交流环电动机 academic 纯理论的accelerometer 加速度测量仪 accommodate 适应accutrol 控制器acoustic wave 声波active 有源的active region 动态区域active filter 有源滤波器 active component 有功分量 active in respect to 相对….呈阻性 active(passive) circuit elements 有(无)源电路元件actuate 激励~驱动actuator 执行机构actuator 执行器adjacent 临近的,接近的 adjacent 相邻的~邻近的 Adjustable-voltage inverter 电压型逆变器admittance 导纳advent 出现air gap 气隙aircraft 飞机air-gap flux 气隙磁通 air-gap line 气隙磁化线 air-gap flux distribution 气隙磁通分布algebraic 代数的algebraic 代数的algebraical 代数的algorithm 算法algorithmic 算法的align 调整~校准allowable temperature rise 允许温升 alloy 合金allude 暗指~直接提到 alnico 铝镍钴合金alphabet 字母表alternating current, AC 交流 aluminum 铝ambient 环境的ambiguity 模棱两可Ammeter 安培计、电流表 ammeter 电流表ampere-turns 安匝(数) amplidyne 微场扩流发电机 amplification 扩大Amplitude Modulation AM调幅 analog electronics 电力电子学 analog-to-digital conversion, ADC 模数转换器analytical 解析的analytical 分析的~分解的 angular 角的anode 阳极、正极antenna 天线aptly 适当地~适宜地 arbitration 仲裁~公断 arc welding 电弧焊armature 电枢armature coil 电枢线圈 armature m.m.f wave 电枢磁势波 armature 衔铁armature 电枢armature circuit 电枢电路 arrangement 结构as a rule of thumb 根据经验 asynchronous machine 异步电机attenuate 衰减audio 音频的automatic station 无人值守电站 automatic oscillograph 自动示波器automatic Voltage regulator(AVR) 自动电压调整器automobile 汽车automobile starter motor 汽车启动机autonomic 自治的autonomous 匿名的autotransformer 自耦变压器 auxiliary 辅助的auxiliary motor 辅助电动机 auxiliary 辅助的backlash 啮合间隙~齿隙 ballast 镇流器bandwidth 带宽bar code reader 条码阅读器 base 基极bearing 轴承bellows 膜盒bilateral circuit 双向电路 bimotored 双马达的binary 二进制binary-coded decimal BCDbiphase 双相的bipolar junction transistor(BJT 双极性晶体管bistable circuit 双稳电路 blend 混合~调和~配料 block diagram 方框图blow (保险丝)烧断bode plot 波特图bolt 螺栓boost 增压boost-buck 升压去磁boredom 讨厌~无趣braking 制动branch circuit 直路breakaway force 起步阻力 breakdown 击穿breakdown torque 极限转矩 bronze 青铜brush 电刷brute 僵化的buck 补偿bushing 套管bushing 高压套bypass 旁路by-product 副产品calibrate 校正calibration 校准~标定~刻度calibration 标定~标准化 call for 需要cam 凸轮cantilever 悬臂capability 容量capacitance effect 电容效应 capacitor 电容器capacitor 电容器capacity 容量capsule 封装carbon 碳carbon-filament lamp 碳丝灯泡 carrier 载波Cartesian coordinates 笛卡儿坐标系cartridge 盒式保险丝 cast-aluminum rotor 铸铝转子 cathode 阴极cease 停止~终了centimeter 厘米centrifugal 离心的~离心力 centrifugal force 离心力 ceramic 陶瓷的chamber 室~腔chao 混乱checksum 检查和chopper circuit 斩波电路 circuit components 电路元件 circuit parameters 电路参数 circuit diagram 电路图 circuit branch 支路circuitry 电路~线路 circumference 圆周circumnavigate 饶过clamp 夹住~夹紧clamp 夹~钳classic 古典的~经典的~传统的clearance 间隙client-server 客户-服务器 client-server model 客户服务器模型clinker-cooler 熟料冷却器 closed-loop 闭环?coast 跟踪惯性coaxial 共轴的,同轴的 cogging 齿槽效应coil winding 线圈绕组 coils 线圈、绕组coincide in phase with 与….同相 coincidence 一致~相等 collector 集电极]collector 集电极commutation 换向commutation condition 换向状况 commutator 换向器commutator 换向器commutator-brush combination 换向器-电刷总线compatible 兼容的complement 补码complex impedance 复数阻抗 complex number 复数compound 紧密、结合 compound generator 复励发电机 compounded 复励compound-wound 复励condominium (国际)共官 conductance 电导conductor 导体conduit 导线~导线管 cone pulley 塔轮~快慢轮 configuration 组态connection 接线端constraint 强制~约束 contact 触点contactor 接触器contiguous 邻近的conveyance 运输工具conveyor 传送机copper bar 铜导条copper end rings 铜端环 core 铁心corona 电晕,放电corridor 通路corridor 通道~走廊 corrosion 腐蚀cost-effective 花费大的 counter emf 反电势counter electromotive force、CEMF 反电势counteract 抵抗~抵消~消除 counterclockwise 逆时针 counterpart 对应物coupling capacitor 结合电容 creep 蠕动criteria 标准~判据 crude 不精细的~粗略的 crystal 晶体crystal 晶体的~水晶~晶体 cubicle 立方体culminate 达到极值点 culprit 犯罪者cumulative compound 积复励 cumulatively compounded motor 积复励电动机Current source inverter 电流型逆变器cutoff 截止,关闭Cyclic Redundancy Check 循环冗余检查cylindrical 圆柱式的damper 减速器dashpot relay 油壶式继电器 dashpot 阻尼器DC link 直流环节dc generator 直流发电机 dc motor 直流电动机 de machine 直流电机decouple 解耦~去除干扰 deenergize 不给…通电 deflection 挠度、挠曲demagnetization 退磁~去磁 demodulation 解调demodulator 解调器demystify 阐明denominator 分母depict 描绘、描写depict 描述depress 压下derivative 导数derive 推倒deteriorate 使….恶化deterioration 损坏,磨损 deterioration 变化~降低品质 deviation 偏差dial 刻度盘dial 刻度盘~调节控制盘 diameter 直径diaphragm 震动膜diaphragm 膜片~挡板diaphragm 膜片diaphragm 膜~隔板dictate 确定differential compound 差复励 differential pressure transducer 差压变送器differential equation 微分方程 differentiation 微分diode 二极管direct axis 直轴direct axis transient time constant 直轴瞬变时间常数direct-current 直流discrete 离散的displacement 位移displacement current 位移电流 dissipate 散发dissipate 浪费distillation 蒸馏distributed system 分布式系统 distribution 分配~配电 doubly excited 双边励磁 drill 钻床due 应得到的dungen 地牢dwelling 住房dynamic response 动态响应 dynamic braking 能耗制动 dynamic-state operation 动态运行 dynamometer 测力计~功率计 e.m.f=electromotive fore 电动势 eddy 涡流eddy current braking 涡流制动 eddy current 涡流effective values 有效值effects of saturation 饱和效应elapse 时间(流逝)elapse 过去~消逝elbow 弯头electric energy 电能electrical stressing 电气应力 electrical device 电气设备 electrode 电极电焊条 electrodynamometer 电测力计 electro-hydraulic 电动液压的electrolytic 电解的electromagnetic torque 电磁转矩 electromagnetic interference 电磁干扰electromechanical 机电的 electronic mail 电子邮件 electro-pneumatic 电动气动的 elusive 难以捉摸的emitter 发射极emitter 发射管放射器、发射极enclosure 外(机)壳enclosure 机壳enclosure 设备外壳encode 编码encoder 编码器end ring 端环energize 励磁energy converter 电能转换器 entity 实体enumerate 列举envision 预见epoch angle 初相角equilibria 平衡equilibrium level 平均值 equivalent T–circuit T型等值电路equivalent circuit 等效电路 error signal 误差信号 error detector 误差检测器 error 误差~偏差escalation 升级~提高 establishment 组织~部门 etiquette 规则excitation system 励磁系统 excited by 励磁exciting voltage 励磁电压 expedite 加速expel 排出~放出expire 期满,终止exponential 指数external armature circuit 电枢外电路 external characteristic 外特性extruded 型材的fabricate 制造faithful 正确的~可靠的 fallout 余波~附带结果fasten 固定~连接feasible 可行的feedback system 反馈系统feedback loop 反馈回路feedback 反馈feedback component 反馈元件feedback signal 反馈信号feeder 馈电线~电源线~馈电板 feedforward 前馈felt 毡ferromagnetic 铁磁的fidelity 保真度fidelity 重现精度~真实~正确 field winding 磁场绕组、励磁绕组 field coils 励磁线圈field current 励磁电流field effect transistor(FET) 场效应管 field pole 磁极figure of merit 品质因数~优值 filter 滤波器fin 飞边fixture 设备~装臵]flicker 闪烁~摇曳flip-flop 触发器fluctuation 升降剥动~不规则的变化 fluorescent 荧光的~有荧光性的flux density 磁通密度flux linkage 磁链flux per pole 每极磁通forced commutation 强迫换流forced-draft 强制通风forging 锻造form-wound 模绕forward transfer function 正向传递函数 forward 转发fraction 分数frame 机座~机壳frequency 频率frequency- domain 频域Frequency Shift Keying(FSK) 移频键控 friction 摩擦full load 满载full-duplex 全双工full-load torque 满载转矩 furnace 炉fuse 保险丝、熔丝fuse 熔断器~保险丝 fuse 熔断器gain 增益gamut 全体~整体gear 齿轮、传动装臵 general-purpose relay 通用继电器 generating 发电generator 发电机generator 发电机generator voltage 发电机电压 Geometrical position 几何位臵 geometry 几何结构glitch 同步glue 胶合~粘贴goggles 护目镜~潜水镜 graphite 石墨grinder 磨床grossly 大概~大体上的 ground-fault protector (GFP)ground-fault circuit interrupter(GFCI) 接地故障保护器~接地故障断路器 gyroscope 陀螺仪half-duplex 半双工hand-wheel 手轮~驾驶盘~操纵盘hardwired 硬接线的harmonic 谐波的havoc 大破坏hazard 危险hazardous 危险的heat sink 散热器heating appliance 电热器 hierarchy 阶梯~等级 high-gain 高增益high-pass filter 高通滤波器 high-performance 高性能的 high-volume 大容量hitherto 迄今,至今hockey puck 冰球hoist 起重机horsepower 马力horsepower 马力horseshoe magnet 马蹄形磁铁 host 主机humidity 湿度hydraulic 液压传动hydraulic 液力的hydraulic 液压的~液压传动装臵hydropower station 水电站 hysteresis 磁滞ideal source 理想电源ideological 思想的imaginary part 虚部immunity 抗扰性impedance 阻抗impulse 推动力in parallel with 并联in series with 串联in terms of 根据~在……方面 in the vicinity of 在…附近~在…左右in(inch,inches) 英寸incident 入射的increment encoder 增量编码器 indicating needle 仪表、指针indispensable 必需的~必不可少的induced current 感生电流 induced-draft fan 吸风机 induction generator 感应发电机 induction coupling 感应耦合 induction machine 感应式电机 induction machine 感应电机 induction motor 感应电动机 induction motor 感应电动机 induction-disc relay 感应圆盘式继电器inductive component 感性(无功)分量inertia 惯性inertial 惯性的~惯量的 inference 干扰infinite voltage gain 无穷大电压增益 infrastructure 基础~底层结构inherent 固有的inhibit 禁止initiate 引起~促进injection molding 注模inrush current 涌流instantaneous electric power 瞬时电功率 instantaneous mechanical power 瞬时机械功率instruction set 指令集insulation 绝缘insulation 绝缘insulation 绝缘insulator string 绝缘子串intake 吸入integrate 求…的积分integrated circuit 集成电路 integration 积分下限integration 积分interactive 交互式interconnection 相互连接interface 接口interface data unit 接口数据单元 interfere with 有害于... internal resistance 内阻interoffice 局间的interrupter 断续(流、电、路)器 intimately 密切地intimately 紧密地~直接的 inventory 存货~清单inventory 存货inverse 倒数inverse time relay 反时限继电器 inversion 反相,反转inverting amplifier 反向放大器 iron-loss 铁损isolation 隔离、分离、绝缘、隔振isolation transformer 隔离变压器 jerk 振动~冲击jog 啮合joint 关节~铰链keyway 键槽killer 迷人的kiln 炉kinematic 运动的~运动学的 knob 旋钮、圆形把手 knob 钮~圆形把手lable 为……标号lagging(trailing) edge 下降沿 laminate 叠制~叠压 laminated core 叠片铁芯 lamination 叠片latch circuit 锁存电路 latching relay 自锁继电器 lathe 车床launcher 发生器~启动装臵 laundry 洗衣房leading edge 上升沿leakage 漏电流leakage reactance 漏磁电抗 leakage flux 漏磁通leakage current 漏电流 leakage 泄漏left-hand rule 左手定则 lever 杆,杠杆lever 手柄~控制杆 liable 有责任的light emitting diode 发光二极管 light-dimmer 调光lightning shielding 避雷 lightning arrester 避雷装臵 limit switch 限位开关 limiter 限幅器line 线电压line trap 限波器linear zone 线性区line-to-neutral 线与中性点间的 link 连杆live conductor 带电导体 load characteristic 负载特性 load-saturation curve 负载饱和曲线locked-rotor 锁定转子 locked-rotor torque 锁定转子转矩 longitudinal 经度了,纵向的 long-shunt 长复励loss 损耗low-pass filter 低通滤波器 lubricant 润滑剂、润滑油lubrication 润滑~注油 magnetic torque 电磁转矩 magnetic amplifier 磁放大器 magnetic circuit 磁路magnetic field 磁场magnetic flux 磁通magnetization curve 磁化曲线 magnetizing current 磁化电流~励磁电流magnetizing reacance 磁化电抗 magneto 磁发电机magnitude 振幅maintenance 维护malfunction 故障manipulations 操作~控制~处理 manipulator 机械手~操作器 manual control 手动控制 margin 余量~裕度mass 物质~块~堆mass-produce 大量生产 mature 成熟的means 手段~工具measurand 被测量~被测量对象 mechanical rectifier 机械式整流器mechanical stressing 机械应力 mechanical rectifier 机械式换向器Megohmmeter 兆欧表merit 优点~长处~指标 metallic 金属的meticulous 详细的micrometer 千分尺mid-frequency band 中频带 milliammeter 毫安表milling machine 铣床mine minder 矿坑卷扬机 mismatch 失配moderate 适度的~适中的 modulation 调制modulator 调制器modulus 模moisture 潮湿、湿气 moisture 潮气~湿度 molecule 分子morally 道德上~道义上 motoring 电动机驱动mount 安装multimeter 万用表multiple axis drive 多轴驱动 multiplexer 多路转换器 mutual flux 交互(主)磁通 mutual-inductor 互感narrowband filter 带通滤波器 neutral 中性的nevertheless 然而newsgroup 新闻组no load 空载no-load 空载nomenclature 术语nominally 标称nonetheless~none the less 仍然~依然nonvolatile 非挥发的nuisance 障碍~公害 number of poles 极数numerator 分子numerical 数值的object-oriented 面向对象的 obsolete 废弃的occupancy 占有~占用~居住 octal-base 八脚的offset 偏心Ohmmeter 欧姆计、电阻表 open-loop 开环operating condition 运行状态 operation amplifier 运算放大器operational calculus 算符演算 optical fiber 光纤orifice 孔~注孔orifice 侧流板~隔板 orthogonal 垂直的~正交的 Oscillation 振荡oscillation 振荡oscillatory 振动的~摆动的 oscilloscope 示波器outgoing 外出了~离开的 out-of-step 不同步的over-current relay 过电流继电器 overhauling 检修overload 过载P.D=potential drop 电压降panel 操纵台~面板 parity 校验pedal 踏板~踏蹬penetrate 透过~渗透 per unit value 标么值 percentage 百分数performance characteristic 工作特性peripheral 外设periphery 周围~圆周 permanent magnet 永磁体 permanent-magnet 永磁permissible 允许的perpendicular 垂直的~正交的perpendicular 垂直的~正交的Personnel 人员、职员 pertinent 有关的per-unit value 标么值 pharmaceutical 药剂~药品 phase 相位(控制) phase displacement 相位差 phase reversal 反相phase sequence 相序philosophy 基本原理 photosensor 光电传感器 pickup current 始动电流piezoelectric 压电的pilot light 信号灯piston 活塞pitch 齿轮pitfall 缺陷pivot 支点pivot 轴~支点~旋转中心pivot 轴~支点plant 装臵~设备plate (电)镀plug fuse 插头式保险丝 plugging 反向制动plunger 可动铁心~插棒式铁心pneumatic 气动的pneumatic 气动的pneumatic 气动的~气动力学的pointer 指针polarity 极性polarity 极性polarity 极性pole 极点Polyphase 多相(的)polyphase rectifier 多相整流器 polyphase rectifier 多相整流器potential transformer 电压互感器 potential distribution 电位分布potential transformer 电压互感器 potentiometer 电位器power frequency 工频power amplifier 功率放大器 power flow diagram 功率流程图 prebias 预偏臵predominant 主要的~突出的 prefix 前缀~把…放在前面 premise 上述各点~前言~根据 primary cell 原生电池primary 最初的~基本的~初级线圈prime 上撇号prime mover 原动机prime motor 原动机primitive 操作~原型prismatic 棱型的private line 专用线路process of self–excitation 自励过程 process 进程profile 轮廓~仿行profile 轮廓prolong 延长propagate 传导、传播propagation 传播~宣传proposition 命题pros and cons 优缺点protective gap 保护性间隙放电 protocol 协议protocol data unit 协议数据单元 protrude 使伸出~突出proximity 距离quadrant 象限quadruple 四合一quantization 量化r.m.s values=root mean square values 均方根值rack mounting 机架安装radial 径向的radial 径向的~辐射状的 radiated 传播random 随机的random-wound 散绕rated 额定的rated torque 额定转矩 rating 额定值~标称值~定额 ratio 比~比率reaction 电感reactive power 无功功率 reactive component 无功分量 reactive in respect to 相对….呈感性real part 实部recast 重做receptacle 插座receptacle 插座~插孔 rectifier 整流器redundant 多余的redundant 多余的~重复的 reference Voltage 基准电压 refinement 改进refinement 明确表达regeneration 再生,、后反馈放大regenerative braking 回馈制动 regulator 调节器relay 继电器release current 释放电流 reluctance 磁阻reluctance 磁阻remarks 附注~要点remote database 远程数据库 remote login 远程登陆 remote terminal 终端representation 代表~表示 reprisal 报复residence 住宅resident program 驻留程序 residential 住宅的~居住的 resolver 解算器resonance 共鸣resonance 共振restriking 电弧再触发 resynchronize 再同步retarding torque 制动转矩retrofit 改型reveal 展现reverse braking 反接制动 revolute 旋转的~转动的 revolutions per minute 转/分 revolutions per second 转/秒 revolving magnetic field 旋转磁场 RF noise 射频干扰rheostat 变阻器~电阻箱 rheostat 变阻器rig 设备right-hand rule 右手定则 rigid 刚性的~坚固的 riiple 纹动ripple 脉动.robustness 鲁棒性rolling 轧制rolling mill 轧钢机rotating commutator 旋转(整流子)换向器rotating magnetic field 旋转磁场 rotor (stator) winding 转子(定子绕组)rotor 转子rotor core 转子铁芯rotor resistance 转子电阻 router 路由器rugged 坚固的runout table 输出轨道 salient 突出的salient poles 凸极salient-pole 凸极式sampling 采样sampling period 采样周期 saturate 使…饱和saturation curve 饱和曲线 saturation effect 饱和效应 scale 刻度、量程scale 改变比例schematic (电路)原理图符号 sealed-off 封的self excited 自励self–excitation process 自励过程self-bias resistor 自偏臵电阻 self-exciting 自励的self-inductor 自感self-latching 自锁semiconductor 半导体separately 单独励磁地separately excited 他励separately excited 他励的series 串励series excited 串励series-wound 串励service data unit 服务数据单元 servo 伺服servomechanism 伺服机构~伺服系统shock 冲击short-circuiting ring 短路环 short-shunt 短复励shunt 分流~分路~并联~旁路 shunt 并励shunt 分路器shunt excited 并励shunt displacement current 旁路位移电流shunt field 并励磁场shunt-wound 并励signal amplifier 小信号放大器 signal-to-noise ration、SNR 信噪比signify 表示silica 硅石、二氧化硅 silicon 硅simulate 模拟~方针simultaneous 同时的Single Side Band(SSB) 单边带 single-phase 单相的singly excited 单边励磁sinusoidal 正弦的sinusoidal time function 正弦时间函数sinusoidal–density wave 正弦磁密度 slip 转差率slip rings 滑环slot 槽~开槽solenoid relay 螺管式继电器 solenoid 线圈solenoids 螺线管solid state 固体solid-state relay 固态继电器 solt 槽sophisticated 复杂的~完善的spare 备用的spark gap 火花放电隙 spatial waveform 空间波形 speed regulation 速度调节 speed-torque characteristic 速度转矩特性speed-torque curve 转速力矩特性曲线sphere 球体spiral 螺旋形的spring 弹簧spring 弹簧square 平方squeeze 压榨~挤~挤榨 squirrel 鼠笼式squirrel cage 鼠笼squirrel-cage 鼠笼式~笼型 stabilization network 稳定网络 stabilize 使稳定stabilizer 稳定器stabilizing transformer 稳定变压器 stand-alone 独立的standby 后备的staor winding 定子绕组 starting current 启动电流 starting torque 启动转矩 static allocation 静态分配 stator 定子stator 定子stator 定子steady–state condition 瞬态暂态steady direct current 恒稳直流电 steer 驾驶~操纵~引导 stepper 步进电机storage battery 蓄电池 strain gage 应变计量器 strategy 策略stress 应力strip 条~带~(跨接)片 strive for 争取structural 结构上的studmounted 拴接式subnet 子网subsequently 其后substantially 主要地~实质上地 subtle 微妙的~巧妙的subtract 减去summation 求和~加法 summer 加法器summing circuit 总和线路反馈系统中的比较环节switchyard 户外配电装臵 symmetry 对称synchronous 同步电动机 synchronous generator 同步发电机 synchronous condenser 同步进相(调相)机synchronous reactance 同步电抗 synchronous machine 同步电机synchronous speed 同步转速 synchronous speed 同步转速 synchroscops 同步指示器 synthesis 综合物tachogenerator 测速发电机 tachometer 转速计tachometer 测速仪tap 抽头tap 抽头taxonomy 分类学~分类 technical specifications 技术条件 telemedicine 远程医疗 terminal 端子terminal voltage 端电压 terminology 术语~专门名词 terminology 术语testbed 测试平台the dielectric 电介质theorem 定理therapy 治疗thermal 热的~热量的 thermocouple 热电偶thermocouple 热电偶thermometer 温度计third harmonic voltage 三次谐波电压three-phase 三相threshold 门限,阈值threshold 门~界限~阈值 throttle 节流阀~风门 thumb 检查、查阅thyristor 晶闸管time constant 时间常数time delay 延时time invariant 时不变的 time-phase 时间相位timing relay 延时继电器 toggle (来回)切换toggle 搬扭~刀闸token 令牌topology 拓扑学toroid 环状物torque 转矩~扭矩trade-off 权衡~折衷 trajectory 轨迹transducer 传感器transducer 变送器transformer 变压器transformer 变压器transformer 变压器transient 瞬态的transient response 瞬态响应 transistor 电子晶体管 transistor 晶体管transistor-to-transistor logic~TTL 晶体管-晶体管逻辑transit 运输translatory 平移的traveling-wave 行波triac 双向可控硅Triac 双向晶闸管trial and error 试错法~试凑法triangular symbol 三角符号 trigger 引起,触发trigger 起动装臵trigonometric transformations 瞬时值trip coil 跳闸线圈troubleshoot 排除故障 truthtable 真值表tuner 调谐器turns 匝数turns ratio 变比、匝比 two-way configuration 二线制 undervoltage 欠电压unidirectional 单方向的~方向不变的unidirectional current 单方向性电流uninterruptible power supply 不间断电源uninverting amplifer 同相放大器 validity 正确性vane 机器的叶~叶片vector equation 向(相)量方程 velodyne 伺服积分器vendor 生产厂商ventilation 通风~流通空气 vernier 游标尺vibration 振动vibration 震动vicinity 附近~邻近~接近 videoconference 可视会议 virtual reality 虚拟现实 virtually 实际上~实质上 virtue 优点viscous 粘稠的viscous friction 粘滞摩擦 volatile 挥发的~易失的 voltage control system 电压控制系统 voltage across the terminals 端电压 volt-ampere characteristics 伏安特性 Voltmeter 电压表、伏特计 voltmeter 电压表wafer 薄片Leonard system 发电机-电动机组系统 Ward-watchdog timer 看门狗定时器 water-tight 防水Watt-hour 瓦时、瓦特小时 watt-hour meter 电度表Wattmeter 瓦特计、电表、功率 wattmeter 电力表waveguide 波导、波导管 wavy groove 起伏的沟槽wear 磨损~损耗weighted 加权的whilst=whilewide area network 广域网windage 通风wind-driven generator 风动发电机 winding 绕组winding 绕组winding loss 绕组(铜)损耗 worldwide shared 全球共享的 wound-rotor 绕线式wrap 捆~缠~环绕wreak 发泄~报复wrench 扳手wye-connected 星形连接yield 产生~提供yoke 轭zener diode 齐纳二极管zero sequence current 零序电流generator 发电机gas insulated substation GIS气体绝缘变电站turbogenerator 汽轮发电机 neutral point 中性点hydrogenerator 水轮发电机 moving contact 动触头hydraulic turbine 水轮机fixed contact 静触头steam turbine 汽轮机arc-extinguishing chamber 灭弧室 dynamo 直流发电机stray capacitance 杂散电容 motor 电动机stray inductance 杂散电感 stator 定子sphere gap 球隙rotor 转子bushing tap grounding wire 套管末屏接地线power transformer 电力变压器 electrostatic voltmeter 静电电压表variable transformer 调压变压器 ammeter 电流表taped transformer 多级变压器 grounding capacitance 对地电容 step up (down) transformer 升(降)压变压器voltage divider 分压器circuit breaker CB断路器surge impedance 波阻抗dead tank oil circuit breaker 多油断路器Schering bridge 西林电桥 live tank oil circuit breaker 少油断路器Rogowski coil 罗可夫斯基线圈 vacuum circuit breaker 真空断路器oscilloscope 示波器sulphur hexafluoride breaker SF6断路器 peak voltmeter 峰值电压表potential transformer PT电压互感器 conductor 导线current transformer CT电流互感器 cascade transformer 串级变压器disconnector 隔离开关coupling capacitor 耦合电容 earthing switch 接地开关test object 被试品synchronous generator 同步发电机 detection impedance 检测阻抗asynchronous machine 异步电机 substation 变电站Insulator 绝缘子hydro power station 水力发电站 lightning arrester 避雷器thermal power station 火力发电站 metal oxide arrester MOA氧化锌避雷器 nuclear power station 核电站 bus bar 母线oil-filled power cable 充油电力电缆 overhead line 架空线mixed divider (阻容)混合分压器 transmission line 传输线XLPE cable 交链聚乙烯电缆 (coaxial) cable (同轴)电缆 relay继电器iron core 铁芯tuned circuit 调谐电路winding 绕组suspension insulator 悬式绝缘子 bushing 套管porcelain insulator 陶瓷绝缘子 front(tail) resistance 波头(尾)电阻glass insulator 玻璃绝缘子 inverter station 换流站flash counter 雷电计数器steel-reinforced aluminum 钢芯铝绞线 charging(damping) resistor 充电(阻尼)电阻conductortank 箱体point plane gap 针板间隙earth(ground) wire 接地线exciting winding 激磁绕组grading ring 均压环trigger electrode 触发电极 highvoltage engineering 高电压工程 glow discharge 辉光放电highvoltage testing technology高电压试验技术harmonic 谐波Power electronics电力电子Automatic control 自动控制 Principles of electric circuits电路原理Digital signal processing 数字信号处理电气工程专业英语词汇表 power system电力系统impulse current 冲击电流power network 电力网络impulse flashover 冲击闪络 insulation 绝缘inhomogenous field 不均匀场 overvoltage 过电压insulation coordination 绝缘配合 aging 老化internal discharge 内部放电 alternating current 交流电lightning stroke 雷电波AC transmission system交流输电系统 lightning overvoltage 雷电过电压arc discharge 电弧放电loss angle,介质,损耗角 attachment coefficient 附着系数 magneticfield 磁场attenuation factor 衰减系数 mean free path 平均自由行程 anode (cathode) 阳极,阴极, mean molecular velocity平均分子速度 breakdown ,电,击穿negative ions 负离子bubble breakdown 气泡击穿non-destructive testing 非破坏性试验 cathode ray oscilloscope 阴极射线示波器non-uniform field 不均匀场 cavity空穴,腔partial discharge 局部放电 corona 电晕peak reverse voltage 反向峰值电压 composite insulation 组合绝缘photoelectric emission 光电发射 critical breakdown voltage 临界击穿电压photon 光子Discharge 放电phase-to-phase voltage 线电压 Dielectric 电介质,绝缘体 polarity effect 极性效应 dielectric constant 介质常数 power capacitor 电力电容dielectric loss介质损耗 quasi-uniform field 稍不均匀场 direct current 直流电radio interference 无线干扰 divider ratio 分压器分压比 rating of equipment 设备额定值 grounding 接地routing testing 常规试验 electric field 电场residual capacitance 残余电容 electrochemical deterioration 电化学腐蚀shielding 屏蔽electron avalanche 电子崩 short circuit testing 短路试验electronegative gas电负性气体 space charge 空间电荷epoxy resin 环氧树脂streamer breakdown 流注击穿 expulsion gap 灭弧间隙surface breakdown 表面击穿 field strength 场强sustained discharge 自持放电 field stress 电场力switching overvoltage 操作过电压 field distortion 场畸变thermal breakdown 热击穿 field gradient 场梯度treeing 树枝放电field emission 场致发射uniform field 均匀场flashover 闪络wave front(tail) 波头,尾, gaseous insulation 气体绝缘 withstand voltage 耐受电压 Prime mover 原动机Power factor 功率因数Torque 力矩Distribution automation system 配电网自动化系统Servomechanism伺服系统Automatic meter reading 自动抄表 Boiler 锅炉Armature 电枢Internal combustion engine 内燃机 Brush 电刷Deenergize 断电Commutator 换向器Underground cable 地下电缆 Counter emf 反电势电气工程专业英语词汇表 Loop system 环网系统Demagnetization 退磁~去磁 Distribution system配电系统 Relay panel 继电器屏Trip circuit 跳闸电路Tertiary winding 第三绕组 Switchboard 配电盘~开关屏 Eddy current 涡流Instrument transducer 测量互感器 Copper loss 铜损Oil-impregnated paper 油浸纸绝缘 Iron loss 铁损Bare conductor 裸导线Leakage flux 漏磁通Reclosing 重合闸Autotransformer 自耦变压器 Distribution dispatch center 配电调度中心Zero sequence current 零序电流 Pulverizer 磨煤机Series (shunt) compensation 串,并,联补偿Drum 汽包~炉筒Restriking 电弧重燃Superheater 过热器Automatic oscillograph 自动录波仪 Peak-load 峰荷Tidal current 潮流Prime grid substation 主网变电站 Trip coil 跳闸线圈Reactive power` 无功功率 Synchronous condenser 同步调相机 Active power 有功功率Main and transfer busbar 单母线带旁路 Shunt reactor 并联电抗器Feeder 馈电线Blackout 断电、停电Skin effect 集肤效应Extra-high voltage (EHV) 超高压 Potential stress 电位应力(电场强度) Ultra-high voltage (UHV) 特高压 Capacitor bank 电容器组Domestic load 民用电crusher 碎煤机Reserve capacity备用容量 pulverizer 磨煤机Fossil-fired power plant 火电厂 baghouse 集尘室Combustion turbine 燃气轮机 Stationary (moving) blade 固定,可动,叶片Right-of-way线路走廊Shaft 转轴Rectifier 整流器Kinetic(potential) energy动,势,能 Inductive (Capacitive) 电感的(电容的) Pumpedstorage power station 抽水蓄能电站 Reactance (impedance) 电抗,阻抗, Synchronous condenser 同步调相机 Reactor 电抗器Light(boiling)-water reactor 轻,沸,水反应堆Reactive 电抗的~无功的 Stator(rotor) 定,转,子 Phase displacement (shift) 相移 Armature 电枢Surge冲击~过电压。
Self-Consistent Thermal Accretion Disk Corona Models for Compact Objects II. Application to
cal conditions in the Comptonizing medium), and he developed a more generalized theory. Using a composite spectrum from EXOSAT, GRANAT, and OSSE observations, Titarchuk (1994) found that this new accretion disk corona (ADC) model, having an optical depth τe ≈ 0.6, a coronal temperature kTC ≈ 150 keV, and a slab geometry allowed for a rough description of Cyg X-1. Although Comptonization apparently accounts for the general behavior of the observed X-ray spectrum of Cyg X-1, it appears that a reprocessing component is needed for the full description of the spectrum. Formal descriptions of the different observations in terms of a reflection component with an underlying Comptonization spectrum give better fits to the data than do pure Comptonization models. The presence of a weak fluorescence line from neutral iron (Kitamoto et al. 1990; Done et al. 1992; Marshall et al. 1993; Ebisawa et al. 1996) and the deviation of the 2–60 keV-band from a pure power law seen by HEAO 1-A2 (Inoue 1989; Done et al. 1992; Gierli´ nski et al. 1997), which is usually interpreted as a Compton reflection hump, both indicate the presence of cold or slightly ionized material present in the source, in addition to the Comptonizing medium. Therefore, since the radiation processes of Comptonization and reprocessing of coronal radiation in the cold accretion disk cover a large range of photon energies ( 2 keV to ∼ 250 keV) in the predicted X-ray spectrum, it is necessary to use-broad-band spectral observations to place meaningful constraints on the models. As we discussed in our companion paper (Dove, Wilms, &
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
–2– 1E1740.7-2942 show power law spectra extending up to hundreds of keV (e.g. Gilfanov et al. 1994; Tanaka & Levin 1995). The characteristic features of reflection have been seen in GBHC as well (e.g. Done et al. 1992). AGN and GBHC are believed to be powered by accretion through an accretion disk. In the unified model for AGN (e.g. Antonucci 1993), it is believed that we are viewing the disks in Seyfert 1 galaxies more or less face on, while for Seyfert 2 galaxies we are viewing the disk more or less edge on through a molecular torus. In GBHC sources we are viewing the binary system along some given (possibly time dependent) direction. The X-ray spectra indicate the existence of both hot X-ray emitting and cold reflecting gas components. The exact geometry is not known, but a currently popular model is the two-phase disk-corona model (e.g. Haardt & Maraschi 1991, 1993, hereafter HM93). The black body disk radiation from the cold disk (in the EUV for AGN, and in the soft X-rays for GBHC) enters the hot corona from one side and gets Comptonized into the X-rays. Part of this X-ray radiation is incident on the cold disk and is partly reflected but mainly reprocessed into soft black body radiation. The remaining part forms the X-ray spectrum leaving the disk-corona system. Both the black body and the Comptonized spectrum are anisotropic so observers at different viewing angles see different spectra (HM93). It immediately clear that such models are neither homogeneous nor spherically symmetric. In order to correctly interpret observed X-ray spectra of AGN and GBHC one needs to know the theoretical spectra for mildly relativistic temperature and for different viewing angles. Theoretical Comptonized spectra have been computed for two decades now. Almost all work make simplifying assumptions that render them useless for interpreting X-ray spectra from sources were anisotropic effects are important. We briefly discuss standard methods for modeling Comptonized spectra from AGN and GBHC at mildly relativistic temperatures. One approach is to treat the photon and pair producing processes as well as the energy and pair balance in great detail, but to make large simplifications regarding the radiative transfer using various prescriptions for the spectral shape and using simple escape probabilities to get the photon density (e.g. Zdziarski 1985; Pietrini & Krolik 1995). Such calculations can only give very approximate relations between the typical spectral shape and other parameters. Other approaches are to do detailed radiative transfer using Monte Carlo methods for geometries such as slabs or spheres (e.g. Hua & Titarchuk 1995) or to improve the analytical theory of Comptonization (e.g. Titarchuk 1994). Normally the following simplifications are made: 1) processes other than Comptonization are neglected, 2) pair balance is not imposed, 3) angle dependence of output spectra is not considered, 4) the soft photon injection is homogeneous throughout the source. There are some exceptions. For example, Skibo et al. (1995) includes bremsstrahlung, pair production, and pair balance, and Zdziarski et al. (1994) assume the soft photons to be injected at one of the slab surfaces. Most work neglect reflection by cold matter. The few papers considering polarized radiative transfer (e.g. Sunyaev & Titarchuk 1985; Haardt & Matt 1993) use the Rayleigh matrix, which is not valid for temperatures and photon energies
Juri Poutanen and Roland Svensson Stockholm Observatory, S-133 36 Saltsj¨ obaden, Sweden; juri@astro.su.se, svensson@astro.su.se ABSTRACT We consider two phase accretion disk-corona models for active galactic nuclei and some X-ray binaries. We describe in detail how one can exactly solve the polarized radiative transfer and Comptonization using the iterative scattering method, while simultaneously solving the energy and pair balance equation for both the cold and hot phases. We take into account Compton scattering, photon-photon pair production, pair annihilation, bremsstrahlung, and double Compton scattering, as well as edisk. We consider coronae having slab geometry as well as coronae consisting of one or more well separated active regions of cylinder or hemisphere geometry. The method is useful for determining the spectral intensity and the polarization emerging in different directions from disk-corona systems. The code is tested against a Monte-Carlo code. We also compare with earlier, less accurate, work. The method is more than an order of magnitude faster than applying Monte Carlo methods to the same problem and has the potential of being used in spectral fitting software such as XSPEC.
