新高二暑期培优讲义改错
第十一讲:连词与状语从句+题型综合练2021年新高二英语暑假精品课程(全国通用版)(解析版)

[高考考情分析]题型分类典题试做命题解读语法填空1.In much of Asia,especially the socalled “ricebowl” cultures of China,Japan,Korea,andVietnam,food is usually eaten with chopsticks.2.Over time,as/when the population grew,peoplebegan cutting food into small pieces so it wouldcook more quickly.1.并列连词(考查的重点:and,but,or,so,when,while);2.状语从句的引导词。
短文改错1.)Before getting into the car,I thought I had learnedthe instructor's orders,so→but/yet once I startedthe car...blank.2.If we stay at home,it is comfortable but→andthere is no need to spend money.3.Though not very big,but the restaurant is popularin our area.1.并列连词的错用或缺失;2.状语从句连接词的错用;3.并列连词和状语从句的连接词的同时使用错误。
[先试做题组]并列连词连词与状语从句+题型综合练第11讲Ⅰ.单句语法填空1.I am not afraid of tomorrow,for I have seen yesterday and I love today.2.He is a shy man,but he is not afraid of anything or anyone.Ⅱ.单句改错1.—Peter,please send us postcards for we'll know where you have visited.—No problem.for→so2.Although history can't be changed,but lessons can be learned to face the future.去掉but或but→yet[再解读要点]1.表并列、递进或顺承关系的并列连词:and,both ...and ...,neither ...nor ...,not only ...but also ...,as well asNot only can a beautiful smile make ourselves happy,but also it enables others to feel delighted.一个灿烂的笑容不仅能使我们自己高兴,而且能使他人感到愉快。
(人教版)2021年新高二英语暑假精品讲义08 倒装

作业081.Only when he reached the tea house ________ it was the same place he'd been in last year.A.he realized B.realized he C.did he realize D.he did realize2.________ both sides accept the agreement will a lasting peace be established in this region.A.As long as B.Only if C.If only D.Unless3.________ to the gift was a note on which he expressed his gratitude for our reception during his stay here. A.To attach B.Attaching C.Attached D.Having attached4.I am glad to find that on top of the mountain __________ from the time of the Roman Empire.A.lies ancient ruins B.lie ancient ruins C.does ancient ruins lie D.do ancient ruins lie5.________is the power of global warming that the climate goes from one extreme to the other.A.Such B.This C.That D.So6.He said before the whole class that only if we put our heart into our lessons _________ great progress. A.we had made B.we could makeC.could we make D.have we made7.On the ocean floor________. However, to bring them to the surface requires expensive equipment and trained people.A.do some sunken ship lie B.some sunken ships do lieC.lie some sunken ships D.some sunken ships lie8.---I can’t stand people killing so many endangered animals for money.---________ I think we should protect them instead.A.So can I.B.Neither I can.C.So I can.D.Neither can I.9.Under no circumstances________ in such a meaningless discussion.A.he participated B.he did participateC.did he participate D.participated he10.If Tom’s wife won’t go to the party,________.A.he will either B.neither will he C.he neither will D.either he will11.As the human population continues to grow, ________ does the effect we have on animals.12.Not until I returned________I realize that a quiet town life was the best for me.13.So touching________the song sound that I couldn’t hold my tears back when I heard it for the first time. 14.He jumped at the opportunity when he saw the advertisement in a newspaper, because barely________(can) he make ends meet.15.Only after one has become a parent________he realize how great his parents are.16.Never________it realistic for Europe to lay too much hope on China since the debt problem will only be solved by European countries themselves.17.Not until he failed a second time________ he begin to take my advice seriously.18.Only after Mary read her essay the second time________she notice the spelling mistake.19.I believe that when the power of love overcomes the love of power,________then will there be peace in this world20.Hardly ________(he, enter) the office when everyone proceeded to greet him merrily.If we are honest with ourselves, most of us have dreamed of 21.(be)rich and famous. Most musicians form a band because of the same interest 22.writing and playing music. They may play anywhere 23.(earn)some extra money as well as achieve their dreams. However, the Monkees, 24.started differently, 25.(begin) as a TV show with musicians playing jokes and music in the programme. Only after a year or so 26.they become more serious and they 27.(produce) their own records and later became even 28.(popular) than the Beatles in the USA.The band once 29.(break) up and then reunited. In memory 30.their former time as a real band, they produced a new record in 1996.1.C【详解】考查倒装句。
高考英语二轮精讲精练讲义 短文改错精讲

语鹅市安置阳光实验学校短文改错经典精讲(上)开篇语本讲在高中学习中的地位及考查形式:改错题型改错题型--题型改变短文改错题型的设置方式会有沿袭,但不再是一行一错或无错。
改为:短文中有10处语言错误,要求你在错误的地方增加、删除或者修改某个单词。
每处错误及其修改均仅限一词,而且只允许修改10处,多者(从第11处起)不计分。
这样的错误设置更接近于真实的文章改错,但是改错难度将明显增大。
改错题型--样卷第一节(10分,每改对一处计1分)As the high school student, I like to talk with foreigners to practise my oral English buta and learn more about other cultures. There were two foreign teachers in our school in this term. Theyareoften go to the English corner so that we can have a chance to practice the language. I seize every chance ∧ talk with them. However, I sometime find they are not so interesting in what I say,to sometimes interestedand it’s hard for I to choose a suitable topic. I would like some advices about how to communicateme adviceproper with foreigners, and what topics to pick.properly考查的词性有:冠词/连词/动词(考时态)/ 介词/不定式(to)/副词(2个)/代词/形容词(分词转化而来)/不可数名词。
高二数学培优补差记录

班级11-3 时间辅导教师类别学生姓名训练内容1.回归直线:如果散点图中点的分布从整体上看大致在一条直线附近,我们就称这两个变量之间具有线性相关关系,这条直线叫作回归直线。
2.回归分析:对具有相关关系的两个变量进行统计分析的一种常用方法。
建立回归模型的基本步骤是:①确定研究对象,明确哪个变量是解释变量,哪个变量是预报变量;②画好确定好的解释变量和预报变量的散点图,观察它们之间的关系(线性关系).③由经验确定回归方程的类型.④按一定规则估计回归方程中的参数(最小二乘法);⑤得出结论后在分析残差图是否异常,若存在异常,则检验数据是否有误,后模型是否合适等.3.利用统计方法解决实际问题的基本步骤:(1)提出问题;(2)收集数据;(3)分析整理数据;(4)进行预测或决策。
4.残差变量的主要来源:(1)用线性回归模型近似真实模型(真实模型是客观存在的,通常我们并不知道真实模型到底是什么)所引起的误差。
可能存在非线性的函数能够更好地描述与之间的关系,但是现在却用线性函数来表述这种关系,结果就会产生误差。
这种由于模型近似所引起的误差包含在中。
(2)忽略了某些因素的影响。
影响变量的因素不只变量一个,可能还包含其他许多因素(例如在描述身高和体重关系的模型中,体重不仅受身高的影响,还会受遗传基因、饮食习惯、生长环境等其他因素的影响),但通常它们每一个因素的影响可能都是比较小的,它们的影响都体现在中。
效果班级11-3 时间辅导教师类别学生姓名训练内容例题:研究某灌溉渠道水的流速与水深之间的关系,测得一组数据如下:水深 1.40 1.50 1.60 1.70 1.80 1.90 2.00 2.10流速 1.70 1.79 1.88 1.95 2.03 2.10 2.16 2.21 (1)求对的回归直线方程;(2)预测水深为1.95时水的流速是多少?分析:本题考查如何求回归直线的方程,可先把有关数据用散点图表示出来,若这些点大致分布在通过散点图中心的一条直线附近,说明这两个变量线性相关,从而可利用我们学过的最小二乘估计思想及计算公式求得线性回归直线方程。
2024年高考数学复习培优讲义专题2-指对同构(朗博同构)(含解析)
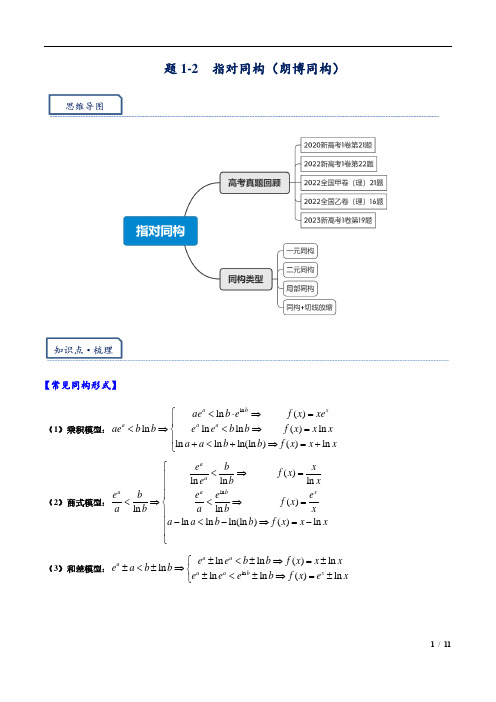
题1-2 指对同构(朗博同构)【常见同构形式】(1)乘积模型:ln ln ()ln ln ln ()ln ln ln ln(ln )()ln a b x aa a aeb e f x xe ae b b e e b b f x x x a a b b f x x x ⎧<⋅⇒=⎪<⇒<⇒=⎨⎪+<+⇒=+⎩(2)商式模型:ln ()ln ln ln ()ln ln ln ln ln(ln )()ln a aa ab x e b xf x e b x e b e e e f x a b a b x a a b b f x x x ⎧<⇒=⎪⎪⎪<⇒<⇒=⎨⎪−<−⇒=−⎪⎪⎩(3)和差模型:ln ln ln ()ln ln ln ln ()ln a a aaa b xe e b bf x x xe a b b e e e bf x e x ⎧±<±⇒=±±<±⇒⎨±<±⇒=±⎩【六大超越函数图像】(6)2020新高考1卷21(2)1.已知函数1()ln x f x ae x lna −=−+,若f (x )≥1,求a 的取值范围.2022新高考1卷第22题2.已知函数()x f x e x =−和()ln g x x x =−,证明:存在直线y b =,其与两条曲线()y f x =和()y g x =共有三个不同的交点,并且从左到右的三个交点的横坐标成等差数列.2022全国甲卷(理)21题3.已知函数()ln xf x x a xx e −=+−.(1)若()0f x ≥,求a 的取值范围;(2)证明:若()f x 有两个零点12,x x ,则121x x <.2023新高考1卷T19(2) 同构+切线放缩或2次求导4.已知函数()()x f x a e a x =+−,证明:当a >0时,3()2ln 2f x a >+.2022全国乙卷(理)16题5.已知1x x =和2x x =分别是函数2()2e x f x a x =−(0a >且1a ≠)的极小值点和极大值点.若12x x <,则a的取值范围是 .题型一 一元同构2023深圳高二下期末·21(2)1.已知2()()x f x axe a R =∈,若关于x 的()2ln 0f x x x −−≥恒成立,求实数a 的取值范围.重点题型·归类精讲2.若关于x 的不等式ln ln 0e xx a a xx+−>对()0,1x ∀∈恒成立,则实数a 的取值范围为( ) A .1,e ⎛⎤−∞ ⎥⎝⎦B .1,e ∞⎡⎫+⎪⎢⎣⎭C .1,1e ⎡⎫⎪⎢⎣⎭D .10,e ⎛⎤ ⎥⎝⎦宁波九校高三上期末·22(2) 3.已知函数1()ln 2f x x x x x ⎛⎫=+− ⎪⎝⎭,e 是自然对数的底数.若不等式2()(1)4axf x a e x ≤+−对0x ∀>恒成立,求实数a 的取值范围.江苏盐城2023届高三5月三模·22 4.已知函数()(ln ).x a f x e e a x =−+ (1)当a =1时,求()f x 的单调递增区间; (2)()0f x ≥恒成立,求a 的取值范围.湖南九校联盟第二次联考·16 5.已知不等式))(1ln (0xa x e a a e −⎡⎤≥>⎢⎥⎣⎦恒成立,则实数a 的最大值为_______湖南省2023届高三下3月考试·16 6.已知e 是自然对数的底数.若()0x ∀∈+∞,,e ln mx m x ≥成立,则实数m 的最小值是 .7.若不等式0x ae lnx lna −+恒成立,则a 的取值范围是( )A .1[,)e +∞B .2[,)e +∞C .[,)2e+∞D .[e ,)+∞湖北鄂东南联考 ·88.已知函数()ln x f x x x xe k −=−−−恒有零点,则实数k 的取值范围是( )A .(],1−∞−B .1,1e⎛⎤−∞−− ⎥⎝⎦C .11,1e⎡⎤−−−⎢⎥⎣⎦D .11,0e⎡⎫−−⎪⎢⎣⎭福建龙岩九校联考·16 9.已知函数mx x m x f −+=)1ln()(,若不等式x e x x f −+>1)(在()+∞,0上恒成立,则实数m 的取值范围是____________ .湖南常德3月模拟10.已知不等式ln()x x a e a +≤−对[1,)x ∀∈+∞恒成立,则a 的取值范围为 .浙江省衢州、丽水、湖州三地市2023高三下学期4月教学质量检测·8 11.对任意的实数0x >,不等式22ln ln 0x ae x a −+≥恒成立,则实数a 的最小值为( )e2eC.2eD.12e2022湖北四地七校高二下期中·712.已知实数a >0,不等式()0x e aln ax ->恒成立,则a 的取值范围是( ) A .1<<a e eB .0<a <1C .0<a <eD .a >e湖南郴州高二下期末·16 13.函数.若对任意,都有,则实数m 的取值范围为_________.2023湖南邵阳二模·8 14.若不等式()1e 1ln 10txt x x ⎛⎫−−−≥ ⎪⎝⎭对任意[)2e 1,x ∞∈++恒成立,则正实数t 的取值范围是( )A. ln2,2e 1∞⎡⎫+⎪⎢+⎣⎭B. ln21,2e 1∞+⎡⎫+⎪⎢+⎣⎭C. ln210,2e 1+⎛⎫ ⎪+⎝⎭ D. ln2ln21,2e 12e 1+⎡⎤⎢⎥++⎣⎦15.已知函数ln 0x f xe a ax a a a ,若关于x 的不等式0f x恒成立,则实数a 的取值范围为( ) A .],0(eB .],0(2eC .],1[2eD .),1(2e()()()e1ln R mxf x m x x m =+−−∈0x >()0f x ≥16.关于x 的不等式ln 1axx e xe a x x−≤−−恒成立,则a 的取值范围为 .2022衡阳市八中高二期末·16 17.已知函数1()(0)a x f x x alnx x a e=++−<,若()0f x 在[2x ∈,)+∞上恒成立,则实数a 的取值范围为 . 2023届郴州三模·1618.设实数0m >,若对任意的21x e ∞⎛⎫∈+ ⎪⎝⎭,,不等式ln 1mx mx x e e m m mx−≥−恒成立,则实数m 的取值范围为 .湖北省部分学校高三下5月适应性考试·14 19.对于任意实数0x >,不等式22e ln ln 0x a x a −+≥恒成立,则a 取值范围是__________.2023·广东惠州·一模T22(2)20.已知函数()2ln f x x a x =−,若函数()(2)e x f x a x x ≥+−恒成立,求实数a 的取值范围.2023·广东深圳·南山区高三上期末联考·22 21.已知定义在()0,∞+上的函数()e ax f x x =. (1)若R a ∈,讨论()f x 的单调性;(2)若0a >,且当()0,x ∈+∞时,不等式2e ln aax xx ax ⎛⎫≥⎪⎝⎭恒成立,求实数a 的取值范围.2023·广东汕头·一模T2222.已知函数()e ln(2)ln 2x f x a x a =−++−.(1)若函数()f x 在2023x =处取得极值,求a 的值及函数的单调区间; (2)若函数()f x 有两个零点,求a 的取值范围.的题型二 二元同构2022届山东聊城一模·823.已知正数x ,y 满足ylnx +ylny =e x ,则xy ﹣2x 的最小值为( ) A .1122n B .222ln ﹣ C .1122n −D .222ln +24.实数x ,y 满足ln ln xe y x y y =+,则2ln xe y x−的最小值为________2022届T8第一次联考·825.设a ,b 都为正数,e 为自然对数的底数,若1a ae b blnb ++<,则( ) A .ab e >B .1a b e +>C .ab e <D .1a b e +<2023茂名市高三一模·1226.(多选)e 是自然对数的底数,,m n ∈R ,已知e ln ln m m n n n m +>+,则下列结论一定正确的是( ) A .若0m >,则0m n −> B .若0m >,则e 0m n −> C .若0m <,则ln 0m n +< D .若0m <,则e 2m n +>河北省衡水中学2023届高三下学期第三次综合素养评价·16 27.若正实数a ,b 满足()1ln ln e a a b a a b −−+≥,则1ab的最小值为 .28.设11110e ,11ln1.111a b ==,则( )A .1ab a <<B .1ab b <<C .1a ab <<D .1b ab <<题型三 局部同构华大新高考五月押题卷·1229.(多选)已知0λ>,若关于x 的方程()1ln 0x e x x xλλλ−−+=存在正零点,则实数λ的值可能为A .1eB .12C .eD .230.已知函数1ln )(−−=x ae x f x ,若0)(≥x f 恒成立,则实数a 的取值范围是 .2023·广东·海珠区高三2月联考·22 31.已知函数()()1e 02x f x ax a =−≠. (1)讨论函数()f x 的单调性; (2)已知函数()()ln xg x f x x=−有两个零点,求实数a 的取值范围.2023·广东3月·中学生标准学术能力诊断测试联考模拟预测T22(2) 部分同构+放缩 32.设()()e xxf x x =∈R ,若(e )()(ln 1)x f x k x ⋅≤⋅+在()1,x ∈+∞上恒成立,求k 的取值范围.2023·广东·深圳中学5月适应性测试T22(1) 部分同构33.已知函数()e ln xf x ax a x x =−−,若不等式()0f x <恒成立,求实数a 的取值范围.题型四 同构+切线放缩2023佛山一模T1134.(多选)若正实数x ,y 满足()1e 1ln x x y y −=+,则下列不等式中可能成立的是( )A .1x y <<B .1y x <<C .1x y <<D .1y x <<巴蜀中学2023届高考适应性月考卷(八)T8——局部构造+切线放缩35.已知函数22ln 1()e x x f x x a x+=−−,当()0,x ∈+∞时,()0f x ≥恒成立,则实数a 的取值范围是( ) A .(2,e 1⎤−∞−⎦B .(],e −∞C .(],2−∞D .(],1−∞2023届湖南四大名校5月“一起考”T736.若当π0,2x ⎛⎫∈ ⎪⎝⎭时,关于x 的不等式2e cos cos lncos 1x x x x x ax −++≥恒成立,则满足条件的a 的最小整数为( ) A. 1 B. 2 C. 3 D. 437.(2023·广东珠海·高三联考模拟考试)已知函数()()()()ln 2R ,e 1xf x x ax ag x x x a x =−−∈=−−+.(1)求函数()f x 的单调区间;(2)若不等式()()f x g x ≤恒成立,求实数a 的取值范围.38.(2023·广东·统考一模)已知函数()1e x f x x +=.(1)求()f x 的极值; (2)当0x >时,()()1ln 2f x a x x ≥+++,求实数a 的取值范围.补充练习杭州一模(高三上期末)T16——同构有一定难度,函数分析也比较麻烦1.已知不等式()ln ln 10,1()xa a a x a a >−>≠对)1,(x ∀∈+∞恒成立,a 的取值范围是________.2023湖北高三九师联盟1月·82.已知a >b >1,若1a a b e be ae a ++=+,则 A .ln(a +b )>1B .ln(a -b )<0C .333a b −+<D .133a b −<湖北名校联合体高三下学期开学考·163.已知关于x 的不等式()1ln 2x e a a ax a −+>−(0)a >恒成立,则实数a 的取值范围为________.4.对0x ∀>,恒有()112ln axa e x x x ⎛⎫+≥+⎪⎝⎭,则实数a 的最小值为________.专题1-2 指对同构(朗博同构)【常见同构形式】(1)乘积模型:ln ln ()ln ln ln ()ln ln ln ln(ln )()ln a b x aa a aeb e f x xe ae b b e e b b f x x x a a b b f x x x ⎧<⋅⇒=⎪<⇒<⇒=⎨⎪+<+⇒=+⎩(2)商式模型:ln ()ln ln ln ()ln ln ln ln ln(ln )()ln a aa ab x e b xf x e b x e b e e e f x a b a b x a a b b f x x x ⎧<⇒=⎪⎪⎪<⇒<⇒=⎨⎪−<−⇒=−⎪⎪⎩(3)和差模型:ln ln ln ()ln ln ln ln ()ln a a aaa b xe e b bf x x xe a b b e e e bf x e x⎧±<±⇒=±±<±⇒⎨±<±⇒=±⎩【六大超越函数图像】(6)2020新高考1卷21(2)1.已知函数1()ln x f x ae x lna −=−+,若f (x )≥1,求a 的取值范围.【答案】[)1+∞, [方法一]:【最优解】:同构由()1f x ≥得1e ln ln 1x a x a −−+≥,即ln 1ln 1ln a x e a x x x +−++−≥+,而ln ln ln x x x e x +=+,所以ln 1ln ln 1ln a x x e a x e x +−++−≥+.令()m h m e m =+,则()10m h m e +'=>,所以()h m 在R 上单调递增.由ln 1ln ln 1ln a x x e a x e x +−++−≥+,可知(ln 1)(ln )h a x h x +−≥,所以ln 1ln a x x +−≥,所以max ln (ln 1)a x x ≥−+. 令()ln 1F x x x =−+,则11()1xF x x x−'=−=. 所以当(0,1)x ∈时,()0,()F x F x '>单调递增; 当(1,)x ∈+∞时,()0,()F x F x '<单调递减. 所以max [()](1)0F x F ==,则ln 0a ≥,即1a ≥. 所以a 的取值范围为1a ≥. [方法二]:换元同构由题意知0,0a x >>,令1x ae t −=,所以ln 1ln a x t +−=,所以ln ln 1a t x =−+. 于是1()ln ln ln ln 1x f x ae x a t x t x −=−+=−+−+.由于()1,ln ln 11ln ln f x t x t x t t x x ≥−+−+≥⇔+≥+,而ln y x x =+在,()0x ∈+∞时为增函数,故t x ≥,即1x ae x −≥,分离参数后有1x xa e −≥.令1()x x g x e −=,所以1112222(1)()x x x x x e xe e x g x e e −−−−−−−=='. 当01x <<时,()0,()g x g x >'单调递增;当1x >时,()0,()g x g x <'单调递减. 所以当1x =时,1()x x g x e−=取得最大值为(1)1g =.所以1a ≥.[方法三]:通性通法1()ln ln x f x ae x a −=−+,11()x f x ae x−'∴=−,且0a >.设()()g x f x =',则121()0,x g x ae x −'=+> ∴g(x)在(0,)+∞上单调递增,即()f x '在(0,)+∞上单调递增, 当1a =时,()01f '=,∴()()11min f x f ==,∴()1f x ≥成立.当1a >时,11a < ,111a e −<∴,111()(1)(1)(1)0a f f a e a a−''∴=−−<,∴存在唯一00x >,使得01001()0x f x ae x −'=−=,且当0(0,)x x ∈时()0f x '<,当0(,)x x ∈+∞时()0f x '>,0101x ae x −∴=,00ln 1ln a x x ∴+−=−, 因此01min 00()()ln ln x f x f x ae x a −==−+000011ln 1ln 2ln 122ln 1a x a a x a x x =++−+≥−+⋅=+>1, ∴()1,f x >∴()1f x ≥恒成立;当01a <<时, (1)ln 1,f a a a =+<<∴(1)1,()1f f x <≥不是恒成立. 综上所述,实数a 的取值范围是[1,+∞). [方法四]:因为定义域为(0,)+∞,且()1f x ≥,所以(1)1f ≥,即ln 1a a +≥. 令()ln S a a a =+,则1()10S a a='+>,所以()S a 在区间(0,)+∞内单调递增. 因为(1)1S =,所以1a ≥时,有()(1)S a S ≥,即ln 1a a +≥. 下面证明当1a ≥时,()1f x ≥恒成立.令1()ln ln x T a ae x a −=−+,只需证当1a ≥时,()1T a ≥恒成立. 因为11()0x T a ea−=+>',所以()T a 在区间[1,)+∞内单调递增,则1min [()](1)ln x T a T e x −==−. 因此要证明1a ≥时,()1T a ≥恒成立,只需证明1min [()]ln 1x T a e x −=−≥即可.由1,ln 1x e x x x ≥+≤−,得1,ln 1x e x x x −≥−≥−.上面两个不等式两边相加可得1ln 1x e x −−≥,故1a ≥时,()1f x ≥恒成立. 当01a <<时,因为(1)ln 1f a a =+<,显然不满足()1f x ≥恒成立.所以a 的取值范围为1a ≥.【整体点评】(2)方法一:利用同构思想将原不等式化成ln 1ln ln 1ln a x x e a x e x +−++−≥+,再根据函数()m h m e m =+的单调性以及分离参数法即可求出,是本题的最优解;方法二:通过先换元,令1x ae t −=,再同构,可将原不等式化成ln ln t t x x +≥+,再根据函数ln y x x =+的单调性以及分离参数法求出;方法三:利用导数判断函数()f x 的单调性,求出其最小值,由min 0f ≥即可求出,解法虽稍麻烦,但是此类题,也是本题的通性通法;方法四:由特殊到一般,利用(1)1f ≥可得a 的取值范围,再进行充分性证明即可2022新高考1卷第22题2.已知函数()x f x e x =−和()ln g x x x =−,证明:存在直线y b =,其与两条曲线()y f x =和()y g x =共有三个不同的交点,并且从左到右的三个交点的横坐标成等差数列. 【解答】易得()f x 在()0,+∞↑,(),0−∞↓;()g x 在()0,1↓,()1,+∞↑只有y b =过()f x 与()g x 交点时,恰有3个不同交点 则有1223()()()()f x f x g x g x b ====,即12122233ln ln x xe x e x x x x x b −=−=−=−= ①∵111122ln ln xxxe x e e x x −==−− ,且1211,xe x <<,∴1212ln xe x x x =⇒= ② 又∵32ln 3332ln ln x x x x ex e x −=−=− ,且3200ln ,x x >>,∴2323ln x x x x e =⇒= ③由①②③可得:()()2132222ln 2xx x e x b x x b x +=+=++−=,证毕2022全国甲卷(理)21题3.已知函数()ln xf x x a x x e −=+−.(1)若()0f x ≥,求a 的取值范围;(2)证明:若()f x 有两个零点12,x x ,则121x x <. 【详解】(1)[方法一]:同构处理 由()0f x ≥得:ln ln 0x x e x x a −++−−≥令ln ,1t x x t −=≥,则()0tf t e t a =+−≥即t a e t ≤+ 令()[),1,tg t e t t =+∈+∞,则()'10tg t e =+>故()tg t e t =+在区间[)1,+∞上是增函数故()()min 11g t g e ==+,即1a e ≤+ 所以a 的取值范围为(,1]e −∞+ [方法二]:常规求导()f x 的定义域为(0,)+∞,则2111()1x f x e x x x ⎛⎫'=−−+ ⎪⎝⎭1111111x x x e e x x x x x ⎛⎫−⎛⎫⎛⎫=−+−=+ ⎪ ⎪ ⎪⎝⎭⎝⎭⎝⎭令()0f x '=,得1x =当(0,1),()0,()x f x f x '∈<单调递减当(1,),()0,()x f x f x >'∈+∞单调递增()(1)1f x f e a ≥=+−, 若()0f x ≥,则10e a +−≥,即1a e ≤+ 所以a 的取值范围为(,1]e −∞+ (2)法一:极值点偏移+同构简化计算由题知,()f x 一个零点小于1,一个零点大于1,不妨设121x x ,要证121x x <,即证121x x <因为121,(0,1)x x ∈,即证()121f x f x ⎛⎫> ⎪⎝⎭, 又因为()()12f x f x =,故只需证()221f x f x ⎛⎫> ⎪⎝⎭,即证11ln ln 0,(1,)x x e x x xe x x x x −+−−−>∈+∞同构,原不等式变形为:()1ln ln 1ln ln x x xxex x ex x+−++−>+ 令()xg x e x =+,则有1(ln )ln g x x g x x ⎛⎫−>+⎪⎝⎭即证:)1ln ln ,(1,x x x x x−>∈+∞+ 即证1()2ln 0(1,,)h x x x xx =+∈<+∞− ()()222121'()10,1x h x x x x x−−=−−=<>,即()h x 递减,故()(1)0h x h <=,证毕. [方法二]:对数平均不等式由题意得:()ln x xe ef x a x x=+−令1xe t x=>,则()ln f t t t a =+−,()1'10f t t =+>所以()ln g t t t a =+−在()1,+∞上单调递增,故()0g t =只有1个解又因为()ln x xe ef x a x x =+−有两个零点12,x x ,故1212x x e e t x x == 两边取对数得:1122ln ln x x x x −=−,即12121ln ln x x x x −=−()121212*ln ln x x x x x x −<−121x x <,即121x x <()121212*ln ln x x x x x x −<−121211212121222112ln ln ln ln ln x x xx xx x x x x x x x x x x −<⇔−⇔<−不妨设121x t x =>,则只需证12ln t t t <−构造()12ln ,1h t t t t t =−+>,则()22211'110h t t t t ⎛⎫=−−=−−< ⎪⎝⎭故()12ln h t t t t=−+在()1,+∞上单调递减故()()10h t h <=,即12ln t t t<−得证2023新高考1卷T19(2) 同构+切线放缩或2次求导4.已知函数()()x f x a e a x =+−,证明:当a >0时,3()2ln 2f x a >+. 解:即证:当a >0时,232ln 2xae a x a +−>+第一步,指数化,同构变形:()ln 2ln 2332ln ln ln 22a xa x ea x a e a x a a +++−>+⇒−+>−+ 第二步,换元:令ln t a x =+,t ∈R ,有23ln 2te t a a −>−+ 第三步,放缩:1t e t −≥(证明略),即证231ln 2a a >−+第四步,构造函数:令23()ln 2g a a a =−+,1'()2g a a a =−,故()g a 在202⎛⎫↑ ⎪ ⎪⎝⎭,,2,2⎫+∞↓⎪⎢⎪⎣⎭22132()ln ln 1122222g a g ⎛≤=−+=+< ⎝⎭2022全国乙卷(理)16题5.已知1x x =和2x x =分别是函数2()2e x f x a x =−(0a >且1a ≠)的极小值点和极大值点.若12x x <,则a的取值范围是 .【答案】1,1e ⎛⎫⎪⎝⎭【详解】[方法一]:转化法,零点的问题转为函数图象的交点因为()2ln 2e xf x a a x '=⋅−,所以方程2ln 2e 0x a a x ⋅−=的两个根为12,x x ,即方程ln e x a a x ⋅=的两个根为12,x x ,即函数ln x y a a =⋅与函数e y x =的图象有两个不同的交点,因为12,x x 分别是函数()22e x f x a x =−的极小值点和极大值点,所以函数()f x 在()1,x −∞和()2,x +∞上递减,在()12,x x 上递增, 所以当时()1,x −∞()2,x +∞,()0f x '<,即e y x =图象在ln x y a a =⋅上方 当()12,x x x ∈时,0fx,即e y x =图象在ln x y a a =⋅下方1a >,图象显然不符合题意,所以01a <<.令()ln x g x a a =⋅,则()2ln ,01xg x a a a '=⋅<<,设过原点且与函数()y g x =的图象相切的直线的切点为()00,ln x x a a⋅,则切线的斜率为()020ln x g x a a '=⋅,故切线方程为()0020ln ln x x y a a a a x x −⋅=⋅−,则有0020ln ln x x a a x a a −⋅=−⋅,解得01ln x a=,则切线的斜率为122ln ln eln a a a a ⋅=, 因为函数ln x y a a =⋅与函数e y x =的图象有两个不同的交点,所以2eln e a <,解得1e e a <<,又01a <<,所以11ea <<,综上所述,a 的取值范围为1,1e ⎛⎫⎪⎝⎭.[方法二]:【通性通法】构造新函数,二次求导 ()2ln 2e x f x a a x '=⋅−=0的两个根为12,x x因为12,x x 分别是函数()22e x f x a x =−的极小值点和极大值点,所以函数()f x 在()1,x −∞和()2,x +∞上递减,在()12,x x 上递增,设函数()()()g 2ln xx f x a a ex '==−,则()()2g 2ln 2x x a a e '=−,若1a >,则()g x '在R 上单调递增,此时若()0g 0x '=,则()f x '在()0-,x ∞上单调递减,在()0,x +∞上单调递增,此时若有1x x =和2x x =分别是函数()22(0x f x a ex a =−>且1)a ≠的极小值点和极大值点,则12x x >,不符合题意;若01a <<,则()g x '在R 上单调递减,此时若()0g 0x '=,则()f x '在()0,x −∞上单调递增,在()0,x +∞上单调递减,令()0g 0x '=,则02(ln )xea a =,此时若有1x x =和2x x =分别是函数()22(0x f x a ex a =−>且1)a ≠的极小值点和极大值点,且12x x <,则需满足()00f x '>,()()00002ln 20ln xe f x a a ex ex a ⎛⎫'=−=−> ⎪⎝⎭,即001ln 1ln x x a a <>,故()002ln ln ln 1ln x e a x a a ==>,所以11ea <<. [方法三]:同构+放缩(简证) ① 先得出01a << ② ()ln ln 2ln ln ln ln x a xx ae ea a ex ea ex x a a ⋅=⇒⋅=⇒=(ln 0x a >)③ 放缩:xxe e ex e x≥⇒≥()()221ln 11ln 01ln ee a a a ea >⇒<⇒−<<⇒<<题型一 一元同构2023深圳高二下期末·21(2)1.已知2()()x f x axe a R =∈,若关于x 的()2ln 0f x x x −−≥恒成立,求实数a 的取值范围.【答案】1a e≥【简证】()2ln 0f x x x −−≥恒成立等价于()22ln 0xaxe x x −−≥恒成立,即()()ln 2ln 22ln 2ln 0x xx x aee x x ae x x +−+=−+≥,则有ln 22ln x xx xa e++≥令2ln t x x =+,t ∈R ,则有max1t t a e e ⎛⎫≥=⎪⎝⎭(构造函数求导得出最值,过程略) 总结:同构+分参2.若关于x 的不等式ln ln 0e xx a a xx+−>对()0,1x ∀∈恒成立,则实数a 的取值范围为( ) A .1,e ⎛⎤−∞ ⎥⎝⎦B .1,e ∞⎡⎫+⎪⎢⎣⎭C .1,1e ⎡⎫⎪⎢⎣⎭D .10,e ⎛⎤ ⎥⎝⎦【答案】B【分析】由题意可知0a >,且ln e ln e xx a xa x >对()0,1x ∀∈恒成立,设()ln x g x x =,则问题转化为()()e xg a g x >在()0,1上恒成立,利用导数说明函数的单调性,再分e 1x a ≥和0e 1x a <<两种情况讨论,结合函数的取值情况及单调性,分别计算可得.重点题型·归类精讲【详解】由题意可知0a >,ln e ln ln e x x a a x x +>,即ln e ln e x x a xa x >对()0,1x ∀∈恒成立. 设()ln x g x x =,则问题转化为()()e xg a g x >在()0,1上恒成立,因为()21ln xg x x−'=,所以当0e x <<时,()0g x '>,当e x >时,()0g x '<, 所以()g x 在()0,e 上单调递增,在()e,+∞上单调递减,又()10g =,所以当()0,1x ∈时,()0g x <;当()1,x ∈+∞时,()0g x >. ①在()0,1x ∈上,若e 1x a ≥恒成立,即1a ≥,()()e0xg a g x ≥>;②在()0,1x ∈上,若0e 1x a <<,则e x a x >恒成立,即1e xxa <<恒成立, 令()e x x h x =,()0,1x ∈,则()10ex xh x −'=>,所以()h x 在()0,1上单调递增, 所以()()11e h x h <=,所以11e a <≤,综上所述,实数a 的取值范围为1,e ∞⎡⎫+⎪⎢⎣⎭.故选:B .宁波九校高三上期末·22(2) 3.已知函数1()ln 2f x x x x x ⎛⎫=+− ⎪⎝⎭,e 是自然对数的底数.若不等式2()(1)4axf x a e x ≤+−对0x ∀>恒成立,求实数a 的取值范围. 【答案】实数a 的取值范围为2,e ⎡⎫+∞⎪⎢⎣⎭.12()(1)42ln 4(1)4ax ax f x a e x x x x a e x x ⎛⎫≤+−⇒+−≤+− ⎪⎝⎭,整理,同乘x 得:()2212ln (1)1ln (1)ax axx x a e x x ax e x ⎛⎫+≤+⇒+≤+ ⎪⎝⎭, 比较一下2种构造方式,方式1:令()x g x xe x =+,()'()11xg x x e =++,易错:由洛必达可知(选填时用)——这里用不了错了!()111lim 1lim 0x x x x x x x e e e −−→−∞→−∞+−∞+=====−+∞−−∞,故()'()110()xg x x e g x =++>⇒↑()11'()111x xx xx x e g x x e e e−−−+++=++=+=,令()1xh x e x =−+,易知()h x ≥2恒成立, 故()11()0'()0()xx x e e x h x g x g x −−++=−−++=−>⇒>⇒↑由()2222ln 21ln (1)ln ln axx ax x x ax e x ex axe ax +≤+⇒+≤+,则有2(ln )()g x g ax ≤,由单调性可知22min ln 2ln x x ax a x e⎛⎫≤⇒≥= ⎪⎝⎭参考ln xy x=图像可以快速得出答案,解答题还是要写一下求导过程. 方式2:()ln g x x x x =+总结:(1)求导通分看极值点即可,注意2个增区间之间用“,”而不是“∪”(2)先同构再判断单调性. 江苏盐城2023届高三5月三模·22 4.已知函数()(ln ).x a f x e e a x =−+ (1)当a =1时,求()f x 的单调递增区间; (2)()0f x ≥恒成立,求a 的取值范围.【答案】(1)()1,+∞(2)(,1]−∞(1)解:当时,,,又,单调递增, ··············································· 2分 又,当时,当时,∴的单调递增区间为()1,+∞. ·························································· 4分 1a =()()1ln x f x e e x =−+()xe f x e x'=−()20xef x e x ''=+>()f x '∴()10f '=∴()0,1x ∈()0f x '<()1,x ∈+∞()0f x '>()f x(2)若恒成立,即恒成立.方法1:,,令, 则,在上单调递增,又,当时,故存在唯一正实数使得, ····················································· 6分 当时,,单调递减,当时,,单调递增,,由恒成立,得,由得,, ······ 8分 ∴,∴,∴,设,则恒成立,故在上递增,而,∴, 又且函数在上是增函数,故的取值范围为. ···································································· 12分 法2:同法一得,由得,∴ ,,故的取值范围为. ················· 12分方法3:令,则,,则,令,则, ················································ 8分 ∵,∴在上单调递增,当时,显然成立;当时,恒成立,即恒成立,可证(过程略),,,即,,综上,的取值范围为(,1]−∞. ······························································ 12分 ()0f x ≥()ln 0x ae e a x −+≥()ln x a af x e e x e a =−−()a x a x e xe e f x e x x−'=−=()x ag x xe e =−()0x x g x e xe '=+>()x ag x xe e ∴=−()0,+∞()00ag e =−<x →+∞()g x →+∞0x 00x a x e e =0x x <()0f x '<()f x 0x x >()0f x '>()f x ()()000min ln x a a f x f x e e x e a ∴==−−()0f x ≥()min 0f x ≥00x a x e e =00ln x x a +=()()00000min (2ln )0x xf x f x e x e x x ∴==−+≥0001(2ln )0x x x −+≥000(2ln )10x x x +−≤00012ln 0x x x +−≤1()2ln h x x x x=+−221()10h x x x '=++>()h x (0,)+∞(1)0h =001x <≤00ln x x a +=ln y x x =+(0,1]a (,1]−∞()()000min ln x a af x f x e e x e a ==−−00x a x e e =00ln x x a +=()000min00011ln ln aa a a a a a e f x e x e a e x e a e x a e a x x x ⎛⎫⎛⎫=−−=−−=+−− ⎪ ⎪⎝⎭⎝⎭()20a a e a e a ≥−−≥()220a e a ∴−≥a (,1]−∞a e t =ln a t =()()ln ln ln x e t t x t tx ≥+=()()()ln ln ln tx xxe tx tx tx e ≥=()(0)xg x xe x =>()()ln()g x g tx ≥()()10x g x x e '=+>()(0)xg x xe x =>()0,+∞()ln 0tx ≤()()ln()g x g tx ≥()ln 0tx >()ln ln ln x tx t x ≥=+ln ln t x x ≤−ln 1x x −≥∴ln 1t ≤∴t e ≤a e e ≤∴1a ≤a方法4:∵恒成立,∴,即,同法3考查函数可得, ··········································· 7分 反之,当时,, 又可证(过程略),∴,∴恒成立,故的取值范围为. ···································································· 12分 补充:同构和型+放缩ln (ln )0(ln )ln ln ln x a x a x a x a x e x x e a x e e a x e a x e x x a e −−−+≥⇒≥+⇒−≥+⇒+≥+=+令()x g x e x =+↑,则有()min ()(ln )ln ln 1g x a g x x a x a x x −≥⇒−≥⇒≤−=总结:(1)两次求导+取点(2)法一和法二是整体求导再用隐零点处理,法三和法四是同构处理相对简单 湖南九校联盟第二次联考·16 5.已知不等式))(1ln (0xa x e a a e −⎡⎤≥>⎢⎥⎣⎦恒成立,则实数a 的最大值为_______ 【答案】2e[]ln ln (1)lnln (1)1ln ln(1)1ln ln(1)1x x x a x a a x e a e a a x e a x x x e a x e−−−≥⇒≥−−−+⇒≥+−⇒−+−≥−令()x g x e x =+↑,则有()2(ln )ln(1)ln ln(1)ln(1)ln 2ln g x a g x x a x x x a a e a −≥−⇒−≥−⇒−−≥⇒≥⇒≥可放缩补充:构造函数求导令ln(1)()g x x x −−=,12()111x g x x x '−=−=−− 故g (x )在(1,2)上单调递减,在(2,+∞)上单调递增,因此min ()(2)2g x g ==. 因为不等式(1)ln(0)xa x e a a e−≥>恒成立,所以Ina ≤2,即2.a e ≤ 总结:指对分离,补全结构,最后的最值可以放缩得出. 补充:对右边的式子配凑也可以()0f x ≥(1)0f ≥a e e a ≥()(0)xg x xe x =>1a ≤1a ≤11x a a x −+≥+−ln 1,1x a x x e x a −≤−≥−+ln x a e a x −≥+()ln x ae e a x ≥+a (,1]−∞湖南省2023届高三下3月考试·166.已知e 是自然对数的底数.若()0x ∀∈+∞,,e ln mx m x ≥成立,则实数m 的最小值是 . 【答案】1e解析:由ln e ln e ln ln mx mx x m x mx x x e x ≥⇒≥=⋅.令()e x f x x =,则()f x 在()0+∞,上单调递增, 且()()ln f mx f x ≥,所以ln mx x ≥,即ln xm x≥对()0x ∀∈+∞,恒成立. 令()ln xg x x =,则()21ln x g x x−'=,所以当()0e x ∈,时,()0g x '>;当()e x ∈+∞,时,()0g x '<, 故()g x 在[)1+∞,上的最大值是1e ,所以1e m ≥,即实数m 的最小值是1e .故答案为:1e. 总结:同乘补全结构即可,入门型7.若不等式0x ae lnx lna −+恒成立,则a 的取值范围是( )A .1[,)e +∞B .2[,)e +∞C .[,)2e+∞D .[e ,)+∞【答案】A 【法一】:同构ln ln ln ln ln 0ln ln ln ln ln x a x a x x ae x a e e a x e a x x x e x +⇒+−+≥⇒≥+≥=+++构造函数()x g x e x =+,故ln ln ln ln (ln )(ln )a x x e a x e x g a x g x ++≥++≥+⇒ 而'()10x g x e =+>,则ln ln a x x +≥,即()max ln ln a x x ≥−令ln y x x =−,则1x y x '−=,故max 1y =−,则1ln 1a a e≥−⇒≥. 对于ln ln a x x +≥还可以直接分类参数:max1ln ln ln ln ln ln x xx xx a x x a x e a ee e ⎛⎫⎛⎫+≥⇒≥−=⇒≥= ⎪ ⎪⎝⎭⎝⎭ 总结:需要同加x 才能补全结构 【法二】:整体求导、取点设()x f x ae lnx lna =−+,则0x >,0a >,1()x f x ae x∴'=−, 易知()f x '在(0,)+∞上为增函数,存在0(0,)x ∈+∞,使得0001()0x f x ae x '=−=, 即01x ae x =, 两边取对数,可得00lna x lnx +=−,当00x x <<时,()0f x '<,函数()f x 单调递减, 当0x x >时,()0f x '>,函数()f x 单调递增,000001()()2x min f x f x ae lnx lna x lna x ∴==−+=++, 不等式0x ae lnx lna −+恒成立,∴00120x lna x ++恒成立, ∴12x lna x +−恒成立, 00001122x x x x +⋅=,当且仅当01x =时取等号, 22lna ∴−,即1ae ,故a 的取值范围是1[e,)+∞.湖北鄂东南联考 ·88.已知函数()ln x f x x x xe k −=−−−恒有零点,则实数k 的取值范围是( )A .(],1−∞−B .1,1e⎛⎤−∞−− ⎥⎝⎦C .11,1e⎡⎤−−−⎢⎥⎣⎦D .11,0e⎡⎫−−⎪⎢⎣⎭方法1:同构要使()ln x f x x x xe k −=−−−恒有零点,只需ln ln l =n x x x k x x xe x x e e −−=−−−− 设ln x x t −=,求导可知(],1t ∈−∞−而t k t e =−,求导可知函数t k t e =−在(],1−∞−上单调递增,故1,1k e ⎛⎤∈−∞−⎥⎝⎦方法2:分参求导ln xk x x xe −=−−,令()ln xg x x x xe −=−−,则()1'()1111x x x g x e x x x e x e −−⎪=⎛⎫+−=−−− ⎝⎭∵110xx e −> 故()ln x g x x x xe −=−−在(]0,1递增,()1,+∞递减,故max 1()(1)1g x g e==−−,故选B.注:由常见不等式1x e x ≥+得到,即1100xx e x x e−−>⇒>; 或者令11()x x xe e h x e x x x −=−=,221'()x x x e h x e−=,因为0x >,故'()0h x > 方法3:直接求导(可以消掉k )()()2111'()1xx x x x xxx x e x xe e x x f x x e e xe xe −−−−−=−+=++=,不难得出x x e −在()0,+∞上恒小于0,故()f x 在()0,1上单调递增,在[)1,+∞上递减,故max 1()(1)1f x f k e ==−−−,当0x →时,()f x →−∞,故()f x 的值域为1,1k e ⎛⎤−∞−−− ⎥⎝⎦,则11101k k e e−−−≥⇒≤−−. 福建龙岩九校联考·169.已知函数mx x m x f −+=)1ln()(,若不等式x e x x f −+>1)(在()+∞,0上恒成立,则实数m 的取值范围是____________ . 【答案】(],1−∞x e x x f −+>1)(在()+∞,0上恒成立等价于ln(1)1x m x mx x e +−>+−第一步,错位同构:()ln(1)1xm x x mx e +−+>−,第二步,构造对应函数:令()xg x mx e =−,则有[]ln(1)()g x g x +>第三步,分析单调性,定义域:易知0ln(1)x x <+<,故()g x 在()0,+∞上单调递减 第四步,由单调性求出参数范围:()min'()001xx g x m e x m e=−≤>⇒≤=总结:错位同构,很少见,最后要注意取等.湖南常德3月模拟10.已知不等式ln()x x a e a +≤−对[1,)x ∀∈+∞恒成立,则a 的取值范围为 . 【答案】11a e −<≤−解析:易得:()ln()ln()x xx a e a x a x a x e +≤−⇒+++≤+,1a >−即:ln()ln()x a x x a e x e +++≤+,构造函数()xg x x e =+,∴()()()ln g x a g x +≤.易知()g x 在[1,)x ∈+∞为增函数;∴()ln x x a ≥+, 令()()ln h x x x a =−+,()111x a h x x a x a+−'=−=++, 当0a ≥时,()0h x '≥,()h x 在[1,)x ∈+∞为增函数,()()10h x h ≥≥,∴01a e ≤≤−;当10a −<<时,11a −>;[1,1)x a ∈−,()0h x '<;()1x a ∈−+∞,时,()0h x '≥; ∴()()min 110h x h a a =−=−≥,∴11a −<≤,综上:11a e −<≤−. 总结:最后不等式要注意x 取值范围 补充:对于()ln x x a ≥+,也可以分参()()()minln ln ln 1x x x x x a e x a e x a a e x e ≥+⇒≥+⇒≥+⇒≤−=−浙江省衢州、丽水、湖州三地市高三下学期4月教学质量检测·811.对任意的实数0x >,不等式22ln ln 0x ae x a −+≥恒成立,则实数a 的最小值为( )e2eC.2eD.12e【答案】D总结:指对分离,补全结构2022湖北四地七校高二下期中·712.已知实数a >0,不等式()0x e aln ax ->恒成立,则a 的取值范围是( ) A .1<<a e eB .0<a <1C .0<a <eD .a >e【解答】解:令f (x )=e x ﹣aln (ax ),a >0,x ∈(0,+∞),f ′(x )=e x ﹣在x ∈(0,+∞)上单调递增,x →0时,f ′(x )→﹣∞;x →+∞时,f ′(x )→+∞. ∴存在唯一x 0>0,使得﹣=0,即=,x 0=lna ﹣lnx 0,∴x =x 0时,函数f (x )取得极小值即最小值,f (x 0)=+ax 0﹣2alna >0,∴2﹣2lna >0,解得0<a <e . 总结:补全结构即可。
2024高二英语暑假培优练(人教版2019)专题6 高二英语重点语法名词性从句知识巩固及练习

完成时间:月日天气:暑假作业 06 高二英语重点语法名词性从句知识巩固及练习核心考点梳理名词性从句名词性从句是指在复合句中起名词作用的句子,功能相当于名词词组。
名词性从句在复合句中能作主语、宾语、表语、同位语等,根据在句中的语法功能可分为主语从句、宾语从句、表语从句和同位语从句。
一、主语从句定义:在复合句中,作主语的句子称为主语从句。
常见的连接词:(连接词在句中不能省略)that, whether, who, what, whatever, whoever, where, when, why, how等。
用法:1. 连接词that 引导That we should learn English well is very important.主语从句中we should learn English well句子完整,不缺成分,用that引导,that 不能省连接词whether,if 引导whether 引导的主语从句可放句首,也可放句中,表“是否”if 引导的主语从句只能引导动词后的宾语Whether I will go or not depend on the weather.It remains to be seen whether / if they can finish the task in time.2. 连接代词what, who, which, whose, whatever, whichever,whoever等引导What matters most is your attitude.3. 连接副词when,where,why,how等引导How he made it is still unknown.4. it作形式主语为了避免句子头重脚轻,通常把形式主语it放在句首,真正的主语放在句子后面。
常见句型:(1) It is + n. + that + 句子It is +(a pity / a shame / a fact …)+ that ...(2) It is + adj. + that + 句子It is +(true / clear / important …)+ that ...(3) It is + 过去分词+ that + 句子It is+ said / announced / expected / believed / hoped…+ that ...(4) It seems /appears / happens / turned out that + 句子(5) It hit sb. /occurred to sb. that + 句子二、宾语从句定义:在复合句中,作宾语的句子称为宾语从句。
(人教版)2021年新高二英语暑假精品讲义09 省略

作业091.Though________ to see us, the professor gave us a warm welcome.A.surprising B.to surprise C.being surprised D.surprised2.No matter how frequently ______, he didn’t ______ and determined to lose weight. A.frustrated; lose heart B.frustrated; lose his heartC.being frustrated; lose hope D.having been frustrated; lose his hope3.He was born in an extended family. His family, is richer than mine.A.if so B.if anything C.if ever D.if not4.These problems may lead to more serious ones if ________unsolved.A.making B.remained C.keeping D.left5.It shames me to say it, but I told a lie when _________ at the meeting by my boss. A.questioning B.having questioned C.questioned D.being questioned 6.While ________ dogs, people should not let them loose. Otherwise, they may be dangerous to others. A.walking B.walked C.having walked D.to walk7.He realized that ____there was a time for action, this was it.A.if ever B.if possible C.if any D.if necessary8.When_______,the museum will be open to the public next month.A.to complete B.completesC.completing D.completed9.My family go to the old lady’s home to help them do some housework and some gardening work whenever _________.A.possible B.is possible C.being possible D.possibly10.If _______, I’ll show the committee what I have found out about the matter.A.permitting B.being permitted C.permitted D.having permitted11.If ________ (give) more care and attention, the boy would not have gone that far on the wrong path. 12.Although ________ (beat) by the opposite team, the players were not discouraged.13.David is lively and cheerful while his sister Catherine is quiet to shyness, never speaking unless ________ (speak) to.14.When________(cross) the road, you can not be too careful.15.Its walls were painted fresh as if________(paint) yesterday.16.He looked backward constantly as if _________(follow) by someone.17.When __________(compare)with the other members of the team, I am at a disadvantage.18.Though____________(tell) to learn new words by heart, he didn't follow the teacher's advice. 19.They had accidentally damaged a gas pipe while ________ (drill).20.She won’t go to Tom’s party unless _______ (invite) .Easy Ways to Build V ocabularyIt's not all that hard to build an advanced and large vocabulary. Like many things in life, it's 21.ongoing process, and the best part of the process is that there's enough room for improvement, 22.means you'll just keep getting better and better. Of course you have to work at it. You wouldn't think that a few 23.(month) of exercise in your teens would be enough 24.the rest of your life, and that's also true for building your vocabulary--you have to keep at it daily, and pretty soon you will find that you have an excellent vocabulary.One of the 25.(effect) ways to build vocabulary is to read good books. You need to 26.(real) read at least one good book a week, preferably a classic. This isn't as hard as it 27.(sound), and it is far better than any other method because you improve your vocabulary while 28.(read) an interesting piece of literature. Another nice thing is that you learn both new words and 29.(they) use unconsciously, meaning that you will tend to use the words 30.(learn) this way in conversations almost automatically.1.D【详解】考查状语从句的省略。
(人教版)2021年新高二英语暑假精品讲义09 省略

作业091.Though________ to see us, the professor gave us a warm welcome.A.surprising B.to surprise C.being surprised D.surprised2.No matter how frequently ______, he didn’t ______ and determined to lose weight. A.frustrated; lose heart B.frustrated; lose his heartC.being frustrated; lose hope D.having been frustrated; lose his hope3.He was born in an extended family. His family, is richer than mine.A.if so B.if anything C.if ever D.if not4.These problems may lead to more serious ones if ________unsolved.A.making B.remained C.keeping D.left5.It shames me to say it, but I told a lie when _________ at the meeting by my boss. A.questioning B.having questioned C.questioned D.being questioned 6.While ________ dogs, people should not let them loose. Otherwise, they may be dangerous to others. A.walking B.walked C.having walked D.to walk7.He realized that ____there was a time for action, this was it.A.if ever B.if possible C.if any D.if necessary8.When_______,the museum will be open to the public next month.A.to complete B.completesC.completing D.completed9.My family go to the old lady’s home to help them do some housework and some gardening work whenever _________.A.possible B.is possible C.being possible D.possibly10.If _______, I’ll show the committee what I have found out about the matter.A.permitting B.being permitted C.permitted D.having permitted11.If ________ (give) more care and attention, the boy would not have gone that far on the wrong path. 12.Although ________ (beat) by the opposite team, the players were not discouraged.13.David is lively and cheerful while his sister Catherine is quiet to shyness, never speaking unless ________ (speak) to.14.When________(cross) the road, you can not be too careful.15.Its walls were painted fresh as if________(paint) yesterday.16.He looked backward constantly as if _________(follow) by someone.17.When __________(compare)with the other members of the team, I am at a disadvantage.18.Though____________(tell) to learn new words by heart, he didn't follow the teacher's advice. 19.They had accidentally damaged a gas pipe while ________ (drill).20.She won’t go to Tom’s party unless _______ (invite) .Easy Ways to Build V ocabularyIt's not all that hard to build an advanced and large vocabulary. Like many things in life, it's 21.ongoing process, and the best part of the process is that there's enough room for improvement, 22.means you'll just keep getting better and better. Of course you have to work at it. You wouldn't think that a few 23.(month) of exercise in your teens would be enough 24.the rest of your life, and that's also true for building your vocabulary--you have to keep at it daily, and pretty soon you will find that you have an excellent vocabulary.One of the 25.(effect) ways to build vocabulary is to read good books. You need to 26.(real) read at least one good book a week, preferably a classic. This isn't as hard as it 27.(sound), and it is far better than any other method because you improve your vocabulary while 28.(read) an interesting piece of literature. Another nice thing is that you learn both new words and 29.(they) use unconsciously, meaning that you will tend to use the words 30.(learn) this way in conversations almost automatically.1.D【详解】考查状语从句的省略。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
新高二暑期培优讲义----短文改错题集训 名词和冠词 高考链接: 1、The airs we breathe in is getting dirtier and dirtier. (2015年全国卷1) 2、Mum taught me some basic step of baking (2015陕西卷) 3、Now I am living in a city, but I miss my hometown in countryside. (2015年新课标全国卷1) 名词与冠词考点归纳 1. 注意可数名词单、复数形式的用法错误。 2.注意不可数名词不能用复数形式。 3. 记住常考的几个不可数名词: advice, fun, progress, information, furniture, equipment, luggage/baggage等。 4. 注意积累冠词a, an , the的常见用法。 名词冠词当堂练 [例1]…give me some advices on how to write like a real writer. [例2]Christie was one of my best friend at high school. [例3] Besides, a friend is always good listener when you need one. [例4] Besides, Cleo tends to bark a average of six hours a day. 补充知识点 1.只修饰可数名词: a large/great number of, a good/great many, dozens/scores of, quite a few 2.只修饰不可数名词:a great deal of, a large amount of, quite a little 3.抽象名词具体化: a success; a failure; a surprise, 4.物质名词表一场、一杯、一份 a heavy rain; a coffee 5.零冠词: 1)三餐、季节和学科 2)表职务、头衔的表语、同位语或补足语 3) 球类运动和棋类游戏 4) by+交通工具 动词 1、 When I was a child, I hoped to live in the city, I think I would be happy there. (2015年新课标全国卷) 2、If you are me, would you talk to them?(2015四川卷) 动词考点归纳 1.谓语动词主要考查时态、语态及语气(虚拟语气为主)、主谓一致、并列谓语时态一致。 2.非谓语动词主要考查点:1)某些动词后只能接不定时作宾语;2)某些动词或短语后只能接动名词作宾语;3)某些动词后既可接不定式、也可接动名词;4)非谓语动词作状语、定语等。 谓语动词当堂练 [例1 My sister saw a lovely cup when we are shopping the other day. [例2] It was very kind of Tom to meet me at the airport and drove me to his home. [例3] He was ill and his parents suggested that he had a medical examination. [例4] The whole supermarket was destroying and the bank beside it was also damaged. [例5] There exist now a park that has a small river running through. 非谓语动词当堂练 [例1 ] The position of the classroom with its view made me felt like I was dreaming. [例2] The boy went over at once, opened his umbrella for the grandpa. [例3] I was accustomed to be the only child at a table full of adults. [例4] I begged him come back to Beijing with me, but he refused. [例5] The pupils will get confused if they are made learn too much. 补充知识点 1. 有些动词后只能接动名词作宾语,常见的有: advise, admit , avoid, consider, enjoy, escape, finish, appreciate, imagine, mention, miss, prevent, quit, resist, risk, suggest等。 2.有些动词后常接不定式作宾语,常见的有: hope, ask, refuse, decide, promise, want, wish, pretend, expect, arrange, plan, demand, manage, agree, fail ,determine, choose, desire等 补充知识点 3. 有些动词后面既可以接不定式,也可以接动名词,但意义不尽相同。意义基本相同的有:intend, attempt, continue, begin, start等,意义不同的有:remember, forget, regret, mean, stop, go on, try等。 4. 常见的to为介词的短语有: look forward to, be addicted/accustomed to, get down to, lead to , contribute to, devote…to, object to,, owing to, pay attention to, be/get/ become used to 形容词和副词 高考链接: 1、 Lots of studies have shown that global warming has already become a very seriously problem. (2015年新课标全国卷1) 2、Mon said, “How nice to see you again! Dad and I were terrible worried”.(2015新课标全国卷2) 3、 My mum makes the better biscuits in the world, so I decided to ask her for help.(2015陕西卷) 形容词和副词考点归纳 1.修饰名词、be动词,系动词后要用形容词 2.修饰动词、形容词以及整个句子用副词 3.形容词和副词原级、比较级和最高级的使用 4. 考查-ed形容词和-ing形容词的区别 形容词副词当堂练 [例1 When she dropped me off, I pulled out the toy slow and gave it back [例2] I learned early in life that I had to be more patient and little aggressive.. [例3] People have been great helped by the computer. [例4] …that would make him exciting and crazy. 介词 高考链接: 1、Unfortunately, on the development of industrialization, the environment has been polluted. (2015年新课标全国卷1) 2、My soccer coach retired in last week.(2015陕西卷) 3、My old classroom was interesting because three side of the classroom were made from glass.(2015浙江卷) 介词考点归纳 1.主要考查介词的意义、用法及固定搭配 2.错误类型:多用、少用与误用介词。 介词当堂练 [例1 In that particular evening, there was a strange excitement in the air. [例2] Last Sunday we took a bus to visit to a farm. [例3] From then on, we’ve kept touch with each other through e-mails. [例4] It was so kind for you to let us bring Anne’s friend, Gina. 代词 高考链接: 1. Close to the school there was a beautiful park with many trees around them.(2015浙江卷) 2. I want to do anything special for him at his retirement party.(2015陕西卷) 3. How do you think I should do?(2015四川卷) 代词考点归纳 1.主要考查人称代词和不定代词的用法 2.有时也考查疑问代词和反身代词的用法 代词当堂练 [例1 I was happy when the toys worked, but when things went wrong, I got angry and broke it. [例2] We asked John and Jerry, and all of them offered a satisfactory explanation. [例3] Yesterday he bought two books, one of that is about American history. [例4] But after a while I asked me what else I was going to do other than repairing shoes. 连词 高考链接: 1、There the air is clean or the mountains are (2015年新课标全国卷1) 2、In fact, I don’t like to go anymore, so I’m afraid I’ll lose their friendship.(2015四川卷) 3、If I was only a child when I studied in that classroom , I will never forget it.(2015浙江卷) 连词考点归纳 主要考查逻辑关系,根据上下文判断是并列,转折,还是因果关系等。 连词当堂练 [例1 All I had to do was to write a story or present it. [例2] I’m sorry, and I didn’t understand your question. Could you please restate it? [例3] Frank was about to leave before he noticed a large packet on the floor. [例4] I’d like to go with you; my hands are full, although.
