国际物理林匹克竞赛试题b_a (12)
第24届国际物理奥林匹克竞赛试题与解

中华物理竞赛网
官方网站 圣才学习网
网 赛 竞 理 物 华 中
中华物理竞赛网
官方网站 圣才学习网
网 赛 竞 理 物 华 中
中华物理竞赛网
官方网站 圣才学习网
网 赛 竞 理 物 华 中
中华物理竞赛网
官方网站 圣才学习网
网 赛 竞 理 物 华 中
中华物理竞赛网
官方网站 圣才学习网
网 赛 竞 理 物 华 中
中华物理竞赛网
官方网站 圣才学习网
网 赛 竞 理 物 华 中
中华物理竞赛网
官方网站 圣才学习网
网 赛 竞 理 物 华 中
中华物理竞赛网
官方网站 圣才学习网
网 赛 竞 理 物 华 中
中华物理竞赛网
官方网站 圣才学习网
网 赛 竞 理 物 华 中
中华物理竞赛网
官方网站 圣才学习网
网 赛 竞 理 物 华 中
中华物理竞赛网
官方网站 圣才学习网
网 赛 竞 理 物 华 中
中华物理竞赛网
官方网站 圣才学习网
网 赛 竞 理 物 华 中
中华物理竞赛网
官方网站 圣才学习网
网 赛 竞 理 物 华 中
中华物理竞赛网
官方网站 圣才学习网
网 赛 竞 理 物 华 中
中华物理竞赛网
官方网站 圣才学习网
网 赛 竞 理 物 华 中
中华物理竞赛网
官方网站 圣才学习网
网 赛 竞 理 物 华 中
中华物理竞赛网
官方网站 圣才学习网
网 赛 竞 理 物 华 中
中华物理竞赛网
官方网站 圣才学习网
各国物理竞赛试题及答案

各国物理竞赛试题及答案一、选择题1. 光在真空中的传播速度是多少?A. 299,792,458 m/sB. 300,000,000 m/sC. 299,792,458 km/sD. 300,000,000 km/s答案:A2. 根据牛顿第三定律,作用力和反作用力的大小关系是?A. 相等B. 不相等C. 作用力大于反作用力D. 反作用力大于作用力答案:A二、填空题3. 根据国际单位制,电荷量的单位是______。
答案:库仑(C)4. 绝对零度的数值是______开尔文。
答案:0三、计算题5. 一个物体的质量为2kg,受到一个大小为10N的恒定力作用,求物体的加速度。
答案:根据牛顿第二定律,F=ma,所以a=F/m=10N/2kg=5m/s²。
四、实验题6. 设计一个实验来验证欧姆定律。
答案:实验步骤如下:- 准备一个定值电阻、一个电源、一个电流表、一个电压表和若干导线。
- 将电阻、电流表和电压表串联连接,并接入电源。
- 记录不同电压下的电流值。
- 绘制电流-电压图,观察是否为直线,斜率即为电阻值,验证欧姆定律。
五、简答题7. 简述波粒二象性的概念。
答案:波粒二象性是指微观粒子如电子、光子等,既表现出波动性也表现出粒子性。
在某些实验条件下,它们表现出波动性,如干涉和衍射现象;而在其他实验条件下,它们表现出粒子性,如光电效应。
这一概念是量子力学的基础之一。
六、论述题8. 论述相对论对现代物理学的影响。
答案:相对论是20世纪初由爱因斯坦提出的物理理论,包括狭义相对论和广义相对论。
狭义相对论提出了时间膨胀和长度收缩的概念,改变了我们对时间和空间的认识。
广义相对论则将引力视为时空的弯曲,为黑洞、宇宙大爆炸等天体物理学现象提供了理论基础。
相对论不仅在理论物理学中占有重要地位,还对现代科技如GPS定位系统等产生了深远影响。
物理奥林匹亚竞赛试题与解答
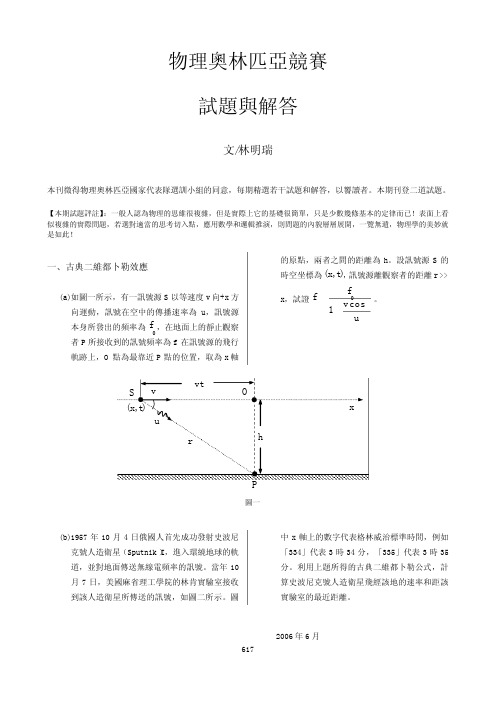
物理奧林匹亞競賽 試題與解答文/林明瑞本刊徵得物理奧林匹亞國家代表隊選訓小組的同意,每期精選若干試題和解答,以饗讀者。
本期刊登二道試題。
【本期試題評註】:一般人認為物理的思維很複雜,但是實際上它的基礎很簡單,只是少數幾條基本的定律而已!表面上看似複雜的實際問題,若選對適當的思考切入點,應用數學和邏輯推演,則問題的內貌層層展開,一覽無遺,物理學的美妙就是如此!一、古典二維都卜勒效應(a) 如圖一所示,有一訊號源S 以等速度v 向+x 方向運動,訊號在空中的傳播速率為u,訊號源本身所發出的頻率為f ,在地面上的靜止觀察者P 所接收到的訊號頻率為f 。
在訊號源的飛行軌跡上,O 點為最靠近P 點的位置,取為x 軸的原點,兩者之間的距離為h。
設訊號源S 的時空坐標為),(t x ,訊號源離觀察者的距離r >>x,試證uv ff cos 10。
(b) 1957年10月4日俄國人首先成功發射史波尼克號人造衛星(Sputnik I ),進入環繞地球的軌道,並對地面傳送無線電頻率的訊號。
當年10月7日,美國麻省理工學院的林肯實驗室接收到該人造衛星所傳送的訊號,如圖二所示。
圖中x 軸上的數字代表格林威治標準時間,例如「334」代表3時34分,「335」代表3時35分。
利用上題所得的古典二維都卜勒公式,計算史波尼克號人造衛星飛經該地的速率和距該實驗室的最近距離。
v),(t x PuS rh xO vt圖一解:(a) 參看圖三,訊號源S 以等速度v 向+x 方向運動,訊號的傳播速率為u,訊號源本身所發出的訊號週期為0,觀察者P 所測得的訊號週期為。
設S 1的時空坐標為),(11t x ,經一個週期後,其時空坐標變為),(22t x ,由圖上的幾何關係可得2121()x x v t t v2121r r t t u u21211()()t t r r u由於r x ,故1221()cos r r x x ,以之代入上式,得211()cosx x u00cos v u 0cos 1v u0cos 1ff v u(b) 人造衛星的軌道雖然是橢圓形,但從地面上觀察,所見者僅為其軌跡的一小段,近乎為一直線,因此衛星訊號的都卜勒效應符合上題所描述的情況。
国际物理林匹克竞赛试题Theoretical_Exam_Solution_2_English
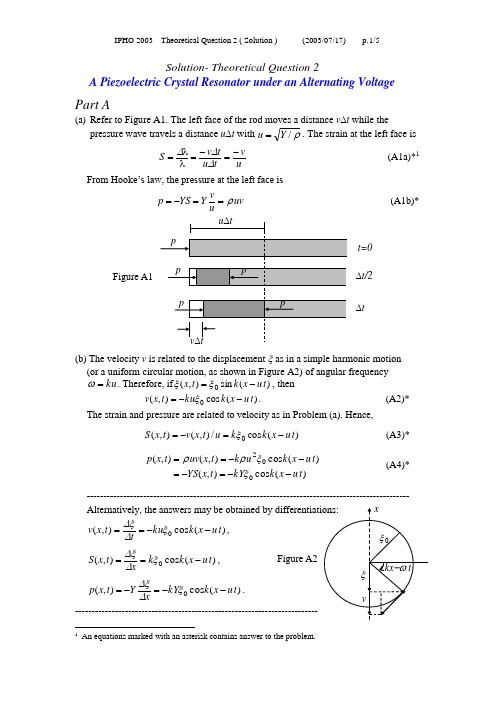
Solution- Theoretical Question 2A Piezoelectric Crystal Resonator under an Alternating Voltage Part A(a) Refer to Figure A1. The left face of the rod moves a distance v ∆t while thepressure wave travels a distance u ∆t with ρ/Y u =. The strain at the left face isuv t u t v S -=-==∆∆∆(A1a)*1 From Hooke’s law, the pressure at the left face is uv uv Y YS p ρ==-= (A1b)*(b) The velocity v is related to the displacement ξ as in a simple harmonic motion (or a uniform circular motion, as shown in Figure A2) of angular frequency ku =ω. Therefore, if )(sin ),(0t u x k t x -=ξξ, then)(cos ),(0t u x k ku t x v --=ξ. (A2)*The strain and pressure are related to velocity as in Problem (a). Hence,)(cos /),(),(0t u x k k u t x v t x S -=-=ξ (A3)*)(cos ),()(cos ),(),(002t u x k kY t x YS t u x k u k t x uv t x p --=-=--==ξξρρ (A4)*-------------------------------------------------------------------------------------------------)(cos ),(0t u x k ku t t x v --==ξ∆ξ∆, )(cos ),(0t u x k k x t x S -==ξ∆ξ∆, )(cos ),(0t u x k kY xY t x p --=-=ξ∆ξ∆. -------------------------------------------------------------------------1 An equations marked with an asterisk contains answer to the problem.t=0∆t/2 Figure A1 ∆t Figure A2Part B(c) Since the angular frequency ω and speed of propagation u are given, the wavelength is given by λ = 2π / k with k = ω / u . The spatial variation of the displacement ξ is therefore described by)2(cos )2(sin )(21b x k B b x k B x g -+-= (B1) Since the centers of the electrodes are assumed to be stationary, g (b /2) = 0. This leads to B 2 = 0. Given that the maximum of g (x ) is 1, we have A = ±1 and)2(sin )(b x u x g -±=ω (B2)* Thus, the displacement ist b x u t x ωωξξcos )2(sin 2),(0-±= (B3) (d) Since the pressure p (or stress T ) must vanish at the end faces of the quartz slab (i.e., x = 0 and x = b ), the answer to this problem can be obtained, by analogy, from the resonant frequencies of sound waves in an open pipe of length b . However, given that the centers of the electrodes are stationary, all evenharmonics of the fundamental tone must be excluded because they have antinodes, rather than nodes, of displacement at the bisection plane of the slab.Since the fundamental tone has a wavelength λ = 2b , the fundamentalfrequency is given by )2/(1b u f =. The speed of propagation u is given by33101045.51065.21087.7⨯=⨯⨯==ρYu m/s (B4) and, given that b =1.00⨯10-2 m, the two lowest standing wave frequencies are)kHz (27321==b u f , )kHz (81823313===bu f f (B5)* -------------------------------------------------------------------------------------------------[Alternative solution to Problems (c) and (d)]: A longitudinal standing wave in the quartz slab has a displacement node at x = b/2. It may be regarded as consisting of two waves traveling in opposite directions. Thus, its displacement and velocity must have the following form)]2(sin )2([sin cos )2(sin 2),(ut b x k ut b x k t b x k t x m m +-+--=-=ξωξξ (B6) t b x k ut b x k ut b x k ku t x v m m ωωξξsin )2(sin 2)]2(cos )2([cos ),(--=+-----= (B7) where ω = k u and the first and second factors in the square brackets represent waves traveling along the +x and –x directions, respectively. Note that Eq. (B6) is identical to Eq. (B3) if we set ξm = ±ξ0.For a wave traveling along the –x direction, the velocity v must be replaced by –v in Eqs. (A1a) and (A1b) so that we haveuv S -= and uv p ρ= (waves traveling along +x ) (B8) uv S = and uv p ρ-= (waves traveling along –x ) (B9) As in Problem (b), the strain and pressure are therefore given byt b x k k ut b x k ut b x k k t x S m m ωξξcos )2(cos 2)]2(cos )2(cos [),(-=+------= (B10) t b x k u ut b x k ut b x k u t x p m m ωωξρωξρcos )2(cos 2)]2(cos )2([cos ),(--=+-+---= (B11) Note that v , S , and p may also be obtained by differentiating ξ as in Problem (b).The stress T or pressure p must be zero at both ends (x = 0 and x = b ) of the slab at all times because they are free. From Eq. (B11), this is possible only if 0)2/cos(=kb or,5,3,1,2====n n b ff b u kb πλπω (B12) In terms of wavelength λ, Eq. (B12) may be written as,5,3,1,2==n nb λ. (B13) The frequency is given by,5,3,1,22====n Y b n b nu u f ρλ. (B14) This is identical with the results given in Eqs. (B4) and (B5).--------------------------------------------------------------------------------------------------- (e) From Eqs. (5a) and (5b) in the Question, the piezoelectric effect leads to the equations)(E d S Y T p -= (B15)E d Y S Yd T pT p )1(2εεσ-+= (B16)Because x = b /2 must be a node of displacement for any longitudinal standingwave in the slab, the displacement ξ and strain S must have the form given in Eqs. (B6) and (B10), i.e., with ku =ω,)cos()2(sin ),(φωξξ+-=t b x k t x m (B17) )cos()2(cos ),(φωξ+-=t b x k k t x S m (B18) where a phase constant φ is now included in the time-dependent factors.By assumption, the electric field E between the electrodes is uniform anddepends only on time:ht V h t V t x E m ωcos )(),(==. (B19) Substituting Eqs. (B18) and (B19) into Eq. (B15), we have]cos )cos()2(cos [t V hd t b x k k Y T m p m ωφωξ-+-= (B20) The stress T must be zero at both ends (x = 0 and x = b ) of the slab at all times because they are free. This is possible only if φ = 0 andhV d kb k m p m =2cos ξ (B21) Since φ = 0, Eqs. (B16), (B18), and (B19) imply that the surface charge density must have the same dependence on time t and may be expressed ast x t x ωσσcos )(),(= (B22)with the dependence on x given byh V d Y b x k kb d Y hV d Y b x k k Yd x m T p T p mT p T m p )]1()2(cos 2cos [)1()2(cos )(222εεεεξσ-+-=-+-= (B23)* (f) At time t , the total surface charge Q (t ) on the lower electrode is obtained by integrating ),(t x σin Eq. (B22) over the surface of the electrode. The result is)]1()2tan 2([)]1()2tan 2()[()]1()2(cos 2cos [)(1),()(1)()(2202202200ααεεεεεσσ-+=-+=-+-===⎰⎰⎰kb kb C d Y kb kb d Y h bw dx d Y b x k kb d Y h w dx w x V wdx t x t V t V t Q Tp T p T b T p T p b m b (B24) where h bw C T ε=0, 322221082.906.427.110)25.2(--⨯=⨯⨯==T p d Y εα (B25)* (The constant α is called the electromechanical coupling coefficient .) Note : The result C 0 = εT bw /h can readily be seen by considering the static limit k = 0 of Eq. (5) in the Question. Since x x ≈tan when x << 1, we have02200)]1([)(/)(lim C C t V t Q k =-+≈→αα (B26)Evidently, the constant C 0 is the capacitance of the parallel-plate capacitor formed by the electrodes (of area bw ) with the quartz slab (of thickness h and permittivityεT) serving as the dielectric medium. It is therefore given by εT bw/h.(B47)*。
第1届国际物理奥林匹克竞赛试题与解答

第1届国际物理奥林匹克竞赛试题与解答(1967年于波兰的华沙)【题1】质量M=0.2kg 的小球静置于垂直柱上,柱高h=5m 。
一粒质量m=0.01kg 、以速度0=500m/s 飞行的子弹水平地穿过球心。
球落在距离柱s =20m 的地面上。
问子弹落在地面何处?子弹动能中有多少转换为热能?解:在所有碰撞情况下,系统的总动量均保持不变:MV mv mv +=0其中v 和V 分别是碰撞后子弹的速度和小球的速度. 两者的飞行时间都是01.12==gh t s 球在这段时间沿水平方向走过20m 的距离,故它在水平方向的速度为:8.1901.120==V (m/s ) 由方程0.01×500=0.01v +0.2×19.8可求出子弹在碰撞后的速度为:v =104m/s子弹也在1.01s 后落地,故它落在与柱的水平距离为S =vt =104×1.01=105m 的地面上。
碰撞前子弹的初始动能为=2021mv 1250 J 球在刚碰撞后的动能为=221MV 39.2 J 子弹在刚碰撞后的动能为=221mv 54 J 与初始动能相比,两者之差为1250 J -93.2 J =1156.8 J这表明原来动能的92.5%被系统吸收而变为热能。
这种碰撞不是完全非弹性碰撞。
在完全弹性碰撞的情形下,动能是守恒的。
而如果是完全非弹性碰撞,子弹将留在球内。
【题2】右图(甲)为无限的电阻网络,其中每个电阻均为r ,求A、B两点间的总电阻。
解:如图(乙)所示 A、B两点间的总电阻应等于C、D两点间的总电阻与电阻r的并联,再与r串联 图(甲) 后的等效电阻。
如果网络是无限的,则A、B两点间的总电阻应等于C、D 两点间的总电阻,设为Rx 。
根据它们的串并联关系有:rR rR r R x x x ++= 图(乙)A B r r r r r r r r A B r rr r r r r r CD解上式可得: r R x 251+= 【题3】给定两个同样的球,其一放在水平面上,另一个以细线悬挂。
奥林匹克物理竞赛试题及答案

奥林匹克物理竞赛试题及答案国际奥林匹克物理竞赛是国际中学生的物理大赛,高中同学可以用来提升物理解题能力。
下面店铺给大家带来奥林匹克物理竞赛试题,希望对你有帮助。
奥林匹克物理竞赛试题国际物理奥林匹克竞赛简介竞赛设立由参赛成员国组成的国际物理奥林匹克委员会。
竞赛章程规定:目的是为增进中学物理教学的国际交流,通过竞赛促进开展物理学科的课外活动,以加强不同国家青年之间的友好关系和人民间的相互了解合作。
同时帮助参赛者发展物理方面的创造力,把从学校学到的知识用于解决实际问题的能力。
国际物理奥林匹克竞赛每年举办一次。
由各会员国轮流主办,并由各代表团团长和一名主办国指定的主席组成国际委员会。
国际委员会的任务是公平合理地评卷,监督章程规定的执行情况,决定竞赛结果。
每一会员国可选派5名高中学生或技术学校学生参加竞赛。
参加者的年龄到竞赛开始的那一天不能超过20岁。
参赛代表队要有2名团长,2名团长是国际委员会的成员,条件是能胜任解答赛题,能参加竞赛试卷的讨论和评分工作,并能通晓一种国际物理奥林匹克的工作语言。
国际物理奥林匹克的工作语言是英文、法文、德文和俄文。
代表团到达主办国时,团长要将参加学生及团长的情况告诉主办国家组织人员。
竞赛于每年6月底举行。
竞赛分两天进行。
第一天进行3道理论计算题竞赛,另一天的竞赛内容是1—2道实验题。
中间有一天的休息。
参赛者可使用计算尺、不带程序编制的计算器和对数表、物理常数表和制图工具,但不能使用数学和物理公式一览表。
竞赛题由参加国提供题目,主办国命题。
在竞赛前,赛题要保密。
竞赛题内容包括中学物理的4个部分(力学、热力学和分子物理学、光学及原子和核物理学、电磁学) ,解题要求用标准的中等数学而不要用高等数学。
主办国提出评卷标准并指定评卷人。
每题满分为10分。
各代表团团长同时对自己团员竞赛卷的复制品进行评定,最后协商决定成绩。
评奖标准是以参赛者前三名的平均分数计为100%,参赛者达90% 以上者为一等奖,78—90%者为二等奖,65—78%者为三等奖,同时发给证书。
物理竞赛考试题及答案

物理竞赛考试题及答案一、选择题(每题5分,共20分)1. 光在真空中的速度是多少?A. 299,792,458 m/sB. 299,792,458 km/sC. 299,792,458 cm/sD. 299,792,458 mm/s答案:A2. 牛顿第三定律描述的是:A. 力的合成B. 力的分解C. 作用力和反作用力D. 力的平衡答案:C3. 根据能量守恒定律,下列哪项描述是错误的?A. 能量可以在不同形式之间转换B. 能量可以在系统中自由创造C. 能量的总量在封闭系统中保持不变D. 能量转换过程中总能量守恒答案:B4. 以下哪种力不是基本力?A. 重力B. 电磁力C. 强核力D. 弱核力答案:A二、填空题(每题5分,共20分)1. 根据库仑定律,两个点电荷之间的力与它们电荷量的乘积成正比,与它们之间距离的________成反比。
答案:平方2. 欧姆定律表明,电流I与电压V和电阻R之间的关系是:I =________。
答案:V/R3. 一个物体在自由落体运动中,其速度v与时间t的关系是:v = ________。
答案:gt4. 根据热力学第一定律,系统内能的增加等于系统吸收的热量Q与系统对外做的功W之和,即:ΔU = ________。
答案:Q + W三、简答题(每题10分,共20分)1. 简述波粒二象性的概念。
答案:波粒二象性是指微观粒子如电子、光子等,既表现出波动性也表现出粒子性。
在某些实验条件下,它们表现出波动性,如干涉和衍射现象;而在另一些条件下,它们表现出粒子性,如光电效应。
这一概念是量子力学的基础之一。
2. 描述牛顿第一定律的内容及其意义。
答案:牛顿第一定律,也称为惯性定律,指出一个物体会保持静止或匀速直线运动状态,除非受到外力的作用。
这一定律的意义在于,它揭示了力是改变物体运动状态的原因,而不是维持物体运动的原因。
四、计算题(每题15分,共30分)1. 一个质量为2kg的物体从静止开始自由下落,忽略空气阻力,求物体下落2秒后的速度和位移。
国际物理奥林匹克竞赛试题.doc

国际物理奥林匹克竞赛试题理论试题题1A 某蹦迪运动员系在一根长弹性绳子的一端,绳的另一端固定在一座高桥上,他自静止高桥向下面的河流下落,末与水面相触,他的质量为m,绳子的自然长度为L,绳子的力常数(使绳子伸长lm所需的力)为k,重力场强度为g。
求出下面各量的表达式。
(a)运动员在第一次达到瞬时静止前所落下的距离y。
(b)他在下落过程中所达到的最大速率v。
(c)他在第一次达到瞬时静止前的下落过程所经历的时间t。
设运动员可以视为系于绳子一端的质点,与m相比绳子的质量可忽略不计,当绳子在伸长时服从胡克定律,在整个下落过程中空气的阻力可忽略不计。
B 一热机工作于两个相同材料的物体之间,两物体的温度分别为T A和T B(T A>T B),每个物体的质量均为m,比热恒定,均为s。
设两个物体的压强保持不变,且不发生相变。
(a)假定热机能从系统获得理论上允许的最大机械能,求出两物体A和B最终达到的温度T?的表达式,给出解题全部过程。
(b)由此得出允许获得的最大功的表达式。
(c)假定热机工作于两箱水之间,每箱水的体积为2.50m3,一箱水的温度为350K,另一箱水的温度为300K。
计算可获得的最大机械能。
已知水的比热容= 4.19×103kg-1K-1,水的密度=1.00 x 103kgm.-3C 假定地球形成时同位素238U和235U已经存在,但不存在它们的衰变产物。
238U和235U的衰变被用来确定地球的年龄T。
(a)同位素238U以4.50×109年为半衰期衰变,衰变过程中其余放射性衰变产物的半衰期比这都短得多,作为一级近似,可忽略这些衰变产物的存在,衰变过程终止于铅的同位素206Ph。
用238U的半衰期、现在238U的数目238N表示出由放射衰变产生的206Pb原子的数目206n。
(运算中以109年为单位为宜)(b)类似地,235U在通过一系列较短半衰期产物后,以0.710×109年为半衰期衰变,终止于稳定的同位素207Pb。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
Experimental Question2
Diffraction and Scattering of Laser Light
The aim of this experiment is to demonstrate and quantify to some extent the reflection,diffraction,and scattering of light,using visible radiation from a Laser Diode source.A metal ruler is employed as a diffraction grating,and a perspex tank,containing water and diluted milk,is used to determine reflection and scattering phenomena.
Section1(6marks)
Place the150mm length metal ruler provided so that it is nearly normal to the incident laser beam,and so that the laserr beam illuminates several rulings on it.Observe a number of“spots”of light on the white paper screen provided,caused by the phenomenon of diffraction.
Draw the overall geometry you have employed and measure the position and separation of these spots with the screen at a distance of approximately1.5metres from the ruler.
Using the relation
Nλ=h sinβ
where N is the order of diffraction
λis the radiation wavelength
h is the grating period
βis the angle of diffraction
and the information obtained from your measurements,determine the wavelength of the laser radiation. Section2(4marks)
Now insert the empty perspex tank provided into the space between the laser and the white paper screen. Set the tank at approximately normal incidence to the laser beam.
(i)Observe a reduction in the emergent beam intensity,and estimate the percentage value of this re-
duction.Some calibrated transmission discs are provided to assist with this estimation.Remember that the human eye has a logarithmic response.
This intensity reduction is caused primarily by reflection losses at the aid/perspex boundaries,of which there are four in this case.THe reflection coefficient for normal incidence at each boundary,R, which is the ratio of the reflectied to incident intensities,is given by
R={(n1−n2)/(n1+n2)}2
where n1and n2are the refractive indices before and after the boundary.The corresponding transmission coefficient,assuming zero absorption in the perspex,isfiven by
T=1−R.
(ii)Assuming a refractive index of1.59for the perspex and neglecting the effect of multiple reflections and cogerence,calculate the intensity transmission coefficient of the empty perspex pare this result with the estimate you made in Part(i)of this Section.
Section3(1mark)
Without moving the perspex tank,repeat the observations and calculations in Section2with the50mL of water provided in a beaker now added to the tank.Assume the refractive index of water to be1.33. Section4(10marks)
(i)Add0.5mL(12drops)of milk(the scattering material)to the50mL of water in the perspex tank,
and stir well.Measure as accurately as possible the total angle through which the laser light is
scattered,and the diameter of the emerging light patch at the exit face of the tank,noting that these
quantities are related.Also estimate the reduction in transmitted intensity,as in earlier sections.
(ii)Add a further0.5mL of milk to the tank,and repeat the measurements requested in part(i).
(iii)Repeat the process in part(ii)until very little or no transmitted laser light can be observed.
(iv)Determine the relationship between scattering angle and milk concentration in the tank.
(v)Use your results,and the relationship
I=I0e−µz=T milk×I0
where I0is the input intensity
I is the emerging intensity
z is the distance in the tank
µis the attenuation coefficient and equals a constant times the concentration of the scatterer
T milk is the transmission coefficient for the milk
to obtain an estimate for the value ofµfor a scatterer concentration of10%.。
