Pascal滑铁卢数学竞赛(Grade 9)-数学Mathematics-2013-试题 exam

合集下载

Hypatia滑铁卢数学竞赛(Grade 11)-数学Mathematics-2008-试题 exam

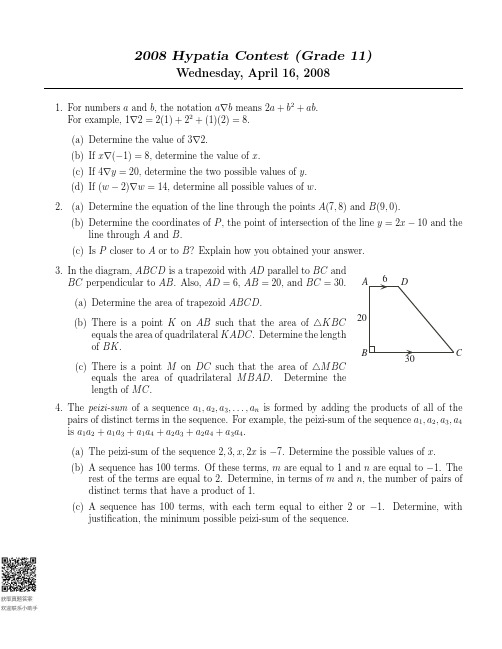
2008Hypatia Contest(Grade11)Wednesday,April16,20081.For numbers a and b,the notation a∇b means2a+b2+ab.For example,1∇2=2(1)+22+(1)(2)=8.(a)Determine the value of3∇2.(b)If x∇(−1)=8,determine the value of x.(c)If4∇y=20,determine the two possible values of y.(d)If(w−2)∇w=14,determine all possible values of w.2.(a)Determine the equation of the line through the points A(7,8)and B(9,0).(b)Determine the coordinates of P,the point of intersection of the line y=2x−10and theline through A and B.(c)Is P closer to A or to B?Explain how you obtained your answer.3.In the diagram,ABCD is a trapezoid with AD parallel to BC andBC perpendicular to AB.Also,AD=6,AB=20,and BC=30.(a)Determine the area of trapezoid ABCD.(b)There is a point K on AB such that the area of KBCequals the area of quadrilateral KADC.Determine the length of BK.(c)There is a point M on DC such that the area of MBCequals the area of quadrilateral MBAD.Determine the length of MC.C4.The peizi-sum of a sequence a1,a2,a3,...,a n is formed by adding the products of all of thepairs of distinct terms in the sequence.For example,the peizi-sum of the sequence a1,a2,a3,a4 is a1a2+a1a3+a1a4+a2a3+a2a4+a3a4.(a)The peizi-sum of the sequence2,3,x,2x is−7.Determine the possible values of x.(b)A sequence has100terms.Of these terms,m are equal to1and n are equal to−1.Therest of the terms are equal to2.Determine,in terms of m and n,the number of pairs of distinct terms that have a product of1.(c)A sequence has100terms,with each term equal to either2or−1.Determine,withjustiﬁcation,the minimum possible peizi-sum of the sequence.。

滑铁卢竞赛数学题

滑铁卢竞赛数学题概述
滑铁卢竞赛数学题通常比较难，涉及的知识点广泛，包括代数、几何、数论、组合数学等多个领域。

以下是一些滑铁卢竞赛数学题的示例：
1. 有100个球，其中有一个与其他99个重量不同，但外观相同。

用一个天平，最少需要称多少次才能确定这个重量不同的球？
2. 一个正方形的面积为1，将其四边中点连接起来，形成另一个正方形。

如此重复，得到第五、第六个正方形，求第五个正方形的面积。

3. 一个圆被分成n个相等的扇形，其中一个是空心的，其他n-1个是实心的。

求空心扇形的圆心角是多少度？
4. 有100个人站成一排，从第1个人开始报数，每次报到奇数的人离开队伍。

经过若干轮后，只剩下一个人。

求这个人最初站在第几位？
5. 有5个不同质因数的最小正整数是多少？
以上仅是滑铁卢竞赛数学题的一些示例，实际上还有更多难题和技巧题。

如果想要深入了解滑铁卢竞赛数学题的解题技巧和策略，建议参考相关的竞赛书籍和资料，或者参加专业的数学竞赛培训课程。

滑铁卢大学欧几里得数学竞赛

该考试是学生申请滑铁卢大学数学学院本科专业的重要参考。

众所周知滑铁卢大学数学学院是全球最大的数学、统计学、计算机科学等学科教学中心比尔•盖茨曾于 2005 年、 2008 年两度造访该大学是比尔•盖茨大学巡回讲座的北美5 所大学之一也是唯一的一所加拿大大学。

考试范围：大部分的题目基于高三或者12年级数学课学习的内容。

我们的竞赛题目主要包括以下的数学内容：Ø 欧几里德几何和解析几何Ø 三角函数，包括函数、图像、性质、正弦余弦定理Ø 指数和对数函数Ø 函数符号Ø 方程组Ø 多项式，包括二次三次方程根的关系、余数定理Ø 数列、数列求和Ø 简单的计算问题Ø 数字的性质考试时间为 2.5 个小时， 10 道题。

每题 10 分，共计 100 分。

考试题有两种，一种只需要给出答案，另一种则需要写出整个解题过程，这种题的最终得分不仅取决于结果正确与否，还与解题思路有关。

Ø 笔试Ø 10道题：大部分要求写出完整的解题步骤；Ø 根据解题的方法和步骤获得相应的分数；Ø 步骤不完整的解题无法得到全部的分数；Ø 竞赛时长为2.5小时；Ø 共100分；Ø 可以使用无编程无绘图功能的计算器；Ø 不可以使用任何可接入互联网的设备，如手机、平板电脑等均不能携带如何准备：Ø CEMC官网可以免费下载历年的竞赛原题以及标准答案；Ø CEMC官网提供各种免费的数学资源；Ø www.cemc.uwaterloo.ca；如何参加：Ø 学校可以申请注册为考点，安排组织欧几里德数学竞赛；Ø 学生需要通过自己所在的学校报名参加欧几里德数学竞赛；Ø 如果学生所在学校未注册考点，学生可以报名在我们北京或者上海的考点参加欧几里德数学竞赛；Ø 竞赛结束之后，学校需要将全部的试卷寄回滑铁卢大学；Ø 改卷结束之后，滑铁卢大学会在CEMC官网录入学生的成绩。

Pascal滑铁卢数学竞赛(Grade 9)-数学Mathematics-2004-试题 exam

(A) 21 (D) 13
(B) 40 (E) 34
(C) 19
20. At the beginning of the game “Clock 7”, the arrow points to one of the seven numbers. On each turn, the arrow is rotated clockwise by the number of spaces indicated by the arrow at the beginning of the turn. For example, if “Clock 7” starts with the arrow pointing at 4, then on the first turn, the arrow is rotated clockwise 4 spaces so that it now points at 1. The arrow will then move 1 space on the next turn, and so on. If the arrow points at 6 after the 21st turn, at which number did the arrow point after the first turn?
1. To win a skateboard, the skill testing question is 5 × (10 – 6) ÷ 2 . The correct answer is
(A)ቤተ መጻሕፍቲ ባይዱ10
(B) 35
(C) 32
(D) 22
(E) 40
2. The average of 2, x and 12 is 8. What is the value of x?

2010PascalSolution滑铁卢竞赛题答案

1.In cents,theﬁve given choices are50,90,95,101,and115cents.The diﬀerences between each of these and$1.00(or100cents),in cents,are100−50=50100−90=10100−95=5101−100=1115−100=15 The diﬀerence between$1.01and$1.00is the smallest(1cent),so$1.01is closest to$1.00.Answer:(D) ing the correct order of operations,(20−16)×(12+8)4=4×204=804=20Answer:(C)3.We divide the750mL ofﬂour into portions of250mL.We do this by calculating750÷250=3.Therefore,750mL is three portions of250mL.Since50mL of milk is required for each250mL ofﬂour,then3×50=150mL of milk is required in total.Answer:(C) 4.There are8ﬁgures in total.Of these,3are triangles.Therefore,the probability is3.Answer:(A) 5.We simplify the left side and express it as a fraction with numerator1:1 9+118=218+118=318=16Therefore,the number that replaces the is6.Answer:(C) 6.There are16horizontal segments on the perimeter.Each has length1,so the horizontalsegments contribute16to the perimeter.There are10vertical segments on the perimeter.Each has length1,so the vertical segments contribute10to the perimeter.Therefore,the perimeter is10+16=26.(We could arrive at this total instead by starting at aﬁxed point and travelling around the outside of theﬁgure counting the number of segments.)Answer:(E) 7.Since33=3×3×3=3×9=27,then√33+33+33=√27+27+27=√81=9Answer:(B)8.The diﬀerence between the two given numbers is7.62−7.46=0.16.This length of the number line is divided into8equal segments.The length of each of these segments is thus0.16÷8=0.02.Point P is three of these segments to the right of7.46.Thus,the number represented is7.46+3(0.02)=7.46+0.06=7.52.Answer:(E)9.A 12by 12grid of squares will have 11interior vertical lines and 11interior horizontal lines.(In the given 4by 4example,there are 3interior vertical lines and 3interior horizontal lines.)Each of the 11interior vertical lines intersects each of the 11interior horizontal lines and creates an interior intersection point.Thus,each interior vertical line accounts for 11intersection points.Therefore,the number of interior intersection points is 11×11=121.Answer:(B)10.Because the central angle for the interior sector “Less than 1hour”is 90◦,then the fraction of the students who do less than 1hour of homework per day is 90◦360◦=14.In other words,25%of the students do less than 1hour of homework per day.Therefore,100%−25%=75%of the students do at least 1hour of homework per day.Answer:(E)11.Solution 1Since there is more than 1four-legged table,then there are at least 2four-legged tables.Since there are 23legs in total,then there must be fewer than 6four-legged tables,since 6four-legged tables would have 6×4=24legs.Thus,there are between 2and 5four-legged tables.If there are 2four-legged tables,then these tables account for 2×4=8legs,leaving 23−8=15legs for the three-legged tables.Since 15is divisible by 3,then this must be the solution,so there are 15÷3=5three-legged tables.(We can check that if there are 3or 4four-legged tables,then the number of remaining legs is not divisible by 3,and if there are 5four-legged tables,then there is only 1three-legged table,which is not allowed.)Solution 2Since there is more than 1table of each type,then there are at least 2three-legged tables and 2four-legged tables.These tables account for 2(3)+2(4)=14legs.There are 23−14=9more legs that need to be accounted for.These must come from a combination of three-legged and four-legged tables.The only way to make 9from 3s and 4s is to use three 3s.Therefore,there are 2+3=5three-legged tables and 2four-legged tables.Answer:(E)12.Solution 1The total area of the rectangle is 3×4=12.The total area of the shaded regions equals the total area of the rectangle (12)minus the area of the unshaded region.The unshaded region is a triangle with base of length 1and height 4;the area of this region is 12(1)(4)=2.Therefore,the total area of the shaded regions is 12−2=10.Solution 2The shaded triangle on the left has base of length 2and height of length 4,so has an area of 12(2)(4)=4.The shaded triangle on the right has base of length3(at the top)and height of length4,sohas an area of12(3)(4)=6.Therefore,the total area of the shaded regions is4+6=10.Answer:(C)13.Since the ratio of boys to girls at Cayley H.S.is3:2,then33+2=35of the students at CayleyH.S.are boys.Thus,there are35(400)=12005=240boys at Cayley H.S.Since the ratio of boys to girls at Fermat C.I.is2:3,then22+3=25of the students at FermatC.I.are boys.Thus,there are25(600)=12005=240boys at Fermat C.I.There are400+600=1000students in total at the two schools.Of these,240+240=480are boys,and so the remaining1000−480=520students are girls.Therefore,the overall ratio of boys to girls is480:520=48:52=12:13.Answer:(B) 14.When the given net is folded,the face numbered5will be opposite the face numbered1.Therefore,the remaining four faces share an edge with the face numbered1,so the product of the numbers is2×3×4×6=144.Answer:(B)15.The percentage10%is equivalent to the fraction110.Therefore,t=110s,or s=10t.Answer:(D)16.Since the base of the folded box measures5cm by4cm,then the area of the base of the boxis5(4)=20cm2.Since the volume of the box is60cm3and the area of the base is20cm2,then the height of the box is60=3cm.Therefore,each of the four identical squares has side length3cm,because the edges of these squares form the vertical edges of thebox.Therefore,the rectangular sheet measures3+5+3=11cm by3+4+3=10cm,and so has area11(10)=110cm2.Answer:(B) 17.Solution1Since SUR is a straight line,then∠RUV=180◦−∠SUV=180◦−120◦=60◦.Since P W and QX are parallel,then∠RV W=∠V T X=112◦.Since UV W is a straight line,then∠RV U=180◦−∠RV W=180◦−112◦=68◦.Since the measures of the angles in a triangle add to180◦,then∠URV=180◦−∠RUV−∠RV U=180◦−60◦−68◦=52◦Solution2Since SUR is a straight line,then∠RUV=180◦−∠SUV=180◦−120◦=60◦.Since P W and QX are parallel,then∠RST=∠RUV=60◦.Since ST X is a straight line,then∠RT S=180◦−∠V T X=180◦−112◦=68◦.Since the measures of the angles in a triangle add to180◦,then∠URV=∠SRT=180◦−∠RST−∠RT S=180◦−60◦−68◦=52◦Answer:(A) 18.Solution1When Catherine adds30litres of gasoline,the tank goes from18full to34full.Since34−18=68−18=58,then58of the capacity of the tank is30litres.Thus,18of the capacity of the tank is30÷5=6litres.Also,the full capacity of the tank is8×6=48litres.Toﬁll the remaining14of the tank,Catherine must add an additional14×48=12litres of gas.Because each litre costs$1.38,it will cost12×$1.38=$16.56toﬁll the rest of the tank. Solution2Suppose that the capacity of the gas tank is x litres.Starting with1of a tank,30litres of gas makes the tank3full,so1x+30=3x or5x=30or x=48.The remaining capacity of the tank is14x=14(48)=12litres.At$1.38per litre,it will cost Catherine12×$1.38=$16.56toﬁll the rest of the tank.Answer:(C) 19.The area of a semi-circle with radius r is1πr2so the area of a semi-circle with diameter d is1 2π(12d)2=18πd2.The semicircles with diameters UV,V W,W X,XY,and Y Z each have equal diameter andthus equal area.The area of each of these semicircles is18π(52)=258π.The large semicircle has diameter UZ=5(5)=25,so has area18π(252)=6258π.The shaded area equals the area of the large semicircle,minus the area of two small semicircles, plus the area of three small semicircles,which equals the area of the large semicircle plus the area of one small semicircle.Therefore,the shaded area equals6258π+258π=6508π=3254π.Answer:(A)20.The sum of the odd numbers from5to21is5+7+9+11+13+15+17+19+21=117Therefore,the sum of the numbers in any row is one-third of this total,or39.This means as well that the sum of the numbers in any column or diagonal is also39.Since the numbers in the middle row add to39,then the number in the centre square is 39−9−17=13.Since the numbers in the middle column add to39,then the number in the middle square in the bottom row is39−5−13=21.591317x21Since the numbers in the bottom row add to39,then the number in the bottom right square is39−21−x=18−x.Since the numbers in the bottom left to top right diagonal add to39,then the number in the top right square is39−13−x=26−x.Since the numbers in the rightmost column add to39,then(26−x)+17+(18−x)=39or 61−2x=39or2x=22,and so x=11.We can complete the magic square as follows:195159131711217Answer:(B) 21.We label the numbers in the empty boxes as y and z,so the numbers in the boxes are thus8,y,z,26,x.Since the average of z and x is26,then x+z=2(26)=52or z=52−x.We rewrite the list as8,y,52−x,26,x.Since the average of26and y is52−x,then26+y=2(52−x)or y=104−26−2x=78−2x.We rewrite the list as8,78−2x,52−x,26,x.Since the average of8and52−x is78−2x,then8+(52−x)=2(78−2x)60−x=156−4x3x=96x=32Therefore,x=32.Answer:(D) 22.Since JKLM is a rectangle,then the angles at J and K are each90◦,so each of SJP andQKP is right-angled.By the Pythagorean Theorem in SJP,we haveSP2=JS2+JP2=522+392=2704+1521=4225Since SP>0,then SP=√4225=65.Since P QRS is a rhombus,then P Q=P S=65.By the Pythagorean Theorem in QKP,we haveKP2=P Q2−KQ2=652−252=4225−625=3600Since KP>0,then KP=√3600=60.(Instead of using the Pythagorean Theorem,we could note instead that SJP is a scaled-up version of a3-4-5right-angled triangle and that QKP is a scaled-up version of a5-12-13 right-angled triangle.This would allow us to use the known ratios of side lengths to calculate the missing side length.)Since KQ and P Z are parallel and P K and W Q are parallel,then P KQW is a rectangle,andso P W=KQ=25.Similarly,JP ZS is a rectangle and so P Z=JS=52.Thus,W Z=P Z−P W=52−25=27.Also,SY RM is a rectangle.Since JM and KL are parallel(JKLM is a rectangle),JK and ML are parallel,and P Q and SR are parallel(P QRS is a rhombus),then∠MSR=∠KQP and∠SRM=∠QP K.Since SMR and QKP have two equal angles,then their third angles must be equal too.Thus,the triangles have the same proportions.Since the hypotenuses of the triangles are equal, then the triangles must in fact be exactly the same size;that is,the lengths of the corresponding sides must be equal.(We say that SMR is congruent to QKP by“angle-side-angle”.) In particular,MR=KP=60.Thus,ZY=SY−SZ=MR−JP=60−39=21.Therefore,the perimeter of rectangle W XY Z is2(21)+2(27)=96.Answer:(D) 23.First,we note that2010=10(201)=2(5)(3)(67)and so20102=223252672.Consider N consecutive four-digit positive integers.For the product of these N integers to be divisible by20102,it must be the case that two diﬀerent integers are divisible by67(which would mean that there are at least68integers in the list)or one of the integers is divisible by672.Since we want to minimize N(and indeed because none of the answer choices is at least68), we look for a list of integers in which one is divisible by672=4489.Since the integers must all be four-digit integers,then the only multiples of4489the we must consider are4489and8978.First,we consider a list of N consecutive integers including4489.Since the product of these integers must have2factors of5and no single integer within10 of4489has a factor of25,then the list must include two integers that are multiples of5.To minimize the number of integers in the list,we try to include4485and4490.Thus our candidate list is4485,4486,4487,4488,4489,4490.The product of these integers includes2factors of67(in4489),2factors of5(in4485and 4490),2factors of2(in4486and4488),and2factors of3(since each of4485and4488is divisible by3).Thus,the product of these6integers is divisible by20102.Therefore,the shortest possible list including4489has length6.Next,we consider a list of N consecutive integers including8978.Here,there is a nearby integer containing2factors of5,namely8975.So we start with the list8975,8976,8977,8978and check to see if it has the required property.The product of these integers includes2factors of67(in8978),2factors of5(in8975),and2 factors of2(in8976).However,the only integer in this list divisible by3is8976,which has only1factor of3.To include a second factor of3,we must include a second multiple of3in the list.Thus,we extend the list by one number to8979.Therefore,the product of the numbers in the list8975,8976,8977,8978,8979is a multiple of 20102.The length of this list is5.Thus,the smallest possible value of N is5.(Note that a quick way to test if an integer is divisible by3is to add its digit and see if this total is divisible by3.For example,the sum of the digits of8979is33;since33is a multiple of3,then8979is a multiple of3.)Answer:(A)24.We label the terms x1,x2,x3,...,x2009,x2010.Suppose that S is the sum of the odd-numbered terms in the sequence;that is,S=x1+x3+x5+···+x2007+x2009We know that the sum of all of the terms is5307;that is,x1+x2+x3+···+x2009+x2010=5307Next,we pair up the terms:each odd-numbered term with the following even-numbered term.That is,we pair theﬁrst term with the second,the third term with the fourth,and so on,until we pair the2009th term with the2010th term.There are1005such pairs.In each pair,the even-numbered term is one bigger than the odd-numbered term.That is, x2−x1=1,x4−x3=1,and so on.Therefore,the sum of the even-numbered terms is1005greater than the sum of the odd-numbered terms.Thus,the sum of the even-numbered terms is S+1005.Since the sum of all of the terms equals the sum of the odd-numbered terms plus the sum of the even-numbered terms,then S+(S+1005)=5307or2S=4302or S=2151.Thus,the required sum is2151.Answer:(C) 25.Before we answer the given question,we determine the number of ways of choosing3objectsfrom5objects and the number of ways of choosing2objects from5objects.Consider5objects labelled B,C,D,E,F.The possible pairs are:BC,BD,BE,BF,CD,CE,CF,DE,DF,EF.There are10such pairs.The possible triples are:DEF,CEF,CDF,CDE,BEF,BDF,BDE,BCF,BCE,BCD.There are10such triples.(Can you see why there are the same number of pairs and triples?)Label the six teams A,B,C,D,E,F.We start by considering team A.Team A plays3games,so we must choose3of the remaining5teams for A to play.As we saw above,there are10ways to do this.Without loss of generality,we pick one of these sets of3teams for A to play,say A plays B,C and D.We keep track of everything by drawing diagrams,joining the teams that play each other witha line.Thus far,we haveAB C DThere are two possible cases now–either none of B,C and D play each other,or at least one pair of B,C,D plays each other.Case1:None of the teams that play A play each otherIn the conﬁguration above,each of B,C and D play two more games.They already play A and cannot play each other,so they must each play E and F.This givesAE FB C DNo further choices are possible.There are10possible schedules in this type of conﬁguration.These10combinations come from choosing the3teams that play A.Case2:Some of the teams that play A play each otherHere,at least one pair of the teams that play A play each other.Given the teams B,C and D playing A,there are3possible pairs(BC,BD,CD).We pick one of these pairs,say BC.(This gives10×3=30conﬁgurations so far.)AB C DIt is now not possible for B or C to also play D.If it was the case that C,say,played D,then we would have the conﬁgurationAB C DE FHere,A and C have each played3games and B and D have each played2games.Teams E and F are unaccounted for thus far.They cannot both play3games in this conﬁguration as the possible opponents for E are B,D and F,and the possible opponents for F are B,D and E,with the“B”and“D”possibilities only to be used once.A similar argument shows thatB cannot play D.Thus,B or C cannot also play D.So we have the conﬁgurationAB C DHere,A has played3games,B and C have each played2games,and D has played1game.B andC must play1more game and cannot playD or A.They must play E and F in some order.There are2possible ways to assign these games(BE and CF,or BF and CE.)This gives30×2=60conﬁgurations so far.Suppose that B plays E and C plays F.AB C DE FSo far,A,B and C each play3games and E,F and D each play1game.The only way to complete the conﬁguration is to join D,E and F.AB C DE FTherefore,there are60possible schedules in this case.In total,there are10+60=70possible schedules.Answer:(E)。

国际中学生数学竞赛含金量排行榜

国际中学生数学竞赛含金量排行榜
国际中学生数学竞赛的含金量因赛事的权威性、参赛选手水平、奖项设置等因素而有所不同。

以下是部分国际中学生数学竞赛及简要介绍：
1. 国际数学奥林匹克（IMO）：这是最具权威性的国际中学生数学竞赛之一，每年有来自世界各地的参赛选手。

IMO的奖项分为金牌、银牌和铜牌，其
中金牌是最高荣誉。

获奖者将获得世界范围内的认可和奖励，对于未来的学术和职业发展具有重要意义。

2. 亚洲太平洋数学奥林匹克（APMO）：这是亚太地区最高水平的数学竞赛，每年有来自多个国家和地区的代表队参赛。

APMO的奖项分为金、银、铜
牌和优秀奖，其中金牌是最高荣誉。

获奖者将获得国际范围内的认可和奖励，对于未来的学术和职业发展具有重要意义。

3. 英国数学奥林匹克（BMO）：这是英国最高水平的数学竞赛，每年有来
自全国各地的参赛选手。

BMO的奖项分为金、银、铜牌和优秀奖，其中金
牌是最高荣誉。

获奖者将获得英国范围内的认可和奖励，对于未来的学术和职业发展具有重要意义。

4. 罗马尼亚数学奥林匹克（RMO）：这是罗马尼亚最高水平的数学竞赛，
每年有来自全国各地的参赛选手。

RMO的奖项分为金、银、铜牌和优秀奖，其中金牌是最高荣誉。

获奖者将获得国际范围内的认可和奖励，对于未来的学术和职业发展具有重要意义。

总的来说，这些国际中学生数学竞赛都具有较高的含金量，获奖者将获得国际范围内的认可和奖励，对于未来的学术和职业发展具有重要意义。

Fryer滑铁卢数学竞赛(Grade 9)-数学Mathematics-2009-试题 exam

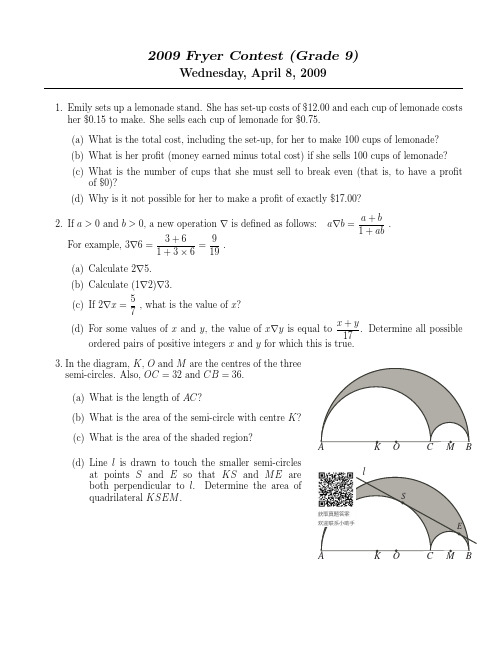
2009Fryer Contest(Grade9)Wednesday,April8,20091.Emily sets up a lemonade stand.She has set-up costs of$12.00and each cup of lemonade costsher$0.15to make.She sells each cup of lemonade for$0.75.(a)What is the total cost,including the set-up,for her to make100cups of lemonade?(b)What is her proﬁt(money earned minus total cost)if she sells100cups of lemonade?(c)What is the number of cups that she must sell to break even(that is,to have a proﬁtof$0)?(d)Why is it not possible for her to make a proﬁt of exactly$17.00?2.If a>0and b>0,a new operation∇is deﬁned as follows:a∇b=a+b 1+ab.For example,3∇6=3+61+3×6=919.(a)Calculate2∇5.(b)Calculate(1∇2)∇3.(c)If2∇x=57,what is the value of x?(d)For some values of x and y,the value of x∇y is equal to x+y17.Determine all possibleordered pairs of positive integers x and y for which this is true.3.In the diagram,K,O and M are the centres of the three semi-circles.Also,OC=32and CB=36.(a)What is the length of AC?(b)What is the area of the semi-circle with centre K?(c)What is the area of the shadedregion?(d)Line l is drawn to touch the smaller semi-circlesat points S and E so that KS and ME are both perpendicular to l.Determine the area of quadrilateral KSEM.2009Fryer Contest Page24.The addition shown below,representing2+22+222+2222+···,has101rows and the lastterm consists of1012’s:2222222222...22 (2222)+222 (2222)···C B A(a)Determine the value of the ones digit A.(b)Determine the value of the tens digit B and the value of the hundreds digit C.(c)Determine the middle digit of the sum.。

滑铁卢数学竞赛

滑铁卢数学竞赛1、21.|x|>3表示的区间是（）[单选题] *A.（-∞，3）B.(-3,3)C. [-3,3]D. （-∞，-3）∪（3，+ ∞）(正确答案)2、15.下列数中，是无理数的为（）[单选题] *A.-3.14B.6/11C.√3(正确答案)D.03、9.一棵树在离地5米处断裂，树顶落在离树根12米处，问树断之前有多高（）[单选题] *A. 17(正确答案)B. 17.5C. 18D. 204、若tan（π-α）>0且cosα>0，则角α的终边在（）[单选题] *A.第一象限B.第二象限C.第三象限D.第四象限(正确答案)5、下列说法正确的是[单选题] *A.带“+”号和带“-”号的数互为相反数B.数轴上原点两侧的两个点表示的数是相反数C.和一个点距离相等的两个点所表示的数一定互为相反数D.一个数前面添上“-”号即为原数的相反数(正确答案)6、16、在中,则( ). [单选题] *A. AB<2AC (正确答案)B. AB=2ACC. AB>2ACD. AB与2AC关系不确定7、260°是第（）象限角？[单选题] *第一象限第二象限第三象限(正确答案)第四象限8、4.已知两圆的半径分别为3㎝和4㎝，两个圆的圆心距为10㎝,则两圆的位置关系是（）[单选题] *Ａ.内切Ｂ.相交Ｃ.外切Ｄ.外离(正确答案)9、下列各角中，是界限角的是（）[单选题] *A. 1200°B. -1140°C. -1350°(正确答案)D. 1850°10、下列各对象可以组成集合的是（）[单选题] *A、与1非常接近的全体实数B、与2非常接近的全体实数(正确答案)C、高一年级视力比较好的同学D、与无理数相差很小的全体实数11、若2?＝a2＝4 ?，则a?等于( ) [单选题] *A. 43B. 82C. 83(正确答案)D. 4?12、47、若△ABC≌△DEF，AB＝2，AC＝4，且△DEF的周长为奇数，则EF的值为（）[单选题] *A．3B．4C．1或3D．3或5(正确答案)13、8. 下列事件中，不可能发生的事件是(? ? )．[单选题] *A．明天气温为30℃B．学校新调进一位女教师C．大伟身长丈八(正确答案)D．打开电视机，就看到广告14、3．中国是最早采用正负数表示相反意义的量，并进行负数运算的国家．若零上10℃记作+10℃，则零下10℃可记作（）[单选题] *A．10℃B．0℃C.-10 ℃(正确答案)D．-20℃15、19．对于实数a、b、c,“a>b”是“ac2(c平方)>bc2(c平方) ; ”的（）[单选题] * A．充分不必要条件B．必要不充分条件(正确答案)C．充要条件D．既不充分也不必要条件16、22．如图棋盘上有黑、白两色棋子若干，找出所有使三颗颜色相同的棋在同一直线上的直线，满足这种条件的直线共有（）[单选题] *A．5条(正确答案)B．4条C．3条D．2条17、f（x）=-2x+5在x=1处的函数值为（）[单选题] *A、-3B、-4C、5D、3(正确答案)18、10. 如图所示，小明周末到外婆家，走到十字路口处，记不清哪条路通往外婆家，那么他一次选对路的概率是(? ? ?)．[单选题] *A．1/2B．1/3(正确答案)C．1/4D．119、15．如图所示，下列数轴的画法正确的是（）[单选题] *A．B．C．(正确答案)D．20、-950°是（）[单选题] *A. 第一象限角B. 第二象限角(正确答案)C. 第三象限角D. 第四象限角21、1．如图，∠AOB＝120°，∠AOC＝∠BOC，OM平分∠BOC，则∠AOM的度数为（）[单选题] *A．45°B．65°C．75°(正确答案)D．80°22、27．下列计算正确的是（）[单选题] *A．（﹣a3）2＝a6(正确答案)B．3a+2b＝5abC．a6÷a3＝a2D．（a+b）2＝a2+b223、16．“x2（x平方）－4x－5=0”是“x=5”的( ) [单选题] *A．充分不必要条件B．必要不充分条件(正确答案)C．充要条件D．既不充分也不必要条件24、19．下列两个数互为相反数的是（）[单选题] *A．（﹣）和﹣（﹣）B．﹣5和(正确答案)C．π和﹣14D．+20和﹣（﹣20）25、13．在数轴上，下列四个数中离原点最近的数是（）[单选题] *A．﹣4(正确答案)B．3C．﹣2D．626、14．命题“?x∈R，?n∈N*，使得n≥x2（x平方）”的否定形式是（）[单选题] * A．?x∈R，?n∈N*，使得n<x2B．?x∈R，?x∈N*，使得n<x2C．?x∈R，?n∈N*，使得n<x2D．?x∈R，?n∈N*，使得n<x2(正确答案)27、8．(2020·课标Ⅱ)已知集合U={－2，－1,0,1,2,3}，A={－1,0,1}，B={1,2}，则?U(A∪B)=( ) [单选题] *A．{－2,3}(正确答案)B．{－2,2,3}C．{－2，－1,0,3}D．{－2，－1,0,2,3}28、7人小组选出2名同学作正副组长，共有选法（）种。

1、下载文档前请自行甄别文档内容的完整性，平台不提供额外的编辑、内容补充、找答案等附加服务。
2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
3、如文档侵犯您的权益，请联系客服反馈,我们会尽快为您处理(人工客服工作时间：9:00-18:30)。

1. The value of (4 + 44 + 444) ÷ 4 is
(A) 111
(B) 123
(C) 459
(D) 489
(E) 456
2. Jing purchased eight identical items. If the total cost was $26, then the cost per item, in dollars, was
7. Scoring: Each correct answer is worth 5 in Part A, 6 in Part B, and 8 in Part C. There is no penalty for an incorrect answer. Each unanswered question is worth 2, to a maximum of 10 unanswered questions.
Friday, February 22, 2013
(outside of North America and South America)
Time: 60 minutes Calculators are permitted Instructions
©2012 University of Waterloo
Mass of Jeff s Pet Atlantic Cod
20
Mass in kg
10
00
2
4
6
8
Age in Years
(A) 3
(B) 7
(C) 4
(D) 6
(E) 5
5. What is the value of 13 + 23 + 33 + 43?
(A) 101
(B) 103
(C) 102
(A) 28
(B) 38
(C) 26
(D) 152
(E) 45
R
Q 3x
x P
S
T
15. If 4n = 642, then n equals
(A) 3
(B) 5
(C) 6
(D) 8
(E) 12
16. An integer x is chosen so that 3x + 1 is an even integer. Which of the following must be an odd integer?
(A) $4.80
(B) $1.50
(C) $4.50
(D) $6.00
(E) $7.50
10. The time on Байду номын сангаас cell phone is 3:52. How many minutes will pass before the phone next shows a time using each of the digits 2, 3 and 5 exactly once?
(A) x + 3
(B) x − 3
(C) 2x
(D) 7x + 4 (E) 5x + 3
17. The graph shows styles of music on a playlist. Country music songs are added to the playlist so that now 40% of the songs are Country. If the ratio of Hip Hop songs to Pop songs remains the same, what percentage of the total number of songs are now Hip Hop?
6. This is a multiple-choice test. Each question is followed by ﬁve possible answers marked A, B, C, D, and E. Only one of these is correct. After making your choice, ﬁll in the appropriate circle on the response form.
(A) −6
(B) 13
(C) 54
(D)
62 3
(E)
−
71 3
13. Which number from the set {1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11} must be removed so that the mean (average) of the numbers remaining in the set is 6.1?
1. Do not open the Contest booklet until you are told to do so.
2. You may use rulers, compasses and paper for rough work.
3. Be sure that you understand the coding system for your response form. If you are not sure, ask your teacher to clarify it. All coding must be done with a pencil, preferably HB. Fill in circles completely.
(A)
(B)
(C)
(D)
(E)
4. The graph shows the mass, in kilograms, of Jeﬀ’s pet Atlantic cod, given its age in years. What is the age of the cod when its mass is 15 kg?
The CENTRE for EDUCATION in MATHEMATICS and COMPUTING
cemc.uwaterloo.ca
Pascal Contest
(Grade 9)
Thursday, February 21, 2013
(in North America and South America)
(D) 105
(E) 104
6.
Erin walks
3 5
of the way home in 30 minutes.
If she continues to walk at the same
rate, how many minutes will it take her to walk the rest of the way home?
4. On your response form, print your school name and city/town in the box in the upper right corner.
5. Be certain that you code your name, age, sex, grade, and the Contest you are writing in the response form. Only those who do so can be counted as eligible students.
(A) 4
(B) 5
(C) 6
(D) 7
(E) 8
14. In the diagram, P QR is a straight line segment and QS = QT . Also, ∠P QS = x◦ and ∠T QR = 3x◦. If ∠QT S = 76◦, the value of x is
SR = 3. Point U is on QR with QU = 2. Point T is
6
on P S with ∠T UR = 90◦. What is the length of T R? P
T
S
(A) 3
(B) 4
(C) 5
(D) 6
(E) 7
3
Q 2U
R
9. Owen spends $1.20 per litre on gasoline. He uses an average of 1 L of gasoline to drive 12.5 km. How much will Owen spend on gasoline to drive 50 km?
How many times does the symbol ♥ occur within the ﬁrst 53 symbols of the pattern?
(A) 25
(B) 26
(C) 27
(D) 28
(E) 29
12. If x = 11, y = −8, and 2x − 3z = 5y, what is the value of z?
(A) 27
(B) 59
(C) 77
(D) 91
(E) 171
Part B: Each correct answer is worth 6.
11. The same sequence of four symbols repeats to form the following pattern:
♥ ♣ ♠ ♥ ♥ ♣ ♠ ♥ ♥ ♣ ♠ ♥ ...
radius 1. What is the area of the shaded region?
P
Q
(A) 16 − π2 (B) 16 − 4π (C) 4 − 4π
(D) 4 − 4π2 (E) 4 − π
S
R