加拿大数学竞赛历年试题(滑铁卢大学)

合集下载

2005滑铁卢竞赛试题答案

1.(a)Answer:a=5Since(a,a)lies on the line3x−y=10,then3a−a=10or2a=10or a=5.(b)Answer:(6,2)Solution1To get from A to B,we move2units to the right and1unit up.xSince C lies on the same straight line as A and B,then to get from B to C we move2 units to the right and1unit up twice,or4units to the right and2units up.Thus,the coordinates of C are(6,2).Solution2Label the origin as O and drop a perpendicular from C to P on the x-axis.xThen AOB is similar to CP B since both are right-angled and they have equal angles at B.Since BC=2AB,then CP=2AO=2(1)=2and BP=2BO=2(2)=4.Therefore,the coordinates of C are(2+4,0+2)=(6,2).(c)By the Pythagorean Theorem,AO2=AB2−OB2=502−402=900,so AO=30.Therefore,the coordinates of A are(0,30).By the Pythagorean Theorem,CD2=CB2−BD2=502−482=196,so CD=14.x Therefore,the coordinates of C are(40+48,14)=(88,14). Since M is the midpoint of AC,then the coordinates of M are1 2(0+88),12(30+14)=(44,22)2.(a)Answer:x=−2Solution1Since y=2x+3,then4y=4(2x+3)=8x+12.Since4y=8x+12and4y=5x+6,then8x+12=5x+6or3x=−6or x=−2.Solution2Since4y=5x+6,then y=54x+64=54x+32.Since y=2x+3and y=54x+32,then2x+3=54x+32or34x=−32or x=−2.Solution3Since the second equation contains a“5x”,we multiply theﬁrst equation by52to obtaina5x term,and obtain52y=5x+152.Subtracting this from4y=5x+6,we obtain32y=−32or y=−1.Since y=−1,then−1=2x+3or2x=−4or x=−2.(b)Answer:a=6Solution1Adding the three equations together,we obtain a−3b+b+2b+7c−2c−5c=−10+3+13 or a=6.Solution2Multiplying the second equation by3,we obtain3b−6c=9.Adding this new equation to theﬁrst equation,we obtain c=−1.Substituting this back into the original second equation,we obtain b=3+2c=1.Substituting into the third equation,a=−2b+5c+13=−2−5+13=6.(c)Solution1Let J be John’s score and M be Mary’s score.Since two times John’s score was60more than Mary’s score,then2J=M+60.Since two times Mary’s score was90more than John’s score,then2M=J+90.Adding these two equations,we obtain2J+2M=M+J+150or J+M=150orJ+M2=75.Therefore,the average of their two scores was75.(Note that we didn’t have to solve for their individual scores.)Solution2Let J be John’s score and M be Mary’s score.Since two times John’s score was60more than Mary’s score,then2J=M+60,so M=2J−60.Since two times Mary’s score was90more than John’s score,then2M=J+90.Substituting theﬁrst equation into the second,we obtain2(2J−60)=J+904J−120=J+903J=210J=70Substituting into M=2J−60gives M=80.Therefore,the average of their scores(ie.the average of70and80)is75.3.(a)Answer:x=50Simplifying using exponent rules,2(1612)+2(816)=2((24)12)+2((23)16)=2(248)+2(248)=4(248)=22(248)=250Therefore,since2x=2(1612)+2(816)=250,then x=50.(b)Solution1We factor the given equation(f(x))2−3f(x)+2=0as(f(x)−1)(f(x)−2)=0.Therefore,f(x)=1or f(x)=2.If f(x)=1,then2x−1=1or2x=2or x=1.If f(x)=2,then2x−1=2or2x=3or x=3..Therefore,the values of x are x=1or x=32Solution2Since f(x)=2x−1and(f(x))2−3f(x)+2=0,then(2x−1)2−3(2x−1)+2=04x2−4x+1−6x+3+2=04x2−10x+6=02x2−5x+3=0(x−1)(2x−3)=0Therfore,x=1or x=3.24.(a)Answer:1415Solution1The possible pairs of numbers on the tickets are(listed as ordered pairs):(1,2),(1,3), (1,4),(1,5),(1,6),(2,3),(2,4),(2,5),(2,6),(3,4),(3,5),(3,6),(4,5),(4,6),and(5,6).There areﬁfteen such pairs.(We treat the pair of tickets numbered2and4as being the same as the pair numbered4and2.)The pairs for which the smaller of the two numbers is less than or equal to4are(1,2), (1,3),(1,4),(1,5),(1,6),(2,3),(2,4),(2,5),(2,6),(3,4),(3,5),(3,6),(4,5),and(4,6).There are fourteen such pairs.Therefore,the probability of selecting such a pair of tickets is14.15Solution 2We ﬁnd the probability that the smaller number on the two tickets is NOT less than or equal to 4.Therefore,the smaller number on the two tickets is at least 5.Thus,the pair of numbers must be 5and 6,since two distinct numbers less than or equal to 6are being chosen.As in Solution 1,we can determine that there are ﬁfteen possible pairs that we can se-lected.Therefore,the probability that the smaller number on the two tickets is NOT less than orequal to 4is 115,so the probability that the smaller number on the two tickets IS less than or equal to 4is 1−115=1415.(b)Solution 1Since ∠HLP =60◦and ∠BLP =30◦,then ∠HLB =∠HLP −∠BLP =30◦.Also,since ∠HLP =60◦and ∠HP L =90◦,then ∠LHP =180◦−90◦−60◦=30◦.Therefore, HBL is isosceles and BL =HB =400m.In BLP ,BL =400m and ∠BLP =30◦,so LP =BL cos(30◦)=400 √32 =200√3m.Therefore,the distance between L and P is 200√3m.Solution 2Since ∠HLP =60◦and ∠BLP =30◦,then ∠HLB =∠HLP −∠BLP =30◦.Also,since ∠HLP =60◦and ∠HP L =90◦,then ∠LHP =180◦−90◦−60◦=30◦.Also,∠LBP =60◦.Let LP =x .HSince BLP is 30◦-60◦-90◦,then BP :LP =1:√3,so BP =1√3LP =1√3x .Since HLP is 30◦-60◦-90◦,then HP :LP =√3:1,so HP =√3LP =√3x .But HP =HB +BP so√3x =400+1√3x3x =400√3+x2x =400√3x =200√3Therefore,the distance from L to P is 200√3m.5.(a)Answer:(6,5)After 2moves,the goat has travelled 1+2=3units.After 3moves,the goat has travelled 1+2+3=6units.Similarly,after n moves,the goat has travelled a total of 1+2+3+···+n units.For what value of n is 1+2+3+···+n equal to 55?The fastest way to determine the value of n is by adding the ﬁrst few integers until we obtain a sum of 55.This will be n =10.(We could also do this by remembering that 1+2+3+···+n =12n (n +1)and solving for n this way.)So we must determine the coordinates of the goat after 10moves.We consider ﬁrst the x -coordinate.Since starting at (0,0)the goat has moved 2units in the positive x direction,4units in the negative x direction,6units in the positive x direction,8units in the negative x direction and 10units in the positive x direction,so its x coordinate should be 2−4+6−8+10=6.Similarly,its y -coordinate should be 1−3+5−7+9=5.Therefore,after having travelled a distance of 55units,the goat is at the point (6,5).(b)Solution 1Since the sequence 4,4r ,4r 2is also arithmetic,then the diﬀerence between 4r 2and 4r equals the diﬀerence between 4r and 4,or4r 2−4r =4r −44r 2−8r +4=0r 2−2r +1=0(r −1)2=Therefore,the only value of r is r =1.Solution 2Since the sequence 4,4r ,4r 2is also arithmetic,then we can write 4r =4+d and 4r 2=4+2d for some real number d .(Here,d is the common diﬀerence in this arithmetic sequence.)Then d =4r −4and 2d =4r 2−4or d =2r 2−2.Therefore,equating the two expressions for d ,we obtain 2r 2−2=4r −4or 2r 2−4r +2=0or r 2−2r +1=0or (r −1)2=0.Therefore,the only value of r is r =1.6.(a)Answer:4πFirst,we notice that whenever an equilateral triangle of side length 3is placed inside acircle of radius3with two of its vertices on the circle,then the third vertex will be at the centre of the circle.This is because if we place XY Z with Y and Z on the circle and connect Y and Z to the centre O,then OY=OZ=3,so OY Z is equilateral(since all three sides have length3).Thus XY Z and OY Z must be the same,so X is at the same point as O. Thus,in the starting position,A is at the centre of the circle.As the triangle is rotated about C,the point B traces out an arc of a circle of radius3. What fraction of the circle is traced out?When point A reaches point A1on the circle,we have AC=3and CA1=3.Since A is at the centre of the circle,then AA1=3as well,so AA1C is equilateral,and∠A1CA=60◦, so the triangle has rotated through60◦.1Therefore,B has traced out60◦360◦=16of a circle of radius3.Notice that A has also traced out an arc of the same length.When A reaches the circle, we have A and C on the circle,so B must be at the centre of the circle.Thus,on the next rotation,B again rotates through16of a circle of radius3as it movesto the circle.On the third rotation,the triangle rotates about B,so B does not move.After three rotations,the triangle will have A at the centre and B and C on the circle,with the net result that the triangle has rotated180◦about the centre of the circle.Thus,to return to its original position,the triangle must undergo three more of these rotations,and B will behave in the same way as it did for theﬁrst three rotations.Thus,in total,B moves four times along an arc equal to16of a circle of radius3.Therefore,the distance travelled by B is4(16)(2π(3))=4π.(b)In order to determine CD,we must determine one of the angles(or at least some infor-mation about one of the angles)in BCD.To do this,we look at∠A use the fact that∠A+∠C=180◦.ADCB 5674Using the cosine law in ABD ,we obtain72=52+62−2(5)(6)cos(∠A )49=61−60cos(∠A )cos(∠A )=15Since cos(∠A )=15and ∠A +∠C =180◦,then cos(∠C )=−cos(180◦−∠A )=−15.(We could have calculated the actual size of ∠A using cos(∠A )=15and then used this to calculate the size of ∠C ,but we would introduce the possibility of rounding error by doing this.)Then,using the cosine law in BCD ,we obtain72=42+CD 2−2(4)(CD )cos(∠C )49=16+CD 2−8(CD ) −150=5CD 2+8CD −1650=(5CD +33)(CD −5)So CD =−335or CD =5.(We could have also determined these roots using the quadratic formula.)Since CD is a length,it must be positive,so CD =5.(We could have also proceeded by using the sine law in BCD to determine ∠BDC and then found the size of ∠DBC ,which would have allowed us to calculate CD using the sine law.However,this would again introduce the potential of rounding error.)7.(a)Answer:Maximum =5,Minimum =1We rewrite by completing the square as f (x )=sin 2x −2sin x +2=(sin x −1)2+1.Therefore,since (sin x −1)2≥0,then f (x )≥1,and in fact f (x )=1when sin x =1(which occurs for instance when x =90◦).Thus,the minimum value of f (x )is 1.To maximize f (x ),we must maximize (sin x −1)2.Since −1≤sin x ≤1,then (sin x −1)2is maximized when sin x =−1(for instance,when x =270◦).In this case,(sin x −1)2=4,so f (x )=5.Thus,the maximum value of f (x )is 5.(b)From the diagram,the x -intercepts of the parabola are x =−k and x =3k .xy Since we are given that y =−14(x −r )(x −s ),then the x -intercepts are r and s ,so r and s equal −k and 3k in some order.Therefore,we can rewrite the parabola as y =−14(x −(−k ))(x −3k ).Since the point (0,3k )lies on the parabola,then 3k =−14(0+k )(0−3k )or 12k =3k 2or k 2−4k =0or k (k −4)=0.Thus,k =0or k =4.Since the two roots are distinct,then we cannot have k =0(otherwise both x -intercepts would be 0).Thus,k =4.This tells us that the equation of the parabola is y =−14(x +4)(x −12)or y =−14x 2+2x +12.We still have to determine the coordinates of the vertex,V .Since the x -intercepts of the parabola are −4and 12,then the x -coordinate of the vertex is the average of these intercepts,or 4.(We could have also used the fact that the x -coordinate is −b2a =−22(−14).)Therefore,the y -coordinate of the vertex is y =−14(42)+2(4)+12=16.Thus,the coordinates of the vertex are (4,16).8.(a)We look at the three pieces separately.If x <−4,f (x )=4so g (x )= 25−[f (x )]2=√25−42=√9=3.So g (x )is the horizontal line y =3when x <−4.If x >5,f (x )=−5so g (x )= 25−[f (x )]2= 25−(−5)2=√0=0.So g (x )is the horizontal line y =0when x >5.So far,our graph looks like this:If−4≤x≤5,f(x)=−x so g(x)=25−[f(x)]2=25−(−x)2=√25−x2.What is this shape?If y=g(x),then we have y=√25−x2or y2=25−x2or x2+y2=25.Therefore,this shape is a section of the upper half(since y is a positive square-root)of the circle x2+y2=25,ie.the circle with centre(0,0)and radius5.We must check the endpoints. When x=−4,we have g(−4)=25−(−4))2=3.When x=5,we have g(5)=√25−52=0.Therefore,the section of the circle connects up with the other two sections of our graph already in place.Thus,ourﬁnal graph is:(b)Solution 1Let the centres of the two circles be O 1and O 2.Join A and B to O 1and B and C to O 2.Designate two points W and X on either side of A on one tangent line,and two points Y and Z oneither side of C on the other tangent line.ZLet ∠XAB =θ.Since W X is tangent to the circle with centre O 1at A ,then O 1A is perpendicular to W X ,so ∠O 1AB =90◦−θ.Since O 1A =O 1B because both are radii,then AO 1B is isosceles,so ∠O 1BA =∠O 1AB =90◦−θ.Since the two circles are tangent at B ,then the line segment joining O 1and O 2passes through B ,ie.O 1BO 2is a straight line segment.Thus,∠O 2BC =∠O 1BA =90◦−θ,by opposite angles.Since O 2B =O 2C ,then similarly to above,∠O 2CB =∠O 2BC =90◦−θ.Since Y Z is tangent to the circle with centre O 2at C ,then O 2C is perpendicular to Y Z .Thus,∠Y CB =90◦−∠O 2CB =θ.Since ∠XAB =∠Y CB ,then W X is parallel to Y Z ,by alternate angles,as required.Solution2Let the centres of the two circles be O1and O2.Join A and B to O1and B and C to O2.Since AO1and BO1are radii of the same circle,AO1=BO1so AO1B is isosceles,so ∠O1AB=∠O1BA.Since BO2and CO2are radii of the same circle,BO2=CO2so BO2C is isosceles,so ∠O2BC=∠O2CB.Since the two circles are tangent at B,then O1BO2is a line segment(ie.the line segment joining O1and O2passes through the point of tangency of the two circles).Since O1BO2is straight,then∠O1BA=∠O2BC,by opposite angles.Thus,∠O1AB=∠O1BA=∠O2BC=∠O2CB.This tells us that AO1B is similar to BO2C,so∠AO1B=∠BO2C or∠AO1O2=∠CO2O1.Therefore,AO1is parallel to CO2,by alternate angles.But A and C are points of tangency,AO1is perpendicular to the tangent line at A and CO2is perpendicular to the tangent line at C.Since AO1and CO2are parallel,then the two tangent lines must be parallel.9.(a)Solution1We have(x−p)2+y2=r2and x2+(y−p)2=r2,so at the points of intersection,(x−p)2+y2=x2+(y−p)2x2−2px+p2+y2=x2+y2−2py+p2−2px=−2pyand so x=y(since we may assume that p=0otherwise the two circles would coincide).Therefore,a and b are the two solutions of the equation(x−p)2+x2=r2or2x2−2px+(p2−r2)=0or x2−px+12(p2−r2)=0.Using the relationship between the sum and product of roots of a quadratic equation andits coeﬃcients,we obtain that a+b=p and ab=12(p2−r2).(We could have solved for a and b using the quadratic formula and calculated these di-rectly.)So we know that a+b=p.Lastly,a2+b2=(a+b)2−2ab=p2−2 12(p2−r2)=r2,as required.Solution2Since the circles are reﬂections of one another in the line y=x,then the two points of intersection must both lie on the line y=x,ie.A has coordinates(a,a)and B has coordinates(b,b).Therefore,(a−p)2+a2=r2and(b−p)2+b2=r2,since these points lie on both circles.Subtracting the two equations,we get(b −p )2−(a −p )2+b 2−a 2=0((b −p )−(a −p ))((b −p )+(a −p ))+(b −a )(b +a )=0(b −a )(a +b −2p )+(b −a )(b +a )=0(b −a )(a +b −2p +b +a )=02(b −a )(a +b −p )=0Since a =b ,then we must have a +b =p ,as required.Since a +b =p ,then a −p =−b ,so substituting back into (a −p )2+a 2=r 2gives (−b )2+a 2=r 2,or a 2+b 2=r 2,as required.(b)We ﬁrst draw a diagram.yxWe know that C has coordinates (p,0)and D has coordinates (0,p ).Thus,the slope of line segment CD is −1.Since the points A and B both lie on the line y =x ,then the slope of line segment AB is 1.Therefore,AB is perpendicular to CD ,so CADB is a kite,and so its area is equal to 12(AB )(CD ).(We could derive this by breaking quadrilateral CADB into CAB and DAB .)Since C has coordinates (p,0)and D has coordinates (0,p ),then CD = p 2+(−p )2= 2p 2.(We do not know if p is positive,so this is not necessarily equal to √2p .)We know that A has coordinates (a,a )and B has coordinates (b,b ),soAB = (a −b )2+(a −b )2=√2a 2−4ab +2b 2= 2(a 2+b 2)−4ab = 2r 2−4 12(p 2−r 2) = 4r 2−2p 2Therefore,the area of quadrilateral CADB is 12(AB )(CD )=124r 2−2p 2 2p 2= 2r 2p 2−p 4To maximize this area,we must maximize 2r 2p 2−p 4=2r 2(p 2)−(p 2)2.Since r is ﬁxed,we can consider this as a quadratic polynomial in p 2.Since the coeﬃcient of (p 2)2is negative,then this is a parabola opening downwards,so we ﬁnd its maximum value by ﬁnding its vertex.The vertex of 2r 2(p 2)−(p 2)2is at p 2=−2r 22(−1)=r 2.So the maximum area of the quadrilateral occurs when p is chosen so that p 2=r 2.Since p 2=r 2,then (a +b )2=p 2=r 2so a 2+2ab +b 2=r 2.Since a 2+b 2=r 2,then 2ab =0so either a =0or b =0,and so either A has coordinates (0,0)or B has coordinates (0,0),ie.either A is the origin or B is the origin.(c)In (b),we calculated that AB = 4r 2−2p 2=√2 2r 2−p 2.Since r and p are integers (and we assume that neither r nor p is 0),then 2r 2−p 2=0,so the minimum possible non-negative value for 2r 2−p 2is 1,since 2r 2−p 2must be an integer.Therefore,the minimum possible distance between A and B should be √2√1=√2.Can we ﬁnd positive integers p and r that give us this value?Yes –if r =5and p =7,then 2r 2−p 2=1,so AB =√2.(There are in fact an inﬁnite number of positive integer solutions to the equation 2r 2−p 2=1or equivalently p 2−2r 2=−1.This type of equation is called Pell’s Equation.)10.(a)We proceed directly.On the ﬁrst pass from left to right,Josephine closes all of the even numbered lockers,leaving the odd ones open.The second pass proceeds from right to left.Before the pass,the lockers which are open are 1,3,...,47,49.On the second pass,she shuts lockers 47,43,39, (3)The third pass proceeds from left to right.Before the pass,the lockers which are open are 1,5,...,45,49.On the third pass,she shuts lockers 5,13, (45)This leaves lockers 1,9,17,25,33,41,49open.On the fourth pass,from right to left,lockers 41,25and 9are shut,leaving 1,17,33,49.On the ﬁfth pass,from left to right,lockers 17and 49are shut,leaving 1and 33open.On the sixth pass,from right to left,locker 1is shut,leaving 33open.Thus,f (50)=33.(b)&(c)Solution 1First,we note that if n =2k is even,then f (n )=f (2k )=f (2k −1)=f (n −1).See Solution 2for this justiﬁcation.Therefore,we only need to look for odd values of n in parts (b)and (c).Suppose that there was an n so that f (n )=2005,ie.2005is the last locker left open.On the ﬁrst pass,Josephine closes every other locker starting at the beginning,so she closes all lockers numbered m with m ≡0(mod 2).This leaves only odd-numbered lockers open,ie.only lockers m with m ≡1or 3(mod 4).On her second pass,she closes every other open locker,starting from the right-hand end.Thus,she will close every fourth locker from the original row.Since we want 2005to be left open and 2005≡1(mod 4),then she must close all lockers numbered m with m ≡3(mod 4).This leaves open only the lockers m with m ≡1(mod 4),or equivalently lockers with m ≡1or 5(mod 8).On her third pass,she closes every other open locker,starting from the left-hand end.Thus,she will close every eighth locker from the original row.Since locker1is still open,then she starts by closing locker5,and so closes all lockers mwith m≡5(mod8).But since2005≡5(mod8),then she closes locker2005on this pass,a contradiction.Therefore,there can be no integer n with f(n)=2005.Next,we show that there are inﬁnitely many positive integers n such that f(n)=f(2005).To do this,weﬁrst make a table of what happens when there are2005lockers in the row.We record the pass#,the direction of the pass,the leftmost locker that is open,therightmost locker that is open,all open lockers before the pass,which lockers will be closedon the pass,and which lockers will be left open after the pass:Pass#Dir.L Open R Open Open To close Leaves Open 1L to R12005All≡0(mod2)≡1(mod2) 2R to L12005≡1,3(mod4)≡3(mod4)≡1(mod4) 3L to R12005≡1,5(mod8)≡5(mod8)≡1(mod8) 4R to L12001≡1,9(mod16)≡9(mod16)≡1(mod16) 5L to R12001≡1,17(mod32)≡17(mod32)≡1(mod32) 6R to L11985≡1,33(mod64)≡33(mod64)≡1(mod64) 7L to R11985≡1,65(mod128)≡65(mod128)≡1(mod128) 8R to L11921≡1,129(mod256)≡1(mod256)≡129(mod256) 9L to R1291921≡129,385(mod512)≡385(mod512)≡129(mod512) 10R to L1291665≡129,641(mod1024)≡129(mod1024)≡641(mod1024) 11L to R6411665≡641,1665(mod2048)≡1665(mod2048)≡641(mod2048) Since there is only one integer between1and2005congruent to641(mod2048),thenthere is only one locker left open:locker641.Notice also that on any pass s,the“class”of lockers which are closed depends on what thenumber of the leftmost(on an odd-numbered pass)or rightmost(on an even-numberedpass)open locker number is congruent to mod2s.Consider n=2005+22a,where22a>2005,ie.a≥6.We show that f(n)=f(2005)=641.(See Solution2for a justiﬁcation of why we mighttry these values of n.)Suppose we were to try to make a table as above to calculate f(n).Then theﬁrst11passes in the table would be identical to the table above,except for therightmost open number;this number in the new table would be the number above plus22a.What will happen after pass11?After pass11,the lockers which are open are lockers with numbers≡641(mod2048).Thus,the leftmost open locker is641and the rightmost is22a+641.As the12th pass starts,the lockers which are still open are those with numbers≡641or2689(mod212).Since the rightmost open locker number(22a+641)is congruent to641(mod212),then alllockers with numbers≡2689(mod212)are closed,leaving open only those lockers withnumbers≡641(mod212).So after this12th pass,the lockers which are open are641,641+212,641+2(212),641+3(212),...,641+22a−12(212)=641+22a.The number of open lockers is22a−12+1.If we can now show that whenever we start with a number of lockers of the form22c+1,thelast locker remaining open is the leftmost locker,then we will be done,since of the lockersleft open above(22a−12+1of them,ie.2to an even power plus1),then the last locker re-maining open will be the leftmost one,that is locker641,so f(22a+2005)=641=f(2005).So consider a row of22c+1lockers.Notice that on any pass,if the number of lockers is odd,then the number of lockers whichwill be closed is one-half of one less than the total number of lockers,and theﬁrst andlast lockers will be left open.So on theﬁrst pass,there are22c−1lockers closed,leaving22c+1−22c−1=22c−1+1lockersopen,ie.an odd number of lockers open.On the next pass,there are22c−2lockers closed(since there are an odd number of lockersopen to begin),leaving22c−2+1lockers open.This continues,until there are21+1=3lockers open just before an even-numbered(ie.right to left)pass.Thus,the middle of these three lockers will be closed,leaving only theoriginal leftmost and rightmost lockers open.On the last pass(an odd-numbered pass from left to right),the rightmost locker will beclosed,leaving only the leftmost locker open.Therefore,starting with a row of22c+1open lockers,the leftmost locker will be the lastremaining open.Translating this to the above,we see that the leftmost locker of the22a−12+1still openis the last left open,ie.f(22a+2005)=641=f(2005)if a≥6.Therefore,there are inﬁnitely many positive integers n for which f(n)=f (2005).Solution2First,we calculate f(n)for n from1to32,to get a feeling for what happens.We obtain 1,1,3,3,1,1,3,3,9,9,11,11,9,9,11,11,1,1,3,3,1,1,3,3,9,9,11,11,9,9,11,11. This will help us to establish some patterns.Next,we establish two recursive formulas for f(n).First,from our pattern,it looks like f(2m)=f(2m−1).Why is this true in general?Consider a row of2m lockers.On theﬁrst pass through,Josephine shuts all of the even numbered lockers,leaving open lockers1,3,...,2m−1.These are exactly the same open lockers as if she had started with2m−1lockers in total. Thus,as she starts her second pass from right to left,the process will be the same now whether she started with2m lockers or2m−1lockers.Therefore,f(2m)=f(2m−1).This tells us that we need only focus on the values of f(n)where n is odd.Secondly,we show that f(2m−1)=2m+1−2f(m).(It is helpful to connect n=2m−1to a smaller case.)Why is this formula true?Starting with2m−1lockers,the lockers left open after theﬁrst pass are1,3,...,2m−1, ie.m lockers in total.Suppose f(m)=p.As Josephine begins her second pass,which is from right to left,we can think of this as being like theﬁrst pass through a row of m lockers.Thus,the last open locker will be the p th locker,counting from the right hand end,from the list1,3,...,2m−1.Theﬁrst locker from the right is2m−1=2m+1−2(1),the second is2m−3=2m+1−2(2), and so on,so the p th locker is2m+1−2p.Therefore,theﬁnal open locker is2m+1−2p,ie.f(2m−1)=2m+1−2p=2m+1−2f(m). Using these two formulae repeatedly,f(4k+1)=f(2(2k+1)−1)=2(2k+1)+1−2f(2k+1)=4k+3−2f(2(k+1)−1)=4k+3−2(2(k+1)+1−2f(k+1))=4k+3−2(2k+3−2f(k+1))=4f(k+1)−3andf(4k+3)=f(2(2k+2)−1)=2(2k+2)+1−2f(2k+2)=4k+5−2f(2k+1)=4k+5−2f(2(k+1)−1)=4k+5−2(2(k+1)+1−2f(k+1))=4k+5−2(2k+3−2f(k+1))=4f(k+1)−1From our initial list of values of f(n),it appears as if f(n)cannot leave a remainder of5 or7when divided by8.So we use these recursive relations once more to try to establish this:f(8l+1)=4f(2l+1)−3(since8l+1=4(2l)+1)=4(2l+3−2f(l+1))−3=8l+9−8f(l+1)=8(l−f(l+1))+9f(8l+3)=4f(2l+1)−1(since8l+3=4(2l)+3)=4(2l+3−2f(l+1))−1=8l+11−8f(l+1)=8(l−f(l+1))+11Similarly,f(8l+5)=8l+9−8f(l+1)and f(8l+7)=8l+11−8f(l+1). Therefore,since any odd positive integer n can be written as8l+1,8l+3,8l+5or8l+7, then for any odd positive integer n,f(n)is either9more or11more than a multiple of8. Therefore,for any odd positive integer n,f(n)cannot be2005,since2005is not9more or11more than a multiple of8.Thus,for every positive integer n,f(n)=2005,since we only need to consider odd values of n.Next,we show that there are inﬁnitely many positive integers n such that f(n)=f(2005). We do this by looking at the pattern we initially created and conjecturing thatf(2005)=f(2005+22a)if22a>2005.(We might guess this by looking at the connection between f(1)and f(3) with f(5)and f(7)and then f(1)through f(15)with f(17)through f(31).In fact,it appears to be true that f(m+22a)=f(m)if22a>m.)Using our formulae from above,f(2005+22a)=4f(502+22a−2)−3(2005+22a=4(501+22a−2)+1) =4f(501+22a−2)−3=4(4f(126+22a−4)−3)−3(501+22a−2=4(125+22a−4)+1)=16f(126+22a−4)−15=16f(125+22a−4)−15=16(4f(32+22a−6)−3)−15(125+22a−4=4(31+22a−6)+1)=64f(32+22a−6)−63=64f(31+22a−6)−63=64(4f(8+22a−8)−1)−63(31+22a−6=4(7+22a−8)+3)=256f(8+22a−8)−127=256f(7+22a−8)−127=256(4f(2+22a−10)−1)−127(7+22a−8=4(1+22a−10)+3)=1024f(2+22a−10)−383=1024f(1+22a−10)−383(Notice that we could have removed the powers of2from inside the functions and used this same approach to show that f(2005)=1024f(1)−383=641.)But,f(22b+1)=1for every positive integer b.Why is this true?We can prove this quickly by induction.For b=1,we know f(5)=1.Assume that the result is true for b=B−1,for some positive integer B≥2.Then f(22B+1)=f(4(22B−2)+1)=4f(22B−2+1)−3=4(1)−3=1by our induction hypothesis.Therefore,if a≥6,then f(1+22a−10)=f(1+22(a−5))=1sof(2005+22a)=1024(1)−383=641=f(2005)so there are inﬁnitely many integers n for which f(n)=f(2005).。

2011EuclidSolution

1.(a)Since (x +1)+(x +2)+(x +3)=8+9+10,then 3x +6=27or 3x =21and so x =7.(b)Since 25+√x =6,then squaring both sides gives 25+√x =36or √x =11.Since √x =11,then squaring both sides again,we obtain x =112=121.Checking, 25+√121=√25+11=√36=6,as required.(c)Since (a,2)is the point of intersection of the lines with equations y =2x −4and y =x +k ,then the coordinates of this point must satisfy both equations.Using the ﬁrst equation,2=2a −4or 2a =6or a =3.Since the coordinates of the point (3,2)satisfy the equation y =x +k ,then 2=3+k or k =−1.2.(a)Since the side length of the original square is 3and an equilateral triangle of side length 1is removed from the middle of each side,then each of the two remaining pieces of each side of the square has length 1.Also,each of the two sides of each of the equilateral triangles that are shown has length 1.1111Therefore,each of the 16line segments in the ﬁgure has length 1,and so the perimeter of the ﬁgure is 16.(b)Since DC =DB ,then CDB is isosceles and ∠DBC =∠DCB =15◦.Thus,∠CDB =180◦−∠DBC −∠DCB =150◦.Since the angles around a point add to 360◦,then∠ADC =360◦−∠ADB −∠CDB =360◦−130◦−150◦=80◦.(c)By the Pythagorean Theorem in EAD ,we have EA 2+AD 2=ED 2or 122+AD 2=132,and so AD =√169−144=5,since AD >0.By the Pythagorean Theorem in ACD ,we have AC 2+CD 2=AD 2or AC 2+42=52,and so AC =√25−16=3,since AC >0.(We could also have determined the lengths of AD and AC by recognizing 3-4-5and 5-12-13right-angled triangles.)By the Pythagorean Theorem in ABC ,we have AB 2+BC 2=AC 2or AB 2+22=32,and so AB =√9−4=√5,since AB >0.3.(a)Solution 1Since we want to make 15−y x as large as possible,then we want to subtract as little as possible from 15.In other words,we want to make y x as small as possible.To make a fraction with positive numerator and denominator as small as possible,wemake the numerator as small as possible and the denominator as large as possible.Since 2≤x ≤5and 10≤y ≤20,then we make x =5and y =10.Therefore,the maximum value of 15−y x is 15−105=13.Solution2Since y is positive and2≤x≤5,then15−yx≤15−y5for any x with2≤x≤5andpositive y.Since10≤y≤20,then15−y5≤15−105for any y with10≤y≤20.Therefore,for any x and y in these ranges,15−yx≤15−105=13,and so the maximumpossible value is13(which occurs when x=5and y=10).(b)Solution1First,we add the two given equations to obtain(f(x)+g(x))+(f(x)−g(x))=(3x+5)+(5x+7)or2f(x)=8x+12which gives f(x)=4x+6.Since f(x)+g(x)=3x+5,then g(x)=3x+5−f(x)=3x+5−(4x+6)=−x−1.(We could alsoﬁnd g(x)by subtracting the two given equations or by using the second of the given equations.)Since f(x)=4x+6,then f(2)=14.Since g(x)=−x−1,then g(2)=−3.Therefore,2f(2)g(2)=2×14×(−3)=−84.Solution2Since the two given equations are true for all values of x,then we can substitute x=2to obtainf(2)+g(2)=11f(2)−g(2)=17Next,we add these two equations to obtain2f(2)=28or f(2)=14.Since f(2)+g(2)=11,then g(2)=11−f(2)=11−14=−3.(We could alsoﬁnd g(2)by subtracting the two equations above or by using the second of these equations.)Therefore,2f(2)g(2)=2×14×(−3)=−84.4.(a)We consider choosing the three numbers all at once.We list the possible sets of three numbers that can be chosen:{1,2,3}{1,2,4}{1,2,5}{1,3,4}{1,3,5}{1,4,5}{2,3,4}{2,3,5}{2,4,5}{3,4,5} We have listed each in increasing order because once the numbers are chosen,we arrange them in increasing order.There are10sets of three numbers that can be chosen.Of these10,the4sequences1,2,3and1,3,5and2,3,4and3,4,5are arithmetic sequences.Therefore,the probability that the resulting sequence is an arithmetic sequence is410or25.(b)Solution 1Join B to D .AConsider CBD .Since CB =CD ,then ∠CBD =∠CDB =12(180◦−∠BCD )=12(180◦−60◦)=60◦.Therefore, BCD is equilateral,and so BD =BC =CD =6.Consider DBA .Note that ∠DBA =90◦−∠CBD =90◦−60◦=30◦.Since BD =BA =6,then ∠BDA =∠BAD =12(180◦−∠DBA )=12(180◦−30◦)=75◦.We calculate the length of AD .Method 1By the Sine Law in DBA ,we have AD sin(∠DBA )=BA sin(∠BDA ).Therefore,AD =6sin(30◦)sin(75◦)=6×12sin(75◦)=3sin(75◦).Method 2If we drop a perpendicular from B to P on AD ,then P is the midpoint of AD since BDA is isosceles.Thus,AD =2AP .Also,BP bisects ∠DBA ,so ∠ABP =15◦.Now,AP =BA sin(∠ABP )=6sin(15◦).Therefore,AD =2AP =12sin(15◦).Method 3By the Cosine Law in DBA ,AD 2=AB 2+BD 2−2(AB )(BD )cos(∠ABD )=62+62−2(6)(6)cos(30◦)=72−72(√32)=72−36√3Therefore,AD = 36(2−√3)=6 2−√3since AD >0.Solution 2Drop perpendiculars from D to Q on BC and from D to R on BA .AThen CQ =CD cos(∠DCQ )=6cos(60◦)=6×12=3.Also,DQ =CD sin(∠DCQ )=6sin(60◦)=6×√32=3√3.Since BC =6,then BQ =BC −CQ =6−3=3.Now quadrilateral BQDR has three right angles,so it must have a fourth right angle and so must be a rectangle.Thus,RD =BQ =3and RB =DQ =3√3.Since AB =6,then AR =AB −RB =6−3√3.Since ARD is right-angled at R ,then using the Pythagorean Theorem and the fact that AD >0,we obtain AD =√RD 2+AR 2= 32+(6−3√3)2= 9+36−36√3+27= 72−36√3which we can rewrite as AD = 36(2−√3)=6 2−√3.5.(a)Let n be the original number and N be the number when the digits are reversed.Sincewe are looking for the largest value of n ,we assume that n >0.Since we want N to be 75%larger than n ,then N should be 175%of n ,or N =74n .Suppose that the tens digit of n is a and the units digit of n is b .Then n =10a +b .Also,the tens digit of N is b and the units digit of N is a ,so N =10b +a .We want 10b +a =74(10a +b )or 4(10b +a )=7(10a +b )or 40b +4a =70a +7b or 33b =66a ,and so b =2a .This tells us that that any two-digit number n =10a +b with b =2a has the required property.Since both a and b are digits then b <10and so a <5,which means that the possible values of n are 12,24,36,and 48.The largest of these numbers is 48.(b)We “complete the rectangle”by drawing a horizontal line through C which meets they -axis at P and the vertical line through B at Q .x A (0,Since C has y -coordinate 5,then P has y -coordinate 5;thus the coordinates of P are (0,5).Since B has x -coordinate 4,then Q has x -coordinate 4.Since C has y -coordinate 5,then Q has y -coordinate 5.Therefore,the coordinates of Q are (4,5),and so rectangle OP QB is 4by 5and so has area 4×5=20.Now rectangle OP QB is made up of four smaller triangles,and so the sum of the areas of these triangles must be 20.Let us examine each of these triangles:• ABC has area 8(given information)• AOB is right-angled at O ,has height AO =3and base OB =4,and so has area 12×4×3=6.• AP C is right-angled at P ,has height AP =5−3=2and base P C =k −0=k ,and so has area 1×k ×2=k .• CQB is right-angled at Q ,has height QB =5−0=5and base CQ =4−k ,andso has area 12×(4−k )×5=10−52k .Since the sum of the areas of these triangles is 20,then 8+6+k +10−52k =20or 4=32k and so k =83.6.(a)Solution 1Suppose that the distance from point A to point B is d km.Suppose also that r c is the speed at which Serge travels while not paddling (i.e.being carried by just the current),that r p is the speed at which Serge travels with no current (i.e.just from his paddling),and r p +c his speed when being moved by both his paddling and the current.It takes Serge 18minutes to travel from A to B while paddling with the current.Thus,r p +c =d 18km/min.It takes Serge 30minutes to travel from A to B with just the current.Thus,r c =d 30km/min.But r p =r p +c −r c =d 18−d 30=5d 90−3d 90=2d 90=d 45km/min.Since Serge can paddle the d km from A to B at a speed of d 45km/min,then it takes him 45minutes to paddle from A to B with no current.Solution 2Suppose that the distance from point A to point B is d km,the speed of the current of the river is r km/h,and the speed that Serge can paddle is s km/h.Since the current can carry Serge from A to B in 30minutes (or 12h),then d r =12.When Serge paddles with the current,his speed equals his paddling speed plus the speed of the current,or (s +r )km/h.Since Serge can paddle with the current from A to B in 18minutes (or 310h),then d r +s =310.The time to paddle from A to B with no current would be d sh.Since d r =12,then r d =2.Since d r +s =310,then r +s d =103.Therefore,s d =r +s d −r d =103−2=43.Thus,d s =34,and so it would take Serge 34of an hour,or 45minutes,to paddle from A to B with no current.Solution 3Suppose that the distance from point A to point B is d km,the speed of the current of the river is r km/h,and the speed that Serge can paddle is s km/h.Since the current can carry Serge from A to B in 30minutes (or 12h),then d r =12or d =1r .When Serge paddles with the current,his speed equals his paddling speed plus the speed of the current,or (s +r )km/h.Since Serge can paddle with the current from A to B in 18minutes (or 310h),then d r +s =310or d =310(r +s ).Since d =12r and d =310(r +s ),then 12r =310(r +s )or 5r =3r +3s and so s =23r .To travel from A to B with no current,the time in hours that it takes is d s =12r 2r =34,or 45minutes.(b)First,we note that a =0.(If a =0,then the “parabola”y =a (x −2)(x −6)is actuallythe horizontal line y =0which intersects the square all along OR .)Second,we note that,regardless of the value of a =0,the parabola has x -intercepts 2and 6,and so intersects the x -axis at (2,0)and (6,0),which we call K (2,0)and L (6,0).This gives KL =4.Third,we note that since the x -intercepts of the parabola are 2and 6,then the axis ofsymmetry of the parabola has equation x =12(2+6)=4.Since the axis of symmetry of the parabola is a vertical line of symmetry,then if theparabola intersects the two vertical sides of the square,it will intersect these at the same height,and if the parabola intersects the top side of the square,it will intersect it at two points that are symmetrical about the vertical line x =4.Fourth,we recall that a trapezoid with parallel sides of lengths a and b and height h hasarea 12h (a +b ).We now examine three cases.Case1:a<0Here,the parabola opens downwards.Since the parabola intersects the square at four points,it must intersect P Q at points M and N.(The parabola cannot intersect the vertical sides of the square since it gets “narrower”towards the vertex.)xx =4Since the parabola opens downwards,then MN<KL=4.Since the height of the trapezoid equals the height of the square(or8),then the area of the trapezoid is1h(KL+MN)which is less than1(8)(4+4)=32.But the area of the trapezoid must be36,so this case is not possible.Case2:a>0;M and N on P QWe have the following conﬁguration:xx =4Here,the height of the trapezoid is8,KL=4,and M and N are symmetric about x=4.Since the area of the trapezoid is36,then12h(KL+MN)=36or12(8)(4+MN)=36or4+MN=9or MN=5.Thus,M and N are each52units from x=4,and so N has coordinates(32,8).Since this point lies on the parabola with equation y=a(x−2)(x−6),then8=a(32−2)(32−6)or8=a(−12)(−92)or8=94a or a=329.Case3:a>0;M and N on QR and P Oxx =4Here,KL=4,MN=8,and M and N have the same y-coordinate.Since the area of the trapezoid is36,then12h(KL+MN)=36or12h(4+8)=36or6h=36or h=6.Thus,N has coordinates(0,6).Since this point lies on the parabola with equation y=a(x−2)(x−6),then 6=a(0−2)(0−6)or6=12a or a=12.Therefore,the possible values of a are329and12.7.(a)Solution1Consider a population of100people,each of whom is75years old and who behave ac-cording to the probabilities given in the question.Each of the original100people has a50%chance of living at least another10years,so there will be50%×100=50of these people alive at age85.Each of the original100people has a20%chance of living at least another15years,so there will be20%×100=20of these people alive at age90.Since there is a25%(or14)chance that an80year old person will live at least another10years(that is,to age90),then there should be4times as many of these people alive at age80than at age90.Since there are20people alive at age90,then there are4×20=80of the original100 people alive at age80.In summary,of the initial100people of age75,there are80alive at age80,50alive at age85,and20people alive at age90.Because50of the80people alive at age80are still alive at age85,then the probability that an80year old person will live at least5more years(that is,to age85)is50=5,or 62.5%.Solution2Suppose that the probability that a75year old person lives to80is p,the probability that an80year old person lives to85is q,and the probability that an85year old person lives to90is r.We want to the determine the value of q.For a75year old person to live at least another10years,they must live another5years (to age80)and then another5years(to age85).The probability of this is equal to pq. We are told in the question that this is equal to50%or0.5.Therefore,pq=0.5.For a75year old person to live at least another15years,they must live another5years (to age80),then another5years(to age85),and then another5years(to age90).The probability of this is equal to pqr.We are told in the question that this is equal to20% or0.2.Therefore,pqr=0.2Similarly,since the probability that an80year old person will live another10years is25%,then qr=0.25.Since pqr=0.2and pq=0.5,then r=pqrpq=0.20.5=0.4.Since qr=0.25and r=0.4,then q=qrr=0.250.4=0.625.Therefore,the probability that an80year old man will live at least another5years is0.625,or62.5%.(b)Using logarithm rules,the given equation is equivalent to22log10x=3(2·2log10x)+16or(2log10x)2=6·2log10x+16.Set u=2log10x.Then the equation becomes u2=6u+16or u2−6u−16=0.Factoring,we obtain(u−8)(u+2)=0and so u=8or u=−2.Since2a>0for any real number a,then u>0and so we can reject the possibility that u=−2.Thus,u=2log10x=8which means that log10x=3.Therefore,x=1000.8.(a)First,we determine theﬁrst entry in the50th row.Since theﬁrst column is an arithmetic sequence with common diﬀerence3,then the50th entry in theﬁrst column(theﬁrst entry in the50th row)is4+49(3)=4+147=151.Second,we determine the common diﬀerence in the50th row by determining the second entry in the50th row.Since the second column is an arithmetic sequence with common diﬀerence5,then the 50th entry in the second column(that is,the second entry in the50th row)is7+49(5) or7+245=252.Therefore,the common diﬀerence in the50th row must be252−151=101.Thus,the40th entry in the50th row(that is,the number in the50th row and the40th column)is151+39(101)=151+3939=4090.(b)We follow the same procedure as in(a).First,we determine theﬁrst entry in the R th row.Since theﬁrst column is an arithmetic sequence with common diﬀerence3,then the R th entry in theﬁrst column(that is,theﬁrst entry in the R th row)is4+(R−1)(3)or 4+3R−3=3R+1.Second,we determine the common diﬀerence in the R th row by determining the second entry in the R th row.Since the second column is an arithmetic sequence with common diﬀerence5,then the R th entry in the second column(that is,the second entry in the R th row)is7+(R−1)(5) or7+5R−5=5R+2.Therefore,the common diﬀerence in the R th row must be(5R+2)−(3R+1)=2R+1.Thus,the C th entry in the R th row(that is,the number in the R th row and the C th column)is3R+1+(C−1)(2R+1)=3R+1+2RC+C−2R−1=2RC+R+C(c)Suppose that N is an entry in the table,say in the R th row and C th column.From(b),then N=2RC+R+C and so2N+1=4RC+2R+2C+1.Now4RC+2R+2C+1=2R(2C+1)+2C+1=(2R+1)(2C+1).Since R and C are integers with R≥1and C≥1,then2R+1and2C+1are each integers that are at least3.Therefore,2N+1=(2R+1)(2C+1)must be composite,since it is the product of two integers that are each greater than1.9.(a)If n=2011,then8n−7=16081and so √8n−7≈126.81.Thus,1+√8n−72≈1+126.812≈63.9.Therefore,g(2011)=2(2011)+1+8(2011)−72=4022+ 63.9 =4022+63=4085.(b)To determine a value of n for which f(n)=100,we need to solve the equation2n−1+√8n−72=100(∗)Weﬁrst solve the equation2x−1+√8x−72=100(∗∗)because the left sides of(∗)and(∗∗)do not diﬀer by much and so the solutions are likely close together.We will try integers n in(∗)that are close to the solutions to(∗∗). Manipulating(∗∗),we obtain4x−(1+√8x−7)=2004x−201=√8x−7(4x−201)2=8x−716x2−1608x+40401=8x−716x2−1616x+40408=02x2−202x+5051=0By the quadratic formula,x=202±2022−4(2)(5051)2(2)=202±√3964=101±√992and so x≈55.47or x≈45.53.We try n=55,which is close to55.47:f(55)=2(55)−1+8(55)−72=110−1+√4332Since √433≈20.8,then1+√4332≈10.9,which gives1+√4332=10.Thus,f(55)=110−10=100.Therefore,a value of n for which f(n)=100is n=55.(c)We want to show that each positive integer m is in the range of f or the range of g ,butnot both.To do this,we ﬁrst try to better understand the “complicated”term of each of the func-tions –that is,the term involving the greatest integer function.In particular,we start witha positive integer k ≥1and try to determine the positive integers n that give 1+√8n −72 =k .By deﬁnition of the greatest integer function,the equation 1+√8n −72 =k is equiv-alent to the inequality k ≤1+√8n −72<k +1,from which we obtain the following set of equivalent inequalities 2k ≤1+√8n −7<2k +22k −1≤√8n −7<2k +14k 2−4k +1≤8n −7<4k 2+4k +14k 2−4k +8≤8n <4k 2+4k +812(k 2−k )+1≤n <12(k 2+k )+1If we deﬁne T k =1k (k +1)=1(k 2+k )to be the k th triangular number for k ≥0,thenT k −1=12(k −1)(k )=12(k 2−k ).Therefore, 1+√8n −72 =k for T k −1+1≤n <T k +1.Since n is an integer,then 1+√8n −72=k is true for T k −1+1≤n ≤T k .When k =1,this interval is T 0+1≤n ≤T 1(or 1≤n ≤1).When k =2,this interval is T 1+1≤n ≤T 2(or 2≤n ≤3).When k =3,this interval is T 2+1≤n ≤T 3(or 4≤n ≤6).As k ranges over all positive integers,these intervals include every positive integer n and do not overlap.Therefore,we can determine the range of each of the functions f and g by examining the values f (n )and g (n )when n is in these intervals.For each non-negative integer k ,deﬁne R k to be the set of integers greater than k 2and less than or equal to (k +1)2.Thus,R k ={k 2+1,k 2+2,...,k 2+2k,k 2+2k +1}.For example,R 0={1},R 1={2,3,4},R 2={5,6,7,8,9},and so on.Every positive integer occurs in exactly one of these sets.Also,for each non-negative integer k deﬁne S k ={k 2+2,k 2+4,...,k 2+2k }and deﬁne Q k ={k 2+1,k 2+3,...,k 2+2k +1}.For example,S 0={},S 1={3},S 2={6,8},Q 0={1},Q 1={2,4},Q 2={5,7,9},and so on.Note that R k =Q k ∪S k so every positive integer occurs in exactly one Q k or in exactly one S k ,and that these sets do not overlap since no two S k ’s overlap and no two Q k ’s overlap and no Q k overlaps with an S k .We determine the range of the function g ﬁrst.For T k −1+1≤n ≤T k ,we have 1+√8n −72=k and so 2T k −1+2≤2n ≤2T k 2T k −1+2+k ≤2n + 1+√8n −72 ≤2T k +k k 2−k +2+k ≤g (n )≤k 2+k +k k 2+2≤g (n )≤k 2+2kNote that when n is in this interval and increases by 1,then the 2n term causes the value of g (n )to increase by 2.Therefore,for the values of n in this interval,g (n )takes precisely the values k 2+2,k 2+4,k 2+6,...,k 2+2k .In other words,the range of g over this interval of its domain is precisely the set S k .As k ranges over all positive integers (that is,as these intervals cover the domain of g ),this tells us that the range of g is precisely the integers in the sets S 1,S 2,S 3,....(We could also include S 0in this list since it is the empty set.)We note next that f (1)=2− 1+√8−72 =1,the only element of Q 0.For k ≥1and T k +1≤n ≤T k +1,we have 1+√8n −72=k +1and so 2T k +2≤2n ≤2T k +12T k +2−(k +1)≤2n − 1+√8n −72 ≤2T k +1−(k +1)k 2+k +2−k −1≤f (n )≤(k +1)(k +2)−k −1k 2+1≤f (n )≤k 2+2k +1Note that when n is in this interval and increases by 1,then the 2n term causes the value of f (n )to increase by 2.Therefore,for the values of n in this interval,f (n )takes precisely the values k 2+1,k 2+3,k 2+5,...,k 2+2k +1.In other words,the range of f over this interval of its domain is precisely the set Q k .As k ranges over all positive integers (that is,as these intervals cover the domain of f ),this tells us that the range of f is precisely the integers in the sets Q 0,Q 1,Q 2,....Therefore,the range of f is the set of elements in the sets Q 0,Q 1,Q 2,...and the range of g is the set of elements in the sets S 0,S 1,S 2,....These ranges include every positive integer and do not overlap.10.(a)Suppose that ∠KAB =θ.Since ∠KAC =2∠KAB ,then ∠KAC =2θand ∠BAC =∠KAC +∠KAB =3θ.Since 3∠ABC =2∠BAC ,then ∠ABC =23×3θ=2θ.Since ∠AKC is exterior to AKB ,then ∠AKC =∠KAB +∠ABC =3θ.This gives the following conﬁguration:BNow CAK is similar to CBA since the triangles have a common angle at C and ∠CAK =∠CBA .Therefore,AKBA=CACBordc=baand so d=bca.Also,CKCA=CACBora−xb=baand so a−x=b2aor x=a−b2a=a2−b2a,as required.(b)From(a),bc=ad and a2−b2=ax and so we obtainLS=(a2−b2)(a2−b2+ac)=(ax)(ax+ac)=a2x(x+c) andRS=b2c2=(bc)2=(ad)2=a2d2In order to show that LS=RS,we need to show that x(x+c)=d2(since a>0).Method1:Use the Sine LawFirst,we derive a formula for sin3θwhich we will need in this solution:sin3θ=sin(2θ+θ)=sin2θcosθ+cos2θsinθ=2sinθcos2θ+(1−2sin2θ)sinθ=2sinθ(1−sin2θ)+(1−2sin2θ)sinθ=3sinθ−4sin3θSince∠AKB=180◦−∠KAB−∠KBA=180◦−3θ,then using the Sine Law in AKB givesx sinθ=dsin2θ=csin(180◦−3θ)Since sin(180◦−X)=sin X,then sin(180◦−3θ)=sin3θ,and so x=d sinθsin2θandc=d sin3θsin2θ.This givesx(x+c)=d sinθsin2θd sinθsin2θ+d sin3θsin2θ=d2sinθsin22θ(sinθ+sin3θ)=d2sinθsin22θ(sinθ+3sinθ−4sin3θ)=d2sinθsin22θ(4sinθ−4sin3θ)=4d2sin2θsin22θ(1−sin2θ)=4d2sin2θcos2θsin22θ=4d2sin2θcos2θ(2sinθcosθ)2=4d2sin2θcos2θ4sin2θcos2θ=d2as required.We could have instead used the formula sin A +sin B =2sinA +B 2 cos A −B 2 toshow that sin 3θ+sin θ=2sin 2θcos θ,from which sin θ(sin 3θ+sin θ)=sin θ(2sin 2θcos θ)=2sin θcos θsin 2θ=sin 22θMethod 2:Extend ABExtend AB to E so that BE =BK =x and join KE .ENow KBE is isosceles with ∠BKE =∠KEB .Since ∠KBA is the exterior angle of KBE ,then ∠KBA =2∠KEB =2θ.Thus,∠KEB =∠BKE =θ.But this also tells us that ∠KAE =∠KEA =θ.Thus, KAE is isosceles and so KE =KA =d.ESo KAE is similar to BKE ,since each has two angles equal to θ.Thus,KA BK =AE KE or d x =c +x dand so d 2=x (x +c ),as required.Method 3:Use the Cosine Law and the Sine LawWe apply the Cosine Law in AKB to obtainAK 2=BK 2+BA 2−2(BA )(BK )cos(∠KBA )d 2=x 2+c 2−2cx cos(2θ)d 2=x 2+c 2−2cx (2cos 2θ−1)Using the Sine Law in AKB ,we get x sin θ=d sin 2θor sin 2θsin θ=d x or 2sin θcos θsin θ=d x and so cos θ=d 2x.Combining these two equations,d2=x2+c2−2cx2d24x2−1d2=x2+c2−cd2x+2cxd2+cd2x=x2+2cx+c2d2+cd2x=(x+c)2xd2+cd2=x(x+c)2d2(x+c)=x(x+c)2d2=x(x+c)as required(since x+c=0).(c)Solution1Our goal is toﬁnd a triple of positive integers that satisfy the equation in(b)and are the side lengths of a triangle.First,we note that if(A,B,C)is a triple of real numbers that satisﬁes the equation in(b)and k is another real number,then the triple(kA,kB,kC)also satisﬁes the equationfrom(b),since(k2A2−k2B2)(k2A2−k2B2+kAkC)=k4(A2−B2)(A2−B2+AC)=k4(B2C2)=(kB)2(kC)2 Therefore,we start by trying toﬁnd a triple(a,b,c)of rational numbers that satisﬁes the equation in(b)and forms a triangle,and then“scale up”this triple to form a triple (ka,kb,kc)of integers.To do this,we rewrite the equation from(b)as a quadratic equation in c and solve for c using the quadratic formula.Partially expanding the left side from(b),we obtain(a2−b2)(a2−b2)+ac(a2−b2)=b2c2which we rearrange to obtainb2c2−c(a(a2−b2))−(a2−b2)2=0By the quadratic formula,c=a(a2−b2)±a2(a2−b2)2+4b2(a2−b2)22b2=a(a2−b2)±(a2−b2)2(a2+4b2)2b2Since∠BAC>∠ABC,then a>b and so a2−b2>0,which givesc=a(a2−b2)±(a2−b2)√a2+4b22b2=(a2−b2)2b2(a±√a2+4b2)Since a2+4b2>0,then √a2+4b2>a,so the positive root isc=(a2−b2)2b2(a+a2+(2b)2)We try to ﬁnd integers a and b that give a rational value for c .We will then check to see if this triple (a,b,c )forms the side lengths of a triangle,and then eventually scale these up to get integer values.One way for the value of c to be rational (and in fact the only way)is for a 2+(2b )2to be an integer,or for a and 2b to be the legs of a Pythagorean triple.Since √32+42is an integer,then we try a =3and b =2,which givesc =(32−22)2·22(3+√32+42)=5and so (a,b,c )=(3,2,5).Unfortunately,these lengths do not form a triangle,since 3+2=5.(The Triangle Inequality tells us that three positive real numbers a ,b and c form a triangle if and only if a +b >c and a +c >b and b +c >a .)We can continue to try small Pythagorean triples.Now 152+82=172,but a =15and b =4do not give a value of c that forms a triangle with a and b .However,162+302=342,so we can try a =16and b =15which givesc =(162−152)2·152(16+√162+302)=31450(16+34)=319Now the lengths (a,b,c )=(16,15,319)do form the sides of a triangle since a +b >c and a +c >b and b +c >a .Since these values satisfy the equation from (b),then we can scale them up by a factor of k =9to obtain the triple (144,135,31)which satisﬁes the equation from (b)and are the side lengths of a triangle.(Using other Pythagorean triples,we could obtain other triples of integers that work.)Solution 2We note that the equation in (b)involves only a ,b and c and so appears to depend only on the relationship between the angles ∠CAB and ∠CBA in ABC .Using this premise,we use ABC ,remove the line segment AK and draw the altitude CF .CBA 3θ2θb aa c os 2θbc os 3θF Because we are only looking for one triple that works,we can make a number of assump-tions that may or may not be true in general for such a triangle,but which will help us ﬁnd an example.We assume that 3θand 2θare both acute angles;that is,we assume that θ<30◦.In ABC ,we have AF =b cos 3θ,BF =a cos 2θ,and CF =b sin 3θ=a sin 2θ.Note also that c =b cos 3θ+a cos 2θ.One way to ﬁnd the integers a,b,c that we require is to look for integers a and b and an angle θwith the properties that b cos 3θand a cos 2θare integers and b sin 3θ=a sin 2θ.Using trigonometric formulae,sin 2θ=2sin θcos θcos 2θ=2cos 2θ−1sin 3θ=3sin θ−4sin 3θ(from the calculation in (a),Solution 1,Method 1)cos 3θ=cos(2θ+θ)=cos 2θcos θ−sin 2θsin θ=(2cos 2θ−1)cos θ−2sin 2θcos θ=(2cos 2θ−1)cos θ−2(1−cos 2θ)cos θ=4cos 3θ−3cos θSo we can try to ﬁnd an angle θ<30◦with cos θa rational number and then integers a and b that make b sin 3θ=a sin 2θand ensure that b cos 3θand a cos 2θare integers.Since we are assuming that θ<30◦,then cos θ>√32≈0.866.The rational number with smallest denominator that is larger than √32is 78,so we try the acute angle θwith cos θ=7.In this case,sin θ=√1−cos 2θ=√158,and sosin 2θ=2sin θcos θ=2×78×√158=7√1532cos 2θ=2cos 2θ−1=2×4964−1=1732sin 3θ=3sin θ−4sin 3θ=3×√158−4×15√15512=33√15128cos 3θ=4cos 3θ−3cos θ=4×343512−3×78=7128To have b sin 3θ=a sin 2θ,we need 33√15128b =7√1532a or 33b =28a .To ensure that b cos 3θand a cos 2θare integers,we need 7128b and 1732a to be integers,andso a must be divisible by 32and b must be divisible by 128.The integers a =33and b =28satisfy the equation 33b =28a .Multiplying each by 32gives a =1056and b =896which satisfy the equation 33b =28a and now have the property that b is divisible by 128(with quotient 7)and a is divisible by 32(with quotient 33).With these values of a and b ,we obtain c =b cos 3θ+a cos 2θ=896×7128+1056×1732=610.We can then check that the triple (a,b,c )=(1056,896,610)satisﬁes the equation from(b),as required.As in our discussion in Solution 1,each element of this triple can be divided by 2to obtain the “smaller”triple (a,b,c )=(528,448,305)that satisﬁes the equation too.Using other values for cos θand integers a and b ,we could obtain other triples (a,b,c )of integers that work.。

滑铁卢竞赛数学题

滑铁卢竞赛数学题概述
滑铁卢竞赛数学题通常比较难，涉及的知识点广泛，包括代数、几何、数论、组合数学等多个领域。

以下是一些滑铁卢竞赛数学题的示例：
1. 有100个球，其中有一个与其他99个重量不同，但外观相同。

用一个天平，最少需要称多少次才能确定这个重量不同的球？
2. 一个正方形的面积为1，将其四边中点连接起来，形成另一个正方形。

如此重复，得到第五、第六个正方形，求第五个正方形的面积。

3. 一个圆被分成n个相等的扇形，其中一个是空心的，其他n-1个是实心的。

求空心扇形的圆心角是多少度？
4. 有100个人站成一排，从第1个人开始报数，每次报到奇数的人离开队伍。

经过若干轮后，只剩下一个人。

求这个人最初站在第几位？
5. 有5个不同质因数的最小正整数是多少？
以上仅是滑铁卢竞赛数学题的一些示例，实际上还有更多难题和技巧题。

如果想要深入了解滑铁卢竞赛数学题的解题技巧和策略，建议参考相关的竞赛书籍和资料，或者参加专业的数学竞赛培训课程。

欧几里得滑铁卢数学竞赛_2010EuclidSolution

Since Bea ﬂies at a constant speed, then the ratio of the two distances equals the ratio of
the corresponding times.
HF 60 minutes 4
Therefore, =
=.
GF 45 minutes 3
(b) Solution 1
Since ∠OP B = 90◦, then OP and P B are perpendicular, so the product of their slopes
is −1.
4−0 4
4−0
4
The slope of OP is
= and the slope of P B is
Since F GH is right-angled at F , then F GH must be similar to a 3-4-5 triangle, and
HG 5
so = .
GF 3
In
particular,
this
means
that
the
ratio
of
the
times
ﬂying
H
to
Wednesday, April 7, 2010
Solutions
©2010 Centre for Education in Mathematics and Computing
2010 Euclid Contest Solutions
Page 2
1. (a) Solution 1 Since 3x = 27, then 3x+2 = 3x32 = 27 · 9 = 243.

2002滑铁卢竞赛试题

Time: 2 1 2 hours
© 2002 Waterloo Mathematics Foundation
Calculators are permitted, provided they are non-programmable and without graphic displays. Do not open this booklet until instructed to do so. The paper consists of 10 questions, each worth 10 marks. Parts of each question can be of two types. SHORT ANSWER parts are worth 2 marks each (questions 1-2) or 3 marks each (questions 3-7). FULL SOLUTION parts are worth the remainder of the 10 marks for the question. Instructions for SHORT ANSWER parts: 1. SHORT ANSWER parts are indicated like this: .
Manulife Financial
Canadian Institute of Actuaries
Equitable Life of Canada
Great West Life and London Life
Chartered Accountants
Sybase Inc. (Waterloo)
iAnywhere Solutions
1 . f ( x)
(ii)

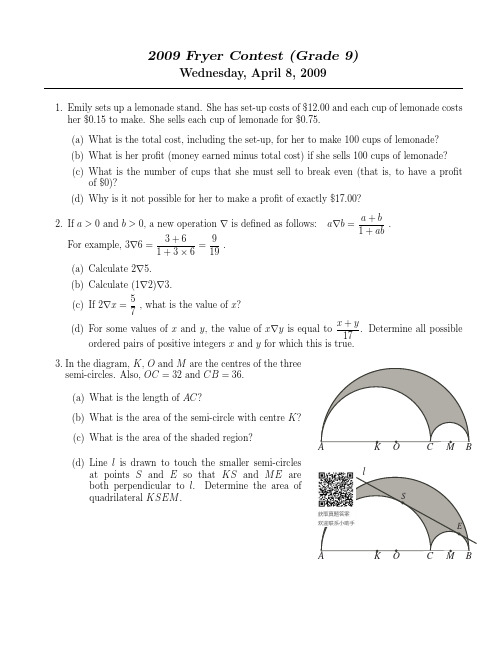
Fryer滑铁卢数学竞赛(Grade 9)-数学Mathematics-2009-试题 exam

2009Fryer Contest(Grade9)Wednesday,April8,20091.Emily sets up a lemonade stand.She has set-up costs of$12.00and each cup of lemonade costsher$0.15to make.She sells each cup of lemonade for$0.75.(a)What is the total cost,including the set-up,for her to make100cups of lemonade?(b)What is her proﬁt(money earned minus total cost)if she sells100cups of lemonade?(c)What is the number of cups that she must sell to break even(that is,to have a proﬁtof$0)?(d)Why is it not possible for her to make a proﬁt of exactly$17.00?2.If a>0and b>0,a new operation∇is deﬁned as follows:a∇b=a+b 1+ab.For example,3∇6=3+61+3×6=919.(a)Calculate2∇5.(b)Calculate(1∇2)∇3.(c)If2∇x=57,what is the value of x?(d)For some values of x and y,the value of x∇y is equal to x+y17.Determine all possibleordered pairs of positive integers x and y for which this is true.3.In the diagram,K,O and M are the centres of the three semi-circles.Also,OC=32and CB=36.(a)What is the length of AC?(b)What is the area of the semi-circle with centre K?(c)What is the area of the shadedregion?(d)Line l is drawn to touch the smaller semi-circlesat points S and E so that KS and ME are both perpendicular to l.Determine the area of quadrilateral KSEM.2009Fryer Contest Page24.The addition shown below,representing2+22+222+2222+···,has101rows and the lastterm consists of1012’s:2222222222...22 (2222)+222 (2222)···C B A(a)Determine the value of the ones digit A.(b)Determine the value of the tens digit B and the value of the hundreds digit C.(c)Determine the middle digit of the sum.。

滑铁卢数学竞赛

滑铁卢数学竞赛1、21.|x|>3表示的区间是（）[单选题] *A.（-∞，3）B.(-3,3)C. [-3,3]D. （-∞，-3）∪（3，+ ∞）(正确答案)2、15.下列数中，是无理数的为（）[单选题] *A.-3.14B.6/11C.√3(正确答案)D.03、9.一棵树在离地5米处断裂，树顶落在离树根12米处，问树断之前有多高（）[单选题] *A. 17(正确答案)B. 17.5C. 18D. 204、若tan（π-α）>0且cosα>0，则角α的终边在（）[单选题] *A.第一象限B.第二象限C.第三象限D.第四象限(正确答案)5、下列说法正确的是[单选题] *A.带“+”号和带“-”号的数互为相反数B.数轴上原点两侧的两个点表示的数是相反数C.和一个点距离相等的两个点所表示的数一定互为相反数D.一个数前面添上“-”号即为原数的相反数(正确答案)6、16、在中,则( ). [单选题] *A. AB<2AC (正确答案)B. AB=2ACC. AB>2ACD. AB与2AC关系不确定7、260°是第（）象限角？[单选题] *第一象限第二象限第三象限(正确答案)第四象限8、4.已知两圆的半径分别为3㎝和4㎝，两个圆的圆心距为10㎝,则两圆的位置关系是（）[单选题] *Ａ.内切Ｂ.相交Ｃ.外切Ｄ.外离(正确答案)9、下列各角中，是界限角的是（）[单选题] *A. 1200°B. -1140°C. -1350°(正确答案)D. 1850°10、下列各对象可以组成集合的是（）[单选题] *A、与1非常接近的全体实数B、与2非常接近的全体实数(正确答案)C、高一年级视力比较好的同学D、与无理数相差很小的全体实数11、若2?＝a2＝4 ?，则a?等于( ) [单选题] *A. 43B. 82C. 83(正确答案)D. 4?12、47、若△ABC≌△DEF，AB＝2，AC＝4，且△DEF的周长为奇数，则EF的值为（）[单选题] *A．3B．4C．1或3D．3或5(正确答案)13、8. 下列事件中，不可能发生的事件是(? ? )．[单选题] *A．明天气温为30℃B．学校新调进一位女教师C．大伟身长丈八(正确答案)D．打开电视机，就看到广告14、3．中国是最早采用正负数表示相反意义的量，并进行负数运算的国家．若零上10℃记作+10℃，则零下10℃可记作（）[单选题] *A．10℃B．0℃C.-10 ℃(正确答案)D．-20℃15、19．对于实数a、b、c,“a>b”是“ac2(c平方)>bc2(c平方) ; ”的（）[单选题] * A．充分不必要条件B．必要不充分条件(正确答案)C．充要条件D．既不充分也不必要条件16、22．如图棋盘上有黑、白两色棋子若干，找出所有使三颗颜色相同的棋在同一直线上的直线，满足这种条件的直线共有（）[单选题] *A．5条(正确答案)B．4条C．3条D．2条17、f（x）=-2x+5在x=1处的函数值为（）[单选题] *A、-3B、-4C、5D、3(正确答案)18、10. 如图所示，小明周末到外婆家，走到十字路口处，记不清哪条路通往外婆家，那么他一次选对路的概率是(? ? ?)．[单选题] *A．1/2B．1/3(正确答案)C．1/4D．119、15．如图所示，下列数轴的画法正确的是（）[单选题] *A．B．C．(正确答案)D．20、-950°是（）[单选题] *A. 第一象限角B. 第二象限角(正确答案)C. 第三象限角D. 第四象限角21、1．如图，∠AOB＝120°，∠AOC＝∠BOC，OM平分∠BOC，则∠AOM的度数为（）[单选题] *A．45°B．65°C．75°(正确答案)D．80°22、27．下列计算正确的是（）[单选题] *A．（﹣a3）2＝a6(正确答案)B．3a+2b＝5abC．a6÷a3＝a2D．（a+b）2＝a2+b223、16．“x2（x平方）－4x－5=0”是“x=5”的( ) [单选题] *A．充分不必要条件B．必要不充分条件(正确答案)C．充要条件D．既不充分也不必要条件24、19．下列两个数互为相反数的是（）[单选题] *A．（﹣）和﹣（﹣）B．﹣5和(正确答案)C．π和﹣14D．+20和﹣（﹣20）25、13．在数轴上，下列四个数中离原点最近的数是（）[单选题] *A．﹣4(正确答案)B．3C．﹣2D．626、14．命题“?x∈R，?n∈N*，使得n≥x2（x平方）”的否定形式是（）[单选题] * A．?x∈R，?n∈N*，使得n<x2B．?x∈R，?x∈N*，使得n<x2C．?x∈R，?n∈N*，使得n<x2D．?x∈R，?n∈N*，使得n<x2(正确答案)27、8．(2020·课标Ⅱ)已知集合U={－2，－1,0,1,2,3}，A={－1,0,1}，B={1,2}，则?U(A∪B)=( ) [单选题] *A．{－2,3}(正确答案)B．{－2,2,3}C．{－2，－1,0,3}D．{－2，－1,0,2,3}28、7人小组选出2名同学作正副组长，共有选法（）种。

Cayley滑铁卢数学竞赛(Grade 10)-数学Mathematics-1998-试题 exam

Chartered Accountants SybaseInc. (Waterloo) IBMCanada Ltd.Canadian Institute of ActuariesDo not open the contest booklet until you are told to do so.You may use rulers, compasses and paper for rough work.Calculators are permitted, providing they are non-programmable and without graphic displays.Part A: Each question is worth 5 credits.1.The value of 03012..()+ is(A ) 0.7(B ) 1(C ) 0.1(D ) 0.19(E ) 0.1092.The pie chart shows a percentage breakdown of 1000 votesin a student election. How many votes did Sue receive?(A ) 550(B ) 350(C ) 330(D ) 450(E ) 9353.The expression a a a 9153× is equal to(A ) a 45(B ) a 8(C ) a 18(D ) a 14(E ) a 214.The product of two positive integers p and q is 100. What is the largest possible value of p q +?(A ) 52(B ) 101(C ) 20(D ) 29(E ) 255.In the diagram, ABCD is a rectangle with DC =12. If the area of triangle BDC is 30, what is the perimeter ofrectangle ABCD ?(A ) 34(B ) 44(C ) 30(D ) 29(E ) 606.If x =2 is a solution of the equation qx –311=, the value of q is (A ) 4(B ) 7(C ) 14(D ) –7(E ) –47.In the diagram, AB is parallel to CD . What is the value ofy ?(A ) 75(B ) 40(C ) 35(D ) 55(E ) 508.The vertices of a triangle have coordinates 11,(), 71,() and 53,(). What is the area of this triangle?(A ) 12(B ) 8(C ) 6(D ) 7(E ) 99.The number in an unshaded square is obtained by adding thenumbers connected to it from the row above. (The ‘11’ is one such number.) The value of x must be (A ) 4(B ) 6(C ) 9(D ) 15(E) 10Scoring:There is no penalty for an incorrect answer.Each unanswered question is worth 2 credits, to a maximum of 20 credits.A BCD DAC B10.The sum of the digits of a five-digit positive integer is 2. (A five-digit integer cannot start with zero.)The number of such integers is(A ) 1(B ) 2(C ) 3(D ) 4(E ) 5Part B: Each question is worth 6 credits.11.If x y z ++=25, x y +=19 and y z +=18, then y equals(A ) 13(B ) 17(C ) 12(D ) 6(E ) –612. A regular pentagon with centre C is shown. The value of xis(A ) 144(B ) 150(C ) 120(D ) 108(E ) 7213.If the surface area of a cube is 54, what is its volume?(A ) 36(B ) 9(C ) 8138(D ) 27(E ) 162614.The number of solutions x y ,() of the equation 3100x y +=, where x and y are positive integers, is(A ) 33(B ) 35(C ) 100(D ) 101(E ) 9715.If y –55= and 28x =, then x y + equals(A ) 13(B ) 28(C ) 3316.Rectangle ABCDhas length 9 and width 5. Diagonal is divided into 5 equal parts at W , X , Y , and Z area of the shaded region.(A ) 36(B ) 365(C ) 18(D ) 41065(E ) 2106517.If N p q =()()()+75243 is a perfect cube, where p and q are positive integers, the smallest possible valueof p q + is(A ) 5(B ) 2(C ) 8(D ) 6(E ) 1218.Q is the point of intersection of the diagonals of one face ofa cube whose edges have length 2 units. The length of QRis(A ) 2(B ) 8(C ) 5(D ) 12(E ) 619.Mr. Anderson has more than 25 students in his class. He has more than 2 but fewer than 10 boys andmore than 14 but fewer than 23 girls in his class. How many different class sizes would satisfy these conditions?(A ) 5(B ) 6(C ) 7(D ) 3(E ) 420.Each side of square ABCD is 8. A circle is drawn through A and D so that it is tangent to BC . What is the radius of thiscircle?(A ) 4(B ) 5(C ) 6(D ) 42(E ) 5.25Part C: Each question is worth 8 credits.21.When Betty substitutes x =1 into the expression ax x c 32–+ its value is –5. When she substitutesx =4 the expression has value 52. One value of x that makes the expression equal to zero is(A ) 2(B ) 52(C ) 3(D ) 72(E ) 422. A wheel of radius 8 rolls along the diameter of a semicircleof radius 25 until it bumps into this semicircle. What is thelength of the portion of the diameter that cannot be touchedby the wheel?(A ) 8(B ) 12(C ) 15(D ) 17(E ) 2023.There are four unequal, positive integers a , b , c , and N such that N a b c =++535. It is also true thatN a b c =++454 and N is between 131 and 150. What is the value of a b c ++?(A ) 13(B ) 17(C ) 22(D ) 33(E ) 3624.Three rugs have a combined area of 2002m . By overlapping the rugs to cover a floor area of 1402m ,the area which is covered by exactly two layers of rug is 242m . What area of floor is covered by three layers of rug?(A ) 122m (B ) 182m (C ) 242m (D) 362m (E ) 422m 25.One way to pack a 100 by 100 square with 10000 circles, each of diameter 1, is to put them in 100rows with 100 circles in each row. If the circles are repacked so that the centres of any three tangent circles form an equilateral triangle, what is the maximum number of additional circles that can be packed?(A ) 647(B ) 1442(C ) 1343(D) 1443(E ) 1344。

1、下载文档前请自行甄别文档内容的完整性，平台不提供额外的编辑、内容补充、找答案等附加服务。
2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
3、如文档侵犯您的权益，请联系客服反馈,我们会尽快为您处理(人工客服工作时间：9:00-18:30)。

Each question is worth 10 marks
Calculating devices are allowed, provided that they do not have any of the following features: (i) internet access, (ii) the ability to communicate with other devices, (iii) information previously stored by students (such as formulas, programs, notes, etc.), (iv) a computer algebra system, (v) dynamic geometry software.
Parts of each question can be of two types: 1. SHORT ANSWER parts indicated by
• worth 3 marks each • full marks given for a correct answer which is placed in the box • part marks awarded only if relevant work is shown in the space provided
WRITE ALL ANSWERS IN THE ANSWER BOOKLET PROVIDED. • Extra paper for your ﬁnished solutions must be supplied by your supervising teacher and inserted into your answer booklet. Write your name, school name, and question number on any inserted pages. • Express answers as simpli√ﬁed exact numbers except where otherwise indicated. For example, π + 1 and 1 − 2 are simpliﬁed exact numbers.
4. For questions marked
, provide a well-organized solution in the answer booklet.

Use mathematical statements and words to explain all of the steps of your solution.
NOTE: 1. Please read the instructions on the front cover of this booklet. 2. Write all answers in the answer booklet provided.
3. For questions marked , place your answer in the appropriate box in the answer booklet and show your work.
Do not discuss the problems or solutions from this contest online for the next 48 hours.
The name, grade, school and location, and score range of some top-scoring students will be published on our website, cemc.uwaterloo.ca. In addition, the name, grade, school and location, and score of some top-scoring students may be shared with other mathematical organizations for other recognition opportunities.
2. FULL SOLUTION parts indicated by
• worth the remainder of the 10 marks for the question • must be written in the appropriate location in the answer booklet • marks awarded for completeness, clarity, and style of presentation • a correct solution poorly presented will not earn full marks
Work out some details in rough on a separate piece of paper before writing your ﬁnished
solution.
5. Diagrams are not drawn to scale. They are intended as aids only.
(outside of North America and South America)
Time:
2
1 2
hours
c 2019 University of Waterloo
Do not open this booklet until instructed to do so.
Number of questions: 10
The CENTRE for EDUCATION in MATHEMATICS and COMPUTING
cemc.uwaterloo.ca
Euclid Contest
Wednesday, April 3, 2019
(in North America and South America)
Thursday, April 4, 2019