Galois滑铁卢数学竞赛(Grade 10)-数学Mathematics-2007-试题 exam

合集下载

Hypatia滑铁卢数学竞赛(Grade 11)-数学Mathematics-2008-试题 exam

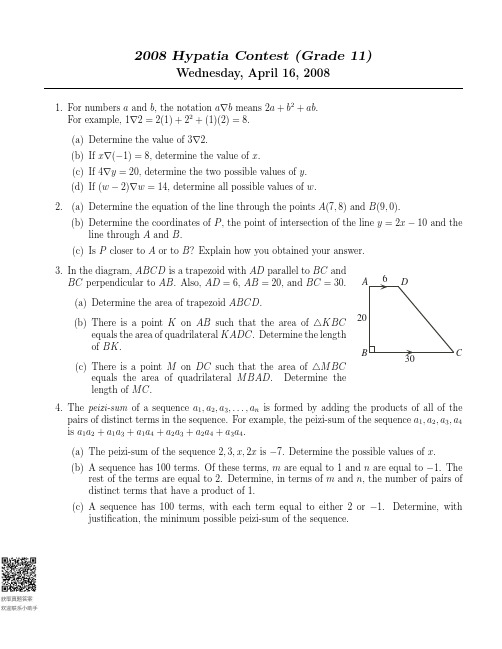
2008Hypatia Contest(Grade11)Wednesday,April16,20081.For numbers a and b,the notation a∇b means2a+b2+ab.For example,1∇2=2(1)+22+(1)(2)=8.(a)Determine the value of3∇2.(b)If x∇(−1)=8,determine the value of x.(c)If4∇y=20,determine the two possible values of y.(d)If(w−2)∇w=14,determine all possible values of w.2.(a)Determine the equation of the line through the points A(7,8)and B(9,0).(b)Determine the coordinates of P,the point of intersection of the line y=2x−10and theline through A and B.(c)Is P closer to A or to B?Explain how you obtained your answer.3.In the diagram,ABCD is a trapezoid with AD parallel to BC andBC perpendicular to AB.Also,AD=6,AB=20,and BC=30.(a)Determine the area of trapezoid ABCD.(b)There is a point K on AB such that the area of KBCequals the area of quadrilateral KADC.Determine the length of BK.(c)There is a point M on DC such that the area of MBCequals the area of quadrilateral MBAD.Determine the length of MC.C4.The peizi-sum of a sequence a1,a2,a3,...,a n is formed by adding the products of all of thepairs of distinct terms in the sequence.For example,the peizi-sum of the sequence a1,a2,a3,a4 is a1a2+a1a3+a1a4+a2a3+a2a4+a3a4.(a)The peizi-sum of the sequence2,3,x,2x is−7.Determine the possible values of x.(b)A sequence has100terms.Of these terms,m are equal to1and n are equal to−1.Therest of the terms are equal to2.Determine,in terms of m and n,the number of pairs of distinct terms that have a product of1.(c)A sequence has100terms,with each term equal to either2or−1.Determine,withjustiﬁcation,the minimum possible peizi-sum of the sequence.。

欧几里得数学竞赛_

欧几里得数学竞赛_摘要：I.欧几里得数学竞赛概述- 竞赛起源与发展- 竞赛难度与影响力II.欧几里得数学竞赛适合人群- 参赛对象与报名方式- 竞赛对申请大学的帮助III.欧几里得数学竞赛考试内容与形式- 竞赛知识点覆盖范围- 考试时间与题型- 评分标准与奖项设置IV.欧几里得数学竞赛备考策略- 备考时间安排- 推荐教材与学习资源- 真题练习与模拟考试V.欧几里得数学竞赛在中国的发展- 我国学生参赛情况- 相关培训机构与课程- 对我国数学教育的启示与影响正文：欧几里得数学竞赛（Euclid Mathematics Contest）是由加拿大滑铁卢大学（University of Waterloo）数学与计算机学院主办的面向全球高中生的数学竞赛，被誉为数学界的托福。

竞赛始于1963年，每年有来自10多个国家和地区、1850多所学校的2万多名学生参加。

该竞赛在数学界中已经得到广泛认可，对学生的申请大学具有很大的帮助。

欧几里得数学竞赛适合人群广泛，参赛对象为全球各地的高中生，报名方式一般由学校统一组织。

竞赛难度较高，知识点覆盖范围广泛，对学生的逻辑思维能力和数学素养有很高的要求。

在我国，许多学生通过参加欧几里得数学竞赛，提高了自身的数学能力，为申请国内外知名大学提供了有力的砝码。

欧几里得数学竞赛的考试内容主要包括代数、几何、组合、数论等多个方面，考试形式为笔试，分为简答题和解答题。

评分标准根据解题过程的准确性、完整性和创新性来评判，奖项分为金、银、铜三个等级。

对于如何备考欧几里得数学竞赛，建议学生合理安排时间，提前准备。

推荐使用一些经典的数学竞赛教材和在线学习资源，如《数学竞赛题型解析》、《欧几里得数学竞赛真题详解》等。

在备考过程中，要注重真题练习和模拟考试，以检验自己的学习效果，逐步提高自己的解题能力。

近年来，随着我国学生对国际数学竞赛的热情逐渐高涨，欧几里得数学竞赛在我国也得到了广泛关注。

越来越多的学生通过参加欧几里得数学竞赛，提升了自己的数学素养，为我国数学教育的发展带来了新的启示和影响。

滑铁卢竞赛数学题

滑铁卢竞赛数学题概述
滑铁卢竞赛数学题通常比较难，涉及的知识点广泛，包括代数、几何、数论、组合数学等多个领域。

以下是一些滑铁卢竞赛数学题的示例：
1. 有100个球，其中有一个与其他99个重量不同，但外观相同。

用一个天平，最少需要称多少次才能确定这个重量不同的球？
2. 一个正方形的面积为1，将其四边中点连接起来，形成另一个正方形。

如此重复，得到第五、第六个正方形，求第五个正方形的面积。

3. 一个圆被分成n个相等的扇形，其中一个是空心的，其他n-1个是实心的。

求空心扇形的圆心角是多少度？
4. 有100个人站成一排，从第1个人开始报数，每次报到奇数的人离开队伍。

经过若干轮后，只剩下一个人。

求这个人最初站在第几位？
5. 有5个不同质因数的最小正整数是多少？
以上仅是滑铁卢竞赛数学题的一些示例，实际上还有更多难题和技巧题。

如果想要深入了解滑铁卢竞赛数学题的解题技巧和策略，建议参考相关的竞赛书籍和资料，或者参加专业的数学竞赛培训课程。

欧几里得滑铁卢数学竞赛_2010EuclidSolution

Since Bea ﬂies at a constant speed, then the ratio of the two distances equals the ratio of
the corresponding times.
HF 60 minutes 4
Therefore, =
=.
GF 45 minutes 3
(b) Solution 1
Since ∠OP B = 90◦, then OP and P B are perpendicular, so the product of their slopes
is −1.
4−0 4
4−0
4
The slope of OP is
= and the slope of P B is
Since F GH is right-angled at F , then F GH must be similar to a 3-4-5 triangle, and
HG 5
so = .
GF 3
In
particular,
this
means
that
the
ratio
of
the
times
ﬂying
H
to
Wednesday, April 7, 2010
Solutions
©2010 Centre for Education in Mathematics and Computing
2010 Euclid Contest Solutions
Page 2
1. (a) Solution 1 Since 3x = 27, then 3x+2 = 3x32 = 27 · 9 = 243.

国际中学生数学竞赛含金量排行榜

国际中学生数学竞赛含金量排行榜
国际中学生数学竞赛的含金量因赛事的权威性、参赛选手水平、奖项设置等因素而有所不同。

以下是部分国际中学生数学竞赛及简要介绍：
1. 国际数学奥林匹克（IMO）：这是最具权威性的国际中学生数学竞赛之一，每年有来自世界各地的参赛选手。

IMO的奖项分为金牌、银牌和铜牌，其
中金牌是最高荣誉。

获奖者将获得世界范围内的认可和奖励，对于未来的学术和职业发展具有重要意义。

2. 亚洲太平洋数学奥林匹克（APMO）：这是亚太地区最高水平的数学竞赛，每年有来自多个国家和地区的代表队参赛。

APMO的奖项分为金、银、铜
牌和优秀奖，其中金牌是最高荣誉。

获奖者将获得国际范围内的认可和奖励，对于未来的学术和职业发展具有重要意义。

3. 英国数学奥林匹克（BMO）：这是英国最高水平的数学竞赛，每年有来
自全国各地的参赛选手。

BMO的奖项分为金、银、铜牌和优秀奖，其中金
牌是最高荣誉。

获奖者将获得英国范围内的认可和奖励，对于未来的学术和职业发展具有重要意义。

4. 罗马尼亚数学奥林匹克（RMO）：这是罗马尼亚最高水平的数学竞赛，
每年有来自全国各地的参赛选手。

RMO的奖项分为金、银、铜牌和优秀奖，其中金牌是最高荣誉。

获奖者将获得国际范围内的认可和奖励，对于未来的学术和职业发展具有重要意义。

总的来说，这些国际中学生数学竞赛都具有较高的含金量，获奖者将获得国际范围内的认可和奖励，对于未来的学术和职业发展具有重要意义。

滑铁卢数学竞赛

滑铁卢数学竞赛滑铁卢数学竞赛是加拿大滑铁卢大学举办的一项年度数学竞赛活动。

该竞赛旨在通过一系列难度不断增加的数学问题，考察参赛者的数学思维能力、解题能力以及创造力。

每年都有来自世界各地的学生参加该比赛，其中包括来自中小学的学生以及大学生。

滑铁卢数学竞赛分为两个阶段，第一阶段为全球性选拔赛，任何人都可以参加。

参赛者需要在线完成一套由滑铁卢大学编制的数学测试，题型涵盖代数、几何、组合数学等多个数学领域。

根据第一阶段的成绩，滑铁卢大学将选拔出前几百名成绩优异的参赛者晋级到第二阶段。

第二阶段为面试阶段，只有第一阶段晋级的学生才可以参加。

参赛者需要前往滑铁卢大学进行现场的笔试和面试。

笔试部分主要考察参赛者的数学基础知识和解题能力，而面试部分则更加注重参赛者的思维过程和解题思路。

面试时，学生需要与评委进行面对面的交流，展示自己的数学思考能力。

滑铁卢数学竞赛的题目通常非常有难度，涉及到一些高级数学概念和方法。

参赛者需要具备扎实的数学基础知识，并且具备独立思考和解决问题的能力。

竞赛的目的不仅是测试学生的数学水平，更重要的是培养他们解决问题的能力和数学思维方式。

参加滑铁卢数学竞赛对于学生来说是一次宝贵的经历。

这个竞赛可以提供一个展示自己数学才能的平台，也可以锻炼参赛者的思维能力和团队合作精神。

在竞赛中，学生们可以结识来自不同国家和地区的志同道合的数学爱好者，分享彼此的数学体验和解题方法。

滑铁卢数学竞赛也为参赛者提供了一些奖励和机会。

根据参赛者在竞赛中的表现，滑铁卢大学会为他们颁发证书和奖状，并且可以获得一些奖金和奖品。

此外，优秀的参赛者还有机会获得滑铁卢大学的奖学金和入学机会，为他们的未来发展开启了一扇大门。

总之，滑铁卢数学竞赛是一个非常有挑战性和有意义的数学竞赛活动。

通过参加这个竞赛，学生们可以提升自己的数学能力，拓展自己的数学视野，同时也能够展示自己的才能和潜力。

无论是对于中小学生还是大学生，参加滑铁卢数学竞赛都是一个值得鼓励和支持的选择。

Fermat滑铁卢数学竞赛(Grade 11)-数学Mathematics-2002-试题 exam

Canadian Instituteof Actuaries Chartered AccountantsSybasei Anywhere SolutionsScoring:There is no penalty for an incorrect answer.Each unanswered question is worth 2, to a maximum of 10 unanswered questions.Part A: Each correct answer is worth 5.1.If x =3, the numerical value of 522–x is(A ) –1(B ) 27(C ) –13(D )–31(E ) 32.332232++ is equal to(A ) 3(B ) 6(C ) 2(D )32(E ) 53.If it is now 9:04 a.m., in 56 hours the time will be(A ) 9:04 a.m.(B ) 5:04 p.m.(C ) 5:04 a.m.(D ) 1:04 p.m.(E ) 1:04 a.m.4.Which one of the following statements is not true?(A ) 25 is a perfect square.(B ) 31 is a prime number.(C ) 3 is the smallest prime number.(D ) 8 is a perfect cube.(E ) 15 is the product of two prime numbers.5. A rectangular picture of Pierre de Fermat, measuring 20 cmby 40 cm, is positioned as shown on a rectangular postermeasuring 50 cm by 100 cm. What percentage of the areaof the poster is covered by the picture?(A ) 24%(B ) 16%(C ) 20%(D ) 25%(E ) 40%6.Gisa is taller than Henry but shorter than Justina. Ivan is taller than Katie but shorter than Gisa. Thetallest of these five people is(A ) Gisa (B ) Henry (C ) Ivan (D ) Justina (E ) Katie7. A rectangle is divided into four smaller rectangles. Theareas of three of these rectangles are 6, 15 and 25, as shown.The area of the shaded rectangle is(A ) 7(B ) 15(C ) 12(D ) 16(E) 108.In the diagram, ABCD and DEFG are squares with equal side lengths, and ∠=°DCE 70. The value of y is (A ) 120(B ) 160(C ) 130(D ) 110(E ) 1409.The numbers 1 through 20 are written on twenty golf balls, with one number on each ball. The golfballs are placed in a box, and one ball is drawn at random. If each ball is equally likely to be drawn,what is the probability that the number on the golf ball drawn is a multiple of 3?(A )320(B )620(C )1020(D )520(E )12010.ABCD is a square with AB x =+16 and BC x =3, as shown.The perimeter of ABCD is(A ) 16(B ) 32(C ) 96(D ) 48(E ) 24Part B: Each correct answer is worth 6.11. A line passing through the points 02,−() and 10,() also passes through the point 7,b (). The numericalvalue of b is(A ) 12(B )92(C ) 10(D ) 5(E ) 1412.How many three-digit positive integers are perfect squares?(A ) 23(B ) 22(C ) 21(D ) 20(E ) 1913. A “double-single” number is a three-digit number made up of two identical digits followed by adifferent digit. For example, 553 is a double-single number. How many double-single numbers are there between 100 and 1000?(A ) 81(B ) 18(C ) 72(D ) 64(E ) 9014.The natural numbers from 1 to 2100 are entered sequentially in 7 columns, with the first 3 rows asshown. The number 2002 occurs in column m and row n . The value of m n + isColumn 1Column 2Column 3Column 4Column 5Column 6Column 7Row 1 1 2 3 4 5 6 7Row 2 8 91011121314Row 315161718192021M M M M M M M M(A ) 290(B ) 291(C ) 292(D ) 293(E ) 294x + 163xA BD C15.In a sequence of positive numbers, each term after the first two terms is the sum of all of the previousterms . If the first term is a ,the second term is 2, and the sixth term is 56, then the value of a is(A ) 1(B ) 2(C ) 3(D ) 4(E ) 516.If ac ad bc bd +++=68 and c d +=4, what is the value of a b c d +++?(A ) 17(B ) 85(C ) 4(D ) 21(E ) 6417.The average age of a group of 140 people is 24. If the average age of the males in the group is 21 andthe average age of the females is 28, how many females are in the group?(A ) 90(B ) 80(C ) 70(D ) 60(E ) 5018. A rectangular piece of paper AECD has dimensions 8 cm by 11 cm. Corner E is folded onto point F , which lies on DC ,as shown. The perimeter of trapezoid ABCD is closest to (A ) 33.3 cm (B ) 30.3 cm (C ) 30.0 cm(D ) 41.3 cm (E ) 35.6 cm 19.If 238610a b =(), where a and b are integers, then b a − equals(A ) 0(B ) 23(C )−13(D )−7(E )−320.In the diagram, YQZC is a rectangle with YC =8 and CZ = 15. Equilateral triangles ABC and PQR , each withside length 9, are positioned as shown with R and B on sidesYQ and CZ , respectively. The length of AP is (A ) 10(B )117(C ) 9(D ) 8(E )72Part C: Each correct answer is worth 8.21.If 31537521219⋅⋅⋅⋅+−=L n n , then the value of n is(A ) 38(B ) 1(C ) 40(D ) 4(E ) 3922.The function f x () has the property that f x y f x f y xy +()=()+()+2, for all positive integers x and y .If f 14()=, then the numerical value of f 8() is(A ) 72(B ) 84(C ) 88(D ) 64(E ) 80continued ...Figure 1Figure 223.The integers from 1 to 9 are listed on a blackboard. If an additional m eights and k nines are added tothe list, the average of all of the numbers in the list is 7.3. The value of k m + is(A ) 24(B ) 21(C ) 11(D ) 31(E ) 8924. A student has two open-topped cylindrical containers. (Thewalls of the two containers are thin enough so that theirwidth can be ignored.) The larger container has a height of20 cm, a radius of 6 cm and contains water to a depth of 17cm. The smaller container has a height of 18 cm, a radius of5 cm and is empty. The student slowly lowers the smallercontainer into the larger container, as shown in the cross-section of the cylinders in Figure 1. As the smaller container is lowered, the water first overflows out of the larger container (Figure 2) and then eventually pours into thesmaller container. When the smaller container is resting onthe bottom of the larger container, the depth of the water in the smaller container will be closest to(A ) 2.82 cm (B ) 2.84 cm (C ) 2.86 cm(D ) 2.88 cm (E ) 2.90 cm25.The lengths of all six edges of a tetrahedron are integers. The lengths of five of the edges are 14, 20,40, 52, and 70. The number of possible lengths for the sixth edge is(A ) 9(B ) 3(C ) 4(D ) 5(E ) 6。

加拿大数学竞赛历年试题(滑铁卢大学)

Each question is worth 10 marks
Calculating devices are allowed, provided that they do not have any of the following features: (i) internet access, (ii) the ability to communicate with other devices, (iii) information previously stored by students (such as formulas, programs, notes, etc.), (iv) a computer algebra system, (v) dynamic geometry software.
Parts of each question can be of two types: 1. SHORT ANSWER parts indicated by
• worth 3 marks each • full marks given for a correct answer which is placed in the box • part marks awarded only if relevant work is shown in the space provided
WRITE ALL ANSWERS IN THE ANSWER BOOKLET PROVIDED. • Extra paper for your ﬁnished solutions must be supplied by your supervising teacher and inserted into your answer booklet. Write your name, school name, and question number on any inserted pages. • Express answers as simpli√ﬁed exact numbers except where otherwise indicated. For example, π + 1 and 1 − 2 are simpliﬁed exact numbers.

1、下载文档前请自行甄别文档内容的完整性，平台不提供额外的编辑、内容补充、找答案等附加服务。
2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
3、如文档侵犯您的权益，请联系客服反馈,我们会尽快为您处理(人工客服工作时间：9:00-18:30)。

2007Galois Contest (Grade 10)
Wednesday,April 18,2007
1.Jim shops at a strange fruit store.Instead of putting prices on each item,the mathematical store owner will answer questions about combinations of items.
(a)In Aisle 1,Jim receives the following answers to his questions:
Jim’s Question Answer
What is the sum of the prices of an Apple and a Cherry?62cents
What is the sum of the prices of a Banana and a Cherry?66cents
What is diﬀerence between the prices of an Apple and a Banana?Which has a higher price?Explain how you obtained your answer.
(b)In Aisle 2,Jim receives the following answers to his questions:
Jim’s Question Answer
What is the sum of the prices of a Mango and a Nectarine?60cents
What is the sum of the prices of a Pear and a Nectarine?60cents
What is the sum of the prices of a Mango and a Pear?68cents
What is the price of a Pear?Explain how you obtained your answer.
(c)In Aisle 3,Jim receives the following answers to his questions:
Jim’s Question Answer
What is the sum of the prices of a Tangerine and a Lemon?60cents
How
much more does a Tangerine cost than a Grapefruit?6cents
What is the sum of the prices of Grapefruit,a Tangerine and a Lemon?94cents
What is the price of a Lemon?Explain how you obtained your
answer.2.(a)In the diagram,what is the perimeter of the sector of the circle with radius 12?Explain how you obtained your answer.
(b)Two sectors of a circle of radius 12are placed side by side,as shown.Determine the area of ﬁgure ABCD .Explain how you obtained
your answer.
A (c)In the diagram,AO
B is a sector of a circle with ∠AOB =60◦.
OY is drawn perpendicular to AB and intersects AB at X .What is the length of XY ?Explain how you obtained your answer.A O B
X Y
12
12
(d)See over...
2007Galois Contest Page2
(d)Two sectors of a circle of radius12overlap as shown.
Determine the area of the shaded region.Explain how you
obtained your answer.
R
3.(a)Each face of a5by5by5wooden cube is divided into1
by1squares.Each square is painted black or white,as
shown.Next,the cube is cut into1by1by1cubes.How
many of these cubes have at least two painted faces?
Explain how you obtained your
answer.
(b)A(2k+1)by(2k+1)by(2k+1)cube,where k is a in the
same manner as the5by5by5cube with white squares in the corners.Again,the cube is cut into1by1by1cubes.
i.In terms of k,how many of these cubes have exactly two white faces?Explain how
you obtained your answer.
ii.Prove that there is no value of k for which the number of cubes having at least two white faces is2006.
4.Jill has a container of small cylindrical rods in six diﬀerent colours.Each colour of rod has a
diﬀerent length as summarized in the chart.
Colour Length
Green3cm
Pink4cm
Yellow5cm
Black7cm
Violet8cm
Red9cm
These rods can be attached together to form a pole.
There are2ways to choose a set of yellow and green rods that will form a pole29cm in length: 8green rods and1yellow rod OR3green rods and4yellow rods.
(a)How many diﬀerent sets of yellow and green rods can be chosen that will form a pole
62cm long?Explain how you obtained your answer.
(b)Among the green,yellow,black and red rods,ﬁnd,with justiﬁcation,two colours for which
it is impossible to make a pole62cm in length using only rods of those two colours.
(c)If at least81rods
of each of the colours green,pink,violet,and red must be used,how
many diﬀerent sets of rods of these four colours can be chosen that will form a pole2007cm in length?Explain how you got your answer.。