各种数学符号英文翻译
数学术语英文对照表

数学术语英文对照表在学习数学时,不可避免地需要掌握一些数学术语。
下面是一些常见的数学术语的中英文对照表:1. 数学 Mathematics2. 数学符号 Mathematical symbol3. 数字 Digit4. 字符 Character5. 算术 Arithmetic6. 代数 Algebra7. 几何 Geometry8. 统计学 Statistics9. 概率 Probability10. 三角学 Trigonometry11. 微积分 Calculus12. 矩阵 Matrix13. 向量 Vector14. 线性代数 Linear algebra15. 数学模型 Mathematical model16. 方程 Equation17. 垂直 Perpendicular18. 平行 Parallel19. 正比例 Direct proportion20. 反比例 Inverse proportion21. 旋转 Rotation22. 缩放 Scaling23. 变形 Deformation24. 函数 Function25. 极限 Limit26. 微分 Differential27. 积分 Integral28. 中心 Central29. 对称 Symmetry30. 相似 Similarity31. 等式 Equality32. 不等式 Inequality33. 方形 Rectangle34. 正方形 Square35. 圆 Circle36. 椭圆 Ellipse37. 球球体 Sphere38. 三角形 Triangle39. 直角三角形 Right triangle40. 等腰三角形 Isosceles triangle41. 等边三角形 Equilateral triangle42. 直线 Line43. 射线 Ray44. 线段 Line segment45. 弧 Arc46. 正弦 Sine47. 余弦 Cosine48. 正切 Tangent49. 余切 Cotangent50. 弦 Chord总之,数学术语是数学学习的基础,掌握数学术语对于提高数学能力以及学好数学至关重要。
常用数学符号英文对照
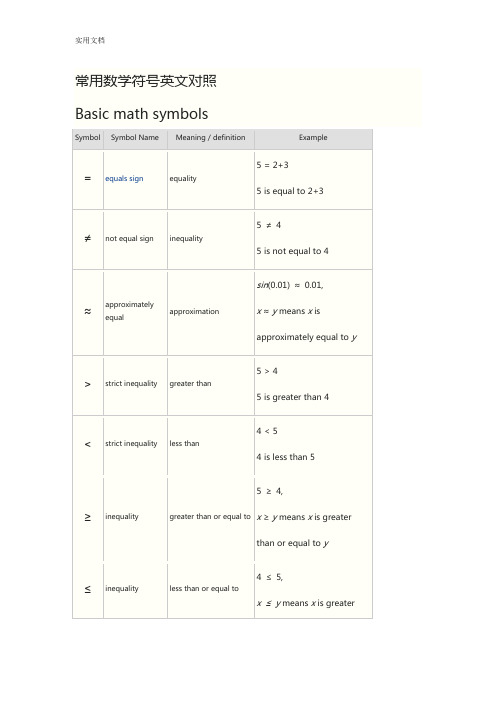
than or equal to y ( ) parentheses calculate expression2 ×(3+5) = 16inside first[ ] brackets calculate expression[(1+2)×(1+5)] = 18inside first+ plus sign addition 1 + 1 = 2−minus sign subtraction 2 −1 = 1±plus - minus both plus and minus3 ±5 = 8 and -2operations±minus - plus both minus and plus3 ±5 = -2 and 8operations* asterisk multiplication 2 * 3 = 6×times sign multiplication 2 ×3 = 6 ·multiplicationmultiplication 2 ·3 = 6dot÷division sign /division 6 ÷2 = 3 obelus/ division slash division 6 / 2 = 3–horizontal line division / fractionmod modulo remainder calculation 7 mod 2 = 1. period decimal point, decimal2.56 = 2+56/100separatora b power exponent 23= 8a^b caret exponent 2 ^ 3= 83√a cube root 3√a ·3√a ·3√a = a3√8 = 24√a fourth root 4√a ·4√a ·4√a ·4√a = a4√16 = ±2n√a n-th root(radical)for n=3, n√8 = 2% percent1% = 1/100 10% ×30 = 3‰per-mille1‰= 1/1000 =0.1%10‰×30 = 0.3ppm per-million1ppm = 1/1000000 10ppm ×30 = 0.0003 ppb per-billion1ppb =1/100000000010ppb ×30 = 3×10-7ppt per-trillion 1ppt = 10-1210ppt ×30 = 3×10-10 Geometry symbolsSymbol Symbol Name Meaning / definition Example ∠angle formed by two rays ∠ABC = 30°measuredangleABC = 30°spherical angle AOB = 30°∟right angle = 90°α= 90°deg degree 1 turn = 360deg α= 60deg ′prime arcminute, 1°= 60′α= 60°59′α= 60°59′″double prime arcsecond, 1′= 60″59″line infinite lineAB line segment line from point A to point Bray line that start from point Aarc arc from point A to point B = 60°⊥perpendicular perpendicular lines (90°angle) AC ⊥ BC| | parallel parallel lines AB | | CD≅congruent to equivalence of geometric shapes and size ∆ABC≅∆XYZ ~ similarity same shapes, not same size ∆ABC~ ∆XYZΔABC≅ΔΔtriangle triangle shapeBCD|x-y| distance distance between points x and y | x-y | = 5πpi constant π = 3.141592654...c = π·d =is the ratio between the circumference andin range ofseries∑∑sigma doublesummation∏capital pi product -product of allvalues in rangeof series∏ x i=x1·x2·...·x ne e constant / Euler's numbere =2.718281828...e = lim (1+1/x)x , x→∞γEuler-Mascheroni constantγ=0.527721566...φgolden ratio golden ratioconstantπpi constant π =3.141592654...is the ratiobetween thecircumferenceand diameter of acirclec = π·d = 2·π·rLinear Algebra SymbolsSymbol Symbol Name Meaning / definition Example ·dot scalar product a · b×cross vector product a × bA⊗B tensor product tensor product of A and B A⊗Binner product[ ] brackets matrix of numbers( ) parentheses matrix of numbers| A | determinant determinant of matrix Adet(A) determinant determinant of matrix A|| x || double vertical bars normA T transpose matrix transpose (A T)ij = (A)ji A†Hermitian matrix matrix conjugate transpose (A†)ij = (A)ji A*Hermitian matrix matrix conjugate transpose (A*)ij = (A)ji A-1inverse matrix A A-1 = Irank(A) matrix rank rank of matrix A rank(A) = 3 dim(U) dimension dimension of matrix A rank(U) = 3Probability and statistics symbols Symbol Symbol Name Meaning / definition Example P(A) probabilityfunctionprobability of event A P(A) = 0.5P(A∩B) probability ofeventsintersectionprobability that ofevents A and BP(A∩B) = 0.5P(A∪B) probability ofevents union probability that ofevents A or BP(A∪B) = 0.5P(A | B) conditionalprobabilityfunctionprobability of event Agiven event B occuredP(A | B) = 0.3f (x) probabilitydensity function(pdf)P(a ≤ x ≤ b) = ∫f (x) dxF(x) cumulativedistributionfunction (cdf)F(x) = P(X≤ x)μpopulationmean mean of populationvaluesμ = 10E(X) expectationvalue expected value ofrandom variable XE(X) = 10E(X | Y) conditionalexpectation expected value ofrandom variable Xgiven YE(X | Y=2) = 5var(X) variance variance of randomvariable Xvar(X) = 4σ2variance variance of populationvaluesσ2 = 4std(X) standarddeviation standard deviation ofrandom variable Xstd(X) = 2σX standarddeviation standard deviationvalue of randomvariable XσX=2median middle value of random variable xcov(X,Y) covariance covariance of randomvariables X and Ycov(X,Y) = 4corr(X,Y) correlation correlation of randomvariables X and Ycorr(X,Y) = 0.6ρX,Y correlation correlation of randomvariables X and YρX,Y = 0.6∑summation summation - sum of all values in range of series∑∑doublesummationdouble summationMo mode value that occurs most frequently in populationMR mid-range MR = (x max+x min)/2 Md sample median half the population isbelow this valueQ1lower / firstquartile 25% of population are below this valueQ2median / secondquartile 50% of population are below this value = median of samplesQ3upper / thirdquartile 75% of population are below this valuex sample mean average / arithmeticmeanx = (2+5+9) / 3 = 5.333s2sample variance population samplesvariance estimators2 = 4s sample standarddeviation population samples standard deviation estimators = 2z x standard score z x = (x-x) / s xX ~ distribution of X distribution of randomvariable XX ~ N(0,3)N(μ,σ2) normaldistributiongaussian distribution X ~ N(0,3)U(a,b) uniformdistribution equal probability inrange a,bX ~ U(0,3)exp(λ) exponentialdistributionf (x) = λe-λx , x≥0gamma(c,λ) gammadistributionf (x) = λc x c-1e-λx /Γ(c), x≥0χ 2(k) chi-squaredistributionf (x) = x k/2-1e-x/2 /( 2k/2 Γ(k/2) )F (k1, k2) F distributionBin(n,p) binomialdistributionf (k) = n C k p k(1-p)n-kPoisson(λ) Poissondistributionf (k) = λk e-λ / k!Geom(p) geometricdistributionf (k) = p(1-p) kHG(N,K,n) hyper-geometricdistributionBern(p) BernoullidistributionCombinatorics SymbolsSymbol Symbol Name Meaning / definition Examplen! factorial n! = 1·2·3·...·n5! = 1·2·3·4·5 = 120 n P k permutation 5P3 = 5! / (5-3)! = 60n C kcombination 5C3 = 5!/[3!(5-3)!]=10Set theory symbolsSymbol Symbol Name Meaning / definition Example { } set a collection of elementsA = {3,7,9,14},B = {9,14,28}A ∩B intersection objects that belong to set A andset BA ∩B = {9,14}A ∪B union objects that belong to set A orset BA ∪B ={3,7,9,14,28}A ⊆B subset subset has fewer elements orequal to the set{9,14,28} ⊆{9,14,28}A ⊂B proper subset / strictsubsetsubset has fewer elements thanthe set{9,14} ⊂{9,14,28}A ⊄B not subset left set not a subset of right set{9,66} ⊄{9,14,28}A ⊇B superset set A has more elements orequal to the set B{9,14,28} ⊇{9,14,28}A ⊃B proper superset / strictsupersetset A has more elements thanset B{9,14,28} ⊃{9,14}A ⊅B not superset set A is not a superset of set B{9,14,28} ⊅{9,66}2A power set all subsets of Apower set all subsets of AA =B equality both sets have the samemembersA={3,9,14},B={3,9,14},A=BA c complement all the objects that do not belong to set AA \B relative complement objects that belong to A and notto BA = {3,9,14},B = {1,2,3},A-B = {9,14}A -B relative complement objects that belong to A and notto BA = {3,9,14},B = {1,2,3},A-B = {9,14}A ∆B symmetric difference objects that belong to A or Bbut not to their intersectionA = {3,9,14},B = {1,2,3},A ∆B ={1,2,9,14}A ⊖B symmetric difference objects that belong to A or Bbut not to their intersectionA = {3,9,14},B = {1,2,3},A ⊖B ={1,2,9,14}a∈A element of set membership A={3,9,14}, 3 ∈Ax∉A not element of no set membershipA={3,9,14}, 1∉ A (a,b) ordered pair collection of 2 elementsA×B cartesian product set of all ordered pairs from Aand B|A| cardinality the number of elements of set AA={3,9,14},|A|=3#A cardinality the number of elements of set AA={3,9,14},#A=3aleph-null infinite cardinality of natural numbers setaleph-one cardinality of countable ordinal numbers setØempty set Ø = { } C = {Ø} universal set set of all possible values0natural numbers / wholenumbers set (withzero)= {0,1,2,3,4,...} 0 ∈01natural numbers / wholenumbers set (without 1= {1,2,3,4,5,...} 6 ∈1zero)integer numbers set = {...-3,-2,-1,0,1,2,3,...} -6 ∈rational numbers set = {x | x=a/b, a,b∈} 2/6 ∈real numbers set = {x | -∞< x <∞} 6.343434∈= {z | z=a+bi, -∞<a<6+2i∈complex numbers set∞, -∞<b<∞}Logic symbolsSymbol Symbol Name Meaning / definition Example ·and and x·y^ caret / circumflex and x ^ y& ampersand and x & y + plus or x + y∨reversed caret or x∨y| vertical line or x | yx' single quote not - negation x'x bar not - negation x¬not not - negation ¬x! exclamation mark not - negation ! x~ tilde negation ~ x ⇒implies⇔equivalent if and only if (iff)↔equivalent if and only if (iff)∀for all∃there exists∄there does not exists∴therefore∵because / sinceCalculus & analysis symbolsSymbol Symbol Name Meaning / definition Example limit limit value of a functionεepsilon represents a very smallnumber, near zeroε→0e e constant /Euler's number e = 2.718281828...e = lim (1+1/x)x ,x→∞y ' derivative derivative - Lagrange'snotation(3x3)' = 9x2y(n)nth derivative n times derivation (3x3)(3) = 18 derivative derivative - Leibniz's notation d(3x3)/dx = 9x2second derivative derivative of derivative d2(3x3)/dx2 = 18xnth derivative n times derivationtime derivative derivative by time - Newton's notationtime secondderivativederivative of derivativeD x y derivative derivative - Euler's notationD x2y second derivative derivative of derivativepartial derivative ∂(x2+y2)/∂x = 2x ∫integral opposite to derivation ∫f(x)dx∫∫double integral integration of function of 2variables∫∫f(x,y)dxdy∫∫∫triple integral integration of function of 3variables ∫∫∫f(x,y,z)dxdydz∮closed contour /line integral∯closed surface integral∰closed volume integral[a,b] closed interval [a,b] = {x | a ≤ x ≤ b}(a,b) open interval (a,b) = {x | a < x < b}i imaginary unit i≡√-1 z = 3 + 2i z* complexconjugatez = a+bi→z*=a-bi z* = 3 - 2iz complexconjugatez = a+bi→z = a-bi z = 3 - 2i∇nabla / del gradient / divergenceoperator∇f (x,y,z) vectorunit vectorx * y convolution y(t) = x(t) * h(t)Laplace transform F(s) = {f (t)}Fourier transform X(ω) = {f (t)}δdelta function∞lemniscate infinity symbol。
数学术语英文对照表

数学术语英文对照表数学是一门具有广泛应用的科学,其术语涉及面广,且大部分都是英文。
因此,了解数学术语的英文对照表对于学习和应用数学都非常重要。
以下是数学术语英文对照表:1. 加法(Addition):指两个或多个数相加的操作。
2. 减法(Subtraction):指从一个数中减去另一个数的操作。
3. 乘法(Multiplication):指两个或多个数相乘的操作。
4. 除法(Division):指将一个数分成几个相等的部分的操作。
5. 平方(Square):指一个数乘以自己的操作。
6. 立方(Cube):指一个数乘以自己两次的操作。
7. 根号(Square Root):指一个数的平方根,即乘以自己等于该数的数。
8. 对数(Logarithm):指以某个数为底的幂的指数。
9. 指数(Exponent):指一个数的幂,即该数自乘若干次的结果。
10. 分数(Fraction):指一个数被另一个数除以的结果。
11. 百分数(Percentage):指一个数乘以100的结果。
12. 四则运算(Arithmetic):指加、减、乘、除四种基本运算。
13. 代数(Algebra):指使用字母和符号来表示数学问题的分支学科。
14. 几何(Geometry):指研究空间中点、线、面等几何图形的学科。
15. 概率(Probability):指描述事件发生可能性的数学分支。
16. 统计(Statistics):指收集、整理、分析数据的学科。
17. 微积分(Calculus):指研究变化和运动的学科。
18. 矩阵(Matrix):指由数列或数表排成的矩形阵列。
19. 向量(Vector):指具有大小和方向的量。
20. 微分方程(Differential Equation):指包含未知函数及其导数的方程。
以上是数学术语英文对照表的部分内容。
掌握这些术语的英文对照是理解数学概念和应用数学的基础。
在学习数学的过程中,我们还需要掌握一些基本的数学符号,如加号(+)、减号(-)、乘号(×或·)、除号(÷或/)、等于号(=)、大于号(>)、小于号(<)、左括号(()、右括号())、逗号(,)、小数点(.)等。
常用数学符号英文对照
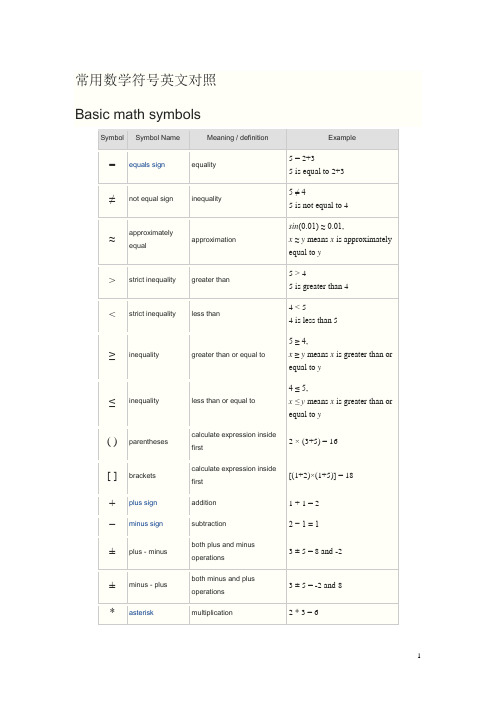
常用数学符号英文对照Basic math symbolsSymbol Symbol Name Meaning / definition Example= equals sign equality 5 = 2+35 is equal to 2+3≠not equal sign inequality 5 ≠ 45 is not equal to 4≈approximatelyequal approximationsin(0.01) ≈ 0.01,x≈y means x is approximatelyequal to y> strict inequality greater than 5 > 45 is greater than 4< strict inequality less than 4 < 54 is less than 5≥inequality greater than or equal to 5 ≥ 4,x≥y means x is greater than or equal to y≤inequality less than or equal to 4 ≤ 5,x ≤ y means x is greater than or equal to y( ) parentheses calculate expression insidefirst2 × (3+5) = 16[ ] brackets calculate expression insidefirst[(1+2)×(1+5)] = 18 + plus sign addition 1 + 1 = 2−minus sign subtraction 2 − 1 = 1±plus - minus both plus and minusoperations3 ± 5 = 8 and -2±minus - plus both minus and plusoperations3 ± 5 = -2 and 8 * asterisk multiplication 2 * 3 = 6×times sign multiplication 2 × 3 = 6 ·multiplication dot multiplication 2 · 3 = 6÷division sign /division 6 ÷ 2 = 3obelus/ division slash division 6 / 2 = 3–horizontal line division / fractionmod modulo remainder calculation 7 mod 2 = 1. period decimal point, decimal2.56 = 2+56/100separatora b power exponent 23= 8a^b caret exponent 2 ^ 3= 8√a square root √a ·√a = a√9 = ±33√a cube root 3√a ·3√a ·3√a = a3√8 = 24√a fourth root 4√a ·4√a ·4√a ·4√a = a4√16 = ±2n√a n-th root (radical) for n=3, n√8 = 2% percent1% = 1/100 10% × 30 = 3‰per-mille1‰ = 1/1000 = 0.1%10‰ × 30 = 0.3ppm per-million1ppm = 1/1000000 10ppm × 30 = 0.0003ppb per-billion 1ppb = 1/1000000000 10ppb × 30 = 3×10-7ppt per-trillion 1ppt = 10-1210ppt × 30 = 3×10-10ABC = 30°AOB = 30°°degree 1 turn = 360°α = 60°deg degree 1 turn = 360deg α = 60deg′prime arcminute, 1° = 60′α = 60°59′″double prime arcsecond, 1′ = 60″α = 60°59′59″line infinite lineAB line segment line from point A to point Bray line that start from point Aarc arc from point A to point B= 60°⊥perpendicular perpendicular lines (90° angle) AC ⊥ BC| | parallel parallel lines AB | | CD≅congruent to equivalence of geometric shapes and size ∆ABC≅∆XYZ ~ similarity same shapes, not same size ∆ABC~ ∆XYZ Δtriangle triangle shape ΔABC≅ΔBCD |x-y| distance distance between points x and y | x-y | = 5πpi constant π = 3.141592654...is the ratio between the circumference and diameter of acirclec = π·d = 2·π·rrad radians radians angle unit 360° = 2π rad c radians radians angle unit 360° = 2πcgrad gradians / gons grads angle unit 360° = 400 gradg gradians / gons grads angle unit 360° = 400 gx x variableto findwhen 2x = 4, then x = 2≡equivalence identical to≜equal by definition equal bydefinition:= equal by definition equal bydefinition~ approximately equal weakapproximation11 ~ 10≈approximately equal approximation sin(0.01) ≈ 0.01∝proportional to proportional to y∝x when y = kx, k constant ∞lemniscate infinity symbol≪much less than much less than 1 ≪1000000≫much greater than much greaterthan1000000 ≫1( ) parentheses calculateexpressioninside first2 * (3+5) = 16[ ] brackets calculateexpressioninside first[(1+2)*(1+5)] = 18{ } braces set⌊x⌋floor brackets rounds numberto lower integer⌊4.3⌋ = 4⌈x⌉ceiling brackets rounds numberto upper integer⌈4.3⌉ = 5x! exclamation mark factorial4! = 1*2*3*4 = 24 | x | single vertical bar absolute value | -5 | = 5f (x) function of x maps values ofx to f(x)f (x) = 3x+5(f∘g) function composition (f∘g) (x)= f (g(x))f (x)=3x,g(x)=x-1 ⇒(f∘g)(x)=3(x-1)(a,b) open interval (a,b) = x∈(2,6)[a ,b ] closed interval[a ,b ] = {x | a ≤ x ≤ b }x ∈ [2,6]∆ delta change / difference∆t = t 1 - t 0∆ discriminantΔ = b 2 - 4ac∑sigmasummation -sum of all values in range of series ∑ x i = x 1+x 2+...+x n∑∑sigmadouble summation∏capital piproduct -product of all values in range of series∏ x i =x 1∙x 2∙...∙x ne e constant / Euler's numbere =2.718281828... e = lim (1+1/x )x , x →∞γ Euler-Mascheroni constant γ =0.527721566...φgolden ratio golden ratio constantπpi constant π =3.141592654...is the ratiobetween the circumference and diameter of a circlec = π·d = 2·π·rA ⊗Btensor product tensor product of A and BA ⊗ Binner product[ ] brackets matrix of numbers ( ) parentheses matrix of numbers| A | determinant determinant of matrix A det(A ) determinant determinant of matrix A|| x || double vertical bars normA T transpose matrix transpose(A T )ij = (A )ji A † Hermitian matrix matrix conjugate transpose (A †)ij = (A )ji A * Hermitian matrix matrix conjugate transpose(A *)ij = (A )jiA -1inverse matrixA A -1 = Irank(A ) matrix rank rank of matrix A rank(A ) = 3 dim(U )dimensiondimension of matrix Arank(U ) = 3Probability and statistics symbolsSymbolSymbol Name Meaning / definitionExampleP (A )probability function probability of event A P (A ) = 0.5P (A ∩ B ) probability ofeventsintersection probability that of events A and BP (A ∩B ) = 0.5P (A ∪B ) probability ofevents unionprobability that of events A or B P (A ∪B ) = 0.5P (A | B )conditionalprobability function probability of event A given event B occuredP (A | B ) = 0.3f (x ) probabilitydensity function (pdf) P (a ≤ x ≤ b ) = ∫ f (x ) dxF (x )cumulativeF (x ) = P (X ≤ x )distribution function (cdf)μ population mean mean of population valuesμ = 10E (X )expectation valueexpected value of random variable X E (X ) = 10E (X | Y )conditional expectationexpected value of random variable X given YE (X | Y=2) = 5var (X ) variance variance of random variable X var (X ) = 4σ2variancevariance of population values σ2 = 4std (X ) standard deviationstandard deviation of random variable X std (X ) = 2σXstandard deviationstandard deviation value of random variable X σX = 2medianmiddle value of random variable xcov (X ,Y )covariance covariance ofrandom variables X and Y cov (X,Y ) = 4corr (X ,Y ) correlationcorrelation ofrandom variables X and Y corr (X,Y ) = 0.6ρX ,Ycorrelation correlation ofrandom variables X and YρX ,Y = 0.6∑summation summation - sum ofall values in range of series∑∑double summationdouble summationMo mode value that occurs most frequently in populationMR mid-range MR = (x max+x min)/2 Md sample median half the population isbelow this valueQ1lower / firstquartile 25% of population are below this valueQ2median /second quartile 50% of population are below this value = median of samplesQ3upper / thirdquartile 75% of population are below this valuex sample mean average / arithmeticmeanx = (2+5+9) / 3 = 5.333s2samplevariance population samples variance estimators2 = 4s samplestandarddeviationpopulation samplesstandard deviationestimators = 2z x standard score z x = (x-x) / s xX ~ distribution of X distribution ofrandom variable XX ~ N(0,3)N(μ,σ2) normaldistributiongaussian distribution X ~ N(0,3)U(a,b) uniformdistributionequal probability inrange a,bX ~ U(0,3)exp(λ)exponentialdistributionf (x) = λe-λx , x≥0gamma(c, λ)gammadistributionf (x) = λ c x c-1e-λx /Γ(c), x≥0χ 2(k) chi-squaredistributionf (x) = x k/2-1e-x/2 /( 2k/2 Γ(k/2) )F (k1, k2) F distributionBin (n ,p )binomial distribution f (k ) = n C k p k (1-p )n-kPoisson (λ)Poisson distribution f (k ) = λk e -λ / k !Geom (p )geometric distribution f (k ) = p (1-p ) kHG (N ,K ,n ) hyper-geometric distributionBern (p ) Bernoulli distributionsubset the set {9,14,28} A ⊄ B not subset left set not a subset of right set{9,66} ⊄{9,14,28} A ⊇B supersetset A has more elements or equalto the set B{9,14,28} ⊇{9,14,28} A ⊃Bproper superset / strictsupersetset A has more elements than setB{9,14,28} ⊃{9,14}A ⊅B not superset set A is not a superset of set B{9,14,28} ⊅{9,66} 2A power set all subsets of Apower set all subsets of AA =B equalityboth sets have the samemembersA={3,9,14},B={3,9,14},A=BA c complementall the objects that do not belongto set AA \B relative complementobjects that belong to A and notto BA = {3,9,14},B = {1,2,3},A-B = {9,14} A - B relative complementobjects that belong to A and notto BA = {3,9,14},B = {1,2,3},A-B = {9,14} A ∆ B symmetric differenceobjects that belong to A or B butnot to their intersectionA = {3,9,14},B = {1,2,3},A ∆B ={1,2,9,14} A ⊖ B symmetric differenceobjects that belong to A or B butnot to their intersectionA = {3,9,14},B = {1,2,3},A ⊖B ={1,2,9,14}a∈A element of set membership A={3,9,14}, 3 ∈Ax∉A not element of no set membership A={3,9,14}, 1 ∉A(a ,b ) ordered pair collection of 2 elementsA×B cartesian product set of all ordered pairs from Aand B|A| cardinality the number of elements of set AA={3,9,14}, |A|=3 #A cardinality the number of elements of set AA={3,9,14}, #A=3aleph-nullinfinite cardinality of naturalnumbers setaleph-one cardinality of countable ordinalnumbers setØ empty set Ø = { }C = {Ø}universal set set of all possible valuesnatural numbers / whole numbers set (with zero) 0 = {0,1,2,3,4,...}0 ∈ 01natural numbers / wholenumbers set (withoutzero)1 = {1,2,3,4,5,...}6 ∈ 1integer numbers set= {...-3,-2,-1,0,1,2,3,...} -6 ∈rational numbers set= {x | x =a /b , a ,b ∈}2/6 ∈real numbers set= {x | -∞ < x <∞} 6.343434∈complex numbers set= {z | z=a +bi ,-∞<a <∞, -∞<b <∞}6+2i ∈∨reversed caret or x∨y | vertical line or x | y x' single quote not - negation x'x bar not - negation x¬not not - negation ¬x ! exclamation mark not - negation ! x ⊕circled plus / oplus exclusive or - xor x⊕y ~ tilde negation ~ x ⇒implies⇔equivalent if and only if (iff)↔equivalent if and only if (iff)∀for all∃there exists∄there does not exists∴therefore∵because / sincee e constant / Euler'snumber e = 2.718281828...e = lim(1+1/x)x ,x→∞y ' derivative derivative - Lagrange's notation (3x3)' = 9x2y '' second derivative derivative of derivative (3x3)'' = 18xy(n)nth derivative n times derivation (3x3)(3) = 18derivative derivative - Leibniz's notation d(3x3)/dx = 9x2second derivative derivative of derivative d2(3x3)/dx2 = 18xnth derivative n times derivationtime derivative derivative by time - Newton's notationtime secondderivativederivative of derivativeD x y derivative derivative - Euler's notationD x2y second derivative derivative of derivativepartial derivative ∂(x2+y2)/∂x = 2x ∫integral opposite to derivation ∫f(x)dx∫∫double integral integration of function of 2variables∫∫f(x,y)dxdy∫∫∫triple integral integration of function of 3variables∫∫∫f(x,y,z)dxdydz∮closed contour / lineintegral∯closed surfaceintegral∰closed volumeintegral[a,b] closed interval [a,b] = {x | a ≤ x ≤ b}(a,b) open interval (a,b) = {x | a < x < b}i imaginary unit i≡ √-1 z = 3 + 2i z* complex conjugate z = a+bi→z*=a-bi z* = 3 - 2i z complex conjugate z = a+bi→z = a-bi z = 3 - 2i ∇nabla / del gradient / divergence operator ∇f (x,y,z)vectorunit vectorx * y convolution y(t) = x(t) * h(t)Laplace transform F(s) = {f (t)}Fourier transform X(ω) = {f (t)}δdelta function∞lemniscate infinity symbol。
数学符号的中英文读法

section; division
分节号
→
arrow
箭号;参见号
+
plus
加号;正号
-
minus
减号;负号
±
plus or minus
正负号
×
is multiplied by
乘号
÷
is divided by
除号
=
is equal to
等于号
≠
is not equal to
不等于号
≡
is equivalent to
Ν
(纽)
Ξ
(可系)
Ο
(奥密克戎)
Π
(派)
Ρ
(若)
Σ
(西格马)
Τ
(套)
Υ
(英文或拉丁字母)
Φ
(斐)
Χ
(喜)
Ψ
(普西)
Ω
(欧米伽)
Mu
Ν ν: 拗
Nu
Ξ ξ: 克西
KeXi
Ο ο: 欧麦克轮 Omicron
∏ π: 派
Pi
Ρ ρ: 柔
Rho
∑ σ: 西格玛
Sigma
Τ τ: 套
Tau
Υ υ: 宇普西龙 Upsilon
Φ φ: fai
Phi
Χ χ: 器
Chi
Ψ ψ: 普赛
Psi
Ω ω: 欧米伽
Omega
希腊字母怎么打:打开 Office 文档之后,在你需要输入希腊字母的时候,先将输入法切换为
ω(欧米伽)。
更全面:
1 Α α alpha a:lf
阿尔法 角度系数
2 Β β beta bet
贝塔 磁通系数 角度系数
你知道这些数学符号和名称如何用英文表达吗?

你知道这些数学符号和名称如何用英文表达吗?加法:addition表达“加”的含义的词:add, and, more, sum, increase, plus, total, together减法:subtraction表达“减”的含义的词:fewer, minus, take away, difference between, take from, reduce, decrease乘法:multiplication表达“乘”的含义的词:multiply, times, groups of, multiplied by, lots of, product除法:division表达“除”的含义的词:divide, share, divisible by, share equally, divide into group, divided by等于:equals表达“等于”的含义的词:makes, totals, is equivalent to, is the same as大于 is greater than小于 is less than运算符 operator数字 digit数 number自然数 natural number整数 integer质数 prime number小数 decimal小数点 decimal point分数 fraction分子 numerator分母 denominator比 ratio正 positive负 negative零 null, zero, nought, nil十进制 decimal system二进制 binary system十六进制 hexadecimal system四舍五入 round下舍入 round down上舍入 round up代数 algebra公式 formula, formulae(pl.)单项式 monomial多项式 polynomial, multinomial系数 coefficient未知数 unknown, x-factor, y-factor, z-factor 等式,方程式 equation一次方程 simple equation二次方程 quadratic equation三次方程 cubic equation四次方程 quartic equation不等式 inequation阶乘 factorial对数 logarithm指数,幂 exponent乘方 power二次方,平方 square三次方,立方 cube四次方 the power of four, the fourth power n次方 the power of n, the nth power开方 evolution, extraction二次方根,平方根 square root三次方根,立方根 cube root四次方根 the root of four, the fourth root n次方根 the root of n, the nth root集合 aggregate元素 element空集 void子集 subset交集 intersection并集 union补集 complement映射 mapping函数 function定义域 domain, field of definition 值域 range常量 constant变量 variable单调性 monotonicity奇偶性 parity周期性 periodicity图象 image数列,级数 series微积分 calculus微分 differential导数 derivative极限 limit无穷大 infinite(a.) infinity(n.)无穷小 infinitesimal积分 integral定积分 definite integral不定积分 indefinite integral有理数 rational number无理数 irrational number实数 real number虚数 imaginary number复数 complex number矩阵 matrix行列式 determinant几何 geometry点 point线 line面 plane体 solid线段 segment射线 radial平行 parallel相交 intersect角 angle角度 degree弧度 radian锐角 acute angle直角 right angle钝角 obtuse angle平角 straight angle周角 perigon底 base边 side高 height三角形 triangle锐角三角形 acute triangle直角三角形 right triangle直角边 leg斜边 hypotenuse勾股定理 Pythagorean theorem 钝角三角形 obtuse triangle不等边三角形 scalene triangle 等腰三角形 isosceles triangle 等边三角形 equilateral triangle四边形 quadrilateral平行四边形 parallelogram矩形 rectangle长 length宽 width菱形 rhomb, rhombus, rhombi(pl.), diamond 正方形 square梯形 trapezoid(美),trapezium(英)直角梯形 right trapezoid等腰梯形 isosceles trapezoid五边形 pentagon六边形 hexagon七边形 heptagon八边形 octagon九边形 enneagon十边形 decagon十一边形 hendecagon十二边形 dodecagon多边形 polygon正多边形 equilateral polygon圆 circle圆心 centre(英), center(美)半径 radius直径 diameter圆周率 pi弧 arc半圆 semicircle扇形 sector环 ring椭圆 ellipse圆周 circumference周长 perimeter面积 area轨迹 locus, loca(pl.)相似 similar全等 congruent立方体 cube四面体 tetrahedron五面体 pentahedron六面体 hexahedron平行六面体 parallelepiped 七面体 heptahedron八面体 octahedron九面体 enneahedron十面体 decahedron十一面体 hendecahedron 十二面体 dodecahedron 二十面体 icosahedron多面体 polyhedron棱锥 pyramid棱柱 prism棱台 frustum of a prism 旋转 rotation轴 axis圆锥 cone圆柱 cylinder圆台 frustum of a cone 球 sphere半球 hemisphere底面 undersurface表面积 surface area体积 volume空间 space坐标系 coordinates坐标轴 x-axis, y-axis, z-axis 横坐标 x-coordinate纵坐标 y-coordinate原点 origin双曲线 hyperbola抛物线 parabola三角 trigonometry正弦 sine余弦 cosine正切 tangent余切 cotangent正割 secant余割 cosecant反正弦 arc sine反余弦 arc cosine反正切 arc tangent反余切 arc cotangent反正割 arc secant反余割 arc cosecant相位 phase周期 period振幅 amplitude内切圆 inscribed circle外切圆 circumcircle统计 statistics平均数 average加权平均数 weighted average方差 variance标准差 root-mean-square deviation, standard deviation 比例 propotion百分比 percent百分点 percentage排列 permutation组合 combination概率,或然率 probability分布 distribution正态分布 normal distribution非正态分布 abnormal distribution图表 graph条形统计图 bar graph柱形统计图 histogram折线统计图 broken line graph曲线统计图 curve diagram扇形统计图 pie diagram公理 axiom定理 theorem计算 calculation运算 operation证明 prove假设 hypothesis, hypotheses(pl.) 命题 proposition。
各种数学符号英文翻译

+ plus加号;正号- minus减号;负号±plus or minus正负号×is multiplied by乘号÷is divided by除号=is equal to等于号≠is not equal to不等于号≡is equivalent to全等于号≌is equal to or approximately equal to等于或约等于号≈is approximately equal to约等于号<is less than小于号>is greater than大于号≮is not less than不小于号≯is not more than不大于号≤is less than or equal to小于或等于号≥is more than or equal to大于或等于号% per cent百分之…‰per mill千分之…∞infinity无限大号∝varies as与…成比例√(square) root平方根∵since; because因为∴hence所以∷equals, as (proportion)等于,成比例∠angle角≲semicircle半圆≰circle圆○circumference圆周πpi 圆周率△triangle三角形≱perpendicular to垂直于∪union of并,合集∩intersection of 交,通集∫the integral of …的积分∑(sigma) summation of总和°degree度′minute分″second秒℃Celsius system摄氏度{open brace, open curly左花括号}close brace, close curly右花括号(open parenthesis, open paren左圆括号)close parenthesis, close paren右圆括号() brakets/ parentheses括号[open bracket 左方括号]close bracket 右方括号[] square brackets方括号.period, dot句号,点|vertical bar, vertical virgule竖线&ersand, and, reference, ref和,引用*asterisk, multiply, star, pointer星号,乘号,星,指针/slash, divide, oblique 斜线,斜杠,除号//slash-slash, comment 双斜线,注释符#pound井号\backslash, sometimes escape反斜线转义符,有时表示转义符或续行符~tilde波浪符.full stop句号,comma逗号:colon冒号;semicolon分号?question mark问号!exclamation mark (英式英语) exclamation point (美式英语)'apostrophe撇号-hyphen连字号-- dash 破折号...dots/ ellipsis省略号"single quotation marks 单引号""double quotation marks 双引号‖parallel 双线号&ersand = and~swung dash 代字号§section; division 分节号→arrow 箭号;参见号。
表示各种数学符号的英文翻译

表示各种数学符号的英文翻译表示各种数学符号的英文翻译有各种各样数学符号,那用英文来表示数学符号的翻译也是有很多的。
以下是店铺为大家整理了关于表示各种各样数学符号的部分英文翻译,一起来看看吧!表示交集的英文翻译:union of1、The new government built up close relations with the Soviet Union, which was especially keen to make use of the port of Berbera.新政府建立了密切的关系,苏联,这是特别热衷于利用伯贝拉港。
2、The College of Textile Engineering of Beijing Union University was one of the six members.北京联合大学纺织工程学院参加了本次调查。
3、Methods:196 consecutive closed Grade I open fracture of lower limb treated with external fixation, nickelclad and bolt or intramedullary rod fixation, major is femoral bone and fracture of fibula, 88 patients who smoked and 108 patients who did not smoke had followed to union and rehabilitate in the same condition.选择196例经外部固定或钢板螺钉、髓内针内固定治疗的连续的闭合或一级开放的下肢骨折患者,主要为股骨、胫腓骨骨折患者,在相同康复治疗下观察88例吸烟患者和108例不吸烟患者的骨折愈合和功能康复效果。
4、Unlike the Soviets Union, who didn't engage in the kind of super-Neo-Capital is m that China does, I don't believe that China will collapse under the weight of itself.跟苏联不同,中国现在在经济上搞得是超级新资本主义,效率极高,我不指望中国会像苏联那样由于自己经济的缺陷而崩溃,中国现在通过跟西方的贸易,很牢固的掌握了国内的民心。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
+ plus加号;正号
- minus减号;负号
±plus or minus正负号
×is multiplied by乘号
÷is divided by除号
=is equal to等于号
≠is not equal to不等于号
≡is equivalent to全等于号
≌is equal to or approximately equal to等于或约等于号≈is approximately equal to约等于号
<is less than小于号
>is greater than大于号
≮is not less than不小于号
≯is not more than不大于号
≤is less than or equal to小于或等于号
≥is more than or equal to大于或等于号
% per cent百分之…
‰per mill千分之…
∞infinity无限大号
∝varies as与…成比例
√(square) root平方根
∵since; because因为
∴hence所以
∷equals, as (proportion)等于,成比例
∠angle角
≲semicircle半圆
≰circle圆
○circumference圆周
πpi 圆周率
△triangle三角形
≱perpendicular to垂直于
∪union of并,合集
∩intersection of 交,通集
∫the integral of …的积分
∑(sigma) summation of总和
°degree度
′minute分
″second秒
℃Celsius system摄氏度
{open brace, open curly左花括号
}close brace, close curly右花括号
(open parenthesis, open paren左圆括号
)close parenthesis, close paren右圆括号
() brakets/ parentheses括号
[open bracket 左方括号
]close bracket 右方括号
[] square brackets方括号
.period, dot句号,点
|vertical bar, vertical virgule竖线
&ersand, and, reference, ref和,引用
*asterisk, multiply, star, pointer星号,乘号,星,指针
/slash, divide, oblique 斜线,斜杠,除号
//slash-slash, comment 双斜线,注释符
#pound井号
\backslash, sometimes escape反斜线转义符,有时表示转义符或续行符~tilde波浪符
.full stop句号
,comma逗号
:colon冒号
;semicolon分号
?question mark问号
!exclamation mark (英式英语) exclamation point (美式英语)
'apostrophe撇号
-hyphen连字号
-- dash 破折号
...dots/ ellipsis省略号
"single quotation marks 单引号
""double quotation marks 双引号
‖parallel 双线号
&ersand = and
~swung dash 代字号
§section; division 分节号
→arrow 箭号;参见号。
