博弈论习题集

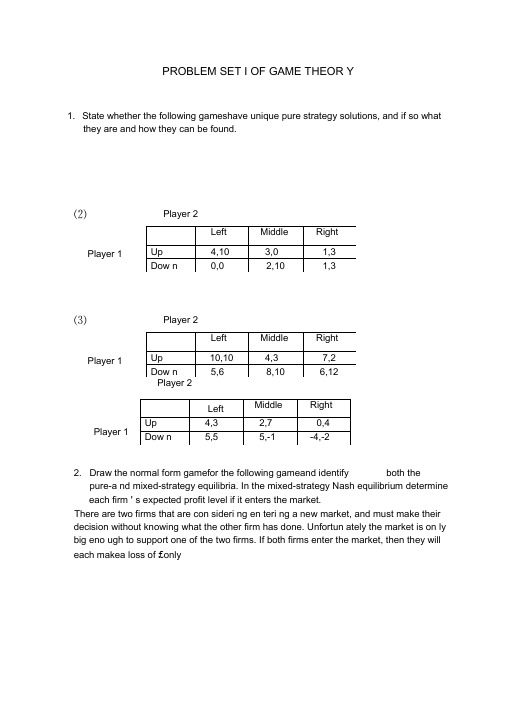
PROBLEM SET I OF GAME THEORY1. State whether the following games have unique pure strategy solutions, and if so what they are and how they can be found.(1) Player 2Player 1(2) Player 2Player 1(3) Player 2Player 12. Draw the normal form game for the following game and identify both the pure-and mixed-strategy equilibria. In the mixed-strategy Nashequilibrium determine each firm ’s expected profit level if it enters the market.There are two firms that are considering entering a new market, and must make their decision without knowing what the other firm has done. Unfortunately the market is only big enough to support one of the two firms. If both firms enter the market, then they will each make a loss of £ onlyone firm enter s the market, th at firm will earn a profit of £50m, and the other firm will just break even.3. Convert the following extensive form game into a normal form game, and identify the Nash equilibria and subgame perfect Nash equilibria. Finally, what is the Nash equilibrium if both players make their moves simultaneously4. Consider an economy consisting of one government and two people. Let x i be the choice of the people, where x i ∈X = {x L , x M , x H }, and i=1, 2， and y the choice of the government, where y ∈Y= {y L , y M , y H }. The payoffs to the government-household are given by the values of u 1(x 1, x 2, y) and u 2(x 1, x 2, y) = u 1(x 2,x 1, y) . These payoffs are entered in the following table:12government ’s policy. Enter the blank with value ranges such that the Nash equilibria are supported.(2)Suppose the government moves first, find Nash Equilibria, the subgame perfect Nash equilibria, and the subgame perfect outcome. Is the outcome efficient Why(3)Show whether there exists Nash equilibrium (in pure strategies) forthe one-period economy when households and the government move simultaneously.(4)If the household choose first, do question (2) again.5.Assume that two players are faced with Rosenthal’s centipede game.Use Bayes’ theorem to calculate the players’ reputation for being co-operative in the following situations if they play across.(1)At the beginning of the game each player believes that there is a 50/50chance that the other player is rational or co-operative. It is assumed that a co-operative player always plays across. Furthermore suppose that a rational player will play across with a probability of (2)At their second move the players again move across. (Continue to assumethat the probability that a rational player plays across remains equal .(3)How would the players’ reputation have changed after the first movehad the other player believed that rational players always play across.(Assume all other probabilities remain the same.)(4)Finally, how would the players’ reputation have changed after thefirst move had the other player believed that rational players never play across. (Again assume all other probabilities remain the same.)6.Assume there are m identical Stackelberg leaders in an industry,indexed j=1,…, m, and n identical Stackelberg followers, indexed k=1,…, n. All firms have a constant marginal cost of c and no fixed costs. The market price, Q, is determined according to the equation , where Q is total industry output, and ɑis a constant. Find the subgame perfect Nash equilibrium supply for the leaders and the followers. Confirm the duopoly results for both Cournot competition and Stackelberg competition, and the generalized Cournot result for n firms derived in Exercise .7.Assume that there are i=1,…, n identical firms in an industry, eachwith constant marginal costs of c and no fixed costs. If the market price, P, is determined by the equation , where Q is total industry output and ɑ is a constant, determine the Cournot-Nash equilibrium output level for each firm. Where happens as n8.Find the separating equilibrium behaviour of the low-cost incumbentin the following two-period model. The incumbent has marginal costs equal to either £4 or £2. Only the incumbent initially knows its exact costs. The entrant observes the incumbent’s output decision in the first period and only enters the market in the second period if it believes that the incumbent has high marginal costs. If entry does occur, the two firms Cournot compete, and we assume that at this stage in the game the incumbent’s true costs are revealed. Price, P, isdetermined by the following equation , where Q is thecombined output of the two firms. Finally, it is assumed that the firms’ discount factor is equal to .9.In the text we argued that a weak government can exploit the privatesector’s uncertainty about the government’s preferences topartially avoid the inflationary bias associated withtime-inconsistent monetary policy. In this exercise we provided a simple model that illustrates this result.Assume that the government, via its monetary policy, can perfectly control inflation. Furthermore the government can be one of two types. Either it is strong or it is weak. A strong government is only concerned about the rate of inflation, and so never inflates the economy. A weak government, however, is concerned about both inflation and unemployment. Specially, its welfare in time-period t is given by the following equation：,where and are the rates of inflation and unemployment in time-period t respectively, and c, d and e are all positive parameters. It is assumed the government does not discount future welfare, and so a weak government attempts to maximize the sum of its per-period welfare over all current and future periods. The constraint facing the government is given by the expectations-augmented Phillips curve. This is written as,where is the expected rate of inflation in period t determined at the beginning of that period, and again ɑand b are positive parameters. The private sector formulates its expectations rationally in accordance with Bayes’ Theorem. Finally, it is assumed that this policy game lasts foronly two periods.(1)Determine the subgame perfect path of inflation if it is commonknowledge the government is weak.(2)Determine the sequential equilibrium path of inflation if there isincomplete information and the private sector’s prior probability that the government is strong is . (Hint: initially determine the necessary condition for the weak government to be indifferent between inflating and not inflating the economy.)。