2016-2017四年级美国大联盟答案
2016-2017第二学期四年级期末测试
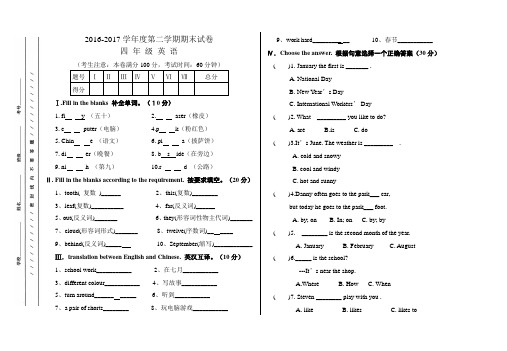
2016-2017学年度第二学期期末试卷四年级英语(考生注意:本卷满分100分,考试时间:60分钟)Ⅰ.Fill in the blanks 补全单词。
(10分)1. fi y (五十)2. aser(橡皮)3. c puter(电脑)4.p k(粉红色)5. Chin e (语文)6. pi a(披萨饼)7. di er(晚餐)8. b s ide(在旁边)9. ni h (第九)10.r d (公路)Ⅱ. Fill in the blanks according to the requirement. 按要求填空。
(20分)1、tooth( 复数)______2、this(复数)________3、leaf(复数)__________4、far(反义词)______5、out(反义词)_______6、they(形容词性物主代词)_______7、cloud(形容词形式)_______ 8、twelve(序数词)__ ____9、behind(反义词)_____ 10、September(缩写)____________Ⅲ.translation between English and Chinese. 英汉互译。
(10分)1、school work___________2、在七月___________3、different colour___________4、写故事___________5、turn around______ _____6、听到___________7、a pair of shorts________ 8、玩电脑游戏___________9、work hard_________ __ 10、春节___________ Ⅳ.Choose the answer. 根据句意选择一个正确答案(30分)( )1. January the first is _______ .A. National DayB. New Year’s DayC. International Workers’ Day( )2. What _________ you like to do?A. areB.isC. do( )3.It’s June. The weather is _________ .A. cold and snowyB. cool and windyC. hot and sunny( )4.Danny often goes to the park___ car,but today he goes to the park___ foot.A. by; onB. In; onC. by; by()5. ________ is the second month of the year.A. JanuaryB. FebruaryC. August ( )6._____ is the school?---It’s near the shop.A.WhereB. HowC. When( )7. Steven ________ play with you .A. likeB. likesC. likes to( )8. How tall_____ he?A. areB. amC. is( )9. I _____ every day.A.go to the schoolB.go schoolC.go to school ( )10.What’s your favourite food______ lunch?A. atB. toC. for( )11. Her sister likes to play her dog.A. ofB. withC. to( )12. Do you like math?Yes, I do. my favourite subject is English.A . And B. But C. Or( )13. What’s your favourite ? ---Sweater.A. subjectsB. colourC. clothes( )14. I live a house beside the park.A. onB. inC. at( )15. The cinema is far our school.A. toB. forC. fromⅤ. Read and choose. 选词填空。
2018年美国“数学大联盟杯赛”(中国赛区)初赛四年级试卷(1)

2017-2018年度美国“数学大联盟杯赛”(中国赛区)初赛(四年级)(初赛时间:2017年11月26日,考试时间90分钟,总分200分)学生诚信协议:考试期间,我确定没有就所涉及的问题或结论,与任何人、用任何方式交流或讨论,我确定我所填写的答案均为我个人独立完成的成果,否则愿接受本次成绩无效的处罚。
请在装订线内签名表示你同意遵守以上规定。
考前注意事项:1. 本试卷是四年级试卷,请确保和你的参赛年级一致;2. 本试卷共4页(正反面都有试题),请检查是否有空白页,页数是否齐全;3. 请确保你已经拿到以下材料:本试卷(共4页,正反面都有试题)、答题卡、答题卡使用说明、英文词汇手册、草稿纸。
考试完毕,请务必将英文词汇手册带回家,上面有如何查询初赛成绩、及如何参加复赛的说明。
其他材料均不能带走,请留在原地。
选择题:每小题5分,答对加5分,答错不扣分,共200分,答案请填涂在答题卡上。
1.Which of the following is the smallest?A) 2.018 B) 20.18 C) 0.218 D) 20182.What is the least common multiple of 20 and 18?A) 90 B) 180 C) 240 D) 3603.The sum of the degree-measures of the exterior angles of a triangle is?A) 180 B) 360 C) 540 D) 7204.In the figure on the right, please put the numbers 1 – 11 in the elevencircles so that the three numbers in every straight line add up to 18.What is the number in the middle circle? Note: There are 5 straightlines in total in this figure.A) 6 B) 7 C) 8 D) 95.I am a lovely cat. When I multiply the digits of a whole numberand the product I get is 8, I put that whole number on my list offavorite numbers. Of the whole numbers from 1000 to 9999,how many would I put on my list of favorite numbers?A) 10 B) 12 C) 16 D) 206.Two planes take off at the same time from the same point to race to apoint and back. Place A travels at 180 miles per hour on the way out and240 miles per hour on the return trip. Plane B covers the entire distance at an averagespeed of 210 miles per hour. Which plane wins the race, or is it a tie?A) plane A wins B) plane B winsC) a tie D) non-deterministic 7.52 × 88 = 44 ×?A) 102 B) 96 C) 104 D) 1248.What is the smallest whole number that leaves a remainder of 4, 5, 6 when divided byeach of 5, 6, 7?A) 29 B) 209 C) 210 D) 20099.In △ABC, m∠A + m∠C = m∠B. What is the degree measure of ∠B?A) 80 B) 90 C) 100 D) 18010.I bought a toy for $10, sold it for $20, rebought it for $30, and resold it for $40. My totalprofit on the 4 transactions was ?A) 10 B) 20 C) 30 D) 4011.What is the greatest number of integers I can choose from the first ten positive integers sothat any 3 of the chosen integers could be the lengths of the three sides of a triangle?A) 4 B) 5 C) 6 D) 712.How many whole numbers between 200 and 400 have all their digits increasing in valuewhen read from left to right?A) 30 B) 36 C) 42 D) 4813.What is the value of 1% of 10% of 100?A) 0.01 B) 0.1 C) 1 D) 1014.If three cats can eat three bowls of food in three minutes, how many minutes will it take100 cats to eat 100 bowls of food?A) 1 B) 3C) 100 D) None of the above15.There are three squares. The area of the smallest one is 2. The side-length of the secondsquare is twice the side-length of the smallest one. And the side-length of the third square is three-times the side-length of the smallest one. The total area of the three squares isA) 12 B) 28 C) 36 D) 7216.A man, who had been married for three years, spent25of his yearly income on his family,14on business, and110on personal travel. If he saved $45000 during those three years, what was his annual income?A) $45000 B) $50000C) $65000 D) None of the above17.Given four different integers, at most how many different sums can be formed bychoosing two, three, or four of them and finding each sum?A) 8 B) 9 C) 10 D) 1118. Max places 100 eggs in 10 baskets, with each basket receiving at least1 egg, but no2 baskets receiving the same number of eggs. What is the greatest number of eggs that may be placed in a basket?A) 45 B) 47 C) 55 D) 6519. 2 + 3 × 4 – 5 =A) 0 B) 6 C) 9 D) 15 20. What is the highest power of 2 that divides 2 × 4 × 6 × 8 × 10? A) 25 B) 27 C) 28 D) 215 21. Which of the following is a prime number?A) 2017B) 2018C) 2015D) 201622. What is the greatest possible number of acute angles in a figure consisting of a triangleand a line passing through two sides of the triangle?A) 5B) 6C) 7D) 823. Amy can solve 5 questions every 3 minutes. Kate can solve 3 questions every 5 minutes.How many more questions Amy can solve than Kate in one hour?A) 15B) 32C) 60D) 6424. Using 3 Ts and 2 Js, in how many different orders can the five letters be arranged? Forexample, TTTJJ and TTJJT are two such different orders.A) 2B) 10C) 20D) 6025. Coastal Coconuts can divide all their coconuts evenly among 8, 9, or10 customers, with 1 coconut left over each time. If Coastal Coconuts has more than 1 coconut, what is the least number of coconuts they could have?A) 561 B) 721C) 831 D) None of the above 26. 35 ÷ 32 =A) 3 B) 9 C) 27 D) 81 27. If the sum of three prime numbers is 30, what is the least prime number?A) 2B) 3C) 5D) 728. Juxtaposing two identical squares to form a rectangle, the perimeter of the rectangle is 12less than the sum of the perimeter of the two squares. What is the side-length of the original square?A) 3B) 6C) 9D) 1229. It takes Mike 2 hours to finish a task. It takes 4 hours for Tom to finish the same task.Mike and Tom worked together on this task for one hour before Mike had to leave. How long will it take Tom to finish the rest of the task?A) 1 B) 2 C) 3 D) 4 30. The number of triangles in the figure on the right isA) 9 B) 10 C) 11 D) 12 31. What is the thousands digit of the product 1234560 × 2345670 × 3456780?A) 8B) 6C) 5D) 032. The sum of nine consecutive positive integers is always divisible byA) 2B) 5C) 7D) 933. You can put as many as 96 books in 6 backpacks. How many backpacks are necessary for144 books?A) 7B) 8C) 9D) 1034. The number of nickels I have is twice the number of dimes I have, and together thesecoins are worth more than $1. The least number of dimes that I can have isA) 5B) 6C) 8D) 1035. The ages of four kids are four consecutive positive integers. The product of their ages is3024. How old is the oldest kid?A) 8B) 9C) 10D) 1136. In the Game of Life, you earn 3 points for flipping a coin to “heads”, and 5 points forflipping a coin to “tails”. In all, how many positive whole number scores are IMPOSSIBLE to get after flipping it one or more times?A) 4B) 5C) 7D) 1137. Four monkeys can eat four bags of peanuts in three minutes. How many monkeys will ittake to eat 100 bags of peanuts in one hour?A) 4 B) 5 C) 20 D) 100 38. The tens digit of the product of the first 100 positive integers isA) 2B) 4C) 8D) 039. Someone put three dimes into my pile of quarters. If I add up the value of these coins,including the dimes, the sum could beA) $6.25B) $7.75C) $8.05D) $9.5040. Brooke's empty tub fills in 20 minutes with the drain plugged, andher full tub drains in 10 minutes with the water off. How manyminutes would it take the full tub to drain while the water is on?A) 12B) 15 C) 20 D) 30。
2018-2019年美国“大联盟”(Math League)思维探索活动第一阶段四年级试卷及答案

2018-2019年度美国“大联盟”(Math League)思维探索活动第一阶段(四年级)(活动日期:2018年11月25日,答题时间:90分钟,总分:200分)学生诚信协议:答题期间,我确定没有就所涉及的问题或结论,与任何人、用任何方式交流或讨论,我确定我所填写的答案均为我个人独立完成的成果,否则愿接受本次成绩无效的处罚。
请在装订线内签名表示你同意遵守以上规定。
考前注意事项:1. 本试卷是四年级试卷,请确保和你的参赛年级一致;2. 本试卷共4页(正反面都有试题),请检查是否有空白页,页数是否齐全;3. 请确保你已经拿到以下材料:本试卷(共4页,正反面都有试题)、答题卡、答题卡使用说明、英文词汇手册、草稿纸。
试卷、答题卡、答题卡使用说明、草稿纸均不能带走,请留在原地。
4. 本试卷题目很多也很难,期待一名学生所有题目全部答对是不现实的,能够答对一半题目的学生就应该受到表扬和鼓励。
选择题:每小题5分,答对加5分,答错不扣分,共200分,答案请填涂在答题卡上。
1.(123 + 456) + 678 = (123 + 678) + ?A) 123 B) 456 C) 579 D) 6782.Bea sharpened 1200 pencils. Half the pencils had erasers, andhalf of all the erasers were pink. How many pencils with pinkerasers did Bea sharpen?A) 200 B) 300C) 400 D) 6003.I have a prime number of pairs of socks. The total number of socks I have could not beA) 26 B) 38 C) 46 D) 544.The product of 500 000 and 200 000 has exactly ? zeros.A) 5 B) 6 C) 10 D) 115.Divide 100 by 10, then multiply the result by 10. The final answer isA)0 B)1 C) 10 D) 1006.At most how many complete 8-minute songs can I sing in 3 hours?A) 22 B) 23 C) 24 D) 1807. 2 × (44 + 44 + 44) = 88 + 88 + ?A) 0 B) 44 C) 66 D) 888. A rectangle has sides of even lengths and perimeter 12. Its area isA) 6 B) 8 C) 9 D) 169.16 × (17 + 1) –? × (15 + 1) = 0A) 15 B) 16 C) 17 D) 1810.The crowd clapped for 840 seconds, stopping at 8:15 P.M. Theystarted clapping at ? P.M.A) 7:59 B) 8:01C) 8:08 D) 8:1411.If each digit of my 5-digit ID code is different, the sum of its digitsis at mostA) 15 B) 25 C) 35 D) 4512.At the museum, adult tickets cost $4 each and child tickets cost $3 each. With $50, I canbuy ? more child tickets than adult tickets.A) 1 B) 4 C) 12 D) 1613.Each day last week I read for a whole number of hours. I read forthe same number of hours each day except Sunday. If I read for 12hours last week, I read for ? hours on Sunday.A) 7 B) 6 C) 5 D) 414.The product 2 × 3 × 4 × 5 × 6 has the same value as the product ? × 3 × 5.A) 12 B) 36 C) 48 D) 6315.The average test grade in my class is a whole number, and the sum of the test grades is2400. Of the following, which could be the total number of test grades?A) 18 B) 21 C) 27 D) 3216.If twice a whole number is 120 less than five times the same whole number, then half thewhole number isA) 10 B) 20 C) 40 D) 6017.The number of bees I have doubles each day. If I had 1024 bees last Friday, the first daythe number of bees was more than 100 was aA) Tuesday B) WednesdayC) Thursday D) Friday18.Each of 6 dogs ate 3 treats from each of 4 bags. If each bag started with 30 treats, the 4bags together ended with ? treats.A) 36 B) 48 C) 72 D) 9619.What is the greatest possible product of two different even whole numbers whose sum is100?A) 196 B) 625 C) 2496 D) 250020. Ed built 3 times as many houses as Bob, who built half as many houses as Ally. If the 3 of them built 96 houses in all, Ed and Ally built a combined total of ? houses.A) 16 B) 32 C) 48D) 8021. How many factors of 2 × 4 × 8 × 16 are multiples of 4?A) 3B) 4C) 8D) 922. When I divide a certain number by 3 or 5, I get a remainder of 2. The sum of the digits ofthe least number for which this is true isA) 1B) 3C) 7D) 823. My 144 fish are split between 2 tanks so that 1 tank has twice as many fish as the other. How many fish must I move from one tank to the other so that both tanks have the same number of fish?A) 24 B) 48 C) 60D) 7224. A 3-digit number is the product of at most ? whole numbers greater than 1.A) 2B) 3C) 9D) 1025. Abby earns $2 for every clam she finds and $3 for every oyster. If Abby finds 5 times asmany oysters as clams, which of the following could be her total earnings?A) $150B) $160C) $170D) $18026. (The average value of the 10 smallest even whole numbers greater than 0) – (the average value of the 10 smallest odd whole numbers) =A) 0B) 1C) 10D) 1127. Ana planted seeds in rows. If the total number of rowsequaled the number of seeds in each row, the number of seeds planted could have beenA) 194B) 216C) 250D) 28928. What is the greatest possible sum of five 2-digit whole numbers if all 10 digits of the fivenumbers are different?A) 270B) 315C) 360D) 48529. I thought I wrote every whole number between 1 and 500 in order from least to greatest, but actually I skipped 3 numbers in a row. If I left out a total of 8 digits, what is the sum of the numbers I skipped?A) 100B) 150C) 300D) 39030. Written backwards, 123 becomes 321. How many whole numbers between 100 and 200 have a larger value when written backwards?A) 70B) 80C) 90D) 9831. The average of four different numbers is 18. And the least of the four numbers is 3. What is the least possible value of the biggest of the four numbers?A) 21B) 23C) 24D) 6032. 3 tigers can eat 36 Big Macs in 6 minutes. How many Big Macs can 12 tigers eat in 3 minutes?A) 18B) 36C) 72D) 28833. In the followi ng sequence, 2, 0, 1, 8, 2, 0, 1, 8, … (repeating), what is its 2018th term?A) 2B) 0C) 1D) 834. 2018a b c is a multiple of 9. What is the least possible value of abc ? (Note: abc is a three-digit number, which means a is not 0.)A) 7B) 100C) 106D) 99735. What is the least common multiple of 84 and 112?A) 28B) 196C) 336D) 940836. In triangle ABC , ∠C = 90°, ∠A = 15°, AB = 20. What is the area of this triangle?A) 20B) 50C) 100D) 20037. ABCD is a rectangle and its perimeter is 22, as shown at the right. EFGH is a square. AH = 6. CF = ?A) 4 B) 5 C) 6D) 838. How many leap years are there between 2018 and 2081?A) 16B) 17C) 18D) 1939. My class was lined up on the gym floor in 8 rows, with 2 students in each row. If our coach rearranged us so that the number of rows was the same as the number of students in each row, how many rows were there after we were rearranged? A) 4B) 6C) 10D) 1640. Of the 100 numbers from 1 to 100, how many of them don’t contain 7 as its digit?A) 65 B) 75C) 80 D) none of the above。
四年级上册英语期末试卷及答案2017

【 - 小学作文】下面是zw234作文网小编为大家带来的四年级上册英语期末试卷及答案2017,希望能帮助到大家!四年级上册英语期末试卷及答案20172016年—2017年四年级上册英语期末试卷(有答案)Listening Part(听力部分)一、Listen and choose. 听录音,选择正确的选项。
( ) 1. A. window B. picture ( ) 2. A. notebook B. storybook( ) 3. A. wall B. tall ( ) 4. A. shoe B. glasses( ) 5. A. table B. phone ( ) 6. A. beef B. bowl( ) 7. A. candy B. cousin ( ) 8. A. driver B. farmer( ) 9. A. soup B. spoon ( ) 10. A. friendly B. fridge二、 Listen and choose. 听录音,选择正确的图片。
( ) 1. A. ( ) 2. A. B.B.( ) 3.A. B. ( ) 4. A. B.( ) 5.A. B.三、Listen and choose. 听录音,选出你所听到的句子。
1. A. Let’s clean the classroom. B. Let’s clean the window.2. A. What’s in your classroom. B. What colour is it?3. A. It’s blue and white. B. It’s near the window.4. A. He has glasses . B. His shoes are blue.5. A. What’s his name? B. What’s for dinner?6. A. Is this your uncle? B. Is this your aunt?7. A. I can use chopsticks. B. I can use spoon.8. A. Where are the keys? B. Are they on the table?9. A. He is a nurse. B. She is a doctor.10. A. It’s near the window. B. He is tall and strong.四、Listen and choose. 听问句,选择正确的答语。
美国小学四年级超级明星数学题解答文件

Jupiter, I1. (a.7; b. 8; c. 3; d. 24)Students could practice making up their own Venn Diagrams aboutthe class by picking characteristics such as eye color and hair color, or clothing combination.In this problem, the difficult part is (d) -- some students will try to use the numbers 7, 8, and3 to get the total in the clubs.2. (30) Dots have been placed in the figure below, to show the right angles.or(36) One could argue for a total of 36. There may be four more in the larger black squares --two in each of corners at the points of overlap. There may also be two more in the V’s -- one at the top and one at the bottom of the figure where the white squares overlap. If students justify their answers, they may receive credit.3. (Monday) Students might make a list --S, M, T, W, T, F, S -- and start counting with Fridayas 7 until they get to 24. Students could think of Fridays as the 7th, 14th, and 21st and count on from the 21st.4. (a. 149; b. 599; c. 30; d. 3×n - 1) The first two parts ask the student to notice that eachsecond number is obtained by multiplying the first number by 3, then subtracting 1. Part (c) asks them to reverse this thinking, and part (d) asks them to generalize the pattern to any number n. The answer for (d) might be written in a number of different but equivalent ways.5. (60 and 12) Students may use "guess and check" by listing the pairs of addends whosesum is 72. Their guessing should get more precise as they get closer to finding the correct pair. They might get a hint about the starting point by noticing that the difference of 48 means that one of the numbers is above 50.6. (d. $3.18 ) The problem has students use their real-world number sense to get an answer.7. (75¢) Three for 25¢ means that nine would cost 75¢; 10¢ each means that nine would cost90¢.Jupiter, II1.(2 years) One-half inch per month means 1 inch every 2 months. Students can thereforecount months “by twos” until they get to 12 inches. It would take 24 months or 2 years for the hair to reach 12 inches or 1 foot in length.2.($1.50) Students at this grade level know intuitively that 50% is 1/2, and they can find 1/2 ofdollar amounts, usually without any actual computation. 1/2 of $6 is $3, and 1/2 of $3 is $1.50.3. (104, 68, 50) The unusual thing about this pattern is that it is much easier to start at the rightend and work to the left. You can see that you are adding 9 at each step.4. (45) Students will likely use a calculator to solve this problem. A few might notice that thesum of the first n counting numbers is n× (n + 1) ÷ 2. Therefore the problem becomesfinding the first or smallest n such that n× (n + 1) ÷ 2 ≥ 1000.5. (6:12 pm) This problem involves elapsed time. Students can add 1:45 and 4:27, but theymust remember that they aren't in the decimal system. They should get 5:72, and since 72 minutes is 1 hour and 12 minutes, 5:72 can be rewritten as 6:12.6. (Maria: 10; Patsy: 8; Colleen; 9; Kenyada: 11) Students might make a list, or they maymake name cards and act the problem out.7. (20 spaces ahead) Each color should come up about 1/3 of the time. However, the orangemoves and the blue moves cancel each other out. Therefore, about 1/3 of the time you would move ahead 2 spaces. 1/3 of 30 spins is 10 spins; therefore, at 2 spaces each move, you would be ahead 20 spaces.8. (She was wrong. x = 33 grams) Students can see intuitively that 1 block can be removedfrom each side of the balance scale, leaving 3 sharpeners and 1 gram to balance 100 grams.Therefore, the 3 sharpeners must weigh 99 grams, and each would weigh 33 grams. x is used simply to introduce the idea of an unknown quantity as a variable.Commentary Jupiter, III1. (The diagonal from upper left to lower right should be ringed.) Give students one star forhaving all the correct products in the chart and another for the correctly-ringed diagonal.2. (12) The ratio of 48 to 60 is the same as the ratio of 24 to 30, or 12 to 15, or 4 to 5. Hewould get the most bags possible by working with the 4 to 5 ratio, putting 9 items (four pieces of candy and 5 pieces of gum) in each bag. This would give 12 bags, since 12 × 4 is48 and 12 × 5 is 60.3. (1:00) The only difficult part of this problem comes if students try to compute 10:45 + 2:15,because they are not in the decimal system with time. The sum of 10:45 and 2:15 is 12:60, which is 1:00. Students with good number sense will probably "count on" from 10:45, using hours and then quarter hours.4. Green Black Yellow Students can be encouraged to solve such logicRed Blue Orange problems by making a chart and proceedingby the process of elimination.5. ($4) Students should have an intuitive feel for this type of problem, rather than subtracting$11.15 from $15.00 and rounding the answer. They should know that $11.15 is close to $11, and $15 - $11 is $4.6. (a. 6; b. 63) First 64 play. Then the 32 winners of those matches play. Next the 16 winnersof those matches play. Then the 8 winners of those matches play. Then the 4 winners of those matches play, and finally the last two winners play. This is 6 rounds of golf, and the winner must play in all of those. Since there are 63 losers, and each one had to play a match to lose, there are 63 matches altogether.7. There are 5 such lines of symmetry, as shown below.Jupiter, IV1. (a. 70; b. 2520) The student can multiply 14 times 5 for (a), and 14 times 180 for (b).2. (65° F) Students can add 15 to 72, then subtract 22.3. (24 ) Students may want to make a list and establish a pattern in order to solve this problem.They might name the pots shown as A, B, C, and D, and then see how many lists they can make, such as ABCD, ABDC, ACBD, ACDB, ADBC, ADCB. Those six are all the orders possible if A is on the left. There would be 6 such arrangements with B starting on the left,6 with C, and 6 with D also, for a total of 24.4. (423) Guess-check-revise is one way to solve the problem. A starting hint is that since thesum of the digits is nine, their average value is 3. Therefore all of the numbers are small numbers.5. (Saturday) Students might use a calendar. They may list S, M, T, W, T, F, S, startcounting with 7 on Tuesday, and count to 25. They may realize that Tuesdays would also be the 14th and the 21st. Then they could count on to the 25th.6. (a. 3 million; b. 36 million; c. 2 1/2 billion) The problem situation calls for estimatedanswers, rather than exact numbers which would be misleading in such a problem. Students should be allowed leeway in their estimates, since they can vary quite a bit. Hopefullystudents will use a calculator to find (a) and continue to use it in finding (b) and (c) byentering only the non-zero digits to fit into the 8-digit calculator.7. (a. 10; b. 9; c. 9 ) Students may use cubes or blocks to construct models. Students withgood spatial visualization can find the answers from the pictures.8. (car and donkeys) Students can approach this in a number of ways. Since the car matches 3elephants in the second picture, three elephants and the car can be “removed” from the last tug of war without affecting the situation. Thus we are left with 1 elephant matched against3 donkeys and asked which side would win. From the first picture, we see that an elephantpulls as much as 2 1/2 donkeys, so 3 donkeys would out pull one elephant. Therefore a car and 3 donkeys would out pull 4 elephants.Jupiter, V1. (a. Answers will vary -- 10 and 11 are the most common answers; b. Answers willvary.) Students should use a calculator to compute:25 × 60 × 16 × 365 × (answer for part a)If part a is 10, the answer is 88 million; if part a is 11, the answer is 96 million.2. (11 quarters, 4 dimes) Some students will randomly use guess-check-revise, while othersrealize that the amount of money in quarters alone should be fairly close to $3.15. Students may begin with 12 quarters (which will not work) and go backwards from there, using guess-check-revise.3. (rectangle: 28 cm; 2 triangles: 32 and 36 cm; 2 parallelograms: 32 and 36 cm) Theseare the four most likely answers, but a quadrilateral could also be built with a perimeter of 36 cm. Note: parallelograms cannot be named as rectangles.4.4 5 6 85 9 6+ 5 9 4 7_________________1 1, 1 1 15. (c. dime) A century is ten times a decade; likewise, a dollar is ten times a dime.6. (12) Students have to consider a problem that is not one usually asked. If 3 is 1/4 of somenumber, what number is it?7. (2 out of 3 chances, or 2/3, or 67%) There are three spaces left, and two of those will resultin a win for the computer. Any of the three spots are equally likely to be selected, so thechance is 2/3 of a win.8. (25, 3, 3, 9) Students familiar with a Venn Diagram should have little difficulty with thisproblem. All the X's are counted for the first answer. Only 3 X's are in the RAP ring only.Three students are in the overlap between rock and country, but not in RAP. There are 9students that are in the RAP and country circles together but not in the rock circle.1. (28 hours, 30 minutes) Students will probably count from 7:15 one morning to 7:15 thenext morning as 24 hours, and then count up by the hour to get to 11:15, finally counting a half hour to 11:45.2. (770 feet) Students may draw the diagram and sub-divide it into two parts. Also, studentscan figure out the missing lengths. 150 ft.+ 200 ft. + 185 ft. + 25 ft. + 35 ft. + 175 ft. = 770 ft. It is interesting to note that the perimeter of this figure is the same as if the figure were a 185 by 200 foot rectangle.3. (a. $33.10; b. 45; c. 1/32) The pattern for (a) is that each number increase by 20¢. For (b),each succeeding number decreases by half. Each next number in (c) is also half of thepreceding number.4. (B) Box A has a 3 out of 5 chance to win with red. Box B has a 2 out of 3 chance to winwith red. If students change ratios so that they are based on the same second number, the result will be obvious. 3 out of 5 is the same as 6 out of 10 or 9 out of 15. 2 out of 3 is the same as 4 out of 6, 6 out of 9, 8 out of 12, and 10 out of 15. But then 10 out of 15 is a better chance than 9 out of ten. Students may run a probability experiment to verify this result.5. (See figure below.) A network of paths such as the one below can be traced without lifting apencil, if it has either 0 or two odd vertices. A vertex is odd if it has an odd number of paths going in or coming out. Furthermore, if you can trace the network, you have to start at one of the odd vertices. You will end up at the other odd vertex. Therefore, the two odd vertices circled below are the only places you can start and trace the path.1. (marble bag) The chance of drawing a blue marble is 1/3; the chance of drawing aweekend day is 2/7. We must compare these fractions to see which is larger. Finding a common denominator (21) allows us to compare the fractions by comparing the numerators.1/3 is 7/21, and 2/7 is 6/21; thus 1/3 is greater than 2/7. Another way to compare thefractions is to use a calculator, change both fractions into decimals, and compare thedecimals.2. (2000 years) Many students will think you must multiply 4 and 2000, but the problemdoesn't call for any computation if you think carefully about the situation.3. (25) Students can use grid paper to make the rectangles that have 20 as a perimeter. The onewith the largest area can then be found by counting unit squares. It is the 5 by 5 square.4. (To get back fewer coins) This way Herman would get back 3 quarters. If he had given theclerk $5, he would have probably received 2 quarters, 2 dimes, and 3 pennies. Many people use a method like that mentioned to avoid carrying extra coins around in their pockets.5. (Juan is 15, Derrick is 5, Tyrone is 10) A suggested strategy is to use guess-check-revise.Guess the youngest person's age, double and triple that amount to get the other ages, and add to see if the sum is 30. If not, revise the youngest person's age appropriately. Another strategy is to think of Derrick’s age as n, Tyrone’s age as 2n, and Juan’s age as 3n. Then the total or 6n is 30, making n = 5.6. (2:38; 2:57; 3:20; 3:48) Students will have to either count backwards or subtract to get eachnew time. Subtraction involves subtracting across non base-ten numerals.7. (See watch to the right.) The time shown is 2:55, and adding4:45 to that gives a time of 7:40. Showing 7:40 will be achallenge for many students, on this watch.CommentaryJupiter, VIII1. (65) Students may use the guess-check-revise method. Some students might get the answerby putting the 36 and 94 on a number line, and deciding the point halfway between. Some students may subtract 36 from 94 (94 - 36 = 58) and add 1/2 of 58 to 36 (29 + 36 = 65).2. 4 , 6or 6 , 4 Perhaps the easiest way to solve each of these2 , 10 or 10 , 2 problems is to focus on the numbers that would give6 , 8 or 8 , 6 the indicated product, and then see which of those7 , 9 or 9 , 7 pairs of numbers would give the indicated sum.3 , 15 or 15 , 31 , 30 or 30 , 13. (10) Students may act out this problem, or they might draw a diagram with A, J, S, C, and Taround a circle. They would then connect each letter with each other letter by a line,counting the lines. AJ S4. (B) This is a two-step problem. Students will first have to find the sum of Karen's grades: 92+ 88 + 99 + 97 + 89 and get 465. Then they will divide 465 by 5 to get 93%, which is a B.Students can use a calculator for such situations.5. 5 0, 6 8 2 The problem involves deducing the two missing numbers, and one way- 4 3, 8 9 6is to work through the standard subtraction algorithm for the numbers.6, 7 8 6 Perhaps the easiest way is to add 6, 786 to 43, 8 6, using number sense to determine the result.6. (33oC) Students should realize that 12°C is too cold, and 120°F is too hot. Therefore byprocess of elimination, 33°C is the correct choice.7. ($0.92) This is a two-step problem. Students first have to decide how much Rachel spent.She bought 12 stamps at 34 cents each: 12 × $0.34= $4.08. Next, the students compute what her change would be. $4.08 from $5.00 is $0.92.8. 8 3 7 6 Students can start by looking for the T value. Three such numbers must8 3 7 6 give a sum of 8 in the ones place -- 6 is a good choice. Then knowing that1 8 3 7 6 1 is “carried” to the next place, the student can solve for N. Proceeding in3 5 1 2 8 this manner solves the problem.9. (a. 70; b. answers will vary.) Part (a) involves multiplying 10 and 7. For part (b), take thenumber that the student put in the first blank, and, using a calculator, divide the number by 7 to get the number in the second blank. The answers will most likely be 9 ÷ 7 = 1 2/7 ≈ 1.3, or 10 ÷ 7 = 1 3/7 ≈ 1.4, or 11 ÷ 7 = 1 4/7 ≈ 1.6. Be lenient in accepting reasonable answers for part (b), since some students will have the right idea but not know how to divide decimals or round their answers.Jupiter, IX1. (96) Students can count the cubes in layers. There would be 16 on each of the 6 layers, or 16 × 6 total cubes.2. ($5) Students can compute 25% of $10, 15% of $10, and 10% of $10 and add to get $5 spent. Then $10 - $5 gives $5 left to spend. Another way is to add the 3 percents (25%, 15%, and 10%) to get 50% spent. Then 50% of $10 was not spent, and 50% of $10 is $5.3. (See diagram below. 3/16) Students might show the circle cutin half, then one of the halves cut in half to get fourths, thenone of those fourths cut into four pieces, and three of themshaded (see the diagram to the right). If so, it would take 16 ofthe smaller pieces to make the whole circle, so each is 1/16. Three shaded sixteenths would be 3/16.5. (3x - $14.62 or 3 × x - $14.62 or x + x + x - $14.62 or any equivalent expression)6. (143) Students will add to find the answer.7.0 18. SB P FG CW RJJupiter, X1. (See figures below) Note that each figure is a square with the same number of dots on eachside as the figure number, plus an extra dot on top.Commentary Jupiter, XI1. (The fold line should divide the corner in two equal parts as shown below.)2. (8) The fractions are either close to 0 or close to 1. The mixed numbers can then be roundedto produce the following whole number computation: 4 + 3 – 2 + 6 – 3, which gives 8.3. (6) Calling the three students A, B, and C, there are 6 ways: ABC, ACB, BAC, BCA, CAB,and CBA4. (10, 15) The problem involves simply counting. The next two problems build on this one.5. (See the shape to the right.)6. (55) The number of circles makes the familiar pattern: 1, 3, 6, 10, 15, 21, 28, 36, 45, 55, ...To get each succeeding term, you add one more than that which you added in the previous term. To get from 1 to 3, you add 2 to 1. To get from 3 to the next number, you add 3. To get the next term, you add 4, then 5, then 6, and so on. Some students may notice that the general term is (n × (n + 1)) ÷ 2. The number of circles in the tenth term would be (10 × 11) ÷ 2 = 55.7. (a. 2, 3, 5, 7, 11, 13, 17, 19, 23, 29, 31, 37, 41, 43, 47; b. 15 out of 49 or 15/49 or anyequivalent; c. the probability of selecting a number that is not prime) The probability of choosing a number that is not prime is 34/49.8. (a. < ; b. < ; c. = )9. (From the left, the 1st, 3rd, and 5th figures should be circled.) The first figure has twosquare faces at its ends. The third figure also has two square bases, although they are“tilted”. The fifth figure has only one square face, the one on which it rests.10. (25) The problem will become, in later years, an algebraic situation of the form 60 = 2x +10. In this case, the two tubes of glue must weigh 50 grams since 50 + 10 = 60. If two tubesof glue weigh 50, then each weighs 25 grams.Jupiter, XII1. (16) Students may need help recognizing that the snail is climbing and then falling.Students may draw pictures or use a number line. Some students will think the answer is 20 days because the snail is making progress at the rate of 1 foot per day. However, thisdiscounts the fact that once the snail reaches the top on the 16th day, it won't fall back four feet that night.2. (3/4, 75%) The circle is divided equally into four regions, so the chance of landing on eachof those regions is 1/4. The chance of landing on any of the three of them is then 3/4.3. ($12.50) Some students will find half of $25 as $12.50, and then subtract that amount from$25 and get $12.50 again. Others will simply say that if the item is on sale for 1/2 off, the price you pay is also 1/2 of the price showing.4. (81) Students may want to use a calendar or set up a chart in order to solve this problem.There would be 20 days left in October, 30 in November, and 31 in December.5. (180) Students may use the guess-check-revise approach. The 2nd clue says that the numberis in the hundreds. It is possible then to write down the multiples of 12 that are in thehundreds, checking to see which are also multiples of 9 in which the units digit is less than the tens digit.6. (a. about 143 mi.; b. about .4 mi.; c. about 2000 ft.; d. about 1300 steps.) Part (a)involves dividing 10,000 by 70; part (b) involves dividing the answer for (a) by 365; part(c) involves multiplying (b)'s answer by 5280, and part (d) is found by dividing (c)’s answerby 1.5 (18 inches = 1.5 feet). If a student uses a calculator and does not round off during the process, he may get closer to 1400steps for answer d..7. (50) Students should divide 800 by 16, which is the number of hours the person is awake andburning calories by fidgeting.8. ($15) $10 for 100 pretzels means he makes pretzels for 10¢ each. If he sells them for 25¢each, he makes 15¢ per pretzel. Therefore 100 pretzels would bring a profit of 100 × 15¢ or $15.Jupiter, XIII1. (8 chances out of 100, 8/100, 8%, 8:100, or a reduced form of these answers, such as4/50, 2/25, and so forth.) Students can obtain such an answer by making a chart of the possibilities. The chart below shows the eight possibilities of success, out of the 100possibilities for the two cards.4. (35 ÷ 7 = 5) The problem is a simple partitioning interpretation of division.5. (a. 5 feet by 4 feet; b. 15 kilograms) Both problems involve number sense. Students caneliminate all the unreasonable answers through their familiarity with the size and weight of a bicycle.6. (a. 9; b. 104; c. Lamps, Television, and Walkman) Students can count the types ofappliances directly from the chart for (a). For (b), the total number of symbols in the chart is 26, and each stands for 4 appliances from the key, so the total is 104. Lamps are most popular with 5 symbols, followed by Television and Walkman with 4 each.7. (189, 180, 117) The pattern involves subtracting 9 each time you move one term to the right.CommentaryJupiter, XIV1. (Charles was correct.) The window “edges out” on the right hand side about half a square unit, and there are six of those square units on that side of the window. Therefore the area is 24 square units, plus the six extra half-squares, or 27 square units altogether. The picture is a little short of taking up the sixth square unit on the right-hand end. Measurement shows that it's about 1/5 of a square unit short on that side, and there are four such squares on that end. Therefore its area is four 1/5's short of being 24 square units. As this problem shows, Charles’ desire to be an architect means that he will probably be quite exact in his measurements.2. (32) The 8 pigs accounted for 1/4 of the total number of animals, since that is the amount left when 1/2 and 1/4 are combined and subtracted from 1. Then the total number of animals is 4 × 8 or 32.3. (a. 15; b. 30; c. 50) For (a), 59 - 32 = 27; 27 ÷ 9 = 3; 5 × 3 = 15. For (b), 86 - 32 = 54; 54 ÷ 9 = 6; 6 × 5 = 30. For (c), 122 - 32 = 90; 90 ÷ 9 = 10; 10 × 5 = 50.4. (140) Students might get this by working backwards. To end up with 60 after multiplying by 5, you must have had 12 at the previous step. To have 12 after dividing by 9, you must have had 108 in the previous step. To have 108 after subtracting 32, you must have had 140 to begin.5. ($4.25) The 17 quarters would be $4.25.6. (a. 3/12 or 1/4 or 0.25 or 25%; b. 9/12 or 0.75 or 75%) In (a), there are 3 months being considered out of twelve, so the chance is 3/12 you will get one of those. In (b), the chances are 9/12 since 9 months out of 12 are being considered.7. (c. 1000) Students can partition the figure into smaller equal-sized pieces, count the dots in one of those, and get an estimate by multiplying. The figure below has about 120 dots in the section that has been counted, and there are 8 such sections, resulting in about 120 × 8 or 960 pots.Commentary Jupiter, XV1. (a. 32; b. $160) There are 10 faces on the side showing, giving 20 altogether on the twosides. There are 8 faces the make up the “steps,” and 4 more on the end. The estimate of the cost can be made by rounding $4.99 to $5 and multiplying 32 × $5.2. (Any picture of a trapezoid is acceptable.)1. (Any model with one shaded cell is correct.) Students will likelythink of dividing the rectangle into thirds either horizontally orvertically, and then one of those thirds into thirds going in theother direction.1. (a. See below; b. rectangle; c. 12)Jupiter, XVIII1. (4 out of 52, 2 out of 26 or 1 out of 13, which could also be written as a fraction (4/52,e.g.), a decimal (approximately 0.08), or a percent (8%)). In each suit, there are two cardsthat are multiples of 5, the 5 and the 10. There are two red suits, diamonds and hearts.Therefore there are four such cards out of 52 in the deck.2. (3/8) The two pizzas shown have not been divided into eighths, as pizzas normally are. Thestudent can divide each of them this way and see that Dad ate 6 pieces, Jenny ate 1 piece, Danny ate 2 pieces, and Mom ate 4 pieces. Therefore 13 pieces were eaten, leaving 3 pieces.Three pieces is 3/8 of a pizza.3. (15) Students should solve this problem intuitively, not by trying to use the equation. Theequation is there simply for them to associate an equation with a real-life situation. They can guess-check-revise to find the weight of an apple, or they can deduce the answer logically as they will be called on later to solve such equations. If three apples and 5 grams weigh 50 grams, then 3 apples by themselves must weigh 45 grams. Therefore each apple weighs 45 ÷3 or 15 grams.4. (26 students) There is extra information in this problem -- 16 classes. The problem is solvedby dividing 104 students by 4.5. (even) Students might want to test this out, by opening a book to several different places andmultiplying the numbers on the facing pages with a calculator.6. (a. $9.60; b. 2 hours and 40 minutes) Students can first multiply each color string by 8, addthose products to get 96, and then multiply by 10 cents. Or, they might add all the colors together for one bracelet and get 12, multiply that amount by 8, and then multiply by 10 cents. For the second question, students can multiply 20 minutes by 8 to get 160 minutes, converting that to 2 hours and 40 minutes.7. (6 meters) Students might estimate this amount visually -- the height of a door is about 2meters, and the width is not quite 1 meter. Therefore, the distance around the outside would be about 1 + 2 + 1 + 2 or 6 meters. Some students might actually measure a door and find approximately the same dimensions. Most interior doors in houses are about 5.5 meters around the outside, which is closer to 6 meters than any of the other answers.8. (11 hours 30 minutes) The trip from Tallahassee takes the longest. Students will probably"count up" from the departing time to the arrival time, getting 6 ½ hours, 11 ½ hours, 5hours, and 8 hours, respectively. On the Tallahassee trip, some might get the time byrealizing that a 12-hour trip would go from 7:30 AM to 7:30 PM, and this would be ½ hour shorter than that, giving 11 ½ hours for the trip.Jupiter, XIX1. (Four possibilities are shown below.) Students can show “fourths” in a number of ways. The square must bedivided into 4 parts, and the parts must have the same area. However, the parts to not have to be the same shape.2. (45) Students might start by listing the numbers greater than 39 but less than 46: {40, 41,42, 43, 44, and 45}. The only number of the list that you count when you count by threes and fives is 45. “It roared an odd number of times.” is not necessary as a clue.3. (771) Students might use a calculator to divide 3855 by 5, getting 771. Students might bechallenged to find the approximate number of pushups per minute -- 13 -- and to thenapproximate the rate of his doing push ups (about 1 every 5 seconds).4. (the perimeter of the square is 4 units; that of the rectangle is 8 units) Some studentsmight misinterpret the problem and try to use all four figures to make the square, and then all four again to make the rectangle. They will find they can't make such a square.5.(The graph should beapproximately equivalent to theone shown.) The change from1988 to 1994 is from 15% to 11%.That same change from 1994 to2000 would result in about 7% in2000.6. (Figure B) Students might find the drawing by tracing over figure A, actually turning thetraced figure three 90° turns, and matching it up with one of the given drawings.7. (40 students) This problem can be solved by working backwards and then adding. OnFriday 12 students got the silly willies; therefore on Thursday, 10 students did; Wednesday, 8 students; Tuesday, 6; and Monday, 4. 12 + 10 + 8 + 6 + 4 = 40.8. (One solution is shown.) Students might start byputting 4 in the center and the two numbers that“surround” it, 3 and 5, on the ends.。
2017年美国“数学大联盟杯赛”初赛四年级试卷

2016-2017年度美国“数学大联盟杯赛”(中国赛区)初赛(四年级)(初赛时间:2016年11月20日,考试时间90分钟,总分200分)学生诚信协议:考试期间,我确定没有就所涉及的问题或结论,与任何人、用任何方式交流或讨论,我确定以下的答案均为我个人独立完成的成果,否则愿接受本次成绩无效的处罚。
如果您同意遵守以上协议请在装订线内签名选择题:每小题5分,答对加5分,答错不扣分,共200分,答案请填涂在答题卡上。
1.Which of the following is the greatest?A) 2.017 B) 20.17 C) 201.7 D) 20172.The sum of the degree-measures of the interior angles of a triangle isA) 180 B) 360 C) 540 D) 7203.100 + 200 + 300 + 400 + 500 = 300 ×?A) 3 B) 4 C) 5 D) 64.100 ÷ 4 = 200 ÷?A) 2 B) 4 C) 8 D) 165.In tonight’s talent show, Jack sang 3 songs. The number of songs that Jill sang is 8 lessthan 4 times the number of songs Jack sang. How many songs did Jill sing?A) 3 B) 4 C) 6 D) 76.Doubling a certain number is the same as adding that number and 36. What is thatnumber?A) 18 B) 36 C) 54 D) 727.The side-lengths of three square farms are 1 km, 2 km, and 3 km respectively. The sum ofthe areas of these three farms is ? km2.A) 6 B) 12 C) 13 D) 148.What is the greatest common factor of 2017 and 20 × 17?A) 1 B) 2 C) 3 D) 59.If a computer can download 2% of the files in 2 seconds, how many seconds does it taketo download all the files?A) 100 B) 200 C) 300 D) 40010.In yesterday’s giant-pie eating, all pies were the same size. Al ate 3/4 of a giant pie, Barbate 4/5 of a giant pie, Cy ate 5/6 of a giant pie, and Di ate 6/7 of a giant pie. Who ate the largest portion?A) Al B) Barb C) Cy D) Di 11.The product of two consecutive positive integers is alwaysA) odd B) evenC) prime D) composite12.In a 5-term sequence, the first term is 2. The value of each term after the first is twice thatof its previous term. What is the product of the 5 terms?A) 24B) 210C) 215D) 24513.Ace, Bo, and Cat performed in a talent show. Bo’s total score was twice that of Ace, andCat’s total score was three times that of Bo. If the sum of all three total scores was 900, what was Cat’s total score?A) 100 B) 200C) 300 D) 60014.The length of each side of triangle T is an integer. If two sides of T have lengths of 2016and 2017, what is the least possible value for the length of the third side?A) 1 B) 2 C) 4032 D) 403315.If the sum of three consecutive whole numbers is 2016, what is the sum of the next threeconsecutive whole numbers?A) 2032 B) 2025 C) 2020 D) 201716.If the sum of a prime and a composite is 2017, what is the least possible value for theproduct of the two numbers?A) 3000 B) 4030 C) 6042 D) 912017.What is the smallest whole number that leaves a remainder of 2 when divided by each of 3,4, 5, and 6?A) 58 B) 60 C) 62 D) 6418.What is the highest power of 2 that divides 1 × 2 × 3 × 4 × 5 × 6 × 7 × 8 × 9?A) 25B) 26C) 27D) 2819.The product of the digits of 23 is 6. How many different whole numbers between 100 and999 have a product of 6?A) 12 B) 9 C) 6 D) 320.What is the value of 1% of 10% of 100%?A) 0.001 B) 0.01 C) 0.1 D) 121.In a box that contains only balls that are red, yellow, or green, 10% of the balls are red, 1/5of the balls are yellow, and 49 balls are green. How many balls are in the box?A) 70 B) 80 C) 90 D) 10022.Of the following, which has the greatest number of positive whole number divisors?A) 24 B) 26 C) 51 D) 2017第1页,共4页第2页,共4页23.If you subtract the sum of the digits of a whole number greater than 9 from the numberitself, the result must be divisible byA) 5 B) 6 C) 9 D) 1224.I bought a painting for $40, sold it for $50, rebought it for $60, and resold it for $70. Mytotal profit on the 4 transactions wasA) $10 B) $20 C) $30 D) $4025.What is the minimum number of whole number divisors of the product of two differentcomposite numbers?A) 5 B) 6 C) 8 D) 926.For each whole number from 1000 to 9999, inclusive, I write the product of its digits.How many of the products I write are even?A) 625 B) 3125 C) 5775 D) 837527.Lisa baked some cookies and cakes. Baking one cookie requires 4 cups of sugar and 3cups of flour, and baking one cake requires 7 cups of sugar and 5 cups of flour. At the end she used 83 cups of sugar and 61 cups of flour. How many cookies did she bake?A) 11 B) 12 C) 13 D) 1428.Working by oneself, Al can build a bridge in 3 years, Barb can build a bridge in 4 years,and Cy can build a bridge in 5 years. Working together, how long, in years, does it take them to build the bridge?A) 12B)6047C)6053D) 129.Jack is a gifted athlete who has trained hardfor the Olympic marathon. In the lasthundred yards he finds the inner strength toincrease his pace and overtakes the runner inthe second place.But then, with the finishing line just feetaway, he is overt aken by two other runners…What medal will Jack receive?A) Gold B) SilverC) Bronze D) None30.If we juxtapose three congruent squares, we get a rectangle with perimeter 64. What is thearea of one of the squares?A) 36 B) 49 C) 64 D) 8131.In a four-digit perfect square, the digits in the hundreds and thousands places are equal,and the digits in the tens and ones places are equal. What is this number?A) 6644 B) 7744 C) 8844 D) 9944 32.For how many of the integers from 100 to 999 inclusive is the product of its digits equal to9?A) 6 B) 7 C) 8 D) 933.What is the smallest positive integer x for which (x + 8) is divisible by 5 and (x + 17) isdivisible by 7?A) 30 B) 31 C) 32 D) 3334.Tom’s new tower was completed. The total value ofthe project, the sum of the cost of the construction andthe cost of the land, was one million dollars. The cost of theconstruction was $900,000 more than the cost of theland. So what did Tom pay for the land?A) $25,000 B) $50,000C) $75,000 D) $90,00035.五个连续正整数的和总是可以被下面哪个数整除?A) 2 B) 3 C) 5 D) 736.从1开始,鲍勃一共喊了2017个数,从第一个数之后的每个数都比前一个数大4。
2017年美国“数学大联盟杯赛”初赛四年级试卷

2016-2017年度美国“数学大联盟杯赛”(中国赛区)初赛(四年级)(初赛时间:2016年11月20日,考试时间90分钟,总分200分)学生诚信协议:考试期间,我确定没有就所涉及的问题或结论,与任何人、用任何方式交流或讨论,我确定以下的答案均为我个人独立完成的成果,否则愿接受本次成绩无效的处罚。
如果您同意遵守以上协议请在装订线内签名选择题:每小题5分,答对加5分,答错不扣分,共200分,答案请填涂在答题卡上。
1.Which of the following is the greatest?A) 2.017 B) 20.17 C) 201.7 D) 20172.The sum of the degree-measures of the interior angles of a triangle isA) 180 B) 360 C) 540 D) 7203.100 + 200 + 300 + 400 + 500 = 300 ×?A) 3 B) 4 C) 5 D) 64.100 ÷ 4 = 200 ÷?A) 2 B) 4 C) 8 D) 165.In tonight’s talent show, Jack sang 3 songs. The number of songs that Jill sang is 8 lessthan 4 times the number of songs Jack sang. How many songs did Jill sing?A) 3 B) 4 C) 6 D) 76.Doubling a certain number is the same as adding that number and 36. What is thatnumber?A) 18 B) 36 C) 54 D) 727.The side-lengths of three square farms are 1 km, 2 km, and 3 km respectively. The sum ofthe areas of these three farms is ? km2.A) 6 B) 12 C) 13 D) 148.What is the greatest common factor of 2017 and 20 × 17?A) 1 B) 2 C) 3 D) 59.If a computer can download 2% of the files in 2 seconds, how many seconds does it taketo download all the files?A) 100 B) 200 C) 300 D) 40010.In yesterday’s giant-pie eating, all pies were the same size. Al ate 3/4 of a giant pie, Barbate 4/5 of a giant pie, Cy ate 5/6 of a giant pie, and Di ate 6/7 of a giant pie. Who ate the largest portion?A) Al B) Barb C) Cy D) Di 11.The product of two consecutive positive integers is alwaysA) odd B) evenC) prime D) composite12.In a 5-term sequence, the first term is 2. The value of each term after the first is twice thatof its previous term. What is the product of the 5 terms?A) 24B) 210C) 215D) 24513.Ace, Bo, and Cat performed in a talent show. Bo’s total score was twice that of Ace, andCat’s total score was three times that of Bo. If the sum of all three total scores was 900, what was Cat’s total score?A) 100 B) 200C) 300 D) 60014.The length of each side of triangle T is an integer. If two sides of T have lengths of 2016and 2017, what is the least possible value for the length of the third side?A) 1 B) 2 C) 4032 D) 403315.If the sum of three consecutive whole numbers is 2016, what is the sum of the next threeconsecutive whole numbers?A) 2032 B) 2025 C) 2020 D) 201716.If the sum of a prime and a composite is 2017, what is the least possible value for theproduct of the two numbers?A) 3000 B) 4030 C) 6042 D) 912017.What is the smallest whole number that leaves a remainder of 2 when divided by each of 3,4, 5, and 6?A) 58 B) 60 C) 62 D) 6418.What is the highest power of 2 that divides 1 × 2 × 3 × 4 × 5 × 6 × 7 × 8 × 9?A) 25B) 26C) 27D) 2819.The product of the digits of 23 is 6. How many different whole numbers between 100 and999 have a product of 6?A) 12 B) 9 C) 6 D) 320.What is the value of 1% of 10% of 100%?A) 0.001 B) 0.01 C) 0.1 D) 121.In a box that contains only balls that are red, yellow, or green, 10% of the balls are red, 1/5of the balls are yellow, and 49 balls are green. How many balls are in the box?A) 70 B) 80 C) 90 D) 10022.Of the following, which has the greatest number of positive whole number divisors?A) 24 B) 26 C) 51 D) 2017第1页,共4页第2页,共4页23.If you subtract the sum of the digits of a whole number greater than 9 from the numberitself, the result must be divisible byA) 5 B) 6 C) 9 D) 1224.I bought a painting for $40, sold it for $50, rebought it for $60, and resold it for $70. Mytotal profit on the 4 transactions wasA) $10 B) $20 C) $30 D) $4025.What is the minimum number of whole number divisors of the product of two differentcomposite numbers?A) 5 B) 6 C) 8 D) 926.For each whole number from 1000 to 9999, inclusive, I write the product of its digits.How many of the products I write are even?A) 625 B) 3125 C) 5775 D) 837527.Lisa baked some cookies and cakes. Baking one cookie requires 4 cups of sugar and 3cups of flour, and baking one cake requires 7 cups of sugar and 5 cups of flour. At the end she used 83 cups of sugar and 61 cups of flour. How many cookies did she bake?A) 11 B) 12 C) 13 D) 1428.Working by oneself, Al can build a bridge in 3 years, Barb can build a bridge in 4 years,and Cy can build a bridge in 5 years. Working together, how long, in years, does it take them to build the bridge?A) 12B)6047C)6053D) 129.Jack is a gifted athlete who has trained hardfor the Olympic marathon. In the lasthundred yards he finds the inner strength toincrease his pace and overtakes the runner inthe second place.But then, with the finishing line just feetaway, he is overt aken by two other runners…What medal will Jack receive?A) Gold B) SilverC) Bronze D) None30.If we juxtapose three congruent squares, we get a rectangle with perimeter 64. What is thearea of one of the squares?A) 36 B) 49 C) 64 D) 8131.In a four-digit perfect square, the digits in the hundreds and thousands places are equal,and the digits in the tens and ones places are equal. What is this number?A) 6644 B) 7744 C) 8844 D) 9944 32.For how many of the integers from 100 to 999 inclusive is the product of its digits equal to9?A) 6 B) 7 C) 8 D) 933.What is the smallest positive integer x for which (x + 8) is divisible by 5 and (x + 17) isdivisible by 7?A) 30 B) 31 C) 32 D) 3334.Tom’s new tower was completed. The total value ofthe project, the sum of the cost of the construction andthe cost of the land, was one million dollars. The cost of theconstruction was $900,000 more than the cost of theland. So what did Tom pay for the land?A) $25,000 B) $50,000C) $75,000 D) $90,00035.五个连续正整数的和总是可以被下面哪个数整除?A) 2 B) 3 C) 5 D) 736.从1开始,鲍勃一共喊了2017个数,从第一个数之后的每个数都比前一个数大4。
2014年美国“数学大联盟杯赛”(中国赛区)初赛三、四年级详解

2013-2014年度美国“数学大联盟杯赛”(中国赛区)初赛答案(三、四年级)一、选择题1. A.Since 0 is a factor, 2 × 0 × 1 × 4 = 0.A) 0B) 7C) 8D) 20142. B.If 2 years ago I was 3 years old, I am now 3 + 2 = 5.A) 4B) 5C) 6D) 233. D.8 + (60 ÷ 4) = 8 + (15) = 23.A) 15B) 17C) 22D) 234. B.(1 + 7) + (2 + 6) + (3 + 5) = 3 × 8 = 24 = 4 + 20.A) 4B) 20C) 24D) 285. C.The prime numbers less than 10 are 2, 3, 5, and 7.A) 2B) 3C) 4D) 56. B.Caleb the dog dreams he has 12 dozen bones. Since 12 dozen = 12 × 12 = 144, there are 144 ÷ 2 = 72 pairs. Caleb will have to dig 72 holes.A) 24B) 72C) 144D) 2887. C.From 9:45 PM to 10:45 PM is 60 mins. From 10:45 PM to 11 PM is 15 mins. From 11 PM to 11:10 PM is 10 mins. That’s (60 + 15 + 10) mins.A) 65B) 75C) 85D) 958. D.From January 1st to January 31st, there are 16 odd-numbered dates. From February 1st to February 21st, there are 11 odd-numbered dates. That’s 27 × $2 = $54.A) $48B) $50C) $52D) $549. C.9 × 9 + 9 × 8 + 9 × 7 + 9 × 6 = 9 × (9 + 8 + 7 + 6).A) 20B) 24C) 30D) 3610.D.Manny weighs three times as much as Murray. Manny also weighs 8000 kg more than Murray, so 8000 kg is twice Murray’s weight. Thus Murray weighs 4000 kg and Manny weighs 12 000 kg.D) 12 00011.B.I have twice as many shirts as hats, and four times as many hats as scarves. If I have 24 shirts, I have 24÷ 2 = 12 hats and 12 ÷ 4 = 3 scarves.A) 2B) 3C) 6D) 1212.C.My coins have a total value of $6.20. If I have 1 of each coin, I have (1 + 5 + 10 + 25)¢ = 41¢. Subtract 41¢ from $6.20 repeatedly until there is 5¢ left. After 15 subtractions, there is 5¢ left. I have 15 + 5 or20 pennies.A) 10B) 15C) 20D) 2513.D.The diagrams demonstrate choices A, B, and C.A) 14 kmB) 10 kmC) 8 kmD) 1 km14.C.(2014 −1014) + (3014 − 2014) = 1000 + 1000 = 2000.A) 0B) 1000C) 2000D) 201415.A.10 + (9 ×8) − (7 × 6) = 10 + 72 − 42 = 40.A) 40B) 110The prime factorization of 72 is 2 × 2 × 2 × 3 × 3. The largest prime is 3.A) 3B) 7C) 36D) 7217.D.6 × 4 = 24 = 96 ÷ 4.A) 6B) 12C) 24D) 9618.C.If 6 cans contain 96 teaspoons of sugar, 1 can contains 96 ÷ 6 = 16 teaspoons of sugar. Thus 15 cans contain 16 × 15 = 240 teaspoons of sugar.A) 192B) 208C) 240D) 28819.C.The largest possible such sum is 98 + 99 = 197.A) 21B) 99C) 197D) 19820.B.Ann sent Wilson hearts with odd numbers with odd tens digits. The number on each heart he received must be two digits with both digits odd. There are 5 possible tens digits and 5 possible ones digits.That’s a total of 5 × 5 = 25 hearts.A) 23B) 25C) 30D) 4521.B.Since Rich ate his favorite sandwich 8 days ago, today is the 9th day of the month. Since the shortest month has 28 days, it is at least 28 − 9 = 19 days until the last day of the month. He must wait 1 more day.A) 1922.D.The factors of 49 are 1, 7, and 49. Since 49 has 3 factors, it has a prime number of factors.A) 6B) 12C) 36D) 4923.D.Dividing a certain two-digit number by 10 leaves a remainder of 9, so it is 19, 29, 39, 49, 59, 69, 79, 89, or 99. The only number listed with remainder 8 when divided by 9 is 89, so the number is 89 and 8 + 9 = 17.A) 7B) 9C) 13D) 1724.A.The whole numbers less than 1000 that can be written as such a product are 0 × 1 × 2, 1 × 2 × 3, 2 × 3 ×4, 3 × 4 × 5, 4 × 5 × 6, 5 × 6 × 7, 6 × 7 × 8, 7 × 8 × 9, 8 × 9 × 10, and 9 × 10 ×11. In all, that’s 10.A) 10B) 11C) 15D) 2125.B.The only such numbers are 5432, 5431, 5430, 5421, 5420, 5410, 5321, 5320, 5310, and 5210. In all, there are 10 such numbers.A) 3B) 10C) 69D) 12026.C.2014 × 400 = 805 600; the hundreds digit is 6.A) 0B) 5C) 6D) 827.B.Greta was 110 cm tall 2 years ago, when she was 10 cm taller than her brother. Her brother was 100 cmB) 130C) 140D) 15028.B.The number 789 678 567 456 is added to the number 987 876 765 654. Since we carry a 1 when adding the left-most digits, the sum has 12 + 1 digits.A) 12B) 13C) 24D) 2529.D.We must find which number among the choices is two more than a multiple of 5. Divide each choice by5 (or recogniz e that any number that ends in “2”or “7” is 2 more than a multiple of 5).A) 4351B) 5215C) 5616D) 646230.C.Of every 11 people, there are 2 adults and 9 children. Since 99 ÷ 11 = 9, there are 9 groups of 11 people.Of these, 9 × 2 = 18 are adults.A) 9B) 11C) 18D) 22二、填空题31.5.32.22.33.4.34.1.35.617.36.21.37.499.38.765.39.69.40.10.。
