南航矩阵论期中考试参考答案.doc
南京航空航天大学矩阵论07-08A试卷及答案.doc

南京航空航天大学研究生考试试卷r 1 1 -2'一、(20 分)设矩阵4= —2 —2 3 ,<-1 -1 1 >(1)求A的特征多项式和A的全部特征值;(2)求A的行列式因子、不变因子和初等因子;(3)求A的最小多项式,并计算A6+3A —2/;(4)写出A的Jordan标准型二、(20分)设Z?2"2是实数域上的全体2x2实矩阵构成的线性空间(按通常矩阵的加法和数与矩阵的乘法)。
(1)求尺2"2的维数,并写山其一组基;(2)设W是全体2x2实对称矩阵的集合,证明:W是/?2x2的子空间,并写出W的维数和一组基;(3)在W中定义闪积G4,B) = Zr(&4),其中人BeW,求出W的一组标准正交基;(4)给出尺〜2上的线性变换7\ T(A) = A+A r, VA G R^2写出线性变换T在(1)中所取基下的矩阵,并求7的核/^r(r)和值域/?(r)。
三、(20分)证明: 是C'w 上的矩阵范数并说明具有相容性(1)求矩阵A 的07?分解;(3)用广义逆判断方程组Av = 6是否相界?若相界,求其通解;若不相容,求其极小最小二乘解 五、(20分)证明:A,, >0, Ar-AgAjAuSO 。
(I-1 1、’2' 1 11,向量/?=11 、0 0b<2>四、(20分)已知矩阵4 =,5 3 2>12、 1)设矩阵汲二3 2 t ,B = 1 1 0.5/t 2; /<2 0.5/ 1 ,,其中f 为实数问当Z 满足什么条件时,A 〉B 成立?Ai A 2 A2 ^22>0,其巾 A u eCkxkau(1)设乂 =2 13 -1 21 ,喇"K, ML, h(2)设4 =(〜)e C ,IX \ 令p=n • max 騸⑶证明:-||<<||<<(2)设 n 阶 Hermite 矩阵 A =(3)己知Hermite 矩陈A =(七)€ (?■ , a ij〉工a ij (= l,2,".,n ),证明:A 正定一、(20 分)(2) VA ,fielV ,V 々e/?,贝ij v (A +B)7= A 7+ B 7= A+B , /. A + B G W ;v (M)7 =kA T = kA ; /.MeW 。
南航矩阵论期中考试试题2013可修改全文

Mid-term Exam of Matrix TheoryPart IRequired Questions (4×15′=60′).Q1.1)Let A = 2−344−686−78,1.Calculate the characteristics polynomial and eigenvalues of A .2.Find the determinant divisors,invariant divisors and elementary divisors of A .2)Given B =(17−645−16)and C =(14−603−13),please determine if B and C are similar or not.And prove your conclusion.Q2.Denote V ={(a 11a 12a 21a 22)∈R 2×2|a 11=a 22}.For any X ∈V ,let T (X )=P X +XP ,where P =(0110).1.Find a basis of V and show the dimension.2.Arbitrarily given A =(a 11a 12a 21a 22)and B =(b 11b 12b 21b 22)in V ,define (A,B )=a 11b 11+2a 12b 12+a 21b 21.Please show that (A,B )is an inner product on V .3.Given an orthonormal basis of V under the inner product of 2.4.Prove that T is a linear transformation on V ,and show the matrix representation of T with respect to the basis given in item 1.Q3.Consider the inner product space C [−1,1]with inner product defined as(f,g )=∫1−1f (x )g (x )dx,∀f (x ),g (x )∈C [−1,1].11.Show that1and3x2−1are orthogonal.2.Determine∥1∥and∥3x2−1∥.3.Let S=L{1,3x2−1}be a subspace of R[x]3,find the optimal approximation of xover S.Q8.Denote R[x]3to be the vector space of zero and polynomials with degree less than3.1.Determine the dimension of R[x]3and give a basis of R[x]3.2.Define the linear transformation D on R[x]3,D(f(x))=f′(x),∀f(x)∈R[x]3.Please give the matrix representation of D with respect to the basis given in the above item.Show R(D)and ker(D).3.Prove that D is not diagonalizable.4.Define the inner product on R[x]3,(f,g)=∫1−1f(x)g(x)dx,∀f(x),g(x)∈R[x]3,please Gram-Schmidt orthogonalize the basis given in item1.Part IIPreferential Questions(2×20′=40′).Q5.For any x∈R n,several definitions are given as follows,∥x∥0=∑x i=0|x i|0,∥x∥p=(m∑i=1|x i|p)1p(0<p<1),∥x∥1=m∑i=1|x i|.(1)1.Please determine if∥x∥0,∥x∥p and∥x∥1are valid vector norms or not.And try todefense your decision.2.Especially when n=2,plot the curves of∥x∥0=1,∥x∥p=1and∥x∥1=1respectively.2Q6.Given A∈R n×n,summarize the necessary and sufficient conditions of A to be di-agonalizable,and prove at least one of them.Determine if the matrix A given in Q1is diagonalizable or not.If yes,please explain why,if not,please give the Jordan canonical form of A.Q7.Given A∈R n×n,denote W={X∈R n×n|AX=XA}.1.Show that W is a subspace of R n×n.2.DenoteD=λ10 00λ2 0............00···λn,whereλ1,λ2,···,λn are different from each other.If A=D,please determine the dimension of W.3.If A is similar to D defined as in item2,please prove that any X∈W is diagonalizable.4.Given some X∈W,if X and A are both diagonalizable,then there exists a nonsingularmatrix P∈R n×n such that P−1XP and P−1AP are diagonal simultaneously.3。
南航双语矩阵论matrixtheory第五章部分习题参考答案

第五章部分习题参考答案#2. Find determinant divisors and elementary divisors of each of the following matrices.(a) 1000100015432λλλλ-⎛⎫ ⎪-⎪ ⎪- ⎪+⎝⎭ (b)001010100000λλλλ⎛⎫⎪ ⎪ ⎪ ⎪⎝⎭Solution(a ) 100010()0015432A λλλλλ-⎛⎫ ⎪- ⎪= ⎪- ⎪+⎝⎭det (())A λ4322345λλλλ=++++100det 10101λλ-⎛⎫⎪-=- ⎪ ⎪-⎝⎭. Hence, the determinant divisors are 123()()()1D D D λλλ===,4324()2345D λλλλλ=++++. Invariant divisor are 123()()()1d d d λλλ===,4324()2345d λλλλλ=++++Unfortunately, it is not easy to factorize 4324()2345d λλλλλ=++++ by hand. With the help of Maple or Matlab, we can see that ()A λ has four distinct linear elementary divisors. (b) 44()D λλ=, 123()()()1D D D λλλ===. There is a unique elementary divisor 4λ #3. Let11a a A a ⎛⎫ ⎪ ⎪= ⎪ ⎪⎝⎭ , a a B a εε⎛⎫ ⎪⎪= ⎪ ⎪⎝⎭ be n n ⨯ matrices, where 0ε≠. Show that A and B are similar.Proof The Smith normal forms of both I A λ- and I B λ-are11()n a λ⎛⎫ ⎪⎪ ⎪ ⎪-⎝⎭. A and B have the same set of elementary divisors. Hence they are similar to each other. #4. Let11a a A a ⎛⎫ ⎪ ⎪= ⎪ ⎪⎝⎭ , 11a a B a ε⎛⎫ ⎪⎪= ⎪ ⎪⎝⎭be n n ⨯ matrices, where 0ε≠. Show that A and B are NOT similar. ProofThe determinant of I A λ- is ()n a λ- . The determinant of I B λ- is ()n a λε--. A and B have distinct characteristic polynomials. Hence, they are not similar.#11. How many possible Jordan forms are there for a 66⨯ complex matrix with characteristic polynomial 42(2)(1)x x +-?Solution The possibilities for the sets of elementary divisors are { 42(2),(1)x x +-}, {4(2),(1),(1)x x x +--}{32(2),(2),(1)x x x ++-}, {3(2),(2),(1),(1)x x x x ++--} {222(2),(2),(1)x x x ++-}, {22(2),(2),(1),(1)x x x x ++--},{22(2),(2),(2),(1)x x x x +++-}, {2(2),(2),(2),(1),(1)x x x x x +++--}{2(2),(2),(2),(2),(1)x x x x x ++++-}, {(2),(2),(2),(2),(1),(1)x x x x x x ++++--}. For each set of elementary divisors, there is a Jordan canonical form up to similarity. There are 10 Jordan canonical forms up to similarity.#12. Classify up to similarity all 33⨯ complex matrices A such that 3A I =. Solution An annihilating polynomial of A is 321(1)()()x x x x ωω-=---, where ω A is diagonalizable.The possibilities for the minimal polynomial of A are1x -, x ω-, 2x ω-;(1x -)(x ω-), (x ω-)(2x ω-), (1x -)(2x ω-);2(1)()()x x x ωω---Up to similarity, all 33⨯ complex matrices A are100010001⎛⎫ ⎪ ⎪ ⎪⎝⎭, 000000ωωω⎛⎫⎪ ⎪ ⎪⎝⎭, 222000000ωωω⎛⎫ ⎪ ⎪ ⎪⎝⎭; 10001000ω⎛⎫⎪ ⎪ ⎪⎝⎭, 1000000ωω⎛⎫ ⎪ ⎪ ⎪⎝⎭; 22000000ωωω⎛⎫ ⎪⎪ ⎪⎝⎭, 2000000ωωω⎛⎫ ⎪ ⎪ ⎪⎝⎭;221000000ωω⎛⎫⎪ ⎪ ⎪⎝⎭,210001000ω⎛⎫⎪ ⎪ ⎪⎝⎭21000000ωω⎛⎫ ⎪ ⎪ ⎪⎝⎭#14. If N is a nilpotent (幂零的) 33⨯ matrix over C , prove that 21128A I N N =+- satisfies2A I N =+, i.e., A is a square root of I N +. Use the binomial series for 1/2(1)t + to obtain asimilar formula for a square root of I N +, where N is any nilpotent n n ⨯ matrix over C .Use the result above to prove that if c is a non-zero complex number and N is a nilpotent complex matrix, then cI N +has a square root. Now use the Jordan form to prove that every non-singular complex n n ⨯ matrix has a square root.Solution If N is an n n ⨯ matrix and k N O =, then k x is an annihilating polynomial for N . The minimal polynomial of N must be of the form p x , where p n ≤ and p k ≤ since the minimal polynomial of a matrix divides its characteristic polynomial. Thus, n N O =.(1) If N is a nilpotent 33⨯ matrix, then 3N O =. By straightforward computation, we can verify that 2A I N =+.(2) If N is an n n ⨯ nilpotent matrix, n N O =.1/22111111(1)(1)((1)1)122222(1)122!(1)!n n t t t t n -----++=+++++- 1/22111111(1)(1)((1)1)122222()22!(1)!n n I N I N N N n -----++=++++-(3) Since1N c is a nilpotent matrix, 1I N c + has a square root 1/21()I N c+. cI N + has a square root 1/21/21()c I N c+.(4) Suppose that 12121()0()000()r d d d r J J P AP J J λλλ-⎛⎫ ⎪⎪==⎪ ⎪ ⎪⎝⎭. Then each ()k d k J λ has asquare root 1/2()k d k J λ since ()k d k J λ is of the form k I N λ+, where 0k λ≠ because A is nonsingular and N is nilpotent.Let 121/211/2211/2()000()000()r d d d r J J B P P J λλλ-⎛⎫⎪⎪=⎪ ⎪⎪⎝⎭, then 2B A =. Hence, A has a squareroot.#20. Prove that the minimal polynomial of a matrix is equal to the characteristic polynomial if andonly if the elementary divisors are relatively prime in pairs.Proof Suppose that a Jordan canonical form of A is1212()000()000()r d d d r J J J J λλλ⎛⎫⎪ ⎪=⎪ ⎪ ⎪⎝⎭(where 12,,,r λλλ are not necessarily distinct. Each ()i d i J λ is a Jordan block.)The minimal polynomial of A is the same as that of J . The characteristic polynomial of A is the same as that of J . The elementary divisors of A are 11()d λλ-, , ()rd r λλ-The minimal polynomial of ()i d i J λ is ()i d i λλ-. The minimal polynomial of J is the least common multiple (最小公倍式) of 11()d λλ-, , ()rd r λλ-. The characteristicpolynomial of J is 1212()()()()rd d d r p λλλλλλλ=--- .The least common divisor of 11()d λλ-, , ()rd r λλ- is equal to the product of11()d λλ-, , ()r d r λλ- if and only if ()j dj λλ-and ()k d k λλ-are relatively prime forj k ≠. Thus the minimal polynomial of a matrix is equal to the characteristic polynomial ifand only if the elementary divisors are relatively prime in pairs.。
南航矩阵论课后习题答案

南航矩阵论课后习题答案南航矩阵论课后习题答案矩阵论是数学中的一个重要分支,广泛应用于各个领域,包括物理学、工程学、计算机科学等等。
南航的矩阵论课程是培养学生数学思维和解决实际问题的重要环节。
在课后习题中,学生需要运用所学的矩阵理论知识,解答各种问题。
下面是南航矩阵论课后习题的一些答案和解析。
1. 已知矩阵A = [1 2 3; 4 5 6; 7 8 9],求A的逆矩阵。
解析:要求一个矩阵的逆矩阵,需要先判断该矩阵是否可逆。
一个矩阵可逆的充要条件是其行列式不为零。
计算矩阵A的行列式,得到det(A) = -3。
因此,矩阵A可逆。
接下来,我们可以使用伴随矩阵法求解逆矩阵。
首先,计算矩阵A的伴随矩阵Adj(A),然后将其除以行列式的值,即可得到逆矩阵。
计算得到A的伴随矩阵为Adj(A) = [-3 6 -3; 6 -12 6; -3 6 -3]。
最后,将伴随矩阵除以行列式的值,即可得到矩阵A的逆矩阵A^-1 = [-1 2 -1; 2 -4 2; -1 2 -1]。
2. 已知矩阵A = [2 1; 3 4],求A的特征值和特征向量。
解析:要求一个矩阵的特征值和特征向量,需要先求解其特征方程。
特征方程的形式为|A - λI| = 0,其中A为给定矩阵,λ为特征值,I为单位矩阵。
计算得到特征方程为|(2-λ) 1; 3 (4-λ)| = (2-λ)(4-λ) - 3 = λ^2 - 6λ + 5 = 0。
解这个二次方程,得到特征值λ1 = 1,λ2 = 5。
接下来,我们可以求解对应于每个特征值的特征向量。
将特征值代入(A - λI)x = 0,即可求解出特征向量。
对于特征值λ1 = 1,解得特征向量x1 = [1; -1];对于特征值λ2 = 5,解得特征向量x2 = [1; 3]。
3. 已知矩阵A = [1 2; 3 4],求A的奇异值分解。
解析:奇异值分解是将一个矩阵分解为三个矩阵的乘积:A = UΣV^T,其中U和V是正交矩阵,Σ是对角矩阵。
南航双语矩阵论-matrix-theory第三章部分题解精选全文

可编辑修改精选全文完整版Solution Key to Some Exercises in Chapter 3 #5. Determine the kernel and range of each of the following linear transformations on 2P(a) (())'()p x xp x σ=(b) (())()'()p x p x p x σ=- (c) (())(0)(1)p x p x p σ=+Solution (a) Let ()p x ax b =+. (())p x ax σ=.(())0p x σ= if and only if 0ax = if and only if 0a =. Thus, ker(){|}b b R σ=∈The range of σis 2()P σ={|}ax a R ∈ (b) Let ()p x ax b =+. (())p x ax b a σ=+-.(())0p x σ= if and only if 0ax b a +-= if and only if 0a =and 0b =. Thus, ker(){0}σ=The range of σis 2()P σ=2{|,}P ax b a a b R +-∈=(c) Let ()p x ax b =+. (())p x bx a b σ=++.(())0p x σ= if and only if 0bx a b ++= if and only if 0a =and 0b =. Thus, ker(){0}σ=The range of σis 2()P σ=2{|,}P bx a b a b R ++∈= 备注: 映射的核以及映射的像都是集合,应该以集合的记号来表达或者用文字来叙述. #7. Let be the linear mapping that maps 2P into 2R defined by10()(())(0)p x dx p x p σ⎛⎫⎪= ⎪⎝⎭⎰ Find a matrix A such that()x A ασαββ⎛⎫+= ⎪⎝⎭.Solution1(1)1σ⎛⎫= ⎪⎝⎭ 1/2()0x σ⎛⎫= ⎪⎝⎭11/211/2()1010x ασαβαββ⎛⎫⎛⎫⎛⎫⎛⎫+=+= ⎪ ⎪⎪⎪⎝⎭⎝⎭⎝⎭⎝⎭Hence, 11/210A ⎛⎫= ⎪⎝⎭#10. Let σ be the transformation on 3P defined by(())'()"()p x xp x p x σ=+a) Find the matrix A representing σ with respect to 2[1,,]x x b) Find the matrix B representing σ with respect to 2[1,,1]x x + c) Find the matrix S such that 1B S AS -=d) If 2012()(1)p x a a x a x =+++, calculate (())n p x σ.Solution (a) (1)0σ= ()x x σ=22()22x x σ=+002010002A ⎛⎫⎪= ⎪ ⎪⎝⎭(b) (1)0σ=()x x σ=22(1)2(1)x x σ+=+000010002B ⎛⎫⎪= ⎪ ⎪⎝⎭(c)2[1,,1]x x +2[1,,]x x =101010001⎛⎫⎪⎪ ⎪⎝⎭The transition matrix from 2[1,,]x x to 2[1,,1]x x + is101010001S ⎛⎫ ⎪= ⎪ ⎪⎝⎭, 1B S AS -=(d) 2201212((1))2(1)n n a a x a x a x a x σ+++=++#11. Let A and B be n n ⨯ matrices. Show that if A is similar to B then there exist n n ⨯ matrices S and T , with S nonsingular, such thatA ST =andB TS =.Proof There exists a nonsingular matrix P such that 1A P BP -=. Let 1S P -=, T BP =. Then A ST =and B TS =.#12. Let σ be a linear transformation on the vector space V of dimension n . If there exist a vector v such that 1()v 0n σ-≠ and ()v 0n σ=, show that(a) 1,(),,()v v v n σσ- are linearly independent.(b) there exists a basis E for V such that the matrix representing σ with respect to the basis E is000010000010⎛⎫⎪⎪⎪⎪⎝⎭Proof(a) Suppose that1011()()v v v 0n n k k k σσ--+++= Then 11011(()())v v v 0n n n k k k σσσ---+++=That is, 12210110()()())()v v v v 0n n n n n k k k k σσσσ----+++==Thus, 0k must be zero since 1()v 0n σ-≠. 211111(()())()v v v 0n n n n k k k σσσσ----++==This will imply that 1k must be zero since 1()v 0n σ-≠.By repeating the process above, we obtain that 011,,,n k k k - must be all zero. Thisproves that1,(),,()v v v n σσ- are linearly independent.(b) Since 1,(),,()v v v n σσ- are n linearly independent, they form a basis for V .Denote 112,(),,()εv εv εv n n σσ-=== 12()εεσ= 23()εεσ= …….1()εεn n σ-= ()ε0n σ=12[(),(),,()]εεεn σσσ121[,,,,]εεεεn n -=000010000010⎛⎫⎪⎪⎪⎪⎝⎭#13. If A is a nonzero square matrix and k A O =for some positive integer k , show that A can not be similar to a diagonal matrix.Proof Suppose that A is similar to a diagonal matrix 12diag(,,,)n λλλ. Then for each i , there exists a nonzero vector x i such that x x i i i A λ= x x x 0k k i i i i i A λλ=== since k A O =.This will imply that 0i λ= for 1,2,,i n =. Thus, matrix A is similar to the zero matrix. Therefore, A O =since a matrix that is similar to the zero matrix must be the zero matrix, whichcontradicts the assumption.This contradiction shows that A can not be similar to a diagonal matrix. OrIf 112diag(,,,)n A P P λλλ-= then 112diag(,,,)k k k k n A P P λλλ-=. k A O = implies that 0i λ= for 1,2,,i n =. Hence, B O =. This will imply that A O =.Contradiction!。
南京航空航天大学2007-2014硕士研究生矩阵论matrixTheory试题

2 3 4 A 4 6 8 6 7 8 。 一(20 分) (1)设
2010 ~ 2011 学年《矩阵论》 课程考试 A 卷
(i)求 A 的特征多项式和 A 的全部特征值; (ii)求 A 的行列式因子,不变因子和初等因子; (iii)写出 A 的 Jordan 标准形;
1 A* A2 A* (3)证明: n 。
1 1 1 1 A 0 0 0 0 四、 (20 分)已知矩阵
(1)求矩阵 A 的 QR 分解;
1 2 0 1 b 1 1 2 1 ,向量 ,
(2)计算 A ;
17 6 14 60 A , B 45 16 3 13 ,试问 A 和 B 是否相似?并说明 (2)设
原因。
2 1 A 1 2 3 1 ,求 A 1 , A 2 , A , A F ; 二(20 分) (1)设
(3)用广义逆判断方程组 Ax b 是否相容?若相容,求其通解;若不相容,求其极小最小二乘解。
五、 (20 分)
(1)设矩阵
问当 t 满足什么条件时, A B 成立?
5 3 2 0 1 A 3 2 t , B 1 1 2 t 2 2 0 .5 t
五(20 分)设
A ( a ij )
为 n 阶 Hermite 矩阵,证明:
3
存在唯一 Hermite 矩阵 B 使得 A B ;
2
(2)
(3) 如果 A 0 ,则 tr ( A)tr ( A ) n 。
1
如果 A 0 ,则 tr ( A ) (tr ( A)) ;
2
南京航空航天大学2009_矩阵论考试考题及答案
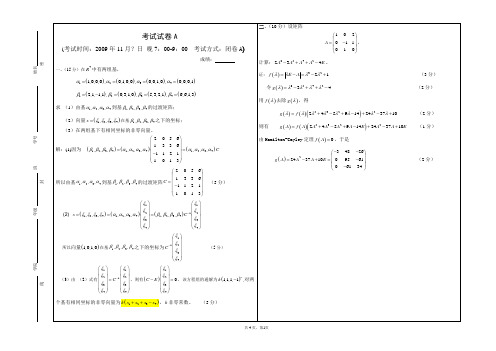
(3),判断方程组 Ax b 是否相容?若相容,求其最小范数解;若不相容,求其极小最小二乘 解。(4 分)
解:
2 0 0 8 1 0 0 4 行 (1): A 0 2 8 0 0 1 4 0 ,故矩阵 A 的满秩分解为: 2 2 8 8 0 0 0 0 2 0 2 0 1 0 0 4 1 0 0 4 A 0 2 CD, C 0 2 , D 。 0 1 4 0 0 1 4 0 2 2 2 2
k
k
k 1
A |||| A k 1 |||| A || || A || k . (5 分)
k
2. || A || 1 lim || A || k 0 lim || A k || 0. (5 分) 3. lim || A k || 0 lim || A k 0 || 0 lim A k 0. (5 分)
学院 ------------------------------ 线 ----------------------------------------------------------------
年级 ----------
从而其极小最小二乘解为:
南航双语矩阵论matrix theory第4章部分习题参考答案

)
If i is a root of p( ) 0 , then p(i ) 0 . We obtain that eigenvalue of C T with eigenvector x (1, i ,, in 2 , in 1 )T .
Exercise 16
Let be an orthogonal transformation on a Euclidean space V (an inner product space over the real number field). If W is a -invariant subspace of V, show that the orthogonal complement of W is also -invariant. Proof Let V W W , where W is -invariant. Let {u1 , u2 ,, uk } be an orthonormal basis for
0 1 T C x 0 0 0 0 1 0 0 0 0 0 0 an 0 an 1 0 an 2 1 a1
T
i i 1 2 2 i i i n2 n 1 n 1 i i i n 1 n n 1 a a a p ( i n i n 1 i 1 i i
C T x i x . Then i is an
(b) If p( ) has n distinct roots, then all roots of p( ) are eigenvalues of C T . We obtain that the characteristic polynomial of C T and p( ) have the same n distinct roots. And also they have the same degree and the same leading coefficient. Hence, the characteristic polynomial of C T is the same as p( ) . Since C and C T have the same characteristic polynomial, we know that p( ) is the characteristic polynomial of C.
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
1)
一组基为q =
.维数为3.
3)
南京航空航天大学双语矩阵论期中考试参考答案(有些答案可能有问题) Q1
1解矩阵A 的特征多项式为
A-2 3 -4
4I-A| =-4 2+6 -8 =A 2(/l-4)
-6 7 A-8
所以矩阵A 的特征值为4 =0(二重)和/^=4.
人・2 3
由于(4-2,3)=1,所以D| (人)二1.又 彳 人+6=“2+4人=?(人)
4-2 3
、=7人+4=代(人)故(们3),代3))=1 •其余的二阶子式(还有7个)都包含因子4, -6 7
所以 D? 3)=1 .最后 det (A (/L))=42(人.4),所以 D 3(A)=/l 2 (2-4). 因此矩阵A 的不变因子为d, (2) = d 2(2) = l, d 3 (2) = r (2-4). 矩阵A 的初等因子为人2, 2-4.
2解矩阵B 与矩阵C 是相似的.矩阵B 和矩阵C 的行列式因子相同且分别为9 3)=1 ,
D 2(/i)=A 2-/l-2 .根据定理:两矩阵相似的充分必要条件是他们有相同的行列式因子.
所以矩阵B 与矩阵c 相似.
Q2
2)设k 是数域p 中任意数,a, 0, /是v 中任意元素.明显满足下而四项.
(") = (",a) ; (a+月,/) = (",/) + (”,刃;(ka,/3) = k(a,/3) ; (a,a)>0,
当且仅当Q = 0时(a,a) = ().所以(。
,/?)是线性空间V 上的内积.
利
用Gram-Schmidt 正交化方法,可以依次求出
,p 2 =%-(%'5)与=
层=%-(%,弟与一(%,弓)役=
T ") = %2+"12 _1
_
02+"12 -o r
%2 +Sn _ i
%1+”21
%2 +腐2 _ 1 0 %1 +021 +。
12 +"12
+811 + %2 +012 %2 +§T1 + G 1 +J1
%2 +0\2 +%1 +目21 T (a )+T (/?)=T (a+").T (ka ) = R 任1 +。
12 。
》+名] 0] +0>7 +4] 灯(a ).所以T 是V 上的线性变换.T (/) = T (%) =
1 1
0 0矩阵A 称为线性变换T 在
0 0
Q3 1)
2)
l|| = 7(n )=V2 , |3X 2
-1|| = ^
(3X 2-1,3X
2
3)
设S 中的任意向量为K I +K2(3X 2-
-(^ + ^(3\2-1))|| = ^ j (x-3K 2x 2-K 1 + K2)2</% =
则£|,旦,§是标准正交基•
4)设Q, ”CV, k 为数域P 上的任意数.
0 丁(。
|,%,。
3)二(。
1,%,%)人. A= 2
2
QIZ 2 7
—+2K
《+—.明显看 5 I
3
出当K!=K 2=O 时上式值
最小。
所以x 在S 上的最佳逼近为0向量.
Q4
1)维数为 3, 一组基为 0=1, a 2=x , a y = x 1.
D (l )=0-l+0x + 0-x 2
2) D (x )=l-l+0 x + 0-x 2 或表示成D (l, x, x 2) = (l, x, x?)A.则线性变换在
D (X 2
)=0-1+2-X + 0-X
2
I
(1,3亍一 1)= j (3x 2
-l )^/x = 0所以 1 和 3X 2
-1 是正交的.
-1
1 %+坊 0 %1+”21 8
3)
4) 0 1 0
基1, X, x2下的矩阵为A= 0 0 2
0 0 0
A -1 |AE-A|= 0 2 0 0
-2 =23 4.当4=0的时候,秩r(AE-A )= 2,所以D 不可对角化.
A
£\ -ii^ii ->
腐=%一(%,£|)弓=X ,£2
~
-
二。
3 - ( %,*1 )与 一 (0,£ ?)《2 = JV~ - I / 3 ,《
Q5
1) Ikllp ,||域0为无效范数,kill 为有效范数.
对于||圳°,当x={i ,i ,i …}时,|HHHII %P G P
不满足齐次性,所以为无效 范数. 对于 x p,当a={0,a,0,(),•••}, 0={a,0,0,(),•••}, (x,/3e. R n , p =—时.
2)
Q6
R + "||p =(2网)2 =4|d ,而妆|| +1加|| = 2同,所以不满足三角不等式.||x||p 为 无效范数,另外根据Minkowski 不等式,只有当P21才满足三角不等式的条件, 也可也得到lk||p 为无效范数.对于凤,容易证明满足非负性,齐次性和三角不等 式。
故为有
效范数.
1) 证明存在可逆矩阵P,使P 'AP=A ,其中A 为对角矩阵.
证明
A 的特征值互异.
A* X】…,* X,…,X n]PA
对于Q1中的矩阵,是不可对角矩阵.因为当特征值为0.
.
. .
因为X], X2,---, Xn线性无关,故P为满秩矩阵,所以AP=PA ,即存在可逆矩阵使得P"AP=A,即A可对角化.
必要性:已知存在可逆矩阵P使P J A P=A,设P=[P|, P2,…,P n],则有
[A P p AP2,..-, APJ = [/11P1, /l2P2,---, /l n P n],可知九为A 的特征值,%为A 的特
征向量.即A有n个线性无关的特征向量.
秩为2壬〃一§,S,・=2/ = 3.根据Q1的初等因子可得Jordan形矩阵为
3) 证明对于A的每一个特征值W都有秩(4义- A) = 〃 - S’,S,.是九的重数.
4) 矩阵A可对角化的充要条件是有n个线性无关的特征向量.
证明:充分性:设A的n个线性无关的特征向量为XI, X2, X3,…,Xn.则, i = 1,2,…,〃。
