KAMENEV-TYPE OSCILLATION CRITERIA FOR DELAY DIFFERENCE EQUATIONS
假肠膜明串珠菌国外检测标准

假肠膜明串珠菌国外检测标准英文回答:The international standards for detecting Enterococcus faecium are important for ensuring the accuracy and reliability of test results. These standards help laboratories and researchers across different countries to compare and validate their findings. There are several key aspects to consider when it comes to the detection of Enterococcus faecium.Firstly, the sampling and collection methods play a crucial role in obtaining accurate results. The international standards provide guidelines on the appropriate techniques for collecting samples fromdifferent sources, such as food, water, or clinical specimens. For example, when testing food samples for Enterococcus faecium, the standards may recommend swabbing the surface of the food item and transferring the swab into a suitable transport medium.Secondly, the isolation and identification of Enterococcus faecium require specific laboratory procedures. The international standards outline the necessary steps and protocols for culturing and identifying the bacteria. These protocols may include using selective media, performing biochemical tests, and confirming the identificationthrough molecular techniques. Following these standardized procedures ensures that the detected Enterococcus faecium strains are accurately identified.Furthermore, the standards also address theantimicrobial susceptibility testing of Enterococcus faecium. Given the rising concern of antibiotic resistance, it is important to determine the susceptibility profile of the bacteria. The international standards provideguidelines on the appropriate methods for testing the susceptibility of Enterococcus faecium to various antibiotics. This information is crucial for guidingclinical treatment and monitoring the emergence ofresistant strains.In addition to the technical aspects, international standards also consider the reporting and interpretation of results. These standards provide guidelines on how to report the presence or absence of Enterococcus faecium, as well as any relevant quantitative data. Standardized reporting ensures that the results are consistent and can be easily compared across different laboratories and studies.中文回答:肠膜明串珠菌的国际检测标准对于确保测试结果的准确性和可靠性非常重要。
假肠膜明串珠菌国外检测标准

假肠膜明串珠菌国外检测标准英文回答:The international standards for detecting Enterococcus faecalis, also known as Vancomycin-resistant enterococci (VRE), in the intestines are well-established. These standards are followed by laboratories around the world to ensure accurate and reliable detection of this pathogen.One commonly used method for detecting VRE is the culture-based method. This involves collecting a stool sample from the patient and culturing it on selective media that promote the growth of VRE. The presence of VRE can then be confirmed through further testing, such as biochemical tests or molecular methods like polymerase chain reaction (PCR). These methods allow for the identification of specific genes or markers associated with VRE.Another approach for detecting VRE is through molecularmethods alone, such as PCR or real-time PCR. These methods directly detect the presence of VRE DNA in the stool sample, without the need for culture. They are often more sensitive and rapid compared to culture-based methods.In addition to these laboratory methods, there are also guidelines and recommendations for the interpretation ofthe test results. These guidelines help to determine the clinical significance of VRE detection and guideappropriate patient management and infection control measures.For example, if a patient is found to be colonized with VRE but does not show any signs or symptoms of infection,it may indicate that the patient is a carrier of thebacteria but not actively infected. In such cases,infection control measures may still be implemented to prevent the spread of VRE to other patients.On the other hand, if a patient is found to be positive for VRE and is showing signs and symptoms of infection, appropriate antibiotic treatment may be initiated based onthe susceptibility testing of the isolated VRE strain. This can help in selecting the most effective antibiotic totreat the infection.中文回答:国际上对于检测肠道中的假肠膜明串珠菌(VRE)的标准已经得到了很好的建立。
一类非线性振动系统的周期运动

一类非线性振动系统的周期运动余晓娟【摘要】In the system of nonlinear oscillating, periodic motion is of prime importance. The paper studies the periodic motion for a class of two-degree-of-freedom nonlinear oscillating systems. This model can be expressed by two mutual coupling second-order nonlinear differential equations. By using the method of Liapunov function and special techniques, periodic solution to the system are obtained.%在非线性振动系统中,周期运动至关重要。
该文研究了一类两自由度非线性振动系统的周期运动,这个系统由两个相互耦合的二阶非线性微分方程表示,运用Liapunov函数方法和特殊技巧,得到了该类系统的周期解。
【期刊名称】《文山学院学报》【年(卷),期】2016(029)003【总页数】3页(P39-41)【关键词】振动系统;周期运动;周期解【作者】余晓娟【作者单位】汉江师范学院数学与财经系,湖北十堰442000【正文语种】中文【中图分类】O175.14在非线性振动系统中,周期运动具有非常重要的作用,但周期解的存在性问题一直都是研究的重点。
对于多自由度系统的周期解,在理论与应用上都有着十分重要的作用,文献[1]研究了如下一类两自由度系统的周期解:其中a1,a2,b1,b2是大于0的常数,f1,f2是关于x,y,sint,cost的连续函数。
本文在此基础上,研究比较复杂的一类两自由度非线性系统,它由两个相互耦合的二阶非线性微分方程组成:其中是阻力,Q(t, x,y),H(t, x,y)是势,f (x,y)cosωt,g(x,y)sinωt是强迫外力。
稳定性英文版

HPLC ASSAY with DETERMINATION OF META-FLUOXETINE HCl.ANALYTICAL METHOD VALIDATION10 and 20mg Fluoxetine Capsules HPLC DeterminationFLUOXETINE HClC17H18F3NO•HClM.W. = 345.79CAS — 59333-67-4STABILITY INDICATINGA S S A Y V A L I D A T I O NMethod is suitable for:ýIn-process controlþProduct ReleaseþStability indicating analysis (Suitability - US/EU Product) CAUTIONFLUOXETINE HYDROCHLORIDE IS A HAZARDOUS CHEMICAL AND SHOULD BE HANDLED ONLY UNDER CONDITIONS SUITABLE FOR HAZARDOUS WORK.IT IS HIGHLY PRESSURE SENSITIVE AND ADEQUATE PRECAUTIONS SHOULD BE TAKEN TO AVOID ANY MECHANICAL FORCE (SUCH AS GRINDING, CRUSHING, ETC.) ON THE POWDER.ED. N0: 04Effective Date:APPROVED::HPLC ASSAY with DETERMINATION OF META-FLUOXETINE HCl.ANALYTICAL METHOD VALIDATION10 and 20mg Fluoxetine Capsules HPLC DeterminationTABLE OF CONTENTS INTRODUCTION........................................................................................................................ PRECISION............................................................................................................................... System Repeatability ................................................................................................................ Method Repeatability................................................................................................................. Intermediate Precision .............................................................................................................. LINEARITY................................................................................................................................ RANGE...................................................................................................................................... ACCURACY............................................................................................................................... Accuracy of Standard Injections................................................................................................ Accuracy of the Drug Product.................................................................................................... VALIDATION OF FLUOXETINE HCl AT LOW CONCENTRATION........................................... Linearity at Low Concentrations................................................................................................. Accuracy of Fluoxetine HCl at Low Concentration..................................................................... System Repeatability................................................................................................................. Quantitation Limit....................................................................................................................... Detection Limit........................................................................................................................... VALIDATION FOR META-FLUOXETINE HCl (POSSIBLE IMPURITIES).................................. Meta-Fluoxetine HCl linearity at 0.05% - 1.0%........................................................................... Detection Limit for Fluoxetine HCl.............................................................................................. Quantitation Limit for Meta Fluoxetine HCl................................................................................ Accuracy for Meta-Fluoxetine HCl ............................................................................................ Method Repeatability for Meta-Fluoxetine HCl........................................................................... Intermediate Precision for Meta-Fluoxetine HCl......................................................................... SPECIFICITY - STABILITY INDICATING EVALUATION OF THE METHOD............................. FORCED DEGRADATION OF FINISHED PRODUCT AND STANDARD..................................1. Unstressed analysis...............................................................................................................2. Acid Hydrolysis stressed analysis..........................................................................................3. Base hydrolysis stressed analysis.........................................................................................4. Oxidation stressed analysis...................................................................................................5. Sunlight stressed analysis.....................................................................................................6. Heat of solution stressed analysis.........................................................................................7. Heat of powder stressed analysis.......................................................................................... System Suitability stressed analysis.......................................................................................... Placebo...................................................................................................................................... STABILITY OF STANDARD AND SAMPLE SOLUTIONS......................................................... Standard Solution...................................................................................................................... Sample Solutions....................................................................................................................... ROBUSTNESS.......................................................................................................................... Extraction................................................................................................................................... Factorial Design......................................................................................................................... CONCLUSION...........................................................................................................................ED. N0: 04Effective Date:APPROVED::HPLC ASSAY with DETERMINATION OF META-FLUOXETINE HCl.ANALYTICAL METHOD VALIDATION10 and 20mg Fluoxetine Capsules HPLC DeterminationBACKGROUNDTherapeutically, Fluoxetine hydrochloride is a classified as a selective serotonin-reuptake inhibitor. Effectively used for the treatment of various depressions. Fluoxetine hydrochloride has been shown to have comparable efficacy to tricyclic antidepressants but with fewer anticholinergic side effects. The patent expiry becomes effective in 2001 (US). INTRODUCTIONFluoxetine capsules were prepared in two dosage strengths: 10mg and 20mg dosage strengths with the same capsule weight. The formulas are essentially similar and geometrically equivalent with the same ingredients and proportions. Minor changes in non-active proportions account for the change in active ingredient amounts from the 10 and 20 mg strength.The following validation, for the method SI-IAG-206-02 , includes assay and determination of Meta-Fluoxetine by HPLC, is based on the analytical method validation SI-IAG-209-06. Currently the method is the in-house method performed for Stability Studies. The Validation was performed on the 20mg dosage samples, IAG-21-001 and IAG-21-002.In the forced degradation studies, the two placebo samples were also used. PRECISIONSYSTEM REPEATABILITYFive replicate injections of the standard solution at the concentration of 0.4242mg/mL as described in method SI-IAG-206-02 were made and the relative standard deviation (RSD) of the peak areas was calculated.SAMPLE PEAK AREA#15390#25406#35405#45405#55406Average5402.7SD 6.1% RSD0.1ED. N0: 04Effective Date:APPROVED::HPLC ASSAY with DETERMINATION OF META-FLUOXETINE HCl.ANALYTICAL METHOD VALIDATION10 and 20mg Fluoxetine Capsules HPLC DeterminationED. N0: 04Effective Date:APPROVED::PRECISION - Method RepeatabilityThe full HPLC method as described in SI-IAG-206-02 was carried-out on the finished product IAG-21-001 for the 20mg dosage form. The method repeated six times and the relative standard deviation (RSD) was calculated.SAMPLENumber%ASSAYof labeled amountI 96.9II 97.8III 98.2IV 97.4V 97.7VI 98.5(%) Average97.7SD 0.6(%) RSD0.6PRECISION - Intermediate PrecisionThe full method as described in SI-IAG-206-02 was carried-out on the finished product IAG-21-001 for the 20mg dosage form. The method was repeated six times by a second analyst on a different day using a different HPLC instrument. The average assay and the relative standard deviation (RSD) were calculated.SAMPLENumber% ASSAYof labeled amountI 98.3II 96.3III 94.6IV 96.3V 97.8VI 93.3Average (%)96.1SD 2.0RSD (%)2.1The difference between the average results of method repeatability and the intermediate precision is 1.7%.HPLC ASSAY with DETERMINATION OF META-FLUOXETINE HCl.ANALYTICAL METHOD VALIDATION10 and 20mg Fluoxetine Capsules HPLC DeterminationLINEARITYStandard solutions were prepared at 50% to 200% of the nominal concentration required by the assay procedure. Linear regression analysis demonstrated acceptability of the method for quantitative analysis over the concentration range required. Y-Intercept was found to be insignificant.RANGEDifferent concentrations of the sample (IAG-21-001) for the 20mg dosage form were prepared, covering between 50% - 200% of the nominal weight of the sample.Conc. (%)Conc. (mg/mL)Peak Area% Assayof labeled amount500.20116235096.7700.27935334099.21000.39734463296.61500.64480757797.52000.79448939497.9(%) Average97.6SD 1.0(%) RSD 1.0ED. N0: 04Effective Date:APPROVED::HPLC ASSAY with DETERMINATION OF META-FLUOXETINE HCl.ANALYTICAL METHOD VALIDATION10 and 20mg Fluoxetine Capsules HPLC DeterminationED. N0: 04Effective Date:APPROVED::RANGE (cont.)The results demonstrate linearity as well over the specified range.Correlation coefficient (RSQ)0.99981 Slope11808.3Y -Interceptresponse at 100%* 100 (%) 0.3%ACCURACYACCURACY OF STANDARD INJECTIONSFive (5) replicate injections of the working standard solution at concentration of 0.4242mg/mL, as described in method SI-IAG-206-02 were made.INJECTIONNO.PEAK AREA%ACCURACYI 539299.7II 540599.9III 540499.9IV 5406100.0V 5407100.0Average 5402.899.9%SD 6.10.1RSD, (%)0.10.1The percent deviation from the true value wasdetermined from the linear regression lineHPLC ASSAY with DETERMINATION OF META-FLUOXETINE HCl.ANALYTICAL METHOD VALIDATION10 and 20mg Fluoxetine Capsules HPLC DeterminationED. N0: 04Effective Date:APPROVED::ACCURACY OF THE DRUG PRODUCTAdmixtures of non-actives (placebo, batch IAG-21-001 ) with Fluoxetine HCl were prepared at the same proportion as in a capsule (70%-180% of the nominal concentration).Three preparations were made for each concentration and the recovery was calculated.Conc.(%)Placebo Wt.(mg)Fluoxetine HCl Wt.(mg)Peak Area%Accuracy Average (%)70%7079.477.843465102.27079.687.873427100.77079.618.013465100.0101.0100%10079.6211.25476397.910080.8011.42491799.610079.6011.42485498.398.6130%13079.7214.90640599.413080.3114.75632899.213081.3314.766402100.399.618079.9920.10863699.318079.3820.45879499.418080.0820.32874899.599.4Placebo, Batch Lot IAG-21-001HPLC ASSAY with DETERMINATION OF META-FLUOXETINE HCl.ANALYTICAL METHOD VALIDATION10 and 20mg Fluoxetine Capsules HPLC DeterminationED. N0: 04Effective Date:APPROVED::VALIDATION OF FLUOXETINE HClAT LOW CONCENTRATIONLINEARITY AT LOW CONCENTRATIONSStandard solution of Fluoxetine were prepared at approximately 0.02%-1.0% of the working concentration required by the method SI-IAG-206-02. Linear regression analysis demonstrated acceptability of the method for quantitative analysis over this range.ACCURACY OF FLUOXETINE HCl AT LOW CONCENTRATIONThe peak areas of the standard solution at the working concentration were measured and the percent deviation from the true value, as determined from the linear regression was calculated.SAMPLECONC.µg/100mLAREA FOUND%ACCURACYI 470.56258499.7II 470.56359098.1III 470.561585101.3IV 470.561940100.7V 470.56252599.8VI 470.56271599.5(%) AverageSlope = 132.7395299.9SD Y-Intercept = -65.872371.1(%) RSD1.1HPLC ASSAY with DETERMINATION OF META-FLUOXETINE HCl.ANALYTICAL METHOD VALIDATION10 and 20mg Fluoxetine Capsules HPLC DeterminationSystem RepeatabilitySix replicate injections of standard solution at 0.02% and 0.05% of working concentration as described in method SI-IAG-206-02 were made and the relative standard deviation was calculated.SAMPLE FLUOXETINE HCl AREA0.02%0.05%I10173623II11503731III10103475IV10623390V10393315VI10953235Average10623462RSD, (%) 5.0 5.4Quantitation Limit - QLThe quantitation limit ( QL) was established by determining the minimum level at which the analyte was quantified. The quantitation limit for Fluoxetine HCl is 0.02% of the working standard concentration with resulting RSD (for six injections) of 5.0%. Detection Limit - DLThe detection limit (DL) was established by determining the minimum level at which the analyte was reliably detected. The detection limit of Fluoxetine HCl is about 0.01% of the working standard concentration.ED. N0: 04Effective Date:APPROVED::HPLC ASSAY with DETERMINATION OF META-FLUOXETINE HCl.ANALYTICAL METHOD VALIDATION10 and 20mg Fluoxetine Capsules HPLC DeterminationED. N0: 04Effective Date:APPROVED::VALIDATION FOR META-FLUOXETINE HCl(EVALUATING POSSIBLE IMPURITIES)Meta-Fluoxetine HCl linearity at 0.05% - 1.0%Relative Response Factor (F)Relative response factor for Meta-Fluoxetine HCl was determined as slope of Fluoxetine HCl divided by the slope of Meta-Fluoxetine HCl from the linearity graphs (analysed at the same time).F =132.7395274.859534= 1.8Detection Limit (DL) for Fluoxetine HClThe detection limit (DL) was established by determining the minimum level at which the analyte was reliably detected.Detection limit for Meta Fluoxetine HCl is about 0.02%.Quantitation Limit (QL) for Meta-Fluoxetine HClThe QL is determined by the analysis of samples with known concentration of Meta-Fluoxetine HCl and by establishing the minimum level at which the Meta-Fluoxetine HCl can be quantified with acceptable accuracy and precision.Six individual preparations of standard and placebo spiked with Meta-Fluoxetine HCl solution to give solution with 0.05% of Meta Fluoxetine HCl, were injected into the HPLC and the recovery was calculated.HPLC ASSAY with DETERMINATION OF META-FLUOXETINE HCl.ANALYTICAL METHOD VALIDATION10 and 20mg Fluoxetine Capsules HPLC DeterminationED. N0: 04Effective Date:APPROVED::META-FLUOXETINE HCl[RECOVERY IN SPIKED SAMPLES].Approx.Conc.(%)Known Conc.(µg/100ml)Area in SpikedSampleFound Conc.(µg/100mL)Recovery (%)0.0521.783326125.735118.10.0521.783326825.821118.50.0521.783292021.55799.00.0521.783324125.490117.00.0521.783287220.96996.30.0521.783328526.030119.5(%) AVERAGE111.4SD The recovery result of 6 samples is between 80%-120%.10.7(%) RSDQL for Meta Fluoxetine HCl is 0.05%.9.6Accuracy for Meta Fluoxetine HClDetermination of Accuracy for Meta-Fluoxetine HCl impurity was assessed using triplicate samples (of the drug product) spiked with known quantities of Meta Fluoxetine HCl impurity at three concentrations levels (namely 80%, 100% and 120% of the specified limit - 0.05%).The results are within specifications:For 0.4% and 0.5% recovery of 85% -115%For 0.6% recovery of 90%-110%HPLC ASSAY with DETERMINATION OF META-FLUOXETINE HCl.ANALYTICAL METHOD VALIDATION10 and 20mg Fluoxetine Capsules HPLC DeterminationED. N0: 04Effective Date:APPROVED::META-FLUOXETINE HCl[RECOVERY IN SPIKED SAMPLES]Approx.Conc.(%)Known Conc.(µg/100mL)Area in spikedSample Found Conc.(µg/100mL)Recovery (%)[0.4%]0.4174.2614283182.66104.820.4174.2614606187.11107.370.4174.2614351183.59105.36[0.5%]0.5217.8317344224.85103.220.5217.8316713216.1599.230.5217.8317341224.81103.20[0.6%]0.6261.3918367238.9591.420.6261.3920606269.81103.220.6261.3920237264.73101.28RECOVERY DATA DETERMINED IN SPIKED SAMPLESHPLC ASSAY with DETERMINATION OF META-FLUOXETINE HCl.ANALYTICAL METHOD VALIDATION10 and 20mg Fluoxetine Capsules HPLC DeterminationED. N0: 04Effective Date:APPROVED::REPEATABILITYMethod Repeatability - Meta Fluoxetine HClThe full method (as described in SI-IAG-206-02) was carried out on the finished drug product representing lot number IAG-21-001-(1). The HPLC method repeated serially, six times and the relative standard deviation (RSD) was calculated.IAG-21-001 20mg CAPSULES - FLUOXETINESample% Meta Fluoxetine % Meta-Fluoxetine 1 in Spiked Solution10.0260.09520.0270.08630.0320.07740.0300.07450.0240.09060.0280.063AVERAGE (%)0.0280.081SD 0.0030.012RSD, (%)10.314.51NOTE :All results are less than QL (0.05%) therefore spiked samples with 0.05% Meta Fluoxetine HCl were injected.HPLC ASSAY with DETERMINATION OF META-FLUOXETINE HCl.ANALYTICAL METHOD VALIDATION10 and 20mg Fluoxetine Capsules HPLC DeterminationED. N0: 04Effective Date:APPROVED::Intermediate Precision - Meta-Fluoxetine HClThe full method as described in SI-IAG-206-02 was applied on the finished product IAG-21-001-(1) .It was repeated six times, with a different analyst on a different day using a different HPLC instrument.The difference between the average results obtained by the method repeatability and the intermediate precision was less than 30.0%, (11.4% for Meta-Fluoxetine HCl as is and 28.5% for spiked solution).IAG-21-001 20mg - CAPSULES FLUOXETINESample N o:Percentage Meta-fluoxetine% Meta-fluoxetine 1 in spiked solution10.0260.06920.0270.05730.0120.06140.0210.05850.0360.05560.0270.079(%) AVERAGE0.0250.063SD 0.0080.009(%) RSD31.514.51NOTE:All results obtained were well below the QL (0.05%) thus spiked samples slightly greater than 0.05% Meta-Fluoxetine HCl were injected. The RSD at the QL of the spiked solution was 14.5%HPLC ASSAY with DETERMINATION OF META-FLUOXETINE HCl.ANALYTICAL METHOD VALIDATION10 and 20mg Fluoxetine Capsules HPLC DeterminationSPECIFICITY - STABILITY INDICATING EVALUATIONDemonstration of the Stability Indicating parameters of the HPLC assay method [SI-IAG-206-02] for Fluoxetine 10 & 20mg capsules, a suitable photo-diode array detector was incorporated utilizing a commercial chromatography software managing system2, and applied to analyze a range of stressed samples of the finished drug product.GLOSSARY of PEAK PURITY RESULT NOTATION (as reported2):Purity Angle-is a measure of spectral non-homogeneity across a peak, i.e. the weighed average of all spectral contrast angles calculated by comparing all spectra in the integrated peak against the peak apex spectrum.Purity Threshold-is the sum of noise angle3 and solvent angle4. It is the limit of detection of shape differences between two spectra.Match Angle-is a comparison of the spectrum at the peak apex against a library spectrum.Match Threshold-is the sum of the match noise angle3 and match solvent angle4.3Noise Angle-is a measure of spectral non-homogeneity caused by system noise.4Solvent Angle-is a measure of spectral non-homogeneity caused by solvent composition.OVERVIEWT he assay of the main peak in each stressed solution is calculated according to the assay method SI-IAG-206-02, against the Standard Solution, injected on the same day.I f the Purity Angle is smaller than the Purity Threshold and the Match Angle is smaller than the Match Threshold, no significant differences between spectra can be detected. As a result no spectroscopic evidence for co-elution is evident and the peak is considered to be pure.T he stressed condition study indicated that the Fluoxetine peak is free from any appreciable degradation interference under the stressed conditions tested. Observed degradation products peaks were well separated from the main peak.1® PDA-996 Waters™ ; 2[Millennium 2010]ED. N0: 04Effective Date:APPROVED::HPLC ASSAY with DETERMINATION OF META-FLUOXETINE HCl.ANALYTICAL METHOD VALIDATION10 and 20mg Fluoxetine Capsules HPLC DeterminationFORCED DEGRADATION OF FINISHED PRODUCT & STANDARD 1.UNSTRESSED SAMPLE1.1.Sample IAG-21-001 (2) (20mg/capsule) was prepared as stated in SI-IAG-206-02 and injected into the HPLC system. The calculated assay is 98.5%.SAMPLE - UNSTRESSEDFluoxetine:Purity Angle:0.075Match Angle:0.407Purity Threshold:0.142Match Threshold:0.4251.2.Standard solution was prepared as stated in method SI-IAG-206-02 and injected into the HPLC system. The calculated assay is 100.0%.Fluoxetine:Purity Angle:0.078Match Angle:0.379Purity Threshold:0.146Match Threshold:0.4272.ACID HYDROLYSIS2.1.Sample solution of IAG-21-001 (2) (20mg/capsule) was prepared as in method SI-IAG-206-02 : An amount equivalent to 20mg Fluoxetine was weighed into a 50mL volumetric flask. 20mL Diluent was added and the solution sonicated for 10 minutes. 1mL of conc. HCl was added to this solution The solution was allowed to stand for 18 hours, then adjusted to about pH = 5.5 with NaOH 10N, made up to volume with Diluent and injected into the HPLC system after filtration.Fluoxetine peak intensity did NOT decrease. Assay result obtained - 98.8%.SAMPLE- ACID HYDROLYSISFluoxetine peak:Purity Angle:0.055Match Angle:0.143Purity Threshold:0.096Match Threshold:0.3712.2.Standard solution was prepared as in method SI-IAG-206-02 : about 22mg Fluoxetine HCl were weighed into a 50mL volumetric flask. 20mL Diluent were added. 2mL of conc. HCl were added to this solution. The solution was allowed to stand for 18 hours, then adjusted to about pH = 5.5 with NaOH 10N, made up to volume with Diluent and injected into the HPLC system.Fluoxetine peak intensity did NOT decrease. Assay result obtained - 97.2%.ED. N0: 04Effective Date:APPROVED::HPLC ASSAY with DETERMINATION OF META-FLUOXETINE HCl.ANALYTICAL METHOD VALIDATION10 and 20mg Fluoxetine Capsules HPLC DeterminationSTANDARD - ACID HYDROLYSISFluoxetine peak:Purity Angle:0.060Match Angle:0.060Purity Threshold:0.099Match Threshold:0.3713.BASE HYDROLYSIS3.1.Sample solution of IAG-21-001 (2) (20mg/capsule) was prepared as per method SI-IAG-206-02 : An amount equivalent to 20mg Fluoxetine was weight into a 50mL volumetric flask. 20mL Diluent was added and the solution sonicated for 10 minutes. 1mL of 5N NaOH was added to this solution. The solution was allowed to stand for 18 hours, then adjusted to about pH = 5.5 with 5N HCl, made up to volume with Diluent and injected into the HPLC system.Fluoxetine peak intensity did NOT decrease. Assay result obtained - 99.3%.SAMPLE - BASE HYDROLYSISFluoxetine peak:Purity Angle:0.063Match Angle:0.065Purity Threshold:0.099Match Threshold:0.3623.2.Standard stock solution was prepared as per method SI-IAG-206-02 : About 22mg Fluoxetine HCl was weighed into a 50mL volumetric flask. 20mL Diluent was added. 2mL of 5N NaOH was added to this solution. The solution was allowed to stand for 18 hours, then adjusted to about pH=5.5 with 5N HCl, made up to volume with Diluent and injected into the HPLC system.Fluoxetine peak intensity did NOT decrease - 99.5%.STANDARD - BASE HYDROLYSISFluoxetine peak:Purity Angle:0.081Match Angle:0.096Purity Threshold:0.103Match Threshold:0.3634.OXIDATION4.1.Sample solution of IAG-21-001 (2) (20mg/capsule) was prepared as per method SI-IAG-206-02. An equivalent to 20mg Fluoxetine was weighed into a 50mL volumetric flask. 20mL Diluent added and the solution sonicated for 10 minutes.1.0mL of 30% H2O2 was added to the solution and allowed to stand for 5 hours, then made up to volume with Diluent, filtered and injected into HPLC system.Fluoxetine peak intensity decreased to 95.2%.ED. N0: 04Effective Date:APPROVED::HPLC ASSAY with DETERMINATION OF META-FLUOXETINE HCl.ANALYTICAL METHOD VALIDATION10 and 20mg Fluoxetine Capsules HPLC DeterminationSAMPLE - OXIDATIONFluoxetine peak:Purity Angle:0.090Match Angle:0.400Purity Threshold:0.154Match Threshold:0.4294.2.Standard solution was prepared as in method SI-IAG-206-02 : about 22mg Fluoxetine HCl were weighed into a 50mL volumetric flask and 25mL Diluent were added. 2mL of 30% H2O2 were added to this solution which was standing for 5 hours, made up to volume with Diluent and injected into the HPLC system.Fluoxetine peak intensity decreased to 95.8%.STANDARD - OXIDATIONFluoxetine peak:Purity Angle:0.083Match Angle:0.416Purity Threshold:0.153Match Threshold:0.4295.SUNLIGHT5.1.Sample solution of IAG-21-001 (2) (20mg/capsule) was prepared as in method SI-IAG-206-02 . The solution was exposed to 500w/hr. cell sunlight for 1hour. The BST was set to 35°C and the ACT was 45°C. The vials were placed in a horizontal position (4mm vials, National + Septum were used). A Dark control solution was tested. A 2%w/v quinine solution was used as the reference absorbance solution.Fluoxetine peak decreased to 91.2% and the dark control solution showed assay of 97.0%. The difference in the absorbance in the quinine solution is 0.4227AU.Additional peak was observed at RRT of 1.5 (2.7%).The total percent of Fluoxetine peak with the degradation peak is about 93.9%.SAMPLE - SUNLIGHTFluoxetine peak:Purity Angle:0.093Match Angle:0.583Purity Threshold:0.148Match Threshold:0.825 ED. N0: 04Effective Date:APPROVED::HPLC ASSAY with DETERMINATION OF META-FLUOXETINE HCl.ANALYTICAL METHOD VALIDATION10 and 20mg Fluoxetine Capsules HPLC DeterminationSUNLIGHT (Cont.)5.2.Working standard solution was prepared as in method SI-IAG-206-02 . The solution was exposed to 500w/hr. cell sunlight for 1.5 hour. The BST was set to 35°C and the ACT was 42°C. The vials were placed in a horizontal position (4mm vials, National + Septum were used). A Dark control solution was tested. A 2%w/v quinine solution was used as the reference absorbance solution.Fluoxetine peak was decreased to 95.2% and the dark control solution showed assay of 99.5%.The difference in the absorbance in the quinine solution is 0.4227AU.Additional peak were observed at RRT of 1.5 (2.3).The total percent of Fluoxetine peak with the degradation peak is about 97.5%. STANDARD - SUNLIGHTFluoxetine peak:Purity Angle:0.067Match Angle:0.389Purity Threshold:0.134Match Threshold:0.8196.HEAT OF SOLUTION6.1.Sample solution of IAG-21-001-(2) (20 mg/capsule) was prepared as in method SI-IAG-206-02 . Equivalent to 20mg Fluoxetine was weighed into a 50mL volumetric flask. 20mL Diluent was added and the solution was sonicated for 10 minutes and made up to volume with Diluent. 4mL solution was transferred into a suitable crucible, heated at 105°C in an oven for 2 hours. The sample was cooled to ambient temperature, filtered and injected into the HPLC system.Fluoxetine peak was decreased to 93.3%.SAMPLE - HEAT OF SOLUTION [105o C]Fluoxetine peak:Purity Angle:0.062Match Angle:0.460Purity Threshold:0.131Match Threshold:0.8186.2.Standard Working Solution (WS) was prepared under method SI-IAG-206-02 . 4mL of the working solution was transferred into a suitable crucible, placed in an oven at 105°C for 2 hours, cooled to ambient temperature and injected into the HPLC system.Fluoxetine peak intensity did not decrease - 100.5%.ED. N0: 04Effective Date:APPROVED::。
带有强迫项的二阶拟线性微分方程的振动准则

带有强迫项的二阶拟线性微分方程的振动准则王培颖【摘要】In this paper,by using integral averaging functions of the formu(t)and the generalized Riccati technique,new interval oscillation criteria areestablished for forced second-order quasi-linear differential equations of the form p (t)y′(t)α-1 y′(t) ( )′+q(t)q(t)β-1 y (t)=e(t).We drop the restriction “φ′(t)≥0”in some existing results.%主要利用一元函数 u(t)型积分平均辅助函数和广义 Riccati 变换技巧,建立带有强迫项的二阶拟线性微分方程 p (t)|y′(t)|α-1 y′(t)()′+q(t)|y (t)|β-1 y (t)=e (t)的新的区间振动准则,去掉了某些已有结果中关于“φ′(t)≥0”的限制。
【期刊名称】《德州学院学报》【年(卷),期】2015(000)004【总页数】5页(P24-28)【关键词】微分方程;区间振动准则;Riccati 变换技巧;强迫项【作者】王培颖【作者单位】广东技术师范学院天河学院,广州 510540【正文语种】中文【中图分类】O175考虑带有强迫项的二阶拟线性微分方程(p(t)|y′(t)|α-1y′(t))′+q(t)|y(t)|β-1y(t)=e(t),t≥t的振动性,其中p,q,e∈C([t0,∞),R),p(t)>0,0<α≤β(α,β是常数),称函数 y(t)∈C1([Ty,∞),R),Ty≥t0,是方程(1)的解,如果p(t)|y′(t)α-1|y′(t)∈C1(Ty,∞)且满足(1)式.主要考虑方程(1)的非平凡解y(t),即sup{|y(t)|:t≥T}>0,T≥Ty,如果它有任意大的零点,方程(1)的非平凡解y(t)称为振动的;否则,称之为非振动的.如果它的所有非平凡解都是振动的,方程(1)称为振动的.当0<α=β时,方程(1)转化为半线性微分方程(p(t)|y′(t)|α-1y′(t))′+q(t)|y(t)|α-1y(t)=e(t≥t当0<α<β时,方程(1)是超半线性微分方程.方程(2)和它的特殊情形(没有强迫项)(p(t)|y′(t)|α-1y′(t))′+q(t)|y(t)|y(t)=0,t≥t的振动性已经被许多学者广泛的研究,可见Elbert[3],Li和Yeh[4].拟线性微分方程(3)和线性微分方程具有类似的性质.例如,当 w=时,方程(3)可转化为方程(4)和α+1阶泛函与线性振动理论中的经典Riccati方程和二次泛函起着相同的作用.当α=1时,方程(2)转化为带有强迫项的线性微分方程对于方程(6),1999年Wong[5]得到下面结果定理1 如果对任意T≥t0,存在T≤s1<t1≤s2<t2,使得令D(si,ti)={u∈C1[si,ti]:u(t)≠0, u( si)=u( ti)=0};i=1,2假定存在u∈ D( si,ti),使得则方程(6)是振动的.上述定理改进了没有强迫项的微分方程的结论.2002年,Li和Cheng [6]用类似的方法和一个正的、非减的函数φ∈C1([t0,∞),R)可以将定理1的结论推广到方程(2)的振动性.定理2 如果对任意T≥t0,存在T≤s1<t1≤s2<2,使得假定存在H∈D(si,ti)和一个正的、非减的函数φ∈C1([t0,∞),R)使得则方程(2)是振动的.当α=1,φ(t)=1,H(t)=t)时,(8)式转化为(7)式.(8)式和(5)式没有联系,并且定理2不能应用于α>1的情形.2007年,Zheng和Meng[7]将定理2推广到方程(1).定理3 如果对任意T≥t0,存在T≤s1<t1≤s2<t2,使得假定存在H∈si,ti)和一个正的、非减的函数φ∈C1([t0,∞),R)使得其中,约定00=1,则方程(1)是振动的.上述定理1-3所用的积分平均辅助函数为一元的.1999年,Manojlovic[8]利用Philos[9]提出的二元函数H(t,s)型积分平均辅助函数,研究了方程(3)的振动性,其中的一个结果如下.定理4 假设存在一个定义在 D={(t,s):t≥s≥t0}→R上的连续函数H,使得H(t,t0)=0,t≥t0,H(t,s)>0,(t,s)∈D.是定义在D上的非负连续函数.如果存在一个正的、非减的函数ρ∈C1([t0,∞),R),使得成立,则方程(3)是振动的.2001年,Wang[10]将定理4中的限制“ρ′(t)≥0”去掉.2004年,Wang和Yang[11]利用二元函数H(t,s)型积分平均辅助函数,研究了方程(3)的区间振动性,其中的一个结果如下.定理5 假设存在c∈(a,b),ρ∈([t0,∞),(0,∞)]),使得则方程(3)的每一个解在(a,b)上至少有一零点.将利用一元函数u(t)型积分平均辅助函数,建立方程(1)的新的区间振动准则.首先给出一个不等式,可见文献[29].引理1 假设X≥0,等号成立有且只有 X=Y.下面给出主要结果.定理6 如果对任意T≥t0,存在T≤s1<t1≤s2<t2,使得令D(si,ti)={u∈ C1[si,ti]:uα+1(t)>0,t∈( si,ti),u(si)=u(ti)=0};i=1,2假定存在H∈D(si,ti)和一个函数φ∈C1([t0,+∞),(0,+∞))使得p(t)dt>0,i=1,2其中约定00=1,则方程(1)是振动的.证明反证法.假设方程(1)有非平凡非振动解 y(t),t∈[t0,∞),则存在T0≥t0使得当t≥Ty(t)≠0.不妨设y(t)>0.令对(10)式求导并利用方程(1)得由假设取t1>s1≥t0,因此在I1=[s1,t1],e(t)≤0,令F′(x)=0,得所以由(11)(12)得即把(13)式两边同乘以 Hα+1(t)并从si到ti积分,而且H(si)=H(ti)=0,得到令据引理1所以∫tisiφ(t) Q e(t) H α+1(t)dt≤∫ tisit)p(t)即当i=1时(14)与(9)矛盾,同理当 y(t)<0.t≥T0>t0,做和(10)式相同的Riccati变换得当 i=2时与(9)式矛盾,定理得证.推论1 若在定理6中令φ(t)≡1,式子(9)变为Qi(H)=∫tisi[Qe(t)Hα+1(t)-p(t)则方程(1)是振动的.推论2 若在定理6中令φ(t)≡1,α=β>0,式子(9)变为Qi(H)=∫tisi[q(t)Hα+1(t)-p(t)则方程(2)是振动的.注定理6去掉了定理2和定理3中关于“φ′(t)≥0”的限制.例考虑带有强迫项的拟线性微分方程方程其中λ,γ都是常数,且λ,γ>0在定理6中取,则取,对任意T≥1,当nπ=2kπ≥T,s1=2kπ,t1=(2k+1)π,于是由定理6得故即因此当时.同理,取s2=(2k+1)π,t2=(2时.所以据定理6得当0<γ<时原方程是振动的.[1]parisonAnd Oscillation Theory of Linear Differential Eq uations[M].New York:Academic Press,1968.[2]燕居让.常微分方程的振动理论[M].太原:山西教育出版社,1992.[3]A.Elbert.A half-linear second order differential equation,Colloq.Math.Soc.Janos Bolyai:Qual itative Theory of Differential Equations[J].Szeged,1979:153-180.[4]H.J.Li,CC.Yeh.Sturmian comparison theorem for half-linear second-order differential equations[J].Proc.Royal Soc. Edinburgh 125A,1995:1193-1204.[5]J.S.W.Wong.Oscillation criteria forA second-order linear differential equation[J].J.Math.Anal.Appl.,1999,231:235-240. [6]W.T.Li,S.S.Cheng.An oscillation criterion for nonhomogeneous half-linear differential equations[J].Appl.Math.Lett.2002,15:259-263.[7]Zhaowen Zheng,Fanwei Meng.Oscillation criteria for forced second-order quasi-linear differential equations[J]put.Modelling,2007,45:1-2,215-220.[8]J.V.Manojlovi’c.Oscillation criteria for second-order half-linear differential equations[J]put.Modelling,1999,30:109-119.[9]Ch.G.Philos.Oscillation theorems for linear differential equations of seco nd order[J].Arch.Math.(Basel),1989,53:482-492.[10]Qi-Ru Wang.OscillationAndAsymptotics for second-order half-linear differential equations[J]put.,2001,122:2,253-266. [11]Qi-Ru Wang,Qi-Gui Yang.Interval criteria for oscillation of second-order half-linear differential equations[J].J.Math.Anal.Appl.,2004,291:1,224-236.[12]Y.G.Sun.New Kamenev-type oscillation criteria for second-order nonlinear differential equations with damping[J].J.Math.Anal.Appl.,20 04,291:341-351.[13]Qi Long,Qi-Ru Wang.New oscillation criteria of second-order nonlinear differential equations[J]put.,2009,212:2,35 7-365[14]Yin-Lian Fu,Qi-Ru Wang. Oscillation criteria for second-order nonlinear damped differential equations.Dynam.SystemsAppl.18(200 9),no.3-4,375-391.[15]D.Cakmak,A.Tiryaki,Oscillation criteria for certain forced second-order nonlinear differential equations[J].Appl.Math.Lett.,2004,17: 275-279.[16]J.Jaros,T.Kusano,A Picone type identity for second order half-linear differential equations[J].Acta enian. 1999, 68 (1):137-151.[17]J.Jaros,T.Kusano,N.Yoshida.Generalized Picone’s formulaAnd forced o scillation for in quasilinear differential equations of the secondorder[J].Arch.Math.(Bero),2002,38:53-59.[18]A.Wintner.A criterion of oscillatory stability[J].Quart.Appl.Math.,1949,7: 115-117.[19]I.V.Kamenev.Integral criterion for oscillation of linear differential equati ons of second order[J].Mat.Zametki,1978,23:249-251.[20]parison theorems for linear differential equations of se cond order[J].Proc.Amer.Math.Soc.,1962,13:603-610.[21]Q.Kong.Interval criteria for oscillation of second-order linear differential equation[J].J.Math.Anal.Appl.,2001,258:244-257. [22]H.L.Hong,W.C.Lian,C.C.Yeh.The oscillation of half-linear differential equations withAn oscillatory coefficient[J]put. Modelling,1996,24:77-86.[23]J.S.W.Wong.Second order nonlinear forced oscillations[J].SIAM J.Math. Anal.,1988,19:667-675.[24]Qi-Ru Wang.Oscillation criteria for nonlinear second order damped differentia l equations[J].Acta Math.Hungar.,2004,102:117-139.[25]Qi-Ru Wang.Interval criteria for oscillation of certain second order nonlinear d ifferential equations[J].Dynam.Cont.Discrete Impulsive Syst.SeriesA:Math.A nal.,2005,12:769-781.[26]Qi-Ru Wang.Interval criteria for oscillation of second-order nonlinear differential equations[J]put.Appl.Math.,2007,205:1,2 31-238.[27]H.W.Wu,Q.R.Wang,Y.T.Xu.OscillationAndAsymptotics for nonlinear seco nd-order differential equations[J].Comput.Math.Appl.,2004,48:61-72. [28]Q.G.Yang.Interval oscillation criteria forA forced second order nonlinea r ordinary differential equations with oscillatory potential[J].Appl.Math.Co mput.,2003,135:49-64.Key words:differential equations; interval oscillation criteria; generalized ric cati transformation; forcing term。
OSCILLATION CRITERIA FOR SECOND ORDER FUNCTIONAL NEUTRAL DIFFERENTIAL EQUATIONS

OS C ILLAT IO N C R IT E R IA F OR SE C ON DOR D E R F U N C T IO N AL NE U T R AL D IF F E R E N T I AL E Q UAT IO N SJ ing Shao(De pt.of Ma th.,J ining Unive rsity,Quf u 273155,Sha ndong,E-m ail:shaojing99500@)Nan Wang,Fanwei Meng(De pt.of Ma th.,Qufu Nor m a l Univer sity,Quf u 273165,Sha ndong)Ann.of Di .Eqs.29:2(2013),188-194Ab st ra ctB y th e sta nda rd int egr a l a ver a ging te c h nique,we obta in som e oscilla tion cr it eria for a second or der funct iona l neu tr al di er entia l equa tion.Our r esu lt s a r e mor e ge nera l t ha n th ose in B.B acul kova ,J.D ˇz ur in a [2].An exa mple is p rovided t o illu st ra t e the r eleva nce of our th eor em s.K ey wo r d s oscilla t ion;second-or der;funct iona l neutr a l di e rent ia l equ at ion;in -t egra l a ver a ging2000M a t h e m a t ic s Su b je c t C la ssi c a t io n 34C 101In t r o d u ct io nIn this paper,we are concerned wit h t he oscillat ory behavior of second order nonlinear neutral delay di erential equat ion[r (t)φ(x(t ))[x(t)+p(t )x(τ(t))]′]′+q(t )f (t,x(t ),x(σ(t)))=0,t ≥t 0,(1.1)where r,p,τ,σ∈C 1([t 0,∞),R ),φ∈C (R ,R ),q ∈C ([t 0,∞),R ),f ∈C (R 3,R )and(C1)r (t)>0,0≤p(t)≤p 0<+∞,q(t)≥0and q(t )is not ident ically zero for large t ;(C2)there exist t wo cont inuously di erent iable funct ions ρ1(t)and ρ2(t )such t hat forarbit rary solut ion x(t )t o equat ion (1.1),0<ρ1(t )≤φ(x(t ))≤ρ2(t ),ρ′1(t)≤φ′(x(t )),for t ≥t 0wit h lim t →+∞∫t t 0[r(s )ρ2(s )]1ds =∞;(C3)for all t ≥t 0,σ(t )≤t ,σ′(t )>0andlim t →+∞σ(t )=∞;(C4)for all t ≥t 0,τ(t )≤t ;(C5) f (t ,x,y)y≥l(t )>0,for y =0,l(t)∈C ([t 0,∞)),xf (t,x,y)>0,for x =0.Let D 0={(t,s ):t 0≤s <t <+∞}and D ={(t,s):t 0≤s ≤t <+∞}.Suppose that H ∈C (D ,R )sat is es t he following t hree condit ions:(A1)H (t ,t )=0,t ≥t 0;H (t,s)>0,t >s ≥t 0;(A2)H has a cont inuous and non-posit ive part ial derivat ive on D 0and h :D 0→R isa cont inuous funct ion wit h Hs(t,s)=h(t ,s )√H (t,s)for all (t ,s )∈D 0;This resea rch was pa rt ia lly suppor ted by t he NNSF of C hina (Gr a nt 11171178a nd 11271225),Science and Technology P ro jec t of High Schools of Sha ndong P r ovinc e (Gra nt J 12LI52)a nd P ro-gra m f or Scie nt i c R esea r ch Innova tion Te am in Colleges and Universities of Sha ndong P rovince.Ma nuscr ipt r eceived April 8,2011;R evised Novemb er 5,2012188No.2J.Shao,etc.,OSCILLATION CRIT ERIA FOR NE UT RAL DIFF.E QS.189(A3)0<infs≥t0[lim inft→+∞H(t,s)H(t,t0)]≤+∞.Recent ly,more and more int erest has been paid t o t he oscillat ion of solut ions to di erent classes of nonlinear second-order di erent ial equat ions wit h damping t erm since t hey are encount ered in m any applicat ions in science and t echnology,such as,describing distribut ed net works wit h losses t ransm ission lines,studying vibrat ing m asses at t ached t o an elast ic bar,as well as in som e variat ional problems,see[7].In general,t he invest igat ion of neutral di erent ial equat ions is m ore di cult t han t hat of ordinary di erent ial equat ions,although cert ain similarit ies in t he behavior of solut ions to ordinary and neut ral di erential equat ions can be observed,see[1-10].M.M.A.El-Sheikh and R.Sallam[1]showed t hat p(t)=0t oget her wit hlim sup t→+∞1H(t,t0)∫tt0[H(t,s)p(s)14r(s)K q′(s)h2(t,s)]ds=∞guarant ees t he oscillation of t he neut ral equat ion(r(t)ψ(u(t))u′(t))′+p(t)f(u(q(t)))=0.By t he comparison t heorems, B.Bacul kova and J.Dˇzurina[2]derived a number of general oscillat ion crit eria for a nonlinear neut ral di erent ial equat ion(r(t)(x(t)+p(t)x(tτ))′)′+q(t)f(x(tσ))=0.Recent ly,M ust afa Hasanbulli and Yuri V.Rogovchenko[8]proved several oscillat ion crit eria for equat ion(1.1)wit hφ(t)=1,τ(t)=tτ,using int egral averaging technique.In t his paper,using t he st andard int egral averaging t echnique,we can obt ain more useful oscillat ion crit eria t han t hose of t he above papers.2M ain T h eor em sT h eor em 2.1Let conditions(C1)-(C5)be satis ed,h and H s atisfy conditions(A1) and(A2).Suppos e t hat t here exists a funct ionβ∈C1([t0,+∞),R)such that,for someα≥1,lim sup t→+∞1H(t,t0)∫tt0[H(t,s)ψ(s)αv(s)r(σ(s))ρ2(σ(s))4σ′(s)h2(t,s)]ds=∞,(2.1)whereψ(t)=v(t)[q(t)l(t)(1p(σ(t)))(r(t)ρ1(t)β(t))′+σ′(t)(r(t)ρ1(t))2r(σ(t))ρ2(σ(t))β2(t)](2.2)andv(t)=exp (2∫tσ′(s)r(s)ρ1(s)β(s)r(σ(s))ρ2(σ(s))ds).(2.3)Then,equat ion(1.1)is oscil latory.P r oof Let x(t)be a non-oscillat ory solution t o equat ion(1.1).Wit hout loss of ge-nerality,we assume t hat x(t)is an eventually posit ive solut ion since t he case when x(t) is event ually negat ive can be t reat ed analogously.Then,t here exist s a t1≥t0such t hat x(t)>0,x(τ(t))>0,x(σ(t))>0,for all t≥t1.De nez(t)=x(t)+p(t)x(τ(t)),t≥t1.190ANN.OF DIF F.E QS.Vol.29Obviously,for t≥t1,z(t)≥x(t)>0,f(t,x(t),x(σ(t)))>0,and r(t)φ(x(t))z′(t)is decrea-sing because(r(t)φ(x(t))z′(t))′=q(t)f(t,x(t),x(σ(t)))<0.F irst ly,we show t hat z′(t)>0for all t≥t1.Otherwise,t here exist s a t2≥t1such that z′(t)<0,for all t≥t2.Since r(t)φ(x(t))z′(t)is decreasing,we can getr(t)φ(x(t))z′(t)≤r(t2)φ(x(t2))z′(t2)<0,t≥t2.(2.4) It follows from(2.4)and(C2)t hat for all t≥t2,z′(t)≤r(t2)φ(x(t2))z′(t2)1r(t)φ(x(t))≤r(t2)φ(x(t2))z′(t2)1r(t)ρ2(t).Int egrat ion of t he above inequalit y from t2t o t yieldsz(t)≤z(t2)+r(t2)φ(x(t2))z′(t2)∫tt21r(s)ρ2(s)ds.(2.5)Let ting t→+∞and using(C2),limt→+∞z(t)=∞which cont radict s t he fact t hat z(t)>0. So z′(t)>0for all t≥t1.Sincex(t)=z(t)p(t)x(τ(t))≥(1p(t))z(t),t≥t1,and limt→∞σ(t)=∞,t here exists a t3≥t1such t hatx(σ(t))≥(1p(σ(t)))z(σ(t)).(2.6) By(C5),we can obt ain that(r(t)φ(x(t))z′(t))′+q(t)l(t)x(σ(t))≤0.(2.7) It follows from(2.6)and(2.7)t hat(r(t)φ(x(t))z′(t))′≤q(t)l(t)(1p(σ(t)))z(σ(t)).(2.8)Letu(t)=v(t)r(t)ρ1(t)[z′(t)z(σ(t))+β(t)].(2.9)Di erent iat ing(2.9)and using(2.8),we conclude t hat,for all t≥t3,u′(t)=v′(t)v(t)u(t)+v(t)(r(t)ρ1(t)z′(t))′z(σ(t))+v(t)(r(t)ρ1(t)β(t))′v(t)r(t)ρ1(t)σ′(t)z′(σ(t))z′(t)(z′(t)z(σ(t)))2≤v′(t)v(t)u(t)v(t)q(t)l(t)(1p(σ(t)))+v(t)(r(t)ρ1(t)β(t))′v(t)σ′(t)(r(t)ρ1(t))22[u(t)1β(t)]2No.2J.Shao,etc.,OSCILLATION CRIT ERIA FOR NE UT RAL DIFF.E QS.191=v(t)q(t)l(t)(1p(σ(t)))+v(t)(r(t)ρ1(t)β(t))′σ′(t)u2(t)r(σ(t))ρ2(σ(t))v(t)v(t)σ′(t)(r(t)ρ1(t))2r(σ(t))ρ2(σ(t))β2(t)=ψ(t)σ′(t)u2(t)r(σ(t))ρ2(σ(t))v(t),t his isu′(t)≤ψ(t)σ′(t)u2(t)2,(2.10) whereψ(t)is de ned by(2.2)and(2.3).M ult iplying(2.10)by H(t,s)and int egrat ing it from t3t o t,we obt ain,for allα≥1,∫t t3[H(t,s)ψ(s)αv(s)r(σ(s))ρ2(σ(s))4σ′(s)h2(t,s)]ds≤∫tt3[H(t,s)u′(s)H(t,s)σ′(s)u2(s)r(σ(s))ρ2(σ(s))v(s)αv(s)r(σ(s))ρ2(σ(s))4σ′(s)h2(t,s)]ds≤H(t,t3)u(t3)∫tt3(α1)σ′(s)u2(s)H(t,s)2ds∫t t3(√σ′(s)u2(s)H(t,s)αr(σ(s))ρ2(σ(s))v(s)√αr(σ(s))ρ2(σ(s))v(s)h2(t,s)4σ′(s))2ds≤H(t,t0)|u(t3)|, correspondingly,∫t t0[H(t,s)ψ(s)αv(s)r(σ(s))ρ2(σ(s))4σ′(s)h2(t,s)]ds≤H(t,t0)[|u(t3)|+∫t3t0|ψ(s)|ds].(2.11)By virt ue of(2.11),we havelim sup t→+∞1H(t,t0)∫tt0[H(t,s)ψ(s)αv(s)r(σ(s))ρ2(σ(s))′h2(t,s)]ds≤|u(t3)|+∫t3t0|ψ(s)|ds<+∞,which cont radict s(2.1).This complet es t he proof.Some useful oscillat ion crit eria can be derived from Theorem2.1by choosing suit able funct ions H and h.For example,let H(t,s)=(t s)n,where n≥1is an int eger,so h(t,s)=n(t s)(n2)/2.Thus,we get t he following oscillat ion criterion.C or ollar y 2.1Suppose t hat t here exists a functionβ∈C1([t0,∞),R)such that,for some int eger n>1a nd someα≥1,lim sup t→+∞1t n∫tt0(t s)n2[(t s)2ψ(s)αv(s)r(σ(s))ρ2(σ(s))′n2]ds=+∞,whereψ(t)and v(t)are a s in T heorem2.1.Then,equat ion(1.1)is oscillatory.192ANN.OF DIF F.E QS.Vol.29Ex am ple 2.1Consider t he following second order neutral di erent ial equat ion for t ≥1[arct an x +πt 2(x(t)+t t +1x(t1))′]′+1t +2t (1+x)2x(t 2)=0.Let r (t )=1/t 2,φ(x)=arct an x +π,p(t)=t /(t +1),τ(t )=t 1,σ(t)=t/2,q(t)=1/(t +2),f (t,x,y)=t (1+x)2y.Choose ρ1(t )=1,ρ2(t)=6,l(t)=t ,β(t )=48/t.Obviously,(C1)-(C5)are sat is ed.Then,v(t )=t 2,ψ(t)=2t 3/(2+t )296/t 2,andlim sup t →+∞1t2∫t 1{(ts )2[2s 3(2+s )296s 2]48α}ds =+∞.An applicat ion of Corollary 2.1wit h n =2est ablishes t he oscillat ion of this equat ion.T he or em 2.2Let conditions (C1)-(C5)be satis ed,h and H satis fy condit ion (A1)-(A3).Suppose that there exi sts a function β∈C 1([t 0,+∞),R)and ∈C ([t 0,+∞),R )such tha t,for some α≥1and al l T ≥t 0,lim supt →+∞1H (t ,T )∫t T[H (t ,s )ψ(s )αv(s)r (σ(s ))ρ2(σ(s))4σ′(s )h 2(t ,s )]ds ≥(T),where ψ(t)and v(t )are as in Theorem 2.1.Suppose fur ther t hatlim supt →+∞∫tt 0σ′(s)2+(s)v(s )r (σ(s ))ρ2(σ(s ))ds =+∞,(2.12)where +(t)=m ax{(t),0}.Then,equat ion (1.1)is oscillatory.P r oof Let x(t )be a non-oscillat ory solut ion t o equat ion (1.1).Without loss of ge-neralit y,we assume t hat x(t )is an event ually positive solut ion since t he case when x(t )is event ually negat ive can be treat ed analogously.Then,there exist s a t 1≥t 0,such that x(t)>0,x(τ(t ))>0,x(σ(t))>0,for all t ≥t 1.Similar t o t he proof of Theorem 2.1,w e get ,for all t ≥t 3and all α≥1,(t 3)≤lim supt →+∞1H (t ,t 3)∫t t 3[H (t,s)ψ(s )αv(s )r (σ(s ))ρ2(σ(s ))4σ′(s )h 2(t ,s )]ds≤u(t 3)lim inft →+∞1H (t,t 3)∫tt 3(α1)σ′(s )u 2(s)H (t ,s )αv(s )r (σ(s ))ρ2(σ(s ))ds.This is(t 3)+lim inft →+∞1H (t,t 3)∫tt 3(α1)σ′(s )u 2(s )H (t ,s )αv(s )r (σ(s ))ρ2(σ(s ))ds ≤u(t 3).So(t 3)≤u(t 3)(2.13)andlim inft →+∞1H (t ,t 3)∫tt 3σ′(s )u 2(s )H (t,s)v(s )r (σ(s ))ρ2(σ(s ))ds ≤α(α1)[u(t 3)(t 3)]<+∞.(2.14)No.2J.Shao,etc.,OSCILLATION CRIT ERIA FOR NE UT RAL DIFF.E QS.193 Now we prove t hat∫+∞t3σ′(s)u2(s)v(s)r(σ(s))ρ2(σ(s))ds<+∞.(2.15)Suppose t o t he cont rary t hat∫+∞t3σ′(s)u2(s)v(s)r(σ(s))ρ2(σ(s))ds=+∞.(2.16)any su cient ly small posit ive const antε>0,t here exist s a t4≥t3such t hat for all t≥t4∫t t3σ′(s)u2(s)v(s)r(σ(s))ρ2(σ(s))ds≥1ε2.(2.17)According to condit ion(A3),t here exists a t5≥t4such t hat for all t≥t5,lim inf t→+∞H(t,s)H(t,t0)>ε>0.(2.18)So,1H(t,t3)∫tt3σ′(s)u2(s)H(t,s)v(s)r(σ(s))ρ2(σ(s))ds=1H(t,t3)∫tt3H(t,s)d(∫st3σ′(ξ)u2(ξ)v(ξ)r(σ(ξ))ρ2(σ(ξ))dξ)≥1ε21H(t,t3)∫tt5(H(t,s)s)ds≥1ε2H(t,t5)H(t,t0)>1ε.Sinceεis an arbit rary posit ive const ant,we obt ainlim inf t→+∞1H(t,t3)∫tt3H(t,s)σ′(s)u2(s)v(s)r(σ(s))ρ2(σ(s))ds=+∞,which cont radict s(2.14).Consequent ly,(2.15)holds.By virt ue of(2.13),∫+∞t3σ′(s)2+(s)v(s)r(σ(s))ρ2(σ(s))ds≤∫+∞t3σ′(s)u2(s)v(s)r(σ(s))ρ2(σ(s))ds<+∞,which cont radict s(2.12).Therefore,equat ion(1.1)is oscillat ory.By choosing H(t,s)=(t s)n,Theorem2.2can be simpli ed as t he following oscillat ioncrit erion for equat ion(1.1).We observe t hat condit ion(A3)holds because limt→+∞H(t,s)H(t,t0)=1.C or ollar y2.2Assume tha t t here exis t functionsβ∈C1([t0,+∞),R)and∈C([t0,+∞), R)such that,for som eα≥1,all T≥t0and some integer n>1,lim sup t→+∞1t n∫tT(t s)n2[(t s)2ψ(s)αv(s)r(σ(s))ρ2(σ(s))4σ′(s)n2]ds≥(T),whereψ(t),v(t)and+(t)are as in Theorem2.2.Suppose furt her tha t(2.12)holds.Then, equat ion(1.1)is oscillatory.Sim ilar t o t he analysis of Theorem2.2,we obt ain t he following oscillat ion crit eria for equat ion(1.1).194ANN.OF DIF F.E QS.Vol.29T he or em 2.3Assume that t here exist functi ons h,H:D→R satisfy condition(A1)-(A3),β∈C1([t0,+∞),R)and∈C([t0,+∞),R)such t hat,for s omeα≥1and all T≥t0,lim inf t→+∞1H(t,T)∫tT[H(t,s)ψ(s)αv(s)r(σ(s))ρ2(σ(s))4σ′(s)h2(t,s)]ds≥(T),whereψ(t),v(t)and+(t)are de ned as in Theorem2.2.Suppose further t hat(2.12)holds. Then,equati on(1.1)is oscillator y.Sim ilar t o Corollary2.2,we can obt ain t he following result by choosing H(t,s)=(t s)n.C or ol lar y2.3Assume that t here exist functionsβ∈C1([t0,+∞),R)and∈C([t0,+∞), R)such that,for someα≥1,all T≥t0and some integer n>1,lim inf t→+∞1t n∫tT(t s)n2[(t s)2ψ(s)αv(s)r(σ(s))ρ2(σ(s))4σ′(s)n2]ds≥(T),whereψ(t),v(t)and+(t)are de ned as in Theorem2.2.Suppose further t hat(2.12)holds. Then,equati on(1.1)is oscillator y.In conclusion,we not e t hat t he result s given here can be very easily generalized t o ob-t ain oscillat ion crit eria for some funct ional di erent ial equat ions,involving several ret arded argum ent s.We also note t hat t he main result s of[1-3]fail t o study(1.1),t hus our result s are more general t han t hose of[1-3].R eferen ces[1]M.M.A.El-Sheikh,R.Sa lla m,Oscilla tion cr iter ia for second or der fun ction al di e rent ia l equa-tions,Appl.Ma th.C omput.,115(2000),113-121.[2] B.B a cul kova,J.Dˇzurina,Oscillat ion theor ems f or second or der neu tr al d i erent ia l equa tions,Com put.M ath.Appl.,61(2011),94-99.[3]Y.R ogovchen ko,F.Tunc ay,Oscilla t ion cr it er ia for secon d or der nonlinea r d i erent ia l equat ionswit h da mping,Nonlinea r Anal.,TM A,69(2008),208-221.Zbl1147.34026.[4]R.Xu, F.Men g,Som e n ew oscillat ion crit eria for second or der quasi-linea r neut ra l delaydi er ential equa tions,Appl.M ath.C omput.,182(2006),797-803.Zbl1115.34341.[5]R.Xu, F.Me ng,Oscillat ion cr iter ia for second ord er qua si-line ar neut ra l dela y di er entialequat ions,Appl.M ath.C om put.,192(2007),216-222.Zbl1131.34319.[6]J.Ya n,Osc illa tion the or em s for second orde r line ar di er ential equa tion with d am ping,P r oc.Am.Ma th.Soc.,98(1986),276-282.[7]J.K.Hale,The or y of Fu nctional Di er ential Equa tions,Spr inger-Ver la g,New York,1977.[8]Must afa Ha sanbulli,Yuri V.R ogovchenko,Oscillat ion cr it er ia for second ord er nonlinea r n eu-tr a l di er ent ia l equa t ions,Appl.Ma put.,215(2010),4392-4399.[9]J.Dˇz ur ina,ckova,Oscillat ion re sults for second-or der nonlinea r di er ent ia l equa tions, Stud.Univ.ˇZilina Ma th.Ser.,17(2003),79-86.[10]W.Shi,P.Wa ng,O scilla tion crit eria of a cla ss of second-or der neu tr al funct iona l di er entialequat ions,Appl.M ath.C om put.,146(2003),211-226.(edited by Liangwei Huang)。
crystal_oscillator_design_and_negative_resistance

particular region of their I-V curve. These active devices exhibit a dip in the I-V curve such that an increase in voltage results in a decrease of current. This behavior, called negative resistance, is very useful in creating microwave oscillators (in the case of a tunnel diode). The important concept to remember when considering these types of devices is that no other passive components are required to create the effect of negative resistance. There are bias networks that are necessary to select the region of operation and associated feedback networks that create an oscillator network, but it is the active device itself that supplies the negative resistance. The I-V curve for a tunnel diode is illustrated in Fig. 1.
2
This has created a new problem since the crystal vendor, semiconductor manufacturer, and the end user -- or customer -- determines the final design of the oscillator. They each may have different design guidelines and measurement methodologies. Negative resistance is a very important oscillator design parameter that can change based on the design guideline or measurement methodology employed. The end customer or isolated vendor may specify parameters that are not compatible for an overall successful oscillator design. The purpose here is to theoretically explain and quantify negative resistance when used in terms of oscillator design: such that the crystal vendor, semiconductor manufacturer, and customer can have a common platform for communication and collaboration in designing a robust crystal oscillator. The first place to start is by defining a negative resistance model (see Fig. 2) for an oscillator and the basic requirements for oscillation to take place. [²]
化学检测相关中英文对照
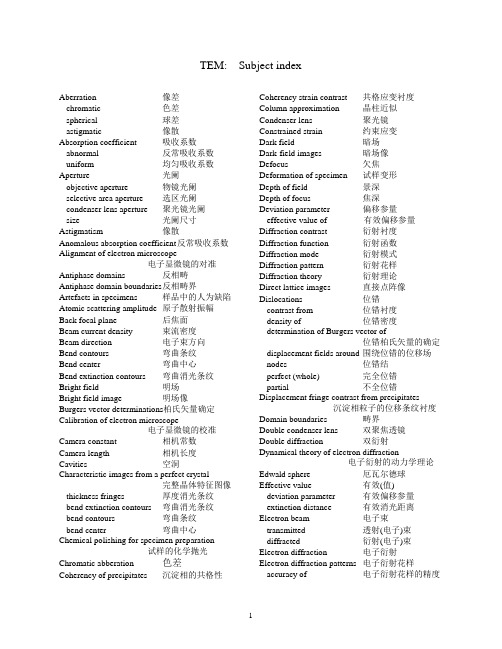
TEM: Subject indexAberration 像差chromatic 色差spherical 球差astigmatic像散Absorption coefficient 吸收系数abnormal 反常吸收系数uniform 均匀吸收系数Aperture 光阑objective aperture 物镜光阑selective area aperture 选区光阑condenser lens aperture 聚光镜光阑size 光阑尺寸Astigmatism 像散Anomalous absorption coefficient 反常吸收系数Alignment of electron microscope电子显微镜的对准Antiphase domains反相畴Antiphase domain boundaries 反相畴界Artefacts in specimens 样品中的人为缺陷Atomic scattering amplitude 原子散射振幅Back focal plane 后焦面Beam current density 束流密度Beam direction 电子束方向Bend contours 弯曲条纹Bend center 弯曲中心Bend extinction contours 弯曲消光条纹Bright field 明场Bright field image 明场像Burgers vector determinations柏氏矢量确定Calibration of electron microscope电子显微镜的校准Camera constant 相机常数Camera length 相机长度Cavities 空洞Characteristic images from a perfect crystal完整晶体特征图像thickness fringes 厚度消光条纹bend extinction contours 弯曲消光条纹bend contours 弯曲条纹bend center 弯曲中心Chemical polishing for specimen preparation试样的化学抛光Chromatic abberation色差Coherency of precipitates 沉淀相的共格性Coherency strain contrast 共格应变衬度Column approximation 晶柱近似Condenser lens 聚光镜Constrained strain 约束应变Dark field 暗场Dark-field images 暗场像Defocus 欠焦Deformation of specimen 试样变形Depth of field 景深Depth of focus 焦深Deviation parameter 偏移参量effective value of 有效偏移参量Diffraction contrast 衍射衬度Diffraction function 衍射函数Diffraction mode 衍射模式Diffraction pattern 衍射花样Diffraction theory 衍射理论Direct lattice images直接点阵像Dislocations 位错contrast from 位错衬度density of 位错密度determination of Burgers vector of位错柏氏矢量的确定displacement fields around 围绕位错的位移场nodes 位错结perfect (whole) 完全位错partial 不全位错Displacement fringe contrast from precipitates沉淀相粒子的位移条纹衬度Domain boundaries 畴界Double condenser lens 双聚焦透镜Double diffraction 双衍射Dynamical theory of electron diffraction电子衍射的动力学理论Edwald sphere 厄瓦尔德球Effective value 有效(值)deviation parameter 有效偏移参量extinction distance 有效消光距离Electron beam 电子束transmitted 透射(电子)束diffracted 衍射(电子)束Electron diffraction 电子衍射Electron diffraction patterns 电子衍射花样accuracy of 电子衍射花样的精度calibration of 电子衍射花样的校准effects of crystal shape电子衍射花样的形状效应indexing of 电子衍射花样的标定Kikuchi lines 菊池线花样polycrystalline ring 多晶环状衍射花样single crystal spot 单晶斑点衍射衍射streaks on 电子衍射花样的芒线Electron gun 电子枪Electron microscope 电子显微镜analytical 分析电镜attachments for 电子显微镜的附件high resolution 高分辩电镜magnification of 电子显微镜的放大倍数ray diagrams for 电子显微镜的光路图resolving power of 电子显微镜的分辩力transmission 透射电镜Electron microscopy 电子显微学(术) analytical 分析电子显微学(术) conventional 常规电子显微学high resolution 高分辩电子显微学(术) transmission 透射电子显微学Electron wavelengths 电子波长Electropolishing for specimen preparation电解抛光制备电镜试样Extinction 消光Extinction contours 消光条纹Extinction distance 消光距离Extrinsic插入型的Faults 缺陷Focus distance 焦距Foil thickness 薄膜厚度measurement of 膜厚测量Fringes 条纹displacement 位移条纹magnetic domain wall 磁畴壁条纹moire Moirre条纹precipitates, from 由沉淀相粒子引起的条纹stacking fault 层错条纹thickness 厚度条纹Goniometer stage 测角台Heating stages 加热台High order Laue Zone 高阶劳厄区High resolution electron microscopy 高分辩电镜Identification of precipitates沉淀相鉴别Illumination of specimen 试样照明contamination by 试样照明引起的污染damage by 试样照明引起的破坏Image 图像bright field 明场像dark field 暗场像intermediate 中间像rotation of 像转Image contrast 图像衬度origin of 图像衬度的来源antiphase domains, from 反相畴图像衬度antiphase domain boundaries, from反相畴界图像衬度cavities, from 空洞图像衬度dislocations, from, 位错的衬度dipoles 位错偶极子的衬度double images 位错双线衬度edges 刃型位错衬度general dislocation 一般位错的衬度inclined 倾斜位错的衬度invisibility criteria for 位错不可见位错loops 位错圈的衬度oscillation effects at 位错衬度的振荡效应partial 不全位错的衬度screws 螺型位错的衬度superdislocations 超位错的衬度surface relaxation effects位错衬度的表面松弛效应visibility rules for 位错不可见规律width of images 位错图像宽度partial dislocations 不全位错的衬度Frank Frank位错的衬度Schockley Schockley位错的衬度precipitates,from, 沉淀相粒子的衬度coherency strain field images沉淀相粒子的共格应变场衬度dislocation ring contrast沉淀相粒子的位错圈衬度displacement fringe contrast沉淀相粒子的位移条纹衬度matrix contrast 沉淀相粒子的基体衬度moire fringes 沉淀相粒子的Morrie条纹衬度orientation contrast 沉淀相粒子的取向衬度structure factor contrast沉淀相粒子的结构因子衬度visibility of 沉淀相粒子的可见性stacking faults, from, 层错引起的衬度determination of nature of 层错性质的确定twin boundaries, from 孪晶界的衬度Image force 镜像力Image formation 图像形成(成像) Ab by’s theory of Abby成像理论Image function 像函数Image mode 图像模式Image plane 像平面Image rotation 像转Inclusions 夹杂Indexing of electron patterns 电子衍射花样标定trier and error 尝试校核法known camera constant 已知相机长度standard diffraction patterns 标准衍射谱法computer simulation 计算机标定法ambiguous 不唯一性Inelastic scattering 非弹性散射Interface contrast 界面衬度Intermediate image 中间像Intermediate image plane 中间像平面Intrinsic 抽出型的Ion bombardment technique for specimen preparation 离子束轰击制样法Kikuchi pattern 菊池线花样Kikuchi lines 菊池线Kikuchi maps 菊池线图Kinematical theory of diffraction contrast运动学衍衬理论Lattice image 点阵像two beam 双束点阵(平面)像many beam 多束点阵像structure image 结构像Lattice plane spacing 点阵面间距Laue circle 劳厄园Laue zones 劳厄区high order 高阶劳厄区Line defect 线缺陷Line of no contrast 无衬度线Magnetic lens 电磁透镜aberrations of 电磁透镜的像差focal length of 电磁透镜的焦距pole-piece of 电磁透镜的极靴Many-beam effects 多束效应Measurements of; dislocation density,位错密度测量elastic strain fields of precipitates沉淀相粒子弹性应变场测量foil thickness 膜厚测量precipitate size, 沉淀相粒子尺寸测量stacking fault energy 层错能测量nodes, by 用位错结测量层错能ribbon widths, by 用层错带宽度测量层错能Microanalysis 微区分析Moire patterns Moire花样from precipitates 沉淀相粒子Moire花样mixed 混合Moire条纹parallel 平行Moire条纹rotation 旋转Moire条纹spacing of Moire条纹间距Nodes, extended threefold, 三维扩展位错结stacking fault energy from三维扩展位错结测量层错能Objective wave function 物波函数Objective lens物镜Operating vector 操作矢量Operation reflection 操作反射Orientation determination 取向确定Orientation relationship 取向关系Parallel moire patterns 平行Moire条纹Partial dislocations, contrast from平行位错的衬度determination of Burgers vectors of位错柏氏矢量的确定Frank Frank位错柏氏矢量确定Shockley Shockley位错柏氏矢量确定Particles 粒子Planar defect 面缺陷Point defects in specimen 试样中的点缺陷Pole-piece of magnetic lens 电磁透镜极靴Precipitates 沉淀相粒子contrast from 沉淀相粒子衬度size of 沉淀相粒子尺寸visibility of 沉淀相粒子可见性Precipitation contrast 沉淀相衬度Projective lens投影镜Reciprocal lattice 倒易点阵construction 倒易点阵的构筑definition of 倒易点阵的定义properties of 倒易点阵的性质Replica 复型Resolution 分辩率Ring diffraction patterns 环状衍射花样Rotation moirre patterns 旋转Moirre花样Satellites on electron diffraction patterns衍射花样卫星斑点Scattering amplitude 散射振幅Scattering of electrons 电子散射Second phase particles 第二相粒子Selected area diffraction 选区电子衍射accuracy of 选区电子衍射的精度Shape effect 形状效应Single crystal diffraction patterns单晶电子衍射花样Specimen 试样contamination of 试样污染cooling of 试样冷却deformation of 试样变形heating of 试样加热microanalysis of 试样微区分析orientation of 试样的取向preparation of 试样制备chemical machining 试样加工chemical polishing, by 用化学抛光制备试样ion bombardment, by 离子轰击制备试样electropolishing 电解抛光制备试样jet machining, by, 电解双喷制样法Specimen holder 试样台top enrty 顶插式试样台side entry 侧插式试样台Spherical aberration 球差Spinodal decomposition 拐点分解Stacking faults 层错contrast of 层错的衬度determination of nature of 确定层错的性质energy of 层错能types of 层错类型Sterogram 极图Stereomicroseopy 体视显微术Stigmator 消像散器Strain fields 应变场Streaks on electron diffraction patterns衍射花样的星芒线Structure factor 结构因子contrast from, 结构因子衬度Subsidiary fringe 副条纹Superlattice 超点阵reflections 超点阵反射Theory of diffraction contrast 衍射衬度理论kinematic 运动学衍衬理论dynamic 动力学衍衬理论Two beam approximation 双束近似Uniform absorption coefficient 反常吸收系数Viewing screen 荧光屏Weak beam technique 弱束技术Weak beam dark field image 弱束暗场象Zone 晶带Zone law晶带定理Zone axis 晶带轴Zone axis patterns 晶带轴花样HREMAiry disc Airy园(盘) Amplitude object 振幅物Amplitude contrast 振幅衬度Astigmatism 像散Astigmator 消像散器Axial 轴向照明Axial alignment 合轴调整Chromatic aberration coefficient色差系数Chromatic aberration 色差Chromatic aberration limited resolution色差限制的分辩率Cluster 偏聚区Coherence 相干性Defocus 欠焦Diffraction contrast 衍射衬度Diffraction limit 衍射极限Diffraction limited resolution 衍射限制的分辩率Diffused circle 弥散园Exact focus 准确聚焦Experimental condition 实验条件Exsolution 脱溶Focus 聚焦, 焦距, 焦点Focal length 焦距Frensnel fringes 菲捏尔条纹Grain boundaries晶界small angle 小角度晶界high angle 大角度晶界symmetrical 对称晶界asymmetrical 不对称晶界tilt 倾斜晶界Guinier-Preston zones GP区HREM images高分辩电镜图像interpretation 高分辩电镜图像的解释information available 高分辩电镜图像的信息image analysis of 图像分析computer simulation of 计算机模拟Illumination 照明axial 轴向照明tilted 倾斜照明Illumination semi-angle 照明半角Image analysis 图像分析Imaging mode 图像模式lattice plane 点阵平面像many beam 多束点阵像structure 结构像Image restoration 图像修复Incident wave 入射波Interaction constant 交互作用常数Interplanar spacing 面间距Internal standards 内标Line to line resolution 线分辩率Multi-slice approximation 多片近似Optical diffraction 光学衍射Optimum defocus 最佳欠焦(量) Optimum resolution 最佳分辩率Optimum illumination semi-angle 最佳照明半角Optimum aperture size 最佳光阑尺寸Order/disorder transition 有序/无序转变Orientation 取向Bragg Bragg取向Laue Laue取向Over focus 过焦Phase change 相位变化induced by defocus 欠焦引起的相位变化by spherical aberration 球差引起的相位变化Phase contrast 相位衬度Phase contrast transfer function 相位衬度传递函数Phase grating 相位光栅Phase grating approximation 相位光栅近似Phase object 相位物Phase object approximation 相位物近似Phase shift 相位变化Phase transition 相转变Phase transformation 相变Point source 点源Point to point 点分辩率Projected potential 投影势Propagation function 传递函数Polymorphism 多型性(转变) Resolution 分辩率line to line 线分辩率point to point 点分辩率Resolution limit 分辩率极限Scattered wave 散射波Spherical aberration 球差Spherical aberration coefficient 球差系数(C S) Spherical aberration limited resolution球差限制的分辩率Weak phase approximation 弱相位近似Tilted illumination 倾斜照明Through focus series 聚焦系列Two beam lattice plane imaging双束点阵平面像Two beam lattice fringe imaging双束点阵条纹像AEMAamorphous carbon 非晶碳EELS absolute quantification 用于EELS绝对定量analytical electron microscope 分析电镜alignment 对中calibration for EELS or EDS EELS或EDS定标analytical electron microscopy 分析电子显微学annular dark-field imaging 环状暗场像annular detector 环状探头apertures 光阑2nd condenser lens (C2) 第二聚光镜光阑effect on microanalysis 对微区分析的影响effect on microdiffraction 对微束衍射的影响effect on probe convergence 对探针会聚性的影响objective 物镜光阑selected area (SA) 选区光阑ultra-thick 超厚光阑Auger electrons俄歇electron spectroscopy 俄歇谱Bbackground spectrum 本底(背底)谱in EELS EELS背底谱subtraction in EDS 扣除EDS谱背底subtraction in EELS 扣除EDS谱背底X-rays 扣除X-射线背底(请参见bremsstrahlung 和continuum)backscattered electrons 背散射电子detector 背散射电子探头images 背散射电子像beam 电子束beam damage 电子束损伤beam-sensitive specimens 电子束敏感试样beam-specimen interactions 电子束-试样交互作用beam spreading 电子束扩展beryllium window 铍窗bremsstrahlung X-rays 背底辐射X-射线bright field detector 明场探头bright field image in STEM STEM 明场像brightness of electron source 电子源亮度Ccalibration 校准, 定标cathode ray tube 阴极射线管cathodoluminescence 阴极荧光(辐射)Cliff-Lorimer equation Cliff-Lorimer 公式condenser lens —first (C1) 第一聚光镜condenser lens —second (C2) 第二聚光镜condenser objective lens 聚光镜物镜contamination 污染use to determine thickness 用于厚度测定continuum X-rays 连续(背底)X-射线convergent beam diffraction 会聚束衍射use to determine thickness 用于厚度测定convergent beam diffraction patterns (CBDP)会聚束衍射花样convergent electron probe 会聚电子探针crystal point group (晶体)点群Ddark field detector 暗场探头dark field image in STEM STEM暗场像deconvolution 解谱, EDS或EELS of EDS spectrum, of EELS spectrumdiad symmetry 二次对称diffraction groups 衍射群diffraction maxima 衍射极大值EEDS (Energy Dispersive Spectroscopy) 能谱(能量色散谱)EDS defector能谱探头EELS spectrometer 电子能量损失谱仪EELS 电子能量损失谱 (electron energy loss spectrum) zero loss peak 零损失峰 plasmon peak 等离子振荡峰 energy loss peaks 能量损失峰 ionization edge 电离损失峰(边) background subtraction 背底扣除elastic scatter 弹性散射electron detectors 电子探头 collection angle 收集角electron energy loss spectrometer 电子能量损失谱仪electron energy loss spectrometry 电子能量损失谱 energy loss processes 电子能量损失过程 imaging/mapping 电子能量损失成象 ionization losses 电离损失 limitations 极限 plasmon losses 等离子振荡损失 spatial resolution 空间分辨率electron-hole pairs 电子-空位对electron probe 电子探针 brightness 亮度 convergence angle 会聚角 current 电流 diameter 直径energy dispersive spectrometer 能谱仪 (See X-ray energy dispersive 58spectrometer)energy filtered images 能量过滤图像extended absorption fine structure 广延吸收精细结构extraction replica 萃取复型 Ffirst order laue zone (FOLZ) 一阶劳厄区fine structure in ionization edge 电离峰(边)精细结构 post-edge (EXAFS) 峰后(EXAFS) pre-edge 峰前forbidden reflections禁止反射full width half maximum 半高宽Gg vector g 矢量Gaussian 高斯Hhard X-rays 硬X-射线higher order laue zone (HOLZ)高阶劳厄区indexing 标定lines高阶劳厄区线 reflections 高阶劳厄区反射 rings高阶劳厄区环HOLZ lines 高阶劳厄区线Iillumination system 照明系统imaging in STEM STEM 成像image enhancement 图像增强Indexing 标定 HOLZ lines 高阶劳厄区线 HOLZ patterns 高阶劳厄区花样 ZOLZ patterns 零阶劳厄区花样inelastic scatter 非弹性散射(See also electron energy loss) effect on EDS 对EDS 的影响 effect on EELS 对EELS 的影响ionization 电离ionization edges 电离损失峰(边) post-edge fine structure 峰后精细结构 pre-edge fine structure 峰前精细结构KKossel patterns (conditions) Kossel 花样Kossel-Möllenstedt fringes use to determine thickness K-M 条纹9用于确定试样厚度)Kossel-Möllenstedt (K-M) patterns K-M花样Llanthanum hexaboride gun 六硼化镧电子枪lattice parameter determination 点阵常数确定lattice strain 点阵应变effect on HOLZ lines 对高阶劳厄区线的影响lenses 透镜auxiliary 辅助透镜condenser 聚光镜condenser-objective 聚光镜-物镜intermediate 中间镜objective 物镜projector投影镜light element analysis by EDS EDS轻元素分析by EELS EELS轻元素分析limitations to X-ray analysis X-射线分析极限low loss electrons 低能量损失电子Mmicrodiffraction 微束衍射microprobe mode 微区探针模式minimum detectable mass 最小可探测质量minimum mass fraction 最小质量分数Nobjective aperture 物镜光阑objective lens 物镜Ppeak to background ratio 峰/背比in EDS spectrum EDS谱in EELS spectrum EELS谱(See also signal to noise ratio) 参见信/噪比phonon energy loss 声子能量损失plasmon energy losses 等离子振荡能量损失probe convergence angle 探针会聚角Qqualitative analysis 定性分析using EDS EDS定性分析using EE LS EE LS定性分析quantitative analysis 定量分析using E DS EDS定量分析using EE LS EE LS定量分析Rradial distribution function 径向分布函数radiation damage 辐射损伤resolution 分辨率of EDS spectrometer EDS谱仪分辨率ot EELS spectrometer EELS谱仪分辨率of STEM image STEM图像分辨率Riecke microdiffraction Riecke法微束衍射Sscanning electron microscope 扫描电镜scanning images 扫描图像scanning transmission electron microscope扫描透射电镜screw axis 螺旋轴second order laue zone (SOLZ) 二阶劳厄区secondary electrons 二次电子detectorsensitivity limits灵敏度极限in EDS EDSin EE LS EE LSspace group 空间群spurious effects 杂散效应signal processing 信号处理signal to noise ratio(See also peak to background ratio) 信/噪比spatial resolution 空间分辨率in EDS EDS in EE LS EE LSin microdiffraction 微束衍射in STEM image STEM图像spurious effects 杂散效应in EDS spectrum EDS谱杂散效应stationary diffraction pattern 稳定衍射花样strain measurements 应变测量symmetry (crystal) (晶体)对称changes 对称变化determination 对称确定systematic absences 系统消光Tterminology of CBDPs 会聚束衍射术语thickness determination 厚度确定transmitted electrons 透射电子triad symmetry 三重(次)对称tungsten hairpin filament 钨灯丝Uultra-thin window 超薄窗ultra-thick condenser apertures 超厚聚光镜光阑Vvalence electron interactions 价电子交互作用wwavelength dispersive spectrometer (WDS)波谱仪weak beam imaging 弱束暗场成象x X-ray(s) X-射线Absorption 吸收fluorescence generation 荧光的产生images/maps 像/成份分布ionization cross section 电离截面microanalysis 微区分析X-ray energy dispersive spectrometerX-射线能谱仪Calibration 校准, 定标collection angle 接收角dead layer 死层dead time 死时间efficiency 效率X-ray peak X-射线峰peak fitting in EDS 能谱峰位拟合X-ray spectrum X-射线谱background subtraction 背底扣除deconvolution 解谱digital filtering 数字过滤Yyttrium-aluminum garnet 钇铝石榴石yttrium-aluminum perovskite 钇铝钙钛矿zZ-contrast 原子序数衬度ZAF correction ZAF校正zero loss peak 零损失峰zero order laue zone (ZOLZ) 零阶劳厄区indexing 标定pattern symmetry 对称性zone axis 晶带轴patterns 晶带轴花样symmetry 对称性。
