同济大学《高等数学D》英文电子教案课件pptfile_5611deaad34f6
合集下载
file_5174fb699d910同济大学高等数学英语版
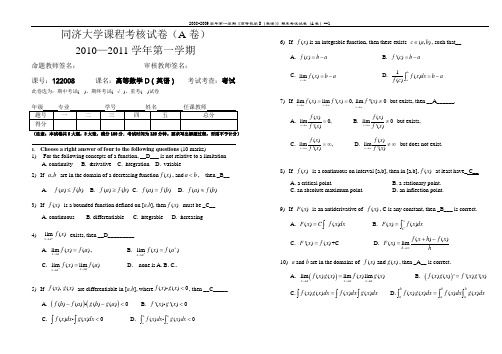
9) If F ( x) is an antiderivative of f ( x) , C is any constant, then _B___ is correct. A. F ( x) = C ∫ f ( x)dx C. F '( x) = f ( x) +C B. F ( x) = ∫ f ( x)dx
If f ( x) = e x , then
∫
f '(ln x) dx = _|x|+C___________. x
d2 f 1 −2sin x = − + f ( x) ln(cos x) + tan x , then 7) = 2 2 dx cos x cos3 x
If F ( x), f ( x), g ( x), h( x) are continuous in (−∞, ∞) . g ( x) ≤ f ( x) ≤ h( x) with
Increasing intervals: ( 5)
3 + 33 −3 + 33 , 0 ), ( , +∞) 4 4
= we have: a)
dy dt
(3 marks) Write out the concave up and concave down intervals of f ( x)
∫ f ( x)dx∫ g ( x)dx < 0
∫
b
a
f ( x)dx ∫ g ( x)dx < 0
a
b
2008-2009 学年第一学期《高等数学 D(英语) 》期末考试试卷(A 卷)--2
2. Fill in the blanks (10 marks)
1)
同济大学版本高数精品课件全册

1+ x
理解为:
f
(
∆
)
=
1− 1+
∆ ∆
(五)函数与图像
2、图像:平面点= 集 C {(x= , y) y f (x), x∈D}。
了解函数的直
例:画函数 y = x 的图像.
观手段!
y
一元函数的图像通常是二
维平面上的一条一维曲线.
注: 由曲线求取对应的函
数往往不易,由函数画图
o
x 像相对容易.
例如, 1 + 2 =3 1 − 2 =−1
负数的引入有实 际意义!如:记 帐有赢利亏欠, 温度有零上零 下…
2. Z(整数环)
对加法、减法都封闭; 对除法不能封闭。
例如, 1 ÷ 2 =0.5
3. Q(有理数域)
对加法、减法、乘法、除法都封闭;有理数域尽管稠密但不 连续,还有客观事物不能用有理数表示。
课后自测
1、 写出所有三角函数和反三角函数的定义域,并画出函数图像。
2、
已知函数
y
=
f
(x)
=
12+
x, x,
0≤ x ≤1 x >1
求
f
(
1 2
)
及
f
(
1 t
)
,
并写出定义域及值域 。
第十节 闭区间上连续函数的性质
一、有界性与最大值最小值定理 二、零点定理与介值定理
一、有界性与最大值最小值定理
二、预备知识
1、基本初等函数 (4) 三角函数
余弦函数 y = cos x 正切函数 y = tan x
余切函数 y = cot x
正割函数 y = sec x 余割函数 y = csc x
理解为:
f
(
∆
)
=
1− 1+
∆ ∆
(五)函数与图像
2、图像:平面点= 集 C {(x= , y) y f (x), x∈D}。
了解函数的直
例:画函数 y = x 的图像.
观手段!
y
一元函数的图像通常是二
维平面上的一条一维曲线.
注: 由曲线求取对应的函
数往往不易,由函数画图
o
x 像相对容易.
例如, 1 + 2 =3 1 − 2 =−1
负数的引入有实 际意义!如:记 帐有赢利亏欠, 温度有零上零 下…
2. Z(整数环)
对加法、减法都封闭; 对除法不能封闭。
例如, 1 ÷ 2 =0.5
3. Q(有理数域)
对加法、减法、乘法、除法都封闭;有理数域尽管稠密但不 连续,还有客观事物不能用有理数表示。
课后自测
1、 写出所有三角函数和反三角函数的定义域,并画出函数图像。
2、
已知函数
y
=
f
(x)
=
12+
x, x,
0≤ x ≤1 x >1
求
f
(
1 2
)
及
f
(
1 t
)
,
并写出定义域及值域 。
第十节 闭区间上连续函数的性质
一、有界性与最大值最小值定理 二、零点定理与介值定理
一、有界性与最大值最小值定理
二、预备知识
1、基本初等函数 (4) 三角函数
余弦函数 y = cos x 正切函数 y = tan x
余切函数 y = cot x
正割函数 y = sec x 余割函数 y = csc x
同济大学高等数学上课件D矢量

与 a 的模相同, 但方向相反的向量称为 a 的负向量, 记作-a ;
因平行向量可平移到同一直线上, 故两向量平行又称 两向量共线 .
假设 k (≥3)个向量经平移可移到同一平面上 , 那么称此 k 个向量共面 .
第四页,共28页。
机动 目录 上页 下页 返回 完毕
二、向量的线性运算
1. 向量的加法
的坐标为 M(x,y,z),那
么
z
O M O N M O O A O BC C
O Axi, O Byj,OC zk
r x i y j zk (x,y,z)
ko i
j
r
M
B y
A
此xi式,y 称 j为,z向k 称 量 r 的为 坐标r 分沿向 解三式个坐, 量 标轴方向的x分向量. N
试 a 与 用 b 表 M ,示 M A ,M B ,M C . D
解: abAC2MC2MA
D
C
baBD2MD2MB b
M A 1 2(ab) MB 1 2(ba)A M C 1 2(ab) M D 1 2(ba)
M aB
第十页,共28页。
机动 目录 上页 下页 返回 完毕
三、空间直角坐标系
1. 空间直角坐标系的根本概念
第十四页,共28页。
机动 目录 上页 下页 返回 完毕
四、利用坐标作向量的线性运算
设 a (a a x,b a y ,( a a zx ) b , b x ,( a b y x ,b b y y ,,b a zz ), b z 为)实数,那么
a(ax,ay,az)
平行向量对应坐标成比例:
当 a 0 时 ,
得两点间的间隔 公式:
A
ABAB ( x 2 x 1 ) 2 ( y 2 y 1 ) 2 ( z 2 z 1 ) 2
因平行向量可平移到同一直线上, 故两向量平行又称 两向量共线 .
假设 k (≥3)个向量经平移可移到同一平面上 , 那么称此 k 个向量共面 .
第四页,共28页。
机动 目录 上页 下页 返回 完毕
二、向量的线性运算
1. 向量的加法
的坐标为 M(x,y,z),那
么
z
O M O N M O O A O BC C
O Axi, O Byj,OC zk
r x i y j zk (x,y,z)
ko i
j
r
M
B y
A
此xi式,y 称 j为,z向k 称 量 r 的为 坐标r 分沿向 解三式个坐, 量 标轴方向的x分向量. N
试 a 与 用 b 表 M ,示 M A ,M B ,M C . D
解: abAC2MC2MA
D
C
baBD2MD2MB b
M A 1 2(ab) MB 1 2(ba)A M C 1 2(ab) M D 1 2(ba)
M aB
第十页,共28页。
机动 目录 上页 下页 返回 完毕
三、空间直角坐标系
1. 空间直角坐标系的根本概念
第十四页,共28页。
机动 目录 上页 下页 返回 完毕
四、利用坐标作向量的线性运算
设 a (a a x,b a y ,( a a zx ) b , b x ,( a b y x ,b b y y ,,b a zz ), b z 为)实数,那么
a(ax,ay,az)
平行向量对应坐标成比例:
当 a 0 时 ,
得两点间的间隔 公式:
A
ABAB ( x 2 x 1 ) 2 ( y 2 y 1 ) 2 ( z 2 z 1 ) 2
第一课同济大学高等数学上预备知识ppt课件

例 设 X 1 ,2 ,3 ,Y 2 ,4 ,6 ,8 ,
T
X Y,
x
2 x,
则T 是 X 到 Y 的映射.
例 设 X 1 ,1 ,Y , ,
X Y
T
x
tan
2
x
则T 是 X 到 Y 的映射.
从使用情况来看,闭胸式的使用比较 广泛。 敞开式 盾构之 中有挤 压式盾 构、全 部敞开 式盾构 ,但在 近些年 的城市 地下工 程施工 中已很 少使用 ,在此 不再说 明。
例 试说明函数 f x 1 sin 1 在 x 0 的任何空心邻
xx
域内是无界函数.
解 只要证明在 x 0 的任何空心邻域内,无论对怎样的
正数 M 0,总是存在该邻域内一点 x 0 ,使得
f x0 M.
1
现设
M
0,取
x0
2n
/
,
2
其中取
n
1
2
M
2
的正整数,
并且使得 x 0 在空心邻域内,
例:设 X R ,Y 1 ,1 ,Z 0 ,1 ,
X Y,
T1
x
sin
x,
Y Z,
T2
y
y2,
则复合映射T2 T1为
X Z, T x(sinx)2.
从使用情况来看,闭胸式的使用比较 广泛。 敞开式 盾构之 中有挤 压式盾 构、全 部敞开 式盾构 ,但在 近些年 的城市 地下工 程施工 中已很 少使用 ,在此 不再说 明。
从使用情况来看,闭胸式的使用比较 广泛。 敞开式 盾构之 中有挤 压式盾 构、全 部敞开 式盾构 ,但在 近些年 的城市 地下工 程施工 中已很 少使用 ,在此 不再说 明。
同济大学高等数学(第七版)上册第一章函数 PPT课件

16 x2 0
(1) (2)
y 2x ln x 16 x2
y log5 (x2 1)
ln x 0 x [1, 4) (4, )
x0
x2 1 0 x (, 1) (1, )
函数定义可简单地归结为构成函数的两个要素: • 定义域 D f : 自变量的变化范围。 • 对应法则 f :自变量与因变量的对应规则。
y y f (x)
f (x)
f (x)
-x o x
x
偶函数图形关于y轴对称,如:y=kx2
设D关于原点对称, 对于x D, 有 f ( x) f ( x) 称 f ( x)为奇函数;
y
y f (x)
-x f (x)
f (x)
o
xx
奇函数的图形关于原点对称,如:y=kx
奇、偶函数经四则运算后仍可在一定条件 下保持相应的奇、偶性。
解: D( 7) 1, 5
D(1 2) 0,
D(D( x)) 1,
(5) 取最值函数
y max{ f ( x), g( x)}
y
f (x)
g( x)
o
x
y min{ f ( x), g( x)}
y
f (x)
g( x)
o
x
例.
已知函数
y
f
(
x)
2 1
x, x,
y
y f (x)
f (x2 )
f (x1)
o
x
I
设函数 f ( x)的定义域为D, 区间I D, 如果对于区间 I 上任意两点x1及 x2 , 当 x1 x2时, 恒有 (2) f ( x1 ) f ( x2 ), 则称函数 f ( x)在区间I上是单调减少的;
高等数学D教学大纲
版本II
第一章 实数系与几何学(学时数:10)
实数系;几何学;空间坐标系(直角坐标系、曲面方程、曲线方程、二次曲面、球面坐标系)。
第二章 函数、极限、求和(学时数:16)
函数的概念;函数的表示;函数的几个特性;初等函数;极限的定义和性质;极限的运算法则;两个重要极限;函数的连续性;无穷级数求和;等比级数求和;正项级数收敛性判别;幂级数。
第二章 函数、极限、求和(学时数:16)
函数的概念;函数的表示;函数的几个特性;初等函数;极限的定义和性质;极限的运算法则;两个重要极限;函数的连续性;无穷级数求和;等比级数求和;正项级数收敛性判别;幂级数。
第三章导数及其应用(学时数:15)
导数定义;求导法则;高阶导数及偏导数简介;微分的概念及计算;
高等数学D教学大纲
(Advanced Mathematics)
课程代码
218.104.1
编写时间
课程名称
高等数学Dຫໍສະໝຸດ 英文名称AdvancedMathematics
学分数
4
周学时
5
任课教师*
张玉娣等
开课院系**
数学学院
预修课程
课程性质:
文科学生的基础课(国际关系学院本科各专业、文科的本科学生)。
基本要求和教学目的:
用导数研究函数(中值定理,函数的单调性,函数的极值,凹凸与拐点,渐近线,函数作图)。
第四章积分(学时数:15)
原函数;不定积分;换元积分法;分部积分法;曲边梯形的面积;定积分;微积分基本定理;无穷限广义积分;无界函数的广义积分;简单的微分方程。
第五章矩阵与线性方程组(学时数:13)
矩阵的定义;矩阵相加(减)和数乘;矩阵的乘法;求逆阵;线性方程组;消元法;线性变换与矩阵;线性规划(归结成线性规划问题的方法举例子,图解法,标准线性规划)
第一章 实数系与几何学(学时数:10)
实数系;几何学;空间坐标系(直角坐标系、曲面方程、曲线方程、二次曲面、球面坐标系)。
第二章 函数、极限、求和(学时数:16)
函数的概念;函数的表示;函数的几个特性;初等函数;极限的定义和性质;极限的运算法则;两个重要极限;函数的连续性;无穷级数求和;等比级数求和;正项级数收敛性判别;幂级数。
第二章 函数、极限、求和(学时数:16)
函数的概念;函数的表示;函数的几个特性;初等函数;极限的定义和性质;极限的运算法则;两个重要极限;函数的连续性;无穷级数求和;等比级数求和;正项级数收敛性判别;幂级数。
第三章导数及其应用(学时数:15)
导数定义;求导法则;高阶导数及偏导数简介;微分的概念及计算;
高等数学D教学大纲
(Advanced Mathematics)
课程代码
218.104.1
编写时间
课程名称
高等数学Dຫໍສະໝຸດ 英文名称AdvancedMathematics
学分数
4
周学时
5
任课教师*
张玉娣等
开课院系**
数学学院
预修课程
课程性质:
文科学生的基础课(国际关系学院本科各专业、文科的本科学生)。
基本要求和教学目的:
用导数研究函数(中值定理,函数的单调性,函数的极值,凹凸与拐点,渐近线,函数作图)。
第四章积分(学时数:15)
原函数;不定积分;换元积分法;分部积分法;曲边梯形的面积;定积分;微积分基本定理;无穷限广义积分;无界函数的广义积分;简单的微分方程。
第五章矩阵与线性方程组(学时数:13)
矩阵的定义;矩阵相加(减)和数乘;矩阵的乘法;求逆阵;线性方程组;消元法;线性变换与矩阵;线性规划(归结成线性规划问题的方法举例子,图解法,标准线性规划)
同济大学《高等数学D》英文电子教案课件pptfile_5623579213949

has farther to go. Therefore, as there are an infinite number of points A must reach where T has already been, he never overtake T.
Some Examples for Limit
f2 ( x) f1(a) f2 (a)
a
One Side Limit (An Informal View)
If the values of f (x) can be made as close as we like to L by taking values of x sufficiently close to a (but greater than a), written as lim f (x) L
Advanced Mathematics D
Chapter Two Limits & Continuity
Enter Calculus World
We have known “Function” This is the tool for us enter the Calculus
World The first adventure is “Limit” Limits is the soul of Calculus as well as
“割之弥细,所失弥小. 割之又割, 以至于不可割,则与圆周合体,而无 所失矣”。
Calculation of a Circle Area
N=4 N=6 N=8 N=10 N=12
Inside Polygo n Area
2 2.598 2.828 2.939 3
Outsid e Polygo n Area
Some Examples for Limit
f2 ( x) f1(a) f2 (a)
a
One Side Limit (An Informal View)
If the values of f (x) can be made as close as we like to L by taking values of x sufficiently close to a (but greater than a), written as lim f (x) L
Advanced Mathematics D
Chapter Two Limits & Continuity
Enter Calculus World
We have known “Function” This is the tool for us enter the Calculus
World The first adventure is “Limit” Limits is the soul of Calculus as well as
“割之弥细,所失弥小. 割之又割, 以至于不可割,则与圆周合体,而无 所失矣”。
Calculation of a Circle Area
N=4 N=6 N=8 N=10 N=12
Inside Polygo n Area
2 2.598 2.828 2.939 3
Outsid e Polygo n Area
同济大学高等数学课件D23高阶导数-PPT文档资料

( n ) (cos x ) cos( x n ) 2
机动 目录 上页 下页 返回 结束
( a ,b 为常数 ),求 bx y (n) . 例5 . 设 ye sin
解:
a x a x y ae sin bx be cos bx a x e ( a sin b x b cos b x )
,
y
(n)
(1)
n1
(n 1 )!
(1 x ) n ( n 1) ! (1 x ) n
规定 0 ! = 1
ln ( 1 x ) , y (n) 思考: y
机动 目录 上页 下页 返回 结束
sin x ,求 y ( n ) . 例4. 设 y
解:
) sin( x y cos x 2
( n ) n ( x ) ( 1 )( 2 ) ( n 1 ) x
机动 目录 上页 下页 返回 结束
(n) 例2. 设 y eax , 求 y .
解:
2 a x 3a x ae , , y a e , y a e , y
第三节 高阶导数
一、高阶导数的概念
二、高阶导数的运算法则
第二章
机动 目录 上页 下页 返回 结束
一、高阶导数的概念
引例:变速直线运动 ss ( t)
速度
ds v , dt
即 v s
d ds dv ( ) 加速度 a d t dt dt
即
a ( s )
机动 目录 上页 下页 返回 结束
ax
a x
b arctan ) e a bsin( bx ) ( a a x 2 2 a x [ ae sin( bx ) a y b be cos( bx )]
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
Age(John)=18, Age(Linda)=17…
BirthDay (Name) :
BirthDay(John)=02/04/1995, BirthDay(Linda)=16/07/1996, …
Sex (Name): Sex(John)=M, Sex(Linda)=F…
Graphs of Functions
Part Two
Functions Limites Derivatives Integration Differential Equations
Functions
What is a function? How does a function play in Calculus? What is any difference between the
Represent of Function
Numerically by tables Geometrically by Graphs Algebraically by formulas Verbally
Example of Function 1
x
0
1
2
3
f (x)
3
4
-1
3
f (0) 3, f (1) 4, f (2) 1, f (3) 3
Different from Social Science
Philosophy v.s Arts Logical v.s Image
Method of Studying Advanced Mathematics
Understanding deeply Deduce step by step Exercise
Advanced Mathematics D
Part One
Introduction
The Aim of this Course
Learn Advanced Mathematics
What is advanced mathematics What is advanced mathematics doing How is mathematical thinking
Example of Function 2
90
80
East(Season) 70
60
West(Season) 50
东部
North(Season)
40 30
西部 北部
20
10
0 第一季度 第二季度 第三季度 第四季度
East(1)=0, East(2)=28, East(3)=90, East(4)=20 West(1)=30, West(2)=38, West(3)=32, West(4)=31, North(1)=45, North(2)=46, North(3)=44, North(1)=43
F G m1m2 r2
Example of Function 6
The Age of the students in Class X The Birth Day of the students in Class X The Sex of the students in Class X
Students in Class X are: John, Linda, …. Age (Name) :
Example of Function 3
Example of Function 4
x 10-3 1.2
1
0.8
error
0.6
0.4
0.2
0
0.01
0.02
0.03
0.04
0.05
0.06
0.07
0.08
0.09
0.1Biblioteka 0.11xExample of Function 5
Newton’s Law of Universal Gravitation:
Not all graph in xy-plane is a function
For every input variable, only one output corresponds
A curve in the xy-plane is the graph of a function, if and only if no vertical line intersects cure more than once
Learn English
What is saying in English about advanced mathematics
Calculus – Advanced Mathematics
Different from High School Mathematics
Calculate v.s Analysis Static v.s Dynamic
y
This is a function
y
This is
not a
function
x x
Domain & Range
Domain – the set of all allowable inputs
Range – the set of the all possible output when input over the domain
If a variable y depends on a variable x in a such way that each value of x determines exactly one value of y, then we say that y is a function of x.
A function f is a rule that associates a unique output with each input. If the input is denoted by x, then the output is denoted by f(x) (read “f of x”)
function in Calculus and the Mathematics in the Middle School?
Basic Concepts
Variables: x,y Value or image Relation Determination Region
Definition of Function
Denoted by Df , and Rf respectively
BirthDay (Name) :
BirthDay(John)=02/04/1995, BirthDay(Linda)=16/07/1996, …
Sex (Name): Sex(John)=M, Sex(Linda)=F…
Graphs of Functions
Part Two
Functions Limites Derivatives Integration Differential Equations
Functions
What is a function? How does a function play in Calculus? What is any difference between the
Represent of Function
Numerically by tables Geometrically by Graphs Algebraically by formulas Verbally
Example of Function 1
x
0
1
2
3
f (x)
3
4
-1
3
f (0) 3, f (1) 4, f (2) 1, f (3) 3
Different from Social Science
Philosophy v.s Arts Logical v.s Image
Method of Studying Advanced Mathematics
Understanding deeply Deduce step by step Exercise
Advanced Mathematics D
Part One
Introduction
The Aim of this Course
Learn Advanced Mathematics
What is advanced mathematics What is advanced mathematics doing How is mathematical thinking
Example of Function 2
90
80
East(Season) 70
60
West(Season) 50
东部
North(Season)
40 30
西部 北部
20
10
0 第一季度 第二季度 第三季度 第四季度
East(1)=0, East(2)=28, East(3)=90, East(4)=20 West(1)=30, West(2)=38, West(3)=32, West(4)=31, North(1)=45, North(2)=46, North(3)=44, North(1)=43
F G m1m2 r2
Example of Function 6
The Age of the students in Class X The Birth Day of the students in Class X The Sex of the students in Class X
Students in Class X are: John, Linda, …. Age (Name) :
Example of Function 3
Example of Function 4
x 10-3 1.2
1
0.8
error
0.6
0.4
0.2
0
0.01
0.02
0.03
0.04
0.05
0.06
0.07
0.08
0.09
0.1Biblioteka 0.11xExample of Function 5
Newton’s Law of Universal Gravitation:
Not all graph in xy-plane is a function
For every input variable, only one output corresponds
A curve in the xy-plane is the graph of a function, if and only if no vertical line intersects cure more than once
Learn English
What is saying in English about advanced mathematics
Calculus – Advanced Mathematics
Different from High School Mathematics
Calculate v.s Analysis Static v.s Dynamic
y
This is a function
y
This is
not a
function
x x
Domain & Range
Domain – the set of all allowable inputs
Range – the set of the all possible output when input over the domain
If a variable y depends on a variable x in a such way that each value of x determines exactly one value of y, then we say that y is a function of x.
A function f is a rule that associates a unique output with each input. If the input is denoted by x, then the output is denoted by f(x) (read “f of x”)
function in Calculus and the Mathematics in the Middle School?
Basic Concepts
Variables: x,y Value or image Relation Determination Region
Definition of Function
Denoted by Df , and Rf respectively
