2008 AMC 12B Problems(答案)
2013 AMC 12B Problems

On a particular January day, the high temperature in Lincoln, Nebraska, was degrees higher than the low temperature, and the average of the high and low temperatures was . In degrees, what was the low temperature in Lincoln that day?SolutionMr. Green measures his rectangular garden by walking two of the sides and finds that it is stepsby steps. Each of Mr. Green’s steps is feet long. Mr. Green expects a half a pound of potatoes per square foot from his garden. How many pounds of potatoes does Mr. Green expect from his garden?SolutionWhen counting from to , is the number counted. When counting backwardsfrom to , is the number counted. What is ?SolutionRay's car averages miles per gallon of gasoline, and Tom's car averages miles per gallon of gasoline. Ray and Tom each drive the same number of miles. What is the cars' combined rate of miles per gallon of gasoline?SolutionThe average age of fifth-graders is . The average age of of their parents is . What is the average age of all of these parents and fifth-graders?SolutionReal numbers and satisfy the equation . What is ?SolutionJo and Blair take turns counting from to one more than the last number said by the other person. Jo starts by saying , so Blair follows by saying . Jo then says , and so on. What is the number said?SolutionLine has equation and goes through . Line has equation and meets line at point . Line has positive slope, goes through point , and meets at point . The area of is . What is the slope of ?SolutionWhat is the sum of the exponents of the prime factors of the square root of the largest perfect square that divides ?SolutionAlex has red tokens and blue tokens. There is a booth where Alex can give two red tokens and receive in return a silver token and a blue token, and another booth where Alex can give three blue tokensand receive in return a silver token and a red token. Alex continues to exchange tokens until no more exchanges are possible. How many silver tokens will Alex have at the end?SolutionTwo bees start at the same spot and fly at the same rate in the following directions. Bee travels foot north, then foot east, then foot upwards, and then continues to repeat this pattern. Beetravels foot south, then foot west, and then continues to repeat this pattern. In what directions are the bees traveling when they are exactly feet away from each other?east, westnorth, southnorth, westup, southup, westSolutionCities , , , , and are connected by roads , , , , , , and . How many different routes are there from to that use each road exactly once? (Such a route will necessarilyvisit some cities more than once.)SolutionThe internal angles of quadrilateral form an arithmetic progression.Triangles and are similar with and . Moreover, theangles in each of these two triangles also form an arithmetic progression. In degrees, what is the largestpossible sum of the two largest angles of ?SolutionTwo non-decreasing sequences of nonnegative integers have different first terms. Each sequence has theproperty that each term beginning with the third is the sum of the previous two terms, and the seventhterm of each sequence is . What is the smallest possible value of ?SolutionThe number is expressed in the form,where and are positive integers and is as small as possible. What is ?SolutionLet be an equiangular convex pentagon of perimeter . The pairwise intersections of the linesthat extend the sides of the pentagon determine a five-pointed star polygon. Let be the perimeter of thisstar. What is the difference between the maximum and the minimum possible values of ?SolutionLet and be real numbers such thatWhat is the difference between the maximum and minimum possible values of ?SolutionBarbara and Jenna play the following game, in which they take turns. A number of coins lie on a table. When it is Barbara’s turn, she must remove or coins, unless only one coin remains, in which case she loses her turn. What it is Jenna’s turn, she must remove or coins. A coin flip determines who goes first. Whoever removes the last coin wins the game. Assume both players use their best strategy. Who will win when the game starts with coins and when the game starts with coins?Barbara will win with coins and Jenna will win with coins.Jenna will win with coins, and whoever goes first will win with coins.Barbara will win with coins, and whoever goes second will win with coins.Jenna will win with coins, and Barbara will win with coins.Whoever goes first will win with coins, and whoever goes second will win with coins. SolutionIn triangle , , , and . Distinct points , , and lie onsegments , , and , respectively, such that , , and . The length of segment can be written as , where and are relatively prime positive integers. What is ?SolutionFor ,points and are the vertices of a trapezoid. What is ?SolutionConsider the set of 30 parabolas defined as follows: all parabolas have as focus the point (0,0) and the directrix lines have the form with a and b integers suchthat and . No three of these parabolas have a common point. How many points in the plane are on two of these parabolas?SolutionLet and be integers. Suppose that the product of the solutions for of the equationis the smallest possible integer. What is ?SolutionBernardo chooses a three-digit positive integer and writes both its base-5 and base-6 representations on a blackboard. Later LeRoy sees the two numbers Bernardo has written. Treating the two numbers as base-10 integers, he adds them to obtain an integer . For example, if , Bernardo writes the numbers 10,444 and 3,245, and LeRoy obtains the sum . For how many choices of are the two rightmost digits of , in order, the same as those of ?SolutionLet be a triangle where is the midpoint of , and is the angle bisectorof with on . Let be the intersection of the median and the bisector . In addition is equilateral with . What is ?SolutionLet be the set of polynomials of the formwhere are integers and has distinct roots of the form with and integers. How many polynomials are in ?Solution。
【VIP专享】2010 AMC 12B Problems

2010Problem 1Makarla attended two meetings during her -hour work day. The first meetingtook minutes and the second meeting took twice as long. What percent of her work day was spent attending meetings?Problem 2A big is formed as shown. What is its area?Problem 3A ticket to a school play cost dollars, where is a whole number. A group of 9th graders buys tickets costing a total of $, and a group of 10graders buystickets costing a total of $. How many values for are possible?Problem 4A month with days has the same number of Mondays and Wednesdays.Howmany of the seven days of the week could be the first day of this month?Lucky Larry's teacher asked him to substitute numbers for , , , , and inthe expression and evaluate the result. Larry ignored the parenthese but added and subtracted correctly and obtained the correct result by coincidence. The number Larry sustitued for , , , and were , , , and ,respectively. What number did Larry substitude for ?Problem 6At the beginning of the school year, of all students in Mr. Wells' math classanswered "Yes" to the question "Do you love math", and answered "No." Atthe end of the school year, answered "Yes" and answerws "No."Altogether, of the students gave a different answer at the beginning and end of the school year. What is the difference between the maximum and the minimum possible values of ?Problem 7Shelby drives her scooter at a speed of miles per hour if it is not raining, andmiles per hour if it is raining. Today she drove in the sun in the morning and in the rain in the evening, for a total of miles in minutes. How many minutesdid she drive in the rain?Problem 8Every high school in the city of Euclid sent a team of students to a math contest. Each participant in the contest received a different score. Andrea's score was the median among all students, and hers was the highest score on her team.Andrea's teammates Beth and Carla placedth and th, respectively. How many schools are in the city?Let be the smallest positive integer such that is divisible by , is a perfectcube, and is a perfect square. What is the number of digits of ?Problem 10The average of the numbers and is . What is ?Problem 11A palindrome between and is chosen at random. What is theprobability that it is divisible by ?Problem 12For what value of doesProblem 13In , and . What is ?Problem 14Let , , , , and be postive integers with and letbe the largest of the sum , , and . What is the smallestpossible value of ?Problem 15For how many ordered triples of nonnegative integers less than arethere exactly two distinct elements in the set , where ?Problem 16Positive integers , , and are randomly and independently selected withreplacement from the set . What is the probability thatis divisible by ?Problem 17The entries in a array include all the digits from through , arranged sothat the entries in every row and column are in increasing order. How many such arrays are there?Problem 18A frog makes jumps, each exactly meter long. The directions of the jumps are chosen independenly at random. What is the probability that the frog's final position is no more than meter from its starting position?Problem 19A high school basketball game between the Raiders and Wildcats was tied at the end of the first quarter. The number of points scored by the Raiders in each ofthe four quarters formed an increasing geometric sequence, and the number of points scored by the Wildcats in each of the four quarters formed an increasing arithmetic sequence. At the end of the fourth quarter, the Raiders had won by one point. Neither team scored more than points. What was the total numberof points scored by the two teams in the first half?Problem 20A geometric sequence has , , and for somereal number . For what value of does ?Problem 21Let , and let be a polynomial with integer coefficients such that, and.What is the smallest possible value of ?Problem 22Let be a cyclic quadralateral. The side lengths of are distinctintegers less than such that . What is the largestpossible value of ?SolutionProblem 23Monic quadratic polynomial and have the property that haszeros at and , and has zeros atand . What is the sum of the minimum values ofand ?Problem 24The set of real numbers for whichis the union of intervals of the form . What is the sum of the lengths of these intervals?Problem 25For every integer , let be the largest power of the largest primethat divides . For example . What is the largestinteger such that divides?。
2009 AMC 12A Problems(答案)
2009 AMC 12A ProblemsProblem 1Kim's flight took off from Newark at 10:34 AM and landed in Miami at 1:18 PM. Both cities are in the same time zone. If her flight took hours and minutes, with, what is ?SolutionThere is hour and minutes between 10:34 AM and noon; and thereis hour and minutes between noon and 1:18 PM. Hence the flight took hoursand minutes, and .Problem 2Which of the following is equal to ?SolutionWe compute:Problem 3What number is one third of the way from to ?SolutionSolution 1We can rewrite the two given fractions as and . (We multiplied all numeratorsand denominators by .)Now it is obvious that the interval between them is divided into three parts by thefractions and .Solution 2The number we seek can be obtained as a weighted average of the two endpoints, where the closer one has weight and the further one . We compute:Problem 4Four coins are picked out of a piggy bank that contains a collection of pennies, nickels, dimes, and quarters. Which of the following could not be the total value of the four coins, in cents?SolutionAs all five options are divisible by , we may not use any pennies. (This is becausea penny is the only coin that is not divisible by , and if we used between andpennies, the sum would not be divisible by .)Hence the smallest coin we can use is a nickel, and thus the smallest amount we canget is . Therefore the option that is not reachable is .We can verify that we can indeed get the other ones:▪▪▪▪Problem 5One dimension of a cube is increased by , another is decreased by , and the thirdis left unchanged. The volume of the new rectangular solid is less than that of thecube. What was the volume of the cube?SolutionLet the original cube have edge length . Then its volume is . The new box hasdimensions , , and , hence its volume is .The difference between the two volumes is . As we are given that the difference is, we have , and the volume of the original cube was .Problem 6Suppose that and . Which of the following is equal to forevery pair of integers ?SolutionWe have .Problem 7The first three terms of an arithmetic sequence are , , andrespectively. The th term of the sequence is . What is ?SolutionAs this is an arithmetic sequence, the difference must be constant:. This solves to . The firstthree terms then are , , and . In general, the -th term is . Solvingwe get .Problem 8Four congruent rectangles are placed as shown. The area of the outer square istimes that of the inner square. What is the ratio of the length of the longer side of each rectangle to the length of its shorter side?SolutionThe area of the outer square is times that of the inner square. Therefore the sideof the outer square is times that of the inner square.Then the shorter side of the rectangle is of the side of the outer square, and thelonger side of the rectangle is of the side of the outer square, hence their ratiois .Problem 9Suppose that and . What is ?SolutionSolution 1As , we have .To compute , set in the first formula. We get.Solution 2Combining the two formulas, we know that .We can rearrange the right hand side to .Comparing coefficients we have , , and . Fromthe second equation we get , and then from the third we get . Hence.Problem 10In quadrilateral , , , , , and is aninteger. What is ?SolutionBy the triangle inequality we have , and also, hence .We got that , and as we know that is an integer, we must have.Problem 11The figures , , , and shown are the first in a sequence of figures. For, is constructed from by surrounding it with a square and placing onemore diamond on each side of the new square than had on each side of itsoutside square. For example, figure has diamonds. How many diamonds arethere in figure ?SolutionSolution 1Color the diamond layers alternately blue and red, starting from the outside. You'll get the following pattern:In the figure , the blue diamonds form a square, and the red diamondsform a square. Hence the total number of diamonds in is.Solution 2When constructing from , we add new diamonds. Let be thenumber of diamonds in . We now know that and.Hence we get:Problem 12How many positive integers less than are times the sum of their digits?SolutionSolution 1The sum of the digits is at most . Therefore the number is at most . Out of the numbers to the one with the largest sum of digits is ,and the sum is . Hence the sum of digits will be at most .Also, each number with this property is divisible by , therefore it is divisible by ,and thus also its sum of digits is divisible by .We only have six possibilities left for the sum of the digits: , , , , , and .These lead to the integers , , , , , and . But for the sum of digitsis , which is not , therefore is not a solution. Similarly we can throwaway , , , and , and we are left with just solution: the number .Solution 2We can write each integer between and inclusive aswhere and . The sum of digits of this number is, hence we get the equation . Thissimplifies to . Clearly for there are no solutions, henceand we get the equation . This obviously has only one valid solution, hence the only solution is the number .Problem 13A ship sails miles in a straight line from to , turns through an angle betweenand , and then sails another miles to . Let be measured in miles.Which of the following intervals contains ?SolutionAnswering the questionTo answer the question we are asked, it is enough to compute for two differentangles, preferably for both extremes (and degrees).Alternately, it is enough to compute for one of the extreme angles. In case it falls inside one of the given intervals, we are done. In case it falls on the boundarybetween two options, we also have to argue whether our is the minimal or themaximal possible value of .Below we show a complete solution in which we also show that all possible values ofdo indeed lie in the given interval.Complete solutionLet be the point the ship would reach if it turned , and the point it wouldreach if it turned . Obviously, is the furthest possible point from , and isthe closest possible point to .Hence the interval of possible values for is .We can find and as follows:Let and be the feet of the heights from and onto . The angles in thetriangle are , , and , hence .Similarly, the angles in the triangle are , , and , henceand .Hence we get:Therefore for any valid the value is surely in the interval .Problem 14A triangle has vertices , , and , and the line divides thetriangle into two triangles of equal area. What is the sum of all possible values of ?SolutionLet's label the three points as , , and .Clearly, whenever the line intersects the inside of the triangle, it will intersect the side . Let be the point of intersection.The triangles and have the same height, which is the distance betweenthe point and the line . Hence they have equal areas if and only if is themidpoint of .The midpoint of the segment has coordinates . This point lies onthe line if and only if . This simplifies to. This is a quadratic equation with roots and .Both roots represent valid solutions, and their sum is .For illustration, below are pictures of the situation for , ,, and .Problem 15For what value of is ?Note: here .SolutionObviously, even powers of are real and odd powers of are imaginary. Hence thereal part of the sum is , and the imaginary part is.Let's take a look at the real part first. We have , hence the real partsimplifies to . If there were an odd number of terms, wecould pair them as follows: , hence the result would be negative. As we need the real part to be , we must have an even number ofterms. If we have an even number of terms, we can pair them as. Each parenthesis is equal to , thus there are ofthem, and the last value used is . This happens for and . Asis not present as an option, we may conclude that the answer is .In a complete solution, we should now verify which of and will giveus the correct imaginary part.We can rewrite the imaginary part as follows:. We need toobtain . Once again we can repeat the same reasoning: If the number of terms were even, the left hand side would be negative, thus thenumber of terms is odd. The left hand side can then be rewritten as. We need parentheses, therefore the last valueused is . This happens when or , and we are done.Problem 16A circle with center is tangent to the positive and -axes and externally tangentto the circle centered at with radius . What is the sum of all possible radii ofthe circle with center ?SolutionLet be the radius of our circle. For it to be tangent to the positive and axes, wemust have . For the circle to be externally tangent to the circle centeredat with radius , the distance between and must be exactly .By the Pythagorean theorem the distance between and is, hence we get the equation .Simplifying, we obtain . By Vieta's formulas the sum of the tworoots of this equation is .(We should actually solve for to verify that there are two distinct positive roots. Inthis case we get .)Problem 17Let and be two differentinfinite geometric series of positive numbers with the same first term. The sum of the first series is , and the sum of the second series is . What is ?SolutionUsing the formula for the sum of a geometric series we get that the sums of thegiven two sequences are and .Hence we have and . This can be rewritten as.As we are given that and are distinct, these must be precisely the two roots ofthe equation .Using Vieta's formulas we get that the sum of these two roots is .Alternate SolutionUsing the formula for the sum of a geometric series we get that the sums of thegiven two sequences are and .Hence we have and . This can be rewritten as.Which can be further rewritten as . Rearranging the equation weget . Expressing this as a difference of squares we get.Dividing by like terms we finally get as desired.Problem 18For , let , where there are zeros between the and the .Let be the number of factors of in the prime factorization of . What is themaximum value of ?SolutionThe number can be written as .For we have . The first value in the parentheses is odd, the second one is even, hence their sum is odd and we have.For we have . For the value in theparentheses is odd, hence .This leaves the case . We have . The value isobviously even. And as , we have , and therefore. Hence the largest power of that divides is , andthis gives us the desired maximum of the function : .Problem 19Andrea inscribed a circle inside a regular pentagon, circumscribed a circle around the pentagon, and calculated the area of the region between the two circles. Bethany did the same with a regular heptagon (7 sides). The areas of the two regions were and , respectively. Each polygon had a side length of . Which ofthe following is true?SolutionIn any regular polygon with side length , consider the isosceles triangle formed bythe center of the polygon and two consecutive vertices and . We are giventhat . Obviously , where is the radius of the circumcircle.Let be the midpoint of . Then , and , where is theradius of the incircle.Applying the Pythagorean theorem on the triangle , we get that .Then the area between the circumcircle and the incircle can be computed as.Hence , , and therefore .Problem 20Convex quadrilateral has and . Diagonals andintersect at , , and and have equal areas. What is?1 Problem2 Solution2.1Solution 12.2Solution 23 See alsoSolutionSolution 1Let denote the area of triangle . , so. Since trianglesand share a base, they also have the same height and thus andwith a ratio of . .Solution 2Using the sine area formula on triangles and , as ,we see thatSince , triangles and are similar. Their ratio is. Since , we must have , .Problem 21Let , where , , and are complex numbers. SupposethatWhat is the number of nonreal zeros of ?SolutionsSolution 1From the three zeroes, we have.Then.Let's do each factor case by case:: Clearly, all the fourth roots are going to be complex.▪: The real roots are , and there are two complex roots.▪: The real roots are , and there are two complex roots.So the answer is .Problem 22A regular octahedron has side length . A plane parallel to two of its opposite facescuts the octahedron into the two congruent solids. The polygon formed by theintersection of the plane and the octahedron has area , where , , and arepositive integers, and are relatively prime, and is not divisible by the square ofany prime. What is ?Solution[Unparseable or potentially dangerous asy code. Unable to convert EPSfile]If the plane divides the octahedron into two congruent solids, it goes through the center of the octahedron. As it is parallel to two opposite faces (colored above in green), it passes through the midpoints of the edges connecting the corresponding vertices of the faces. The distance between the center and any of the midpoints, aswell as the distance between any consecutive midpoints, is found to be (by midline and so forth). Thus, the intersection of the plane and the octahedron is aregular hexagon, and the answer is , and.Problem 23Functions and are quadratic, , and the graph of containsthe vertex of the graph of . The four -intercepts on the two graphs have-coordinates , , , and , in increasing order, and . Thevalue of is , where , , and are positive integers, and isnot divisible by the square of any prime. What is ?SolutionThe two quadratics are rotations of each other about . Since we are only dealing with differences of roots, we can translate them to be symmetric about. Now and . Say our translated versions of andare and , respectively, so that . Let be a root of anda root of by symmetry. Note that since they each contain each other's vertex, , , , and must be roots of alternating polynomials, so is a root of and a root ofThe vertex of is half the sum of its roots, or . We are told that the vertex of one quadratic lies on the other, soLet and divide through by , since this is a timed competition and it will drastically simplify computations. We know and that, orSo . Since , .The answer is , and.Problem 24The tower function of twos is defined recursively as follows: andfor . Let and . What is the largest integer such thatis defined?SolutionWe just look at the last three logarithms for the moment, and use the fact that. We wish to find:Now we realize that is much smaller than . So we approximate this, remembering we have rounded down, as:We have used logarithms so far. Applying more to the left of our expression,we get . Then we can apply the logarithm more times, until we get to .So our answer is approximately . But we rounded down, so that means that after logarithms we get a number slightly greater than , sowe can apply logarithms one more time. We can be sure it is small enough so that the logarithm can only be applied more time since is the largestanswer choice. So the answer is .Alternative SolutionLet where there are 's. is defined iffiff . Note , so. Thus, we seek the largest such that . Nownote thatso satisfies the inequality. Since it is the largest choice, it is the answer.ProblemThe first two terms of a sequence are and . For ,What is ?SolutionConsider another sequence such that , and.The given recurrence becomesIt follows that . Since , all terms inthe sequence will be a multiple of .Now consider another sequence such that , and. The sequence satisfies.As the number of possible consecutive two terms is finite, we know that the sequence is periodic. Write out the first few terms of the sequence until it starts to repeat.Note that and . Thus has a period of :.It follows that and . ThusOur answer is .。
amc12真题及答案

amc12真题及答案Problem 1What is the value of ?SolutionProblem 2For what value of does ?SolutionProblem 3The remainder can be defined for all real numbers and with bywhere denotes the greatest integer less than or equal to . What is the value of ?SolutionProblem 4The mean, median, and mode of the data values are all equal to . What is the value of ?SolutionProblem 5Goldbach's conjecture states that every even integer greater than 2 can be written as the sum of two prime numbers (for example, ). So far, no one has been able to prove that the conjecture is true, and no one has found a counterexample to show that the conjecture is false. What would a counterexample consist of?SolutionProblem 6A triangular array of coins has coin in the first row, coins in the second row, coins in the third row, and so on up to coins in the th row. What is the sum of the digits of ?SolutionProblem 7Which of these describes the graph of ?SolutionProblem 8What is the area of the shaded region of the given rectangle?SolutionProblem 9The five small shaded squares inside this unit square are congruent and have disjoint interiors. The midpoint of each side of the middle square coincides with one of the vertices of the other four small squares as shown. The common side length is , where and are positive integers. What is ?SolutionProblem 10Five friends sat in a movie theater in a row containing seats, numbered to from left to right. (The directions "left" and "right" are from the point of view of the people as they sit in the seats.) During the movie Ada went to the lobby to get some popcorn. When she returned, she found that Bea had moved two seatsto the right, Ceci had moved one seat to the left, and Dee and Edie had switched seats, leaving an end seat for Ada. In which seat had Ada been sitting before she got up?SolutionProblem 11Each of the students in a certain summer camp can either sing, dance, or act. Some students have more than one talent, but no student has all three talents. Thereare students who cannot sing, students who cannot dance, and students who cannot act. How many students have two ofthese talents?SolutionProblem 12In , , , and . Point lies on , and bisects . Point lies on ,and bisects . The bisectors intersect at . What is the ratio : ?SolutionProblem 13Let be a positive multiple of . One red ball and green balls are arranged in a line in random order. Let be the probability that at least of the green balls are on the same side of the red ball. Observe that andthat approaches as grows large. What is the sum of the digits of the least value of such that ?SolutionProblem 14Each vertex of a cube is to be labeled with an integer from through , with each integer being used once, in such a way that the sum of the four numbers on the verticesof a face is the same for each face. Arrangements that can be obtained from each other through rotations of the cube are considered to be the same. How many different arrangements are possible?SolutionProblem 15Circles with centers and , having radii and , respectively, lie on the same side of line and are tangent to at and , respectively, with between and . The circle with center is externally tangent to each of the other two circles. What is the area of triangle ?SolutionProblem 16The graphs of and are plotted on the same set of axes. How many points in the plane with positive -coordinates lie on two or more of the graphs?SolutionProblem 17Let be a square. Let and be the centers, respectively, of equilateral triangles with bases and each exterior to the square. What is the ratio of the area of square to the area of square ?SolutionProblem 18For some positive integer the number has positive integer divisors, including and the number How many positive integer divisors does thenumber have?SolutionProblem 19Jerry starts at on the real number line. He tosses a fair coin times. When he gets heads, he moves unit in the positive direction; when he gets tails, he moves unit in the negative direction. The probability that he reaches at some time during this process is where and are relatively prime positive integers. What is (For example, he succeeds if his sequence of tosses is ) SolutionProblem 20A binary operation has the properties that and that for all nonzero real numbers and (Here the dot represents the usual multiplication operation.) The solution to the equation can be written as where and are relatively prime positive integers. What isSolutionProblem 21A quadrilateral is inscribed in a circle of radius Three of the sides of this quadrilateral have length What is the length of its fourth side?SolutionProblem 22How many ordered triples of positive integers satisfy and ?SolutionProblem 23Three numbers in the interval are chosen independently and at random. What is the probability that the chosen numbers are the side lengths of a triangle with positive area?SolutionProblem 24There is a smallest positive real number such that there exists a positive real number such that all the roots of the polynomial are real. In fact, for this value of the value of is unique. What is the value ofSolutionProblem 25Let be a positive integer. Bernardo and Silvia take turns writing and erasing numbers on a blackboard as follows: Bernardo starts by writing the smallest perfect square with digits. Every time Bernardo writes a number, Silvia erases the last digits of it. Bernardo then writes the next perfect square, Silvia erases the last digits of it, and this process continues until the last two numbers that remain on the board differ by at least 2. Let be the smallest positive integer not written on the board. For example, if , then the numbers that Bernardo writes are , and the numbersshowing on the board after Silvia erases are and , and thus . What is the sum of the digits of ?2016 AMC 12A Answer Key1 B2 C3 B4 D5 E6 D7 D8 D9 E10 B11 E12 C13 A14 C15 D16 D17 B18 D19 B20 A21 E22 A23 C24 B25 E。
2007 AMC 12B Problems and Solution

2007 AMC 12B Problems and Solution Problem 1Isabella's house has 3 bedroom s. Each bedroom is 12 feet long, 10 feet wide, and 8 feet high. Isabella must paint the walls of all the bedrooms. Doorways and windows, which will not be painted, occupy 60 square feet in each bedroom. How many square feet of walls m ust be painted?SolutionThere are four walls in each bedroom, since she can't paint floors or ceilings. So we calculate the num ber of square feet of wall there is in one bedroom:We havethree bedrooms, so she must paint square feet of wall.Problem 2A college student drove his com pact car 120 miles home for the weekend and averaged 30 miles per gallon. On the return trip the student drove his parents' SUV and averaged only 20 miles per gallon. What was the average gas mileage, in miles per gallon, for the round trip?SolutionThe trip was miles long and took gallons. Therefore,the average m ileage wasThe point is the center of the circle circum scribed about triangle , withand , as shown. What is the degree m easure of ?SolutionProblem 4At Frank's Fruit Market, 3 bananas cost as m uch as 2 apples, and 6 apples cost as much as 4 oranges. How many oranges cost as m uch as 18 bananas?Solution18 bananas cost the sam e as 12 apples, and 12 apples cost the sam e as 8 oranges,so 18 bananas cost the sam e as oranges.The 2007 AMC 12 contests will be scored by awarding 6 points for each correct response, 0 points for each incorrect response, and 1.5 points for each problem left unanswered. After looking over the 25 problems, Sarah has decided to attem pt the first 22 and leave the last 3 unanswered. How many of the first 22 problems must she solve correctly in order to score at least 100 points?SolutionShe must get at least points, and that can only be possible byanswering at least questions correctly.Problem 6Triangle has side lengths , , and . Two bugs startsimultaneously from and crawl along the sides of the triangle in oppositedirections at the sam e speed. They m eet at point . What is ?SolutionOne bug goes to . The path that he takes is units long. The lengthof isProblem 7All sides of the convex pentagon are of equal length, and. What is the degree m easure of ?SolutionSince and are right angles, and equals , is a square, and is5. Since and are also 5, triangle is equilateral. Angle is thereforeProblem 8Tom's age is years, which is also the sum of the ages of his three children. His ageyears ago was twice the sum of their ages then. What is ?SolutionTom's age years ago was . The ages of his three children years ago wassince there are three people. If his age years ago was twice the sum ofthe children's ages then,Problem 9A function has the property that for all real numbers .What is ?SolutionProblem 10Som e boys and girls are having a car wash to raise m oney for a class trip to China.Initially % of the group are girls. Shortly thereafter two girls leave and two boys arrive, and then % of the group are girls. How many girls were initially in thegroup?SolutionIf we let be the num ber of people initially in the group, the is the number of girls. If two girls leave and two boys arrive, the number of people in the group is stillbut the num ber of girls is . Since only of the group are girls,The num ber of girls isProblem 11The angles of quadrilateral satisfy . What isthe degree m easure of , rounded to the nearest whole number?SolutionThe sum of the interior angles of any quadrilateral isProblem 12A teacher gave a test to a class in which of the students are juniors and areseniors. The average score on the test was . The juniors all received the sam e score, and the average score of the seniors was . What score did each of thejuniors receive on the test?SolutionWe can assum e there are people in the class. Then there will be junior andseniors. The sum of everyone's scores is Since the average score ofthe seniors was the sum of all the senior's scores is The only score that has not been added to that is the junior's score, which isProblem 13A traffic light runs repeatedly through the following cycle: green for seconds,then yellow for seconds, and then red for seconds. Leah picks a randomthree-second tim e interval to watch the light. What is the probability that the color changes while she is watching?SolutionThe traffic light runs through a second cycle.Letting reference the m oment it turns green, the light changes at threedifferent tim es: , , andThis m eans that the light will change if the beginning of Leah's interval lies in, orThis gives a total of seconds out ofProblem 14Point is inside equilateral . Points , , and are the feet of theperpendiculars from to , , and , respectively. Given that ,, and , what is ?SolutionDrawing , , and , is split into three sm aller triangles. Thealtitudes of these triangles are given in the problem as , , and .Summing the areas of each of these triangles and equating it to the area of the entire triangle, we get:where is the length of a sideProblem 15The geom etric series has a sum of , and the terms involving odd powers of have a sum of . What is ?SolutionSolution 1The sum of an infinite geom etric series is given by where is the first term and is the common ratio.In this series,The series with odd powers of is given asIt's sum can be given byDoing a little algebraSolution 2The given series can be decomposed as follows:Clearly . We obtain that, hence .Then from we get , and thus . Problem 16Each face of a regular tetrahedron is painted either red, white, or blue. Two colorings are considered indistinguishable if two congruent tetrahedra with those colorings can be rotated so that their appearances are identical. How many distinguishable colorings are possible?SolutionA tetrahedron has 4 sides. The ratio of the number of faces with each color must be one of the following:, , , orThe first ratio yields appearances, one of each color.The second ratio yields appearances, three choices for the first color, andtwo choices for the second.The third ratio yields appearances since the two colors areinterchangeable.The fourth ratio yields appearances. There are three choices for the first color, andsince the second two colors are interchangeable, there is only one distinguishable pair that fits them.The total is appearancesSolution 2Every colouring can be represented in the form, where is the num ber of white faces, is the number of red faces, and is the number of blue faces. Everydistinguishable colouring pattern can be represented like this in exactly one way, and every ordered whole number triple with a total sum of 4 represents exactly one colouring pattern (if two tetrahedra have rearranged colours on their faces, it is always possible to rotate one so that it m atches the other).Therefore, the number of colourings is equal to the num ber of ways 3 distinguishable nonnegative integers can add to 4. If you have 6 cockroaches in a row, this number is equal to the num ber of ways to pick two of the cockroaches to eat for dinner (because the rem aining cockroaches in between are separated in to three sections with a non-negative number of cockroaches each), which isProblem 17If is a nonzero integer and is a positive number such that , what isthe m edian of the set ?SolutionNote that if is positive, then, the equation will have no solutions for . Thisbecom es more obvious by noting that at , . The LHS quadraticfunction will increase faster than the RHS logarithmic function, so they will never intersect.This puts as the sm allest in the set since it m ust be negative.Checking the new equation:Near , but at ,This implies that the solution occurs som ewhere in between:This also implies thatThis m akes our set (ordered)The m edian isProblem 18Let , , and be digits with . The three-digit integer lies one third of theway from the square of a positive integer to the square of the next larger integer. The integer lies two thirds of the way between the sam e two squares. What is?SolutionThe difference between and is given byThe difference between the two squares is three tim es this amount orThe difference between two consecutive squares is always an odd number, therefore is odd. We will show that must be 1. Otherwise we would belooking for two consecutive squares that are at least 81 apart. But already theequation solves to , and has m ore than threedigits.The consecutive squares with common difference are and .One third of the way between them is and two thirds of the way is .This gives , , .Problem 19Rhombus , with side length , is rolled to form a cylinder of volum e bytaping to . What is ?SolutionWhere andProblem 20The parallelogram bounded by the lines , , , andhas area . The parallelogram bounded by the lines ,, , and has area . Given that , , , andare positive integers, what is the sm allest possible value of ?SolutionThis solution is incomplete. You can help us out by completing it.Plotting the parallelogram on the coordinate plane, the 4 corners are at. Because , wehave that or that , whichgives (consider a hom othety, or dilation, that carries the first parallelogram to the second parallelogram; because the area increases by , it follows that thestretch along the diagonal is ). The area of triangular half of the parallelogram onthe right side of the y-axis is given by , so substituting:Thus , and we verify that , will give us a minimum value for . Then.Solution 2This solution is incomplete. You can help us out by completing it.The key to this solution is that area is invariant under translation. By suitably shifting the plane, the problem is mapped to the linesand . Now, thearea of the parallelogram contained by is the former is equal to the area of arectangle with sides and , , and the area contained bythe latter is . Thus, and must be even if the form erquantity is to equal . so is a m ultiple of . Putting this alltogether, the minimal solution for , so the sum is . Problem 21The first positive integers are each written in base . How m any of thesebase-representations are palindromes? (A palindrome is a number that reads the sam e forward and backward.)SolutionAll numbers of six or less digits in base 3 have been written.The form of each palindrome is as follows1 digit -2 digits -3 digits -4 digits -5 digits -6 digits -Where are base 3 digitsSince , this gives a total ofpalindromes so far.7 digits - , but not all of the num bers are less thanCase:All of these numbers are less than giving more palindromesCase: ,All of these numbers are also small enough, giving more palindromesCase: ,It follows that , since any other would make the value too large. This leavesthe number as . Checking each value of d, all of the three are sm all enough, so that gives more palindromes.Summing our cases there areProblem 22Two particles m ove along the edges of equilateral in the directionstarting simultaneously and moving at the sam e speed. Onestarts at , and the other starts at the midpoint of . The midpoint of the linesegm ent joining the two particles traces out a path that encloses a region . Whatis the ratio of the area of to the area of ?SolutionFirst, notice that each of the midpoints of ,, and are on the locus.Suppose after som e time the particles have each been displaced by a short distance, to new positions and respectively. Consider and drop aperpendicular from to hit at . Then, and .From here, we can use properties of a triangle to determine thelengths and as m onomials in . Thus, the locus of the midpoint will be linear between each of the three special points m entioned above. It follows that the locus consists of the only triangle with those three points as vertices. Com paring inradii between this "midpoint" triangle and the original triangle, the area containedby must be of the total area.Problem 23How many non-congruent right triangles with positive integer leg lengths have areas that are num erically equal to tim es their perimeters?SolutionUsing Euclid's formula for generating primit ive triples: , ,where and are relatively prime positive integers, exactly one of which being even.Since we do not want to restrict ourselves to only primitives, we will add a factor ofk. , ,Now we do som e casework.Forwhich has solutions , , ,Removing the solutions that do not satisfy the conditions of Euclid's formula, theonly solutions are andForhas solutions , , both of which are valid.Forhas solutions , of which only is valid.Forhas solution , which is valid.This m eans that the solutions for aresolutionsProblem 24How many pairs of positive integers are there such that andis an integer?SolutionCombining the fraction, must be an integer.Since the denominator contains a factor of ,Rewriting as for some positive integer , we can rewrite the fraction asSince the denominator now contains a factor of , we get.But since , we must have , and thus .For the original fraction simplifies to .For that to be an integer, must divide , and therefore we must have. Each of these values does indeed yield an integer.Thus there are four solutions: , , , and the answer isProblem 25Points and are located in 3-dim ensional space withand .The plane of is parallel to . What is the area of ?SolutionLet , and . Since , we could let ,, and . Now to get back to we need another vertex. Now if we look at this configuration as if it was two dim ensions, we would see a square missing a side if we don't draw . Now we can bend thesethree sides into an equilateral triangle, and the coordinates change: ,, , , and . Checking for all the requirements, they are all satisfied. Now we find the area of triangle . Itis a triangle, which is an isosceles right triangle. Thus the area of it is.。
2013年 AMC 12B真题含答案

2013AMC12BProblem1On a particular January day,the high temperature in Lincoln,Nebraska,was degrees higher thanthe low temperature,and the average of the high and low temperatures was.In degrees,what was the low temperature in Lincoln that day?内布拉斯加州林肯市在一月份的某天,高温比低温高16度,且高温和低温的平均值是3度,问林肯市那天的低温是多少度?Problem2Mr.Green measures his rectangular garden by walking two of the sides and finds that it is stepsby steps.Each of Mr.Green’s steps is feet long.Mr.Green expects a half a pound of potatoesper square foot from his garden.How many pounds of potatoes does Mr.Green expect from his garden?格林先生通过用自己的步长来测量他的矩形花园,发现大小是15步x20步,格林先生的每一步是2英尺长,他期望他的花园每平方英尺的土地可以产出半磅的土豆,问格林先生期望他的花园总共可以产出多少磅的土豆?Problem3When counting from to,is the number counted.When counting backwardsfrom to,is the number counted.What is?当从3数到201,数到的第51个数是53,当从201倒数到3,数到的第n个数是53,问n是多少Ray's car averages miles per gallon of gasoline,and Tom's car averages miles per gallon ofgasoline.Ray and Tom each drive the same number of miles.What is the cars'combined rate of miles per gallon of gasoline?Ray的汽车每加仑汽油可以行驶40英里,Tom的汽车每加仑汽油可以行驶10英里。
2016年 AMC 12B真题含答案

2016AMC12BProblem1What is the value of when?当时,的值是多少?Problem2The harmonic mean of two numbers can be calculated as twice their product divided by their sum. The harmonic mean of and is closest to which integer?两个数的调和平均值可以由它们乘积的2倍除以它们的和得到。
那么1和2016的调和平均值最接近下面哪个整数?Problem3Let.What is the value of?令,则的值是多少?The ratio of the measures of two acute angles is,and the complement of one of these twoangles is twice as large as the complement of the other.What is the sum of the degree measures of the two angles?两个锐角的度数比值是,且其中一个角的补角是另一个角的补角的2倍,那么这2个角的度数之和是多少?Problem5The War of started with a declaration of war on Thursday,June,.The peace treaty toend the war was signed days later,on December,.On what day of the week was thetreaty signed?1812英美之战以1812年6月18日星期四的宣战开始,结束战争的和平协议的签订是在919天后的1814年12月24日,问签订协议的那天是星期几?(A)Friday|周五(B)Saturday|周六(C)Sunday|周日(D)Monday|周一(E)Tuesday|周二Problem6All three vertices of lie on the parabola defined by,with at the originand parallel to the-axis.The area of the triangle is.What is the length of?的3个顶点位于抛物线上,其中点A在原点,BC和x轴平行.,三角形的面积为64,问BC的长度为多少?Josh writes the numbers.He marks out ,skips the next number,marksout ,and continues skipping and marking out the next number to the end of the list.Then he goesback to the start of his list,marks out the first remaining number,skips the next number,marks out ,skips ,marks out ,and so on to the end.Josh continues in this manner until only one number remains.What is that number?Josh 写下一列数字1,2,3,…,99,100,他划掉1,跳过2,划掉3,并继续跳过和划掉接下来的数字,直到这列数字的末尾。
2010年 AMC 12B真题含答案
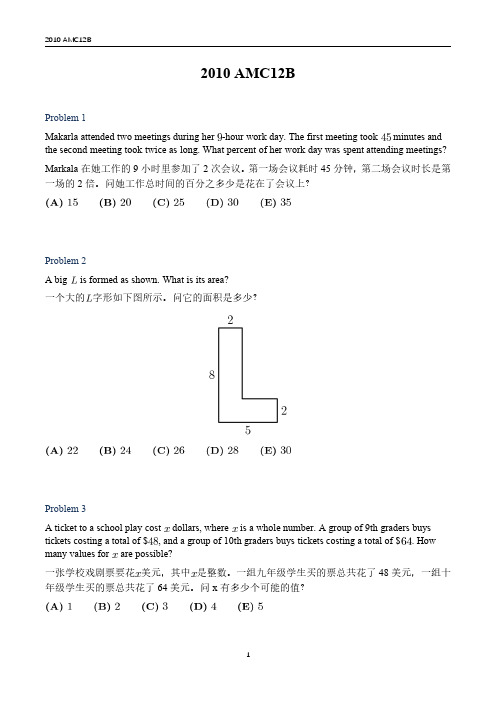
2010AMC12BProblem1Makarla attended two meetings during her-hour work day.The first meeting took minutes andthe second meeting took twice as long.What percent of her work day was spent attending meetings? Markala在她工作的9小时里参加了2次会议。
第一场会议耗时45分钟,第二场会议时长是第一场的2倍。
问她工作总时间的百分之多少是花在了会议上?Problem2A big is formed as shown.What is its area?一个大的字形如下图所示。
问它的面积是多少?Problem3A ticket to a school play cost dollars,where is a whole number.A group of9th graders buystickets costing a total of$,and a group of10th graders buys tickets costing a total of$.How many values for are possible?一张学校戏剧票要花美元,其中是整数。
一组九年级学生买的票总共花了48美元,一组十年级学生买的票总共花了64美元。
问x有多少个可能的值?A month with days has the same number of Mondays and Wednesdays.How many of the sevendays of the week could be the first day of this month?某个月共有31天,其中周一的天数和周三的天数相同。
一周7天有多少天可能成为这个月的第一天?Problem5Lucky Larry's teacher asked him to substitute numbers for,,,,and in theexpression and evaluate the rry ignored the parentheses but added and subtracted correctly and obtained the correct result by coincidence.The numbers Larry substituted for,,,and were,,,and,respectively.What number did Larry substitutefor?幸运的Lany的老师叫他分别将值代入,,,和,以求得表达式的值。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
2008 AMC 12B Problems Problem 1 A basketball player made baskets during a game. Each basket was worth either or points. How many different numbers could represent the total points scored by the player?
Solution If the basketball player makes three-point shots and two-point shots, he scores points. Clearly every value of yields a different number of total points. Since he can make any number of three-point shots between and inclusive, the number of different point totals is .
Problem 2 A block of calendar dates is shown. The order of the numbers in the second row is to be reversed. Then the order of the numbers in the fourth row is to be reversed. Finally, the numbers on each diagonal are to be added. What will be the positive difference between the two diagonal sums?
Solution After reversing the numbers on the second and fourth rows, the block will look like this: The difference between the two diagonal sums is: .
Problem 3 A semipro baseball league has teams with players each. League rules state that a player must be paid at least dollars, and that the total of all players' salaries for each team cannot exceed dollars. What is the maximum possiblle salary, in dollars, for a single player?
Solution We want to find the maximum any player could make, so assume that everyone else makes the minimum possible and that the combined salaries total the maximum of
The maximum any player could make is dollars (answer choice C) Problem 4 On circle , points and are on the same side of diameter , , and . What is the ratio of the area of the smaller sector to the area of the circle? Solution . Since a circle has , the desired ratio is .
Problem 5 A class collects dollars to buy flowers for a classmate who is in the hospital. Roses cost dollars each, and carnations cost dollars each. No other flowers are to be used. How many different bouquets could be purchased for exactly dollars?
Solution The class could send just carnations (25 of them). They could also send 22 carnations and 2 roses, 19 carnations and 4 roses, and so on, down to 1 carnation and 16 roses. There are 9 total possibilities (from 0 to 16 roses, incrementing by 2 at each step), which is answer choice C.
Problem 6 Postman Pete has a pedometer to count his steps. The pedometer records up to steps, then flips over to on the next step. Pete plans to determine his mileage for a year. On January Pete sets the pedometer to . During the year, the pedometer flips from to forty-four times. On December the pedometer reads . Pete takes steps per mile. Which of the following is closest to the number of miles Pete walked during the year?
Solution Every time the pedometer flips, Pete has walked steps. Therefore, Pete has walked a total of steps, which is
miles, which is closest to answer choice A.
Problem 7 For real numbers and , define . What is ?
Solution
Problem 8 Points and lie on . The length of is times the length of , and the length of is times the length of . The length of is what fraction of the length of ?
Solution Since and , . Since and , . Thus, . Problem 9 Points and are on a circle of radius and . Point is the midpoint of the minor arc . What is the length of the line segment ?
Solution Trig Solution: Let be the angle that subtends the arc AB. By the law of cosines,
The half-angle formula says that
, which is answer choice A. Other Solution
Define D as the midpoint of AB, and R the center of the circle. R, C, and D are collinear, and since D is the midpoint of AB, , and so
. Since , , and so Problem 10 Bricklayer Brenda would take hours to build a chimney alone, and bricklayer Brandon would take hours to build it alone. When they work together they talk a lot, and their combined output is decreased by bricks per hour. Working together, they build the chimney in hours. How many bricks are in the chimney?
Solution Let be the number of bricks in the house. Without talking, Brenda and Brandon lay and bricks per hour respectively, so together they lay per hour together.
Since they finish the chimney in hours, . Thus, .
Problem 11 A cone-shaped mountain has its base on the ocean floor and has a height of 8000 feet. The top of the volume of the mountain is above water. What is the depth of the ocean at the base of the mountain in feet?
Solution In a cone, radius and height each vary inversely with increasing height (i.e. the radius of the cone formed by cutting off the mountain at feet is half that of the original mountain). Therefore, volume varies as the inverse cube of increasing
