Igcse 数学 历年真题 2
2011 June igcse 英文数学试卷

This document consists of 19 printed pages and 1 blank page.IB11 06_0580_43/4RP© UCLES 2011[Turn over*8044643715*UNIVERSITY OF CAMBRIDGE INTERNATIONAL EXAMINATIONS International General Certificate of Secondary EducationMATHEMATICS 0580/43Paper 4 (Extended) May/June 20112 hours 30 minutesCandidates answer on the Question Paper.Additional Materials: Electronic calculatorGeometrical instrumentsMathematical tables (optional)Tracing paper (optional)READ THESE INSTRUCTIONS FIRSTWrite your Centre number, candidate number and name on all the work you hand in. Write in dark blue or black pen.You may use a pencil for any diagrams or graphs.Do not use staples, paper clips, highlighters, glue or correction fluid. DO NOT WRITE IN ANY BARCODES.Answer all questions.If working is needed for any question it must be shown below that question. Electronic calculators should be used.If the degree of accuracy is not specified in the question, and if the answer is not exact, give the answer to three significant figures. Give answers in degrees to one decimal place. For π use either your calculator value or 3.142.At the end of the examination, fasten all your work securely together.The number of marks is given in brackets [ ] at the end of each question or part question. The total of the marks for this paper is 130.© UCLES 20110580/43/M/J/11For Examiner's Use1 Lucy works in a clothes shop.(a) In one week she earned $277.20.(i) She spent 81of this on food.Calculate how much she spent on food. Answer(a)(i) $ [1](ii) She paid 15% of the $277.20 in taxes. Calculate how much she paid in taxes. Answer(a)(ii) $ [2](iii) The $277.20 was 5% more than Lucy earned in the previous week. Calculate how much Lucy earned in the previous week. Answer(a)(iii) $ [3](b) The shop sells clothes for men, women and children.(i) In one day Lucy sold clothes with a total value of $2200 in the ratio men : women : children = 2 : 5 : 4. Calculate the value of the women’s clothes she sold. Answer(b)(i) $ [2](ii) The $2200 was 7344of the total value of the clothes sold in the shop on this day. Calculate the total value of the clothes sold in the shop on this day. Answer(b)(ii) $ [2]© UCLES 2011 0580/43/M/J/11[Turn overUsex(a) (i) Draw the reflection of shape X in the x -axis. Label the image Y . [2](ii) Draw the rotation of shape Y , 90° clockwise about (0, 0). Label the image Z . [2](iii) Describe fully the single transformation that maps shape Z onto shape X .Answer(a)(iii)[2](b) (i) Draw the enlargement of shape X , centre (0, 0), scale factor21. [2](ii) Find the matrix which represents an enlargement, centre (0, 0), scale factor 21.Answer(b)(ii)[2](c) (i) Draw the shear of shape X with the x -axis invariant and shear factor –1.[2](ii) Find the matrix which represents a shear with the x -axis invariant and shear factor –1.Answer(c)(ii)[2]© UCLES 20110580/43/M/J/11Use(x + 5) cm2x cmx cmNOT TO SCALEThe diagram shows a square of side (x + 5) cm and a rectangle which measures 2x cm by x cm. The area of the square is 1 cm 2 more than the area of the rectangle.(a) Show that x 2 – 10x – 24 = 0 . Answer(a) [3]© UCLES 2011 0580/43/M/J/11[Turn overFor Examiner's Use(b) Find the value of x . Answer(b) x = [3](c) Calculate the acute angle between the diagonals of the rectangle. Answer(c) [3]© UCLES 2011 0580/43/M/J/11For Examiner's Use4NOT TO SCALEThe circle, centre O , passes through the points A , B and C . In the triangle ABC , AB = 8 cm, BC = 9 cm and CA = 6 cm. (a) Calculate angle BAC and show that it rounds to 78.6°, correct to 1 decimal place. Answer(a) [4](b) M is the midpoint of BC .(i) Find angle BOM . Answer(b)(i) Angle BOM = [1]© UCLES 2011 0580/43/M/J/11[Turn overFor Examiner's Use(ii) Calculate the radius of the circle and show that it rounds to 4.59 cm, correct to 3 significantfigures.Answer(b)(ii) [3](c) Calculate the area of the triangle ABC as a percentage of the area of the circle. Answer(c) % [4]© UCLES 2011 0580/43/M/J/11ForExaminer's Use5 (a) Complete the table of values for the function f(x ), where f(x ) = x 2 + 21x , x ≠ 0 .xO 3 O 2.5 O 2 O 1.5 O 1 O 0.50.5 1 1.5 2 2.5 3 f(x ) 6.41 2.69 4.25 4.252.69 6.41[3](b) On the grid, draw the graph of y = f(x ) for O 3 Y x Y O 0.5 and 0.5 Y x Y 3 .[5]© UCLES 2011 0580/43/M/J/11[Turn overFor Examiner's Use(c) (i) Write down the equation of the line of symmetry of the graph.Answer(c)(i)[1](ii) Draw the tangent to the graph of y = f(x ) where x = O 1.5. Use the tangent to estimate the gradient of the graph of y = f(x ) where x = O 1.5. Answer(c)(ii) [3](iii) Use your graph to solve the equation x 2 + 21x= 3.Answer(c)(iii) x = or x = or x = or x = [2](iv) Draw a suitable line on the grid and use your graphs to solve the equation x 2 + 21x = 2x .Answer(c)(iv) x =or x =[3]© UCLES 2011 0580/43/M/J/11For Examiner's Use6CumulativefrequencyMass (kilograms)mThe masses of 200 parcels are recorded. The results are shown in the cumulative frequency diagram above.(a) Find(i) the median, Answer(a)(i) kg [1](ii) the lower quartile, Answer(a)(ii) kg [1](iii) the inter-quartile range, Answer(a)(iii) kg [1](iv) the number of parcels with a mass greater than 3.5 kg. Answer(a)(iv) [2]© UCLES 2011 0580/43/M/J/11[Turn overFor Examiner's Use(b) (i) Use the information from the cumulative frequency diagram to complete the groupedfrequency table.Mass (m ) kg0 I m Y 44 I m Y 66 I m Y 77 I m Y 10Frequency 36 50[2](ii) Use the grouped frequency table to calculate an estimate of the mean. Answer(b)(ii) kg [4](iii) Complete the frequency density table and use it to complete the histogram.Mass (m ) kg 0 I m Y 4 4 I m Y 6 6 I m Y 7 7 I m Y 10Frequency density916.7FrequencydensityMass (kilograms)m[4]© UCLES 20110580/43/M/J/11ForExaminer's Use7 Katrina puts some plants in her garden.The probability that a plant will produce a flower is107. If there is a flower, it can only be red, yellow or orange.When there is a flower, the probability it is red is 32 and the probability it is yellow is 41.(a) Draw a tree diagram to show all this information. Label the diagram and write the probabilities on each branch. Answer(a) [5](b) A plant is chosen at random. Find the probability that it will not produce a yellow flower. Answer(b) [3](c) If Katrina puts 120 plants in her garden, how many orange flowers would she expect? Answer(c) [2]© UCLES 2011 0580/43/M/J/11[Turn overFor Examiner's Use8A(a) Draw accurately the locus of points, inside the quadrilateral ABCD , which are 6 cm from thepoint D . [1](b) Using a straight edge and compasses only, construct(i) the perpendicular bisector of AB , [2](ii) the locus of points, inside the quadrilateral, which are equidistant from AB and from BC . [2](c) The point Q is equidistant from A and from B and equidistant from AB and from BC .(i) Label the point Q on the diagram. [1](ii) Measure the distance of Q from the line AB . Answer(c)(ii) cm [1](d) On the diagram, shade the region inside the quadrilateral which is• less than 6 cm from Dand• nearer to A than to Band• nearer to AB than to BC . [1]© UCLES 2011 0580/43/M/J/11For Examiner's Use9 f(x ) = 3x + 1 g(x ) = (x + 2)2(a) Find the values of(i) gf(2), Answer(a)(i)[2](ii) ff(0.5). Answer(a)(ii)[2](b) Find f –1(x ), the inverse of f(x ). Answer(b)[2](c) Find fg(x ). Give your answer in its simplest form. Answer(c)[2]© UCLES 2011 0580/43/M/J/11[Turn overFor Examiner's Use(d) Solve the equation x 2 + f(x ) = 0. Show all your working and give your answers correct to 2 decimal places. Answer(d) x = or x =[4]UseBABCD is a parallelogram.DC, M is the midpoint of BC and N is the midpoint of LM.pq.(i)Find the following in terms ofp and q, in their simplest form.(a)Answer(a)[1](b)Answer(a)[2](c)Answer(a)[2] (ii) N lies on the line AC.Answer(a)(ii) [1]© UCLES 2011 0580/43/M/J/11© UCLES 2011 0580/43/M/J/11[Turn overUseEH J2x°75°(x + 15)°NOT TO SCALEEFG is a triangle. HJ is parallel to FG . Angle FEG = 75°. Angle EFG = 2x ° and angle FGE = (x + 15)°.(i) Find the value of x . Answer(b)(i) x = [2](ii) Find angle HJG . Answer(b)(ii) Angle HJG = [1]© UCLES 2011 0580/43/M/J/11For Examiner's Use11 (a) (i) The first three positive integers 1, 2 and 3 have a sum of 6. Write down the sum of the first 4 positive integers. Answer(a)(i) [1](ii) The formula for the sum of the first n integers is21)(+n n . Show the formula is correct when n = 3. Answer(a)(ii) [1](iii) Find the sum of the first 120 positive integers. Answer(a)(iii) [1](iv) Find the sum of the integers121 + 122 + 123 + 124 + …………………………… + 199 + 200.Answer(a)(iv)[2](v) Find the sum of the even numbers 2 + 4 + 6 + …………………………+ 800.Answer(a)(v)[2]© UCLES 20110580/43/M/J/11For Examiner's Use(b) (i) Complete the following statements about the sums of cubes and the sums of integers.13 = 1 1 = 113 + 23 = 9 1 + 2 = 3 13 + 23 + 33 =1 +2 +3 =13 + 23 + 33 + 43 =1 +2 +3 +4 =[2](ii) The sum of the first 14 integers is 105. Find the sum of the first 14 cubes. Answer(b)(ii) [1](iii) Use the formula in part(a)(ii) to write down a formula for the sum of the first n cubes. Answer(b)(iii) [1](iv) Find the sum of the first 60 cubes. Answer(b)(iv) [1](v) Find n when the sum of the first n cubes is 278 784. Answer(b)(v) n = [2]BLANK PAGEPer mission to r epr oduce items wher e thir d-par ty owned mater ial pr otected by copyr ight is included has been sought and clear ed wher e possible. Ever y reasonable effort has been made by the publisher (UCLES) to trace copyright holders, but if any items requiring clearance have unwittingly been included, the publisher will be pleased to make amends at the earliest possible opportunity.University of Cambridge International Examinations is part of the Cambridge Assessment Group. Cambridge Assessment is the brand name of University of Cambridge Local Examinations Syndicate (UCLES), which is itself a department of the University of Cambridge.© UCLES 2011 0580/43/M/J/11。
IGCSE数学
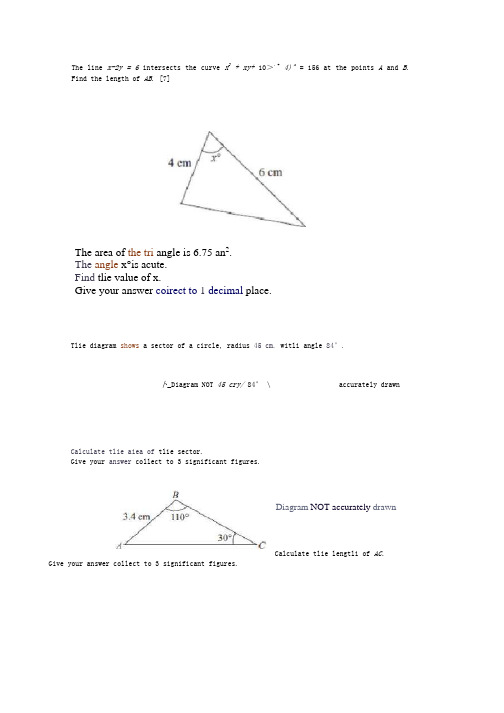
The line x-2y = 6 intersects the curve x2 + xy+ 10>,+4)^ = 156 at the points A and B.Find the length of AB. [7]The area of the tri angle is 6.75 an2.The angle x°is acute.Find tlie value of x.Give your answer coirect to 1 decimal place.Tlie diagram shows a sector of a circle, radius 45 cm. witli angle 84°.卜_Diagram NOT 45 cry/84° \ accurately drawnCalculate tlie aiea of tlie sector.Give your answer collect to 3 significant figures.Diagram NOT accurately drawnCalculate tlie lengtli of AC.Give your answer collect to 3 significant figures.A cone has slant height 4 cm and base radius r cm.In the diagram the lines AB and CD are parallel. The lines AD and BC intersect at X.Angle XDC = 35。
and angle CXD = 120°. (a) (i) Write down the size of angle BAX.Answer(a)( i) Angle BAX = .................................. [........................................................................... 1 ](ii) Write down the size of angle ABX.Answer(a)( ii) Angle ABX = ............................... [1 .............................................................................. ](b) Complete the statementTriangle AXB is .................................................... t o triangle DXC. [1 ](c) AB — 8.3cm, BX — 5.5cm and CD — 16.6cm.Calculate the length of CX.Diagram NOT accurately drawnTlie total surface area of tlie cone is33 2—兀 cnr.4Calculate the value of r.NOT TOSCALEIn quadrilateral ABCD, AB = 77 m, BC = 120 m, CD = 60 m and diagonal AC = 55 m. Angle CAD = 45°, angle BAC = x° and angle ADC = y°. (a) Calculate the value of x. (b) Calculate the value of y.(c) The bearing of D from A is 090°. Find the bearing of(i) A from C, (ii) B from A.NOTTG SCALEDiagram NOT accurately drawnA, B, C and D are four points on the circumference of a circle. The chords AC and BDintersect at E.AE =3.6 ctn. CE = 2.8 cm, DE = 2.4 cm and AD = 4.9 cm. (a' Calculate the length of BE.(b‘ Calculate the size of angle AED.Give your answer correct to 3 significant figures, cABCD is a cyclic quadrilateral.AB = 9.5cm, ffC=lLlcm, angle ABC = 70°and angle CAD = 37°. (a) Calculate the length of AC. (b) Explain why angle ADC = Il0°. (c) Calculate the length of AD.(d) A point E lies on the circle such that triangle ACE is isosceles, with EA = EC.(i) Write down the size of angle A£C. <(ii) Calculate the area of triangle ACE.NOT TO SCALE44°Diagram NOTaccurately drawnL08°Q, R, sand y are points on the circumference of a circle. PU is a tangent io the circle at J.PQR is a straight line.Angle PQT=L08°.Angle STR = 44。
IGCSE真题_20140116
Turn over P42864A©2014 Pearson Education Ltd.1/1/1/1/*P42864A0120*Instructionst Use black ink or ball-point pen.t Fill in the boxes at the top of this page with your name,centre number and candidate number.t Answer all questions.t A nswer the questions in the spaces provided– there may be more space than you need.t Show all the steps in any calculations and state the units.t SInformationt The total mark for this paper is 60.t T he marks for each question are shown in brackets– use this as a guide as to how much time to spend on each question.Advicet Read each question carefully before you start to answer it.t Keep an eye on the time.t Write your answers neatly and in good English.t Try to answer every question.t Check your answers if you have time at the end.2*P42864A0220*3*P42864A0320*Turn overBLANK PAGE4*P42864A0420*5*P42864A0520*Turn over6*P42864A0620*2 Bromine, chlorine, fluorine and iodine are elements in Group 7 of the Periodic Table.(a) Which two of these elements have the darkest colours?(1)....................................................................................................................................and ....................................................................................................................................(b) The equation for the reaction between hydrogen and chlorine isH 2 + Cl 2 o 2HClDifferent names are used for the product, depending on its state symbol.(i) What are the names used for HCl(g) and HCl(aq)?(2)HCl(g) .................................................................................................................................................................................................................................................................HCl(aq) ............................................................................................................................................................................................................................................................... (ii) The presence of HCl(g) can be confirmed by adding ammonia (NH 3) gas.State the observation in the reaction between HCl(g) and ammonia gas and write a chemical equation for the reaction.(2)observation ......................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................chemical equation ................................................................................................................................................................................................................................ (iii) The presence of chloride ions in HCl(aq) can be shown by mixing it with silvernitrate solution and dilute nitric acid.State the result of this test and complete the chemical equation for the reaction by adding the state symbols.(3)result .......................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................AgNO 3(.......................) + HCl(aq) o AgCl(.......................) + HNO 3(.......................)7*P42864A0720*Turn over8*P42864A0820*3 Tungsten is a useful metal. It has the chemical symbol W. (a) One method of extracting tungsten involves heating a tungsten compound (WO 3)with hydrogen.(i) Suggest the chemical name of WO 3(1)....................................................................................................................................................................................................................................................................................(ii) Balance the equation for the reaction between WO 3 and hydrogen.(1)WO 3 + ............................H 2 o ............................W + ............................H 2O(iii) Why is this reaction described as reduction?(1)........................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................(b) Scheelite is an ore of tungsten. The main compound in scheelite has the percentage composition by mass Ca = 13.9%, W = 63.9%, O = 22.2%.Calculate the empirical formula of this compound.(3)empirical formula = ...................................................9*P42864A0920*Turn over(c) Tungsten can also be obtained by reacting tungsten fluoride with hydrogen.The equation for this reaction isWF 6 + 3H 2 o W + 6HF(i) In an experiment, a chemist used 59.6g of tungsten fluoride. What is the maximum mass of tungsten he could obtain from 59.6 g of tungsten fluoride?Relative formula mass of tungsten fluoride = 298(2)maximum mass = ................................................... g(ii) Starting with a different mass of tungsten fluoride, he calculates that the massof tungsten formed should be 52.0 g. In his experiment he actually obtains 47.5 g of tungsten.What is the percentage yield of tungsten in this experiment?(2)percentage yield = ................................................... %(Total for Question 3 = 10 marks)10*P42864A01020*4 A student investigated the neutralisation of acids by measuring the temperature changeswhen alkalis were added to acids of known concentrations.He used this apparatus to add different volumes of sodium hydroxide solution to a fixed volume of dilute nitric acid.He used this method. Ɣ measure the temperature of 25.0 cm 3 of the acid in the polystyrene cupƔ add the sodium hydroxide solution in 5.0 cm 3 portions until a total of 30.0 cm 3has been added(a) State two properties of the sodium hydroxide solution that should be kept constantfor each 5.0 cm 3 portion.(2)1 ...................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................2 ...................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................polystyrene cup11*P42864A01120*Turn over12*P42864A01220*13*P42864A01320*Turn over14*P42864A01420*(f) Another student used sulfuric acid instead of nitric acid in her experiments.She started with 25.0 cm 3 of sulfuric acid of concentration 0.650 mol/dm 3. She added 0.500 mol/dm 3 sodium hydroxide solution until the acid was completely neutralised.The equation for this reaction is2NaOH + H 2SO 4 o Na 2SO 4 + 2H 2O(i) Calculate the amount, in moles, of sulfuric acid used.(2)amount = ................................. mol(ii) Calculate the amount, in moles, of sodium hydroxide needed to neutralise thisamount of sulfuric acid.(1)amount = ................................. mol(iii) Calculate the volume, in cm 3, of sodium hydroxide solution needed to neutralisethis amount of sulfuric acid.(2)volume = ................................. cm 3(Total for Question 4 = 18 marks)15*P42864A01520*Turn over16*P42864A01620*(c) The equation for one reaction that could occur in process 2 is C x H y o C 5H 12 + 2C 2H 4(i) Deduce the formula of C x H y(1)....................................................................................................................................................................................................................................................................................(ii) Give the name of the compound C 5H 12(1)....................................................................................................................................................................................................................................................................................(iii) Draw the displayed formula of C 2H 4(1)(d) The structural formula of chloroethene formed in process 3 is CH 2CHClThe polymer formed in process 4 is poly(chloroethene).Draw the displayed formula for the repeat unit of poly(chloroethene).(2)17*P42864A01720*(e) Poly(chloroethene) is formed by addition polymerisation. Nylon is formed by condensation polymerisation.(i) How does condensation polymerisation differ from addition polymerisation?(1)........................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................(ii) Poly(chloroethene) and nylon do not biodegrade easily.What is meant by the term biodegrade ?(2)................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................(iii) What feature of addition polymers makes it difficult for them to biodegrade?(1)........................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................(Total for Question 5 = 13 marks)(TOTAL FOR PAPER = 60 MARKS)18*P42864A01820*BLANK PAGE19*P42864A01920*BLANK PAGE20*P42864A02020*BLANK PAGE。
0606_m19_qp_12(IGCSE 数学考试2019年3月真题

Identities Formulae for ∆ABC
2. TRIGONOMETRY
sin2 A + cos2 A = 1 sec2 A = 1 + tan2 A cosec2 A = 1 + cot2 A
a sin A
=
b sin B
=
c sin C
a2 = b2 + c2 – 2bc cos A
At the end of the examination, fasten all your work securely together. The number of marks is given in brackets [ ] at the end of each question or part question. The total number of marks for this paper is 80.
∆
=
1 2
bc
sin
A
© UCLES 2019
0606/12/F/M/19
3
1 (a) Given that
பைடு நூலகம்
= { x : 1 1 x 1 20},
A = {multiples of 3},
B = {multiples of 4},
find
(i)
n (A),
[1]
(ii)
n (A + B) .
[1]
*6715046212*
Cambridge Assessment International Education Cambridge International General Certificate of Secondary Education
Igcse-数学-历年真题

4400/4HEdexcel IGCSEMathematicsPaper 4HHigher TierFriday 11 June 2010 – AfternoonTime: 2 hoursMaterials required for examination Items included with question papersRuler graduated in centimetres and Nilmillimetres, protractor, compasses,pen, HB pencil, eraser, calculator.Tracing paper may be used.Instructions to CandidatesIn the boxes above, write your centre number, candidate number, your surname, initials and signature.Check that you have the correct question paper.Answer ALL the questions. Write your answers in the spaces provided in this question paper.You must NOT write on the formulae page. Anything you write on the formulae page will gain NO credit.If you need more space to complete your answer to any question, use additional answer sheets.Information for CandidatesThe marks for individual questions and the parts of questions are shown in round brackets: e.g. (2).There are 22 questions in this question paper. The total mark for this paperis 100.You may use a calculator.Advice to CandidatesWrite your answers neatly and in good English.N36905AIGCSE MATHEMATICS 4400 FORMULA SHEET – HIGHER TIERAnswer ALL TWENTY TWO questions.Write your answers in the spaces provided.You must write down all stages in your working.1. Solve 6 y – 9 = 3 y + 7y = ................................(Total 3 marks) 2. The diagram shows two towns, A and B, on a map.(a) By measurement, find the bearing of B from A.....................................(2)C is another town.The bearing of C from A is 050.(b) Find the bearing of A from C.....................................(2)(Total 4 marks)3. A spinner can land on red or blue or yellow.The spinner is biased.The probability that it will land on red is 0.5The probability that it will land on blue is 0.2Imad spins the spinner once.(a) Work out the probability that it will land on yellow......................................(2)Janet spins the spinner 30 times.(b)Work out an estimate for the number of times the spinner will land on blue......................................(2)(Total 4 marks)4. Rosetta drives 85 kilometres in 1 hour 15 minutes.(a) Work out her average speed in kilometres per hour...................................... km/h(2)Rosetta drives a total distance of 136 kilometres.(b) Work out 85 as a percentage of 136................................. %(2)Sometimes Rosetta travels by train to save money.The cost of her journe y by car is £12The cost of her journey by train is 15% less than the cost of her journey by car.(c)Work out the cost of Rosetta’s journey by train.£ ...................................(3)(Total 7 marks)5.Calculate the value of x.Give your answer correct to 3 significant figures.x = ................................(Total 3 marks)6. A = {2, 3, 4, 5}B = {4, 5, 6, 7}(a)(i) List the members of A B......................................(ii) How many members are in A B?.....................................(2)ℰ = {3, 4, 5, 6, 7}P = {3, 4, 5}Two other sets, Q and R, each contain exactly three members.P Q = {3, 4}P R = {3, 4}Set Q is not the same as set R.(b)(i) Write down the members of a possible set Q......................................(ii) Write down the members of a possible set R......................................(2)(Total 4 marks)7. Rectangular tiles have width (x + 1) cm and height (5x – 2) cm.Some of these tiles are used to form a large rectangle.The large rectangle is 7 tiles wide and 3 tiles high.The perimeter of the large rectangle is 68 cm.(a) Write down an equation in x...............................................................................................................(3)(b) Solve this equation to find the value of x.x = ................................(3)(Total 6 marks)8. Show that 121 141 = 1519. The depth of water in a reservoir increases from 14 m to 15.75 m.Work out the percentage increase.................................. %(Total 3 marks)10. Quadrilaterals ABCD and PQRS are similar.AB corresponds to PQ.BC corresponds to QR.CD corresponds to RS.Find the value of(a) xx = ...............................(2)(b) yy = ...............................(1)(Total 3 marks)11. Simplify fully6x + 43x.....................................(Total 3 marks)12.(a)Find the equation of the line L......................................(3)(b) Find the three inequalites that define the unshaded region shown in the diagram below................................................................................................................(3)(Total 6 marks)13. (a) Solve x 2– 8x + 12 = 0.....................................(3)(b) Solve the simultaneous equationsy = 2x4x – 5y = 9x = ................................y = ................................(3)(Total 6 marks)14.The area of the triangle is 6.75 cm2.The angle x° is acute.Find the value of x.Give your answer correct to 1 decimal place.x = ................................(Total 3 marks)15. The unfinished histogram shows information about the heights, h metres, ofsome trees.(a) Calculate an estimate for the number of trees with heights in theinterval 4.5 < h ≤ 10.....................................(3)(b) There are 75 trees with heights in the interval 10 < h ≤ 13Use this information to complete the histogram.(2)(Total 5 marks)16. A bag contains 3 white discs and 1 black disc.John takes at random 2 discs from the bag without replacement.(a) Complete the probability tree diagram.First disc Second disc(3)(b)Find the probability that both discs are white......................................(2)All the discs are now replaced in the bag.Pradeep takes at random 3 discs from the bag without replacement.(c)Find the probability that the disc left in the bag is white......................................(3)(Total 8 marks)17. The diagram shows a sector of a circle, radius 45 cm, with angle 84°.Calculate the area of the sector.Give your answer correct to 3 significant figures.............................. cm2(Total 3 marks) 18.Calculate the length of AC.Give your answer correct to 3 significant figures................................ cm(Total 3 marks)19. A cone has slant height 4 cm and base radius r cm.The total surface area of the cone is 433π cm 2.Calculate the value of r .r = ................................(Total 4 marks)20. f(x) = (x – 1)2(a) Find f(8).....................................(1)The domain of f is all values of x where x ≥ 7(a)Find the range of f......................................(2)xg(x) =x1(c) Solve the equation g(x) = 1.2.....................................(2)(d) (i) Express the inverse function g –1 in the form g –1(x) = .......g –1(x) = ...................................(ii) Hence write down gg(x) in terms of x.gg(x) = ....................................(6)(Total 11 marks)21.In the diagram OA= a and OC= c.(a) Find CA in terms of a and c......................................(1)The point B is such that AB=1c.2(b) Give the mathematical name for the quadrilateral OABC......................................(1)The point P is such that OP= a + k c, where k ≥ 0(c) State the two conditions relating to a + k c that must be true for OAPCto be a rhombus.(2)(Total 4 marks)22. (a) Work out 5.2 × 102+ 2.3 × 104Give your answer in standard form......................................(2)a × 102 +b × 104 =c × 104(b) Express c in terms of a and b.c = ................................(2)(Total 4 marks)TOTAL FOR PAPER = 100 MARKSEND。
Igcse 数学 历年真题

4400/4HEdexcel IGCSEMathematicsPaper 4HHigher TierFriday 11 June 2010 – AfternoonTime: 2 hoursMaterials required for examination Items included with question papersRuler graduated in centimetres and Nilmillimetres, protractor, compasses,pen, HB pencil, eraser, calculator.Tracing paper may be used.Instructions to CandidatesIn the boxes above, write your centre number, candidate number, your surname, initials and signature.Check that you have the correct question paper.Answer ALL the questions. Write your answers in the spaces provided in this question paper.You must NOT write on the formulae page. Anything you write on the formulae page will gain NO credit.If you need more space to complete your answer to any question, use additional answer sheets.Information for CandidatesThe marks for individual questions and the parts of questions are shown in round brackets: . (2).There are 22 questions in this question paper. The total mark for this paperis 100.You may use a calculator.Advice to CandidatesWrite your answers neatly and in good English.This publication may be reproduced only in accordance with Edexcel Limited copyright policy.©2010 Edexcel Limited.Printer’s Log. No. N36905AIGCSE MATHEMATICS 4400 FORMULA SHEET – HIGHER TIERAnswer ALL TWENTY TWO questions.Write your answers in the spaces provided.You must write down all stages in your working.1. Solve 6 y – 9 = 3 y + 7y = ................................(Total 3 marks) 2. The diagram shows two towns, A and B, on a map.(a) By measurement, find the bearing of B from A.....................................(2)C is another town.The bearing of C from A is 050.(b) Find the bearing of A from C.....................................(2)(Total 4 marks)3. A spinner can land on red or blue or yellow.The spinner is biased.The probability that it will land on red isThe probability that it will land on blue isImad spins the spinner once.(a) Work out the probability that it will land on yellow......................................(2)Janet spins the spinner 30 times.(b)Work out an estimate for the number of times the spinner will land on blue......................................(2)(Total 4 marks)4. Rosetta drives 85 kilometres in 1 hour 15 minutes.(a) Work out her average speed in kilometres per hour...................................... km/h(2)Rosetta drives a total distance of 136 kilometres.(b) Work out 85 as a percentage of 136................................. %(2)Sometimes Rosetta travels by train to save money.The cost of her journey by car is £12The cost of her journey by train is 15% less than the cost of her journey by car.(c)Work out the cost of Rosetta’s journ ey by train.£ ...................................(3)(Total 7 marks)5.Calculate the value of x.Give your answer correct to 3 significant figures.x = ................................(Total 3 marks)6. A = {2, 3, 4, 5}B = {4, 5, 6, 7}(a)(i) List the members of A B......................................(ii) How many members are in A B?.....................................(2)ℰ = {3, 4, 5, 6, 7}P = {3, 4, 5}Two other sets, Q and R, each contain exactly three members.P Q = {3, 4}P R = {3, 4}Set Q is not the same as set R.(b)(i) Write down the members of a possible set Q......................................(ii) Write down the members of a possible set R......................................(2)(Total 4 marks)7. Rectangular tiles have width (x + 1) cm and height (5x – 2) cm.Some of these tiles are used to form a large rectangle.The large rectangle is 7 tiles wide and 3 tiles high.The perimeter of the large rectangle is 68 cm.(a) Write down an equation in x...............................................................................................................(3)(b) Solve this equation to find the value of x.x = ................................(3)(Total 6 marks)8. Show that 121 141 = 1519. The depth of water in a reservoir increases from 14 m to m.Work out the percentage increase.................................. %(Total 3 marks)10. Quadrilaterals ABCD and PQRS are similar.AB corresponds to PQ.BC corresponds to QR.CD corresponds to RS.Find the value of(a) xx = ...............................(2)(b) yy = ...............................(1)(Total 3 marks)11. Simplify fully6x + 43x.....................................(Total 3 marks)12.(a)Find the equation of the line L......................................(3)(b) Find the three inequalites that define the unshaded region shown in the diagram below................................................................................................................(3)(Total 6 marks)13. (a) Solve x 2– 8x + 12 = 0.....................................(3)(b) Solve the simultaneous equationsy = 2x4x – 5y = 9x = ................................y = ................................(3)(Total 6 marks)14.The area of the triangle is cm2.The angle x° is acute.Find the value of x.Give your answer correct to 1 decimal place.x = ................................(Total 3 marks)15. The unfinished histogram shows information about the heights, h metres, ofsome trees.(a) Calculate an estimate for the number of trees with heights in theinterval < h ≤ 10.....................................(3)(b) There are 75 trees with heights in the interval 10 < h ≤ 13Use this information to complete the histogram.(2)(Total 5 marks)16. A bag contains 3 white discs and 1 black disc.John takes at random 2 discs from the bag without replacement.(a) Complete the probability tree diagram.First disc Second disc(3)(b)Find the probability that both discs are white......................................(2)All the discs are now replaced in the bag.Pradeep takes at random 3 discs from the bag without replacement.(c)Find the probability that the disc left in the bag is white......................................(3)(Total 8 marks)17. The diagram shows a sector of a circle, radius 45 cm, with angle 84°.Calculate the area of the sector.Give your answer correct to 3 significant figures.............................. cm2(Total 3 marks) 18.Calculate the length of AC.Give your answer correct to 3 significant figures................................ cm(Total 3 marks)19. A cone has slant height 4 cm and base radius r cm.The total surface area of the cone is 433π cm 2.Calculate the value of r .r = ................................(Total 4 marks)20. f(x) = (x – 1)2(a) Find f(8).....................................(1)The domain of f is all values of x where x ≥ 7(a)Find the range of f......................................(2)xg(x) =x1(c) Solve the equation g(x) =.....................................(2)(d) (i) Express the inverse function g –1 in the form g –1(x) = .......g –1(x) = ...................................(ii) Hence write down gg(x) in terms of x.gg(x) = ....................................(6)(Total 11 marks)21.In the diagram OA= a and OC= c.(a) Find CA in terms of a and c......................................(1)The point B is such that AB=1c.2(b) Give the mathematical name for the quadrilateral OABC......................................(1)The point P is such that OP= a + k c, where k ≥ 0(c) State the two conditions relating to a + k c that must be true for OAPCto be a rhombus.(2)(Total 4 marks)22. (a) Work out × 102+ × 104Give your answer in standard form......................................(2)a × 102 +b × 104 =c × 104(b) Express c in terms of a and b.c = ................................(2)(Total 4 marks)TOTAL FOR PAPER = 100 MARKSEND。
8.4-2006年剑桥O-level数学试卷P2
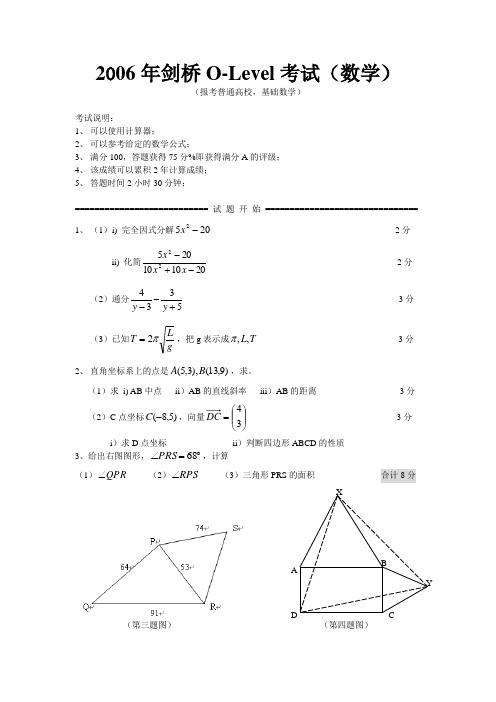
2006年剑桥O-Level 考试(数学)(报考普通高校,基础数学)考试说明:1、 可以使用计算器;2、 可以参考给定的数学公式;3、 满分100,答题获得75分%即获得满分A 的评级;4、 该成绩可以累积2年计算成绩;5、 答题时间2小时30分钟;=========================== 试 题 开 始 =============================== 1、 (1)i) 完全因式分解2052-x 2分ii) 化简20101020522-+-x x x 2分(2)通分5334+--y y 3分 (3)已知gLT π2=,把g 表示成T L ,,π 3分 2、 直角坐标系上的点是)9,13(),3,5(B A ,求。
(1)求 i) AB 中点 ii )AB 的直线斜率 iii )AB 的距离 3分(2)C 点坐标)5,8(-C ,向量⎪⎪⎭⎫⎝⎛=34 3分i )求D 点坐标 ii )判断四边形ABCD 的性质 3、给出右图图形,︒=∠68PRS ,计算(1)QPR ∠ (2)RPS ∠ (3)三角形PRS 的面积 合计8分(第三题图) (第四题图)4、如图所示矩形ABCD ,三角形ABX 和BCY 是等边三角形。
(1)求XBY ∠(2)证明三角形AXD 和BXY 是全等的 (3)证明︒=∠60DXY(4)证明三角形DXY 是等边三角形 8分 5、(1)某天英镑和美元的汇率为1英镑=1.65美元。
在同一天,英镑和欧元的汇率为1英镑=1.44欧元。
4分i) Alan 换500英镑到美元,可以换出多少美元?ii)Brenda 用900欧元换成英镑,可以换出多少英镑?iii)Clare 用792美元换欧元,问可以换出多少欧元? (2)制作电视机的成本是15000元i)出售给零售商,按照成本获益8%。
计算零售商的零售价? 1分 ii) 零售商出售电视机给商店,获益8%。
商店卖给个人john 也是按照盈利8%出售。
2007年IGCSEchemistry真题paper2

(a) (i) Complete the equation for the burning of hydrogen.
For Examiner's
Use
H2 + O2 →
(ii) State an adverse effect of lead on health.
For Examiner's
Use
[1]
[1]
(c) Structure A is sulphur. Explain why burning fossil fuels containing sulphur is harmful to the environment.
SS S
S
S
SS S
A
HH HCCH
HH B
H HCH
H C
N
H
H
H
D
Na+ Cl – Na+ Cl – Na+ Cl – Na+ Cl – Na+ Cl – Na+ Cl – Na+ Cl – Na+ Cl – Na+ Cl – Na+ Cl –
E
Pb Pb Pb Pb Pb Pb Pb Pb Pb
Answer all questions. A copy of the periodic table is printed on page 16.
At the end of the examination, fasten all your work securely together. The number of marks is given in brackets [ ] at the end of each question or part question.
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
4400/4HEdexcel IGCSEMathematicsPaper 4HHigher TierFriday 11 June 2010 – AfternoonTime: 2 hoursMaterials required for examination Items included with question papersRuler graduated in centimetres and Nilmillimetres, protractor, compasses,pen, HB pencil, eraser, calculator.Tracing paper may be used.Instructions to CandidatesIn the boxes above, write your centre number, candidate number, your surname, initials and signature.Check that you have the correct question paper.Answer ALL the questions. Write your answers in the spaces provided in this question paper. You must NOT write on the formulae page. Anything you write on the formulae page will gain NO credit.If you need more space to complete your answer to any question, use additional answer sheets. Information for CandidatesThe marks for individual questions and the parts of questions are shown in round brackets: e.g. (2). There are 22 questions in this question paper. The total mark for this paper is 100.You may use a calculator.Advice to CandidatesWrite your answers neatly and in good English.This publication may be reproduced only in accordance withEdexcel Limited copyright policy.©2010 Edexcel Limited.Printer’s Log. No. N36905AIGCSE MATHEMATICS 4400 FORMULA SHEET – HIGHER TIERAnswer ALL TWENTY TWO questions.Write your answers in the spaces provided.You must write down all stages in your working.1. Solve 6 y – 9 = 3 y + 7y = ................................(Total 3 marks) 2. The diagram shows two towns, A and B, on a map.(a) By measurement, find the bearing of B from A.....................................︒(2)C is another town.The bearing of C from A is 050︒.(b) Find the bearing of A from C.....................................︒(2)(Total 4 marks)3. A spinner can land on red or blue or yellow.The spinner is biased.The probability that it will land on red is 0.5The probability that it will land on blue is 0.2Imad spins the spinner once.(a) Work out the probability that it will land on yellow......................................(2)Janet spins the spinner 30 times.(b) Work out an estimate for the number of times the spinner will land on blue......................................(2)(Total 4 marks)4. Rosetta drives 85 kilometres in 1 hour 15 minutes.(a) Work out her average speed in kilometres per hour...................................... km/h(2)Rosetta drives a total distance of 136 kilometres.(b) Work out 85 as a percentage of 136................................. %(2)Sometimes Rosetta travels by train to save money.The cost of her journey by car is £12The cost of her journey by train is 15% less than the cost of her journey by car.(c)Work out the cost of Rose tta’s journey by train.£ ...................................(3)(Total 7 marks)5.Calculate the value of x.Give your answer correct to 3 significant figures.x = ................................(Total 3 marks)6. A = {2, 3, 4, 5}B = {4, 5, 6, 7}(a)(i) List the members of A ⋂B......................................(ii) How many members are in A ⋃B?.....................................(2)ℰ = {3, 4, 5, 6, 7}P = {3, 4, 5}Two other sets, Q and R, each contain exactly three members.P ⋂Q = {3, 4}P ⋂R = {3, 4}Set Q is not the same as set R.(b)(i) Write down the members of a possible set Q......................................(ii) Write down the members of a possible set R......................................(2)(Total 4 marks)7. Rectangular tiles have width (x + 1) cm and height (5x – 2) cm.Some of these tiles are used to form a large rectangle.The large rectangle is 7 tiles wide and 3 tiles high.The perimeter of the large rectangle is 68 cm.(a) Write down an equation in x...............................................................................................................(3)(b) Solve this equation to find the value of x.x = ................................(3)(Total 6 marks)8. Show that 121 141 = 1519.The depth of water in a reservoir increases from 14 m to 15.75 m. Work out the percentage increase.................................. %(Total 3 marks)10. Quadrilaterals ABCD and PQRS are similar.AB corresponds to PQ.BC corresponds to QR.CD corresponds to RS.Find the value of(a) xx = ...............................(2)(b) yy = ...............................(1)(Total 3 marks)11. Simplify fully6x + 43x.....................................(Total 3 marks)12.(a)Find the equation of the line L......................................(3)(b) Find the three inequalites that define the unshaded region shown in the diagram below................................................................................................................(3)(Total 6 marks)13. (a) Solve x 2– 8x + 12 = 0.....................................(3)(b) Solve the simultaneous equationsy = 2x4x – 5y = 9x = ................................y = ................................(3)(Total 6 marks)14.The area of the triangle is 6.75 cm2.The angle x°is acute.Find the value of x.Give your answer correct to 1 decimal place.x = ................................(Total 3 marks)15. The unfinished histogram shows information about the heights, h metres, of some trees.(a) Calculate an estimate for the number of trees with heights in the interval 4.5 < h ≤ 10.....................................(3)(b) There are 75 trees with heights in the interval 10 < h ≤ 13Use this information to complete the histogram.(2)(Total 5 marks)16. A bag contains 3 white discs and 1 black disc.John takes at random 2 discs from the bag without replacement.(a) Complete the probability tree diagram.First disc Second disc(3)(b)Find the probability that both discs are white......................................(2)All the discs are now replaced in the bag.Pradeep takes at random 3 discs from the bag without replacement.(c)Find the probability that the disc left in the bag is white......................................(3)(Total 8 marks)17. The diagram shows a sector of a circle, radius 45 cm, with angle 84°.Calculate the area of the sector.Give your answer correct to 3 significant figures.............................. cm2(Total 3 marks) 18.Calculate the length of AC.Give your answer correct to 3 significant figures................................ cm(Total 3 marks)19. A cone has slant height 4 cm and base radius r cm.The total surface area of the cone is 433π cm 2.Calculate the value of r .r = ................................(Total 4 marks)20. f(x) = (x – 1)2(a) Find f(8).....................................(1)The domain of f is all values of x where x ≥ 7(a)Find the range of f......................................(2)xg(x) =x1(c) Solve the equation g(x) = 1.2.....................................(2)(d) (i) Express the inverse function g –1 in the form g –1(x) = .......g –1(x) = ...................................(ii) Hence write down gg(x) in terms of x.gg(x) = ....................................(6)(Total 11 marks)21.In the diagram OA= a and OC= c.(a) Find CA in terms of a and c......................................(1)The point B is such that AB=1c.2(b) Give the mathematical name for the quadrilateral OABC......................................(1)The point P is such that OP= a + k c, where k ≥ 0(c) State the two conditions relating to a + k c that must be true for OAPC to be a rhombus.(2)(Total 4 marks)22. (a) Work out 5.2 × 102 + 2.3 × 104Give your answer in standard form......................................(2)a × 102 +b × 104 =c × 104(b) Express c in terms of a and b.c = ................................(2)(Total 4 marks)TOTAL FOR PAPER = 100 MARKSEND。
