自控第三章作业答案
《自动控制原理》---丁红主编---第三章习题答案
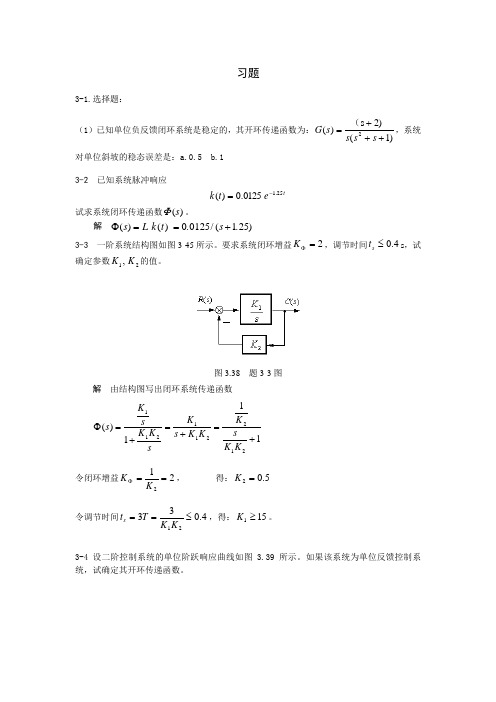
习题3-1.选择题:(1)已知单位负反馈闭环系统是稳定的,其开环传递函数为:)1(2)s )(2+++=s s s s G (,系统对单位斜坡的稳态误差是:a.0.5 b.1 3-2 已知系统脉冲响应t e t k 25.10125.0)(-=试求系统闭环传递函数)(s Φ。
解 Φ()()./(.)s L k t s ==+001251253-3 一阶系统结构图如图3-45所示。
要求系统闭环增益2=ΦK ,调节时间4.0≤s t s ,试确定参数21,K K 的值。
图3.38 题3-3图解 由结构图写出闭环系统传递函数111)(212211211+=+=+=ΦK K sK K K s K sK K s K s令闭环增益212==ΦK K , 得:5.02=K 令调节时间4.03321≤==K K T t s ,得:151≥K 。
3-4 设二阶控制系统的单位阶跃响应曲线如图 3.39 所示。
如果该系统为单位反馈控制系统,试确定其开环传递函数。
图3.39 题3-4图 解:由图2.8知,开环传递函数为3-5 设角速度指示随动统结构图如图3-40所示。
若要求系统单位阶跃响应无超调,且调节时间尽可能短,问开环增益K 应取何值,调节时间s t 是多少?图3-40 题3-5图解:依题意应取 1=ξ,这时可设闭环极点为02,11T -=λ。
写出系统闭环传递函数Ks s Ks 101010)(2++=Φ 闭环特征多项式20022021211010)(⎪⎪⎭⎫ ⎝⎛++=⎪⎪⎭⎫ ⎝⎛+=++=T s T s T s K s s s D 比较系数有 ⎪⎪⎩⎪⎪⎨⎧=⎪⎪⎭⎫ ⎝⎛=K T T 101102200 联立求解得 ⎩⎨⎧==5.22.00K T 因此有 159.075.40''<''==T t s3-6 图3.41所示为某控制系统结构图,是选择参数K 1和K 2,使系统的ωn =6,ξ=1.3-7 已知系统的特征方程,试判别系统的稳定性,并确定在右半s 平面根的个数及纯虚根。
自动控制原理第三章课后习题答案

3-1(1) )(2)(2.0t r t c= (2) )()()(24.0)(04.0t r t c t c t c=++ 试求系统闭环传递函数Φ(s),以及系统的单位脉冲响应g(t)和单位阶跃响应c(t)。
已知全部初始条件为零。
解:(1) 因为)(2)(2.0s R s sC =闭环传递函数ss R s C s 10)()()(==Φ 单位脉冲响应:s s C /10)(= 010)(≥=t t g单位阶跃响应c(t) 2/10)(s s C = 010)(≥=t t t c(2))()()124.004.0(2s R s C s s =++ 124.004.0)()(2++=s s s R s C 闭环传递函数124.004.01)()()(2++==s s s R s C s φ 单位脉冲响应:124.004.01)(2++=s s s C t e t g t 4sin 325)(3-= 单位阶跃响应h(t) 16)3(61]16)3[(25)(22+++-=++=s s s s s s Ct e t e t c t t 4sin 434cos 1)(33----=3-2 温度计的传递函数为11+Ts ,用其测量容器内的水温,1min 才能显示出该温度的98%的数值。
若加热容器使水温按10ºC/min 的速度匀速上升,问温度计的稳态指示误差有多大?解法一 依题意,温度计闭环传递函数11)(+=ΦTs s 由一阶系统阶跃响应特性可知:o o T c 98)4(=,因此有 min 14=T ,得出 min 25.0=T 。
视温度计为单位反馈系统,则开环传递函数为Ts s s s G 1)(1)()(=Φ-Φ= ⎩⎨⎧==11v T K用静态误差系数法,当t t r ⋅=10)( 时,C T Ke ss ︒===5.21010。
解法二 依题意,系统误差定义为 )()()(t c t r t e -=,应有 1111)()(1)()()(+=+-=-==ΦTs TsTs s R s C s R s E s e C T s Ts Ts ss R s s e s e s ss ︒==⋅+=Φ=→→5.210101lim )()(lim 23-3 已知二阶系统的单位阶跃响应为)1.536.1sin(5.1210)(2.1o tt et c +-=-试求系统的超调量σ%、峰值时间tp 和调节时间ts 。
自动控制原理第三章答案

n
临界阻尼:ts 4.75T 4.75
1
4.75
n
1 0.95s 5
3-3 原系统传递函数为 G(s) 0.2s 1 , 现采用如题所示的负反馈方式,欲将反 馈系统的调节时间减小为原来的0.1倍, 并且保证原放大倍数不变,试确定参数 K0 , KH的值。 解:原系统传递函数 新系统传递函数
K 10
0
1 10K 10 (时间常数为
H
1 ) 10
K 0.9
H
问题 非标准形式 10K 0 1 1 10K H , 0 .2 s 1 Ts 1 1 10K H
3
3-4
已知系统的单位阶跃响应为 试求取系统的传递函数
y(t ) 1 e
t
e
2t
Y(s) X(s)
n
2
问题 1、没有完成 2、计算错误
0.146
8
1 KK
1
2
3-9 设题3-9图(a)所示的单位 阶跃响应如题3-9图(b)所示。 试确定系统参数K1,K2和a。
解:据题意
K K (s) s(s a ) K K K K s as K s 2 s 1 s(s a )
(s) s(0.1s 1)
K 1 s(0.1s 1) K 10K 0.1s s K s 10s 10K
2 2
对应二阶系统标准形式,取ζ=1,得
问题
1、没有求调节时间 2、临界阻尼,调节时间 计算错误
2 10 5
n n
5 10K K 2.5 10
t
p
0.1
1.1 1.0 100% 10% 1.1 根据二阶欠阻尼系统指标计算公式
自控第三章作业答案

P3.4 The open-loop transfer function of a unity negative feedback system is)1(1)(+=s s s GDetermine the rise time, peak time, percent overshoot and setting time (using a 5% setting criterion).Solution: Writing he closed-loop transfer function 2222211)(nn ns s s s s ωςωωΦ++=++=we get 1=n ω, 5.0=ς. Since this is an underdamped second-order system with 5.0=ς, thesystem performance can be estimated as follows.Rising time.sec 42.25.0115.0arccos 1arccos 22≈-⋅-=--=πςωςπn r tPeak time.sec 62.35.011122≈-⋅=-=πςωπn p tPercent overshoot %3.16% 100% 100225.015.01≈⨯=⨯=--πςπςσee pSetting time.sec 615.033=⨯=≈ns t ςω(using a 5% setting criterion)P3.5 A second-order system gives a unit step response shown in Fig. P3.5. Find the open-loop transfer function if the system is a unit negative-feedback system.Solution: By inspection we have %30% 100113.1=⨯-=pσSolving the formula for calculating the overshoot,3.021==-ςπςσep, we have362.0ln ln 22≈+-=pp σπσςSince .sec 1=p t , solving the formula for calculating the peak time, 21ςωπ-=n p t , we gets e c / 7.33rad n =ωHence, the open-loop transfer function is )4.24(7.1135)2()(2+=+=s s s s s G n nςωωP3.6 A feedback system is shown in Fig. P3.6(a), and its unit step response curve is shown in Fig. P3.6(b). Determine the values of 1k , 2k , and a ..1.1Figure P3.5Solution: The transfer function between the input and output is given by2221)()(k as sk k s R s C ++=The system is stable and we have, from the response curve,21lim )(lim 122210==⋅++⋅=→∞→k sk as sk k s t c s tBy inspection we have %9% 10000.211.218.2=⨯-=pσSolving the formula for calculating the overshoot, 09.021==-ςπςσep, we have608.0ln ln 22≈+-=pp σπσςSince .sec 8.0=p t , solving the formula for calculating the peak time,21ςωπ-=n p t , we gets e c / 95.4rad n =ωThen, comparing the characteristic polynomial of the system with its standard form, we have22222n n s s k as s ωςω++=++5.2495.4222===n k ω02.695.4608.022=⨯⨯==n a ςωP3.8 For the servomechanism system shown in Fig. P3.8, determine the values of k and a that satisfy the following closed-loop system design requirements. (a) Maximum of 40% overshoot. (b) Peak time of 4s.Solution: For the closed-loop transfer function we have 22222)(nn ns sks k sk s ωςωωαΦ++=++=hence, by inspection, we getk n=2ω, αςωk n =2, and nnkωςςωα22==Taking consideration of %40% 10021=⨯=-ςπςσepresults in280.0=ς.In this case, to satisfy the requirement of peak time, 412=-=ςωπn p t , we have.s e c / 818.0r a d n =ω.2.2(a)(b)Figure P3.6Figure P3.8Hence, the values ofkandaare determined as67.02==n k ω, 68.02==nωςαP3.10 A control system is represented by the transfer function)13.04.0)(56.2(33.0)()(2+++=s ss s R s CEstimate the peak time, percent overshoot, and setting time (%5=∆), using the dominant polemethod, if it is possible.Solution: Rewriting the transfer function as]3.0)2.0)[(56.2(33.0)()(22+++=s s s R s Cwe get the poles of the system: 3.02.02 1j s ±-=,, 56.23-=s . Then, 2 1,s can be considered as a pair of dominant poles, because )Re()Re(32 1s s <<,.Method 1. After reducing to a second-order system, the transfer function becomes13.04.013.0)()(2++=s ss R s C (Note:1)()(lim==→s R s C k s Φ)which results in sec / 36.0rad n =ω and 55.0=ς. The specifications can be determined ass e c 0.42112ςωπ-=n p t , %6.12% 10021=⨯=-ςπςσeps e c 67.2011ln 12=⎪⎪⎪⎭⎫⎝⎛-=ς∆ςωns t Method 2. Taking consideration of the effect of non-dominant pole on the transient components cause by the dominant poles, we haves e c 0.8411)(231=--∠-=ςωπn p s s t%6.13% 10021313=⨯-=-ςπςσes s s ps e c 6.232ln 1313=⎪⎪⎭⎫⎝⎛-⋅=ss s t ns ∆ςωP3.13 The characteristic equations for certain systems are given below. In each case, determine the value of k so that the corresponding system is stable. It is assumed that k is positive number.(a) 02102234=++++k s s s s (b) 0504)5.0(23=++++ks s k sSolution: (a) 02102234=++++k s s s s .The system is stable if and only if⎪⎪⎩⎪⎪⎨⎧<⇒>=>9 022010102203k k D ki.e. the system is stable when 90<<k .(b) 0504)5.0(23=++++ks s k s . The system is stable if and only if⎪⎩⎪⎨⎧>-+⇒>-+⇒>+=>>+0)3.3)(8.34( 05024 041505.00 ,05.022k k k k k k D k ki.e. the system is stable when 3.3>k .P3.14 The open-loop transfer function of a negative feedback system is given by)12.001.0()(2++=s ss Ks G ςDetermine the range of K and ς in which the closed-loop system is stable. Solution: The characteristic equation is02.001.023=+++K s s s ς The system is stable if and only if⎪⎩⎪⎨⎧<⇒>-⇒>=>>ςςς20 001020 0101.02.002.0 ,02K K .ς.K D kThe required range is20>>K ς.P3.17 A unity negative feedback system has an open-loop transfer function )16)(13()(++=s s s K s GDetermine the range ofkrequired so that there are no closed-loop poles to the right of the line1-=s . Solution: The closed-loop characteristic equation is18)6)(3( 0)16)(13(=+++⇒=+++K s s s K s s si.e. 01818923=+++K s s sLetting 1~-=s s resulting in 0)1018(~3~6~ 018)5~)(2~)(1~(23=-+++⇒=+++-K s s s K s s sUsing Lienard-Chipart criterion, all closed-loop poles locate in the right-half s~-plane, i.e. to theright of the line 1-=s , if and only if⎪⎩⎪⎨⎧<⇒>-⇒>-=>⇒>-14 08.182 0311018695 ,010182K K K D K KThe required range is 91495 <<K , or56.10.56 <<KP3.18 A system has the characteristic equation0291023=+++k s s sDetermine the value of k so that the real part of complex roots is 2-, using the algebraic criterion.Solution: Substituting 2~-=s s into the characteristic equation yields 02~292~102~ 23=+-+-+-k s s s )()()( 0)26(~~4~ 23=-+++k s s sThe Routh array is established as shown.If there is a pair of complex roots with real part of 2-, then026=-ki.e. 30=k . In the case of 30=k , we have the solution of the auxiliary equation j s ±=~, i.e. j s ±-=2.3s 1 12s 4 26-k1s 0sP3.22 The open-loop transfer function of a unity negative feedback system is given by)1)(1()(21++=s T s T s Ks GDetermine the values of K , 1T , and 2T so that the steady-state error for the input, bt a t r +=)(, is less than 0ε. It is assumed that K , 1T , and 2T are positive, a and b are constants. Solution: The characteristic polynomial is K s s T T s T T s ++++=221321)()(∆Using L-C criterion, the system is stable if and only if2121212121212 0 01T T T T K T KT T T T T K T T D +<⇒>-+⇒>+=Considering that this is a 1-type system with a open-loop gain K , in the case of 2121T T T T K +<,we have 00.. εεεεεbK Kb v ss r ss ss>⇒<=+=Hence, the required range for K is21210T T T T K b+<<εP3.24 The block diagram of a control system is shown in Fig. P3.24, where )()()(s C s R s E -=. Select the values of τ and b so that the steady-state error for a ramp input is zero.Solution: Assuming that all parameters are positive, the system must be stable. Then, the error response is)()1)(1()(1)()()(21s R K s T s T b s K s C s R s E ⎥⎦⎤⎢⎣⎡++++-=-=τ)()1)(1()1()(2121221s R Ks T s T Kb s K T T sT T ⋅+++-+-++=τLetting the steady-state error for a ramp input to be zero, we get 221212210.)1)(1()1()(lim )(lim sv K s T s T Kb s K T T sT T s s sE s s r ss ⋅+++-+-++⋅==→→τεwhich results in ⎩⎨⎧=-+=-0121τK T T Kb I.e. KT T 21+=τ,Kb 1=.P3.26 The block diagram of a system is shown in Fig. P3.26. In each case, determine the steady-state error for a unit step disturbance and a unit ramp disturbance, respectively. (a) 11)(K s G =,)1()(222+=s T s K s GFigure P3.24Figure P3.26(b)ss T K s G )1()(111+=,)1()(222+=s T s K s G , 21T T >Solution: (a) In this case the system is of second-order and must be stable. The transfer function from disturbance to error is given by 212212.)1(1)(K K Ts s K G G G s d e ++-=+-=ΦThe corresponding steady-state errors are 1212.11)1(lim K s K K Ts s K s s p ss -=⋅++-⋅=→ε∞→⋅++-⋅=→2212.1)1(lim sK K Ts s K s s ass ε(b) Now, the transfer function from disturbance to error is given by )1()1()(121222.+++-=s T K K s T s sK s d e Φand the characteristic polynomial is21121232)(K K s T K K s s T s +++=∆ Using L-C criterion,0)(121211212212>-==T T K K T K K T K K Dthe system is stable. The corresponding steady-state errors are 01)1()1(lim 1212220.=⋅+++-⋅=→ss T K K s T s sK s s p ss ε121212220.11)1()1(lim K ss T K K s T s sK s s a ss -=⋅+++-⋅=→ε。
自动控制原理第三章课后习题 答案()

3-1 设系统的微分方程式如下:(1) )(2)(2.0t r t c= (2) )()()(24.0)(04.0t r t c t c t c=++ 试求系统闭环传递函数Φ(s),以及系统的单位脉冲响应g(t)和单位阶跃响应c(t)。
已知全部初始条件为零。
解:(1) 因为)(2)(2.0s R s sC =闭环传递函数ss R s C s 10)()()(==Φ 单位脉冲响应:s s C /10)(= 010)(≥=t t g单位阶跃响应c(t) 2/10)(s s C = 010)(≥=t t t c(2))()()124.004.0(2s R s C s s =++ 124.004.0)()(2++=s s s R s C `闭环传递函数124.004.01)()()(2++==s s s R s C s φ单位脉冲响应:124.004.01)(2++=s s s C t e t g t 4sin 325)(3-= 单位阶跃响应h(t) 16)3(61]16)3[(25)(22+++-=++=s s s s s s C t e t e t c t t 4sin 434cos 1)(33----=3-2 温度计的传递函数为11+Ts ,用其测量容器内的水温,1min 才能显示出该温度的98%的数值。
若加热容器使水温按10ºC/min 的速度匀速上升,问温度计的稳态指示误差有多大解法一 依题意,温度计闭环传递函数11)(+=ΦTs s 由一阶系统阶跃响应特性可知:o o T c 98)4(=,因此有 min 14=T ,得出 min 25.0=T 。
视温度计为单位反馈系统,则开环传递函数为Tss s s G 1)(1)()(=Φ-Φ=⎩⎨⎧==11v TK !用静态误差系数法,当t t r ⋅=10)( 时,C T Ke ss ︒===5.21010。
解法二 依题意,系统误差定义为 )()()(t c t r t e -=,应有 1111)()(1)()()(+=+-=-==ΦTs TsTs s R s C s R s E s e C T sTs Ts ss R s s e s e s ss ︒==⋅+=Φ=→→5.210101lim )()(lim 203-3 已知二阶系统的单位阶跃响应为)1.536.1sin(5.1210)(2.1o tt et c +-=-试求系统的超调量σ%、峰值时间tp 和调节时间ts 。
自动控制原理第3章习题解答

(2) k (t ) = 5t + 10 sin( 4t + 45 )
0
(3) k (t ) = 0.1(1 − e 解: (1) Φ ( s ) =
−t / 3
)
0.0125 s + 1.25
1
胡寿松自动控制原理习题解答第三章
(2) k (t ) = 5t + 10 sin 4t cos 45 + 10 cos 4t sin 45
3s 4 + 10s 3 + 5s 2 + s + 2 = 0
试用劳思稳定判据和赫尔维茨判据确定系统的稳定性。 解: 列劳思表如下:
s4 s3 s2 s1 s0
3 5 2 10 1 47 2 10 1530 0 − 47 2
由劳思表可以得到该系统不稳定。 3-12 已知系统特征方程如下,试求系统在 s 右半平面的根数及虚根值。 (1)
2ξω n = 70
ξ=
7 2 6
根据(3-17)
h(t ) = 1 +
e − t / T1 e − t / T12 + T2 / T1 − 1 T1 / T2 − 1
解:根据公式(3-17)
3
胡寿松自动控制原理习题解答第三章
自控第三章答案

K
p
不稳定
稳定
0
K
d
不稳定
不稳定
临界阻尼轨迹: D ( s ) s 4 K d s 4 K
2 p
0 出现重根时
p
临界阻尼条件为: 即: K
2 d
4 K
2
2 d
4 4K
0 线。
K p , 以纵轴为对称轴的抛物 K K
2 d 2 d
过阻尼区: 欠阻尼区: K
B3.15 分析图B3.15所示的两个系统,引入与不引入反馈时 系统的稳定性 。
解 不引入反馈 显然不稳定。 引入反馈 D ( s ) s ( s 1 )( s 5 ) 10 ( s 1 ) 0 闭环稳定。 (s ) 10 ( s 1 ) s ( s 1 )( s 5 )
3
赫尔维茨判据: 9 100 D2 20 1 100 9 80 0
1 20 4 100
2
1
0
故系统是稳定的。
(3)s4+4s3+13s2+36s+K=0
解
(1 ) 劳思判据: s s s s s
4
1 4 4 36 K K
13 36 K K
K 0
3
2
1
0
若系统稳定,则
36 K 0 0 K 36 K 0
( 2 )由
G (s )
7(s 1) s ( s 4 )( s 2 s 2 )
2
0 . 875 ( s 1 ) s ( 0 . 25 s 1 )( 0 . 5 s s 1 )
2
可知系统为
1
型的,于是
自控-第三章作业答案-超调量计算

作业3-11,3-12,3-15.。
参考答案(知识点:二阶振荡系统的动态特性指标计算)3-11已知系统结构如图所示,求:(1) 4K =,0,τ=时系统参数,?n ωζ=,性能指标%,?s t σ=(2) 如果要求0.707?0K ζτ===,,其中(3) 4K =,为改善性能加s τ使%5%σ<,求τ=?习题3-11系统结构图(1) K s s K s G s G s ++=+=Φ2)(1)()(=)0,4(,2222==++τωζωωK s s nn n 2==K n ω,25.021==n ωζ %47%100%21=⨯=--ζζπσe 36s n t s ζω==,⎪⎪⎩⎪⎪⎨⎧===⇒===⇒=5.021********n n n n K K ωωζωζω (2) K s s K s G s G s ++=+=Φ2)(1)()()707.0,0,(,2222==++=ςτωζωωK s s nn n 求 5.0)707.021(707.01222=⨯==⇒⎭⎬⎫==n n K ωζζω; (3) )41(4)1(41)1(4)(ττ++=+++=s s s s s s s s G k ,24144s s s τΦ=+++()() 457.0412707.024*******=-⨯⨯=-=⇒⎩⎨⎧=+=n n n ζωτωτζω 注意:教材树P73最佳阻尼比的定义:0707ς=.时,系统的最大超调量435πσ-=<.%%%=e ,1.3调节时间最短,即平稳性和快速性最佳。
本题的启示:(1)求得原系统的超调量47σ=%%非常大,(2)为了降低超调<5%,降低了开环增益K 。
(注意:求解稳态误差时,为了提高精度,可以增大开环增益。
当设计者进行系统参数设定时,需要兼顾动静态指标) (3)为了降低超调<5%,在前向通道环节引入了微分环节。
3-12已知系统的单位阶跃响应曲线如图所示,求系统的闭环传递函数。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
P3.4 The open-loop transfer function of a unity negative feedback system is)1(1)(+=s s s GDetermine the rise time, peak time, percent overshoot and setting time (using a 5% setting criterion).Solution: Writing he closed-loop transfer function 2222211)(nn ns s s s s ωςωωΦ++=++=we get 1=n ω, 5.0=ς. Since this is an underdamped second-order system with 5.0=ς, thesystem performance can be estimated as follows.Rising time.sec 42.25.0115.0arccos 1arccos 22≈-⋅-=--=πςωςπn r tPeak time.sec 62.35.011122≈-⋅=-=πςωπn p tPercent overshoot %3.16% 100% 100225.015.01≈⨯=⨯=--πςπςσee pSetting time.sec 615.033=⨯=≈ns t ςω(using a 5% setting criterion)P3.5 A second-order system gives a unit step response shown in Fig. P3.5. Find the open-loop transfer function if the system is a unit negative-feedback system.Solution: By inspection we have %30% 100113.1=⨯-=pσSolving the formula for calculating the overshoot,3.021==-ςπςσep, we have362.0ln ln 22≈+-=pp σπσςSince .sec 1=p t , solving the formula for calculating the peak time, 21ςωπ-=n p t , we gets e c / 7.33rad n =ωHence, the open-loop transfer function is )4.24(7.1135)2()(2+=+=s s s s s G n nςωωP3.6 A feedback system is shown in Fig. P3.6(a), and its unit step response curve is shown in Fig. P3.6(b). Determine the values of 1k , 2k , and a ..1.1Figure P3.5Solution: The transfer function between the input and output is given by2221)()(k as sk k s R s C ++=The system is stable and we have, from the response curve,21lim )(lim 122210==⋅++⋅=→∞→k sk as sk k s t c s tBy inspection we have %9% 10000.211.218.2=⨯-=pσSolving the formula for calculating the overshoot, 09.021==-ςπςσep, we have608.0ln ln 22≈+-=pp σπσςSince .sec 8.0=p t , solving the formula for calculating the peak time,21ςωπ-=n p t , we gets e c / 95.4rad n =ωThen, comparing the characteristic polynomial of the system with its standard form, we have22222n n s s k as s ωςω++=++5.2495.4222===n k ω02.695.4608.022=⨯⨯==n a ςωP3.8 For the servomechanism system shown in Fig. P3.8, determine the values of k and a that satisfy the following closed-loop system design requirements. (a) Maximum of 40% overshoot. (b) Peak time of 4s.Solution: For the closed-loop transfer function we have 22222)(nn ns sks k sk s ωςωωαΦ++=++=hence, by inspection, we getk n=2ω, αςωk n =2, and nnkωςςωα22==Taking consideration of %40% 10021=⨯=-ςπςσepresults in280.0=ς.In this case, to satisfy the requirement of peak time, 412=-=ςωπn p t , we have.s e c / 818.0r a d n =ω.2.2(a)(b)Figure P3.6Figure P3.8Hence, the values ofkandaare determined as67.02==n k ω, 68.02==nωςαP3.10 A control system is represented by the transfer function)13.04.0)(56.2(33.0)()(2+++=s ss s R s CEstimate the peak time, percent overshoot, and setting time (%5=∆), using the dominant polemethod, if it is possible.Solution: Rewriting the transfer function as]3.0)2.0)[(56.2(33.0)()(22+++=s s s R s Cwe get the poles of the system: 3.02.02 1j s ±-=,, 56.23-=s . Then, 2 1,s can be considered as a pair of dominant poles, because )Re()Re(32 1s s <<,.Method 1. After reducing to a second-order system, the transfer function becomes13.04.013.0)()(2++=s ss R s C (Note:1)()(lim==→s R s C k s Φ)which results in sec / 36.0rad n =ω and 55.0=ς. The specifications can be determined ass e c 0.42112ςωπ-=n p t , %6.12% 10021=⨯=-ςπςσeps e c 67.2011ln 12=⎪⎪⎪⎭⎫⎝⎛-=ς∆ςωns t Method 2. Taking consideration of the effect of non-dominant pole on the transient components cause by the dominant poles, we haves e c 0.8411)(231=--∠-=ςωπn p s s t%6.13% 10021313=⨯-=-ςπςσes s s ps e c 6.232ln 1313=⎪⎪⎭⎫⎝⎛-⋅=ss s t ns ∆ςωP3.13 The characteristic equations for certain systems are given below. In each case, determine the value of k so that the corresponding system is stable. It is assumed that k is positive number.(a) 02102234=++++k s s s s (b) 0504)5.0(23=++++ks s k sSolution: (a) 02102234=++++k s s s s .The system is stable if and only if⎪⎪⎩⎪⎪⎨⎧<⇒>=>9 022010102203k k D ki.e. the system is stable when 90<<k .(b) 0504)5.0(23=++++ks s k s . The system is stable if and only if⎪⎩⎪⎨⎧>-+⇒>-+⇒>+=>>+0)3.3)(8.34( 05024 041505.00 ,05.022k k k k k k D k ki.e. the system is stable when 3.3>k .P3.14 The open-loop transfer function of a negative feedback system is given by)12.001.0()(2++=s ss Ks G ςDetermine the range of K and ς in which the closed-loop system is stable. Solution: The characteristic equation is02.001.023=+++K s s s ς The system is stable if and only if⎪⎩⎪⎨⎧<⇒>-⇒>=>>ςςς20 001020 0101.02.002.0 ,02K K .ς.K D kThe required range is20>>K ς.P3.17 A unity negative feedback system has an open-loop transfer function )16)(13()(++=s s s K s GDetermine the range ofkrequired so that there are no closed-loop poles to the right of the line1-=s . Solution: The closed-loop characteristic equation is18)6)(3( 0)16)(13(=+++⇒=+++K s s s K s s si.e. 01818923=+++K s s sLetting 1~-=s s resulting in 0)1018(~3~6~ 018)5~)(2~)(1~(23=-+++⇒=+++-K s s s K s s sUsing Lienard-Chipart criterion, all closed-loop poles locate in the right-half s~-plane, i.e. to theright of the line 1-=s , if and only if⎪⎩⎪⎨⎧<⇒>-⇒>-=>⇒>-14 08.182 0311018695 ,010182K K K D K KThe required range is 91495 <<K , or56.10.56 <<KP3.18 A system has the characteristic equation0291023=+++k s s sDetermine the value of k so that the real part of complex roots is 2-, using the algebraic criterion.Solution: Substituting 2~-=s s into the characteristic equation yields 02~292~102~ 23=+-+-+-k s s s )()()( 0)26(~~4~ 23=-+++k s s sThe Routh array is established as shown.If there is a pair of complex roots with real part of 2-, then026=-ki.e. 30=k . In the case of 30=k , we have the solution of the auxiliary equation j s ±=~, i.e. j s ±-=2.3s 1 12s 4 26-k1s 0sP3.22 The open-loop transfer function of a unity negative feedback system is given by)1)(1()(21++=s T s T s Ks GDetermine the values of K , 1T , and 2T so that the steady-state error for the input, bt a t r +=)(, is less than 0ε. It is assumed that K , 1T , and 2T are positive, a and b are constants. Solution: The characteristic polynomial is K s s T T s T T s ++++=221321)()(∆Using L-C criterion, the system is stable if and only if2121212121212 0 01T T T T K T KT T T T T K T T D +<⇒>-+⇒>+=Considering that this is a 1-type system with a open-loop gain K , in the case of 2121T T T T K +<,we have 00.. εεεεεbK Kb v ss r ss ss>⇒<=+=Hence, the required range for K is21210T T T T K b+<<εP3.24 The block diagram of a control system is shown in Fig. P3.24, where )()()(s C s R s E -=. Select the values of τ and b so that the steady-state error for a ramp input is zero.Solution: Assuming that all parameters are positive, the system must be stable. Then, the error response is)()1)(1()(1)()()(21s R K s T s T b s K s C s R s E ⎥⎦⎤⎢⎣⎡++++-=-=τ)()1)(1()1()(2121221s R Ks T s T Kb s K T T sT T ⋅+++-+-++=τLetting the steady-state error for a ramp input to be zero, we get 221212210.)1)(1()1()(lim )(lim sv K s T s T Kb s K T T sT T s s sE s s r ss ⋅+++-+-++⋅==→→τεwhich results in ⎩⎨⎧=-+=-0121τK T T Kb I.e. KT T 21+=τ,Kb 1=.P3.26 The block diagram of a system is shown in Fig. P3.26. In each case, determine the steady-state error for a unit step disturbance and a unit ramp disturbance, respectively. (a) 11)(K s G =,)1()(222+=s T s K s GFigure P3.24Figure P3.26(b)ss T K s G )1()(111+=,)1()(222+=s T s K s G , 21T T >Solution: (a) In this case the system is of second-order and must be stable. The transfer function from disturbance to error is given by 212212.)1(1)(K K Ts s K G G G s d e ++-=+-=ΦThe corresponding steady-state errors are 1212.11)1(lim K s K K Ts s K s s p ss -=⋅++-⋅=→ε∞→⋅++-⋅=→2212.1)1(lim sK K Ts s K s s ass ε(b) Now, the transfer function from disturbance to error is given by )1()1()(121222.+++-=s T K K s T s sK s d e Φand the characteristic polynomial is21121232)(K K s T K K s s T s +++=∆ Using L-C criterion,0)(121211212212>-==T T K K T K K T K K Dthe system is stable. The corresponding steady-state errors are 01)1()1(lim 1212220.=⋅+++-⋅=→ss T K K s T s sK s s p ss ε121212220.11)1()1(lim K ss T K K s T s sK s s a ss -=⋅+++-⋅=→ε。
