IGCSE数学试卷pastpaper
a level数学备考经验分享容易拿a的数学,应该怎么备考 testdaily厚朴优学

a level数学备考经验分享容易拿a的数学,应该怎么备考testdaily厚朴优学a level数学介绍ciea level数学(9709)分为4张paper: p1、s1、m1、p3。
其中m1是力学(mechanics),s1是统计学(statistics),而p1和p3就是纯数(pure math)。
p1通常在a level的第一年学习,p3是在第二年学习。
p1包括一元二次方程、二次函数、三角函数、平面几何、圆的计算、数列、微分、积分这几大知识点,考试是考10道或者11道题,1小时45分钟做完。
p1考纲p3包括代数多项式、指数函数、三角函数、微分、积分、解高次方程、微分方程、向量、复数等,考试也是1小时45分钟10道题。
p3考纲p1作为igcse数学到a level数学的一个过渡,难度并不高,其中的一些知识点比如说基础的三角函数、二次函数及方程等都是体制内初中就已经学过的东西。
而微积分这些听起来“高大上”,其实很基础很容易“上手”。
但是对于p1千万不能掉以轻心,因为p3算是p1的进阶版,在p1学过的知识点上进行了深入扩展和拔高,比如说p1学的三角函数还是sin/cos/tan,p3就加入了cosec/cot/sec,如果基础没打好,p3是很难学懂的。
a level数学,务实基础才是关键。
a level数学备考工具“工欲善其事,必先利其器”,要想在a level纯数部分“顺利摘星”,首先要像做一桌满汉全席那样备好“食材”。
1. 计算器a level数学大考允许使用计算器,不仅可以用于加减乘除运算,还需要用计算器解方程、做微积分和三角函数。
大多数同学用的都是这款卡西欧fx-991科学计算器。
能画图的计算器是不允许的。
2. cie官方公式表大考时,剑桥官方会提供公式表,里面含盖了a level数学和高数所有的重要公式,但是要注意的是,不是每一个你需要的公式都会包括,所以不要过度依赖公式表,该好好背还是要背的。
2011 June igcse 英文数学试卷

This document consists of 19 printed pages and 1 blank page.IB11 06_0580_43/4RP© UCLES 2011[Turn over*8044643715*UNIVERSITY OF CAMBRIDGE INTERNATIONAL EXAMINATIONS International General Certificate of Secondary EducationMATHEMATICS 0580/43Paper 4 (Extended) May/June 20112 hours 30 minutesCandidates answer on the Question Paper.Additional Materials: Electronic calculatorGeometrical instrumentsMathematical tables (optional)Tracing paper (optional)READ THESE INSTRUCTIONS FIRSTWrite your Centre number, candidate number and name on all the work you hand in. Write in dark blue or black pen.You may use a pencil for any diagrams or graphs.Do not use staples, paper clips, highlighters, glue or correction fluid. DO NOT WRITE IN ANY BARCODES.Answer all questions.If working is needed for any question it must be shown below that question. Electronic calculators should be used.If the degree of accuracy is not specified in the question, and if the answer is not exact, give the answer to three significant figures. Give answers in degrees to one decimal place. For π use either your calculator value or 3.142.At the end of the examination, fasten all your work securely together.The number of marks is given in brackets [ ] at the end of each question or part question. The total of the marks for this paper is 130.© UCLES 20110580/43/M/J/11For Examiner's Use1 Lucy works in a clothes shop.(a) In one week she earned $277.20.(i) She spent 81of this on food.Calculate how much she spent on food. Answer(a)(i) $ [1](ii) She paid 15% of the $277.20 in taxes. Calculate how much she paid in taxes. Answer(a)(ii) $ [2](iii) The $277.20 was 5% more than Lucy earned in the previous week. Calculate how much Lucy earned in the previous week. Answer(a)(iii) $ [3](b) The shop sells clothes for men, women and children.(i) In one day Lucy sold clothes with a total value of $2200 in the ratio men : women : children = 2 : 5 : 4. Calculate the value of the women’s clothes she sold. Answer(b)(i) $ [2](ii) The $2200 was 7344of the total value of the clothes sold in the shop on this day. Calculate the total value of the clothes sold in the shop on this day. Answer(b)(ii) $ [2]© UCLES 2011 0580/43/M/J/11[Turn overUsex(a) (i) Draw the reflection of shape X in the x -axis. Label the image Y . [2](ii) Draw the rotation of shape Y , 90° clockwise about (0, 0). Label the image Z . [2](iii) Describe fully the single transformation that maps shape Z onto shape X .Answer(a)(iii)[2](b) (i) Draw the enlargement of shape X , centre (0, 0), scale factor21. [2](ii) Find the matrix which represents an enlargement, centre (0, 0), scale factor 21.Answer(b)(ii)[2](c) (i) Draw the shear of shape X with the x -axis invariant and shear factor –1.[2](ii) Find the matrix which represents a shear with the x -axis invariant and shear factor –1.Answer(c)(ii)[2]© UCLES 20110580/43/M/J/11Use(x + 5) cm2x cmx cmNOT TO SCALEThe diagram shows a square of side (x + 5) cm and a rectangle which measures 2x cm by x cm. The area of the square is 1 cm 2 more than the area of the rectangle.(a) Show that x 2 – 10x – 24 = 0 . Answer(a) [3]© UCLES 2011 0580/43/M/J/11[Turn overFor Examiner's Use(b) Find the value of x . Answer(b) x = [3](c) Calculate the acute angle between the diagonals of the rectangle. Answer(c) [3]© UCLES 2011 0580/43/M/J/11For Examiner's Use4NOT TO SCALEThe circle, centre O , passes through the points A , B and C . In the triangle ABC , AB = 8 cm, BC = 9 cm and CA = 6 cm. (a) Calculate angle BAC and show that it rounds to 78.6°, correct to 1 decimal place. Answer(a) [4](b) M is the midpoint of BC .(i) Find angle BOM . Answer(b)(i) Angle BOM = [1]© UCLES 2011 0580/43/M/J/11[Turn overFor Examiner's Use(ii) Calculate the radius of the circle and show that it rounds to 4.59 cm, correct to 3 significantfigures.Answer(b)(ii) [3](c) Calculate the area of the triangle ABC as a percentage of the area of the circle. Answer(c) % [4]© UCLES 2011 0580/43/M/J/11ForExaminer's Use5 (a) Complete the table of values for the function f(x ), where f(x ) = x 2 + 21x , x ≠ 0 .xO 3 O 2.5 O 2 O 1.5 O 1 O 0.50.5 1 1.5 2 2.5 3 f(x ) 6.41 2.69 4.25 4.252.69 6.41[3](b) On the grid, draw the graph of y = f(x ) for O 3 Y x Y O 0.5 and 0.5 Y x Y 3 .[5]© UCLES 2011 0580/43/M/J/11[Turn overFor Examiner's Use(c) (i) Write down the equation of the line of symmetry of the graph.Answer(c)(i)[1](ii) Draw the tangent to the graph of y = f(x ) where x = O 1.5. Use the tangent to estimate the gradient of the graph of y = f(x ) where x = O 1.5. Answer(c)(ii) [3](iii) Use your graph to solve the equation x 2 + 21x= 3.Answer(c)(iii) x = or x = or x = or x = [2](iv) Draw a suitable line on the grid and use your graphs to solve the equation x 2 + 21x = 2x .Answer(c)(iv) x =or x =[3]© UCLES 2011 0580/43/M/J/11For Examiner's Use6CumulativefrequencyMass (kilograms)mThe masses of 200 parcels are recorded. The results are shown in the cumulative frequency diagram above.(a) Find(i) the median, Answer(a)(i) kg [1](ii) the lower quartile, Answer(a)(ii) kg [1](iii) the inter-quartile range, Answer(a)(iii) kg [1](iv) the number of parcels with a mass greater than 3.5 kg. Answer(a)(iv) [2]© UCLES 2011 0580/43/M/J/11[Turn overFor Examiner's Use(b) (i) Use the information from the cumulative frequency diagram to complete the groupedfrequency table.Mass (m ) kg0 I m Y 44 I m Y 66 I m Y 77 I m Y 10Frequency 36 50[2](ii) Use the grouped frequency table to calculate an estimate of the mean. Answer(b)(ii) kg [4](iii) Complete the frequency density table and use it to complete the histogram.Mass (m ) kg 0 I m Y 4 4 I m Y 6 6 I m Y 7 7 I m Y 10Frequency density916.7FrequencydensityMass (kilograms)m[4]© UCLES 20110580/43/M/J/11ForExaminer's Use7 Katrina puts some plants in her garden.The probability that a plant will produce a flower is107. If there is a flower, it can only be red, yellow or orange.When there is a flower, the probability it is red is 32 and the probability it is yellow is 41.(a) Draw a tree diagram to show all this information. Label the diagram and write the probabilities on each branch. Answer(a) [5](b) A plant is chosen at random. Find the probability that it will not produce a yellow flower. Answer(b) [3](c) If Katrina puts 120 plants in her garden, how many orange flowers would she expect? Answer(c) [2]© UCLES 2011 0580/43/M/J/11[Turn overFor Examiner's Use8A(a) Draw accurately the locus of points, inside the quadrilateral ABCD , which are 6 cm from thepoint D . [1](b) Using a straight edge and compasses only, construct(i) the perpendicular bisector of AB , [2](ii) the locus of points, inside the quadrilateral, which are equidistant from AB and from BC . [2](c) The point Q is equidistant from A and from B and equidistant from AB and from BC .(i) Label the point Q on the diagram. [1](ii) Measure the distance of Q from the line AB . Answer(c)(ii) cm [1](d) On the diagram, shade the region inside the quadrilateral which is• less than 6 cm from Dand• nearer to A than to Band• nearer to AB than to BC . [1]© UCLES 2011 0580/43/M/J/11For Examiner's Use9 f(x ) = 3x + 1 g(x ) = (x + 2)2(a) Find the values of(i) gf(2), Answer(a)(i)[2](ii) ff(0.5). Answer(a)(ii)[2](b) Find f –1(x ), the inverse of f(x ). Answer(b)[2](c) Find fg(x ). Give your answer in its simplest form. Answer(c)[2]© UCLES 2011 0580/43/M/J/11[Turn overFor Examiner's Use(d) Solve the equation x 2 + f(x ) = 0. Show all your working and give your answers correct to 2 decimal places. Answer(d) x = or x =[4]UseBABCD is a parallelogram.DC, M is the midpoint of BC and N is the midpoint of LM.pq.(i)Find the following in terms ofp and q, in their simplest form.(a)Answer(a)[1](b)Answer(a)[2](c)Answer(a)[2] (ii) N lies on the line AC.Answer(a)(ii) [1]© UCLES 2011 0580/43/M/J/11© UCLES 2011 0580/43/M/J/11[Turn overUseEH J2x°75°(x + 15)°NOT TO SCALEEFG is a triangle. HJ is parallel to FG . Angle FEG = 75°. Angle EFG = 2x ° and angle FGE = (x + 15)°.(i) Find the value of x . Answer(b)(i) x = [2](ii) Find angle HJG . Answer(b)(ii) Angle HJG = [1]© UCLES 2011 0580/43/M/J/11For Examiner's Use11 (a) (i) The first three positive integers 1, 2 and 3 have a sum of 6. Write down the sum of the first 4 positive integers. Answer(a)(i) [1](ii) The formula for the sum of the first n integers is21)(+n n . Show the formula is correct when n = 3. Answer(a)(ii) [1](iii) Find the sum of the first 120 positive integers. Answer(a)(iii) [1](iv) Find the sum of the integers121 + 122 + 123 + 124 + …………………………… + 199 + 200.Answer(a)(iv)[2](v) Find the sum of the even numbers 2 + 4 + 6 + …………………………+ 800.Answer(a)(v)[2]© UCLES 20110580/43/M/J/11For Examiner's Use(b) (i) Complete the following statements about the sums of cubes and the sums of integers.13 = 1 1 = 113 + 23 = 9 1 + 2 = 3 13 + 23 + 33 =1 +2 +3 =13 + 23 + 33 + 43 =1 +2 +3 +4 =[2](ii) The sum of the first 14 integers is 105. Find the sum of the first 14 cubes. Answer(b)(ii) [1](iii) Use the formula in part(a)(ii) to write down a formula for the sum of the first n cubes. Answer(b)(iii) [1](iv) Find the sum of the first 60 cubes. Answer(b)(iv) [1](v) Find n when the sum of the first n cubes is 278 784. Answer(b)(v) n = [2]BLANK PAGEPer mission to r epr oduce items wher e thir d-par ty owned mater ial pr otected by copyr ight is included has been sought and clear ed wher e possible. Ever y reasonable effort has been made by the publisher (UCLES) to trace copyright holders, but if any items requiring clearance have unwittingly been included, the publisher will be pleased to make amends at the earliest possible opportunity.University of Cambridge International Examinations is part of the Cambridge Assessment Group. Cambridge Assessment is the brand name of University of Cambridge Local Examinations Syndicate (UCLES), which is itself a department of the University of Cambridge.© UCLES 2011 0580/43/M/J/11。
0606_m19_qp_12(IGCSE 数学考试2019年3月真题

Identities Formulae for ∆ABC
2. TRIGONOMETRY
sin2 A + cos2 A = 1 sec2 A = 1 + tan2 A cosec2 A = 1 + cot2 A
a sin A
=
b sin B
=
c sin C
a2 = b2 + c2 – 2bc cos A
At the end of the examination, fasten all your work securely together. The number of marks is given in brackets [ ] at the end of each question or part question. The total number of marks for this paper is 80.
∆
=
1 2
bc
sin
A
© UCLES 2019
0606/12/F/M/19
3
1 (a) Given that
பைடு நூலகம்
= { x : 1 1 x 1 20},
A = {multiples of 3},
B = {multiples of 4},
find
(i)
n (A),
[1]
(ii)
n (A + B) .
[1]
*6715046212*
Cambridge Assessment International Education Cambridge International General Certificate of Secondary Education
Igcse-数学-历年真题

4400/4HEdexcel IGCSEMathematicsPaper 4HHigher TierFriday 11 June 2010 – AfternoonTime: 2 hoursMaterials required for examination Items included with question papersRuler graduated in centimetres and Nilmillimetres, protractor, compasses,pen, HB pencil, eraser, calculator.Tracing paper may be used.Instructions to CandidatesIn the boxes above, write your centre number, candidate number, your surname, initials and signature.Check that you have the correct question paper.Answer ALL the questions. Write your answers in the spaces provided in this question paper.You must NOT write on the formulae page. Anything you write on the formulae page will gain NO credit.If you need more space to complete your answer to any question, use additional answer sheets.Information for CandidatesThe marks for individual questions and the parts of questions are shown in round brackets: e.g. (2).There are 22 questions in this question paper. The total mark for this paperis 100.You may use a calculator.Advice to CandidatesWrite your answers neatly and in good English.N36905AIGCSE MATHEMATICS 4400 FORMULA SHEET – HIGHER TIERAnswer ALL TWENTY TWO questions.Write your answers in the spaces provided.You must write down all stages in your working.1. Solve 6 y – 9 = 3 y + 7y = ................................(Total 3 marks) 2. The diagram shows two towns, A and B, on a map.(a) By measurement, find the bearing of B from A.....................................(2)C is another town.The bearing of C from A is 050.(b) Find the bearing of A from C.....................................(2)(Total 4 marks)3. A spinner can land on red or blue or yellow.The spinner is biased.The probability that it will land on red is 0.5The probability that it will land on blue is 0.2Imad spins the spinner once.(a) Work out the probability that it will land on yellow......................................(2)Janet spins the spinner 30 times.(b)Work out an estimate for the number of times the spinner will land on blue......................................(2)(Total 4 marks)4. Rosetta drives 85 kilometres in 1 hour 15 minutes.(a) Work out her average speed in kilometres per hour...................................... km/h(2)Rosetta drives a total distance of 136 kilometres.(b) Work out 85 as a percentage of 136................................. %(2)Sometimes Rosetta travels by train to save money.The cost of her journe y by car is £12The cost of her journey by train is 15% less than the cost of her journey by car.(c)Work out the cost of Rosetta’s journey by train.£ ...................................(3)(Total 7 marks)5.Calculate the value of x.Give your answer correct to 3 significant figures.x = ................................(Total 3 marks)6. A = {2, 3, 4, 5}B = {4, 5, 6, 7}(a)(i) List the members of A B......................................(ii) How many members are in A B?.....................................(2)ℰ = {3, 4, 5, 6, 7}P = {3, 4, 5}Two other sets, Q and R, each contain exactly three members.P Q = {3, 4}P R = {3, 4}Set Q is not the same as set R.(b)(i) Write down the members of a possible set Q......................................(ii) Write down the members of a possible set R......................................(2)(Total 4 marks)7. Rectangular tiles have width (x + 1) cm and height (5x – 2) cm.Some of these tiles are used to form a large rectangle.The large rectangle is 7 tiles wide and 3 tiles high.The perimeter of the large rectangle is 68 cm.(a) Write down an equation in x...............................................................................................................(3)(b) Solve this equation to find the value of x.x = ................................(3)(Total 6 marks)8. Show that 121 141 = 1519. The depth of water in a reservoir increases from 14 m to 15.75 m.Work out the percentage increase.................................. %(Total 3 marks)10. Quadrilaterals ABCD and PQRS are similar.AB corresponds to PQ.BC corresponds to QR.CD corresponds to RS.Find the value of(a) xx = ...............................(2)(b) yy = ...............................(1)(Total 3 marks)11. Simplify fully6x + 43x.....................................(Total 3 marks)12.(a)Find the equation of the line L......................................(3)(b) Find the three inequalites that define the unshaded region shown in the diagram below................................................................................................................(3)(Total 6 marks)13. (a) Solve x 2– 8x + 12 = 0.....................................(3)(b) Solve the simultaneous equationsy = 2x4x – 5y = 9x = ................................y = ................................(3)(Total 6 marks)14.The area of the triangle is 6.75 cm2.The angle x° is acute.Find the value of x.Give your answer correct to 1 decimal place.x = ................................(Total 3 marks)15. The unfinished histogram shows information about the heights, h metres, ofsome trees.(a) Calculate an estimate for the number of trees with heights in theinterval 4.5 < h ≤ 10.....................................(3)(b) There are 75 trees with heights in the interval 10 < h ≤ 13Use this information to complete the histogram.(2)(Total 5 marks)16. A bag contains 3 white discs and 1 black disc.John takes at random 2 discs from the bag without replacement.(a) Complete the probability tree diagram.First disc Second disc(3)(b)Find the probability that both discs are white......................................(2)All the discs are now replaced in the bag.Pradeep takes at random 3 discs from the bag without replacement.(c)Find the probability that the disc left in the bag is white......................................(3)(Total 8 marks)17. The diagram shows a sector of a circle, radius 45 cm, with angle 84°.Calculate the area of the sector.Give your answer correct to 3 significant figures.............................. cm2(Total 3 marks) 18.Calculate the length of AC.Give your answer correct to 3 significant figures................................ cm(Total 3 marks)19. A cone has slant height 4 cm and base radius r cm.The total surface area of the cone is 433π cm 2.Calculate the value of r .r = ................................(Total 4 marks)20. f(x) = (x – 1)2(a) Find f(8).....................................(1)The domain of f is all values of x where x ≥ 7(a)Find the range of f......................................(2)xg(x) =x1(c) Solve the equation g(x) = 1.2.....................................(2)(d) (i) Express the inverse function g –1 in the form g –1(x) = .......g –1(x) = ...................................(ii) Hence write down gg(x) in terms of x.gg(x) = ....................................(6)(Total 11 marks)21.In the diagram OA= a and OC= c.(a) Find CA in terms of a and c......................................(1)The point B is such that AB=1c.2(b) Give the mathematical name for the quadrilateral OABC......................................(1)The point P is such that OP= a + k c, where k ≥ 0(c) State the two conditions relating to a + k c that must be true for OAPCto be a rhombus.(2)(Total 4 marks)22. (a) Work out 5.2 × 102+ 2.3 × 104Give your answer in standard form......................................(2)a × 102 +b × 104 =c × 104(b) Express c in terms of a and b.c = ................................(2)(Total 4 marks)TOTAL FOR PAPER = 100 MARKSEND。
Igcse 数学 历年真题

4400/4HEdexcel IGCSEMathematicsPaper 4HHigher TierFriday 11 June 2010 – AfternoonTime: 2 hoursMaterials required for examination Items included with question papersRuler graduated in centimetres and Nilmillimetres, protractor, compasses,pen, HB pencil, eraser, calculator.Tracing paper may be used.Instructions to CandidatesIn the boxes above, write your centre number, candidate number, your surname, initials and signature.Check that you have the correct question paper.Answer ALL the questions. Write your answers in the spaces provided in this question paper.You must NOT write on the formulae page. Anything you write on the formulae page will gain NO credit.If you need more space to complete your answer to any question, use additional answer sheets.Information for CandidatesThe marks for individual questions and the parts of questions are shown in round brackets: . (2).There are 22 questions in this question paper. The total mark for this paperis 100.You may use a calculator.Advice to CandidatesWrite your answers neatly and in good English.This publication may be reproduced only in accordance with Edexcel Limited copyright policy.©2010 Edexcel Limited.Printer’s Log. No. N36905AIGCSE MATHEMATICS 4400 FORMULA SHEET – HIGHER TIERAnswer ALL TWENTY TWO questions.Write your answers in the spaces provided.You must write down all stages in your working.1. Solve 6 y – 9 = 3 y + 7y = ................................(Total 3 marks) 2. The diagram shows two towns, A and B, on a map.(a) By measurement, find the bearing of B from A.....................................(2)C is another town.The bearing of C from A is 050.(b) Find the bearing of A from C.....................................(2)(Total 4 marks)3. A spinner can land on red or blue or yellow.The spinner is biased.The probability that it will land on red isThe probability that it will land on blue isImad spins the spinner once.(a) Work out the probability that it will land on yellow......................................(2)Janet spins the spinner 30 times.(b)Work out an estimate for the number of times the spinner will land on blue......................................(2)(Total 4 marks)4. Rosetta drives 85 kilometres in 1 hour 15 minutes.(a) Work out her average speed in kilometres per hour...................................... km/h(2)Rosetta drives a total distance of 136 kilometres.(b) Work out 85 as a percentage of 136................................. %(2)Sometimes Rosetta travels by train to save money.The cost of her journey by car is £12The cost of her journey by train is 15% less than the cost of her journey by car.(c)Work out the cost of Rosetta’s journ ey by train.£ ...................................(3)(Total 7 marks)5.Calculate the value of x.Give your answer correct to 3 significant figures.x = ................................(Total 3 marks)6. A = {2, 3, 4, 5}B = {4, 5, 6, 7}(a)(i) List the members of A B......................................(ii) How many members are in A B?.....................................(2)ℰ = {3, 4, 5, 6, 7}P = {3, 4, 5}Two other sets, Q and R, each contain exactly three members.P Q = {3, 4}P R = {3, 4}Set Q is not the same as set R.(b)(i) Write down the members of a possible set Q......................................(ii) Write down the members of a possible set R......................................(2)(Total 4 marks)7. Rectangular tiles have width (x + 1) cm and height (5x – 2) cm.Some of these tiles are used to form a large rectangle.The large rectangle is 7 tiles wide and 3 tiles high.The perimeter of the large rectangle is 68 cm.(a) Write down an equation in x...............................................................................................................(3)(b) Solve this equation to find the value of x.x = ................................(3)(Total 6 marks)8. Show that 121 141 = 1519. The depth of water in a reservoir increases from 14 m to m.Work out the percentage increase.................................. %(Total 3 marks)10. Quadrilaterals ABCD and PQRS are similar.AB corresponds to PQ.BC corresponds to QR.CD corresponds to RS.Find the value of(a) xx = ...............................(2)(b) yy = ...............................(1)(Total 3 marks)11. Simplify fully6x + 43x.....................................(Total 3 marks)12.(a)Find the equation of the line L......................................(3)(b) Find the three inequalites that define the unshaded region shown in the diagram below................................................................................................................(3)(Total 6 marks)13. (a) Solve x 2– 8x + 12 = 0.....................................(3)(b) Solve the simultaneous equationsy = 2x4x – 5y = 9x = ................................y = ................................(3)(Total 6 marks)14.The area of the triangle is cm2.The angle x° is acute.Find the value of x.Give your answer correct to 1 decimal place.x = ................................(Total 3 marks)15. The unfinished histogram shows information about the heights, h metres, ofsome trees.(a) Calculate an estimate for the number of trees with heights in theinterval < h ≤ 10.....................................(3)(b) There are 75 trees with heights in the interval 10 < h ≤ 13Use this information to complete the histogram.(2)(Total 5 marks)16. A bag contains 3 white discs and 1 black disc.John takes at random 2 discs from the bag without replacement.(a) Complete the probability tree diagram.First disc Second disc(3)(b)Find the probability that both discs are white......................................(2)All the discs are now replaced in the bag.Pradeep takes at random 3 discs from the bag without replacement.(c)Find the probability that the disc left in the bag is white......................................(3)(Total 8 marks)17. The diagram shows a sector of a circle, radius 45 cm, with angle 84°.Calculate the area of the sector.Give your answer correct to 3 significant figures.............................. cm2(Total 3 marks) 18.Calculate the length of AC.Give your answer correct to 3 significant figures................................ cm(Total 3 marks)19. A cone has slant height 4 cm and base radius r cm.The total surface area of the cone is 433π cm 2.Calculate the value of r .r = ................................(Total 4 marks)20. f(x) = (x – 1)2(a) Find f(8).....................................(1)The domain of f is all values of x where x ≥ 7(a)Find the range of f......................................(2)xg(x) =x1(c) Solve the equation g(x) =.....................................(2)(d) (i) Express the inverse function g –1 in the form g –1(x) = .......g –1(x) = ...................................(ii) Hence write down gg(x) in terms of x.gg(x) = ....................................(6)(Total 11 marks)21.In the diagram OA= a and OC= c.(a) Find CA in terms of a and c......................................(1)The point B is such that AB=1c.2(b) Give the mathematical name for the quadrilateral OABC......................................(1)The point P is such that OP= a + k c, where k ≥ 0(c) State the two conditions relating to a + k c that must be true for OAPCto be a rhombus.(2)(Total 4 marks)22. (a) Work out × 102+ × 104Give your answer in standard form......................................(2)a × 102 +b × 104 =c × 104(b) Express c in terms of a and b.c = ................................(2)(Total 4 marks)TOTAL FOR PAPER = 100 MARKSEND。
2018年深圳国际交流学院G1入学考试数学 数学模拟试卷1

!!
为(
)
A. 6 + 2 − 1 B. 6 − 2 + 1 C. 6 − 2 − 1 D. 6 + 2 + 1
3.为确保信息安全,信息需加密传输,发送方由明文→密文(加密),接收方由密文→明文(解
密),已知加密规则为:明文 a,b,c,d 对应密文 a+2b,2b+c,2c+3d,4d.例如,明文 1,2,
=
!! !!
=
!! !!
=
������(������
≠
0,1),
则称抛物线 C1,C2 互为“友好抛物线”.关于“友好抛物线”有以下说法:(1) C1,C2 开口方向、 开口大小相同; (2) C1,C2 的对称轴相同;(3) 如果 y2 的最值为 m,则 y1 的最值为 km; (4 ) 如果 C2 与 x 轴的两交点间距离为 d,则 C1 与 x 轴的两交点间距离也为 d.其中正确的结论是
3,4 对应密文 5,7,18,16.当接收方收到密文 14,9,23,28 时,则解密得到的明文为(
)
A. 7,6,1,4
B. 6,4,1,7
C. 4,6,1,7
D. 1,6,4,7
4.若一元二次方程式 ax(x+1)+(x+1)(x+2)+bx(x+2)=2 的两根为 0、2,则|3a+4b|的值
(把所有正确结论的序号都填在横线上).
2
12.如图是我省某地一座抛物线形拱桥,桥拱在竖直平面内,与水平桥面相交于 A、B 两点,拱
桥最高点 C 到 AB 的距离为 9m,AB=36m,D、E 为拱桥底部的两点,且 DE ⊥AB,点 E 到直线
G10 Final Test Paper(国际学校数学)
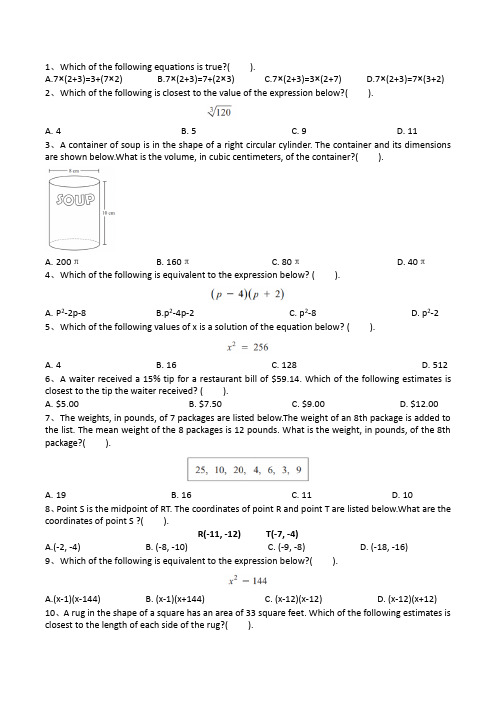
1、Which of the following equations is true?( ).A.7×(2+3)=3+(7×2)B.7×(2+3)=7+(2×3)C.7×(2+3)=3×(2+7)D.7×(2+3)=7×(3+2)2、Which of the following is closest to the value of the expression below?( ).A.4B. 5C. 9D. 113、A container of soup is in the shape of a right circular cylinder. The container and its dimensions are shown below.What is the volume, in cubic centimeters, of the container?( ).A.200πB. 160πC. 80πD. 40π4、Which of the following is equivalent to the expression below? ( ).A.P2-2p-8B.p2-4p-2C. p2-8D. p2-25、Which of the following values of x is a solution of the equation below? ( ).A.4B. 16C. 128D. 5126、A waiter received a 15% tip for a restaurant bill of $59.14. Which of the following estimates is closest to the tip the waiter received? ( ).A.$5.00B. $7.50C. $9.00D. $12.007、The weights, in pounds, of 7 packages are listed below.The weight of an 8th package is added to the list. The mean weight of the 8 packages is 12 pounds. What is the weight, in pounds, of the 8th package?( ).A.19B. 16C. 11D. 108、Point S is the midpoint of RT. The coordinates of point R and point T are listed below.What are the coordinates of point S ?( ).R(-11, -12) T(-7, -4)A.(-2, -4)B. (-8, -10)C. (-9, -8)D. (-18, -16)9、Which of the following is equivalent to the expression below?( ).A.(x-1)(x-144)B. (x-1)(x+144)C. (x-12)(x-12)D. (x-12)(x+12)10、A rug in the shape of a square has an area of 33 square feet. Which of the following estimates is closest to the length of each side of the rug?( ).A.415feetB. 435feetC.436feetD. 418feet11、A set of data is shown in the scatterplot below,Which of the following equations best represents the line of best fit for the data in the scatterplot?( ).A.221--=x yB. 121+-=x yC.221-=x yD. 121+=x y12、A parallelogram and some of its dimensions are shown below.The area of the parallelogram is 90 square inches. What is h, the height in inches of the parallelogram?( ).A. 6B. 8C. 10D. 12 13、Which of the following expressions is equivalent to 17?( ). A.3173⋅ B.31731⋅ C.3317 D. 3317 14、Two groups are going on a trip to a theater. The first group has 30 students and 4 adult chaperones. The second group has 25 students and 4 adult chaperones. The cost, in dollars, for each student ticket, s, and each adult ticket, a, can be determined using the system of equations below. ( ).What is the cost for each student ticket?A. $5B. $20C. $25D. $30Questions 15 and 16 are short -answer questionsWhat is the value of the expression below?16、The line plot below shows the number of red items of clothing owned by each student in a class.What is the median number of red items of clothing owned by the students in the class?17、Rectangle ABCD is similar to rectangle EFGH. The rectangles and some of their dimensions are shown in the diagram below.Based on the dimensions in the diagram, what is the value of x?18、The equation below has two solutions.One solution of the equation is 3. What is the other solution of the equation?19、A company packages fruit baskets of different weights and ships them to customers. The company charges a flat fee for packaging the baskets. The total packaging and shipping cost in dollars, y, of a fruit basket weighing x pounds is represented by the line on the graph below.a.What is the y-intercept of the line on the graph?b. What does the y-intercept of the line represent in this situation?c. What is the slope of the line on the graph? Show or explain how you got your answer.d. What does the slope of the line represent in this situation?e. Write an equation that represents the line on the graph.f. Use the equation you wrote in part (e) to determine the weight, in pounds, of the heaviest fruit basket that could be packaged and shipped for $50. Show or explain how you got your answer.。
IGCSE Maths past paper数学考试题

6
Ruler graduated in centimetres and
Nil
millimetres, protractor, compasses,
7
pen, HB pencil, eraser, calculator.
Tracing paper may be used.
8
9
Instructions to Candidates
10
In the boxes above, write your centre number and candidate number, your surname, initial(s) and
signature.
11
The paper reference is shown at the top of this page. Check that you have the correct question paper.
Surface area of sphere = 4πr2
r
l
h
r
hyp
θ adj
adj = hyp × cos θ opp = hyp × sin θ opp opp = adj × tan θ
or sinθ = opp hyp
In any triangle ABC C
b
a
cosθ = adj hyp
Mathematics
Paper 3H
Higher Tier
Monday 10 May 2004 – Morning Time: 2 hours
Page Leave Numbers Blank
3
4
5
Materials required for examination Items included with question papers
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
UNIVERSITY OF CAMBRIDGE INTERNATIONAL EXAMINATIONSInternational General Certificate of Secondary Education*8006817548*ADDITIONAL MATHEMATICS 0606/12Paper 1 October/November 20122 hoursCandidates answer on the Question Paper.Additional Materials:Electronic calculator.READ THESE INSTRUCTIONS FIRSTWrite your Centre number, candidate number and name on all the work you hand in.Write in dark blue or black pen.Y ou may use a pencil for any diagrams or graphs.Do not use staples, paper clips, highlighters, glue or correction fluid.Answer all the questions.Give non-exact numerical answers correct to 3 significant figures, or 1 decimal place in the case of angles in degrees, unless a different level of accuracy is specified in the question.The use of an electronic calculator is expected, where appropriate.Y ou are reminded of the need for clear presentation in your answers.At the end of the examination, fasten all your work securely together.The number of marks is given in brackets [ ] at the end of each question or part question.The total number of marks for this paper is 80.For Examiner’s Use 1 2 3 4 5 6 7 8 91011TotalMathematical Formulae1. ALGEBRA Quadratic EquationFor the equation ax2 + bx + c = 0,xba =−2Binomial Theorem(a + b)n = a n + (n1)a n–1b + (n2)a n–2b2 + … + (n r)a n–r b r + … + b n, wheren is a positive integer and (n r)= n!(n – r)!r!2. TRIGONOMETRYIdentitiessin2A + cos2A = 1sec2A = 1 + tan2Acosec2A = 1 + cot2AFormulae for ∆ABCasin A =bsin B =csin Ca2 = b2 + c2 – 2bc cos A∆ = 12bc sin A0606/12/O/N/12© UCLES 20120606/12/O/N/12© UCLES 2012[Turn overFor Examiner’s Use1It is given that a = 43 , b = –12 and c = 212 .(i) Find |a + b + c |. [2](ii)Find λ and μ such that λ a + μ b = c . [3]0606/12/O/N/12© UCLES 2012For Examiner’s Use2 (i) Find the inverse of the matrix2 –1–1 1.5 . [2](ii) Hence find the matrix A such that 2 –1–1 1.5 A = 1 6–0.5 4 . [3]3 (i) Show that cot θ +sin θcos θ= cosec θ. [5](ii)Ex plain why the equation cot θ + sin θ1 + cos θ = 12has no solution.[1]0606/12/O/N/12© UCLES 2012[Turn overForExaminer’s Use4Given that log a pq = 9 and log a p 2q = 15, find the value of(i) log a p and of log a q , [4](ii) log p a + log q a . [2]0606/12/O/N/12© UCLES 2012For Examiner’s Use5 The line x – 2y =6 intersects the curve x 2 + xy + 10y + 4y 2 = 156 at the points A and B .Find the length of AB . [7]0606/12/O/N/12© UCLES 2012[Turn overFor Examiner’s Use6( 3 – 1) and without using a calculator, find the value of sin θ in the forma +b 2, where a and b are integers.[5]0606/12/O/N/12© UCLES 2012For Examiner’s Use7Solutions to this question by accurate drawing will not be accepted.The vertices of the trapezium ABCD are the points A (–5, 4), B (8, 4), C (6, 8) and D . The line AB is parallel to the line DC . The lines AD and BC are extended to meet at E and angle AEB = 90°.(i) Find the coordinates of D and of E . [6](ii) Find the area of the trapezium ABCD. [2]ForExaminer’sUse© UCLES 2012[Turn over0606/12/O/N/120606/12/O/N/12© UCLES 2012For Examiner’s Use8BThe diagram shows an isosceles triangle OBD in which OB = OD = 18 cm and angleBOD = 1.5 radians. An arc of the circle, centre O and radius 10 cm, meets OB at A and OD at C .(i) Find the area of the shaded region.[3](ii) Find the perimeter of the shaded region. [4]For Examiner’s Use 9 (a) (i) Using the axes below, sketch for 0 р x р π, the graphs ofy = sin 2x and y = 1 + cos 2x . [4]y––– (ii) Write down the solutions of the equation sin 2x – cos 2x = 1, for 0 р x р π. [2] (b) (i) Write down the amplitude and period of 5 cos 4x – 3. [2] (ii) Write down the period of 4 tan 3x. [1]For Examiner’s Use 10 A function f is such that f(x ) = 4x 3 + 4x 2 + ax + b . It is given that 2x – 1 is a factor of both f(x )and f ʹ(x ).(i) Show that b = 2 and find the value of a . [5] Using the values of a and b from part (i),(ii) find the remainder when f(x ) is divided by x + 3,[2]ForExaminer’s Use(iii) express f(x ) in the form f(x ) = (2x – 1)(px 2 + qx + r ), where p , q and r are integers to befound, [2] (iv) find the values of x for which f(x ) = 0. [2]For Examiner’s Use11 Answer only one of the following two alternatives.EITHERA curve is such that y = 5x 21 + x 2 . (i) Show that d y d x = kx (1 + x 2)2 , where k is an integer to be found. [4] (ii) Find the coordinates of the stationary point on the curve and determine the nature of thisstationary point. [3](iii) By using your result from part (i), find ͵x (1 + x 2)2 d x and hence evaluate ͵2–1x (1 + x 2)2 d x .[4] ORA curve is such that y = Ax 2 +B x 2 – 2 , where A and B are constants. (i) Show thatd y d x = – 2x (2A + B )(x 2 – 2)2 . [4] It is given that y = –3 andd y d x = –10 when x = 1. (ii) Find the value of A and of B . [3] (iii) Using your values of A and B , find the coordinates of the stationary point on the curve, anddetermine the nature of this stationary point. [4]Start your answer to Question 11 here.Indicate which question you are answering. EITHEROR..........................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................Use ....................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................Use ................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................ Permission to reproduce items where third-party owned material protected by copyright is included has been sought and cleared where possible. Every reasonable effort has been made by the publisher (UCLES) to trace copyright holders, but if any items requiring clearance have unwittingly been included, the publisher will be pleased to make amends at the earliest possible opportunity.University of Cambridge International Examinations is part of the Cambridge Assessment Group. Cambridge Assessment is the brand name of University of Cambridge Local Examinations Syndicate (UCLES), which is itself a department of the University of Cambridge.。
