结构动力学课件—1dyanmics of structures-ch1 ch2-PPT课件
合集下载
结构动力学—1dyanmics of structures-ch1 ch
For most problems in structural dynamics it may be assumed that mass does not vary with time, in which case Eq. (13) may be written
the second term is called the inertial force resisting the acceleration of the mass.
The equation of motion for the simple system is most easily formulated by directly expressing the equilibrium of all forces acting on the mass using d'Alembert's principle.
FIGURE 1-3 Sine-series representation of simple beam deflection.
CHAPTER 1. OVERVIEW OF STRUCTURAL DYNAMICS
Discrete Models
FIGURE 1-4 Lumped-mass id(ea)a1l9i9z9a年t台io湾n集o集f地a震集si鹿m大p桥l破e坏b状e态am.
(a) 1999年台湾集集地震集鹿大桥破坏状态
Tasman Bridge Derwent River, Hobart, Australia (1975)
CHAPTER 1. OVERVIEW OF STRUCTURAL DYNAMICS
1.2 ESSENTIAL CHARACTERISTICS OF A DYNAMIC PROBLEM • timevarying nature of the dynamic problem • inertial forces (more fundamental distinction)
the second term is called the inertial force resisting the acceleration of the mass.
The equation of motion for the simple system is most easily formulated by directly expressing the equilibrium of all forces acting on the mass using d'Alembert's principle.
FIGURE 1-3 Sine-series representation of simple beam deflection.
CHAPTER 1. OVERVIEW OF STRUCTURAL DYNAMICS
Discrete Models
FIGURE 1-4 Lumped-mass id(ea)a1l9i9z9a年t台io湾n集o集f地a震集si鹿m大p桥l破e坏b状e态am.
(a) 1999年台湾集集地震集鹿大桥破坏状态
Tasman Bridge Derwent River, Hobart, Australia (1975)
CHAPTER 1. OVERVIEW OF STRUCTURAL DYNAMICS
1.2 ESSENTIAL CHARACTERISTICS OF A DYNAMIC PROBLEM • timevarying nature of the dynamic problem • inertial forces (more fundamental distinction)
结构动力学课件PPT
地震作用
200 0 -200
t(sec)
0 5 10 15 20 25 30 35 40 45 50
结构在确定性荷载作用下的响应分析通 常称为结构振动分析。 结构在随机荷载作用下的响应分析， 被称为结构的随机振动分析。 本课程主要学习确定性荷载作用下的结 构振动分析。
§1-3 动力问题的基本特性
§2-5 广义单自由度体系：刚体集合
刚体的集合（弹性变形局限于局部弹性
元件中） 分布弹性（弹性变形在整个结构或某些 元件上连续形成） 只要可假定只有单一形式的位移，使得 结构按照单自由度体系运动，就可以按 照单自由度体系进行分析。
E2-1
A
x
x p( x,t ) = p a ( t )
1
令：
5l FE (t ) q(t ) 8
y FE (t )
FE(t) 定义为体系的等效动荷载或等效干扰力。其通用表达式
P FE (t )
含义：等效动荷载直接作用在质量自由度上产生的动位移与
实际动荷载产生的位移相等！
已经知道柔度和刚度k 之间的关系为： k 表达式成为：
简支梁： 比较： 刚架： 基本质量弹簧体系：
大型桥梁结构 的有限元模型
§1-5 运动方程的建立
定义
在结构动力分析中，描述体系质量运动规律的数学 方程，称为体系的运动微分方程，简称运动方程。 运动方程的解揭示了体系在各自由度方向的位移 随时间变化的规律。 建立运动方程是求解结构振动问题的重要基础。 常用方法：直接平衡法、虚功法、变分法。
（2-3）
刚度法: 取每一运动质量为隔离体，通过分析所受 的全部外力，建立质量各自由度的瞬时力平衡方 程，得到体系的运动方程。
结构动力学课件—dyanmics of structures-ch(2)
184 BEAM FLEXURE: ORTHOGONALITY OF VIBRATION MODE SHAPES
CHAPTER 18. ANALYSIS OF UNDAMPED FREE VIBRATION
The second orthogonality condition, For a nonuniform beam, the equation of motion in free vibrations
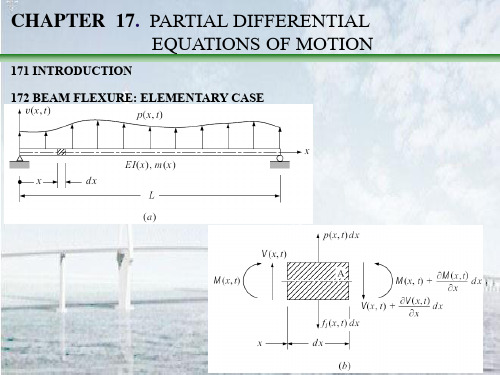
CHAPTER 17. PARTIAL DIFFERENTIAL EQUATIONS OF MOTION
173 BEAM FLEXURE: INCLUDING AXIALFORCE EFFECTS
momentequilibrium equation now becomes
Rotation of beam axis
Example E182. Cantilever Beam
CHAPTER 18. ANALYSIS OF UNDAMPED FREE VIBRATION
CHAPTER 18. ANALYSIS OF UNDAMPED FREE VIBRATION
CHAPTER 18. ANALYSIS OF UNDAMPED FREE VIBRATION
183 BEAM FLEXURE: WITH DISTRIBUTED ELASTIC SUPPORT Separating variables as before
giving two independent equations
CHAPTER 18. ANALYSIS OF UNDAMPED FREE VIBRATION
equilibrium relationship for vertical forces
CHAPTER 18. ANALYSIS OF UNDAMPED FREE VIBRATION
The second orthogonality condition, For a nonuniform beam, the equation of motion in free vibrations
CHAPTER 17. PARTIAL DIFFERENTIAL EQUATIONS OF MOTION
173 BEAM FLEXURE: INCLUDING AXIALFORCE EFFECTS
momentequilibrium equation now becomes
Rotation of beam axis
Example E182. Cantilever Beam
CHAPTER 18. ANALYSIS OF UNDAMPED FREE VIBRATION
CHAPTER 18. ANALYSIS OF UNDAMPED FREE VIBRATION
CHAPTER 18. ANALYSIS OF UNDAMPED FREE VIBRATION
183 BEAM FLEXURE: WITH DISTRIBUTED ELASTIC SUPPORT Separating variables as before
giving two independent equations
CHAPTER 18. ANALYSIS OF UNDAMPED FREE VIBRATION
equilibrium relationship for vertical forces
结构动力学课件-dyanmicsofstructures-ch
See Eq.(13-13)
the speed of convergence can be accelerated by shifting to a value very close to the root that is sought, it is good practice to shift at intervals during iteration, as better approximations of the root are obtained.
!Repeat the procedure to obtain higher modes!
CHAPTER 13. VIBRATION ANALYSIS BY MATRIX ITERATION
136 INVERSE ITERATION--THE PREFERRED PROCEDURE
Not narrow banded
CHAPTER 13. VIBRATION ANALYSIS BY MATRIX ITERATION
134 ANALYSIS OF HIGHER MODES Modal orthogonality properties
CHAPTER 13. VIBRATION ANALYSIS BY MATRIX ITERATION
Divided by
CHAPTER 13. VIBRATION ANALYSIS BY MATRIX ITERATION
Example E131.
Frame used in example Stodola analysis: (a) structural system; (b) flexibility influence coefficients ( x 3,600).
the speed of convergence can be accelerated by shifting to a value very close to the root that is sought, it is good practice to shift at intervals during iteration, as better approximations of the root are obtained.
!Repeat the procedure to obtain higher modes!
CHAPTER 13. VIBRATION ANALYSIS BY MATRIX ITERATION
136 INVERSE ITERATION--THE PREFERRED PROCEDURE
Not narrow banded
CHAPTER 13. VIBRATION ANALYSIS BY MATRIX ITERATION
134 ANALYSIS OF HIGHER MODES Modal orthogonality properties
CHAPTER 13. VIBRATION ANALYSIS BY MATRIX ITERATION
Divided by
CHAPTER 13. VIBRATION ANALYSIS BY MATRIX ITERATION
Example E131.
Frame used in example Stodola analysis: (a) structural system; (b) flexibility influence coefficients ( x 3,600).
结构动力学1 56页
《结构动力学》
Dynamics of Structures
雷庆关 2011年3月
2019/12/11
Dynamics of Structures
1
参考教材
1.《结构力学(Ⅱ)》龙驭球、包世华主编，高等教育出版社 2.《结构动力学及其应用》陆伟民、刘雁编著，同济大学出版社 3.《结构动力学》包世华编著，武汉理工大学出版社 4.《结构动力学》杨茀康编著，人民交通出版社
输入 （动力荷载）
结构 （系统）
第四类问题：控制问题
输入 （动力荷载）
结构 （系统）
输出 （动力反应）
输出 （动力反应）
控制系统 （装置、能量）
2019/12/11Dynamics of Structures
本课程主要介绍结构的反应分析,其主要任务是：
讨论结构在动力荷载作用下反应的分析方法。 寻找结构固有动力特性、动力荷载和结构反应三者 间的相互关系，即结构在动力荷载作用下的反应规 律，为结构的动力可靠性（安全、舒适）设计提供 依据。
两个质点 一个DOF
两个质点 三个DOF
复杂体系可通过附加链 杆法确定体系的自由度
（2）体系动力自由度与其超静定次数无关
（3）体系动力自由度决定了结构动力计算的精度
转化
2019/12/11Dynamics of Structures
1.2 单自由度体系的自由振动
1.2.1 单自由度体系自由振动微分方程的建立 1.2.2 自由振动微分方程的解答 1.2.3 结构的自振周期和自振频率 1.2.4 阻尼对自由振动的影响
y
y2
EI
EI 2EI
(a)单自由度
v(t) u(t)
θ(t)
(c)三个自由度
Dynamics of Structures
雷庆关 2011年3月
2019/12/11
Dynamics of Structures
1
参考教材
1.《结构力学(Ⅱ)》龙驭球、包世华主编，高等教育出版社 2.《结构动力学及其应用》陆伟民、刘雁编著，同济大学出版社 3.《结构动力学》包世华编著，武汉理工大学出版社 4.《结构动力学》杨茀康编著，人民交通出版社
输入 （动力荷载）
结构 （系统）
第四类问题：控制问题
输入 （动力荷载）
结构 （系统）
输出 （动力反应）
输出 （动力反应）
控制系统 （装置、能量）
2019/12/11Dynamics of Structures
本课程主要介绍结构的反应分析,其主要任务是：
讨论结构在动力荷载作用下反应的分析方法。 寻找结构固有动力特性、动力荷载和结构反应三者 间的相互关系，即结构在动力荷载作用下的反应规 律，为结构的动力可靠性（安全、舒适）设计提供 依据。
两个质点 一个DOF
两个质点 三个DOF
复杂体系可通过附加链 杆法确定体系的自由度
（2）体系动力自由度与其超静定次数无关
（3）体系动力自由度决定了结构动力计算的精度
转化
2019/12/11Dynamics of Structures
1.2 单自由度体系的自由振动
1.2.1 单自由度体系自由振动微分方程的建立 1.2.2 自由振动微分方程的解答 1.2.3 结构的自振周期和自振频率 1.2.4 阻尼对自由振动的影响
y
y2
EI
EI 2EI
(a)单自由度
v(t) u(t)
θ(t)
(c)三个自由度
第一章结构动力学概述1(长沙理工大学结构动力学)祥解PPT课件
结构动力学是研究动荷作用下结构动力反应规律的 学科。
1） 结构动力学的研究内容
当前结构动力学的研究内容可用下图表示
第一类问题：响应分析——正问题
输入 （动力荷载）
结构 （系统）
输出 （动力反应）
9
第二类问题：参数（或称系统）识别
输入 （动力荷载）
结构 （系统）
输出 （动力反应）
10
第三类问题：荷载识别。
结构动力学讲义
Tel.: Email:
1
教材 《结构动力学》
（杨茀康 人民交通出版社 张子明 清华大学出版社）
2
第一章、结构动力学概论
1.1 概述 1.2 基本概念 1.3 弹性系统的动力自由度 1.4 结构振动中的能量耗散-阻尼力 1.5结构运动方程的建立
3
1.1概述
首先请大家看中国唐山、日 本阪神地震及TOCOMA大桥 在风载下垮塌录像，希望能从 中体会到学习结构动力学的重 要性。更希望大家能学好结构 动力学！
m y(x)
广义坐标个数即 为自由度个数
m
结点位移个数即 为自由度个数
18
3. 自由度的确定
4)
1) 平面上的一个质点
• 理论计算法
对所设计的结构建立数学模型，通过计算机计算从理论 上验证设计是否满足动力性的要求。
• 试验量测法
用来检验数学模型的正确性，为理论计算提供确切数据。
• 试验与计算混合法
重要结构的动力研究常常需要将数值计算和试验结合起 来，一方面利用数值计算为结构试验提供依据，另一方 面，根据试验结果，不断修正模型以便数学模型能更好 地反映实际情况。
4
5
美国的TOCOMA大桥在风载下垮塌的案例
1940年7月1日，造型优美的塔科马钢铁大桥建成通车。大桥刚投入使用
1） 结构动力学的研究内容
当前结构动力学的研究内容可用下图表示
第一类问题：响应分析——正问题
输入 （动力荷载）
结构 （系统）
输出 （动力反应）
9
第二类问题：参数（或称系统）识别
输入 （动力荷载）
结构 （系统）
输出 （动力反应）
10
第三类问题：荷载识别。
结构动力学讲义
Tel.: Email:
1
教材 《结构动力学》
（杨茀康 人民交通出版社 张子明 清华大学出版社）
2
第一章、结构动力学概论
1.1 概述 1.2 基本概念 1.3 弹性系统的动力自由度 1.4 结构振动中的能量耗散-阻尼力 1.5结构运动方程的建立
3
1.1概述
首先请大家看中国唐山、日 本阪神地震及TOCOMA大桥 在风载下垮塌录像，希望能从 中体会到学习结构动力学的重 要性。更希望大家能学好结构 动力学！
m y(x)
广义坐标个数即 为自由度个数
m
结点位移个数即 为自由度个数
18
3. 自由度的确定
4)
1) 平面上的一个质点
• 理论计算法
对所设计的结构建立数学模型，通过计算机计算从理论 上验证设计是否满足动力性的要求。
• 试验量测法
用来检验数学模型的正确性，为理论计算提供确切数据。
• 试验与计算混合法
重要结构的动力研究常常需要将数值计算和试验结合起 来，一方面利用数值计算为结构试验提供依据，另一方 面，根据试验结果，不断修正模型以便数学模型能更好 地反映实际情况。
4
5
美国的TOCOMA大桥在风载下垮塌的案例
1940年7月1日，造型优美的塔科马钢铁大桥建成通车。大桥刚投入使用
《结构动力计算》PPT课件
Psint
1 k
1 EI
1 2
l 2
l 4
2 3
l 4
2
l3 48EI
2
k m
1
m
1
m
48EIg Ql3
EI
0.5l
0.5l
1
48
2.11011 7.48 105 9.8 35 103 43
57.43 / s
2.
荷载频率：
2n
60
2
500 60
52.36 / s
EI
0.25l MM1
3.
动力系数：
其中,
c 2m
为阻尼比, c为阻尼系数。
22
阻尼比ξ是结构阻尼的重要参数 。
§10.4 阻尼对振动的影响
1. 阻尼对体系自振频率的影响
考虑阻尼时体系的自振频率
r 1 2
＜1为小阻尼，体系具有振动的性质；自振频率减小
＞1（大阻尼）和=1（临界阻尼）时，体系不具有
振动的性。
通常ξ很小，一般结构可取 r≈ 。
的自振周期。EI1=3.528107Nm2.
I=∞
l=6m
• 结构的刚度系数即使柱顶发生单
位位移时，在柱顶需施加的力。 EI1
EI1
考虑梁AB的平衡可得：
k
24EI1
3
l
1
1
结构的自振频率和周期：
k m
2
24EI1g Wl 3
EI1
T
2
2
Wl 3 24EI1g
T 2
24
20 103 63 3.528 107 9.8
4. 最大动位移（振幅）： yd max P 5.03mm
结构动力学课件—dyanmics of structures-ch13 14 15
CHAPTER 13. VIBRATION ANALYSIS BY MATRIX ITERATION
11.1 PRELIMINARY COMMENTS
132 FUNDAMENTAL MODE ANALYSIS
CHAPTER 13. VIBRATION ANALYSIS BY MATRIX ITERATION
The matrix iteration procedure can now be formulated with this sweeping matrix so that it converges toward the second mode of vibration.
CHAPTER 13. VIBRATION ANALYSIS BY MATRIX ITERATION
CHAPTER 14. SELECTION OF DYNAMIC DEGREES OF FREEDOM
141 FINITEELEMENT DEGREES OF FREEDOM
OneDimensional Elements Two and ThreeDimensional Elements
142 KINEMATIC CONSTRAINTS
If this component is removed from the assumed shape, the vector which remains may be said to be purified:
This puried trial vector will now converge toward the secondmode shape in the iteration process. However, roundoff errors are introduced in the numerical operations which permit firstmode components to reappear in the trial vector; therefore it is necessary to repeat this purification operation during each cycle of the iterative solution to ensure its convergence to the second mode.
11.1 PRELIMINARY COMMENTS
132 FUNDAMENTAL MODE ANALYSIS
CHAPTER 13. VIBRATION ANALYSIS BY MATRIX ITERATION
The matrix iteration procedure can now be formulated with this sweeping matrix so that it converges toward the second mode of vibration.
CHAPTER 13. VIBRATION ANALYSIS BY MATRIX ITERATION
CHAPTER 14. SELECTION OF DYNAMIC DEGREES OF FREEDOM
141 FINITEELEMENT DEGREES OF FREEDOM
OneDimensional Elements Two and ThreeDimensional Elements
142 KINEMATIC CONSTRAINTS
If this component is removed from the assumed shape, the vector which remains may be said to be purified:
This puried trial vector will now converge toward the secondmode shape in the iteration process. However, roundoff errors are introduced in the numerical operations which permit firstmode components to reappear in the trial vector; therefore it is necessary to repeat this purification operation during each cycle of the iterative solution to ensure its convergence to the second mode.
《结构动力学》PPT课件
重物落在结构上（突然加载和突然卸载）
④快速移动荷载——高速通过桥梁的火车、汽车
⑤随机荷载——地震的激振、风力脉动作用
荷载变化极不规律，只能用概率方法求其统计规律
a
2
周期荷载（简谐）
周期荷载（非简谐）
冲击荷载（急剧增大、急剧减少）
a
3
随机荷载
a
4
内容：自由振动
无阻尼 单、多自由度
强迫振动
有阻尼 无限多自由度
myky0 达朗伯尔原理 隔离体平衡方程
微分方程
y 2y 0
k 1
m m
a
11
（2）柔度法——列位移方程 ——弹性体系（非隔离体）（图14 – 5c）
运动过程，质量只受惯性力——按静力荷载考虑， I my
m在时刻 t 的位移等于惯性力作用下的静力位移
即 y my
单自由度体系 myy0 1 k
周期运动 y( t + T ) = y( t )
y(t)asint2
asint2y(tT)
自振周期 频率
T＝ 2
每隔一段时间就重复原来运动 单位：秒（S）
f 1 T 2
单位时间内的振动次数 ， 单位： 1／秒（1/S）
园频率（频率）＝2 ＝2f
Ta
2π秒内完成的 振动次数
16
＝k 1 g g m m W st
②动力反应 动内力/位移随时间变化的规律 ——最大值——设计依据
a
5
§14－2 结构振动的自由度
振动自由度
——为了确定全部质量位置所需的独立几何参数的数目
集中质量法：突出主要质量——静力等效
单自由度结构
多自由度结构
a
结构动力学(课用ppt)
10/28/2015 29
10/28/2015
30
10/28/2015
18
（4）一般任意荷载 荷载的幅值变化复杂、难以用解析函数解析表示的荷 载。 由环境振动引起的地脉动、地震引起的地震动， 以及脉动风引起的结构表面的风压时程等。
10/28/2015
19
1.5 结构动力分析中的自由度
一. 自由度的定义
结构动力学和静力学的一个本质区别：考虑惯性力的影响
结构产生动力反应的内因（本质因素）：惯性力 惯性力的产生是由结构的质量引起的 动力自由度（数目）：在动力计算中，一个体系的动力自由度是指为了确定 运动过程中任一时刻全部质体位置所需的独立的几何参数数目。
独立参数也称为体系的广义坐标，可以是位移、转角或其它广义量。
10/28/2015
20
二. 自由度的简化 实际结构都是无限自由度体系，这不仅导致分析困难，而且从工程 角度也没必要。常用简化方法有：
张亚辉 林家浩 编著, 结构动力学基础，大连理工大学出版社，2007. 刘晶波等编著，结构动力学，机械工业出版社，2005. 张子明等编著，结构动力学，河海大学出版社，2001.
10/28/2015
3
第一章 绪论
1.1 动力问题的基本特征 1.2 结构动力分析的目的
1.3 结构动力学研究的内容
1.4 动力荷载类型
注意！
振动体系的自由度数与计算假定有关，而与集中质量的数目和 超静定次数无关，如下图所示的体系。
10/28/2015
26
2、广义坐标法
广义坐标：能决定体系几何位置的彼此独立的量，称为该体系的广义坐标
变形曲线可用三角级数的和来表示：
nx nx u( x, t ) bn sin bn (t ) sin L L n 1 n 1
10/28/2015
30
10/28/2015
18
（4）一般任意荷载 荷载的幅值变化复杂、难以用解析函数解析表示的荷 载。 由环境振动引起的地脉动、地震引起的地震动， 以及脉动风引起的结构表面的风压时程等。
10/28/2015
19
1.5 结构动力分析中的自由度
一. 自由度的定义
结构动力学和静力学的一个本质区别：考虑惯性力的影响
结构产生动力反应的内因（本质因素）：惯性力 惯性力的产生是由结构的质量引起的 动力自由度（数目）：在动力计算中，一个体系的动力自由度是指为了确定 运动过程中任一时刻全部质体位置所需的独立的几何参数数目。
独立参数也称为体系的广义坐标，可以是位移、转角或其它广义量。
10/28/2015
20
二. 自由度的简化 实际结构都是无限自由度体系，这不仅导致分析困难，而且从工程 角度也没必要。常用简化方法有：
张亚辉 林家浩 编著, 结构动力学基础，大连理工大学出版社，2007. 刘晶波等编著，结构动力学，机械工业出版社，2005. 张子明等编著，结构动力学，河海大学出版社，2001.
10/28/2015
3
第一章 绪论
1.1 动力问题的基本特征 1.2 结构动力分析的目的
1.3 结构动力学研究的内容
1.4 动力荷载类型
注意！
振动体系的自由度数与计算假定有关，而与集中质量的数目和 超静定次数无关，如下图所示的体系。
10/28/2015
26
2、广义坐标法
广义坐标：能决定体系几何位置的彼此独立的量，称为该体系的广义坐标
变形曲线可用三角级数的和来表示：
nx nx u( x, t ) bn sin bn (t ) sin L L n 1 n 1
结构动力学课件—2dyanmics of structures-ch
FIGURE 3-4 Rate of buildup of resonant response from rest.
CHAPTER 3. RESPONSE TO HARMONIC LOADING
34 ACCELEROMETERS AND DISPLACEMENT METERS
At this point it is convenient to discuss the fundamental principles on which the operation of an important class of dynamic measurement devices is based. These are seismic instruments, which consist essentially of a viscously damped oscillator as shown in Fig. 35. The system is mounted in a housing which may be attached to the surface where the motion is to be measured. The response is measured in terms of the motion v(t) of the mass
33 RESONANT RESPONSE
The steadystate response amplitude of an undamped system tends toward infinity as the
frequency ratio approaches unity. For low values of damping, it is seen in this same
CHAPTER 3. RESPONSE TO HARMONIC LOADING
34 ACCELEROMETERS AND DISPLACEMENT METERS
At this point it is convenient to discuss the fundamental principles on which the operation of an important class of dynamic measurement devices is based. These are seismic instruments, which consist essentially of a viscously damped oscillator as shown in Fig. 35. The system is mounted in a housing which may be attached to the surface where the motion is to be measured. The response is measured in terms of the motion v(t) of the mass
33 RESONANT RESPONSE
The steadystate response amplitude of an undamped system tends toward infinity as the
frequency ratio approaches unity. For low values of damping, it is seen in this same
结构动力学 ppt课件
i (0) i (l ) 0
--基函数（或形状函数） 课件 i ( x)PPT
9
ai ---广义坐标
3) 有限元法 和静力问题一样，可通过将实 际结构离散化为有限个单元的集合， 将无限自由度问题化为有限自由度 来解决。
m
三. 自由度的确定
集中质量法：独立质量位移数即为自由度数； 广义坐标法：广义坐标个数即为自由度个数； 有限元法：独立结点位移数即为自由度数；
第三类问题：荷载识别。
PPT课件
5
第四类问题：控制问题
输入 （动力荷载） 结构 （系统） 控制系统 （装置、能量） 输出 （动力反应）
本课程主要介绍结构的反应分析 任务 讨论结构在动力荷载作用下反应的分析的方法。寻找 结构固有动力特性、动力荷载和结构反应三者间的相互关 系，即结构在动力荷载作用下的反应规律，为结构的动力 可靠性（安全、舒适）设计提供依据。
PPT课件
10
例. 自由度的确定
1) 平面上的一个质点 3) 计轴向变形时 W=2 不计轴向变形时 W=1 W=2 为减少动力自由度，梁与 刚架一般可不计轴向变形。
y2
y1
W=2
2)Βιβλιοθήκη 弹性支座不减少动力自由度PPT课件
11
4)
y1
W=1
5) W=2
6)
EI
W=1
PPT课件
12
§1.4
体系的运动方程
形式上的平衡方程，实质上的运动方程
PPT课件
13
一、柔度法
P(t )
l
EI
m m (t ) y y(t )
=1
11
(t )] 11[ P(t ) m y
相关主题
- 1、下载文档前请自行甄别文档内容的完整性，平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益，请联系客服反馈,我们会尽快为您处理(人工客服工作时间：9:00-18:30)。
(a) 2019年台湾集集地震集鹿大桥破坏状态
FIGURE 1-2 Basic difference between static and dynamic loads: (a) static loading; (b) dynamic loading.
CHAPTER 1. OVERVIEW OF STRUCTURAL DYNAMICS
CHAPTER 1. OVERVIEW OF STRUCTURAL DYNAMICS
1.2 ESSENTIAL CHARACTERISTICS OF A DYNAMIC PROBLEM • timevarying nature of the dynamic problem • inertial forces (more fundamental distinction)
known as d'Alembert's principle
CHAPTER 1. OVERVIEW OF STRUCTURAL DYNAMICS
1.4.2 Principle of Virtual Displacements
However, if the structural system is reasonably complex involving a number of interconnected mass points or bodies of finite size, the direct equilibration of all the forces acting in the system may be difficult. Frequently, the various forces involved may readily be expressed in terms of the displacement degrees of freedom, but their equilibrium relationships may be obscure. In this case, the principle of virtual displacements can be used to formulate the equations of motion as a substitute for the direct equilibrium relationships. The principle of virtual displacements may be expressed as follows. If a system which is in equilibrium under the action of a set of externally applied forces is subjected to a virtual displacement, i.e., a displacement pattern compatible with the system's constraints, the total work done by the set of forces will be zero.
制作人 Jilu Bridge(in Taiwan) in Jiji Earthquake of 2019
(a) 2019年台湾集集地震集鹿大桥破坏状态
The Damages of Kobe Bridge(Japan) in Kobe Earthquake of 2019
For most problems in structural dynamics it may be assumed that mass does not vary with time, in which case Eq. (13) may be written
the second term is called the inertial force resisting the acceleration of the mass.
FIGURE 1-3 Sine-series representation of simple beam deflection.
CHAPTER 1. OVERVIEW OF STRUCTURAL DYNAMICS
Discrete Models
FIGURE 1-4 (a) 2019年台湾集集地震集鹿大桥破坏状态 Lumped-mass idealization of a simple beam.
Dynamics of Structures
Junjie Wang Dept. of Bridge Engineering 2019.01
CHAPTER 1. OVERVIEW OF STRUCTURAL DYNAMICS
1.1 BACKGROUND
(a) simple harmonic; (b) complex;
(a) 2019年台湾集集地震集鹿大桥破坏状态
FIGURE 1-5 Typical finite-element beam coordinates.
CHAPTER 1. OVERVIEW OF STRUCTURAL DYNAMICS
1.4 FORMULATION OF THE EQUATIONS OF MOTION
1.4.1 Direct Equilibration Using d'Alembert's Principle
The equations of motion of any dynamic system represent expressions of Newton's second law of motion, which states that the rate of change of momentum of any mass particle m is equal to the force acting on it. This relationship can be expressed mathematically by the differential equation
CHAPTER 1. OVERVIEW OF STRUCTURAL DYNAMICS
FEM Athird method of expressing the displacements of any given structure in terms of a nite number of discrete displacement coordinates, which combines certain features of both the lumpedmass and the generalizedcoordinate procedures
1.3 SOLUTIONS TO A DYNAMIC PROBLEM
Continuous Models (partial differential equations; generalized displacement, sum of a series)
(a) 2019年台湾集集地震集鹿大桥破坏状态
CHAPTER 1. OVERVIEW OF STRUCTURAL DYNAMICS
(a) 2019年台湾集集地震集鹿大桥破坏状态
Sunshine Skyway Bridge Tampa Bay, Florida (1980)
Tasman Bridge Derwent River, Hobart, Australia (1975)
(c) impulsive;
(d) long-duration.
FIGURE 1-1 Characteristics and sources of typical dynamic loadings
CHAPTER 1. OVERVIEW OF STRUCTURAL DYNAMICS
集鹿大桥
制作人：同济大学桥梁工程系 孙利民